Numerical Simulation on Size Effect of Fracture Toughness of Concrete Based on Mesomechanics
Abstract
1. Introduction
2. Numerical Model and Examples
2.1. Generation of Concrete Mesostructure
2.2. Constitutive Relations and Failure Criteria
2.3. Determination of Property Parameters
2.4. Determination of Mesh Size
3. Results and Discussion
3.1. Cracking Process
3.2. Peak Stress
3.3. Fracture Toughness
3.4. Discussion of the Size Effect on Fracture Toughness and Tensile Strength
4. Conclusions
- (1)
- The numerical model of concrete mesofracture, considering initial defects, can simulate the fracture process and predict the peak load of concrete, so it is suitable for determining concrete fracture parameters and tensile strength;
- (2)
- Based on the above mesofracture numerical model, when the height of a concrete specimen reaches 600 mm (W/dmax = 60), the fracture toughness KIC calculated from Pmax and the initial notch length according to the linear elastic fracture mechanics formula is independent of the specimen size;
- (3)
- The tensile strength (ft) and the fracture toughness (KIC) which are independent in specimens of concrete can be obtained by the application of the mesofracture numerical model and the BEM. This property can be well expressed by ∆a at peak load (Pmax), and the relationship between ∆a and the maximum aggregate diameter (dmax) can be established by introduced a discrete coefficient (β). Discrete values of β range from 1.0 to 1.4 are a sufficient approximation to predict the ft and KIC values of concrete.
Author Contributions
Funding
Conflicts of Interest
References
- Mariotte, E. 1686 Traite du Mouvement des Eaux, (Posthumously); De La Hire, M., Ed.; Random House: London, UK, 1718; p. 249. [Google Scholar]
- Griffith, A.A. Phenomena of rupture and flow in solids. Philos. Trans. R. Soc. B 1921, 221A, 179–180. [Google Scholar]
- Wittmann, F.H. Size effect on fracture energy of concrete. Eng. Fract. Mech. 1990, 35, 107–115. [Google Scholar] [CrossRef]
- Wu, Z.M.; Zhao, G.F. Fracture toughness of large size concrete specimens. J. Hydraul. Eng. 1997, 6, 67–70. [Google Scholar]
- Hoover, C.G.; Bazant, Z.P. Universal size-shape effect law based on comprehensive concrete fracture tests. ASCE J. Eng. Mech. 2014, 140, 473–479. [Google Scholar] [CrossRef]
- Carpinteri, A.; Ferro, G. Size effects on tensile fracture properties: A unified explanation based on disorder and fractality of concrete microstructure. Mater. Struct. 1994, 27, 563–571. [Google Scholar] [CrossRef]
- Duan, K.; Hu, X.Z.; Wittmann, F.H. Scaling of quasi-brittle fracture: Boundary and size effect. Mech. Mater. 2006, 38, 128–141. [Google Scholar] [CrossRef]
- Weibull, W. The phenomenon of rupture in solids. Proc. R. Swed. Inst. Eng. Res. 1939, 153, 1–55. [Google Scholar]
- Weibull, W. A Statistical distribution function of wide applicability. ASME J. Appl. Mech. 1951, 18, 293–297. [Google Scholar]
- Bazant, Z.P.; Estenssoro, L.F. Surface singularity and crack propagation. Int. J. Solids Struct. 1979, 15, 405–426. [Google Scholar] [CrossRef]
- Bazant, Z.P.; Joong-koo, K. Consequences of diffusion theory for shrinkage of concrete. Mater. Struct. 1991, 24, 323–326. [Google Scholar] [CrossRef]
- Tvergaad, V.; Needleman, A.J. Effect of crack meandering on dynamic ductile fracture. J. Mech. Phys. Solids 1992, 40, 447–471. [Google Scholar] [CrossRef]
- Huang, H.Y.; Zhang, Z.M. Statistical analysis of concrete fracture energy. Water Resour. Hydraul. Eng. 2004, 02, 95–97. [Google Scholar]
- Huang, Y.B.; Qian, J.S. Brittleness and strength size effect of high and super high strength concretes. Eng. Mech. 2005, 35, 15–17. [Google Scholar]
- Hu, X.Z.; Guan, J.F.; Wang, Y.S. Comparison of boundary and size effect models based on new developments. Eng. Fract. Mech. 2017, 175, 146–167. [Google Scholar] [CrossRef]
- Murthy, A.R.; Ganesh, P. Effect of steel fibres and nano silica on fracture properties of medium strength concrete. Adv. Concr. Constr. 2019, 7, 143–150. [Google Scholar]
- Murthy, A.R.; Ganesh, P.; Kumar, S.S.; Iyer, N.R. Fracture energy and tension softening relation for nano-modified concrete. Struct. Eng. Mech. 2015, 54, 1201–1216. [Google Scholar] [CrossRef]
- Mungule, M.; Raghuprasad, B.K. Meso-scale studies in fracture of concrete: A numerical simulation. Comput. Struct. 2011, 89, 912–920. [Google Scholar] [CrossRef]
- Leite, J.P.B.; Slowik, V.; Apel, J. Computational model of mesoscopic structure of concrete for simulation of fracture processes. Comput. Struct. 2006, 85, 1293–1303. [Google Scholar] [CrossRef]
- Zhang, S.L.; Zhang, C.S.; Liao, L. Numerical study of the effect of ITZ on the failure behaviour of concrete by using particle element modelling. Constr. Build. Mater. 2018, 170, 776–789. [Google Scholar] [CrossRef]
- Landis, E.N.; Bolander, J.E. Explicit representation of physical processes in concrete fracture. J. Phys. D Appl. Phys. 2009, 42, 214002. [Google Scholar] [CrossRef]
- Wang, J.; Guan, Q.Y.; Kong, Y.T.; Yu, S.S. Influence of interface initial defects on concrete uniaxial tensile strength. Adv. Sci. Tech. Water Resour. 2016, 36, 46–49. [Google Scholar]
- Suchorzewski, J.; Tejchman, J.; Nitka, M. Experimental and numerical investigations of concrete behaviour at meso-level during quasi-static splitting tension. Theor. Appl. Fract. Mech. 2018, 96, 720–739. [Google Scholar] [CrossRef]
- Suchorzewski, J.; Tejchman, J.; Nitka, M. Discrete element method simulations of fracture in concrete under uniaxial compression based on its real internal structure. Int. J. Dam. Mech. 2018, 27, 578–607. [Google Scholar] [CrossRef]
- Wang, J.; Li, Q.B.; Qing, L.B.; Guan, J.F. 3D simulation of concrete strength under uniaxial compressive load. Eng. Mech. 2014, 31, 39–44. [Google Scholar] [CrossRef]
- Xu, S.L.; Zhang, X.F.; Zheng, S. Experimental determination of double-k fracture parameters of small aggregate concrete. J. Hydrol. Eng. 2006, 5, 543–553. [Google Scholar]
- Schlangen, J.E. Simple lattice model for numerical simulation of fracture of concrete materials and structures. Mater. Struct. 1992, 25, 534–542. [Google Scholar] [CrossRef]
- Zimbelmann, R. Contribution of cement-aggregate bond. CEME Counc. Res. 1985, 15, 801–808. [Google Scholar] [CrossRef]
- Larbi, J.A. The Cement Paste-Aggregate Interfacial Zone in Concrete; University Press: Delft, The Netherlands, 1991; Volume 127. [Google Scholar]
- Ollivier, J.P.; Maso, J.C.; Bourdette, B. Interfacial transition zone in concrete. Adv. Cem. Based Master. 1995, 2, 30–38. [Google Scholar] [CrossRef]
- Bentur, A. Microstructure interfacial effects and micromechanics of cementitious composites. Adv. Cem. Mater. Ceram. Trans. 1990, 16, 523–549. [Google Scholar]
- Chen, Z.Y.; Older, I. The interface zone between marble and tricalcium silicate. Cem. Concr. Resear. 1987, 17, 784–792. [Google Scholar]
- Kong, Y.T. Study on the Effect of Structure and Mechanical Properties of Interfacial Transition Zone on the Strength of Concrete; Zhengzhou University: Zhengzhou, China, 2017. [Google Scholar]
- Qing, L.B.; Shi, X.Y. Determining tensile strength of concrete based on experimental loads in fracture test. Eng. Fract. Mech. 2018, 202, 87–102. [Google Scholar] [CrossRef]
- Qing, L.B.; Dong, M.W.; Guan, J.F. Determining initial fracture toughness of concrete for split-tension specimens based on the extreme theory. Eng. Fract. Mech. 2018, 189, 427–438. [Google Scholar] [CrossRef]
- Chen, H.S.; Sun, W.; Stroeven, P. Review on the study of effect of ITZ on the macro properties of cementitious composites. J. Build. Mater. 2005, 8, 51–62. [Google Scholar]
- Xu, S.; Reinhardt, H.W. Determination of double-determination of Double-K criterion for crack propagation in quasi-brittle fracture part I: Experimental investigation of crack propagation. Int. J. Fract. 1999, 98, 111–149. [Google Scholar] [CrossRef]
- Zhao, H.T.; Jiang, K.D.; Yang, R.; Tang, Y.M.; Liu, J.P. Experimental and theoretical analysis on coupled effect of hydration, temperature and humidity in early-age cement-based materials. Int. J. Heat Mass Transf. 2020, 146, 118784. [Google Scholar] [CrossRef]
- Skarzyński, L.; Suchorzewski, J. Mechanical and fracture properties of concrete reinforced with recycled and industrial steel fibers using Digital Image Correlation technique and X-ray micro computed tomography. Constr. Build. Mater. 2018, 183, 283–299. [Google Scholar] [CrossRef]
- Qing, L.B.; Cao, G.R.; Guan, J.F. Experimental study on allowable damage scale of concrete based on DIC method. Eng. Mech. 2019, 36, 115–121. [Google Scholar]
- Zhou, R.X.; Chen, H.M. Mesoscopic investigation of size effect in notched concrete beams: The role of fracture process zone. Eng. Fract. Mech. 2019, 212, 136–152. [Google Scholar] [CrossRef]
- European Committee for Standardization. Test Method for Metallic Fiber Concrete-Measuring the Flexural Tensile Strength (Limit of Proportionality (LOP), Residual; BS EN14651: 2005+A1:2007; BSI: Brussels, Belgium, 2007. [Google Scholar]
- Zheng, S. The Research of Net Stress Intensity Factor on Concrete Crack Tip for Multiple Specimen; Dalian University of Technology: Dalian, China, 2005. [Google Scholar]
- Golewski, G.L. Green concrete composite incorporating fly ash with high strength and fracture toughness. J. Clean. Prod. 2017, 172, 218–226. [Google Scholar] [CrossRef]
- Golewski, G.L. Effect of curing time on the fracture toughness of fly ash concrete composites. Compos. Struct. 2018, 185, 105–112. [Google Scholar] [CrossRef]
- Golewski, G.L. Estimation of the optimum content of fly ash in concrete composite based on the analysis of fracture toughness tests using various measuring systems. Constr. Build. Mater. 2019, 213, 142–155. [Google Scholar] [CrossRef]
- Dong, W.; Zhang, X.; Zhang, B.S. Influence of sustained loading on fracture properties of concrete. Eng. Fract. Mech. 2018, 200, 134–145. [Google Scholar] [CrossRef]
- Feng, J.; Sun, W.; Li, B. Numerical study of size effect in concrete penetration with LDPM. Def. Technol. 2018, 14, 560–569. [Google Scholar] [CrossRef]
- Suchorzewski, J. Investigations of size effect in concrete during splitting using DEM combined with X-Ray Micro-CT scans. In Proceedings of the 10th International Conference on Fracture Mechanics of Concrete and Concrete Structures, Berkeley, CA, USA, 24–26 June 2019. [Google Scholar]
- Suchorzewski, J.; Tejchman, J.; Nitka, M.; Bobinski, J. Meso-scale analyses of size effect in brittle materials using DEM. Granul. Matter 2019, 21, 1–19. [Google Scholar] [CrossRef]
- Jin, L.; Yu, W.X.; Du, X.L. Mesoscopic numerical simulation of dynamic size effect on the splitting-tensile strength of concrete. Eng. Fract. Mech. 2019, 209, 317–332. [Google Scholar] [CrossRef]
- Duan, K.; Hu, X.Z. Scaling of specimen boundary effect on quasi-brittle fracture. Struct. Integr. Fract. 2004, 2004, 47–68. [Google Scholar]
- Guan, J.F.; Li, C.M.; Wang, J. Determination of fracture parameter and prediction of structural fracture using various concrete specimen types. Theor. Appl. Fract. Mech. 2019, 100, 114–127. [Google Scholar] [CrossRef]
- Guan, J.F.; Yao, X.H.; Bai, W.F.; Chen, J.H.; Fu, J.W. Determination of fracture toughness and tensile strength of concrete by small size specimens. Eng. Mech. 2019, 36, 70–87. [Google Scholar]
- Guan, J.F.; Xie, C.P.; Hu, X.Z.; Yao, X.H.; Bai, W.F. Determination of toughness and strength of hot rolled carbon steel based on boundary effect theory. Eng. Mech. 2019, 3, 231–239. [Google Scholar]
- Guan, J.F.; Hu, X.Z.; Wang, Y.S.; Jiang, B.; Bai, W.F. Effect of fracture toughness and tensile strength on fracture based on boundary effect theory. J. Hydraul. Eng. 2016, 47, 1298–1306. [Google Scholar]
- Wang, Y.S.; Hu, X.Z. Determination of tensile strength and fracture toughness of granite using notched three-point-bend samples. Rock Mech. Rock Eng. 2017, 50, 17–28. [Google Scholar] [CrossRef]
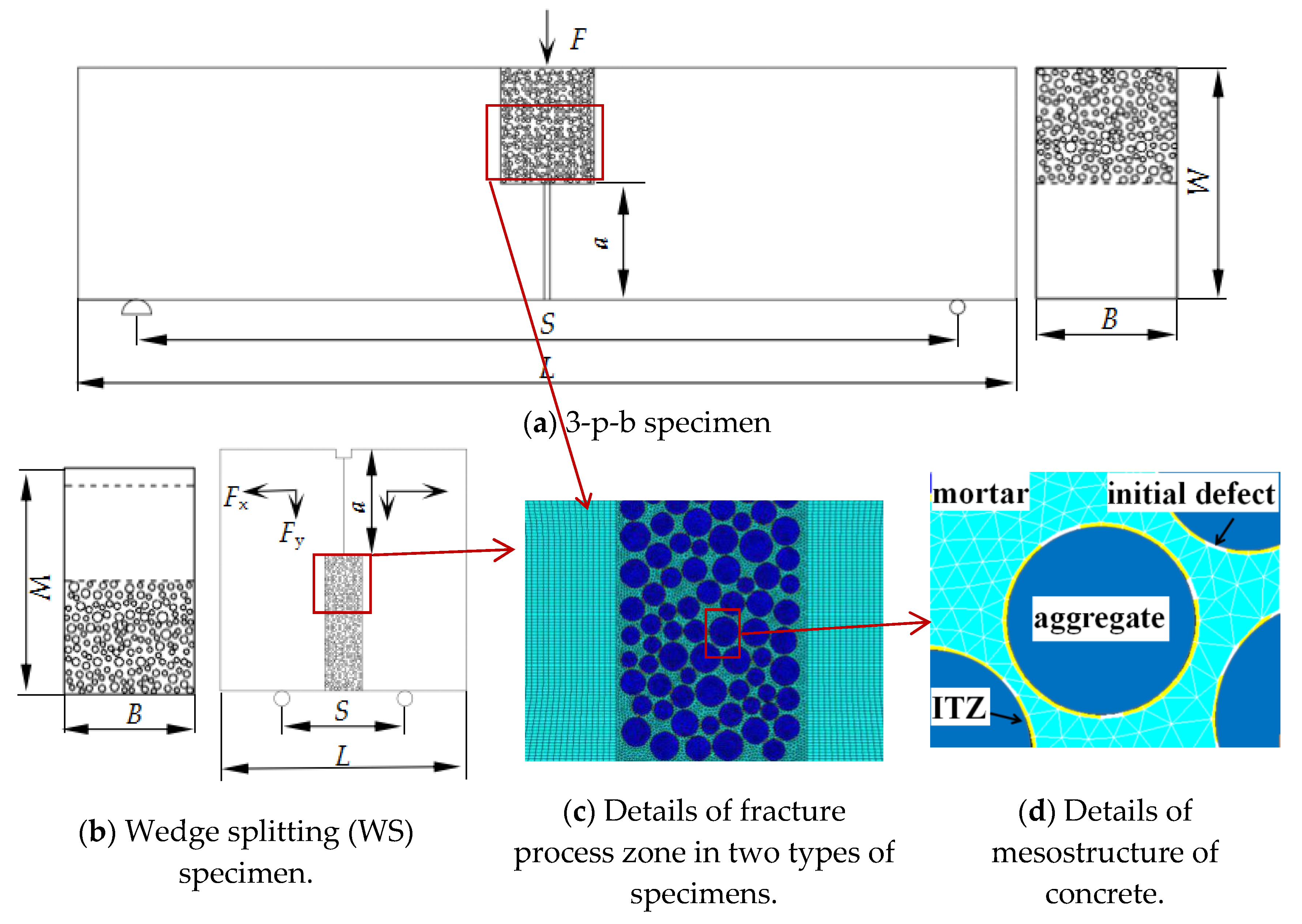
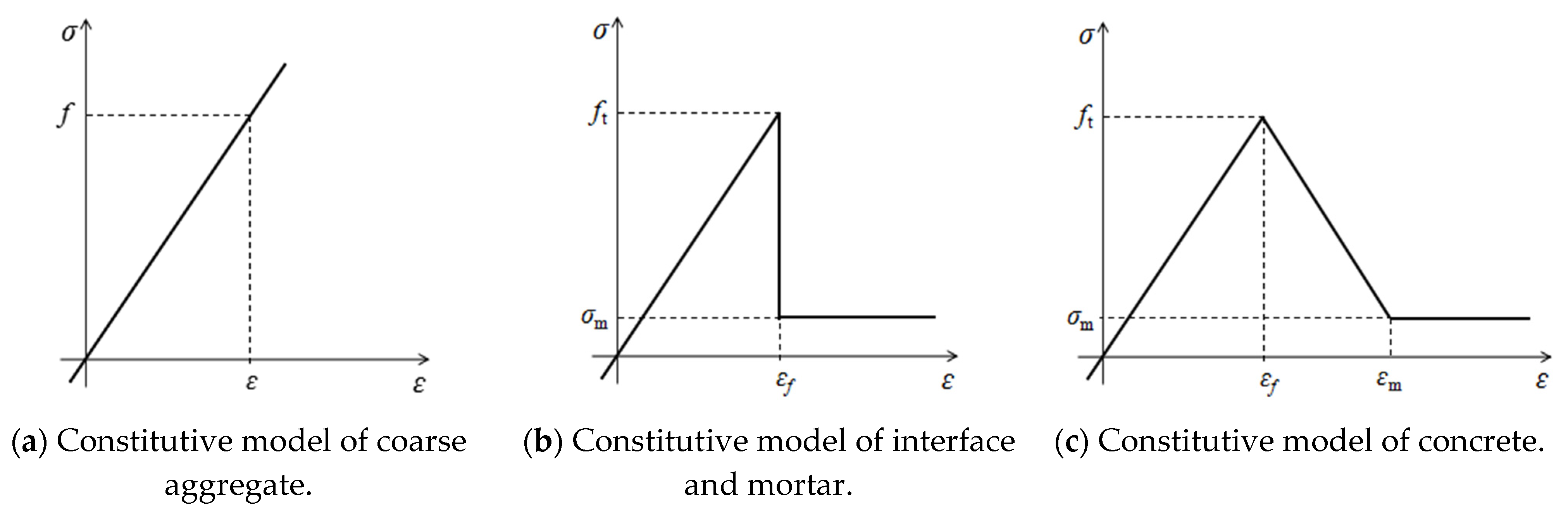
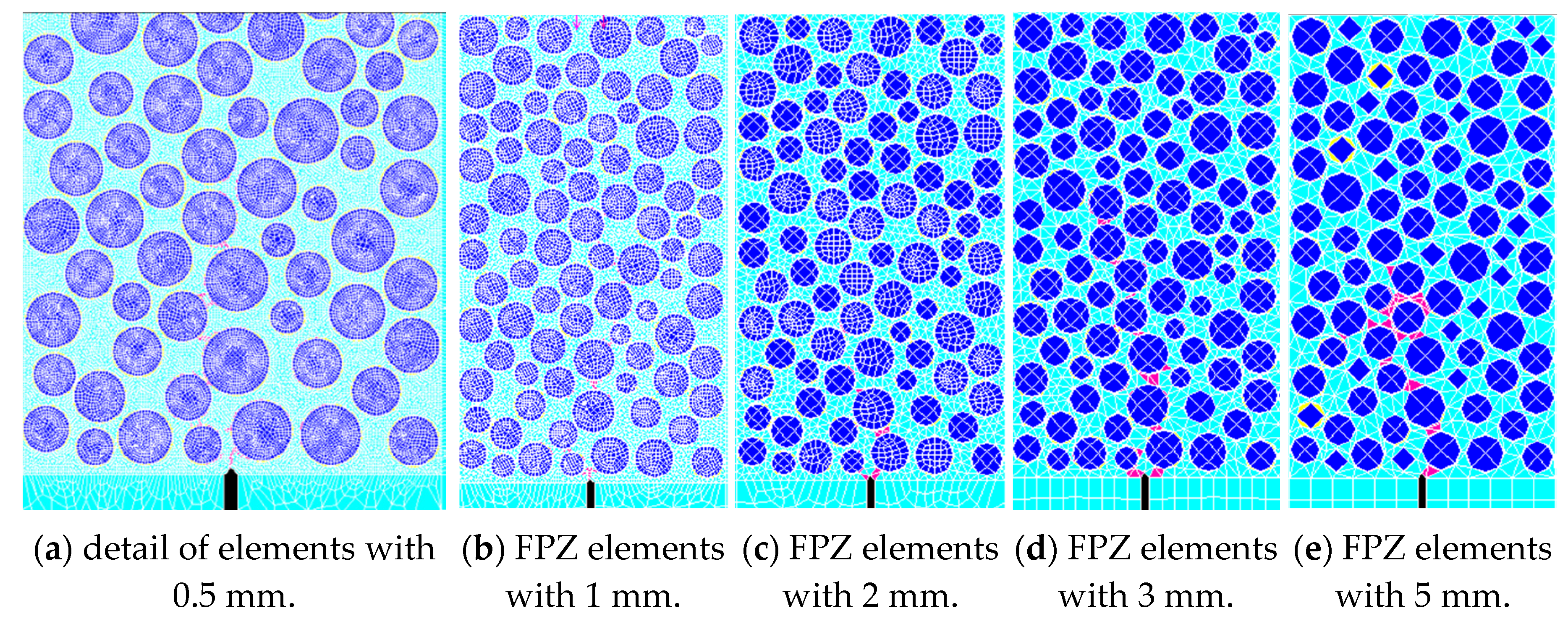
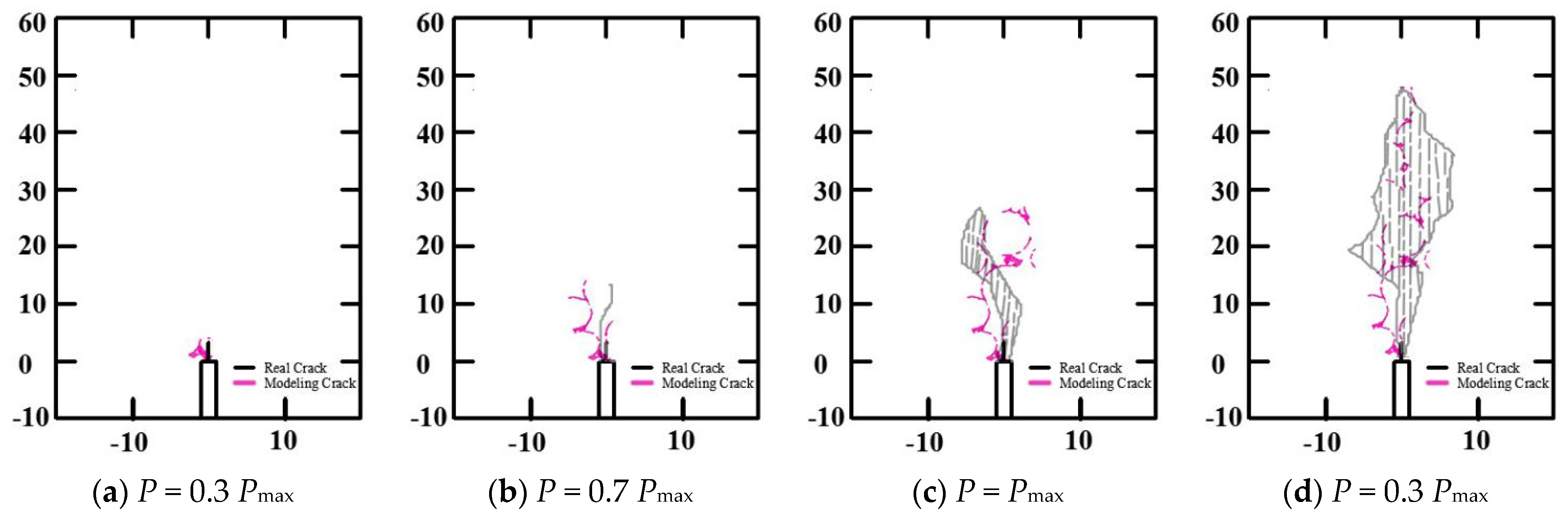

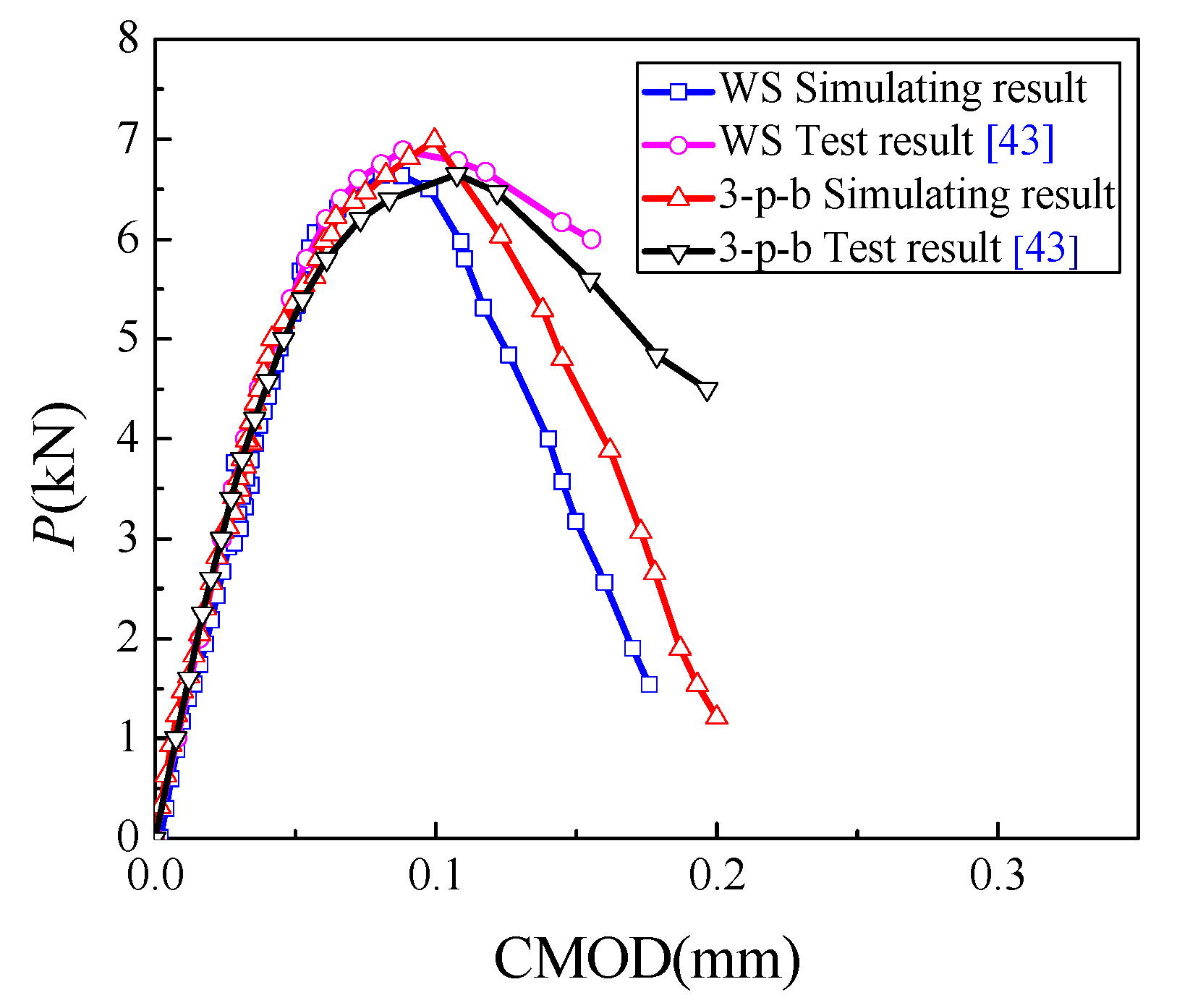

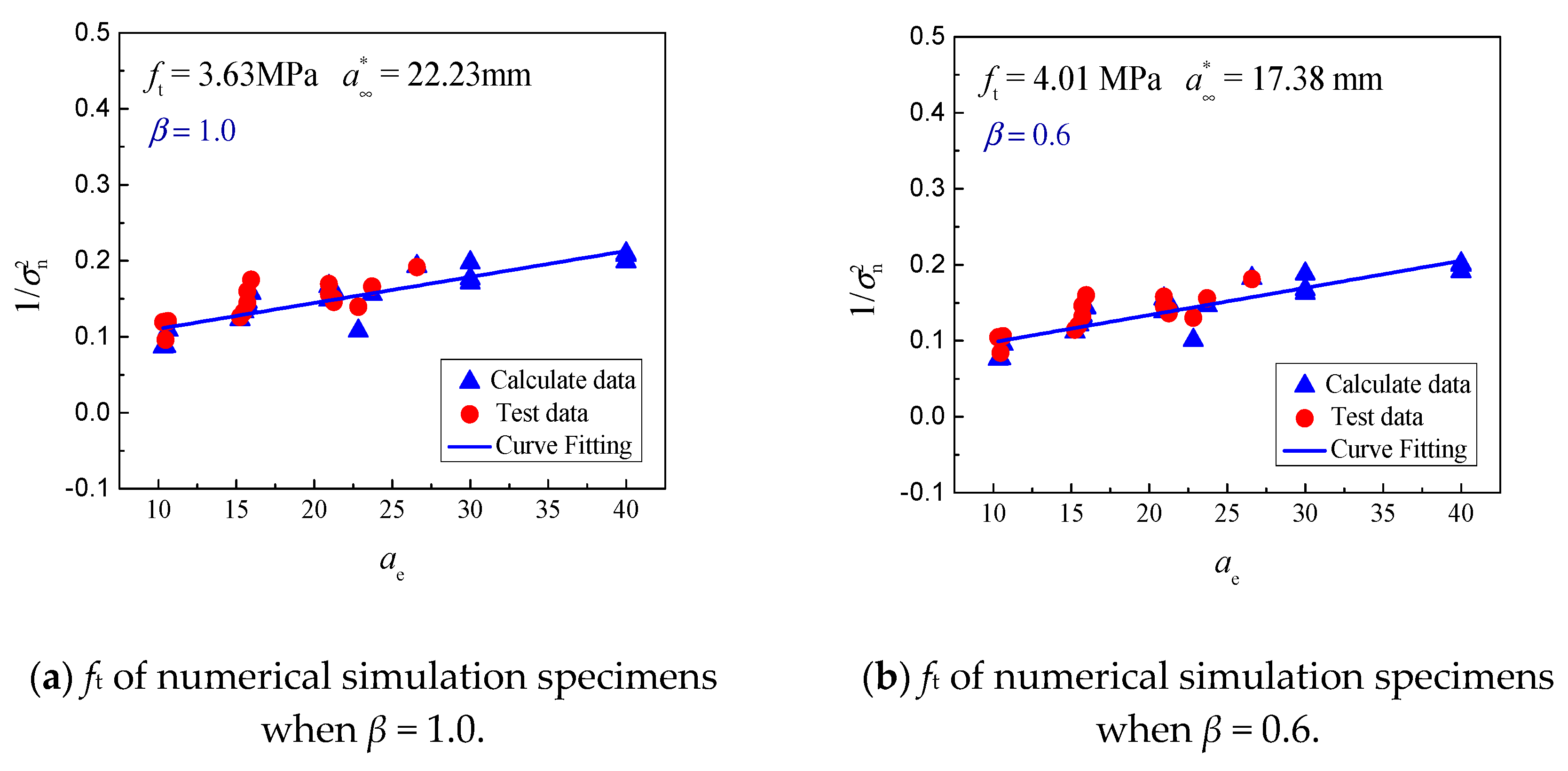

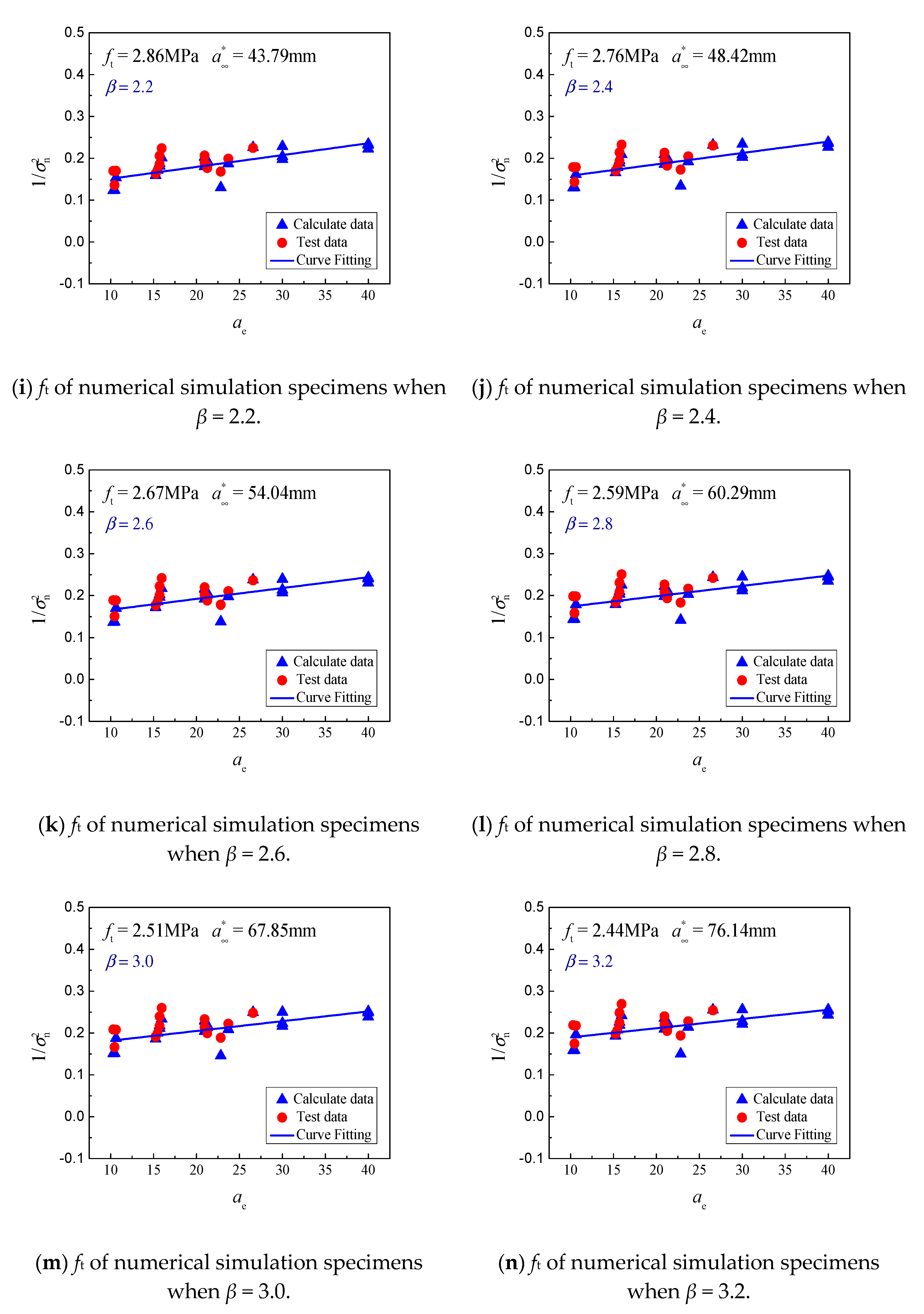
| Microscopic Components | Elastic Modulus (GPa) | Strength (MPa) | Poisson’s Ratio | Volume Content (%) |
|---|---|---|---|---|
| Aggregate | 60 | — | 0.168 | 45 |
| Mortar | 18 | 2.5 | 0.2 | — |
| Interface | 5.4 | 0.83 | 0.2 | — |
| Concrete | 20 | 1.0 | 0.168 | — |
| Interface initial defects | 5 × 10−6 | — | — | 30 |
| Mesh Size (mm) | Element Number | Simulation Results Pmax (kN) | Relative Error of Pmax /% |
|---|---|---|---|
| 0.5 | 67458 | 6.58 | 4.1 |
| 1 | 19403 | 6.84 | 0.2 |
| 2 | 8530 | 7.02 | 2.3 |
| 3 | 5467 | 9.08 | 32.3 |
| 5 | 4119 | 10.24 | 49.3 |
| Specimen Number | a/W | W (mm) | B (mm) | Test Result fL (MPa) | Simulation Results fL (MPa) | Relative Error (%) |
|---|---|---|---|---|---|---|
| I-W200-1 | 0.46 | 200 | 200 | 0.91 | 0.94 | 0.0 |
| I-W200-2 | 0.47 | 200 | 200 | 0.92 | 0.89 | |
| I-W200-3 | 0.47 | 200 | 200 | 0.88 | 0.88 | |
| Average | 0.90 | 0.90 | ||||
| I-W400-1 | 0.45 | 400 | 200 | 0.70 | 0.73 | 5.0 |
| I-W400-2 | 0.46 | 400 | 198 | 0.93 | 0.82 | |
| I-W400-3 | 0.46 | 400 | 199 | 0.78 | 0.96 | |
| Average | 0.80 | 0.84 | ||||
| I-W600-1 | 0.46 | 600 | 193 | 0.79 | 0.76 | 3.9 |
| I-W600-2 | 0.46 | 599 | 200 | 0.73 | 0.71 | |
| I-W600-3 | 0.46 | 600 | 193 | 0.74 | 0.70 | |
| I-W600-5 | 0.46 | 599 | 200 | 0.78 | 0.73 | |
| Average | 0.76 | 0.73 | ||||
| I-W800-1 | 0.45 | 799 | 196 | 0.59 | 0.64 | 8.7 |
| I-W800-2 | 0.46 | 800 | 194 | 0.75 | 0.62 | |
| I-W800-4 | 0.46 | 798 | 200 | 0.70 | 0.60 | |
| I-W800-5 | 0.46 | 801 | 200 | 0.71 | 0.66 | |
| Average | 0.69 | 0.63 | ||||
| I-W1000-1 | 0.45 | 997 | 200 | 0.55 | 0.53 | 5.0 |
| I-W1000-3 | 0.45 | 997 | 200 | 0.58 | 0.54 | |
| I-W1000-4 | 0.45 | 999 | 196 | 0.65 | 0.70 | |
| I-W1000-5 | 0.45 | 1000 | 200 | 0.60 | 0.51 | |
| Average | 0.60 | 0.57 | ||||
| I-W1200-0 | 0.45 | 1198 | 200 | 0.51 | 0.50 | 5.6 |
| I-W1200-1 | 0.46 | 1200 | 201 | 0.52 | 0.52 | |
| I-W1200-2 | 0.45 | 1200 | 200 | 0.58 | 0.50 | |
| Average | 0.54 | 0.51 | ||||
| I-W1500-1 | 0.5 | 1500 | 200 | — | 0.44 | — |
| I-W1500-2 | 0.5 | 1500 | 200 | — | 0.44 | |
| I-W1500-3 | 0.5 | 1500 | 200 | — | 0.43 | |
| Average | 0.44 | |||||
| I-W2000-1 | 0.5 | 2000 | 200 | — | 0.40 | — |
| I-W2000-2 | 0.5 | 2000 | 200 | — | 0.37 | |
| I-W2000-3 | 0.5 | 2000 | 200 | — | 0.38 | |
| Average | 0.38 |
| Specimen Number | a/W | W (mm) | B (mm) | Test Result fL (MPa) | Simulation Results fL (MPa) | Relative Error (%) |
|---|---|---|---|---|---|---|
| II-T200-2 | 0.47 | 200 | 200 | 3.84 | 4.03 | 8.9 |
| II-T200-3 | 0.48 | 200 | 200 | 3.46 | 4.06 | |
| II-T200-4 | 0.46 | 199 | 199 | 3.47 | 3.65 | |
| Average | 3.59 | 3.91 | ||||
| II-T300-1 | 0.47 | 298 | 200 | 2.85 | 2.99 | 2.7 |
| II-T300-2 | 0.47 | 297 | 195 | 3.10 | 3.14 | |
| II-T300-3 | 0.46 | 298 | 198 | 2.75 | 2.90 | |
| II-T300-4 | 0.48 | 298 | 200 | 3.14 | 3.14 | |
| II-T300-5 | 0.49 | 298 | 200 | 3.23 | 3.27 | |
| Average | 3.01 | 3.09 | ||||
| II-T400-1 | 0.46 | 401 | 199 | 2.86 | 2.76 | 0.7 |
| II-T400-2 | 0.46 | 400 | 196 | 2.88 | 2.79 | |
| II-T400-3 | 0.47 | 396 | 199 | 2.84 | 2.92 | |
| II-T400-4 | 0.47 | 396 | 197 | 2.75 | 2.78 | |
| Average | 2.83 | 2.81 | ||||
| II-T500-1 | 0.53 | 499 | 200 | 2.68 | 2.76 | 5.6 |
| II-T500-2 | 0.46 | 500 | 196 | 2.50 | 2.50 | |
| II-T500-3 | 0.55 | 500 | 198 | 2.39 | 2.72 | |
| Average | 2.52 | 2.66 | ||||
| II-T600-1 | 0.5 | 600 | 200 | — | 2.40 | — |
| II-T600-2 | 0.5 | 600 | 200 | — | 2.54 | |
| II-T600-3 | 0.5 | 600 | 200 | — | 2.58 | |
| Average | 2.51 | |||||
| II-T800-1 | 0.5 | 800 | 200 | — | 2.36 | — |
| II-T800-2 | 0.5 | 800 | 200 | — | 2.30 | |
| II-T800-3 | 0.5 | 800 | 200 | — | 2.31 | |
| Average | 2.32 |
| Specimen Number | KIC/(MPa·m1/2) | Average | Test KIC/(MPa·m1/2) | Test Average |
|---|---|---|---|---|
| I-W200-1 | 0.7238 | 0.7329 | 0.7331 | 0.7236 |
| I-W200-2 | 0.7433 | 0.7337 | ||
| I-W200-3 | 0.7316 | 0.7039 | ||
| I-W400-1 | 0.8376 | 0.9553 | 0.8099 | 0.9161 |
| I-W400-2 | 0.9318 | 1.0553 | ||
| I-W400-3 | 1.0964 | 0.883 | ||
| I-W600-1 | 1.0655 | 1.0111 | 1.1039 | 1.0607 |
| I-W600-2 | 0.9860 | 1.0153 | ||
| I-W600-3 | 0.9776 | 1.0333 | ||
| I-W600-5 | 1.0153 | 1.0901 | ||
| I-W800-1 | 1.0326 | 1.0140 | 0.9596 | 1.1062 |
| I-W800-2 | 0.9965 | 1.2062 | ||
| I-W800-4 | 0.9662 | 1.1212 | ||
| I-W800-5 | 1.0607 | 1.1377 | ||
| I-W1000-1 | 0.9655 | 1.0383 | 0.9993 | 1.0846 |
| I-W1000-3 | 0.9862 | 1.053 | ||
| I-W1000-4 | 1.2689 | 1.187 | ||
| I-W1000-5 | 0.9325 | 1.0992 | ||
| I-W1200-0 | 0.9948 | 1.0085 | 1.0166 | 1.0657 |
| I-W1200-1 | 1.0334 | 1.0183 | ||
| I-W1200-2 | 0.9973 | 1.1622 | ||
| I-W1500-1 | 1.1118 | 1.0984 | — | — |
| I-W1500-2 | 1.1097 | — | ||
| I-W1500-3 | 1.0734 | — | ||
| I-W2000-1 | 1.1495 | 1.1065 | — | — |
| I-W2000-2 | 1.0702 | — | ||
| I-W2000-3 | 1.0999 | — |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, J.; Wu, Q.; Guan, J.; Zhang, P.; Fang, H.; Hu, S. Numerical Simulation on Size Effect of Fracture Toughness of Concrete Based on Mesomechanics. Materials 2020, 13, 1370. https://doi.org/10.3390/ma13061370
Wang J, Wu Q, Guan J, Zhang P, Fang H, Hu S. Numerical Simulation on Size Effect of Fracture Toughness of Concrete Based on Mesomechanics. Materials. 2020; 13(6):1370. https://doi.org/10.3390/ma13061370
Chicago/Turabian StyleWang, Juan, Qianqian Wu, Junfeng Guan, Peng Zhang, Hongyuan Fang, and Shaowei Hu. 2020. "Numerical Simulation on Size Effect of Fracture Toughness of Concrete Based on Mesomechanics" Materials 13, no. 6: 1370. https://doi.org/10.3390/ma13061370
APA StyleWang, J., Wu, Q., Guan, J., Zhang, P., Fang, H., & Hu, S. (2020). Numerical Simulation on Size Effect of Fracture Toughness of Concrete Based on Mesomechanics. Materials, 13(6), 1370. https://doi.org/10.3390/ma13061370





