Theoretical Investigation on Failure Behavior of Ogive-Nose Projectile Subjected to Impact Loading
Abstract
1. Introduction
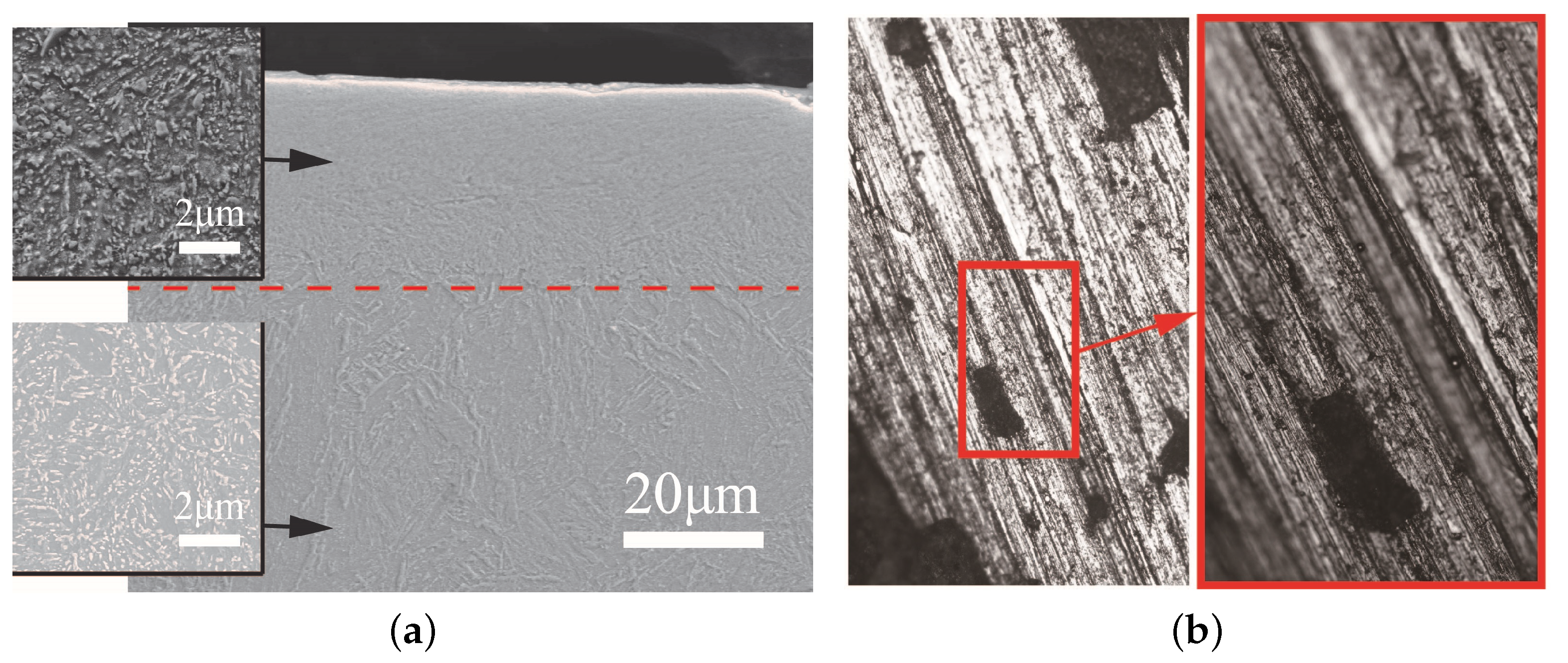
2. Microscopic Analysis of the Recovered Projectile
3. Abrasion Model
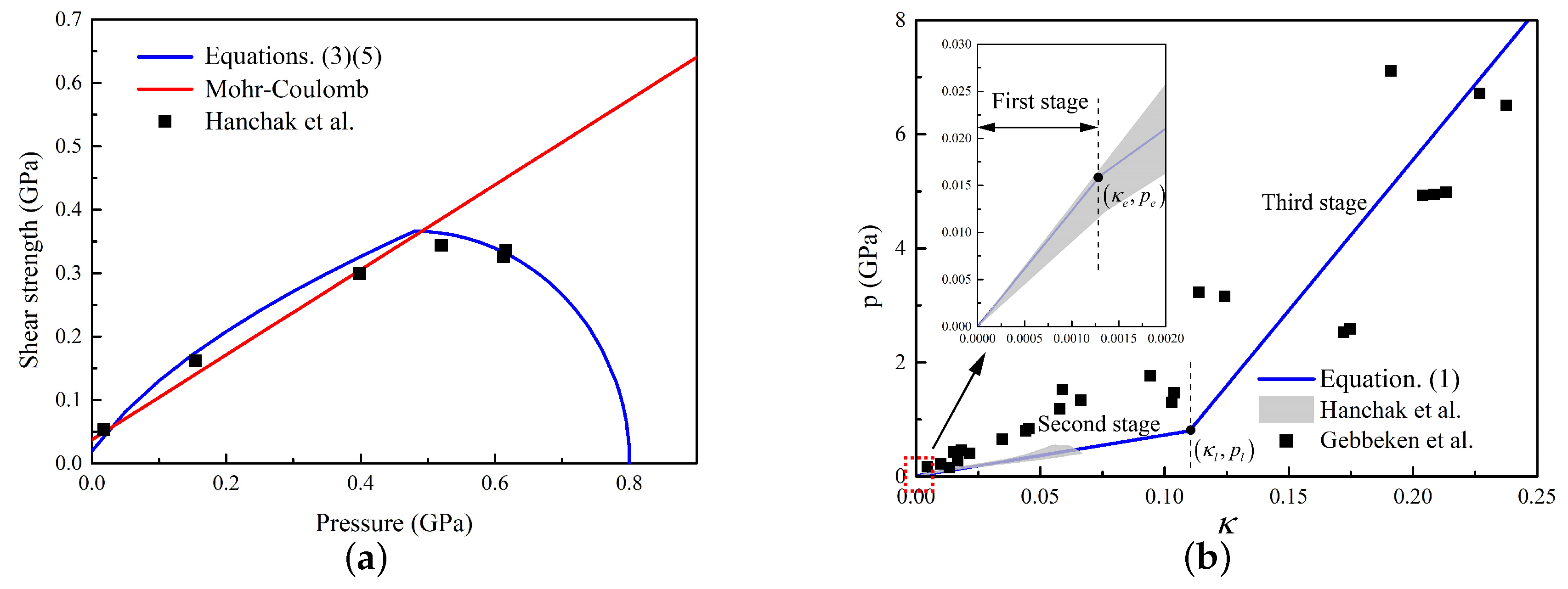
3.1. Constitutive Model
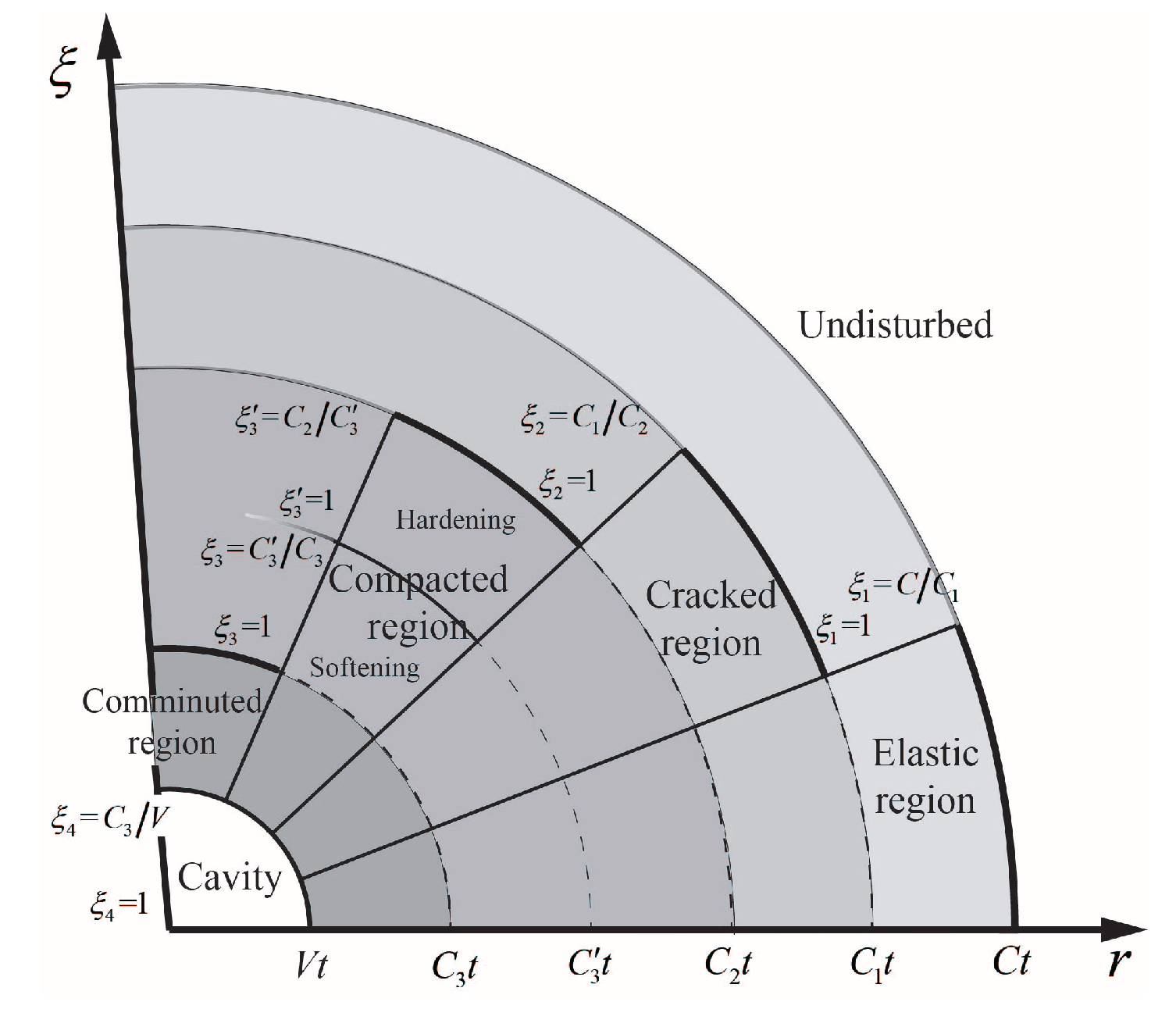
3.2. Equations of Projectile Motion
| Algorithm 1 The flow chat of cavity expansion theory. |
|
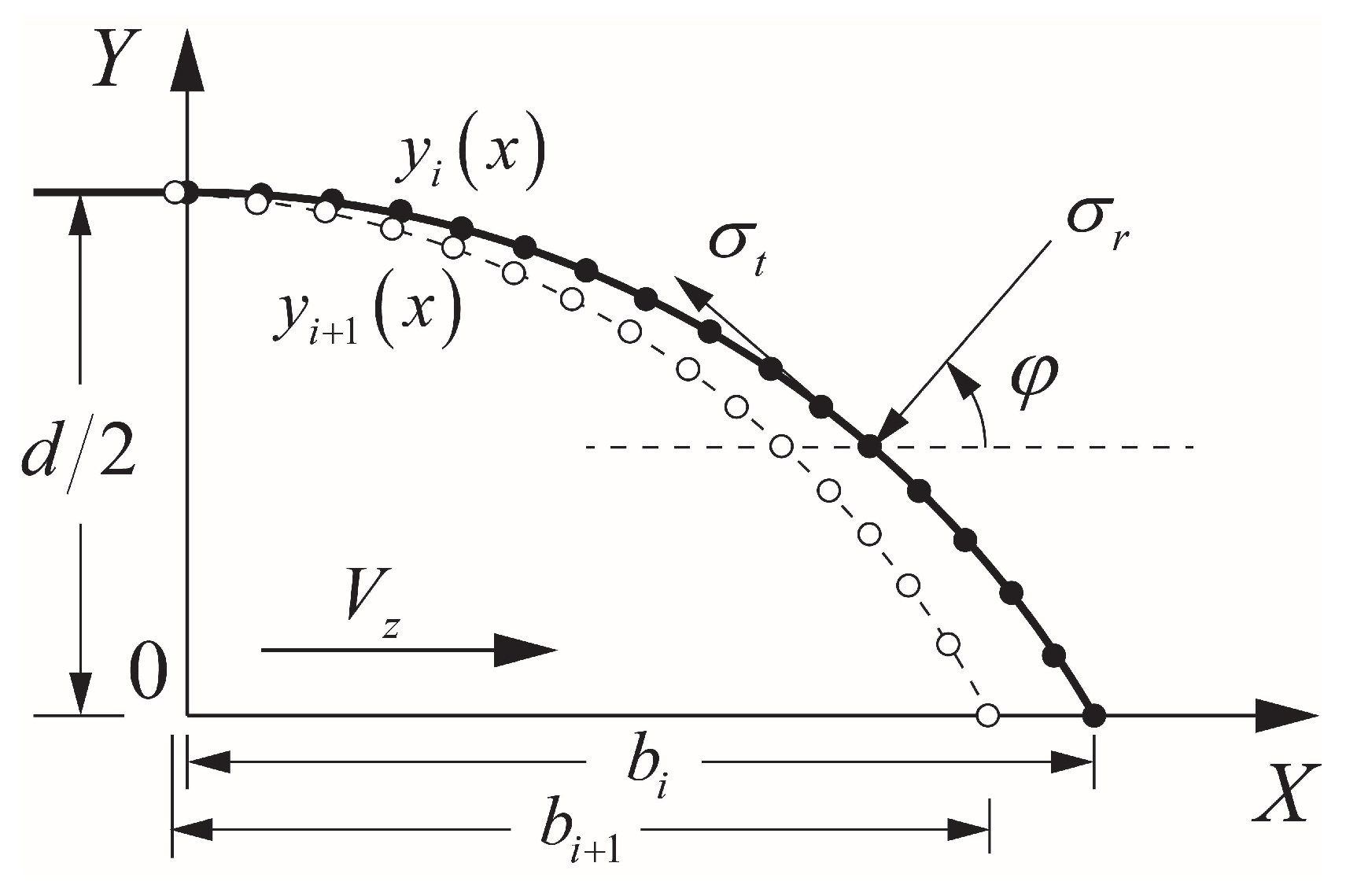
3.3. Mass Abrasion
| Algorithm 2 Calculation of mass loss in any increment of time . |
|
4. Results and Discussion
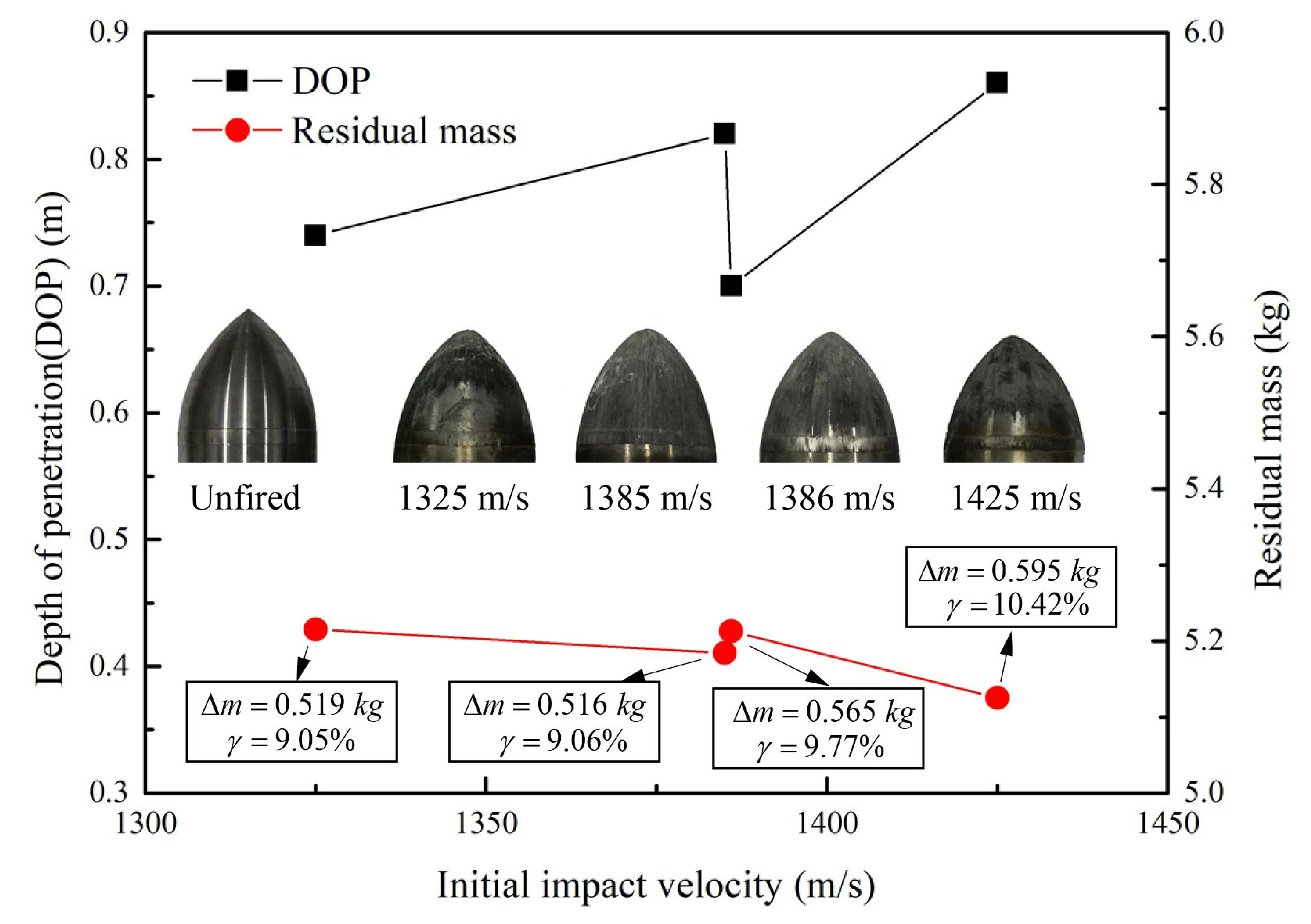
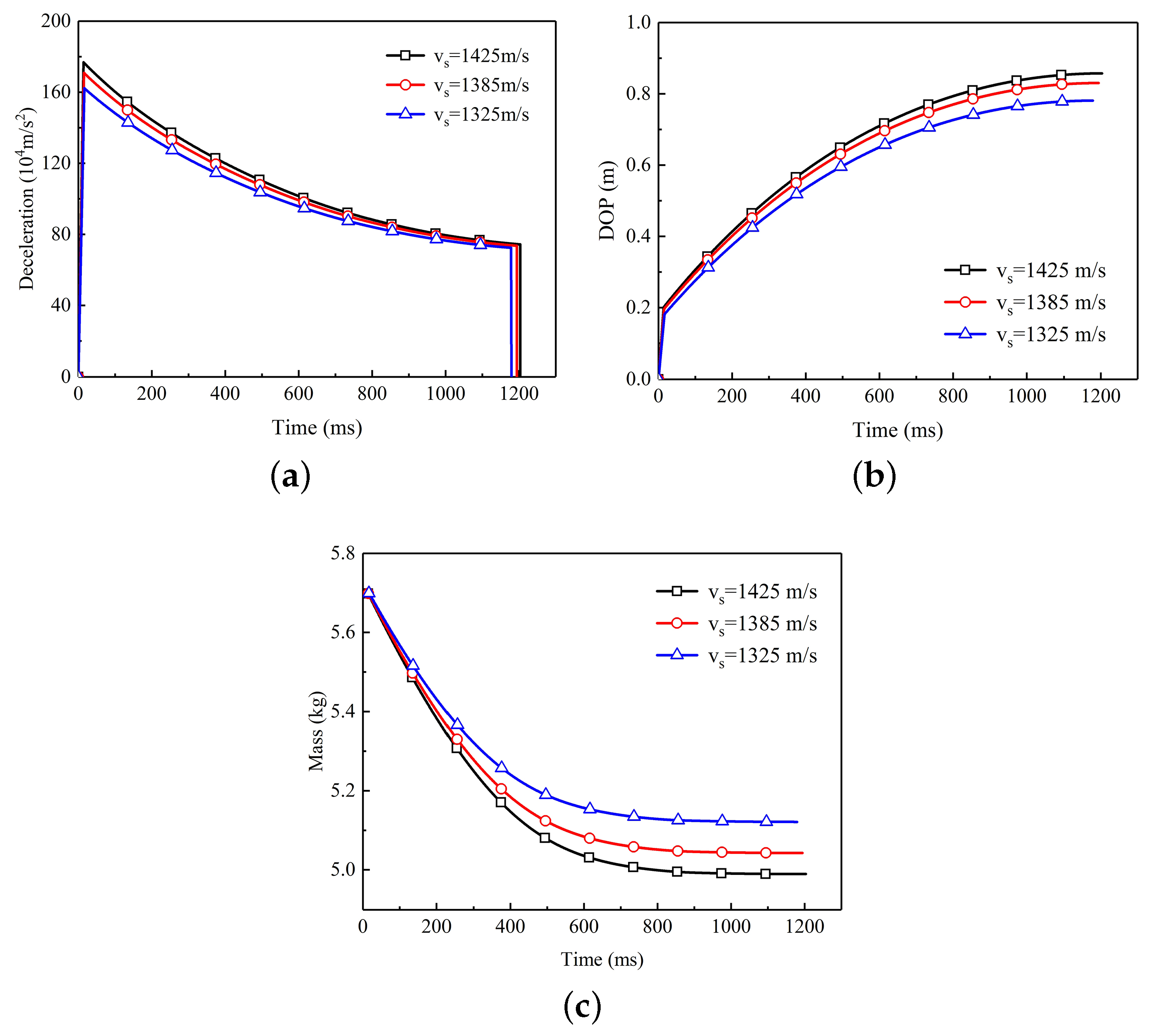
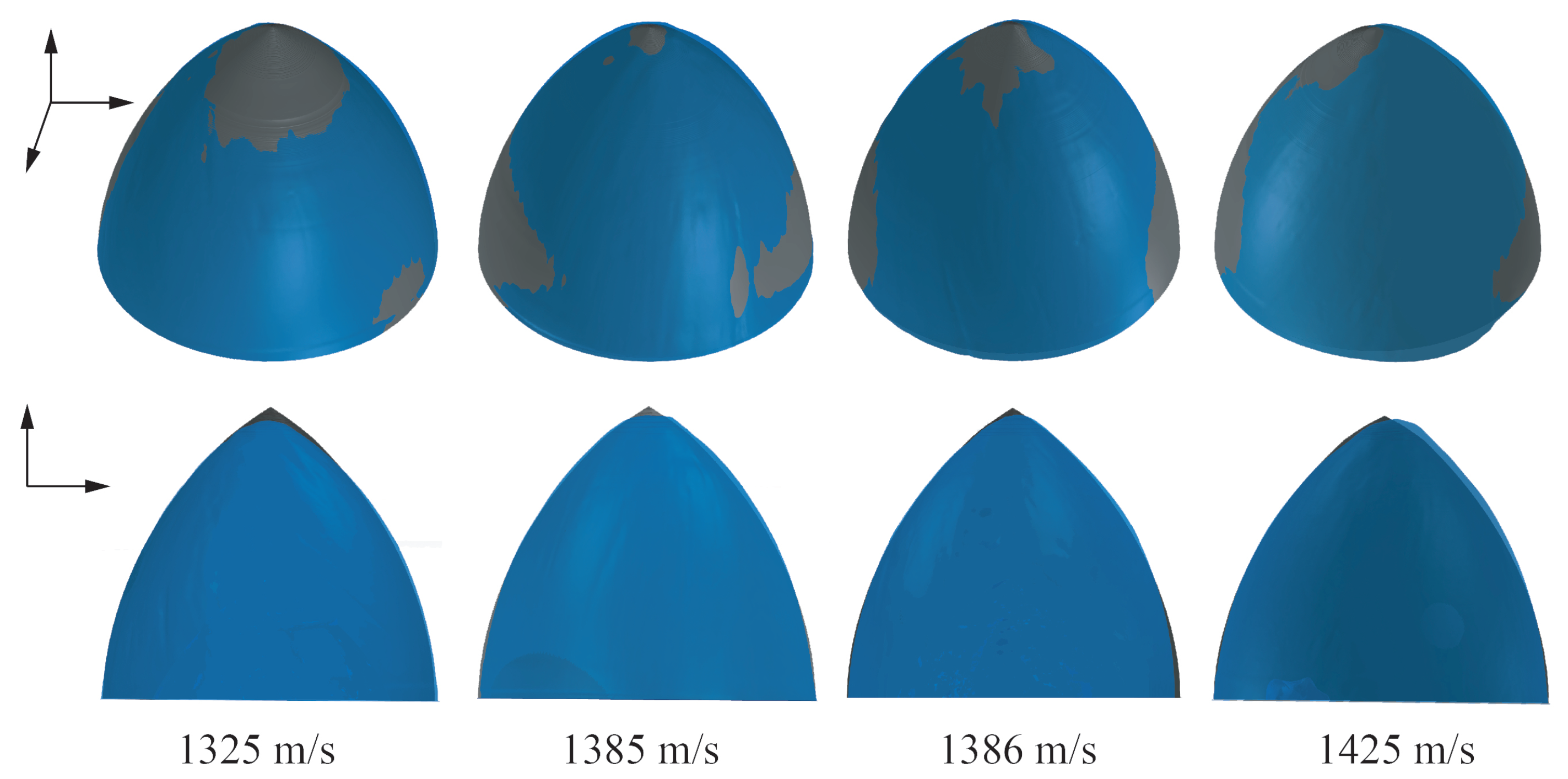
4.1. Comparison of the Predicted Results with the Experimental Data
4.2. Comparison of the Predicted Results with Published Experimental Data and Other Abrasion Models
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
Abbreviations
| SEM | Scanning electron microscopy |
| DOP | Depth of penetration |
| HAZ | Heat affected zone |
| CRH | Caliber-radius-head |
References
- Xiao, X.K.; Mu, Z.C.; Pan, H.; Lou, Y.S. Effect of the Lode parameter in predicting shear cracking of 2024-T351 aluminum alloy Taylor rods. Int. J. Impact Eng. 2018, 120, 185–201. [Google Scholar] [CrossRef]
- Hamed, S.; Keith, D.; Rooholamin, D.; Abolgazl, D. Scaled models for failure under impact loading. Int. J. Impact Eng. 2019, 129, 36–56. [Google Scholar]
- Ning, J.; Chen, L. Fuzzy interface treatment in Eulerian method. Sci. China Ser. E Technol. Sci. 2004, 47, 550–568. [Google Scholar] [CrossRef]
- Liu, B.; Guedes, C. Plastic response and failure of rectangular cross-section tubes subjected to transverse quasi-static and low-velocity impact loads. Int. J. Impact Eng. 2015, 90, 213–227. [Google Scholar] [CrossRef]
- Li, J.; Hao, L.; Li, J. Theoretical modeling and numerical simulations of plasmas generated by shock waves. Sci. China Technol. Sci. 2019, 62, 2204–2212. [Google Scholar] [CrossRef]
- Rosenberg, Z.; Vayig, Y. The scaling issue in the penetration of concrete targets by rigid projectiles—Revisited. Int. J. Impact Eng. 2020, 140, 1–7. [Google Scholar] [CrossRef]
- Ning, J.; Song, W.; Yang, G. Failure analysis of plastic spherical shells impacted by a projectile. Int. J. Impact Eng. 2006, 32, 1464–1484. [Google Scholar] [CrossRef]
- Chen, X.W.; Li, Q.M. Transition from nondeformable projectile penetration to semihydrodynamic penetration. J. Eng. Mech-ASCE 2004, 130, 123–127. [Google Scholar] [CrossRef]
- Li, Q.M.; Chen, X.W. Dimensionless formulae for penetration depth of concrete target impacted by a non-deformable projectile. Int. J. Impact Eng. 2003, 28, 93–116. [Google Scholar] [CrossRef]
- Forrestal, M.J.; Altman, B.S.; Cargile, J.D.; Hanchak, S.J. An empirical equation for penetration depth of ogive-nose projectiles into concrete targets. Int. J. Impact Eng. 1994, 15, 395–405. [Google Scholar] [CrossRef]
- Forrestal, M.J.; Tzou, D.Y. A spherical cavity-expansion penetration model for concrete targets. Int. J. Solid Struct. 1997, 34, 4127–4146. [Google Scholar] [CrossRef]
- Xu, X.; Ma, T.; Liu, H.; Ning, J. A three-dimensional coupled Euler-PIC method for penetration problems. Int. J. Numer. Methods Eng. 2019, 119, 737–756. [Google Scholar] [CrossRef]
- Forrestal, M.J.; Frew, D.J.; Hanchak, S.J.; Brar, N.S. Penetration of grout and concrete targets with ogive-nose steel projectiles. Int. J. Impact Eng. 1996, 18, 465–476. [Google Scholar] [CrossRef]
- Frew, D.J.; Hanchak, S.J.; Green, M.L.; Forrestal, M.J. Penetration of concrete targets with ogive-nose steel rods. Int. J. Impact Eng. 1998, 21, 489–497. [Google Scholar] [CrossRef]
- Zhang, Y.D.; Lu, Z.C.; Wen, H.M. On the penetration of semi-infinite concrete targets by ogival-nosed projectiles at different velocities. Int. J. Impact Eng. 2019, 129, 128–140. [Google Scholar] [CrossRef]
- Feng, J.; Song, M.; Sun, W. Thick plain concrete targets subjected to high speed penetration of 30CrMnSiNi2A steel projectiles: Tests and analyses. Int. J. Impact Eng. 2018, 122, 305–317. [Google Scholar] [CrossRef]
- Yang, J.C.; Zuo, X.J.; He, X. Experimental Study of projectile mass loss in high velocity penetration of concrete target. J. Exp. Mech. 2012, 27, 122–127. [Google Scholar]
- Liu, C.; Zhang, X.F.; Chen, H.H. Experimental and theoretical study on steel long-rod projectile penetration into concrete targets with elevated impact velocities. Int. J. Impact Eng. 2020, 138, 305–317. [Google Scholar] [CrossRef]
- Ning, J.; Ren, H.; Guo, T.; Li, P. Dynamic response of alumina ceramics impacted by long tungsten projectile. Int. J. Impact Eng. 2013, 62, 60–74. [Google Scholar] [CrossRef]
- Silling, S.A.; Forrestal, M.J. Mass loss from abrasion on ogive-nose steel projectiles that penetrate concrete targets. Int. J. Impact Eng. 2007, 34, 1814–1820. [Google Scholar] [CrossRef]
- Wen, H.M.; Yang, Y.; He, T. Effects of abrasion on the penetration of ogival-nosed projectiles into concrete targets. Lat. Am. J. Solids Struct. 2010, 7, 413–422. [Google Scholar] [CrossRef]
- Chen, X.W.; He, L.L.; Yang, S.Q. Modeling on mass abrasion of kinetic energy penetrator. Eur. J. Mech. A Solids 2010, 29, 7–17. [Google Scholar] [CrossRef]
- Jones, S.E.; Foster, J.C.; Toness, O.A.; DeAngelis, R.J.; Rule, W.K. An estimate for mass loss from high velocity steel penetrators. In Proceedings of the ASME PVP-435 Conference on Thermal–Hydraulic Problems, Sloshing Phenomena, and Extreme Loads on Structures, Vancouver, BC, Canada, 5–9 August 2002; pp. 227–237. [Google Scholar]
- Davis, R.N.; Neely, A.M.; Jones, S.E. Mass loss and blunting during high-speed penetration. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2004, 218, 1053–1062. [Google Scholar] [CrossRef]
- He, L.L.; Chen, X.W.; He, X. Parametric study on mass loss of penetrators. Acta Mech. Sin. 2010, 26, 585–597. [Google Scholar] [CrossRef]
- Ouyang, H.; Chen, X. Modeling on mass loss and nose blunting of high-speed penetrator into concrete target. Int. J. Prot. Struct. 2018, 10, 3–25. [Google Scholar]
- Guo, L.; He, Y.; Zhang, N.S.; Pang, C.X.; Hao, Z. On the mass loss of a projectile based on the Archard theory. Explos. Shock Waves 2014, 34, 622–629. [Google Scholar]
- Ning, J.; Ma, T.; Fei, G. Multi-material Eulerian method and parallel computation for 3D explosion and impact problems. Int. J. Comput. Methods 2014, 11, 1350079. [Google Scholar] [CrossRef]
- Luk, V.K.; Forrestal, M.J. Penetration into semi-infinite reinforced-concrete targets with spherical and ogival nose projectiles. Int. J. Impact Eng. 1987, 6, 291–301. [Google Scholar] [CrossRef]
- Meng, C.M.; Tan, Q.H.; Jiang, Z.G.; Song, D.Y.; Liu, F. Approximate solutions of finite dynamic spherical cavity-expansion models for penetration into elastically confined concrete targets. Int. J. Impact Eng. 2018, 114, 182–193. [Google Scholar] [CrossRef]
- Deng, Y.J.; Song, W.J.; Chen, X.W. Spherical cavity-expansion model for penetration of reinforced-concrete targets. Acta Mech. Sin. 2019, 35, 535–551. [Google Scholar] [CrossRef]
- Ottosen, N.S. A failure criterion for concrete. J. Eng. Mech. Div. ASCE 1979, 105, 127–141. [Google Scholar]
- Holmquist, T.J.; Johnson, G.R.; Cook, W.H. A computational constitutive model for concrete subjected to large strains, high strain rate, and high pressures. In Proceedings of the 14th International Symposium on Ballistics, Quebec City, QC, Canada, 26–29 September 1993; pp. 591–600. [Google Scholar]
- Ren, G.M.; Wu, H.; Fang, Q.; Kong, X.Z. Parameters of Holmquist-Johnson-Cook model for high-strength concrete-like materials under projectile impact. Int. J. Prot. Struct. 2017, 8, 352–367. [Google Scholar] [CrossRef]
- Roscoe, K.H.; Schofied, M.A. On the yielding of soils. Geotechnique 1958, 8, 22–53. [Google Scholar] [CrossRef]
- Sandler, I.S. Review of the development of Cap Models for geomaterials. Shock Vib. 2005, 21, 67–71. [Google Scholar] [CrossRef]
- Feng, J.; Li, W.; Wang, X.; Song, M.; Ren, H.; Li, W. Dynamic spherical cavity expansion analysis of rate-dependent concrete material with scale effect. Int. J. Impact Eng. 2015, 84, 24–37. [Google Scholar] [CrossRef]
- Liu, Z.L.; Sun, W.W.; Wang, X.M.; Feng, J. Spherical cavity-expansion model for concrete targets based on cap model and penetration resistance analysis. Acta Armamentarii 2015, 32, 2209–2216. [Google Scholar]
- Hanchak, S.J.; Forrestal, M.J.; Young, E.R.; Ehrgott, J.Q. Perforation of concrete slabs with 48 MPa (7 ksi) and 140 MPa (20 ksi) unconfined compressive strengths. Int. J. Impact Eng. 1992, 12, 1–7. [Google Scholar] [CrossRef]
- Gebbeken, N.; Greulich, S.; Pietzsch, A. Hugoniot properties for concrete determined by full-scale detonation experiments and flyer-plate-impact tests. Int. J. Impact Eng. 2006, 32, 2017–2031. [Google Scholar] [CrossRef]
- Satapathy, S. Dynamic spherical cavity expansion in brittle ceramics. Int. J. Solids Struct. 2001, 38, 5833–5845. [Google Scholar] [CrossRef]
- He, L.; Chen, X. Analyses of the penetration process considering mass loss. Eur. J. Mech. A Solids 2011, 30, 145–157. [Google Scholar] [CrossRef]
- Rabinowicz, E.; Dunn, L.; Russell, P. A study of abrasive wear under three-body conditions. Wear 1961, 4, 345–355. [Google Scholar] [CrossRef]
- Guo, L.; He, Y.; Zhang, X.; He, Y.; Deng, J.; Guan, Z. Thermal-mechanical analysis on the mass loss of high-speed projectiles penetrating concrete targets. Eur. J. Mech. A Solids 2017, 65, 159–177. [Google Scholar] [CrossRef]
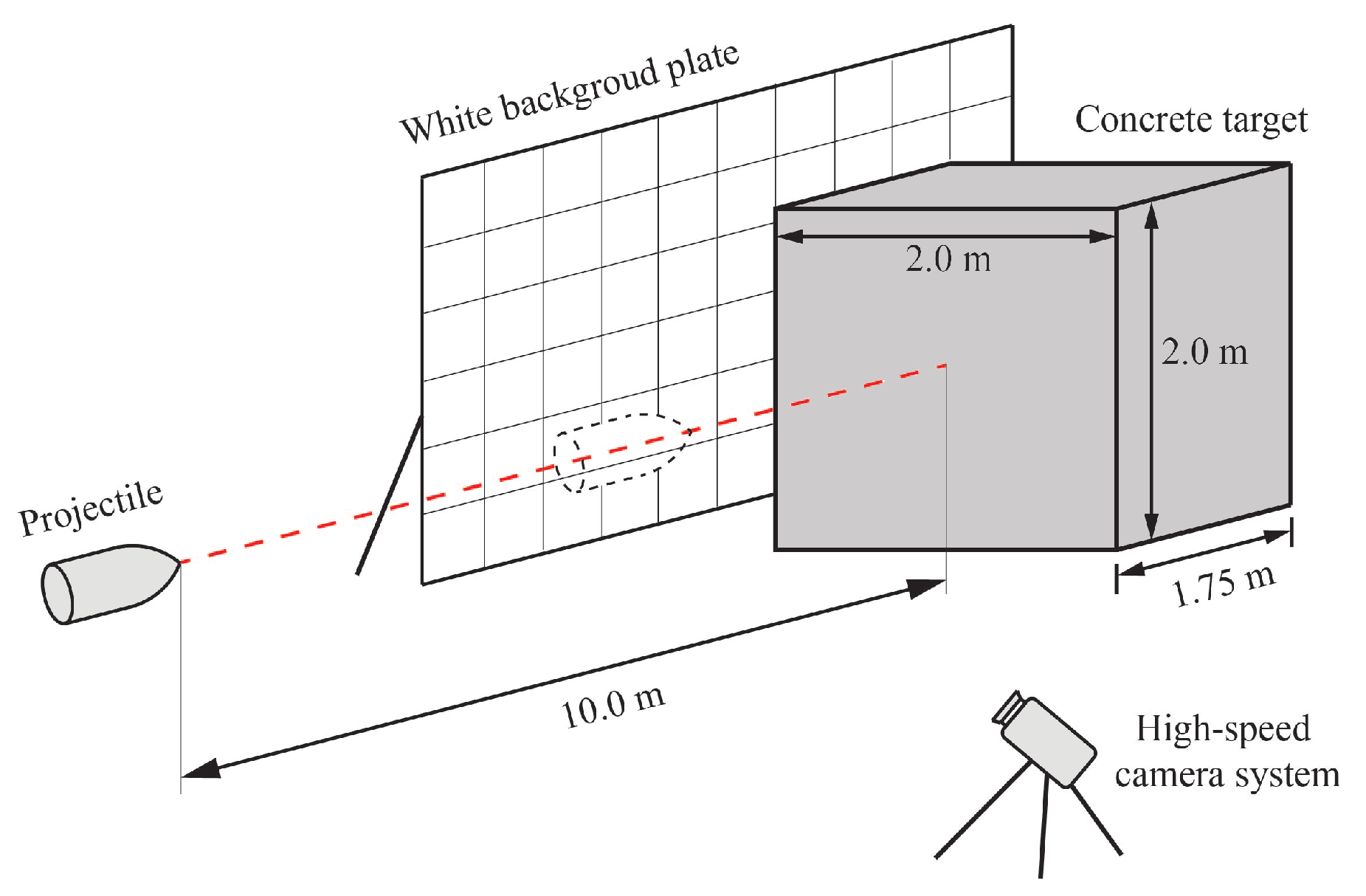
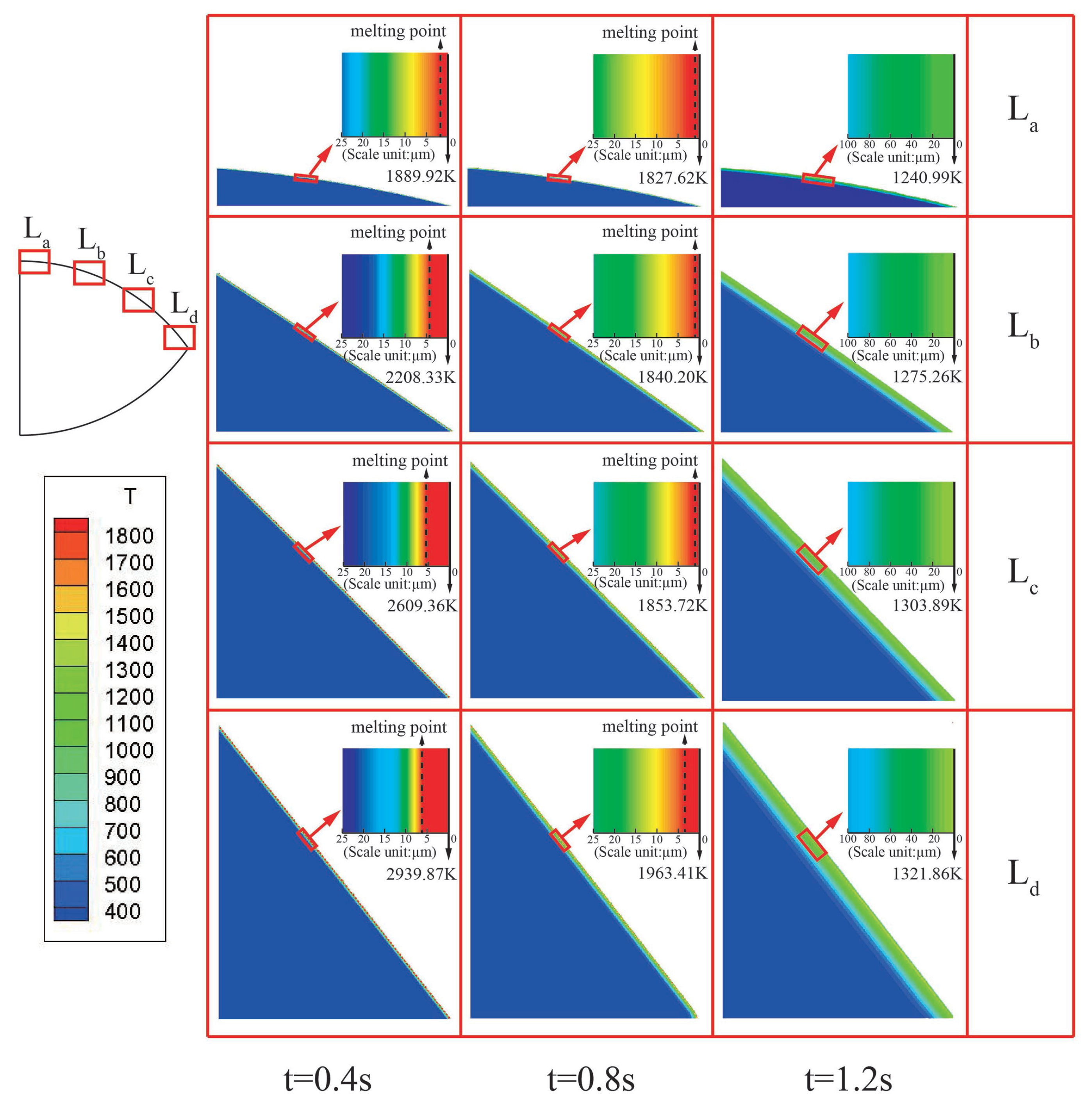
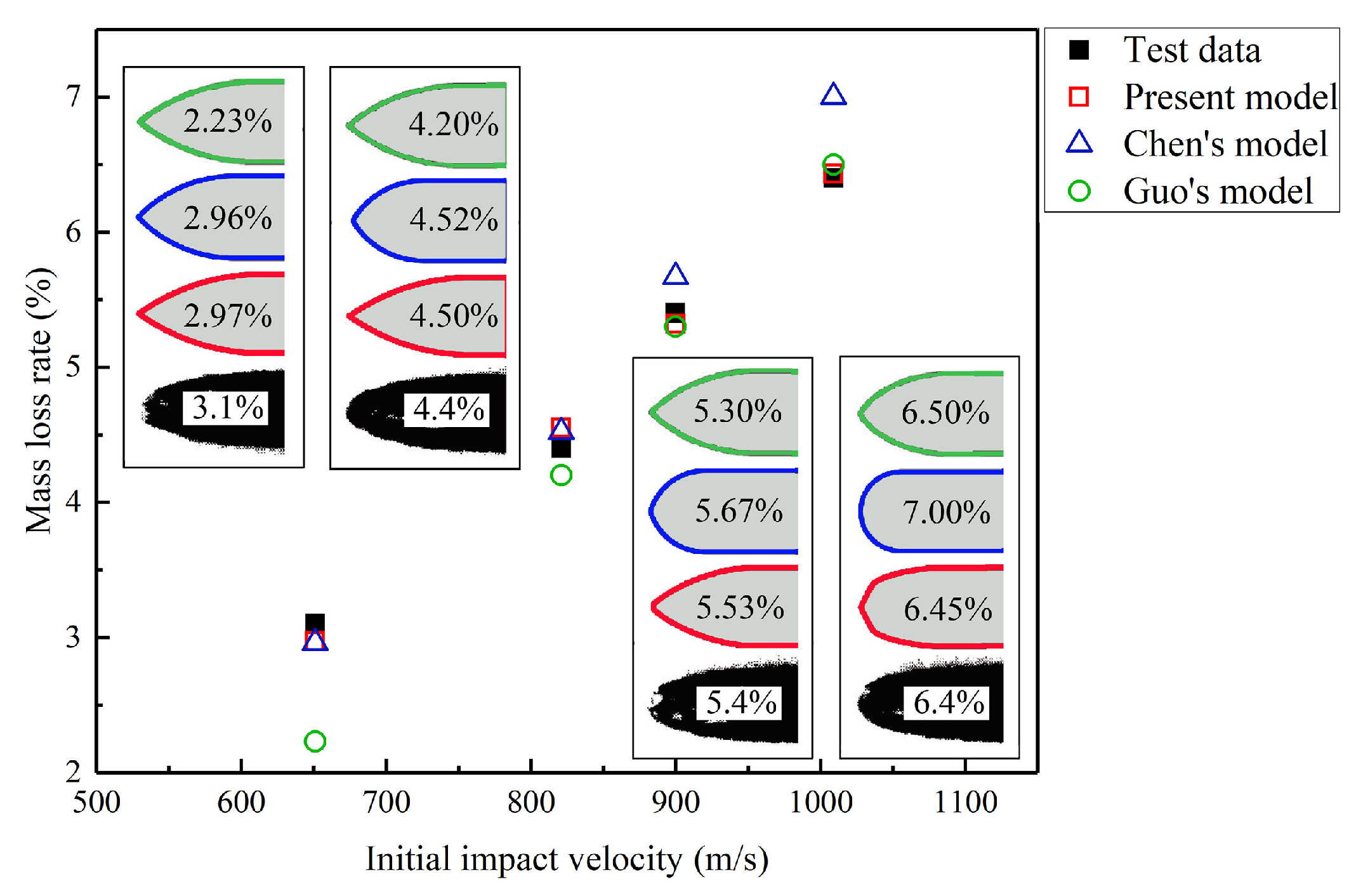
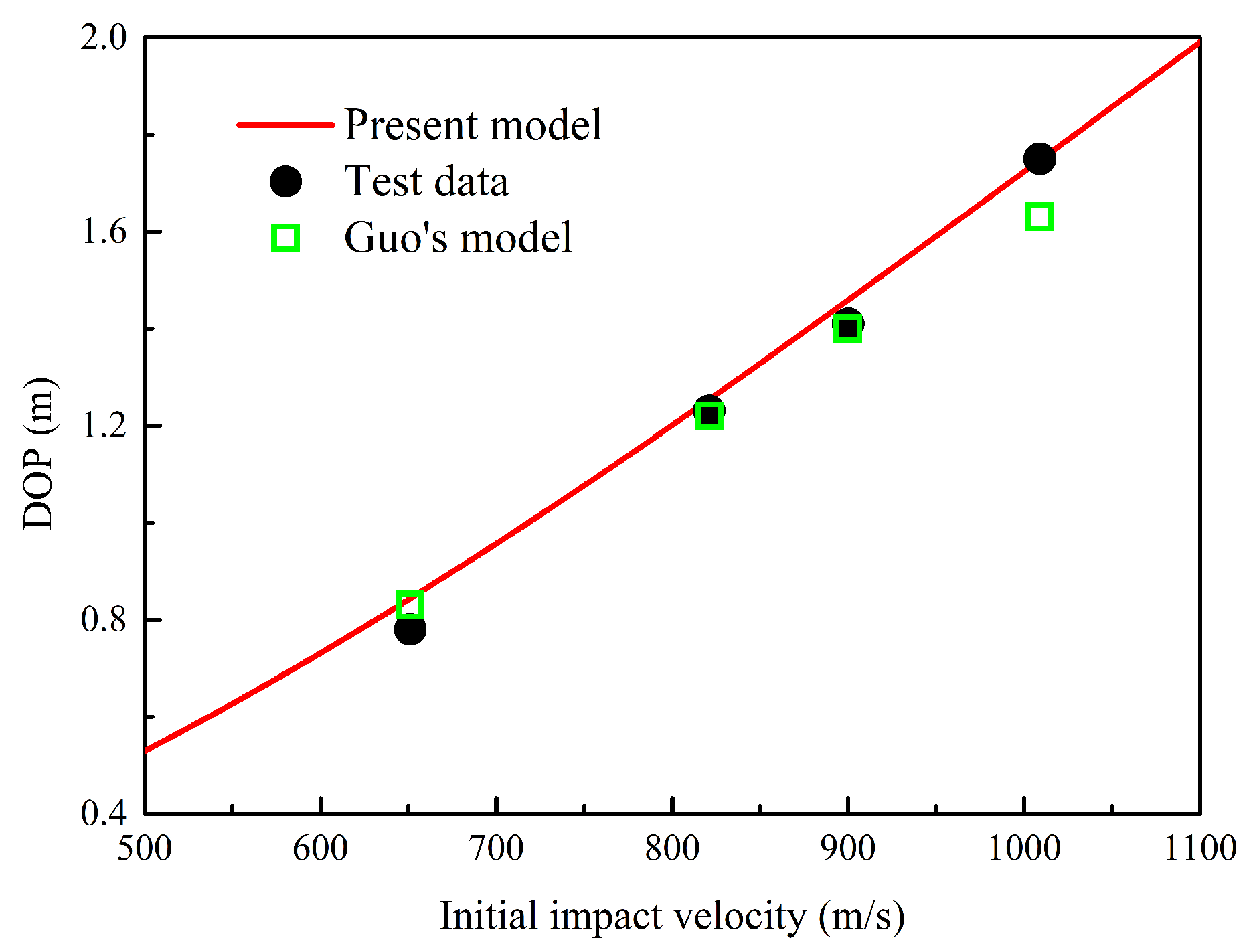
| R | |||||||
| 50.0 | 4.0 | 476.0 | 800.0 | 0.0013 | 0.11 | 0.91 | 2300.0 |
| 7.18 | 52.8 | 1.8076 | 14.4863 | 0.9914 | 4.0962 | 0.22 | 209 |
| Test No. | Initial Impact Velocity (m/s) | DOP (m) | Test Data (m) | Error | Mass Loss Rate (%) | Test Data (%) | Error |
|---|---|---|---|---|---|---|---|
| No. 1 | 1325 | 0.79 | 0.74 | 6.7% | 9.6 | 9.05 | 6.1% |
| No. 2 | 1385 | 0.83 | 0.82 | 1.2% | 10.4 | 9.06 | 14.8% |
| No. 3 | 1386 | 0.83 | 0.70 | 18.6% | 10.5 | 9.77 | 7.5% |
| No. 4 | 1425 | 0.86 | 0.86 | 0.1% | 11.4 | 10.42 | 9.4% |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, Z.; Xu, X. Theoretical Investigation on Failure Behavior of Ogive-Nose Projectile Subjected to Impact Loading. Materials 2020, 13, 5372. https://doi.org/10.3390/ma13235372
Li Z, Xu X. Theoretical Investigation on Failure Behavior of Ogive-Nose Projectile Subjected to Impact Loading. Materials. 2020; 13(23):5372. https://doi.org/10.3390/ma13235372
Chicago/Turabian StyleLi, Zhao, and Xiangzhao Xu. 2020. "Theoretical Investigation on Failure Behavior of Ogive-Nose Projectile Subjected to Impact Loading" Materials 13, no. 23: 5372. https://doi.org/10.3390/ma13235372
APA StyleLi, Z., & Xu, X. (2020). Theoretical Investigation on Failure Behavior of Ogive-Nose Projectile Subjected to Impact Loading. Materials, 13(23), 5372. https://doi.org/10.3390/ma13235372





