Selected Concrete Models Studied Using Willam’s Test
Abstract
1. Introduction
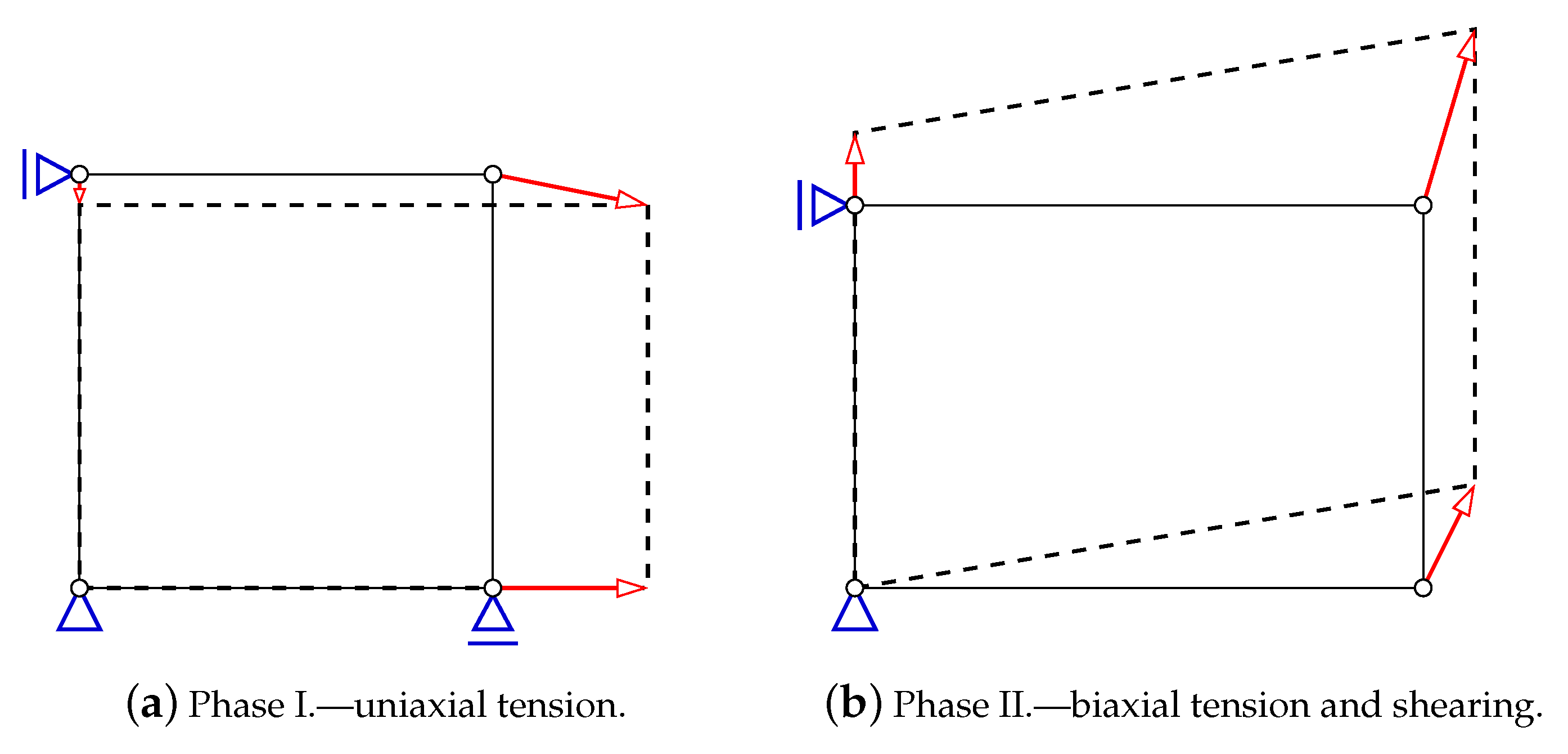
- Phase I.
- Uniaxial horizontal tension with vertical contraction due to the Poisson’s effect, according to the relation between the strain increments:where and are horizontal and vertical strain components, respectively, is shear strain and is Poisson’s ratio. In Figure 1a, the scheme of prescribed displacements corresponds to the uniaxial tensile strain state. Such relation is valid until the tensile strength is attained.
- Phase II.
- Immediately after the tensile strength is reached, the change of configuration is enforced, Figure 1b. Now, the proportions for the strain increments are arranged in the following way:This relation induces tension in two directions with additional shear effect. As a result of such combination, a rotation of principal strain axes occurs; however, tension regime is preserved. At the beginning, the rate of rotation is fast, but, during the evolution, it goes down gradually.
- Condition 1:
- The maximum principal stress is lower than or at most equal to the given uniaxial tensile strength.
- Condition 2:
- All stress components should converge to zero at the final stage.
2. Overview of Studied Models
2.1. Concrete Damaged Plasticity (CDP)
2.2. Concrete Smeared Cracking (CSC)
2.3. Damage-Plasticity (DAP)
2.4. Isotropic Damage (IDA)
3. Testing of Considered Models
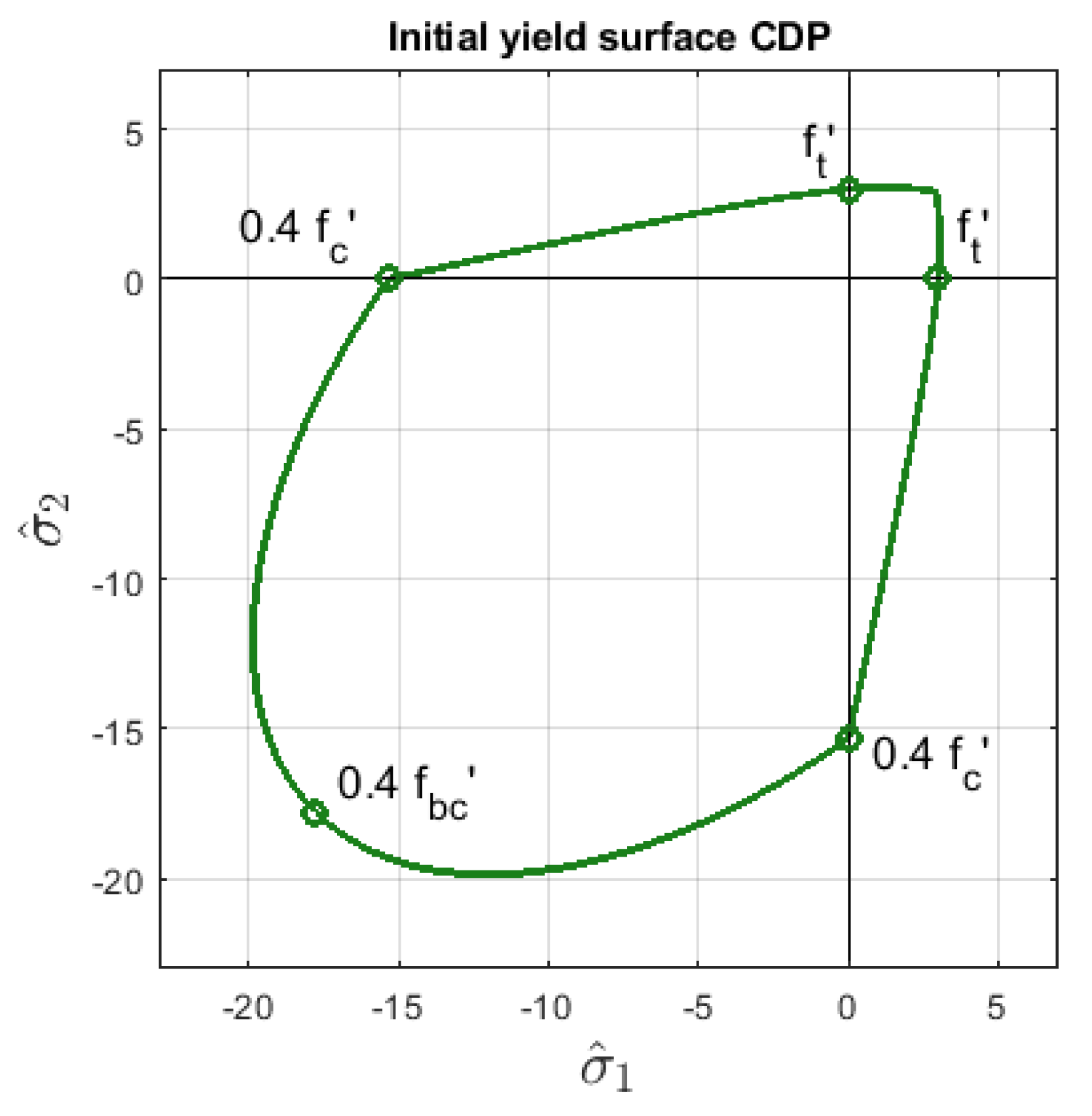
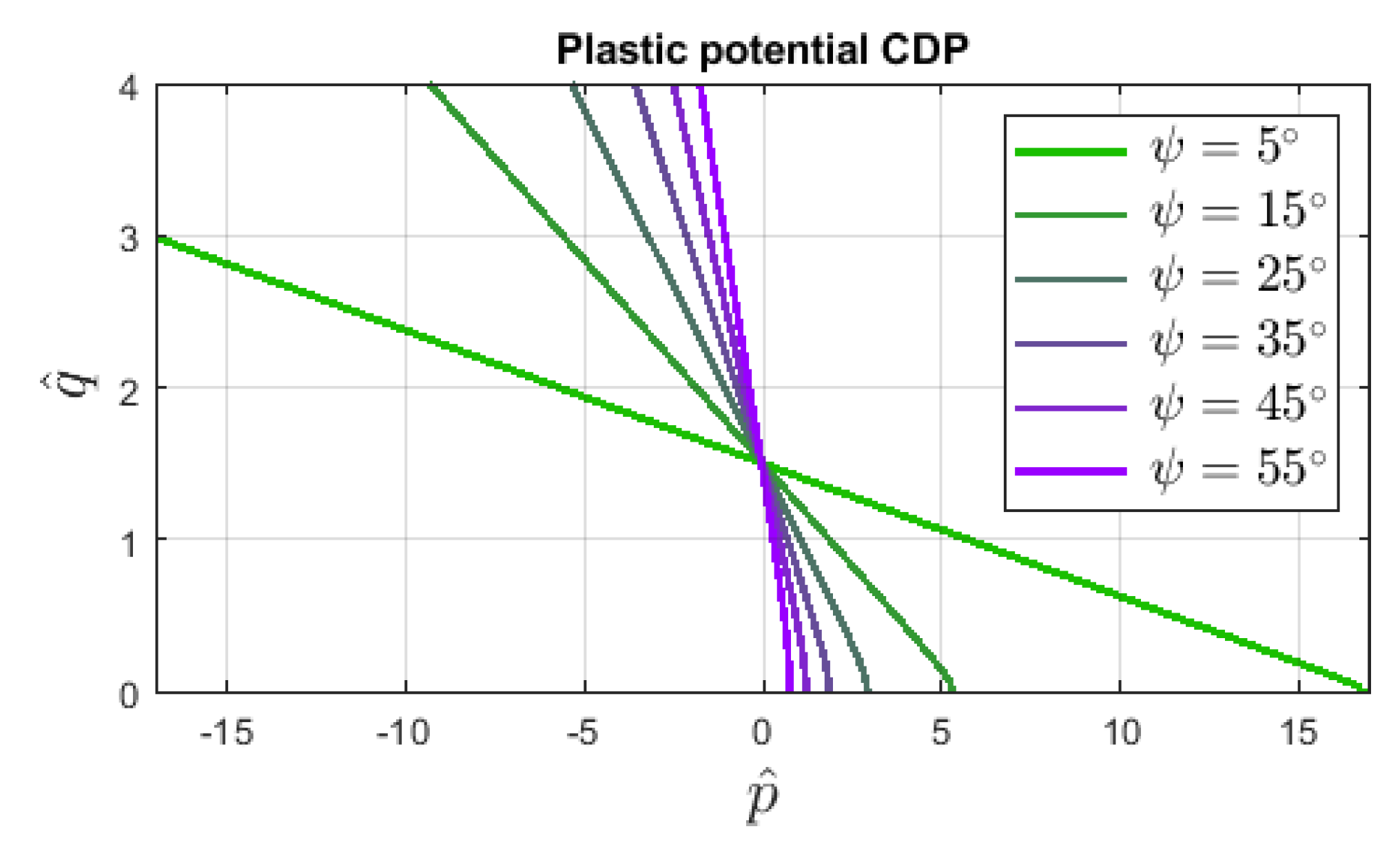
3.1. Concrete Damaged Plasticity (CDP)
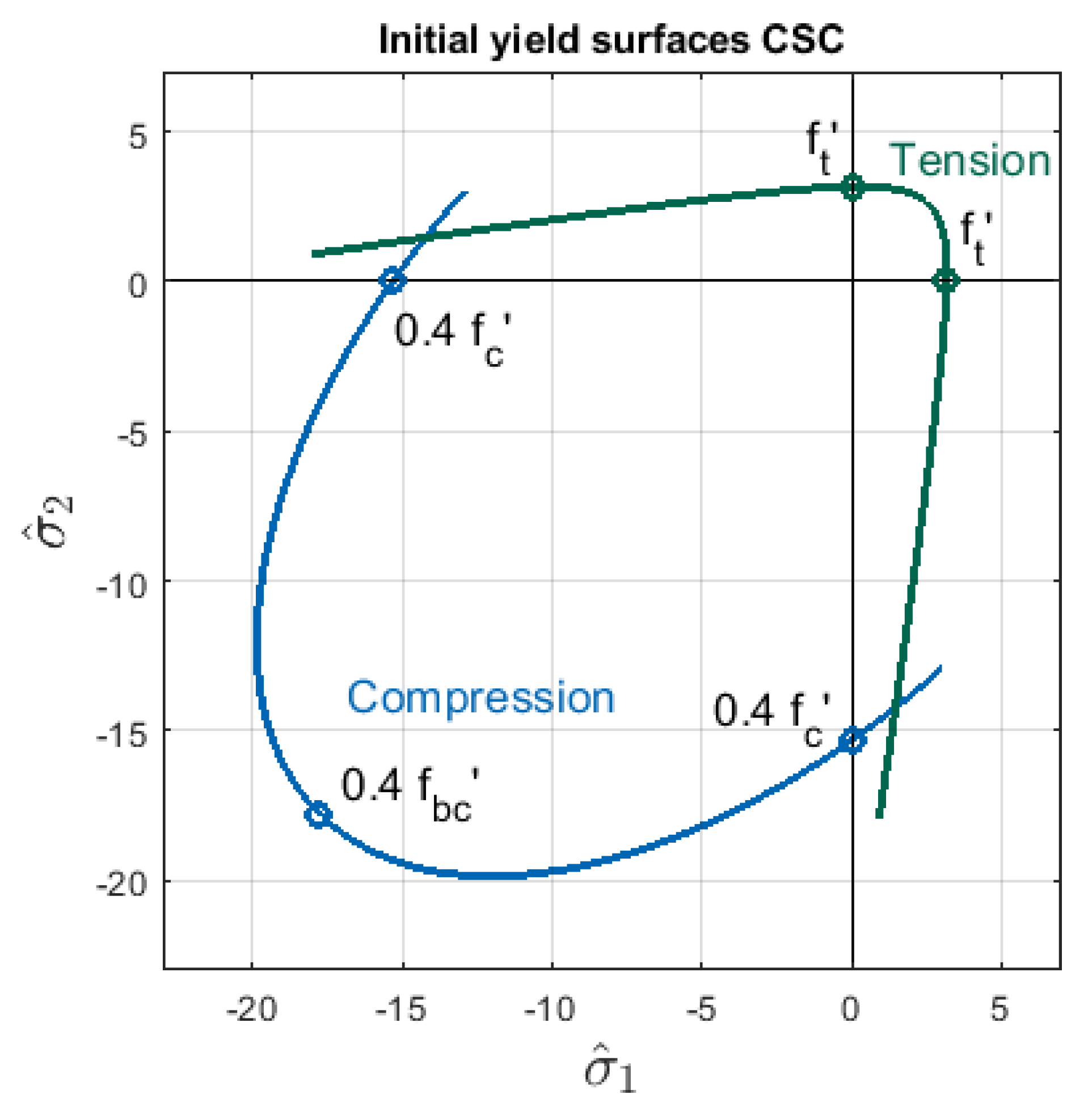
3.2. Concrete Smeared Cracking (CSC)
- the ratio of the biaxial compressive stress to the uniaxial compressive stress equals as the default value,
- the ratio of the uniaxial tensile strength to the maximum uniaxial compressive strength equals for Willam’s test,
- the ratio of the principal plastic strain for biaxial and uniaxial compression, respectively, equals as the default value,
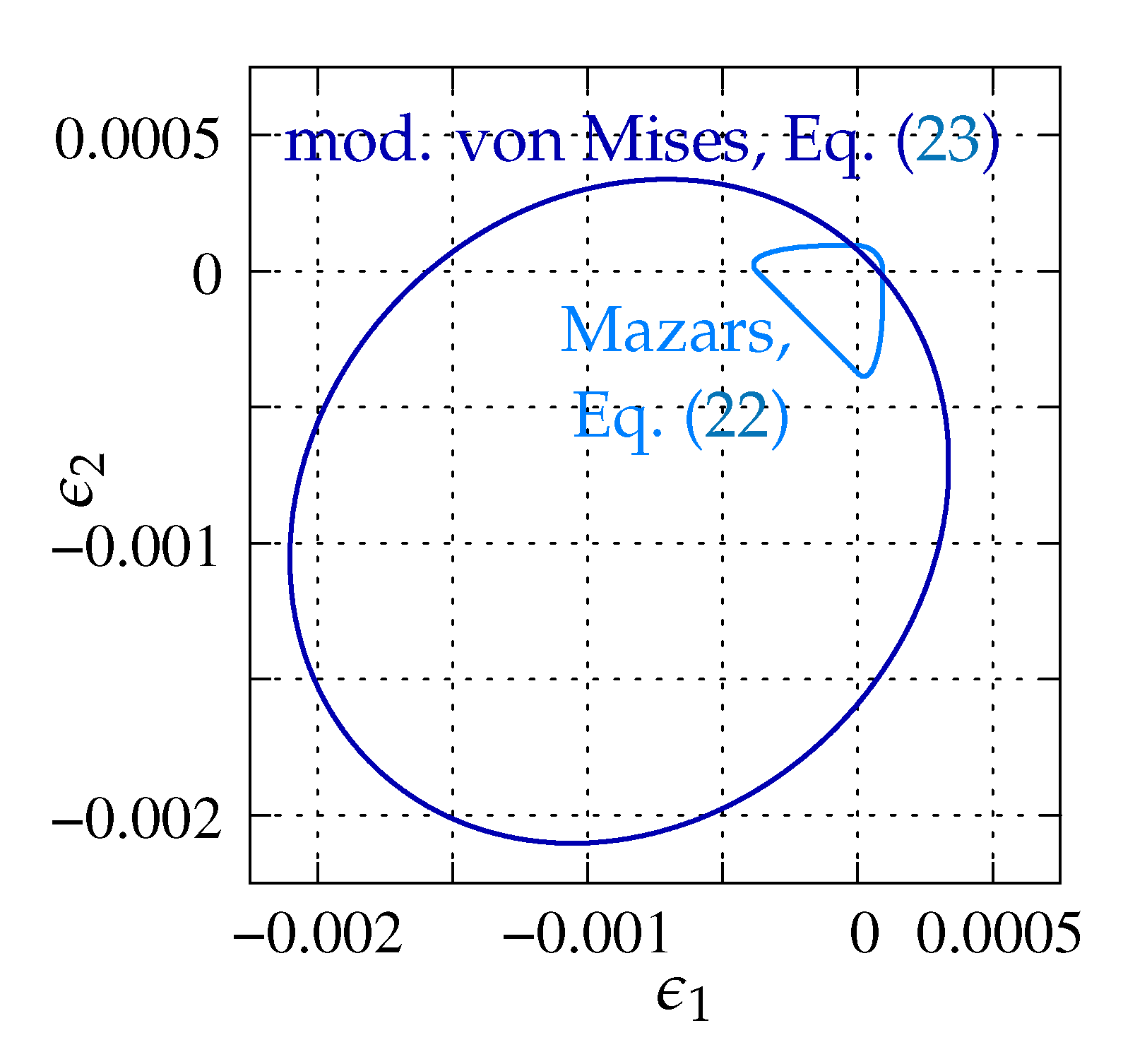
- the ratio of the tensile principal stress at cracking, when is at the ultimate compressive stress, to the tensile cracking stress for uniaxial tension equals as the default value.
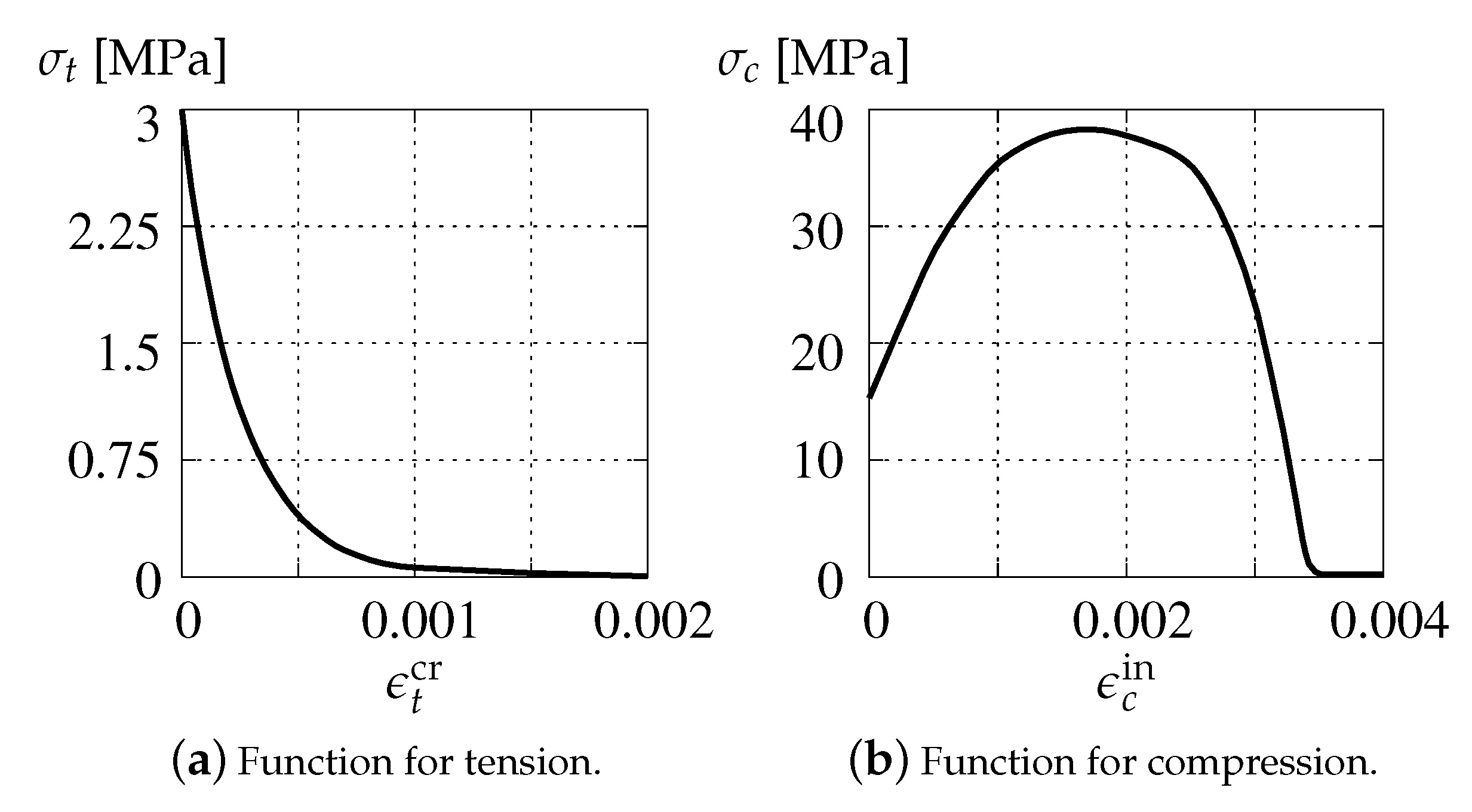
3.3. Damage-Plasticity (DAP)
3.4. Isotropic Damage (IDA)
4. Discussion—Comparison of Models for Principal Stresses and Their Directions
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Abbreviations
| CDP | concrete damaged plasticity model |
| CSC | concrete smeared cracking model |
| DAP | damage-plasticity model |
| FE | finite element |
| FEM | finite element method |
| IDA | isotropic damage model |
| MCFT | modified compression field theory |
| RC | reinforced concrete |
References
- Willam, K.; Pramono, E.; Sture, S. Fundamental issues of smeared crack models. In Proceedings of the SEM-RILEM International Conference on Fracture of Concrete and Rock, Houston, TX, USA, 17–19 June 1987; Shah, S., Swartz, S., Eds.; 1989; pp. 142–157. [Google Scholar] [CrossRef]
- Winnicki, A.; Cichoń, C. Numerical analysis of the plain concrete model prediction for nonproportional loading paths. In Advances in Finite Element Technology; Topping, B., Ed.; Civil-Comp Press: Edinburgh, UK, 1996; pp. 331–339. [Google Scholar]
- Pivonka, P.; Ožbolt, J.; Lackner, R.; Mang, H.A. Comparative studies of 3D-constitutive models for concrete: Application to mixed-mode fracture. Int. J. Numer. Methods Eng. 2004, 60, 549–570. [Google Scholar] [CrossRef]
- SIMULIA. Abaqus Theory Manual (6.14); Dassault Systemes: Providence, RI, USA, 2014. [Google Scholar]
- Taylor, R. FEAP—A Finite Element Analysis Program; Version 7.4, User Manual; University of California: Berkeley, CA, USA, 2001. [Google Scholar]
- Kupfer, H.; Hilsdorf, H.K.; Rusch, H. Behavior of Concrete Under Biaxial Stresses. Am. Concr. Inst. J. 1969, 66, 655–666. [Google Scholar] [CrossRef]
- Carol, I.; Rizzi, E.; Willam, K. On the formulation of anisotropic elastic degradation: II. Generalized pseudo-Rankine model for tensile damage. Int. J. Solids Struct. 2001, 38, 519–546. [Google Scholar] [CrossRef]
- State of the Art Report on Finite Element Analysis of Reinforced Concrete; ASCE Committee 447; American Society of Civil Engineers: New York, NY, USA, 1982.
- Chen, W.F. Plasticity in Reinforced Concrete; McGraw-Hill: New York, NY, USA, 1988. [Google Scholar]
- Hofstetter, G.; Mang, H.A. Computational Mechanics of Reinforced Concrete Structures; Vieweg: Braunschweig, Wiesbaden, Germany, 1995. [Google Scholar]
- Häußler-Combe, U. Computational Methods for Reinforced Concrete Structures; Ernst & Sohn—A Wiley Brand: Berlin, Germany, 2015. [Google Scholar] [CrossRef]
- Sucharda, O.; Konecny, P. Recommendation for the modelling of 3D nonlinear analysis of RC beam tests. Comput. Concr. 2018, 21, 11–20. [Google Scholar] [CrossRef]
- Lubliner, J.; Oliver, J.; Oller, S.; Oñate, E. A plastic-damage model for concrete. Int. J. Solids Struct. 1989, 25, 299–326. [Google Scholar] [CrossRef]
- Lee, J.; Fenves, G.L. Plastic-damage model for cyclic loading of concrete structures. ASCE J. Eng. Mech. 1998, 124, 892–900. [Google Scholar] [CrossRef]
- Lemaitre, J. Evaluation of Dissipation and Damage in Metals. In Proceedings of the First International Conference on Mechanical Behavior of Materials, Kyoto, Japan, 15–20 August 1971; Volume 1, p. 20. [Google Scholar]
- Simo, J.C.; Ju, J.W. Strain- and stress-based continuum damage models—I. Formulation. Int. J. Solids Struct. 1987, 23, 821–840. [Google Scholar] [CrossRef]
- Willam, K.J.; Warnke, E.P. Constitutive Model for Triaxial Behavior of Concrete. In Proceedings of the Concrete Structure Subjected to Triaxial Stresses, Bergamo, Italy, 17–19 May 1974; International Association for Bridge and Structural Engineering, IABSE Proceedings: Zurich, Switzerland, 1975; Volume 19, pp. 1–30. [Google Scholar]
- Ottosen, N.S. A Failure Criterion for Concrete. ASCE J. Eng. Mech. Div. 1977, 103, 527–535. [Google Scholar]
- Menétrey, P.; Willam, K.J. Triaxial Failure Criterion for Concrete and Its Generalization. ACI Struct. J. 1995, 92, 311–318. [Google Scholar] [CrossRef]
- Kmiecik, P.; Kamiński, M. Modelling of reinforced concrete structures and composite structures with concrete strength degradation taken into consideration. Arch. Civ. Mech. Eng. 2011, 11, 623–636. [Google Scholar] [CrossRef]
- Earij, A.; Alfano, G.; Cashell, K.; Zhou, X. Nonlinear three-dimensional finite-element modelling of reinforced-concrete beams: Computational challenges and experimental validation. Eng. Fail. Anal. 2017, 82, 92–115. [Google Scholar] [CrossRef]
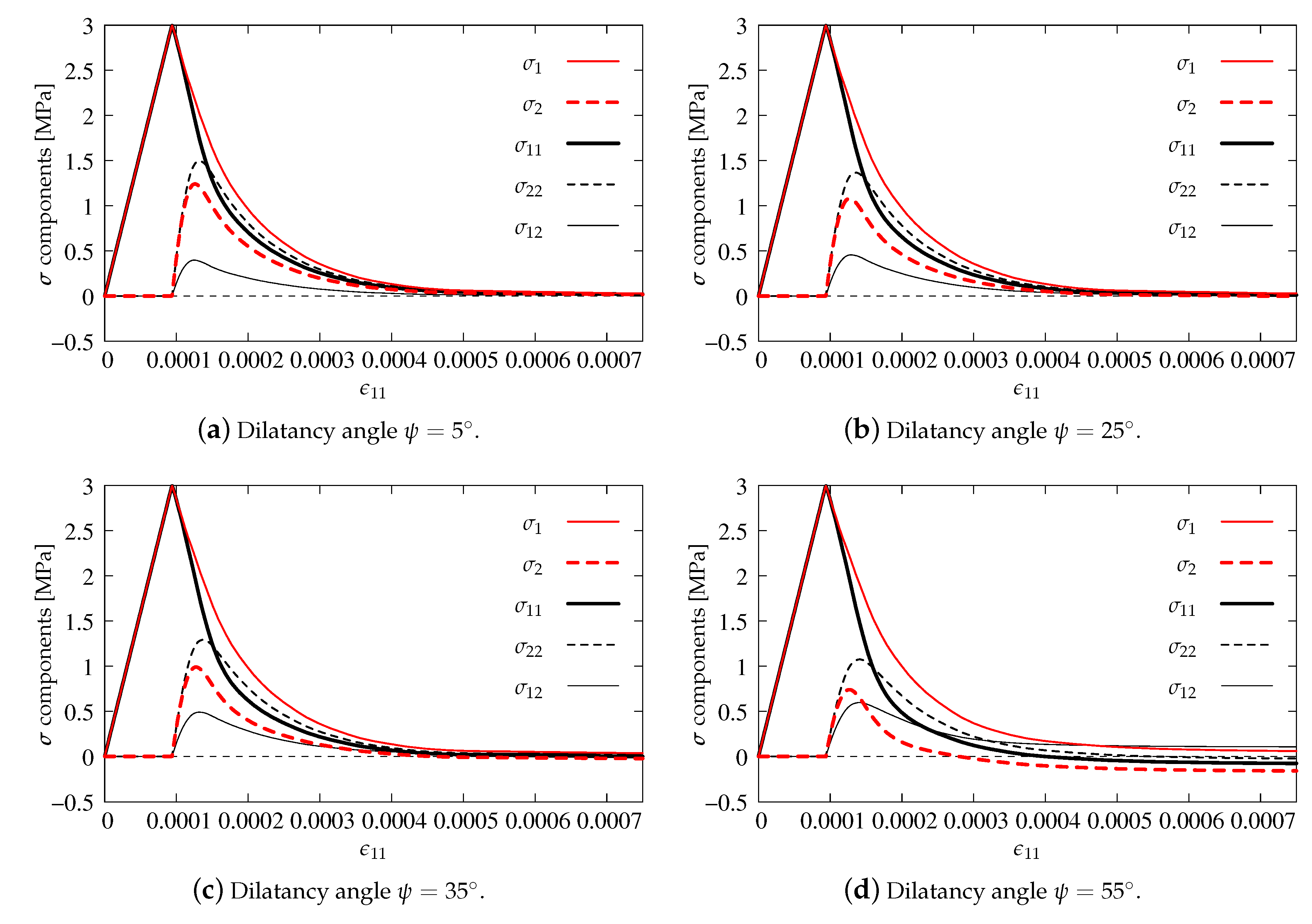
- Wosatko, A.; Winnicki, A.; Polak, M.A.; Pamin, J. Role of dilatancy angle in plasticity-based models of concrete. Arch. Civ. Mech. Eng. 2019, 19, 1268–1283. [Google Scholar] [CrossRef]
- Wosatko, A.; Genikomsou, A.; Pamin, J.; Polak, M.A.; Winnicki, A. Examination of two regularized damage-plasticity models for concrete with regard to crack closing. Eng. Fract. Mech. 2018, 194, 190–211. [Google Scholar] [CrossRef]
- Duvaut, G.; Lions, I.J. Les Inequations en Mechanique et en Physique; Dunod: Paris, France, 1972. [Google Scholar]
- Szczecina, M.; Winnicki, A. Relaxation time in CDP model used for analyses of RC structures. Procedia Eng. 2017, 193, 369–376. [Google Scholar] [CrossRef]
- Rashid, Y.R. Ultimate strength analysis of prestressed concrete pressure vessels. Nucl. Engng. Des. 1968, 7, 334–344. [Google Scholar] [CrossRef]
- Rots, J.G.; Blaauwendraad, J. Crack models for concrete: Discrete or smeared? Fixed, multi-directional or rotating? Heron 1989, 34, 1–59. [Google Scholar]
- Feenstra, P.H. Computational Aspects of Biaxial Stress in Plain and Reinforced Concrete. Ph.D. Thesis, Delft University of Technology, Delft, The Netherlands, 1993. [Google Scholar]
- Weihe, S.; Kröplin, B.; de Borst, R. Classification of smeared crack models based on material and structural properties. Int. J. Solids Struct. 1998, 35, 1289–1308. [Google Scholar] [CrossRef]
- Bažant, Z.P.; Oh, B. Crack band theory for fracture of concrete. RILEM Mater. Struct. 1983, 16, 155–177. [Google Scholar] [CrossRef]
- Jirásek, M.; Bauer, M. Numerical aspects of the crack band approach. Comput. Struct. 2012, 110–111, 60–78. [Google Scholar] [CrossRef]
- Červenka, J.; Červenka, V.; Laserna, S. On crack band model in finite element analysis of concrete fracture in engineering practice. Eng. Fract. Mech. 2018, 197, 27–47. [Google Scholar] [CrossRef]
- Vecchio, F.J.; Collins, M.P. The Modified Compression-Field Theory for Reinforced Concrete Elements Subjected to Shear. ACI J. 1986, 83, 219–231. [Google Scholar]
- Simo, J.C.; Ju, J.W. Strain- and stress-based continuum damage models—II. Computational aspects. Int. J. Solids Struct. 1987, 23, 841–869. [Google Scholar] [CrossRef]
- de Borst, R.; Pamin, J.; Geers, M.G.D. On coupled gradient-dependent plasticity and damage theories with a view to localization analysis. Eur. J. Mech. A/Solids 1999, 18, 939–962. [Google Scholar] [CrossRef]
- Mazars, J. Application de la Mécanique de L’edommagement au Comportement non Linéaire et à la Rupture du béton de Structure. Ph.D. Thesis, Université Paris, Paris, France, 1984. [Google Scholar]
- de Vree, J.H.P.; Brekelmans, W.A.M.; van Gils, M.A.J. Comparison of nonlocal approaches in continuum damage mechanics. Comput. Struct. 1995, 55, 581–588. [Google Scholar] [CrossRef]
- Mazars, J.; Pijaudier-Cabot, G. Continuum damage theory—Application to concrete. ASCE J. Eng. Mech. 1989, 115, 345–365. [Google Scholar] [CrossRef]
- Peerlings, R.H.J.; de Borst, R.; Brekelmans, W.A.M.; de Vree, J.H.P. Gradient-enhanced damage for quasi-brittle materials. Int. J. Numer. Methods Eng. 1996, 39, 3391–3403. [Google Scholar] [CrossRef]
- Pijaudier-Cabot, G.; Benallal, A. Strain localization and bifurcation in a nonlocal continuum. Int. J. Solids Struct. 1993, 30, 1761–1775. [Google Scholar] [CrossRef]
- Jirásek, M. Nonlocal models for damage and fracture: Comparison of approaches. Int. J. Solids Struct. 1998, 35, 4133–4145. [Google Scholar] [CrossRef]
- Grassl, P.; Jirásek, M. Plastic model with non-local damage applied to concrete. Int. J. Numer. Anal. Methods Geomech. 2006, 30, 71–90. [Google Scholar] [CrossRef]
- Skrzypek, J.; Ganczarski, A. Modelling of Material Damage and Failure of Structures: Theory and Applications; Springer: Berlin, Germany; New York, NY, USA, 1999. [Google Scholar] [CrossRef]
- Lemaitre, J.; Desmorat, R. Engineering Damage Mechanics: Ductile, Creep, Fatigue and Brittle Failures; Springer: Berlin, Germany, 2005. [Google Scholar] [CrossRef]
- Comi, C. A non-local model with tension and compression damage mechanisms. Eur. J. Mech. A/Solids 2001, 20, 1–22. [Google Scholar] [CrossRef]
- Wu, J.Y.; Li, J.; Faria, R. An energy release rate-based plastic-damage model for concrete. Int. J. Solids Struct. 2006, 43, 583–612. [Google Scholar] [CrossRef]
- Pröchtel, P.; Häußler-Combe, U. On the dissipative zone in anisotropic damage models for concrete. Int. J. Solids Struct. 2008, 45, 4384–4406. [Google Scholar] [CrossRef][Green Version]
- Wosatko, A. Gradient damage with volumetric-deviatoric decomposition and one strain measure. Mech. Control 2011, 30, 254–263. [Google Scholar]
- Desmorat, R.; Gatuingt, F.; Ragueneau, F. Nonlocal anisotropic damage model and related computational aspects for quasi-brittle materials. Eng. Fract. Mech. 2007, 74, 1539–1560. [Google Scholar] [CrossRef]
- Ragueneau, F.; Desmorat, R.; Gatuingt, F. Anisotropic damage modelling of biaxial behavior and rupture of concrete structures. Comput. Concr. 2008, 5, 417–434. [Google Scholar] [CrossRef]
- Pamin, J.; Wosatko, A.; Desmorat, R. A volumetric upgrade of scalar gradient damage model. In Proceedings of the EURO-C 2014 International Conference Computational Modelling of Concrete Structures, St. Anton Am Arlberg, Austria, 24–27 March 2014; Taylor and Francis: Boca Raton, FL, USA; London, UK; New York, NY, USA; Leiden, The Netherlands, 2014; pp. 289–298. [Google Scholar] [CrossRef]
- Hognestad, E. A Study of Combined Bending Axial Load in Reinforced Concrete Members; Bulletin Series No. 399; The Engineering Experimental Station, University of Illinoins: Urbana, IL, USA, 1951; Volume 49. [Google Scholar]
- International Federation for Structural Concrete (FIB) (Ed.). fib Model Code for Concrete Structures 2010; Ernst & Sohn: Lausanne, Switzerland, 2013. [Google Scholar] [CrossRef]
- Stoner, J.G.; Polak, M.A. Finite element modelling of GFRP reinforced concrete beams. Comput. Concr. 2020, 25, 369–382. [Google Scholar] [CrossRef]
- Pamin, J.; de Borst, R. Stiffness degradation in gradient-dependent coupled damage-plasticity. Arch. Mech. 1999, 51, 419–446. [Google Scholar]
- Jankowiak, T.; Łodygowski, T. Quasi-Static Failure Criteria for Concrete. Arch. Civ. Eng. 2010, 56, 123–154. [Google Scholar] [CrossRef]
- Nzabonimpa, J.D.; Hong, W.K.; Kim, J. Nonlinear finite element model for the novel mechanical beam-column joints of precast concrete-based frames. Comput. Struct. 2017, 189, 31–48. [Google Scholar] [CrossRef]

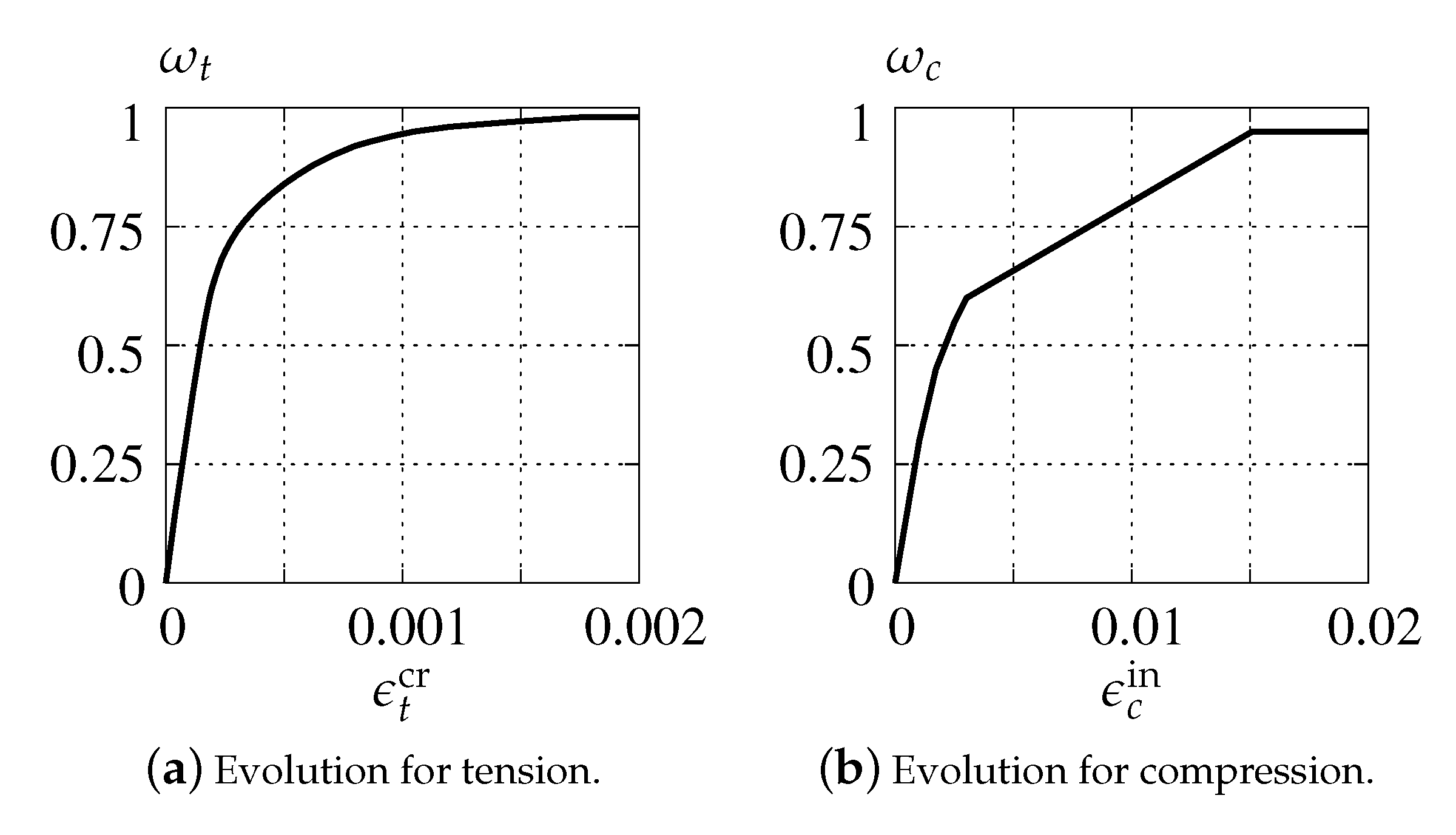
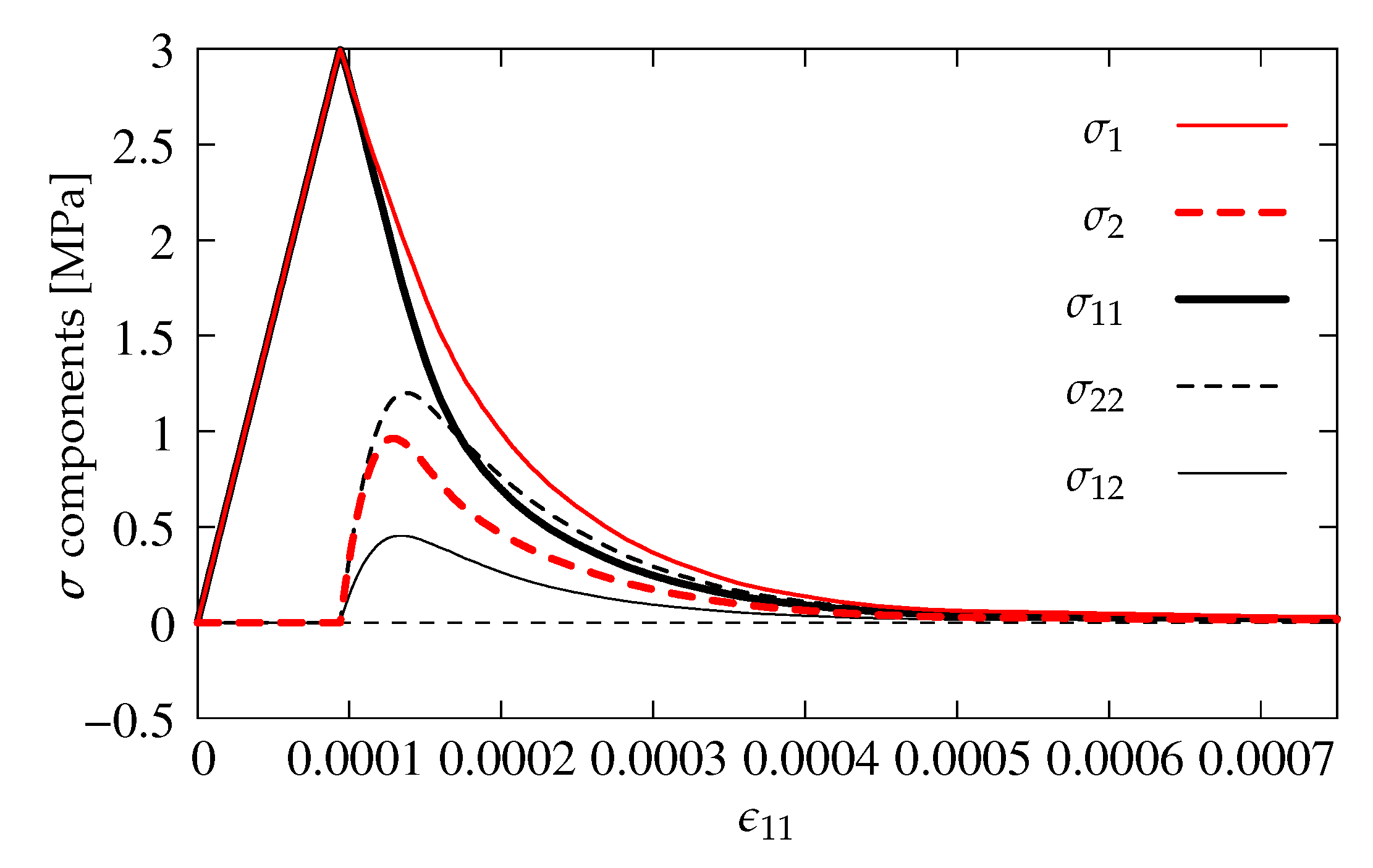
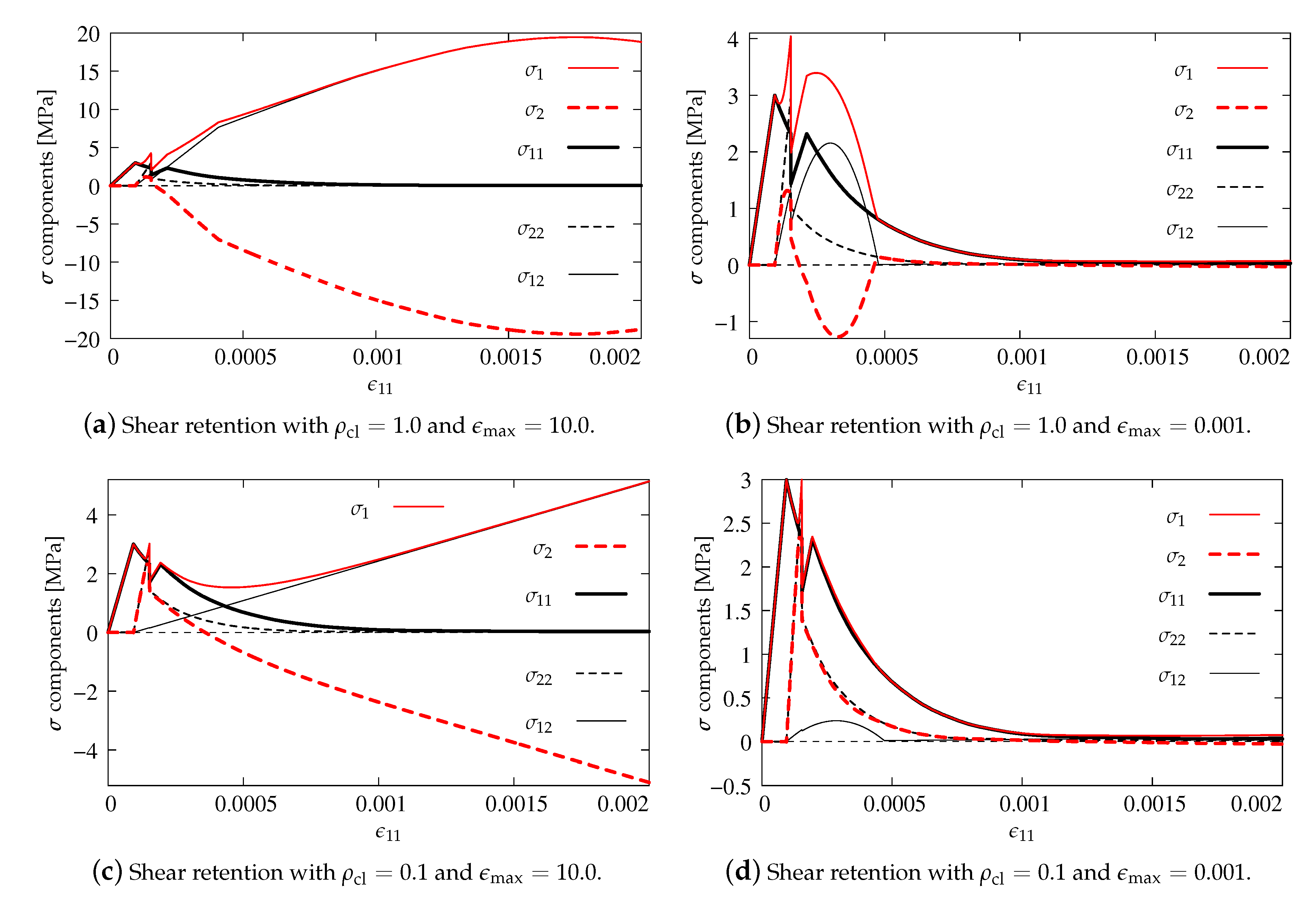
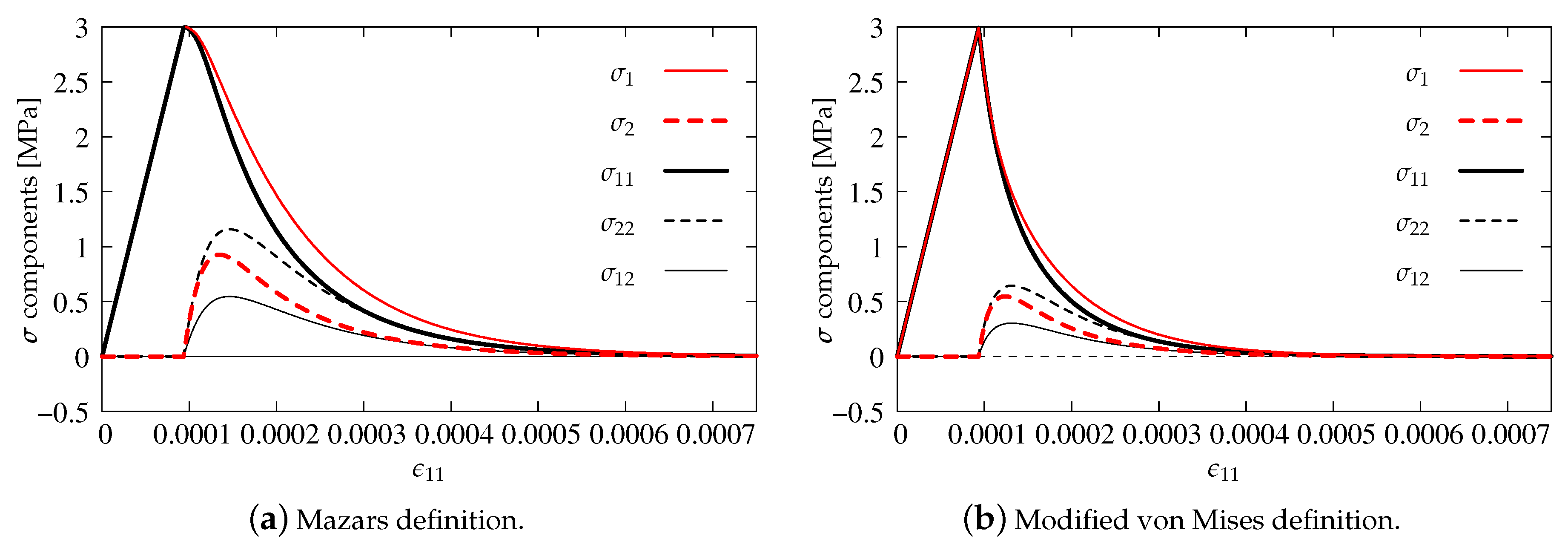



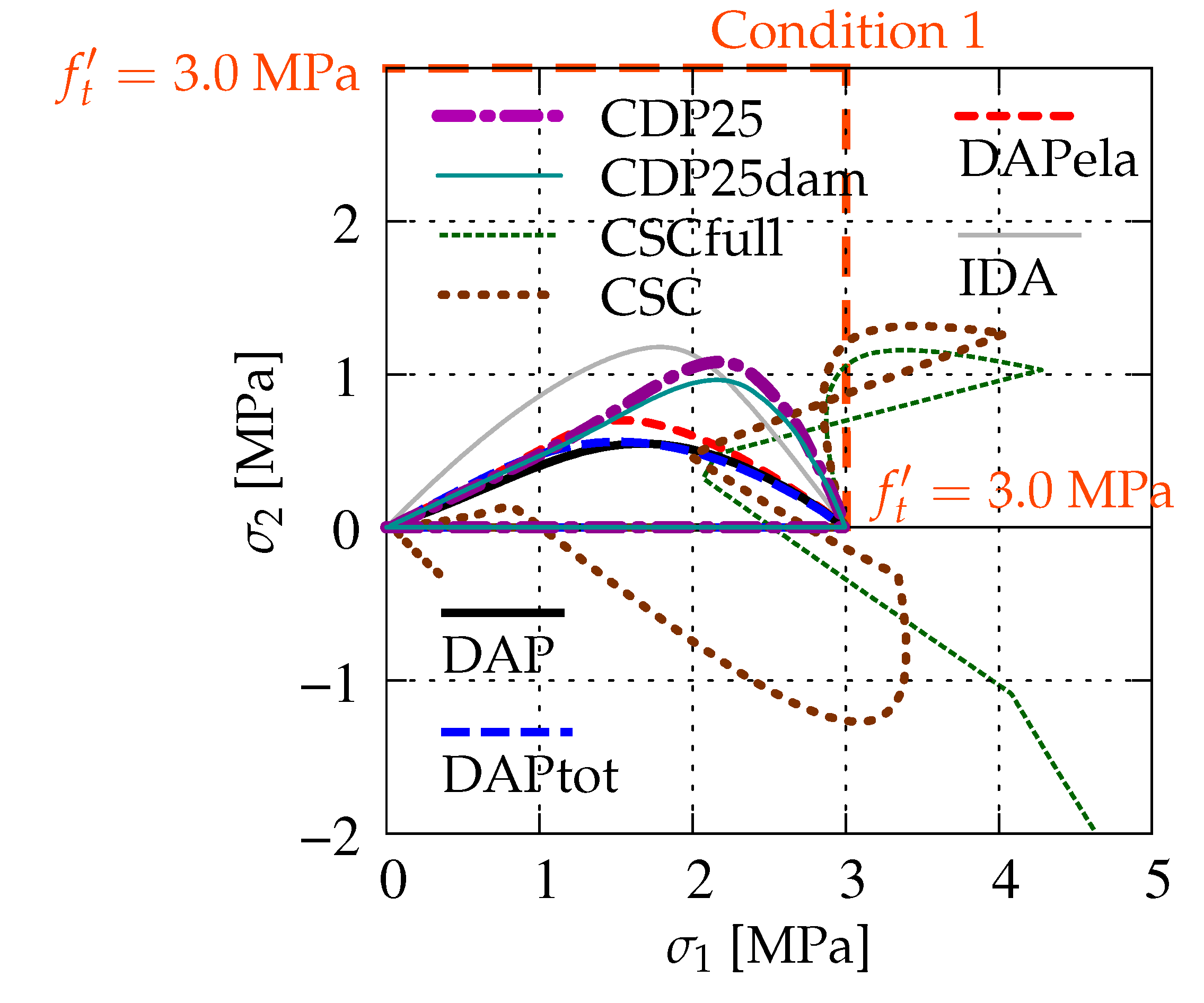

| Acronym | Model | Crucial details | Figure |
|---|---|---|---|
| CDP25 | concrete damaged plasticity | dilatancy angle | Figure 9b |
| CDP25dam | concrete damaged plasticity | dilatancy angle , tensile damage—Figure 8a | Figure 10 |
| CSCfull | concrete smeared cracking | shear retention: , | Figure 11a |
| CSC | concrete smeared cracking | shear retention: , | Figure 11b |
| DAP | damage | modified von Mises definition | Figure 12b |
| DAPtot | damage-plasticity | coupling by total strains | Figure 13a |
| DAPela | damage-plasticity | coupling by elastic strains | Figure 13b |
| IDA | isotropic damage | power | Figure 14c |
| Model | Condition | Final | Restriction | |
|---|---|---|---|---|
| Acronym | 1 | 2 | Assessment | of Usage |
| CDP | + | conditionally | ||
| CSC | − | no | ||
| DAP | + | + | yes | |
| IDA | + | conditionally | ||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wosatko, A.; Szczecina, M.; Winnicki, A. Selected Concrete Models Studied Using Willam’s Test. Materials 2020, 13, 4756. https://doi.org/10.3390/ma13214756
Wosatko A, Szczecina M, Winnicki A. Selected Concrete Models Studied Using Willam’s Test. Materials. 2020; 13(21):4756. https://doi.org/10.3390/ma13214756
Chicago/Turabian StyleWosatko, Adam, Michał Szczecina, and Andrzej Winnicki. 2020. "Selected Concrete Models Studied Using Willam’s Test" Materials 13, no. 21: 4756. https://doi.org/10.3390/ma13214756
APA StyleWosatko, A., Szczecina, M., & Winnicki, A. (2020). Selected Concrete Models Studied Using Willam’s Test. Materials, 13(21), 4756. https://doi.org/10.3390/ma13214756





