Physical Fundamentals of Biomaterials Surface Electrical Functionalization
Abstract
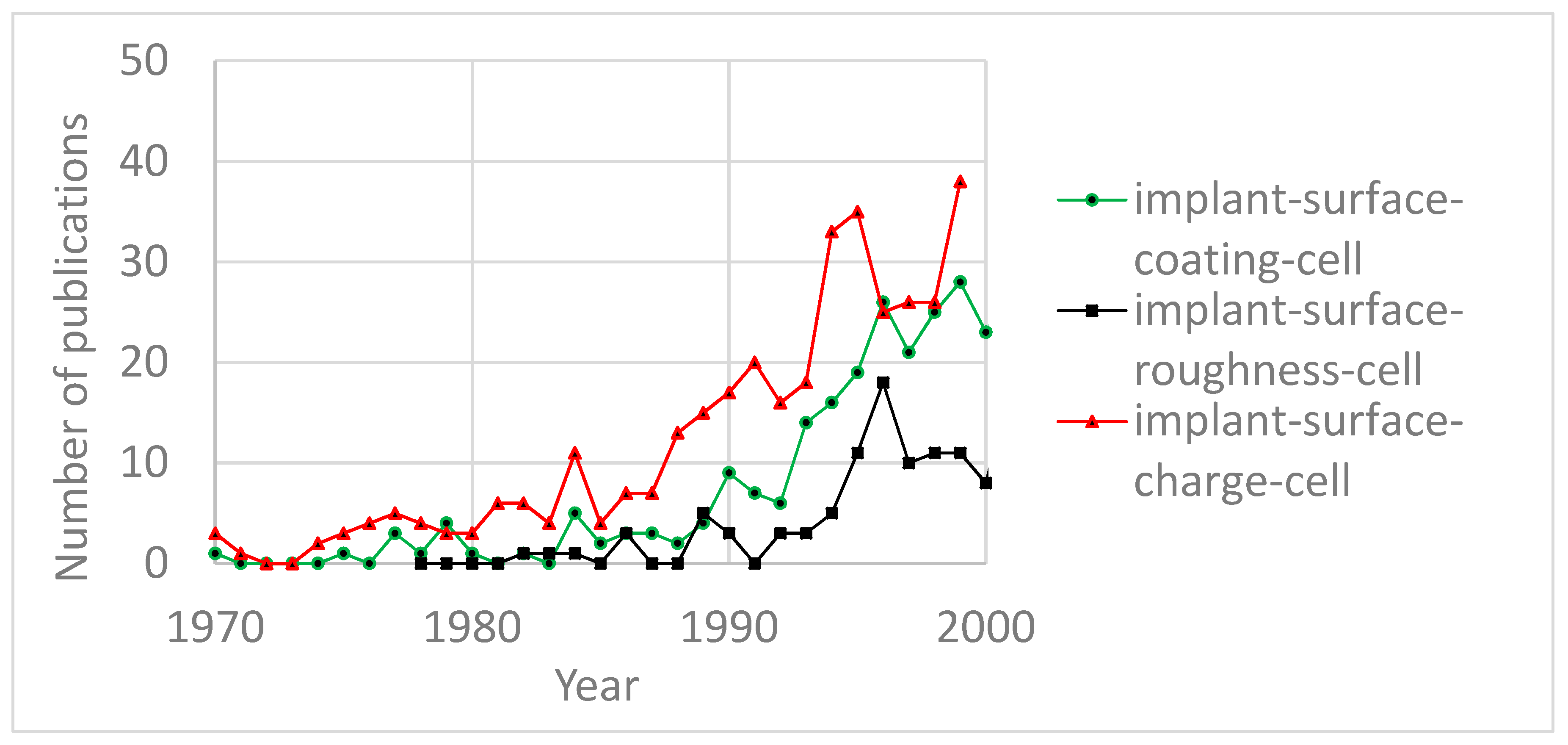
:1. Introduction
- the engineering of a surface’s geometry (morphology)
- the engineering of a surface’s physicochemical properties.
- there are no model experiments that decouple the influence of surface charge on attachment of microorganisms from surface roughness, i.e., cell deposition studies on smooth surfaces with evenly distributed surface charge are necessary.
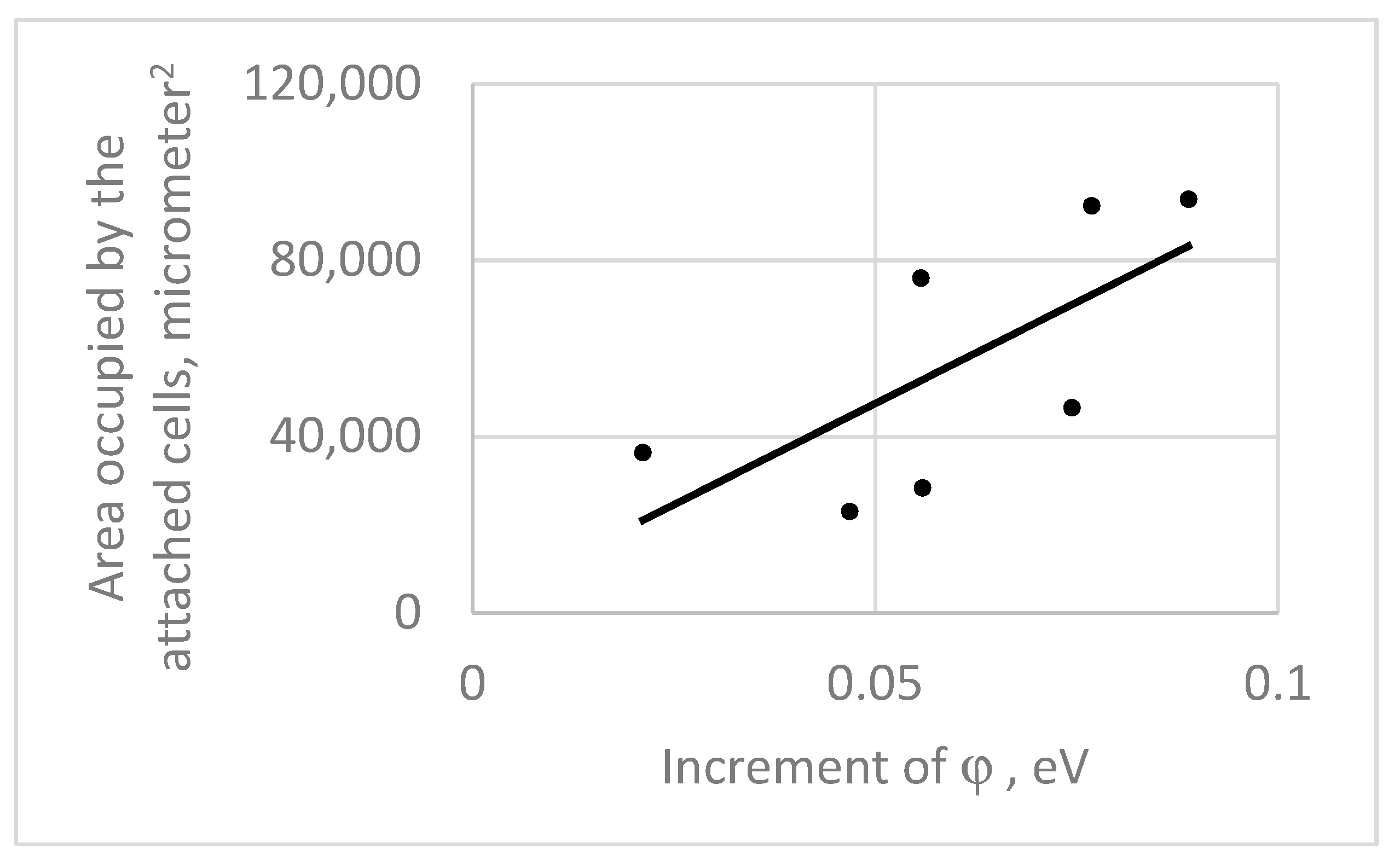
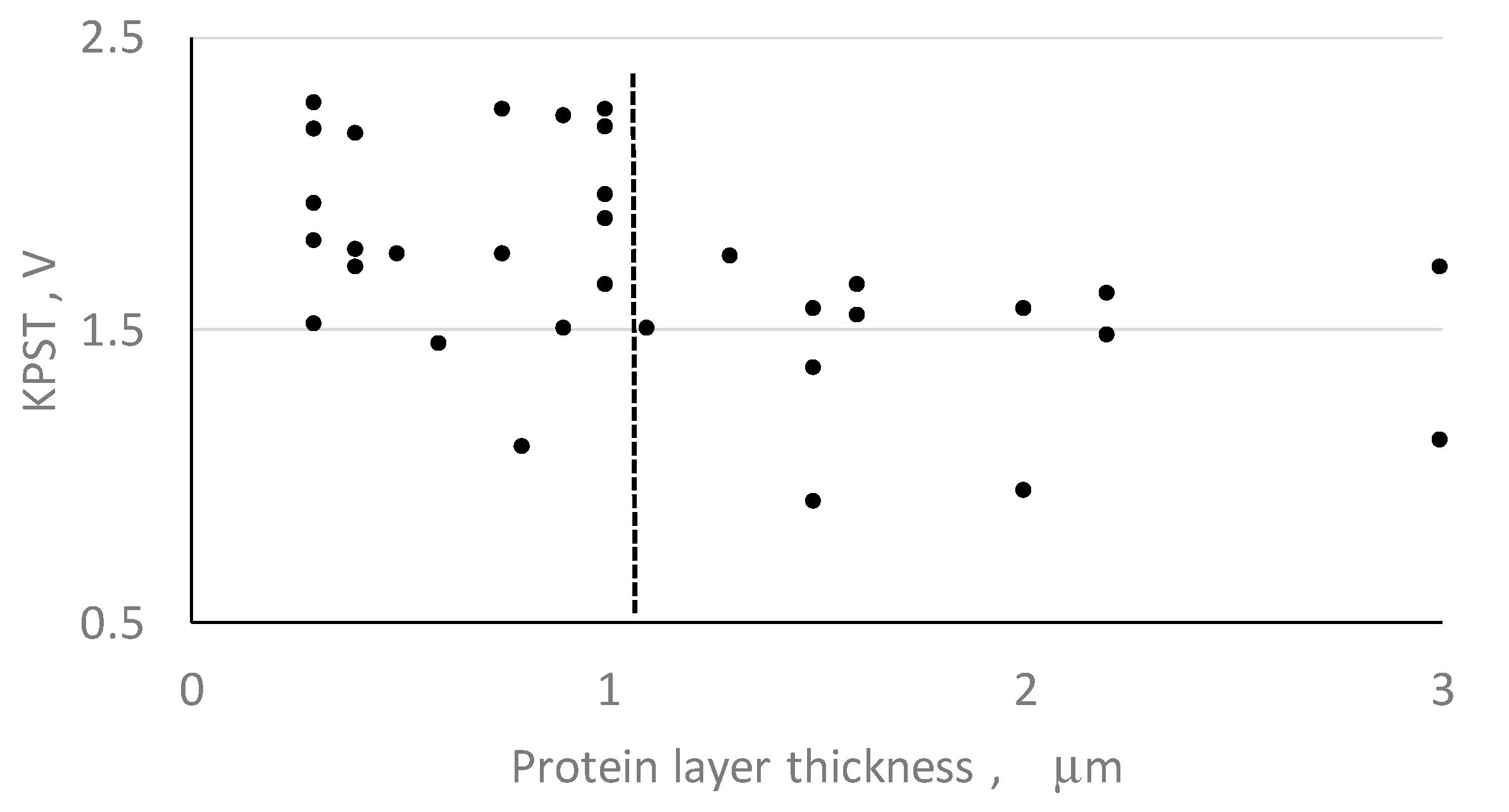
- the influence of a protein layer (which forms around cells after they become attached to a surface within a host) on surface charge shielding.
- there are no model experiments that identify the influence of controlled surface roughness on the attachment of microorganisms when surface charge is constant, i.e., cell deposition studies on surfaces with controlled roughness are necessary.
- since real HAp ceramics have complex point defects in their structure, their influence on the accumulation of charge on the surfaces of HAp ceramics needs to be studied with numerical simulations preceding physical experiments.
- the influence of oxygen vacancies of HAp on its surface electrical charge remains unknown (HAp (Ca5(PO4)3OH) has the highest amount (60%) of oxygen atoms against the others); this should first be assessed using numerical simulations;
- experimental evidence that point defects of HAp influence its surface electrical charge.
2. Attachment of Microorganisms to the Even Charged Surface
2.1. Specimens and Methods
2.2. Results and Discussion
3. Protein Layer as a Surface Charge Shield
3.1. Specimens and Methods
3.2. Results and Discussion
4. Controlled Surface Roughness to Attach Microorganisms at the Constant Surface Charge Condition
4.1. Specimens and Methods
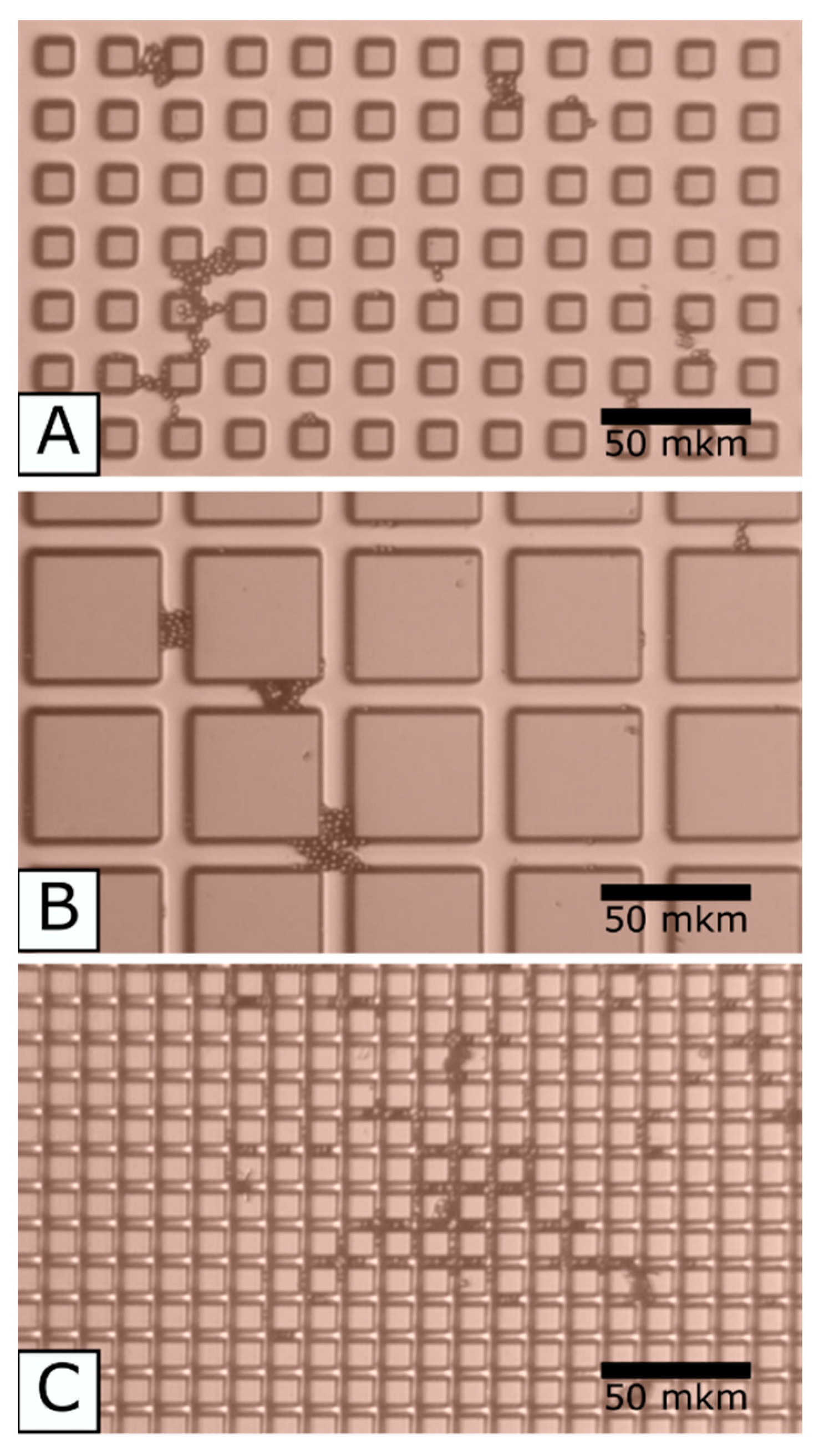
- equal cross-sections, however, different distances between the pillars (A + C).
- equal distances between the pillars, however, different cross-sections (A + B).
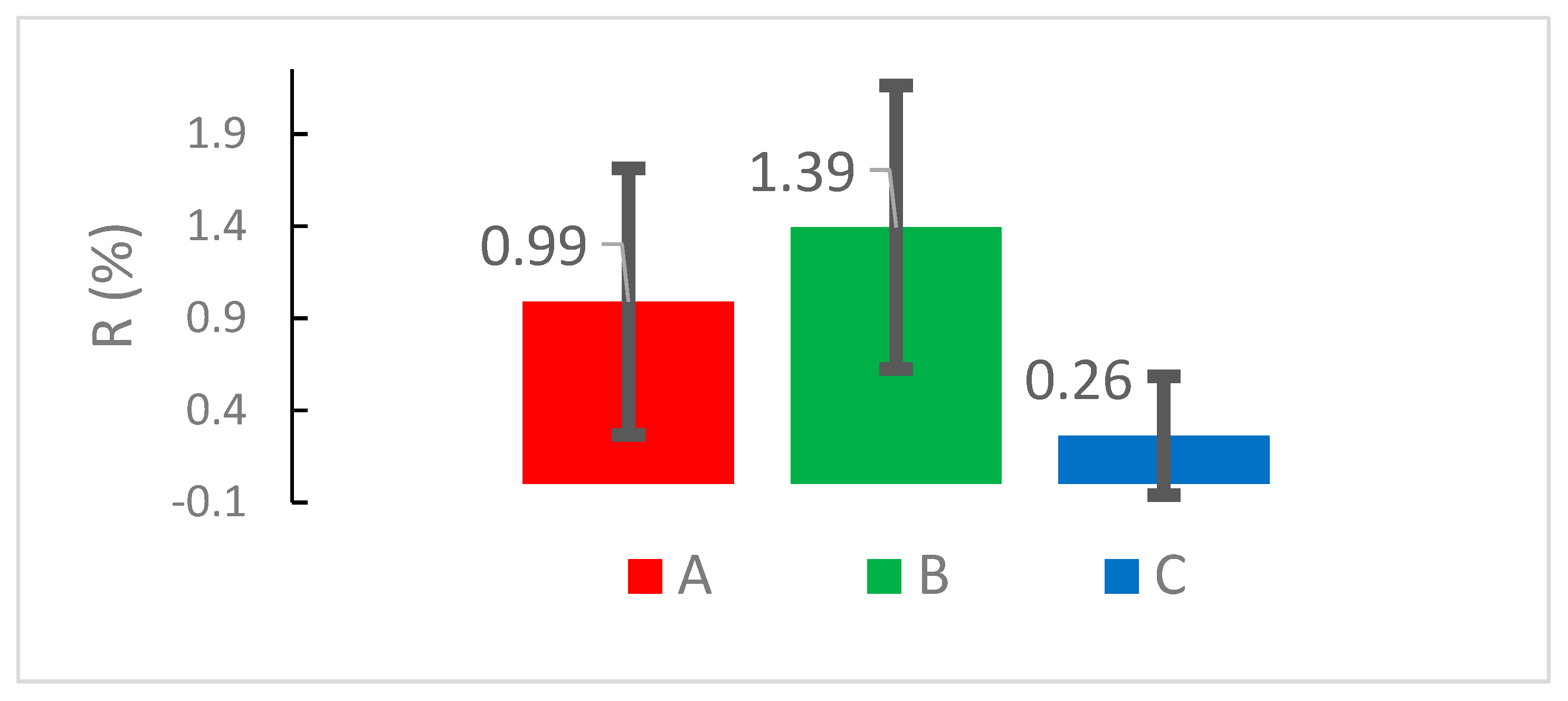
4.2. Results and Discussion
5. Complex Point Defects of HAp toward Induction of Its Surface Electrical Charge
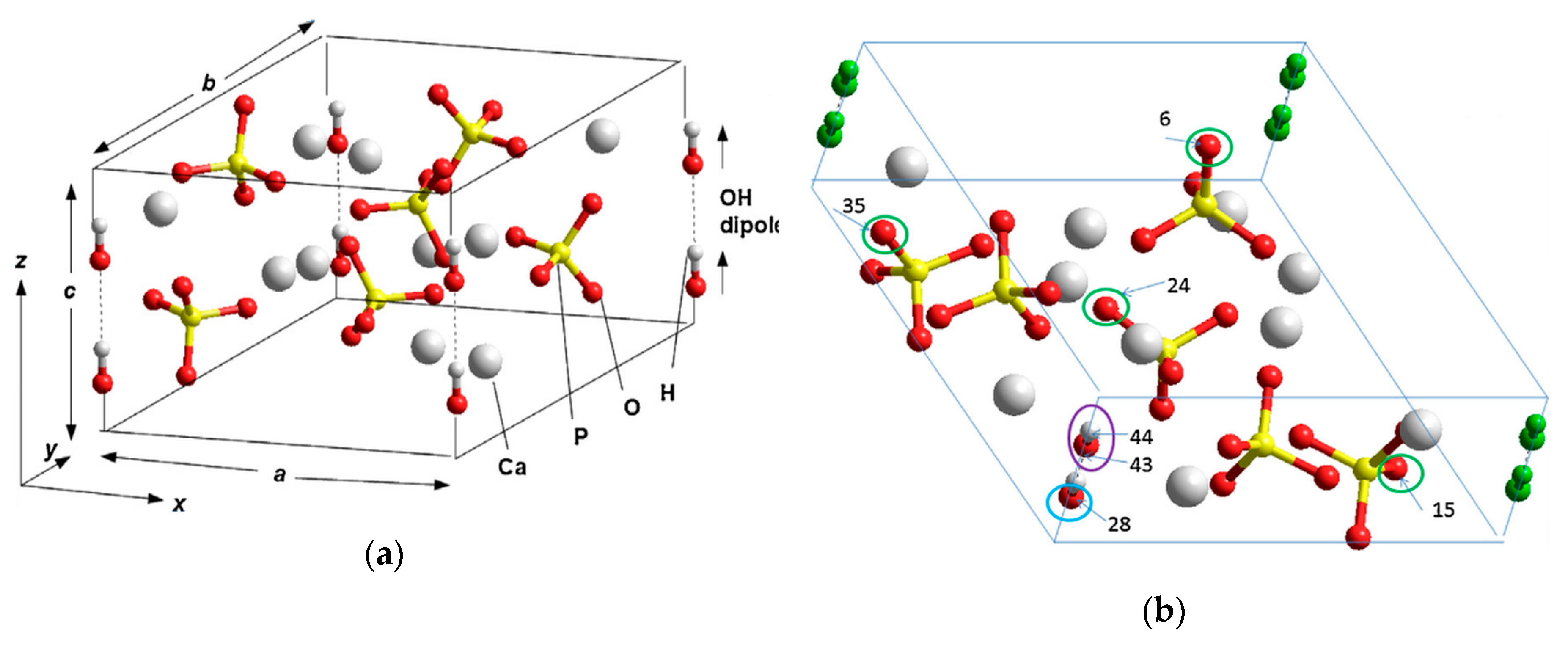
5.1. The Main Particularities of HAp Structure
- as a rule, either ceramics or a powder of various kinds of micro- and nanoparticles with a set of grains of different sizes is used (with its own statistical spread around some average size).
- basically, in this case, the crystal structure of HAp grains has a hexagonal phase (P63), stochastically disordered with respect to the orientation of OH groups (dipoles) in OH-channels, although they can be ordered (that is, parallel oriented OH structures), as well as monoclinic phases (P21).
- the main thing is that in this case, HAp has many different structural defects and this structural imperfection and inhomogeneity of it leads both to a scatter of its physical properties observed in the experiments and to noticeable differences in its physical properties from those theoretically calculated data by various methods for periodic crystal structures of HAp without defects.
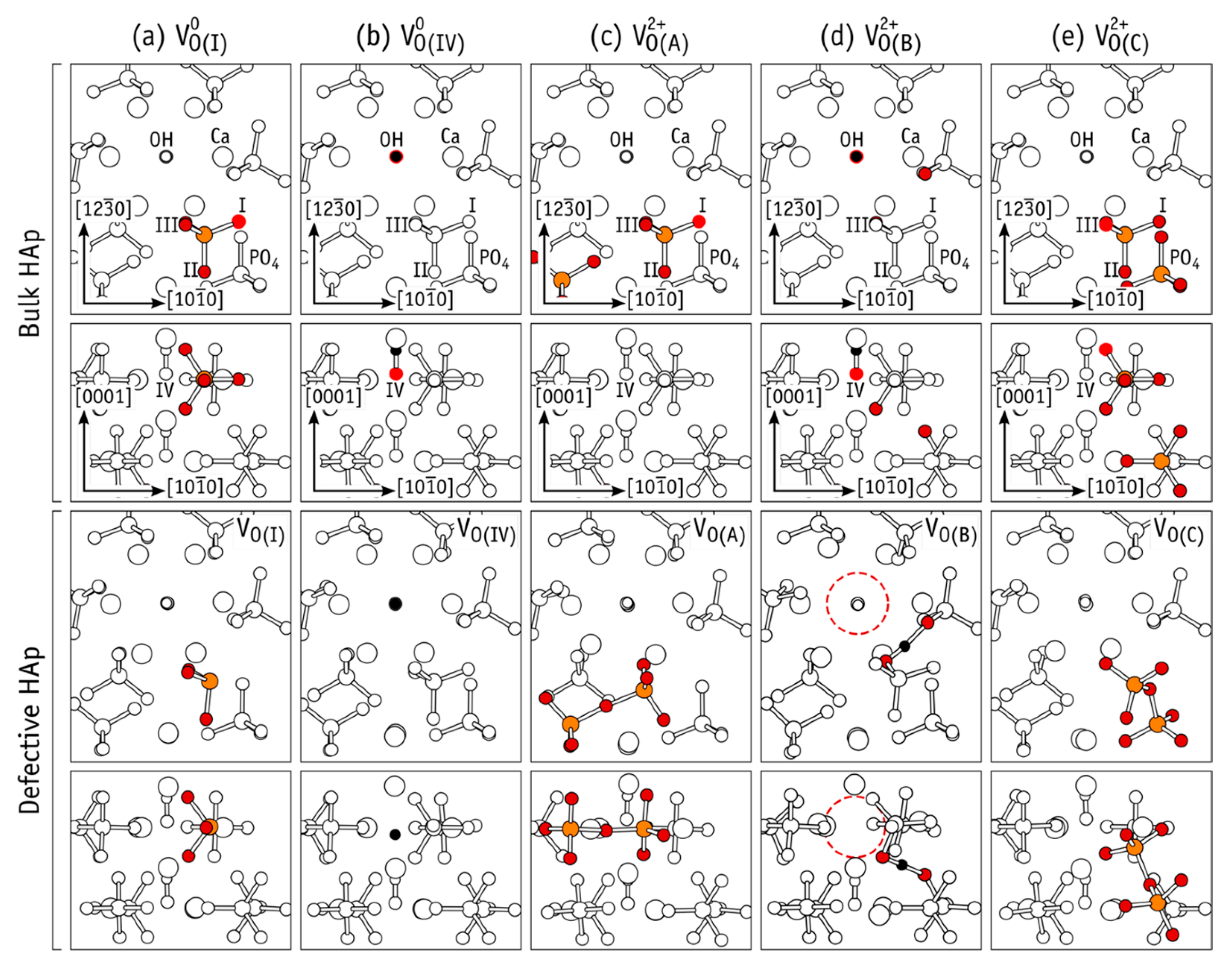
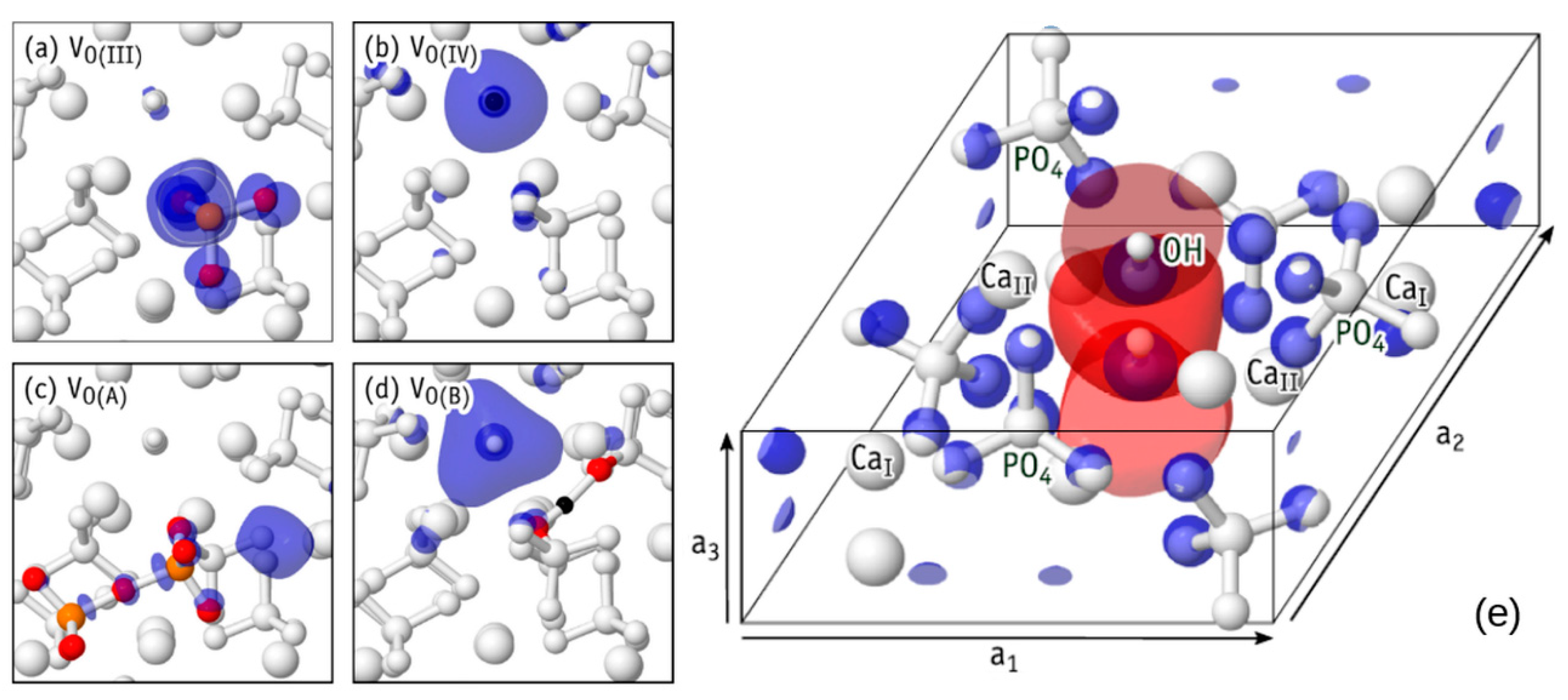
5.2. Oxygen Originated Complex HAp Defects
5.2.1. Kohn−Sham Energy Levels of Neutral VO Defects
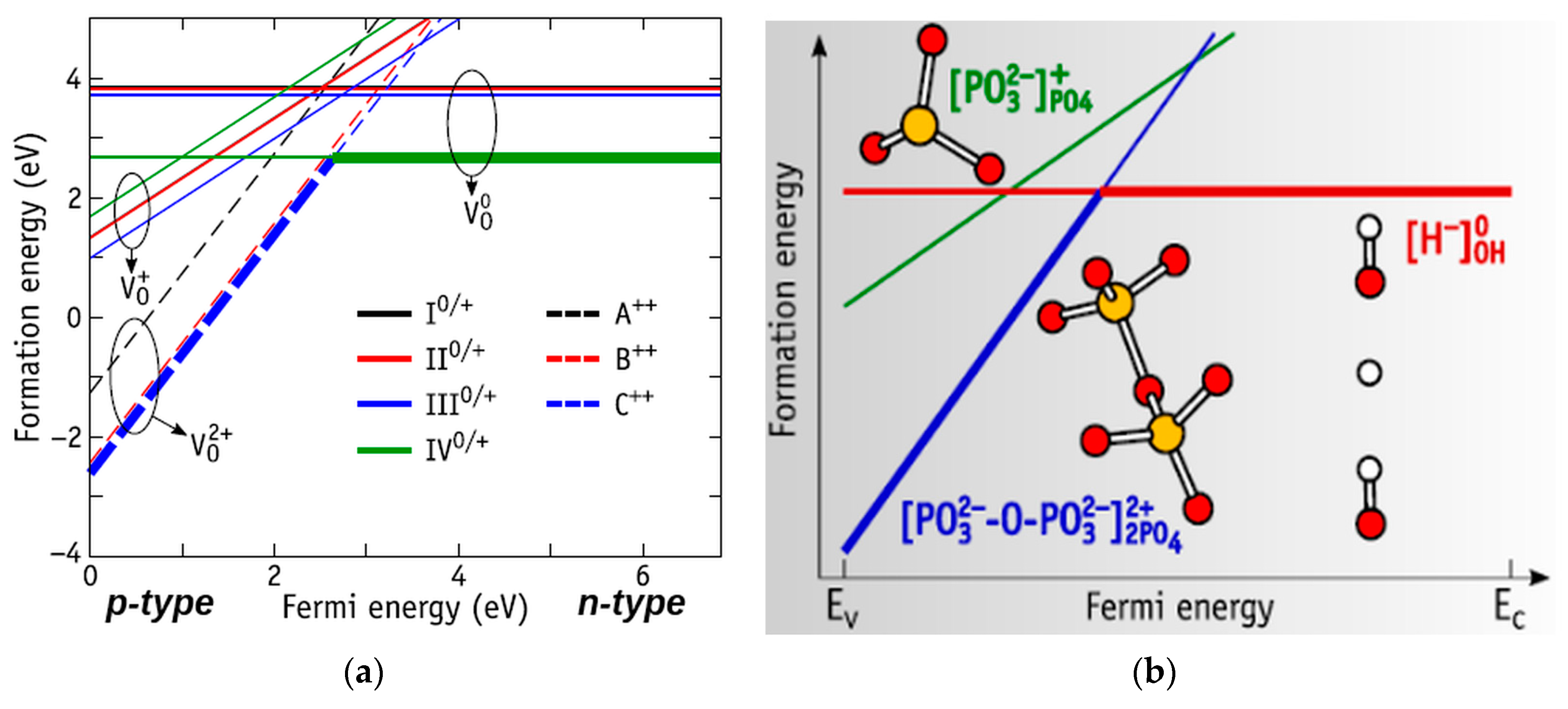
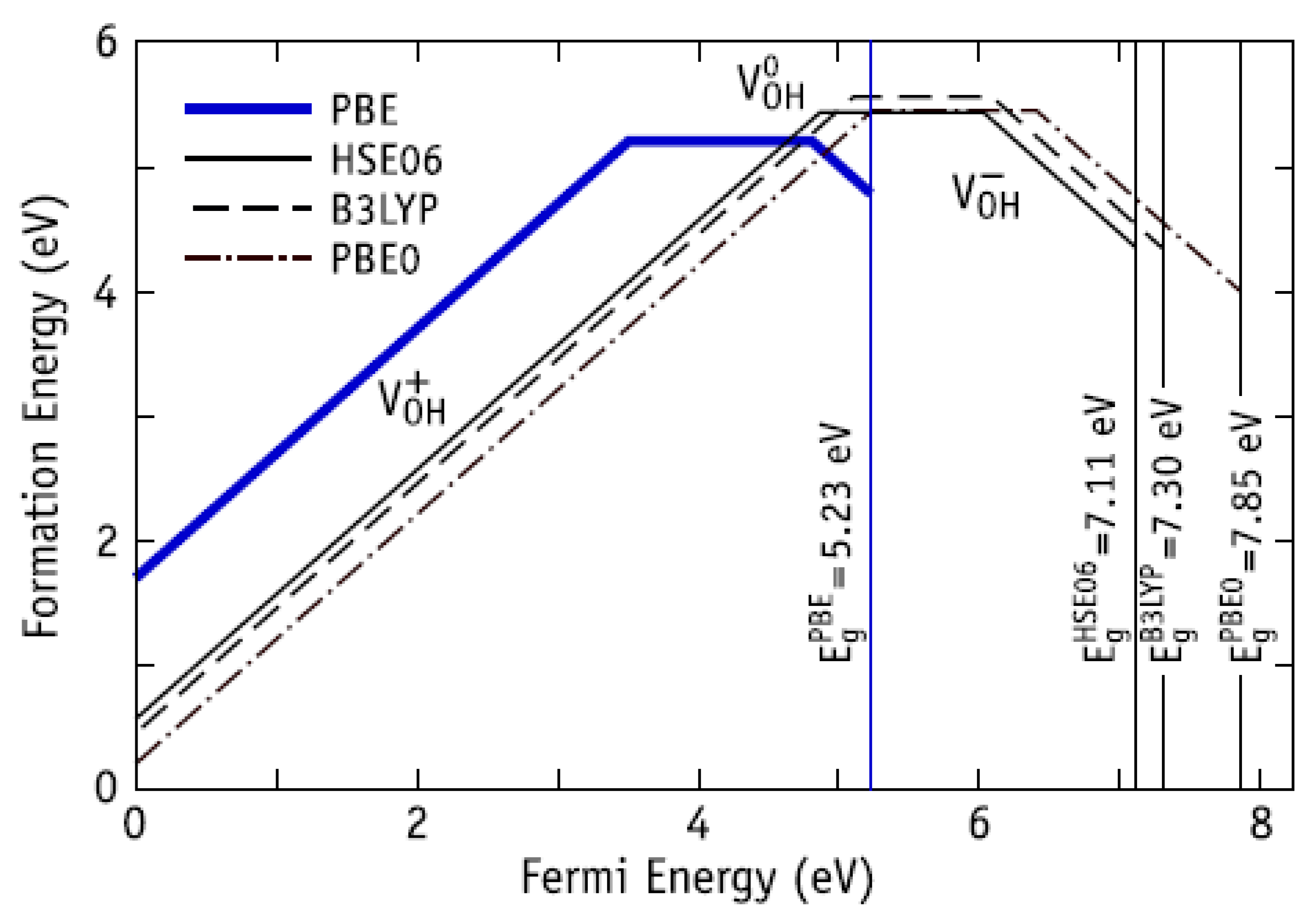
5.2.2. Generation of Defects and Dependence on Their Charge State
6. Engineering of Surface Electrical Charge of HAp due to Its Structural Imperfections
6.1. Specimens and Methods
- Annealing:
- vacuum condition: 0.001–0.00115 Pa.
- temperature: 542–546 °C.
- heating rate: 15 °C/min.
- annealing time: 30 min.
- cooling down to room temperature.
- Gamma-ray irradiation:
- photon energy: 18 MeV.
- the dose rate: 1000 MU/min.
- the ionizing radiation dose: 10 Gy.
- distance from the radiation source to sample: 1 m.
- radiation field: 10 × 10 cm2.
- Hydrogenation:
- temperature: 23 °C.
- pressure of hydrogen: 60 ± 2 atmospheres.
- hydrogenation time: 6 h.
- Microwave irradiation:
- power: 800 W.
- working time: 6.5 min.
- Annealing was provided in a vacuum condition (10−4 Pa) using the custom-made equipment [12].
- Gamma-ray irradiation: linear accelerator CLINAC, Varian Medical Systems Inc., Charlottesville, VA, USA.
- Hydrogenation: autoclave, HPM-P, PREMEX, Lyss, Switzerland.
- Microwave (MW) irradiation: microwave oven, SAMSUNG, Seoul, South Korea.
6.2. Results and Discussion
- the hydrogen atoms are knocked out during annealing and then captured by unsaturated hydrogen bonds that were natively present in the unit cell (sub-subgroups 1.1, 2.2, 5.1, and 6.1).
- the oxygen atoms are knocked out during annealing (sub-subgroups 1.2, 2.1, 3.2, and 6.1).
- the hydrogen atoms are knocked out during annealing without following capturing by unsaturated hydrogen bonds that were natively present in the unit cell (sub-subgroup 1.1).
- the OH-groups are knocked out during annealing (sub-subgroups 1.2, 2.1, and 3.1).
7. Conclusions
- Immobilization of the microorganisms can be achieved on the even surface of the substrate, characterized with a couple of nanometer roughness. The attachment of the cells can be controlled because of the surface electrical functionalization (deposition of the electrical charge).
- The protein layer having a thickness below 1 µm is not crucial to shield the electrical charge deposited on the substrate surface.
- The micrometric roughness of the substrate surface attaches the cells, the capillary phenomenon can play an important role to get the cell into the roughness valleys. The cells located at the valleys connect them with the bridges that stretch over the morphology pinnacles and cover the latter.
- The electrical charge deposition on the semiconductor or dielectric substrate can be delivered because of production of the point defects, hydroxyapatite being the example.
- The spatial arrangements of various atoms of the hydroxyapatite lattice, i.e., PO4 and OH groups, oxygen vacancies, interstitial H atoms, etc., give the instruments to deposit the electrical charge on the substrate.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Ayatollahi, M.; Geramizadeh, B.; Zakerinia, M.; Ramzi, M.; Yaghobi, R.; Hadadi, P.; Rezvani, A.R.; Aghdai, M.; Azarpira, N.; Karimi, H. Human Bone Marrow-derived Mesenchymal Stem Cell: A Source for Cell-Based Therapy. Int. J. Organ Transpl. Med. 2012, 3, 32–41. [Google Scholar]
- Derjaguin, B.V.; Landau, L.D. A Theory of the Stability of Strongly Charged Lyophobic Sols and the Coalescence of Strongly Charged Particles in Electrolytic Solutions. Acta Phys. Chim. 1941, 14, 633–642. [Google Scholar]
- Kobayashi, T.; Nakamura, S.; Yamashita, K. Enhanced osteobonding by negative surface charges of electrically polarized hydroxyapatite. J. Biomed. Mater. Res. 2001, 57, 477–484. [Google Scholar] [CrossRef]
- Dekhtyar, Y.; Polyaka, N.; Sammons, R. Electrically Charged Hydroxyapatite Enhances Immobilization and Proliferation of Osteoblasts. Ifmbe Proc. 2008, 20, 23–25. [Google Scholar]
- Dekhtyar, Y.; Khlusov, I.A.; Polyaka, N.; Sammons, R.L.; Tyulkin, F. Influence of Bioimplant Surface Electrical Potential on Osteoblast Behavior and Bone Tissue Formation. Ifmbe Proc. 2010, 29, 800–803. [Google Scholar]
- Khlusov, I.A.; Dekhtyar, Y.; Sharkeev, Y.; Pichugin, V.; Khlusova, M.; Polyaka, N.; Tyulkin, F.; Vendinya, V.; Legostaeva, E.; Litvinova, L.S.; et al. Nanoscale Electrical Potential and Roughness of a Calcium Phosphate Surface Promotes the Osteogenic Phenotype of Stromal Cells. Materials 2018, 11, 978. [Google Scholar] [CrossRef] [Green Version]
- Dekhtyar, Y.; Lancere, L.; Polyaka, N.; Sudnikovich, A.; Tyulkin, F.; Valters, V. PMMA Wettability Caused by Ultraviolet Radiation. Adv. Mater. Res. 2011, 222, 322–325. [Google Scholar] [CrossRef]
- Aronov, D.; Molotskii, M.; Rosenman, G. Charge-induced wettability modification. Appl. Phys. Lett. 2007, 90, 104104. [Google Scholar] [CrossRef]
- Palcevskis, E.; Dindune, A.; Dekhtyar, Y.; Polyaka, N.; Veljovic, D.; Sammons, R.L. The Influence of Surface Treatment by Hydrogenation on the Biocompatibility of Different Hydroxyapatite Materials. Mater. Sci. Eng. 2011, 23, 5–8. [Google Scholar] [CrossRef]
- Bystrov, V.; Bystrova, A.V.; Dekhtyar, Y. HAP nanoparticle and substrate surface electrical potential towards bone cells adhesion: Recent results review. Adv. Colloid Interface Sci. 2017, 249, 213–219. [Google Scholar] [CrossRef]
- Dekhtyar, Y.; Selutina, M.; Jung, C. Alteration of electrostatic potential of the titanium implant surface by antibacterial copper deposits. Eur. Cells Mater. 2016, 32, 22. [Google Scholar]
- Akmene, R.J.; Balodis, A.J.; Dekhtyar, Y.D.; Markelova, G.N.; Matvejevs, J.V.; Rozenfelds, L.B.; Sagalovich, G.L.; Smirnovs, J.S.; Tolkachovs, A.A.; Upmins, A.I. Exoelectron emission spectrometer complete set of surface local investigation. Surf. Phys. Chem. Mech. 1993, 8, 125–128, (In Russian: Poverhn. Fiz. Him. Meh.). [Google Scholar]
- Behari, J.; Kumar, H.; Aruna, R. Effect of ultraviolet light on the dielectric behaviour of bone at microwave frequencies. Adv. Biomed. Eng. 1982, 10, 139–144. [Google Scholar]
- Tengvall, P. 4.6 Protein Interactions with Biomaterials ☆. In Comprehensive Biomaterials; Elsevier BV: Neuchâtel, Switzerland, 2017; pp. 70–84. [Google Scholar]
- Bystrov, V.; Bystrova, N.; Dekhtyar, Y.; Filippov, S.; Karlov, A.; Katashev, A.; Meissner, C.; Paramonova, E.; Patmalnieks, A.; Polyaka, N.; et al. Size depended electrical properties of hydroxyapatite nanoparticles. In Proceedings of the World Congress on Medical Physics and Biomedical Engineering, IFMBE, Seoul, Korea, 27 August–1 September 2006. [Google Scholar]
- Seah, M.P.; Dench, W.A. Quantitative electron spectroscopy of surfaces: A standard data base for electron inelastic mean free paths in solids. Surf. Interface Anal. 1979, 1, 2–11. [Google Scholar] [CrossRef]
- Zein, I. by Jeffries Wyman JR. Studies on the dielectric constant of protein solutions. J. Biol. Chem. 1931, 90, 443–476. [Google Scholar]
- Hanafy, M.S. Electric field affected the molecular structure of the total serum protein of the mice blood. Romanian. J. Biophys. 2006, 16, 205–214. [Google Scholar]
- Morita, M.; Ohmi, T.; Hasegawa, E.; Kawakami, M.; Ohwada, M. Growth of native oxide on a sincon surface. J. Appl. Phys. 1990, 68, 1272. [Google Scholar] [CrossRef]
- Feldmann, H. Yeast Cell Architecture and Functions. In Yeast: Molecular and Cell Biology, 2nd ed.; Wiley-VCH Verlag: Weinheim, Germany, 2012; pp. 5–24. [Google Scholar]
- Elliot, J.C. Structure and Chemistry of the Apatites and Other Calcium Phosphates, 1st ed.; Elsevier: Amsterdam, The Netherlands, 1994. [Google Scholar]
- Bystrov, V.; Coutinho, J.; Bystrova, A.V.; Dekhtyar, Y.D.; Pullar, R.C.; Poronin, A.; Palcevskis, E.; Dindune, A.; Alkan, B.; Durucan, C.; et al. Computational study of hydroxyapatite structures, properties and defects. J. Phys. D Appl. Phys. 2015, 48, 195302. [Google Scholar] [CrossRef]
- Hughes, J.M.; Cameron, M.; Crowley, K.D. Structural variation in natural F, OH, and Cl apatites. Am Miner. 1989, 74, 870–876. [Google Scholar]
- Aronov, D.; Rosen, R.; Ron, E.; Rosenman, G. Electron-induced surface modification of hydroxyapatite-coated implant. Surf. Coat. Technol. 2008, 202, 2093–2102. [Google Scholar] [CrossRef]
- Aronov, D.; Chaikina, M.; Haddad, J.; Karlov, A.; Mezinskis, G.; Oster, L.; Pavlovska, I.; Rosenman, G. Electronic states spectroscopy of Hydroxyapatite ceramics. J. Mater. Sci. Mater. Electron. 2007, 18, 865–870. [Google Scholar] [CrossRef]
- Kittel, C. Introduction to Solid State Physics, 8th ed.; John Willey & Sons: New York, NY, USA, 2004. [Google Scholar]
- Bystrov, V.; Coutinho, J.; Avakyan, L.A.; Bystrova, A.V.; Paramonova, E.V. Piezoelectric, ferroelectric, and optoelectronic phenomena in hydroxyapatite with various defect levels. Ferroelectrics 2020, 559, 45–55. [Google Scholar] [CrossRef]
- Bystrov, V.; Piccirillo, C.; Tobaldi, D.; Castro, P.; Coutinho, J.; Kopyl, S.; Pullar, R.C. Oxygen vacancies, the optical band gap (Eg) and photocatalysis of hydroxyapatite: Comparing modelling with measured data. Appl. Catal. B Env. 2016, 196, 100–107. [Google Scholar] [CrossRef]
- Hohnberg, P.; Kohn, W. Inhomogeneous Electron Gas. Phys. Rev. A 1964, 136, B864–B871. [Google Scholar] [CrossRef] [Green Version]
- Kohn, W.; Sham, L.J. Self-Consistent Equations Including Exchange and Correlation Effects. Phys. Rev. 1965, 140, A1133–A1138. [Google Scholar] [CrossRef] [Green Version]
- Britney, P.R.; Jones, R. LDA Calculations Using a Basis of Gaussian Orbitals. Phys. Status Solidi B Basic Res. 2000, 217, 131–171. [Google Scholar]
- Cho, J.; Garcia-Molina, H.; Page, L. Efficient crawling through URL ordering. Comput. Netw. Isdn Syst. 1998, 30, 161–172. [Google Scholar] [CrossRef]
- Perdew, J.P.; Burke, K.; Ernzerhof, M. Generalized Gradient Approximation Made Simple. Phys. Rev. Lett. 1996, 77, 3865–3868. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Avakyan, L.; Paramonova, E.V.; Coutinho, J.; Öberg, S.; Bystrov, V.S.; Bugaev, L.A. Optoelectronics and defect levels in hydroxyapatite by first-principles. J. Chem. Phys. 2018, 148, 154706. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Bystrov, V.S.; Avakyan, A.L.; Paramonova, E.V.; Coutinho, J. Sub-Band Gap Absorption Mechanisms Involving Oxygen Vacancies in Hydroxyapatite. J. Phys. Chem. C 2019, 123, 4856–4865. [Google Scholar] [CrossRef] [Green Version]
- Kresse, G.; Furthmüller, J. Efficient iterative schemes for ab initio total-energy calculations using a plane-wave basis set. Phys. Rev. B Condens. Matter Mater. Phys. 1996, 54, 11169–11186. [Google Scholar] [CrossRef] [PubMed]
- Kresse, G.; Joubert, D. From ultrasoft pseudopotentials to the projector augmented-wave method. Phys. Rev. B 1999, 59, 1758–1775. [Google Scholar] [CrossRef]
- VASP (Vienna Ab initio Simulation Package). Available online: https://www.vasp.at (accessed on 11 July 2020).
- Lee, C.; Yang, W.; Parr, R.G. Development of the Colle-Salvetti correlation-energy formula into a functional of the electron density. Phys. Rev. B 1988, 37, 785–789. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Becke, A.D. A new mixing of Hartree–Fock and local density--functional theories. J. Chem. Phys. 1993, 98, 1372–1377. [Google Scholar] [CrossRef]
- Blöchl, P.E. Projector augmented-wave method. Phys. Rev. B Condens. Matter Mater. Phys. 1994, 50, 17953–17979. [Google Scholar]
- Calderín, L.; Stott, M.J.; Rubio, A. Electronic and crystallographic structure of apatites. Phys. Rev. B 2003, 67, 134106. [Google Scholar] [CrossRef] [Green Version]
- Rulis, P.; Ouyang, L.; Ching, W.Y. Electronic structure and bonding in calcium apatite crystals: Hydroxyapatite, fluorapatite, chlorapatite, and bromapatite. Phys. Rev. B 2004, 70, 155104. [Google Scholar] [CrossRef]
- Matsunaga, K.; Kuwabara, A. First-principles study of vacancy formation in hydroxyapatite. Phys. Rev. B 2007, 75, 014102. [Google Scholar] [CrossRef] [Green Version]
- Slepko, A.; Demkov, A.A. First-principles study of the biomineral hydroxyapatite. Phys. Rev. B 2011, 84, 134108. [Google Scholar] [CrossRef] [Green Version]
- Haverty, D.; Tofail, S.A.M.; Stanton, K.T.; McMonagle, J.B. Structure and stability of hydroxyapatite: Density functional calculation and Rietveld analysis. Phys. Rev. B 2005, 71, 094103. [Google Scholar] [CrossRef]
- De Leeuw, N.H. Computer simulations of structures and properties of the biomaterial hydroxyapatite. J. Mater. Chem. 2010, 20, 5376. [Google Scholar] [CrossRef]
- Mostafa, N.Y.; Brown, P.W. Computer simulation of stoichiometric hydroxyapatite: Structure and substitutions. J. Phys. Chem. Solids 2007, 68, 431–437. [Google Scholar] [CrossRef]
- Astala, R.; Stott, M.J. First-principles study of hydroxyapatite surfaces and water adsorption. Phys. Rev. B 2008, 78, 075427. [Google Scholar] [CrossRef]
- Hu, S.; Jia, F.; Marinescu, C.; Cimpoesu, F.; Qi, Y.; Tao, Y.; Stroppa, A.; Ren, W. Ferroelectric polarization of hydroxyapatite from density functional theory. Rsc Adv. 2017, 7, 21375–21379. [Google Scholar] [CrossRef] [Green Version]
- Bystrov, V.; Paramonova, E.V.; Dekhtyar, Y.D.; Bystrova, A.V.; Pullar, R.C.; Kopyl, S.; Tobaldi, D.; Piccirillo, C.; Avakyan, L.A.; Coutinho, J. Surface modified hydroxyapatites with various functionalized nanostructures: Computational studies of the vacancies in HAp. Ferroelectrics 2017, 509, 105–112. [Google Scholar] [CrossRef]
- Bystrova, A.V.; Dekhtyar, Y.D.; Popov, A.; Coutinho, J.; Bystrov, V.S. Modified Hydroxyapatite Structure and Properties: Modeling and Synchrotron Data Analysis of Modified Hydroxyapatite Structure. Ferroelectrics 2015, 475, 135–147. [Google Scholar] [CrossRef]
- European PERCERAMICS project NMP3-CT-2003-504937 Funded by the European Commission. Available online: http://www.perceramics.vip.lv (accessed on 17 July 2011).
- Bystrov, V.S.; Paramonova, E.V.; Dekhtyar, Y.; Katashev, A.; Karlov, A.; Polyaka, N.; Bystrova, A.V.; Patmalnieks, A.; Kholkin, A. Computational and experimental studies of size and shape related physical properties of hydroxyapatite nanoparticles. J. Phys. Condens. Matter 2011, 23, 065302. [Google Scholar] [CrossRef] [PubMed]
- Silin, A.R.; Trukhin, A.I. Point defects and elementary excitations in crystalline and glassy SiO2; Zinatne: Riga, Latvia, 1985; p. 244. (in Russian) [Google Scholar]
- Pohl, H.A. The Motion and Precipitation of Suspensoids in Divergent Electric Fields. J. Appl. Phys. 1951, 22, 869–871. [Google Scholar] [CrossRef]

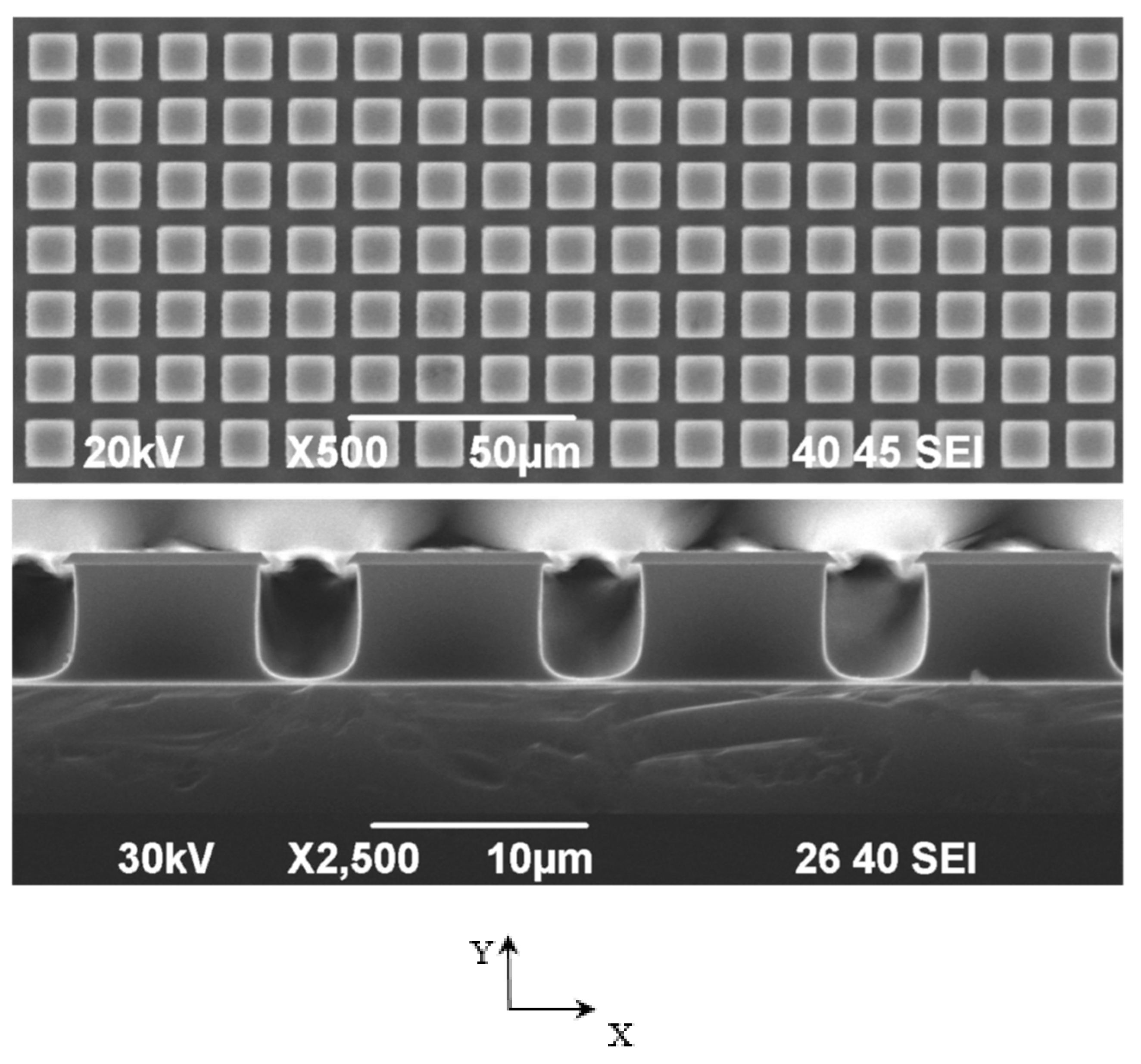

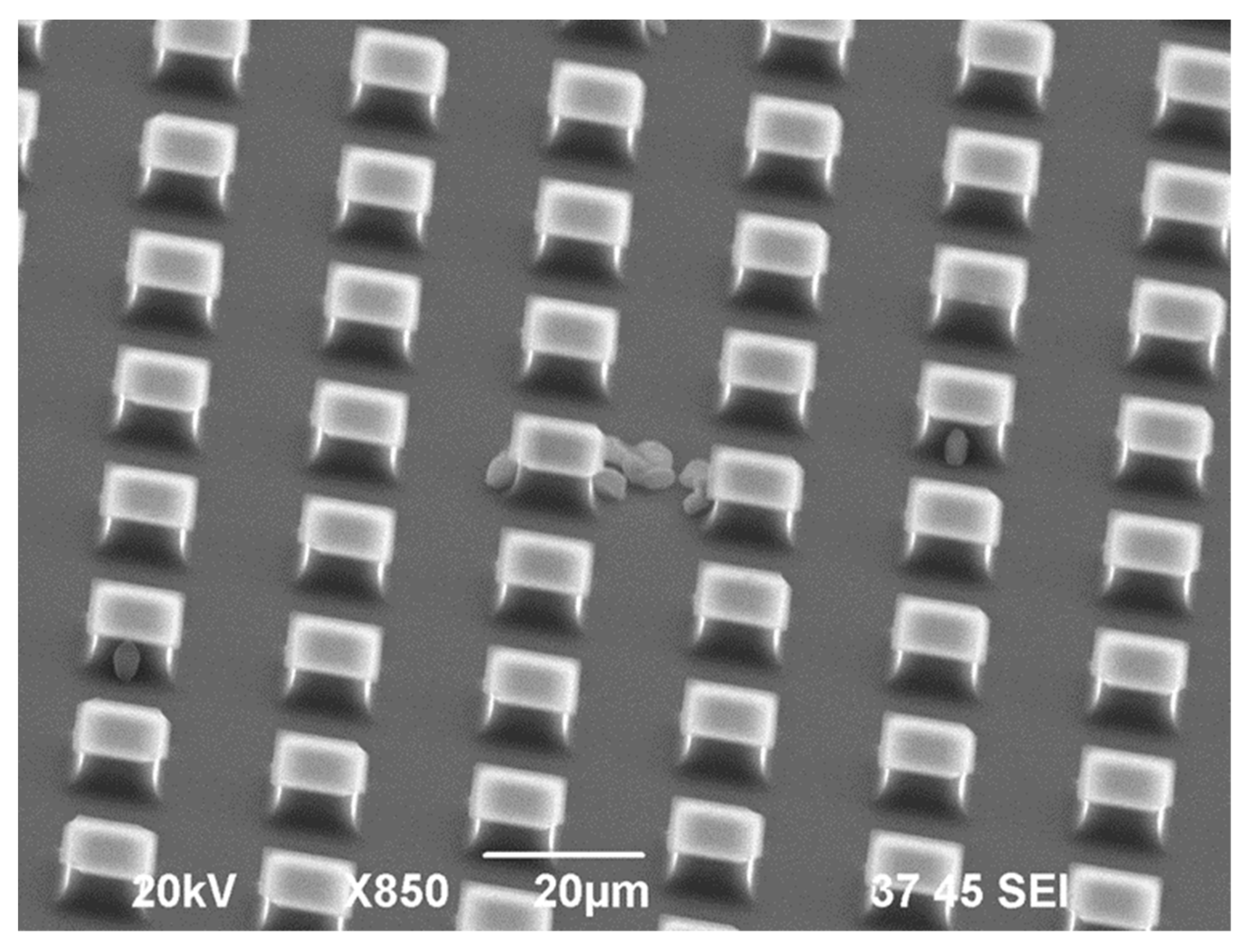

| Substrate | Cross-section Side, μm | Distance between the Pillars, μm | Number of Pillars on the Single Substrate | Number of Substrates |
|---|---|---|---|---|
| A | 10.4 ± 0.08 | 13.48 ± 0.12 | 42,516 | 4 |
| B | 45.46 ± 0.12 | 12.78 ± 0.16 | 7248 | 4 |
| C | 9.44 ± 0.08 | 4.49 ± 0.05 | 124,439 | 4 |
| Phase | Group | a, Å | b, Å | c, Å |
|---|---|---|---|---|
| Hexagonal | P63/m | 9.417 | 9.417 | 6.875 |
| Monoclinic | P21/b | 9.480 | 18.960 | 6.830 |
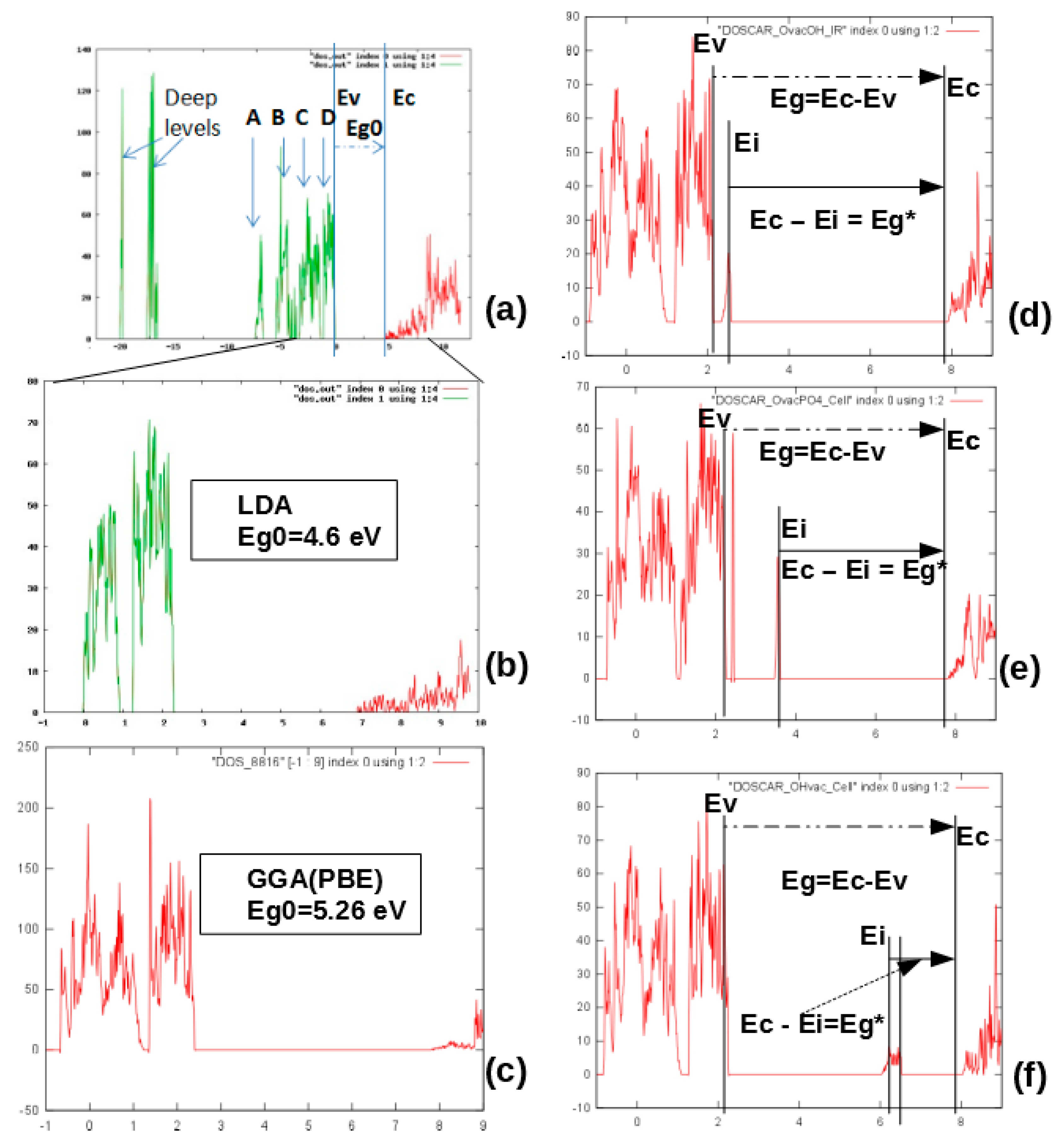
| Defect type | LDA | GGA (PBE) | ||||||
|---|---|---|---|---|---|---|---|---|
| Eg = Ec − Ev, eV | ΔEg= Eg* − Eg ~Δφ, eV | Ei − Ev*, eV | Ec* − Ei = Ei0, eV | Eg = Ec − Ev, eV | ΔEg = Eg* − Eg ~Δφ, eV | Ei − Ev*, eV | Ec*− Ei = Ei0, eV | |
| HAp in P63/m | 4.6 | – | – | – | 5.26 | – | – | – |
| H vac | 4.92 | +0.32 | 0.1 (½ occ.) | 4.82 | – | – | – | – |
| O(OH) vac | 5.15 | +0.55 | 0.1 (1 occ.) | 5.05 | 5.72 | +0.46 | 0.27 | 5.45 |
| OH vac | 5.49 | +0.89 | 3.11–3.82 peaks: 3.40 3.53 3.66 (½ occ.) | 2.38–1.67 peaks: 2.09 1.96 1.83 | 5.75 | +0.49 | 3.66–4.28 peaks: 3.96 4.11 4.17 | 1.97–1.35 peaks: 1.78 1.63 1.57 |
| O(PO4) * vac | 4.7 ± 0.13 | +0.1 | 1.11 ± 0.3 | 3.59 ± 0.3 | 5.34 ± 0.1 | +0.08 | 1.23 ± 0.3 | 4.11 ± 0.3 |
| H int | 5.12 | +0.52 | Peaks: 1.20 1.45 1.75 (½ occ.) | Peaks: 3.92 3.67 3.37 | – | – | – | – |
| 2H _HB Hydrogen filling of unsaturated hydrogen bonds | 4.63 | +0.03 | Peaks: 1.71 0.89 (1 occ.) | Peaks: 2.92 3.74 | – | – | – | – |
| Defect type | PBE | B3LYP | ||||||
|---|---|---|---|---|---|---|---|---|
| Eg*= =Ec* − Ev*, eV | ΔEg= =Eg* − Eg~ ~Δφ, eV | Ei − Ev*, eV | Ec* − Ei = = Eig0, eV | Eg*= =Ec* − Ev*, eV | ΔEg= Eg* − Eg~ ~Δφ, eV | Ei − Ev*, eV | Ec* − Ei = = Eig0, eV | |
| VO(I) | 5.0674 | −0.1626 | 1.1496 ~1.15 | 3.9178 | 7.0497 | −0.2503 | 1.4291 ~ 1.43 | 5.6206 |
| VO(II) | 5.2004 | −0.0296 | 1.3167 ~1.32 | 3.8837 | 7.2311 | −0.0689 | 1.6512 ~ 1.65 | 5.5799 |
| VO(III) | 5.1393 | −0.0907 | 1.3811 ~1.38 | 3.7582 | 7.1333 | −0.1667 | 1.6845 ~ 1.68 | 5.4488 |
| VO(IV) | 5.3004 | +0.0704 | 0.4189 ~0.42 | 4.8815 | 7.3842 | +0.0842 | 0.7347 ~ 0.73 | 6.6495 |
| Eg of initial defect-free HAp (P63/m) | 5.23 | – | – | – | 7.3 | – | – | – |
| Induced Defect | Treatments |
|---|---|
| Vacancy OH | Annealing 500–900 °C [25] |
| Vacancy O | Gamma-ray irradiation [55] |
| Vacancy H | Gamma-ray irradiation [55] |
| Interstitial H | Hydrogenation [56] + MW irradiation |
| Hydrogen filling of unsaturated hydrogen bonds | Hydrogenation [56] |
| Group of Samples | Sequencing | ||||||
|---|---|---|---|---|---|---|---|
| PE (Measurement of the Native Specimens) | Annealing | PE (Measurement of the Annealed) | Hydrogenation | MW Irradiation | Gamma-Ray Irradiation | PE (Measurement of the Specimens Processed in 4–6 Sequences) | |
| 1 | + | + | + | – | – | – | + |
| 2 | + | + | + | – | – | + | + |
| 3 | + | + | + | + | – | – | + |
| 4 | + | + | + | – | + | – | + |
| 5 | + | + | + | + | + | – | + |
| Subgroup Number | Calculated φ (Table 3 and [22]), eV | Type of Defect | Measured φ, eV |
|---|---|---|---|
| 1 | 4.6 4.63 | HAp without defects 2 additional H atoms that fill unsaturated hydrogen bonds | φ0 = 4.51 ± 0.15 |
| 2 | 4.63 | 2 additional H atoms that fill unsaturated hydrogen bonds | φ0 = 4.49 ± 0.09 |
| 4.92 | H–vacancy | φ1 = 4.84 ± 0.06 | |
| 3 | 4.63 | 2 additional H atoms that fill unsaturated hydrogen bonds | φ0 = 4.5 ± 0.1 |
| 5.12 5.15 | H–interstitial O–vacancy | φ1 = 5.20 ± 0.04 | |
| 4 | 4.63 | 2 additional H atoms that fill unsaturated hydrogen bonds | φ0 = 4.47 ± 0.05 |
| 5.49 | OH–vacancy | φ1 = 5.37 ± 0.05 | |
| 5 | 4.92 | H–vacancy | φ0 = 4.98 ± 0.04 |
| 5.15 | O–vacancy | φ1 = 5.25 ± 0.04 | |
| 6 | 5.12 5.15 | H–interstitial O–vacancy | φ0 = 5.06 ± 0.04 |
| φ1 = 5.23 ± 0.04 |
| Subgroup Number | Sub-Subgroup Number | Calculated φ (Table 3 and [22]), eV | Type of Defect | φ Measured after Annealing, eV |
|---|---|---|---|---|
| 1 | 1.1 | 4.63 | 2 additional H atoms that fill unsaturated hydrogen bonds | φ0 = 4.74 |
| 4.92 | H–vacancy | φ1 = 4.90 | ||
| 1.2 | 4.63 | 2 additional H atoms that fill unsaturated hydrogen bonds | φ0 = 4.68 | |
| 5.15 | O–vacancy | φ1 = 5.05 | ||
| 2 | 2.1 | 4.63 | 2 additional H atoms that fill unsaturated hydrogen bonds | φ0 = 4.53 |
| 5.15 | O–vacancy | φ1 = 5.25 | ||
| 2.2 | 4.63 | 2 additional H atoms that fill unsaturated hydrogen bonds | φ0 = 4.53 | |
| 4.92 | H–vacancy | φ1 = 5.00 | ||
| 3 | 3.1 | 4.63 | 2 additional H atoms that fill unsaturated hydrogen bonds | φ0 = 4.60 |
| 5.49 | OH–vacancy | φ1 = 5.30 | ||
| 3.2 | 4.63 | 2 additional H atoms that fill unsaturated hydrogen bonds | φ0 = 4.60 | |
| 5.15 | O–vacancy | φ1 = 5.20 | ||
| 4 | 4.1 | 4.63 | 2 additional H atoms that fill unsaturated hydrogen bonds | φ0 = 4.62 |
| 4.92 | H–vacancy | φ1 = 5.00 | ||
| 5 | 5.1 | 4.63 | 2 additional H atoms that fill unsaturated hydrogen bonds | φ0 = 4.85 |
| 4.92 | H–vacancy | φ1 = 5.08 | ||
| 6 | 6.1 | 4.63 | 2 additional H atoms that fill unsaturated hydrogen bonds | φ0 = 4.70 |
| 5.15 | O–vacancy | φ1 = 5.15 |
| Subgroup Number | Calculated φ (Table 3 and [22]), eV | Type of Defect | Measured φ, eV |
|---|---|---|---|
| 2.1 | 5.49 | OH–vacancy | φ0 = 5.6 |
| 4.92 | H–vacancy | φ1 = 4.93 | |
| 2.2 | 4.92 | H–vacancy | φ0 = 4.93 |
| 5.15 | O–vacancy | φ1 = 5.12 |
| Subgroup Number | Calculated φ (Table 3 and [22]), eV | Type of Defect | Measured φ, eV |
|---|---|---|---|
| 3.1 | 5.49 | OH–vacancy | φ0 = 5.49 |
| 4.92 | H–vacancy | φ1 = 4.95 | |
| 5.15 | O–vacancy | φ2 = 5.20 | |
| 3.2 | 4.63 | 2 additional H atoms that fill unsaturated hydrogen bonds | φ0 = 4.62 |
| 5.49 | OH–vacancy | φ1 = 5.30 |
| Subgroup Number | Calculated φ (Table 3 and [22]), eV | Type of Defect | Measured φ, eV |
|---|---|---|---|
| 4.1 | 4.63 | 2 additional H atoms that fill unsaturated hydrogen bonds | φ0 = 4.50 |
| 4.92 | H–vacancy | φ1 = 5.00 | |
| 5.12 5.15 | H–interstitial O–vacancy | φ2 = 5.18 | |
| 4.2 | 5.12 5.15 | H–interstitial O–vacancy | φ0 = 5.17 |
| 5.49 | OH–vacancy | φ1 = 5.33 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Baltacis, K.; Bystrov, V.; Bystrova, A.; Dekhtyar, Y.; Freivalds, T.; Raines, J.; Rozenberga, K.; Sorokins, H.; Zeidaks, M. Physical Fundamentals of Biomaterials Surface Electrical Functionalization. Materials 2020, 13, 4575. https://doi.org/10.3390/ma13204575
Baltacis K, Bystrov V, Bystrova A, Dekhtyar Y, Freivalds T, Raines J, Rozenberga K, Sorokins H, Zeidaks M. Physical Fundamentals of Biomaterials Surface Electrical Functionalization. Materials. 2020; 13(20):4575. https://doi.org/10.3390/ma13204575
Chicago/Turabian StyleBaltacis, Karlis, Vladimir Bystrov, Anna Bystrova, Yuri Dekhtyar, Talivaldis Freivalds, Jan Raines, Krista Rozenberga, Hermanis Sorokins, and Martins Zeidaks. 2020. "Physical Fundamentals of Biomaterials Surface Electrical Functionalization" Materials 13, no. 20: 4575. https://doi.org/10.3390/ma13204575
APA StyleBaltacis, K., Bystrov, V., Bystrova, A., Dekhtyar, Y., Freivalds, T., Raines, J., Rozenberga, K., Sorokins, H., & Zeidaks, M. (2020). Physical Fundamentals of Biomaterials Surface Electrical Functionalization. Materials, 13(20), 4575. https://doi.org/10.3390/ma13204575






