Model-Based Residual Stress Design in Multiphase Seamless Steel Tubes
Abstract
1. Introduction
- (i)
- external cooling only, that is, the coolant is applied only to the tube’s outer surface;
- (ii)
- both, external and internal cooling for mainly martensitic microstructure, that is, an additional cooling is applied through a cooling device from inside the tube;
- (iii)
- and both external and internal cooling, mainly to adjust a bainitic microstructure.
2. Materials and Methods
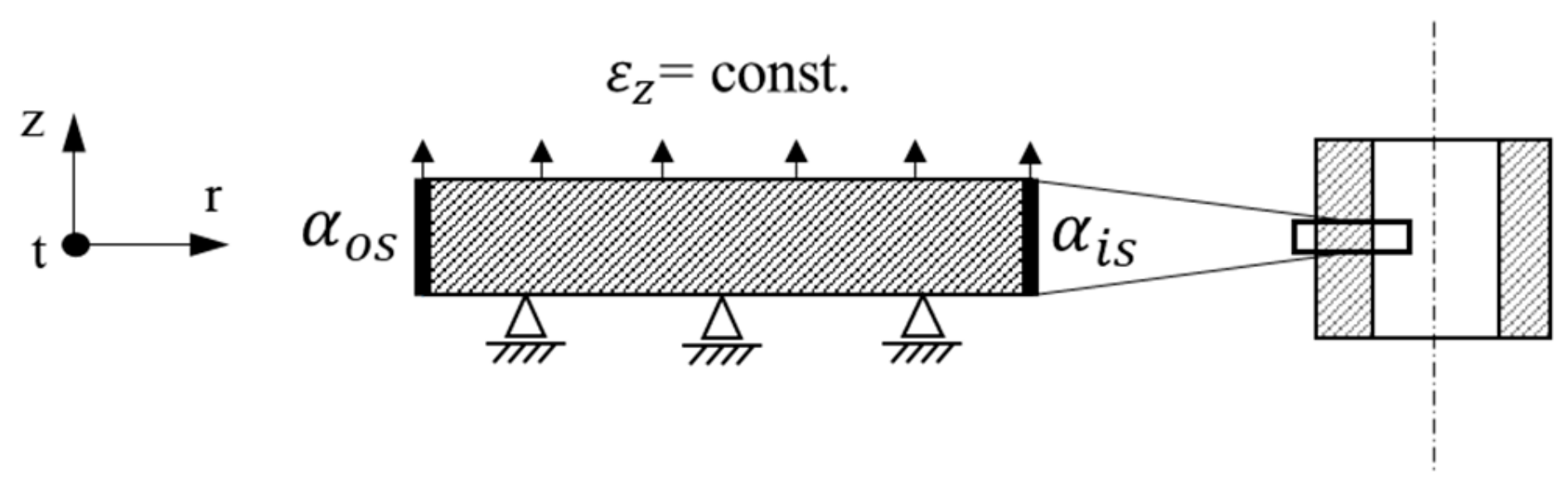
2.1. Model Description
2.2. Design Strategy
3. Results
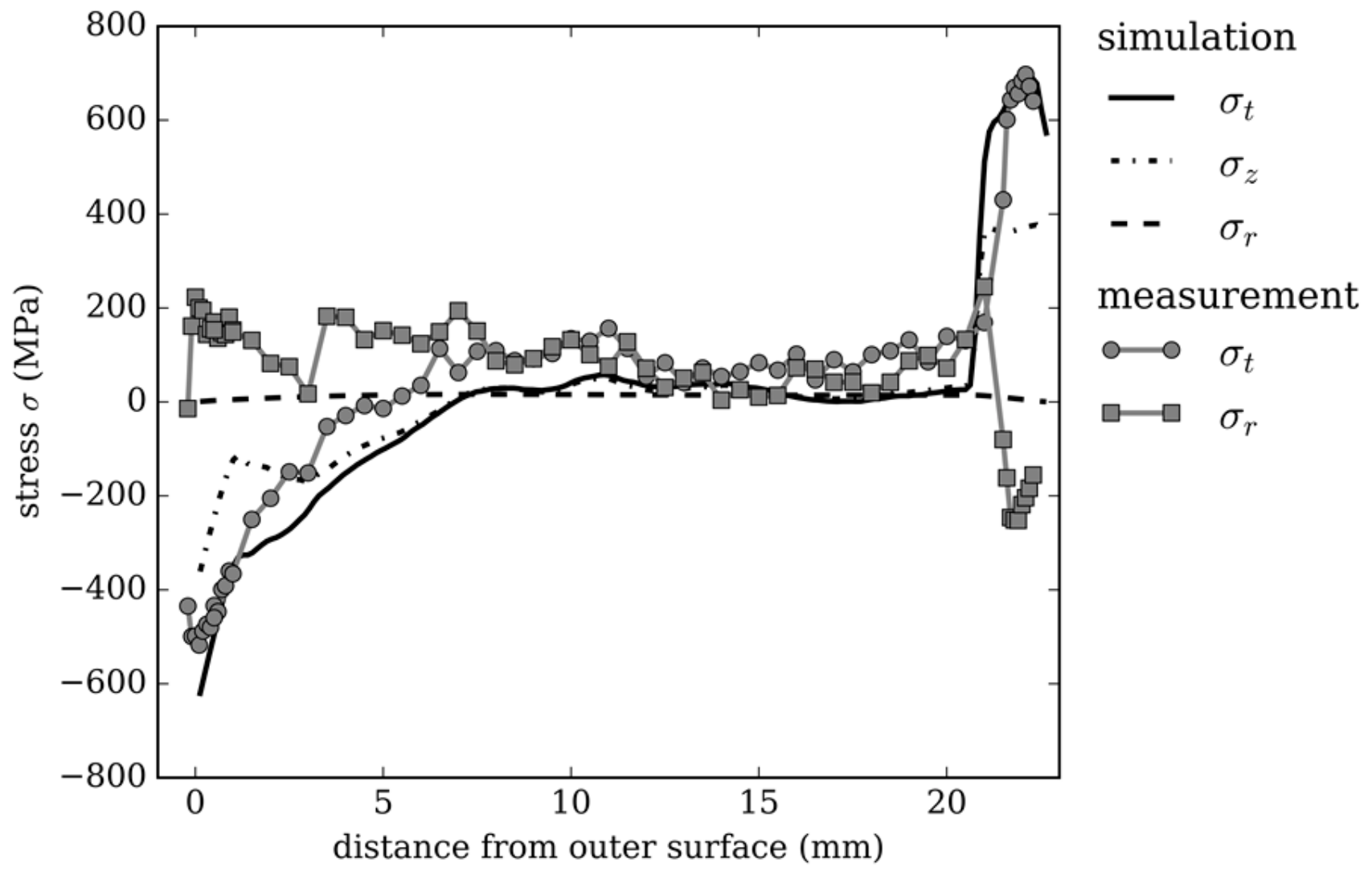
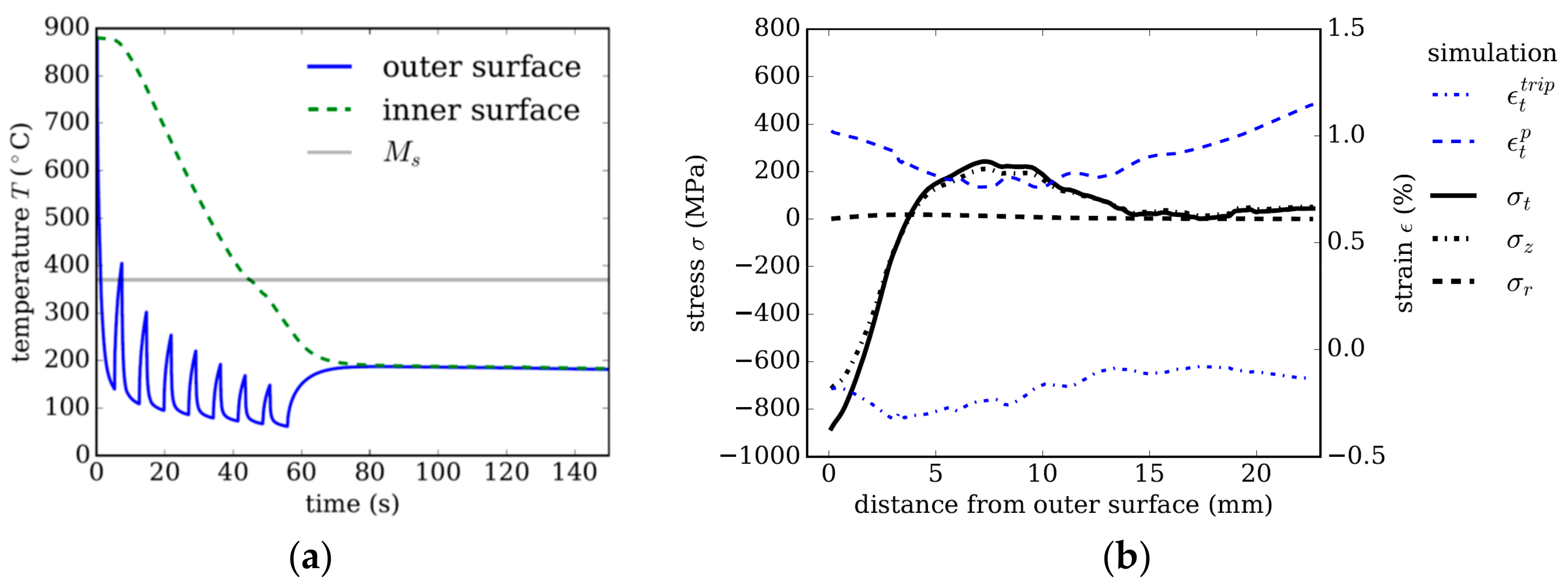
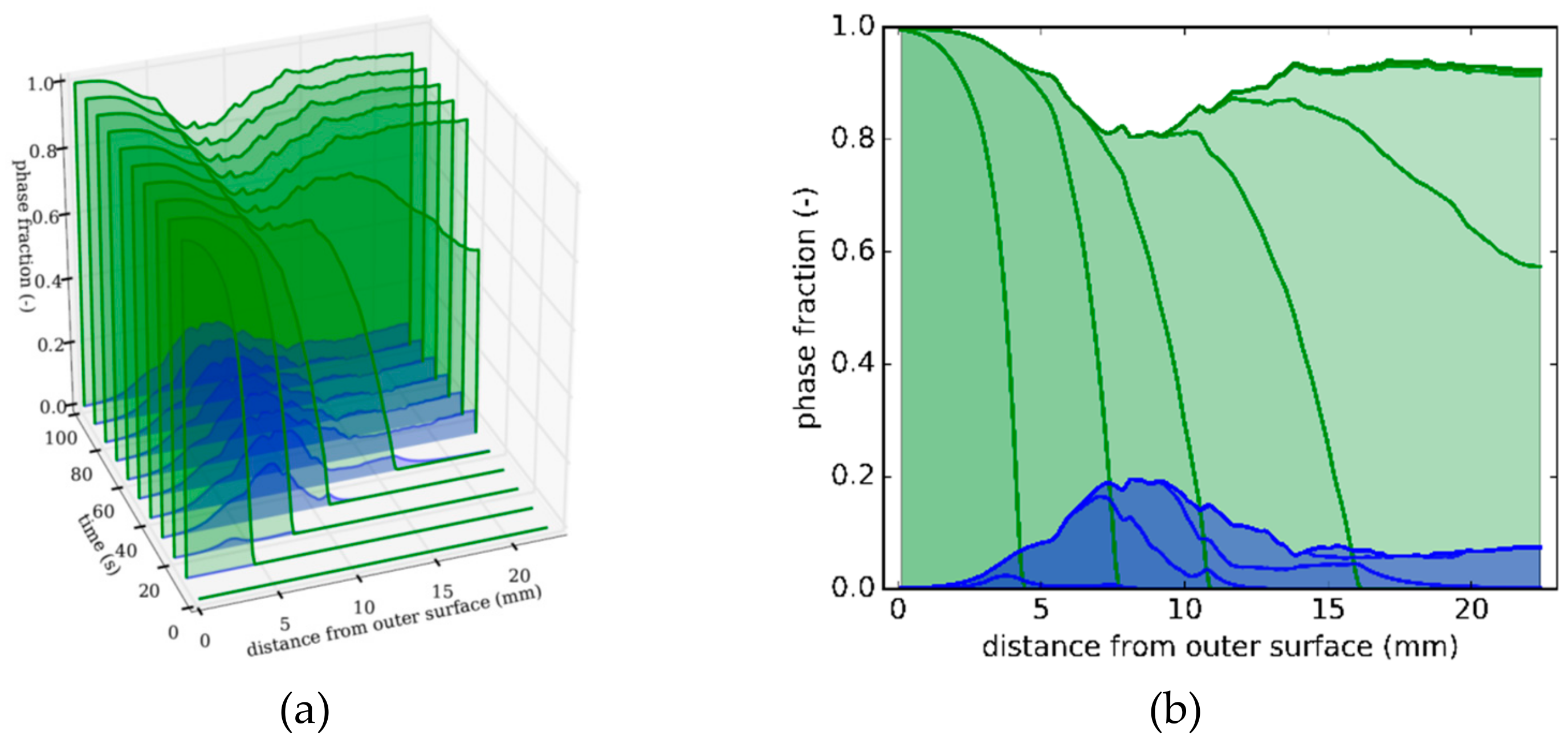
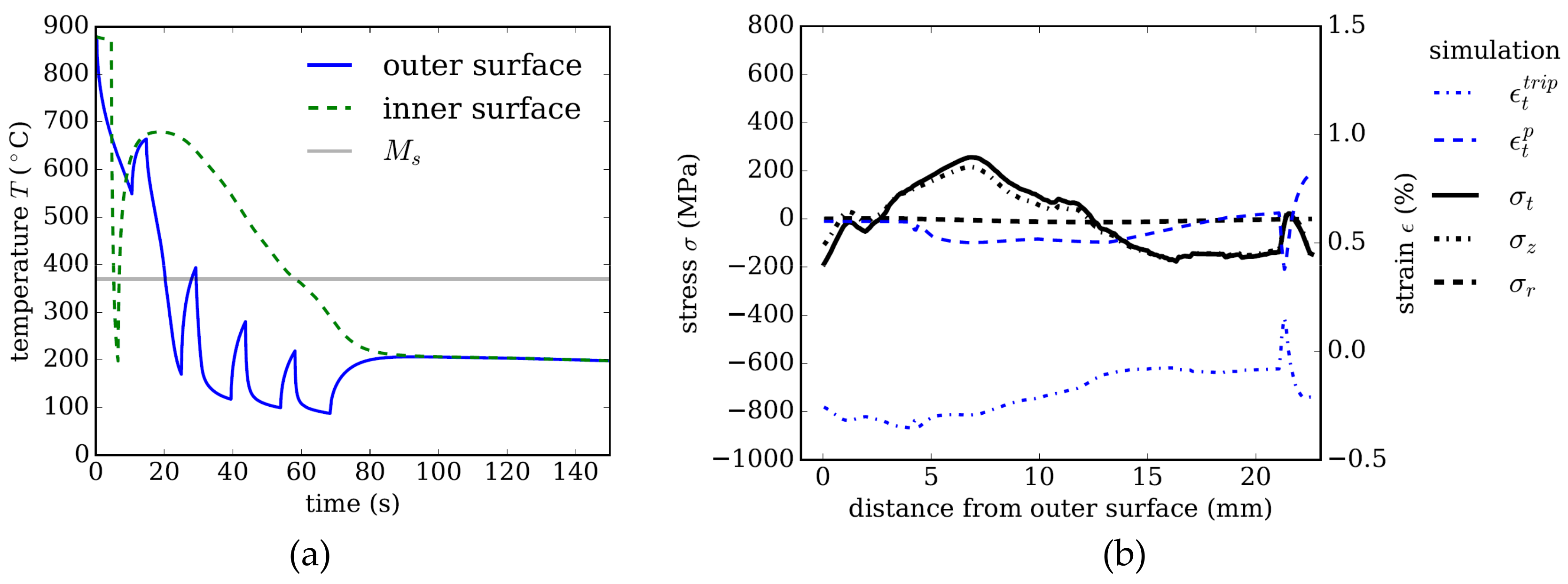
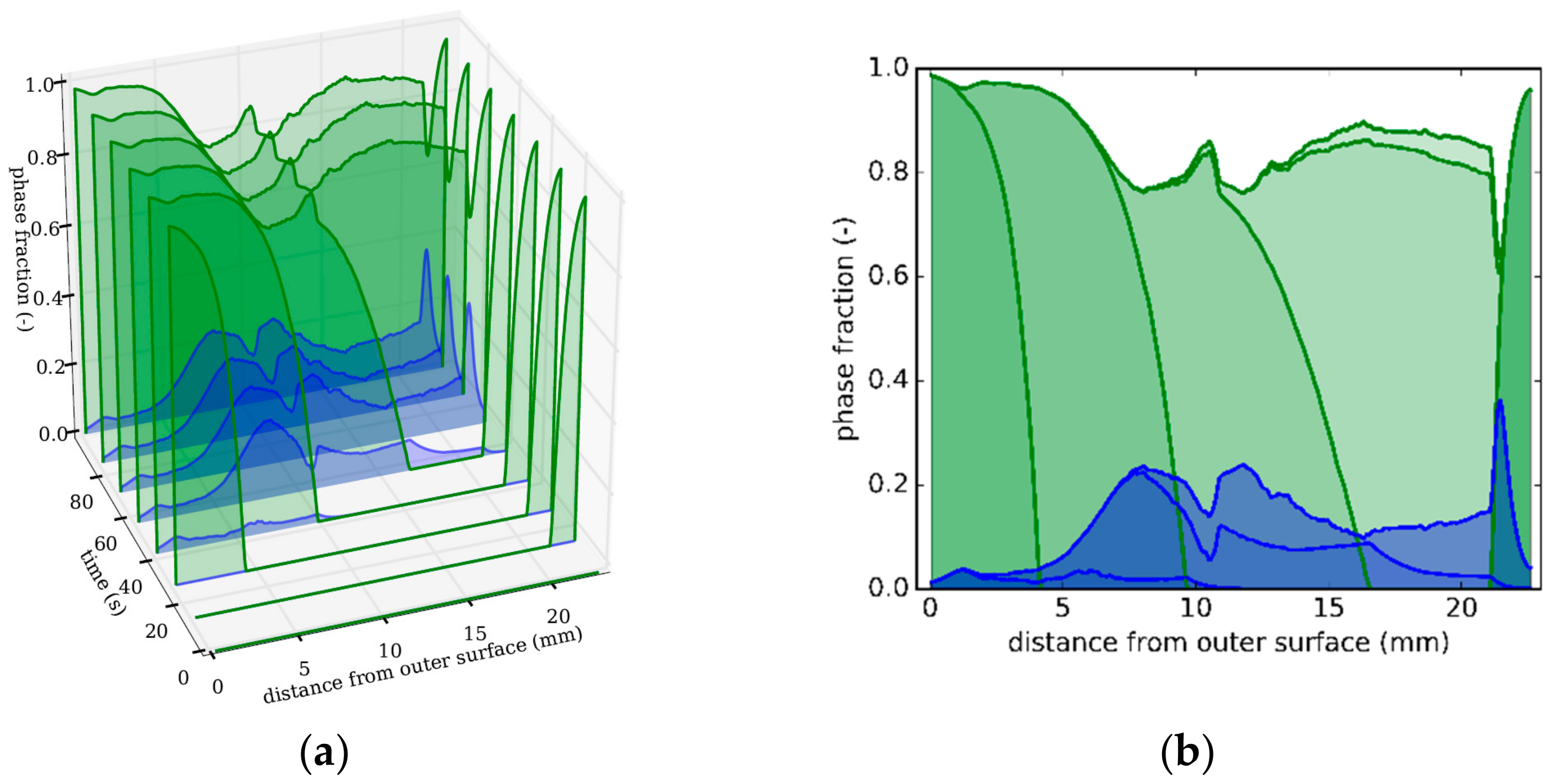
3.1. Strategy 1
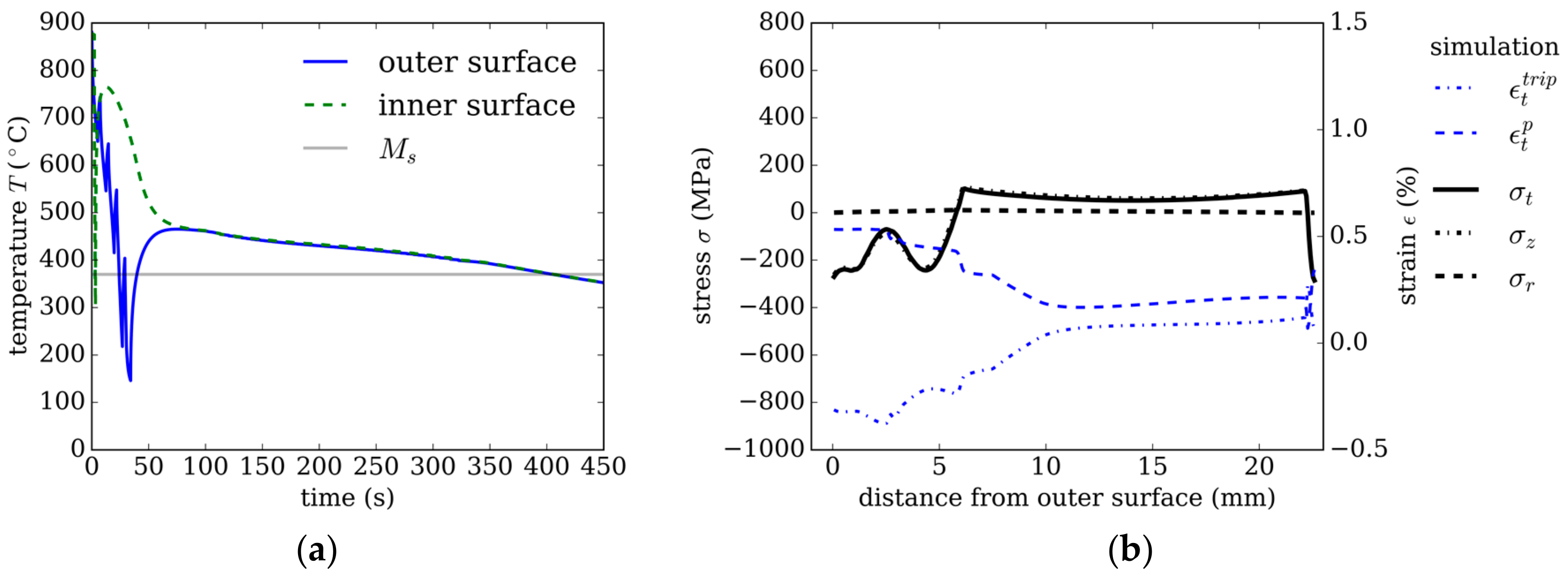
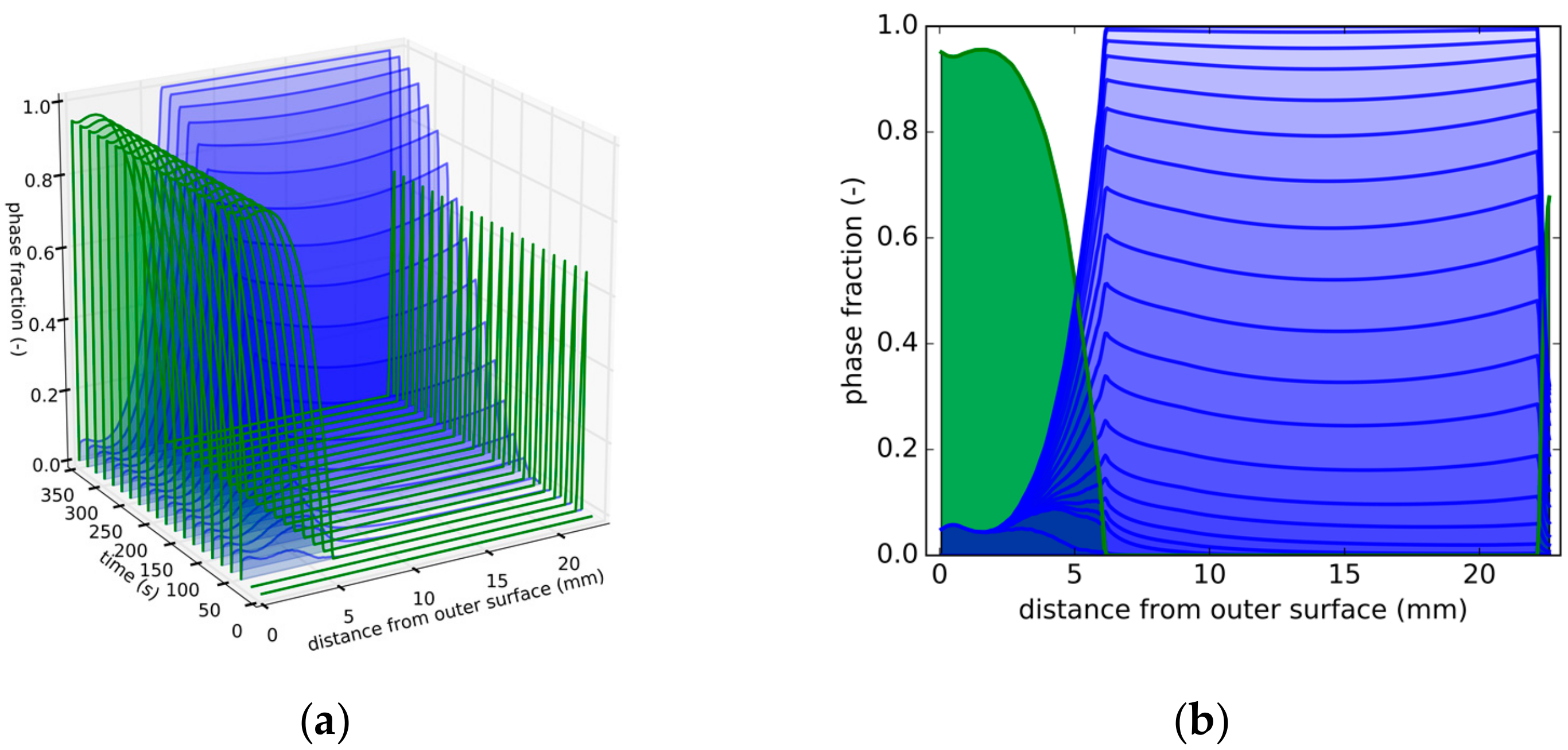
3.2. Strategy 2
3.3. Strategy 3
4. Discussion and Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Denis, S.; Farias, D.; Simon, A. Mathematical model coupling phase transformations and temperature evolutions in steels. ISIJ Int. 1992, 32, 316–325. [Google Scholar] [CrossRef]
- Rohde, J.; Jeppsson, A. Literature review of heat treatment simulations with respect to phase transformation, residual stresses and distortion. Scand. J. Metall. 2000, 29, 47–62. [Google Scholar] [CrossRef]
- Gür, C.H.; Pan, J. Handbook of Thermal Process Modeling of Steels; CRC Press: Boca Raton, FL, USA, 2009; ISBN 978-0-8493-5019-1. [Google Scholar]
- Fu, G.; Lourenço, M.I.; Duan, M.; Estefen, S.F. Influence of the welding sequence on residual stress and distortion of fillet welded structures. Mar. Struct. 2016, 46, 30–55. [Google Scholar] [CrossRef]
- Bhatti, A.A.; Barsoum, Z.; Murakawa, H.; Barsoum, I. Influence of thermo-mechanical material properties of different steel grades on welding residual stresses and angular distortion. Mat. Des. (1980–2015) 2015, 65, 878–889. [Google Scholar] [CrossRef]
- Islam, M.; Buijk, A.; Rais-Rohani, M.; Motoyama, K. Simulation-based numerical optimization of arc welding process for reduced distortion in welded structures. Finite Elem. Anal. Des. 2014, 84, 54–64. [Google Scholar] [CrossRef]
- Prime, M.B.; Prantil, V.C.; Rangaswamy, P.; García, F.P. Residual stress measurement and prediction in a hardened steel ring. MSF 2000, 347–349, 223–228. [Google Scholar] [CrossRef]
- Eck, S.; Prevedel, P.; Marsoner, S.; Ecker, W.; Illmeier, M. Using finite element simulation to optimize the heat treatment of tire protection chains. J. Mat. Eng. Perform. 2014, 23, 1288–1295. [Google Scholar] [CrossRef]
- Schemmel, M.; Prevedel, P.; Schöngrundner, R.; Ecker, W.; Antretter, T. Size effects in residual stress formation during quenching of cylinders made of hot-work tool steel. Adv. Mater. Sci. Eng. 2015, 2015, 1–7. [Google Scholar] [CrossRef]
- Greenwood, G.W.; Johnson, R.H. The deformation of metals under small stresses during phase transformations. Proc. R. Soc. A Math. Phys. Eng. Sci. 1965, 283, 403–422. [Google Scholar]
- Deng, D. FEM prediction of welding residual stress and distortion in carbon steel considering phase transformation effects. Mater. Des. 2009, 30, 359–366. [Google Scholar] [CrossRef]
- Pietzsch, R.; Brzoza, M.; Kaymak, Y.; Specht, E.; Bertram, A. Minimizing the distortion of steel profiles by controlled cooling. Steel Res. Int. 2005, 76, 399–407. [Google Scholar] [CrossRef]
- Nallathambi, A.K.; Kaymak, Y.; Specht, E.; Bertram, A. Sensitivity of material properties on distortion and residual stresses during metal quenching processes. J. Mater. Process. Technol. 2010, 210, 204–211. [Google Scholar] [CrossRef]
- Dassault Systems. ABAQUS Standard. Available online: https://www.3ds.com/products-services/simulia/products/abaqus/abaqusstandard/ (accessed on 13 January 2020).
- Wendelstorf, J.; Spitzer, K.-H.; Wendelstorf, R. Spray water cooling heat transfer at high temperatures and liquid mass fluxes. Int. J. Heat Mass Transf. 2008, 51, 4902–4910. [Google Scholar] [CrossRef]
- Brunbauer, S.; Winter, G.; Antretter, T.; Staron, P.; Ecker, W. Residual stress and microstructure evolution in steel tubes for different cooling conditions—Simulation and verification. Mater. Sci. Eng. A 2019, 747, 73–79. [Google Scholar] [CrossRef]
- Koistinen, D.P.; Marburger, R.E. A general equation prescribing the extent of the austenite-martensite transformation in pure iron-carbon alloys and plain carbon steels. Acta Metall. 1959, 7, 59–60. [Google Scholar] [CrossRef]
- Garrett, R.P.; Xu, S.; Lin, J.; Dean, T.A. A model for predicting austenite to bainite phase transformation in producing dual phase steels. Int. J. Mach. Tools Manuf. 2004, 44, 831–837. [Google Scholar] [CrossRef]
- Mahnken, R.; Schneidt, A.; Tschumak, S.; Maier, H.J. On the simulation of austenite to bainite phase transformation. Comput. Mater. Sci. 2011, 50, 1823–1829. [Google Scholar] [CrossRef]
- Mahnken, R.; Schneidt, A.; Antretter, T.; Ehlenbröker, U.; Wolff, M. Multi-scale modeling of bainitic phase transformation in multi-variant polycrystalline low alloy steels. Int. J. Solids Struct. 2015, 54, 156–171. [Google Scholar] [CrossRef]
- Lusk, M.; Krauss, G.; Jou, H.-J. A balance principle approach for modeling phase transformation kinetics. J. Phys. IV Fr. 1995, 5, C8-279–C8-284. [Google Scholar] [CrossRef][Green Version]
- Leblond, J.B.; Devaux, J.; Devaux, J.C. Mathematical modelling of transformation plasticity in steels I: Case of ideal-plastic phases. Int. J. Plast. 1989, 5, 551–572. [Google Scholar] [CrossRef]
- Neubert, S.; Pittner, A.; Rethmeier, M. Experimental determination of TRIP-parameter K for mild- and high-strength low-alloy steels and a super martensitic filler material. SpringerPlus 2016, 5, 754. [Google Scholar] [CrossRef] [PubMed]
- Moyer, J.M.; Ansell, G.S. The volume expansion accompanying the martensite transformation in iron-carbon alloys. MTA 1975, 6, 1785–1791. [Google Scholar] [CrossRef]
- Norström, L.-Å. The influence of nitrogen and grain size on yield strength in Type AISI 316L austenitic stainless steel. Metal Sci. 1977, 11, 208–212. [Google Scholar] [CrossRef]
- Sente Software Ltd. JMatPro. Available online: https://www.sentesoftware.co.uk/jmatpro (accessed on 13 January 2020).
- Heinze, C.; Pittner, A.; Rethmeier, M.; Babu, S.S. Dependency of martensite start temperature on prior austenite grain size and its influence on welding-induced residual stresses. Comput. Mater. Sci. 2013, 69, 251–260. [Google Scholar] [CrossRef]
- Chilton, J.M.; Kelly, P.M. The strength of ferrous martensite. Acta Metall. 1968, 16, 637–656. [Google Scholar] [CrossRef]
- Morito, S.; Yoshida, H.; Maki, T.; Huang, X. Effect of block size on the strength of lath martensite in low carbon steels. Mater. Sci. Eng. A 2006, 438–440, 237–240. [Google Scholar] [CrossRef]

| Title | Internal Cooling | External Cooling | Cooling Speed |
|---|---|---|---|
| (kgm−2s−1) | (kgm−2s−1) | (ms−1) | |
| Strategy 1 | - | 80 | 0.2 |
| Strategy 2 | 100 | 10 | 0.2 |
| Strategy 3 | 100 | 10 | 0.1 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Leitner, S.; Winter, G.; Klarner, J.; Antretter, T.; Ecker, W. Model-Based Residual Stress Design in Multiphase Seamless Steel Tubes. Materials 2020, 13, 439. https://doi.org/10.3390/ma13020439
Leitner S, Winter G, Klarner J, Antretter T, Ecker W. Model-Based Residual Stress Design in Multiphase Seamless Steel Tubes. Materials. 2020; 13(2):439. https://doi.org/10.3390/ma13020439
Chicago/Turabian StyleLeitner, Silvia, Gerald Winter, Jürgen Klarner, Thomas Antretter, and Werner Ecker. 2020. "Model-Based Residual Stress Design in Multiphase Seamless Steel Tubes" Materials 13, no. 2: 439. https://doi.org/10.3390/ma13020439
APA StyleLeitner, S., Winter, G., Klarner, J., Antretter, T., & Ecker, W. (2020). Model-Based Residual Stress Design in Multiphase Seamless Steel Tubes. Materials, 13(2), 439. https://doi.org/10.3390/ma13020439





