Influence of Particle Size on Toughening Mechanisms of Layered Silicates in CFRP
Abstract
:1. Introduction
2. Materials and Methods
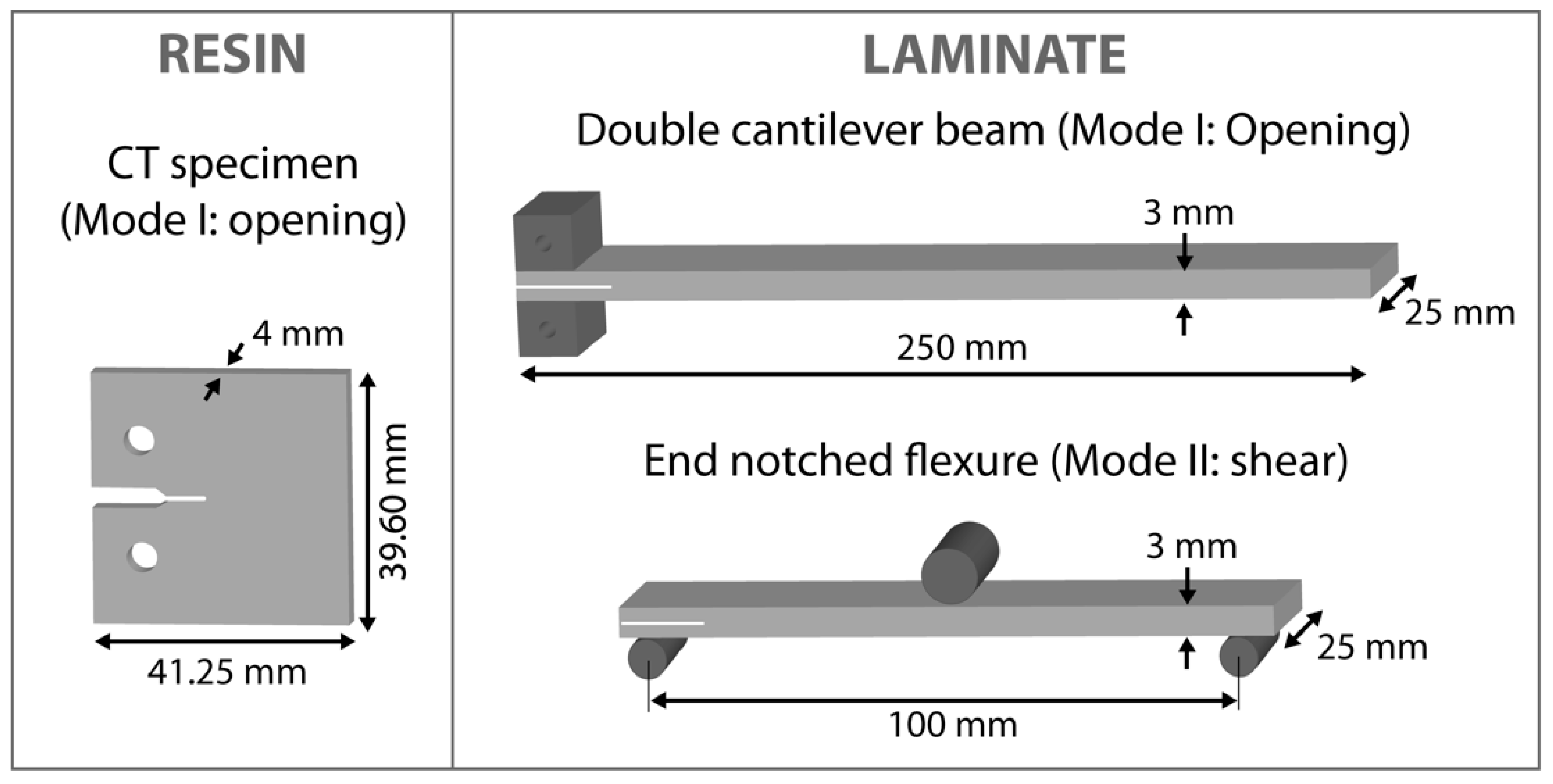
2.1. Materials and Sample Preparation
2.2. Characterization
3. Results and Discussion
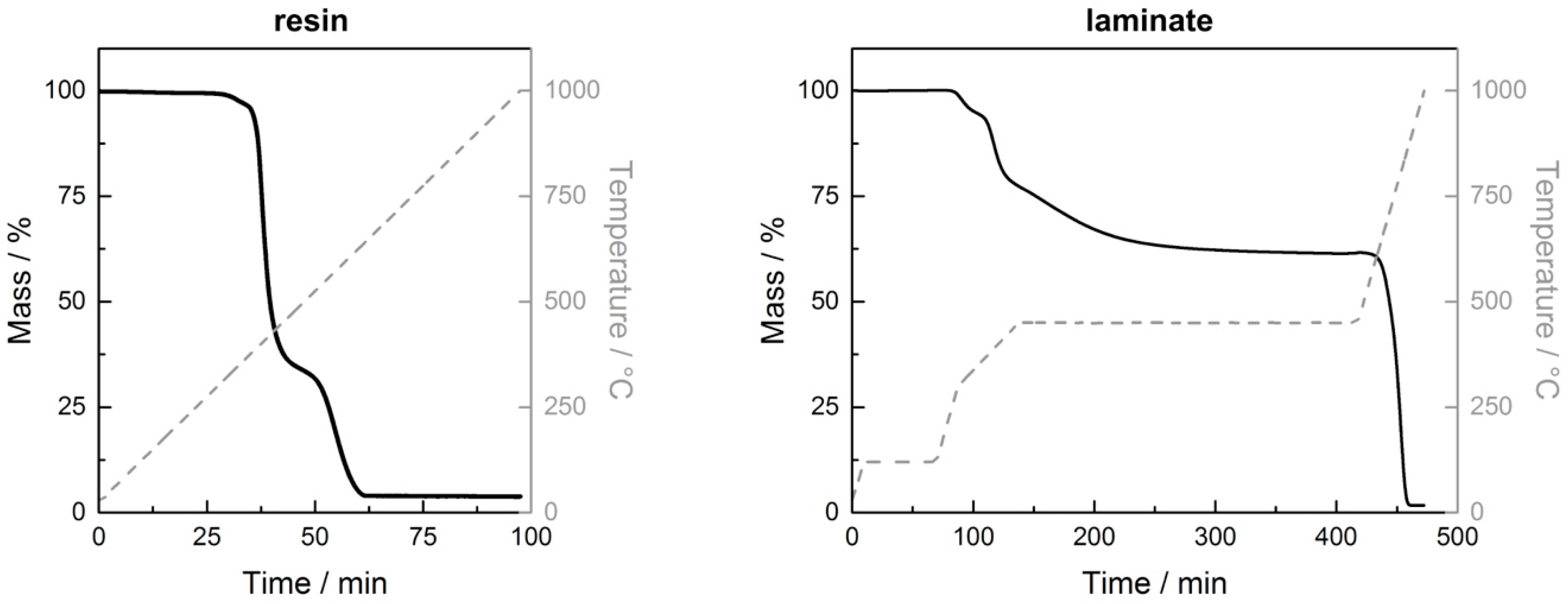
3.1. Filler and Fiber Contents
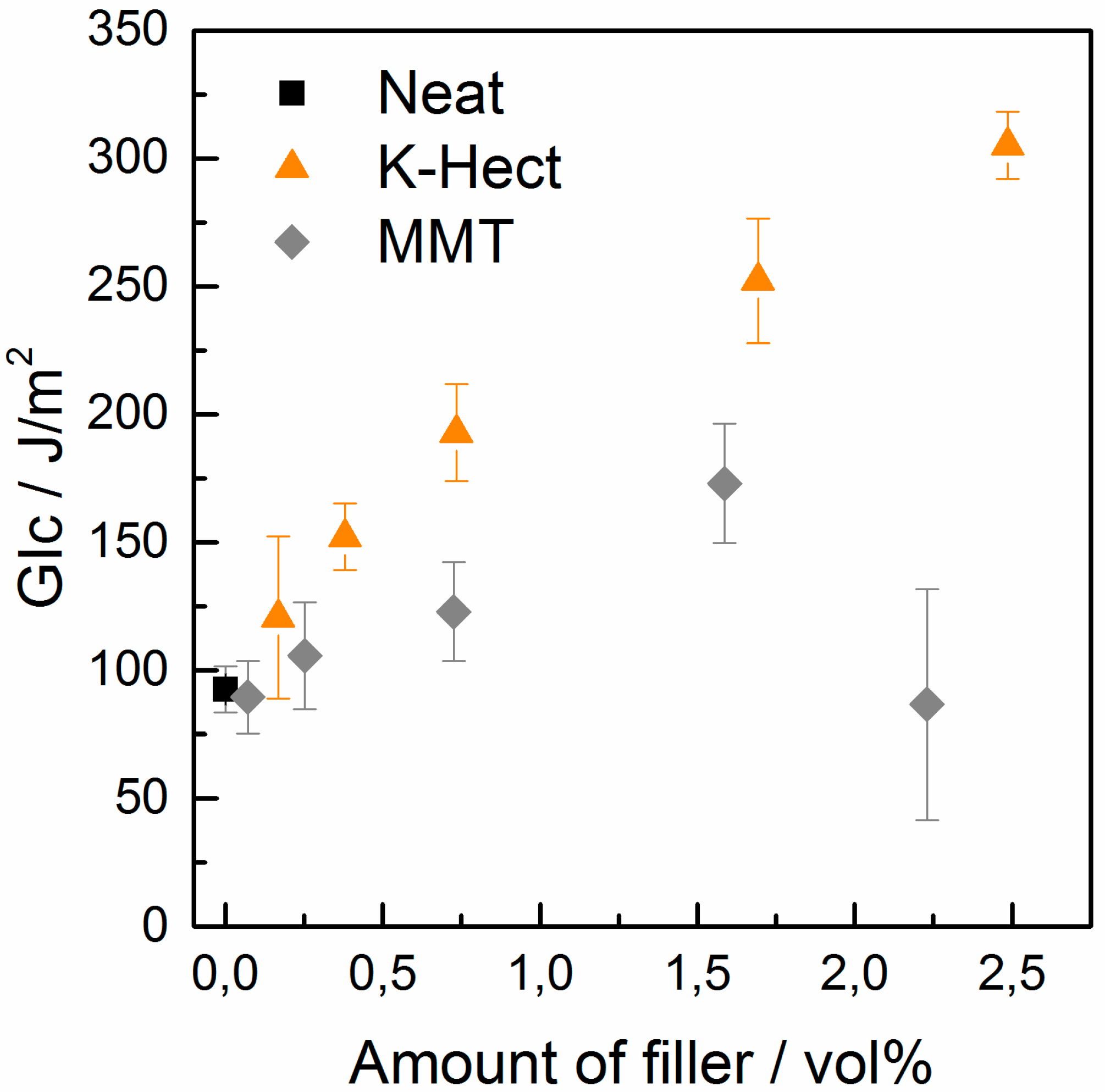
3.2. Properties of Epoxy-Clay-Nanocomposites
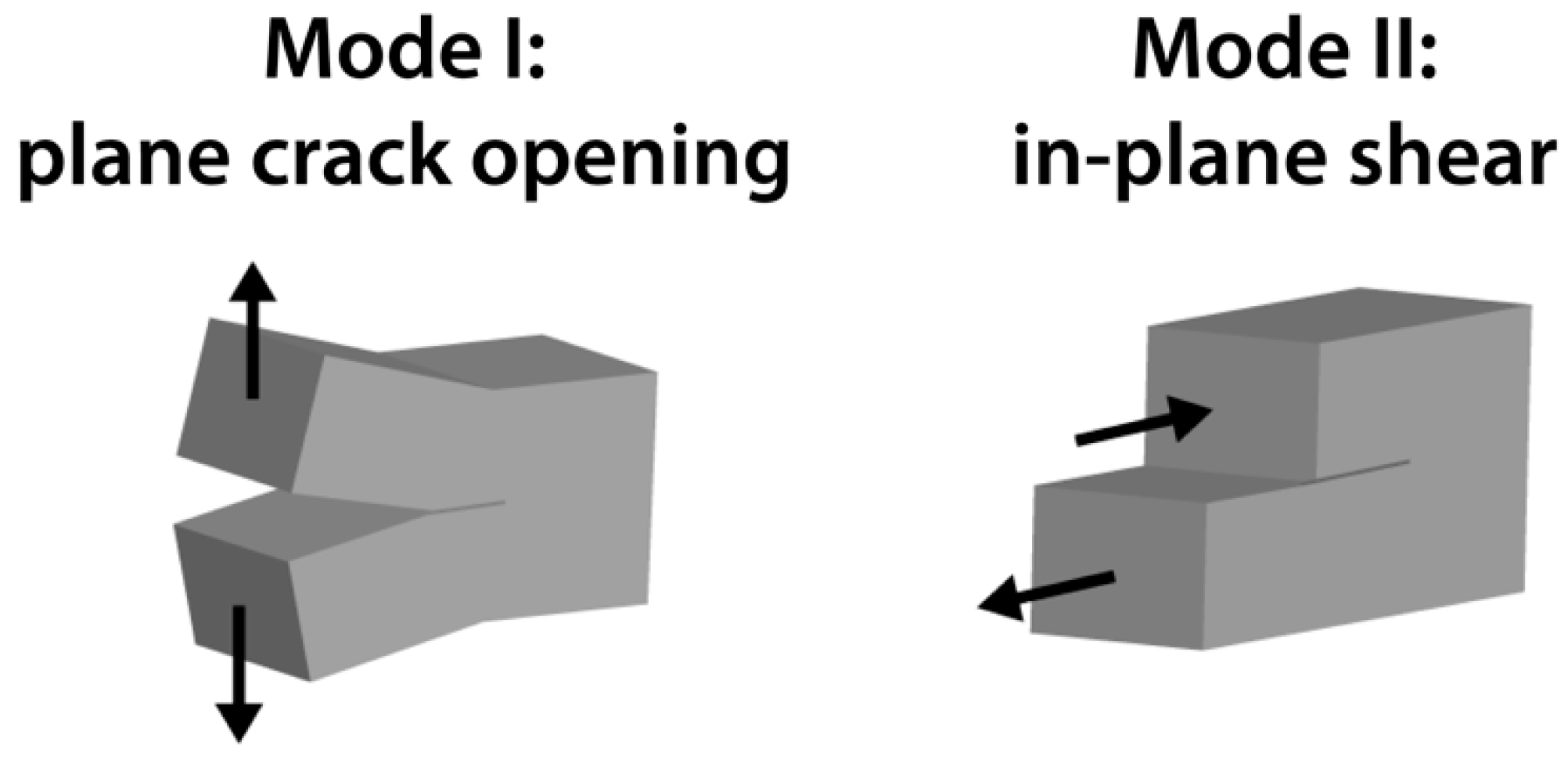
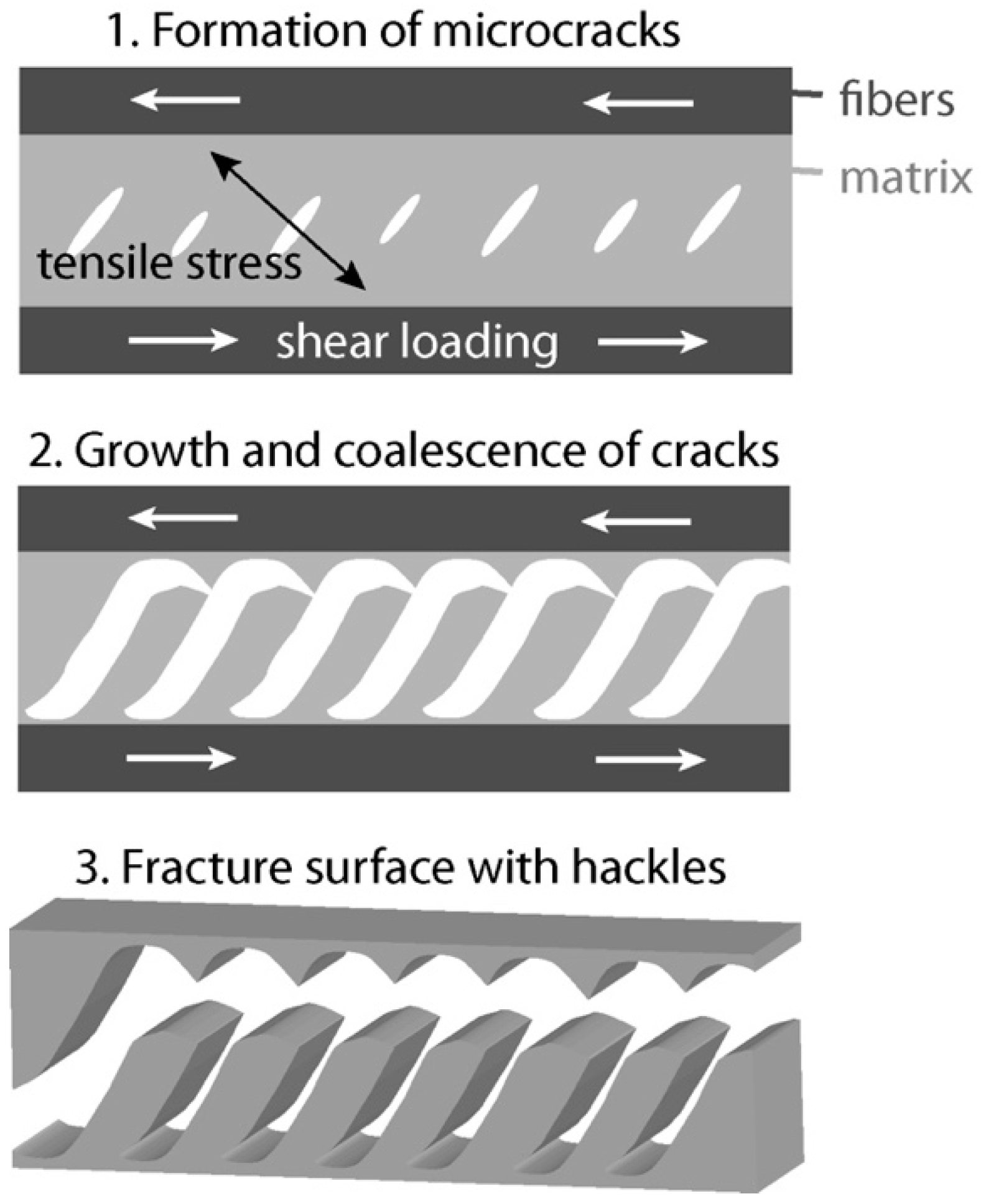
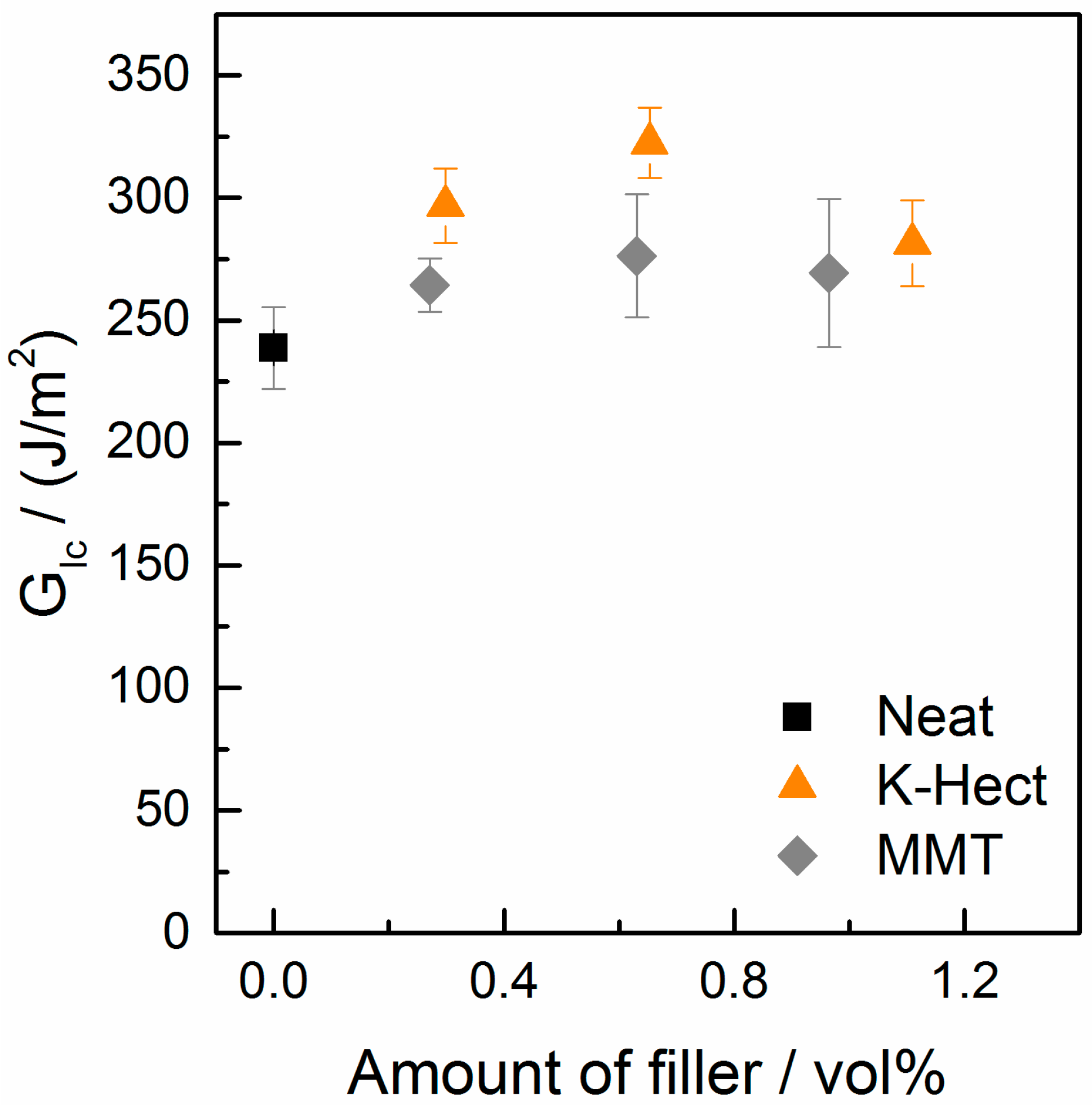
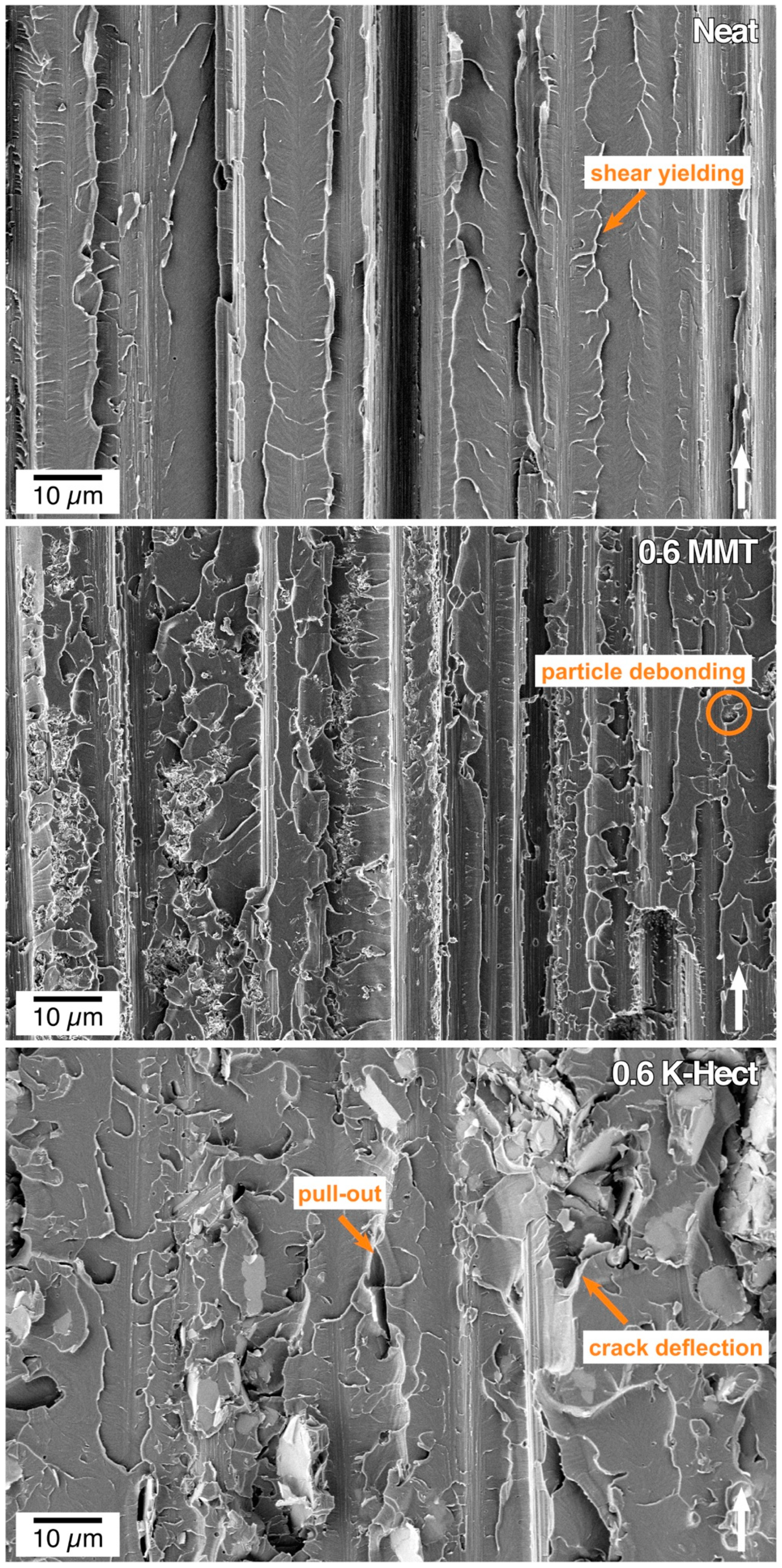
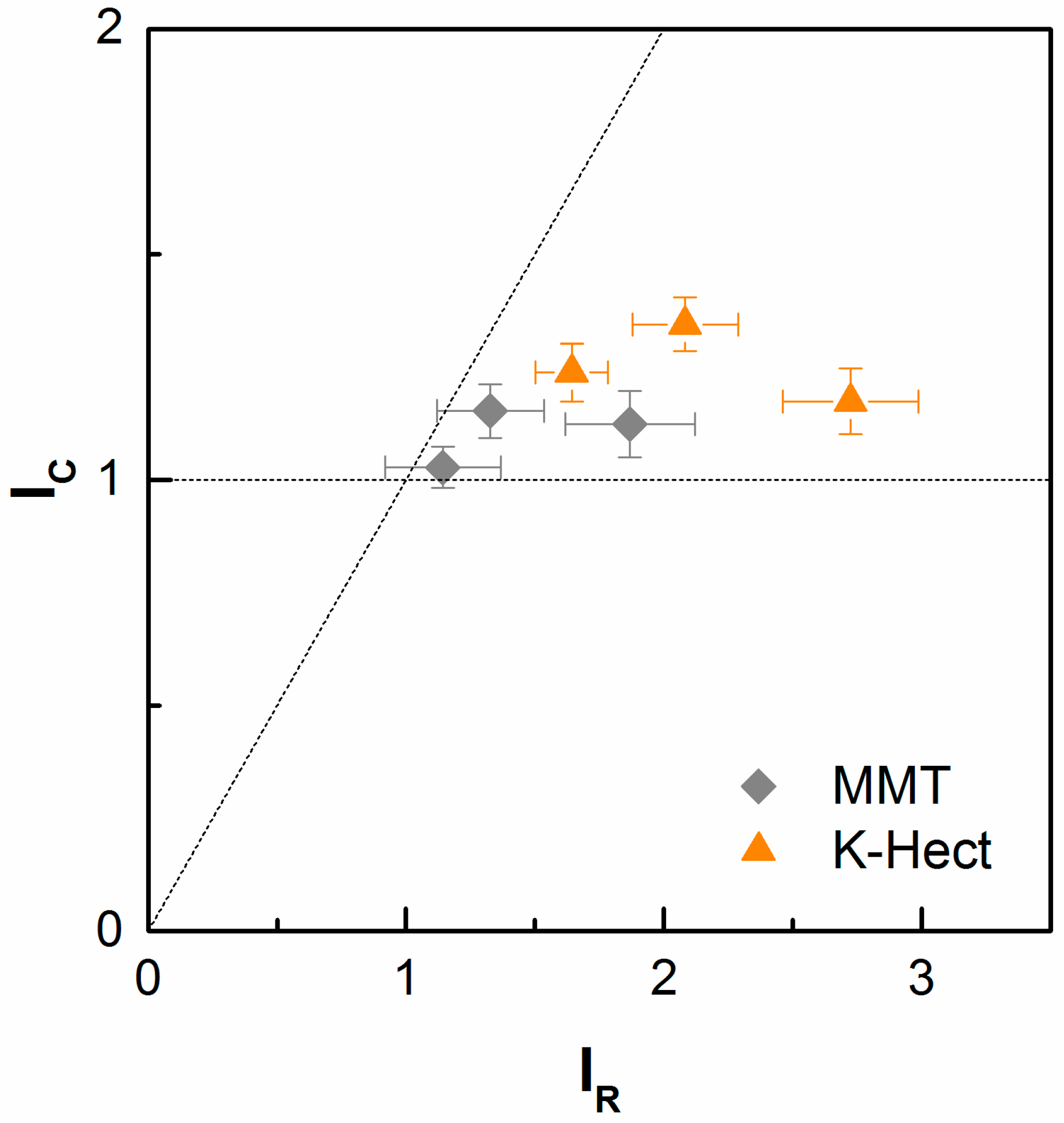
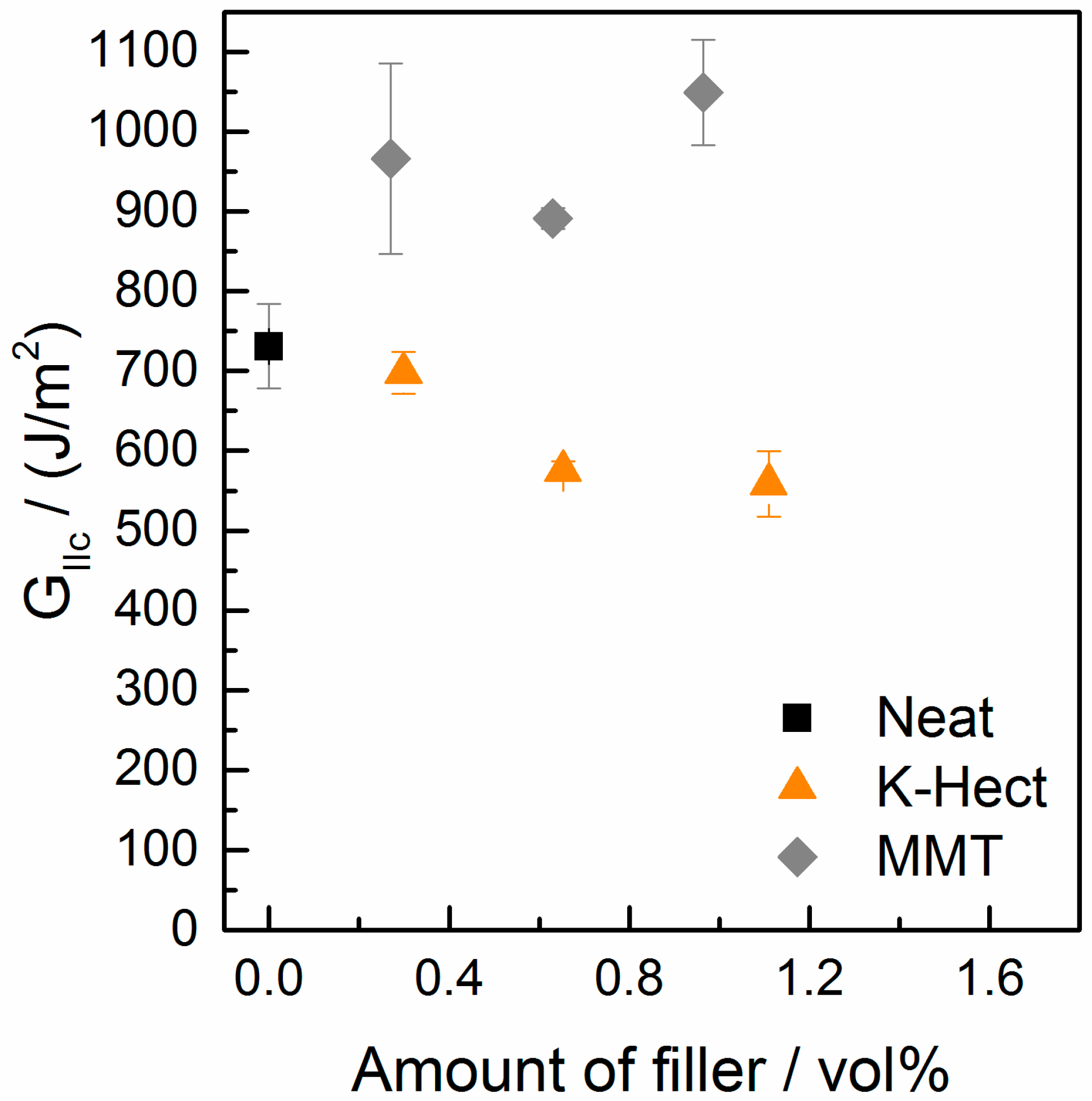
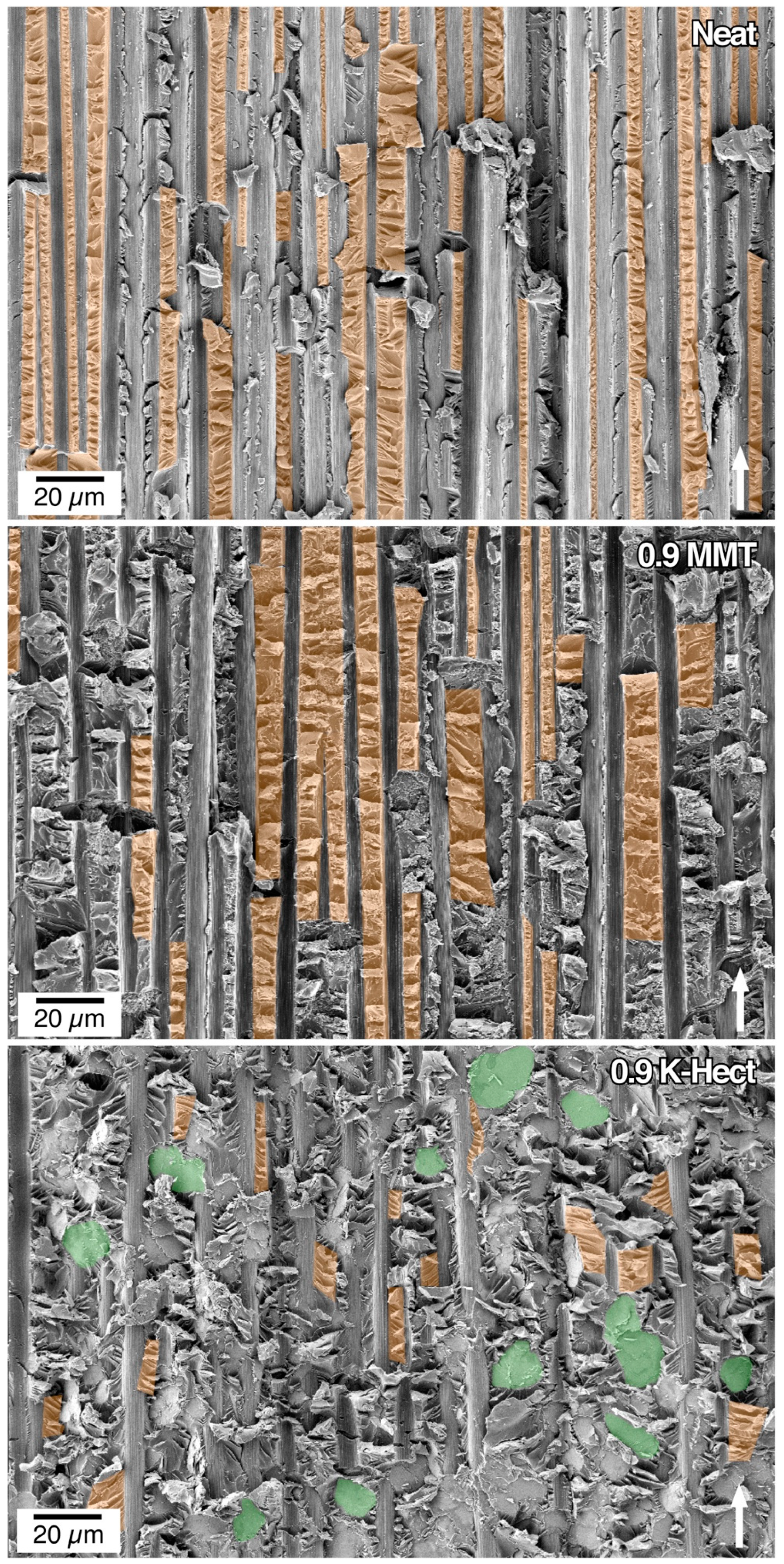
3.3. Properties of CFRP
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Soutis, C. Aerospace engineering requirements in building with composites. In Polymer Composites in the Aerospace Industry; Elsevier BV: Amsterdam, The Netherlands, 2020; pp. 3–22. [Google Scholar]
- Fishpool, D.T.; Rezai, A.; Baker, D.; Ogin, S.; A Smith, P. Interlaminar toughness characterisation of 3D woven carbon fibre composites. Plast. Rubber Compos. 2013, 42, 108–114. [Google Scholar] [CrossRef] [Green Version]
- Qin, W.; Chen, C.; Zhou, J.; Meng, J. Synergistic Effects of Graphene/Carbon Nanotubes Hybrid Coating on the Interfacial and Mechanical Properties of Fiber Composites. Materials 2020, 13, 1457. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Ramirez, V.A.; Hogg, P.J.; Sampson, W. The influence of the nonwoven veil architectures on interlaminar fracture toughness of interleaved composites. Compos. Sci. Technol. 2015, 110, 103–110. [Google Scholar] [CrossRef]
- Shivakumar, K.N.; Panduranga, R.; Sharpe, M. Interleaved Polymer Matrix Composites-A Review. In Proceedings of the 54th AIAA/ASME/ASCE/AHS/ASC Structures, Structural Dynamics, and Materials Conference; American Institute of Aeronautics and Astronautics (AIAA), Boston, MA, USA, 8–11 April 2013. [Google Scholar]
- Liu, K.; Macosko, C.W. Can nanoparticle toughen fiber-reinforced thermosetting polymers? J. Mater. Sci. 2018, 54, 4471–4483. [Google Scholar] [CrossRef]
- Bagheri, R.; Marouf, B.T.; Pearson, R.A. Rubber-Toughened Epoxies: A Critical Review. Polym. Rev. 2009, 49, 201–225. [Google Scholar] [CrossRef]
- Chen, L.; Wu, L.; Jiang, Q.; Tian, D.; Zhong, Z.; Wang, Y.; Fu, H.-J. Improving Interlaminar Fracture Toughness and Impact Performance of Carbon Fiber/Epoxy Laminated Composite by Using Thermoplastic Fibers. Molecules 2019, 24, 3367. [Google Scholar] [CrossRef] [Green Version]
- Becker, O.; Varley, R.J.; Simon, G.P. Morphology, thermal relaxations and mechanical properties of layered silicate nanocomposites based upon high-functionality epoxy resins. Polymer 2002, 43, 4365–4373. [Google Scholar] [CrossRef]
- Domun, N.; Paton, K.R.; Hadavinia, H.; Sainsbury, T.; Zhang, T.; Mohamud, H. Enhancement of Fracture Toughness of Epoxy Nanocomposites by Combining Nanotubes and Nanosheets as Fillers. Materials 2017, 10, 1179. [Google Scholar] [CrossRef] [Green Version]
- Kothmann, M.H.; Bakis, G.; Zeiler, R.; Ziadeh, M.; Breu, J.; Altstädt, V. Fatigue Crack Growth Behaviour of Epoxy Nanocomposites—Influence of Particle Geometry. In Self-Organized Morphology in Nanostructured Materials; Springer Science and Business Media LLC: New York, NY, USA, 2017; pp. 23–32. [Google Scholar]
- Carolan, D.; Kinloch, A.J.; Sprenger, S.; Taylor, A.C.; Ivankovic, A. Toughened carbon fibre-reinforced polymer composites with nanoparticle-modified epoxy matrices. J. Mater. Sci. 2016, 52, 1767–1788. [Google Scholar] [CrossRef] [Green Version]
- Domun, N.; Hadavinia, H.; Sainsbury, T.; Liaghat, G.; Vahid, S.; Zhang, T. Improving the fracture toughness and the strength of epoxy using nanomaterials—A review of the current status. Nanoscale 2015, 7, 10294–10329. [Google Scholar] [CrossRef] [Green Version]
- Kinloch, A.J.; Taylor, A.C. The mechanical properties and fracture behaviour of epoxy-inorganic micro and nano-composites. J. Mater. Sci. 2006, 41, 3271–3297. [Google Scholar] [CrossRef] [Green Version]
- Kothmann, M.H.; Ziadeh, M.; Bakis, G.; de Anda, A.R.; Breu, J.; Altstädt, V. Analyzing the influence of particle size and stiffness state of the nanofiller on the mechanical properties of epoxy/clay nanocomposites using a novel shear-stiff nano-mica. J. Mater. Sci. 2015, 50, 4845–4859. [Google Scholar] [CrossRef]
- Bakis, G.; Kothmann, M.H.; Zeiler, R.; Brückner, A.; Ziadeh, M.; Breu, J.; Altstädt, V. Influence of size, aspect ratio and shear stiffness of nanoclays on the fatigue crack propagation behavior of their epoxy nanocomposites. Polymer 2018, 158, 372–380. [Google Scholar] [CrossRef]
- Jung, A.; Peter, K.; Demco, D.E.; Jehnichen, D.; Moeller, M. Nanocomposites Based on Layered Silicates and Epoxy Resins: Measurement of Clay Dispersion and Exfoliation using TEM, Solid-State NMR, and X-ray Diffraction Methods. Macromol. Chem. Phys. 2012, 213, 389–400. [Google Scholar] [CrossRef]
- Agubra, V.A.; Owuor, P.S.; Hosur, M. Influence of Nanoclay Dispersion Methods on the Mechanical Behavior of E-Glass/Epoxy Nanocomposites. Nanomaterials 2013, 3, 550–563. [Google Scholar] [CrossRef]
- Zotti, A.; Zuppolini, S.; Zarrelli, M.; Borriello, A. Fracture Toughening Mechanisms in Epoxy Adhesives. Adhes. Appl. Prop. 2016, 237–269. [Google Scholar] [CrossRef] [Green Version]
- Guo, Q. Thermosets: Structure, Properties, and Applications; Elsevier Science: Amsterdam, The Netherlands, 2017. [Google Scholar]
- Fischer, B.; Ziadeh, M.; Pfaff, A.; Breu, J.; Altstädt, V. Impact of large aspect ratio, shear-stiff, mica-like clay on mechanical behaviour of PMMA/clay nanocomposites. Polymer 2012, 53, 3230–3237. [Google Scholar] [CrossRef]
- Montazeri, A.; Kamrava, S.; Yeganeh, M. Assessment of dominant factors affecting the fracture toughness of polymer/clay nanocomposites using finite element method. J. Reinf. Plast. Compos. 2017, 36, 1545–1553. [Google Scholar] [CrossRef]
- Lange, F.F. The interaction of a crack front with a second-phase dispersion. Philos. Mag. 1970, 22, 0983–0992. [Google Scholar] [CrossRef]
- Evans, A.G. The strength of brittle materials containing second phase dispersions. Philos. Mag. 1972, 26, 1327–1344. [Google Scholar] [CrossRef]
- Green, D.; Nicholson, P.S.; Embury, J.D. Fracture of a brittle particulate composite – Part 1 Experimental Aspects. J. Mater. Sci. 1979, 14, 1413–1420. [Google Scholar] [CrossRef]
- Green, D.; Nicholson, P.S.; Embury, J.D. Fracture of a brittle particulate composite – Part 2 Theoretical Aspects. J. Mater. Sci. 1979, 14, 1657–1661. [Google Scholar] [CrossRef]
- Siddiqui, N.A.; Woo, R.S.; Kim, J.-K.; Leung, C.K.; Munir, A. Mode I interlaminar fracture behavior and mechanical properties of CFRPs with nanoclay-filled epoxy matrix. Compos. Part A Appl. Sci. Manuf. 2007, 38, 449–460. [Google Scholar] [CrossRef] [Green Version]
- Wang, K.; Chen, L.; Wu, J.; Toh, M.L.; He, C.; Yee, A.F. Epoxy Nanocomposites with Highly Exfoliated Clay: Mechanical Properties and Fracture Mechanisms. Macromolecules 2005, 38, 788–800. [Google Scholar] [CrossRef]
- Zerda, A.S.; Lesser, A.J. Intercalated clay nanocomposites: Morphology, mechanics, and fracture behavior. J. Polym. Sci. Part B Polym. Phys. 2001, 39, 1137–1146. [Google Scholar] [CrossRef]
- Ziadeh, M.; Fischer, B.; Schmid, J.; Altstädt, V.; Breu, J. On the importance of specific interface area in clay nanocomposites of PMMA filled with synthetic nano-mica. Polymer 2014, 55, 3770–3781. [Google Scholar] [CrossRef]
- Becker, O.; Varley, R.J.; Simon, G.P. Use of layered silicates to supplementarily toughen high performance epoxy-carbon fiber composites. J. Mater. Sci. Lett. 2003, 22, 1411–1414. [Google Scholar] [CrossRef]
- Tang, Y.; Ye, L.; Zhang, Z.; Friedrich, K. Interlaminar fracture toughness and CAI strength of fibre-reinforced composites with nanoparticles—A review. Compos. Sci. Technol. 2013, 86, 26–37. [Google Scholar] [CrossRef]
- Quaresimin, M.; Varley, R.J. Understanding the effect of nano-modifier addition upon the properties of fibre reinforced laminates. Compos. Sci. Technol. 2008, 68, 718–726. [Google Scholar] [CrossRef]
- Subramaniyan, A.K.; Sun, C. Interlaminar Fracture Behavior of Nanoclay Reinforced Glass Fiber Composites. J. Compos. Mater. 2008, 42, 2111–2122. [Google Scholar] [CrossRef]
- Tsai, J.-L.; Wu, M.-D. Organoclay Effect on Mechanical Responses of Glass/Epoxy Nanocomposites. J. Compos. Mater. 2008, 42, 553–568. [Google Scholar] [CrossRef]
- Quaresimin, M.; Salviato, M.; Zappalorto, M. Fracture and interlaminar properties of clay-modified epoxies and their glass reinforced laminates. Eng. Fract. Mech. 2012, 81, 80–93. [Google Scholar] [CrossRef]
- Phonthammachai, N.; Li, X.; Wong, S.; Chia, H.; Tjiu, W.W.; He, C. Fabrication of CFRP from high performance clay/epoxy nanocomposite: Preparation conditions, thermal–mechanical properties and interlaminar fracture characteristics. Compos. Part A Appl. Sci. Manuf. 2011, 42, 881–887. [Google Scholar] [CrossRef]
- Selzer, R.; Krey, J. Fractography of interlaminar fracture surfaces of CF/PI and CF/BMI composites. J. Mater. Sci. 1994, 29, 2951–2956. [Google Scholar] [CrossRef]
- Siddique, A.; Abid, S.; Shafiq, F.; Nawab, Y.; Wang, H.; Shi, B.; Saleemi, S.; Sun, B. Mode I fracture toughness of fiber-reinforced polymer composites: A review. J. Ind. Text. 2019. [Google Scholar] [CrossRef]
- Kalo, H.; Möller, M.W.; Ziadeh, M.; Dolejš, D.; Breu, J. Large scale melt synthesis in an open crucible of Na-fluorohectorite with superb charge homogeneity and particle size. Appl. Clay Sci. 2010, 48, 39–45. [Google Scholar] [CrossRef]
- Möller, M.W.; Handge, U.A.; Kunz, D.A.; Lunkenbein, T.; Altstädt, V.; Breu, J. Tailoring Shear-Stiff, Mica-like Nanoplatelets. ACS Nano 2010, 4, 717–724. [Google Scholar] [CrossRef] [PubMed]
- Ziadeh, M.; Chwalka, B.; Kalo, H.; Schütz, M.R.; Breu, J. A simple approach for producing high aspect ratio fluorohectorite nanoplatelets utilizing a stirred media mill. Clay Miner. 2012, 47, 341–353. [Google Scholar] [CrossRef]
- Ziadeh, M.; Weiss, S.; Fischer, B.; Förster, S.; Altstädt, V.; Müller, A.H.; Breu, J.; Müller, A.H.E. Towards completely miscible PMMA nanocomposites reinforced by shear-stiff, nano-mica. J. Colloid Interface Sci. 2014, 425, 143–151. [Google Scholar] [CrossRef]


© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hutschreuther, J.; Kunz, R.; Breu, J.; Altstädt, V. Influence of Particle Size on Toughening Mechanisms of Layered Silicates in CFRP. Materials 2020, 13, 2396. https://doi.org/10.3390/ma13102396
Hutschreuther J, Kunz R, Breu J, Altstädt V. Influence of Particle Size on Toughening Mechanisms of Layered Silicates in CFRP. Materials. 2020; 13(10):2396. https://doi.org/10.3390/ma13102396
Chicago/Turabian StyleHutschreuther, Julia, Raphael Kunz, Josef Breu, and Volker Altstädt. 2020. "Influence of Particle Size on Toughening Mechanisms of Layered Silicates in CFRP" Materials 13, no. 10: 2396. https://doi.org/10.3390/ma13102396
APA StyleHutschreuther, J., Kunz, R., Breu, J., & Altstädt, V. (2020). Influence of Particle Size on Toughening Mechanisms of Layered Silicates in CFRP. Materials, 13(10), 2396. https://doi.org/10.3390/ma13102396





