Active Adjustment of Surface Accuracy for a Large Cable-Net Structure by Shape Memory Alloy
Abstract
:1. Introduction
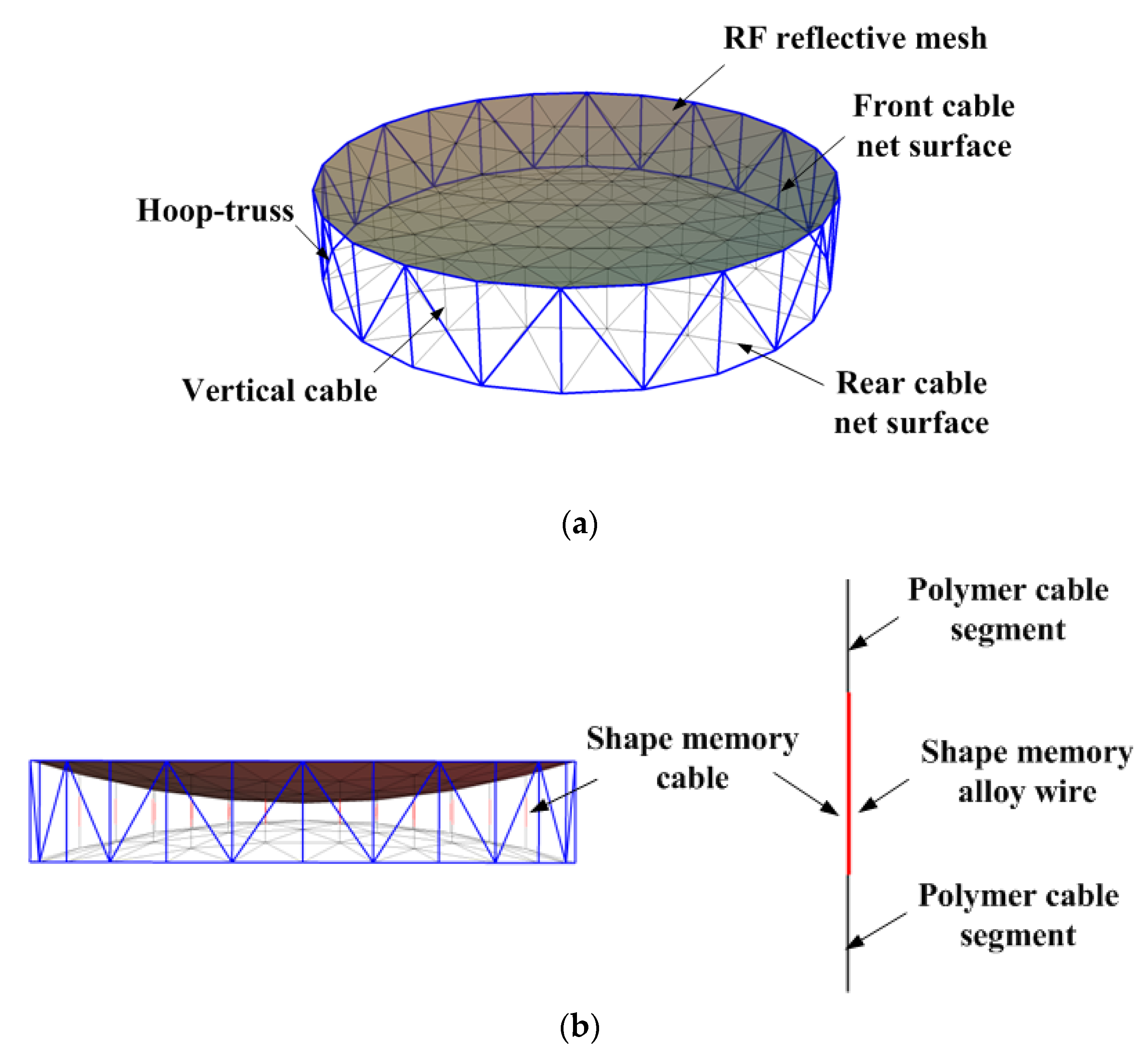
2. Finite Element Modeling for the SMC Structure
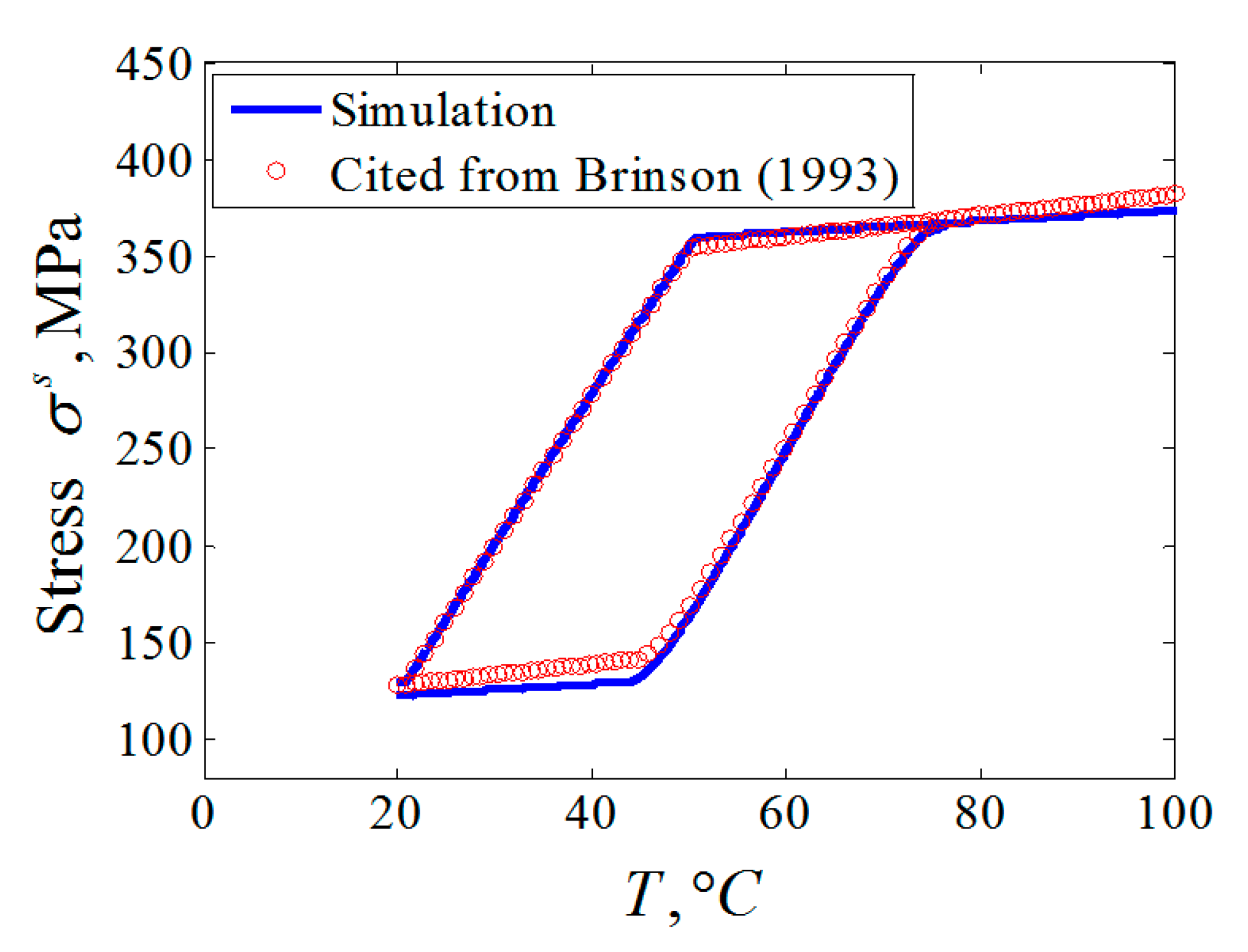
2.1. Constitutive Equation
2.2. Finite Element Modeling for the One-Dimensional Cable Element of SMA
2.3. Finite Element Modeling for the SMC Structure
3. Optimization Model for Shape Adjustment
3.1. Design Variables
3.2. Objective Functions
3.3. Optimization Model
4. Solution of the Optimization Model
4.1. Treatment of Objective Functions
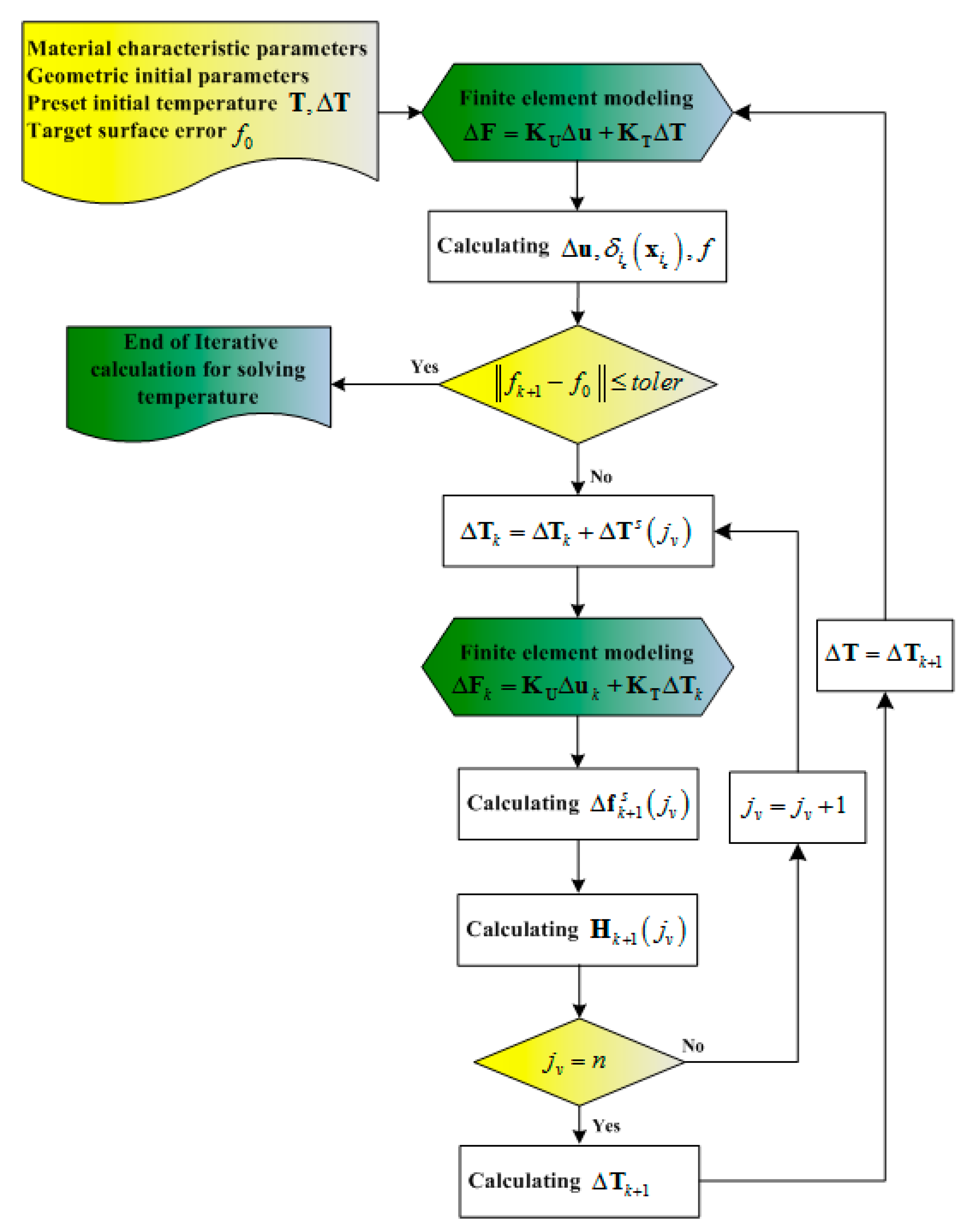
4.2. Iterative Procedure
- 1)
- Given the initial state of the (k+1)th iterative substep: , and , where is the designated temperature increment vector of the SMA cables for the optimization calculation, is the temperature vector of the SMA cables of kth iterative substep, and is the surface error of kth iterative substep.
- 2)
- Therefore, the temperature vector of the SMA cables of the (k+1)th iterative substep for the optimization calculation can be given as: .
- 3)
- The surface error vector corresponding to the SMA cable element is given as: under the temperature increment of the SMA cable element, where is the objective surface error.
- 4)
- These results were used to create the small perturbation influence matrix for optimization as: .
- 5)
- The least squares solution for the temperature vector of the SMA cables of the (k+1)th iterative substep can be calculated by .
- 6)
- Finally, the temperature vector of the SMA cables of the (k+1)th iterative substep for the surface error is given as: . The iterative termination conditions were set as: .
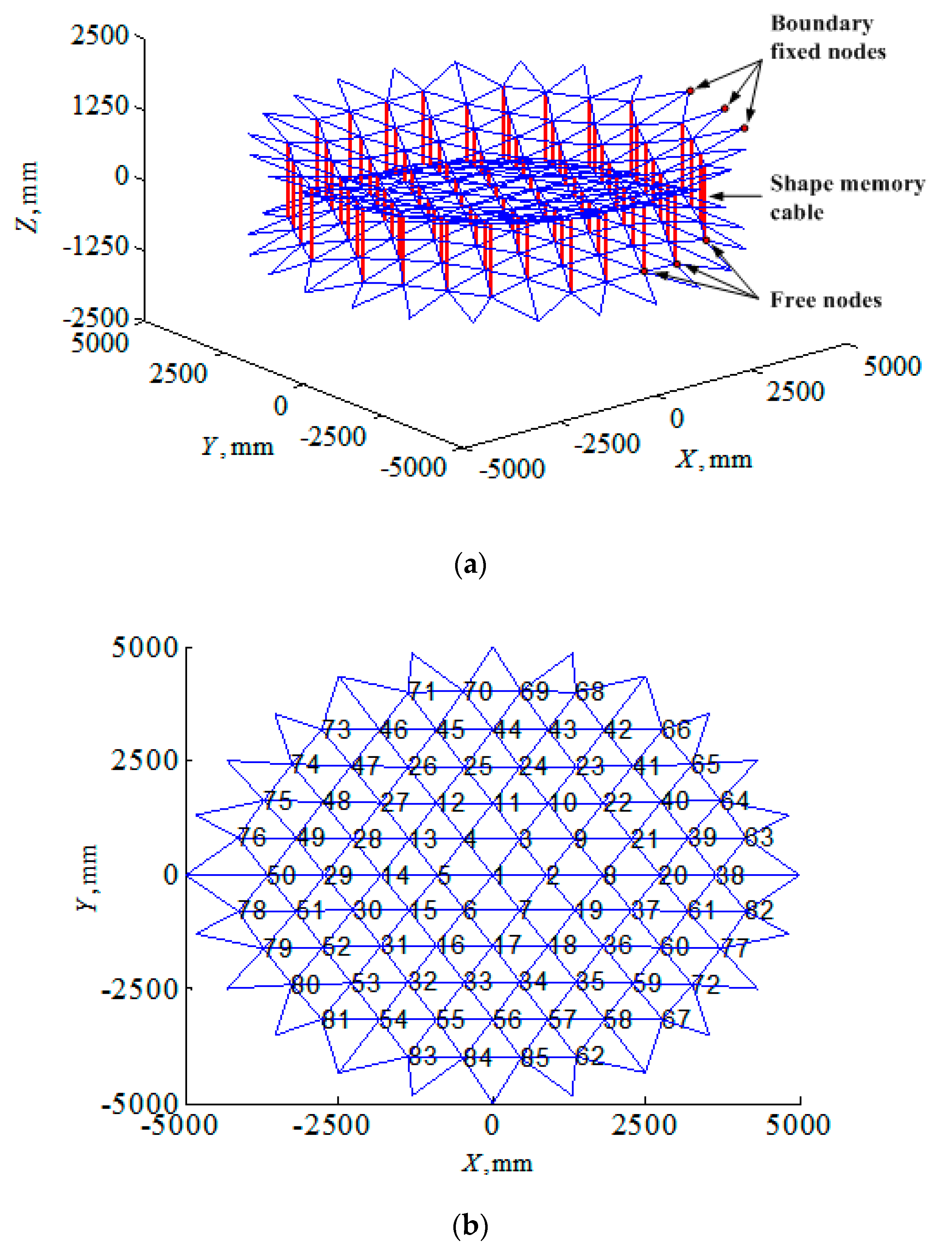
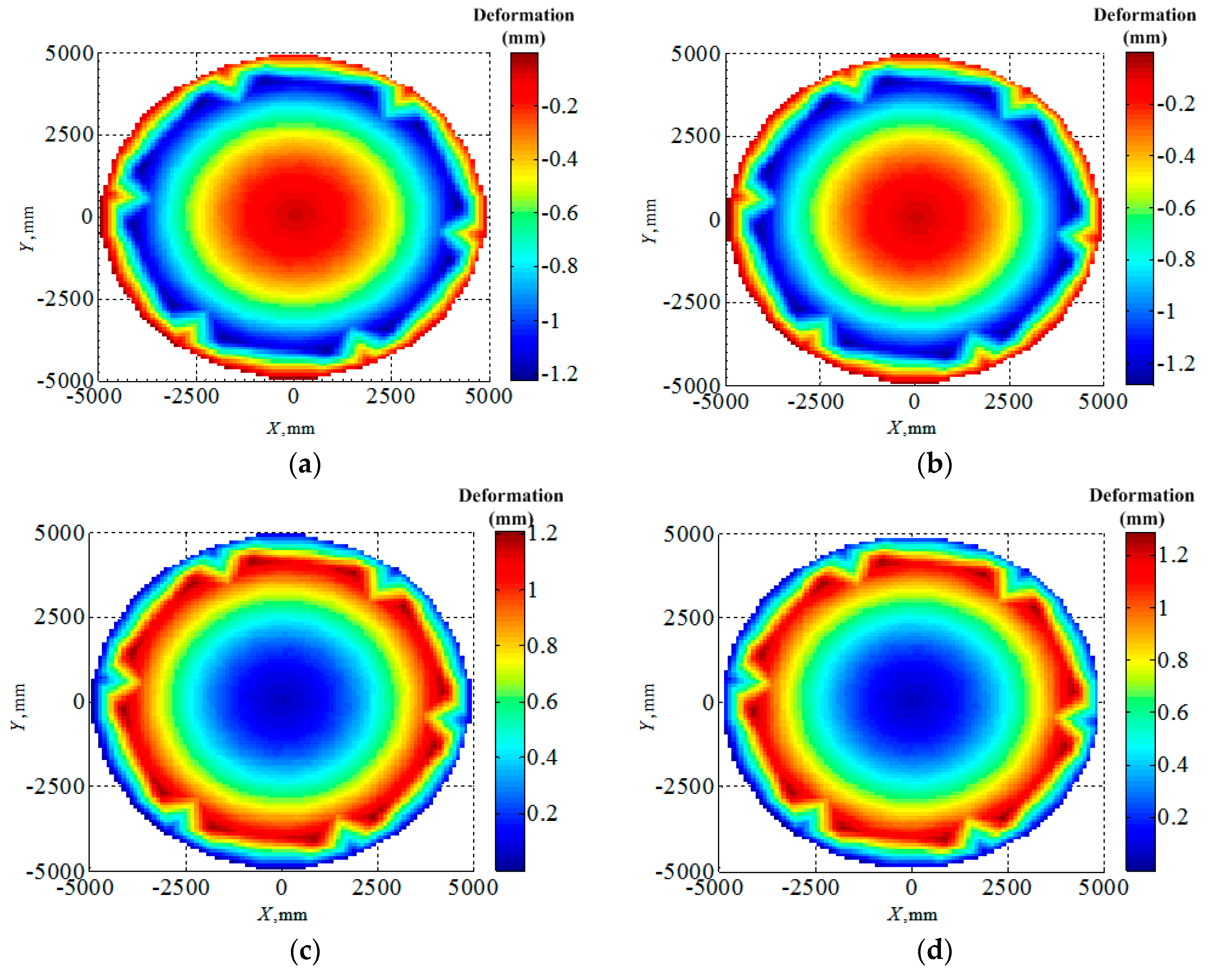
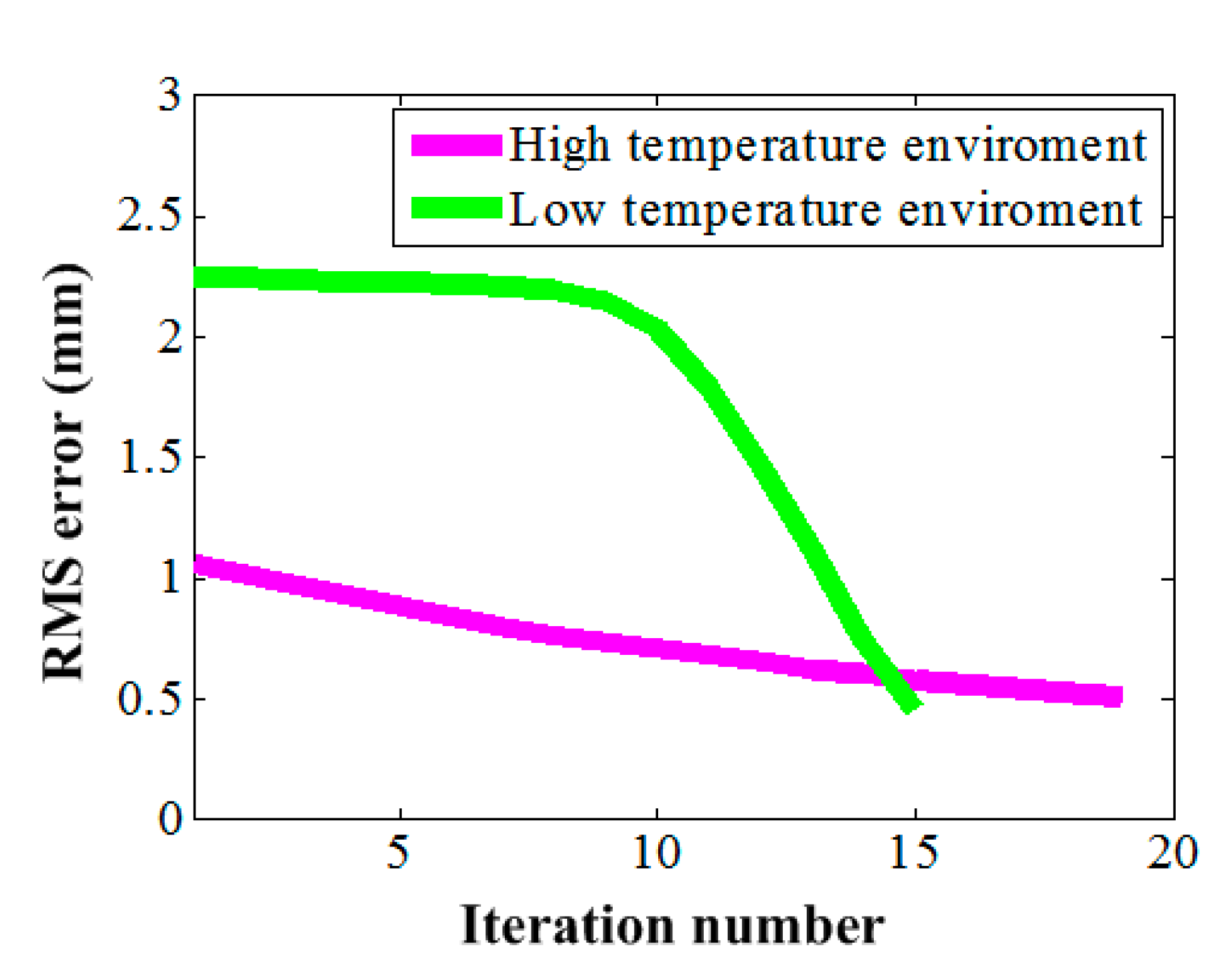
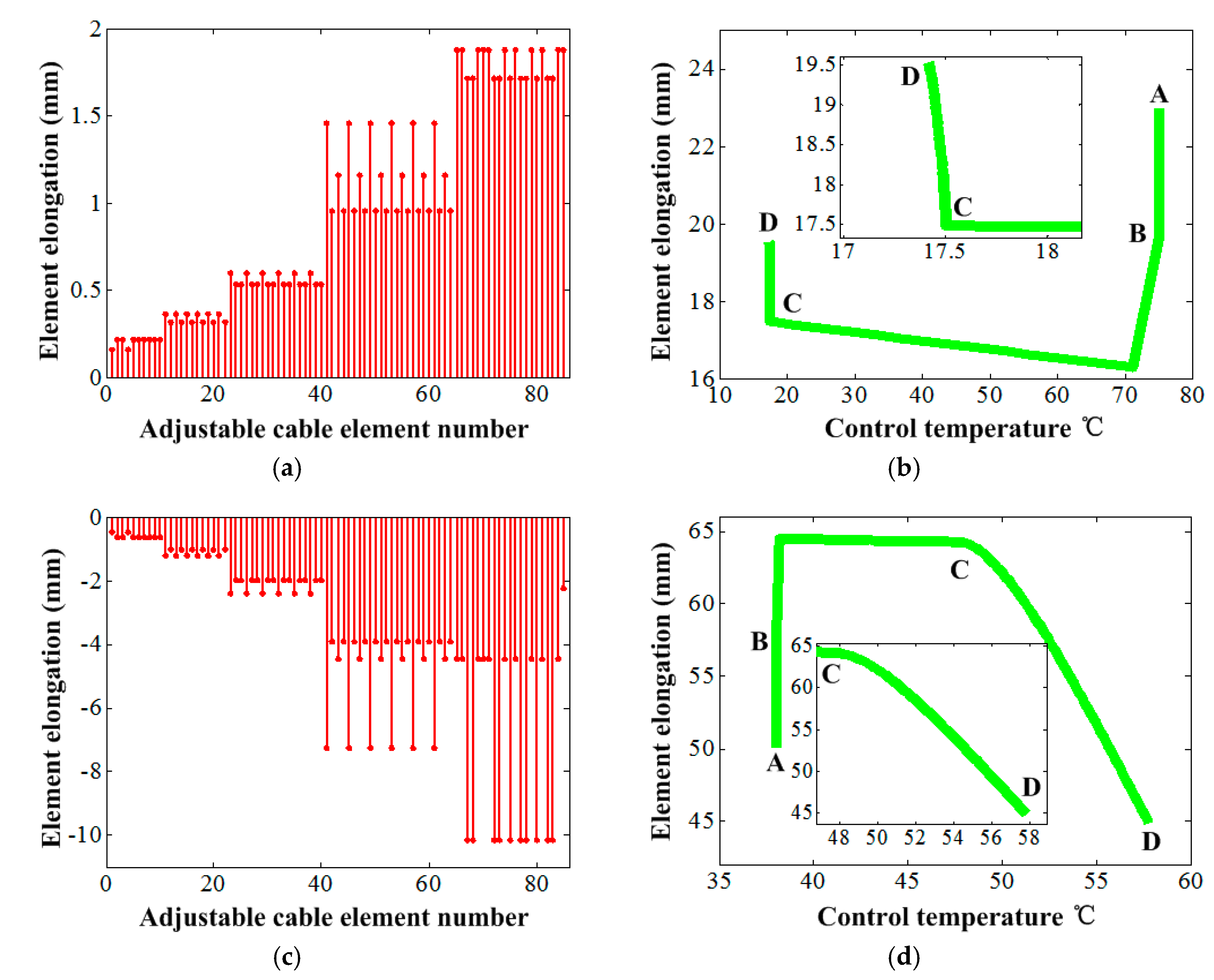
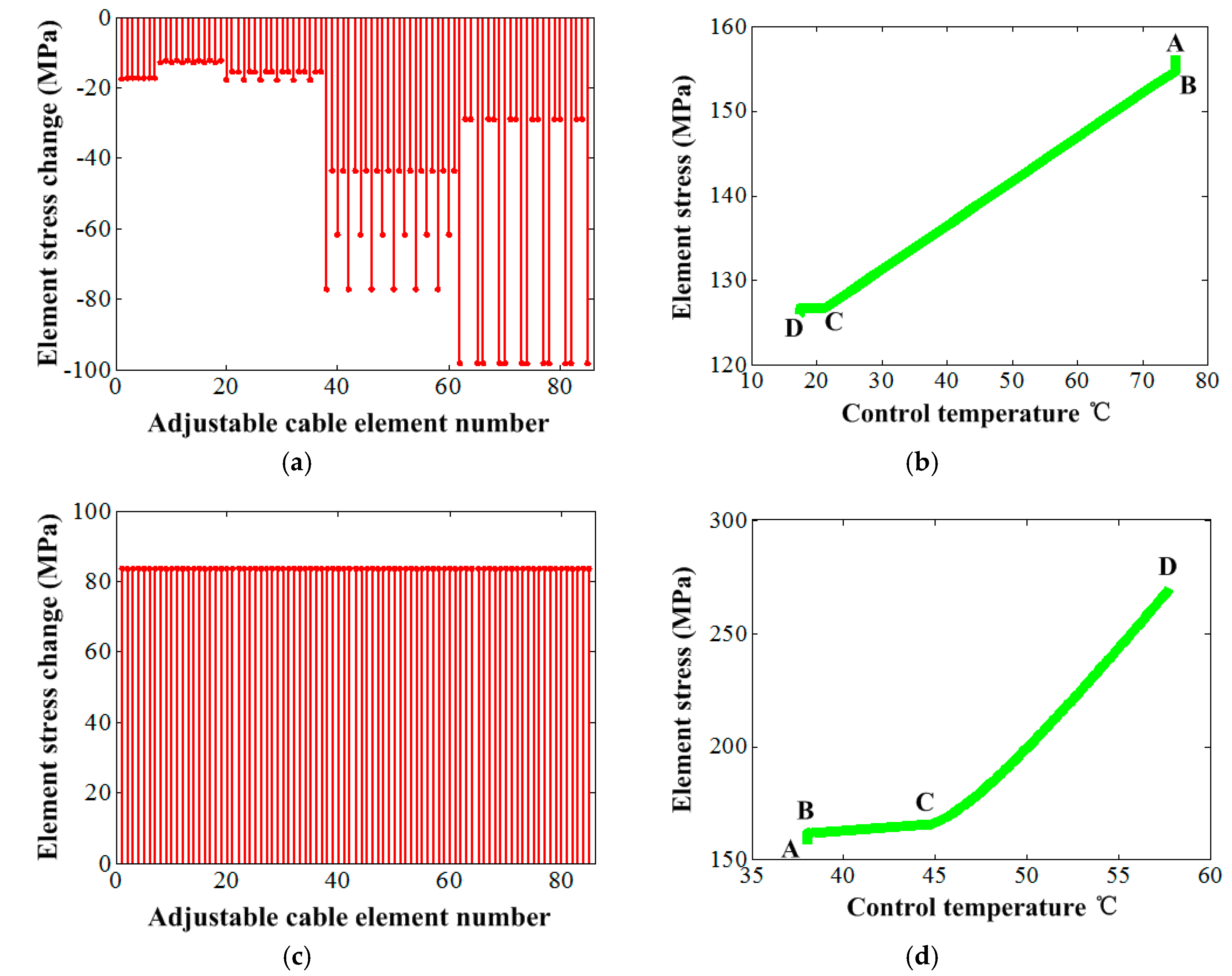
5. Numerical Simulation
6. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Meguro, A.; Shintate, K.; Usui, M.; Tsujihata, A. In-orbit deployment characteristics of large deployable antenna reflector onboard Engineering Test Satellite VIII. Acta Astronaut. 2009, 65, 1306–1316. [Google Scholar] [CrossRef]
- Mobrem, M.; Kuehn, S.; Spier, C.; Slimko, E. Design and performance of astromesh reflector onboard soil moisture active passive spacecraft. In Proceedings of the IEEE Aerospace Conference, Big Sky, MT, USA, 3–10 March 2012; pp. 1–10. [Google Scholar]
- Tibert, G.A. Deployable Tensegrity Structure for Space Applications. Ph.D. Thesis, Royal Institute of Technololy, Department of Mechanics. Stockholm, Sweden, 2002. [Google Scholar]
- Scialino, L. Large deployable reflectors for telecom and earth observation applications. CEAS Space J. 2013, 5, 125–146. [Google Scholar] [CrossRef]
- Liu, W.; Li, D.X. Simple technique for form-finding and tension determining of cable-network antenna reflectors. J. Spacecr. Rocket. 2013, 50, 479–481. [Google Scholar]
- Tanaka, H.; Natori, M.C. Shape control of space antennas consisting of cable networks. Acta Astronaut. 2004, 55, 519–527. [Google Scholar] [CrossRef]
- Jin, M.; Yasaka, T.; Miura, K. Shape control of the tension truss antenna. AIAA J. 1989, 28, 316–322. [Google Scholar]
- Xu, X.; Luo, Y.Z. Multi-objective shape control of prestressed structures with genetic algorithms. J. Aerosp. Eng. 2008, 222, 1139–1147. [Google Scholar] [CrossRef]
- Di, J.J.; Zhao, Y.; Sun, Q. Shape adjustment based on optimization for cable mesh deployable antenna. In Proceedings of the International Symposium on System and Control in Aeronautics and Astronautics (ISSCAA), Harbin, China, 8–10 June 2010; pp. 1090–1094. [Google Scholar]
- Tabata, M.; Yamamoto, K.; Inoue, T. Shape adjustment of a flexible space antenna reflector. J. Intell. Mater. Syst. Struct. 1992, 3, 646–658. [Google Scholar] [CrossRef]
- Du, J.L.; Zong, Y.L.; Bao, H. Shape adjustment of cable mesh antennas using sequential quadratic programming. Aerosp. Sci. Technol. 2013, 30, 26–32. [Google Scholar] [CrossRef]
- Tanaka, H. Surface error estimation and correction of a space antenna based on antenna gain analyses. Acta Astronaut. 2011, 68, 1062–1069. [Google Scholar] [CrossRef]
- Ruze, J. Antenna tolerance theory-a review. Proc. IEEE 1966, 54, 633–640. [Google Scholar] [CrossRef]
- Zong, Y.L.; Zhang, S.X.; Du, J.L.; Yang, G.G.; Xu, W.Y.; Hu, N.G. Shape Control of Cable-Network Antennas Considering the RF Performance. IEEE Trans. Antennas Propag. 2016, 64, 839–848. [Google Scholar] [CrossRef]
- Yoon, H.S. Design, modeling, and optimization of a mechanically reconfigurable smart reflector antenna system. IEEE Trans. Antennas Propag. 2002, 50, 628–637. [Google Scholar]
- Wang, Z.; Li, T.; Cao, Y. Active shape adjustment of cable net structures with PZT actuators. Aerosp. Sci. Technol. 2013, 26, 160–168. [Google Scholar] [CrossRef]
- Liu, W.; Li, D.X. Placement optimization of smart piezoelectric rods for shape control of large cable-network antenna structures. Adv. Mater. Res. 2013, 705, 602–608. [Google Scholar] [CrossRef]
- Hu, J.Q.; Lin, S.; Dai, F.H. Pattern reconfigurable antenna based on morphing bistable composite laminates. IEEE Trans. Antennas Propag. 2017, 65, 2196–2207. [Google Scholar] [CrossRef]
- Mazlouman, S.J.; Mahanfar, A.; Menon, C. Reconfigurable axial-mode helix antennas using shape memory alloys. IEEE Trans. Antennas Propag. 2011, 59, 1070–1077. [Google Scholar] [CrossRef]
- Mazlouman, S.J.; Mahanfar, A.; Menon, C. Square ring antenna with reconfigurable patch using shape memory alloy actuation. IEEE Trans. Antennas Propag. 2012, 60, 5627–5634. [Google Scholar] [CrossRef]
- Kalra, S.; Bhattacharya, B.; Munjal, B.S. Development of shape memory alloy actuator integrated flexible poly-ether-ether-ketone antenna with simultaneous beam steering and shaping ability. J. Intell. Mater. Syst. Struct. 2018, 29, 3634–3647. [Google Scholar] [CrossRef]
- Kalra, S.; Bhattacharya, B.; Munjal, B.S. Design of shape memory alloy actuated intelligent parabolic antenna for space applications. Smart Mater. Struct. 2017, 26, 095015. [Google Scholar] [CrossRef]
- Tanaka, T. A thermomechanical sketch of shape memory effect: One dimensional tensile behavior. Res. Mech. 1986, 18, 251–263. [Google Scholar]
- Liang, C.; Rogers, C.A. One-dimensional thermomechanical constitutive relations for shape memory materials. J. Intell. Mater. Syst. Struct. 1990, 1, 207–234. [Google Scholar] [CrossRef]
- Brinson, L.C. One-Dimensional constitutive behavior of shape memory alloys: Thermomechanical derivation with non-constant material functions and redefined martensite internal variable. J. Intell. Mater. Syst. Struct. 1993, 4, 229–242. [Google Scholar] [CrossRef]
- Boyd, J.G.; Lagoudas, D.C. Thermomechanical response of shape memory composites. J. Intell. Mater. Syst. Struct. 1994, 5, 333–346. [Google Scholar] [CrossRef]
- Boyd, J.G.; Lagoudas, D.C. A thermodynamical constitutive model for shape memory materials. Part I. The monolithic shape memory alloy. Int. J. Plast. 1996, 12, 805–842. [Google Scholar] [CrossRef]
- Boyd, J.G.; Lagoudas, D.C. A thermodynamical constitutive model for shape memory materials. Part II. The SMA composite material. Int. J. Plast. 1996, 12, 843–873. [Google Scholar] [CrossRef]
- Webb, G.V.; Lagoudas, D.C.; Kurdila, A.J. Hysteresis modeling of SMA actuators for control applications. J. Intell. Mater. Syst. Struct. 1998, 9, 432–448. [Google Scholar] [CrossRef]
- Lagoudas, D.C.; Shu, S.G. Residual deformation of active structures with SMA actuators. Int. J. Mech. Sci. 1999, 41, 595–619. [Google Scholar] [CrossRef]
- Hartl, D.J.; Lagoudas, D.C.; Calkins, F.T. Advanced methods for the analysis, design, and optimization of SMA-based aerostructures. Smart Mater. Struct. 2011, 20, 1–6. [Google Scholar] [CrossRef]
- Phillips, F.R.; Wheeler, R.W.; Geltmacher, A.B.; Lagoudas, D.C. Evolution of internal damage during actuation fatigue in shape memory alloys. Int. J. Fatigue 2019, 124, 315–327. [Google Scholar] [CrossRef]
- Xu, L.; Baxevanis, T.; Lagoudas, D.C. A three-dimensional constitutive model for the martensitic transformation in polycrystalline shape memory alloys under large deformation. Smart Mater. Struct. 2019, 28, 074004. [Google Scholar] [CrossRef] [Green Version]
- Auricchio, F.; Conti, M.; Morganti, S. Shape memory alloy: From constitutive modeling to finite element analysis of stent deployment. Comput. Model. Eng. Sci. 2010, 57, 225–244. [Google Scholar]
- Auricchio, F.; Coda, A.; Reali, A. SMA numerical modeling versus experimental results: Parameter identification and model prediction capabilities. J. Mater. Eng. Perform. 2009, 18, 649–654. [Google Scholar] [CrossRef]
- Scalet, G.; Niccoli, F.; Garion, C.; Chiggiato, P.; Maletta, C.; Auricchio, F. A three-dimensional phenomenological model for shape memory alloys including two-way shape memory effect and plasticity. Mech. Mater. 2019, 136, 103085. [Google Scholar] [CrossRef]
- Peigney, M.; Scalet, G.; Auricchio, F. A time integration algorithm for a 3D constitutive model for SMAs including permanent inelasticity and degradation effects. Int. J. Numer. Methods Eng. 2018, 115, 1053–1082. [Google Scholar] [CrossRef]
- Brinson, L.C.; Huang, M.S. Simplifications and comparisons of shape memory alloy constitutive models. J. Intell. Mater. Syst. Struct. 1996, 7, 108–114. [Google Scholar] [CrossRef]
- Bekker, A.; Brinson, L.C. Phase diagram based description of the hysteresis behavior of shape memory alloys. Acta Mater. 1998, 46, 3649–3665. [Google Scholar] [CrossRef]
- Schek, H.J. The force density method for form finding and computation of general networks. Comput. Methods Appl. Mech. Eng. 1974, 3, 115–134. [Google Scholar] [CrossRef]

| - |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jiang, X.; Pan, F.; Fan, Y.; Du, J.; Zhu, M.; Chen, Z. Active Adjustment of Surface Accuracy for a Large Cable-Net Structure by Shape Memory Alloy. Materials 2019, 12, 2619. https://doi.org/10.3390/ma12162619
Jiang X, Pan F, Fan Y, Du J, Zhu M, Chen Z. Active Adjustment of Surface Accuracy for a Large Cable-Net Structure by Shape Memory Alloy. Materials. 2019; 12(16):2619. https://doi.org/10.3390/ma12162619
Chicago/Turabian StyleJiang, Xiangjun, Fengqun Pan, Yesen Fan, Jingli Du, Mingbo Zhu, and Zhen Chen. 2019. "Active Adjustment of Surface Accuracy for a Large Cable-Net Structure by Shape Memory Alloy" Materials 12, no. 16: 2619. https://doi.org/10.3390/ma12162619
APA StyleJiang, X., Pan, F., Fan, Y., Du, J., Zhu, M., & Chen, Z. (2019). Active Adjustment of Surface Accuracy for a Large Cable-Net Structure by Shape Memory Alloy. Materials, 12(16), 2619. https://doi.org/10.3390/ma12162619







