Modelling Cyclic Behaviour of Martensitic Steel with J2 Plasticity and Crystal Plasticity
Abstract
1. Introduction
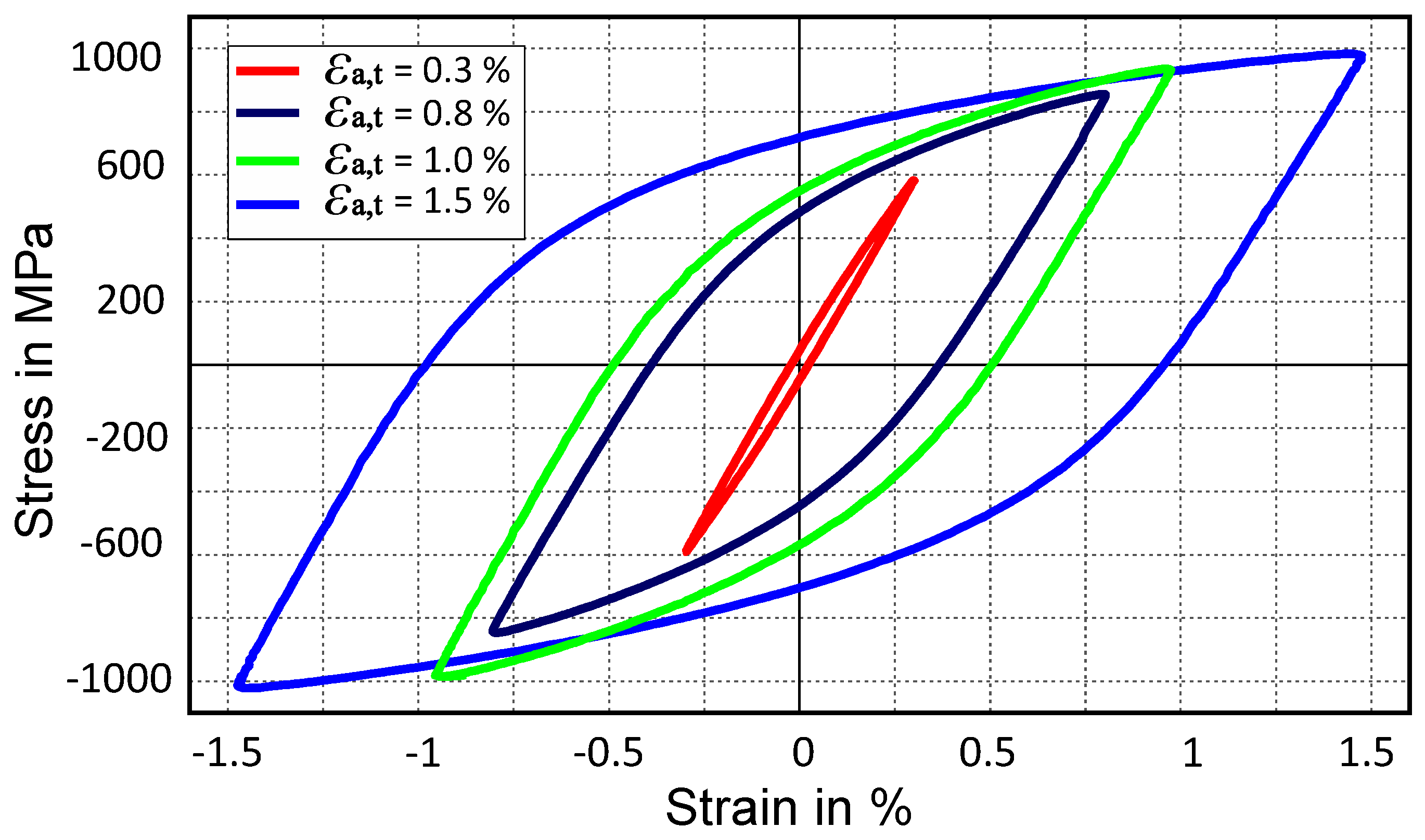
2. Materials and Experiments
3. Modelling Methodology and Constitutive Model Optimization
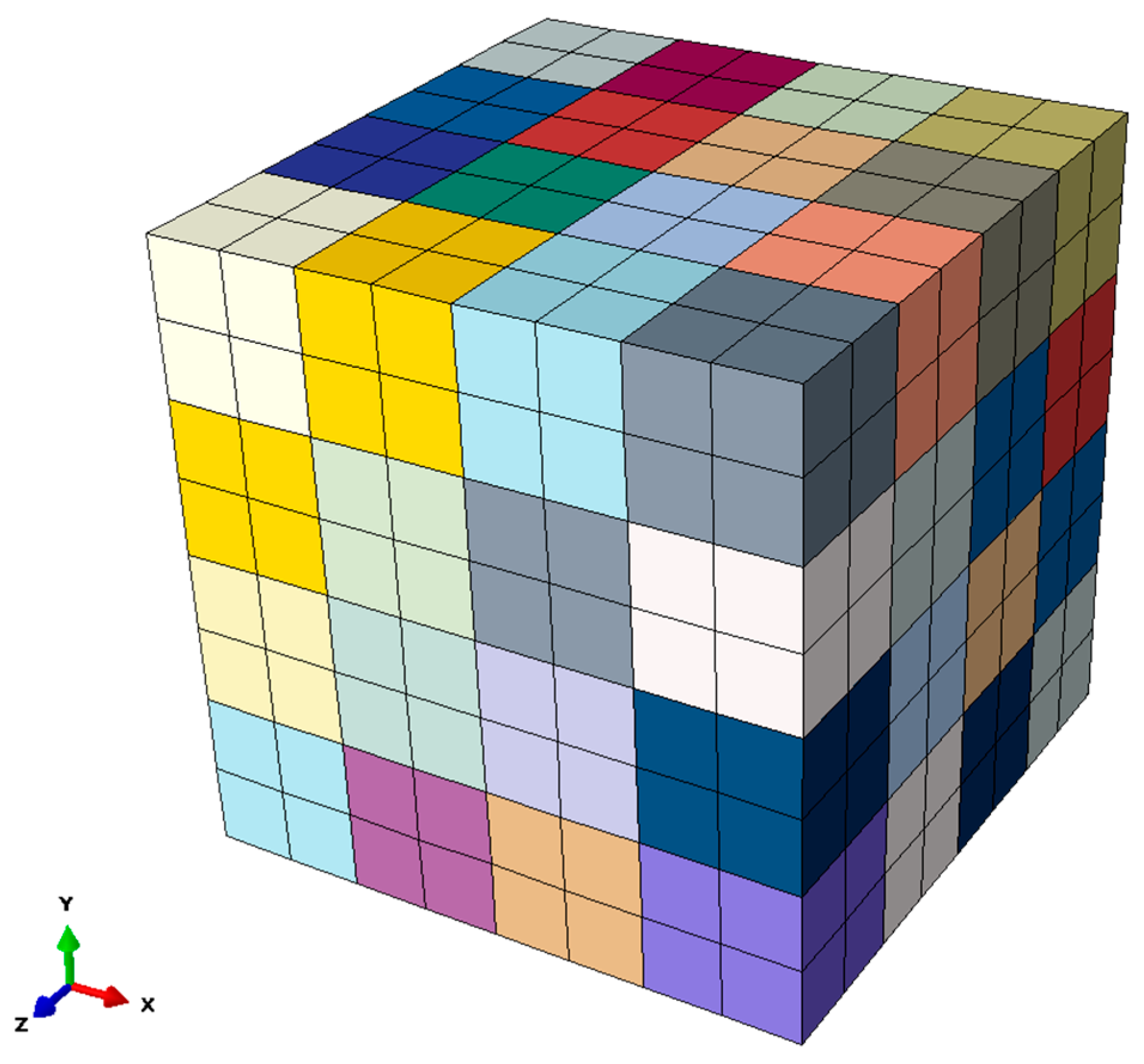
3.1. Numerical Model
3.2. Material Model
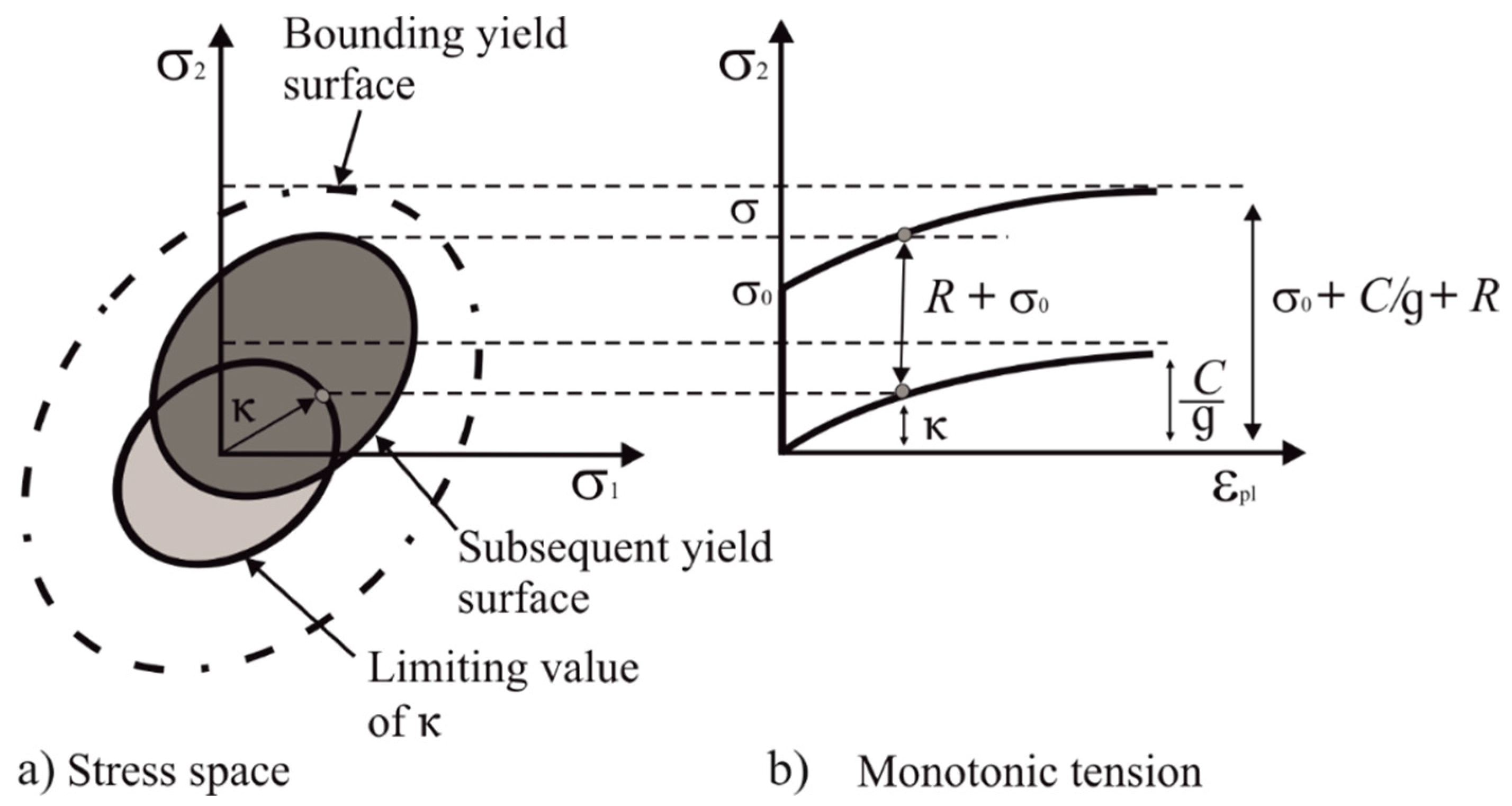
3.2.1. J2/Von Mises plasticity
3.2.2. Crystal Plasticity (CP)
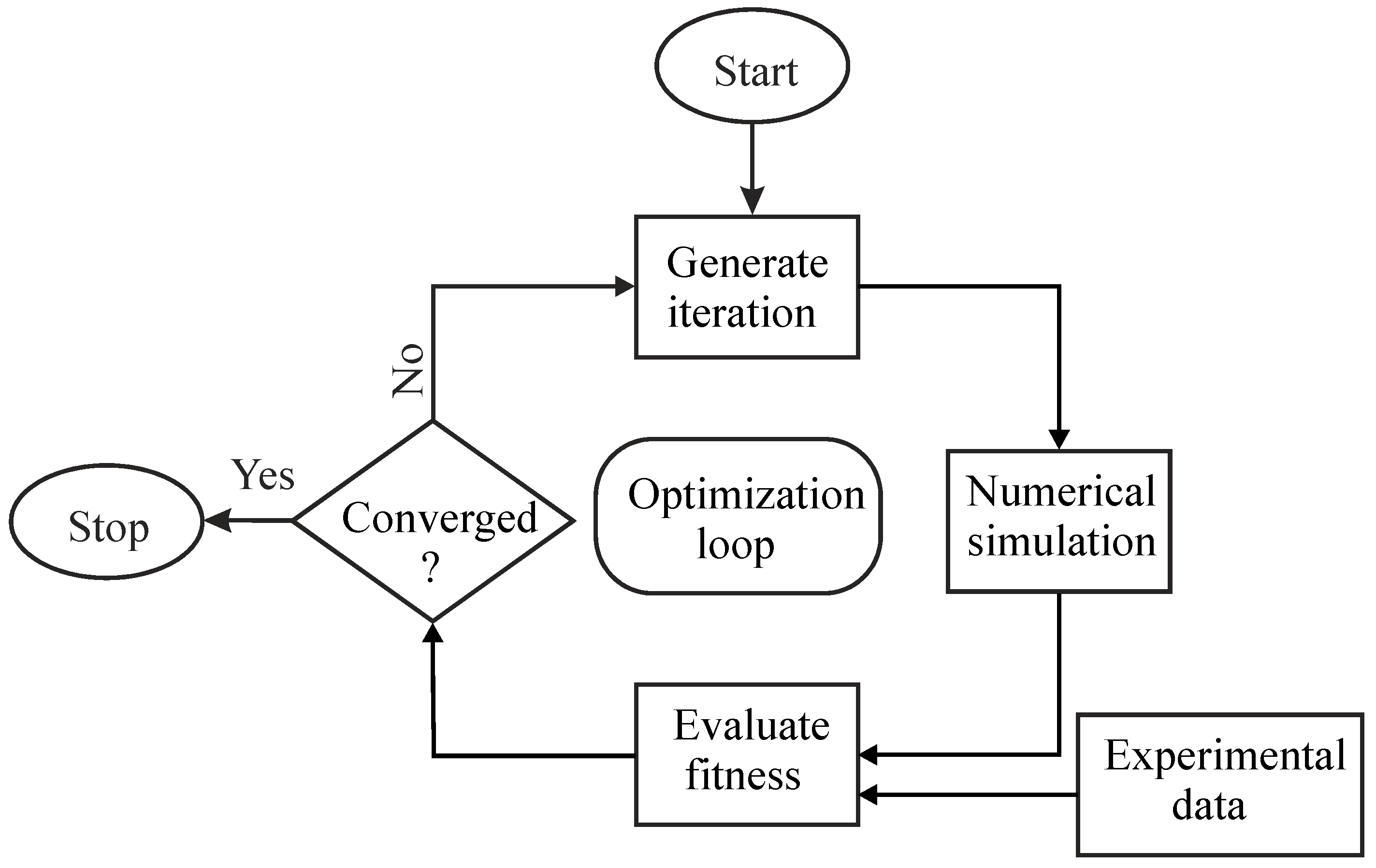
3.3. Optimization Algorithm
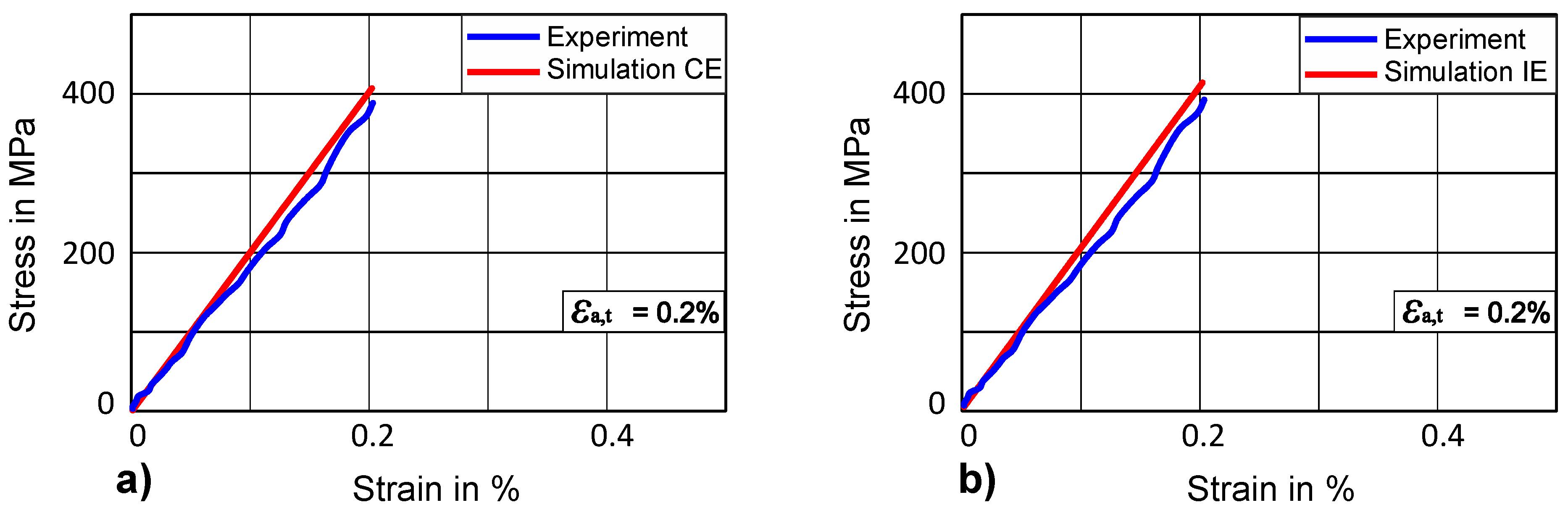
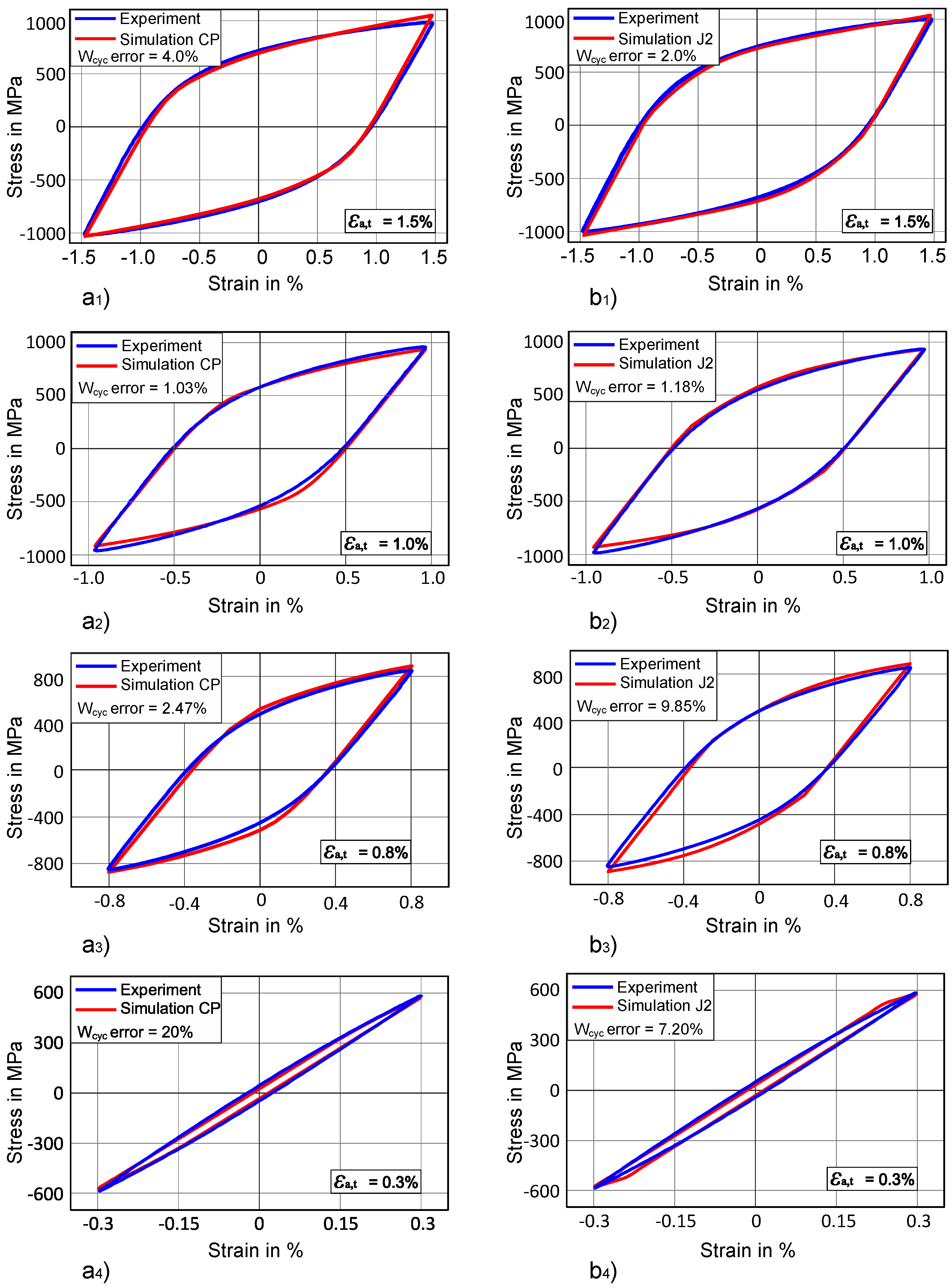
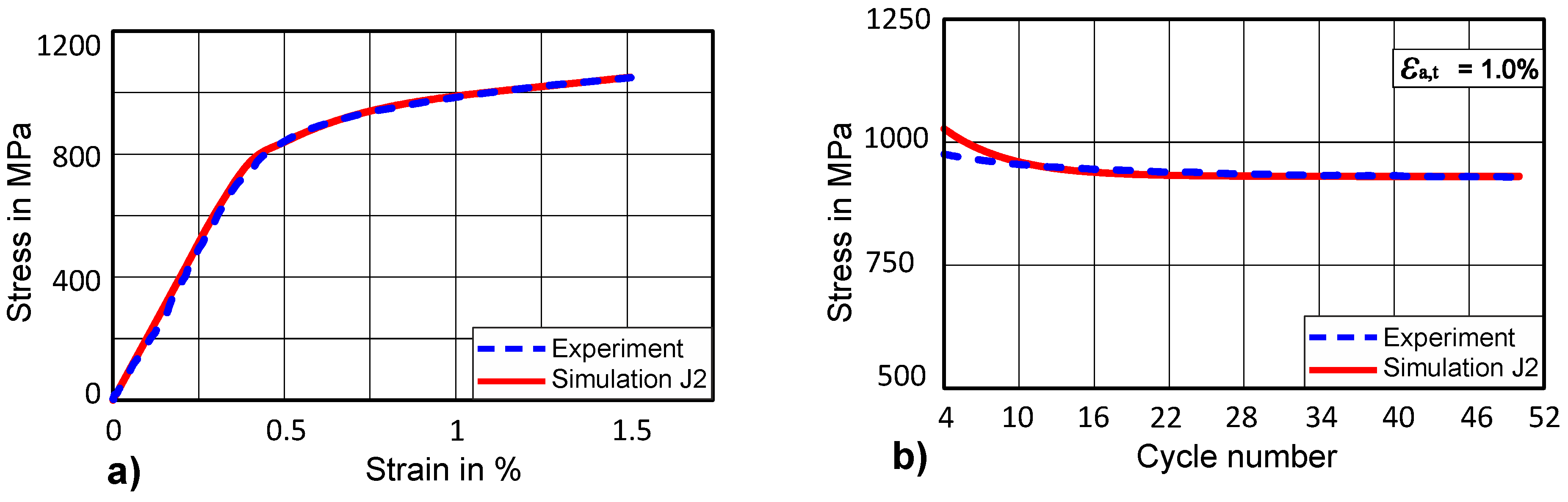
4. Results and Discussion
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
Nomenclature
| LCF | Low cycle fatigue |
| 2D | Two-dimensional |
| 3D | Three-dimensional |
| RVE | Representative volume element |
| S | Deviatoric stress |
| Backstress | |
| Initial yield stress | |
| R | Isotropic hardening |
| b | Rate of isotropic hardening |
| Q | Maximum variation in the size of yield surface |
| Equivalent plastic strain | |
| Total-strain amplitude | |
| Ci | Kinematic hardening moduli for J2 |
| i | Rate of kinematic hardening moduli for J2 |
| N | Number of backstress terms for J2 |
| Ai | Kinematic hardening moduli for CP |
| Bi | Rate of kinematic hardening moduli for CP |
| NB | Number of backstress terms for CP |
| Plastic strain | |
| CP | Crystal plasticity |
| Resolved shear stress | |
| CRSS () | Critical resolved shear stress |
| UMAT | User material subroutine |
| m | Rate sensitivity of the slip |
| Reference shear rate | |
| DP | Dual phase |
| Shear rate with respect to slip system | |
| Resolved backstress on the glide system | |
| , | Material parameters |
| () | Evolution of CRSS |
| , | Slip hardening parameters |
| Saturated CRSS | |
| Hardening matrix | |
| FEM | Finite element method |
| C11, C12, C44 | Elastic constants |
| Wcyc | Dissipated strain energy |
| E | Young’s modulus |
| HV | Vickers’ hardness |
| CPE4 | Four-node plane strain element |
References
- Reed, R.P. Nitrogen in austenitic stainless steels. JOM 1989, 41, 16–21. [Google Scholar] [CrossRef]
- Berns, H. Manufacture and Application of High Nitrogen Steels. ISIJ Int. 1996, 36, 909–914. [Google Scholar] [CrossRef]
- Chang, B.; Zhang, Z. Low cycle fatigue behavior of a high nitrogen austenitic stainless steel under uniaxial and non-proportional loadings based on the partition of hysteresis loops. Mater. Sci. Eng. A 2012, 547, 72–79. [Google Scholar] [CrossRef]
- Simmons, J.W. Overview: High-nitrogen alloying of stainless steels. Mater. Sci. Eng. A 1996, 207, 159–169. [Google Scholar] [CrossRef]
- Schymura, M.; Fischer, A. Metallurgical aspects on the fatigue of solution-annealed austenitic high interstitial steels. Int. J. Fatigue 2013, 61, 1–9. [Google Scholar] [CrossRef]
- Güler, S.; Schymura, M.; Fischer, A.; Droste, M.; Biermann, H. The influence of the nitrogen/nickel-ratio on the cyclic behavior of austenitic high strength steels with twinning-induced plasticity and transformation-induced plasticity effects. Materwiss. Werkst. 2018, 49, 61–72. [Google Scholar] [CrossRef]
- Degallaix, S.; Armas, A.F.; Marinelli, M.C.; Heren, S. On the cyclic softening behavior of SAF 2507 duplex stainless steel. Acta Mater. 2006, 54, 5041–5049. [Google Scholar]
- Vogt, J. Fatigue properties of high nitrogen steels. J. Mater. Process. Tech. 2001, 117, 364–369. [Google Scholar] [CrossRef]
- Pola, J. Analysis of the hysteresis loop in stainless steels II. Austenitic—ferritic duplex steel and the effect of nitrogen. Mater. Sci. Eng. A 2001, 297, 154–161. [Google Scholar] [CrossRef]
- Bru, A. Numerical simulation of micro-crack initiation of martensitic steel under fatigue loading. Int. J. Fatigue 2006, 28, 963–971. [Google Scholar]
- Gu, C.; Lian, J.; Bao, Y.; Xiao, W.; Münstermann, S. Numerical Study of the Effect of Inclusions on the Residual Stress Distribution in High-Strength Martensitic Steels During Cooling. Appl. Sci. 2019, 9, 455. [Google Scholar] [CrossRef]
- Roters, F.; Eisenlohr, P.; Hantcherli, L.; Tjahjanto, D.D.; Bieler, T.R.; Raabe, D. Overview of constitutive laws, kinematics, homogenization and multiscale methods in crystal plasticity finite-element modeling: Theory, experiments, applications. Acta Mater. 2010, 58, 1152–1211. [Google Scholar] [CrossRef]
- Bažant, Z.P. Mechanics of Solid Materials; Cambridge University Press: Cambridge, UK, 1992; Volume 19. [Google Scholar]
- Ziegler, H. A modification of Prager’s hardening rule. Quart. Appl. Math. 1959, 17, 55–65. [Google Scholar] [CrossRef]
- Bauschinger, J. Begründer der Mechanisch-Technischen Versuchsanstalten. Available online: https://www.hausarbeiten.de/document/132971 (accessed on 23 May 2019).
- Mróz, Z. On the description of anisotropic workhardening. J. Mech. Phys. Solids 1967, 15, 163–175. [Google Scholar] [CrossRef]
- Iwan, W.D. On a Class of Models for the Yielding Behavior of Continuous and Composite Systems. J. Appl. Mech. 1967, 34, 612. [Google Scholar] [CrossRef]
- Dafalias, Y.F.; Popov, E.P. Ein Modell für Werkstoffe mit nichtlinearer Verfestigung unter zusammengesetzter Belastung. Acta Mech. 1975, 21, 173–192. [Google Scholar] [CrossRef]
- Dafalias, Y.F.; Popov, E.P. Plastic Internal Variables Formalism of Cyclic Plasticity. J. Appl. Mech. 1976, 43, 645. [Google Scholar] [CrossRef]
- Krieg, R.D. A Practical Two Surface Plasticity Theory. J. Appl. Mech. 1975, 42, 641–646. [Google Scholar] [CrossRef]
- Rezaiee-Pajand, M.; Sinaie, S. On the calibration of the Chaboche hardening model and a modified hardening rule for uniaxial ratcheting prediction. Int. J. Solids Struct. 2009, 46, 3009–3017. [Google Scholar] [CrossRef]
- Armstrong, P.J.; Frederick, C.O. A Mathematical Representation of the Multiaxial Bauschinger Effect; Central Electricity Generating Board: London, UK, 1966; Volume 731. [Google Scholar]
- Chaboche, J.L.; Dang Van, K.; Cordier, G. Modelization of the Strain Memory Effect on the Cyclic Hardening of 316 Stainless Steel; North-Holland Publishing Co.: Amsterdam, The Netherlands, 1979. [Google Scholar]
- Chaboche, J.L. Time-independent constitutive theories for cyclic plasticity. Int. J. Plast. 1986, 2, 149–188. [Google Scholar] [CrossRef]
- Ohno, N.; Wang, J.-D. Kinematic hardening rules with critical state of dynamic recovery, part I: Formulation and basic features for ratchetting behavior. Int. J. Plast. 1993, 9, 375–390. [Google Scholar] [CrossRef]
- Mcdowell, D.L. Stress state of cyclic ratchetting behavior of two rail steels introduced modifications of the AF rule to more accurately model ratchetting effects A. Int. J. Plast. 1995, 11, 397–421. [Google Scholar] [CrossRef]
- Ohno, N. Kinematic hardening model suitable for ratchetting with steady-state. Int. J. Plast. 2000, 16, 225–240. [Google Scholar]
- Chaboche, J.L. A review of some plasticity and viscoplasticity constitutive theories. Int. J. Plast. 2008, 24, 1642–1693. [Google Scholar] [CrossRef]
- Liu, S.; Liang, G. Optimization of Chaboche kinematic hardening parameters by using an algebraic method based on integral equations. J. Mech. Mater. Struct. 2017, 12, 439–455. [Google Scholar] [CrossRef]
- Bari, S.; Hassan, T. Anatomy of coupled constitutive models for ratcheting simulation. Int. J. Plast. 2000, 16, 381–409. [Google Scholar] [CrossRef]
- Schäfer, B.; Song, X.; Sonnweber-Ribic, P.; ul Hassan, H.; Hartmaier, A. Micromechanical Modelling of the Cyclic Deformation Behavior of Martensitic SAE 4150—A Comparison of Different Kinematic Hardening Models. Metals 2019, 9, 368. [Google Scholar] [CrossRef]
- Moeini, G.; Ramazani, A.; Sundararaghavan, V.; Koenke, C. Micromechanical modeling of fatigue behavior of DP steels. Mater. Sci. Eng. A 2017, 689, 89–95. [Google Scholar] [CrossRef]
- Moeini, G.; Ramazani, A.; Myslicki, S.; Sundararaghavan, V.; Könke, C. Low Cycle Fatigue Behaviour of DP Steels: Micromechanical Modelling vs. Validation. Metals 2017, 7, 265. [Google Scholar] [CrossRef]
- Boeff, M.; Hassan, H. ul; Hartmaier, A. Micromechanical modeling of fatigue crack initiation in polycrystals. J. Mater. Res. 2017, 32, 4375–4386. [Google Scholar] [CrossRef]
- Velay, V.; Bernhart, G.; Penazzi, L. Cyclic behavior modeling of a tempered martensitic hot work tool steel. Int. J. Plast. 2006, 22, 459–496. [Google Scholar] [CrossRef]
- Ridha Hambli, A.P. Comparison between 2D and 3D numerical modeling of superplastic forming processes Ridha. Comput. Methods Appl. Mech. Eng. 2007, 190, 171–182. [Google Scholar]
- Segurado, J.; Llorca, J. Simulation of the deformation of polycrystalline nanostructured Ti by computational homogenization. Comput. Mater. Sci. 2013, 76, 3–11. [Google Scholar] [CrossRef]
- Kulosa, M.; Neumann, M.; Boeff, M.; Gaiselmann, G.; Schmidt, V.; Hartmaier, A. A Study on Microstructural Parameters for the Characterization of Granular Porous Ceramics Using a Combination of Stochastic and Mechanical Modeling. Int. J. Appl. Mech. 2017, 9, 1750069. [Google Scholar] [CrossRef]
- Boeff, M. Micromechanical Modelling of Fatigue Crack Initiation and Growth. Ph.D. Thesis, Ruhr-Universität, Bochum, Germany, December 2016. [Google Scholar]
- Smit, R.J.M.; Brekelmans, W.A.M.; Meijer, H.E.H. Prediction of the mechanical behavior of nonlinear heterogeneous systems by multi-level finite element modeling. Comput. Methods Appl. Mech. Eng. 1998, 155, 181–192. [Google Scholar] [CrossRef]
- Novak, J.S.; Benasciutti, D.; De Bona, F.; Stanojević, A.; De Luca, A.; Raffaglio, Y. Estimation of material parameters in nonlinear hardening plasticity models and strain life curves for CuAg alloy. IOP Conf. Ser. Mater. Sci. Eng. 2016, 119, 12020. [Google Scholar] [CrossRef]
- Lemaitre, J.; Chaboche, J.-L. Mechanics of Solid Materials; Cambridge University Press: Cambridge, UK, 1990. [Google Scholar]
- Chaboche, J.L. Constitutive equations for cyclic plasticity and cyclic viscoplasticity. Int. J. Plast. 1989, 5, 247–302. [Google Scholar] [CrossRef]
- Frederick, C.O.; Armstrong, P.J. A mathematical representation of the multiaxial Bauschinger effect. Mater. High Temp. 2007, 24, 1–26. [Google Scholar] [CrossRef]
- Roters, F.; Eisenlohr, P.; Bieler, T.R.; Raabe, D. Crystal Plasticity Finite Element Methods: In Materials Science and Engineering; John Wiley & Sons: Hoboken, NJ, USA, 2010. [Google Scholar]
- Sachtleber, M.; Zhao, Z.; Raabe, D. Experimental investigation of plastic grain interaction. Mater. Sci. Eng. A 2002, 336, 81–87. [Google Scholar] [CrossRef]
- Rice, J.R. Inelastic constitutive relations for solids: An internal-variable theory and its application to metal plasticity. J. Mech. Phys. Solids 1971, 19, 433–455. [Google Scholar] [CrossRef]
- Peirce, D.; Asaro, R.J.; Needleman, A. An analysis of nonuniform and localized deformation in ductile single crystals. Acta Metall. 1982, 30, 1087–1119. [Google Scholar] [CrossRef]
- Peirce, D.; Asaro, R.J.; Needleman, A. Material rate dependence and localized deformation in crystalline solids. Acta Metall. 1983, 31, 1951–1976. [Google Scholar] [CrossRef]
- Hutchinson, J. Bounds and self-consistent estimates for creep of polycrystalline materials. Proc. R. Soc. Lond. A Math. Phys. Eng. Sci. 1976, 348, 101–127. [Google Scholar] [CrossRef]
- ALT, H.; GODAU, M. Computing the Fréchet Distance Between Two Polygonal Curves. Int. J. Comput. Geom. Appl. 2004, 5, 75–91. [Google Scholar] [CrossRef]
- Witowski, K. Identification of Material Parameters with LS-OPT. Available online: https://www.dynamore.de/de/download/papers/2014-ls-dyna-forum/documents/workshops/identification-of-material-parameters-with-ls-opt-r (accessed on 23 May 2019).
- Zitzler, E.; Laumanns, M.; Thiele, L. SPEA2: Improving the Strength Pareto Evolutionary Algorithm; TIK-Report; TIK: Zurich, Switzerland, May 2001. [Google Scholar]
- Agius, D.; Kajtaz, M.; Kourousis, K.I.; Wallbrink, C.; Wang, C.H.; Hu, W.; Silva, J. Sensitivity and optimisation of the Chaboche plasticity model parameters in strain-life fatigue predictions. Mater. Des. 2017, 118, 107–121. [Google Scholar] [CrossRef]
- Mahmoudi, A.H.; Pezeshki-Najafabadi, S.M.; Badnava, H. Parameter determination of Chaboche kinematic hardening model using a multi objective Genetic Algorithm. Comput. Mater. Sci. 2011, 50, 1114–1122. [Google Scholar] [CrossRef]
- Kim, S.A.; Johnson, W.L. Elastic constants and internal friction of martensitic steel, ferritic-pearlitic steel, and α-iron. Mater. Sci. Eng. A 2007, 452–453, 633–639. [Google Scholar] [CrossRef]
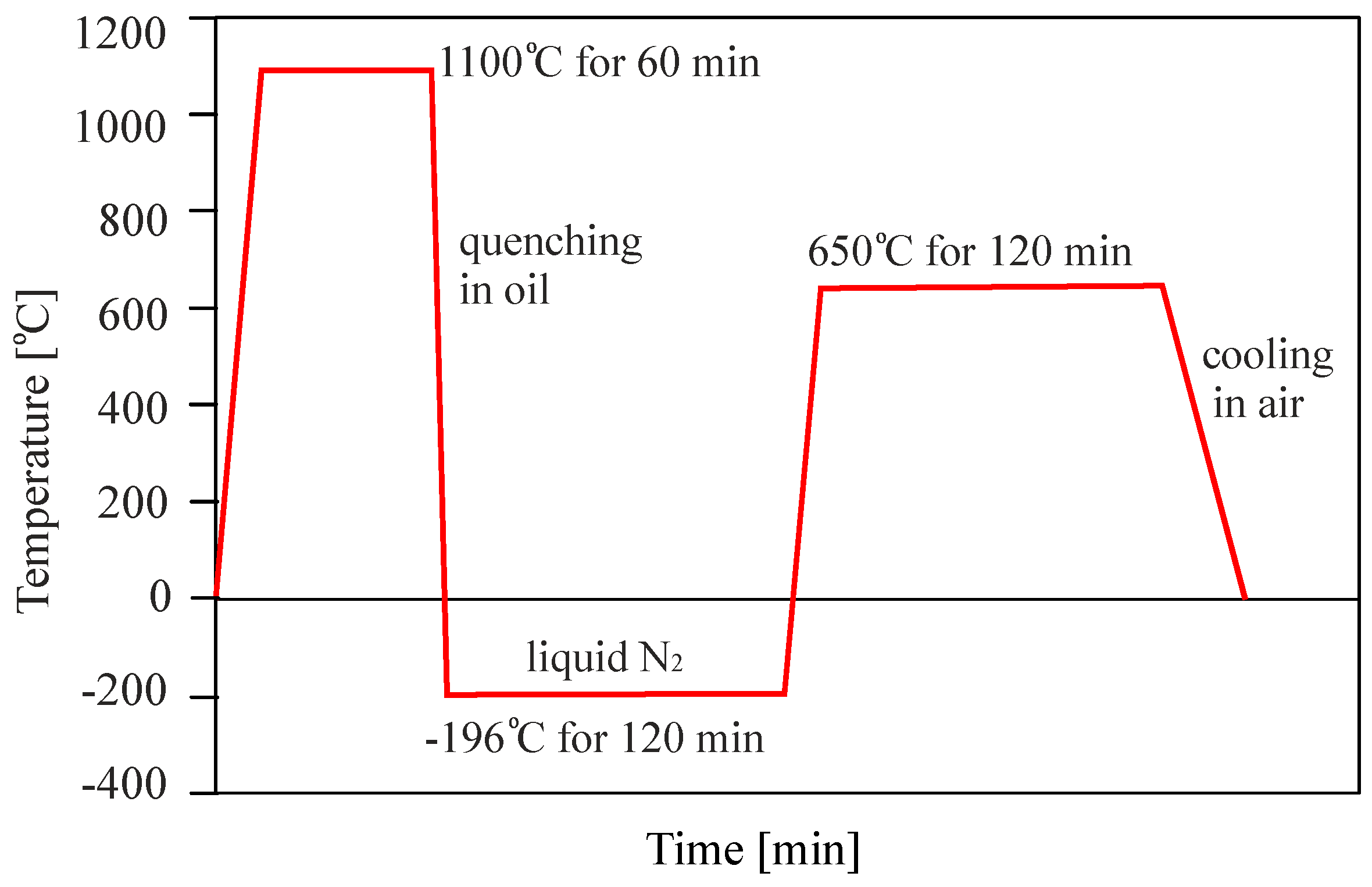
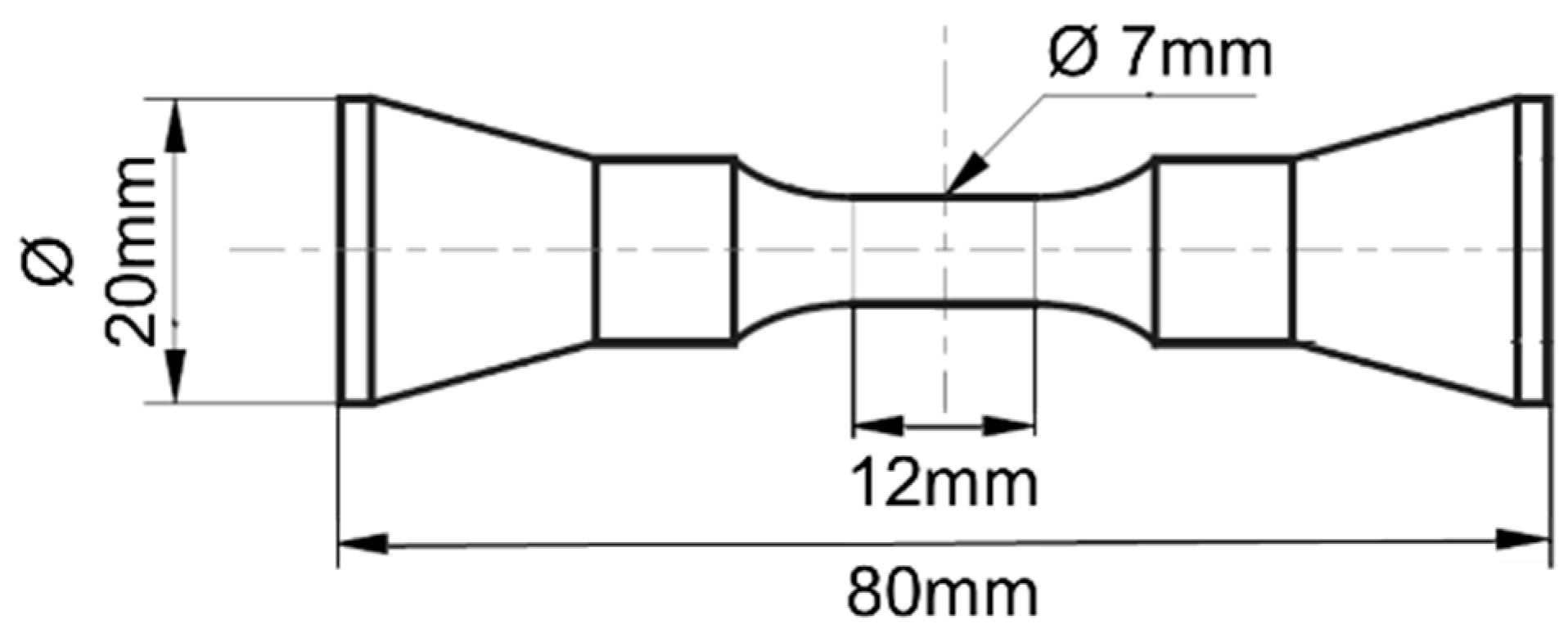
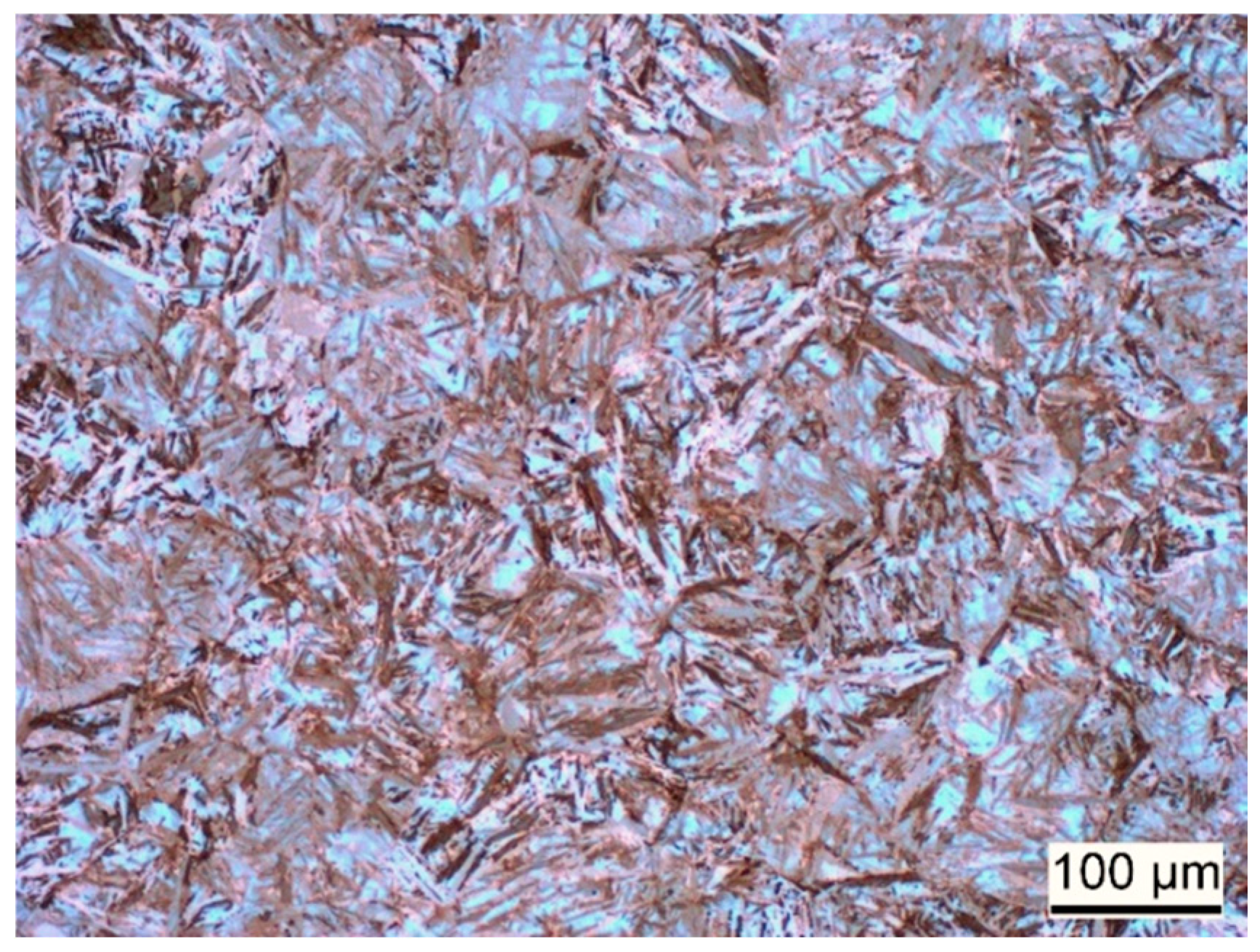
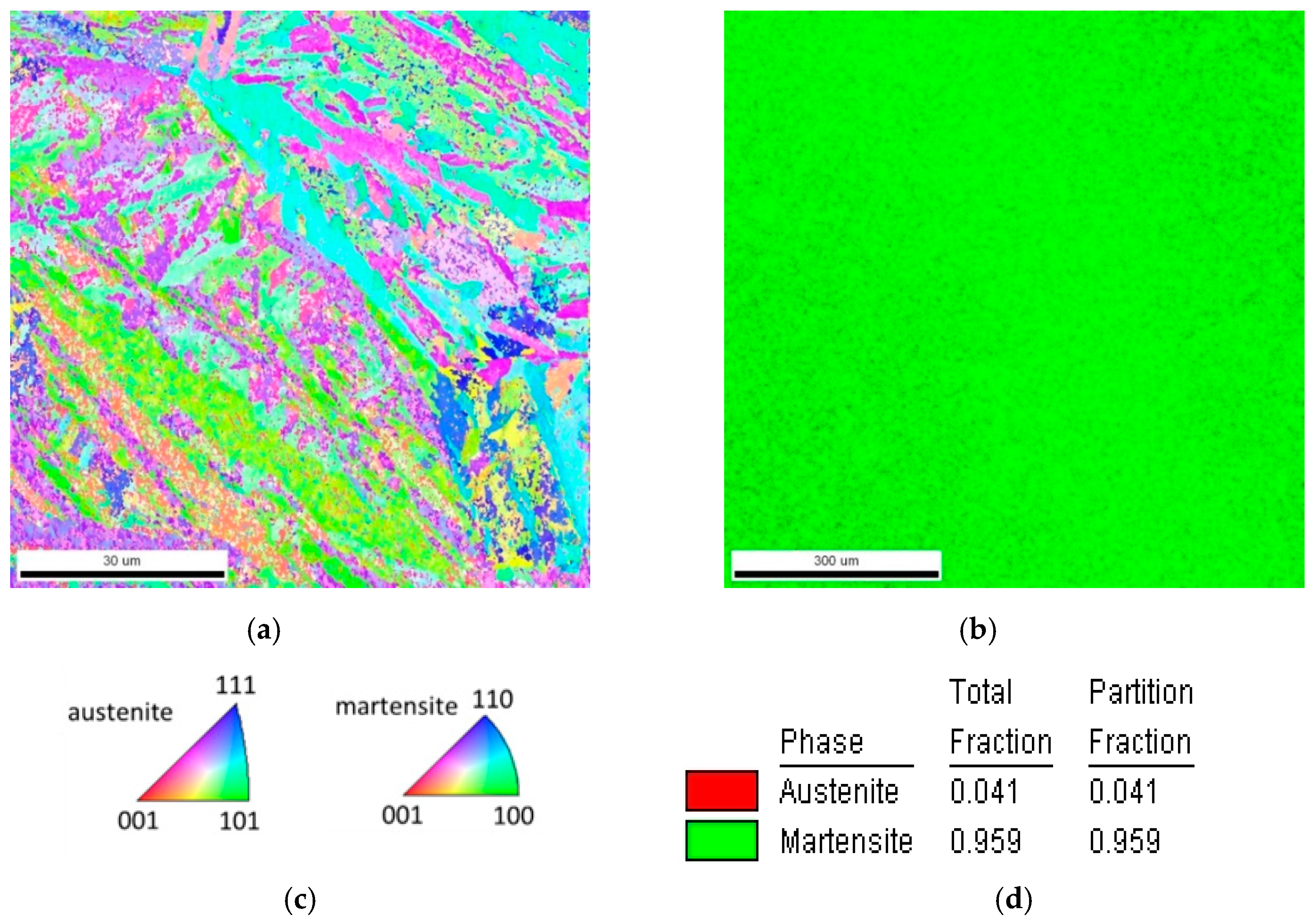

| Element | Cr | Mo | Si | Mn | N | C | Ni | P | Al | V | Ti | Cu | S |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| wt.% | 15.3 | 1.0 | 0.7 | 0.4 | 0.4 | 0.3 | 0.2 | 0.02 | 0.01 | 0.03 | 0.003 | 0.05 | 0.001 |
| Elastic Constants | Kinematic Hardening Parameters | Isotropic Hardening Parameters | |||
|---|---|---|---|---|---|
| C11 (GPa) | 263.001 | A1 (MPa) | 9598 | 0 (s−1) | 0.001 |
| C12 (GPa) | 111.693 | A2 (MPa) | 287609 | h0 (MPa) | 3800 |
| C44 (GPa) | 78.939 | B1 | 3128 | τc,0 (MPa) | 260.301 |
| B2 | 0 | τc,s (MPa) | 204.172 | ||
| n | 1.998 | ||||
| Elastic Constants | Kinematic Hardening Parameters | Isotropic Hardening Parameters | |||
|---|---|---|---|---|---|
| E (GPa) | 206 | C1 (MPa) | 130764 | (MPa) | 754.2 |
| v | 0.3 | C2 (MPa) | 21892 | Q (MPa) | −199.9 |
| 1 | 478 | b | 10.4 | ||
| 2 | 0 | ||||
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sajjad, H.M.; Hanke, S.; Güler, S.; ul Hassan, H.; Fischer, A.; Hartmaier, A. Modelling Cyclic Behaviour of Martensitic Steel with J2 Plasticity and Crystal Plasticity. Materials 2019, 12, 1767. https://doi.org/10.3390/ma12111767
Sajjad HM, Hanke S, Güler S, ul Hassan H, Fischer A, Hartmaier A. Modelling Cyclic Behaviour of Martensitic Steel with J2 Plasticity and Crystal Plasticity. Materials. 2019; 12(11):1767. https://doi.org/10.3390/ma12111767
Chicago/Turabian StyleSajjad, Hafiz Muhammad, Stefanie Hanke, Sedat Güler, Hamad ul Hassan, Alfons Fischer, and Alexander Hartmaier. 2019. "Modelling Cyclic Behaviour of Martensitic Steel with J2 Plasticity and Crystal Plasticity" Materials 12, no. 11: 1767. https://doi.org/10.3390/ma12111767
APA StyleSajjad, H. M., Hanke, S., Güler, S., ul Hassan, H., Fischer, A., & Hartmaier, A. (2019). Modelling Cyclic Behaviour of Martensitic Steel with J2 Plasticity and Crystal Plasticity. Materials, 12(11), 1767. https://doi.org/10.3390/ma12111767







