Electrical Conductivity and Optical Properties of Pulsed Laser Deposited LaNi5 Nanoscale Films
Abstract
1. Introduction
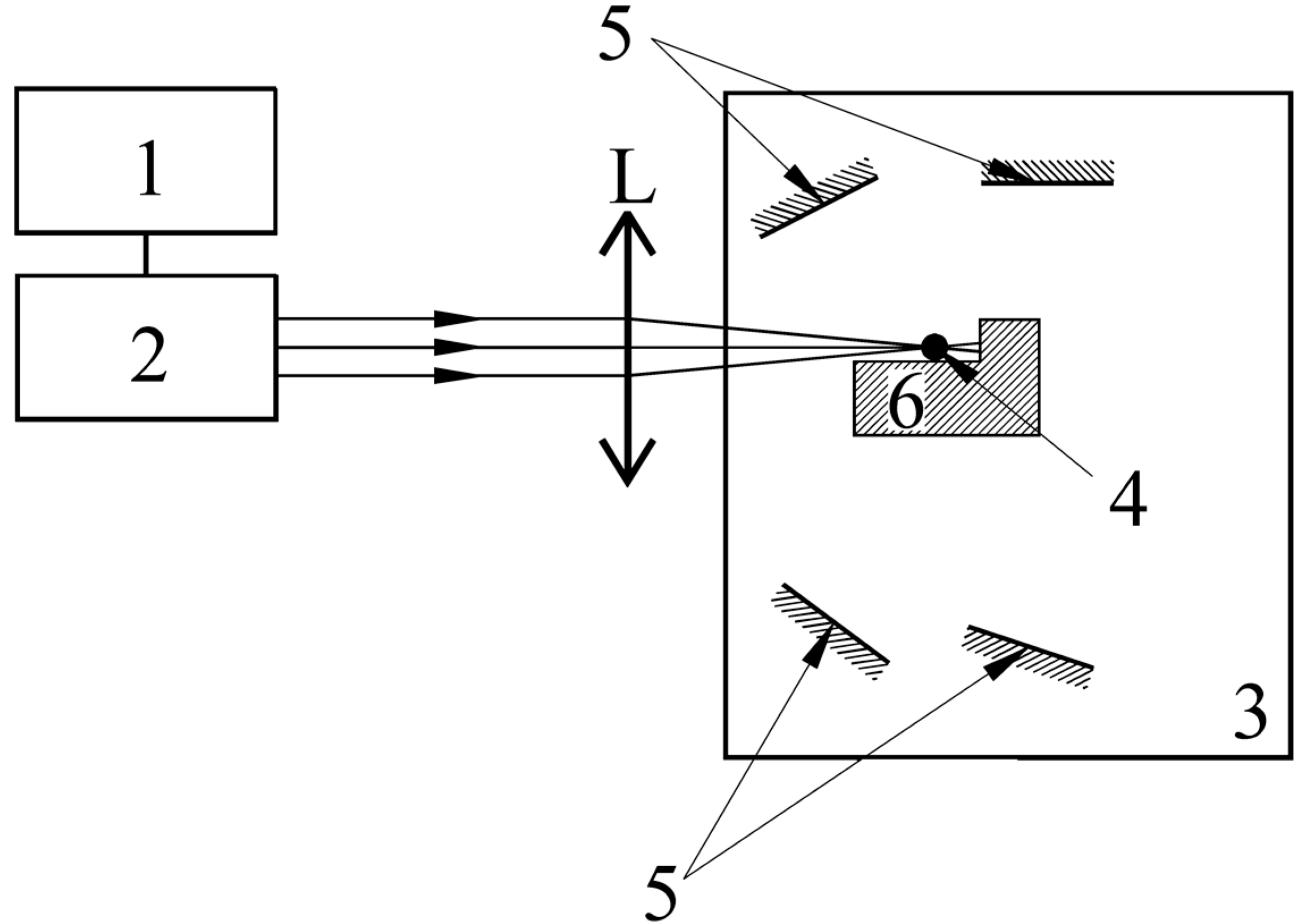
2. Materials and Methods
3. Results and Discussions
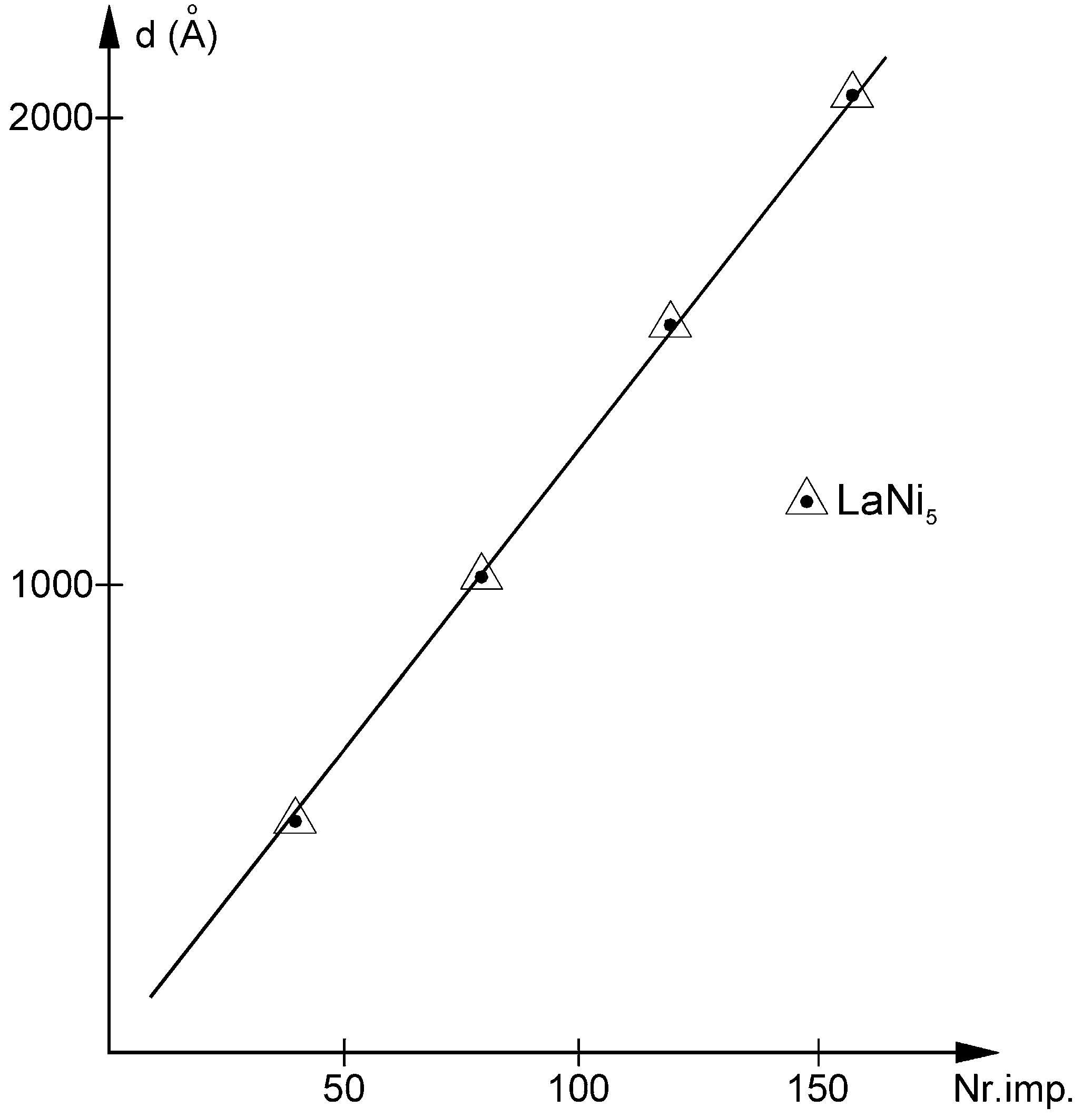
3.1. Film Thickness and Phase Composition
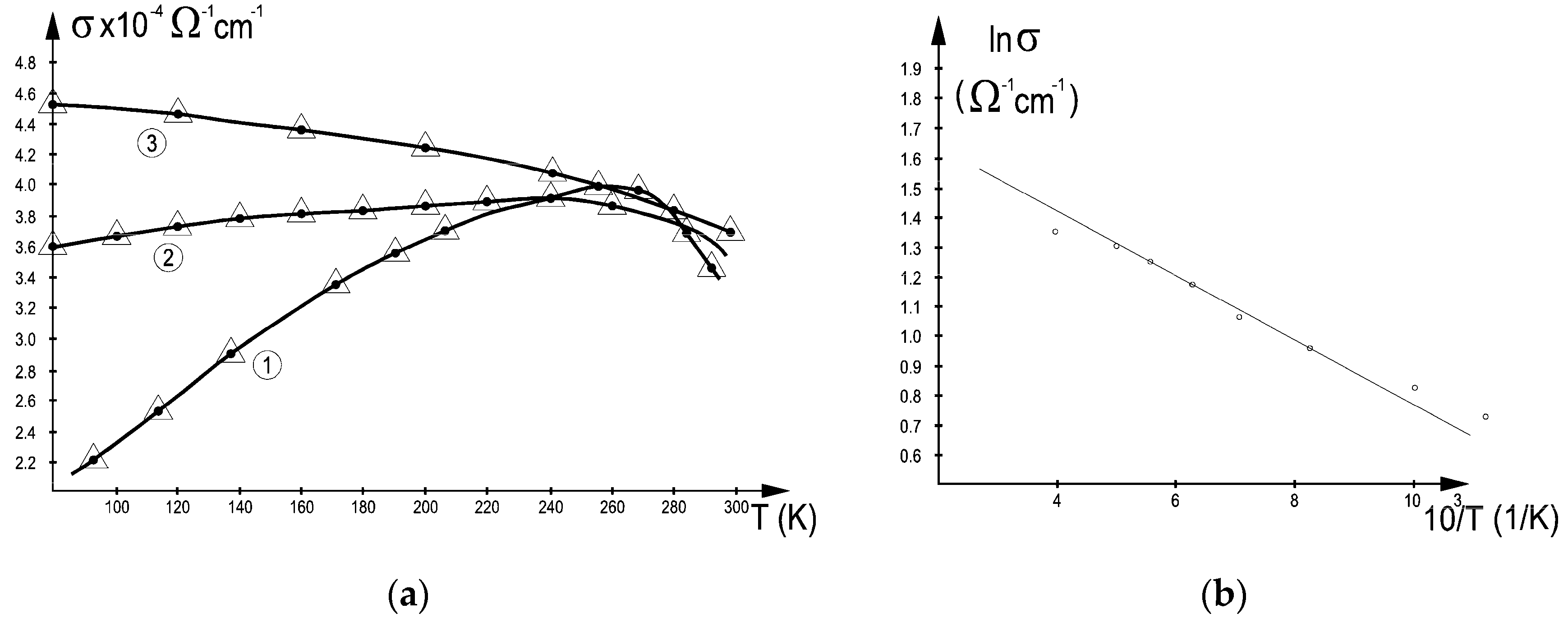
3.2. Electrical Conductivity
3.3. Optical Reflectance and Transmittance
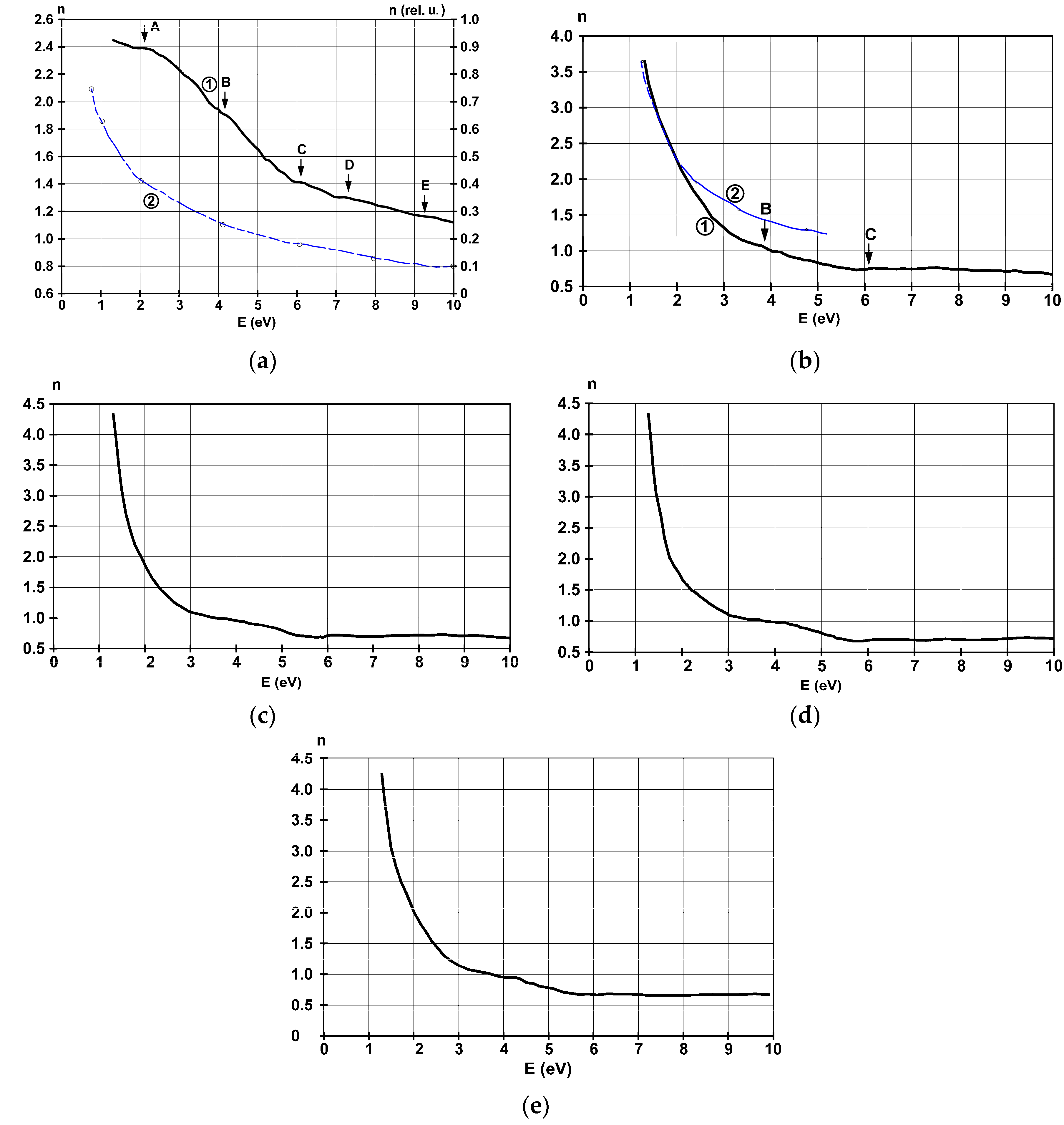
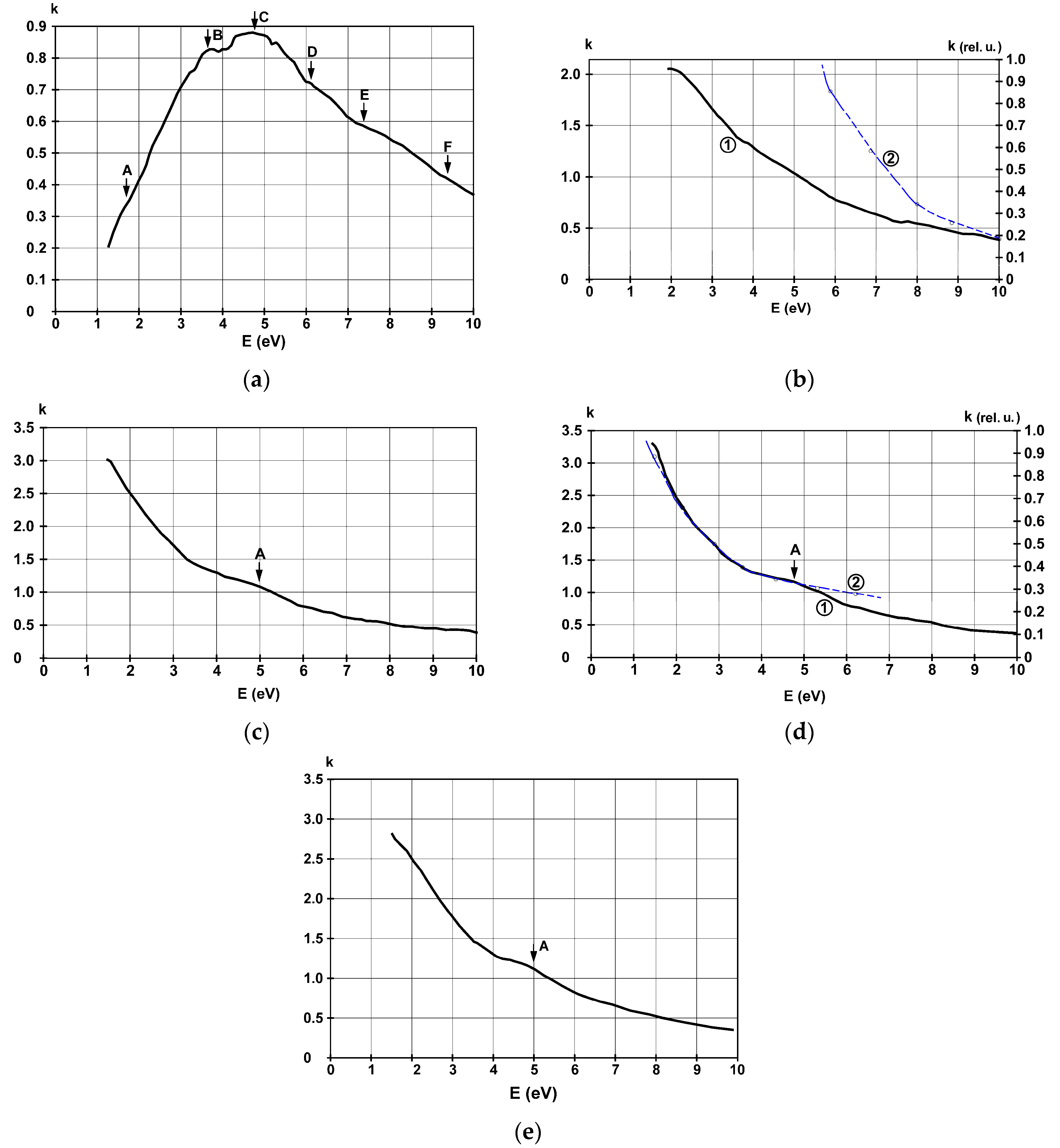
3.4. Refractive Index and Extinction Coefficient
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Xiao, P.; Huang, J.; Dong, T.; Xie, J.; Yuan, J.; Luo, D.; Liu, B. Room-Temperature Fabricated Thin-Film Transistors Based on Compounds with Lanthanum and Main Family Element Boron. Molecules 2018, 23, 1373. [Google Scholar] [CrossRef] [PubMed]
- Mattox, T.M.; Coffman, D.K.; Roh, I.; Sims, C.; Urban, J.J. Moving the Plasmon of LaB6 from IR to Near-IR via Eu-Doping. Materials 2018, 11, 226. [Google Scholar] [CrossRef] [PubMed]
- Jiang, S.Z.; Guo, Z.N.; Liu, G.X.; Gyimah, G.K.; Li, X.Y.; Dong, H.S. A Rapid One-Step Process for Fabrication of Biomimetic Superhydrophobic Surfaces by Pulse Electrodeposition. Materials 2017, 10, 1229. [Google Scholar] [CrossRef] [PubMed]
- Xu, P.F.; Wu, L.; Dong, L.Q.; Xu, X. Chemoselective Polymerization of Polar Divinyl Monomers with Rare-Earth/Phosphine Lewis Pairs. Molecules 2018, 23, 360. [Google Scholar] [CrossRef] [PubMed]
- Tekumalla, S.; Seetharaman, S.; Almajid, A.; Gupta, M. Mechanical Properties of Magnesium-Rare Earth Alloy Systems: A Review. Metals 2015, 5, 1–39. [Google Scholar] [CrossRef]
- Pan, F.; Zhang, J.; Chen, H.L.; Su, Y.H.; Kuo, C.L.; Su, Y.H.; Chen, S.H.; Lin, K.J.; Hsieh, P.H.; Hwang, W.S. Effects of Rare Earth Metals on Steel Microstructures. Materials 2016, 9, 417. [Google Scholar] [CrossRef] [PubMed]
- Malik, S.K.; Arlinghaus, F.J.; Wallace, W.E. Calculation of the spin-polarized energy-band structure of LaNi5 and GdNi5. Phys. Rev. B 1982, 25, 6488–6491. [Google Scholar] [CrossRef]
- Ito, T.; Ido, H. Electronic structures and magnetic properties of LaCo5, LaNi5, and LaCo3Ni2. J. Appl. Phys. 2005, 97, 10A313. [Google Scholar] [CrossRef]
- Grechnev, G.E.; Logosha, A.V.; Panfilov, A.S.; Kuchin, A.G.; Vasijev, A.N. Effect of pressure on the magnetic properties of YNi5, LaNi5, and CeNi5. Low Temp. Phys. 2011, 37, 138–143. [Google Scholar] [CrossRef]
- Escorne, M.; Lamloumi, J.; Percheron-Guegan, A.; Achard, J.C.; Mauger, A. Spin freezing properties in LaNi5−xFex. J. Magn. Magn. Mater. 1987, 65, 63–70. [Google Scholar] [CrossRef]
- Burzo, E.; Chioncel, L.; Costina, I.; Chiuzbaian, S.G. Electronic structure and magnetic properties of GdxLa1−xNi5 system. J. Phys. Condens. Matter 2006, 18, 4861–4877. [Google Scholar] [CrossRef]
- Schlapbach, L.; Zuttel, A. Hydrogen-storage materials for mobile applications. Nature 2001, 414, 353–358. [Google Scholar] [CrossRef] [PubMed]
- Yan, S.; Kwo-Hsiung Young, K.H.; Zhao, X.; Mei, Z.; Simon Ng, K.Y. Electron Backscatter Diffraction Studies on the Formation of Superlattice Metal Hydride Alloys. Batteries 2017, 3, 40. [Google Scholar] [CrossRef]
- Qiu, S.J.; Huang, J.L.; Chu, H.L.; Zou, Y.J.; Xiang, C.L.; Zhang, H.Z.; Xu, F.; Sun, L.X.; Ouyang, L.Z.; Zhou, H.Y. Influence of Zr Addition on Structure and Performance of Rare Earth Mg-Based Alloys as Anodes in Ni/MH Battery. Metals 2015, 5, 565–577. [Google Scholar] [CrossRef]
- Denys, R.V.; Yartys, V.A.; Gray, E.M.; Webb, C.J. LaNi5-Assisted Hydrogenation of MgNi2 in the Hybrid Structures of La1.09Mg1.91Ni9D9.5 and La0.91Mg2.09Ni9D9.4. Energies 2015, 8, 3198–3211. [Google Scholar] [CrossRef]
- Pinatel, E.R.; Palumbo, M.; Massimino, F.; Rizzi, P.; Baricco, M. Hydrogen sorption in the LaNi5−xAlx-H system (0 ≤ x ≤ 1). Intermetallics 2015, 62, 7–16. [Google Scholar] [CrossRef]
- Kukkapalli, V.K.; Kim, S. Optimization of Internal Cooling Fins for Metal Hydride Reactors. Energies 2016, 9, 447. [Google Scholar] [CrossRef]
- Shafiee, S.; Mccay, M.H.; Kuravi, S. The Effect of Magnetic Field on Thermal-Reaction Kinetics of a Paramagnetic Metal Hydride Storage Bed. Appl. Sci. 2017, 7, 1006. [Google Scholar] [CrossRef]
- Mori, K.; Iwase, K.; Oba, Y.; Fukunaga, T.; Sugiyama, M. Surface Observation of LaNi5 under Deuterium Atmosphere Using Small-Angle Neutron Scattering. Mater. Trans. 2014, 55, 1643–1646. [Google Scholar] [CrossRef]
- Rivero-Mendoza, D.E.; Stanley, J.N.G.; Scott, J.; Aguey-Zinsou, K.F. An Alumina-Supported Ni-La-Based Catalyst for Producing Synthetic Natural Gas. Catalysts 2016, 6, 170. [Google Scholar] [CrossRef]
- Nuchkrua, T.; Leephakpreeda, T. Actuation of Pneumatic Artificial Muscle via Hydrogen Absorption/Desorption of Metal Hydride-LaNi5. Adv. Mech. Eng. 2015, 7, 364306. [Google Scholar] [CrossRef]
- Haque, N.; Hughes, A.; Lim, S.; Vernon, C. Rare Earth Elements: Overview of Mining, Mineralogy, Uses, Sustainability and Environmental Impact. Resources 2014, 3, 614–635. [Google Scholar] [CrossRef]
- Zhou, B.L.; Li, Z.X.; Chen, C.C. Global Potential of Rare Earth Resources and Rare Earth Demand from Clean Technologies. Minerals 2017, 7, 203. [Google Scholar] [CrossRef]
- Ge, J.P.; Lei, Y.L.; Zhao, L.R. China’s Rare Earths Supply Forecast in 2025: A Dynamic Computable General Equilibrium Analysis. Minerals 2016, 6, 95. [Google Scholar] [CrossRef]
- Wang, X.B.; Yao, M.T.; Li, J.S.; Zhang, K.X.; Zhu, H.; Zheng, M.S. China’s Rare Earths Production Forecasting and Sustainable Development Policy Implications. Sustainability 2017, 9, 1003. [Google Scholar] [CrossRef]
- Schreiber, A.; Marx, J.; Zapp, P.; Hake, J.F.; Vossenkaul, D.; Friedrich, B. Environmental Impacts of Rare Earth Mining and Separation Based on Eudialyte: A New European Way. Resources 2016, 5, 32. [Google Scholar] [CrossRef]
- Saleem, H.A. Social and Environmental Impact of the Rare Earth Industries. Resources 2014, 3, 123–134. [Google Scholar] [CrossRef]
- Machacek, E.; Richter, J.L.; Lane, R. Governance and Risk-Value Constructions in Closing Loops of Rare Earth Elements in Global Value Chains. Resources 2017, 6, 59. [Google Scholar] [CrossRef]
- Otsuki, A.; Dodbiba, G.; Fujita, T. Two-Liquid Flotation for Separating Mixtures of Ultra-Fine Rare Earth Fluorescent Powders for Material Recycling—A Review. Colloids Interfaces 2018, 2, 7. [Google Scholar] [CrossRef]
- Li, Y.; Yu, H.; Zheng, S.; Miao, Y.; Yin, S.; Li, P.; Bian, Y. Direct Quantification of Rare Earth Elements Concentrations in Urine of Workers Manufacturing Cerium, Lanthanum Oxide Ultrafine and Nanoparticles by a Developed and Validated ICP-MS. Int. J. Environ. Res. Public Health 2016, 13, 350. [Google Scholar] [CrossRef] [PubMed]
- Ault, T.; Krahn, S.; Croff, A. Radiological Impacts and Regulation of Rare Earth Elements in Non-Nuclear Energy Production. Energies 2015, 8, 2066–2081. [Google Scholar] [CrossRef]
- Butnariu, M.; Negrea, P.; Lupa, L.; Ciopec, M.; Negrea, A.; Pentea, M.; Sarac, I.; Samfira, I. Remediation of Rare Earth Element Pollutants by Sorption Process Using Organic Natural Sorbents. Int. J. Environ. Res. Public Health 2015, 12, 11278–11287. [Google Scholar] [CrossRef] [PubMed]
- Liu, W.; Aguey-Zinsou, K.F. Low temperature synthesis of LaNi5 nanoparticles for hydrogen storage. Int. J. Hydrogen Energy 2016, 41, 1679–1687. [Google Scholar] [CrossRef]
- Liu, W.; Aguey-Zinsou, K.F. Synthesis of highly dispersed nanosized LaNi5 on carbon: Revisiting particle size effects on hydrogen storage properties. Int. J. Hydrogen Energy 2016, 41, 14429–14436. [Google Scholar] [CrossRef]
- Todoran, R.; Todoran, D.; Racolta, D.; Szakacs, Z. Electrical and Optical Properties of CeNi5 Nanoscale Films. Nanoscale Res. Lett. 2016, 11, 253. [Google Scholar] [CrossRef]
- Todoran, D.; Todoran, R.; Szakacs, Z. Some optical properties of CeNi5 thin films. J. Optoelectron. Adv. Mater. 2013, 15, 54–57. [Google Scholar]
- Gondoni, P.; Ghidelli, M.; Di Fonzo, F.; Russo, V.; Bruno, P.; Marti-Rujas, J.; Bottani, C.E.; Bassi, A.L.; Casari, C.S. Structural and functional properties of Al:ZnO thin films grown by Pulsed Laser Deposition at room temperature. Thin Solid Films 2012, 520, 4707–4711. [Google Scholar] [CrossRef]
- Gondoni, P.; Ghidelli, M.; Di Fonzo, F.; Carminati, M.; Russo, V.; Bassi, A.L.; Casari, C.S. Structure-dependent optical and electrical transport properties of nanostructured Al-doped ZnO. Nanotechnology 2012, 23, 365706. [Google Scholar] [CrossRef] [PubMed]
- Hummel, R.E.; Dubroca, T. Differential Reflectance Spectroscopy. In Analysis of Surfaces Encyclopedia of Analytical Chemistry; Editor Meyers, R.A., Ed.; John Wiley & Sons, Ltd.: Chichester, UK, 2006; ISBN 978-0-470-02731-8. [Google Scholar] [CrossRef]
- Skoryna, J.; Marczyska, A.; Pacanowski, S.; Szajeka, A.; Smardza, L. XPS Valence Band Studies of LaNi5−xMx (M = Al, Co; x = 0; 1) Alloy Thin Films. Acta Phys. Pol. A 2015, 127, 430–432. [Google Scholar] [CrossRef]
- Burzo, E.; Chiuzbaian, S.G.; Chioncel, L.; Neumann, M. Magnetic and electronic properties of the LaNi5−xCux system. J. Phys. Condens. Matter 2000, 12, 5897–5904. [Google Scholar] [CrossRef]
- Burzo, E.; Chiuzbaian, S.G.; Neumann, M.; Chioncel, L. Magnetic and electronic properties of the LaNi5−xAlx system. J. Phys. Condens. Matter 2002, 14, 8057–8065. [Google Scholar] [CrossRef]
- Chen, D.; Xu, C. Investigations of the optical properties of LaNi5 and LaNi4.5Sn0.5. Appl. Mech. Mater. 2013, 321–324, 495–498. [Google Scholar] [CrossRef]
- Kalita, A.; Kalita, M.P.C. Williamson-Hall analysis and optical properties of small sized ZnO nanocrystals. Physica E 2017, 92, 36–40. [Google Scholar] [CrossRef]
- Valdes, L.B. Resistivity measurements on germanium for transistors. Proc. Inst. Rad. Eng. 1954, 42, 420–427. [Google Scholar] [CrossRef]
- Kramers, H.A. The law of dispersion and Bohr’s theory of spectra. Nature 1924, 113, 673–674. [Google Scholar] [CrossRef]
- Kronig, R.D.L. On the theory of dispersion of X-rays. J. Opt. Soc. Am. 1926, 12, 547–557. [Google Scholar] [CrossRef]
- Todoran, R.; Todoran, D.; Szakacs, Z. Valence band gaps and plasma energies for galena, sphalerite, and chalcopyrite natural minerals using differential optical reflectance spectroscopy. Russ. J. Phys. Chem. A 2015, 89, 2422–2425. [Google Scholar] [CrossRef]
- Todoran, D.; Todoran, R.; Anitas, E.M.; Szakacs, Z. Dynamic Determination of Some Optical and Electrical Properties of Galena Natural Mineral: Potassium Ethyl Xanthate Solution Interface. Russ. J. Phys. Chem. A 2017, 91, 2613–2620. [Google Scholar] [CrossRef]
- Todoran, R.; Todoran, D.; Szakacs, Z. Optical luminescence studies of the ethyl xanthate adsorption layer on the surface of sphalerite minerals. Spectrochim. Acta A Mol. Biomol. Spectrosc. 2016, 152, 591–595. [Google Scholar] [CrossRef] [PubMed]
- Yang, H.H.U.; D’Archangel, J.; Sundheimer, M.L.; Tucker, E.; Boreman, G.D.; Raschke, M.B. Optical dielectric function of silver. Phys. Rev. B 2015, 91, 235137. [Google Scholar] [CrossRef]
- Witten, T.A.; Sander, L.M. Diffusion-limited aggregation, a kinetic critical phenomenon. Phys. Rev. Lett. 1981, 47, 1400–1403. [Google Scholar] [CrossRef]
- Anitas, E.M.; Slyamov, A.M.; Szakacs, Z. Microstructural characterization of surface fractals using small-angle scattering. Rom. J. Phys. 2018, 63, 104. [Google Scholar]



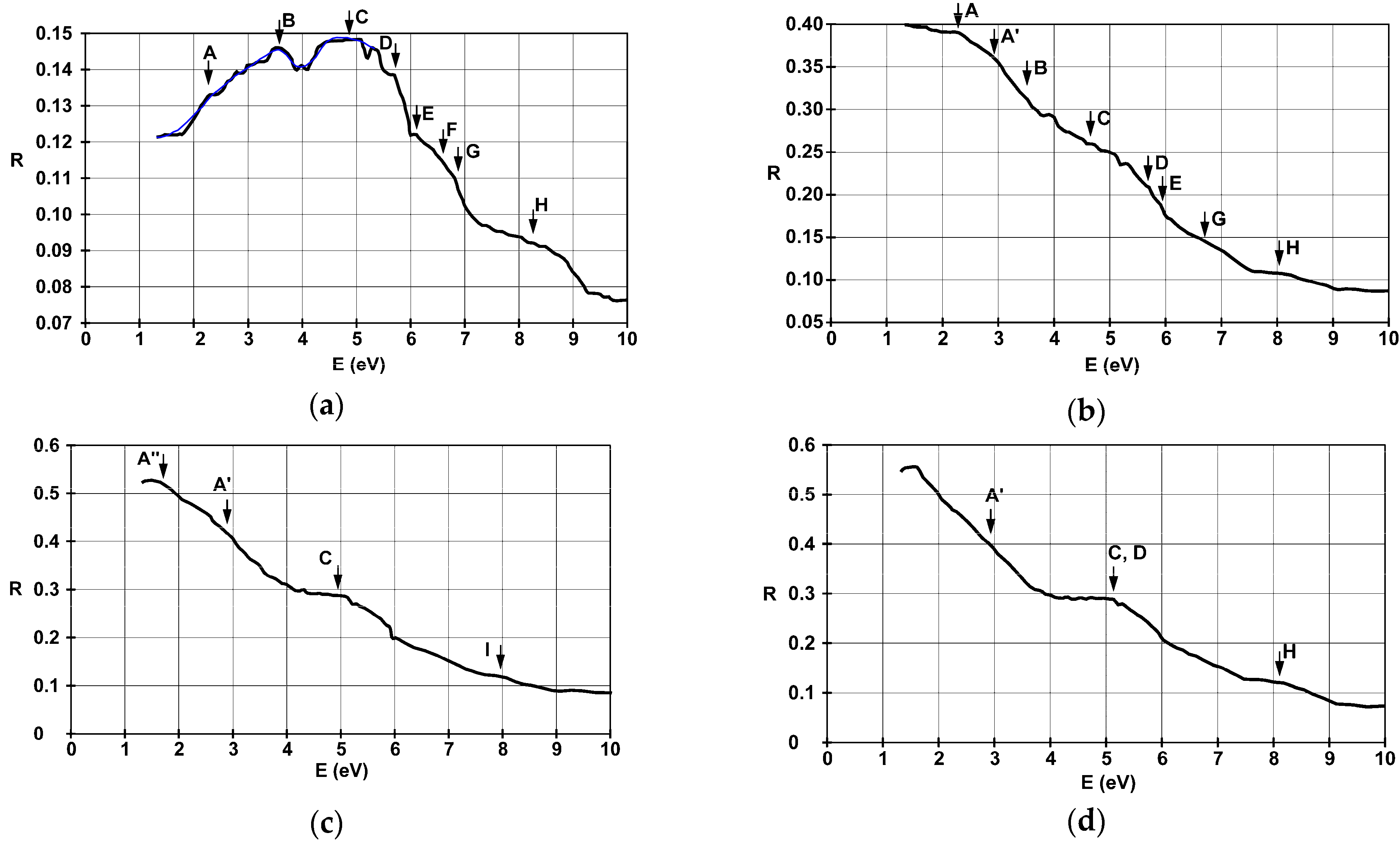
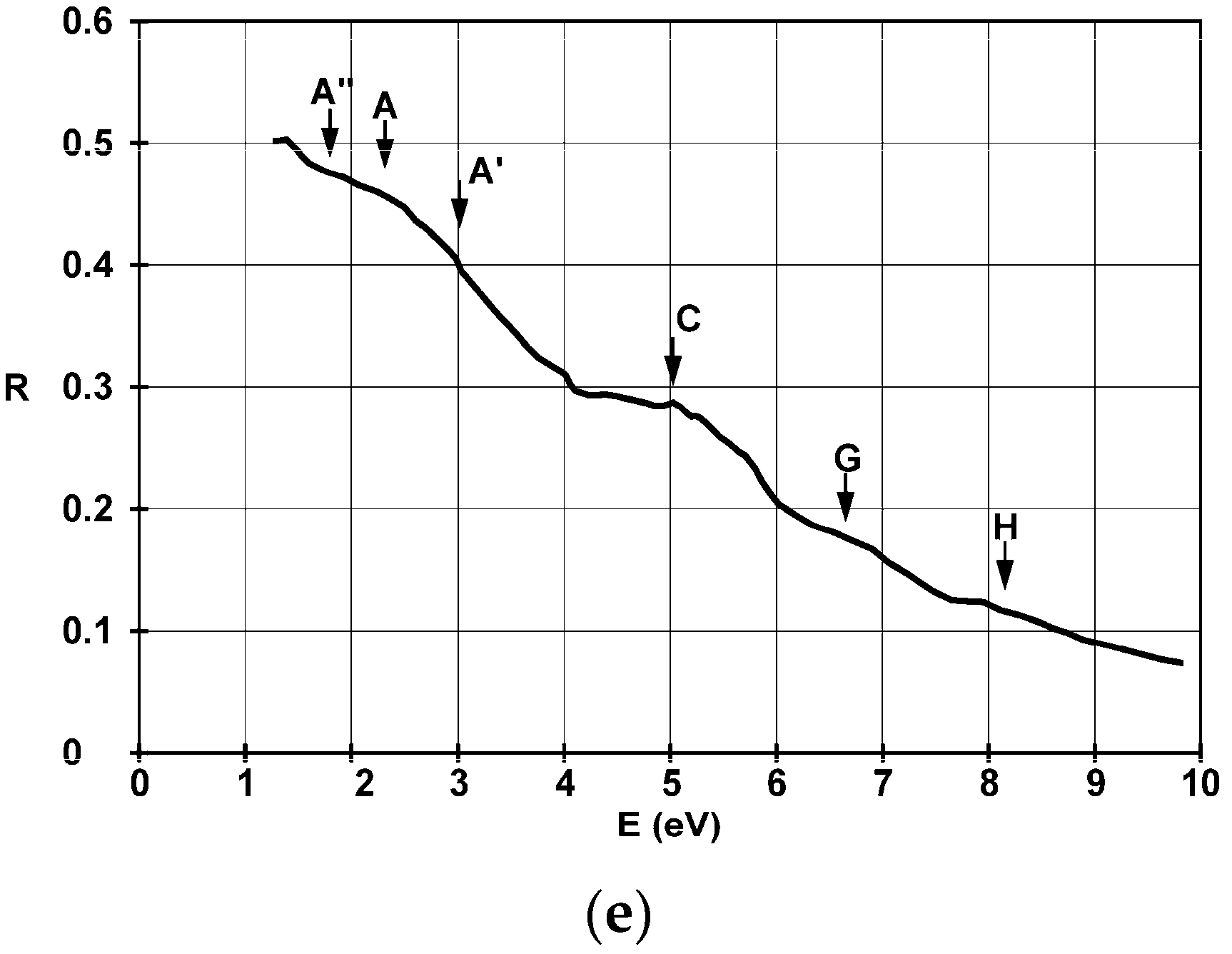
| Substrate | Film Thickness | Identified Allowed Interband Transitions (eV) | |||||||
|---|---|---|---|---|---|---|---|---|---|
| A | B | C | D | E | F | G | H | ||
| Glass | 4.5 nm | 2.2 | 3.4 | 4.9 | 5.7 | 6.1 | 6.5 | 6.8 | 8.2 |
| 22 nm | 2.3; 3 | 3.5 | 4.9 | 5.8 | 6 | - | 6.8 | 8.2 | |
| 38 nm | 1.7; 2.9 | - | 4.7–5.2 | - | - | - | - | 8.1 | |
| 42 nm | 1.6; 3 | - | 5.2 | - | - | - | - | 7.8–8.2 | |
| SiO2 | 36 nm | 1.9; 2.4; 3 | - | 5.1 | - | - | - | 6.7 | 8.1 |
| Film Thickness | Skin Depth |
|---|---|
| 4.5 nm | 60 nm |
| 22 nm | 9.8 nm |
| 38 nm | 9.5 nm |
| 42 nm | 9.1 nm |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Todoran, D.; Todoran, R.; Szakács, Z.; Anitas, E. Electrical Conductivity and Optical Properties of Pulsed Laser Deposited LaNi5 Nanoscale Films. Materials 2018, 11, 1475. https://doi.org/10.3390/ma11081475
Todoran D, Todoran R, Szakács Z, Anitas E. Electrical Conductivity and Optical Properties of Pulsed Laser Deposited LaNi5 Nanoscale Films. Materials. 2018; 11(8):1475. https://doi.org/10.3390/ma11081475
Chicago/Turabian StyleTodoran, Daniela, Radu Todoran, Zsolt Szakács, and Eugen Anitas. 2018. "Electrical Conductivity and Optical Properties of Pulsed Laser Deposited LaNi5 Nanoscale Films" Materials 11, no. 8: 1475. https://doi.org/10.3390/ma11081475
APA StyleTodoran, D., Todoran, R., Szakács, Z., & Anitas, E. (2018). Electrical Conductivity and Optical Properties of Pulsed Laser Deposited LaNi5 Nanoscale Films. Materials, 11(8), 1475. https://doi.org/10.3390/ma11081475





