1. Introduction
The production process of 6xxx series aluminium sheets consists in a succession of thermomechanical steps designed to improve the strength of the product while reaching the desired geometry. The initial billet with its specific chemical composition is produced by continuous or batch casting. Each subsequent step brings irreversible changes in the microstructure that directly affect the mechanical properties of the material. A combination of recrystallised, finely grained, and precipitation hardened microstructure brings the best mechanical strength to the sheet while preserving a reasonable ductility for further shaping processes.
It is now a well-established practice to model the industrial processes with finite element methods, which require material data as an input. Modelling allows to roughly calculate the properties of the final product, supporting the design and optimisation of the production processes. As the models can only be as good as our understanding of the physical phenomena they are meant to represent, an experimental investigation is always needed to validate their output and to understand the underlying physical phenomena.
Phenomenological models consist in setting up a constitutive equation linking the flow stress to the strain, the strain rate, and the temperature, and optimising it so that it best represents the main features of the flow curves. The equation usually features a power law dependency for the strain and the strain rate, and an activation energy for the thermal dependency [
1,
2,
3,
4]. Such models present the advantages of being easy to set up and requiring almost no computational power, but they do not provide any insight on the physics of the problem at hand.
Physical models are more deeply connected to the microstructure evolution. The modification of the microstructure depends essentially on the material itself, its initial state, and its thermomechanical history. In the last decades, metallurgists have developed models to describe the strain hardening of metallic alloys during forming processes using physical-based microstructural approaches. Such approaches usually consist of three main features [
5,
6,
7,
8]; a set of independent internal variables representative of the microstructure (classically dislocation densities, subgrain sizes, precipitation state, etc.), the evolution rates of these variables, and a constitutive equation to link the microscopic variables with the flow stress of the material. The difficulty in observing the underlying mechanisms responsible for the variable evolution often leads to the appearance of a large amount of model parameters [
7,
8].
During deformation, the microstructure of metallic materials develops permanently, leading to important dynamic variations in the macroscopic stress required to further deform the material. Strain hardening, for example, results directly from the multiplication of microscopic defects such as dislocations. These variations essentially depend on the strain, the strain rate, and the temperature of work. After deformation, for example in between passes in a rolling process, the microstructure may undergo static recovery, that is, the annihilation and rearrangement of microstructural defects that also affects the flow stress of the material. Flow stresses developed during hot rolling at elevated temperatures can be modelled using the total dislocation density as a single internal variable to represent the microstructure [
6], but modelling the behaviour of aluminium alloys from room to moderate temperatures requires at least two kinds of internal variables [
6]. In rolled aluminium products, static recrystallisation occurs after cold rolling during a recrystallisation or a solution treatment. The dominant mechanism for the nucleation new grains is strain-induced boundary migration [
9,
10], whereby subgrains lying on the boundaries of existing deformed grains bulge into the neighbouring grain and grow further after reaching the critical size for nucleation. As the motion of boundaries is a diffusional process, it is influenced by the temperature. The strain grade directly influences the number of potential nuclei being available for further growth.
In this work we propose a physically based constitutive model applicable to cold and hot working over a wide range of strain rates using three internal variables. Two kinds of dislocation densities that can evolve are responsible for the strain hardening, while the third kind accounts for the deformation and can be derived directly from the Orowan equation [
11]. Simple evolution rates are split in a dynamic part and a static part, enabling static recovery. A subsequent recrystallisation model featuring the same internal variables was developed that considers the level of strain reached during earlier deformation and encompasses the competition between recrystallisation and static recovery. The physical phenomena were derived and validated from experimental results obtained during cold and hot deformation and further recrystallisation of a 6082 aluminium alloy.
2. Materials and Methods
2.1. Material
A commercial 6082 aluminium alloy with the chemical composition shown in
Table 1 was studied. The material was delivered after hot rolling into a plate of thickness 3.9 mm and subsequent air cooling at room temperature. No homogenisation treatment was applied before rolling.
2.2. Experimental Methods
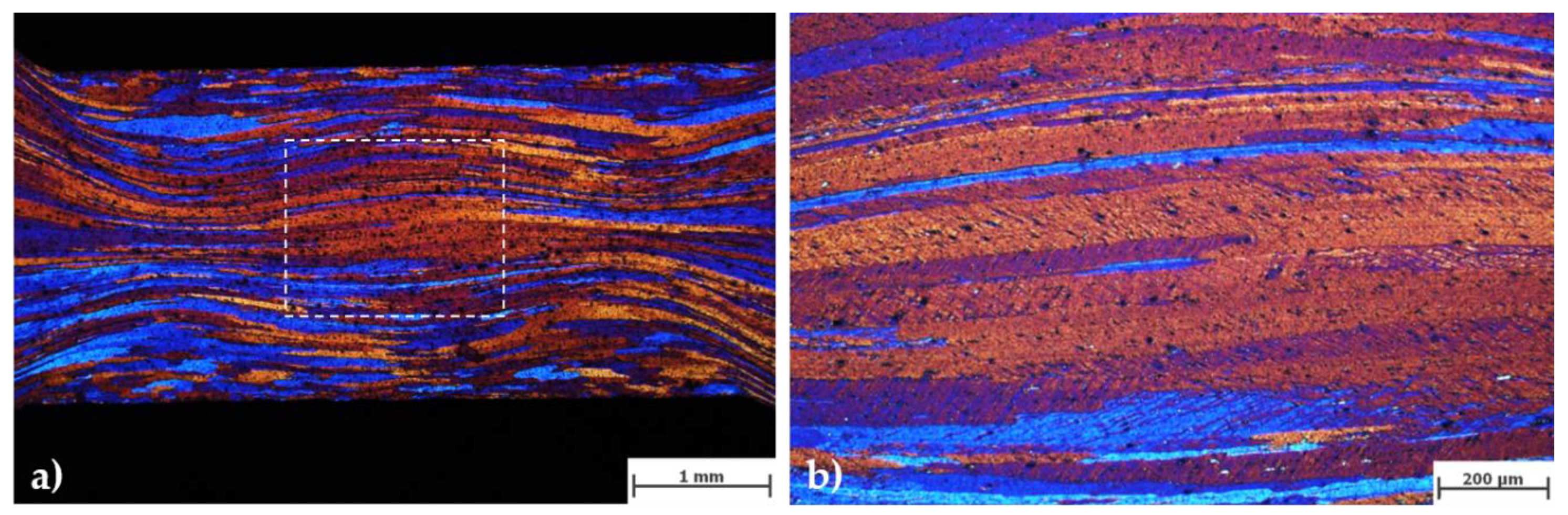
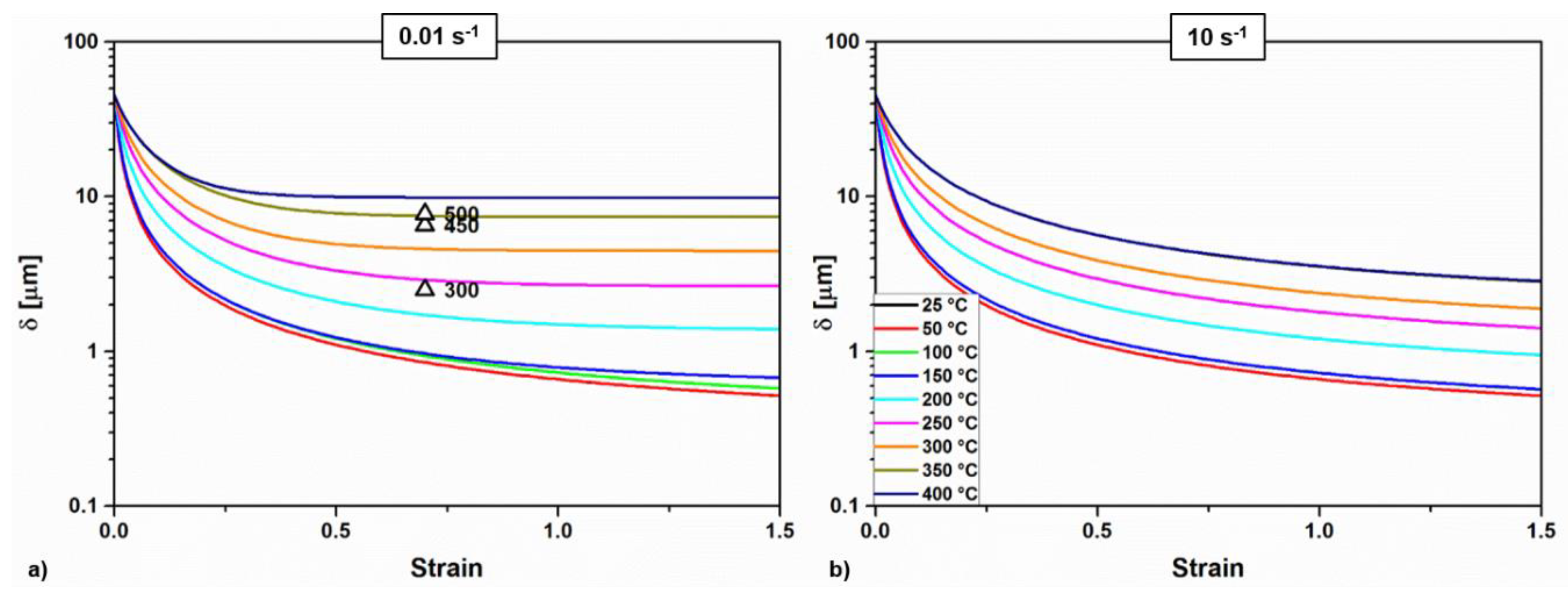
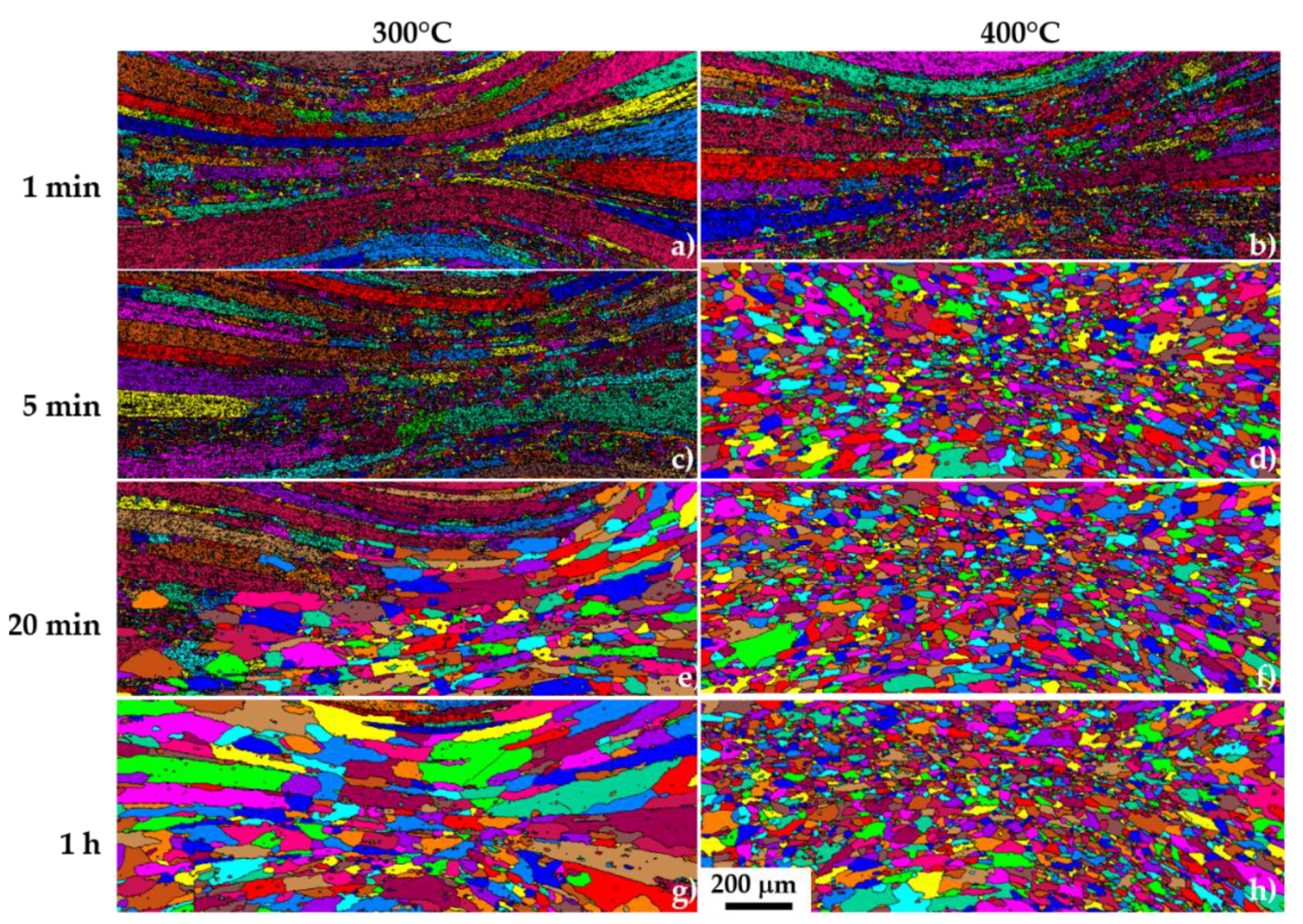
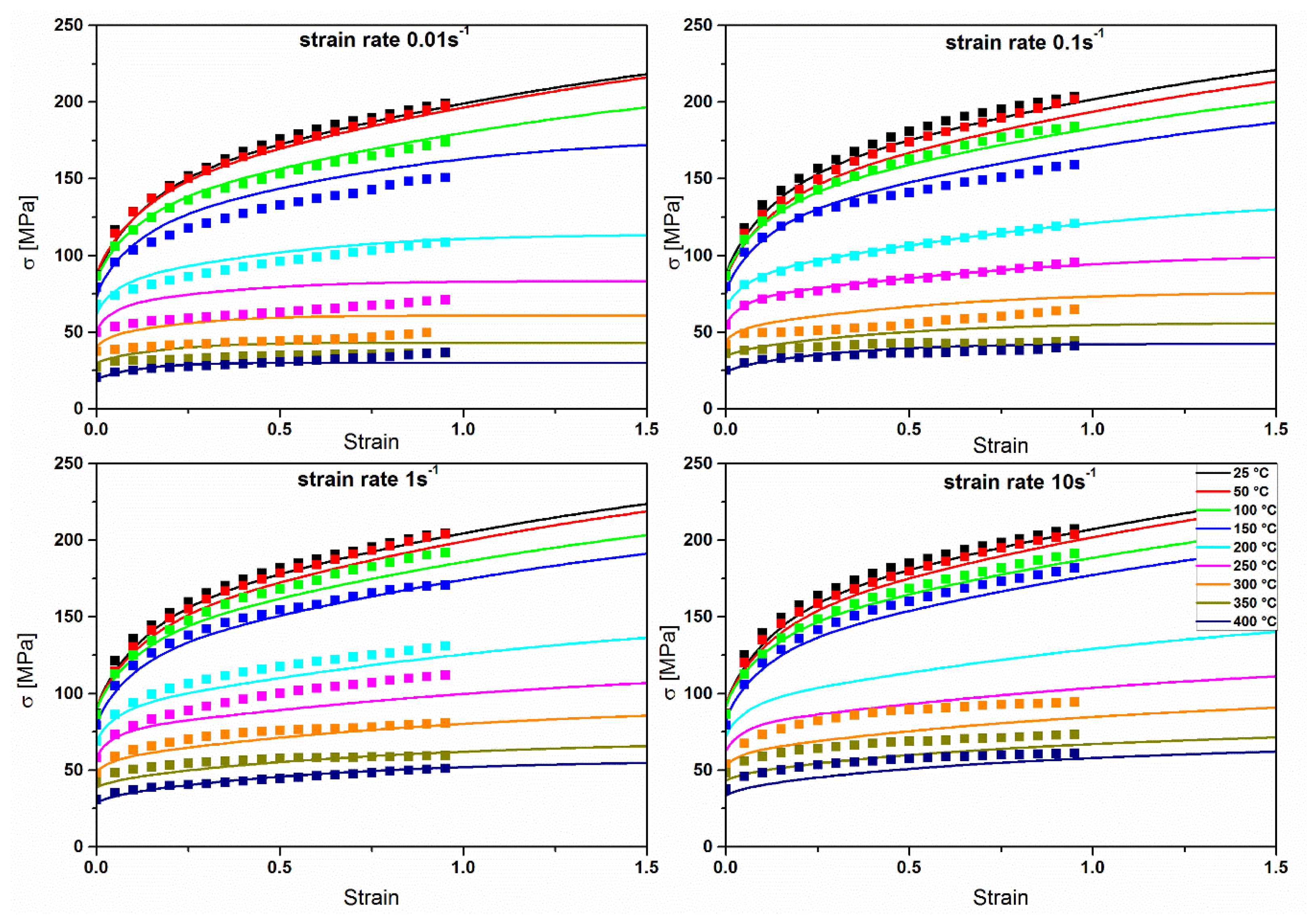
Samples of size 10 mm in the rolling direction and 20 mm in the transverse direction were cut out of the plate and compressed down to a thickness of 1.5 mm in plane strain condition using a Gleeble® 3800 machine (Dynamic Systems Inc., 323 NY 355 Poestenkill, NY, USA). The experiments were carried out between 25 °C and 400 °C, at strain rates of 0.01, 0.1, 1, and 10 s−1. The temperature was controlled by a J type thermocouple welded on the surface of the samples. Samples deformed at room temperature and 10 s−1 were then annealed in an oven at 300 °C and 400 °C for 10 s, 1 min, 5 min, and 1 h to induce recrystallisation.
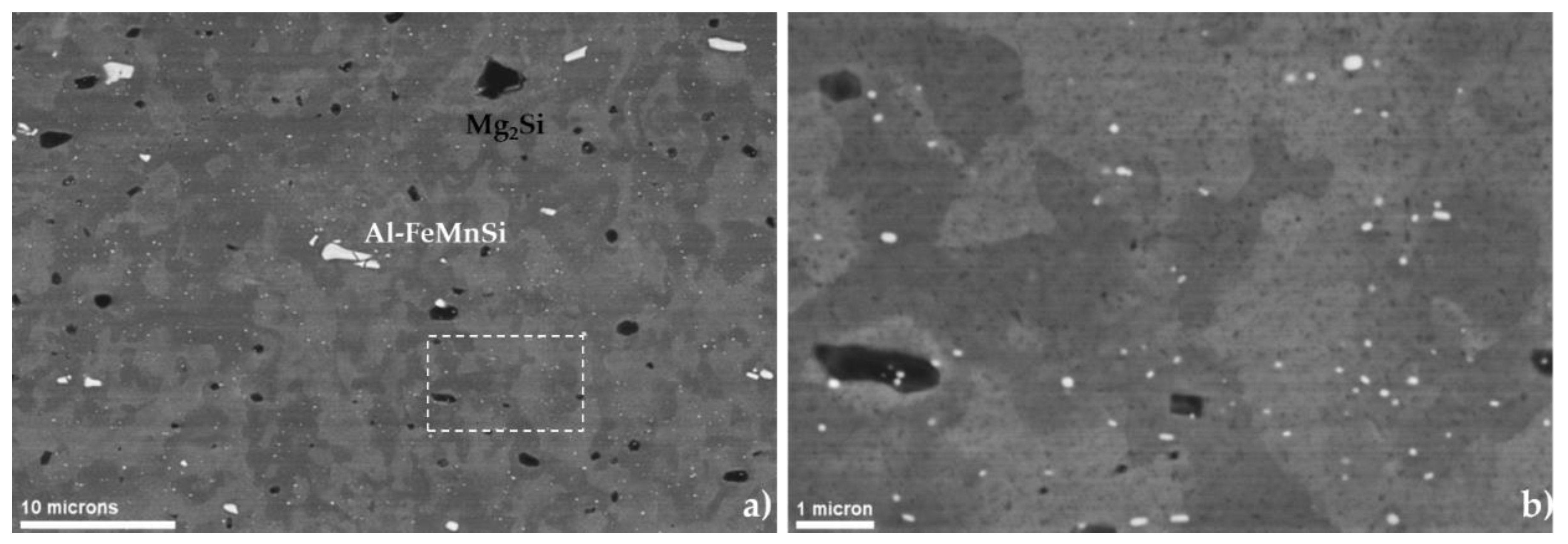
Light optical microscopy was carried out to determine the grain shape and size. Polarised light was used after metallographic preparation of the sample with Barker’s reagent (5 mL HBF4 48%vol in 200 mL water). Additionally, the precipitates were characterised in scanning electron microscope (SEM) Zeiss Ultra 55 (Carl-Zeiss AG, Oberkochen, Germany) with the use of a backscattered electron detector (BSE) which shows material contrast, and an energy dispersive X-ray detector (EDS) EDAX Genesis (EDAX Business Unit AMETEK GmbH, Weiterstadt, Germany). For these characterisations, the SEM was operated at a primary beam energy of only 6 kV to gain high surface sensitive measurements. The intermetallic phases were detected using BSE detector. The density
of particles intersecting the sample surface, as well as the average area of intersection
of individual particles, were readily measured from the obtained micrographs. Assuming many randomly distributed spheres of radius
, the averaging of the area of intersection of individual particles with the sample surface over
, is written as:
For a homogeneous distribution of particles, the volume fraction of particles
reads:
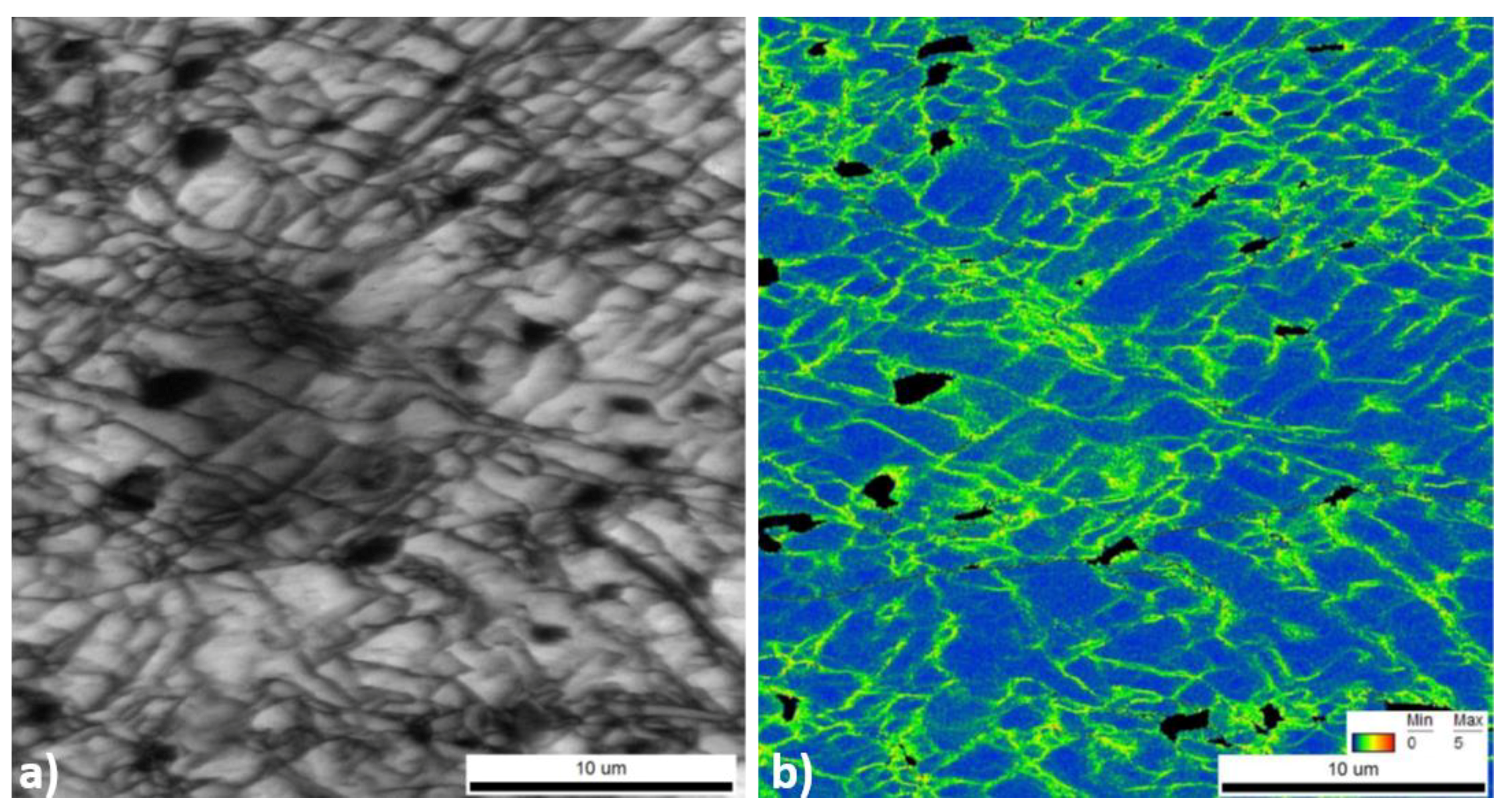
The as-received, deformed, and annealed samples were investigated by electron backscattered diffraction (EBSD) coupled with EDS. These investigations provided information about the grain and subgrain structure and of the intermetallic phases. All coupled EBSD–EDS investigations were performed at a primary beam energy of 20 kV on the Zeiss Ultra equipped with an EBSD system from EDAX-TSL. A tolerance angle of 11° was used to determine the subgrain structure.
Finite element simulations of the plane strain tests were carried out with the software DEFORM
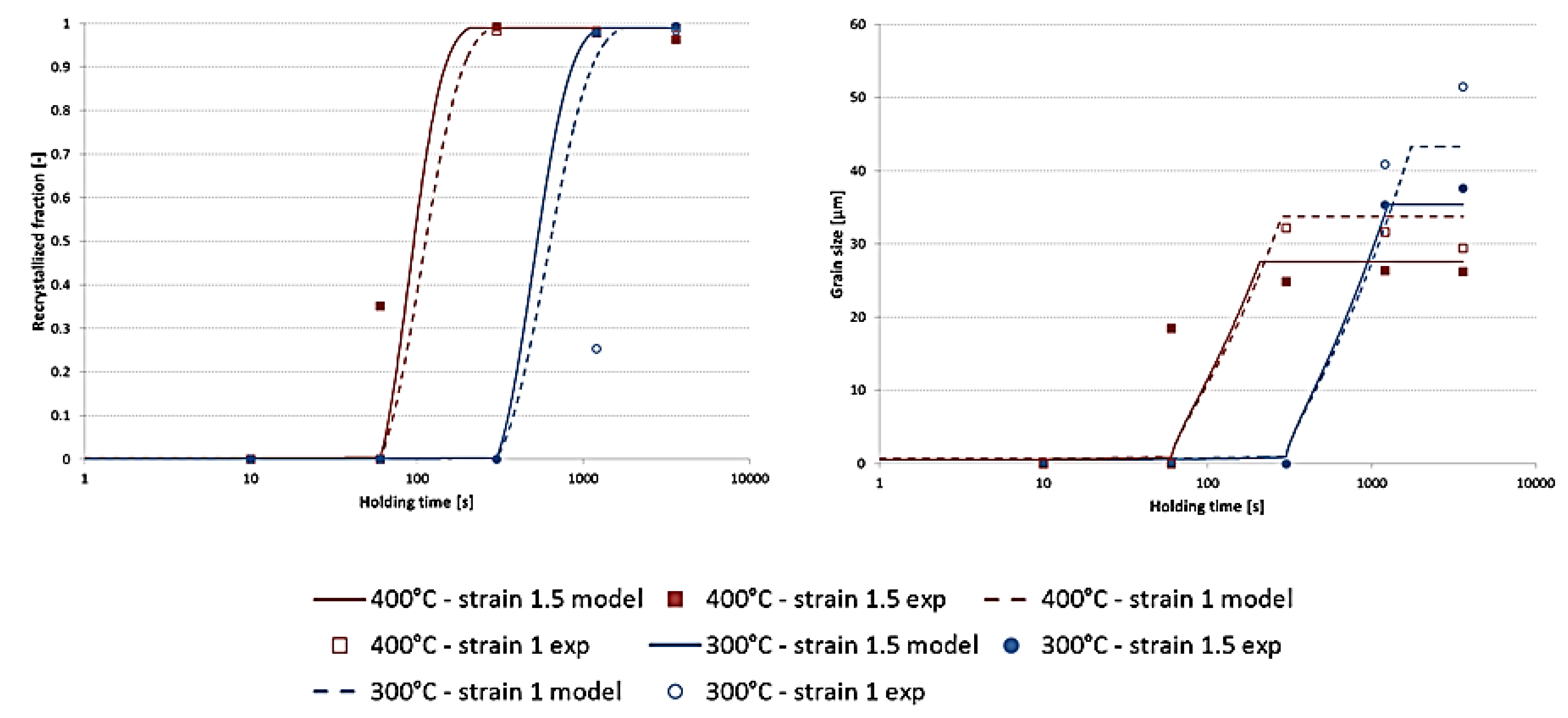
TM 2D (Scientific Forming Technologies Corporation, Columbus, OH, USA) to obtain the distribution of the equivalent strain values. The recrystallisation grade and the recrystallised grain size were characterised in the regions of strain 1 and 1.5 for both annealing temperatures. Grain orientation spread (GOS) maps were produced from EBSD measurements to distinguish recrystallised from non-recrystallised grains [
12] defining a maximum spread limit of 3°.
2.3. Modelling Methods
2.3.1. Microstructure Representation
The microstructure is assumed to be composed of well-defined subgrains, whose walls and interiors are populated with dislocations of respective densities
and
. It is emphasised that, although
usually stands for the dislocation density within the subgrains [
7,
8], it represents here the total length of wall dislocation per unit volume of the material. The later definition yields lower densities than the former. Additionally, mobile dislocations of density
travel across several subgrains before being stored in some manner, accounting for the macroscopic strain. The total density of dislocations
hence reads:
The subgrain size
in the deformed material can be calculated out of the dislocation densities. Two methods are available in the literature [
9]. If the subgrain boundaries are assumed to be tilt boundaries and if the wall dislocation density is averaged over the microstructure, then:
is a shape factor and is the average crystal orientation difference between each side of the boundary. This approach works fine at low to intermediate temperatures.
2.3.2. Constitutive Equation
The governing constitutive equation is chosen to have the following form:
where
is the flow stress of the material and
is the Taylor factor, accounting for the polycrystalline nature of the material [
13]. The athermal shear stress
resolved on the slip plane translates the long-range interaction of dislocations via their elastic strain field, and reads [
14]:
is a stress constant,
is the temperature dependent shear modulus, and
b is the Burgers vector. The effective resolved shear stress
is the additional stress required for mobile dislocations to be able to cut through the forest of dislocations cutting the slip plane and hindering them locally on their way through the microstructure. It is given by:
is the mean free path of dislocations,
is the Debye vibrational frequency of the material,
is the energy barrier of forest dislocations,
has the dimension of a volume and is classically referred to as the activation volume,
is the Boltzmann constant, and
T is the temperature. The glide velocity
of mobile dislocations is given by the Orowan equation:
where
is the rate of strain. The contribution of the intermetallic phases to the resolved shear stress
is the Orowan stress [
15]:
and , respectively, being the volume fraction of dispersoids and their equivalent radius.
2.3.3. Rate Equations
The evolution rates of
and
are each given by a Kocks–Mecking type of equation [
16] supplemented by a static annihilation term [
17]:
with
.
,
and
are dimensionless model parameters.
is an equilibrium dislocation density of a fully recrystallised material.
is the diffusion coefficient:
where
is the activation energy for self-diffusion. In Equation (10), the evolution rates are split into a dynamic part, linked to the strain rate, and a static part, diffusion driven. Although the kinetics of static mechanisms are negligible with respect to dynamic mechanisms, such a form of the model allows for diffusional phenomena when the strain rate is low or null and the temperature high enough.
2.3.4. Recrystallisation Model
The driving force for static recrystallisation and static recovery being related to the local stored energy, both processes happen simultaneously and competitively during annealing. The dislocation density decrease due to static recovery can be calculated by setting
in Equation (10). If the subgrain growth is assumed to be driven by capillarity [
9]:
being the mobility of low angle grain boundaries and
their specific energy, given by a Read and Shockley relationship [
18] of the form:
where
is the critical orientation difference for a low angle boundary to turn into a high angle boundary,
is the natural exponential, and
is the Poisson coefficient of the material. Since the mobile dislocation density is negligible with respect to the subgrain interior dislocation density, the difference in stored volume energy
between non-recrystallised and recrystallised grains reads:
Capillarity also tends to promote the growth of recrystallised grains, which translates in a driving pressure
:
where
is the specific energy of high angle grain boundaries and
is the mean grain size. Second phase particles hinder the movement of boundaries by exerting a retarding pressure
on them. The Zener mechanism [
19] yields the following equation:
The total driving pressure for recrystallisation
is then classically given by:
The growth rate of recrystallised grains
can be written as:
being the mobility of high angle grain boundaries. The grain radius is then calculated by integrating Equation (18) over time.
The factor 2 accounts for the fact that
is the diameter of recrystallised grains. The incubation time
, that is, the time it takes for the nuclei to reach the critical size and start growing, is temperature dependent and must be adjusted. According to the JMAK theory [
20], the extended recrystallised volume can be calculated as the product of the number
of nuclei available in the microstructure with the volume of a recrystallised grain. For site saturated nucleation, meaning that the
nuclei are created during deformation prior to annealing, this yields an equation of the type:
where
is a shape factor and
the Avrami exponent. The fraction of recrystallised material
is then given by:
The number of nuclei reaching the critical size for nucleation is written as follows:
where
is a model parameter. The activation energy is taken as the product of the specific energy of high angle boundaries with a typical area, as for strain induced boundary migration to happen, subgrains have to bulge into the neighbouring grain and displace the existing boundary. Grain growth after recrystallisation is driven only by capillarity. Hence, the driving pressure is the same as in Equation (17), without the term
. The grain size is again given by integrating the growth rate, but now starting at the time of end of recrystallisation.
2.3.5. Parameter Initialisation
The parameters of the flow stress model were initialised as follows:
,
nm [
21],
[
22],
s
−1,
and
eV. The shear modulus reads
in GPa (temperature in K) [
23]. The microstructure being initially fully recrystallised,
and
were initially taken equally low and equal to
m/m
3. Since the model must be able to work out the yield stress of the material, a least square optimisation method was run on
,
and
to best capture the temperature and strain rate dependency of the yield stress, with
let free to vary with the strain rate. The following values were worked out:
1/m
2,
m
3, and
eV. The rate parameters
and
were optimised to best capture the strain hardening of the material, while the
(
x =
i,
w) were taken equal to 1.
The parameters of the recrystallisation model were initialised as follows:
J·m
−2 [
9]. It was assumed that the subgrain misorientation already reaches, at very small strain values, a mean value of
° [
8]. The critical misorientation was selected as
= 15°.
classically has an Arrhenius expression of the form
and the literature provides wide ranges of values for both
and
. The following was determined from our recrystallisation experiments and used here:
m
4·J
−1·s
−1 and
eV. The mobility of the low angle grain boundaries
was taken equal to
[
24]. The parameter
was equal to
.
4. Discussion and Conclusions
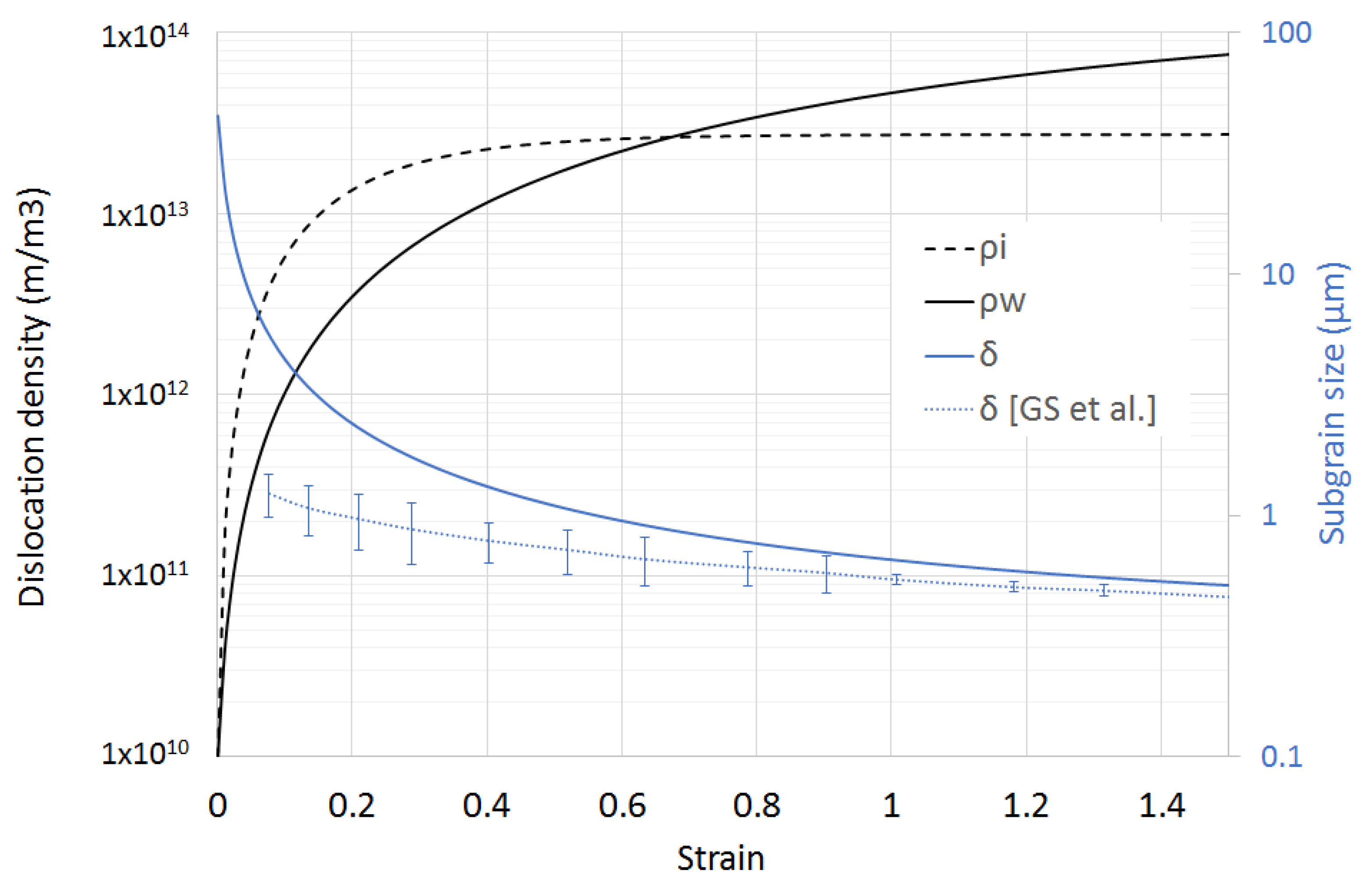
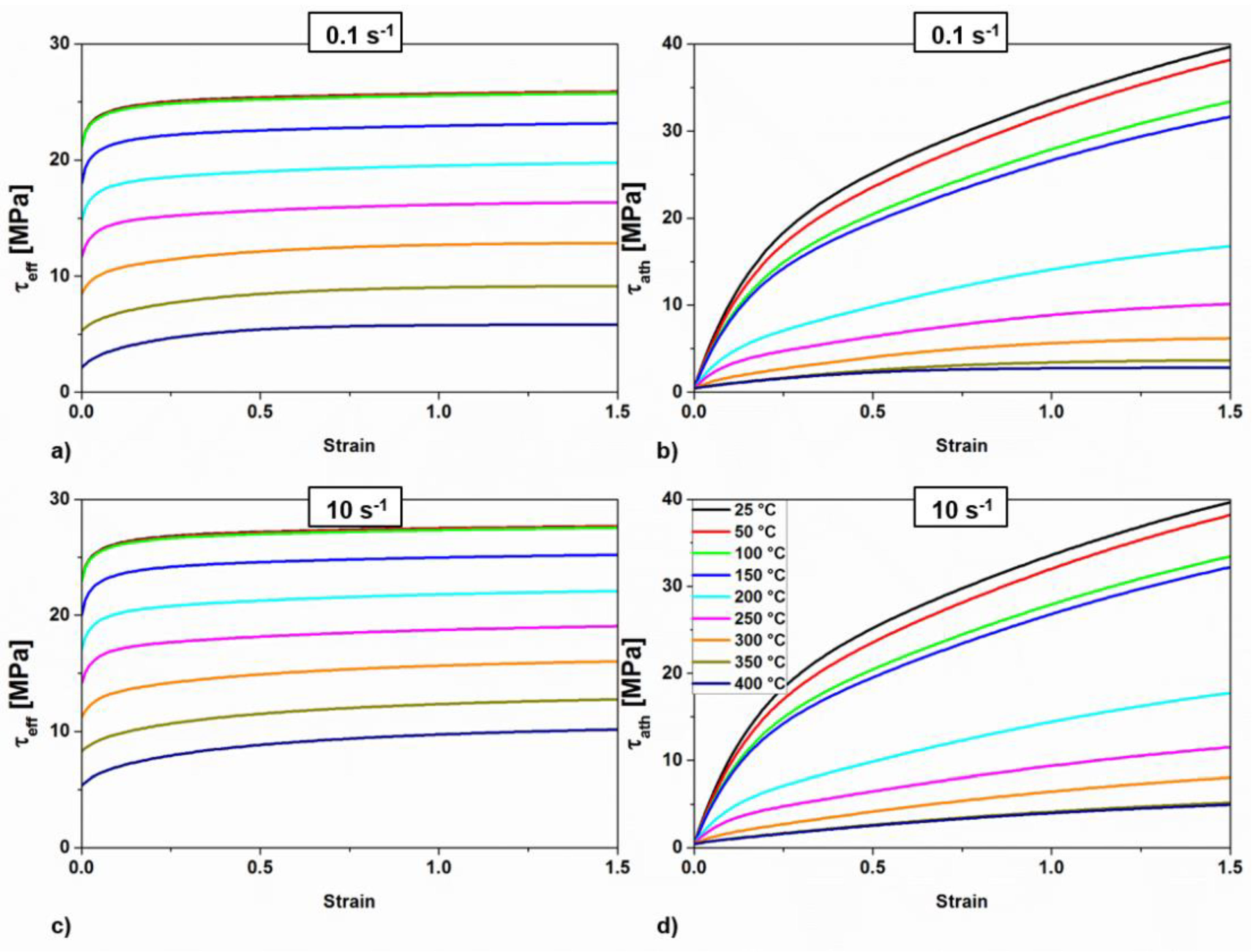
The flow stress model uses a relatively common approach to constitutive modelling. A strong hypothesis, based on the Orowan equation, is that the mobile dislocation density depends only on the strain rate, and remains constant during deformation at constant strain rate. The dislocation rate parameters are plotted against the temperature in
Figure 12. The decrease of the hardening parameter
with the temperature indicates that the storage of dislocations becomes less effective when the temperature increases, that is, that the mobile dislocations travel a longer distance before being immobilised, indicating an improved capacity for bypassing local obstacles when the temperature increases. The results also indicate that the hardening due to subgrain formation is promoted at lower temperatures since
decreases with increasing temperatures. As expected,
increases with the temperature, meaning that the dynamic recovery of cell interior dislocations is promoted. Interestingly,
does not depend on the temperature. This seems to indicate that the wall dislocations, being already arranged in a configuration of low energy, are not appreciably affected by dynamic climb.
The evolution of grain size after recrystallisation in samples deformed by plane strain compression can be explained as follows. As the critical bulge size for Strain Induced Boundary Migration has an inverse relationship to the stored energy of deformation, more grains can nucleate in the middle of the deformation crosses, where the accumulated strain is larger, than in regions of lower strain. The new grains impinge on each other upon growth and limit the grain size in the deformation crosses. Furthermore, the recrystallised grains are elongated in the direction of the alignment of the large aluminides. This is especially obvious during annealing at 300 °C between the deformation crosses. The grain growth in the transverse direction is severely limited in comparison to the rolling direction. In those regions where the intermetallic phases are located, the pinning effect on the moving boundaries increases accordingly, enough to stop them. At higher deformations and annealing temperatures, the same trend would be observed if the grains did not impinge on each other before reaching the bigger aluminides. The small aluminides, homogeneously distributed, might still slow down the boundary migration but cannot completely pin the grain boundaries during recrystallisation. Within the time frame of the measurements, the grain size appears to be stabilised. As grain growth after recrystallisation is driven only by capillarity effects, the pressure on the boundaries is much lower than during recrystallisation. Pinning by the homogeneously distributed smaller aluminides can then effectively counter balance this pressure and prevent any further boundary movement. Although above 350 °C, the Mg2Si phase dissolves, it does not affect the recrystallisation kinetics, because these particles are too coarse and too widely spaced to effectively pin the boundaries. The effects of the finely dispersed aluminides and of the lines of coarse aluminides are dominant. The most critical parameter in determining the final grain size appeared to be density of nuclei. The more grains nucleate, the less space they must grow and the lower the final grain size. By slightly increasing the density of nuclei being created, the predicted grain radius can be decreased to match the experimental measurements.