Spiral Bevel Gears Face Roughness Prediction Produced by CNC End Milling Centers
Abstract
:1. Introduction
2. Spiral Bevel Gears Manufacturing Process in Multitasking Machines
2.1. Design
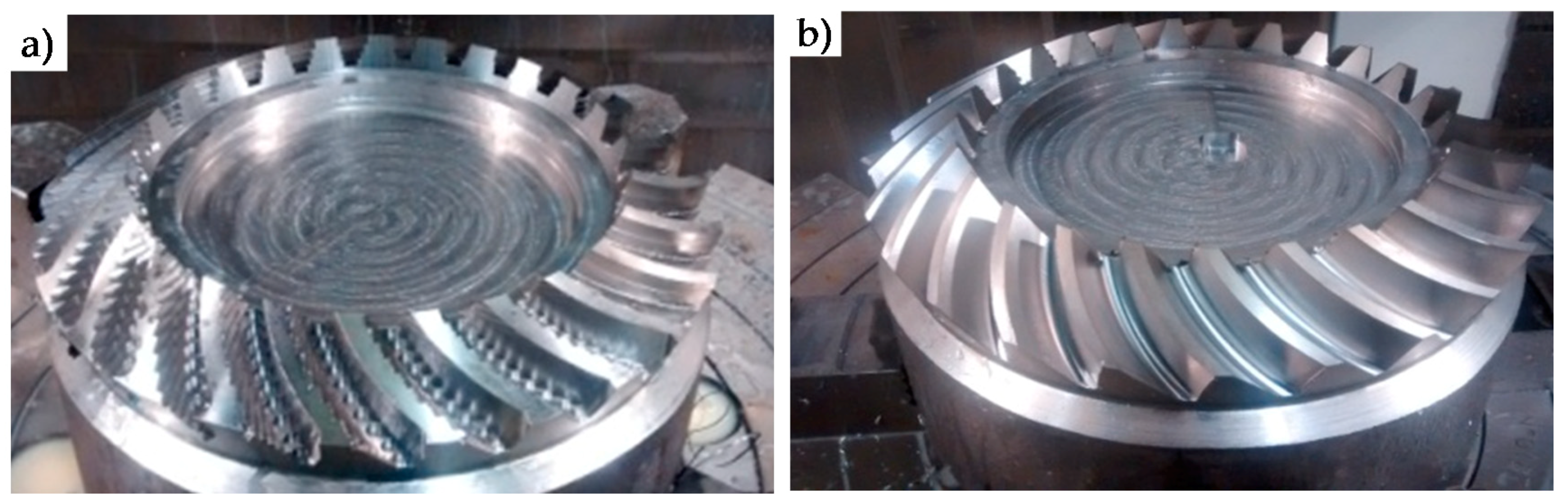
2.2. Manufacturing
2.3. Equipment—Multiprocess Machine
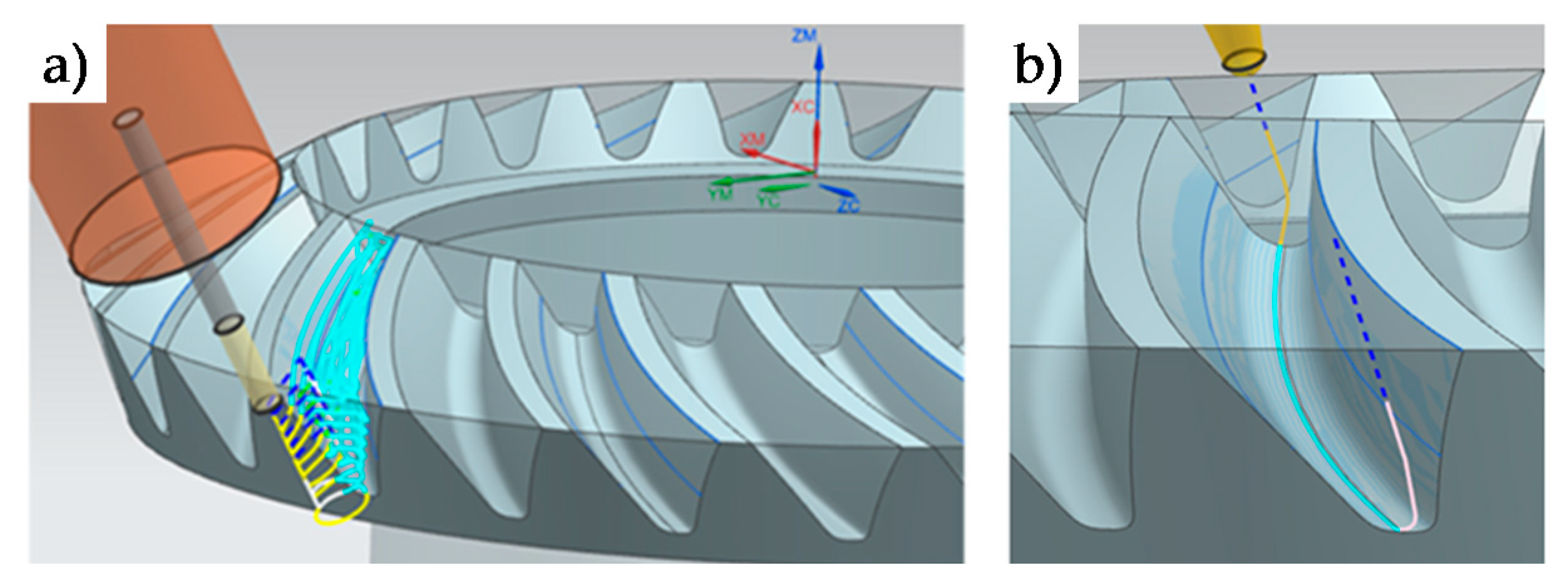
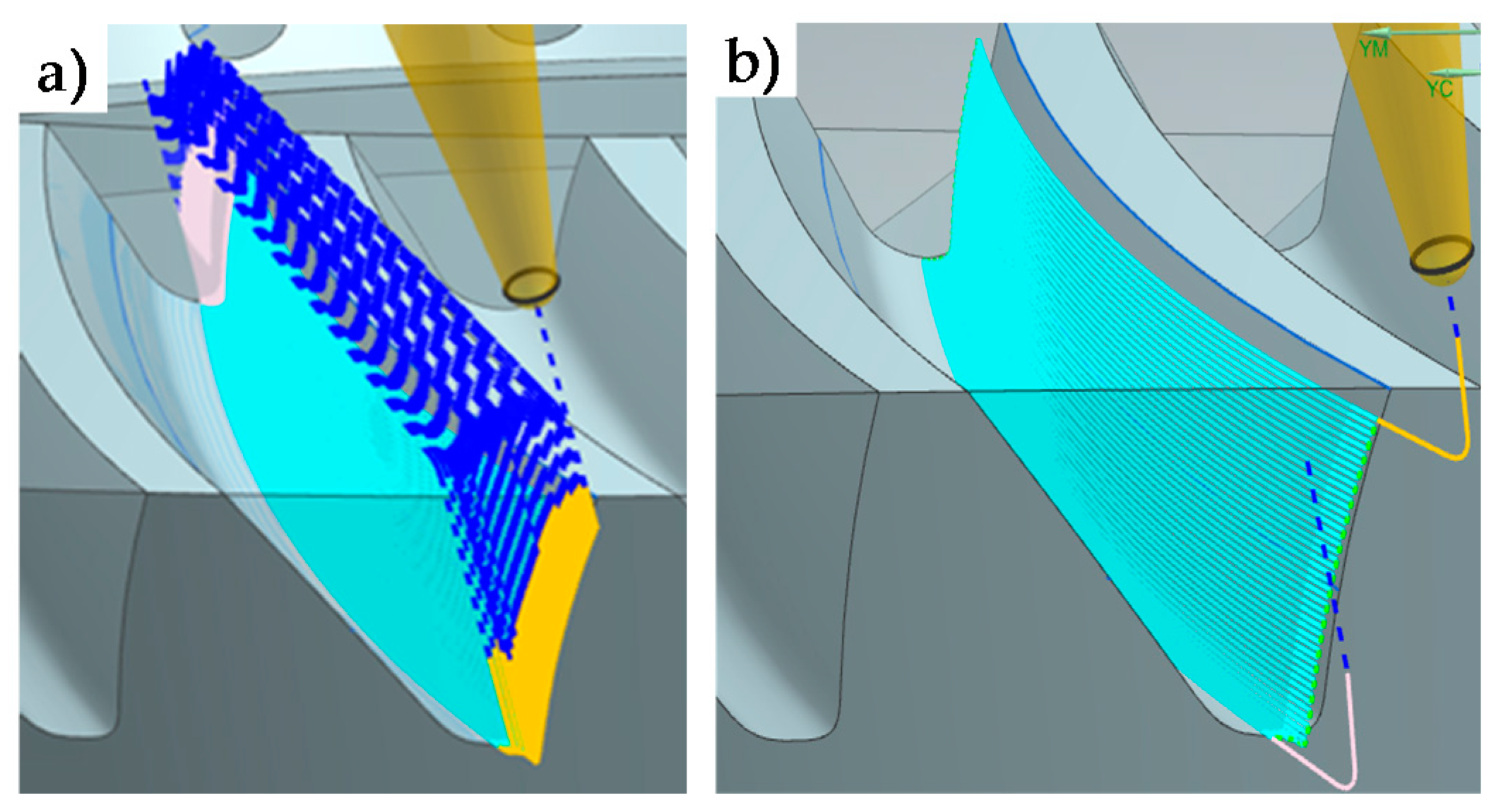
2.4. Machining Strategies
2.5. Machining
3. Predictive Model of Topography on Gear Flank
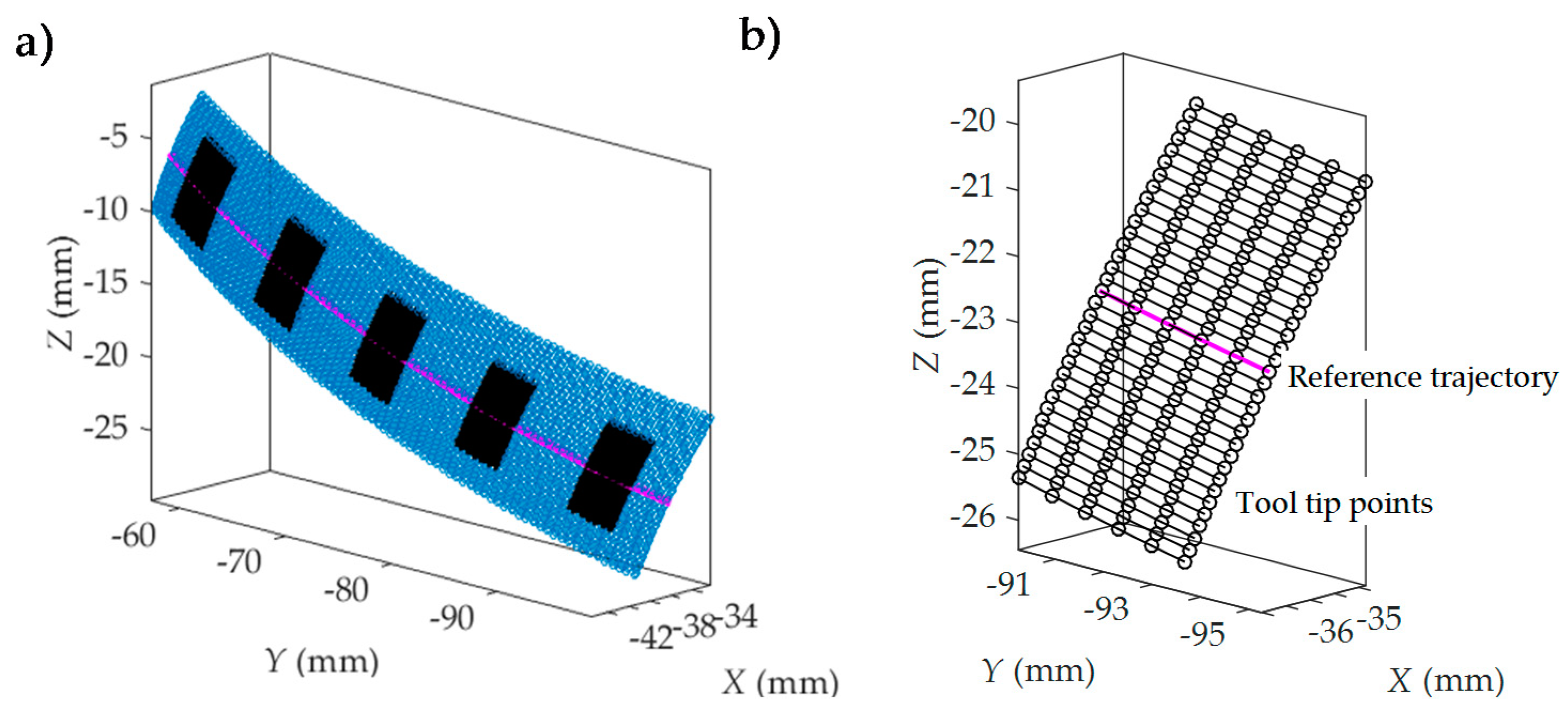
- Interdental gap points and trajectories representation (Figure 5). In this first step, for each interpolation point the model obtains from the machining program (CL data): the tool tip point position (xj, yj, zj) and the tool axis orientation defined by a direction vector (uj, vj, wj) in the workpiece reference system XYZ.Milling trajectories can be obtained by means of the coordinates of successive tool tip points. Figure 5a shows the tool tip point positions obtained from the machining program of an interdental gap. In Figure 5b, the milling trajectories are represented. In the next steps, milling trajectories are evaluated after the elimination of initial and final noncutting movements, as shown in Figure 6a.
- Simulation area definition (Figure 6). Secondly, as can be seen in Figure 6a, five simulation areas are defined (in black) in the feed direction. Each of these simulation areas is defined by 6 points in the feed direction and about 30 points in the direction perpendicular to the feed direction. The dimension of the simulation area depends on the programmer’s criteria. The reference trajectory for the study is defined in magenta, corresponding to the one located in the middle of the simulation area. As an example, Figure 6b shows the milling trajectories followed by the tool tip in one of the simulation areas considered in Figure 6a. Black points in each milling trajectory represent the interpolation points given by the machining program for the selected simulation area. Next, the surface topography generated in each simulation area is predicted. In order to achieve this, a local reference system OWXWYWZW is defined for each simulation area (Step 4). First, the positions of the tool center point (C) in the selected simulation area are deduced in Step 3.
- Tool center position determination (Figure 7). In order to obtain the coordinates of the tool center point (C) (Figure 7f), tool tip point coordinates (xj, yj, zj) and tool axis direction vector (uj, vj, wj) given in the machining program and the ball end mill, radius R needs to be taken into account. First, the line passing through the point (xj, yj, zj) and parallel to the vector (uj, vj, wj) is considered by means of the following equation:
- 4.
- Definition of workpiece local coordinate system. In order to predict the surface topography generated in each simulation area, a local coordinate system OWXWYWZW attached to the workpiece (gear tooth surface) is defined. The definition of this local coordinate system for each simulation area is based on the tool tip point positions and the tool axis orientations given in the machining program for the reference milling trajectory shown in magenta in Figure 6 and Figure 7. The origin OW and the axis ZW are the elements of this system that are obtained first. In order to achieve this, a set of auxiliary elements (a point M and two unit vectors and ) is considered.Firstly, two interpolation points, named A and B, which are located in the center of the reference trajectory (Figure 7b) are selected. The middle point D of the linear interpolation between points A and B is considered. The coordinates (xD, yD, zD) of point D are calculated as a function of the coordinates (xA, yA, zA) and (xB, yB, zB) of points A and B:
- 5.
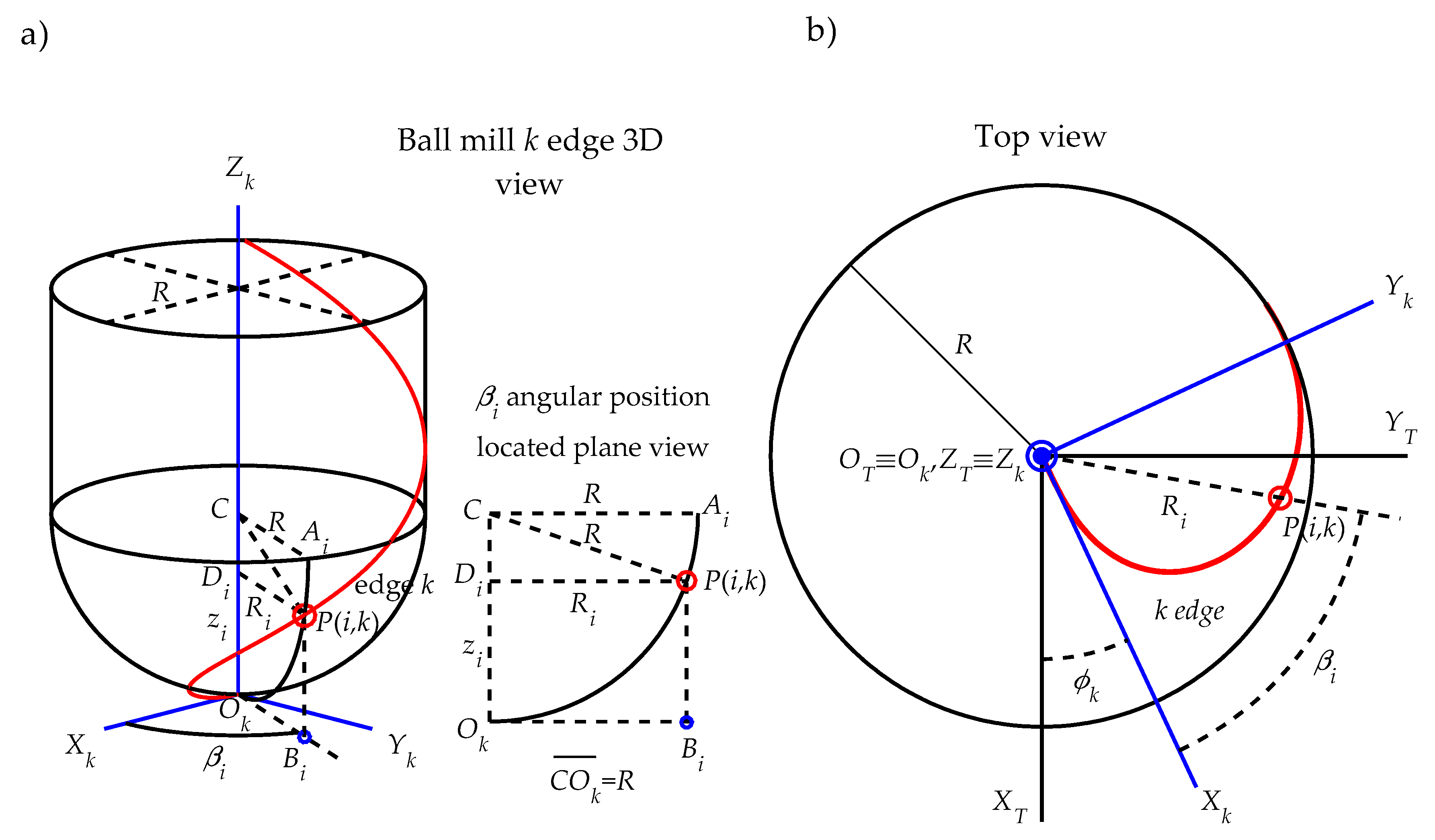
- Tool geometric modelization (Figure 8). The roughness model is developed for a ball end mill geometry, whose behavior resembles that of a conical tool that only cuts with the spherical area of the tool tip for gear teeth finishing trajectories. Figure 8a shows schematically the 3D geometry of a ball end mill of radius R and helix angle i0. For simplicity, only one of the edges is represented in the figure (Figure 8b), but the developed model is generalized for a Nt edge mill. It is assumed that the cutting edge represented in this figure represents one of the edges of the milling cutter, which is referred to as a k edge, where k = 1, 2, ..., Nt. To define the position of a point located on the edge k, a reference system OTXTYTZT attached to the ball end mill is defined:
- ■
- The reference system origin OT is located on the tool tip being coincident with the tool axis
- ■
- The axis ZT corresponds to the tool axis
- ■
- The axis XT is radial and tangent to edge 1 projection in the plane containing point OT and perpendicular to ZT axis
- ■
- The axis YT is perpendicular to axes XT and YT forming a right-handed system.
- 6.
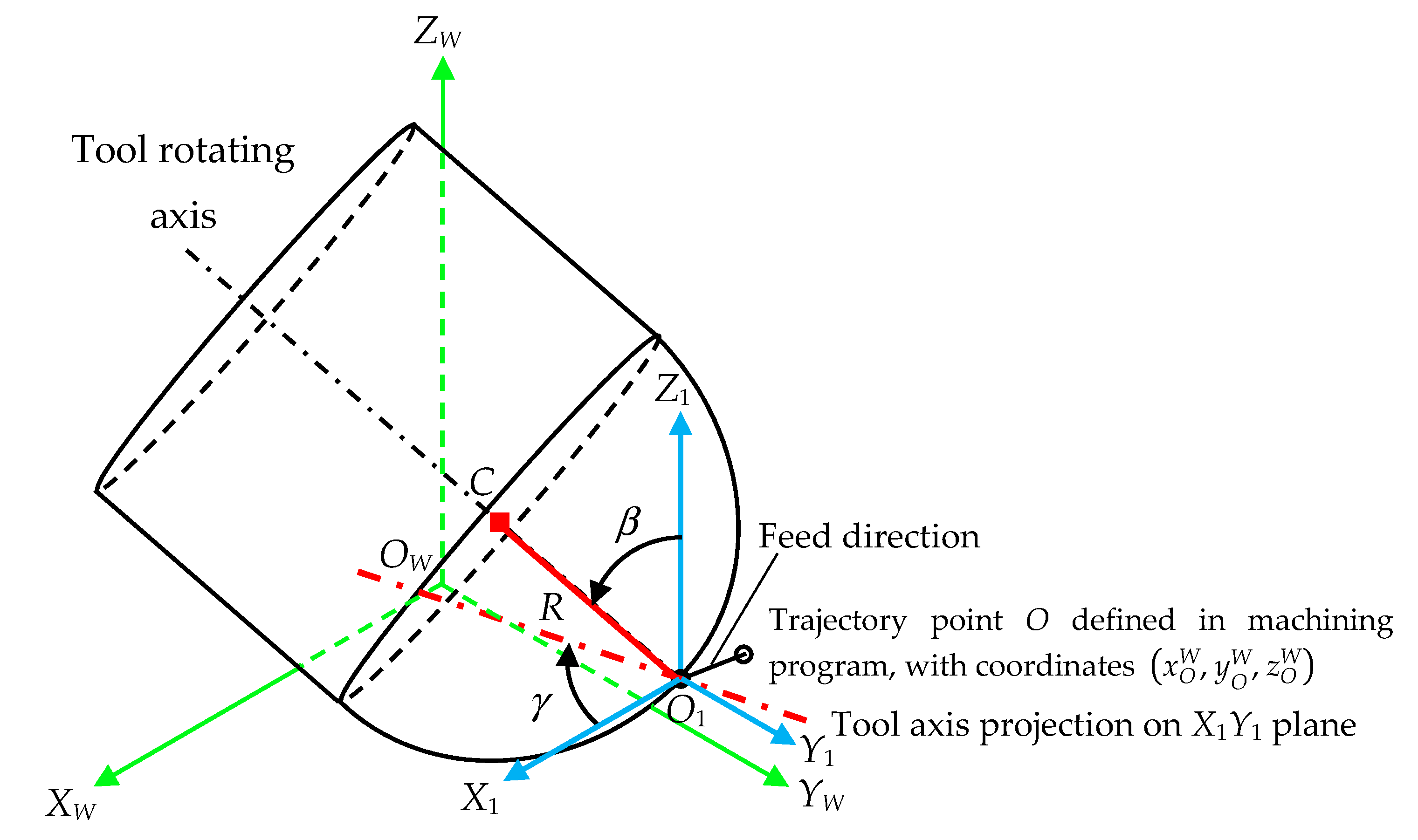
- Tool axis orientation and points trajectories determination (Figure 9 and Figure 10). In this step, the trajectory followed by any cutting edge point in a five-axis milling operation is expressed as a function of cutting parameters, tool axis orientation and milling trajectories defined in the machining program. The cutting parameters are the feed value (F) in mm/min and the spindle speed (S) in rpm. Therefore, the tool feed in mm per revolution can be calculated as:
- ■
- The tilt angle β is defined as the angle between the tool rotating axis and the axis Z1, which is parallel to axis ZW.
- ■
- The lead angle γ is the angle between the projection of the tool rotating axis into plane X1Y1 (which is parallel to plane XWYW) with respect to axis XW direction.
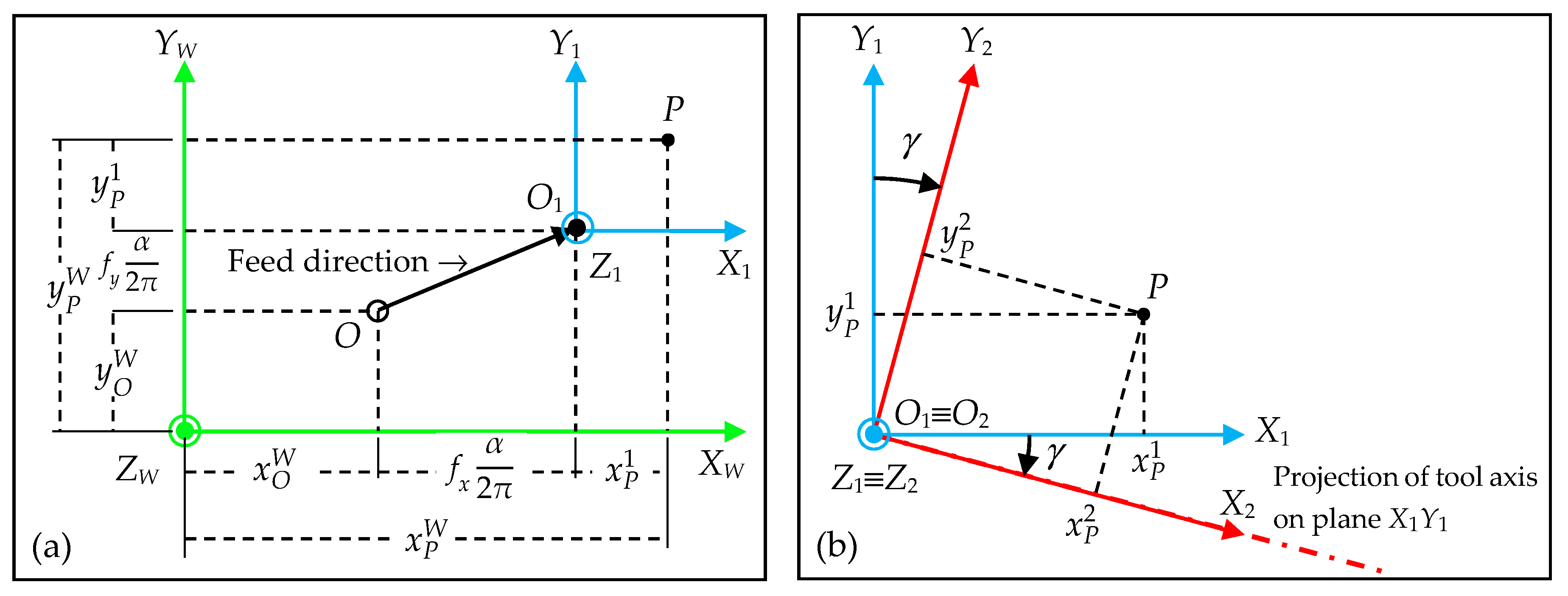
- The current position of the tool tip point given by the coordinates of point O1 (Figure 9). A translation of system O1X1Y1Z1 with respect to system OWXWYWZW (Figure 10a) is considered. The system O1X1Y1Z1 is shifted in XW, YW and ZW by distances xO1W, yO1W and zO1W respectively. The homogeneous transformation matrix TW1(fx, fy, fz, α) of this translation in XW, YW and ZW is:
- The lead angle γ (Figure 10b). The system O2X2Y2Z2 is rotated by an angle γ about axis Z1 in clockwise direction. The homogeneous transformation matrix T12(γ) of this rotation about Z1-axis is:
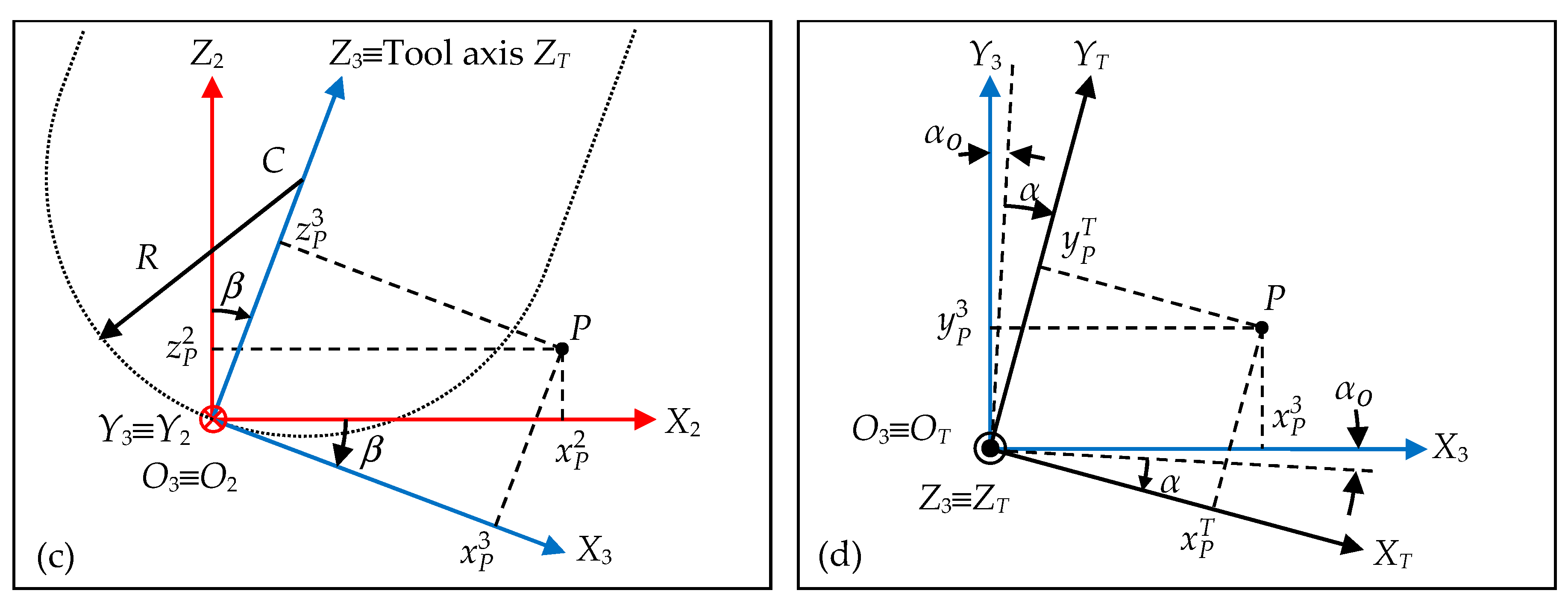
- The tilt angle β (Figure 10c). The system O3X3Y3Z3 is rotated by an angle about axis Y2 in a clockwise direction. The homogeneous transformation matrix T23(β) of this rotation about Y2-axis is:
- The rotation angle α (Figure 10d). The tool system OTXTYTZT is rotated by an angle α about axis Z3 in clockwise direction. The homogeneous transformation matrix T3T(α) of this rotation about Z3-axis is:where αO is the tool rotation angle when the tool tip point is located at point O.
- 7.
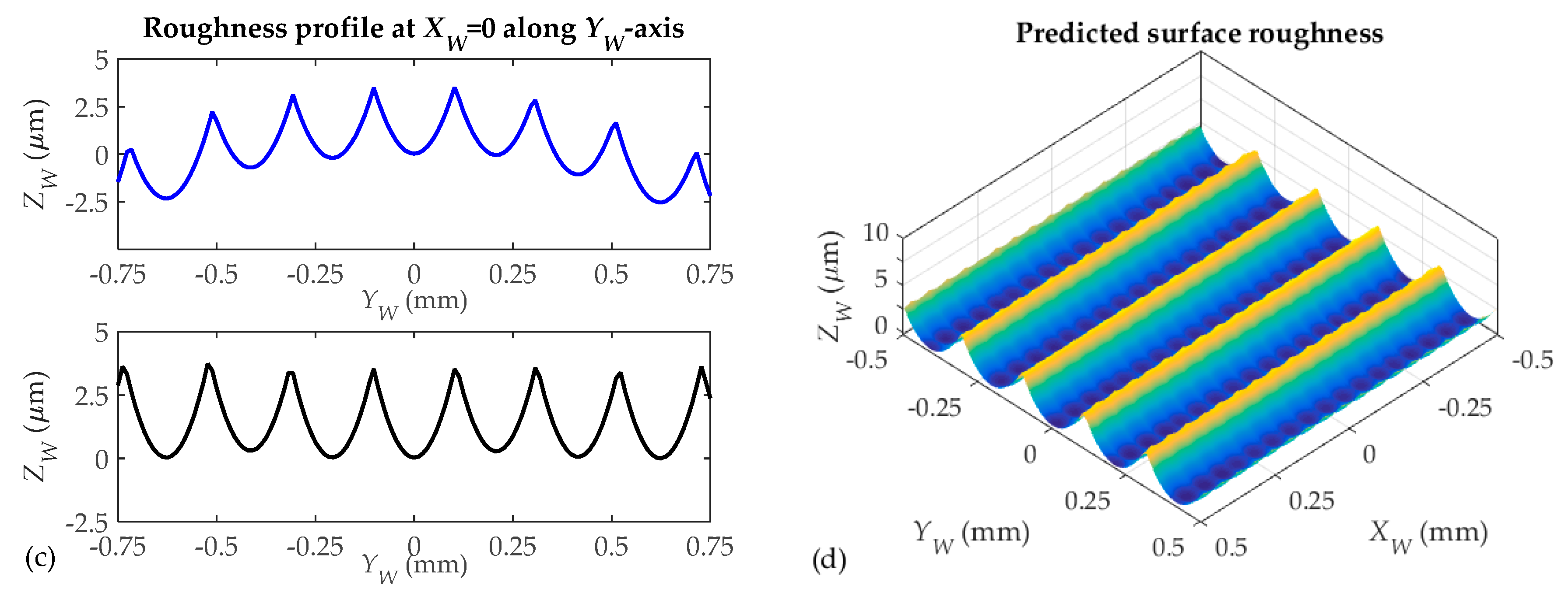
- Surface topographies determination (Figure 11). The surface topography in the gear teeth is obtained from successive point positions of cutting edge points located on the Nt edges with the tool rotating movement. The surface topography is simulated in a rectangular area defined along axes XW and YW, as shown in Figure 7e. This area is divided into a discrete number of planes perpendicular to axes XW and YW. For each plane, taking into account the trajectories of cutting edge points given by Equation (33), the model predicts the area swept by the tool cutting edges during the tool rotation and feed motion. The profile generated at each plane is obtained from the lowest positions of marks left by cutting edge points. By considering the profiles generated in those planes, the 3D surface topography in the rectangular area can be predicted. This allows the profiles generated along axes XW and YW to be analyzed. As an example, Figure 11 shows the surface topography predicted for the simulation area selected in Figure 6b. In Figure 11a, a 3D representation of the predicted surface topography is shown. When the 2D profiles generated at two planes (YW = 0 and XW = 0) are considered (Figure 11b,c), it can be observed that profiles are not only composed of roughness marks left by tool cutting edges. In Figure 11b, the straight sections defining the tool trajectory (red line) can be observed. In Figure 11c, the form of the gear tooth surface can also be observed. In order to analyze the surface roughness, the effect of tool trajectory in each milling pass is removed for each profile predicted along YW-axis. As a consequence, roughness profiles (in black) are obtained. In Figure 11c, the form of the gear surface is also removed from the predicted profile and the roughness profile in black is obtained. In Figure 11d, the predicted surface roughness without the influence of the milling trajectory is shown. It can be observed that the step over between milling passes has a significant influence on the topography and the roughness peak-to-valley values. However, for this case, the effect of tool feed on roughness is less.
4. Model Validation Results
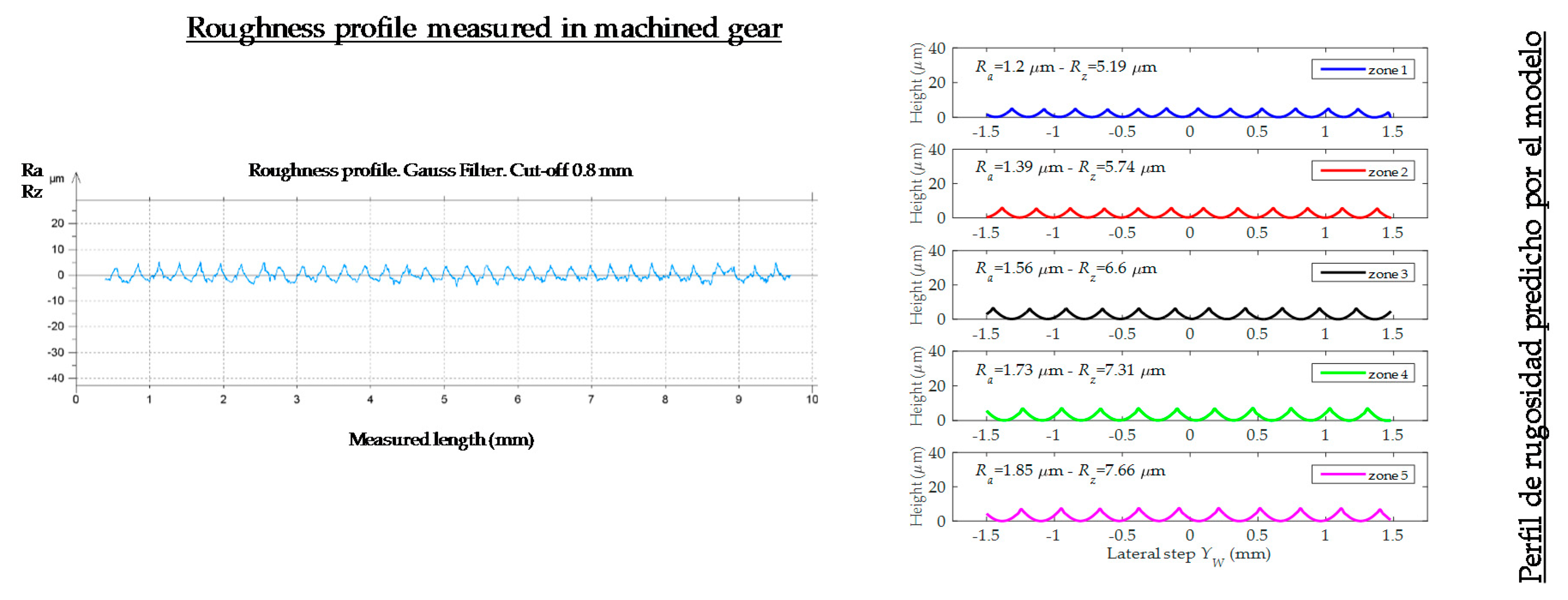
4.1. Gear Roughness Measurement
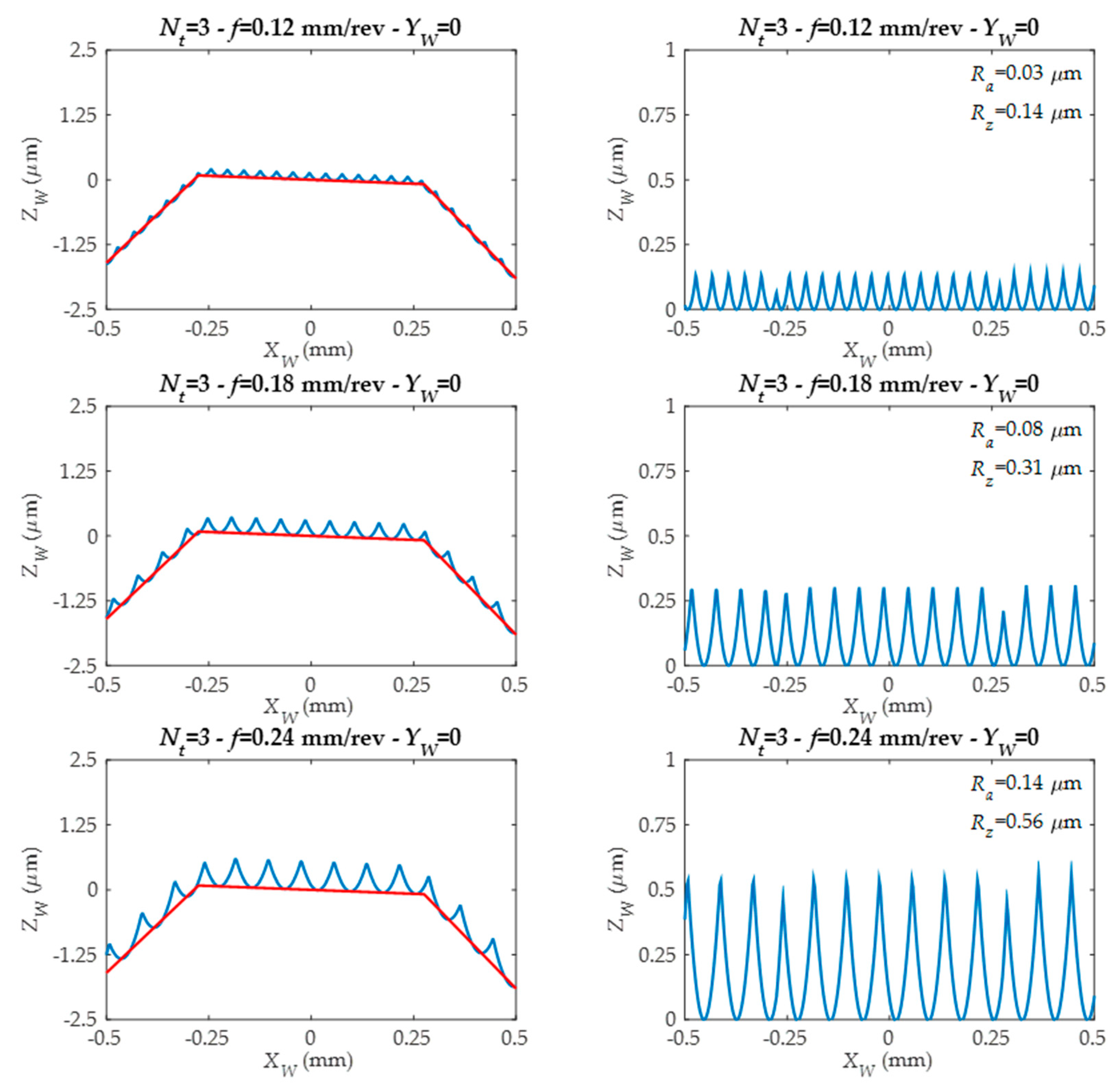
4.2. Gear Roughness Prediction
4.3. Gear Roughness Predictive Model Validation
5. Discussion
6. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Qin, Z.; Luo, Y.; Li, K.; Peng, H. Optimal design of a novel hybrid Electric powertrain for tracked vehicles. Energies 2017, 10, 2141. [Google Scholar] [CrossRef]
- Xu, G.; Hua, D.; Dai, W.; Zhang, X. Design and performance analysis of a coal bed gas drainage machine based on incomplete non-circular gears. Energies 2017, 10, 1933. [Google Scholar] [CrossRef]
- Stadtfeld, H.J. Gleason Bevel Gear Technology: The Science of Gear Engineering and Modern Manufacturing Methods for Angular Transmissions; Gleason Works: Rochester, NY, USA, 2014; ISBN 9780615964928. [Google Scholar]
- Watson, H.J. Gear Milling. In Modern Gear Production; Elsevier: New York, NY, USA, 1970; Chapter 8; pp. 146–158. ISBN 9780080158358. [Google Scholar]
- Lechner, G. Klingelnberg spiral bevel gears. J. Mech. 1968, 3, 217–218. [Google Scholar] [CrossRef]
- Calleja, A.; Gonzalez, H.; Polvorosa, R.; Ortega, N.; Lopez-de-Lacalle, L.N. Multitasking machines: Evolution, resources, processes and scheduling. DYNA 2017, 92, 637–642. [Google Scholar] [CrossRef]
- Klocke, F.; Brumm, M.; Staudt, J. Quality and surface of gears manufactured by free form milling with standard tools. In Proceedings of the International Gear Conference, Lyon, France, 26–28 August 2014; pp. 26–28. [Google Scholar]
- Shih, Y.P.; Sun, Z.H.; Wu, F.C. A disk tool cutting method for bevel gear manufacture on a five-axis machine. Int. J. Adv. Manuf. Technol. 2018, 94, 855–865. [Google Scholar] [CrossRef]
- Suh, S.H.; Jih, W.S.; Hong, H.D.; Chung, D.H. Sculptured surface machining of spiral bevel gears with CNC milling. Int. J. Mach. Tools Manuf. 2001, 41, 833–850. [Google Scholar] [CrossRef]
- Uzun, M. The investigation on manufacturing time of a new type concave-convex gear by a CNC milling machine. Int. J. Adv. Manuf. Technol. 2015, 77, 1275–1280. [Google Scholar] [CrossRef]
- Dudás, I.; Bodzás, S.; Dudás, I.S.; Mándy, Z. Development of spiroid worm gear drive having arched profile in axial section and a new technology of spiroid worm manufacturing with lathe center displacement. Int. J. Adv. Manuf. Technol. 2015, 79, 1881–1892. [Google Scholar] [CrossRef]
- Li, J.B.; Ma, H.J.; Deng, X.Z. An approach to realize the networked closed-loop manufacturing of spiral bevel gears. Int. J. Adv. Manuf. Technol. 2017, 89, 1469. [Google Scholar] [CrossRef]
- Lin, C.; Fan, Y.; Zhang, Z. Additive manufacturing with secondary processing of curve-face gears. Int. J. Adv. Manuf. Technol. 2016, 86, 9–20. [Google Scholar] [CrossRef]
- Li, Z.; Wang, B.; Ma, W. Comparison of ironing finishing and compressing finishing as post-forging for net-shape manufacturing. Int. J. Adv. Manuf. Technol. 2016, 86, 3333–3343. [Google Scholar] [CrossRef]
- Hyatt, G.; Piber, M.A.; Chaphalkar, N.; Kleinhenz, O.; Mori, M. Review of New Strategies for Gear Production. Procedia CIRP 2014, 14, 72–76. [Google Scholar] [CrossRef]
- Kawasaki, K.; Tsuji, I.; Abe, Y.; Gunbara, H. Manufacturing method of large sized spiral bevel gears in cyclo palloid system using multi axis control and multitasking machine tool. In Proceedings of the International Conference on Gears, Garching, Germany, 4–6 October 2012; Volume 1, pp. 337–348. [Google Scholar]
- Bouquet, J.; Hensgen, L.; Klink, A.; Jacobs, T.; Klocke, F.; Lauwers, B. Fast production of gear prototypes—A comparison of technologies. Procedia CIRP 2014, 14, 77–82. [Google Scholar] [CrossRef]
- Tang, J.; Yang, X. Research on manufacturing method of planning for spur face-gear with 4-axis CNC planer. Int. J. Adv. Manuf. Technol. 2016, 82, 847–858. [Google Scholar] [CrossRef]
- Deng, X.; Li, G.; Wei, B.; Deng, J. Face-milling spiral bevel gear tooth surfaces by application of 5-axis CNC machine tool. Int. J. Adv. Manuf. Technol. 2014, 71, 1049–1057. [Google Scholar] [CrossRef]
- Álvarez, A.; Calleja, A.; Ortega, N.; López de Lacalle, LN. Five-Axis Milling of Large Spiral Bevel Gears: Toolpath Definition, Finishing, and Shape Errors. Metals 2018, 8, 353. [Google Scholar] [CrossRef]
- Fuentes, A.A.; Ruiz-Orzaez, R.; Gonzalez-Perez, I. Numerical approach for determination of rough-cutting machine-tool settings for fixed setting face-milled spiral bevel gears. Mech. Mach. Theory 2017, 112, 22–42. [Google Scholar] [CrossRef]
- Litvin, F.L.; Wang, A.G.; Handschu, R.F. Computerized generation and simulation of meshing and contact of spiral bevel gears. Comput. Methods Appl. Mech. Eng. 1998, 158, 35–64. [Google Scholar] [CrossRef]
- Artoni, A.; Gabiccini, M.; Guiggiani, M. Optimization of the loaded contact pattern in hypoid gears by automatic topography modification. ASME J. Mech. Des. 2010, 131, 011008. [Google Scholar] [CrossRef]
- Stadtfeld, H.J.; Gaiser, U. The ultimate motion graph. ASME J. Mech. Des. 2000, 122, 317–322. [Google Scholar] [CrossRef]
- Ding, H.; Tang, J.; Zhou, Y.; Zhong, J.; Wan, G. A multi-objective design for machine setting correction correlating to high tooth contact performance in spiral bevel gears based on nonlinear interval number optimization. Mech. Mach. Theory 2017, 113, 85–108. [Google Scholar] [CrossRef]
- Ding, H.; Tang, J.; Zhong, J.; Zhou, Y. A hybrid modification approach of machine-tool setting considering high tooth contact performance in spiral bevel and hypoid gears. J. Manuf. Syst. 2016, 41, 228–238. [Google Scholar] [CrossRef]
- Bae, I.; Schirru, V. An Approach to Find Optimal Topological Modification to Duplicate Tooth Flank Form of the Existing Gear. In Proceedings of the International Gear Conference, Lyon, France, 26–28 August 2014; pp. 34–43. [Google Scholar]
- Zhou, Y.; Zezhong, C.C.; Tang, J. A New Method of Designing the Tooth Surfaces of Spiral Bevel Gears With Ruled Surface for Their Accurate Five-Axis Flank Milling. J. Manuf. Sci. Eng. 2017, 139, 061004. [Google Scholar] [CrossRef]
- Zhou, Y.; Zezhong, C.C.; Tang, J.; Liu, S. An innovative approach to NC programming for accurate five-axis flank milling of spiral bevel or hypoid gears. Comput. Aided Des. 2017, 84, 15–24. [Google Scholar] [CrossRef]
- Calleja, A.; Bo, P.; González, H.; Bartoň, M.; López de Lacalle, LN. Highly accurate 5-axis flank CNC machining with conical tools. Int. J. Adv. Manuf. Technol. 2018, 97, 1605–1615. [Google Scholar] [CrossRef]
- Litvin, F.L.; Fuentes, A. Gear Geometry and Applied Theory; PTR Prentice Hall: Englewood Cliffs, NJ, USA, 2004. [Google Scholar]
- Artetxe, E.; Olvera, D.; López de Lacalle, L.N.; Campa, F.J.; Olvera, D.; Lamikiz, A. Solid subtraction model for the surface topography prediction in flank milling of thin-walled integral blade rotors (IBRs). Int. J. Adv. Manuf. Technol. 2016, 90, 741–752. [Google Scholar] [CrossRef]
- Xing, Y.; Qin, S.; Wang, T. Study of Subdivision Surface Modelling for Spiral Bevel Gear Manufacturing. In Proceedings of the 6th CIRP-Sponsored International Conference on Digital Enterprise Technology; Springer: Berlin, Germany, 2010; Volume 66. [Google Scholar] [CrossRef]
- Teixeira, J.; Guingand, M.; de Vaujany, JP. Designing and Manufacturing Spiral Bevel Gears Using 5-Axis Computer Numerical Control (CNC) Milling Machines. J. Mech. Des. 2013, 135, 024502. [Google Scholar] [CrossRef]





| Spiral Bevel Gearing Design and Parameters | ||
|---|---|---|
 | Gear heel pitch diameter (Dp) | 200 mm |
| Gear outside diameter (De) | 207.6 mm | |
| Teeth number (Z) | 25 | |
| Spiral angle (β) | 35° | |
| Face angle | 59.5° | |
| Pressure angle (α) | 20° | |
| Roughing Strategy | Teeth Number | Tool | |
|---|---|---|---|
| R-I | Cavity mill (3 + 1-axis) Follow periphery | 1–25 | Frontal mill Ø4 mm |
| R-II | Variable contour (5-axis) Stream line | 1–25 | Conical mill Ø3 mm |
| Finishing Strategy | Teeth Number | Tool | |
|---|---|---|---|
| F-I | Surface area + Relative vector (3 + 2 axes) Cut pattern: ZIG (1–6) ZIG/ZAG(7–12) (Scallop 0.01–0.02–0.03 mm) | 1–12 | Conical mill Ø3 mm 8° Taper Ball nose TiAlN Number of flutes3 Helix angle 45° |
| F-II | Surface area + Relative vector (5 axes) Cut pattern: ZIG (13–18) ZIG/ZAG(19–25) (Scallop 0.01–0.02–0.03 mm) | 13–25 | |
| Teeth Number | Machining | Strategy | Scallops (mm) | Measured Roughness Values | |
|---|---|---|---|---|---|
| Ra (µm) | Rz (µm) | ||||
| 1 | 3 + 2 AXES | ZIG | 0.01 | 1.68 | 8.64 |
| 2 | 3 + 2 AXES | ZIG | 0.01 | ||
| 3 | 3 + 2 AXES | ZIG | 0.02 | 2.72 | 13.03 |
| 4 | 3 + 2 AXES | ZIG | 0.02 | ||
| 5 | 3 + 2 AXES | ZIG | 0.03 | 4.66 | 20.83 |
| 6 | 3 + 2 AXES | ZIG | 0.03 | ||
| 7 | 3 + 2 AXES | ZIG-ZAG | 0.01 | 1.91 | 10.12 |
| 8 | 3 + 2 AXES | ZIG-ZAG | 0.01 | ||
| 9 | 3 + 2 AXES | ZIG-ZAG | 0.02 | 2.71 | 15.43 |
| 10 | 3 + 2 AXES | ZIG-ZAG | 0.02 | ||
| 11 | 3 + 2 AXES | ZIG-ZAG | 0.03 | 4.83 | 22.20 |
| 12 | 3 + 2 AXES | ZIG-ZAG | 0.03 | ||
| 13 | 5 AXES | ZIG | 0.01 | 1.34 | 7.78 |
| 14 | 5 AXES | ZIG | 0.01 | ||
| 15 | 5 AXES | ZIG | 0.02 | 2.62 | 13.44 |
| 16 | 5 AXES | ZIG | 0.02 | ||
| 17 | 5 AXES | ZIG | 0.03 | 4.58 | 19.63 |
| 18 | 5 AXES | ZIG | 0.03 | ||
| 19 | 5 AXES | ZIG-ZAG | 0.01 | 4.24 | 20.03 |
| 20 | 5 AXES | ZIG-ZAG | 0.01 | ||
| 21 | 5 AXES | ZIG-ZAG | 0.02 | 6.86 | 31.62 |
| 22 | 5 AXES | ZIG-ZAG | 0.02 | ||
| 23 | 5 AXES | ZIG-ZAG | 0.02 | ||
| 24 | 5 AXES | ZIG-ZAG | 0.03 | 7.68 | 35.63 |
| 25 | 5 AXES | ZIG-ZAG | 0.03 | ||
| Teeth N° | Machining | Strategy | Scallop (mm) | Zone 1 | Zone 2 | Zone 3 | Zone 4 | Zone 5 | |||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Ra (µm) | Rz (µm) | Ra (µm) | Rz (µm) | Ra (µm) | Rz (µm) | Ra (µm) | Rz (µm) | Ra (µm) | Rz (µm) | ||||
| 1, 2 | 3 + 2 AXES | ZIG | 0.01 | 1.2 | 5.19 | 1.39 | 5.74 | 1.56 | 6.6 | 1.73 | 7.31 | 1.85 | 7.66 |
| 2.1 | 9 | 1.89 | 8.68 | 1.65 | 7.92 | 1.41 | 6.92 | 1.18 | 6.72 | ||||
| 3, 4 | 3 + 2 AXES | ZIG | 0.02 | 2.44 | 10.43 | 2.8 | 11.75 | 3.27 | 13.5 | 3.49 | 15.38 | 3.89 | 16.42 |
| 4.33 | 18.71 | 3.82 | 16.37 | 3.39 | 14.47 | 2.88 | 13.15 | 2.36 | 11.1 | ||||
| 5, 6 | 3 + 2 AXES | ZIG | 0.03 | 3.72 | 16.39 | 4.31 | 18.6 | 4.93 | 20.16 | 5.42 | 22.35 | 5.7 | 24.45 |
| 5.96 | 26.13 | 5.57 | 23.29 | 4.71 | 20.94 | 3.97 | 17.4 | 3.28 | 14.62 | ||||
| 7, 8 | 3 + 2 AXES | ZIG-ZAG | 0.01 | 1.19 | 5.16 | 1.35 | 5.77 | 1.65 | 9.29 | 1.66 | 7.07 | 1.83 | 7.75 |
| 2.2 | 9.58 | 1.93 | 8.63 | 8.63 | 7.89 | 1.46 | 7.3 | 1.19 | 6.2 | ||||
| 9, 10 | 3 + 2 AXES | ZIG-ZAG | 0.02 | 2.28 | 9.8 | 2.63 | 11.44 | 11.44 | 14.38 | 3.34 | 17.21 | 3.47 | 15.01 |
| 4.32 | 18.36 | 3.86 | 16.5 | 16.5 | 14.43 | 2.9 | 12.7 | 2.36 | 10.53 | ||||
| 11, 12 | 3 + 2 AXES | ZIG-ZAG | 0.03 | 3.41 | 14.75 | 3.96 | 16.95 | 16.95 | 19.71 | 5.07 | 20.07 | 5.35 | 19.98 |
| 6.17 | 27.68 | 5.58 | 24.67 | 24.67 | 21.78 | 4.15 | 18.08 | 3.32 | 14.68 | ||||
| 13, 14 | 5 AXES | ZIG | 0.01 | 1.11 | 4.63 | 1.3 | 5.35 | 1.48 | 5.97 | 1.62 | 6.67 | 1.82 | 7.34 |
| 2.3 | 9.68 | 2.19 | 8.93 | 1.93 | 8.21 | 1.68 | 7.42 | 1.46 | 6.52 | ||||
| 15, 16 | 5 AXES | ZIG | 0.02 | 2.25 | 9.2 | 2.59 | 10.52 | 2.95 | 11.93 | 3.32 | 13.39 | 3.6 | 14.66 |
| 4.92 | 19.95 | 4.51 | 18.28 | 4.06 | 16.59 | 3.54 | 15.13 | 3.15 | 13.45 | ||||
| 17, 18 | 5 AXES | ZIG | 0.03 | 3.43 | 14.42 | 4.05 | 16.66 | 4.6 | 18.75 | 5.3 | 21.1 | 5.49 | 22.9 |
| 7.16 | 29.17 | 6.56 | 26.78 | 5.84 | 25.04 | 1.58 | 23.39 | 4.89 | 20.33 | ||||
| 19, 20 | 5 AXES | ZIG-ZAG | 0.01 | 1.06 | 4.61 | 1.24 | 5.5 | 1.41 | 6.23 | 1.58 | 6.59 | 1.72 | 7.12 |
| 2.5 | 9.61 | 2.22 | 8.63 | 2.02 | 7.67 | 1.92 | 7.35 | 1.59 | 6.37 | ||||
| 21, 22, 23 | 5 AXES | ZIG-ZAG | 0.02 | 2.22 | 8.34 | 2.47 | 9.59 | 2.86 | 11.05 | 3.25 | 12.44 | 3.43 | 13.34 |
| 5.16 | 20.07 | 4.61 | 17.6 | 4.25 | 16.78 | 3.77 | 15.46 | 3.36 | 13.91 | ||||
| 24, 25 | 5 AXES | ZIG-ZAG | 0.03 | 3.2 | 11.76 | 3.71 | 13.62 | 4.27 | 16.91 | 4.87 | 19.54 | 5.37 | 20.01 |
| 7.35 | 30.22 | 6.72 | 27.63 | 5.91 | 25.06 | 5.5 | 22.63 | 4.76 | 19.97 | ||||
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Álvarez, Á.; Calleja, A.; Arizmendi, M.; González, H.; Lopez de Lacalle, L.N. Spiral Bevel Gears Face Roughness Prediction Produced by CNC End Milling Centers. Materials 2018, 11, 1301. https://doi.org/10.3390/ma11081301
Álvarez Á, Calleja A, Arizmendi M, González H, Lopez de Lacalle LN. Spiral Bevel Gears Face Roughness Prediction Produced by CNC End Milling Centers. Materials. 2018; 11(8):1301. https://doi.org/10.3390/ma11081301
Chicago/Turabian StyleÁlvarez, Álvaro, Amaia Calleja, Mikel Arizmendi, Haizea González, and Luis Norberto Lopez de Lacalle. 2018. "Spiral Bevel Gears Face Roughness Prediction Produced by CNC End Milling Centers" Materials 11, no. 8: 1301. https://doi.org/10.3390/ma11081301
APA StyleÁlvarez, Á., Calleja, A., Arizmendi, M., González, H., & Lopez de Lacalle, L. N. (2018). Spiral Bevel Gears Face Roughness Prediction Produced by CNC End Milling Centers. Materials, 11(8), 1301. https://doi.org/10.3390/ma11081301








