Nonlinear Buckling Analysis of Functionally Graded Graphene Reinforced Composite Shallow Arches with Elastic Rotational Constraints under Uniform Radial Load
Abstract
:1. Introduction
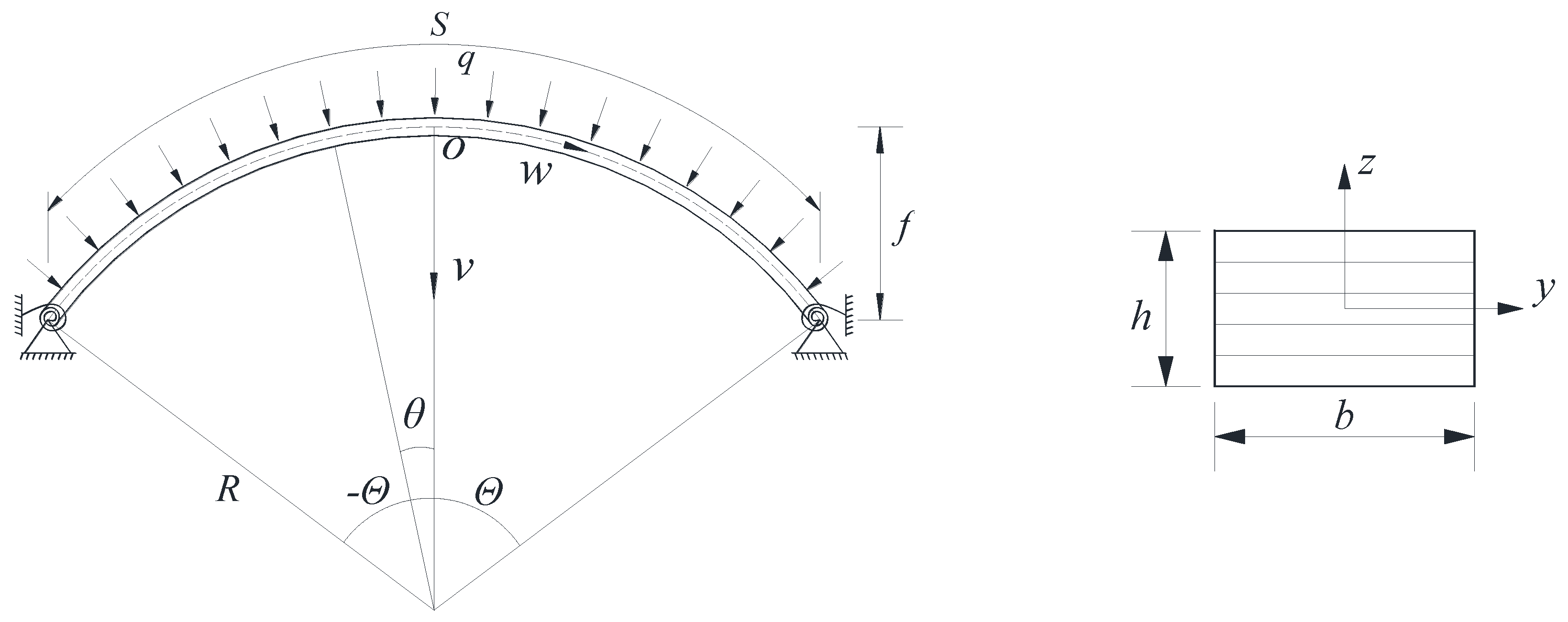
2. Materials Model of FG-GPLRC Shallow Arches
3. Nonlinear Equilibrium Equation
4. Buckling Analysis
4.1. Limit Instability Buckling
4.2. Bifurcation Buckling
5. Comparison and Discussion
5.1. Effect of the Number of Nanocomposite Layers on the Numerical Results
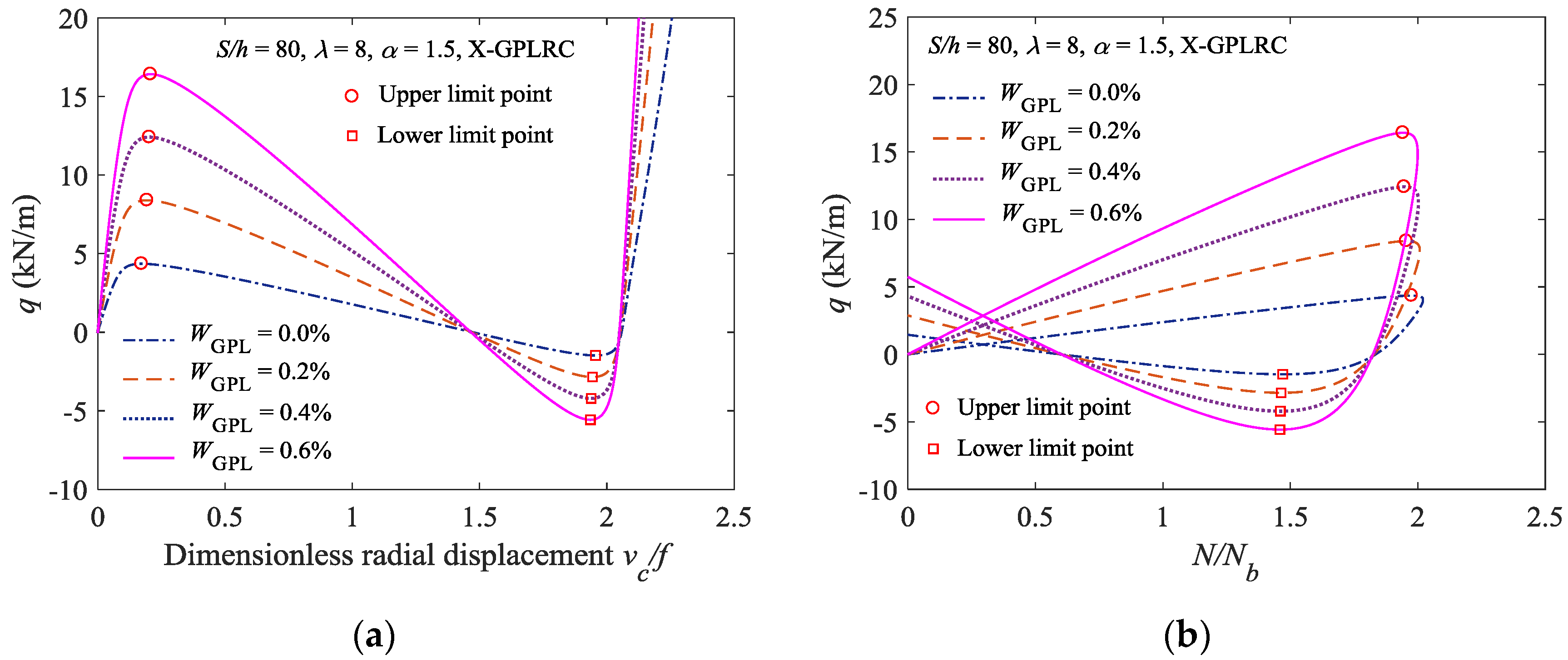
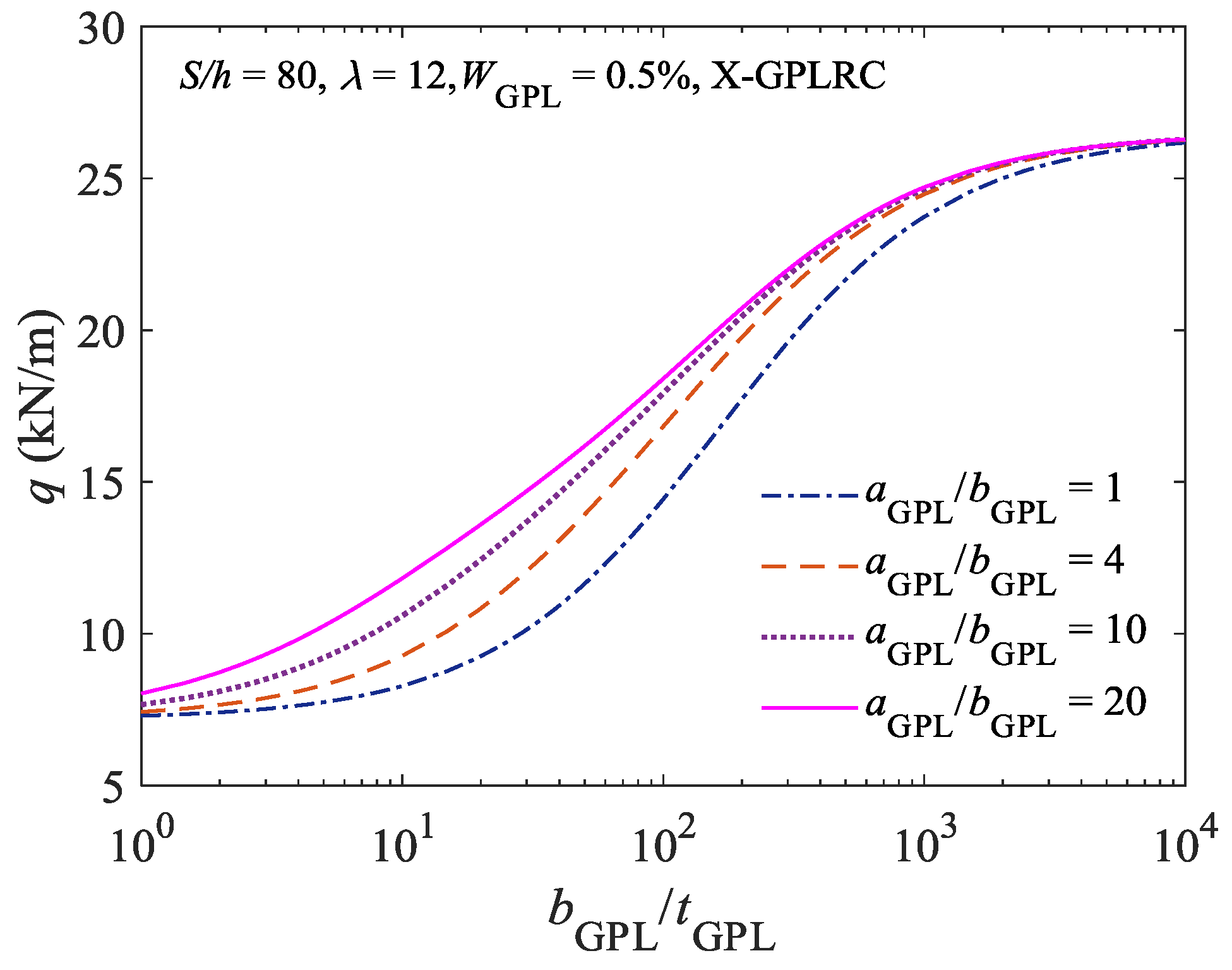
5.2. Limit Point Buckling Analysis
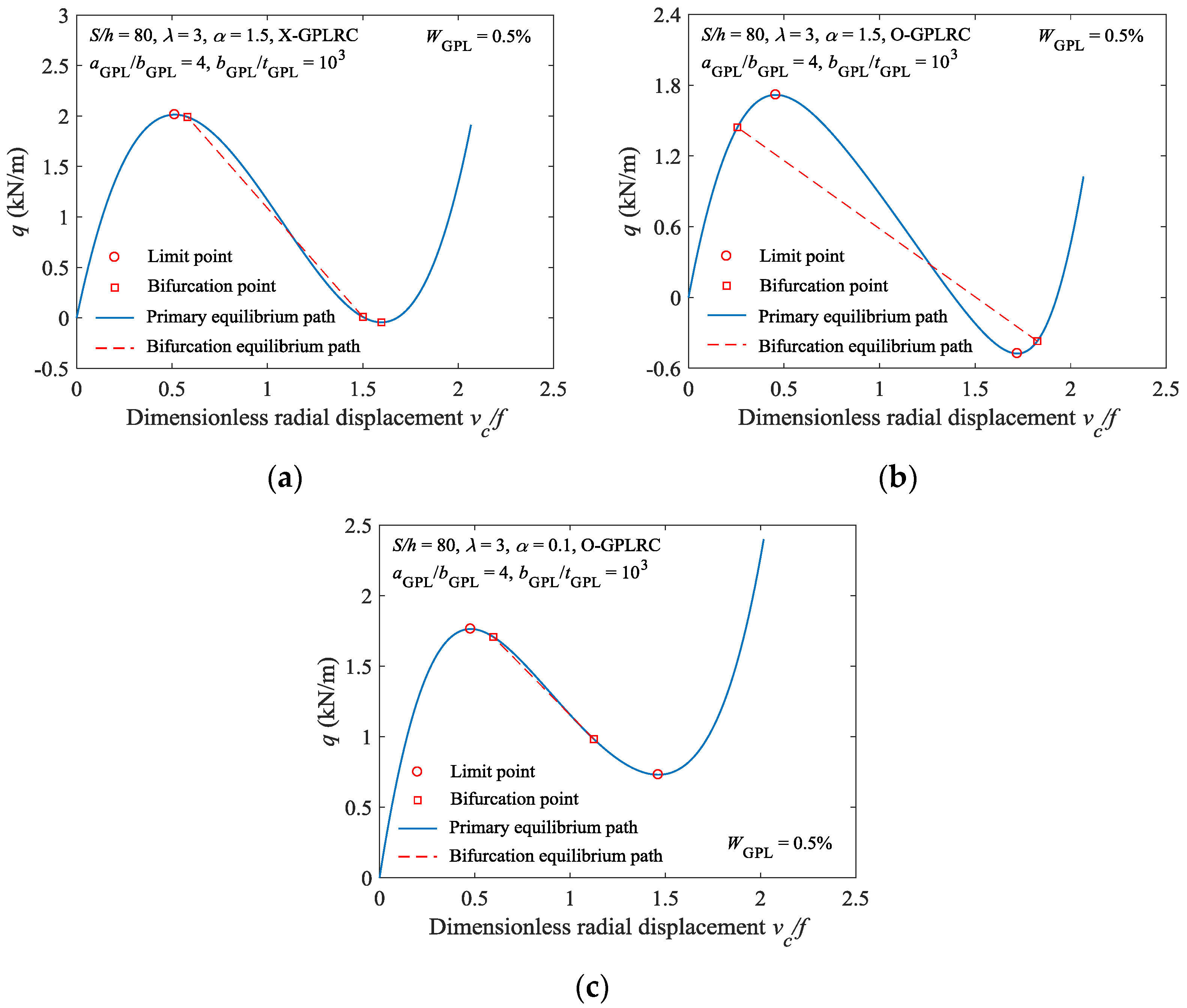
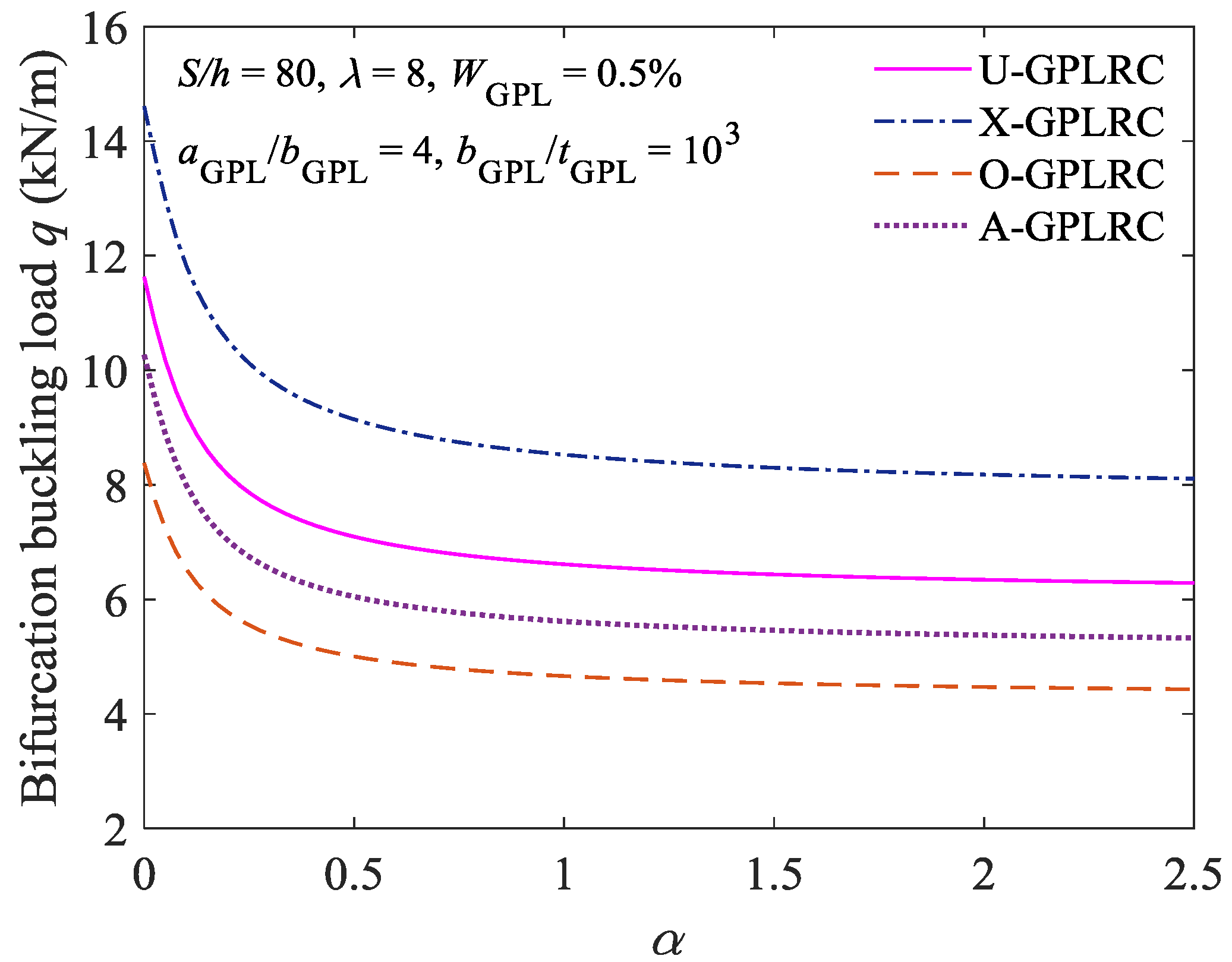
5.3. Bifurcation Buckling Analysis
6. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Strankowski, M.; Korzeniewski, P.; Strankowska, J.; Anu, A.S.; Thomas, S. Morphology, mechanical and thermal properties of thermoplastic polyurethane containing reduced graphene oxide and graphene nanoplatelets. Materials 2018, 11, 82. [Google Scholar] [CrossRef] [PubMed]
- Gigot, A.; Fontana, M.; Pirri, C.F.; Rivolo, P. Graphene/ruthenium active species aerogel as electrode for supercapacitor applications. Materials 2018, 11, 57. [Google Scholar] [CrossRef] [PubMed]
- Wang, L.; Wang, W.; Fu, Y.; Wang, J.; Lvov, Y.; Liu, J.; Lu, Y.; Zhang, L. Enhanced electrical and mechanical properties of rubber/graphene film through layer-by-layer electrostatic assembly. Compos. Part B Eng. 2016, 90, 457–464. [Google Scholar] [CrossRef]
- Xu, W.; Wang, L.F.; Jiang, J.N. Finite element analysis of strain gradient middle thick plate model on the vibration of graphene sheets. Chin. J. Theor. Appl. Mech. 2015, 47, 751–761. (In Chinese) [Google Scholar]
- Lee, C.; Wei, X.; Kysar, J.W.; Hone, J. Measurement of the elastic properties and intrinsic strength of monolayer graphene. Science 2008, 321, 385–388. [Google Scholar] [CrossRef] [PubMed]
- Rafiee, M.A.; Rafiee, J.; Wang, Z.; Song, H.; Yu, Z.Z.; Koratkar, N. Enhanced mechanical properties of nanocomposites at low graphene content. ACS Nano 2009, 3, 3884–3890. [Google Scholar] [CrossRef] [PubMed]
- Rafiee, M.A.; Rafiee, J.; Srivastava, I.; Wang, Z.; Song, H.; Yu, Z.Z. Fracture and fatigue in graphene nanocomposites. Small 2010, 6, 179–183. [Google Scholar] [CrossRef] [PubMed]
- Liu, J.; Yan, H.; Jiang, K. Mechanical properties of graphene platelet-reinforced alumina ceramic composites. Ceram. Int. 2013, 39, 6215–6221. [Google Scholar] [CrossRef]
- Liu, J.; Li, Z.; Yan, H.; Jiang, K. Spark plasma sintering of alumina composites with graphene platelets and silicon carbide nanoparticles. Adv. Eng. Mater. 2014, 16, 1111–1118. [Google Scholar] [CrossRef]
- Ji, X.Y.; Cao, Y.P.; Feng, X.Q. Micromechanics prediction of the effective elastic moduli of graphene sheet-reinforced polymer nanocomposites. Model. Simul. Mater. Sci. Eng. 2010, 18, 045005. [Google Scholar] [CrossRef]
- Zhao, X.; Zhang, Q.; Chen, D.; Lu, P. Enhanced mechanical properties of graphene-based poly(vinyl alcohol) composites. Macromolecules 2010, 43, 2357–2363. [Google Scholar] [CrossRef]
- Shen, H.S. Nonlinear bending of functionally graded carbon nanotube-reinforced composite plates in thermal environments. Compos. Struct. 2009, 91, 9–19. [Google Scholar] [CrossRef]
- Yang, J.; Wu, H.; Kitipornchai, S. Buckling and postbuckling of functionally graded multilayer graphene platelet-reinforced composite beams. Compos. Struct. 2017, 161, 111–118. [Google Scholar] [CrossRef]
- Feng, C.; Kitipornchai, S.; Yang, J. Nonlinear bending of polymer nanocomposite beams reinforced with non-uniformly distributed graphene platelets (GPLS). Compos. Part B Eng. 2017, 110, 132–140. [Google Scholar] [CrossRef]
- Song, M.; Kitipornchai, S.; Yang, J. Free and forced vibrations of functionally graded polymer composite plates reinforced with graphene nanoplatelets. Compos. Struct. 2016, 159, 579–588. [Google Scholar] [CrossRef]
- Song, M.T.; Yang, J.; Kitipornchai, S.; Song, M.T.; Yang, J.; Kitipornchai, S. Bending and buckling analyses of functionally graded polymer composite plates reinforced with graphene nanoplatelets. Compos. Part B Eng. 2018, 134, 106–113. [Google Scholar] [CrossRef]
- Feng, C.; Kitipornchai, S.; Yang, J. Nonlinear free vibration of functionally graded polymer composite beams reinforced with graphene nanoplatelets (GPLS). Eng. Struct. 2017, 140, 110–119. [Google Scholar] [CrossRef]
- Bateni, M.; Eslami, M.R. Non-linear in-plane stability analysis of fg circular shallow arches under uniform radial pressure. Thin Wall Struct. 2015, 94, 302–313. [Google Scholar] [CrossRef]
- Bateni, M.; Eslami, M.R. Non-linear in-plane stability analysis of fgm circular shallow arches under central concentrated force. Int. J. Nonlinear Mech. 2014, 60, 58–69. [Google Scholar] [CrossRef]
- Pi, Y.L.; Bradford, M.A.; Tin-Loi, F. Nonlinear analysis and buckling of elastically supported circular shallow arches. Int. J. Solids. Struct. 2007, 44, 2401–2425. [Google Scholar] [CrossRef]
- Pi, Y.L.; Bradford, M.A.; Tin-Loi, F. Non-linear in-plane buckling of rotationally restrained shallow arches under a central concentrated load. Int. J. Solids. Struct. 2008, 43, 1–17. [Google Scholar] [CrossRef]
- Pi, Y.L.; Bradford, M.A. Non-linear in-plane postbuckling of arches with rotational end restraints under uniform radial loading. Int. J. Nonlinear Mech. 2009, 44, 975–989. [Google Scholar] [CrossRef]
- Zeng, Y.; Yi, Z.; Yang, D. Analysis of buckling for a circular arch with elastic rotational constraints under a central concentrated load. Chin. J. Theor. Appl. Mech. 2016, 33, 904–909. (In Chinese) [Google Scholar]
- Liu, A.; Lu, H.; Fu, J.; Pi, Y.L. Lateral-torsional buckling of circular steel arches under arbitrary radial concentrated load. J. Struct. Eng. 2017, 143, 04017129. [Google Scholar] [CrossRef]
- Liu, A.R.; Huang, Y.H.; Fu, J.Y.; Yu, Q.C.; Rao, R. Experimental research on stable ultimate bearing capacity of leaning-type arch rib systems. J. Constr. Steel Res. 2015, 114, 281–292. [Google Scholar] [CrossRef]
- Liu, A.R.; Lu, H.W.; Fu, J.Y.; Pi, Y.L. Lateral-torsional buckling of fixed circular arches having a thin-walled section under a central concentrated load. Thin Wall Struct. 2017, 118, 46–55. [Google Scholar] [CrossRef]
- Liu, A.R.; Bradford, M.A.; Pi, Y.L. In-plane nonlinear multiple equilibria and switches of equilibria of pinned–fixed arches under an arbitrary radial concentrated load. Arch. Appl. Mech. 2017, 87, 1909–1928. [Google Scholar] [CrossRef]
- Liu, A.R.; Yang, Z.C.; Bradford, M.A.; Pi, Y.L. Nonlinear dynamic buckling of fixed shallow arches under an arbitrary step radial point load. J. Eng. Mech. 2018, 144, 04018012. [Google Scholar] [CrossRef]
- Pi, Y.L.; Bradford, M.A. Lateral–torsional elastic buckling of rotationally restrained arches with a thin-walled section under a central concentrated load. Thin Wall Struct. 2013, 73, 18–26. [Google Scholar] [CrossRef]
- Pi, Y.L.; Bradford, M.A. Lateral-torsional buckling analysis of arches having in-plane rotational end restraints under uniform radial loading. J. Eng. Mech. 2013, 139, 1602–1609. [Google Scholar] [CrossRef]
- Pi, Y.L.; Bradford, M.A.; Liu, A.R. Nonlinear equilibrium and buckling of fixed shallow arches subjected to an arbitrary radial concentrated load. Int. J. Struct. Stab. Dyn. 2017, 17, 1750082. [Google Scholar] [CrossRef]
- Affdl, J.C.H.; Kardos, J.L. The halpin-tsai equations: A review. Polym. Eng. Sci. 1976, 16, 344–352. [Google Scholar] [CrossRef]
- Yi, X.S. An Introduction to Composite Materials. Concise Encycl. Compos. Mater. 2018, 2, 44–49. [Google Scholar]
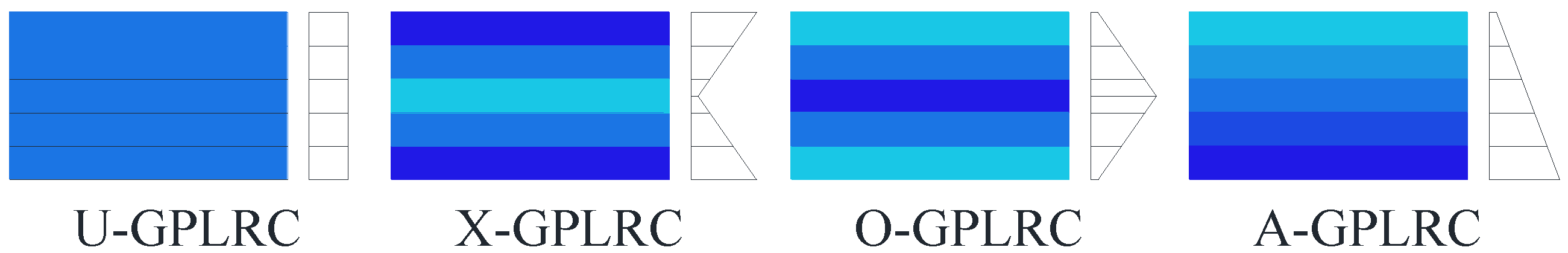


| NL | Limit Point Buckling | Bifurcation Buckling |
|---|---|---|
| 4 | 13.823 | 7.776 |
| 6 | 14.218 | 8.138 |
| 10 | 14.419 | 8.175 |
| 20 | 14.503 | 8.228 |
| 1000 | 14.531 | 8.247 |
| α | U | X | O | A | Pure |
|---|---|---|---|---|---|
| 0 | 22.836 | 28.202 | 16.941 | 20.406 | 8.584 |
| 1.5 | 18.952 | 24.110 | 13.580 | 16.873 | 7.124 |
| ∞ | 18.764 | 23.913 | 13.418 | 16.158 | 7.053 |
| Patterns | U | X | O | A |
|---|---|---|---|---|
| λb | 2.3584 | 2.6912 | 1.9702 | 2.4167 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Huang, Y.; Yang, Z.; Liu, A.; Fu, J. Nonlinear Buckling Analysis of Functionally Graded Graphene Reinforced Composite Shallow Arches with Elastic Rotational Constraints under Uniform Radial Load. Materials 2018, 11, 910. https://doi.org/10.3390/ma11060910
Huang Y, Yang Z, Liu A, Fu J. Nonlinear Buckling Analysis of Functionally Graded Graphene Reinforced Composite Shallow Arches with Elastic Rotational Constraints under Uniform Radial Load. Materials. 2018; 11(6):910. https://doi.org/10.3390/ma11060910
Chicago/Turabian StyleHuang, Yonghui, Zhicheng Yang, Airong Liu, and Jiyang Fu. 2018. "Nonlinear Buckling Analysis of Functionally Graded Graphene Reinforced Composite Shallow Arches with Elastic Rotational Constraints under Uniform Radial Load" Materials 11, no. 6: 910. https://doi.org/10.3390/ma11060910
APA StyleHuang, Y., Yang, Z., Liu, A., & Fu, J. (2018). Nonlinear Buckling Analysis of Functionally Graded Graphene Reinforced Composite Shallow Arches with Elastic Rotational Constraints under Uniform Radial Load. Materials, 11(6), 910. https://doi.org/10.3390/ma11060910








