Compressive Behavior and Constitutive Model of Austenitic Stainless Steel S30403 in High Strain Range
Abstract
:1. Introduction
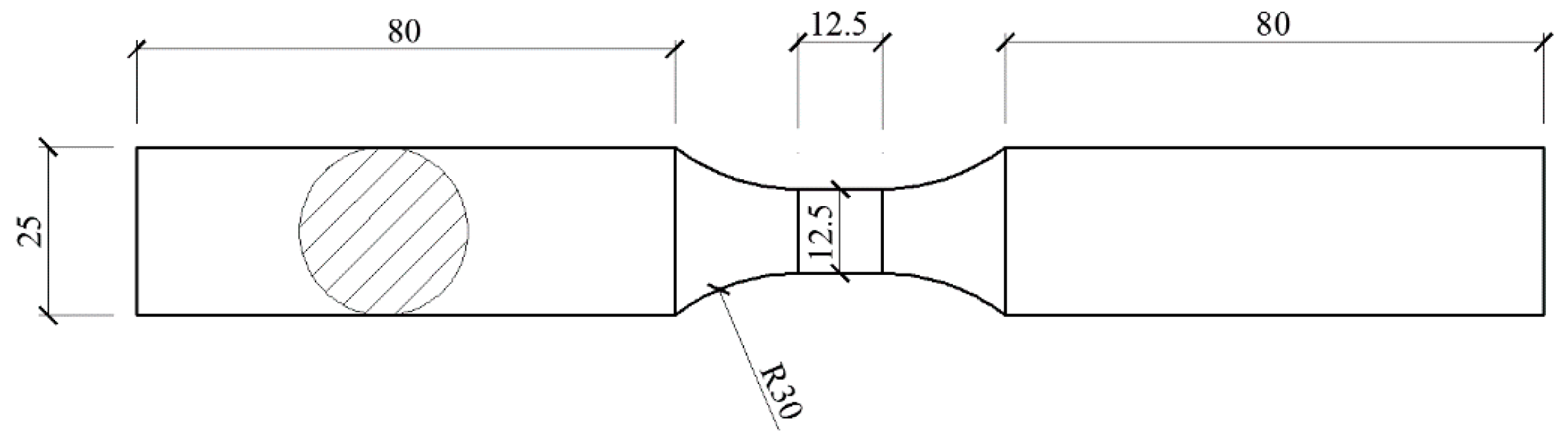
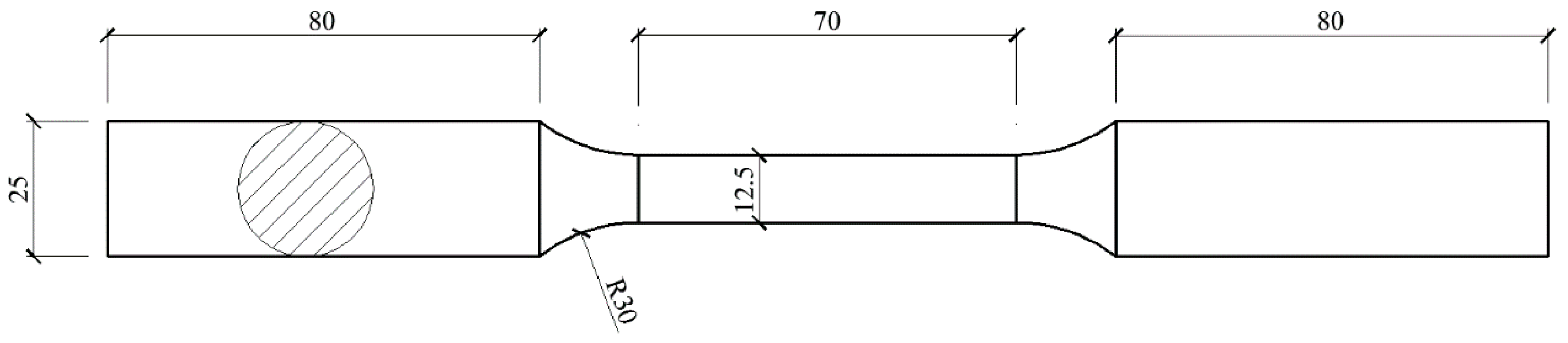
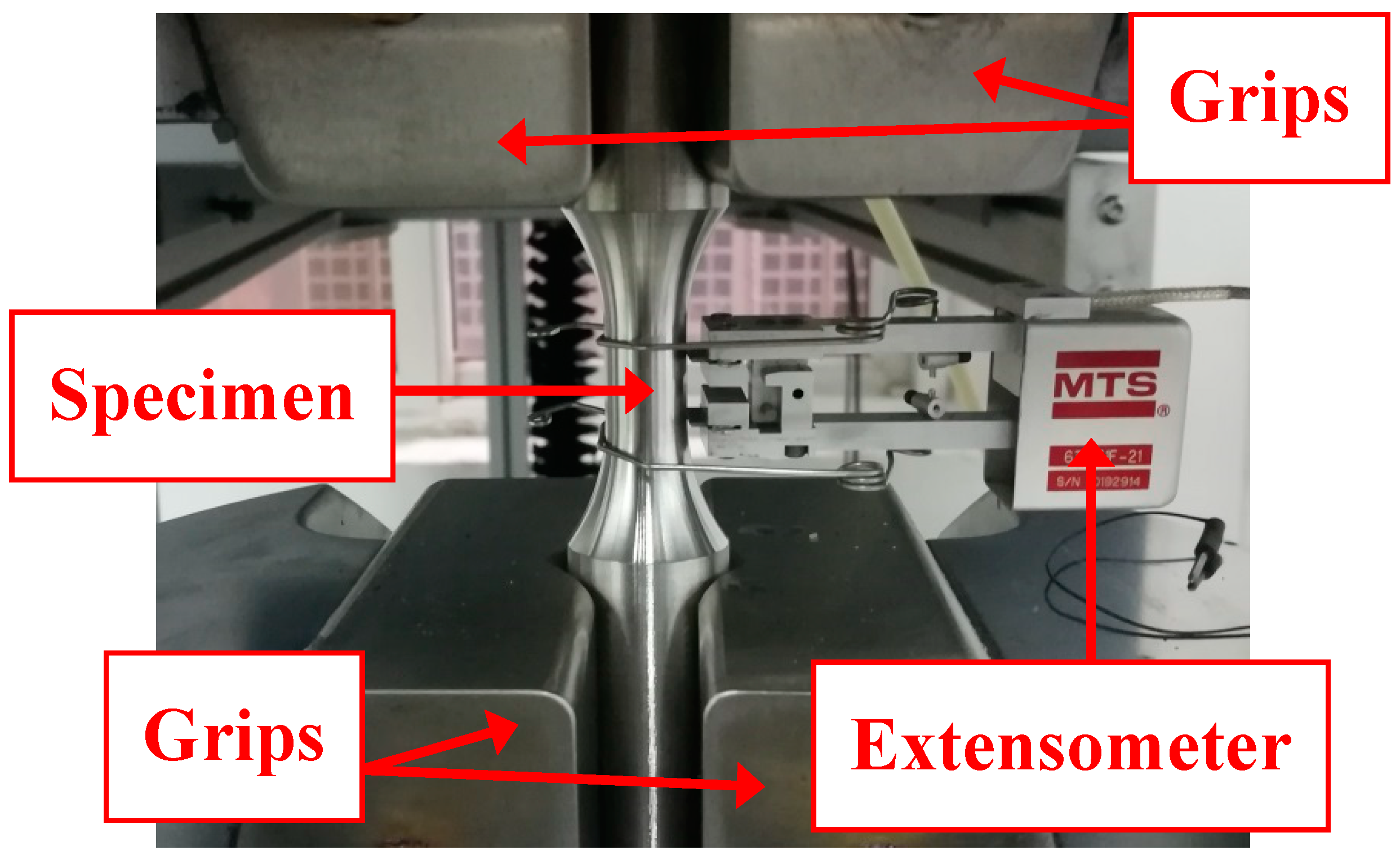
2. Experimental Details
3. Results and Discussion
3.1. Comparison of Tensile Test Results
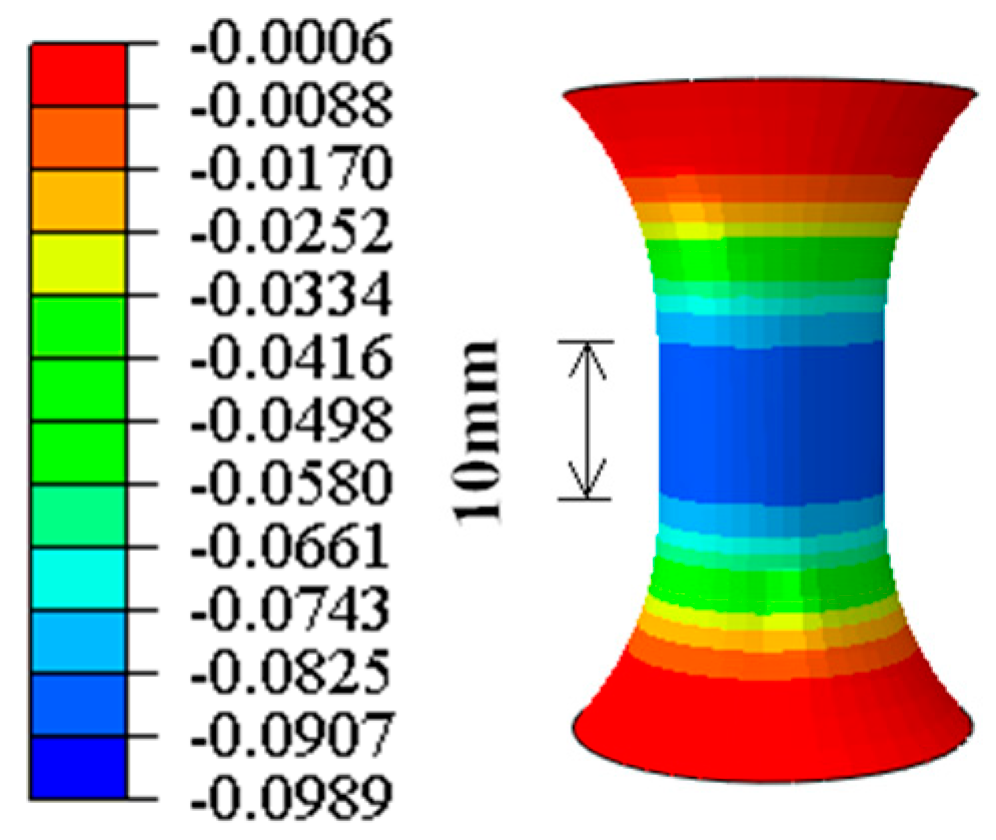
3.2. Compression Test
3.3. Compressive Constitutive Model of Austenite Stainless Steel
3.3.1. Constitutive Models
3.3.2. Reliability of Constitutive Models
4. Conclusions
- (1)
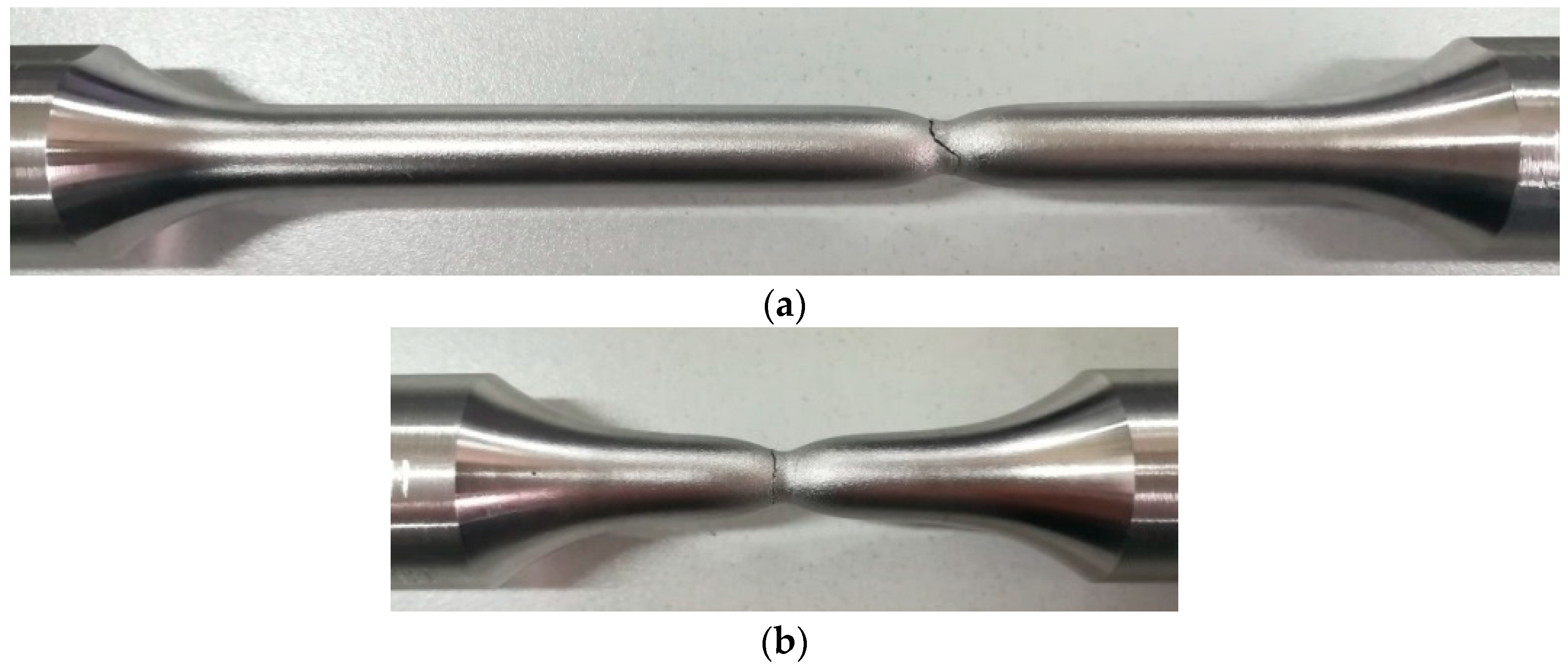
- When the length-to-diameter ratio of the specimen is 1:1, the specimens do not buckle under high compressive strain of 10%. The small-gauge-length specimen with length-to-diameter ratio 1:1 is suitable for compression experiment in the high strain range.
- (2)
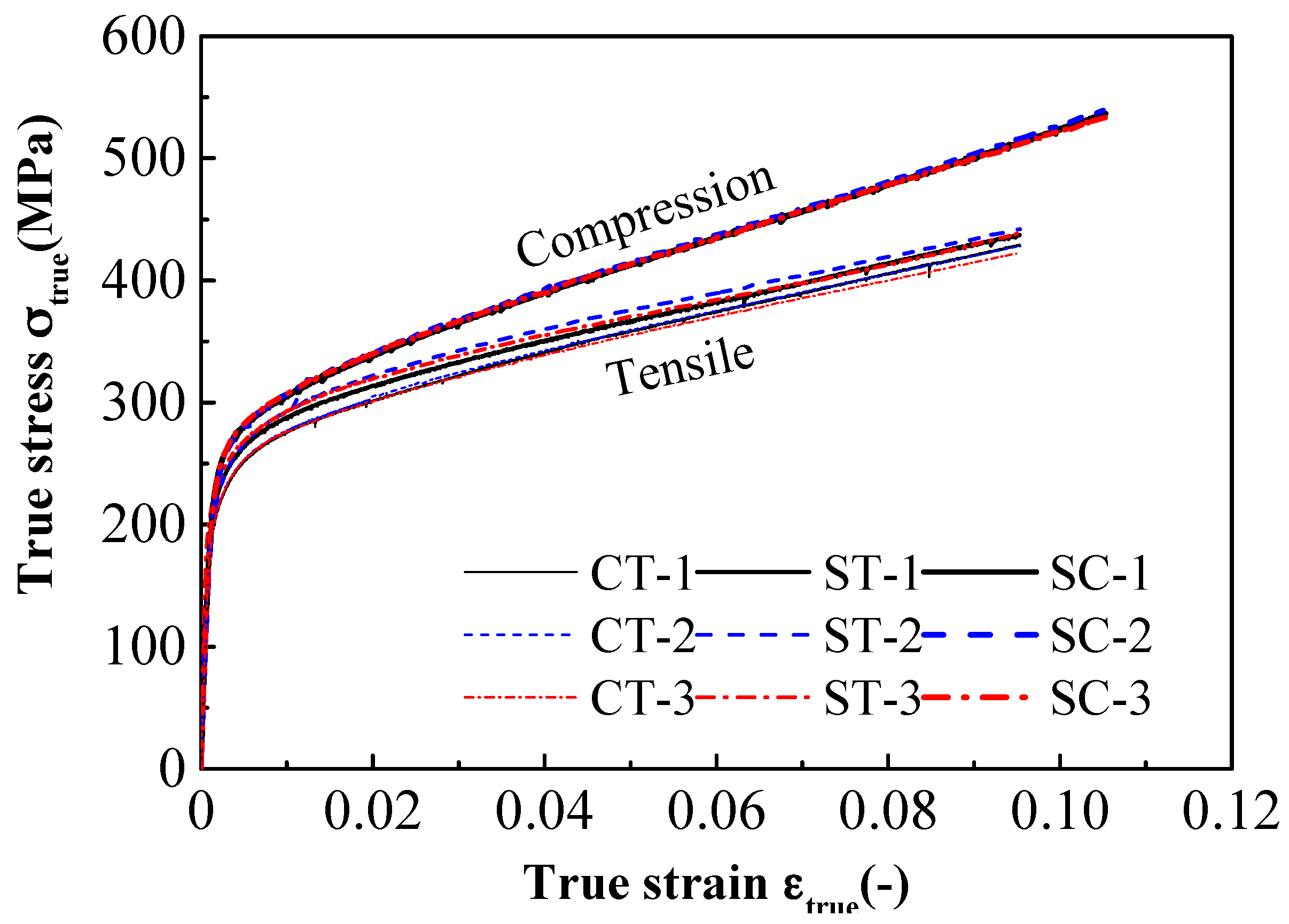
- There is an obvious material anisotropy of the stress-strain response in the tension and compression of austenitic stainless steel under high strain conditions up to 10%. The compressive nominal yield strength is higher than the nominal tensile yield strength. The compressive initial Young’s modulus is close to the tensile initial Young’s modulus.
- (3)
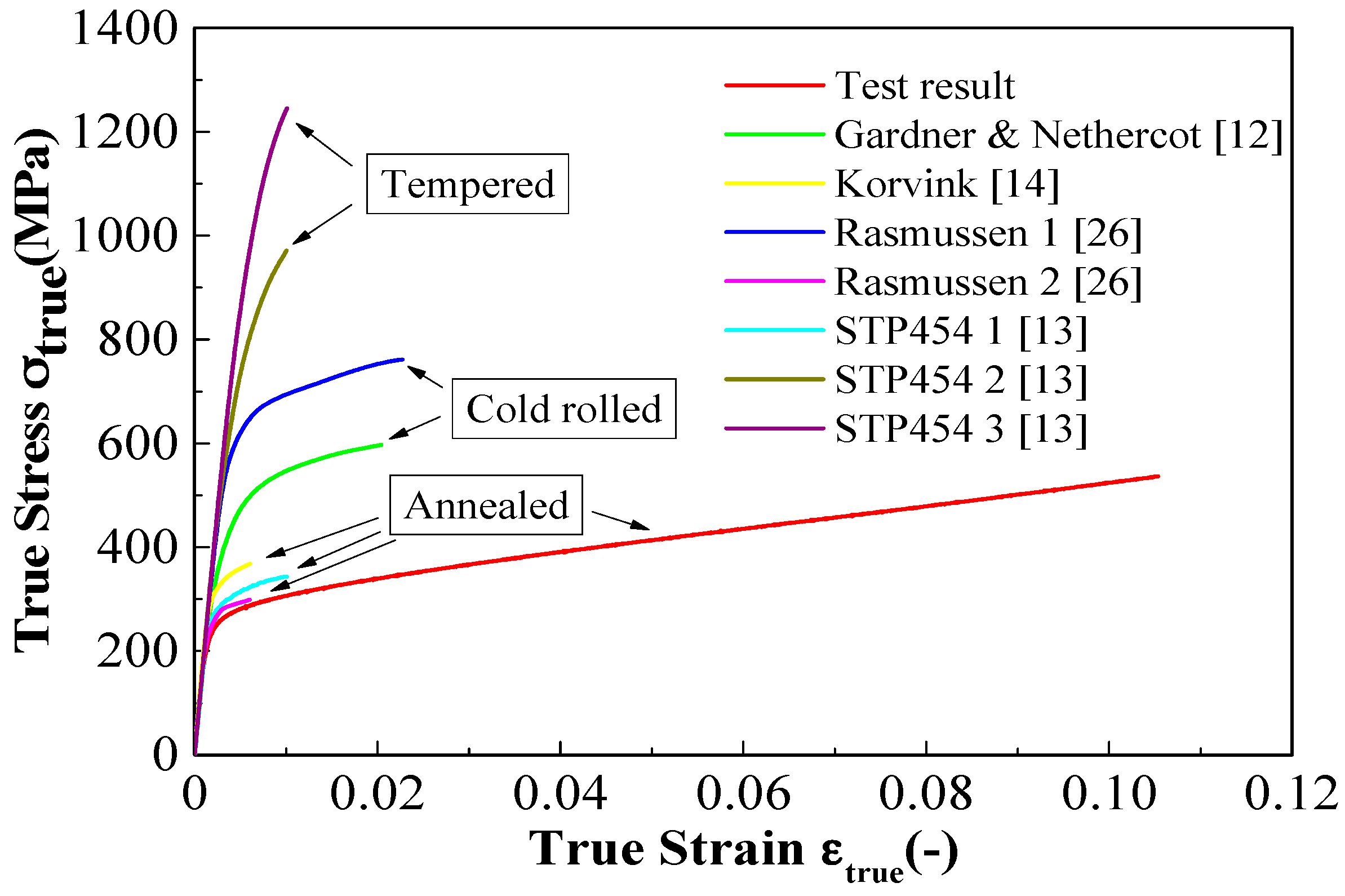
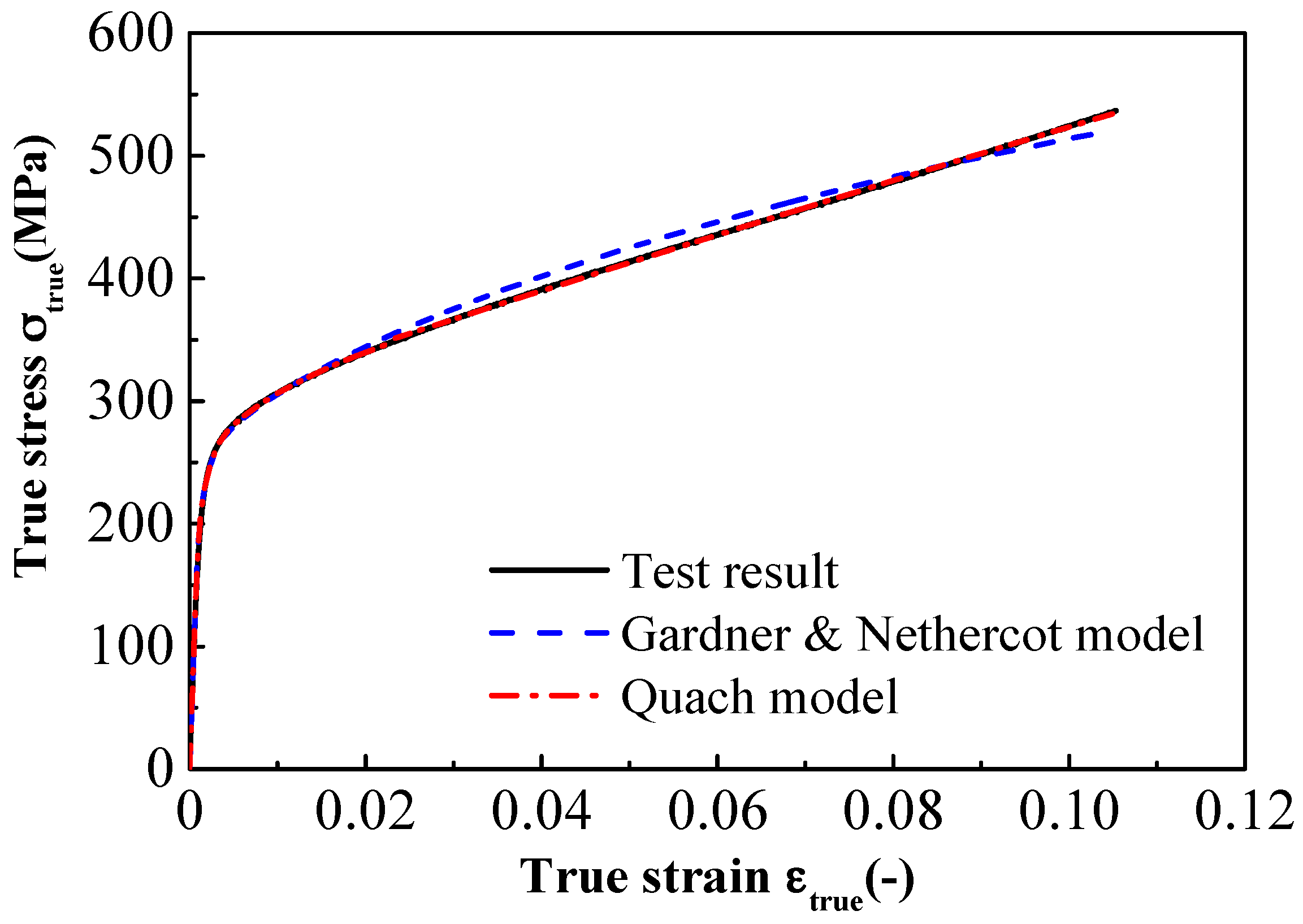
- The compressive stress-strain curve is a two-stage strain-hardening curve and the second strain-hardening stage is represented by a straight line. The Quach model agrees with the test results and can accurately describe the strain-hardening behavior of austenitic stainless steel under high compressive strains up to 10%.
Author Contributions
Acknowledgments
Conflicts of Interest
References
- Yu, W.W.; Laboube, R. Cold-Formed Steel Design, 4th ed.; John Wiley & Sons: Hoboken, NJ, USA, 2010. [Google Scholar]
- Tao, Z.; Uy, B.; Liao, F.Y.; Han, L.H. Nonlinear analysis of concrete-filled square stainless steel stub columns under axial compression. J. Constr. Steel Res. 2011, 67, 1719–1732. [Google Scholar] [CrossRef]
- Patton, M.; Singh, K. Numerical modeling of lean duplex stainless steel hollow columns of square, L-, T-, and +-shaped cross sections under pure axial compression. Thin-Walled Struct. 2012, 53, 1–8. [Google Scholar] [CrossRef]
- Patton, M.; Singh, K. Buckling of fixed-ended lean duplex stainless steel hollow columns of square, L-, T-, and +-shaped sections under pure axial compression—A finite element study. Thin-Walled Struct. 2013, 63, 106–116. [Google Scholar] [CrossRef]
- Patel, V.; Liang, Q.Q.; Hadi, M. Nonlinear analysis of axially loaded circular concrete-filled stainless steel tubular short columns. J. Constr. Steel Res. 2014, 101, 9–18. [Google Scholar] [CrossRef] [Green Version]
- Patel, V.; Liang, Q.Q.; Hadi, M. Nonlinear analysis of circular high strength concrete-filled stainless steel tubular slender beam-columns. Eng. Struct. 2017, 130, 1–13. [Google Scholar] [CrossRef] [Green Version]
- Perdahcıoğlu, E.; Geijselaers, H.; Huétink, J. Constitutive modeling of metastable austenitic stainless steel. Int. J. Mater. Form. 2008, 1, 97–100. [Google Scholar] [CrossRef] [Green Version]
- Zaeraa, R.; Casado, A.; Fernández-Sáez, J.; Rusinek, A.; Pesci, R. A constitutive model for analyzing martensite formation in austenitic steels deforming at high strain rates. Int. J. Plast. 2012, 29, 77–101. [Google Scholar] [CrossRef] [Green Version]
- Haghdadi, N.; Martin, D.; Hodgson, P. Physically-based constitutive modelling of hot deformation behavior in a LDX 2101 duplex stainless steel. Mater. Des. 2016, 106, 420–427. [Google Scholar] [CrossRef]
- Gardner, L.; Ashraf, M. Structural design for non-linear metallic materials. Eng. Struct. 2006, 28, 926–934. [Google Scholar] [CrossRef]
- Quach, W.M.; Teng, J.G.; Chung, K.F. Three-stage full-range stress-strain model for stainless steels. J. Struct. Eng. 2008, 134, 1518–1527. [Google Scholar] [CrossRef]
- Gardner, L.; Nethercot, D. Experiments on stainless steel hollow sections-Part 1: Material and cross-sectional behavior. J. Constr. Steel Res. 2004, 60, 1291–1318. [Google Scholar] [CrossRef]
- Johnson, A.; Kelsen, G. Stainless Steel in Structural Applications, Stainless Steel for Architecture; STP 454; American Society for Testing and Materials: West Conshohocken, PA, USA, 1969. [Google Scholar]
- Korvink, S.; van den Berg, G.; van der Merwe, P. Web crippling of stainless steel cold-formed beams. J. Constr. Steel Res. 1995, 34, 225–248. [Google Scholar] [CrossRef]
- Buchanan, C.; Matilainen, V.; Salminen, A.; Gardner, L. Structural performance of additive manufactured metallic material and cross-sections. J. Constr. Steel Res. 2017, 136, 35–48. [Google Scholar] [CrossRef] [Green Version]
- National Standards of the People’s Republic of China. Stainless Steel Hot-Rolled Steel Sheets and Strips GB/T 4237-2015; National Standards of the People’s Republic of China: Beijing, China, 2015. [Google Scholar]
- National Standard of the People’s Republic of China. Metal Material Compression Test Method GB 7314-2005; National Standards of the People’s Republic of China: Beijing, China, 2005. [Google Scholar]
- Dusicka, P.; Itani, A.; Buckle, I. Cyclic response of plate steels under large inelastic strains. J. Constr. Steel Res. 2007, 63, 156–164. [Google Scholar] [CrossRef]
- Marsh, G.M.; Robb, A.D.; Topper, T.H. Techniques and Equipment for Axial Fatigue Testing of Sheet Steel; SAE Paper No. 730578; SAE: Warrendale, PA, USA, 1973. [Google Scholar]
- Zhou, F.; Chen, Y.; Wu, Q. Dependence of the cyclic response of structural steel on loading history under large inelastic strains. J. Constr. Steel Res. 2015, 104, 64–73. [Google Scholar] [CrossRef]
- Gardner, L. A New Approach to Structural Stainless Steel Design. Ph.D. Thesis, Imperial College London, London, UK, 2002. [Google Scholar]
- China Engineering Construction Association Standards. Technical Specification for Stainless Steel Structures CECS 410, 2015; China Engineering Construction Association Standards: Beijing, China, 2015. [Google Scholar]
- National Standards of the People’s Republic of China. Metallic Materials Tensile Test Part 1: Room Temperature Test Method GB/T228.1-2010; National Standards of the People’s Republic of China: Beijing, China, 2010. [Google Scholar]
- Wu, Q.; Chen, Y.; Zhou, F. Cyclic loading tests of high strength steel under larger inelastic strains. J. Build. Struct. 2014, 35, 89–94. (In Chinese) [Google Scholar]
- Hertzberg, R. Deformation and Fracture Mechanics of Engineering Materials, 4th ed.; Wiley: New York, NY, USA, 1995. [Google Scholar]
- Rasmussen, K.; Burns, T.; Bezkorovainy, P.; Bambach, M. Numerical modelling of stainless steel plates in compression. J. Constr. Steel Res. 2003, 59, 1345–1362. [Google Scholar] [CrossRef]
- Lebedev, A.; Kosarchuk, V. Influence of phase transformations on the mechanical properties of austenitic stainless steels. Int. J. Plast. 2000, 16, 749–767. [Google Scholar] [CrossRef]
- Haghdadi, N.; Cizek, P.; Beladi, H.; Hodgson, P. The austenite microstructure evolution in a duplex stainless steel subjected to hot deformation. Philos. Mag. 2017, 97, 1209–1237. [Google Scholar] [CrossRef]
- Olsson, A. Stainless Steel Plasticity—Material Modelling and Structural Applications. Ph.D. Thesis, Luleå University of Technology, Luleå, Sweden, 2001. [Google Scholar]

| 0.03 | 0.75 | 2.00 | 0.045 | 0.030 | 17.50 | 8.00 |
| Grade | Nominal Yield Strength σ0.2/MPa | Tensile Strength σu/MPa | Elongation after Fracture εu/% | Longitudinal/Transverse Strain Reinforcement Coefficient n |
|---|---|---|---|---|
| S30403 | 180 | 485 | 40 | 6/8 |
| Test No. | Initial Modulus E0/MPa | Secant Modulus E0.2/MPa | Nominal Yield Strength σ0.2 a/MPa | Proof Stress of 1.0% Strain σ1.0 a/MPa | Proof Stress of 10.0% Strain σ10.0 a/MPa | Ultimate Strength σu a/MPa | Elongation after Fracture εu/% |
|---|---|---|---|---|---|---|---|
| CT-1 | 203,626 | 16,382 | 233 | 275 | 395 | 721 | 57 |
| CT-2 | 194,100 | 14,981 | 236 | 278 | 396 | 715 | 56 |
| CT-3 | 195,331 | 15,214 | 240 | 277 | 396 | 725 | 59 |
| mean value | 197,686 | 15,526 | 236 | 277 | 396 | 720 | 57 |
| Standard deviation | 4230 | 613 | 2.87 | 1.25 | 0.47 | 4.11 | 1.25 |
| ST-1 | 194,689 | 15,596 | 242 | 290 | 406 | 720 | 94 |
| ST-2 | 196,896 | 16,330 | 244 | 295 | 409 | 727 | 93 |
| ST-3 | 195,460 | 15,784 | 245 | 292 | 407 | 723 | 92 |
| mean value | 195,682 | 15,903 | 244 | 292 | 407 | 723 | 93 |
| Standard deviation | 915 | 311 | 1.25 | 2.05 | 1.25 | 2.87 | 0.82 |
| SC-1 | 206,405 | 15,196 | 267 | 317 | 600 b | - | - |
| SC-2 | 195,471 | 16,014 | 267 | 317 | 604 b | - | - |
| SC-3 | 203,590 | 17,046 | 269 | 314 | 600 b | - | - |
| mean value | 201,822 | 16,085 | 268 | 316 | 601 b | - | - |
| Standard deviation | 4636 | 757 | 0.94 | 1.41 | 1.89 | - | - |
| Model | E0/GPa | σ0.2/MPa | ||||
|---|---|---|---|---|---|---|
| G & N | 202 | 268 | 8.5 | 1.5 | - | - |
| Quach | 8.5 | 1.7 | 296 | 2390 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Peng, Y.; Chu, J.; Dong, J. Compressive Behavior and Constitutive Model of Austenitic Stainless Steel S30403 in High Strain Range. Materials 2018, 11, 1023. https://doi.org/10.3390/ma11061023
Peng Y, Chu J, Dong J. Compressive Behavior and Constitutive Model of Austenitic Stainless Steel S30403 in High Strain Range. Materials. 2018; 11(6):1023. https://doi.org/10.3390/ma11061023
Chicago/Turabian StylePeng, Yang, Jiang Chu, and Jun Dong. 2018. "Compressive Behavior and Constitutive Model of Austenitic Stainless Steel S30403 in High Strain Range" Materials 11, no. 6: 1023. https://doi.org/10.3390/ma11061023
APA StylePeng, Y., Chu, J., & Dong, J. (2018). Compressive Behavior and Constitutive Model of Austenitic Stainless Steel S30403 in High Strain Range. Materials, 11(6), 1023. https://doi.org/10.3390/ma11061023





