Geometrically Nonlinear Field Fracture Mechanics and Crack Nucleation, Application to Strain Localization Fields in Al-Cu-Li Aerospace Alloys
Abstract
:1. Introduction
2. Notations
3. Incompatible Elasto-Plastic Continuum Theory
3.1. Disconnections and the Incompatibility of the Total Distortion
3.2. Dislocations and the Incompatibility of the Plastic Distortion
3.3. Composition of Incompatibilities and Elastic Distortion
4. Elasto-Static Incompatible Media
5. Transport
6. Elasto-Plastic Incompatible Media
7. Disconnection Nucleation
Thermally-Activated Crack Nucleation in Elastic-Brittle Materials
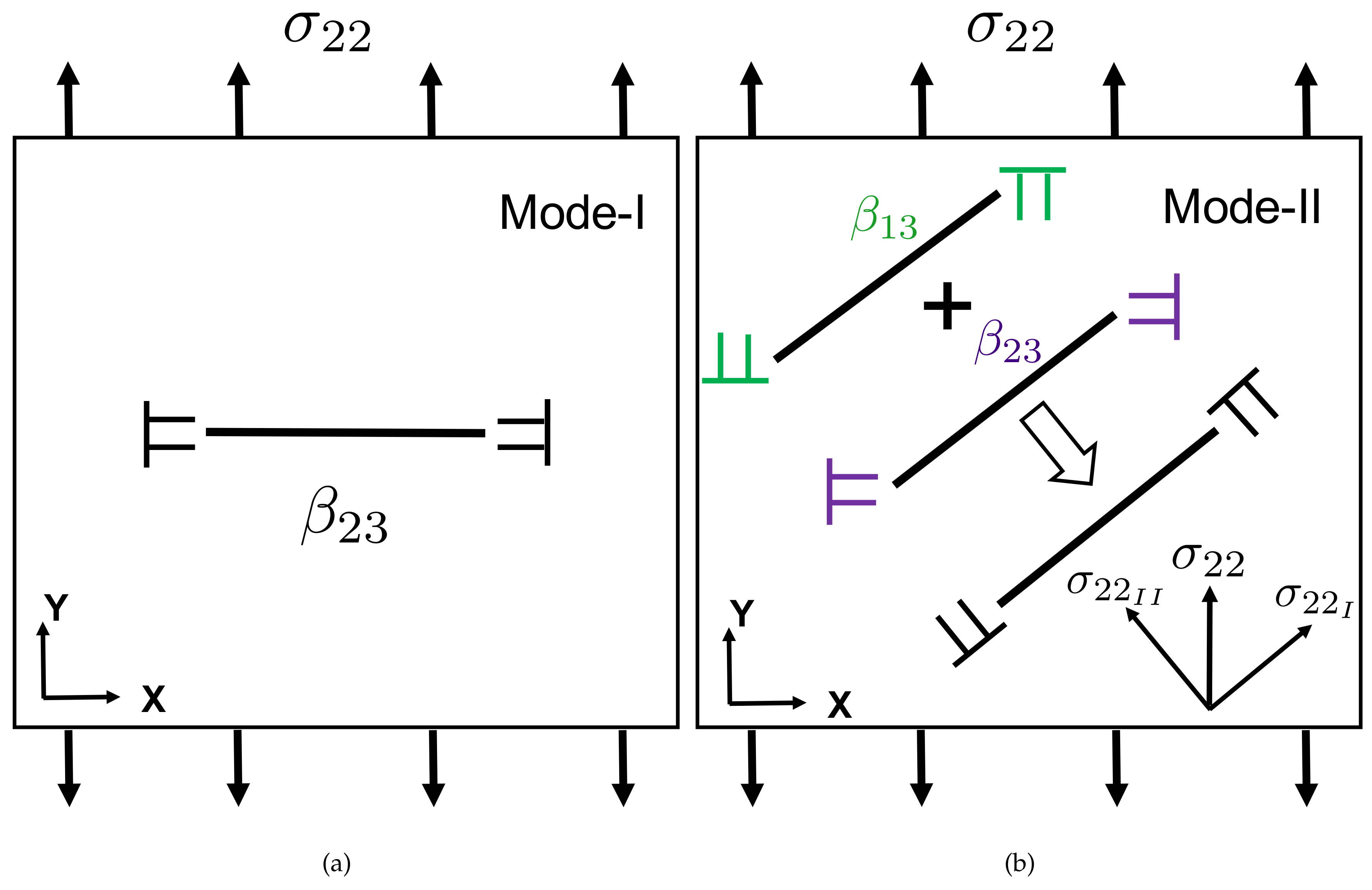
8. Application to DIC Methods and Strain Localization-Induced Fracture in Al-Cu-Li Alloys
8.1. DIC Setup and Estimation of Disconnection Densities from DIC Data
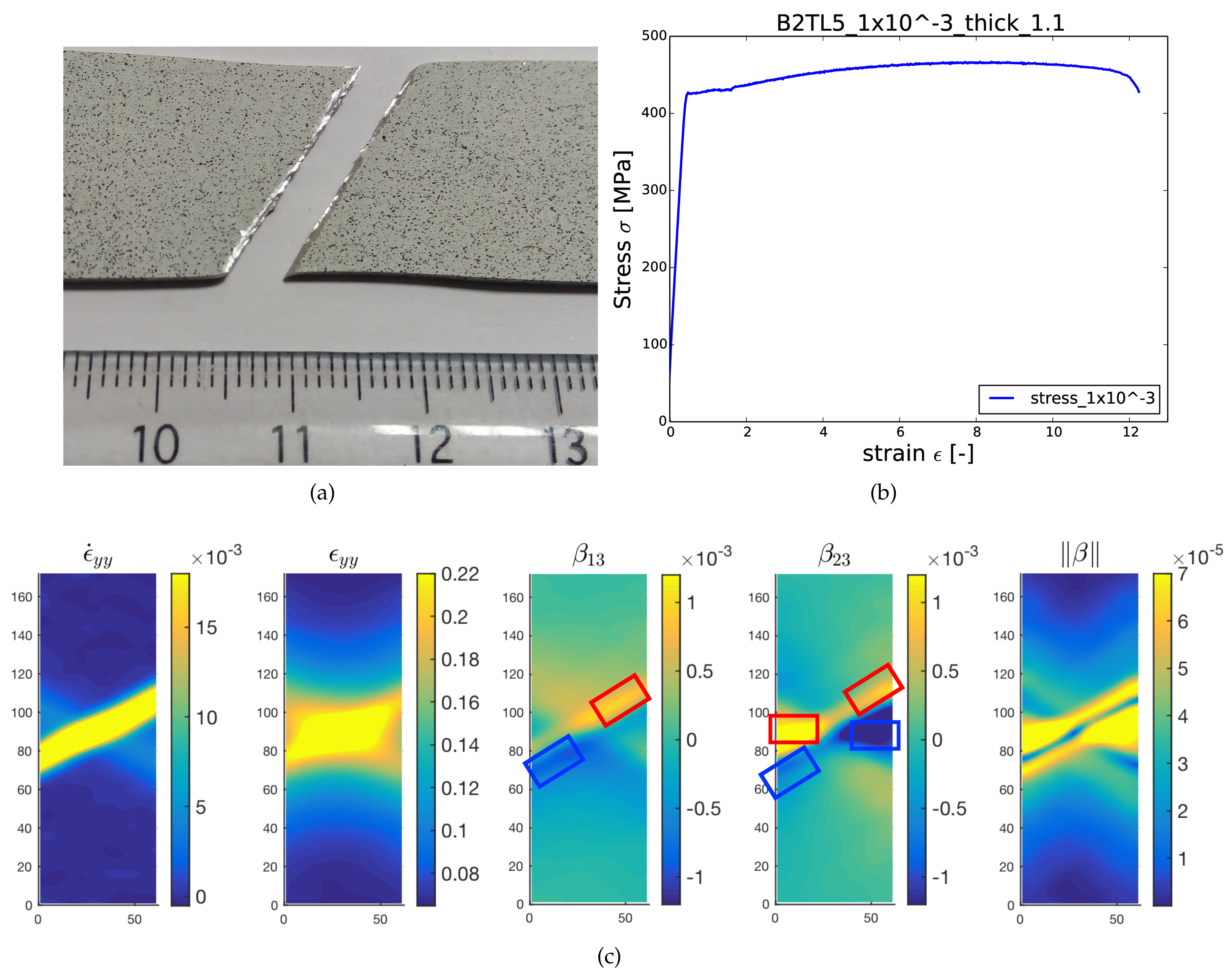
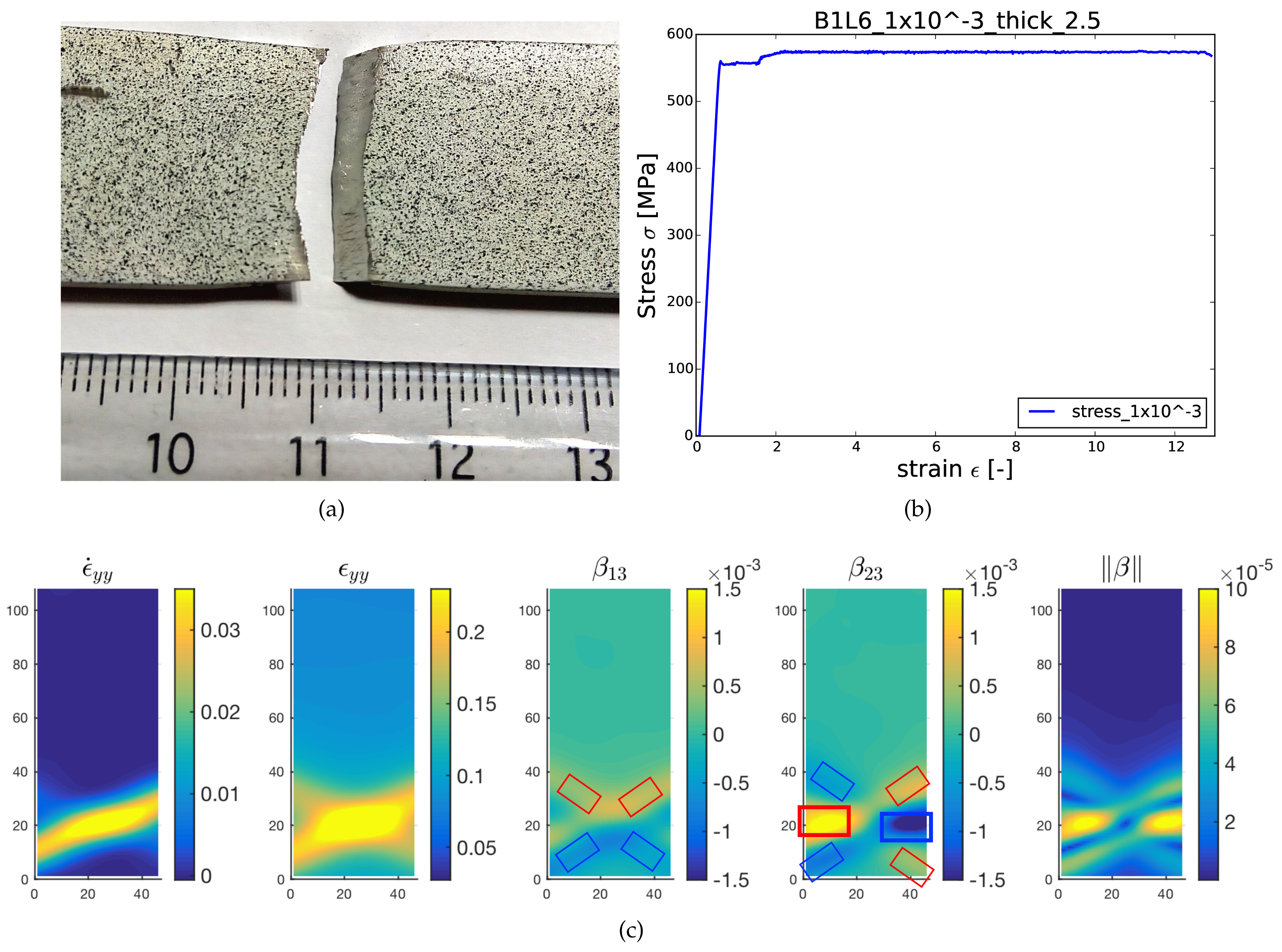
8.2. Analysis of Sample Fracture Using Disconnection Densities
8.3. Mixed Mode Fractured Specimen
8.4. Mode I Dominated Fractured Specimen
9. Conclusions
Acknowledgments
Author Contributions
References
- Eshelby, J.D. The determination of the elastic field in an ellipsoidal inclusion, and related problems. Proc. R. Soc. Lond. A 1957, 241, 376–396. [Google Scholar] [CrossRef]
- Friedel, J. Dislocations; Pergamon Press: Oxford, UK, 1964. [Google Scholar]
- Hirth, J.P. Dislocations, steps and disconnections at interfaces. J. Phys. Chem. Solids 1994, 55, 985–989. [Google Scholar] [CrossRef]
- Kondo, K. RAAG Memoirs of the Unifying Study of the Basic Problems in Engineering Sciences by Means of Geometry; Unifying Study Group: New York, NY, USA, 1955. [Google Scholar]
- Marcinkowski, M.J. The differential geometry of fracture. Acta Mech. 1978, 30, 175–195. [Google Scholar] [CrossRef]
- Nabarro, F.R.N. Dislocations in Solids; Oxford University Press: Oxford, UK, 1967. [Google Scholar]
- Barnett, D.M.; Asaro, R.J. The fracture mechanics of slit-like cracks in anisotropic elastic media. J. Mech. Phys. Solids 1972, 20, 353–366. [Google Scholar] [CrossRef]
- Bilby, B.A.; Cottrell, B.H.; Swinden, K.H. The spread of plastic yield from a notch. Proc. R. Soc. Lond. A 1965, 285, 22–23. [Google Scholar] [CrossRef]
- Hills, D.A.; Kelly, P.A.; Dai, D.N.; Korsunsky, A.M. Solution of Crack Problems: The Distributed Dislocation Technique; Springer: Dordrecht, The Netherlands, 1996. [Google Scholar]
- Mura, T. Micromechanics of Defects in Solids; Martinus Nijhoff Publishers: Dordrecht, The Netherlands, 1987. [Google Scholar]
- Weertman, J. Dislocation Based Fracture Mechanics; World Publishing Co.: Singapore, 1996. [Google Scholar]
- Kröner, E. Kontinuumstheorie der Versetzungen und Eigenspannungen; Ergeb. Agnew. Math. 5; Springer: Berlin, Germany, 1958. [Google Scholar]
- Fressengeas, C.; Taupin, V. A field theory of distortion incompatibility for coupled fracture and plasticity. J. Mech. Phys. Solids 2014, 68, 45–65. [Google Scholar] [CrossRef]
- Maugin, G.A. Configurational Forces: Thermodynamics, Physics, Mathematics, and Numerics; Chapman & Hall/CRC: Boca Raton, FL, USA, 2011. [Google Scholar]
- Miyamoto, H.; Kageyama, K. Extension of J-integral to the general elasto-plastic problem and suggestion of a new method for its evaluation. In Proceedings of the 1st International Conference on Numerical Methods in Fracture Mechanics, West Glamorgan, UK, 9–13 January 1978; Luxmoore, A.R., Owen, D.R.J., Eds.; pp. 261–275. [Google Scholar]
- Simha, N.K.; Fischer, F.D.; Shan, G.X.; Chen, C.R.; Kolednik, O. J-integral and crack driving force in elastic-plastic materials. J. Mech. Phys. Solids 2008, 56, 2876–2895. [Google Scholar] [CrossRef]
- Fressengeas, C.; Taupin, V. A field theory of strain/curvature incompatibility for coupled fracture and plasticity. Int. J. Solids Struct. 2016, 82, 16–38. [Google Scholar] [CrossRef]
- Griffith, A.A. The phenomenon of rupture and flow in solids. Phil. Trans. R. Soc. Lond. A 1921, 221, 163–198. [Google Scholar] [CrossRef]
- Pomeau, Y. Brisure spontanée de cristaux bidimensionnels courbés. C. R. Acad. Sci. Paris 1992, 314, 553–556. [Google Scholar]
- Rabinovitch, A.; Friedman, M.; Bahat, D. Failure time in heterogeneous materials—Non-homogeneous nucleation. Europhys. Lett. 2004, 67, 969–975. [Google Scholar] [CrossRef]
- Gupta, S.; Beaudoin, A.J.; Chevy, J. Strain rate jump induced negative strain rate sensitivity (NSRS) in aluminum alloy 2024: Experiments and constitutive modeling. Mater. Sci. Eng. A 2017, 683, 143–152. [Google Scholar] [CrossRef]
- Morgeneyer, T.F.; Taillandier-Thomas, T.; Helfen, L.; Baumbach, T.; Sinclair, I.; Roux, S.; Hild, F. In situ 3-D observation of early strain localization during failure of thin Al alloy (2198) sheet. Acta Mater. 2014, 69, 78. [Google Scholar] [CrossRef] [Green Version]
- Morgeneyer, T.F.; Taillandier-Thomas, T.; Buljac, A.; Helfen, L.; Hild, F. On strain and damage interactions during tearing: 3D in situ measurements and simulations for a ductile alloy (AA2139-T3). J. Mech. Phys. Solids 2016, 96, 550–571. [Google Scholar] [CrossRef] [Green Version]
- Jiang, B. The least-squares finite element method. In Theory and Computation in Fluid Dynamics and Electromagnetics; Springer Series in Scientific Computation; Springer: Berlin, Germany, 1998. [Google Scholar]
- Fressengeas, C.; Taupin, V.; Capolungo, L. An elasto-plastic theory of dislocation and disclination fields. Int. J. Solids Struct. 2011, 48, 3499–3509. [Google Scholar] [CrossRef]
- Lee, E.H. Elastic-plastic deformation at finite strains. J. Appl. Phys. 1969, 36, 1–6. [Google Scholar] [CrossRef]
- Acharya, A. Jump condition for GND evolution as a constraint on slip transmission at grain boundaries. Phil. Mag. 2007, 87, 1349–1359. [Google Scholar] [CrossRef]
- Garg, A.; Acharya, A.; Maloney, C.E. A study of conditions for dislocation nucleation in coarser-than-atomistic scale models. J. Mech. Phys. Solids 2015, 75, 76–92. [Google Scholar] [CrossRef]
- Varadhan, S.; Beaudoin, A.J.; Acharya, A.; Fressengeas, C. Dislocation transport using an explicit Galerkin/least-squares formulation. Modell. Simul. Mater. Sci. Eng. 2006, 14, 1245–1270. [Google Scholar] [CrossRef]
- Coleman, B.D.; Gurtin, M.E. Thermodynamics with internal state variables. J. Chem. Phys. 1967, 47, 597–613. [Google Scholar] [CrossRef]
- Guarino, A.; Ciliberto, S.; Garcimartín, A. Failure time and microcrack nucleation. Europhys. Lett. 1999, 47, 456–461. [Google Scholar] [CrossRef]
- Dias, C.L.; Kröger, J.; Vernon, D.; Grant, M. Nucleation of cracks in a brittle sheet. Phys. Rev. E 2009, 80, 066109. [Google Scholar] [CrossRef] [PubMed]
- Hounsome, L.S.; Jones, R.; Martineau, P.M.; Fisher, D.; Shaw, M.J.; Briddon, P.R.; Öberg, S. Origin of brown coloration in diamond. Phys. Rev. B 2006, 73, 125203. [Google Scholar] [CrossRef]
- Rabier, J.; Pizzagalli, L. Dislocation dipole annihilation in diamond and silicon. J. Phys. Conf. Ser. 2011, 281, 012025. [Google Scholar] [CrossRef]
- Golubovic, L.; Feng, S. Rate of microcrack nucleation. Phys. Rev. A 1991, 43, 5223–5227. [Google Scholar] [CrossRef] [PubMed]
- Gradshteyn, I.S.; Ryzhik, I.M. Table of Integrals, Series and Products; Academic Press: New York, NY, USA, 1965. [Google Scholar]
- Di Gioacchino, F.; da Fonseca, J.Q. Plastic Strain Mapping with Sub-micron Resolution Using Digital Image Correlation. Exp. Mech. 2013, 53, 743–754. [Google Scholar] [CrossRef]
- Di Gioacchino, F.; da Fonseca, J.Q. An experimental study of the polycrystalline plasticity of austenitic stainless steel. Int. J. Plast. 2015, 74, 92–109. [Google Scholar] [CrossRef]
- Chu, T.C.; Ranson, W.F.; Sutton, M.A.; Peters, W.H. Applications of digital-image-correlation techniques to experimental mechanics. Exp. Mech. 1985, 25, 232–244. [Google Scholar] [CrossRef]
- Sutton, M.A.; Cheng, M.Q.; Peters, W.H.; Chao, Y.J.; McNeill, S.R. Application of an optimized digital image correlation method to planar deformation analysis. Image Vis. Comp. 1986, 4, 143–150. [Google Scholar] [CrossRef]
- Fagerholt, E.; Rvik, T.B.; Hopperstad, O.S. Measuring discontinuous displacement fields in cracked specimens using digital image correlation to mesh adaptation and crack-path optimization. Opt. Lasers Eng. 2013, 51, 299–310. [Google Scholar] [CrossRef]
- Liu, J.; Lyons, J.; Sutton, M.A.; Reynolds, A. Experimental characterization of crack tip deformation fields in alloy 718 at high temperatures. J. Eng. Mat. Tech. Trans. ASME 1998, 120, 71–78. [Google Scholar] [CrossRef]
- McNeill, S.R.; Peters, W.H.; Sutton, M.A. Estimation of stress intensity factor by digital image correlation. Eng. Fract. Mech. 1987, 28, 101–112. [Google Scholar] [CrossRef]
- Valle, V.; Hedan, S.; Cosenza, P.; Fauchille, A.L.; Berdjane, M. Digital Image Correlation Development for the Study of Materials Including Multiple Crossing Cracks. Exp. Mech. 2015, 55, 379–391. [Google Scholar] [CrossRef]
- Chen, J.; Madi, Y.; Morgeneyer, T.F.; Besson, J. Plastic flow and ductile rupture of a 2198 Al-Cu-Li aluminum alloy. Comput. Mater. Sci. 2011, 50, 1365–1371. [Google Scholar] [CrossRef]
- Acharya, A. A model of crystal plasticity based on the theory of continuously distributed dislocations. J. Mech. Phys. Solids 2001, 49, 761–784. [Google Scholar] [CrossRef]
- Roy, A.; Acharya, A. Finite element approximation of Field Dislocation Mechanics. J. Mech. Phys. Solids 2005, 53, 143. [Google Scholar] [CrossRef]
- Berbenni, S.; Taupin, V.; Djaka, K.S.; Fressengeas, C. A numerical spectral approach for solving elasto-static field dislocation and g-disclination mechanics. Int. J. Solids Struct. 2014, 51, 4157–4175. [Google Scholar] [CrossRef]
- Djaka, K.S.; Taupin, V.; Berbenni, S.; Fressengeas, C. A numerical spectral approach to solve the dislocation density transport equation. Modell. Simul. Mater. Sci. Eng. 2015, 23, 065008. [Google Scholar] [CrossRef]

© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gupta, S.; Taupin, V.; Fressengeas, C.; Jrad, M. Geometrically Nonlinear Field Fracture Mechanics and Crack Nucleation, Application to Strain Localization Fields in Al-Cu-Li Aerospace Alloys. Materials 2018, 11, 498. https://doi.org/10.3390/ma11040498
Gupta S, Taupin V, Fressengeas C, Jrad M. Geometrically Nonlinear Field Fracture Mechanics and Crack Nucleation, Application to Strain Localization Fields in Al-Cu-Li Aerospace Alloys. Materials. 2018; 11(4):498. https://doi.org/10.3390/ma11040498
Chicago/Turabian StyleGupta, Satyapriya, Vincent Taupin, Claude Fressengeas, and Mohamad Jrad. 2018. "Geometrically Nonlinear Field Fracture Mechanics and Crack Nucleation, Application to Strain Localization Fields in Al-Cu-Li Aerospace Alloys" Materials 11, no. 4: 498. https://doi.org/10.3390/ma11040498
APA StyleGupta, S., Taupin, V., Fressengeas, C., & Jrad, M. (2018). Geometrically Nonlinear Field Fracture Mechanics and Crack Nucleation, Application to Strain Localization Fields in Al-Cu-Li Aerospace Alloys. Materials, 11(4), 498. https://doi.org/10.3390/ma11040498



