Optical Properties of Complex Plasmonic Materials Studied with Extended Effective Medium Theories Combined with Rigorous Coupled Wave Analysis
Abstract
1. Introduction
2. Materials and Methods
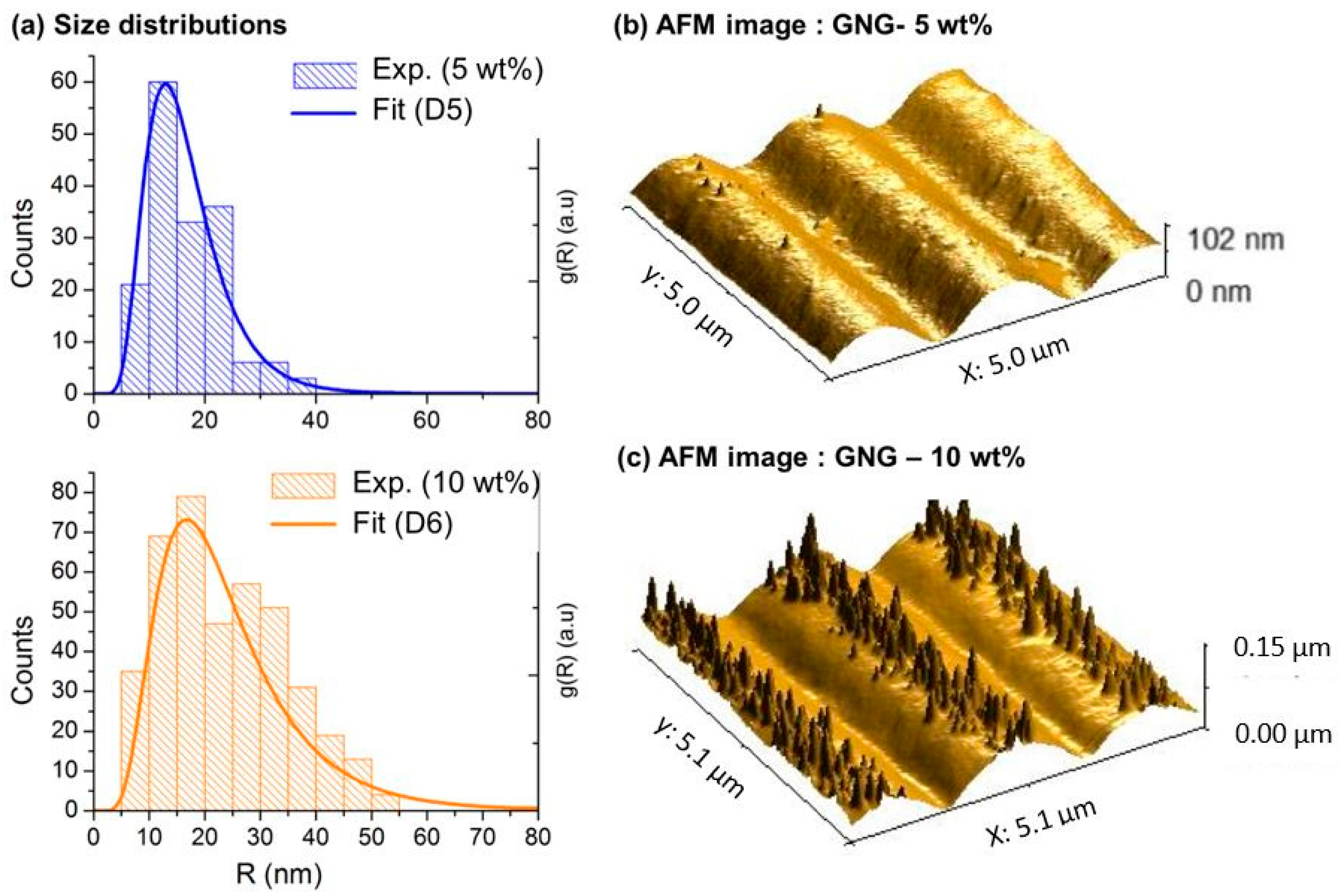
2.1. Fabrication of Gold Nanoparticle Gratings
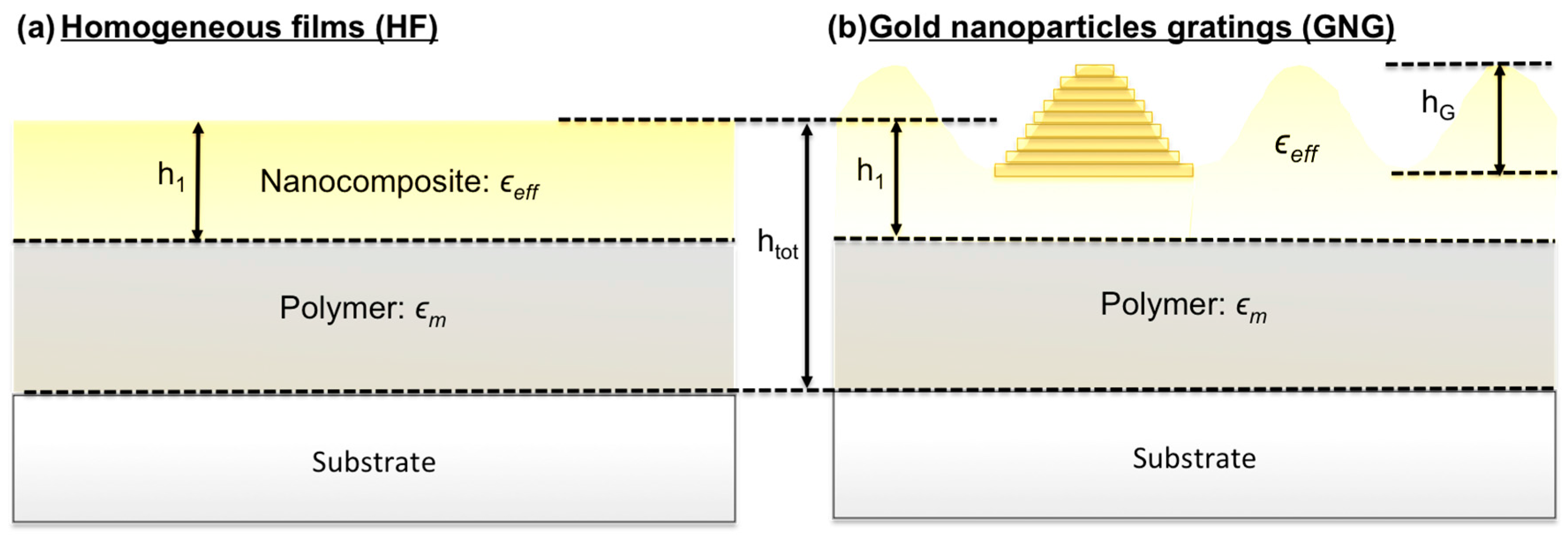
2.2. Analytical and Numerical Models
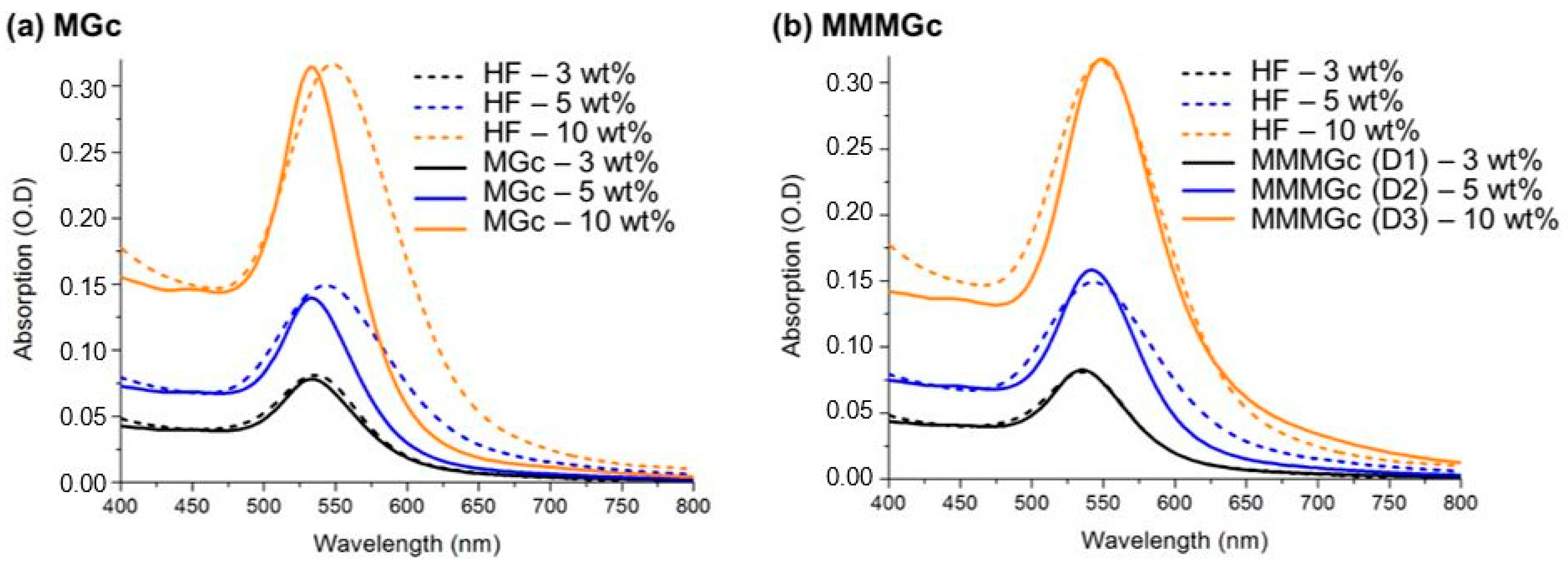
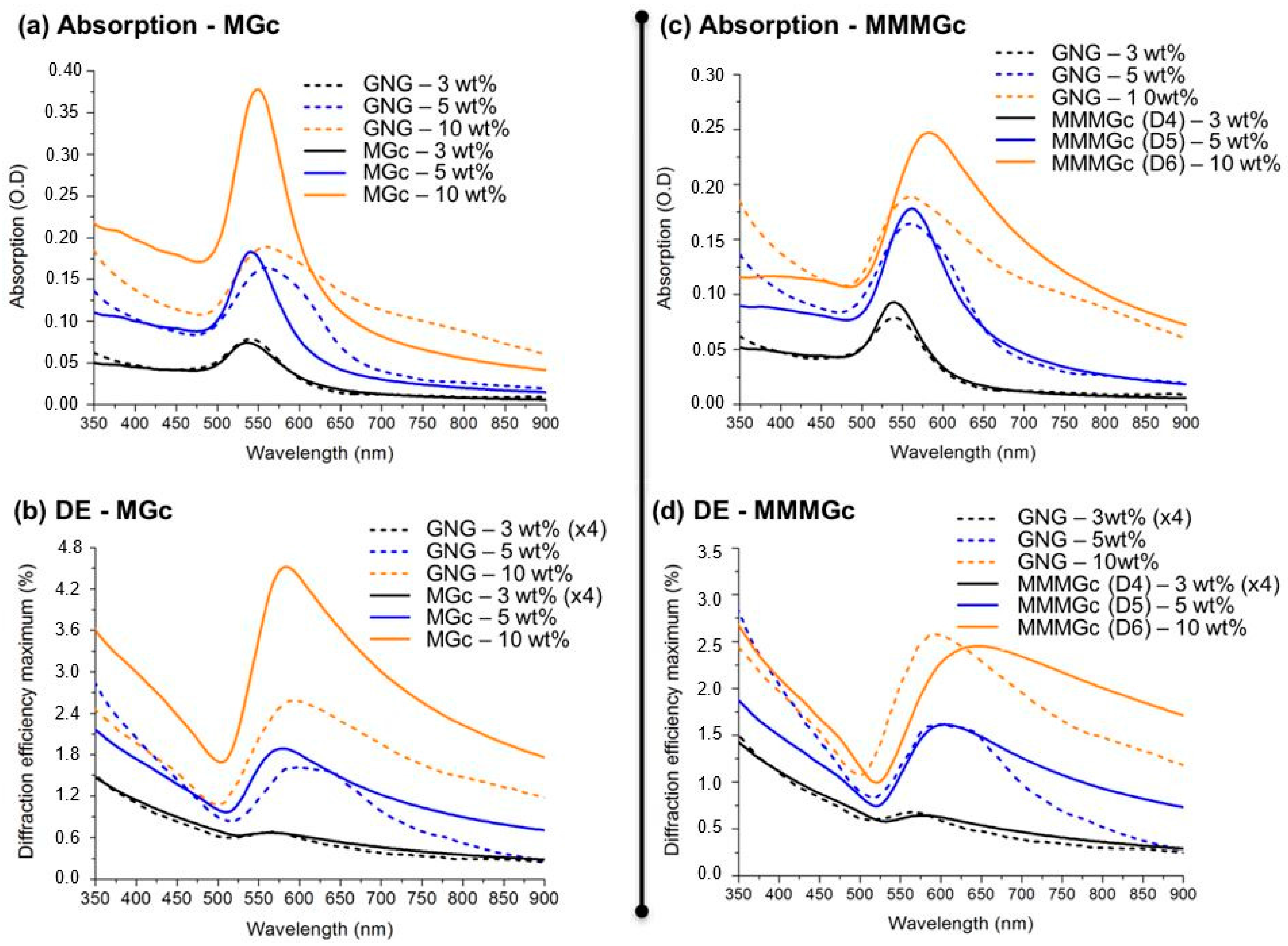
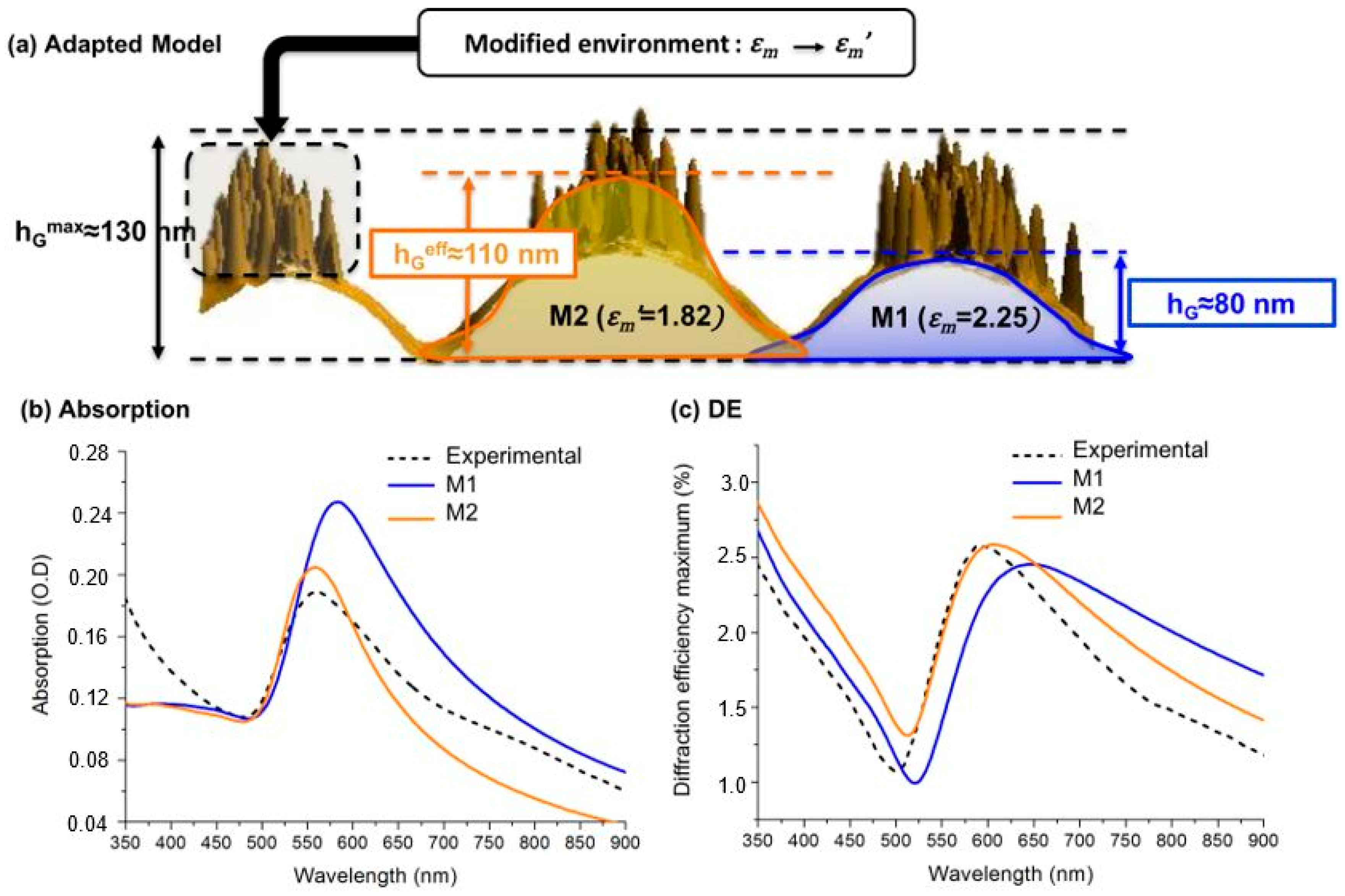
3. Results and Discussion
4. Conclusions
Supplementary Materials
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Hedayati, M.; Faupel, F.; Elbahri, M. Review of Plasmonic Nanocomposite Metamaterial Absorber. Materials 2014, 7, 1221–1248. [Google Scholar] [CrossRef] [PubMed]
- Malafronte, A.; Capretti, A.; Pepe, G.P.; Forestiere, C.; Miano, G.; Auriemma, F.; De Rosa, C.; Di Girolamo, R. Simple Theoretical Considerations for Block-Copolymer-Based Plasmonic Metamaterials. Macromol. Symp. 2016, 359, 72–78. [Google Scholar] [CrossRef]
- Stefik, M.; Guldin, S.; Vignolini, S.; Wiesner, U.; Steiner, U. Block copolymer self-assembly for nanophotonics. Chem. Soc. Rev. 2015, 44, 5076–5091. [Google Scholar] [CrossRef] [PubMed]
- Stratakis, E.; Kymakis, E. Nanoparticle-based plasmonic organic photovoltaic devices. Mater. Today 2013, 16, 133–146. [Google Scholar] [CrossRef]
- Babu, V.J.; Vempati, S.; Sundarrajan, S.; Sireesha, M.; Ramakrishna, S. Effective nanostructred morphologies for efficient hybrid solar cells. Sol. Energy 2014, 106, 1–22. [Google Scholar] [CrossRef]
- Cocoyer, C.; Rocha, L.; Fiorini-Debuisschert, C.; Sicot, L.; Vaufrey, D.; Sentein, C.; Geffroy, B.; Raimond, P. Implementation of a submicrometer patterning technique in azopolymer films towards optimization of photovoltaic solar cells efficiency. Thin Solid Films 2006, 511, 517–522. [Google Scholar] [CrossRef]
- Soum-Glaude, A.; Di Giacomo, L.; Quoizola, S.; Laurent, T.; Flamant, G. Selective Surfaces for Solar Thermal Energy Conversion in CSP: From Multilayers to Nanocomposites. In Nanotechnology for Energy Sustainability; Wiley: Hoboken, NJ, USA, 2017; pp. 231–248. [Google Scholar]
- Sellappan, R.; Nielsen, M.G.; González-Posada, F.; Vesborg, P.C.K.; Chorkendorff, I.; Chakarov, D. Effects of plasmon excitation on photocatalytic activity of Ag/TiO2 and Au/TiO2 nanocomposites. J. Catal. 2013, 307, 214–221. [Google Scholar] [CrossRef]
- Wang, M.; Ye, M.; Iocozzia, J.; Lin, C.; Lin, Z. Plasmon-mediated solar energy conversion via photocatalysis in noble metal/semiconductor composites. Adv. Sci. 2015, 3, 1600024. [Google Scholar] [CrossRef] [PubMed]
- Ma, X.C.; Dai, Y.; Yu, L.; Huang, B.B. Energy transfer in plasmonic photocatalytic composites. Light Sci. Appl. 2016, 5, e16017. [Google Scholar] [CrossRef]
- Mubeen, S.; Lee, J.; Singh, N.; Krämer, S.; Stucky, G.D.; Moskovits, M. An autonomous photosynthetic device in which all charge carriers derive from surface plasmons. Nat. Nanotechnol. 2013, 8, 247–251. [Google Scholar] [CrossRef] [PubMed]
- DeSario, P.A.; Pietron, J.J.; De Vantier, D.E.; Brintlinger, T.H.; Stroud, R.M.; Rolison, D.R. Plasmonic enhancement of visible-light water splitting with Au–TiO2 composite aerogels. Nanoscale 2013, 5, 8073. [Google Scholar] [CrossRef] [PubMed]
- Erwin, W.R.; Coppola, A.; Zarick, H.F.; Arora, P.; Miller, K.J.; Bardhan, R. Plasmon enhanced water splitting mediated by hybrid bimetallic Au–Ag core–shell nanostructures. Nanoscale 2014, 6, 12626–12634. [Google Scholar] [CrossRef] [PubMed]
- Maguire, J.F. Polymer Nanocomposites With Prescribed Morphology: Going Beyond Nanoparticle-Filled Polymers (Preprint). Chem. Mater. 2007, 19, 2736–2751. [Google Scholar]
- Lu, X.; Song, D.P.; Ribbe, A.; Watkins, J.J. Chiral Arrangements of Au Nanoparticles with Prescribed Handedness Templated by Helical Pores in Block Copolymer Films. Macromolecules 2017, 50, 5293–5300. [Google Scholar] [CrossRef]
- Ye, W.; Long, R.; Huang, H.; Xiong, Y. Plasmonic nanostructures in solar energy conversion. J. Mater. Chem. C 2016, 5, 1008–1021. [Google Scholar] [CrossRef]
- Davis, T.J.; Gómez, D.E.; Roberts, A. Plasmonic circuits for manipulating optical information. Nanophotonics 2017, 6, 543–559. [Google Scholar] [CrossRef]
- Juhl, A.T.; Busbee, J.D.; Koval, J.J.; Natarajan, L.V.; Tondiglia, V.P.; Vaia, R.A.; Bunning, T.J.; Braun, P.V. Holographically directed assembly of polymer nanocomposites. ACS Nano 2010, 4, 5953–5961. [Google Scholar] [CrossRef] [PubMed]
- Gallinet, B.; Butet, J.; Martin, O.J.F. Numerical methods for nanophotonics: Standard problems and future challenges. Laser Photonics Rev. 2015, 9, 577–603. [Google Scholar] [CrossRef]
- Draine, B.T. The discrete-dipole approximation and its application to interstellar graphite grains. Astrophys. J. 1988, 333, 848. [Google Scholar] [CrossRef]
- Yurkin, M.A.; Hoekstra, A.G. The discrete dipole approximation: An overview and recent developments. J. Quant. Spectrosc. Radiat. Transf. 2007, 106, 558–589. [Google Scholar] [CrossRef]
- Taflove, A.; Oskooi, A.; Johnson, S.G. Advances in FDTD Computational Electrodynamics: Photonics and Nanotechnology; Artech House Publishers: Norwood, MA, USA, 2013; ISBN 1608071707. [Google Scholar]
- Trügler, A. Modeling the Optical Response of Metallic Nanoparticles. In Optical Properties of Metallic Nanoparticles; Springer Publishing: New York, NY, USA, 2011; pp. 101–1127. [Google Scholar]
- Hohenester, U.; Trügler, A. MNPBEM—A Matlab toolbox for the simulation of plasmonic nanoparticles. Comput. Phys. Commun. 2012, 183, 370–381. [Google Scholar] [CrossRef]
- Myroshnychenko, V.; Rodríguez-Fernández, J.; Pastoriza-Santos, I.; Funston, A.M.; Novo, C.; Mulvaney, P.; Liz-Marzán, L.M.; García de Abajo, F.J. Modelling the optical response of gold nanoparticles. Chem. Soc. Rev. 2008, 37, 1792–1805. [Google Scholar] [CrossRef] [PubMed]
- Choy, T.C. Effective Medium Theory: Principles and Applications; Oxford University Press: New York, NY, USA, 2015. [Google Scholar]
- Markel, V.A. Introduction to the Maxwell Garnett approximation: Tutorial. J. Opt. Soc. Am. A 2016, 33, 1244–1256. [Google Scholar] [CrossRef] [PubMed]
- Knop, K. Rigorous diffraction theory for transmission phase gratings with deep rectangular grooves. J. Opt. Soc. Am. 1978, 68, 1206–1210. [Google Scholar] [CrossRef]
- Li, L. Multilayer modal method for diffraction gratings of arbitrary profile, depth, and permittivity: Addendum. J. Opt. Soc. Am. A 1994, 11, 1685. [Google Scholar] [CrossRef]
- Jackson, J.D. Classical Electrodynamics, 3rd ed.; Wiley: Hoboken, NJ, USA, 1999. [Google Scholar]
- Nadal, E.; Barros, N.; Glénat, H.; Laverdant, J.; Schmool, D.S.; Kachkachi, H. Plasmon-enhanced diffraction in nanoparticle gratings fabricated by in situ photo-reduction of gold chloride doped polymer thin films by laser interference patterning. J. Mater. Chem. C 2017, 5, 3553–3560. [Google Scholar] [CrossRef]
- Johnson, P.B.; Christy, R.W. Optical Constants of the Noble Metals. Phys. Rev. B 1972, 6, 4370–4379. [Google Scholar] [CrossRef]
- Derkachova, A.; Kolwas, K.; Demchenko, I. Dielectric Function for Gold in Plasmonics Applications: Size Dependence of Plasmon Resonance Frequencies and Damping Rates for Nanospheres. Plasmonics 2016, 11, 941–951. [Google Scholar] [CrossRef] [PubMed]
- Ashcroft, N.W.; Mermin, N.D. Solid State Physics; Holt, Rinehart and Winston: New York, NY, USA, 1976. [Google Scholar]
- Lermé, J.; Celep, G.; Broyer, M.; Cottancin, E.; Pellarin, M.; Arbouet, A.; Christofilos, D.; Guillon, C.; Langot, P.; Del Fatti, N.; et al. Effects of confinement on the electron and lattice dynamics in metal nanoparticles. Eur. Phys. J. D At. Mol. Opt. Plasma Phys. 2005, 34, 199–204. [Google Scholar] [CrossRef]
- Ruppin, R. Evaluation of extended Maxwell-Garnett theories. Opt. Commun. 2000, 182, 273–279. [Google Scholar] [CrossRef]
- Havel, H.; Fritz, S.; Hilger, A.; Kreibig, U.; Vollmer, M. Width of cluster plasmon resonances: Bulk dielectric functions and chemical interface damping. Phys. Rev. B 1993, 48, 18178–18188. [Google Scholar] [CrossRef]
- Hu, M.; Novo, C.; Funston, A.; Wang, H.; Staleva, H.; Zou, S.; Mulvaney, P.; Xia, Y.; Hartland, G.V. Dark-field microscopy studies of single metal nanoparticles: Understanding the factors that influence the linewidth of the localized surface plasmon resonance. J. Mater. Chem. 2008, 18, 1949–1960. [Google Scholar] [CrossRef] [PubMed]
- Berciaud, S.; Cognet, L.; Tamarat, P.; Lounis, B. Observation of intrinsic size effects in the optical response of individual gold nanoparticles. Nano Lett. 2005, 5, 515–518. [Google Scholar] [CrossRef] [PubMed]
- Battie, Y.; Resano-Garcia, A.; Chaoui, N.; Zhang, Y.; En Naciri, A. Extended Maxwell-Garnett-Mie formulation applied to size dispersion of metallic nanoparticles embedded in host liquid matrix. J. Chem. Phys. 2014, 140, 044705. [Google Scholar] [CrossRef] [PubMed]
- Mie, G. Beiträge zur Optik trüber Medien, speziell kolloidaler Metallösungen. Ann. Phys. 1908, 330, 377–445. [Google Scholar] [CrossRef]
- Yilmaz, E.; Ertas, G.; Bengu, E.; Suzer, S. Photopatterning of PMMA Films with Gold Nanoparticles: Diffusion of AuCl4− Ions. J. Phys. Chem. C 2010, 114, 18401–18406. [Google Scholar] [CrossRef]
- Ghosh, S.K.; Pal, T. Interparticle coupling effect on the surface plasmon resonance of gold nanoparticles: From theory to applications. Chem. Rev. 2007, 107, 4797–4862. [Google Scholar] [CrossRef] [PubMed]
- Lee, Y.H.; Lee, T.K.; Song, I.; Yu, H.; Lee, J.; Ko, H.; Kwak, S.K.; Oh, J.H. Boosting the Performance of Organic Optoelectronic Devices Using Multiple-Patterned Plasmonic Nanostructures. Adv. Mater. 2016, 28, 4976–4982. [Google Scholar] [CrossRef] [PubMed]



© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Nadal, E.; Barros, N.; Glénat, H.; Kachakachi, H. Optical Properties of Complex Plasmonic Materials Studied with Extended Effective Medium Theories Combined with Rigorous Coupled Wave Analysis. Materials 2018, 11, 351. https://doi.org/10.3390/ma11030351
Nadal E, Barros N, Glénat H, Kachakachi H. Optical Properties of Complex Plasmonic Materials Studied with Extended Effective Medium Theories Combined with Rigorous Coupled Wave Analysis. Materials. 2018; 11(3):351. https://doi.org/10.3390/ma11030351
Chicago/Turabian StyleNadal, Elie, Noémi Barros, Hervé Glénat, and Hamid Kachakachi. 2018. "Optical Properties of Complex Plasmonic Materials Studied with Extended Effective Medium Theories Combined with Rigorous Coupled Wave Analysis" Materials 11, no. 3: 351. https://doi.org/10.3390/ma11030351
APA StyleNadal, E., Barros, N., Glénat, H., & Kachakachi, H. (2018). Optical Properties of Complex Plasmonic Materials Studied with Extended Effective Medium Theories Combined with Rigorous Coupled Wave Analysis. Materials, 11(3), 351. https://doi.org/10.3390/ma11030351






