Optimization of Anodic Porous Alumina Fabricated from Commercial Aluminum Food Foils: A Statistical Approach
Abstract
:1. Introduction
2. Results
2.1. Characterization of the Preliminary APA Dataset
2.2. DoE Design of the New APA Dataset
2.3. Statistical Analysis of Datasets 1 and 2
3. Discussion
3.1. Previous Literature on the Use of DoE Applied to APA Fabrication
3.2. General Considerations and Limitations of Present Work
3.3. Discussion of Present Results
3.4. Confirmation Experiment
4. Materials and Methods
4.1. APA Fabrication
4.2. SEM Imaging and Image Analysis
4.3. DoE Method
5. Conclusions
- Our goal of fabricating comparatively good quality nanoporous alumina (i.e., controlled mean pore size and spacing as well as oxide thickness) for future large-scale production from inexpensive raw material, instead of ultrapure aluminum as currently used in academic research, is feasible. This will pave the way for real applications of this nanostructured material, for example in advanced composites for dentistry, aerospace and automotive uses, thanks to the mechanical interlocking, allowing the removal of the bonding agent phase, as well as in chemical and bio-sensors and in catalysis.
- In particular, we aimed at reaching target pore size and spacing of 100–150 nm and 150–275 nm for pore infiltration by the resin, and target thickness of 6–8 µm, best for subsequent ball-milling of the peeled-off alumina membranes to be used as the inorganic filler of dental composites, according to previously established procedures, in the shortest possible time. Based on the scanning electron microscopy imaging and the subsequent statistical analysis, the working parameters satisfying these requirements have been identified as 125 V, 20 °C and 7 h.
- We can partly confirm the expected behavior between operating parameters and emerging geometry, within the limitations emerging due to a batch effect, pointing out the issue or experimental repeatability in the process.
- The successful run of a confirmation experiment pointed out the possible implementation of an ‘industrial’ process of APA membrane fabrication for the identified application, when all processing parameters are carefully controlled. Most of the plots, which were used in order to determine an optimal design region, can be easily adapted to control the industrial process.
Supplementary Materials
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Sulka, G.D. Highly Ordered Anodic Porous Alumina Formation by Self-Organized Anodizing. In Nanostructured Materials in Electrochemistry; Wiley-VCH Verlag GmbH & Co. KGaA: Weinheim, Germany, 2008; pp. 1–116. [Google Scholar]
- Losic, D.; Santos, A. Nanoporous Alumina: Fabrication, Structure, Properties and Applications; Springer: Heidelberg, Germany, 2015. [Google Scholar]
- Diggle, J.; Downie, T.; Goulding, C. Anodic Oxide Films on Aluminum. Chem. Rev. 1969, 69, 365–405. [Google Scholar] [CrossRef]
- Runge, J.M.; Sung, M. Microcrystalline Anodic Aluminum Oxide Coatings: A Revolutionary Basis Technology for Corrosion Protection; Sanford Process Corporation: Woonsocket, RI, USA, 2011. [Google Scholar]
- Patermarakis, G.; Kapiris, G. Processes, parameters and mechanisms controlling the normal and abnormal growth of porous anodic alumina films. J. Solid State Electrochem. 2013, 17, 1133–1158. [Google Scholar] [CrossRef]
- Pashchanka, M.; Schneider, J.J. Origin of self-organisation in porous anodic alumina films derived from analogy with Rayleigh–Bénard convection cells. J. Mater. Chem. 2011, 21, 18761–18767. [Google Scholar] [CrossRef]
- Zhang, L.; Thompson, G.E.; Curioni, M.; Skeldon, P. Anodizing of Aluminum in Sulfuric Acid/Boric Acid Mixed Electrolyte. J. Electrochem. Soc. 2013, 160, 179–184. [Google Scholar] [CrossRef]
- Stępniowski, W.J.; Forbot, D.; Norek, M.; Michalska-Domańska, M.; Król, A. The impact of viscosity of the electrolyte on the formation of nanoporous anodic aluminum oxide. Electrochim. Acta 2014, 133, 57–64. [Google Scholar] [CrossRef]
- Dimogerontakis, T.; Tsangaraki-Kaplanoglou, I. The influence of certain sulfonic triphenylmethane dyes, as additives, on anodizing of aluminum in phosphoric acid. Thin Solid Films 2001, 385, 182–189. [Google Scholar] [CrossRef]
- Salerno, M.; Patra, N.; Cingolani, R. Use of Ionic Liquid in Fabrication, Characterization, and Processing of Anodic Porous Alumina. Nanoscale Res. Lett. 2009, 4, 865–872. [Google Scholar] [CrossRef] [PubMed]
- Shingubara, S. Fabrication of nanomaterials using porous alumina templates. J. Nanopart. Res. 2003, 5, 17–30. [Google Scholar] [CrossRef]
- Lee, W.; Park, S.J. Porous anodic aluminum oxide: Anodization and templated synthesis of functional nanostructures. Chem. Rev. 2014, 114, 7487–7556. [Google Scholar] [CrossRef] [PubMed]
- Kumeria, T.; Santos, A. Nanoporous Alumina. Springer Ser. Mater. Sci. 2015, 219, 293–318. [Google Scholar]
- Choi, J.; Schilling, J.; Nielsch, K.; Hillebrand, R.; Reiche, M.; Wehrspohn, R.B.; Gösele, U. Large-area porous alumina photonic crystals via imprint method. Mater. Res. Soc. Symp. Proc. 2002, 722, 2–7. [Google Scholar] [CrossRef]
- Walsh, R.J.; Chumanov, G. Silver Coated Porous Alumina as a New Substrate for Surface-Enhanced Raman Scattering. Appl. Spectrosc. 2001, 55, 1695–1700. [Google Scholar] [CrossRef]
- Das, G.; Patra, N.; Gopalakrishnan, A.; Zaccaria, R.P.; Toma, A.; Thorat, S.; Di Fabrizio, E.; Diaspro, A.; Salerno, M. Fabrication of large-area ordered and reproducible nanostructures for SERS biosensor application. Analyst 2012, 137, 1785–1792. [Google Scholar] [CrossRef] [PubMed]
- Karlsson, M.; Pålsgård, E.; Wilshaw, P.; Di Silvio, L. Initial in vitro interaction of osteoblasts with nano-porous alumina. Biomaterials 2003, 24, 3039–3046. [Google Scholar] [CrossRef]
- Salerno, M.; Caneva-Soumetz, F.; Pastorino, L.; Patra, N.; Diaspro, A.; Ruggiero, C. Adhesion and proliferation of osteoblast-like cells on anodic porous alumina substrates with different morphology. IEEE Trans. Nanobiosci. 2013, 12, 106–111. [Google Scholar] [CrossRef] [PubMed]
- Poinern, G.E.J.; Shackleton, R.; Mamun, S.I.; Fawcett, D. Significance of novel bioinorganic anodic aluminum oxide nanoscaffolds for promoting cellular response. Nanotechnol. Sci. Appl. 2011, 4, 11–24. [Google Scholar] [CrossRef] [PubMed]
- Brüggemann, D. Nanoporous Aluminium Oxide Membranes as Cell Interfaces. J. Nanomater. 2013, 2013, 1–18. [Google Scholar] [CrossRef]
- Salerno, M.; Giacomelli, L.; Larosa, C. Biomaterials for the programming of cell growth in oral tissues: The possible role of APA Bioinformation. Bioinformation 2010, 5, 291–293. [Google Scholar] [CrossRef]
- Kumeria, T.; Santos, A.; Rahman, M.M.; Ferre-Borrull, J.; Marsal, L.F.; Losic, D. Advanced Structural Engineering of Nanoporous Photonic Structures: Tailoring Nanopore Architecture to Enhance Sensing Properties. ACS Photonics 2014, 1, 1298–1306. [Google Scholar] [CrossRef]
- Toccafondi, C.; Thorat, S.; La Rocca, R.; Scarpellini, A.; Salerno, M.; Dante, S.; Das, G. Multifunctional substrates of thin porous alumina for cell biosensors. J. Mater. Sci. Mater. Med. 2014, 25, 2411–2420. [Google Scholar] [CrossRef] [PubMed]
- Gultepe, E.; Nagesha, D.; Sridhar, S.; Amiji, M. Nanoporous inorganic membranes or coatings for sustained drug delivery in implantable devices. Adv. Drug Deliv. Rev. 2010, 62, 305–315. [Google Scholar] [CrossRef] [PubMed]
- Santos, A.; Sinn Aw, M.; Bariana, M.; Kumeria, T.; Wang, Y.; Losic, D. Drug-releasing implants: Current progress, challenges and perspectives. J. Mater. Chem. B 2014, 2, 6157–6182. [Google Scholar] [CrossRef]
- Michalska-Domańska, M.; Norek, M.; Stępniowski, W.J.; Budner, B. Fabrication of high quality anodic aluminum oxide (AAO) on low purity aluminum—A comparative study with the (AAO) produced on high purity aluminum. Electrochim. Acta 2013, 105, 424–432. [Google Scholar] [CrossRef]
- Thorat, S.B.; Diaspro, A.; Salerno, M. In vitro investigation of coupling-agent-free dental restorative composite based on nano-porous alumina fillers. J. Dent. 2014, 42, 279–286. [Google Scholar] [CrossRef] [PubMed]
- Runge, J.M. Anodizing for Design and Function. J. Mater. Sci. Nanotechnol. 2014, 1. [Google Scholar] [CrossRef]
- Monfared, N.A.; Atre, S.V.; Kishton, R.; Varadarajan, S. Synthesis and characterization of nanoporous alumina films with controlled physical and chemical attributes. NSTI-Nanotech. 2009, 1, 16–19. [Google Scholar]
- Nemati, M.; Santos, A.; Law, C.S.; Losic, D. Assessment of Binding A ffi nity between Drugs and Human Serum Albumin Using Nanoporous Anodic Alumina Photonic Crystals. Anal. Chem. 2016, 88, 5971–5980. [Google Scholar] [CrossRef] [PubMed]
- Chen, C.A.; Jhan, J.; Chen, J.; Huang, K. Analysis of Experimental Parameters for Submicron Porous Anodic Alumina Structures; National Taiwan University of Science and Technology: Taipei, Taiwan, 2010. [Google Scholar]
- Hassanzadeh, N.; Omidvar, H.; Poorbafrani, M.; Tabaian, S.H. Influence of Anodizing Parameters on Pore Diameter of Anodic Aluminium Oxide (AAO) Films Using Taguchi Design. Arab. J. Sci. Eng. 2013, 38, 1305–1312. [Google Scholar] [CrossRef]
- Santos, A.; Montero-Moreno, J.M.; Bachmann, J.; Nielsch, K.; Formentín, P.; Ferre-Borrull, J.; Pallares, J.; Marsal, L.F. Understanding Pore Rearrangement during Mild to Hard Transition in Bilayered Porous Anodic Alumina Membranes. ACS Appl. Mater. Interfaces 2011, 3, 1925–1932. [Google Scholar] [CrossRef] [PubMed]
- Wang, J.; Li, H.; Sun, Y.; Bai, B.; Zhang, Y.; Fan, Y. Anodization of Highly Ordered TiO2 Nanotube Arrays Using Orthogonal Design and Its Wettability. Int. J. Electrochem. Sci. 2016, 11, 710–723. [Google Scholar]
- Barmala, M.; Moheb, A.; Emadi, R. Applying Taguchi method for optimization of the synthesis condition of nano-porous alumina membrane by slip casting method. J. Alloys Compd. 2009, 485, 778–782. [Google Scholar] [CrossRef]
- Runge, J.M. The Metallurgy of Anodizing Aluminum; Springer: Heidelberg, Germany, 2017. [Google Scholar]
- Salerno, M.; Stepniowski, W.J.; Cieslak, G.; Norek, M.; Michalska-Domanska, M.; Karczewski, K.; Chilimoniuk, P.; Polkowski, W.; Józwik, P.; Bojar, Z. Advanced image analysis of the surface pattern emerging in Ni3Al intermetallic alloys on anodization. Front. Mater. 2016, 3. [Google Scholar] [CrossRef]
- Toccafondi, C.; Stępniowski, W.J.; Leoncini, M.; Salerno, M. Advanced morphological analysis of patterns of thin anodic porous alumina. Mater. Charact. 2014, 94, 26–36. [Google Scholar] [CrossRef]
- Wu, C.F.J.; Hamada, M. Experiments Planning, Anaysis, and Parameter Design Optimization, 2nd ed; John Wiley & Sons, Inc.: New York, NY, USA, 2000. [Google Scholar]
- R Development Core Team. R: A Language and Environment for Statistical Computing. Available online: www.R-project.org (accessed on 8 June 2016).
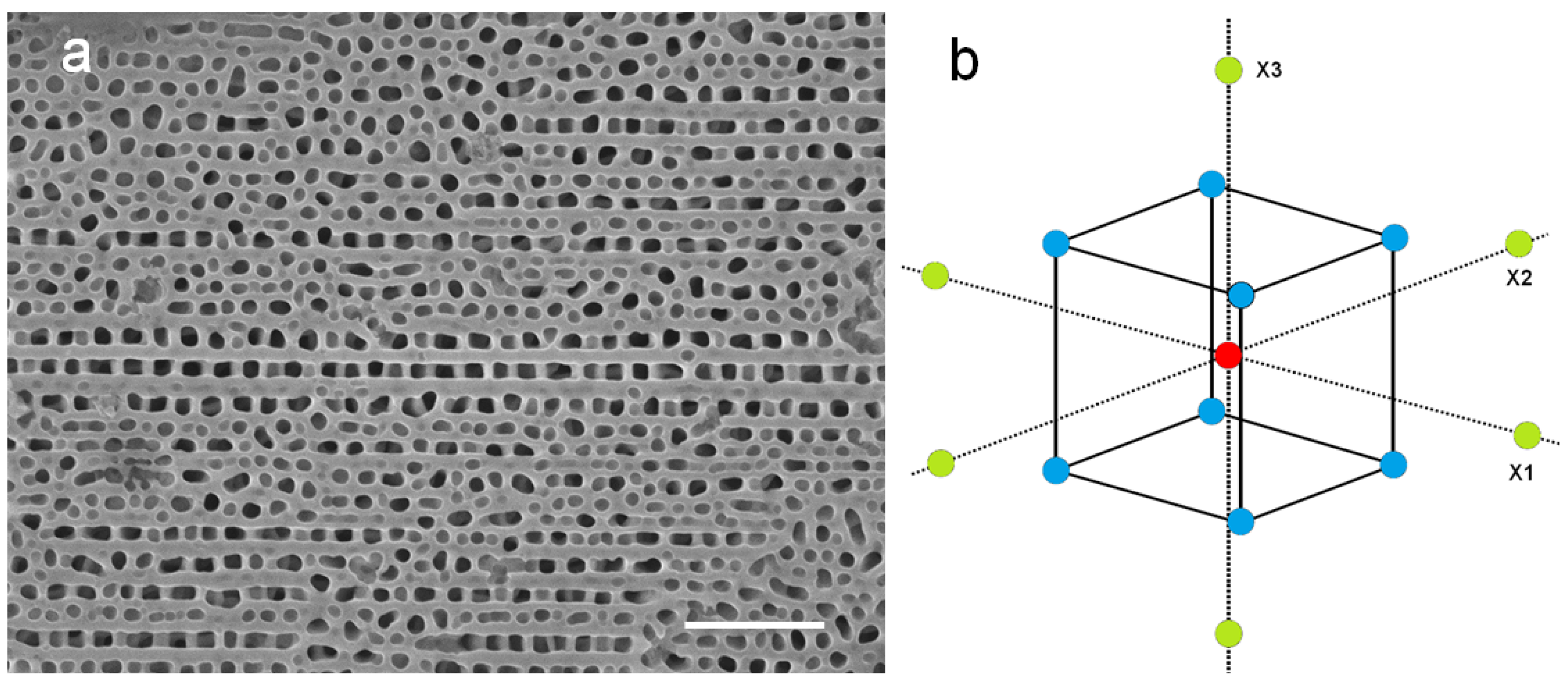

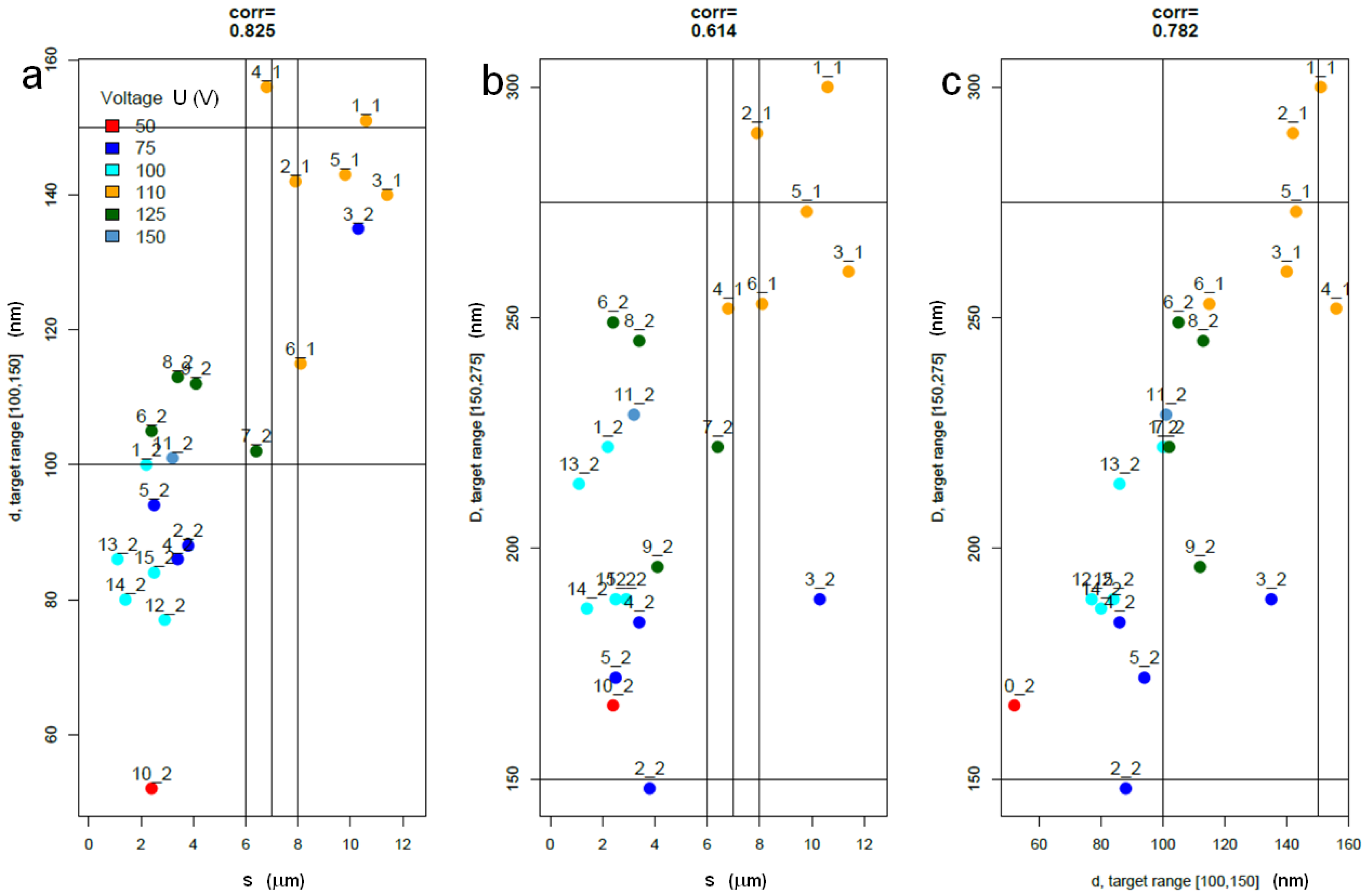
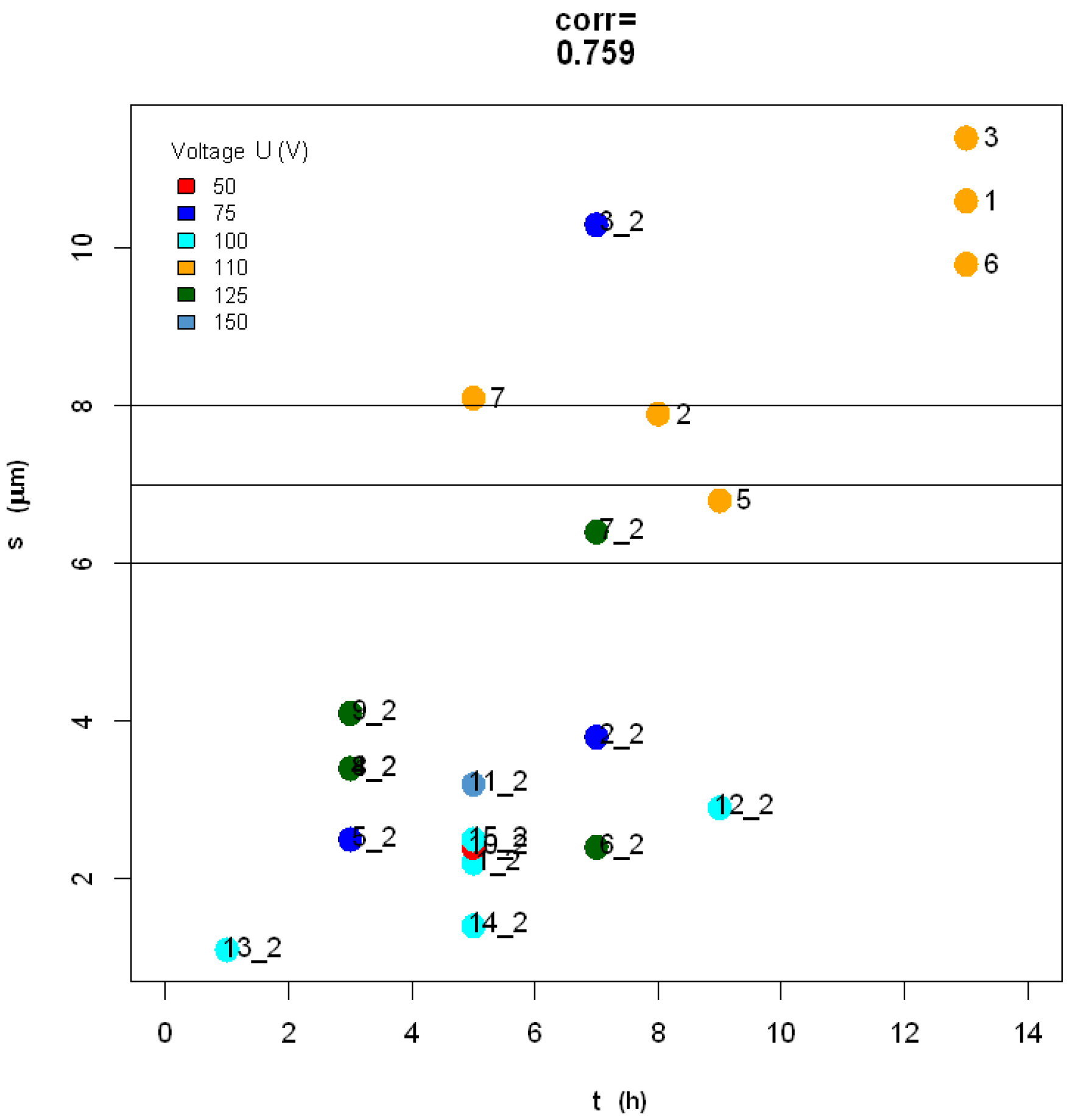
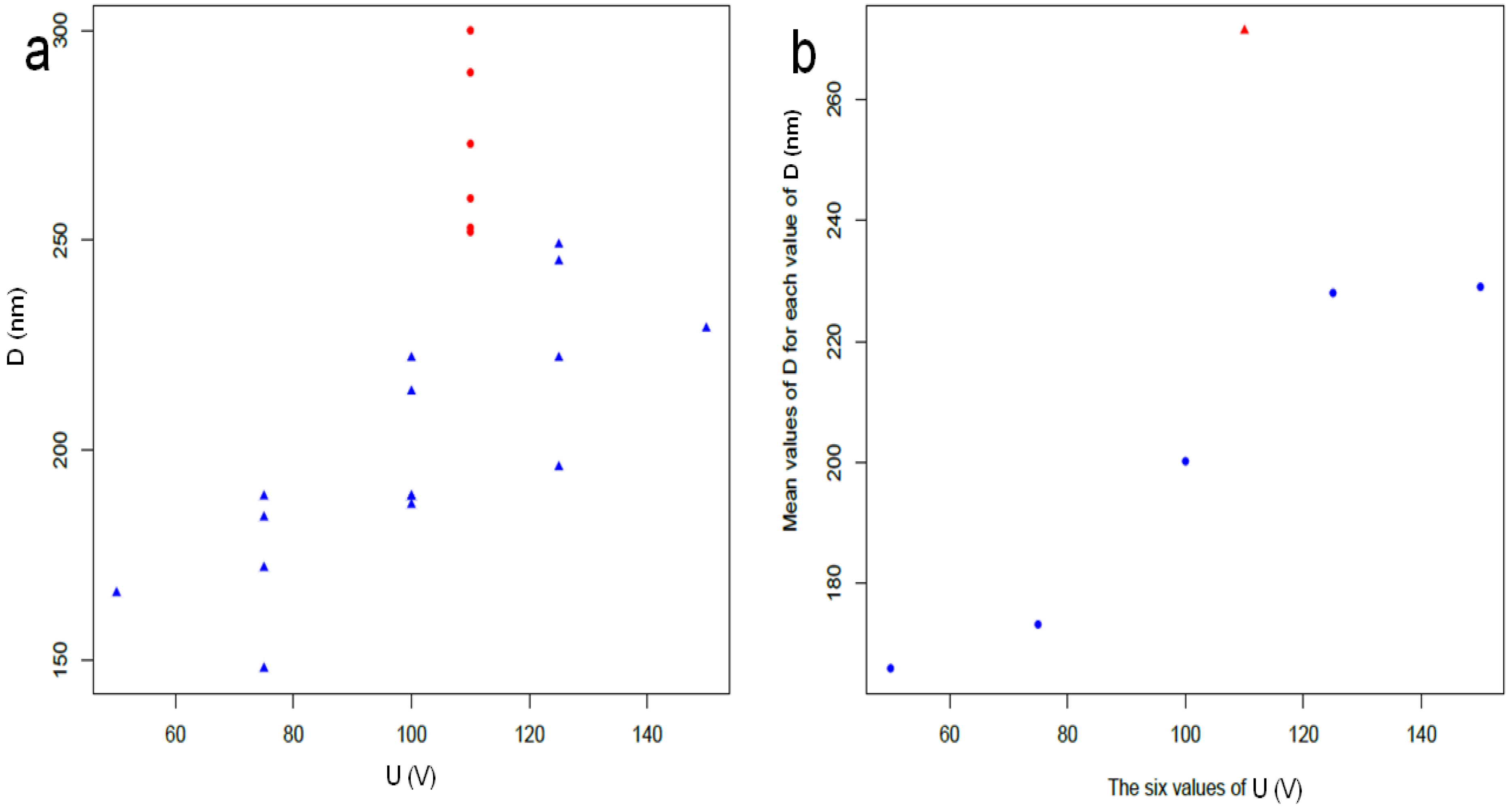
| Factor | Level No. | 1 | 2 | 3 | 4 | 5 | 6 | 7 |
|---|---|---|---|---|---|---|---|---|
| U | Level | 50 | 75 | 100 | 110 | 125 | 150 | - |
| Replicates | 1 | 4 | 5 | 6 | 4 | 1 | - | |
| T | Level | 5 | 8 | 10 | 15 | 20 | 25 | - |
| Replicates | 4 | 2 | 4 | 6 | 4 | 1 | - | |
| t | Level | 1 | 3 | 5 | 7 | 8 | 9 | 13 |
| Replicates | 1 | 4 | 6 | 4 | 1 | 2 | 3 |
| Datapoint | Factors | Main Responses | Secondary Responses | ||||||
|---|---|---|---|---|---|---|---|---|---|
| ID | Type | U (V) | T (°C) | t (h) | d (nm) | D (nm) | s (μm) | σ (μm−2) | p (%) |
| 1_2 | Central | 100 | 15 | 5 | 100 | 222 | 2.2 | 26 | 20 |
| 2_2 | Corner | 75 | 10 | 7 | 88 | 148 | 3.8 | 58 | 35 |
| 3_2 | 75 | 20 | 7 | 135 | 189 | 10.3 | 32 | 53 | |
| 4_2 | 75 | 10 | 3 | 86 | 184 | 3.4 | 37 | 22 | |
| 5_2 | 75 | 20 | 3 | 94 | 172 | 2.5 | 43 | 30 | |
| 6_2 | 125 | 10 | 7 | 105 | 249 | 2.4 | 21 | 18 | |
| 7_2 | 125 | 20 | 7 | 102 | 222 | 6.4 | 26 | 21 | |
| 8_2 | 125 | 10 | 3 | 113 | 245 | 3.4 | 21 | 21 | |
| 9_2 | 125 | 20 | 3 | 112 | 196 | 4.1 | 33 | 33 | |
| 10_2 | Axial | 50 | 15 | 5 | 52 | 166 | 2.4 | 28 | 9 |
| 11_2 | 150 | 15 | 5 | 101 | 229 | 3.2 | 24 | 19 | |
| 12_2 | 100 | 15 | 9 | 77 | 189 | 2.9 | 36 | 17 | |
| 13_2 | 100 | 15 | 1 | 86 | 214 | 1.1 | 28 | 16 | |
| 14_2 | 100 | 25 | 5 | 80 | 187 | 1.4 | 37 | 18 | |
| 15_2 | 100 | 5 | 5 | 84 | 189 | 2.5 | 36 | 20 | |
| T | t | U | s | d | D | p | σ | |
|---|---|---|---|---|---|---|---|---|
| T | 1.00 | −0.23 | 0.29 | 0.17 | −0.25 | 0.06 | 0.23 | −0.18 |
| t | −0.52 | 1.00 | 0.05 | 0.64 | 0.23 | −0.16 | −0.31 | 0.26 |
| U | −0.12 | 0.14 | 1.00 | −0.35 | 0.03 | 0.21 | 0.02 | 0.11 |
| s | −0.37 | 0.76 | 0.12 | 1.00 | −0.10 | 0.19 | 0.29 | −0.25 |
| d | −0.45 | 0.63 | 0.41 | 0.83 | 1.00 | 0.90 | 0.96 | −0.04 |
| D | −0.51 | 0.56 | 0.61 | 0.61 | 0.78 | 1.00 | −0.87 | −0.29 |
| p | −0.01 | 0.24 | −0.12 | 0.54 | 0.59 | −0.03 | 1.00 | 0.14 |
| σ | 0.37 | −0.42 | −0.53 | −0.51 | −0.62 | −0.91 | 0.17 | 1.00 |
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Riccomagno, E.; Shayganpour, A.; Salerno, M. Optimization of Anodic Porous Alumina Fabricated from Commercial Aluminum Food Foils: A Statistical Approach. Materials 2017, 10, 417. https://doi.org/10.3390/ma10040417
Riccomagno E, Shayganpour A, Salerno M. Optimization of Anodic Porous Alumina Fabricated from Commercial Aluminum Food Foils: A Statistical Approach. Materials. 2017; 10(4):417. https://doi.org/10.3390/ma10040417
Chicago/Turabian StyleRiccomagno, Eva, Amirreza Shayganpour, and Marco Salerno. 2017. "Optimization of Anodic Porous Alumina Fabricated from Commercial Aluminum Food Foils: A Statistical Approach" Materials 10, no. 4: 417. https://doi.org/10.3390/ma10040417
APA StyleRiccomagno, E., Shayganpour, A., & Salerno, M. (2017). Optimization of Anodic Porous Alumina Fabricated from Commercial Aluminum Food Foils: A Statistical Approach. Materials, 10(4), 417. https://doi.org/10.3390/ma10040417







