Research on Multi-Machine Pre-Synchronization Control and Optimization Based on Parallel Recovery Black Start

Abstract

With the increasing prevalence of renewable energy, microgrids play a crucial role in enhancing distributed energy efficiency and system flexibility. However, the intermittent and unpredictable nature of renewable energy generation presents significant challenges for microgrid restoration and stable operation. Black-start technology, a key method for autonomous power restoration, is essential for ensuring reliable microgrid operation. Grid-forming virtual synchronous generators (VSGs), with inherent inertia support and regulation capabilities, autonomously establish the voltage, meeting the power supply demands of black-start processes. However, during the pre-synchronization of multiple distributed energy resources in black-start scenarios, rapid phase-angle adjustments can cause frequency fluctuations due to the coupling between the frequency and phase angle. This coupling often leads to frequency overshoot and decreased system stability. To address this challenge, this paper proposes an enhanced parallel restoration strategy for a multi-source black start. Optimizing phase-angle control reduces the dependency on phase-locked loops (PLLs), mitigates phase-angle difference jumps, and accelerates the pre-synchronization process. Furthermore, a linear active disturbance rejection controller (LADRC) dynamically compensates for frequency fluctuations, effectively decoupling the frequency from the phase angle. This approach improves synchronization accuracy and enhances parallel reliability among multiple distributed energy resources (DERs). Simulation results show that the proposed method suppresses frequency overshoot and system disturbances during a multi-source black start, significantly enhancing microgrid restoration capability and operational stability.

1. Introduction

The ongoing global energy transition and the widespread integration of renewable energy sources have profoundly impacted the operations of traditional power grids. Renewable energy sources such as photovoltaic (PV) and wind power, due to their inherent intermittency and variability, pose significant challenges to the stability and reliability of power systems [1,2]. In this context, microgrids have emerged as a key solution for integrating distributed energy sources, offering controllability and flexibility to address the challenges posed by renewable energy grid integration. However, traditional grid-following inverters face difficulties in adapting to systems with a growing share of renewable energy, leading to the development of grid-forming VSG technology. A VSG improves the dynamic response and operational stability of microgrids by emulating the inertia and regulatory characteristics of synchronous generators [3,4]. As power systems expand, microgrid structures and operations are becoming more complex and variable, which increases the risk of localized failures that could escalate into widespread outages or even system collapse. Microgrids must independently restore power supplies through black-start technology. VSG-based grid control strategies offer frequency and voltage support, making them ideal for the short-term voltage requirements of black-start operations. Consequently, VSG-based black-start strategies can quickly establish voltage and frequency references after a power system failure, ensuring reliable startup conditions for other devices [5,6].

In [7,8], VSG control strategies for single inverters are primarily explored, though these are often limited by capacity constraints. To enhance system capacity, a control strategy for multi-machine energy storage systems is proposed in [9]. While standalone photovoltaic systems lack black-start capability, integrating them with energy storage systems improves black-start efficiency. However, maintaining the stability of the photovoltaic system requires a high level of precision and reliability [10]. In [11,12], a sequential recovery black-start strategy is proposed, where a designated black-start microsource first establishes the system frequency and voltage before gradually synchronizing additional microsources. This approach ensures high startup reliability and a relatively simple control system structure. However, its main limitation is the underutilization of black-start capabilities, as only one microsource is designated for the black start, which limits improvements in the reliability of the microgrid power supply. Furthermore, sequential recovery requires the startup of each microsource followed by grid connection, leading to excessive time consumption that conflicts with the rapid startup demands of black-start processes [13].

In contrast, Ref. [14] proposes a parallel restoration strategy designed to achieve faster grid restoration. This approach enables multiple black-start-capable microsources to start simultaneously, forming several small subsystems. It offers rapid restoration and operational flexibility and aligns with the structural characteristics of microgrids, facilitating the swift re-establishment of power supply across the entire system. However, in parallel systems, even minor mismatches before synchronization can lead to significant disturbances [15]. Achieving simultaneous grid connection of multiple small subsystems before parallelization presents greater challenges and is more vulnerable to disturbances. In [16], the causes of oscillations induced by micro-power control mode transitions during black-start operations are investigated, and a smooth switching control method based on controller state tracking is proposed. This method enables seamless mode switching by refining the implementation of the control strategy, facilitating the rapid black start of the microgrid. However, this approach has limitations in achieving precise parallel synchronization, resulting in significant disturbances during parallel connection. Ref. [17] describes a method to synchronize microsources through power control loops; however, the synchronization time is relatively long. In [18,19,20], pre-synchronization is employed before the parallel connection of multiple microsources to eliminate amplitude and phase-angle differences in their output voltages. This approach effectively mitigates the risk of black-start failure caused by current surges resulting from amplitude or phase mismatches during parallel connection. However, it overlooks the coupling between the frequency and phase angle during pre-synchronization, making the system prone to frequency perturbations due to rapid phase-angle changes, which can significantly impact the overall parallel black-start system [21]. With the advancement of artificial intelligence algorithms, integrating intelligent algorithms with proportional–integral–derivative (PID) control has proven highly effective in addressing challenges related to strong coupling and nonlinearity in control systems [22,23]. For instance, Ref. [24] proposes an intelligent learning PID control algorithm that adapts and optimizes PID parameters to manage strong coupling between the frequency and phase angle. While this approach enhances system robustness and stability, it also presents challenges such as increased design complexity and longer computation times. Ref. [25] introduces a model predictive control (MPC)-based VSG strategy that analyzes the coupling mechanism between the frequency and phase angle. By incorporating frequency variations to adaptively adjust the weighting coefficients of MPC and dynamically modifying the power reference value of the VSG, this strategy alleviates the synchronization impact caused by frequency–phase-angle coupling to some extent. However, it faces challenges in solving the constrained optimization problems inherent to the MPC framework.

Compared with existing studies, this paper addresses several limitations in multi-machine black-start pre-synchronization strategies. While the methods in [16,17] rely heavily on multiple PLLs and suffer from phase-angle jumps, this study improves the traditional phase-angle difference measurement, reducing PLL usage and eliminating sudden phase-angle changes, thus accelerating the pre-synchronization process. Furthermore, unlike [18,19,20], which overlook the frequency overshoot caused by the coupling between the phase angle and frequency during rapid phase adjustments, this research integrates a LADRC to dynamically adjust the frequency in response to phase-angle fluctuations. By leveraging the LADRC’s strong disturbance rejection and fast dynamic response, the proposed method effectively suppresses frequency overshoot and mitigates adverse coupling effects. In contrast to the complex objective functions used in [24,25] to model frequency and phase-angle differences, the proposed approach adopts a more streamlined control algorithm, enhancing system robustness and simplifying implementation.

Building on these insights, this paper proposes an enhanced phase-angle control strategy combined with a LADRC to improve pre-synchronization accuracy and system stability. The key contributions are as follows:

(1)

Enhanced phase-angle measurement: By refining the phase-angle difference calculation, the proposed method reduces PLL dependence and eliminates phase-angle jumps, accelerating pre-synchronization.

(2)

Frequency–phase-angle decoupling: The integration of the LADRC mitigates the coupling effect between the frequency and phase angle, effectively suppressing frequency overshoot and ensuring smoother dynamic responses.

(3)

Improved system robustness: The simplified control strategy avoids complex objective functions, striking a balance between synchronization speed and system stability.

Ultimately, this strategy enhances synchronization accuracy among black-start microsources, reduces disturbances during parallel grid connection, and strengthens the microgrid’s recovery capability, offering a practical solution to the challenges of multi-machine black start.

2. Multi-VSG Black-Start Strategy

2.1. Comparison of Grid Recovery Strategies

Orphaned grid black start refers to the independent operation of a power system initiated by self-starting units, without the support of an external grid. The process involves progressively restoring the system by gradually expanding the recovery scope until full restoration is achieved. This typically occurs after an initial startup or following a complete shutdown due to faults.

The black-start process consists of three main stages [26]: the startup of black-start microsources, grid restoration, and load casting. Among these, grid restoration is a critical component, typically classified into two types, serial and parallel restoration, as summarized in

Table 1. Comparison of grid recovery strategies.

Grid Recovery Strategy	Serial Recovery	Parallel Recovery
Startup sequence	The process begins by starting a single black-start microsource	The simultaneous activation of multiple black-start microsources
Recovery process	The other microsources are sequentially synchronized with the black-start microsource and then operate in parallel	After each group operates under a load, a synchronized parallel connection is established to form an independent subsystem
Advantages and disadvantages	The simple control process leads to slower grid recovery	Fast grid recovery requires high synchronization performance

.

In microgrid black-start restoration, serial recovery begins with a single black-start microsource to establish the reference voltage, followed by the sequential synchronization of other microsources. This method is simple but slower, making it challenging to meet fast power supply demands. In contrast, parallel recovery activates multiple microsources simultaneously under a load, with subsystems pre-synchronized for stable operation, accelerating recovery. However, pre-synchronizing multiple subsystems is more complex, and small deviations can significantly affect stability, increasing synchronization performance requirements. Therefore, improving the pre-synchronization performance of multiple black-start microsources is indispensable for faster grid recovery and ensuring system safety.

To meet the isolated microgrid black-start requirements, multiple black-start microsources are precisely controlled by VSGs, providing stable frequency and voltage support. Additionally, the black-start microsource VSG’s DC side employs a photovoltaic energy storage strategy, ensuring sufficient capacity and effectively stabilizing the DC bus voltage.

2.2. Multi-VSG Black-Start Control

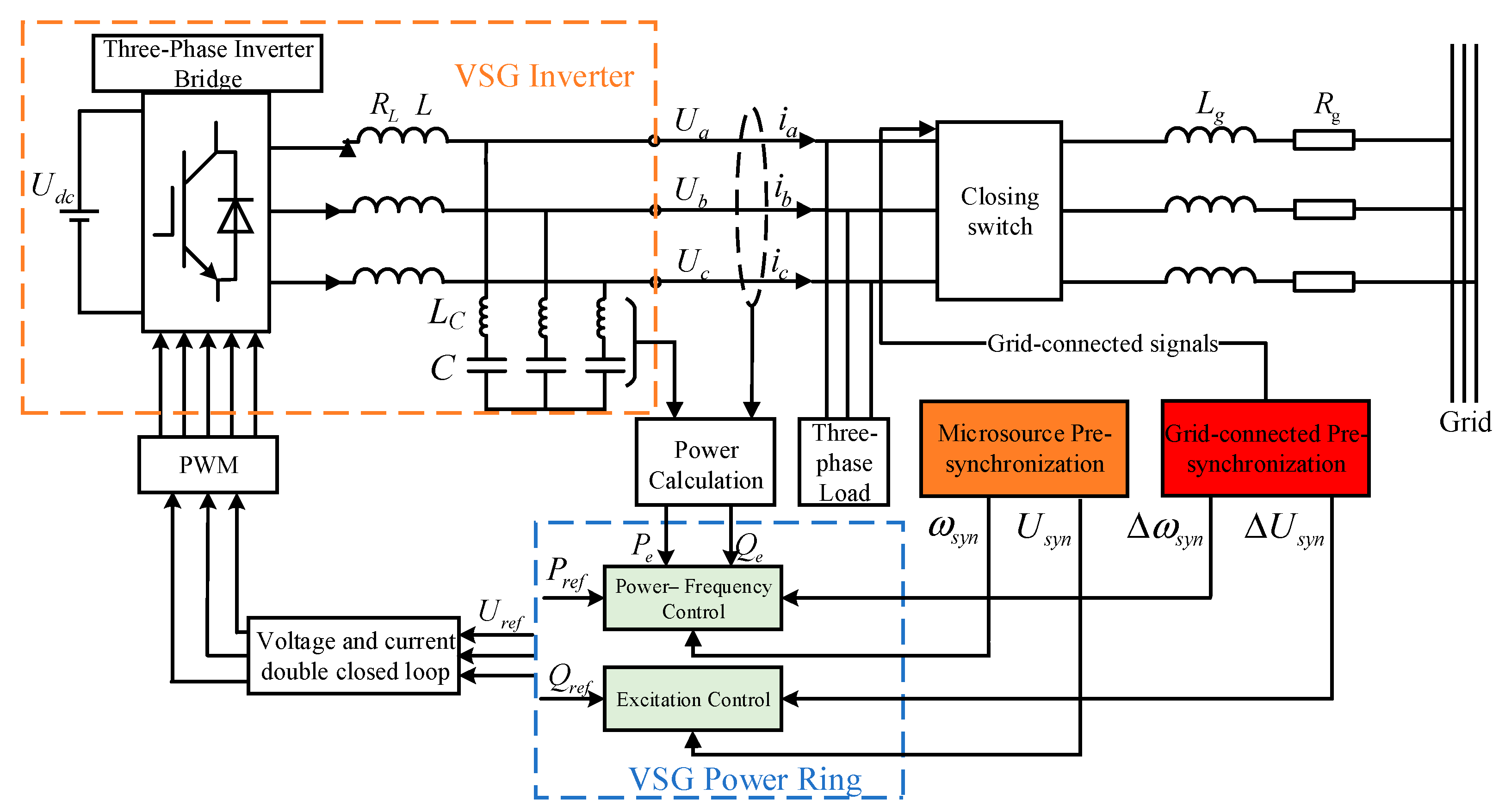
2.2.1. VSG Networking/Parallel Modeling

The block diagram of the VSG control structure is shown in Figure 1. U_dc is the DC-side voltage; L, R_L and L_C, C are the filter impedance and filter capacitance, respectively; U_i (i = a, b, c) is the three-phase output voltage of the inverter side; i (i = a, b, c) is the output current of the inverter; and L_g and R_g constitute the grid impedance.

The VSG Power Ring mainly contains active and reactive loops, which simulate the primary voltage regulation and primary frequency regulation characteristics of synchronous generators, respectively. In VSG active-frequency control, virtual inertia and damping are implemented to emulate the external characteristics of synchronous generators, offering voltage and frequency support to the system.

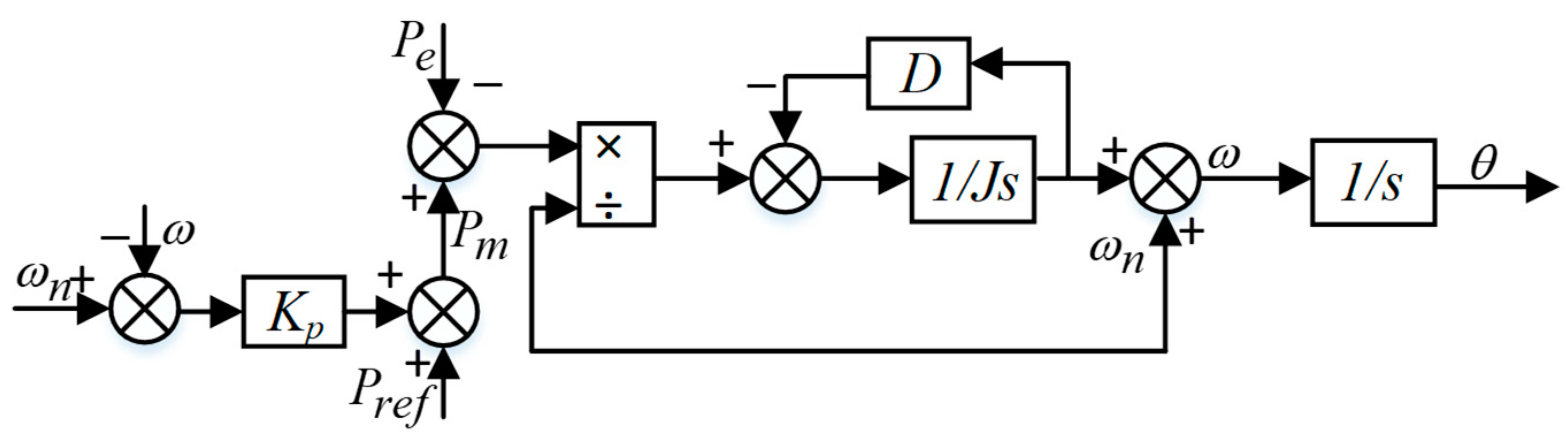
(1)

Active-Frequency Control:

The active-frequency control equation for the VSG is expressed as follows [27]:

$$\begin{array}{ll}& J{\displaystyle \frac{d\omega }{t}}=T_m-T_e-D\left(\omega -{\omega }_n\right)={\displaystyle \frac{P_m}{\omega }}-{\displaystyle \frac{P_e}{\omega }}-D\left(\omega -{\omega }_n\right)\\ & {\displaystyle \frac{d\theta }{dt}}=\omega \end{array}$$

(1)

The VSG governing equation is

$$P_m=P_{ref}+K_p(\omega -{\omega }_n)$$

(2)

In the above equation, J represents the virtual inertia, and D denotes the damping coefficient. T_e and T_m correspond to the electromagnetic and mechanical torques, respectively, while P_m and P_e refer to the mechanical and electromagnetic power. ω_n is the rated angular velocity, and ω is the actual angular velocity. θ is the power angle. The active power reference is denoted by P_ref, and K_p represents the droop coefficient. Figure 2 illustrates the block diagram of the power–frequency control system.

However, the change in frequency affects the terminal voltage of the generator through electromagnetic coupling, so it is necessary to further introduce the voltage-reactive power equation to regulate the excitation voltage so as to maintain the voltage stability of the system. The voltage-reactive power equation regulates the terminal voltage and reactive power by controlling the excitation voltage to ensure that the system voltage is stabilized while the frequency is regulated.

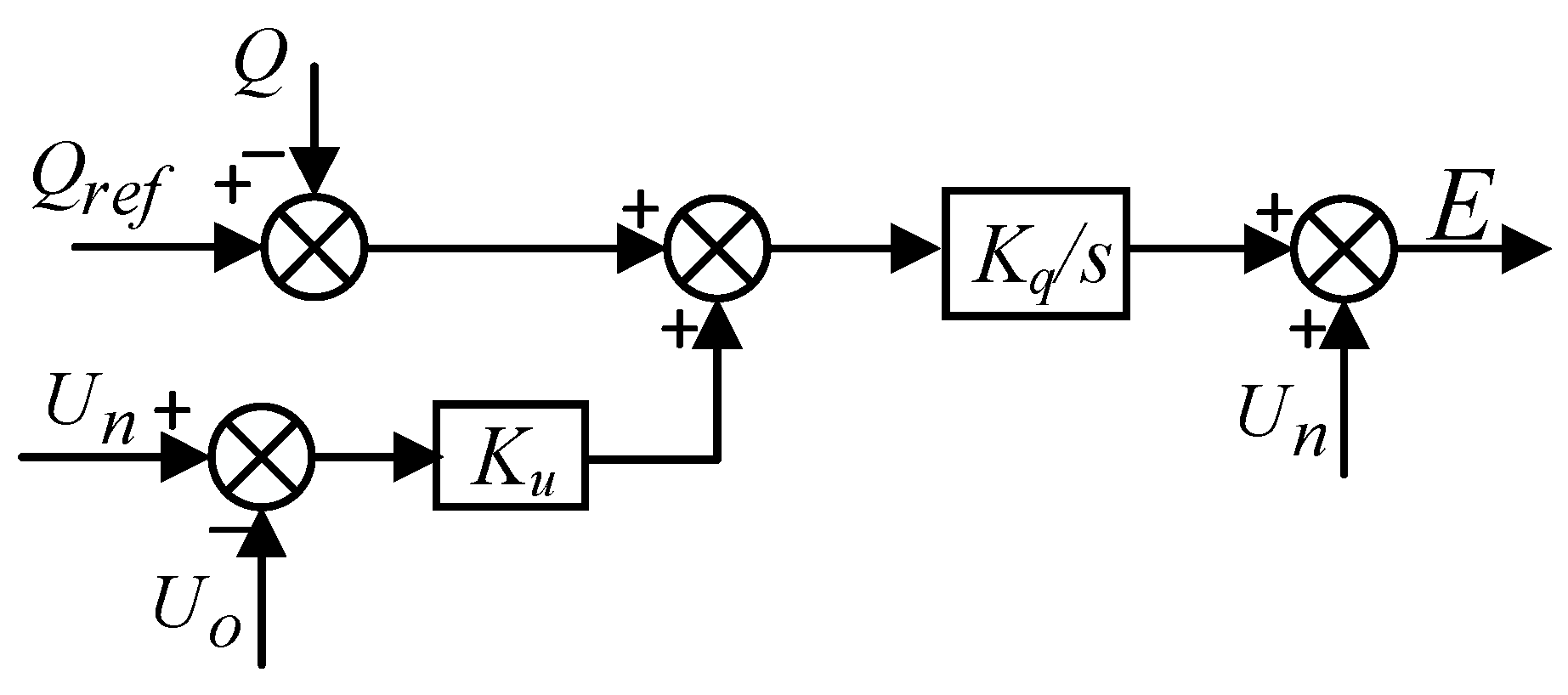
(2)

Voltage-Reactive Control:

The reactive power-voltage equation for VSG is

$$E=U_n+{\displaystyle \frac{K_q}{s}}[Q_{ref}-Q+K_u(U_n-U_0)]$$

(3)

The reactive power reference is denoted by Q_ref. U₀ and U_n represent the rated and actual voltages, respectively, while K_u and K_q denote the droop and integration coefficients, respectively. Figure 3 illustrates the block diagram of the excitation control system.

The desired voltage is achieved by adjusting the voltage amplitude and phase through the active and reactive power control loops, respectively. The output is then processed via a voltage–current dual closed-loop system to generate the final PWM waveform.

During grid restoration, pre-synchronization among black-start microsources generates the frequency perturbation ω_syn and voltage perturbation U_syn required for frequency and excitation control, enabling voltage synchronization between microsources and ultimately forming a parallel network. Following the islanded black start, the synchronized microsource voltages are further aligned with the grid voltage by generating additional frequency perturbation Δω_syn and voltage perturbation ΔU_syn through pre-synchronization. These perturbations are superimposed onto the active and reactive power loops.

Therefore, in order to improve the black-start capability, the analysis of the pre-synchronization mechanism between black-start microsources is essential.

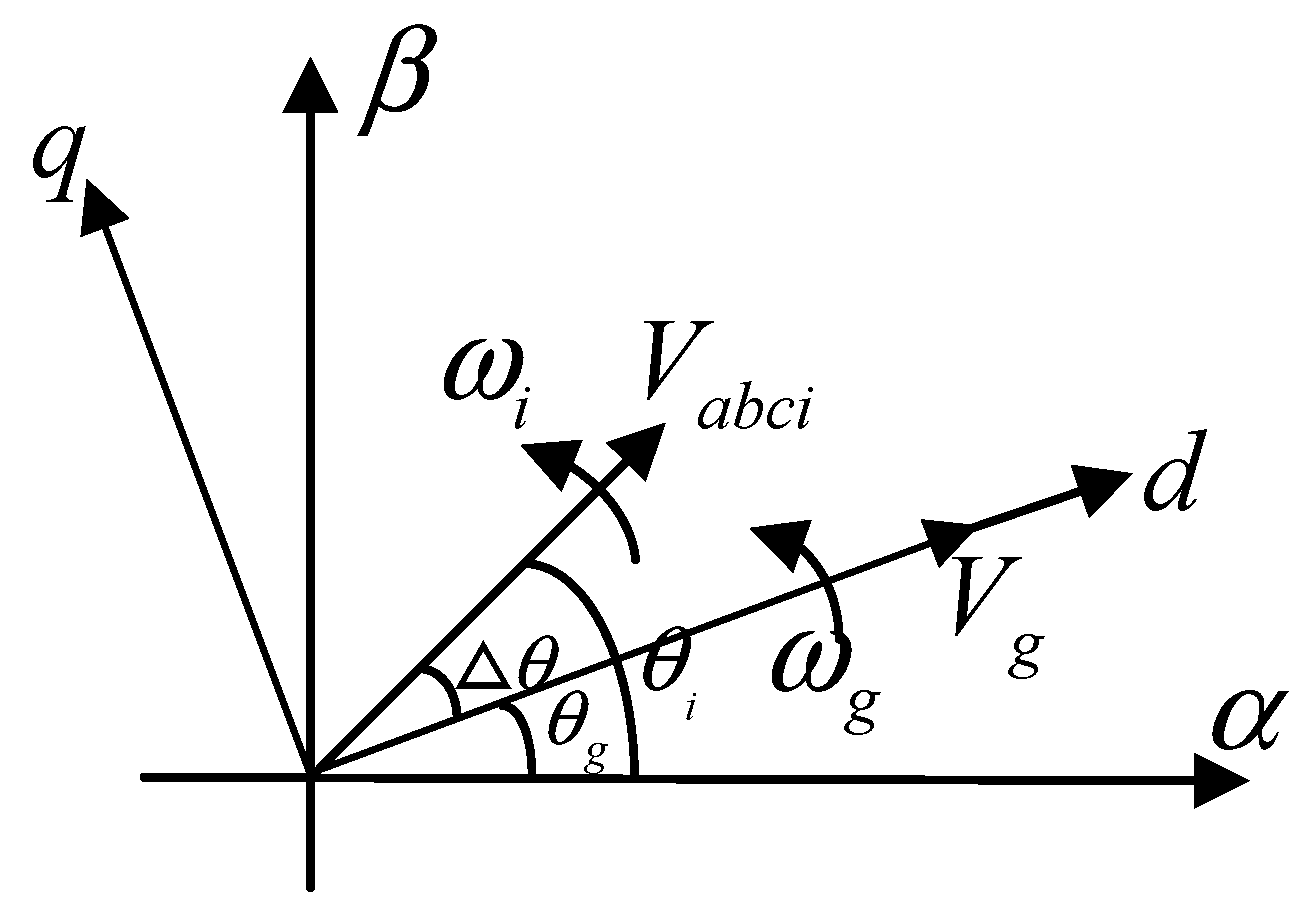
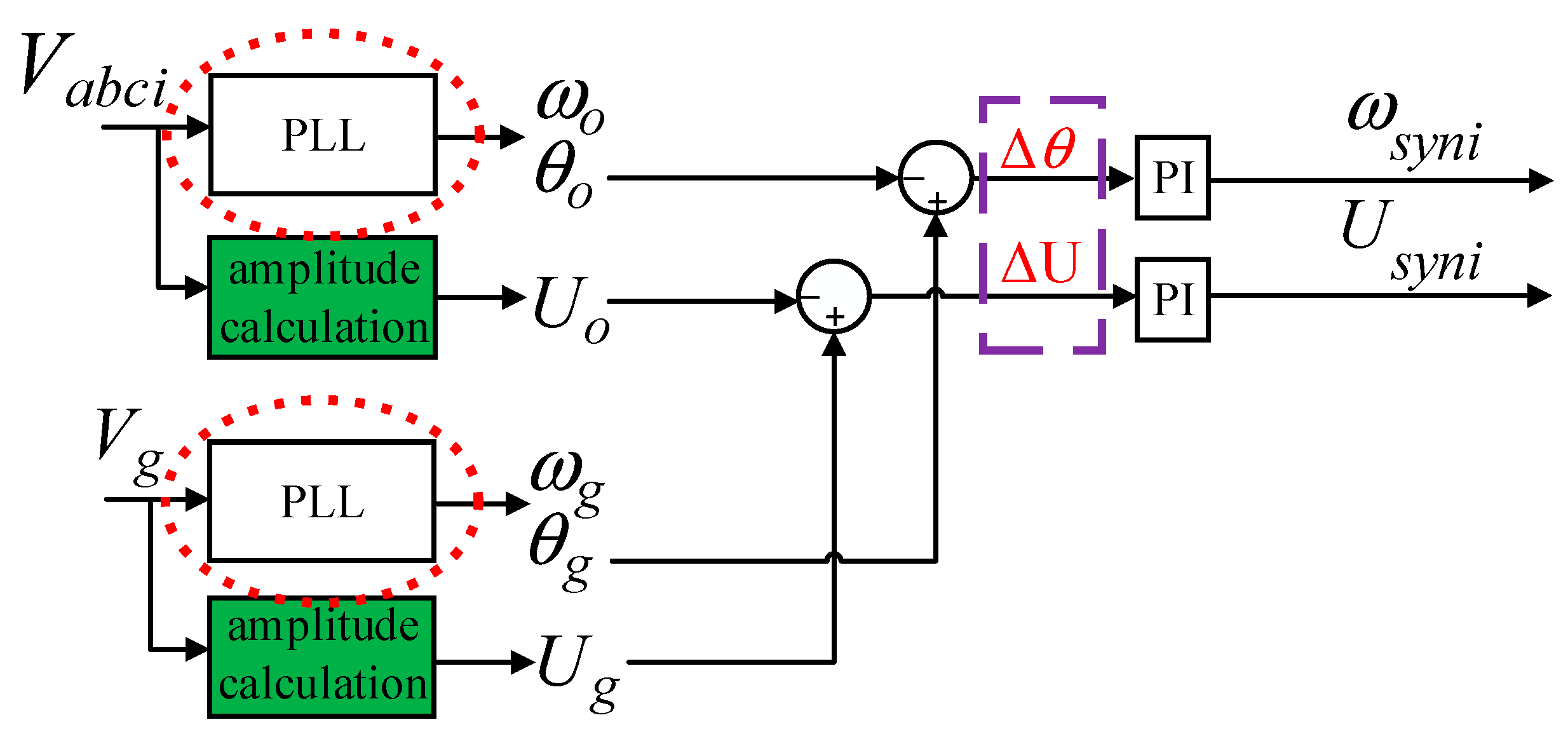
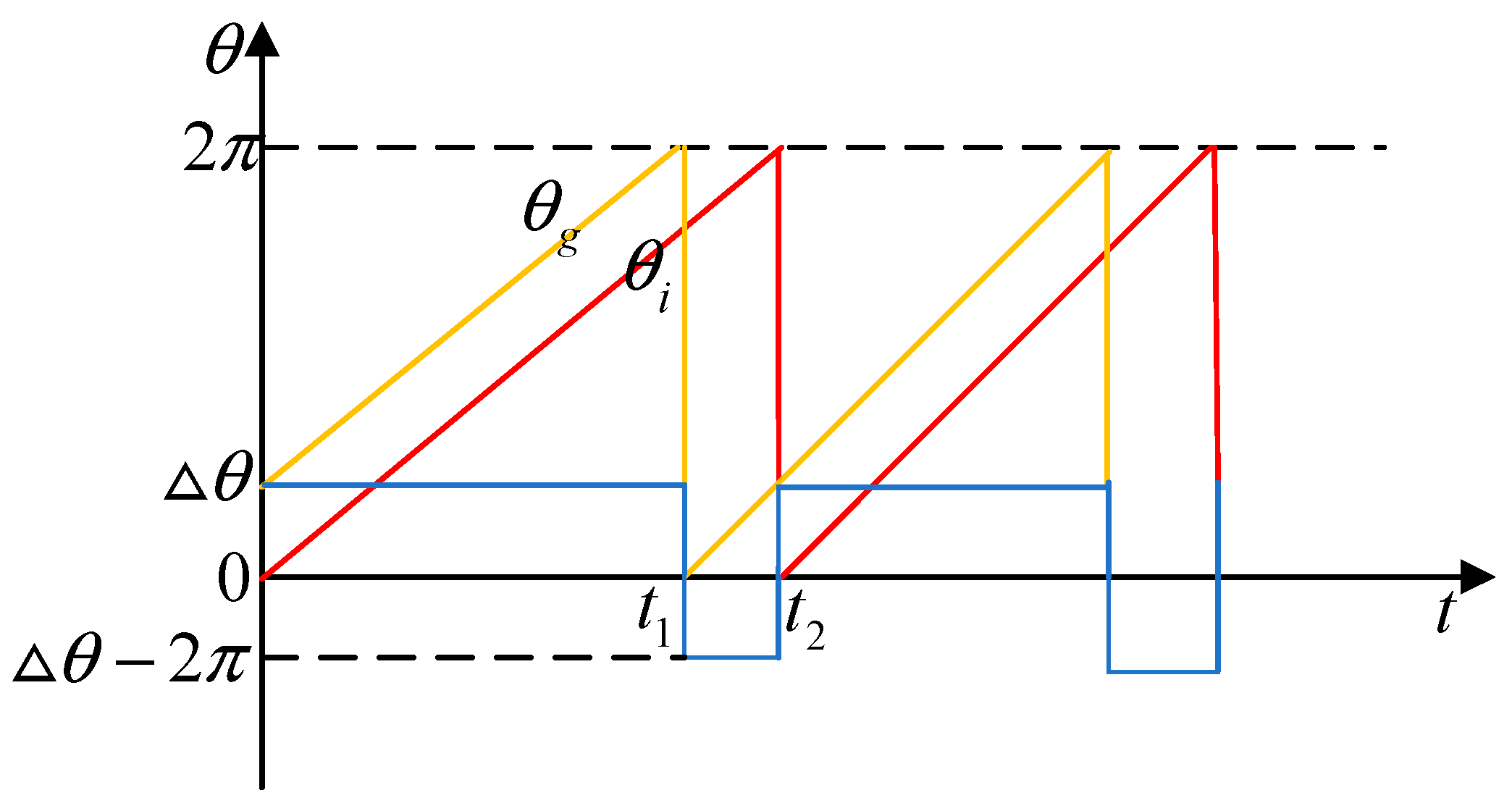
2.2.2. Multi-Machine Pre-Synchronized Phase-Angle Control Strategy

If three photovoltaic energy storage VSGs are used as black-start microsources, VSG₁ is designated as the main reference power supply to establish the reference voltage. Before synchronization, the other two VSGs must align their output voltages with VSG₁ to avoid impacts during system integration that could disrupt the black-start process. Due to parallel recovery, the three black-start microsource VSGs operate independently before being integrated in parallel. VSG₂ and VSG₃ are synchronized with VSG₁ individually, with each synchronization process occurring independently. The overall system microsource synchronization can be compared to the pre-synchronization process of a single machine.

In islanded operation, differences in angular frequency, phase angle, and amplitude may exist between the output voltages of VSG_i (i = 2, 3), V_abci, and the output voltage of VSG₁, V_g. These differences can cause an instantaneous voltage disparity at the common coupling point (PCC), with a peak value approximated at 2 U. The voltage difference is calculated as follows [28]:

$$\begin{array}{c}\Delta U=V_g-V_{abci}\\ =U_g\sin \left({\omega }_{g}t+{\theta }_g\right)-U_{\mathrm{o}}\sin \left({\omega }_{i}t+{\theta }_i\right)\\ \approx 2U\end{array}$$

(4)

The deviations in the components of the two voltages are governed by nonlinear control, resulting in a complex process due to the coupling between the frequency and phase angle. In contrast, amplitude control is linear. Thus, the critical aspect of pre-synchronization is managing the coupling between the frequency and phase angle.

During multi-machine pre-synchronization, the output voltage of VSG_i synchronously tracks the voltage of VSG₁. The voltage vector diagram illustrating this tracking process is shown in Figure 4.

As shown in the figure, the two voltages rotate at their respective angular frequencies, causing a time-varying Δθ. If Δθ can be constrained within an allowable range and the linear adjustment of the amplitude is appropriately performed, the two vectors can coincide, achieving pre-synchronization [18,19,20]. The block diagram is shown in Figure 5.

Using a conventional PLL to obtain the phase angle presents challenges. Not only is the PLL complex to control, but it also causes Δθ jumps when directly extracting Δθ. The voltage phase varies from 0 to 2π, with a discontinuity from 2π back to 0 in each cycle. For example, in Figure 6, at time t₁, θ_g reaches a maximum of 2π and then jumps to 0 at the next moment. However, due to the lag of θ_i, it has not yet reached its peak, causing to decrease by 2π, and thus, Δθ becomes Δθ−2π between t₁ and t₂.

Jumps in the sign of Δθ alter the original adjustment trend and prolong the synchronization adjustment time. Thus, the impact of Δθ jumps can be eliminated by enhancing the phase-angle controller.

To address the over-reliance on the PLL and Δθ, the conventional phase-angle difference acquisition method has been improved, and a new phase-angle control variable has been introduced.

Since sin(Δθ ± 2π) = sinΔθ, when Δθ increases or decreases by 2π, sinΔθ remains continuous without any sign jumps.

To verify the feasibility of this scheme, the approximation scheme errors and impacts are analyzed. Using the Taylor expansion, sinΔθ can be expressed as follows:

$$\mathrm{s}\mathrm{i}\mathrm{n}\Delta \theta =\Delta \theta -{\displaystyle \frac{1}{6}}(\Delta \theta {)}^3+O((\Delta \theta {)}^5)$$

(5)

The approximation error E is given by

$$E=\mathrm{s}\mathrm{i}\mathrm{n}(\Delta \theta )-\Delta \theta =-{\displaystyle \frac{1}{6}}(\Delta \theta {)}^3+O((\Delta \theta {)}^5)$$

(6)

For small Δθ, the higher-order terms can be neglected, making the substitution sinΔθ ≈ Δθ valid. However, as Δθ increases, the nonlinear terms introduce a compression effect, causing the input to the controller to shrink. This shows that when Δθ is small, the difference between sinΔθ and Δθ mainly comes from the cubic nonlinear term. The characteristics of this error are as follows:

(1)

Negligible for small angles (e.g., <10°);

(2)

Rapidly increasing for larger angles (e.g., >30°), following a cubic relationship.

The PI controller takes sinΔθ as input and outputs the frequency compensation Δω:

$$\Delta \omega =K_p\mathrm{s}\mathrm{i}\mathrm{n}(\Delta \theta )+K_i\int \mathrm{s}\mathrm{i}\mathrm{n}(\Delta \theta )dt$$

(7)

Introducing the error E, we obtain

$$\Delta \omega =K_p(\Delta \theta +E)+K_i[(\Delta \theta +E)]dt$$

(8)

Breaking it down into the traditional method and the error contribution,

$$\Delta \omega =\underset{\mathrm{Traditional} \mathrm{method}}{\underbrace{K_p\Delta \theta +K_i\int \Delta \theta dt}}+\underset{\mathrm{Error} \mathrm{contribution}}{\underbrace{K_{p}E+K_i\int Edt}}$$

(9)

The frequency compensation error is

$$\Delta {\omega }_{smor}=K_{p}E+K_i\int Edt$$

(10)

By substituting $E=-{\displaystyle \frac{1}{6}}(\Delta \theta {)}^3$,

$$\Delta {\omega }_{srrar}=-{\displaystyle \frac{K_p}{6}}(\Delta \theta {)}^3-{\displaystyle \frac{K_i}{6}}[(\Delta \theta {)}^3]dt$$

(11)

Assuming Δθ changes linearly over a short period,

$$\Delta \theta (t)\approx \Delta {\theta }_0+\Delta \omega t$$

(12)

The integral becomes

$$\int (\Delta \theta {)}^{3}dt\approx (\Delta {\theta }_0{)}^{3}t$$

(13)

The final error expression is

$$\Delta {\omega }_{srrar}=-{\displaystyle \frac{K_p}{6}}(\Delta \theta {)}^3-{\displaystyle \frac{K_i}{6}}(\Delta {\theta }_0{)}^{3}t$$

(14)

This indicates that the instantaneous error term is proportional to the cube of Δθ. Accumulated Error Term: The error accumulation is more pronounced as time grows linearly and Δθ increases.

Integrating Δω_error,

$${\theta }_{srnor}(t)=-{\displaystyle \frac{K_p}{6}}(\Delta \theta {)}^{3}t-{\displaystyle \frac{K_i}{12}}(\Delta {\theta }_0{)}^3{t}^2$$

(15)

From the above equation, we can see that the first-order term increases linearly with time, affecting the transient response, and the second-order term shows that synchronization errors accumulate at an accelerating rate over time.

Let us compute the instantaneous and cumulative errors for two cases.

When Δθ = 10° (0.1745 rad), t = 0.1 s,

$$\begin{array}{ll}{\theta }_{srrov}(0.1)=& -{\displaystyle \frac{K_p}{6}}(0.1745{)}^3\times 0.1-{\displaystyle \frac{K_i}{12}}(0.1745{)}^3\times (0.1{)}^2\\ & \approx -0.000088K_p-0.0000044K_i\end{array}$$

(16)

When Δθ = 30°(0.5236 rad), t = 0.1 s,

$$\begin{array}{c}{\theta }_{srnor}(0.1)=-{\displaystyle \frac{K_p}{6}}(0.5236{)}^3\times 0.1-{\displaystyle \frac{K_i}{12}}(0.5236{)}^3\times (0.1{)}^2\\ \approx -0.0024K_p-0.00012K_i\end{array}$$

(17)

From the above error results, we know that the approximation sinΔθ ≈ Δθ is highly accurate for angles smaller than 10°, with minimal impact on synchronization. When Δθ exceeds 30°, the cubic nonlinear term causes rapid error growth, leading to frequency compensation deviations and accumulated synchronization errors.

To avoid the impact of Δθ sign jumps on the pre-synchronization control process, pre-synchronization control can also be implemented by replacing sinΔθ with Δθ. The specific formula is

$$\mathrm{s}\mathrm{i}\mathrm{n}\Delta \theta =\mathrm{s}\mathrm{i}\mathrm{n}({\theta }_g-{\theta }_i)={\displaystyle \frac{U_{g\beta }{U}_{\mathrm{o}\alpha }-U_{g\alpha }{U}_{o\beta }}{\sqrt{U_{\mathrm{o}\alpha }^2+U_{\mathrm{o}\beta }^2}\times \sqrt{U_{g\alpha }^2+U_{g\beta }^2}}}$$

(18)

The schematic diagram is illustrated in Figure 7.

Although the improved phase-angle control method significantly accelerates pre-synchronization, it overlooks the coupling between the frequency and phase angle.

For VSG_i (i = 2, 3), the relationship between the frequency and phase angle is described by

$${\displaystyle \frac{d\Delta {\theta }_i}{dt}}={\omega }_i-{\omega }_g$$

(19)

This equation indicates that frequency changes directly affect the phase-angle difference. The output active power P_ei of VSG_i is related to the phase-angle difference $\Delta {\theta }_i$ by

$$P_{ei}\approx {\displaystyle \frac{V_i{V}_g}{X_i}}\mathrm{s}\mathrm{i}\mathrm{n}\Delta {\theta }_i$$

(20)

Rapid changes in $\Delta {\theta }_i$ cause fluctuations in P_ei, which in turn affect the frequency dynamics of VSG_i:

$$J_i{\displaystyle \frac{d{\omega }_i}{dt}}=P_{mi}-P_{si}-D_i({\omega }_i-{\omega }_g)$$

(21)

In a discrete period T_s, the relationship between the phase angle and angular frequency can be expressed as follows:

$${\displaystyle \frac{{\theta }_i(k+1)-{\theta }_i(k)}{T_s}}\approx {\omega }_i(k+1)=\Delta {\omega }_i(k+1)+{\omega }_g$$

(22)

Thus, from the above frequency–phase-angle coupling relationship, it follows that when sinΔθ ≈ Δθ rapidly converges to zero, it induces rapid frequency fluctuations, leading to frequency overruns. During the parallel black start of multiple machines, unresolved frequency disturbances can cause significant impacts at the moment of gate closure and paralleling. In severe cases, this may lead to a black-start failure of the microgrid.

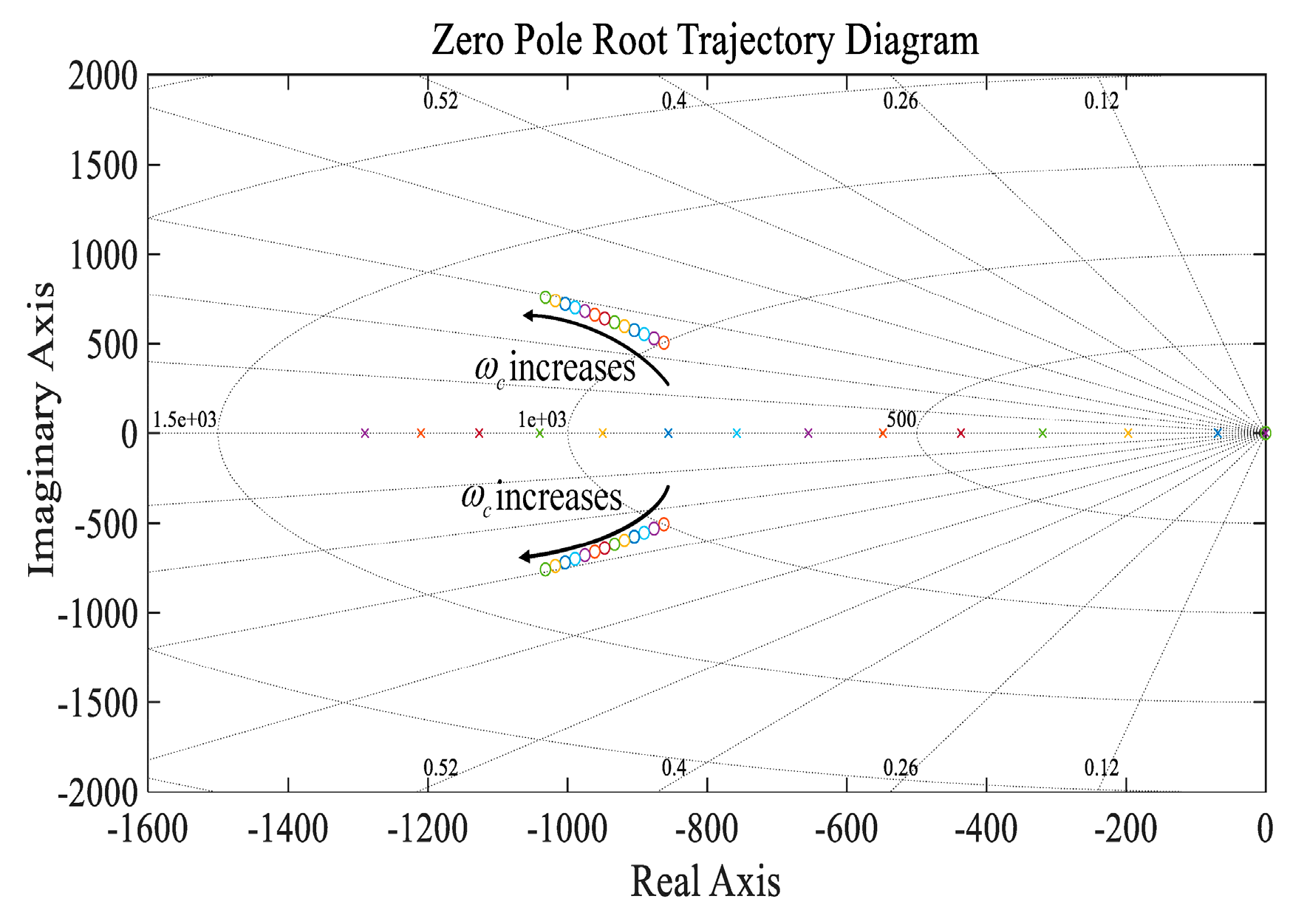
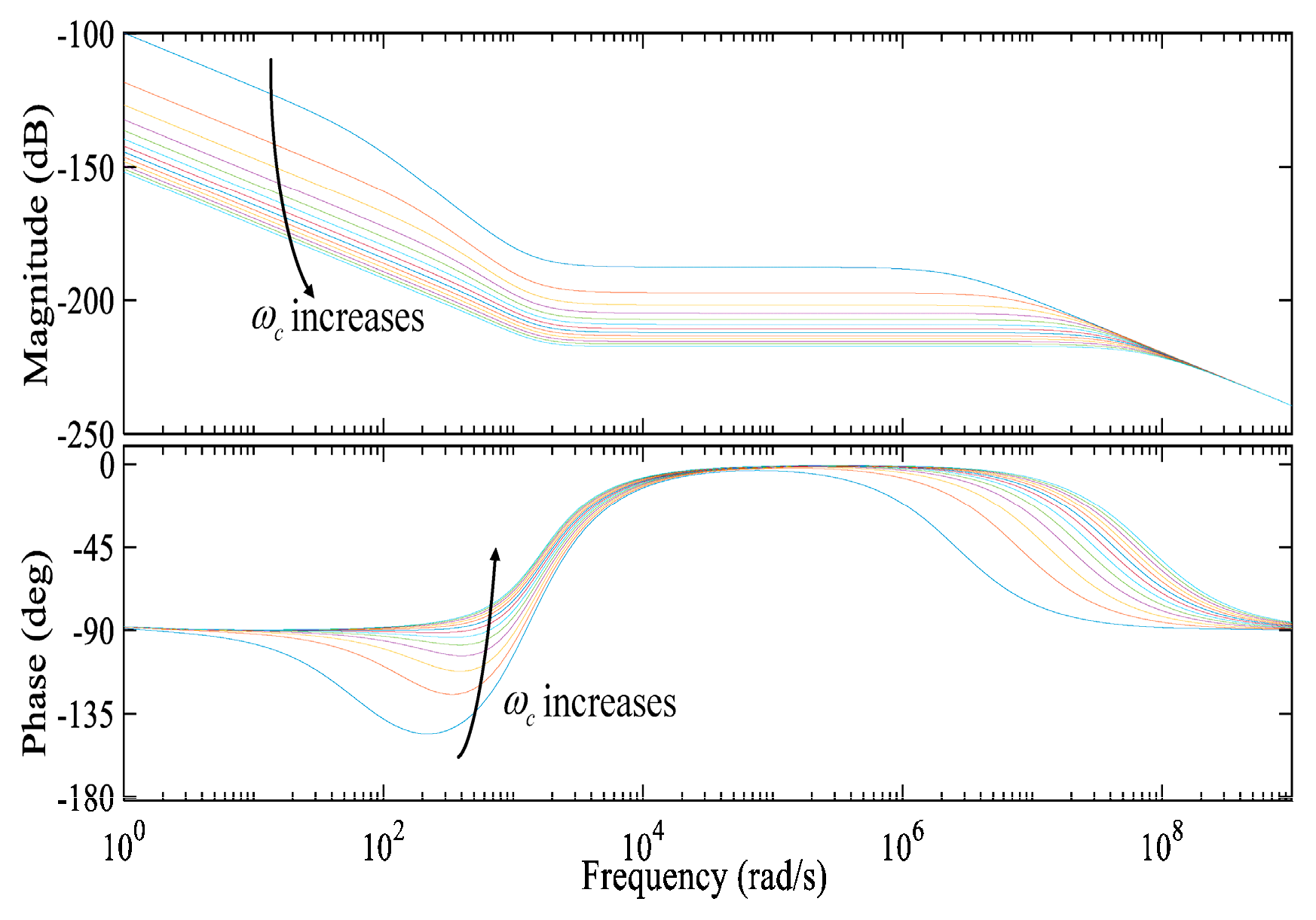
2.2.3. LADRC Adaptive Adjustment

Traditional PI controllers struggle to handle nonlinear control issues, such as frequency and phase-angle adjustments. Therefore, LADRC control is introduced due to its strong immunity and fast dynamic response, enabling rapid adjustments to suppress frequency fluctuations, thus minimizing overshoot or deviation.

The second-order LADRC consists of a linear extended state observer (LESO) and linear state error feedback (LSEF). The entire LADRC is integrated into the active loop of VSG_i (i = 2, 3) to regulate the output frequency. The frequency output dynamics can be modeled as a second-order linear system. In traditional VSG power–frequency control, the nominal angular frequency ωn serves as the reference frequency. The power–frequency control loop of the VSG adjusts the power compensation based on the deviation between the nominal angular frequency and the actual output angular frequency, thereby regulating the system frequency. However, this control method, relying on a fixed reference frequency, exhibits a limited ability to suppress external disturbances, especially under large disturbances. Since ωn remains constant, frequency deviations may accumulate over time.

To address this issue, the LADRC is introduced to replace the fixed nominal angular frequency ωn as the dynamic reference, forming a closed-loop connection with the system’s output frequency. Specifically, the LESO in the LADRC continuously observes the total disturbance within the system, including unmodeled dynamics and external disturbances, dynamically estimating the frequency state and its variation. Simultaneously, linear state error feedback (LSEF) calculates the frequency deviation and, combined with the observed disturbance, generates a dynamic compensation signal, u. This compensation signal adaptively corrects frequency deviations and rapidly mitigates the impact of disturbances, ensuring the dynamic stability of the frequency. Figure 8 shows the block diagram of LADRC-VSG adaptive control.

The reference frequency ω_n represents the original expected value. The system output corresponds to the actual frequency. b₀ denotes the system gain.

The second-order LADRC system comprises the LESO and LSEF [29]. Consider a linear second-order single-input, single-output system object as follows:

$$\ddot{\omega }=-a_1\dot{\omega }-a_2\omega +r+bu$$

(23)

In the equation, u and ω represent the system’s input and output, respectively. r denotes the external disturbance, a₁ and a₂ are the system parameters, and b is the system gain, approximated as b ≈ b₀, where b₀ is the nominal value. Defining x₁ = ω, x₂ = ω, and x₃ = f(ω_n,$\dot{\omega }$,r) = −a₁$\dot{\omega }$ − a₂ω + r + (b − b₀)u as the total disturbance, which accounts for internal system uncertainties and external disturbances, this leads to the equation of state as follows:

$$\left\{\begin{array}{c}{\dot{x}}_1=x_2\\ {\dot{x}}_2=x_3+b_{0}u\\ {\dot{x}}_3=\dot{f}(\omega ,\dot{\omega },r)\\ \omega =x_1\end{array}\right.$$

(24)

In the equation, x₁ and x₂ represent the state variables of the system.

During the pre-synchronization of the three VSG black-start microsources, VSG₁ serves as the reference power supply, and VSG_i (i = 2, 3) is synchronized with VSG₁ using independent LADRC frequency control.

It should be noted in particular that, before the closing of the gate, VSG_i (i = 2, 3) has only independent coupling with VSG₁, and at this time, there is no direct coupling between VSG_i (i = 2, 3) and VSG₁, and thus, the total perturbation x₃ mainly originates from the following:

(1)

VSG₁’s own frequency perturbation d₁(t).

The output frequency of VSG1 may fluctuate slightly due to external perturbations or self-regulation. This affects the pre-synchronization process of VSG_i (i = 2, 3), causing them to require additional frequency adjustment to match VSG₁.

(2)

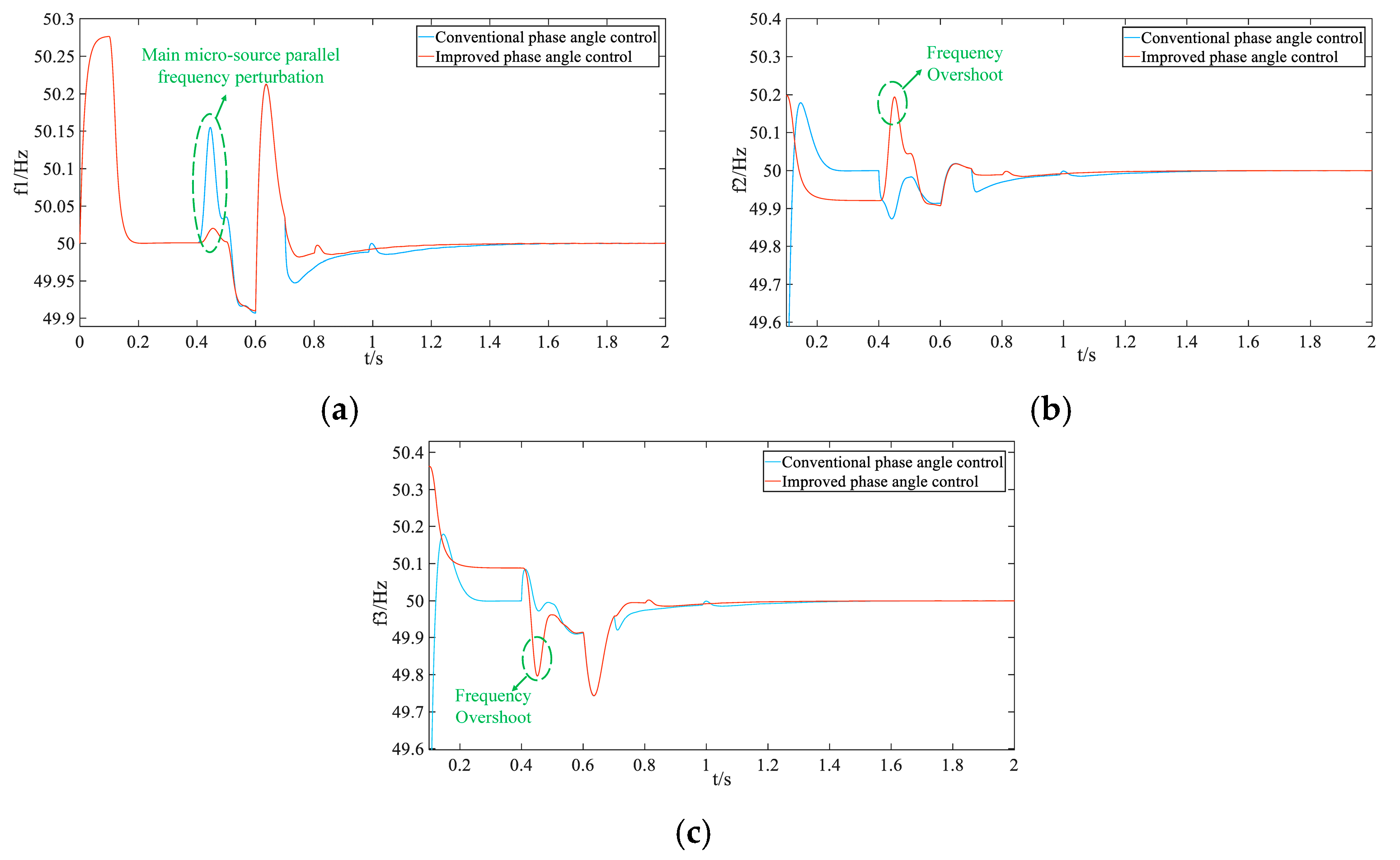
Frequency overshoot due to frequency–phase-angle coupling during pre-synchronization of VSG_i (i = 2, 3) and VSG₁.

Due to the inherent coupling between the frequency and phase angle, VSG_i (i = 2, 3) may experience transient frequency overshoot during frequency adjustment. This overshoot depends on the control strategy for pre-synchronization and the LADRC control parameters. According to the kinetic equation, x₃ can be decomposed into the following three parts.

Here,$\int k_p\Delta {\dot{\theta }}_{i}dt$ is the frequency perturbation caused by the rapid phase angle, since VSG_i (i = 2, 3) needs to adjust the phase angle gradually to match VSG₁, and $\Delta {\dot{\theta }}_i$ reflects the rapid change in the phase angle during the adjustment process.$\int k\Delta {\theta }_{i}dt$ is the frequency shift caused by the accumulation of phase-angle deviations, which causes a steady-state error in the frequency if there is a persistent deviation of the phase angle of VSG_i (i = 2, 3) from that of VSG₁. d₁(t) denotes the self-wavering fluctuation of VSG₁, and small perturbations of VSG₁ will be transmitted through the synchronization process of VSG_i (i = 2, 3) and cause the control system to be inaccurate. d₁(t) denotes the frequency shift caused by the phase-angle deviation. Due to self-fluctuations, small perturbations of VSG₁ will be transmitted through the VSG_i (i = 2, 3) synchronization process and lead to additional adjustments in the control system.

The LESO primarily estimates the system’s state (frequency), its rate of change, and external disturbances. The mathematical formulation of a LESO for a second-order system is as follows:

$$\begin{array}{c}{\dot{Z}}_1=Z_2+{\beta }_1(\omega -Z_1)\\ {\dot{Z}}_2=Z_3+{\beta }_2(\omega -Z_1)+b_{0}u\\ {\dot{Z}}_3={\beta }_3(\omega -Z_1)\end{array}$$

(25)

Z₁, Z₂, and Z₃ represent the current frequency estimate, the rate of change in frequency, and the total disturbance observation, respectively. β₁, β₂, and β₃ are the observer gains, responsible for adjusting the observer’s sensitivity to states and the speed of response. As can be seen, when Z₁ can accurately track the output ω, the error ω − Z₁ will converge to 0, and thus, Z₃ ≈ 0. During the dynamic response process, Z₃ will be continuously updated to reflect the real-time total perturbation x₃ of the system; i.e., Z₃ is actually an online estimate of x₃ in real time, which is used for the estimation of the frequency–phase-angle coupling, as well as the total perturbation triggered by the uncertain fluctuations within VSG₁ itself, and finally, the effect of Z₃ is reflected in the control input u, which is adjusted in real time by PD control.

Linear state error feedback (LSEF) is responsible for regulating the system’s control input via state feedback to achieve frequency regulation. Based on the LADRC control strategy, the input u guides the frequency toward the target value while suppressing overshoot. This process can be mathematically expressed as follows:

$$u={\displaystyle \frac{1}{b_0}}\left(-Z_3+x-k_p(Z_1-x)-k_d{Z}_2\right)$$

(26)

where k_p and k_d are the pending parameters of the PD controller, and k_p and k_d can be expressed as follows:

$$\left\{\begin{array}{c}{k}_p={\omega }_c^2\\ k_d=2\xi {\omega }_c\end{array}\right.$$

(27)

where ω_c is the controller bandwidth; ξ is the damping ratio. The characteristic equation of the LESO can be expressed as follows:

$$\lambda (s)=s^3+{\beta }_1{s}^2+{\beta }_{2}s+{\beta }_3$$

(28)

where the complex frequency s = jw. Choosing the ideal characteristic equation λ(s) = (s + ω₀)³, we have

$$\left\{\begin{array}{c}{\beta }_1=3{\omega }_0\\ {\beta }_2=3{\omega }_0^2\\ {\beta }_3={\omega }_0^2\end{array}\right.$$

(29)

where ω₀ is the observer bandwidth.

According to Figure 8, the closed-loop transfer function is deduced as follows:

$$\left\{\begin{array}{c}\omega (s)={\displaystyle \frac{E(s)}{X(s)}}{P}_{ref}(s)+{\displaystyle \frac{F(s)}{X(s)}}{P}_s(s)+{\displaystyle \frac{T(s)}{X(s)}}{\omega }_n(s)\\ E(s)=-b{s}^3+b({\beta }_1+k_d)s^2+b({\beta }_1{k}_d+{\beta }_2+k_p)s\\ F(s)=b{s}^3-b({\beta }_1+k_d)s^2-b({\beta }_1{k}_d+{\beta }_2+k_p)s\\ T(s)=k_p[k_f+(D+Js){\omega }_n](s^3+{\beta }_1{s}^2+{\beta }_{2}s+{\beta }_3)\\ X\left(s\right)=x_1{s}^4+x_2{s}^3+x_3{s}^2+x_{4}s+x_5\end{array}\right.$$

(30)

Among them,

$$\left\{\begin{array}{c}{x}_1{s}^4+x_2{s}^3+x_3{s}^2+x_{4}s+x_5=0\\ x_1=J{\omega }_{n}b\\ x_2=bJ{\omega }_n({\beta }_1+k_d)+b(k_f+D{\omega }_n)+J{\omega }_n(k_p{\beta }_1+k_d{\beta }_2+{\beta }_3)\\ x_3=bJ{\omega }_n\left(k_d{\beta }_1+k_d{\beta }_2+{k_d\beta }_3+{\beta }_2+k_p\right)+\\ \left(k_f+D{\omega }_n\right)(b{\beta }_1+k_{d}b+{k_d\beta }_1+{\beta }_3+k_d{\beta }_2)\\ x_4=J{\omega }_n{k}_p{\beta }_3+\left(k_f+D{\omega }_n\right)\left(k_{d}b{\beta }_1+b{\beta }_2+k_{p}b\right)+k_d{\beta }_3+k_p{\beta }_2\\ x_5=\left(k_f+D{\omega }_n\right)k_p{\beta }_3\end{array}\right.$$

(31)

Therefore, in order to determine the system’s PD gain coefficient, this study thoroughly investigates the impact of the ω_c parameter on system performance.

As can be seen from Figure 9, with the increase in ω_c, since the closed-loop poles gradually move away from the imaginary axis, the response speed of the system also increases accordingly. At the same time, the damping of the system does not exhibit a monotonic characteristic. When ω_c is at its minimum value, the system damping reaches its maximum. Therefore, ω_c should not be set too large; otherwise, it will lead to excessive overshoot. As shown in Figure 10, with the increase in ω_c, the phase margin in the middle- and low-frequency bands increases, the dynamic performance of the system is improved, and the disturbance is well suppressed. Therefore, the value of ω_c can be increased within a certain range until the system noise reaches its minimum, which helps enhance the system’s anti-interference capability. Once the parameter ω_c is determined within the specified range according to the system stability requirements, the specific values of the proportional gain K_p and the derivative gain K_d can be accurately determined by virtue of the established relationship between ω_c and the gain coefficients of the PD controller.

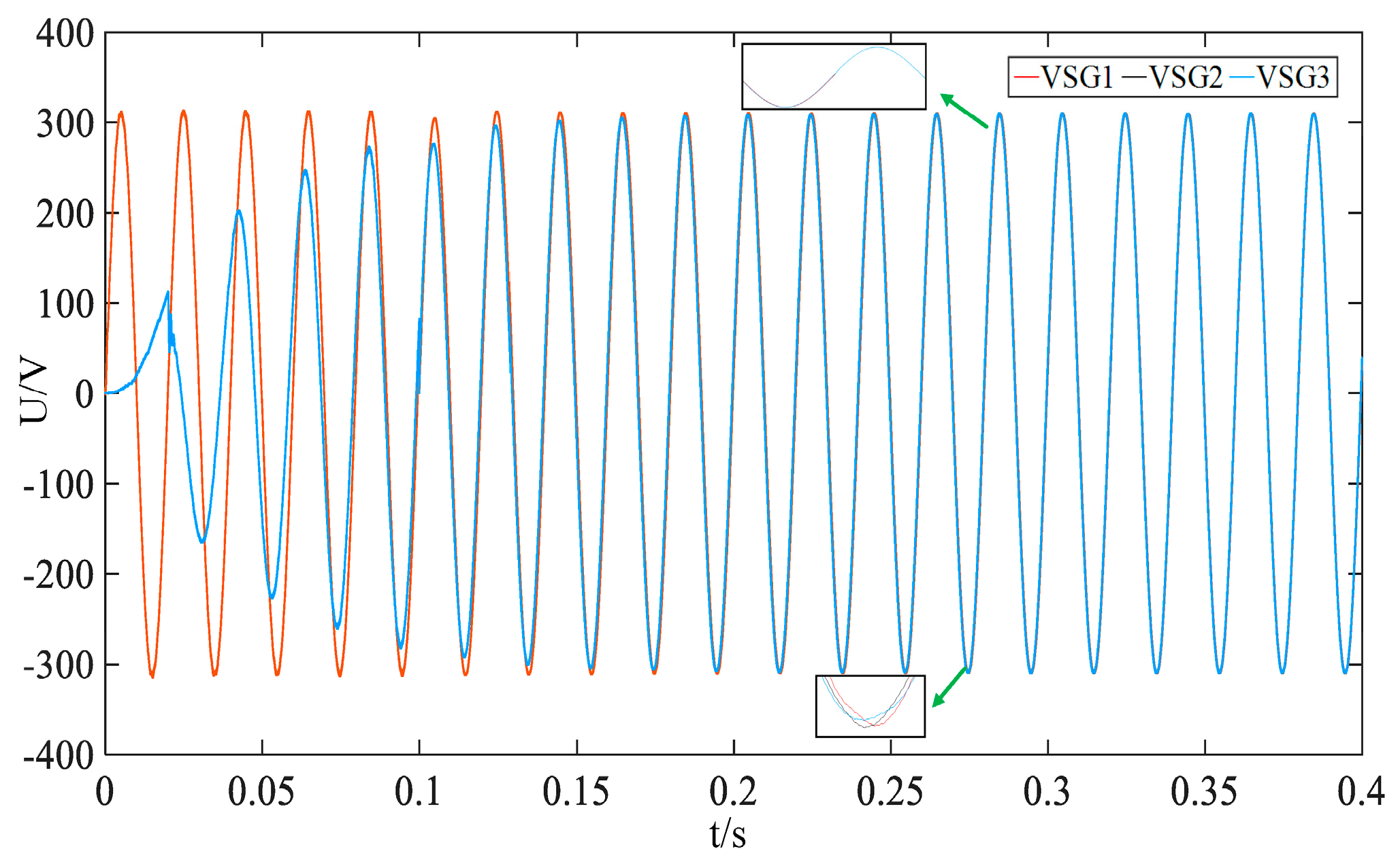
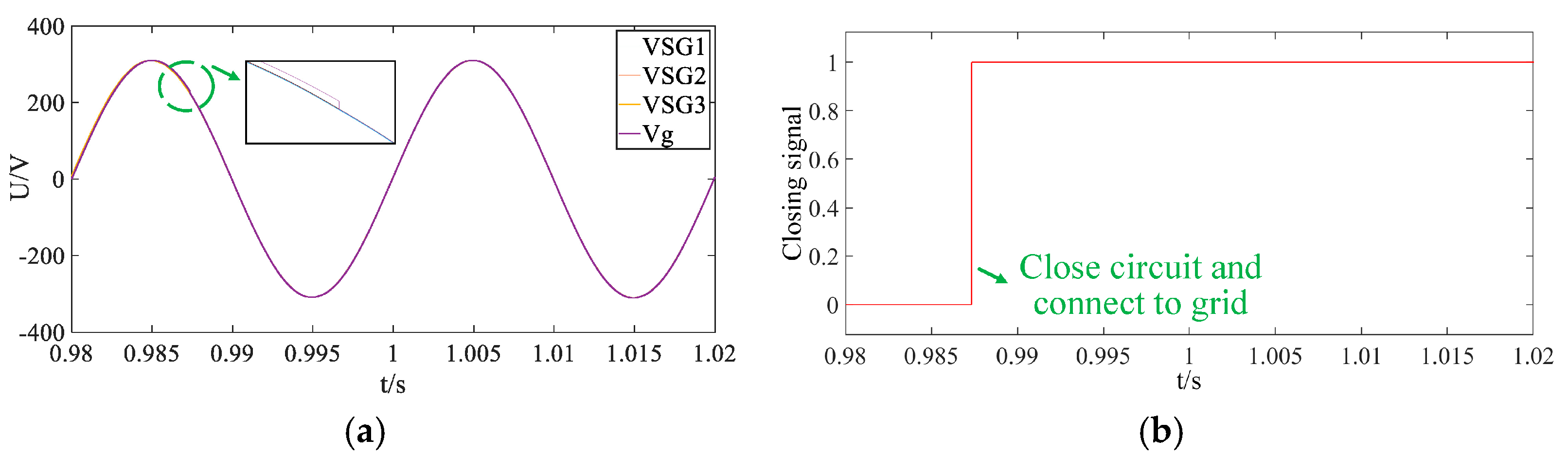
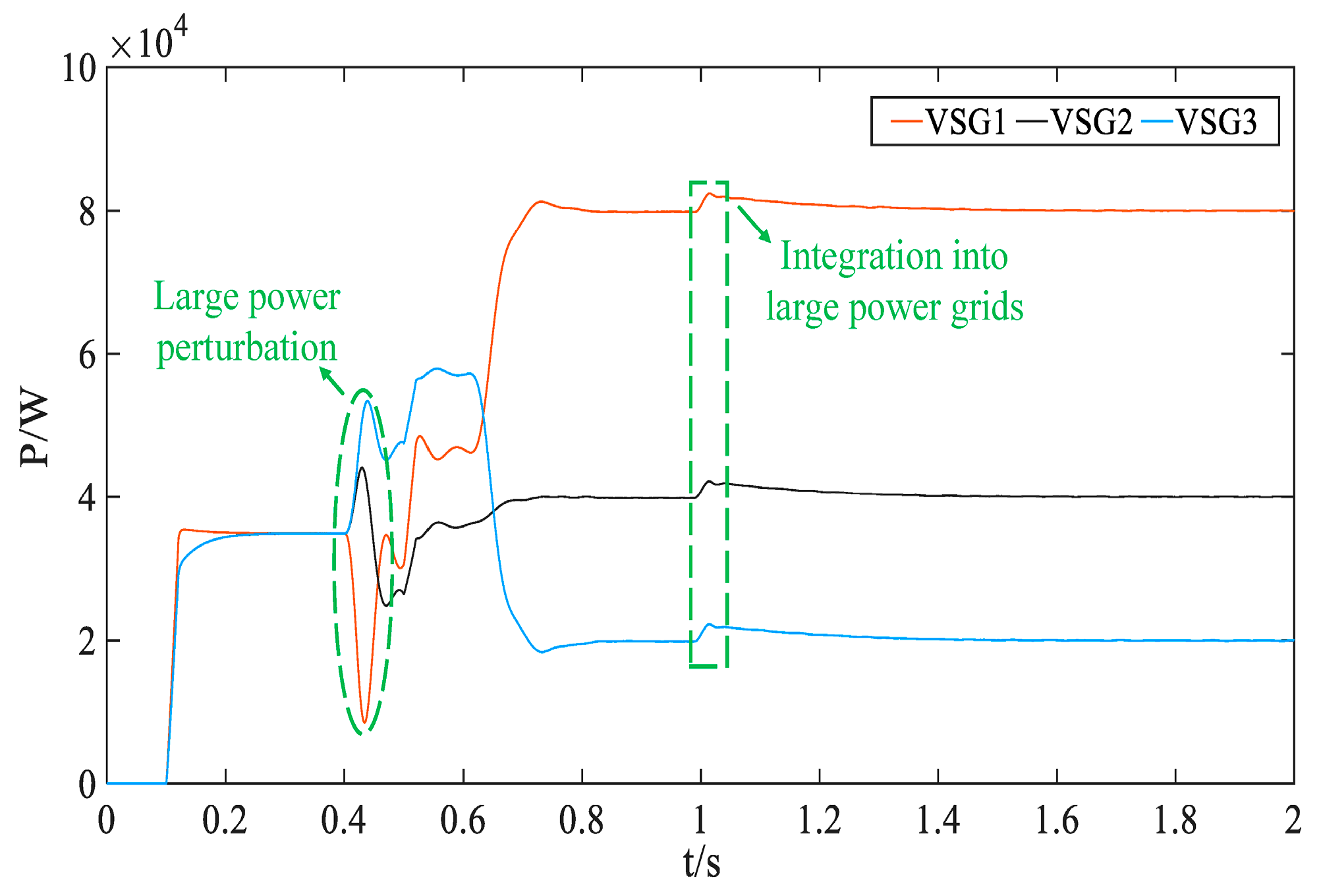
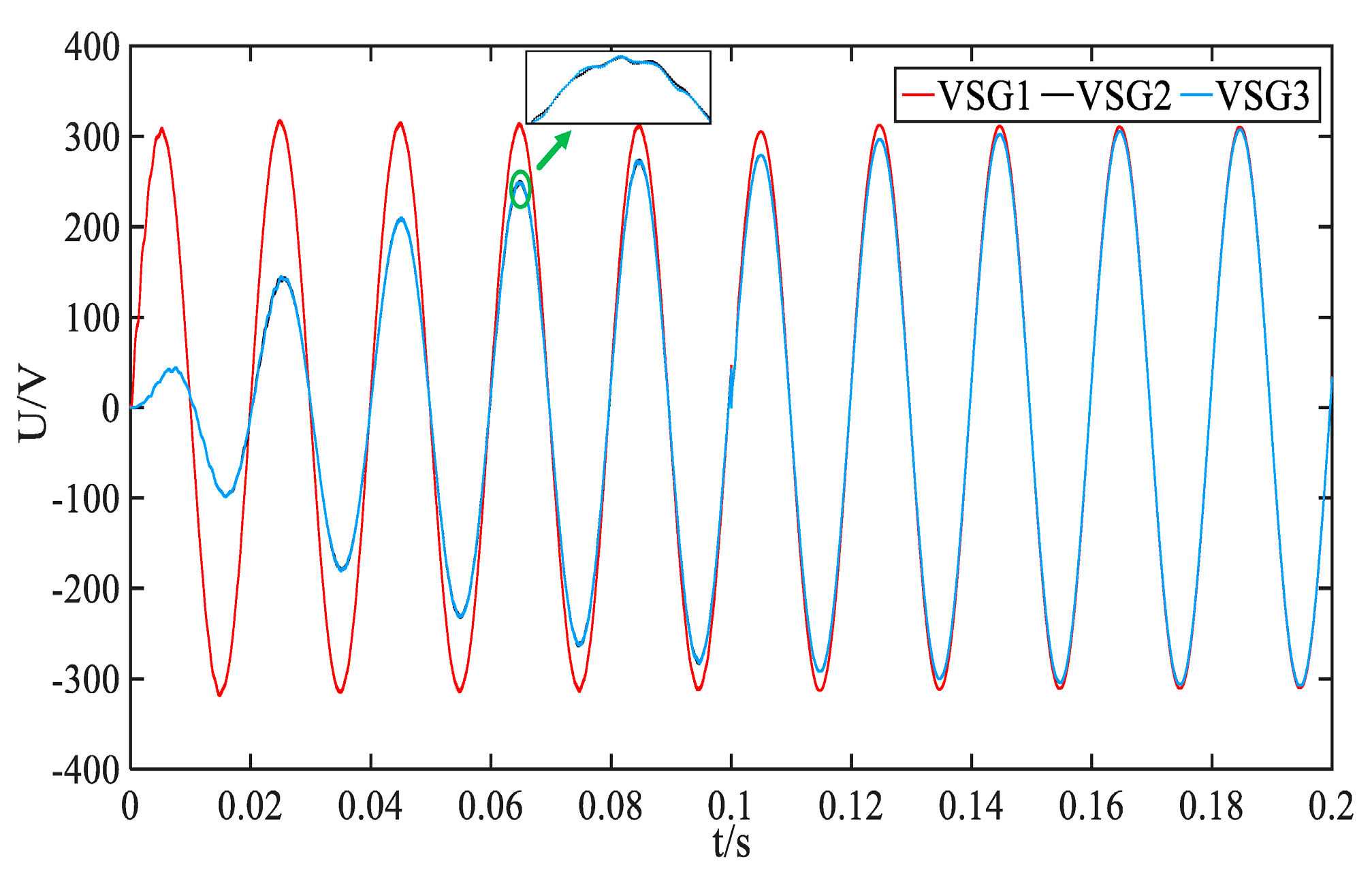
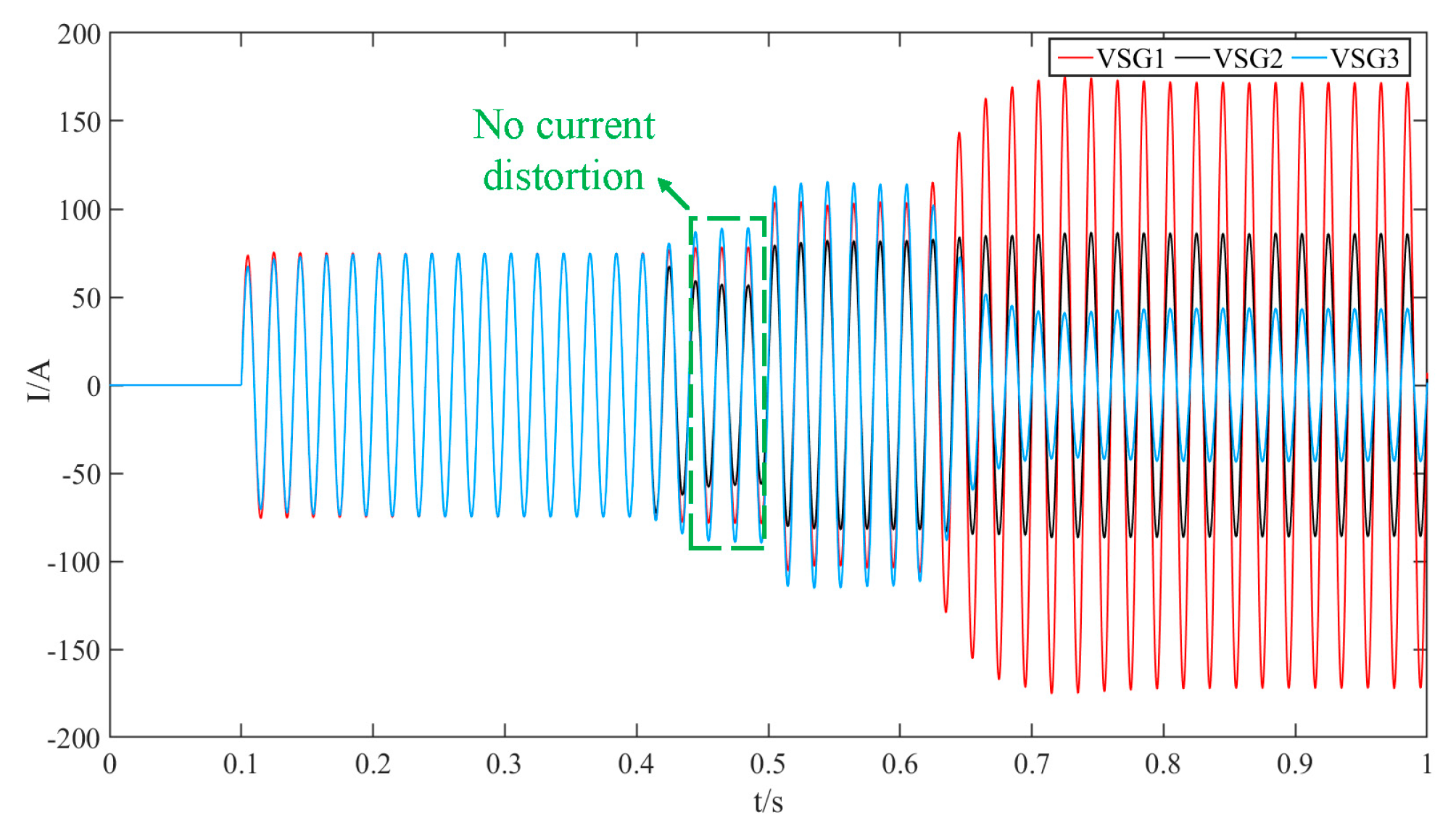
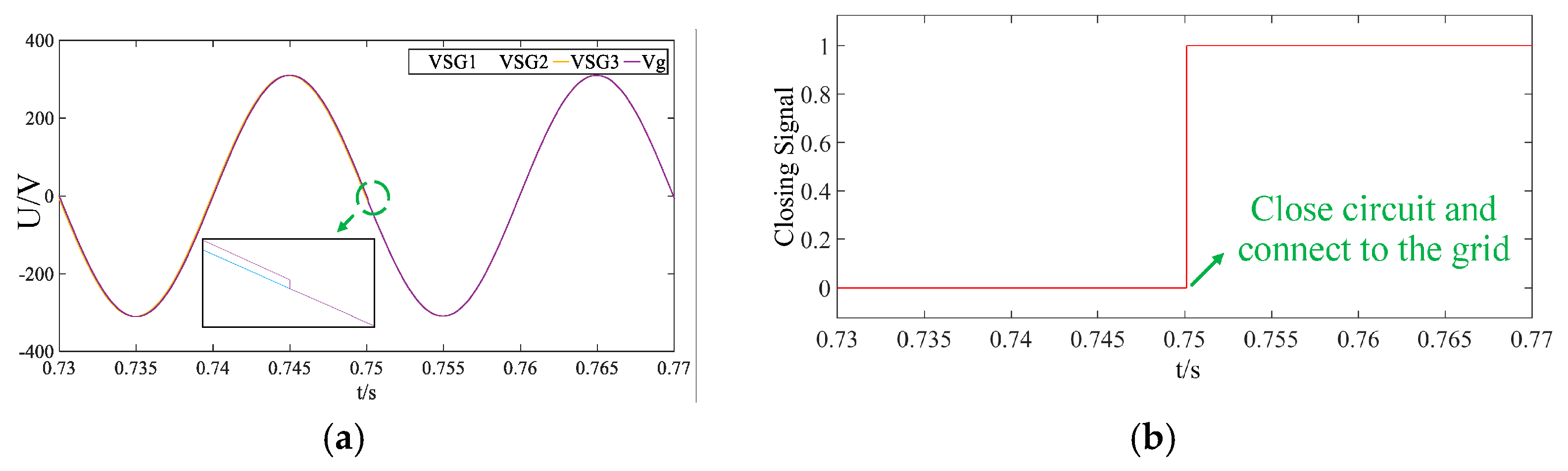
3. Microgrid Black-Start Process

Figure 11 illustrates the overall control block diagram for the black-start process. The main line parameters are mentioned in Figure 3. U_xi (x = a, b, c; I =a, b, c) denotes the three-phase output voltage on the inverter side, and i_xi (x = a, b, c; i = a, b, c) is the inverter’s output current. VSG_i (i = 2, 3) incorporates LADRC-based frequency modulation and an improved phase-angle control pre-synchronization strategy. The parameters ω_syn1, U_syn1, ω_syn2, and U_syn2 represent frequency and voltage compensation, respectively, to achieve synchronization with VSG₁. Finally, Δω_syn and ΔU_syn provide frequency and voltage compensation during integration with the main grid.

Figure 12 illustrates specific implementation details of the control schematic block diagram in Figure 11. It is roughly divided into four links: Microsource Initiation, Parallel Network Operation, Load Shedding, and Pre-synchronized Grid Connection.

4. Discussion of Black-Start Performance Analysis

To evaluate the effectiveness of the proposed multi-machine black-start strategy, a simulation model was developed in Simulink. The model verified the strategy’s capability to rapidly restore the voltage. The simulation involved three photovoltaic-storage VSGs with equal initial capacities before the black start was completed. Following grid connection, the capacities were adjusted to a 4:2:1 ratio. A parallel recovery strategy was implemented, with a total simulation time of 2 s. Loads were introduced at 0.1 s intervals, switches were closed for parallel operation at 0.4 s, and the remaining load was connected at 0.5 s. The system simulation parameters for each VSG_i (i = 1, 2, 3) are detailed in

Table 2. Simulation parameters.

Parameter	Symbol	Value
Initial active command value	Prefi	35 kW
Reactive power reference value	Qrefi	0 var
Moment of inertia	Ji	0.3 kg·m2
Damping coefficient	Di	10 N·m·s·rad−1
DC voltage rating	Udci	800 V
Grid voltage	Ug	380 V
Rated frequency	fni	50 Hz
Switching frequency	fsi	10 kHz
Filter capacitance	Ci	20 × 10−6 F
Filter inductance	Li	0.005 H

.

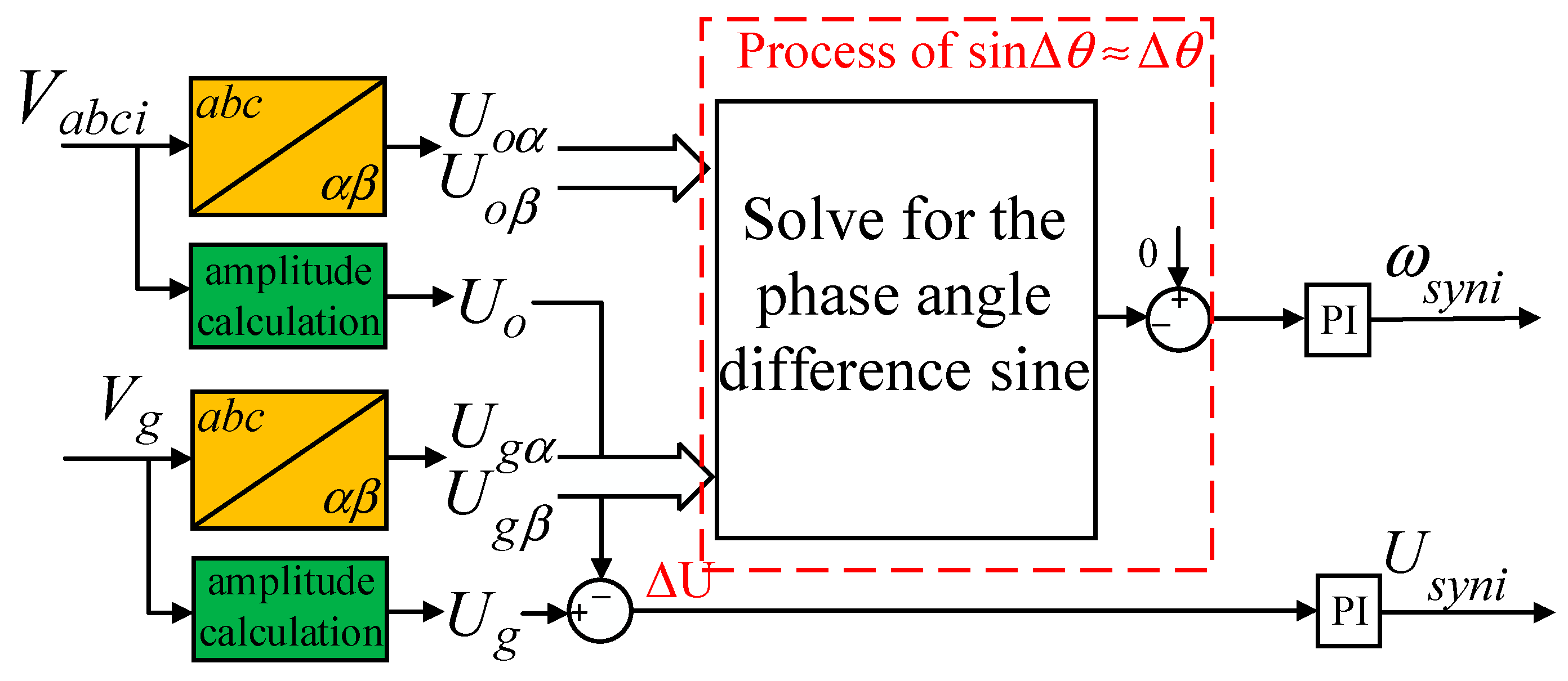
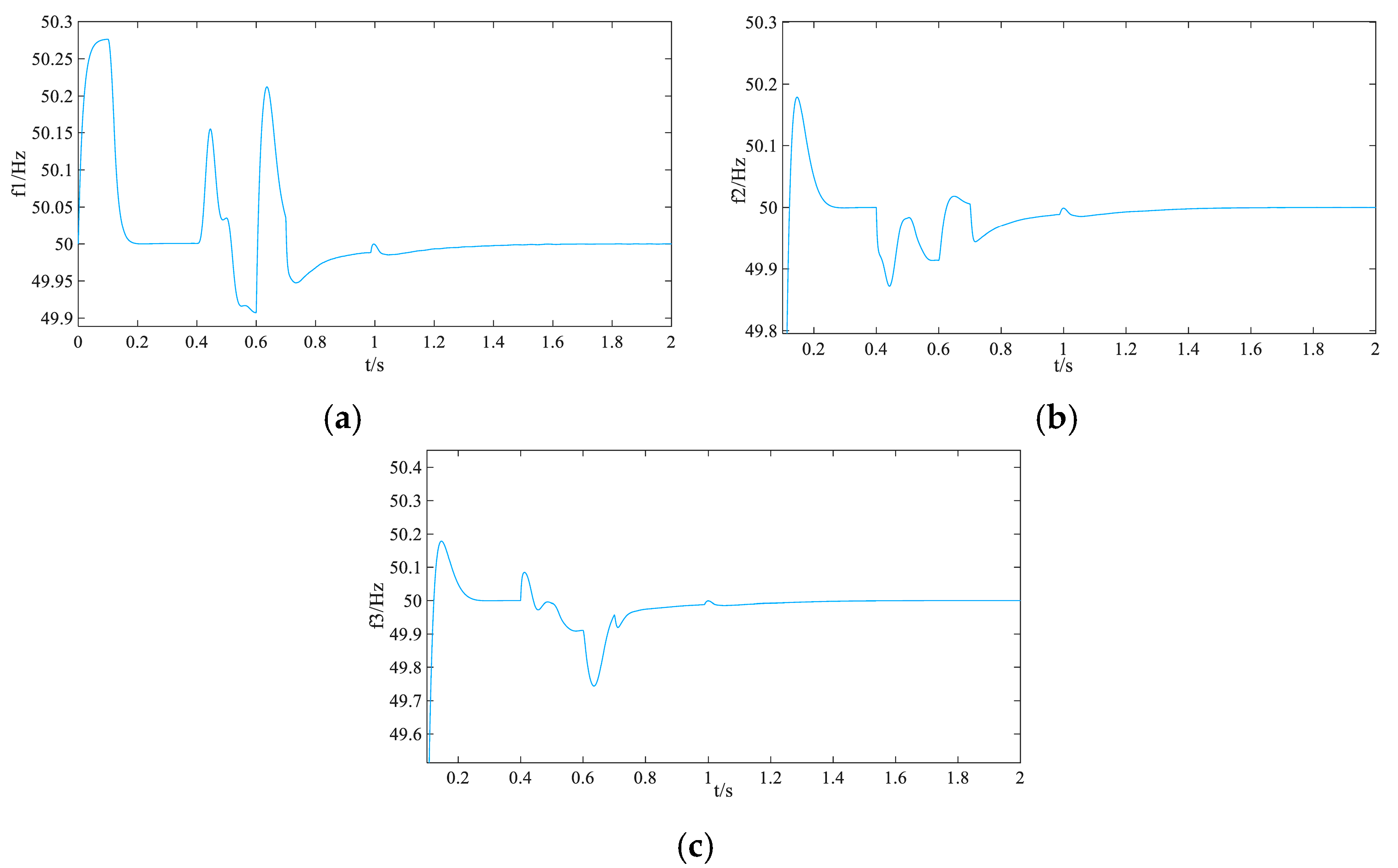
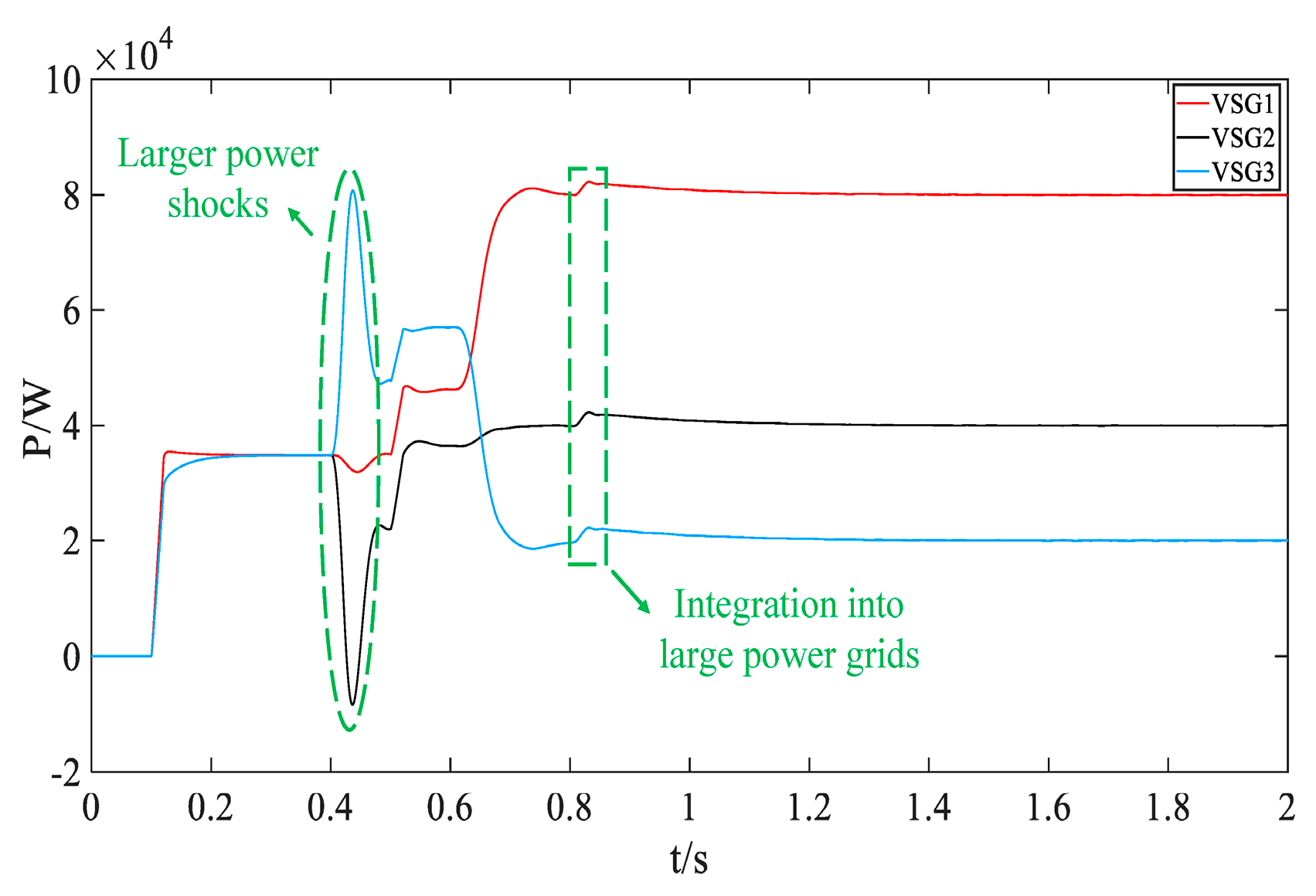
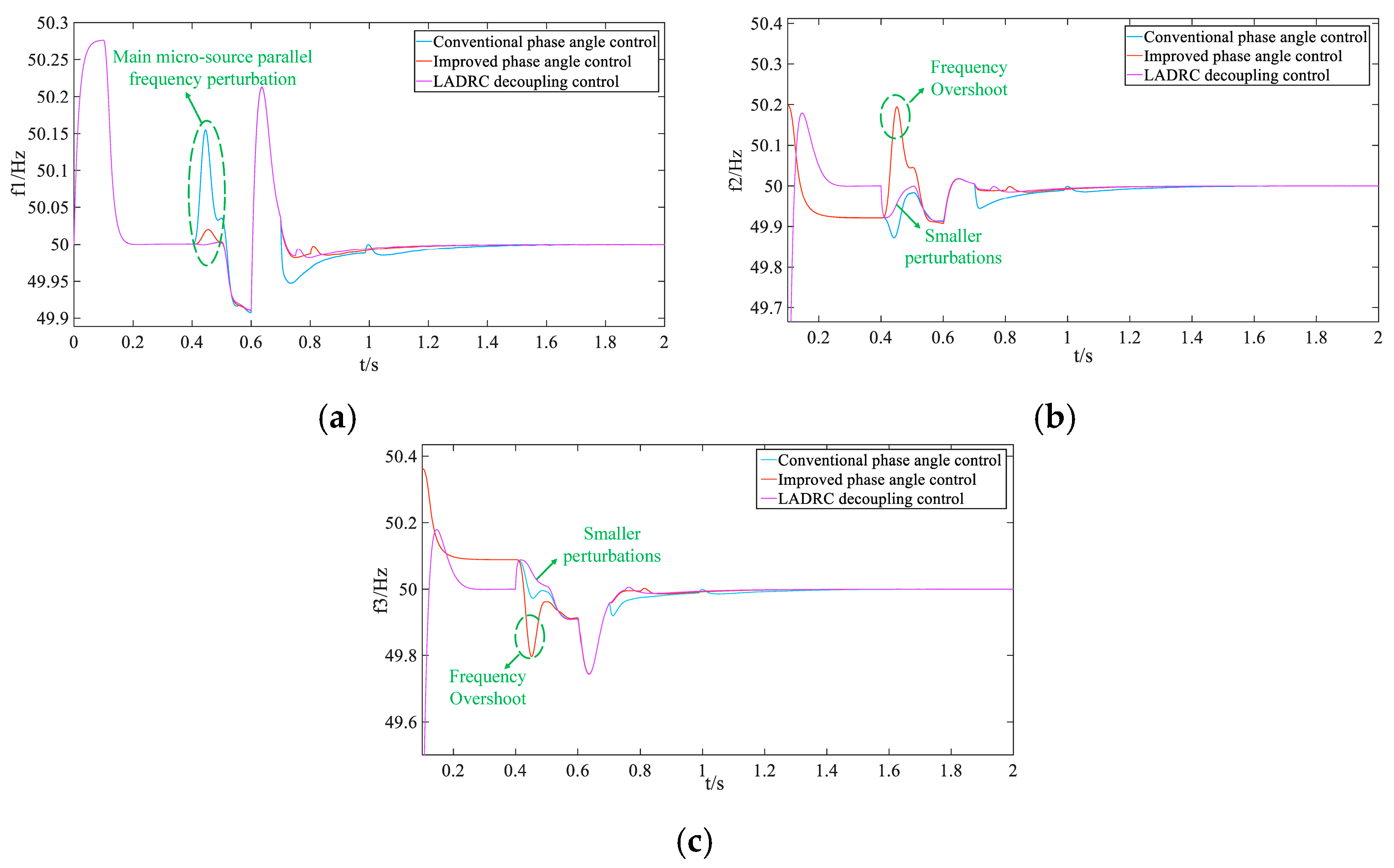
4.1. Traditional Phase-Angle Control for Multi-Machine Black Start

The traditional pre-synchronization control method is first applied to the multi-machine black-start process to verify the zero-start voltage boost. Figure 13 and Figure 14 show the A-phase AC bus voltage and current established by the three VSGs. During the voltage buildup of VSG₂ and VSG₃, voltage distortion occurs under traditional phase-angle control. Once stability is achieved, the voltage quality meets the required standards. To prevent system shutdown from excessive load at the early stage of the black start, a load is introduced at 0.1 s. Although the synchronization of the microsources is relatively slow, they eventually achieve parallel operation. Current generation begins at 0.1 s, with distortion observed during the parallel connection, and increases after 0.5 s as the load switches. Following the islanded black start, the system transitions to grid-connected operation, resulting in current variations due to capacity changes. As shown in Figure 15, voltage synchronization is completed at approximately 0.985 s, validating the effectiveness of the zero-start voltage boost in the black-start simulation.

Figure 16 and Figure 17 illustrate the frequency and power waveforms during the black-start process. Owing to the limitations of traditional phase-angle control in pre-synchronization, both frequency and power experience noticeable fluctuations immediately after the parallel connection at 0.4 s. Nevertheless, the system gradually stabilizes, ultimately achieving a steady-state operation.

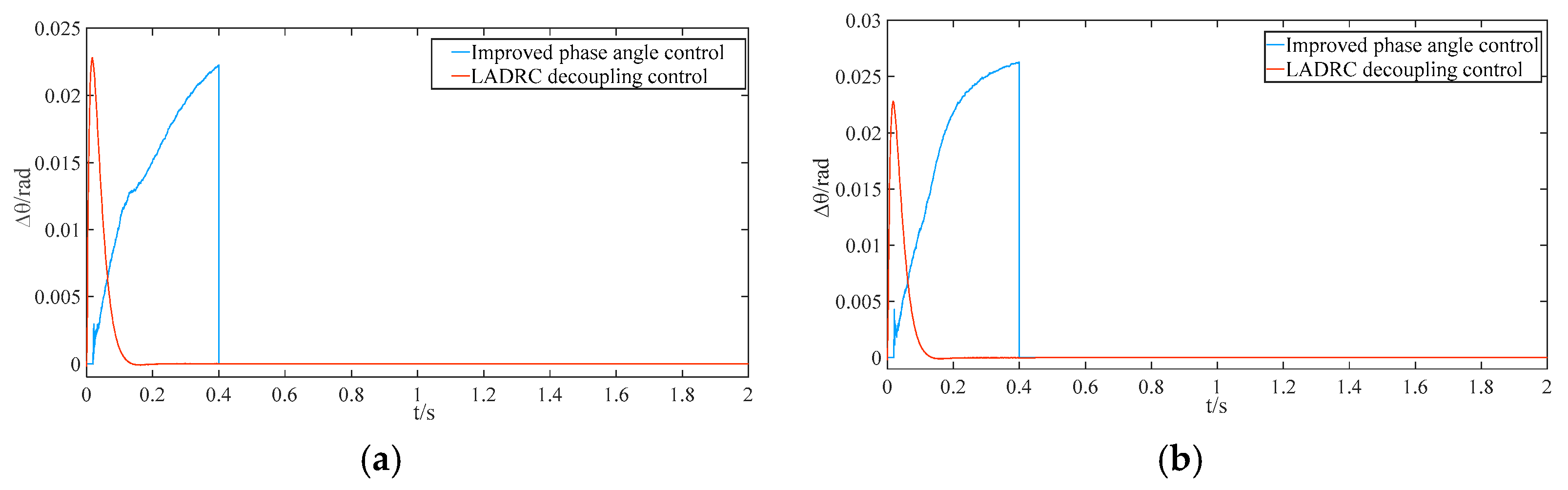
4.2. Improved Phase-Angle Control for Multi-Machine Black Start

Compared with the conventional phase-angle control strategy, the improved phase-angle control further enhances the black-start capability by improving the pre-synchronization performance among multiple black-start microsources.

Figure 18 shows that with improved phase-angle control during pre-synchronization, the three microsources achieve rapid synchronization, minimizing disturbances during grid connection. After load switching at 0.5 s, the current increases, and grid integration is completed at approximately 0.8 s, with fluctuations due to capacity changes, as illustrated in Figure 19. Compared to traditional methods, both voltage and current performance are notably enhanced. Figure 20 further demonstrates that the islanded black-start system achieves faster grid connection than the conventional phase-angle control approach.

While the improved phase-angle control during pre-synchronization accelerates synchronization and enhances voltage and current quality in the black-start process, the rapid adjustment of phase angles in VSG₂, VSG₃, and the main microsource induces frequency overshoot during their parallel connection due to the coupling between the frequency and phase-angle. As shown in Figure 21, the main reference source experiences minimal disturbance at the moment of synchronization. However, Figure 22 reveals significant power fluctuations when VSG₂ and VSG₃ are connected in parallel, which, if left unregulated, could result in black-start failure.

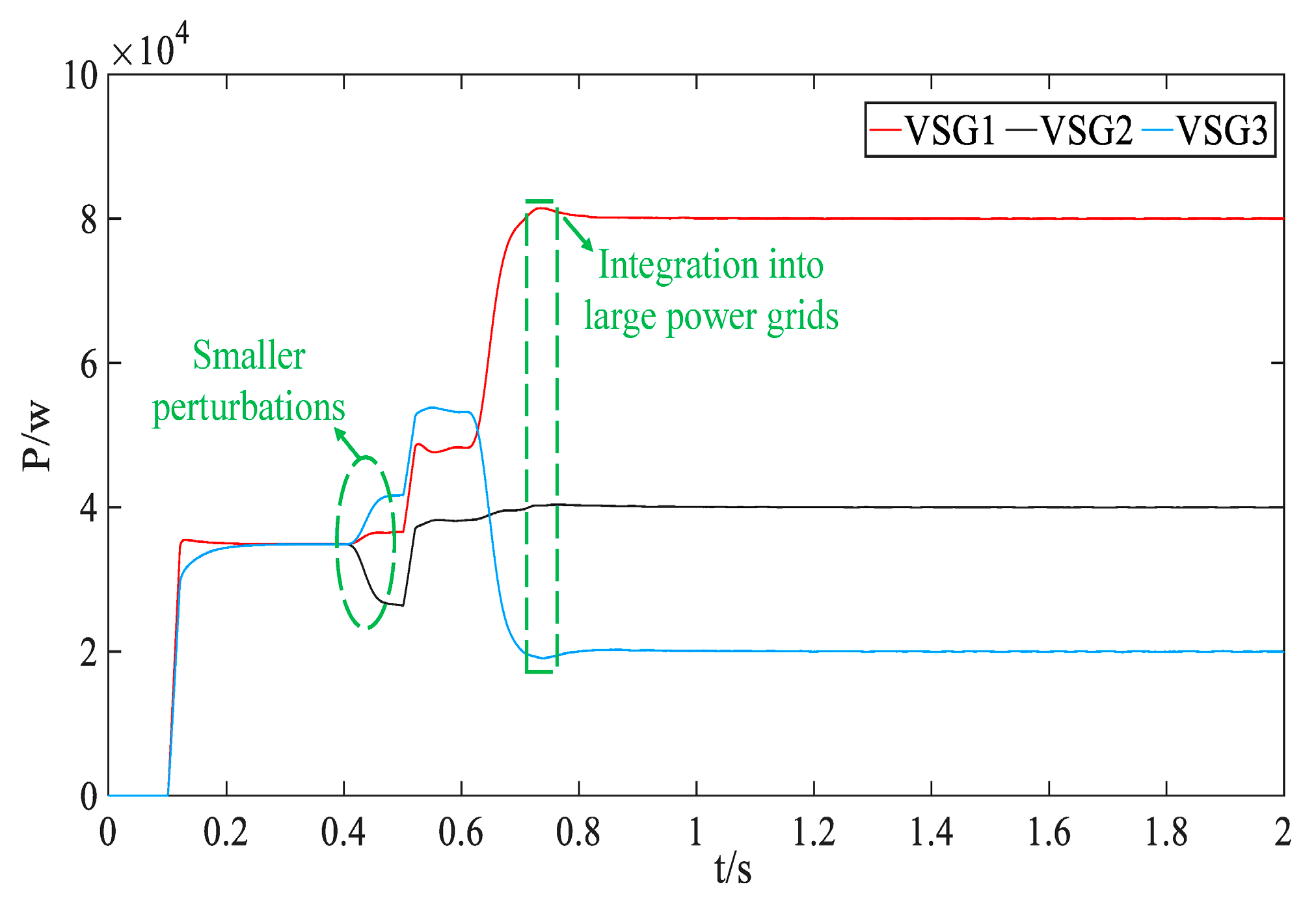
4.3. LADRC Decoupled Control of Multi-Machine Black Start

This study aims to enhance the voltage and current quality during the black-start process while preventing black-start failure due to the frequency overshoot of microsources in the pre-synchronization process with improved phase-angle control. The proposed solution addresses the issue of frequency overshoot caused by rapid changes in the phase-angle difference during the synchronous parallel connection of VSG₂ and VSG₃ with the main reference microsource, VSG₁, by introducing LADRC control. The frequencies of VSG₂ and VSG₃ are reduced as the total system perturbation is adaptively adjusted. Once the perturbation is reduced, the overall system disturbance decreases, and the frequency of the main reference source, VSG₁, is improved when the three VSGs are synchronized in parallel. Figure 23 compares the microsource frequency waveforms under LADRC decoupling control and improved phase-angle control. After achieving stable frequency control, the phase-angle control of the micro-source synchronization becomes smoother and more controllable, leading to faster stabilization. Figure 24 illustrates the phase-angle difference control compared to the improved phase-angle control.

If the frequency and phase angle are not promptly controlled during the parallel networking of black-start microsources, instantaneous power fluctuations can severely impact black-start performance. Figure 25 compares the output power under LADRC decoupling control, which mitigates the coupling effect between the frequency and phase angle, resulting in smoother instantaneous power synchronization. This accelerates and stabilizes parallel recovery, enhancing system robustness. As shown in Figure 26, the system connects to the grid approximately 0.75 s after completing the islanded black start, boosting system capacity and reducing the risk of prolonged island operation.

4.4. Engineering Realization Discussion

Although the LADRC decoupling control strategy proposed in this paper effectively suppresses the frequency–phase-angle coupling problem during the VSG black-start microsource pre-synchronization process in theory and through simulation, it may still face the following challenges in practical microgrid applications:

(1)

Computational Latency and Complexity: The LADRC relies on a LESO for the real-time observation and compensation of the system frequency and disturbances. However, in practical microgrids, constrained by hardware computational capabilities and high-speed data sampling conditions, a high-order LESO may introduce computational delays, thereby compromising frequency compensation effectiveness. To address this, reduced-order extended state observers (RESOs) can be adopted to simplify computational complexity, while distributed control strategies can be integrated to alleviate central control burdens and reduce data processing and transmission delays, thereby enhancing the timeliness of compensation signals.

(2)

Hardware Implementation and Communication Architecture Compatibility: VSGs are typically distributed across various nodes in a microgrid, requiring the frequency, phase angle, and other data to be transmitted via communication links to a central controller or among themselves. However, issues such as communication delays and packet loss may cause dynamic compensation signals from the LADRC to lag or fail, adversely affecting pre-synchronization performance. Therefore, in engineering implementations, it is essential to optimize hardware architecture and communication protocols, such as adopting edge computing-based LADRC control units to reduce reliance on remote data, ensuring real-time and stable regulation capabilities of compensation signals.

5. Conclusions

This paper presents an enhanced pre-synchronization strategy integrating optimized phase-angle control with LADRC adaptive regulation to improve synchronization accuracy and reliability in multi-microsource parallel recovery during a black start. By minimizing PLL dependency and avoiding phase-angle jumps, the approach accelerates synchronization while introducing a LADRC for the real-time compensation of frequency fluctuations. This suppresses frequency overshoot from frequency–phase-angle coupling, enabling precise and coordinated optimization of both parameters. Finally, a comparison of the voltage and current quality generated by the three strategies during the black-start process, along with the frequency characteristics of the parallel networking process of black-start microsources, demonstrates that the proposed strategy effectively reduces the impacts of parallel connection, improves synchronization performance, and enhances the resilience of the microgrid during the black-start process.

However, practical implementation necessitates addressing computational latency and communication architecture compatibility. Future research could optimize LESO computational complexity by introducing reduced-order observers (RESOs), leverage distributed control and edge computing to minimize latency, and enhance communication link robustness to improve microgrid dynamic stability.