Applying Structure Exchange to Battery Charging to Enhance Light-Load Efficiency
Abstract
1. Introduction
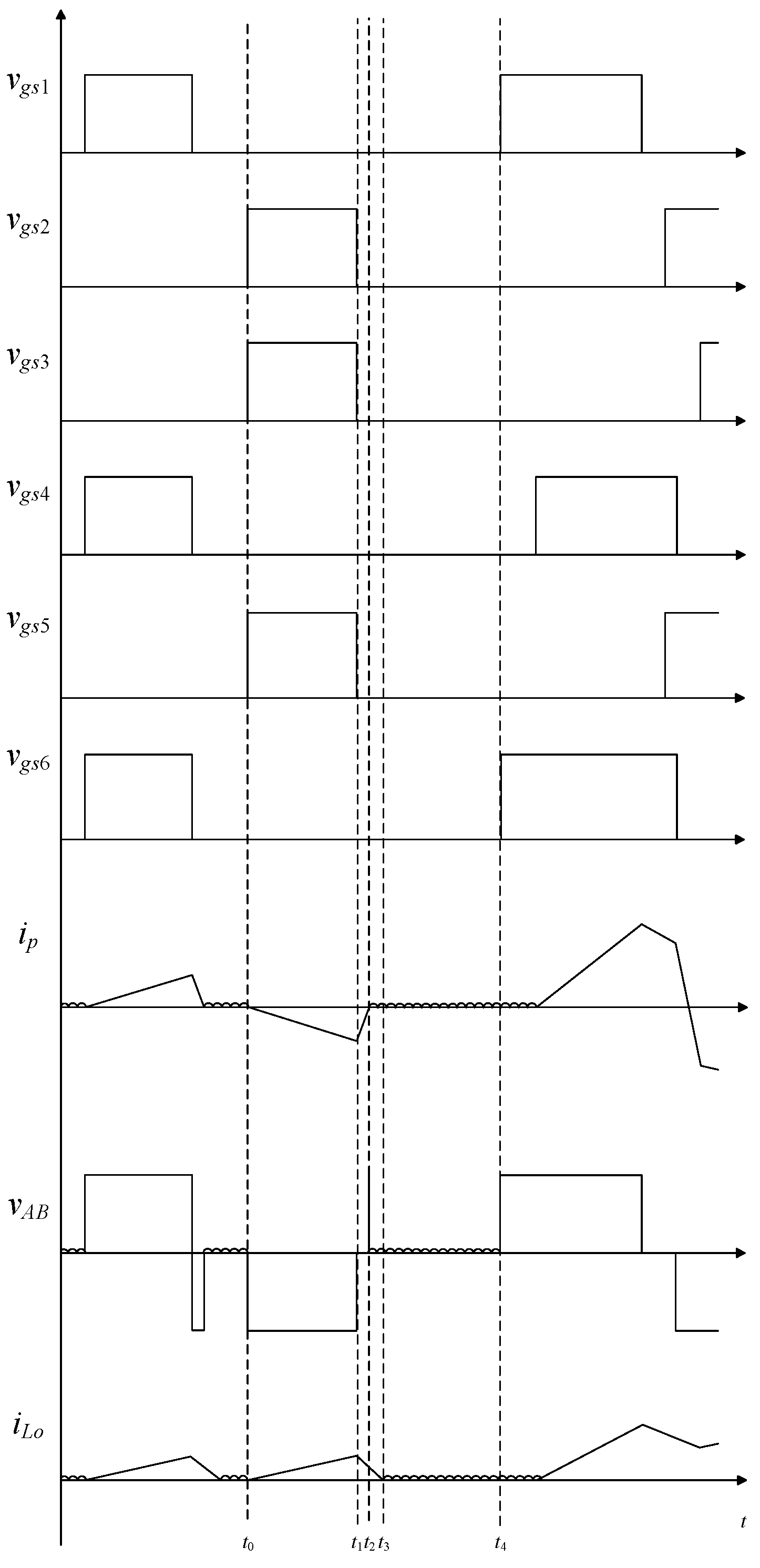
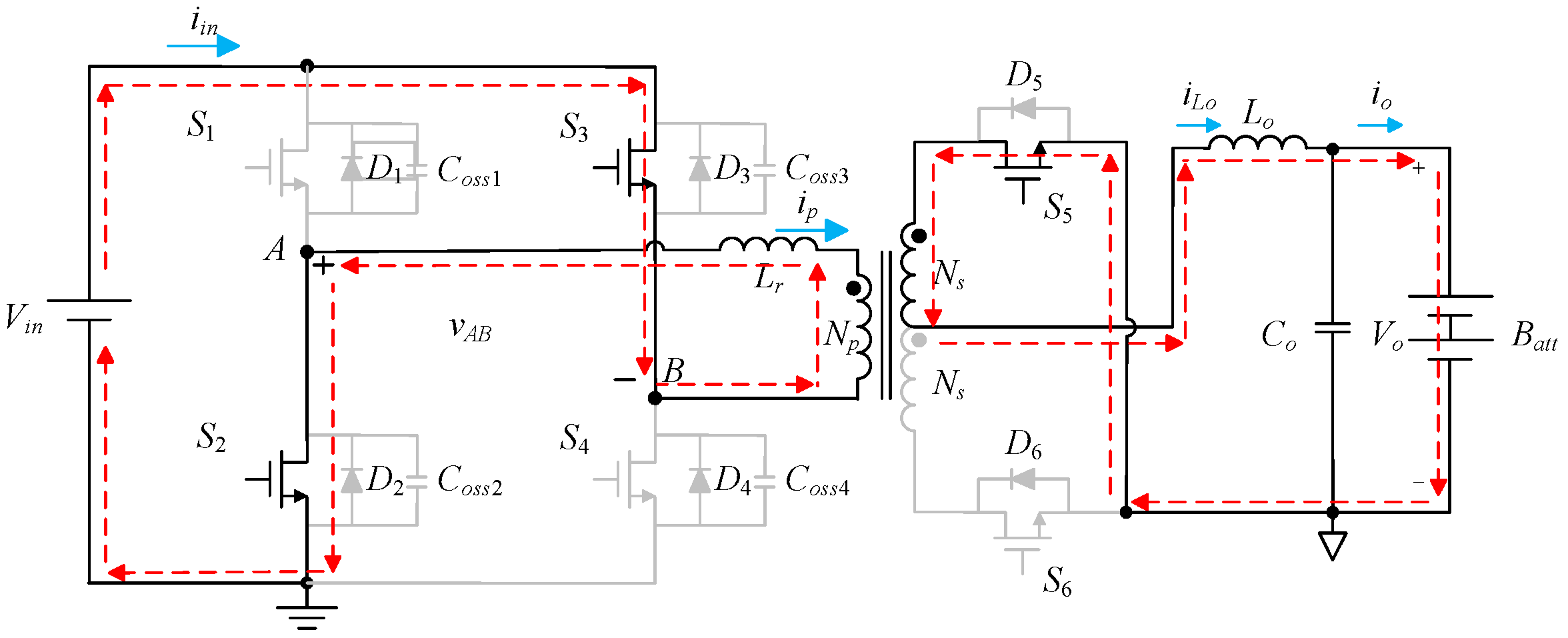
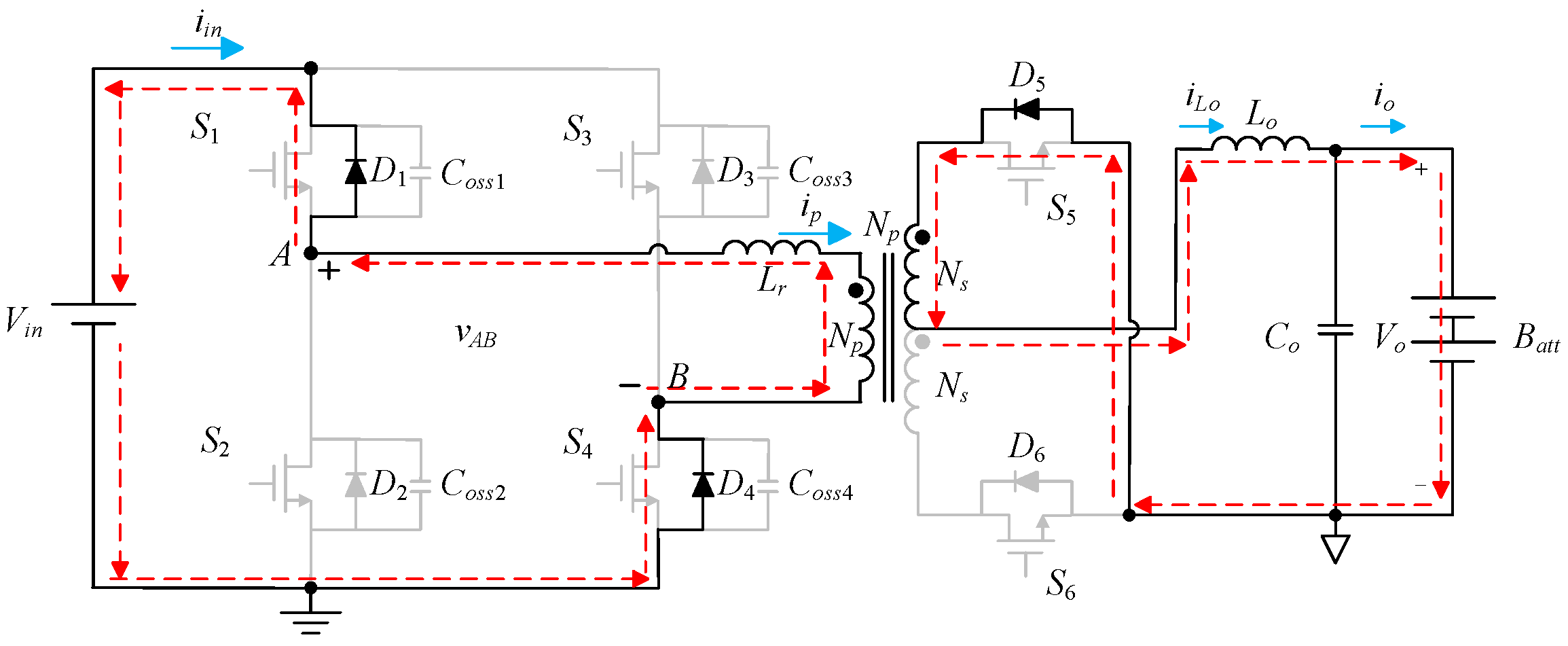
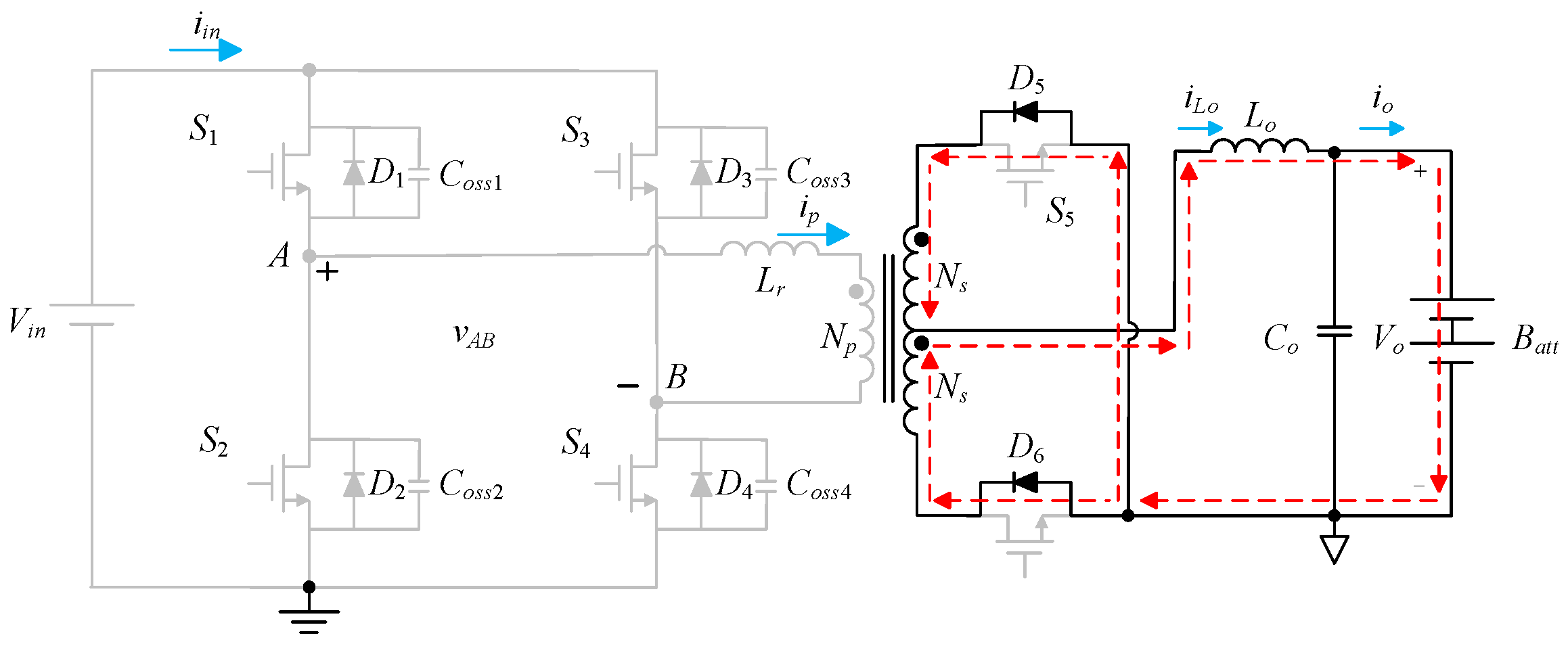
2. Operating Principle of Structure Exchange
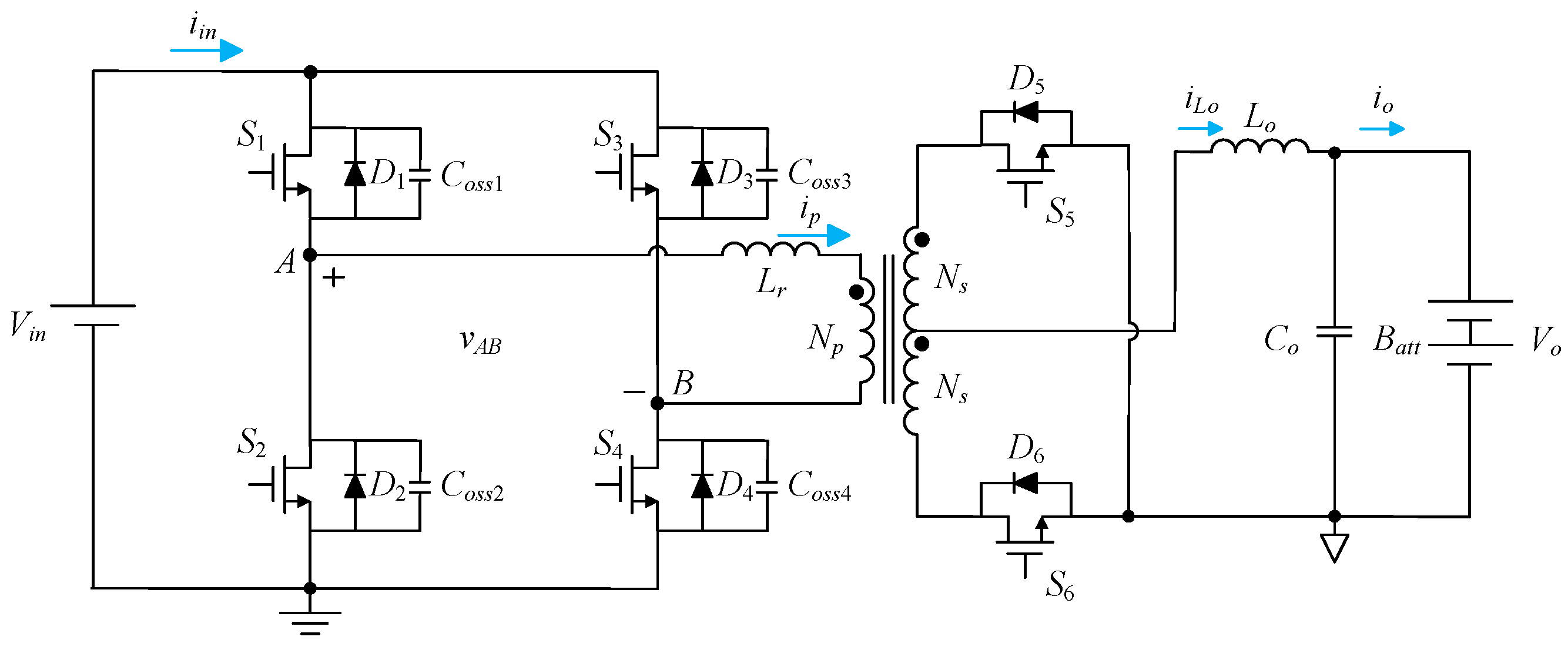
2.1. Circuit Symbol Definitions and Assumptions
- (1)
- Vin, Vo, iin, and io are the input voltage, the output voltage, the input current, and the output current, respectively;
- (2)
- VAB is the voltage across the primary transformer;
- (3)
- Ip is the current of the primary transformer;
- (4)
- iLo is the current of the output inductor;
- (5)
- Ts is the switching period, and fs is the switching frequency;
- (6)
- D and Deff denote the duty cycle and the effective duty cycle, respectively;
- (7)
- Np and Ns represent the number of coils on the primary side and the number of coils on the secondary side, respectively;
- (8)
- The output capacitances of the power switches, called Coss1, Coss2, Coss3, and Coss4, are all equal;
- (9)
- The output capacitances are not considered for the secondary-side power switches;
- (10)
- Since the resonant time is comparatively short, the resonant current can be considered linear as a result.
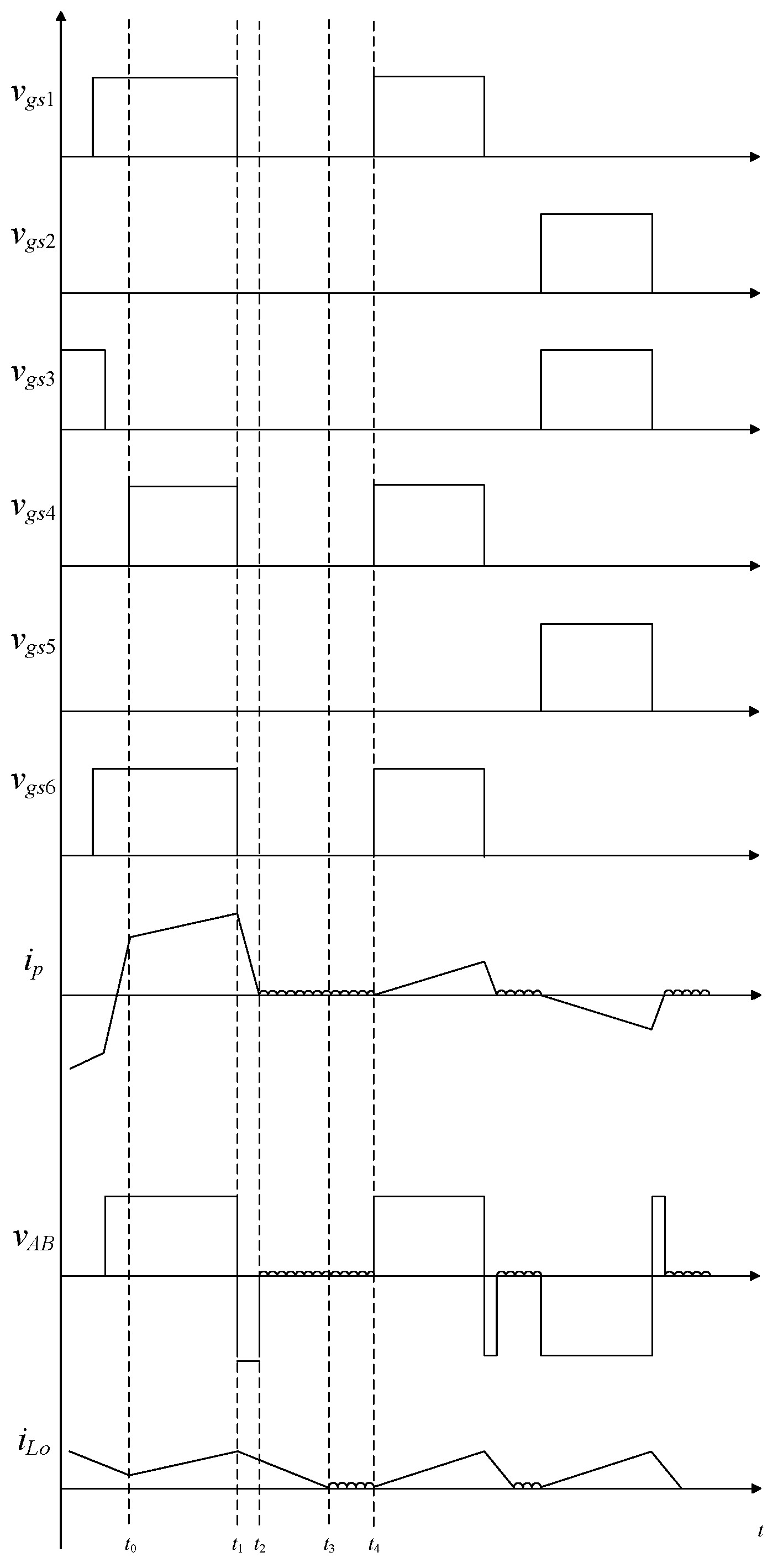
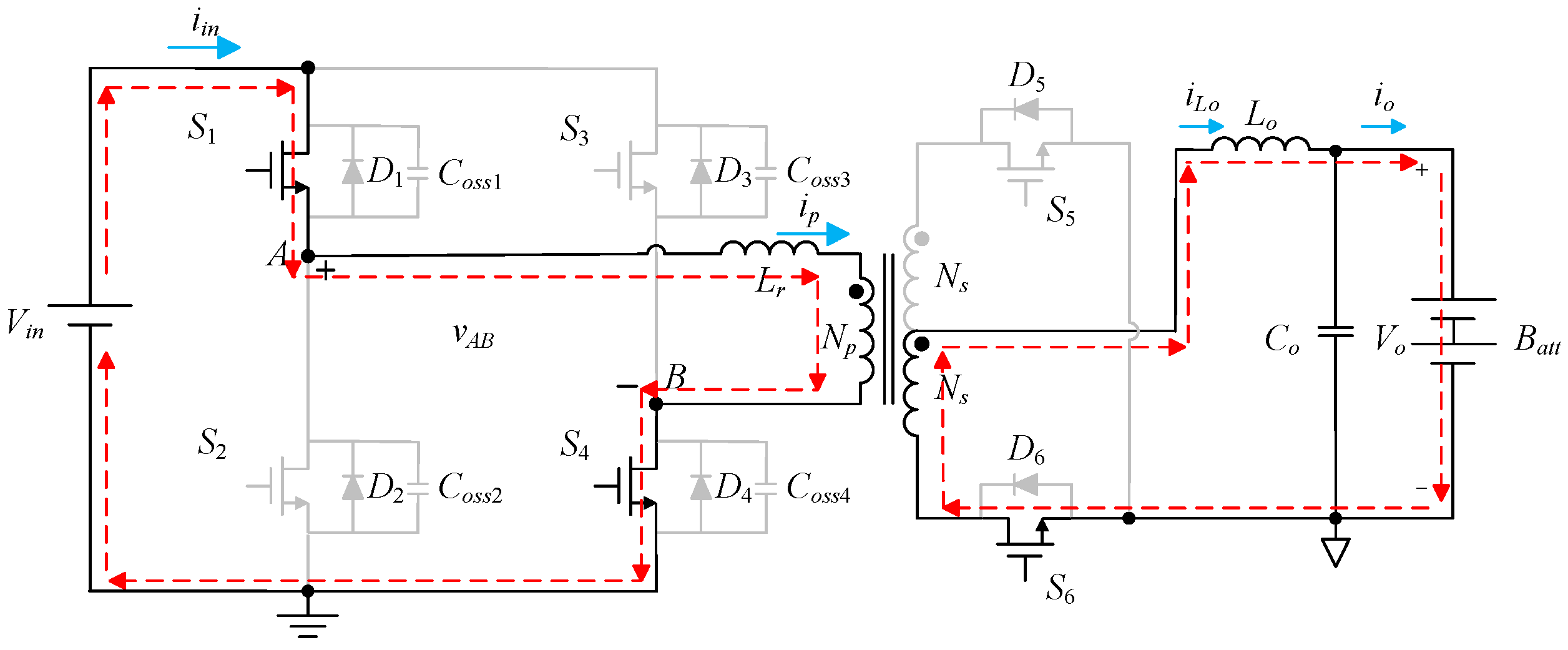
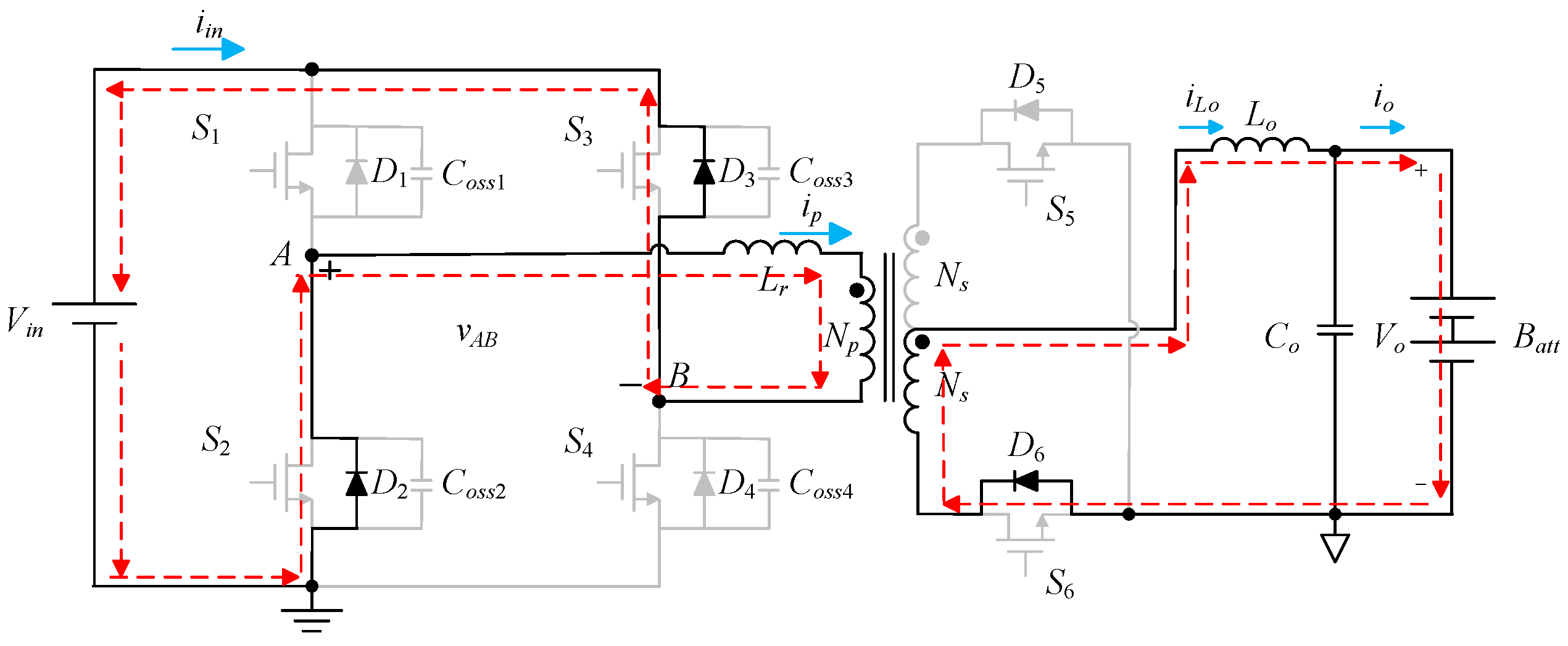
2.2. FBPSS Transferred to Two-Switch Forward Structure (TSFS)
2.3. TSFS Transferred to FBPSS
3. Design Considerations
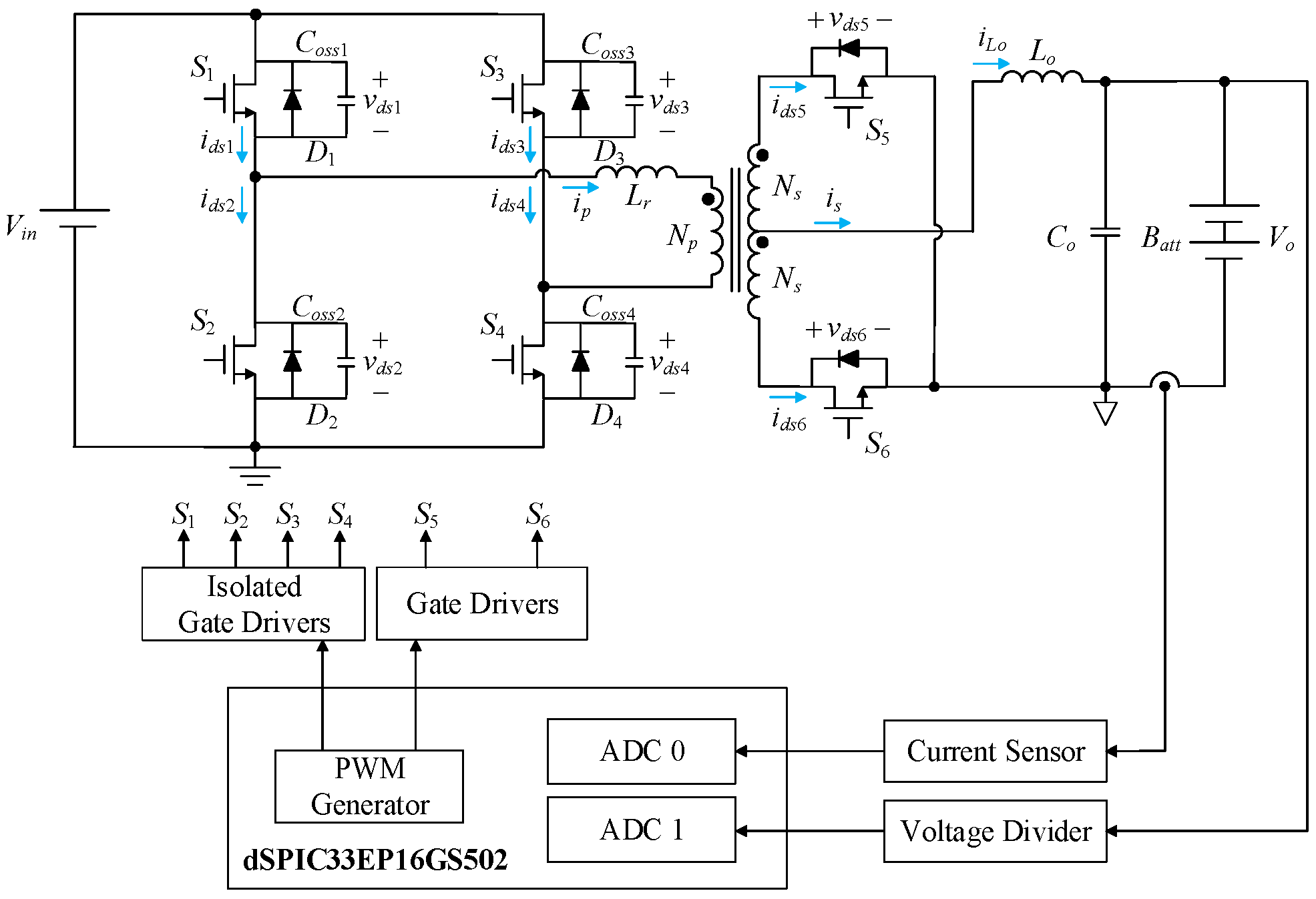
3.1. System Configuration
3.2. System Specifications
3.3. Design of Resonant Capacitor and Inductor
4. Control Program Flowchart
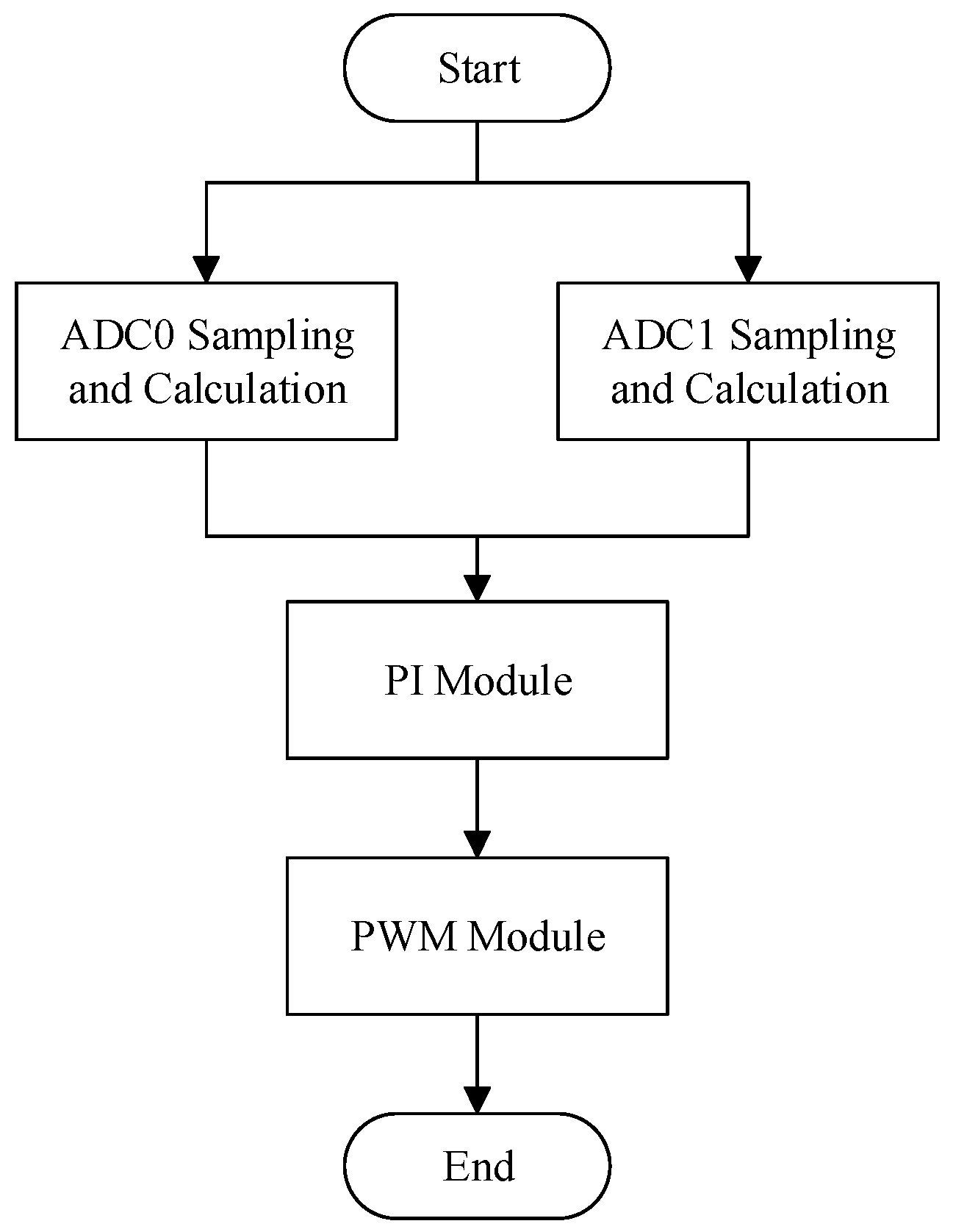
4.1. Main Program Flowchart
4.2. ADC Module
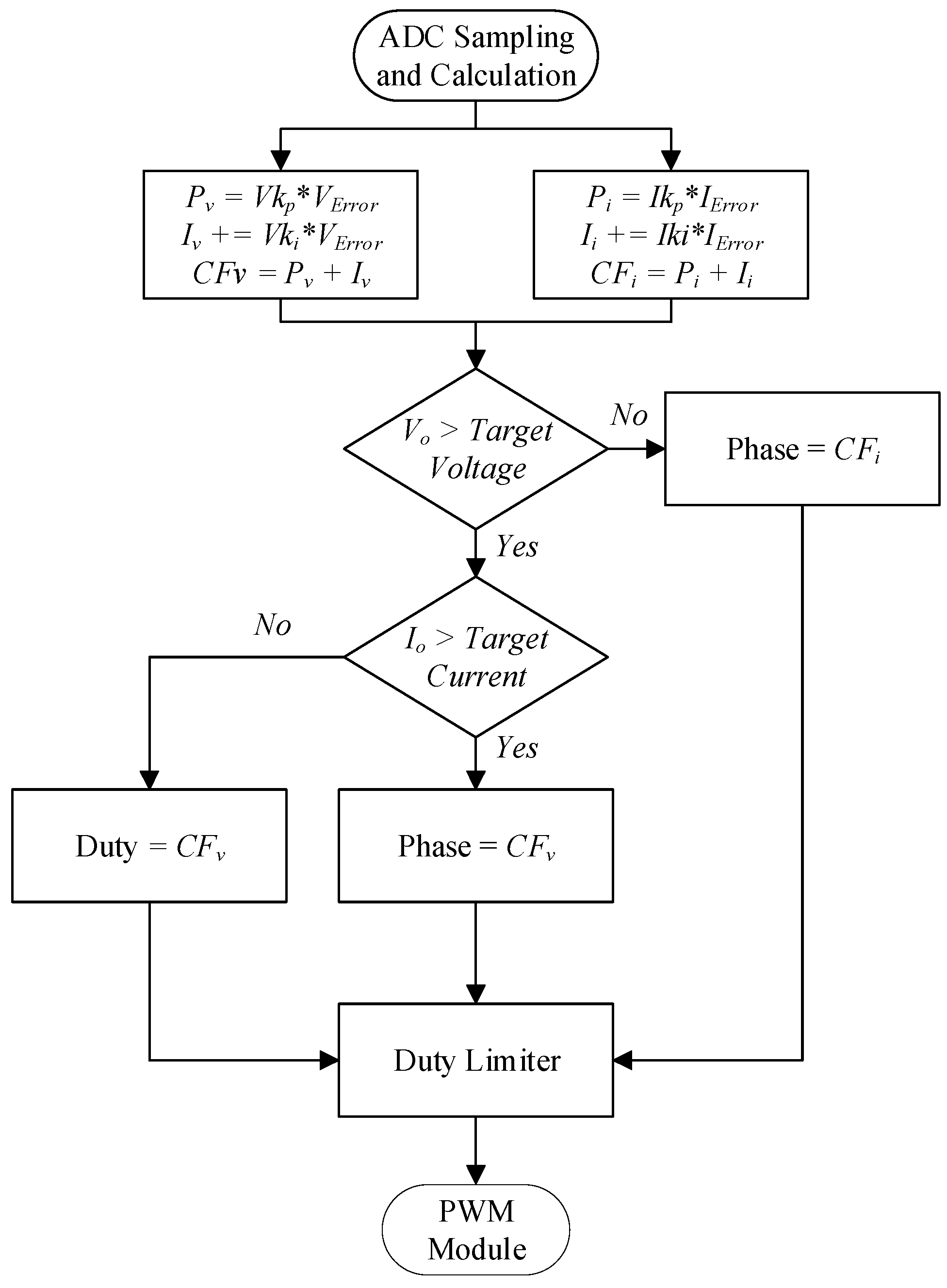
4.3. PI Module
4.4. PWM Module
5. Experimental Results
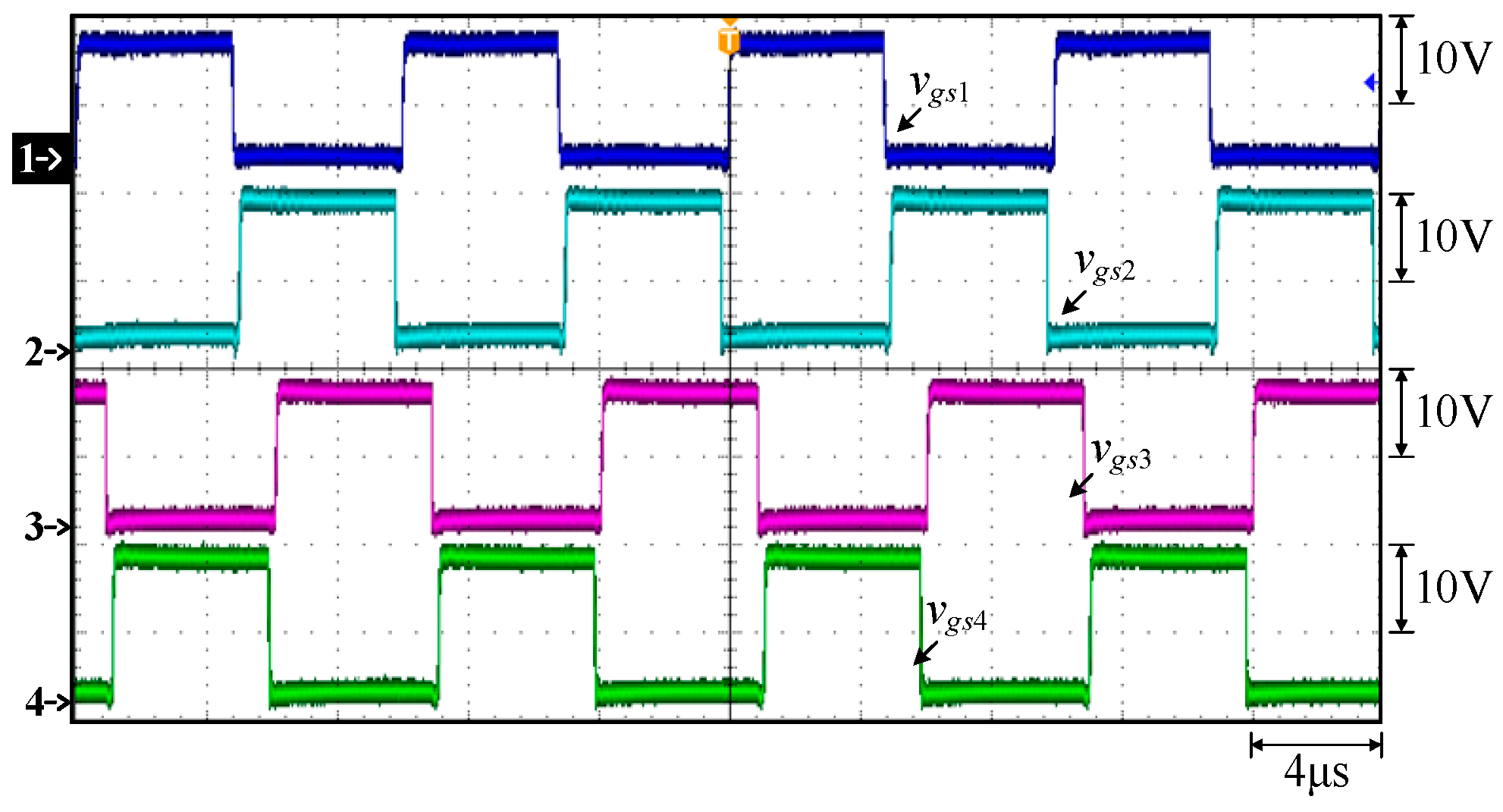
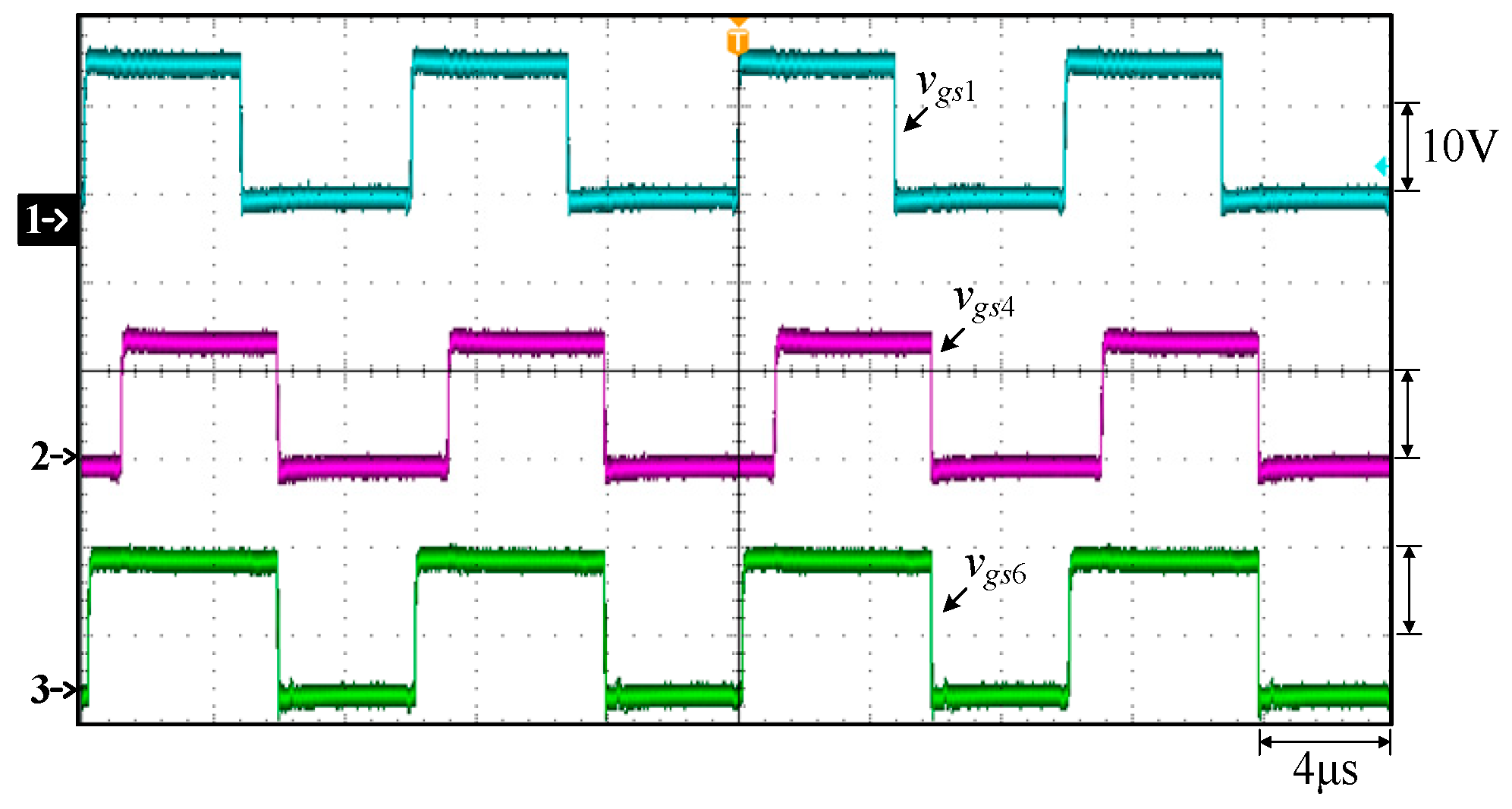
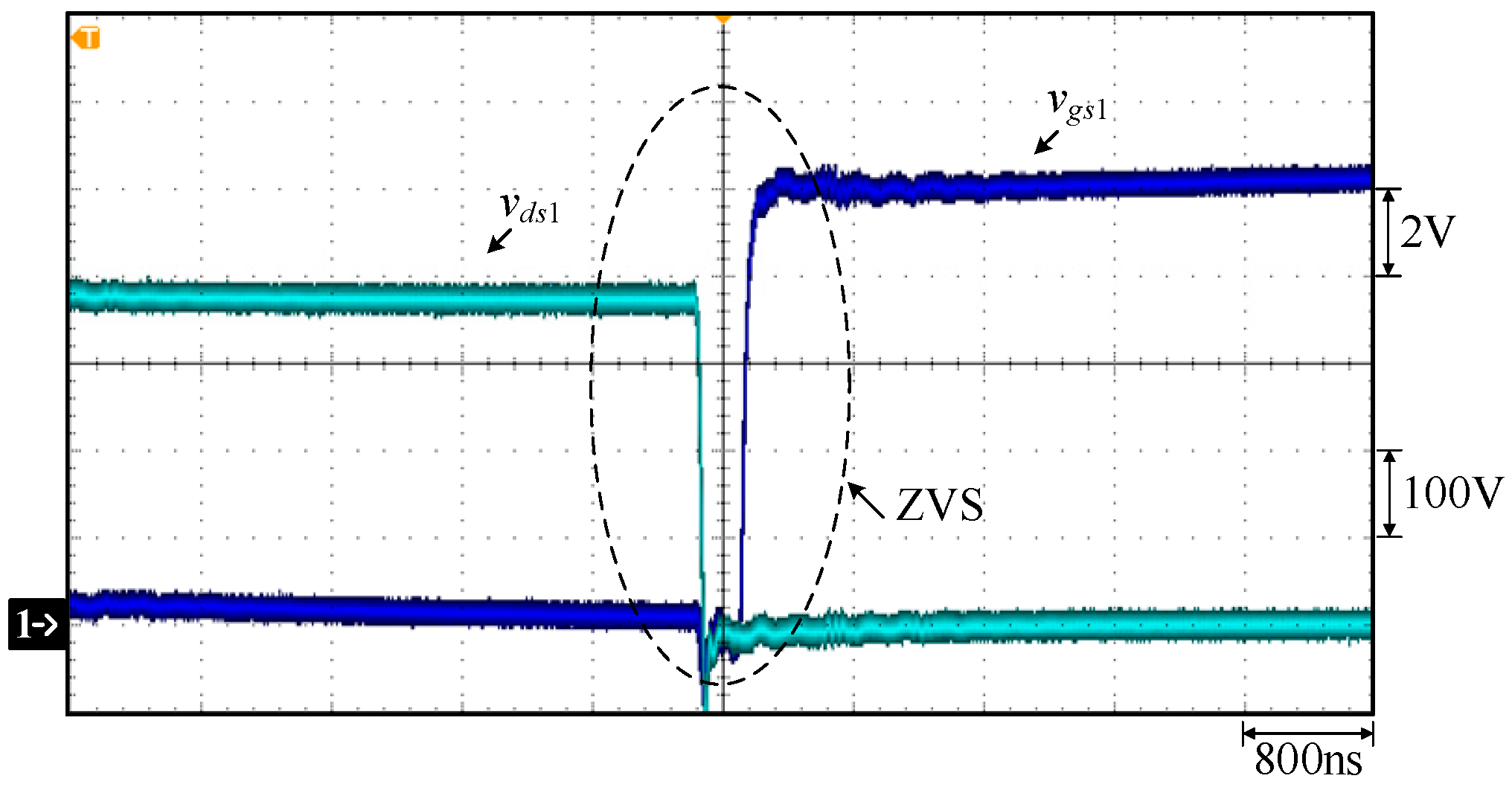
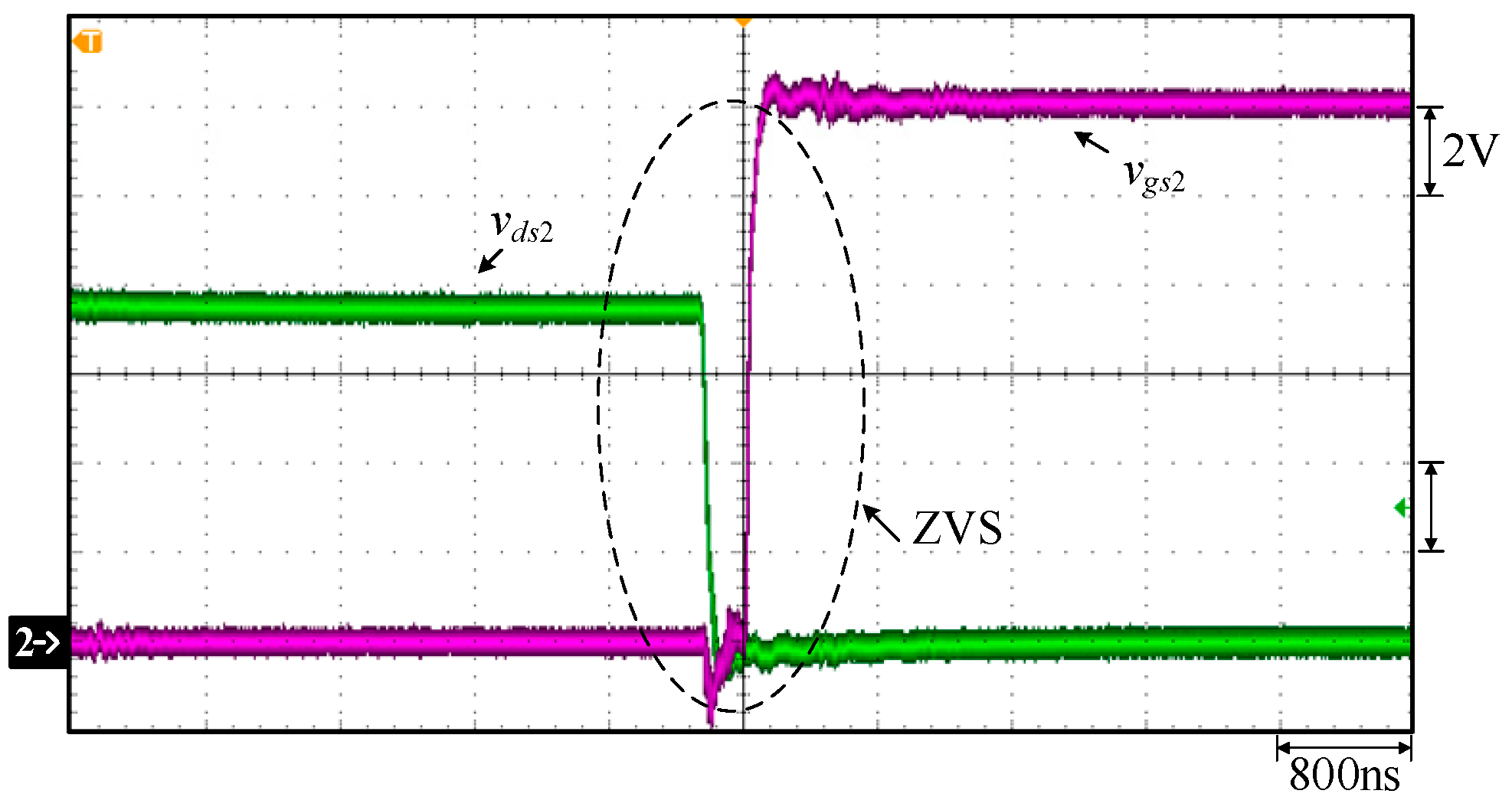
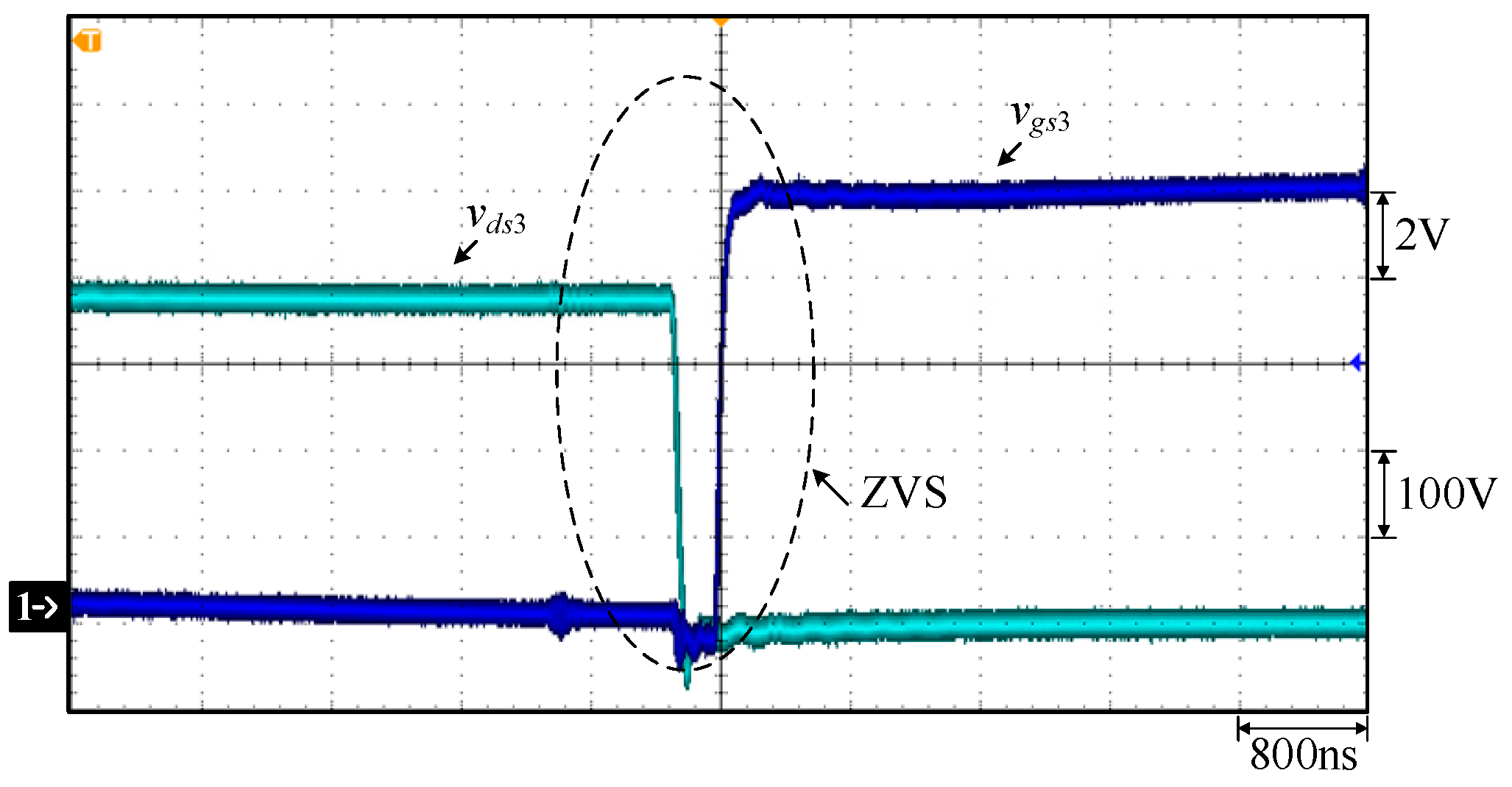
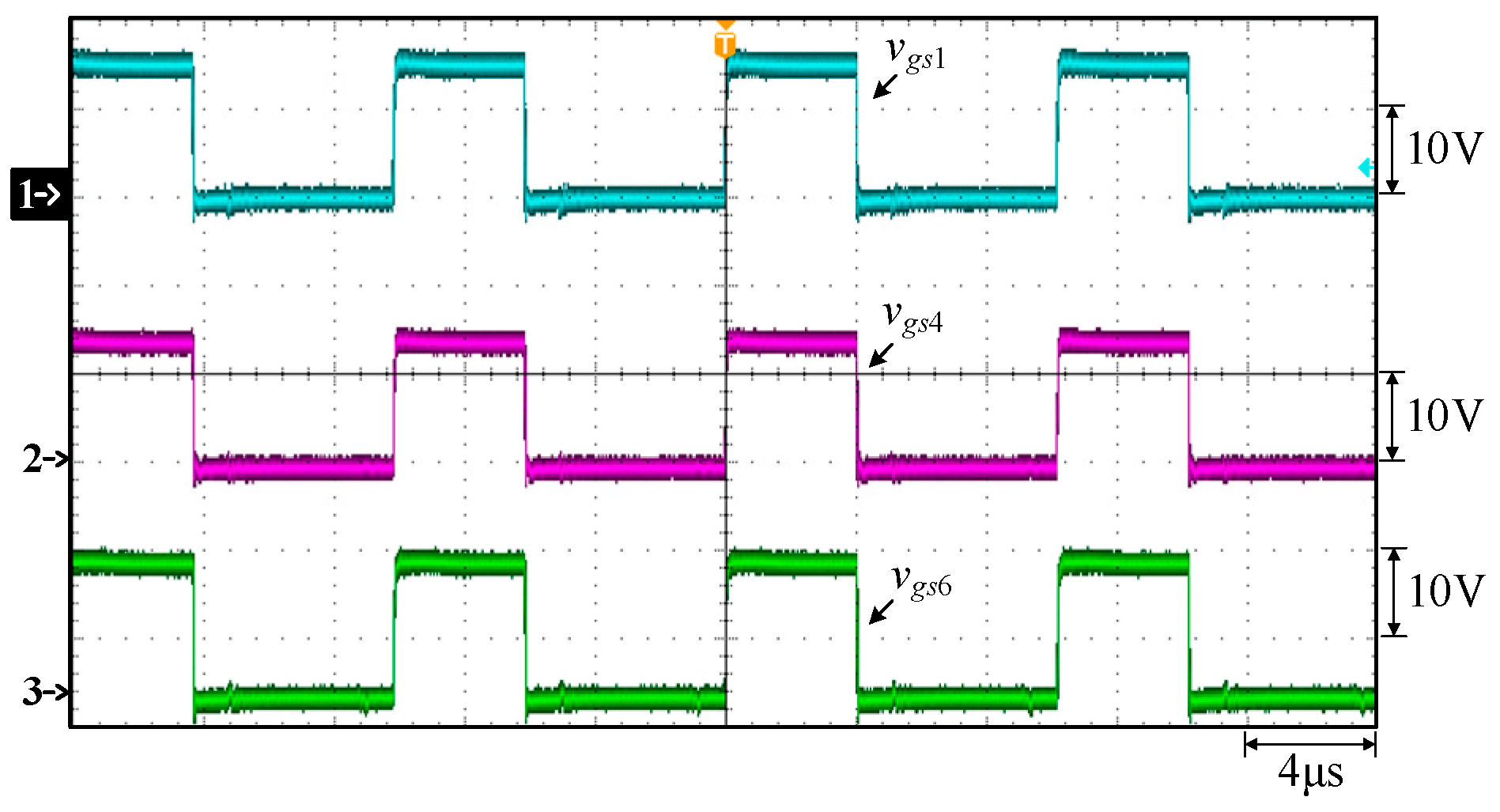
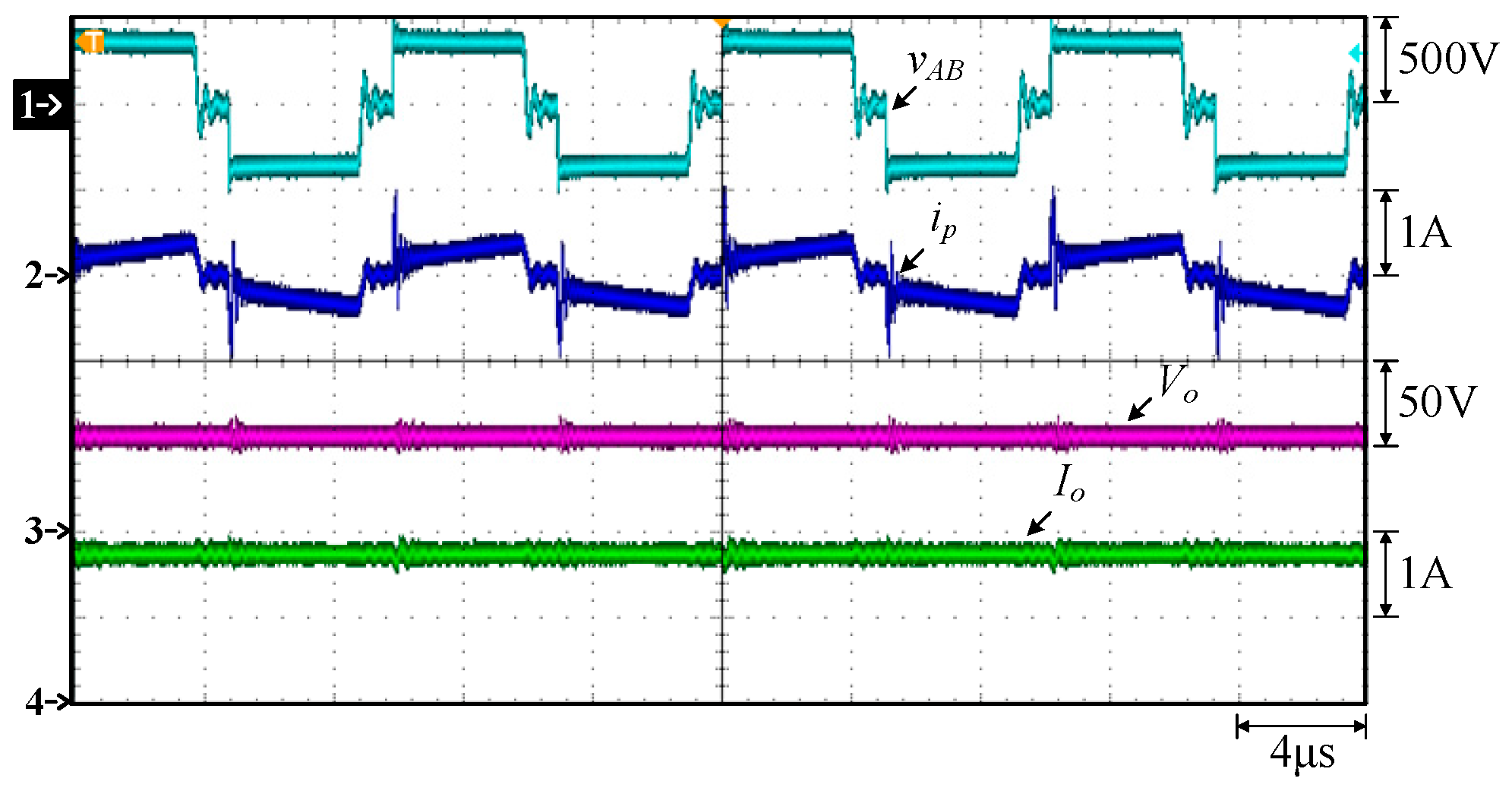
5.1. Measured Waveforms Under Constant Current at 40 V and 17 A for FBPSS
5.2. Measured Waveforms Under Constant Voltage at 58.4 V and 1.7 A for TSFS
5.3. Measured Waveforms for Transferring FBPSS to TSFS
5.4. Measured Waveforms for Transferring TSFS to FBPSS
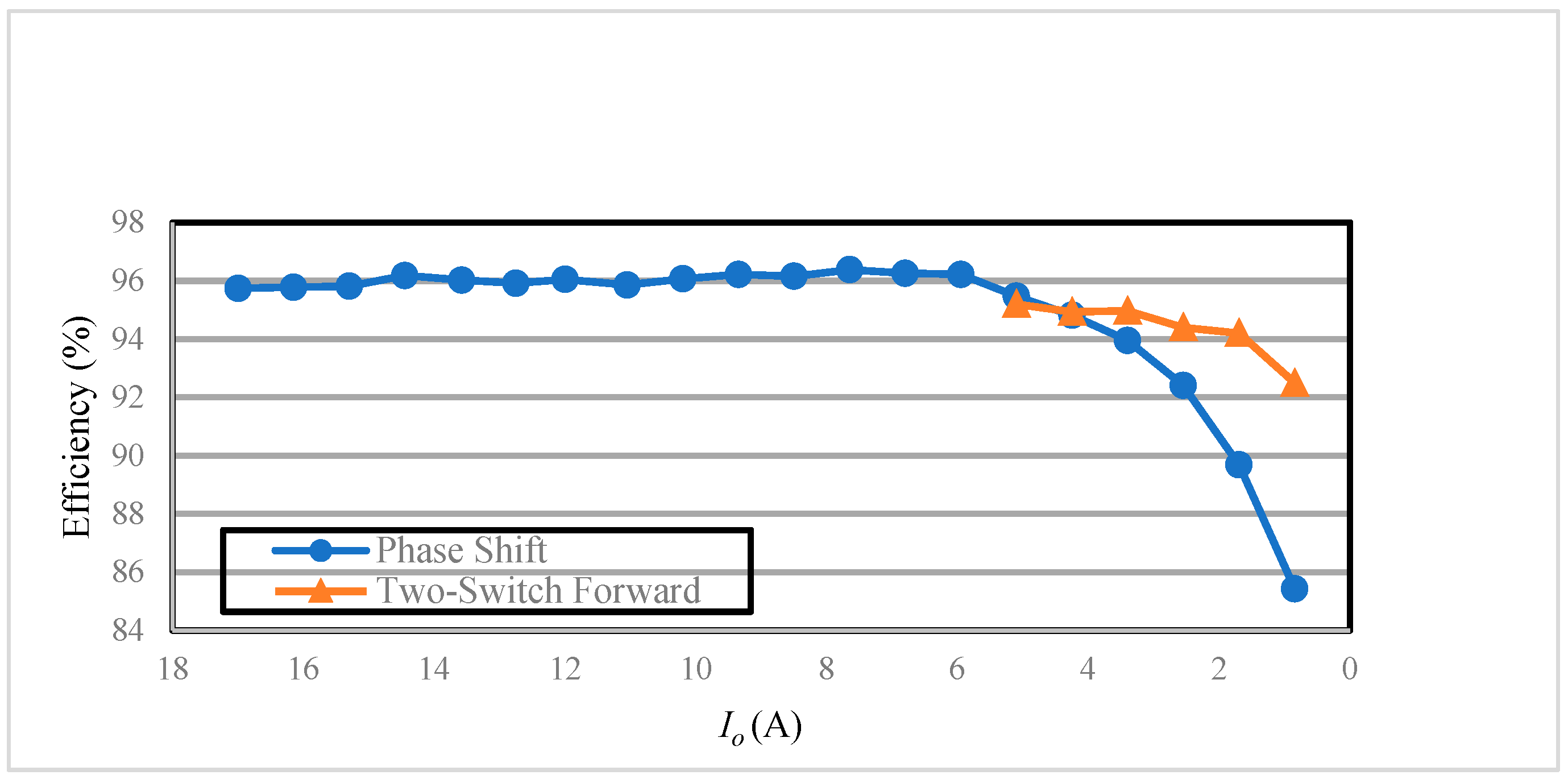
5.5. Efficiency Measurement
5.6. Comparison Between the Proposed Converter and Other Topologies
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Noh, G.; Lee, J.; Ha, J.-I. Design and analysis of single-inductor power converter for both battery balancing and voltage regulation. IEEE Trans. Ind. Electron. 2022, 69, 2874–2884. [Google Scholar] [CrossRef]
- Deng, R.; Liu, Y.; Chen, W.; Liang, H. A survey on electric buses-energy storage, power management, and charging scheduling. IEEE Trans. Intell. Transp. Syst. 2021, 22, 9–22. [Google Scholar] [CrossRef]
- Rahman, M.S.; Rahman, S.F.; Rahman, M.A.; Al Mansur, A. Advancements and Challenges in Electric Vehicle Battery Technology and Charging Solutions: Towards a Sustainable Transportation. In Proceedings of the 6th International Conference on Sustainable Technologies for Industry 5.0 (STI), Dhaka, Bangladesh, 14–15 December 2024; pp. 1–5. [Google Scholar]
- King, B. Selecting Optimal Topology. TI, 2017. Available online: https://www.ti.com/content/dam/videos/external-videos/en-us/2/3816841626001/5589612393001.mp4/subassets/Selecting-Optimal-Topology.pdf (accessed on 17 January 2023).
- Lee, F.C. High-frequency quasi-resonant and multi-resonant converter technologies. In Proceedings of the 14 Annual Conference of Industrial Electronics Society, Singapore, 24–28 October 1988; pp. 509–521. [Google Scholar]
- Phetphimoon, W.; Bhumkittipich, K.; Prabpal, P.; Yupapin, P.; Kongjeen, Y. Phase-shifted full-bridge zvs dc-dc converter with synchronous double rectifiers for battery charging applications. Int. Trans. Electr. Energy Syst. 2022, 2022, 1–12. [Google Scholar] [CrossRef]
- Wang, X.; Zhao, Q.; Zhao, Z.; Meng, F. Full-bridge dc-dc converter with synchronous rectification based on GaN transistors. J. Low Power Electron. Appl. 2025, 15, 25. [Google Scholar] [CrossRef]
- Gautam, K.K.; Chatterjee, A.; Prasad, D. Inherent constant current constant voltage (CCCV) charging of electric vehicles by using lcl resonant converter. In Proceedings of the 2nd International Conference for Innovation in Technology (INOCON), Bangalore, India, 10–12 March 2023; pp. 1–6. [Google Scholar]
- Di, Z.; Donglai, Z.; Zicai, W.; Hua, Z.; Ying, Z. Current-sharing active-clamped forward power converter with self-driven synchronous rectifier. In Proceedings of the IEEE 3rd International Conference on Electronics Technology (ICET), Chengdu, China, 8–11 May 2020; pp. 334–338. [Google Scholar]
- Wang, J.; Lu, B. Open loop synchronous rectifier driver for LLC resonant converter. In Proceedings of the IEEE Applied Power Electronics Conference and Exposition (APEC), Long Beach, CA, USA, 17–21 March 2013; pp. 2048–2051. [Google Scholar]
- Ho, G.K.Y.; Yu, R.; Pong, B.M.H. Current driven synchronous rectifier for LLC resonant converter with a novel integrated current transformer. In Proceedings of the IET International Conference on Power Electronics, Machines and Drives (PEMD 2012), Bristol, UK, 27–29 March 2012; pp. 1–5. [Google Scholar]
- Fei, C.; Li, Q.; Lee, F.C. Digital implementation of adaptive synchronous rectifier driving scheme for high-frequency LLC converters with microcontroller. IEEE Trans. Power Electron. 2018, 33, 5351–5361. [Google Scholar] [CrossRef]
- López-Santos, O.; Zambrano-Prada, D.A.; Valderrama-Blavi, H.; Flores-Bahamonde, F.; Pinzón, C.A.T.; Martínez-Salamero, L. Experimental efficiency comparison between the llc resonant converter and the phase shifted full bridge converter operating as battery chargers. In Proceedings of the 50th Annual Conference of the IEEE Industrial Electronics Society (IECON), Chicago, IL, USA, 20–23 October 2024; pp. 1–6. [Google Scholar]
- Werulkar, A.S.; Kulkarni, P.S. Efficiency improvement of a dual boost converter with zero voltage switching technique for battery charging application of a solar home lighting system. In Proceedings of the IEEE First International Conference on Smart Technologies for Power, Energy and Control (STPEC), Nagpur, India, 25–26 September 2020; pp. 1–6. [Google Scholar]
- Geda, M.G.; Song, H.; Do, B.P.; Choi, S. Light load efficiency enhancement strategy for single-stage ac-dc by using adaptive switching frequency. In Proceedings of the IEEE 10th International Power Electronics and Motion Control Conference (IPEMC2024-ECCE Asia), Kitakyushu, Japan, 12–15 May 2024; pp. 1–5. [Google Scholar]
- Lu, Y.; Wu, H.; Dong, X.; Xing, Y. Light load efficiency improvement for distributed battery energy storage system. In Proceedings of the 42nd Annual Conference of the IEEE Industrial Electronics Society (IECON), Florence, Italy, 23–26 October 2016; pp. 1–5. [Google Scholar]
- Dhiman, S.; Vasudevan, K. Optimal design of battery charging converters based on energy efficiency. In Proceedings of the IEEE 12th Energy Conversion Congress & Exposition-Asia (ECCE-Asia), Singapore, 24–27 May 2021; pp. 1–6. [Google Scholar]
- Sharma, D.K.; B K Vardhan, H.; Hulipalled, V.R. EV home charging infrastructure & low battery AI algorithm. In Proceedings of the Third International Conference on Smart Technologies in Computing, Electrical and Electronics (ICSTCEE), Bengaluru, India, 16–17 December 2022; pp. 1–9. [Google Scholar]
- Rakshith, S.K.; Rohit, S.; Sutha, S.; Goyal, H.R. Smart energy: Artificial intelligence (AI) in charging and battery management systems. In Proceedings of the 5th International Conference on Intelligent Communication Technologies and Virtual Mobile Networks (ICICV), Tirunelveli, India, 4–6 January 2024; pp. 68–73. [Google Scholar]
- KS, K.K.; Chitra, L.; Abraham, J.M.; Joseph, N.; TK, Y.K. The role of IOT & AI in battery management of electric vehicles. In Proceedings of the International Conference on Signal Processing, Computation, Electronics, Power and Telecommunication (IConSCEPT), Kollam, India, 8–9 March 2023; pp. 1–7. [Google Scholar]
- Tran, D.; Vu, H.; Yu, S.; Choi, W. A novel soft-switching full-bridge converter with a combination of a secondary switch and a nondissipative snubber. IEEE Trans. Power Electron. 2018, 33, 1440–1452. [Google Scholar] [CrossRef]
- Lim, C.; Han, J.; Park, M.; Kim, K.; Moon, G. Phase-shifted full-bridge DC-DC converter with high efficiency and reduced output filter using center-tapped clamp circuit. In Proceedings of the IEEE Applied Power Electronics Conference and Exposition (APEC), Anaheim, CA, USA, 17–21 March 2019; pp. 1710–1715. [Google Scholar]
- Lim, C.; Jeong, Y.; Lee, M.; Yi, K.; Moon, G. Half-bridge integrated phase-shifted full-bridge converter with high efficiency using center-tapped clamp circuit for battery charging systems in electric vehicles. IEEE Trans. Power Electron. 2020, 35, 4934–4945. [Google Scholar] [CrossRef]
- Lee, I.; Moon, G. Phase-shifted PWM converter with a wide ZVS range and reduced circulating current. IEEE Trans. Power Electron. 2013, 28, 908–919. [Google Scholar] [CrossRef]
- Kim, Y.-D.; Cho, K.-M.; Kim, D.-Y.; Moon, G.-W. Wide-range ZVS phase-shift full-bridge converter with reduced conduction loss caused by circulating current. IEEE Trans. Power Electron. 2013, 28, 3308–3316. [Google Scholar] [CrossRef]
- Kim, D.; Kim, C.; Moon, G. Variable delay time method in the phase-shifted full-bridge converter for reduced power consumption under light load conditions. IEEE Trans. Power Electron. 2013, 28, 5120–5127. [Google Scholar] [CrossRef]
- Liu, B.H.; Teng, J.H.; Lin, M.Y.; Huang, C.C. Light-load conversion efficiency improvement strategy for phase-shift full-bridge converters. In Proceedings of the IEEE International Future Energy Electronics Conference and ECCE Asia (IFEEC 2017-ECCE Asia), Kaohsiung, Taiwan, 3–7 June 2017; pp. 488–493. [Google Scholar]
- Sun, L.; Zhang, X.; Liu, B.; Wang, S.; Wang, Y.; Xu, D. Efficiency optimization of phase-shift full-bridge through adaptive dead-time and burst-mode at very light load. In Proceedings of the International Conference on Electrical Machines and Systems (ICEMS), Harbin, China, 11–14 August 2019; pp. 1–5. [Google Scholar]
- Arabsalmanabadi, B.; Tashakor, N.; Javadi, A.; Al-Haddad, K. Charging techniques in Lithium-ion battery charger: Review and new solution. In Proceedings of the 44th Annual Conference of the IEEE Industrial Electronics Society (IECON), Washington, DC, USA, 21–23 October 2018; pp. 5731–5738. [Google Scholar]
- Lan, Z.; Li, Y.; Gao, F.; Qiao, F. Research and implementation of step high voltage constant current closed-loop pre-charging technology. In Proceedings of the Advanced Electronic Materials, Computers and Software Engineering (AEMCSE), Changsha, China, 24–26 April 2020; pp. 810–815. [Google Scholar]
- Wang, S.; Liu, Y.; Wang, X. Resonant converter for battery charging applications with CC/CV output profiles. IEEE Access 2020, 8, 54879–54886. [Google Scholar] [CrossRef]
- Sun, T.-W.; Tsai, T.-H. A battery management system using interleaved pulse charging with charge and temperature balancing based on NARX network. IEEE Trans. Circuits Syst. I Regul. Pap. 2022, 69, 1811–1819. [Google Scholar] [CrossRef]
- Ahmadi, F.; Adib, E.; Azari, M. Soft switching bidirectional converter for reflex charger with minimum switches. IEEE Trans. Ind. Electron. 2020, 67, 8355–8362. [Google Scholar] [CrossRef]
- Lee, Y.-L.; Chen, H.-H.; Hwu, K.-I. Applying a current sharing method based on partial energy processing to multiphase LLC resonant converters. Energies 2024, 17, 3859. [Google Scholar] [CrossRef]












| Topology | Output Power |
|---|---|
| Flyback Converter | Below 100 W |
| Forward Converter | Between 100 W and 500 W |
| Half-Bridge Converter | |
| Full-Bridge Converter | Above 500 W |
| Rated Input Voltage (Vin) | 380 V |
| Rated Input Voltage (Vo) | 58.4 V |
| Rated Output Current (Io,rated) | 17 A |
| Minimum Output Current (Io,min) | 1.7 A |
| Output Power (Po) | 1 kW |
| Switching Frequency (fs) | 100 kHz |
| Component | Specification |
|---|---|
| SiC FET Switches S1, S2, S3, S4 | UJ3C065080T3S |
| SR MOSFET Switches S5, S6 | IPP200N25N3G |
| Resonant Capacitor | 488 µF |
| Resonant Inductor | 60.4 µH |
| Additional Resonant Inductor Lext | 48.2 µH |
| Output Inductor Lo | 51.4 µH |
| Output Capacitor Co | 660 µF/63 V |
| [6] | [7] | [13] | [14] | [15] | [16] | [28] | Proposed | ||
| Circuit Structure | Full-Bridge + SR | Full-Bridge + SR | Full-Bridge + Resonant Tank+ Full-Bridge Rectifier | Dual-Boost | Full-Bridge + Resonant Tank+ Full-Bridge Converter | DAHB+ Buck-Boost | Half-Bridge + Resonant Tank+ Full-Bridge Rectifier | Full-Bridge + Two-Switches | |
| Rated-load Power (W) | 1176 | --- | 1100 | 37 | 3700 | 1000 | 2000 | 1000 | |
| Output Voltage (V) | 75 | 24 | 110 | 15 | 630 | 400 | 0 | 58.4 | |
| Switching Frequency (kHz) | 300 | 200 | 55 | 20 | 130 | --- | 28 | 100 | |
| Switches | GaN Transistor | Mosfet | Mosfet | Mosfet | Mosfet | Mosfet | IGBT | SiC FET | |
| Light-load Power (W) | 120 | --- | 100 | 6.2 | 370 | 50 | --- | 100 | |
| Light-load Power Ratio (%) | 10.0 | 40.0 | 9.1 | 16.76 | 10.0 | 5.0 | --- | 10.0 | |
| Light-load Efficiency (%) | 91.8 | 88.1 | 91.2 | 91.3 | 92.5 | 77.3 | 88.9 | 92.4 | |
| Control Strategy | Phase-Shift | Phase-Shift | LLC Resonant | PWM | Adaptive-Frequency | PWM | Phase-Shift | Phase-Shift + Structure Exchange | |
| Isolation | v | v | x | x | v | x | v | v | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hwu, K.-I.; Tseng, P.-C.; Shieh, J.-J. Applying Structure Exchange to Battery Charging to Enhance Light-Load Efficiency. Energies 2025, 18, 2699. https://doi.org/10.3390/en18112699
Hwu K-I, Tseng P-C, Shieh J-J. Applying Structure Exchange to Battery Charging to Enhance Light-Load Efficiency. Energies. 2025; 18(11):2699. https://doi.org/10.3390/en18112699
Chicago/Turabian StyleHwu, Kuo-Ing, Pei-Ching Tseng, and Jenn-Jong Shieh. 2025. "Applying Structure Exchange to Battery Charging to Enhance Light-Load Efficiency" Energies 18, no. 11: 2699. https://doi.org/10.3390/en18112699
APA StyleHwu, K.-I., Tseng, P.-C., & Shieh, J.-J. (2025). Applying Structure Exchange to Battery Charging to Enhance Light-Load Efficiency. Energies, 18(11), 2699. https://doi.org/10.3390/en18112699








