Topology Optimization Design of Phase Change Liquid Cooling Composite Plate
Abstract
1. Introduction
2. Phase Change-Liquid Cooling Channel Optimization
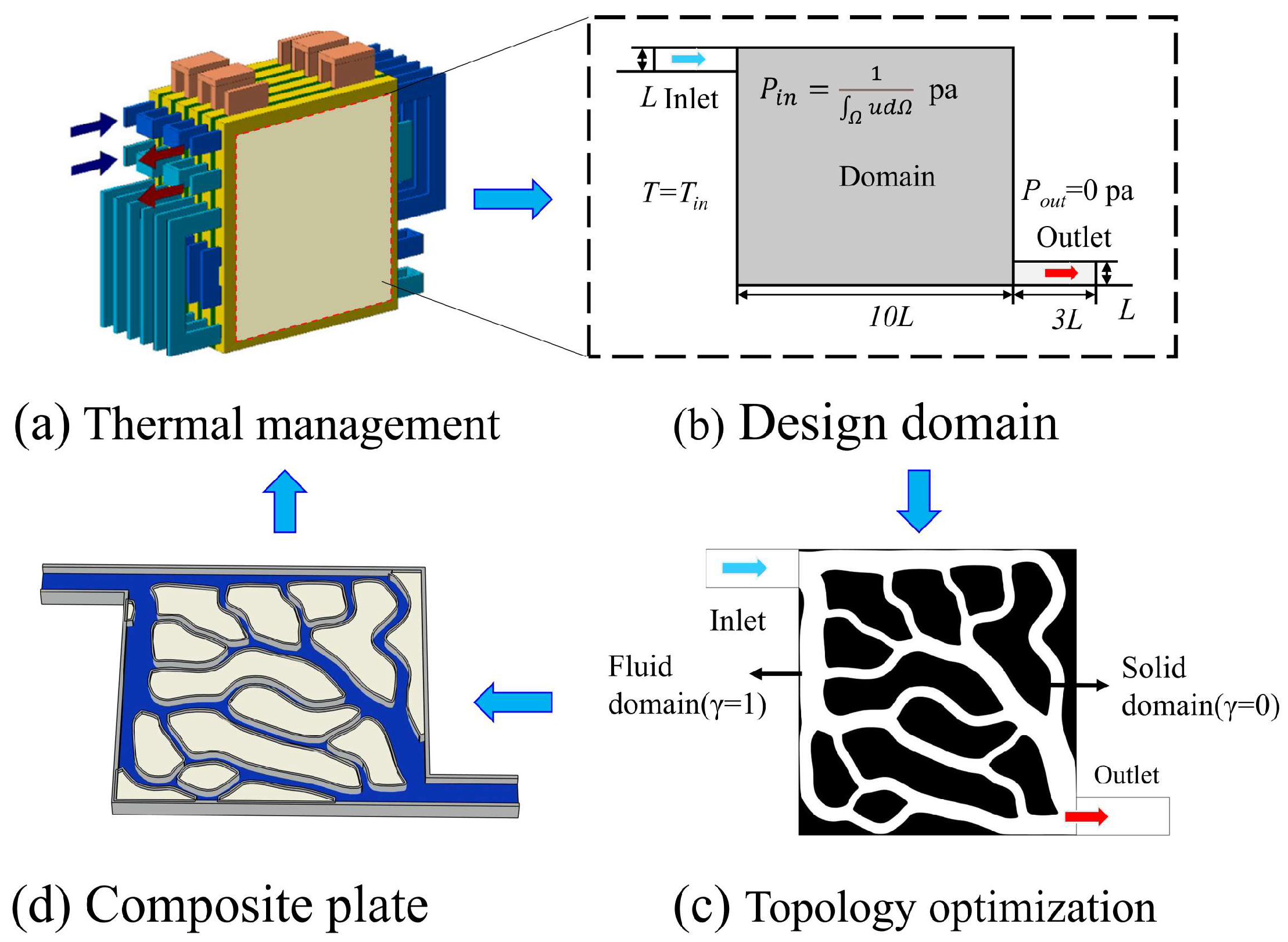
2.1. Physical Model
2.2. Governing Equations
2.2.1. Fluid Model
2.2.2. Conjugate Heat Transfer Model
2.3. Optimization Problem
2.3.1. Optimization Mathematical Model
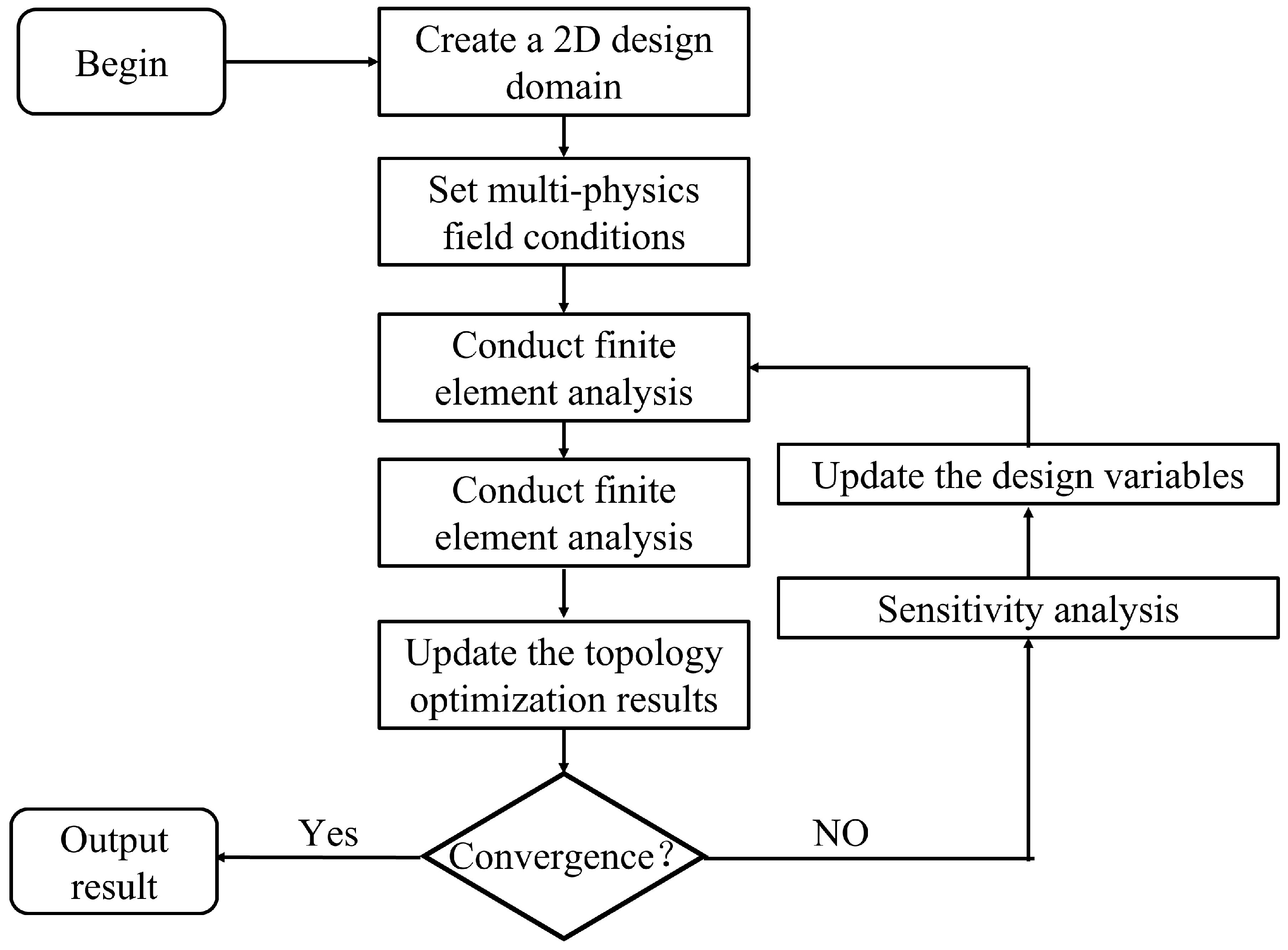
2.3.2. Model Solution
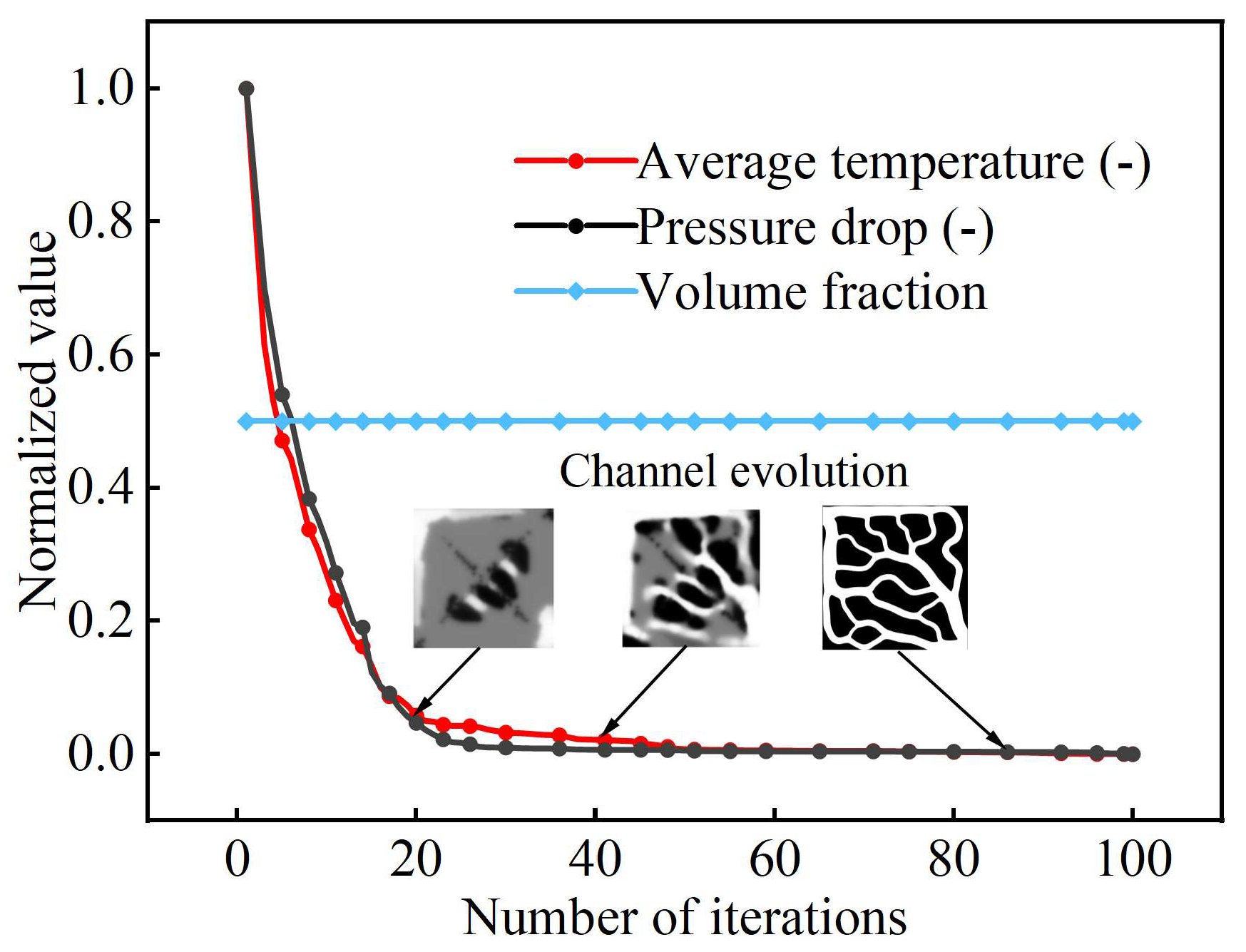
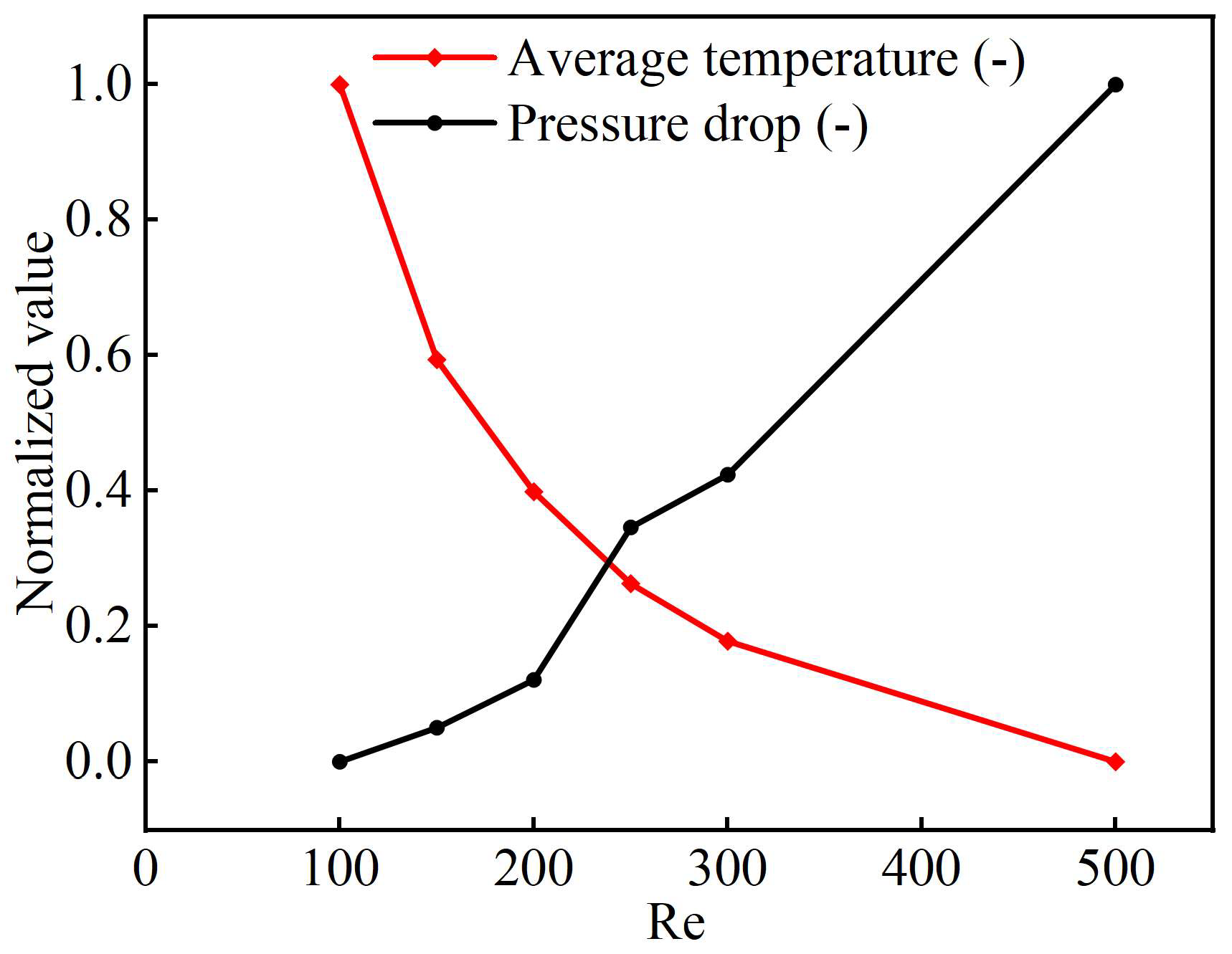
2.4. Analysis of Topology Optimization Results
3. Phase Change Liquid Cooling Coupled Heat Transfer Model
3.1. Phase Change Heat Transfer Model
3.1.1. Governing Equations
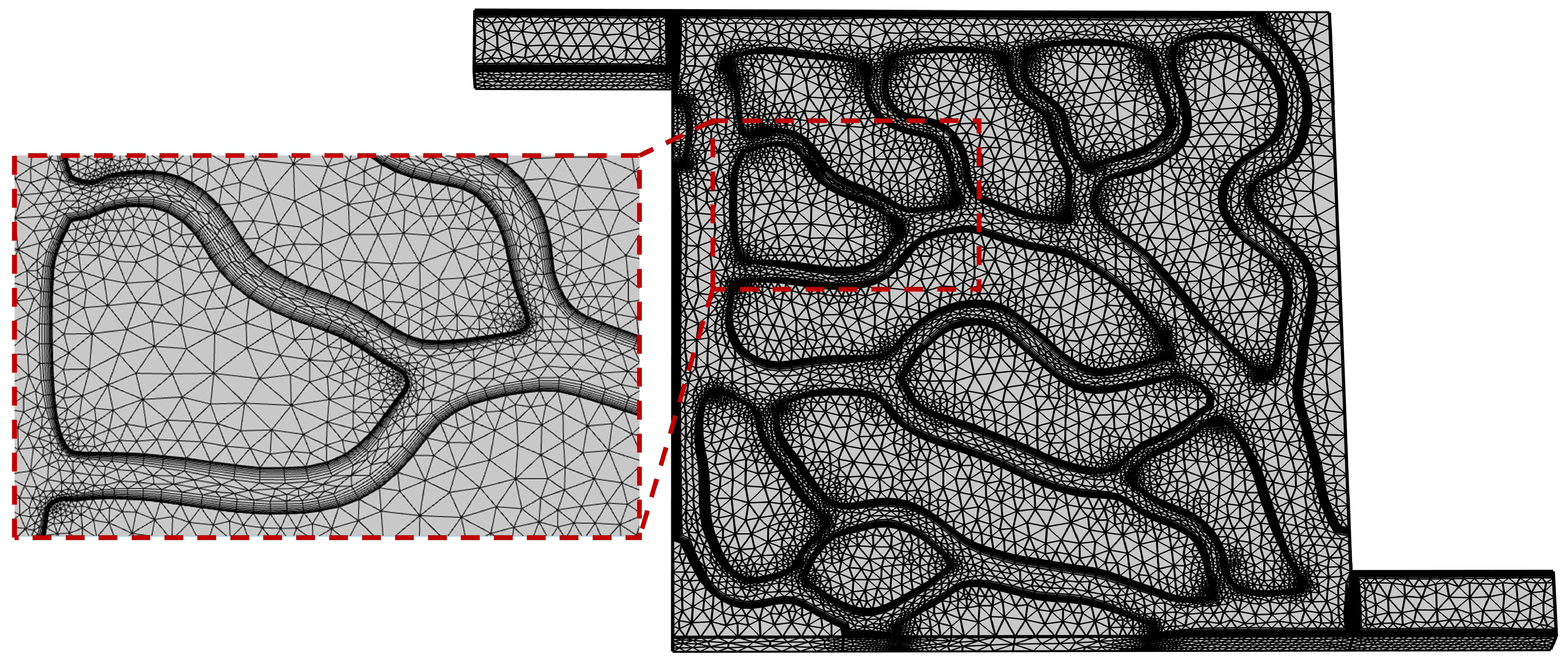
3.1.2. Mesh Independence Verification
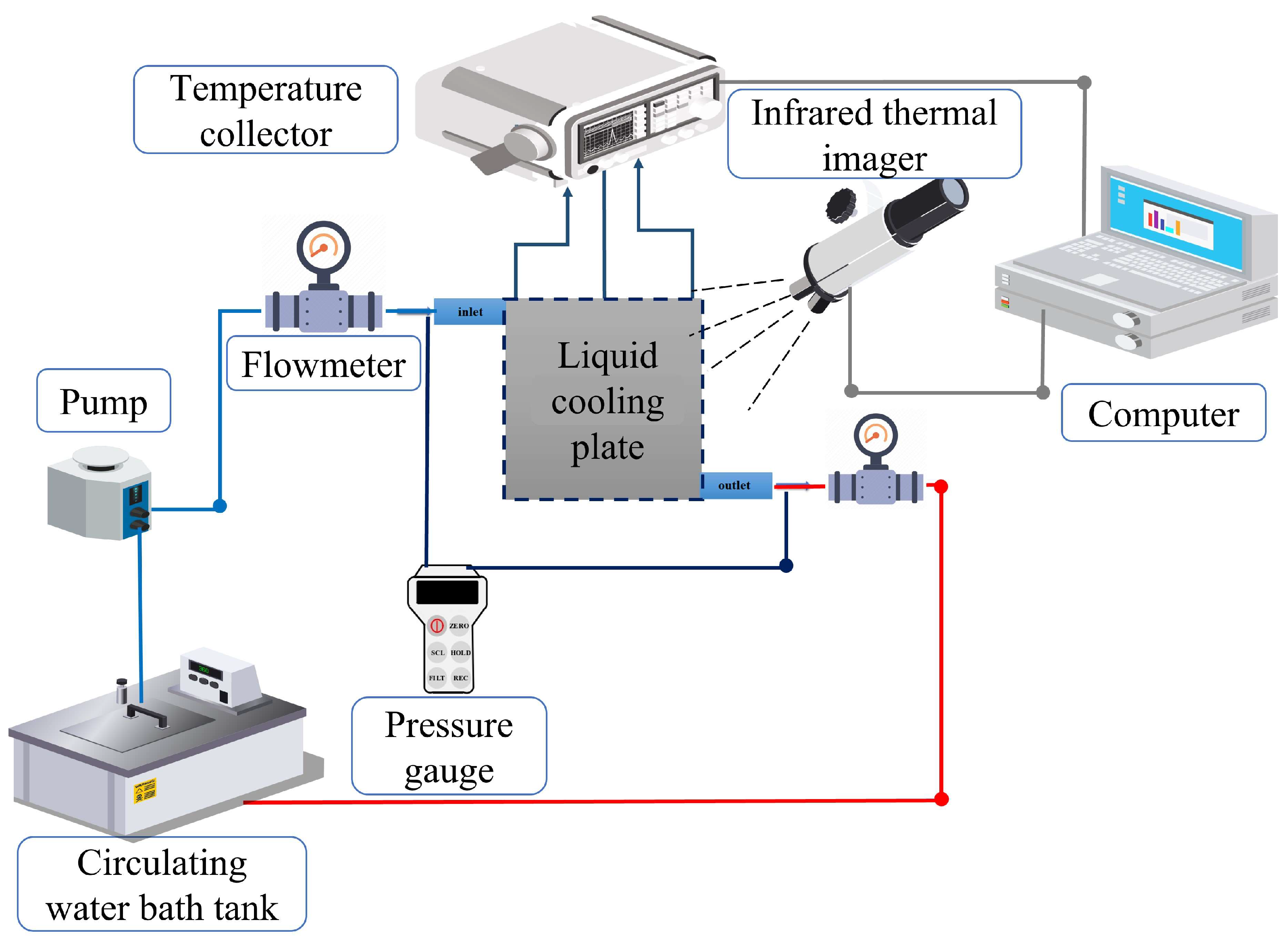
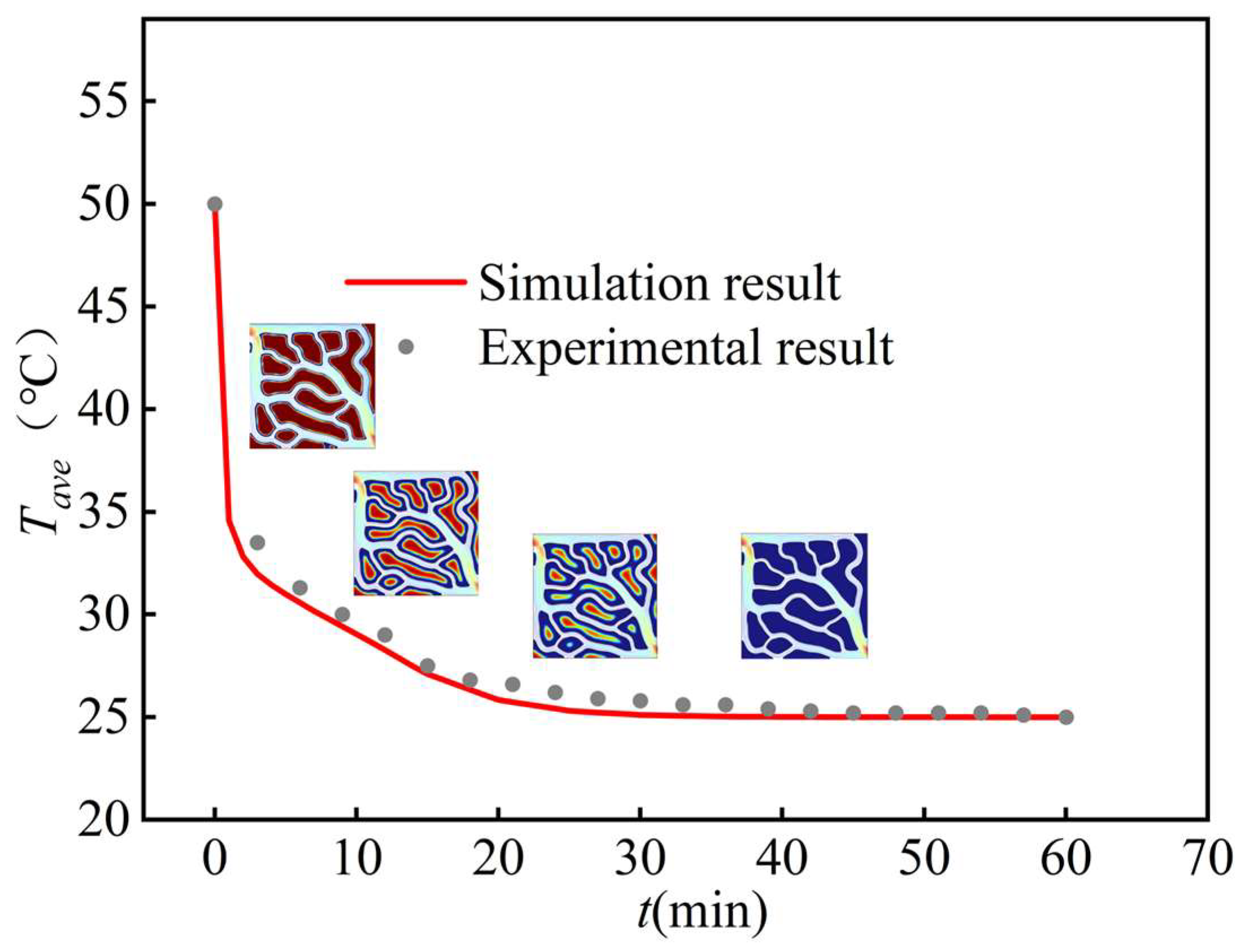
3.2. Experimental Validation
4. Performance Comparison Between Topological Channels and Conventional Channels
4.1. Physical Model
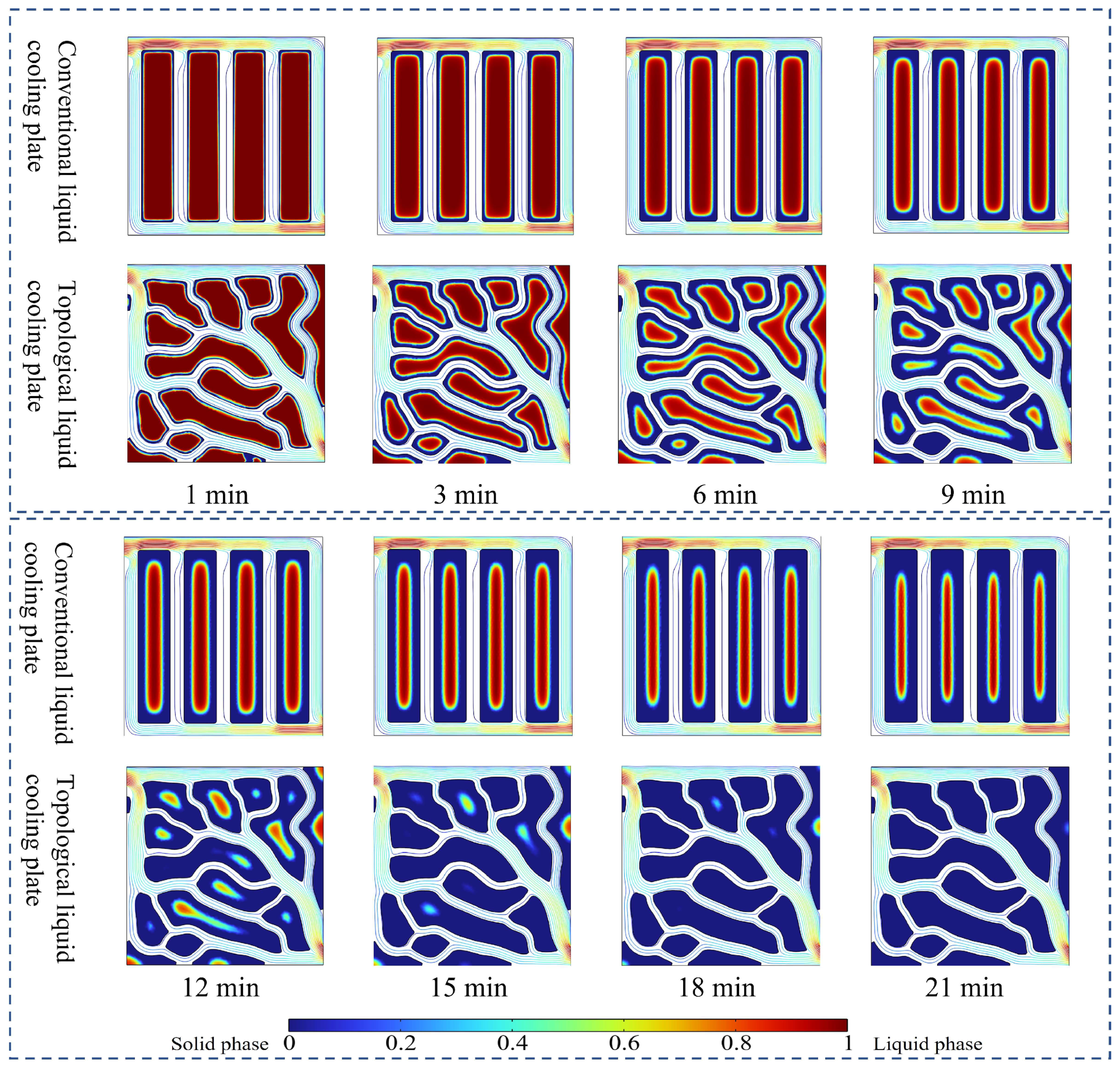
4.2. Phase Change and Temperature Distribution Characteristics
4.3. Resistance Characteristics
4.4. Heat Transfer Performance
5. Conclusions
- (1)
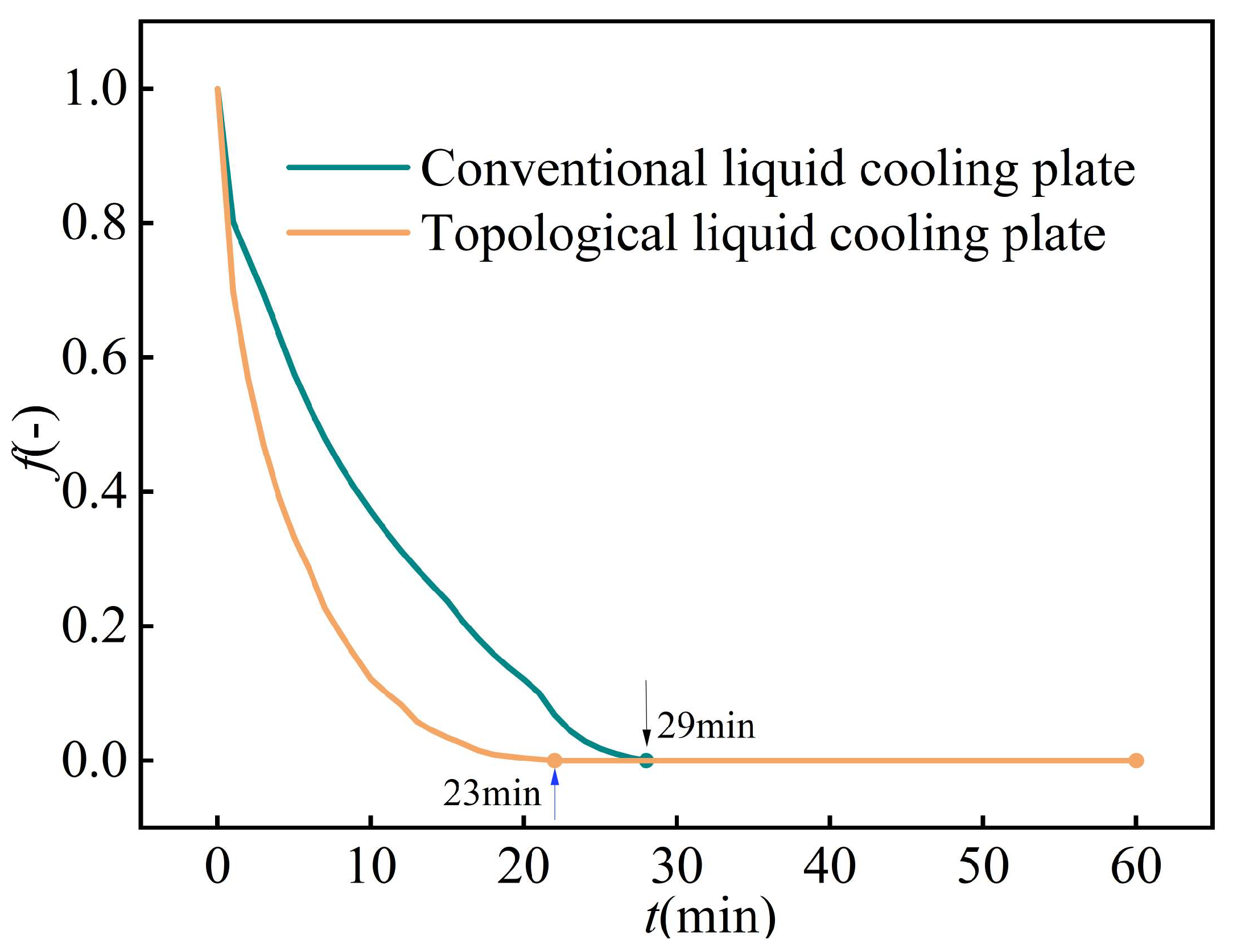
- During the phase change solidification process, the PCM in the conventional and topological liquid cooling plates reached full solidification at 29 min and 23 min, respectively, with the topological design reducing the complete phase change solidification time by 17.2%. This can be attributed to the discrete phase change regions of the multi-branch structure, which shortened the thermal conduction distance of the phase change material and accelerated the phase-change process.
- (2)
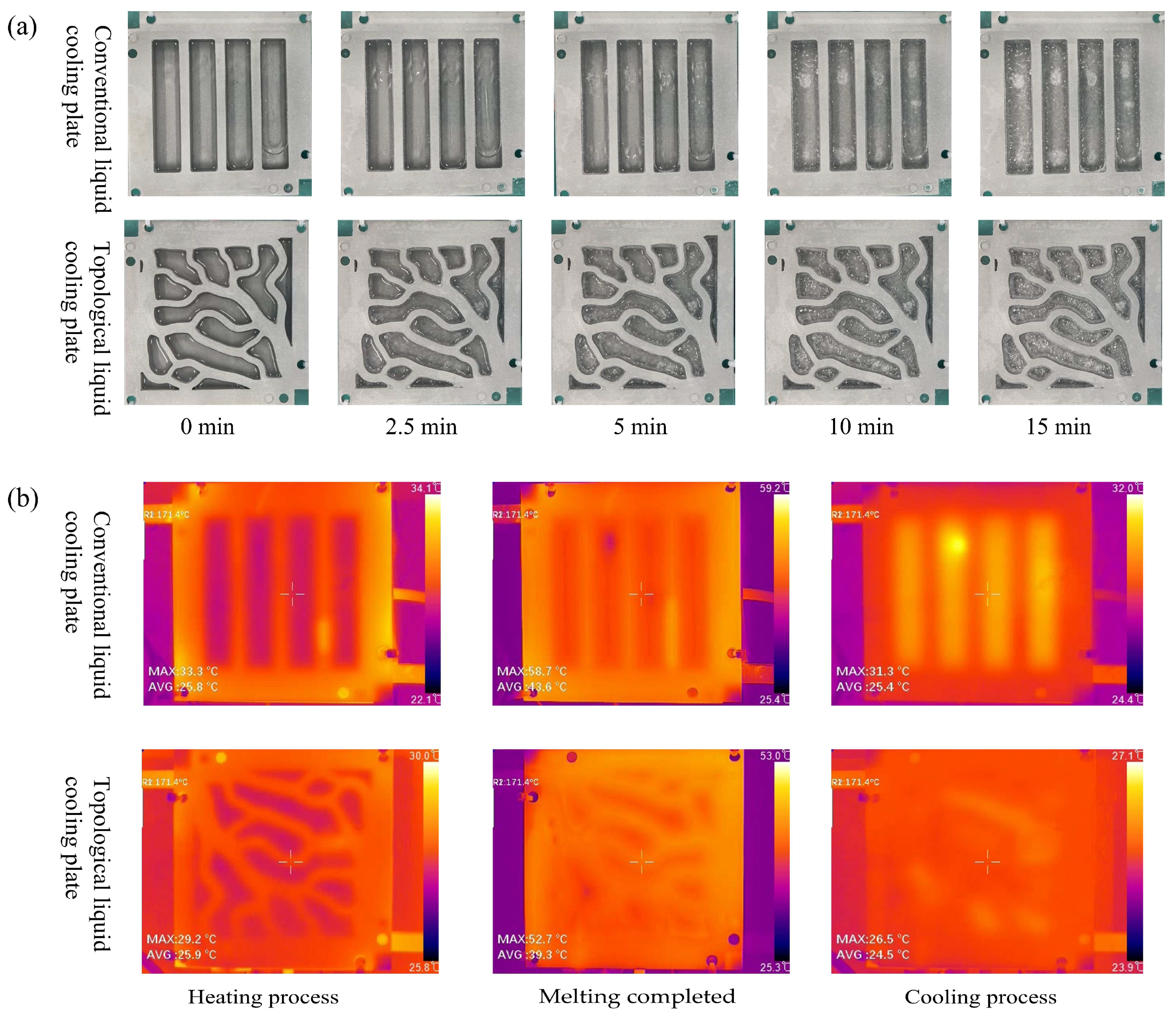
- The pressure drop (ΔP) and pumping power (Ppump) of the topologically optimized liquid cooling plate were 38.28% and 38.02% lower, respectively, compared to the conventional liquid cooling plate. This indicates that the flow channel structure of the topologically optimized liquid cooling plate allows for more uniform coolant flow, resulting in smaller pressure losses within the channels. This effectively reduces flow resistance, thereby lowering the pumping power requirement and improving the overall energy efficiency of the cooling system. The experiments show that when the PCM in the liquid cooling plates had completely melted, the conventional plate exhibited maximum and average temperatures of 58.7 °C and 43.6 °C, while the topological plate showed 52.7 °C and 39.3 °C (10.22% and 9.86% lower, respectively). This demonstrates that the topological design achieves a more reasonable structural PCM distribution, effectively suppresses local heat accumulation, and enhances the thermal equilibrium capability of the phase change system.
- (3)
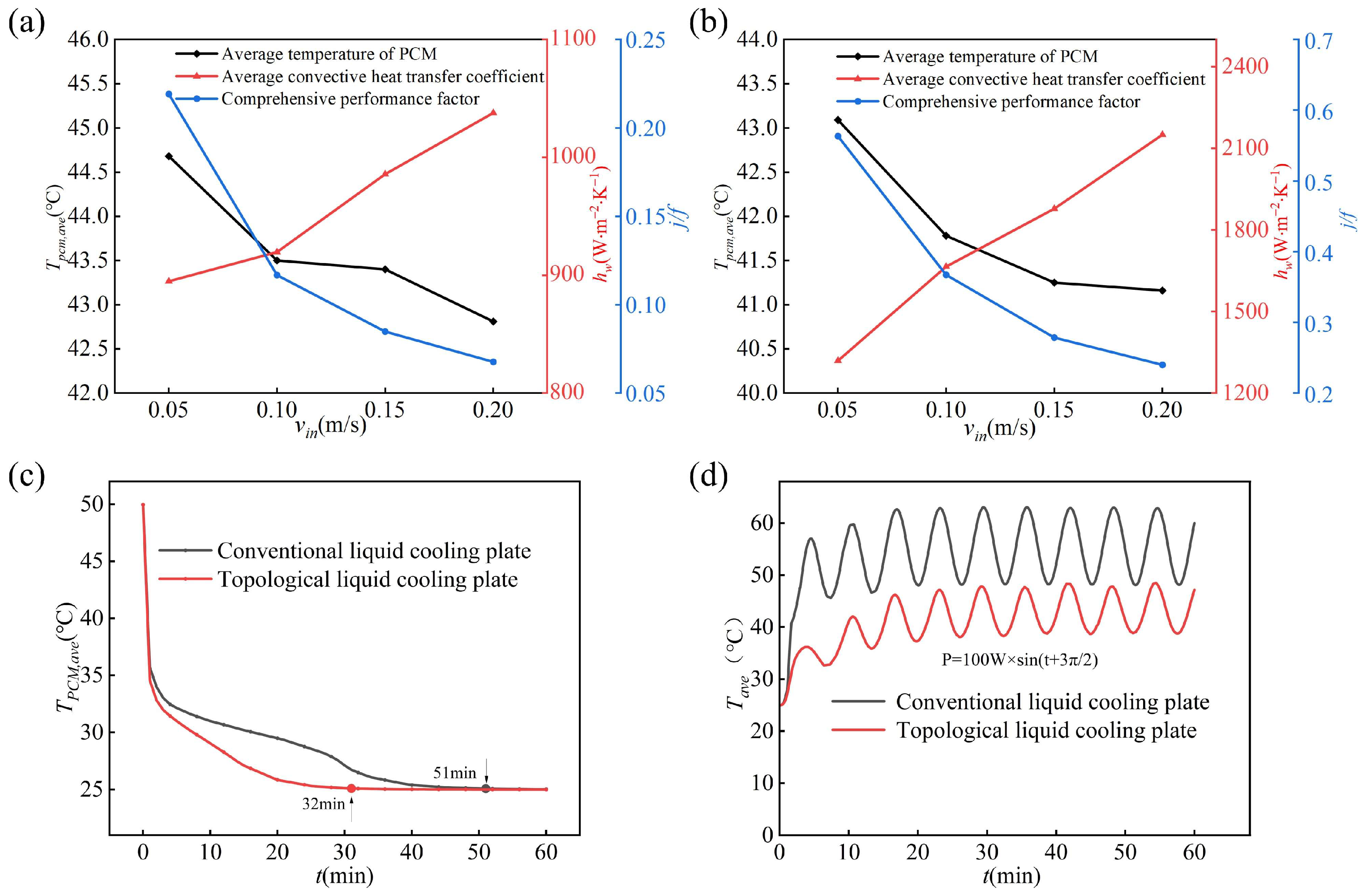
- During the cooling process, the average temperature of the phase change material (PCM) in the topology-optimized liquid cooling plate was lower by 3.6 °C than that of the conventional design. At a flow velocity of 0.05 m/s, the average convective heat transfer coefficient hw of the topologically optimized liquid cooling plate reached 1319.06 W/(m2·K), which was approximately 47.5% higher than that of the conventional liquid cooling plate (894.876 W/(m2·K)). As the flow velocity increased from 0.05 m/s to 0.2 m/s, hw further improved by 38.65%. However, Tpcm,ave decreased by only 0.62 °C. This limited temperature reduction is primarily attributable to the low thermal conductivity of paraffin (0.2 W/m·K), which restricts effective heat transfer within the PCM. Moreover, the increase in flow velocity led to a significant decline in the overall performance factor j/f, which dropped by 57.14%. This suggests that while enhanced convection improves heat transfer, it also substantially increases system energy consumption, thereby reducing the overall thermal performance. This is because the cooling performance of the liquid cooling plate is not solely determined by the convective heat transfer capability of the coolant but is also constrained by the thermal response rate of the PCM. Due to its limited thermal conductivity, the latent heat released within the PCM cannot be conducted rapidly to the heat exchange interface, resulting in solidification being confined to the interfacial region. Consequently, when the flow velocity—and, thus, the Reynolds number—increased, Tpcm,ave showed only a marginal decrease. Benefiting from superior heat transfer performance and temperature uniformity, the topology-optimized liquid cooling plate exhibits better thermal response characteristics and steady-state regulation capability under transient heat load, compared to the conventional design.
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Lu, H.; Tang, X. A flexible optimization study on air-cooled battery thermal management system by considering of system volume and cooling performance. J. Energy Storage 2023, 72, 108527. [Google Scholar] [CrossRef]
- Peng, X.; Cui, X.; Liao, X.; Garg, A. A Thermal Investigation and Optimization of an Air-Cooled Lithium-Ion Battery Pack. Energies 2020, 13, 2956. [Google Scholar] [CrossRef]
- Shengxin, E.; Liu, Y.; Cui, Y.; Wu, A.; Yin, H. Effects of composite cooling strategy including phase change material and cooling air on the heat dissipation performance improvement of lithium ion power batteries pack in hot climate and its catastrophe evaluation. Energy 2023, 283, 129074. [Google Scholar] [CrossRef]
- Santosh, C.; Jie, L.; Venkateswarlu, B.; Woo, J.S.; Chul, K.S. Numerical simulation of lithium-ion battery thermal management systems: A comparison of fluid flow channels and cooling fluids. J. Energy Storage 2023, 73, 108940. [Google Scholar]
- Xu, J.; Chen, Z.; Qin, J.; Pan, M. A lightweight and low-cost liquid-cooled thermal management solution for high energy density prismatic lithium-ion battery packs. Appl. Therm. Eng. 2022, 203, 117871. [Google Scholar] [CrossRef]
- Yu, Z.P.; Zhang, J.K.; Pan, W.G. A review of battery thermal management systems about heat pipe and phase change materials. J. Energy Storage 2023, 62, 106827. [Google Scholar] [CrossRef]
- Jiang, K.; Liao, G.; E, J.; Zhang, F.; Chen, J.; Leng, E. Thermal management technology of power lithium-ion batteries based on the phase transition of materials: A review. J. Energy Storage 2020, 32, 101816. [Google Scholar] [CrossRef]
- Ouikhalfan, M.; Sari, A.; Hekimoglu, G.; Gencel, O.; Tyagi, V.V. Thermal energy storage properties, thermal conductivity, chemical/and thermal reliability of three different organic phase change materials doped with hexagonal boron nitride. Surf. Interfaces 2022, 32, 102176. [Google Scholar] [CrossRef]
- Ben Hamida, M.B.; Nguyen, L.M.Q.; Hajjar, A.; Izadi, M.; Sheremet, M.A.; Alrasheedi, N.H.; Hajlaoui, K. Role of applying PCMs on thermal behavior of innovative unit roof enclosure. J. Energy Storage 2024, 77, 109918. [Google Scholar] [CrossRef]
- Nguyen, L.M.Q.; Hajjar, A.; Izadi, M.; Sheremet, M.A.; Ben Hamida, M.B. Numerical study on thermal resistance of PCMs incorporated in novel roof structures against energy loss. J. Energy Storage 2023, 74, 109506. [Google Scholar] [CrossRef]
- Ben Hamida, M.B. Thermal management of square light emitting diode arrays: Modeling and parametric analysis. Multidiscip. Model. Mater. Struct. 2024, 20, 363–383. [Google Scholar] [CrossRef]
- Ben Salah, S.; Ben Hamida, M.B. Alternate PCM with air cavities in LED heat sink for transient thermal management. Int. J. Numer. Methods Heat Fluid Flow 2019, 29, 4377–4393. [Google Scholar] [CrossRef]
- Li, W.Q.; Qu, Z.G.; He, Y.L.; Tao, Y.B. Experimental study of a passive thermal management system for high-powered lithium ion batteries using porous metal foam saturated with phase change materials. J. Power Sources 2014, 255, 9–15. [Google Scholar] [CrossRef]
- Ye, Y.; Zhang, Z.Y.; Ma, Y.W.; Zhang, Y.Y.; Liu, J.J.; Yan, K.; Cheng, H.H. Performance optimization of hydrogen storage reactors based on PCM coupled with heat transfer fins or metal foams. Int. J. Hydrogen Energy 2024, 92, 392–400. [Google Scholar] [CrossRef]
- Izadi, M.; Fagehi, H.; Imanzadeh, A.; Altnji, S.; Bechir Ben Hamida, M.; Sheremet, M.A. Influence of finned charges on melting process performance in a thermal energy storage. Therm. Sci. Eng. Prog. 2023, 37, 101547. [Google Scholar] [CrossRef]
- Afaynou, I.; Faraji, H.; Choukairy, K.; Khallaki, K.; Akrour, D. Effectiveness of a PCM-based heat sink with partially filled metal foam for thermal management of electronics. Int. J. Heat Mass Transf. 2024, 235, 126196. [Google Scholar] [CrossRef]
- Sheikh, Y.; Hamdan, M.O.; Sakhi, S. A review on micro-encapsulated phase change materials (EPCM) used for thermal management and energy storage systems: Fundamentals, materials, synthesis and applications. J. Energy Storage 2023, 72, 108472. [Google Scholar] [CrossRef]
- Yang, Y.; Chen, L.; Yang, L.; Du, X. Numerical study of combined air and phase change cooling for lithium-ion battery during dynamic cycles. Int. J. Therm. Sci. 2021, 165, 106968. [Google Scholar] [CrossRef]
- Yu, X.K.; Tao, Y.B.; Deng, Q.Q. Experimental study on thermal management of batteries based on the coupling of metal foam-paraffin composite phase change materials and air cooling. J. Energy Storage 2024, 84, 110891. [Google Scholar] [CrossRef]
- Yang, H.; Li, M.; Wang, Z.; Ma, B. A compact and lightweight hybrid liquid cooling system coupling with Z-type cold plates and PCM composite for battery thermal management. Energy 2023, 263, 126026. [Google Scholar] [CrossRef]
- Kang, Z.; Wang, X.; Yin, R.; Xu, L.; Wu, J.; Wang, S.; Peng, Q. Optimizing of coupled phase change materials and liquid cooling thermal management for Li-ion battery pack. Appl. Therm. Eng. 2025, 273, 126508. [Google Scholar] [CrossRef]
- Kim, S.; Stavins, R.A.; Shoham, E.; Ziskind, G.; Miljkovic, N.; King, W.P. High power transient thermal management with dynamic phase change material and liquid cooling. Int. J. Heat Mass Transf. 2025, 246, 126998. [Google Scholar] [CrossRef]
- Yang, W.; Zhou, F.; Zhou, H.; Wang, Q.; Kong, J. Thermal performance of cylindrical lithium-ion battery thermal management system integrated with mini-channel liquid cooling and air cooling. Appl. Therm. Eng. 2020, 175, 115331. [Google Scholar] [CrossRef]
- Zhang, Y.; Zuo, W.; E, J.; Li, J.; Li, Q.; Sun, K.; Zhou, K.; Zhang, G. Performance comparison between straight channel cold plate and inclined channel cold plate for thermal management of a prismatic LiFePO4 battery. Energy 2022, 248, 123637. [Google Scholar] [CrossRef]
- Chen, Y.; Chen, K.; Dong, Y.; Wu, X. Bidirectional symmetrical parallel mini-channel cold plate for energy efficient cooling of large battery packs. Energy 2022, 242, 122553. [Google Scholar] [CrossRef]
- Guo, R.; Li, L. Heat dissipation analysis and optimization of lithium-ion batteries with a novel parallel-spiral serpentine channel liquid cooling plate. Int. J. Heat Mass Transf. 2022, 189, 122706. [Google Scholar] [CrossRef]
- Zuo, W.; Zhang, Y.; E, J.; Li, J.; Li, Q.; Zhang, G. Performance comparison between single S-channel and double S-channel cold plate for thermal management of a prismatic LiFePO4 battery. Renew. Energy 2022, 192, 46–57. [Google Scholar] [CrossRef]
- Chen, X.; Su, Y.; Zhang, Y.; Shen, J.; Xu, X.; Wang, X.; Zhou, F. Performance of thermal management system based on PCM/forked liquid-cold plate for 18650 cylindrical battery. J. Energy Storage 2024, 91, 112071. [Google Scholar] [CrossRef]
- Fan, Y.; Wang, Z.; Fu, T.; Wu, H. Numerical investigation on lithium-ion battery thermal management utilizing a novel tree-like channel liquid cooling plate exchanger. Int. J. Heat Mass Transf. 2022, 183, 122143. [Google Scholar] [CrossRef]
- Zhao, D.; Lei, Z.; An, C. Research on battery thermal management system based on liquid cooling plate with honeycomb-like flow channel. Appl. Therm. Eng. 2023, 218, 119324. [Google Scholar] [CrossRef]
- Fan, X.; Meng, C.; Yang, Y.; Lin, J.; Li, W.; Zhao, Y.; Xie, S.; Jiang, C. Numerical optimization of the cooling effect of a bionic fishbone channel liquid cooling plate for a large prismatic lithium-ion battery pack with high discharge rate. J. Energy Storage 2023, 72, 108239. [Google Scholar] [CrossRef]
- Borrvall, T.; Petersson, J. Topology optimization of fluids in Stokes flow. Int. J. Numer. Methods Fluids 2003, 41, 77–107. [Google Scholar] [CrossRef]
- Fawaz, A.; Hua, Y.; Le Corre, S.; Fan, Y.; Luo, L. Topology optimization of heat exchangers: A review. Energy 2022, 252, 124053. [Google Scholar] [CrossRef]
- Lurie, S.A.; Rabinskiy, L.N.; Solyaev, Y.O. Topology optimization of the wick geometry in a flat plate heat pipe. Int. J. Heat Mass Transf. 2019, 128, 239–247. [Google Scholar] [CrossRef]
- Pietropaoli, M.; Montomoli, F.; Gaymann, A. Three-dimensional fluid topology optimization for heat transfer. Struct. Multidiscip. Optim. 2019, 59, 801–812. [Google Scholar] [CrossRef]
- Li, H.; Kondoh, T.; Jolivet, P.; Furuta, K.; Yamada, T.; Zhu, B.; Zhang, H.; Izui, K.; Nishiwaki, S. Optimum design and thermal modeling for 2D and 3D natural convection problems incorporating level set-based topology optimization with body-fitted mesh. Int. J. Numer. Methods Eng. 2022, 123, 1954–1990. [Google Scholar] [CrossRef]
- Subramaniam, V.; Dbouk, T.; Harion, J.L. Topology optimization of conjugate heat transfer systems: A competition between heat transfer enhancement and pressure drop reduction. Int. J. Heat Fluid Flow 2019, 75, 165–184. [Google Scholar] [CrossRef]
- Li, H.; Ding, X.; Meng, F.; Jing, D.; Xiong, M. Optimal design and thermal modelling for liquid-cooled heat sink based on multi-objective topology optimization: An experimental and numerical study. Int. J. Heat Mass Transf. 2019, 144, 118638. [Google Scholar] [CrossRef]
- Kondoh, T.; Matsumori, T.; Kawamoto, A. Drag minimization and lift maximization in laminar flows via topology optimization employing simple objective function expressions based on body force integration. Struct. Multidiscip. Optim. 2012, 45, 693–701. [Google Scholar] [CrossRef]
- Ebadi, S.; Al-Jethelah, M.; Tasnim, S.H.; Mahmud, S. An investigation of the melting process of RT-35 filled circular thermal energy storage system. Open Physices 2018, 16, 574–580. [Google Scholar] [CrossRef]
- Moench, S.; Dittrich, R. Influence of Natural Convection and Volume Change on Numerical Simulation of Phase Change Materials for Latent Heat Storage. Energies 2022, 15, 2746. [Google Scholar] [CrossRef]
- Monika, K.; Chakraborty, C.; Roy, S.; Dinda, S.; Singh, S.A.; Datta, S.P. Parametric investigation to optimize the thermal management of pouch type lithium-ion batteries with mini-channel cold plates. Int. J. Heat Mass Transf. 2021, 164, 120568. [Google Scholar] [CrossRef]
- Guo, C.; Liu, H.-L.; Guo, Q.; Shao, X.-D.; Zhu, M.-L. Investigations on a novel cold plate achieved by topology optimization for lithium-ion batteries. Energy 2022, 261, 125097. [Google Scholar] [CrossRef]
- Wei, L.-S.; Liu, H.-L.; Tang, C.-G.; Tang, X.-P.; Shao, X.-D.; Gongnan, X. Investigation of novel type of cylindrical lithium-ion battery heat exchangers based on topology optimization. Energy 2024, 304, 131886. [Google Scholar] [CrossRef]





| Boundary Conditions | Parameters | Values |
|---|---|---|
| Length (mm) | L | 15 |
| Projection slope | β | 8 |
| Projection point | θβ | 0.5 |
| Penalty coefficient | q | 0.01 |
| Inlet temperature | Tin | 0 |
| Inlet pressure (Pa) | Pin | 3.33 |
| Volume constraint | Vf | 0.5 |
| Weight factor | W | 0.5 |
| Thermophysical Properties | Paraffin (PCM) | Aluminum | Water |
|---|---|---|---|
| Solid density ρs/(kg·m−3) | 800 | 2700 | - |
| Liquid density ρl/(kg·m−3) | 850 | - | 998.2 |
| Specify heat at constant pressure Cp/(kJ·kg·K−1) | 2000 | 900 | 4182 |
| Thermal conductivity k/(W·m−1·K−1) | 0.2 | 238 | 0.6 |
| Latent heat Lh/(kJ·kg−1) | 220 | - | - |
| Phase change temperature T/°C | 37 | - | - |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xia, X.; Luo, J.; Li, J.; Wei, L. Topology Optimization Design of Phase Change Liquid Cooling Composite Plate. Energies 2025, 18, 2652. https://doi.org/10.3390/en18102652
Xia X, Luo J, Li J, Wei L. Topology Optimization Design of Phase Change Liquid Cooling Composite Plate. Energies. 2025; 18(10):2652. https://doi.org/10.3390/en18102652
Chicago/Turabian StyleXia, Xinqiang, Jiancheng Luo, Jiabao Li, and Lixia Wei. 2025. "Topology Optimization Design of Phase Change Liquid Cooling Composite Plate" Energies 18, no. 10: 2652. https://doi.org/10.3390/en18102652
APA StyleXia, X., Luo, J., Li, J., & Wei, L. (2025). Topology Optimization Design of Phase Change Liquid Cooling Composite Plate. Energies, 18(10), 2652. https://doi.org/10.3390/en18102652





