Research on Surface Charge Migration Characteristics of Two-Layered Polymer Film Based on Bipolar Charge Transport Model
Abstract
1. Introduction
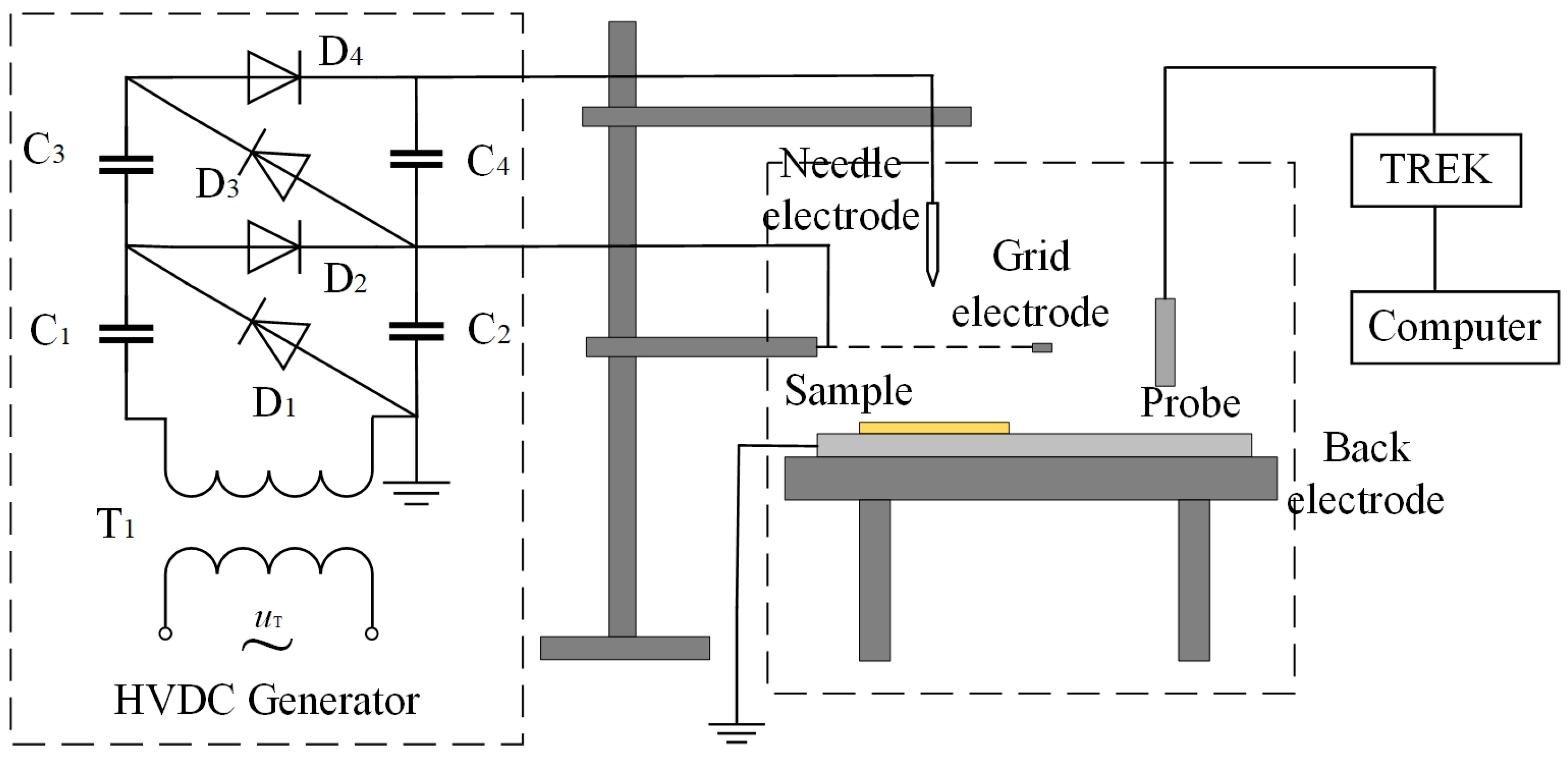
2. Mathematical Model
3. Results and Discussion
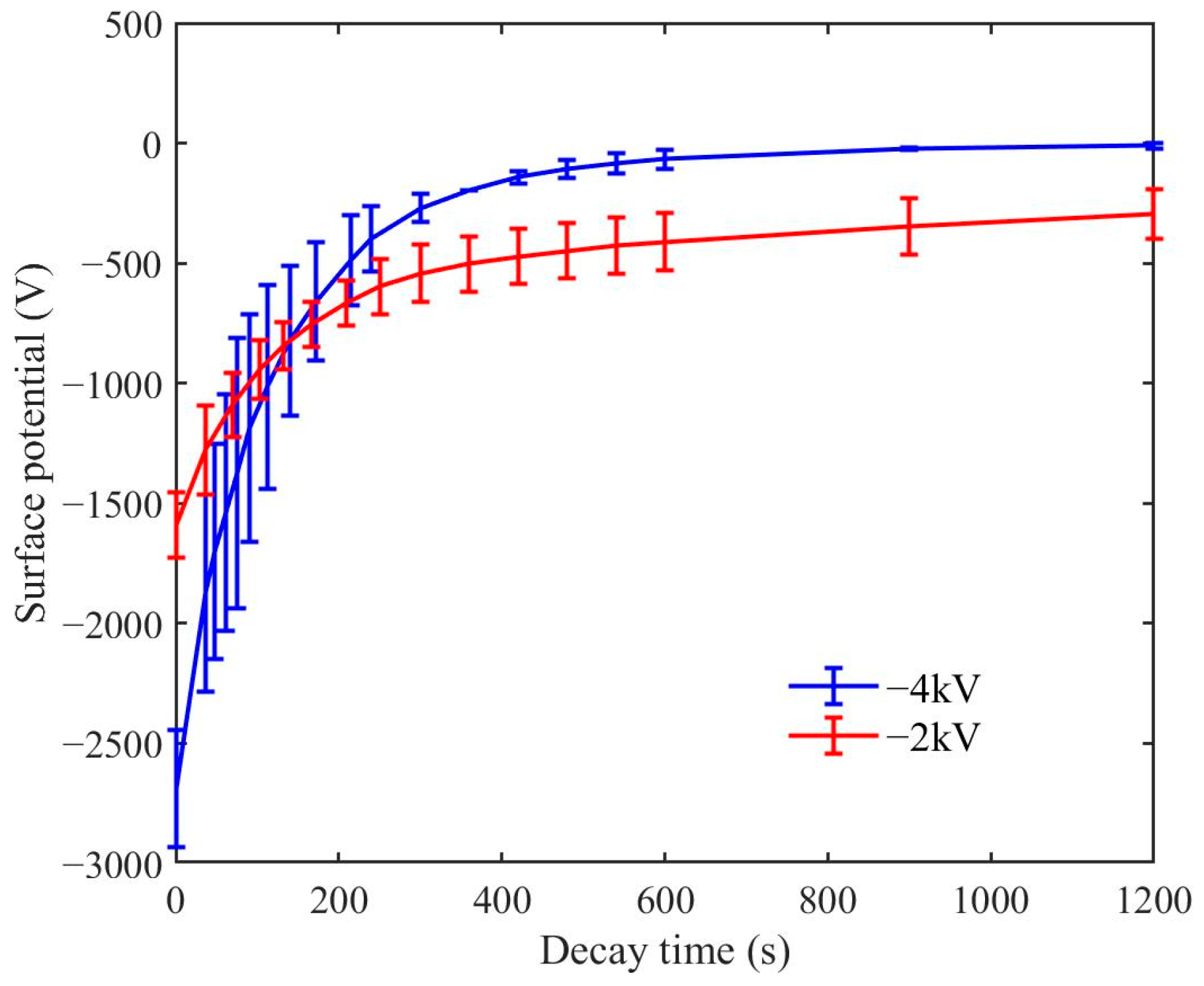
3.1. Benchmark
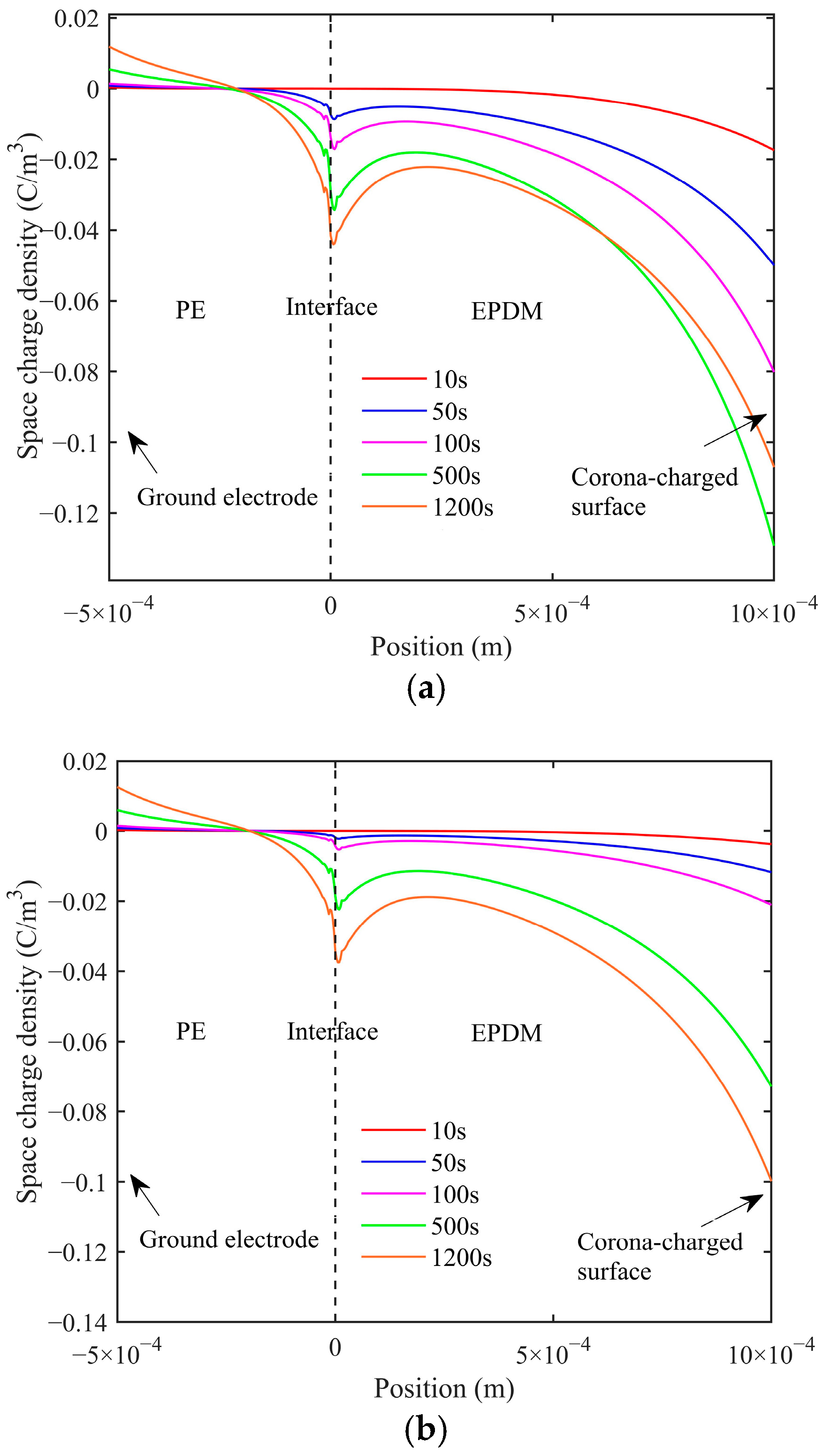
3.2. Effect of Injection on SPD Characteristics
3.3. Effect of Trap on SPD Characteristics
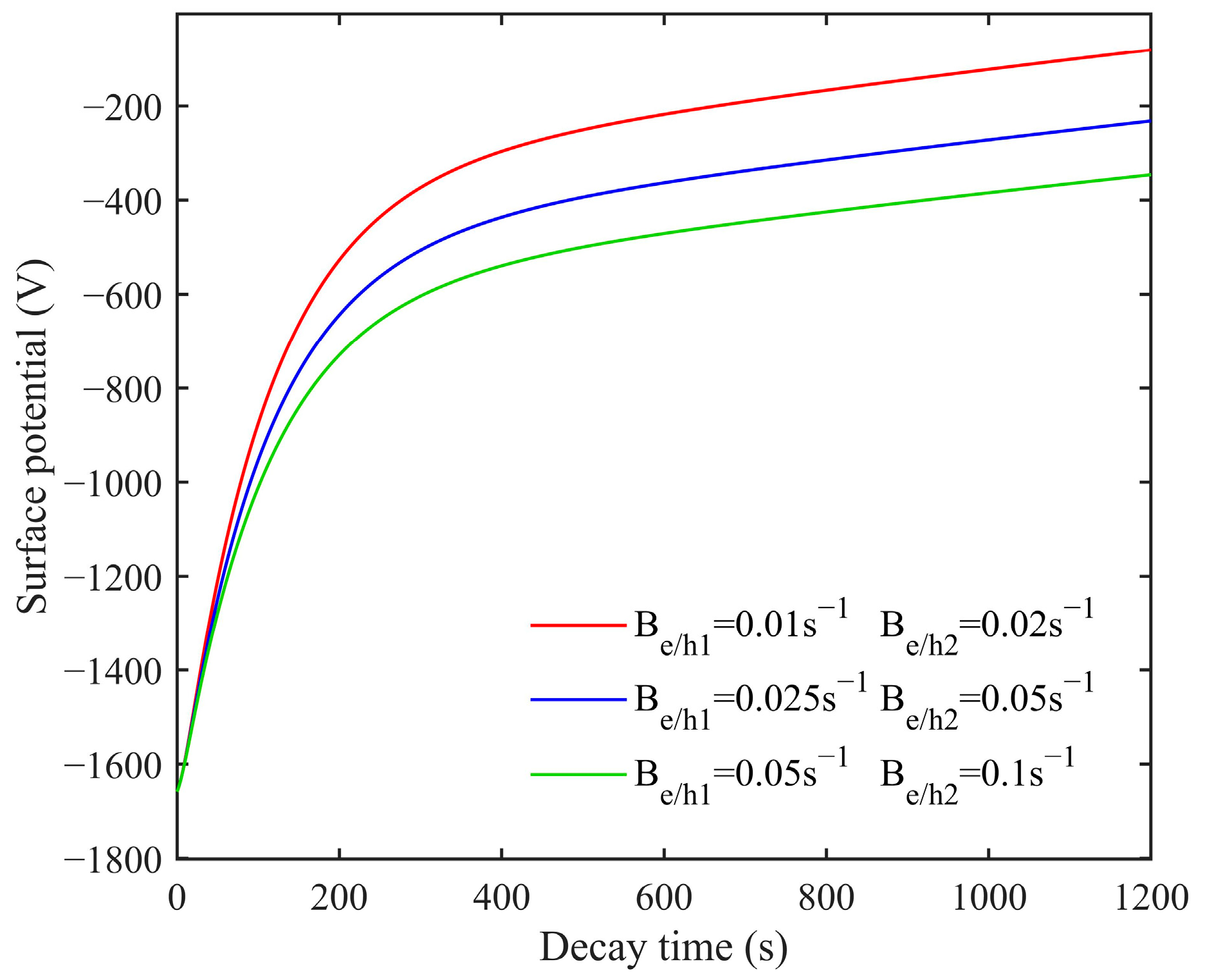
3.3.1. Trapping Coefficient
3.3.2. Trap Depth
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Li, Z.; Du, B. Polymeric insulation for HVDC extruded cables: Challenges and development directions. IEEE Electr. Insul. Mag. 2018, 34, 30–43. [Google Scholar] [CrossRef]
- Liu, Y.; Cai, X.; Cai, S. Study on space charge accumulation characteristics of 320 kV cross-linked polyethylene cable joints. Polym. Sci. Ser. A 2022, 64, 415–423. [Google Scholar] [CrossRef]
- Wang, Y.; Shuai, S.; Sun, Y.; Yang, X.; Liu, C. Effect of nano-MgO doping in XLPE on charge transport and electric field distribution in composite insulation of HVDC cable joint. Energies 2022, 15, 6948. [Google Scholar] [CrossRef]
- Wang, Y.; Wang, Y.; Yang, X.; Ma, A.; Sun, Y.; Yin, Y. Charge transport in full-size HVDC cable joint with modeling of XLPE/EPDM interface. IEEE Trans. Dielectr. Electr. Insul. 2021, 28, 2117–2125. [Google Scholar] [CrossRef]
- Han, Y.; Li, S.; Min, D. Trap energy distribution in polymeric insulating materials through surface potential decay method. IEEE Trans. Dielectr. Electr. Insul. 2018, 25, 639–648. [Google Scholar] [CrossRef]
- Du, B.; Li, X.; Jiang, J. Surface charge accumulation and decay on direct-fluorinated oil-impregnated paper. IEEE Trans. Dielectr. Electr. Insul. 2016, 23, 3094–3101. [Google Scholar] [CrossRef]
- Du, B.; Li, Z.; Li, J. Effects of direct fluorination on space charge accumulation in HTV silicone rubber. IEEE Trans. Dielectr. Electr. Insul. 2016, 23, 2353–2360. [Google Scholar] [CrossRef]
- Du, B.; Li, J.; Du, W. Surface charge accumulation and decay on direct fluorinated polyimide/Al2O3 nanocomposites. IEEE Trans. Dielectr. Electr. Insul. 2013, 20, 1764–1771. [Google Scholar] [CrossRef]
- Li, G.; Gu, Z.; Xing, Z.; Zhang, C.; Guo, S.; Hao, C.; Lei, Q. Space charge and trap distributions and charge dynamic migration characteristics in polypropylene under strong electric field. ECS J. Solid State Sci. Technol. 2022, 11, 083003. [Google Scholar] [CrossRef]
- Min, D.; Cho, M.; Khan, A.; Li, S. Surface and volume charge transport properties of polyimide revealed by surface potential decay with genetic algorithm. IEEE Trans. Dielectr. Electr. Insul. 2012, 19, 600–608. [Google Scholar] [CrossRef]
- Li, Z.; Yang, Z.; Du, B. Surface charge transport characteristics of ZnO/silicone rubber composites under impulse superimposed on DC voltage. IEEE Access 2018, 7, 3008–3017. [Google Scholar] [CrossRef]
- Wang, Y.; Wang, Y.; Yang, X. Interfacial charge dynamics of XLPE/EPDM double layers by simultaneous measurement of space charge and relaxation current. IEEE Trans. Dielectr. Electr. Insul. 2021, 28, 569–577. [Google Scholar] [CrossRef]
- Qi, B.; Yang, Z.; Yang, X.; Luo, Y.; Huang, M.; Gao, C.; Zhang, Y.; Li, C. A simplified method to estimate surface trap distribution: Potential decay by charge dissipation along surface. IEEE Trans. Dielectr. Electr. Insul. 2023, 30, 928–935. [Google Scholar] [CrossRef]
- Wang, F.; Wang, L.; Chen, S.; Zhong, L.; Sun, Q. Charge carriers on insulating surfaces: An experimental study. IEEE Trans. Dielectr. Electr. Insul. 2023, 30, 2665–2672. [Google Scholar] [CrossRef]
- Gödrich, S.; Schmidt, H.; Papastavrou, G. Stability of charge distributions in electret films on the nm-scale. ACS Appl. Mater. Interf. 2022, 14, 4500–4509. [Google Scholar] [CrossRef] [PubMed]
- Rabiee, S.; Sohrabi, M.; Afarideh, H. Electrostatic surface potential stability of corona charged PTFE electret films by some parametric optimization for radiation protection dosimetry. J. Electrostat. 2025, 133, 104013. [Google Scholar] [CrossRef]
- Huang, M.; Zhou, Y.; Chen, W.; Lu, L.; Jin, F.; Huang, J. Space charge dynamics at the physical interface in oil-paper insulation under DC voltage. IEEE Trans. Dielectr. Electr. Insul. 2015, 22, 1739–1746. [Google Scholar] [CrossRef]
- Qin, Y.; Ge, Y.; Zhao, J.; He, Y.; Lv, Z.; Wu, K.; Zhao, Y.; Zhou, Y. Characteristics of charge accumulation at the interface of double-Layer XLPE under DC voltage. IEEE Trans. Dielectr. Electr. Insul. 2025, 32, 707–716. [Google Scholar] [CrossRef]
- Das, S.; Gupta, N. Effect of ageing on space charge distribution in homogeneous and composite dielectrics. IEEE Trans. Dielectr. Electr. Insul. 2015, 22, 541–547. [Google Scholar] [CrossRef]
- Perrin, C.; Griseri, V.; Laurent, C. Measurement of internal charge distribution in dielectrics using the pulsed electro-acoustic method in non contact mode. IEEE Trans. Dielectr. Electr. Insul. 2008, 15, 958–964. [Google Scholar] [CrossRef]
- Rychkov, D.; Gerhard, R. Stabilization of positive charge on polytetrafluoroethylene electret films treated with titanium-tetrachloride vapor. Appl. Phys. Lett. 2011, 98, 139–312. [Google Scholar] [CrossRef]
- Chen, G.; Xu, Z.; Zhang, L. Measurement of the surface potential decay of corona-charged polymer films using the pulsed electroacoustic method. Meas. Sci. Technol. 2007, 18, 1453–1458. [Google Scholar] [CrossRef]
- Zhang, L.; Xu, Z.; Chen, G. Decay of electric charge on corona charged polyethylene. J. Phys. D Appl. Phys. 2007, 40, 7085–7089. [Google Scholar] [CrossRef]
- Liu, Y.; Cai, X.; Cai, S. Analysis of influence factors on space charge accumulation characteristics of 320 kV XLPE cable. Polym. Sci. 2022, 64, 415–423. [Google Scholar]
- Lan, L.; Wu, J.; Yin, Y.; Zhong, Q. Investigation on heterocharge accumulation in crosslinked polyethylene: Experiment and simulation. Jpn. J. Appl. Phys. 2014, 53, 071702. [Google Scholar] [CrossRef]
- Cai, X.; Wang, X.; Pang, D.; Zou, X.; Lu, Z. Investigation of current density, recombination rate and space charge density in polyethylene thin films based on bipolar charge transport model. Mater. Res. Express 2019, 6, 096451. [Google Scholar] [CrossRef]
- Baudoin, F.; Roy, S.; Teyssedre, G.; Laurent, C. Bipolar charge transport model with trapping and recombination: An analysis of the current versus applied electric field characteristic in steady state conditions. J. Phys. D Appl. Phys. 2008, 41, 025306. [Google Scholar] [CrossRef]
- Roy, S.; Segur, P.; Teyssedre, G.; Laurent, C. Description of bipolar charge transport in polyethylene using a fluid model with a constant mobility: Model prediction. J. Phys. D Appl. Phys. 2004, 37, 298–305. [Google Scholar] [CrossRef]
- Min, D.; Li, S. Simulation on the influence of bipolar charge injection and trapping on surface potential decay of polyethylene. IEEE Trans. Dielectr. Electr. Insul. 2014, 21, 1627–1636. [Google Scholar] [CrossRef]
- Li, J.; Liang, H.; Du, B.; Song, P.; Kong, X.; Li, Z. Numerical simulation of interface charge behaviors between LDPE/EPDM for HVDC cable accessory insulation based on the bipolar charge transport model. High Vol. Eng. 2018, 44, 1443–1449. [Google Scholar]
- Li, J.; Du, B.; Xing, Y. Trap distribution and flashover characteristics of Carbon-Black-Filled EPDM in low temperature. IEEE Trans. Appl. Supercond. 2016, 26, 0610305. [Google Scholar] [CrossRef]
- Zhong, X.; Zheng, Y.; Dang, B.; Miao, X.; He, J. Polarity effect on space charge distributions in polymer under DC electric field. Proc. CSEE 2016, 36, 6693–6701. [Google Scholar]
- Li, J.; Gao, Y.; Song, Z.; Peng, J.; Xu, X. On the crosslinking temperature dependence of charge transport behavior in XLPE film deduced from surface potential decay measurements. J. Electrostat. 2021, 109, 103539. [Google Scholar] [CrossRef]
- Li, Y.; Gao, B.; Li, X.; Yang, Y.; Xu, P.; Wang, Z. Evolution of trapping parameters of multi-layer oil-paper insulating medium at different partial discharge stages. High Voltage Eng. 2021, 47, 260–268. [Google Scholar]
- Tian, F.; Hou, C. A trap regulated space charge suppression model for LDPE based nanocomposites by simulation and experiment. IEEE Trans. Dielectr. Electr. Insul. 2018, 25, 2169–2177. [Google Scholar] [CrossRef]
- Anh, H.; Serdyuk, Y.; Gubanski, S. Charge transport in LDPE nanocomposites Part II—Computational approach. Polymers 2016, 8, 103. [Google Scholar] [CrossRef]
- Liu, Y.; Liu, H.; Yu, L.; Gao, L. Effect of thermal stress on the space charge distribution of 160 kV HVDC cable insulation material. IEEE Trans. Dielectr. Electr. Insul. 2017, 24, 1355–1364. [Google Scholar] [CrossRef]
- Li, H.; Li, J.; Ma, Y.; Yan, Q. Effects of thermal aging on the crystal structures of the XLPE cable insulating material at different temperatures. Proc. CSEE 2017, 37, 6740–6748. [Google Scholar]


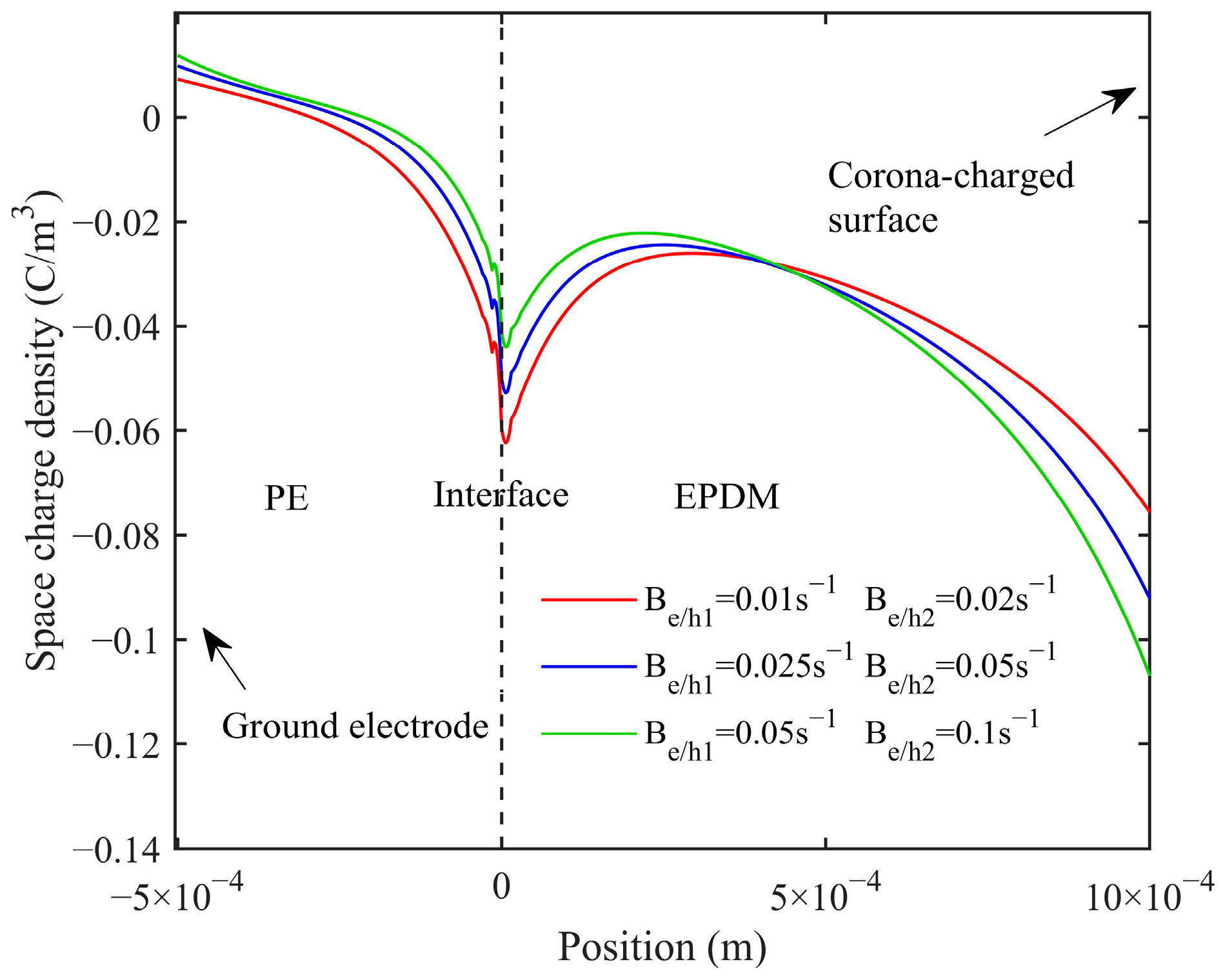
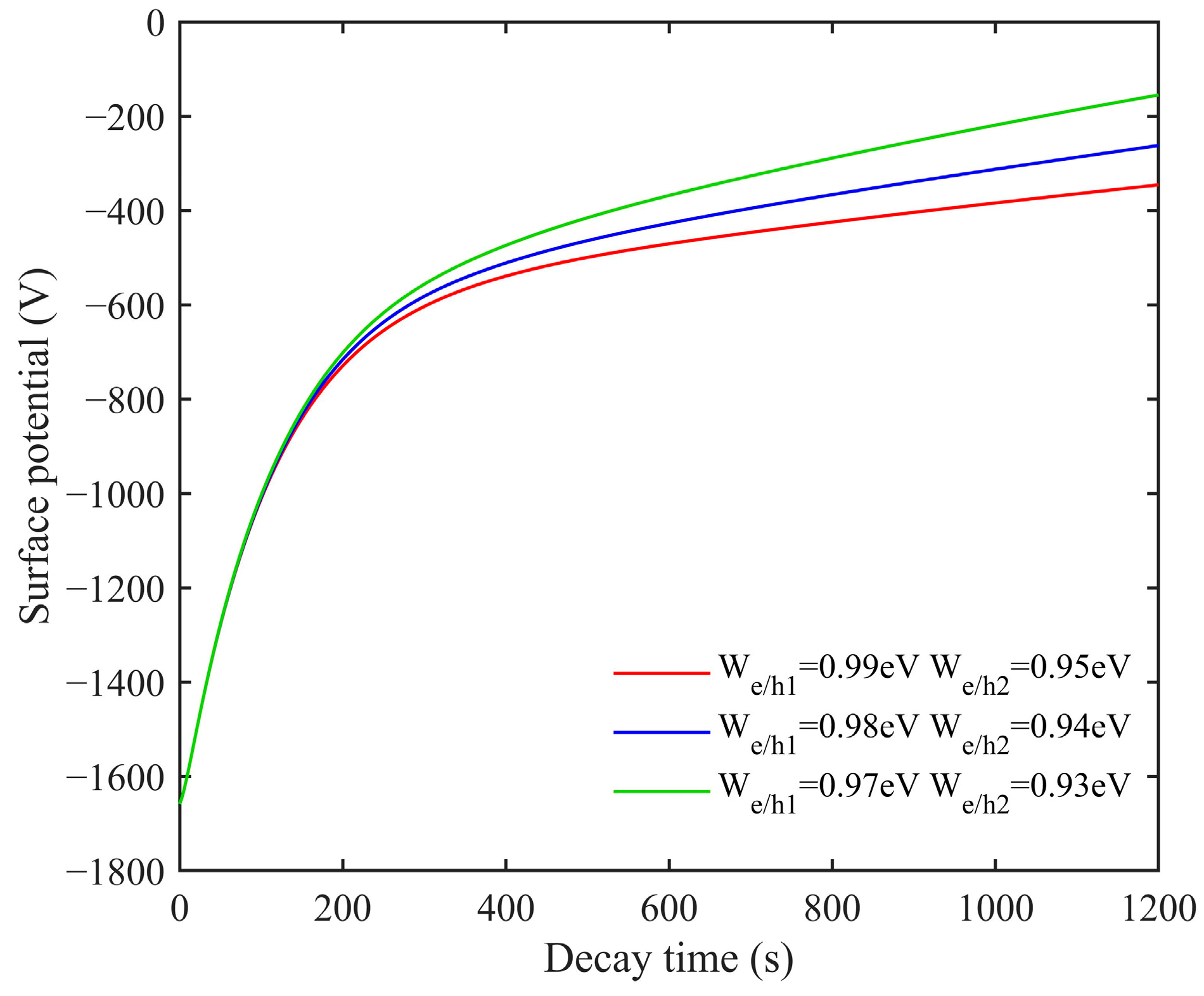
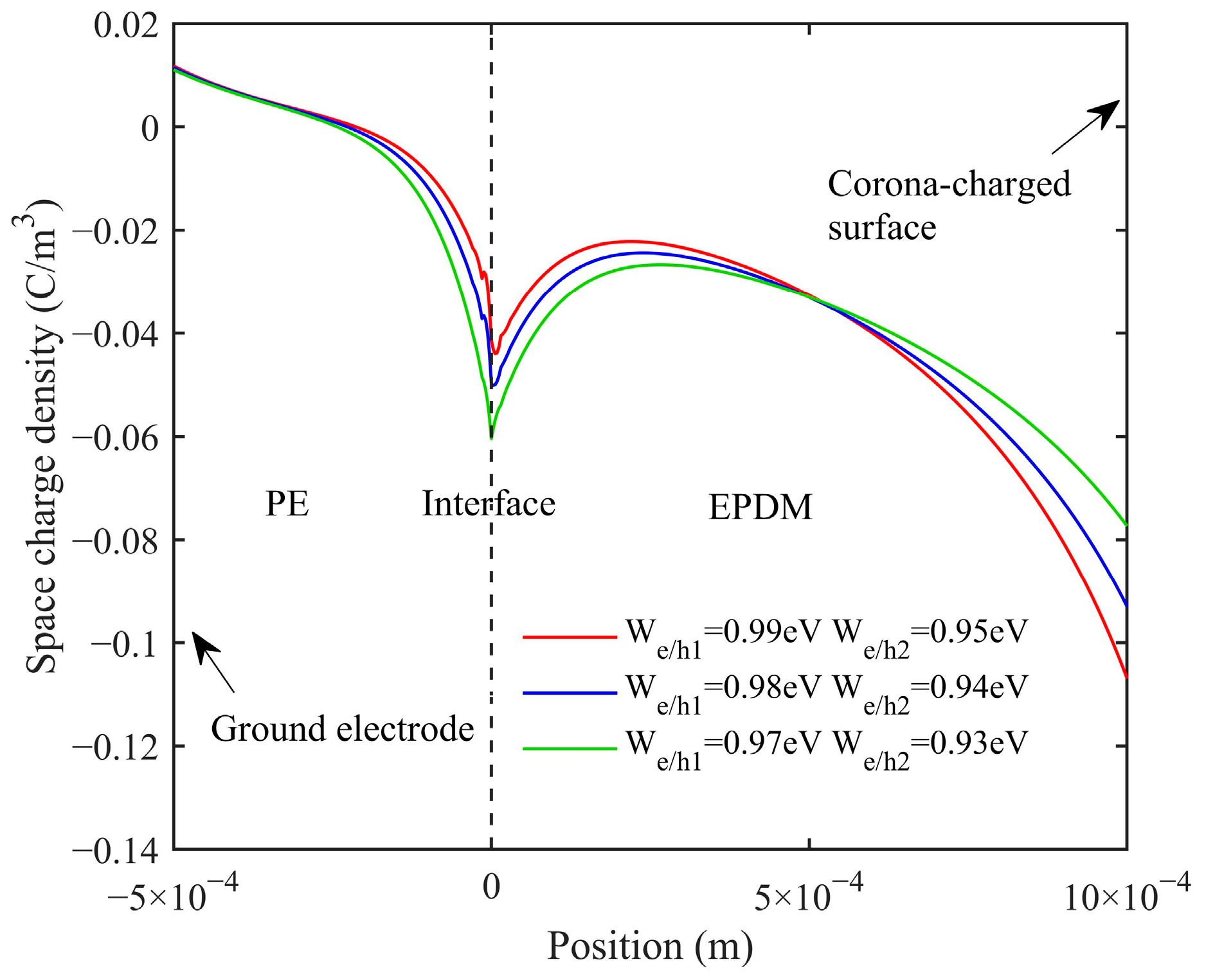
| Parameters | PE | EPDM | Unit |
|---|---|---|---|
| Injection barrier of corona-charged surface, wst | / | 0.93 [29] | eV |
| Injection barrier between polymer and grounded electrode, win | 1.3 [30] | / | eV |
| Trapping coefficient for electrons, Be | 0.05 [24] | 0.1 [30] | s−1 |
| Trapping coefficient for holes, Bh | 0.05 [24] | 0.1 [30] | s−1 |
| Trap depth for electrons, we | 0.99 [24] | 0.95 [30] | eV |
| Trap depth for holes, wh | 0.99 [24] | 0.95 [30] | eV |
| Trapped density for electrons, n0et | 100 [28] | 100 [30] | C/m3 |
| Trapped density for holes, n0ht | 100 [28] | 100 [30] | C/m3 |
| Mobility for electrons, μe | 7.5 × 10−14 [4] | 1.35 × 10−13 [4] | m2·V−1·s−1 |
| Mobility for holes, μh | 2.4 × 10−14 [4] | 1 × 10−13 [4] | m2·V−1·s−1 |
| Recombination coefficient, S0 S1 S2 | 6.4 × 10−22 [28] | 6.4 × 10−22 [28] | m3·s−1 |
| Recombination coefficient, S3 | 0 [28] | 0 [28] | m3·s−1 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, Y.; Cai, X. Research on Surface Charge Migration Characteristics of Two-Layered Polymer Film Based on Bipolar Charge Transport Model. Energies 2025, 18, 2552. https://doi.org/10.3390/en18102552
Liu Y, Cai X. Research on Surface Charge Migration Characteristics of Two-Layered Polymer Film Based on Bipolar Charge Transport Model. Energies. 2025; 18(10):2552. https://doi.org/10.3390/en18102552
Chicago/Turabian StyleLiu, Yuqi, and Xinjing Cai. 2025. "Research on Surface Charge Migration Characteristics of Two-Layered Polymer Film Based on Bipolar Charge Transport Model" Energies 18, no. 10: 2552. https://doi.org/10.3390/en18102552
APA StyleLiu, Y., & Cai, X. (2025). Research on Surface Charge Migration Characteristics of Two-Layered Polymer Film Based on Bipolar Charge Transport Model. Energies, 18(10), 2552. https://doi.org/10.3390/en18102552





