Enhanced Three-Phase Shunt Active Power Filter Utilizing an Adaptive Frequency Proportional-Integral–Resonant Controller and a Sensorless Voltage Method
Abstract
1. Introduction
2. Grid-Connected Inverter and PIR Control Approach
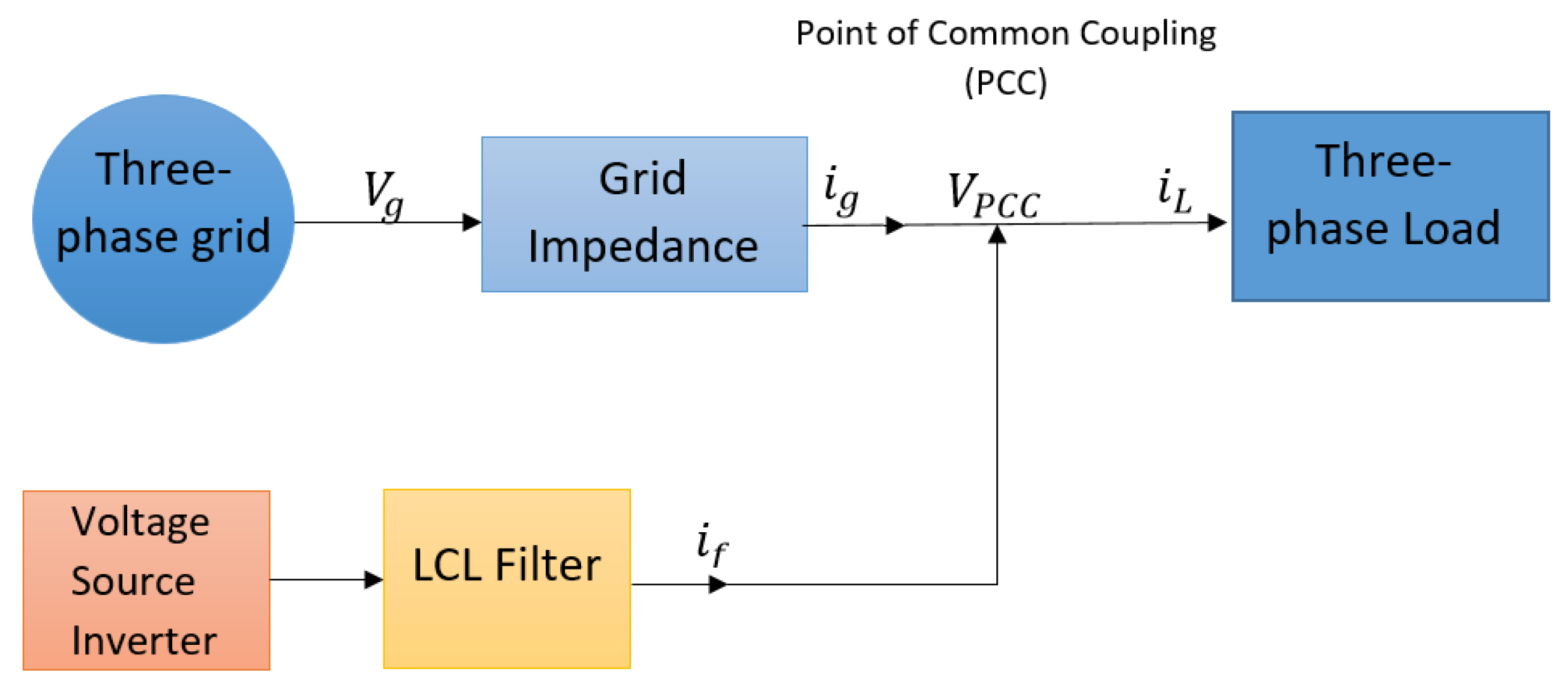
2.1. Design and Configuration of Shunt Active Power Filter
2.2. Symmetrical Components Extraction Method
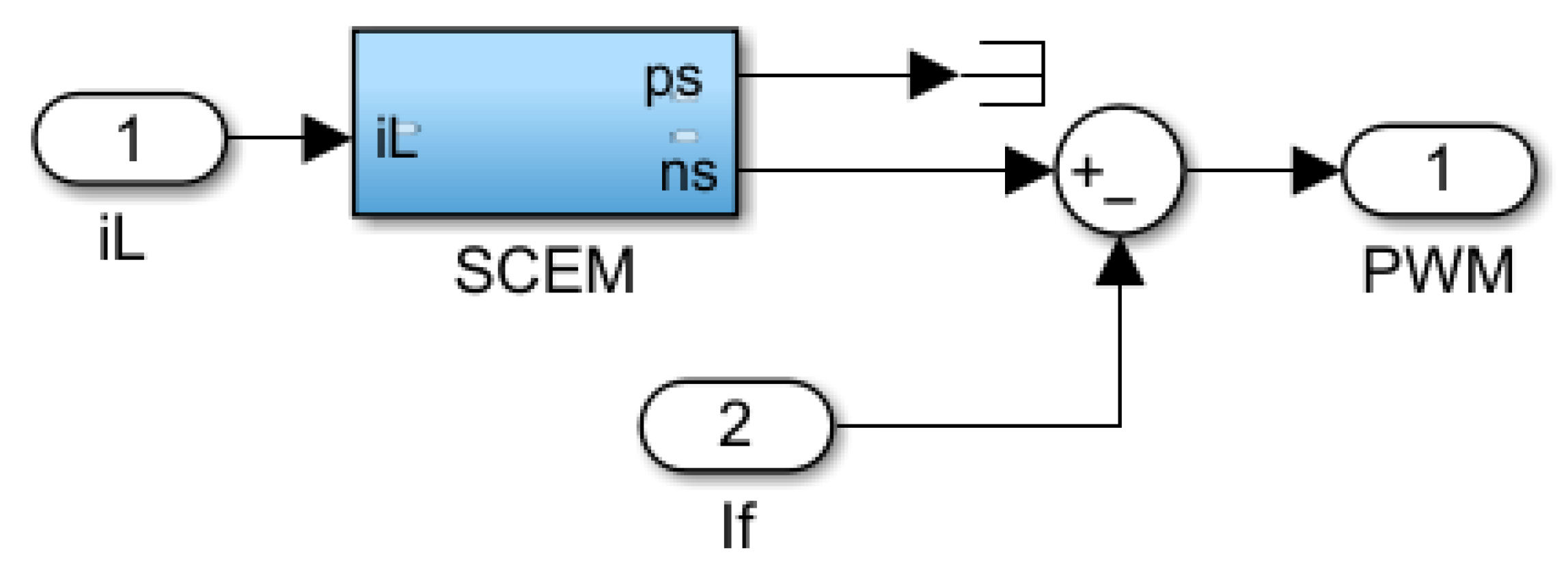
3. SAPF Method Using Only Current Measurements
3.1. SAPF Employing the PIR Controller
- The proportional gain () is configured to a relatively low value to ensure system stability while minimizing overshoot in the operation of the SAPF.
- The integral gain () is carefully selected to remain minimal. While increasing can effectively reduce steady-state errors, it may also lead to undesirable effects such as overshoot and oscillations, thereby impacting system performance.
- Achieving optimal control performance requires tuning the resonant gain () and adjusting the angular frequency () to align precisely with the grid frequency. However, excessive values of can compromise the system stability, necessitating careful calibration.
- Ultimately, the selection of PIR controller gains demands a meticulous balance between achieving sufficient gain and preserving the overall system stability and reliability.
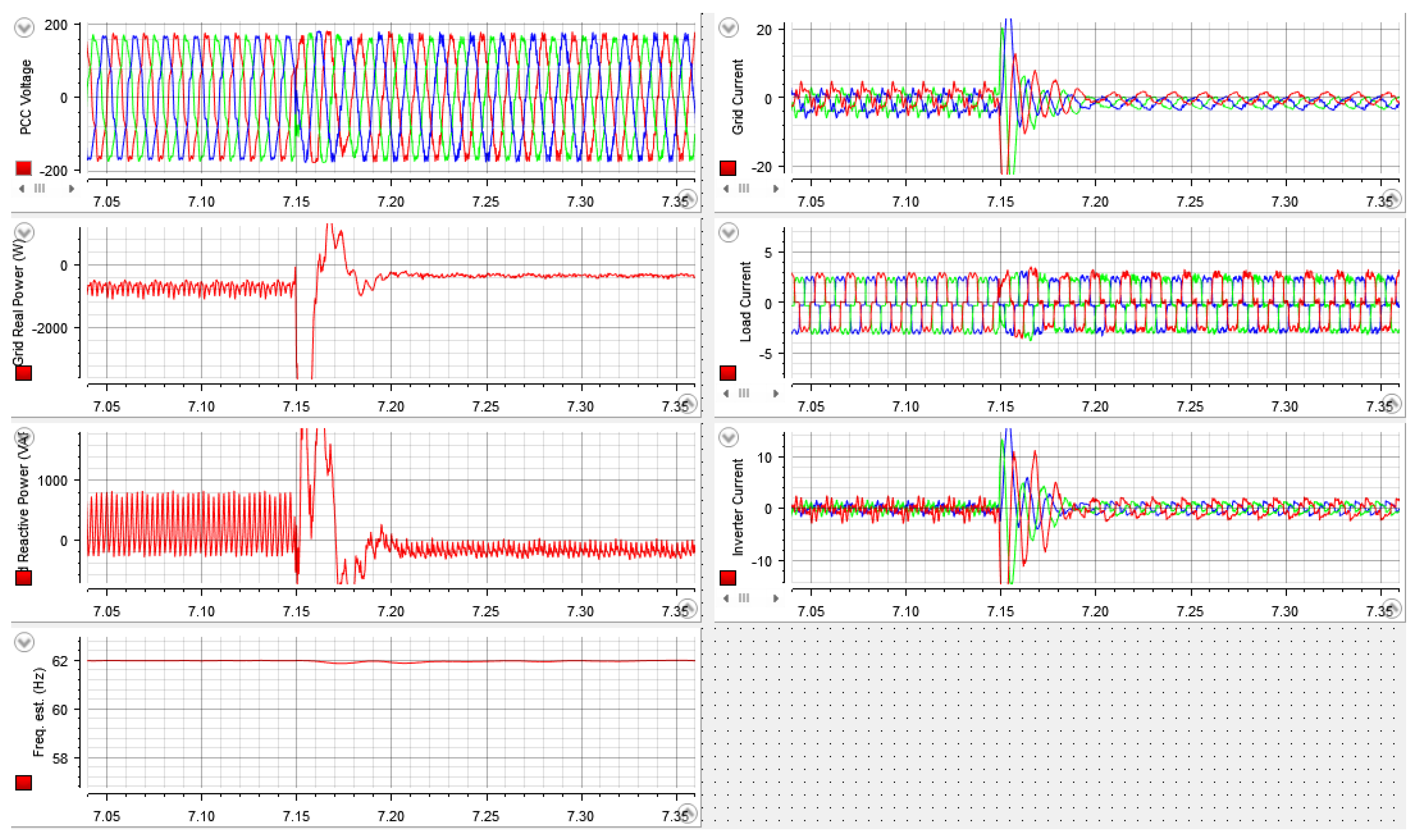
3.2. Challenges of SAPF Control Under Frequency Variation
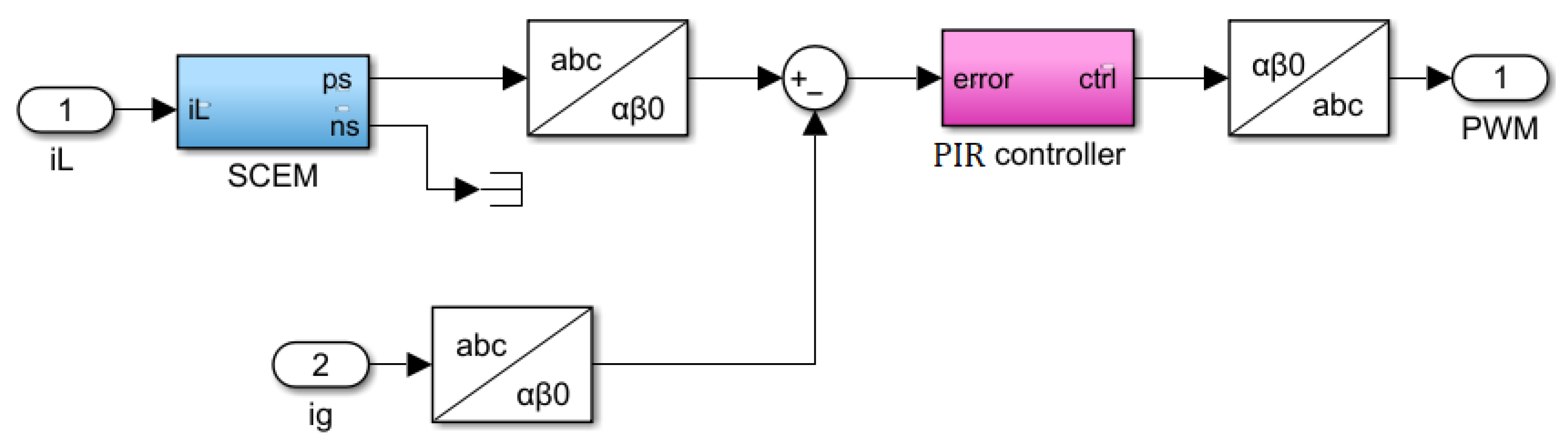
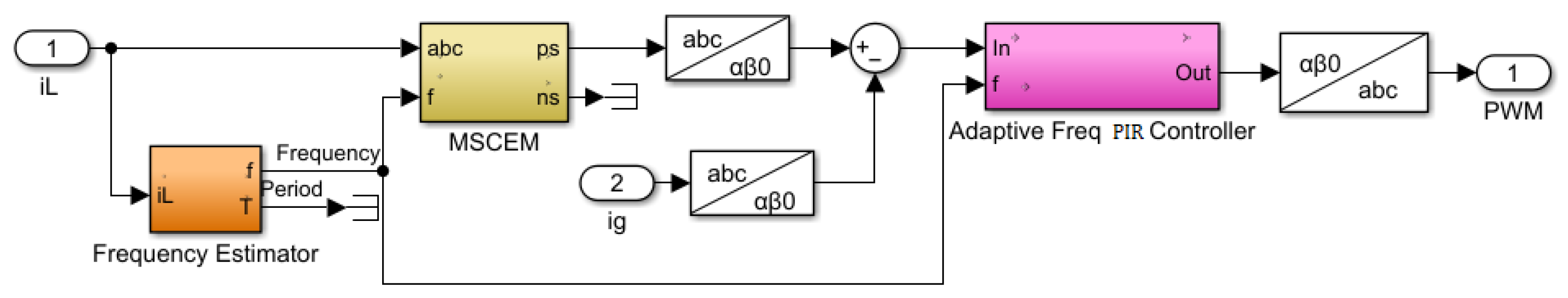
4. The Proposed Method
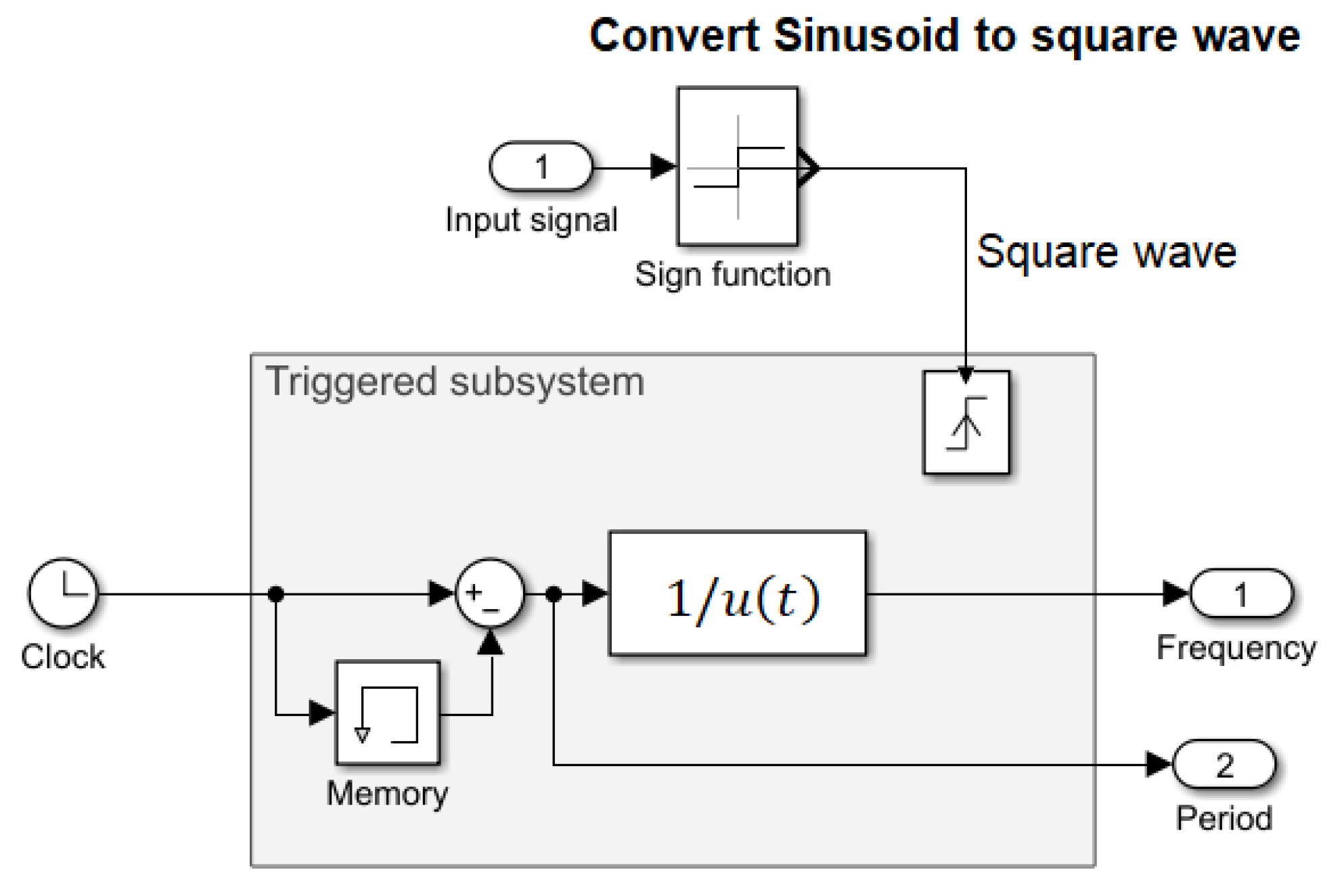
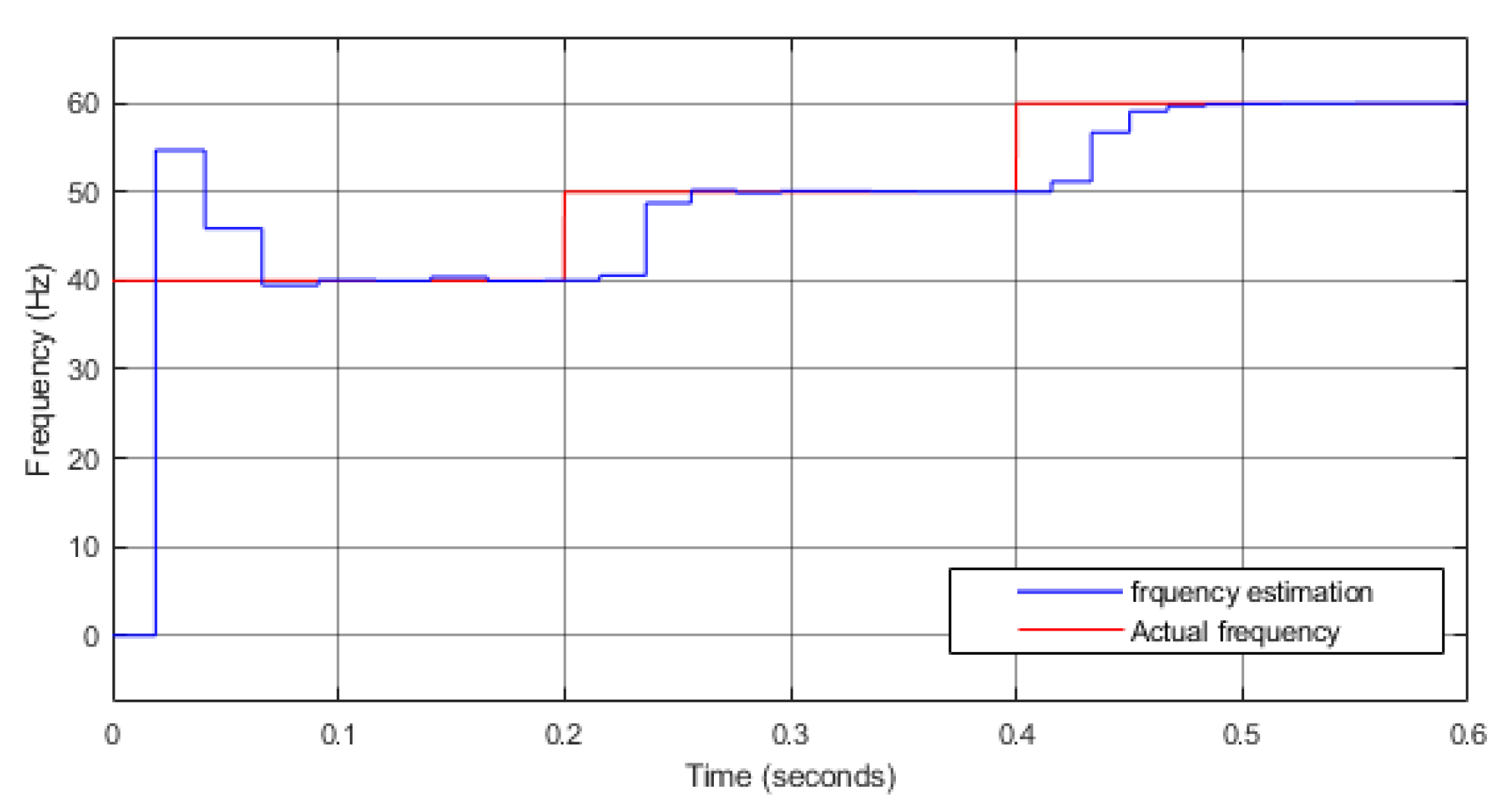
4.1. Frequency Estimation Technique
4.2. Enhanced Symmetrical Components Extraction Method
4.3. Frequency Adaptive Proportional-Integral-Resonant (PIR) Controller
4.4. The Integrated Control Approach
- Incorporating a frequency estimation mechanism,
- Enhancing the SCEM to account for variations in frequency,
- Using the estimated frequency, dynamically adjust the frequency parameter of the PIR controller.
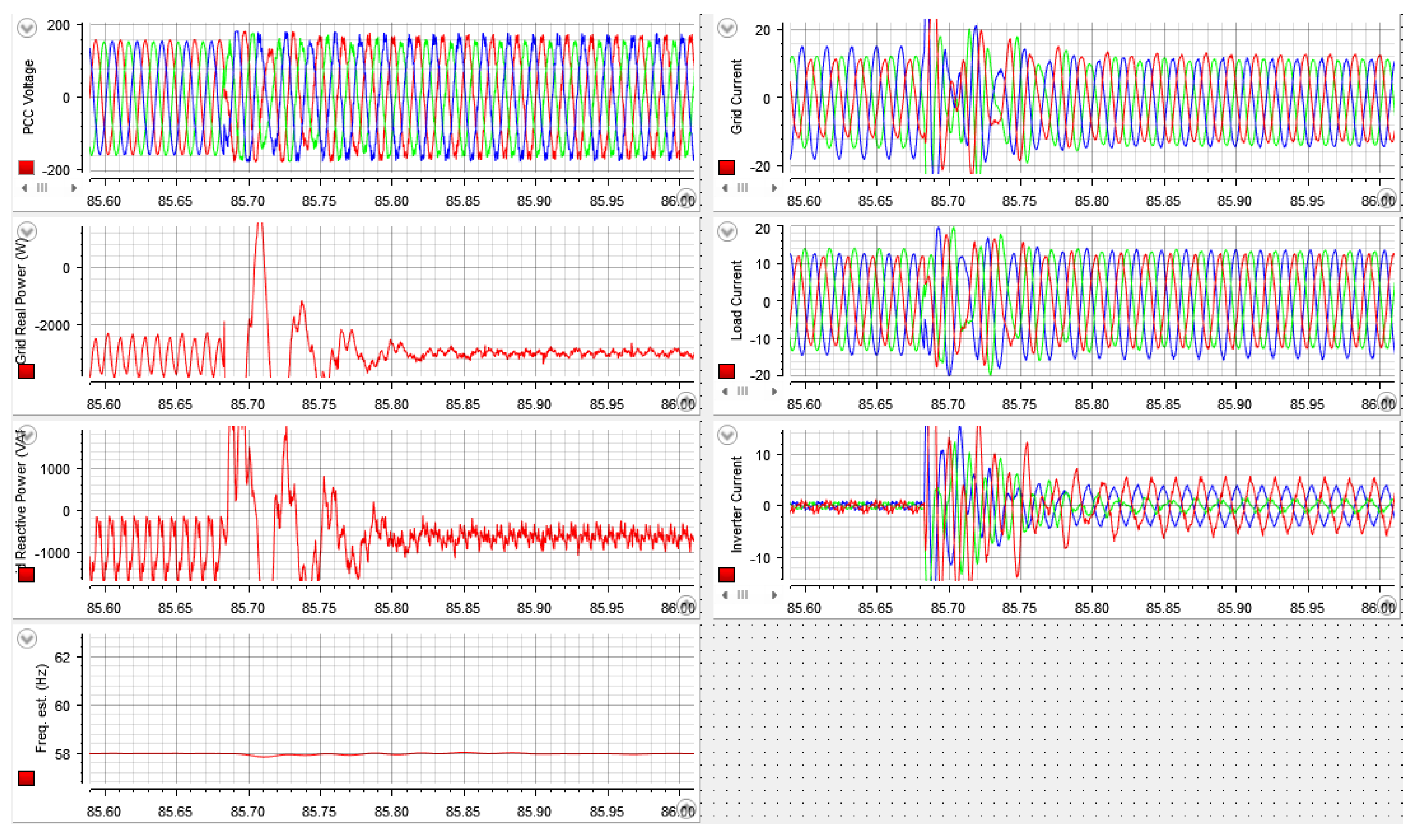
5. Case Studies
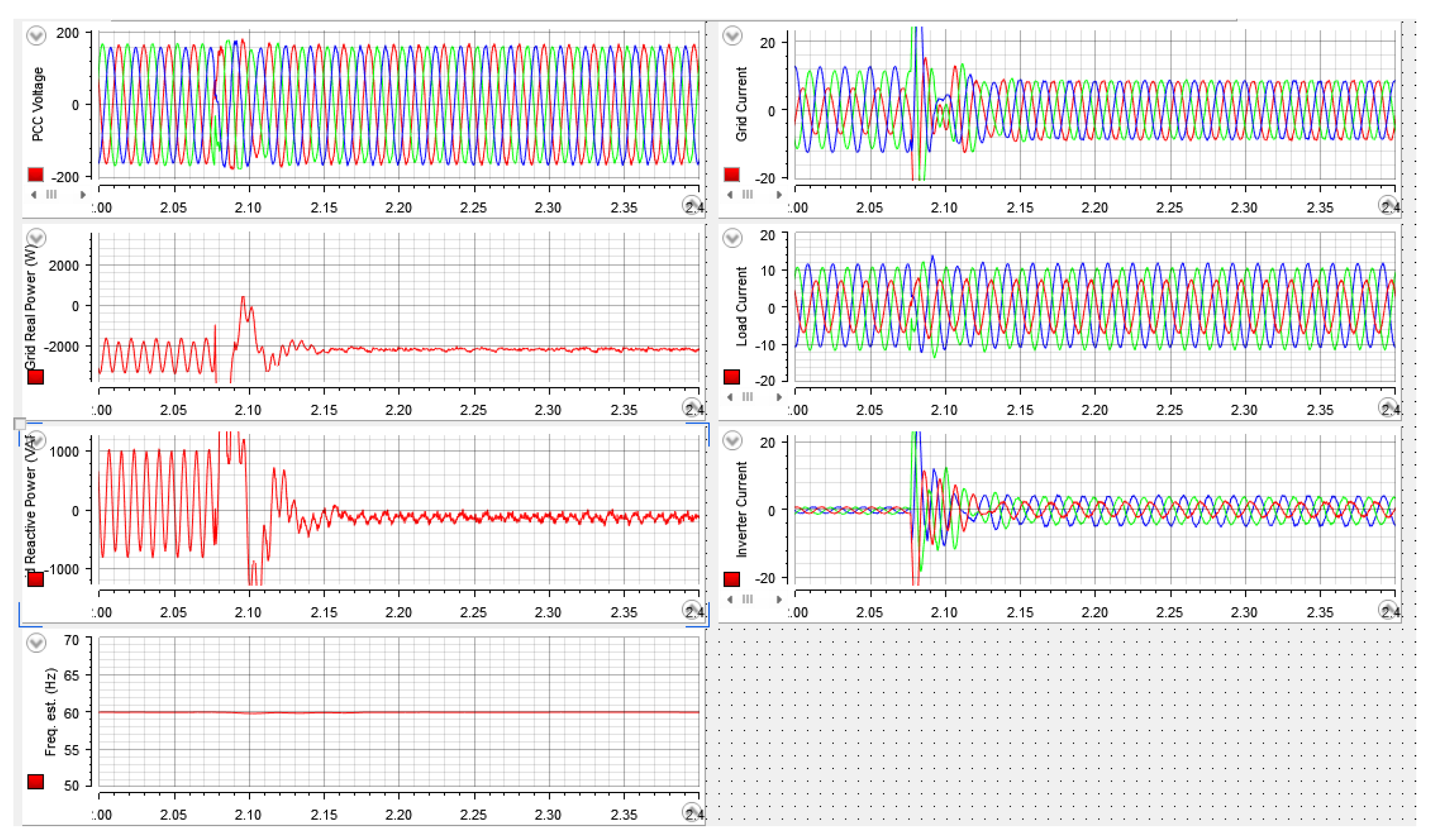
5.1. Simulation Results
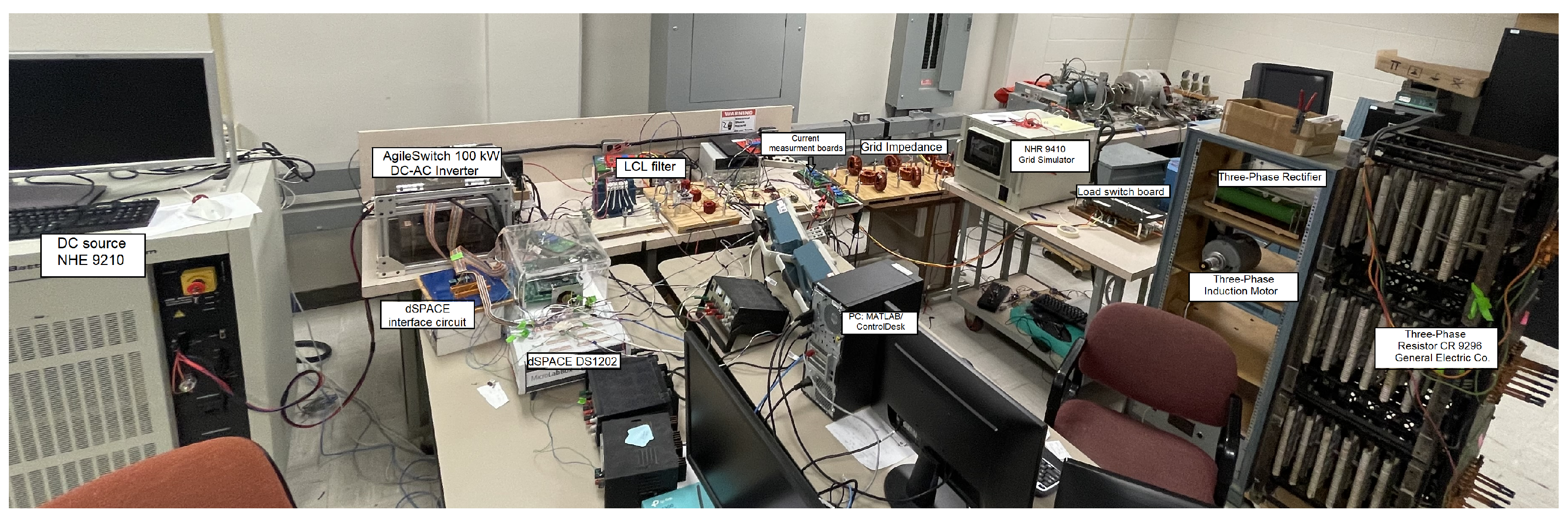
5.2. Hardware Results
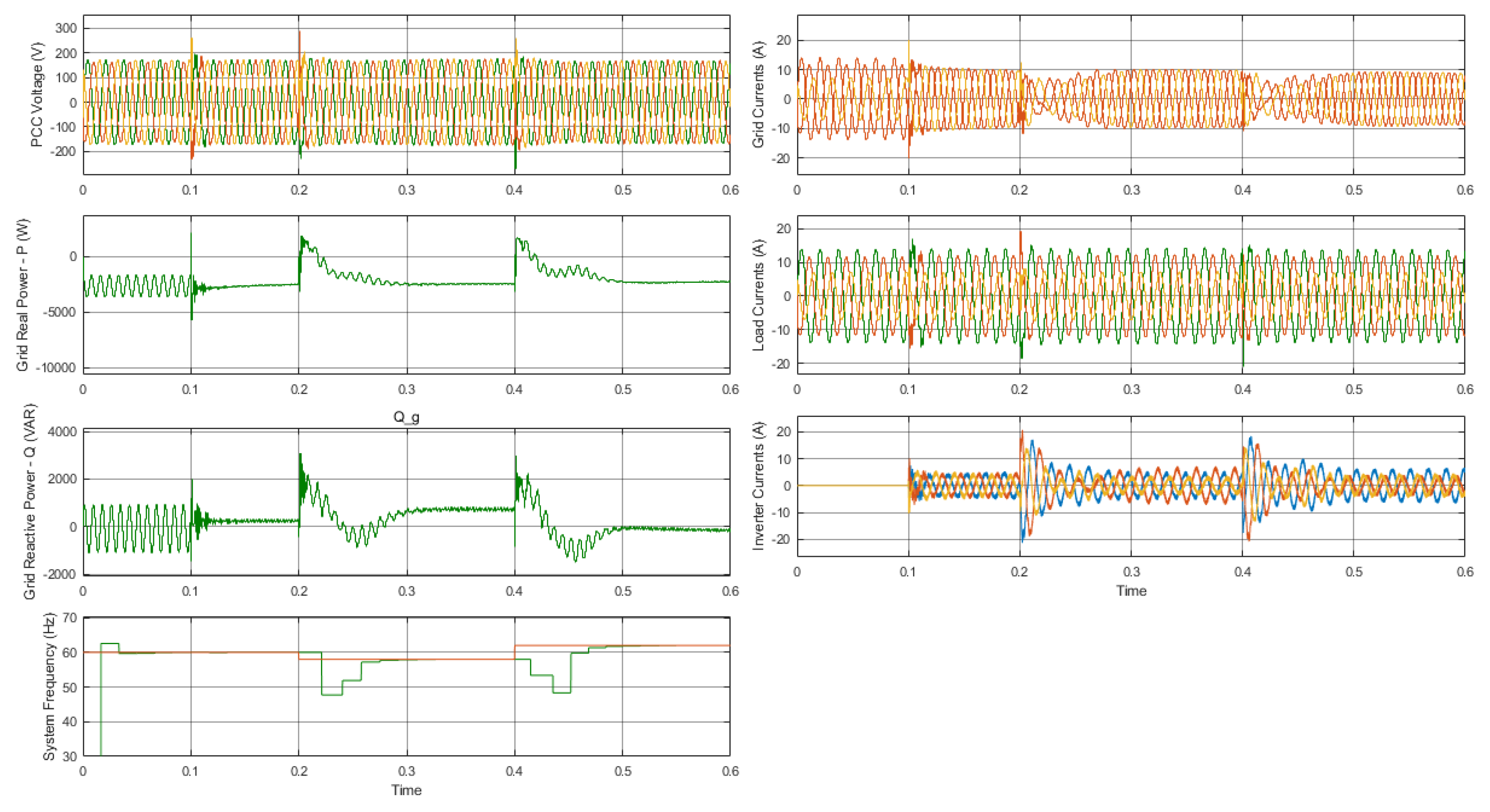
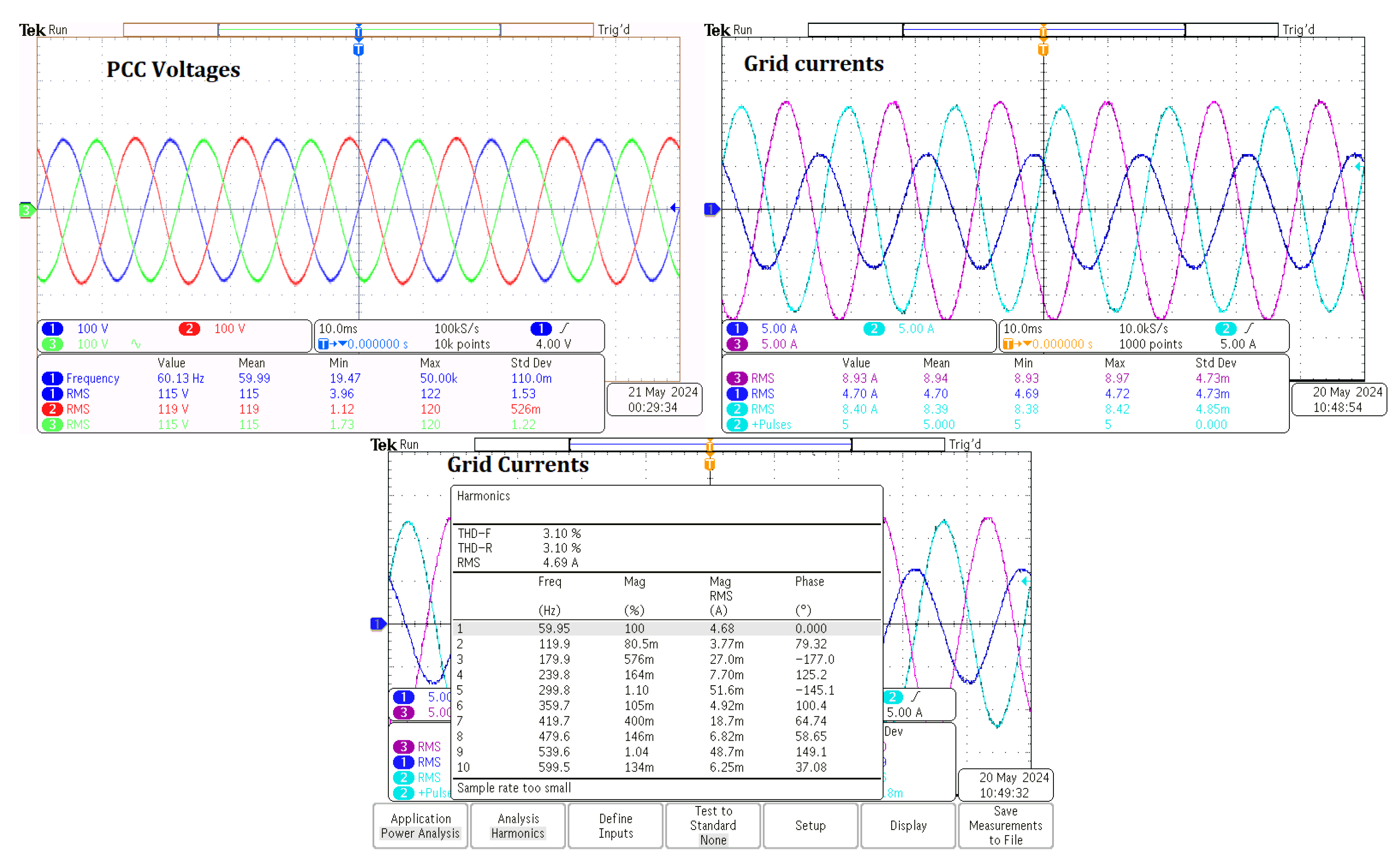
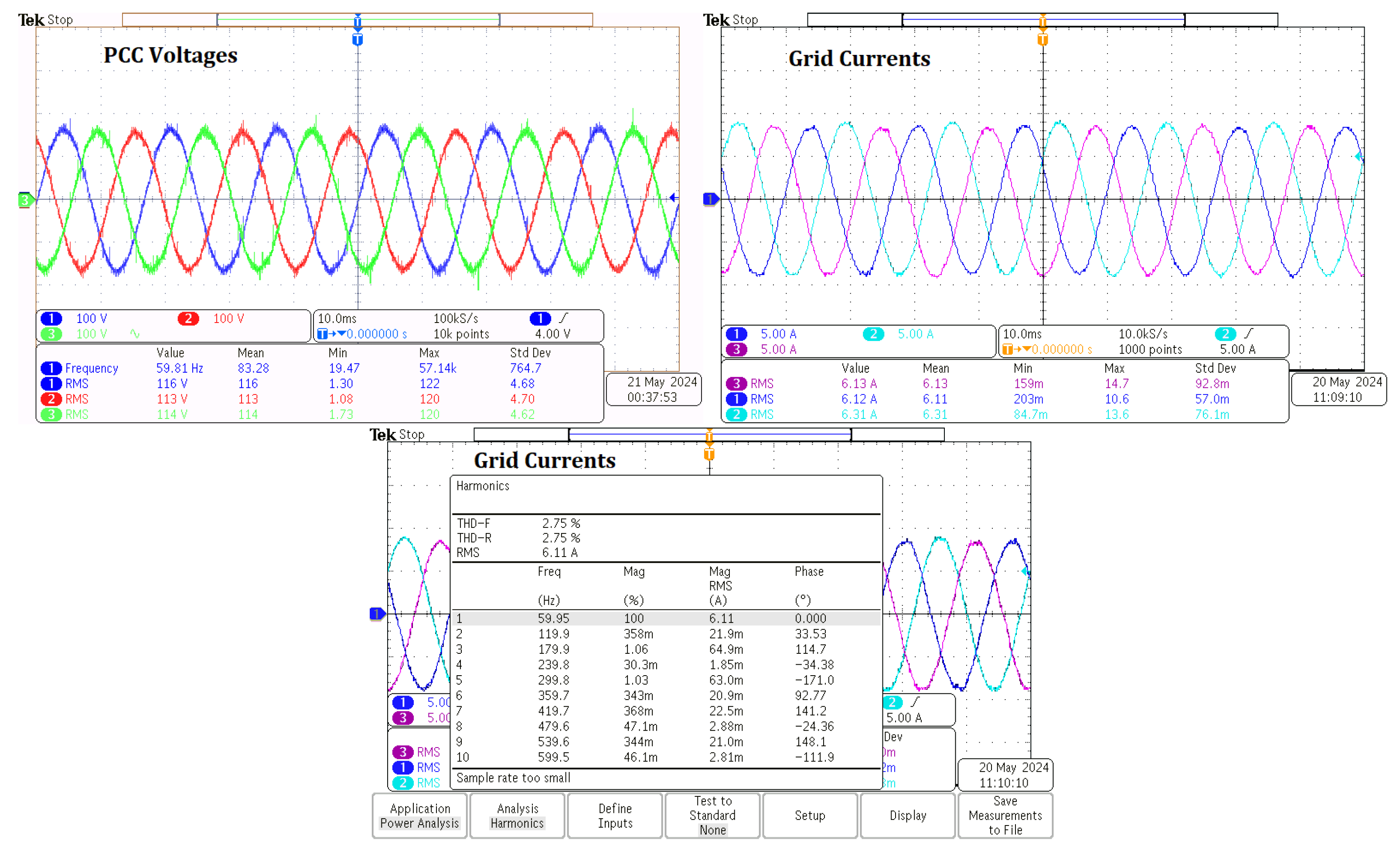
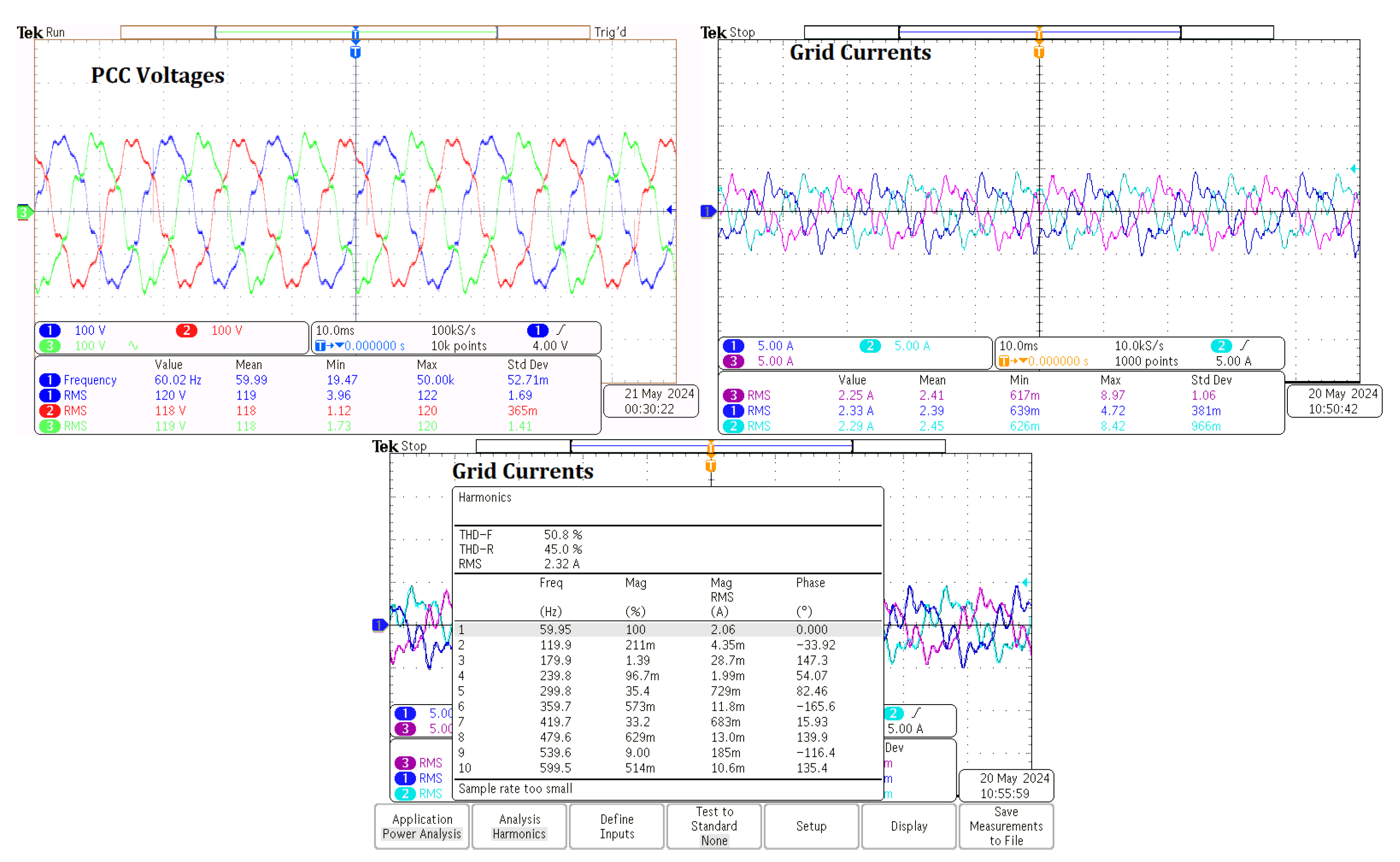
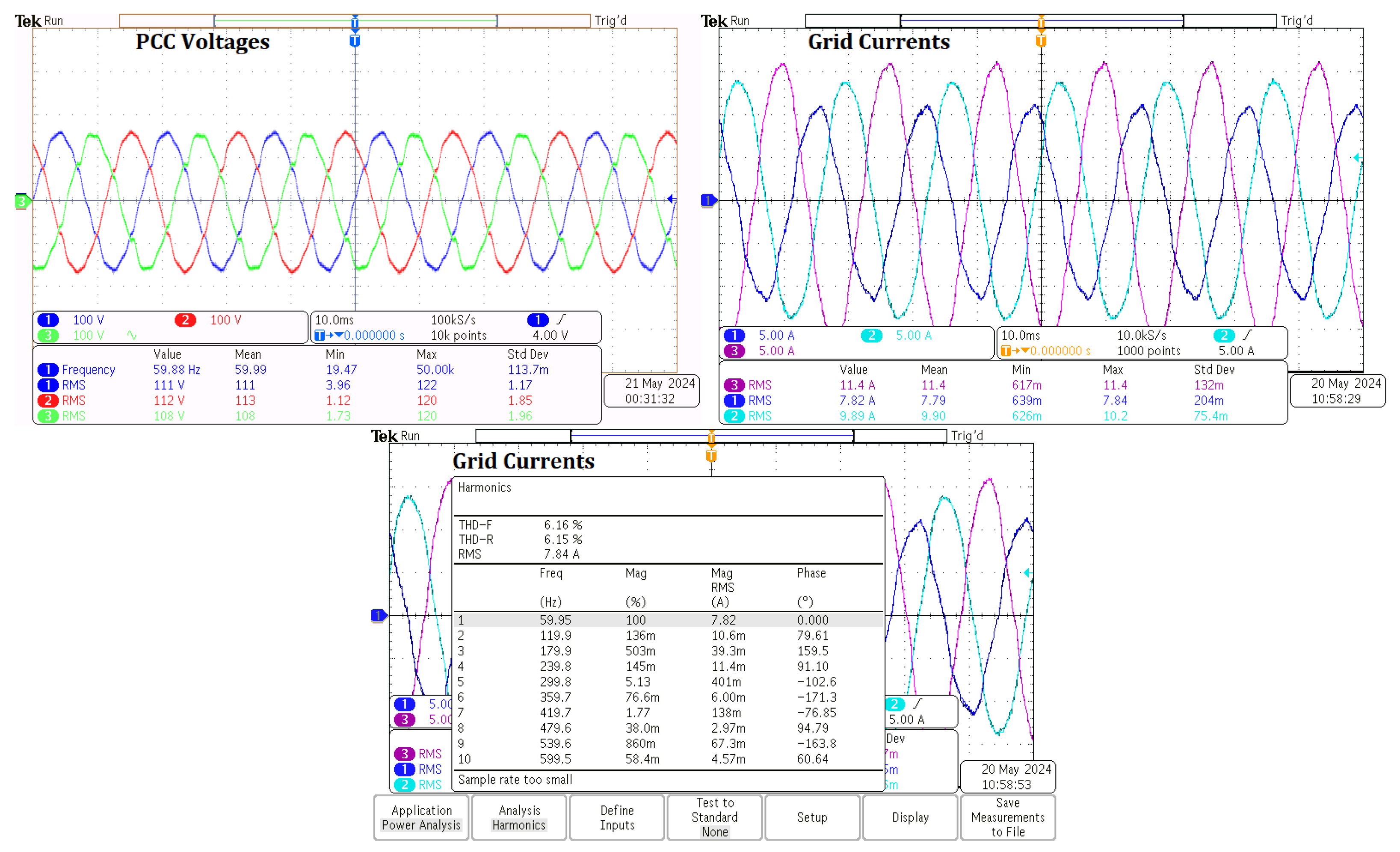
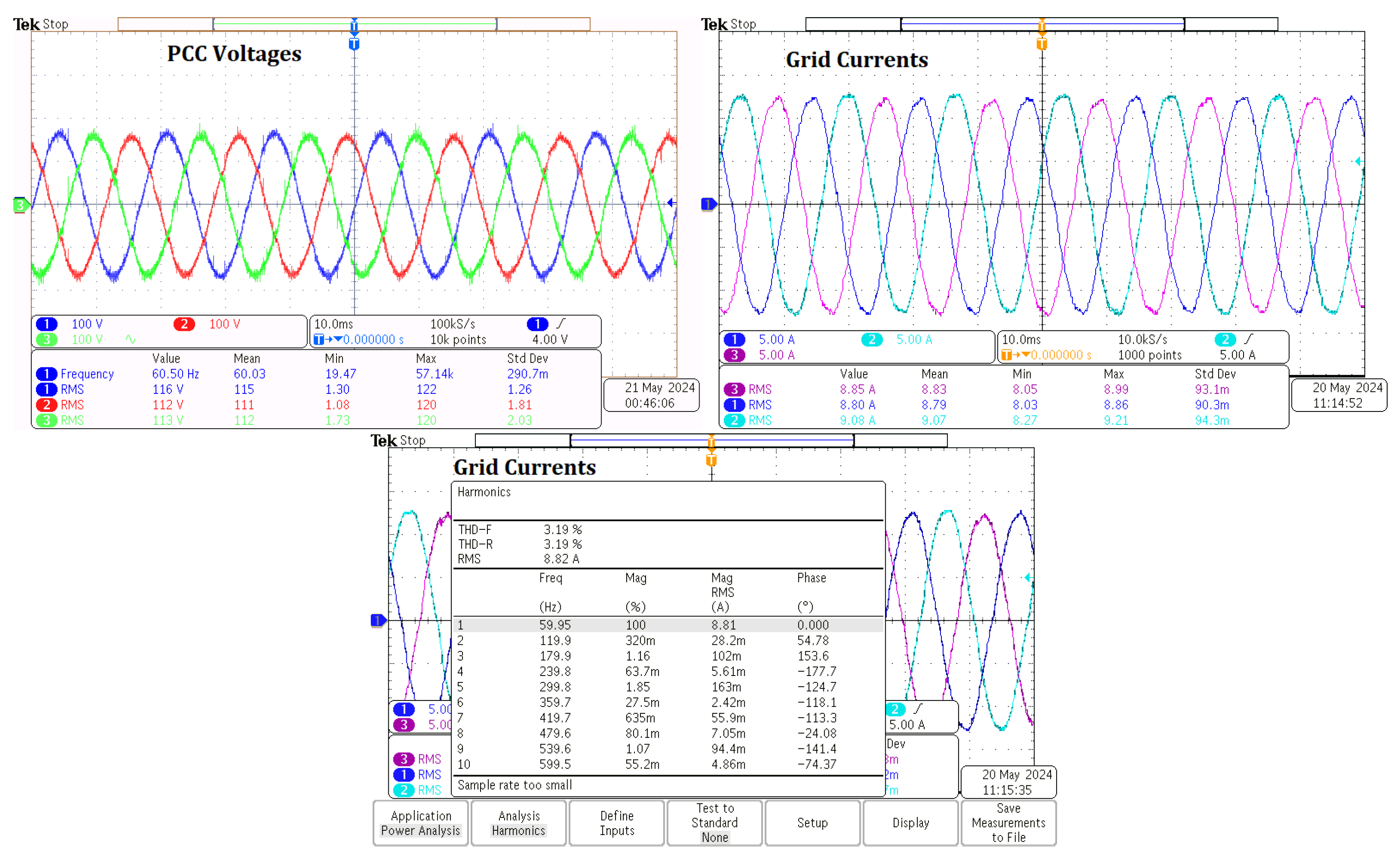
5.2.1. Unbalanced Three-Phase Resistive Load
5.2.2. Three-Phase Rectifier with a Resistor Load
5.2.3. Three-Phase Induction Motor, Three-Phase Rectifier with a Resistor, and Unbalanced Three-Phase Resistive Loads
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Gupta, N.; Singh, S.P.; Dubey, S.P. Neural network based shunt active filter for harmonic and reactive power compensation under non-ideal mains voltage. In Proceedings of the 2010 5th IEEE Conference on Industrial Electronics and Applications, Taichung, Taiwan, 15–17 June 2010; pp. 370–375. [Google Scholar] [CrossRef]
- Singh, B.; Al-Haddad, K.; Chandra, A. A review of active filters for power quality improvement. IEEE Trans. Ind. Electron. 1999, 46, 960–971. [Google Scholar] [CrossRef]
- Salmerón, P.; Litrán, S.P. A Control Strategy for Hybrid Power Filter to Compensate Four-Wires Three-Phase Systems. IEEE Trans. Power Electron. 2010, 25, 1923–1931. [Google Scholar] [CrossRef]
- Lam, C.-S.; Choi, W.-H.; Wong, M.-C.; Han, Y.-D. Adaptive DC-Link Voltage-Controlled Hybrid Active Power Filters for Reactive Power Compensation. IEEE Trans. Power Electron. 2012, 27, 1758–1772. [Google Scholar] [CrossRef]
- Watanabe, E.H.; Aredes, M.; Afonso, J.L.; Pinto, J.G.; Monteiro, L.F.C.; Akagi, H. Instantaneous p–q power theory for control of compensators in micro-grids. In Proceedings of the 2010 International School on Nonsinusoidal Currents and Compensation, Lagow, Poland, 15–18 June 2010; pp. 17–26. [Google Scholar] [CrossRef]
- Sozański, K.P. Control circuit for active power filter with an instantaneous reactive power control algorithm modification. In Proceedings of the 2010 International School on Nonsinusoidal Currents and Compensation, Lagow, Poland, 15–18 June 2010; pp. 230–233. [Google Scholar] [CrossRef]
- Lyons, R.; Howard, C. Improvements to the Sliding Discrete Fourier Transform Algorithm. IEEE Signal Process. Mag. 2021, 38, 119–127. [Google Scholar] [CrossRef]
- Sozanski, K.; Jarnut, M. Three-phase active power filter using the sliding DFT control algorithm. In Proceedings of the 2005 European Conference on Power Electronics and Applications, Dresden, Germany, 11–14 September 2005; p. 10. [Google Scholar] [CrossRef]
- Karimi, S.; Poure, P.; Saadate, S. High performances reference current generation for shunt active filter under distorted and unbalanced conditions. In Proceedings of the IEEE—PESC Power Electronics Specialists Conference, Rhodes, Greece, 15–19 June 2008; pp. 195–201. [Google Scholar]
- Song, H.-S.; Joo, I.-W.; Nam, K. Source voltage sensorless estimation scheme for PWM rectifiers under unbalanced conditions. IEEE Trans. Ind. Electron. 2003, 50, 1238–1245. [Google Scholar] [CrossRef]
- Ahmed, K.H.; Massoud, A.M.; Finney, S.J.; Williams, B.W. Sensorless current control of three-phase inverter-based distributed generation. IEEE Trans. Power Del. 2009, 24, 919–929. [Google Scholar] [CrossRef]
- Malinowski, M.; Bernet, S. A Simple Voltage Sensorless Active Damping Scheme for Three-Phase PWM Converters With an LCL Filter. IEEE Trans. Ind. Electron. 2008, 55, 1876–1880. [Google Scholar] [CrossRef]
- Al-Gahtani, S.; Nelms, R.M. A New Voltage Sensorless Control Method for a Shunt Active Power Filter for Unbalanced Conditions. In Proceedings of the 2019 IEEE International Conference on Environment and Electrical Engineering and 2019 IEEE Industrial and Commercial Power Systems Europe (EEEIC/I & CPS Europe), Genova, Italy, 11–14 June 2019; pp. 1–6. [Google Scholar] [CrossRef]
- Al-Gahtani, S.; Nelms, R.M. Performance of a Shunt Active Power Filter for Unbalanced Conditions Using Only Current Measurements. Energies 2021, 14, 397. [Google Scholar] [CrossRef]
- Alathamneh, M.; Ghanayem, H.; Nelms, R.M. Power Control of a Three-phase Grid-connected Inverter using a PI Controller under Unbalanced Conditions. In Proceedings of the SoutheastCon 2022, Mobile, AL, USA, 26 March–3 April 2022; pp. 447–452. [Google Scholar] [CrossRef]
- Alathamneh, M.; Ghanayem, H.; Nelms, R.M. Bidirectional Power Control for a Three-Phase Grid-Connected Inverter under Unbalanced Grid Conditions Using a Proportional-Resonant and a Modified Time Domain Symmetrical Components Extraction Method. Energies 2022, 15, 9564. [Google Scholar] [CrossRef]
- Alepuz, S.; Busquets-Monge, S.; Bordonau, J.; Martínez-Velasco, J.A.; Silva, C.A.; Pontt, J.; Rodríguez, J. Control Strategies Based on Symmetrical Components for Grid-Connected Converters Under Voltage Dips. IEEE Trans. Ind. Electron. 2009, 56, 2162–2173. [Google Scholar] [CrossRef]
- Ivanović, Z.R.; Adžić, E.M.; Vekić, M.S.; Grabić, S.U.; Čelanović, N.L.; Katić, V.A. HIL Evaluation of Power Flow Control Strategies for Energy Storage Connected to Smart Grid Under Unbalanced Conditions. IEEE Trans. Power Electron. 2012, 27, 4699–4710. [Google Scholar] [CrossRef]
- Srinath, N.S.; Sundarabalan, C.K.; Sharma, J.; Balasundar, C.; Vijayakumar, V. Quasi newton least mean fourth control for multifunctional grid tied solar photovoltaic system. Sustain. Energy Technol. Assessments 2023, 57, 103272. [Google Scholar] [CrossRef]
- Appala Naidu, T.; Arya, S.R.; Maurya, R.; Padmanaban, S. Performance of DVR using optimized PI controller based gradient adaptive variable step LMS control algorithm. IEEE J. Emerg. Sel. Top. Ind. Electron. 2021, 2, 155–163. [Google Scholar] [CrossRef]
- Naidu, T.A.; Arya, S.R.; Maurya, R.; Singh, B.; Al-Durra, A. Combined variable step size LMS for DVR with error regulator gain tuning through ant-lion optimization. In Proceedings of the IEEE Industry Applications Society Annual. Meeting (IAS), Detroit, MI, USA, 10–16 October 2020; pp. 1–8. [Google Scholar]
- Routray, A.; Pradhan, A.K.; Rao, K.P. A novel Kalman filter for frequency estimation of distorted signals in power systems. IEEE Trans. Instrum. Meas. 2002, 51, 469–479. [Google Scholar] [CrossRef]
- Germani, A.; Manes, C.; Palumbo, P. Polynomial extended Kalman filter. IEEE Trans. Autom. Control 2005, 50, 2059–2064. [Google Scholar] [CrossRef]
- Yang, X.; Zhang, Z.; Xu, M.; Li, S.; Zhang, Y.; Zhu, X.-F.; Ouyang, X.; Alù, A. Digital non-foster-inspired electronics for broadband impedance matching. Nat. Commun. 2024, 15, 4346. [Google Scholar] [CrossRef] [PubMed]
- Tian, W.; Wang, L.; Yu, J.; Lv, Y.; Zhang, S.; Xu, Q.; Gao, B. A hybrid impedance matching network for underwater acoustic transducers. IEEE Trans. Power Electron. 2023, 38, 7622–7633. [Google Scholar] [CrossRef]
- Ghanayem, H.; Alathamneh, M.; Nelms, R.M. Adaptive PIR Current Controllers for Torque Ripple Reduction of PMSM using Decoupled Speed and Flux Control. In Proceedings of the 2023 IEEE 14th Annual Ubiquitous Computing, Electronics & Mobile Communication Conference (UEMCON), New York, NY, USA, 12–14 October 2023; pp. 440–444. [Google Scholar] [CrossRef]
- Yang, X.; Zhou, H.; Alathamneh, M.; Nelms, R.M. An Evolutionary Annealing–Simplex Method for Inductance Value Selection for LCL Filters. Energies 2023, 16, 4192. [Google Scholar] [CrossRef]

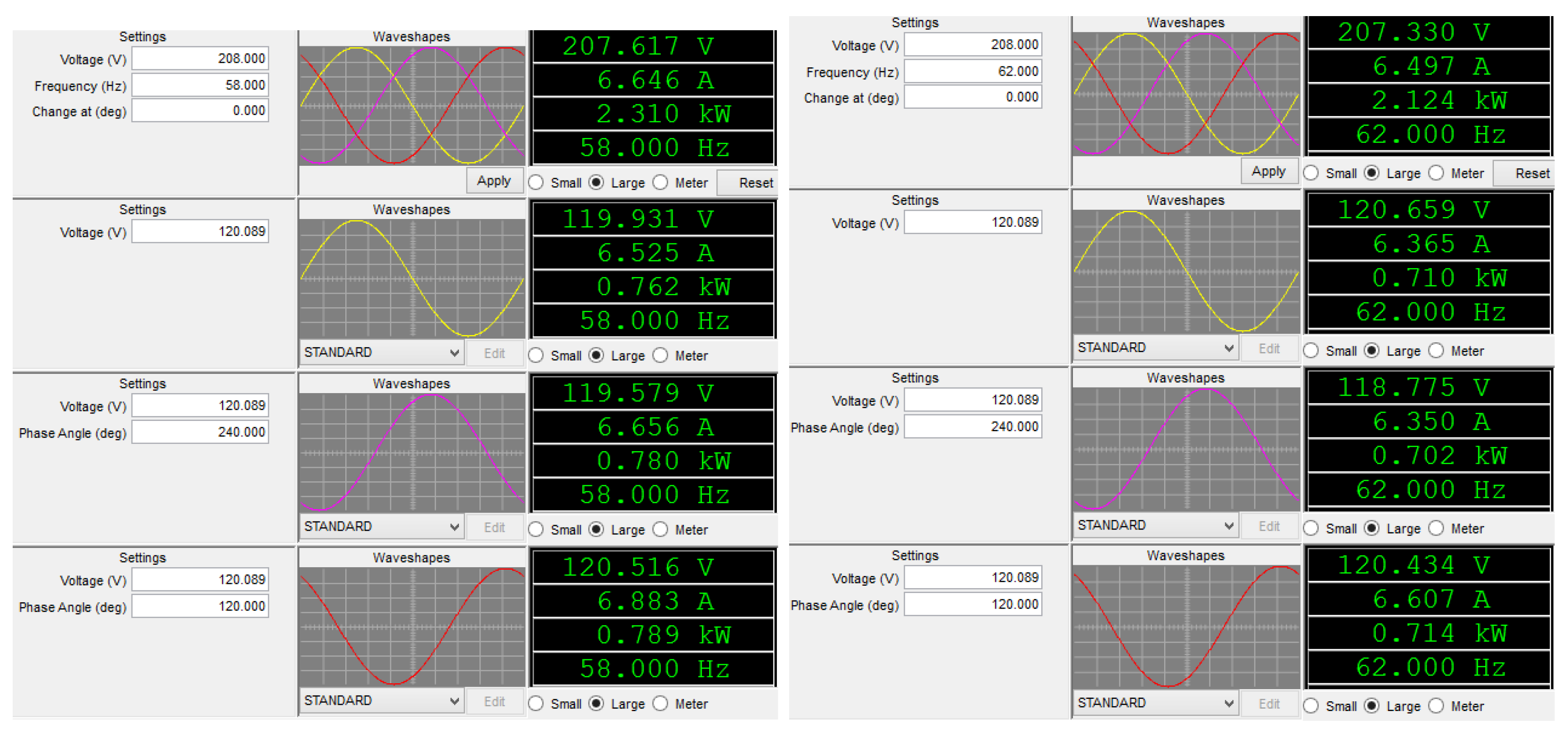

| f | Grid frequency | 58, 60, 62 Hz |
| Grid phase voltage | 120 V RMS | |
| Switching frequency | 5 kHz | |
| DC source voltage | 400 V | |
| L1 | Inverter side inductance | 2.3 mH |
| L2 | Grid side inductance | 0.58 mH |
| C | Filter capacitance | 15 F |
| R | Damping resistor | 1.5 |
| Unbalanced grid Impedance for phases a, b, c | 5.1, 4.5, 3 mH | |
| Three-phase load | 8, 16, 32 | |
| Three-phase AC-DC rectifier resistor | 100 |
| Load | Relative Error of | Relative Error of |
|---|---|---|
| Unbalanced R-Load | 0.334% | 0.968% |
| Three-phase Rectifier Load | 0.667% | 1.093% |
| Three-phase Induction Motor, | 0.957% | 2% |
| Three-phase Rectifier, | ||
| and Unbalanced R-Load |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ghanayem, H.; Alathamneh, M.; Yang, X.; Seo, S.; Nelms, R.M. Enhanced Three-Phase Shunt Active Power Filter Utilizing an Adaptive Frequency Proportional-Integral–Resonant Controller and a Sensorless Voltage Method. Energies 2025, 18, 116. https://doi.org/10.3390/en18010116
Ghanayem H, Alathamneh M, Yang X, Seo S, Nelms RM. Enhanced Three-Phase Shunt Active Power Filter Utilizing an Adaptive Frequency Proportional-Integral–Resonant Controller and a Sensorless Voltage Method. Energies. 2025; 18(1):116. https://doi.org/10.3390/en18010116
Chicago/Turabian StyleGhanayem, Haneen, Mohammad Alathamneh, Xingyu Yang, Sangwon Seo, and R. M. Nelms. 2025. "Enhanced Three-Phase Shunt Active Power Filter Utilizing an Adaptive Frequency Proportional-Integral–Resonant Controller and a Sensorless Voltage Method" Energies 18, no. 1: 116. https://doi.org/10.3390/en18010116
APA StyleGhanayem, H., Alathamneh, M., Yang, X., Seo, S., & Nelms, R. M. (2025). Enhanced Three-Phase Shunt Active Power Filter Utilizing an Adaptive Frequency Proportional-Integral–Resonant Controller and a Sensorless Voltage Method. Energies, 18(1), 116. https://doi.org/10.3390/en18010116






