Electromagnetic Performance Analysis of Dual-Three-Phase Dual-Rotor Flux-Switching Permanent Magnet Machines †
Abstract
1. Introduction
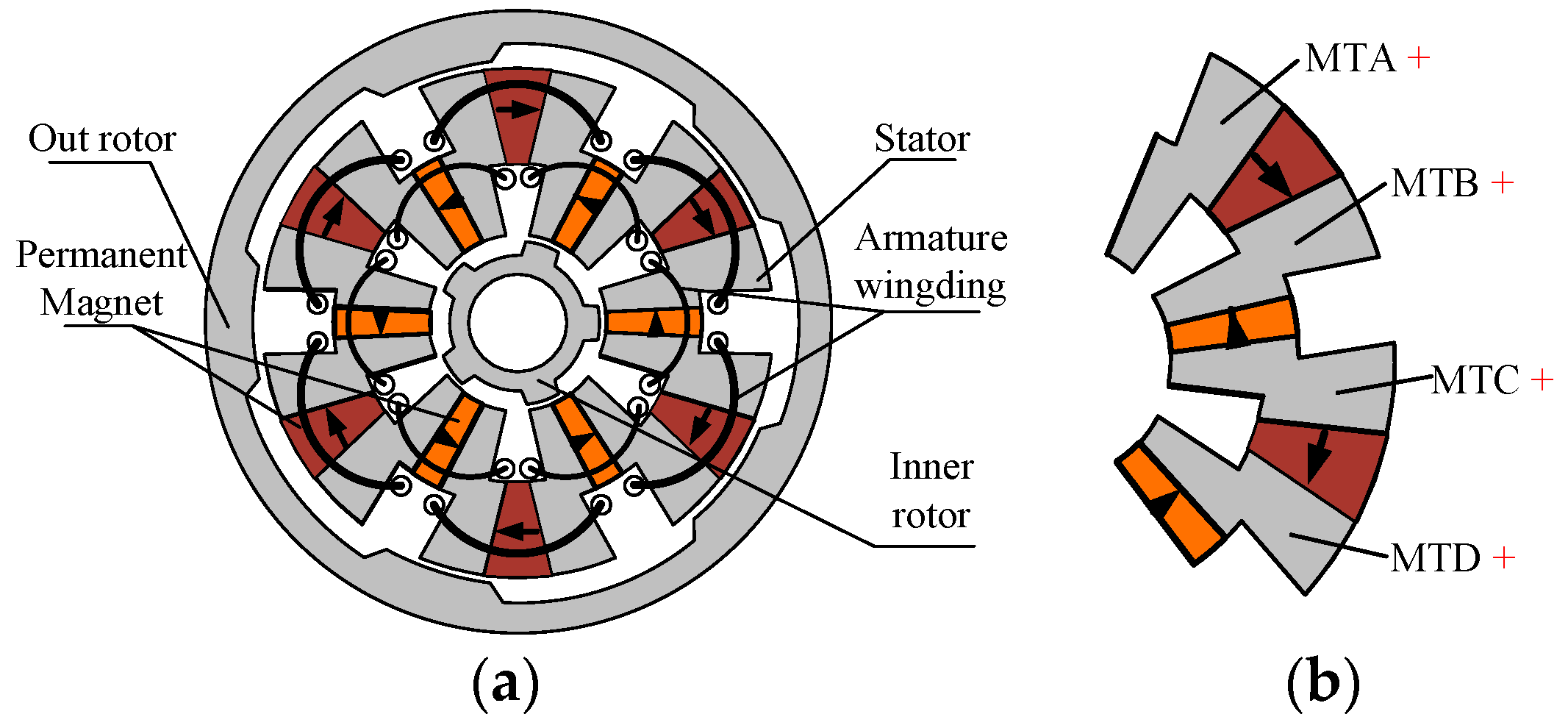
2. Machine Topology
3. Operating Principle and Key Parameter Design
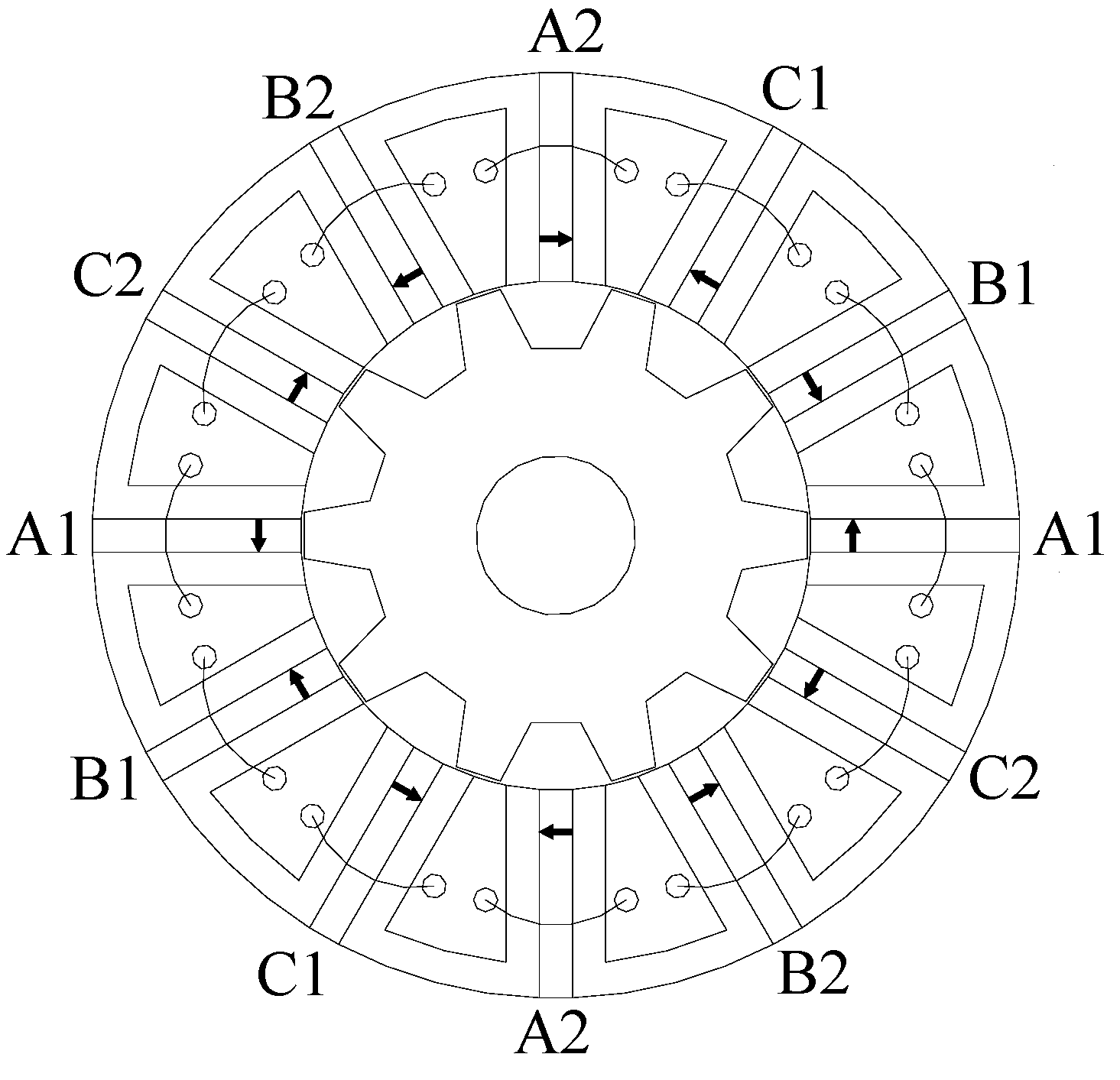
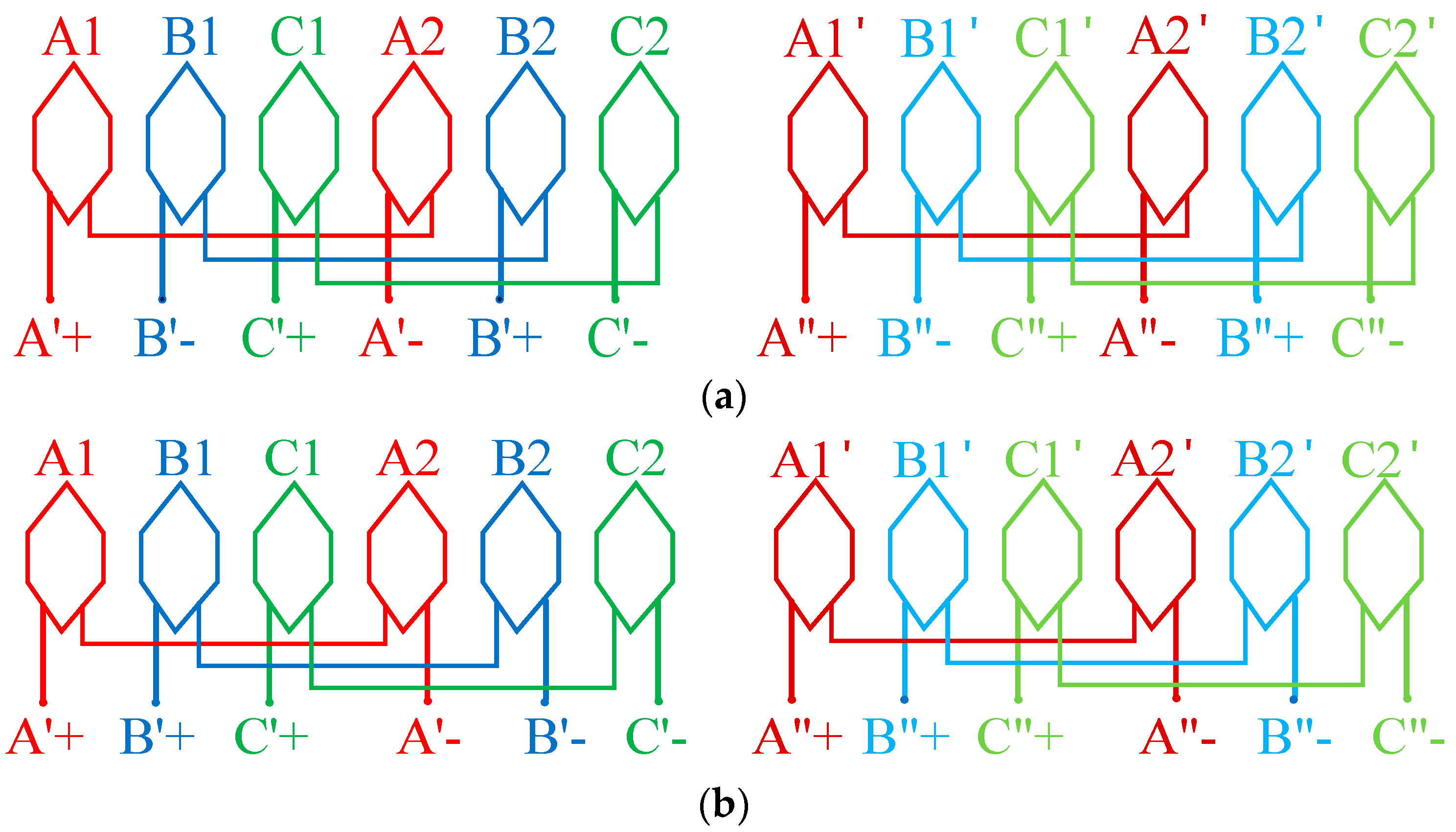
3.1. Operating Principle
3.2. Design Considerations
4. Discussion of Electromagnetic Performance Analysis
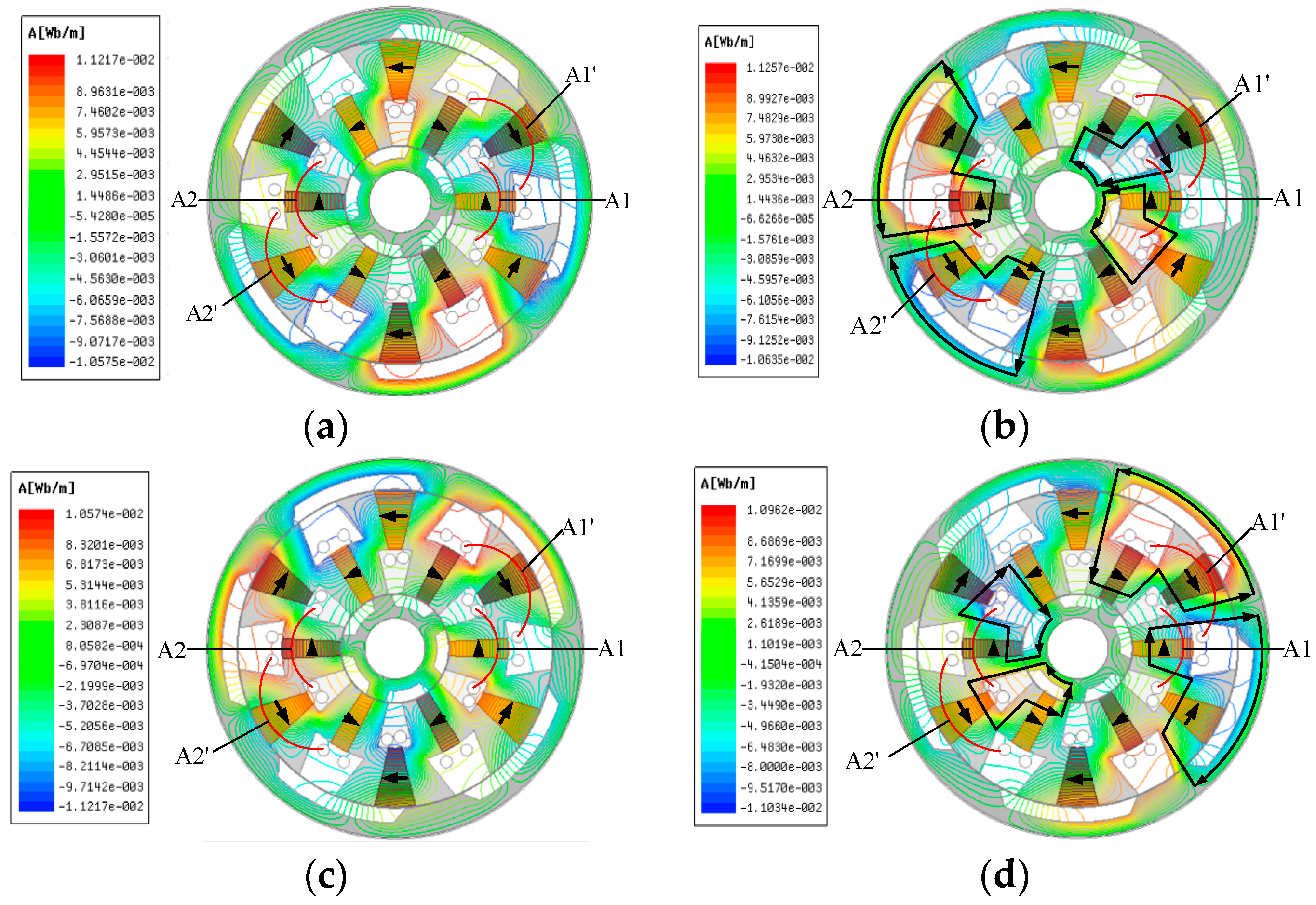
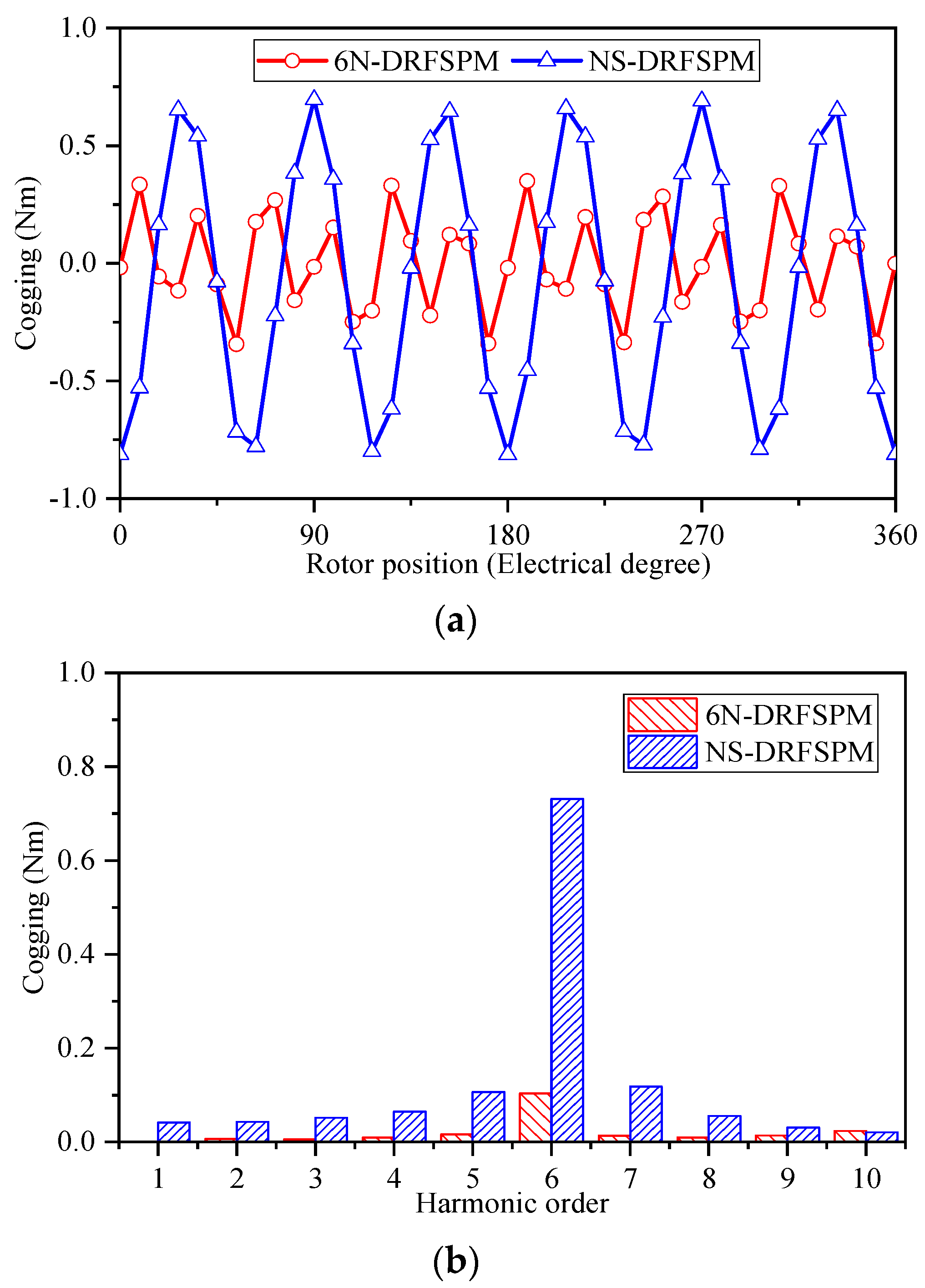
4.1. No-Load Performance
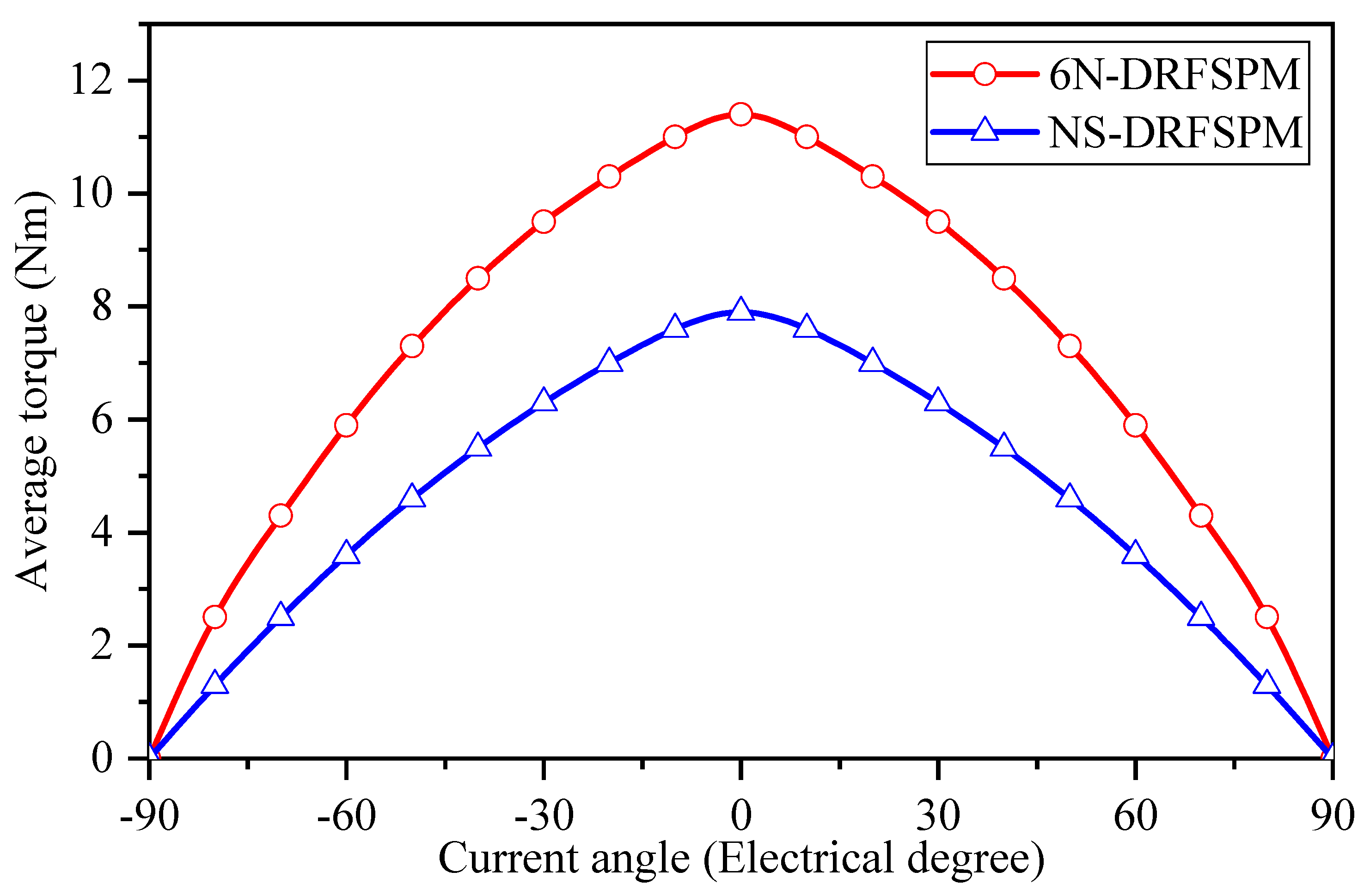
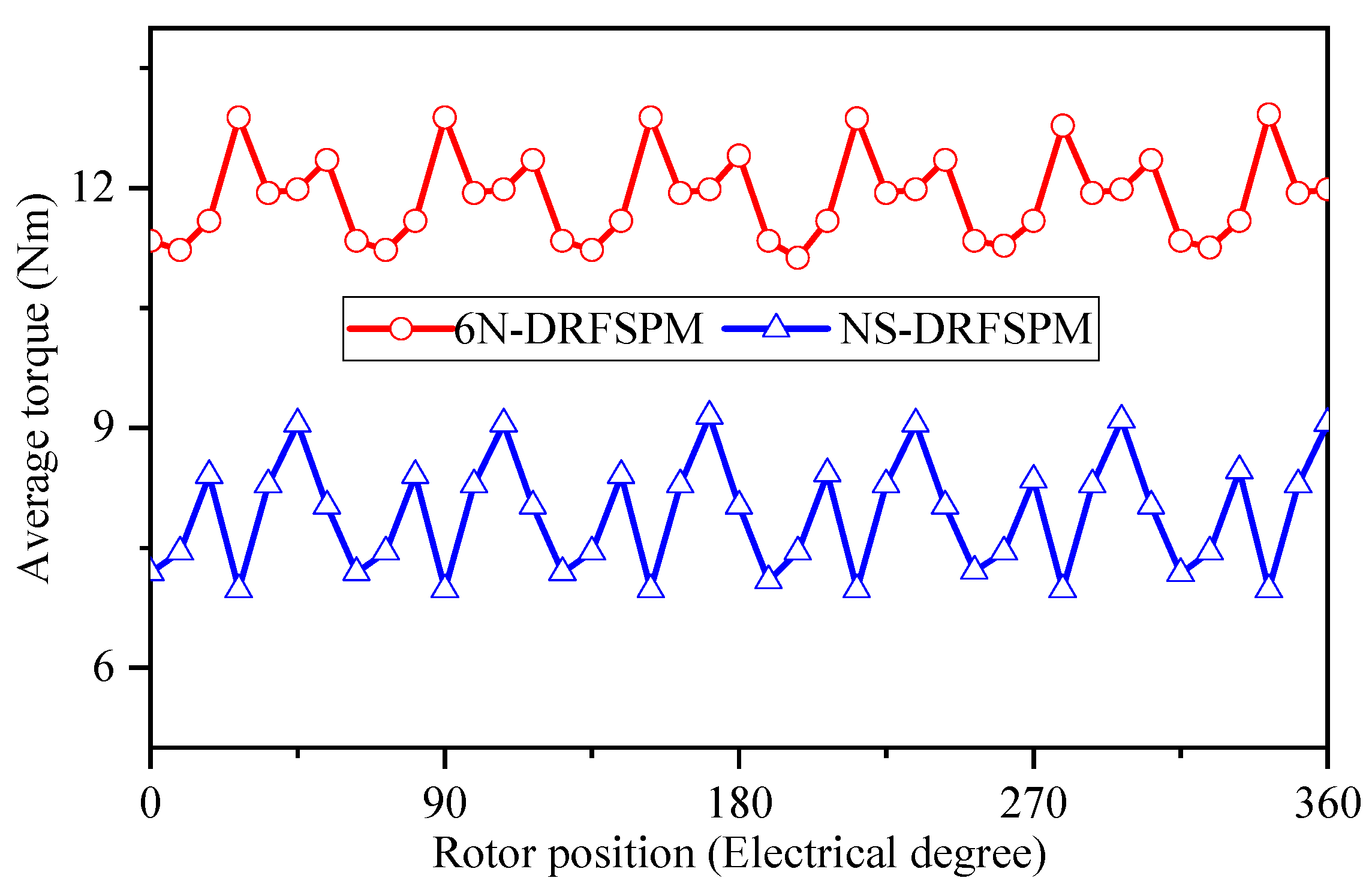
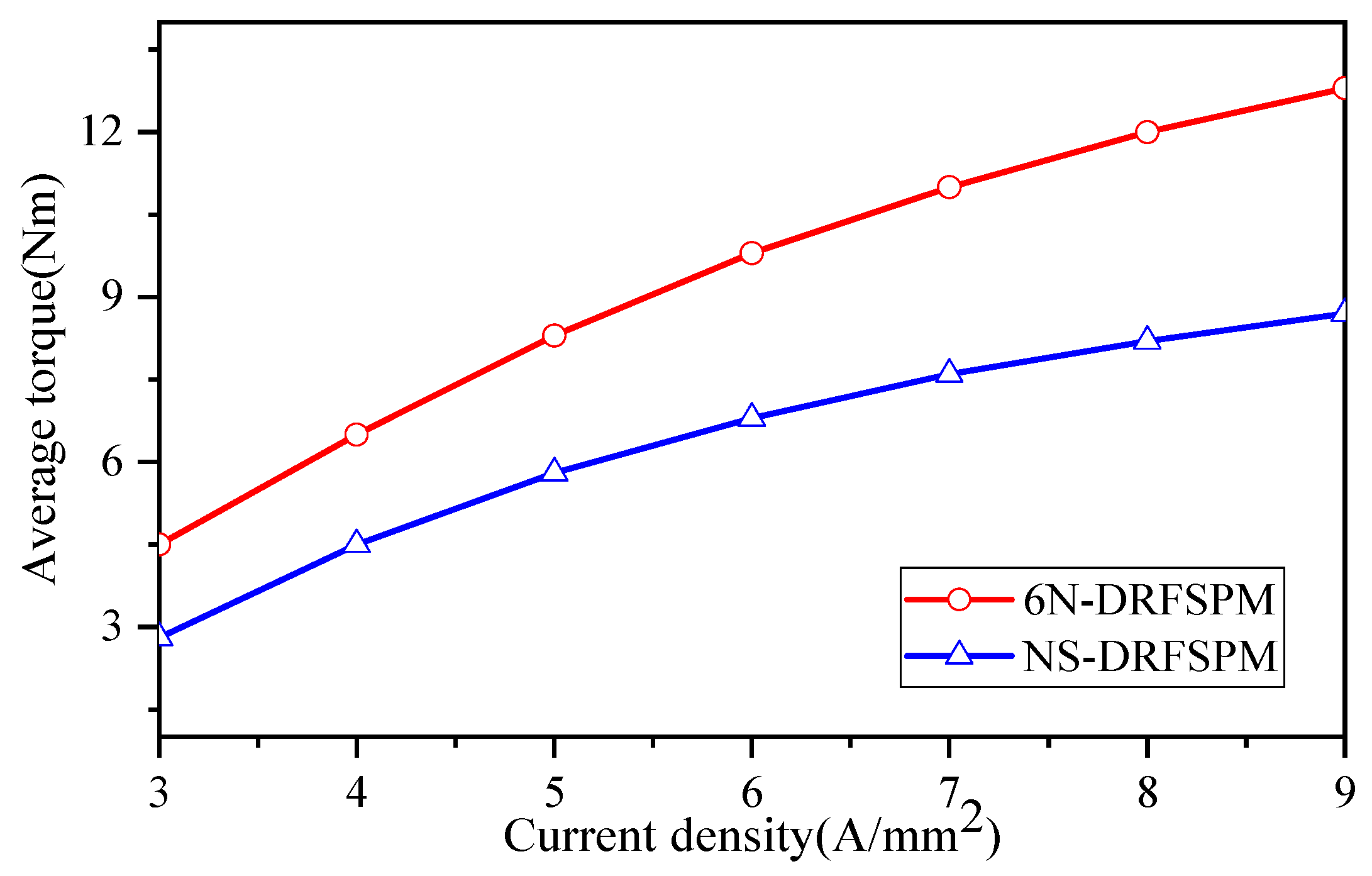
4.2. Torque Performance
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Pfaff, G.; Weschta, A.; Wick, A.F. Design and Experimental Results of a Brushless AC Servo Drive. IEEE Trans. Ind. Appl. 1984, IA-20, 814–821. [Google Scholar] [CrossRef]
- Zhu, Z.Q.; Howe, D. Electrical Machines and Drives for Electric, Hybrid, and Fuel Cell Vehicles. Proc. IEEE 2007, 95, 746–765. [Google Scholar] [CrossRef]
- Chau, K.T.; Chan, C.C.; Liu, C. Overview of Permanent-Magnet Brushless Drives for Electric and Hybrid Electric Vehicles. IEEE Trans. Ind. Electron. 2008, 55, 2246–2257. [Google Scholar] [CrossRef]
- El-Refaie, A.M.; Shah, M.R.; Qu, R.; Kern, J.M. Effect of Number of Phases on Losses in Conducting Sleeves of Surface PM Machine Rotors Equipped with Fractional-Slot Concentrated Windings. IEEE Trans. Ind. Appl. 2008, 44, 1522–1532. [Google Scholar] [CrossRef]
- Lu, K.; Li, X.; Zhao, Y.; Yi, P.; Yan, B.; Hua, W. A novel three-vector-based model predictive flux control with low computation complexity for SPMSM. IEEE Trans. Transp. Electrif. 2023. early access. [Google Scholar] [CrossRef]
- Barcaro, M.; Bianchi, N. Interior PM Machines Using Ferrite to Replace Rare-Earth Surface PM Machines. IEEE Trans. Ind. Appl. 2014, 50, 979–985. [Google Scholar] [CrossRef]
- Wu, X.; Wrobel, R.; Mellor, P.H.; Zhang, C.A. Computationally Efficient PM Power Loss Mapping for Brushless AC PM Machines with Surface-Mounted PM Rotor Construction. IEEE Trans. Ind. Electron. 2015, 62, 7391–7401. [Google Scholar] [CrossRef]
- Reddy, P.B.; El-Refaie, A.M.; Huh, K.K.; Tangudu, J.K.; Jahns, T.M. Comparison of Interior and Surface PM Machines Equipped with Fractional-Slot Concentrated Windings for Hybrid Traction Applications. IEEE Trans. Energy Convers. 2012, 27, 593–602. [Google Scholar] [CrossRef]
- Niu, S.; Chau, K.T.; Li, J.; Li, W. Eddy-Current Analysis of Double-Stator Inset-Type Permanent Magnet Brushless Machines. IEEE Trans. Appl. Supercond. 2010, 20, 1097–1101. [Google Scholar]
- Wu, Z.Z.; Zhu, Z.Q. Analysis of Air-Gap Field Modulation and Magnetic Gearing Effects in Switched Flux Permanent Magnet Machines. IEEE Trans. Magn. 2015, 51, 8105012. [Google Scholar] [CrossRef]
- Hua, W.; Cheng, M.; Zhu, Z.Q.; Howe, D. Analysis and Optimization of Back EMF Waveform of a Flux-Switching Permanent Magnet Motor. IEEE Trans. Energy Convers. 2008, 23, 727–733. [Google Scholar] [CrossRef]
- Cui, S.; Cheng, Y.; Chan, C.C. A Basic Study of Electrical Variable Transmission and Its Application in Hybrid Electric Vehicle. In Proceedings of the 2006 IEEE Vehicle Power and Propulsion Conference, Windsor, UK, 6–8 September 2006; pp. 1–4. [Google Scholar]
- Zheng, P.; Liu, R.; Thelin, P.; Nordlund, E.; Sadarangani, C. Research on the Parameters and Performances of a 4QT Prototype Machine Used for HEV. IEEE Trans. Magn. 2007, 43, 443–446. [Google Scholar] [CrossRef]
- Xinhua, G.; Xuhui, W.; Xu, L.; Feng, Z.; Jun, L. Simulation and experiment analysis of dynamic process for an EVT based on dual mechanical port electric machine. In Proceedings of the 2009 IEEE 6th International Power Electronics and Motion Control Conference, Wuhan, China, 17–20 May 2009; pp. 2010–2014. [Google Scholar]
- Chau, K.T.; Zhang, D.; Jiang, J.Z.; Liu, C.; Zhang, Y. Design of a Magnetic-Geared Outer-Rotor Permanent-Magnet Brushless Motor for Electric Vehicles. IEEE Trans. Magn. 2007, 43, 2504–2506. [Google Scholar] [CrossRef]
- Sun, L.; Cheng, M.; Jia, H. Analysis of a Novel Magnetic-Geared Dual-Rotor Motor with Complementary Structure. IEEE Trans. Ind. Electron. 2015, 62, 6737–6747. [Google Scholar] [CrossRef]
- Hao, L.; Lin, M.; Li, W.; Luo, H.; Fu, X.; Jin, P. Novel Dual-Rotor Axial Field Flux-Switching Permanent Magnet Machine. IEEE Trans. Magn. 2012, 48, 4232–4235. [Google Scholar] [CrossRef]
- Zhao, W.; Lipo, T.A.; Kwon, B.I. A Novel Dual-Rotor, Axial Field, Fault-Tolerant Flux-Switching Permanent Magnet Machine with High Torque Performance. IEEE Trans. Magn. 2015, 51, 1–4. [Google Scholar] [CrossRef]
- Xiang, Z.; Quan, L.; Zhu, X. A New Partitioned-Rotor Flux-Switching Permanent Magnet Motor with High Torque Density and Improved Magnet Utilization. IEEE Trans. Appl. Supercond. 2016, 26, 5201905. [Google Scholar] [CrossRef]
- Zhou, L.; Hua, W.; Cheng, M. Analysis and optimization of key dimensions of co-axial dual-mechanical-port flux-switching permanent magnet machines for fuel-based extended range electric vehicles. CES Trans. Electr. Mach. Syst. 2017, 1, 292–299. [Google Scholar] [CrossRef]
- Zhou, L.; Hua, W. Influences of Stator Teeth Number on PM Coupling Levels of Co-Axial Dual-Mechanical-Port Flux-Switching PM Machines. IEEE Trans. Magn. 2019, 55, 8104007. [Google Scholar] [CrossRef]
- Toliyat, H.A.; Lipo, T.A.; White, J.C. Analysis of a concentrated winding induction machine for adjustable speed drive applications. I. Motor analysis. IEEE Trans. Energy Convers. 1991, 6, 679–683. [Google Scholar] [CrossRef]
- Demir, Y.; Aydin, M. A Novel Dual Three-Phase Permanent Magnet Synchronous Motor with Asymmetric Stator Winding. IEEE Trans. Magn. 2016, 52, 8105005. [Google Scholar] [CrossRef]
- Toliyat, H.A.; Xu, L.; Lipo, T.A. A five-phase reluctance motor with high specific torque. IEEE Trans. Ind. Appl. 1992, 28, 659–667. [Google Scholar] [CrossRef]
- Shao, L.; Hua, W.; Cheng, M. Investigation on phase shift between multiple-winding sets in multiphase flux-switching permanent magnet machines. In Proceedings of the 2015 IEEE Energy Conversion Congress and Exposition (ECCE), Montreal, QC, Canada, 20–24 September 2015; pp. 6942–6947. [Google Scholar]
- Hu, M.; Hua, W.; Huang, W.; Meng, J. Digital Current Control of an Asymmetrical Dual Three-Phase Flux-Switching Permanent Magnet Machine. IEEE Trans. Ind. Electron. 2020, 67, 4281–4291. [Google Scholar] [CrossRef]
- Shao, B.; Zhu, Z.Q.; Feng, J.; Guo, S.; Li, Y.; Liao, W. Compensation of Selective Current Harmonics for Switching-Table-Based Direct Torque Control of Dual Three-Phase PMSM Drives. IEEE Trans. Ind. Appl. 2021, 57, 2505–2515. [Google Scholar] [CrossRef]
- Luo, Y.; Liu, C. A Simplified Model Predictive Control for a Dual Three-Phase PMSM With Reduced Harmonic Currents. IEEE Trans. Ind. Electron. 2018, 65, 9079–9089. [Google Scholar] [CrossRef]
- Zhao, G.; Li, Z.; Hua, W.; Ding, S.; Su, P.; Jiang, X. Analysis of a novel dual-three-phase dual-rotor flux-switching permanent magnet machine. In Proceedings of the 2022 IEEE 20th Biennial Conference on Electromagnetic Field Computation (CEFC), Denver, CO, USA, 24–26 October 2022; pp. 1–2. [Google Scholar]
- Li, X.; Wei, Z.; Zhao, Y.; Wang, X.; Hua, W. Design and analysis of surface-mounted permanent-magnet field-modulation machine for achieving high power factor. IEEE Trans. Ind. Electron. 2023. early access. [Google Scholar] [CrossRef]
- Cheng, M.; Han, P.; Hua, W. General Airgap Field Modulation Theory for Electrical Machines. IEEE Trans. Ind. Electron. 2017, 64, 6063–6074. [Google Scholar] [CrossRef]
- Zhu, X.; Cheng, M.; Wang, Y.; Hua, W.; Han, P.; Wang, W. Mathematical Analysis Model of Double-Stator Field Modulation HTS Machine Based on General Airgap Field Modulation Theory. IEEE Trans. Energy Convers. 2022, 37, 475–486. [Google Scholar] [CrossRef]
- Zhao, W.; Hu, Q.; Ji, J.; Ling, Z.; Li, Z. Torque Generation Mechanism of Dual-Permanent-Magnet-Excited Vernier Machine by Air-Gap Field Modulation Theory. IEEE Trans. Ind. Electron. 2023, 70, 9799–9810. [Google Scholar] [CrossRef]




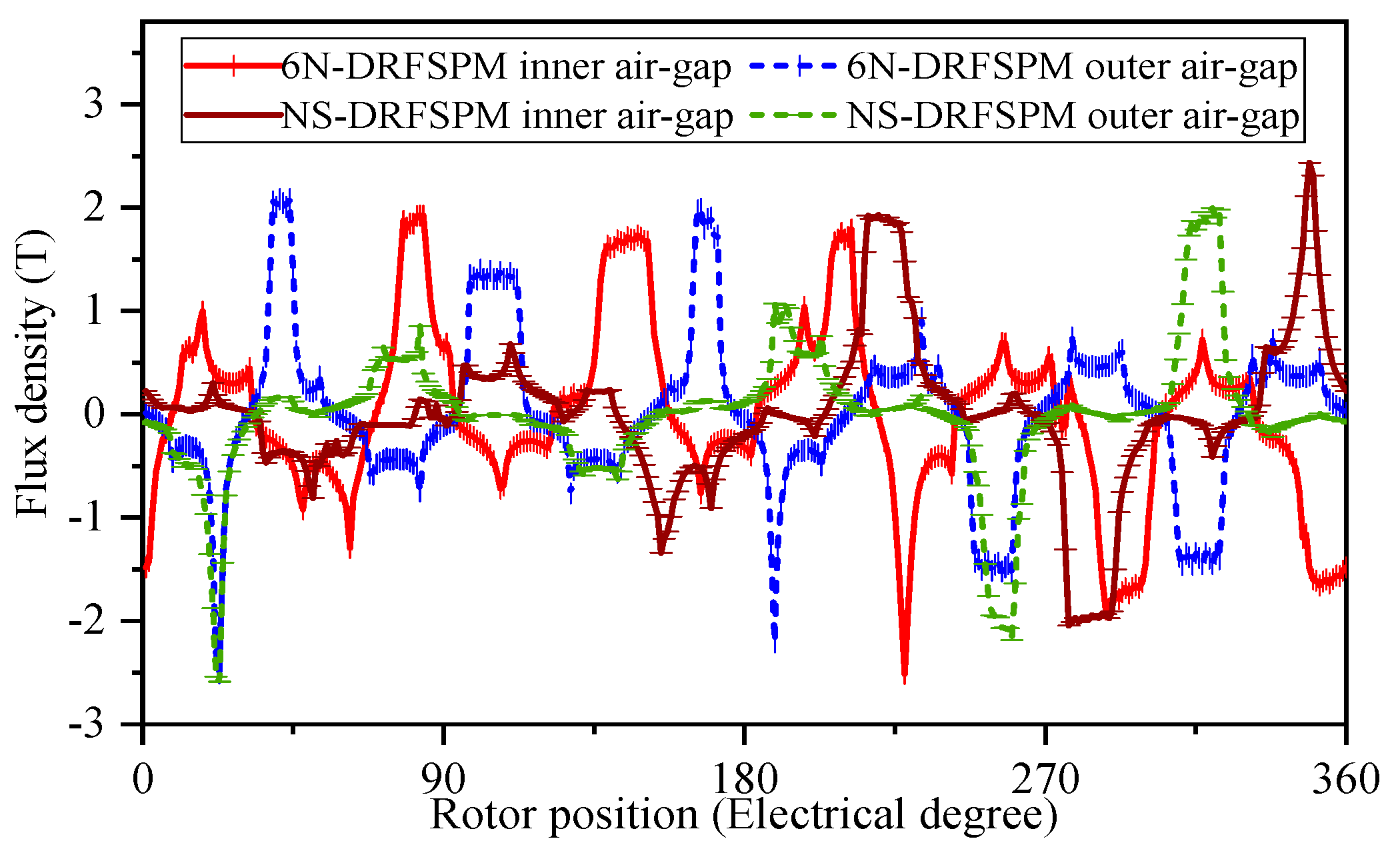
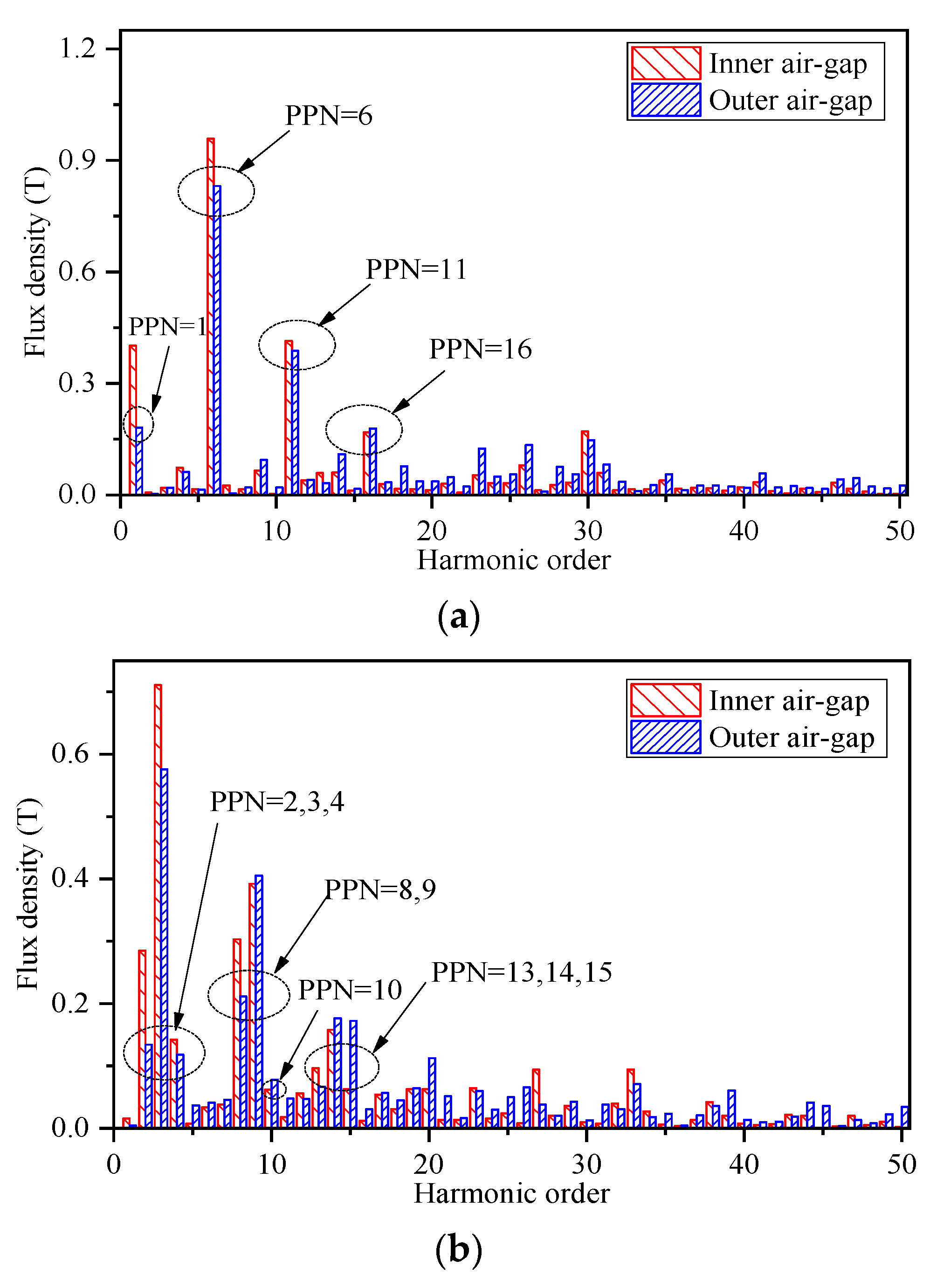
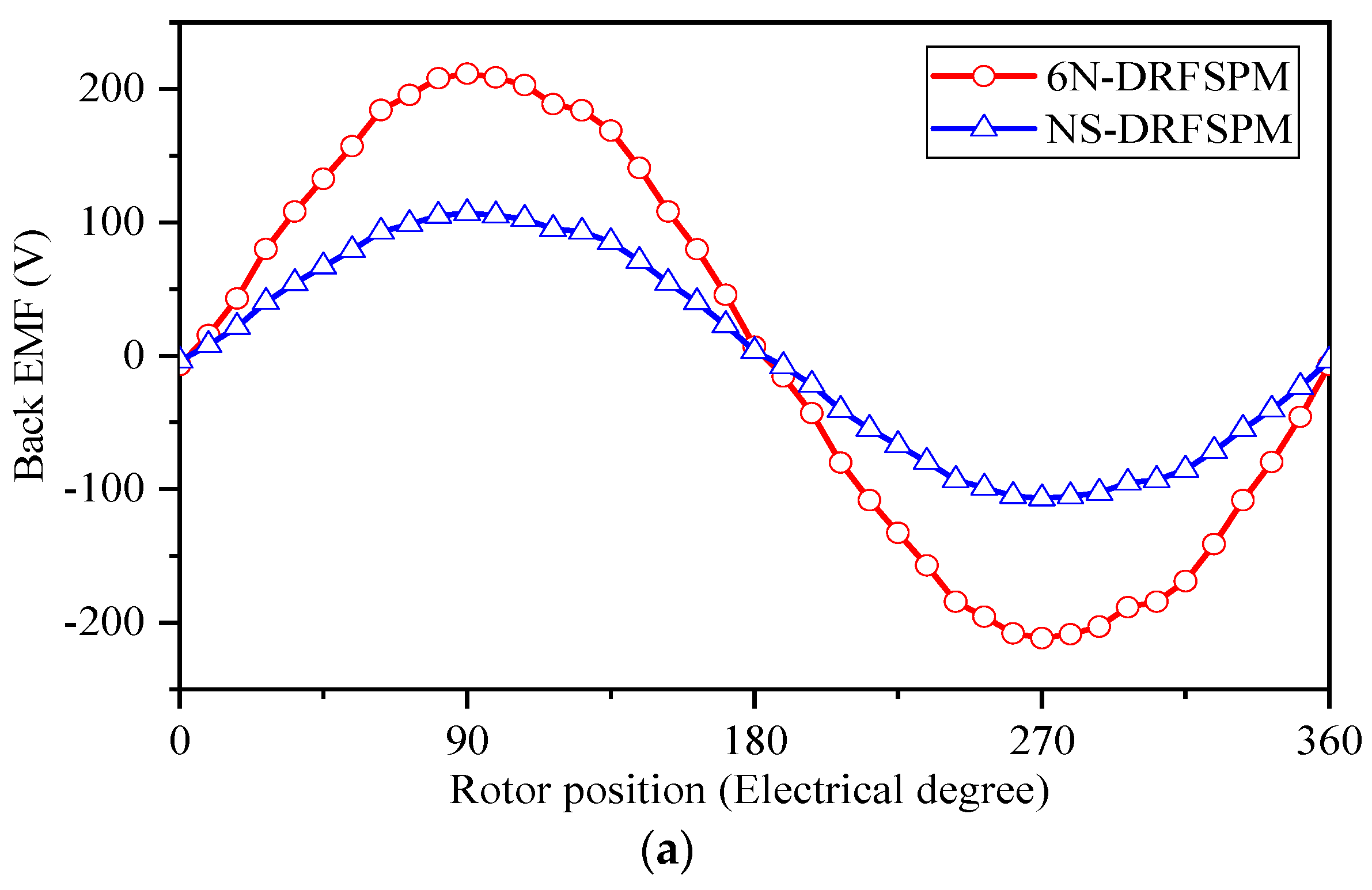
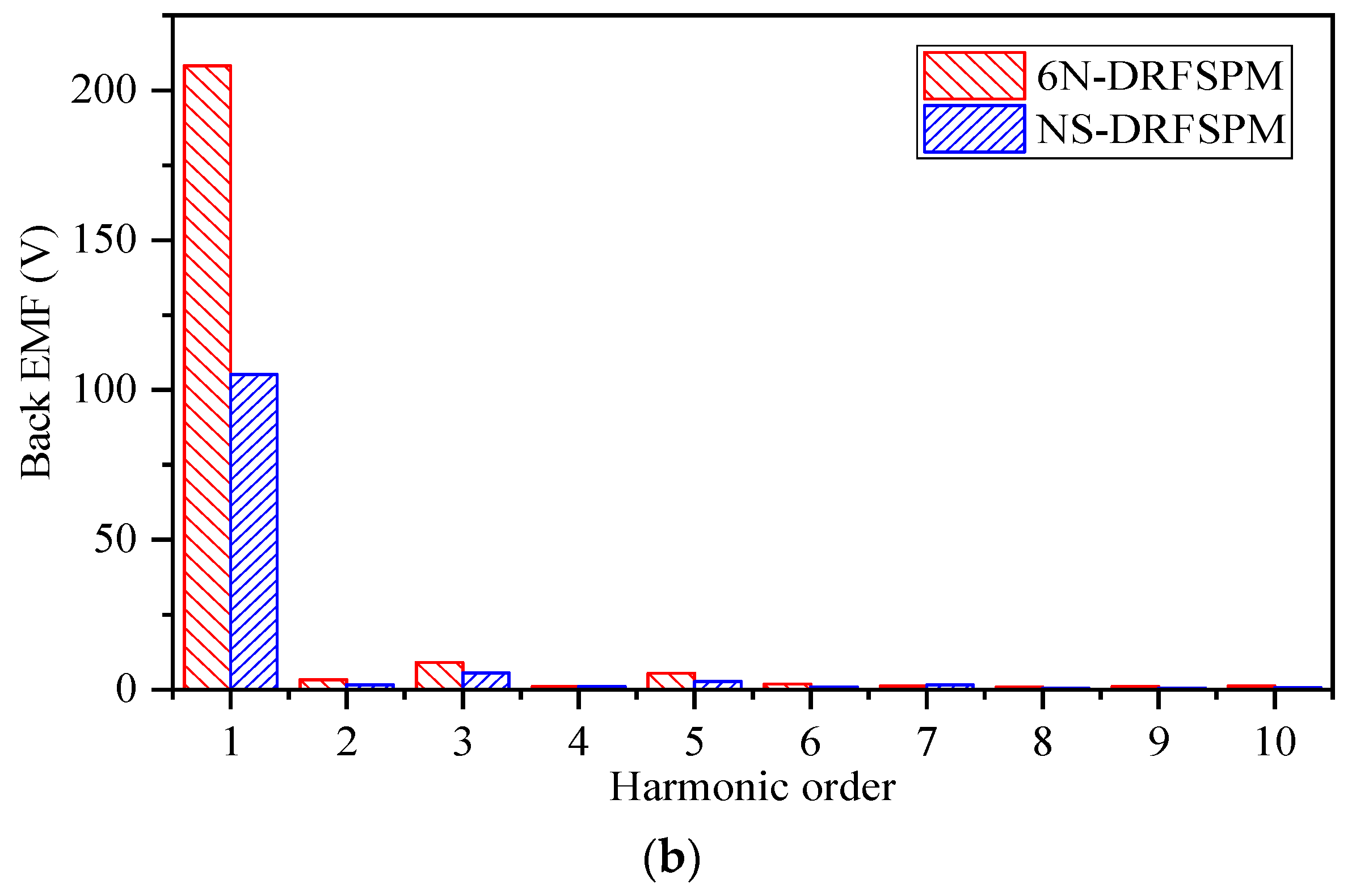
| Items | Harmonic Order | (m, n) |
|---|---|---|
| 6N-DRFSPM inner air gap | m = 0, 1, 2, … n = 1, 2, 3, … | |
| 6N-DRFSPM outer air gap | m = 0, 1, 2, … n = 1, 2, 3, … | |
| NS-DRFSPM inner air gap | m = 0, 1, 2, … n = 1, 2, 3, … | |
| NS-DRFSPM outer air gap | m = 0, 1, 2, … n = 1, 2, 3, … |
| Items | 6N-DRFSPM/NS-DRFSPM |
|---|---|
| Pole number of inner/outer rotors | 5/5 |
| Outer diameter of inner/outer rotors (mm) | 128/36.6 |
| Outer diameter of stator (mm) | 107.3 |
| Active length of inner/outer air gaps (mm) | 0.35/0.35 |
| Stack length (mm) | 75 |
| Slot package factor (kpf) | 0.45 |
| PM type | NdFeB |
| Remanent (T) | 1.2 |
| Coercivity (kA/m) | 909 |
| Rated speed (rpm) | 1500 |
| Coil number per phase | 4 |
| Number of turns per coil | 70 |
| Items | 6N-DRFSPM | NS-DRFSPM |
|---|---|---|
| Amplitude of fundamental phase back-EMF (V) | 208.2 | 105.2 |
| THD of phase back-EMF | 4.98% | 5.56% |
| Cogging torque (Nm) | 0.62 | 1.59 |
| Average torque (Nm) | 11.71 | 7.92 |
| Torque ripple | 17.2% | 28.3% |
| Copper loss (W) | 82.5 | 82.5 |
| Iron loss (W) | 37.3 | 31.4 |
| Efficiency at Jsa = 7.5 A/mm2 and 1500 r/min | 0.938 | 0.916 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, Y.; Zhao, G.; Li, Z.; Chang, Z.; Ding, S.; Zhou, Y. Electromagnetic Performance Analysis of Dual-Three-Phase Dual-Rotor Flux-Switching Permanent Magnet Machines. Energies 2024, 17, 2102. https://doi.org/10.3390/en17092102
Chen Y, Zhao G, Li Z, Chang Z, Ding S, Zhou Y. Electromagnetic Performance Analysis of Dual-Three-Phase Dual-Rotor Flux-Switching Permanent Magnet Machines. Energies. 2024; 17(9):2102. https://doi.org/10.3390/en17092102
Chicago/Turabian StyleChen, Yizhi, Guishu Zhao, Zhengliang Li, Zhe Chang, Shuye Ding, and Yuheng Zhou. 2024. "Electromagnetic Performance Analysis of Dual-Three-Phase Dual-Rotor Flux-Switching Permanent Magnet Machines" Energies 17, no. 9: 2102. https://doi.org/10.3390/en17092102
APA StyleChen, Y., Zhao, G., Li, Z., Chang, Z., Ding, S., & Zhou, Y. (2024). Electromagnetic Performance Analysis of Dual-Three-Phase Dual-Rotor Flux-Switching Permanent Magnet Machines. Energies, 17(9), 2102. https://doi.org/10.3390/en17092102








