Author Contributions
Conceptualization, P.B., E.V. and Y.A.; methodology, P.B., E.V. and Y.A.; software, P.B.; validation, P.B., E.V. and Y.A.; investigation, P.B., E.V. and Y.A.; writing—original draft preparation, P.B.; writing—review and editing, P.B., E.V. and Y.A.; supervision, E.V. and Y.A.; project administration, Y.A.; funding acquisition, Y.A. All authors have read and agreed to the published version of the manuscript.
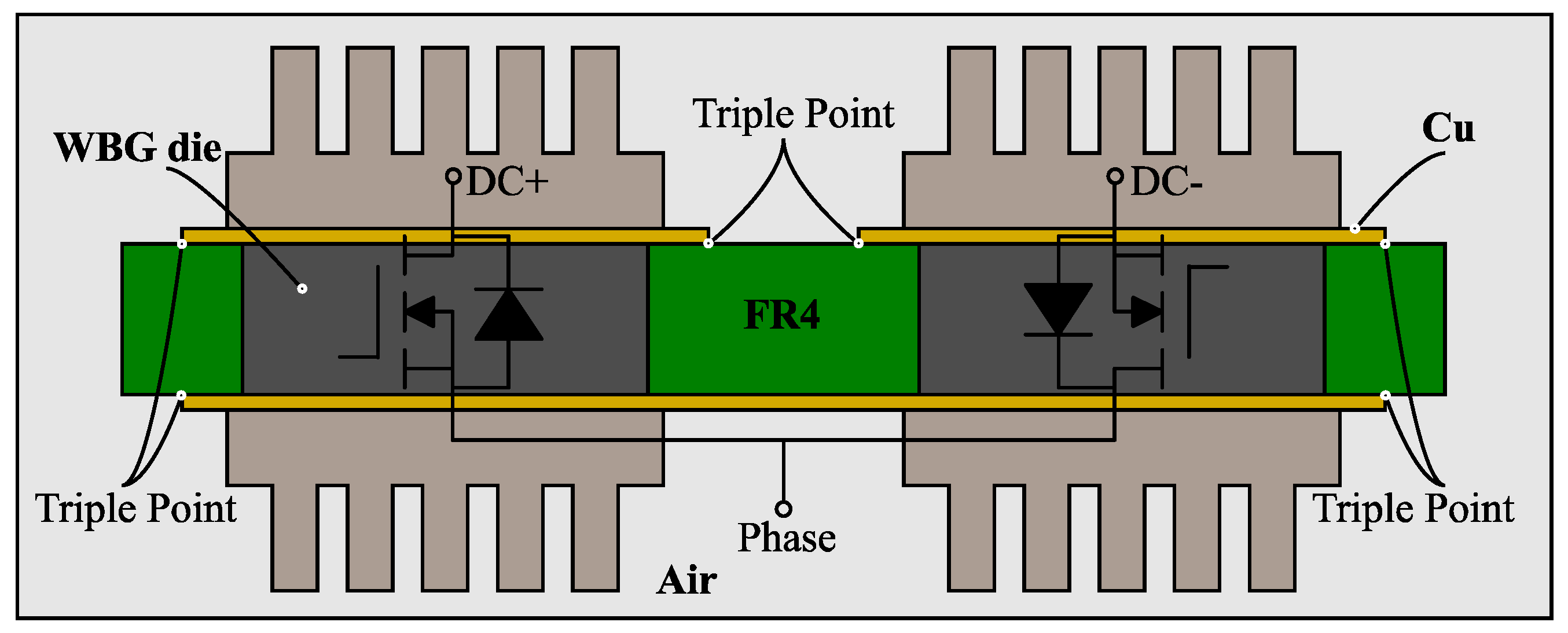
Figure 1.
PCB-embedded switching cell made of two flip-chipped WBG dies.
Figure 1.
PCB-embedded switching cell made of two flip-chipped WBG dies.
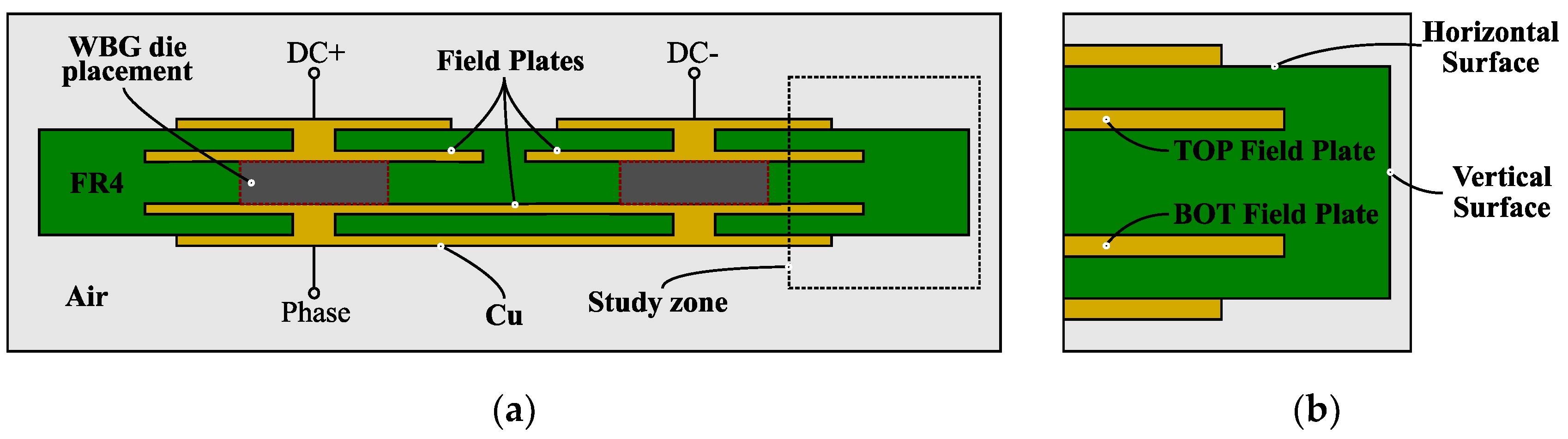
Figure 2.
(a) A field plate concept dedicated to PCB-embedded assemblies with the study zone located inside the dashed rectangle and zoomed in (b) with the representation of both horizontal and vertical surfaces.
Figure 2.
(a) A field plate concept dedicated to PCB-embedded assemblies with the study zone located inside the dashed rectangle and zoomed in (b) with the representation of both horizontal and vertical surfaces.
Figure 3.
(a) Schematic pattern of PCB geometry without FPs and (b) zoom (blue square of (a)) on the modified outer metallization edge with simulation mesh around the triple point and the dot representing point A, the electric field measurement point.
Figure 3.
(a) Schematic pattern of PCB geometry without FPs and (b) zoom (blue square of (a)) on the modified outer metallization edge with simulation mesh around the triple point and the dot representing point A, the electric field measurement point.
Figure 4.
Equipotential lines and field mapping results for PCB without FPs.
Figure 4.
Equipotential lines and field mapping results for PCB without FPs.
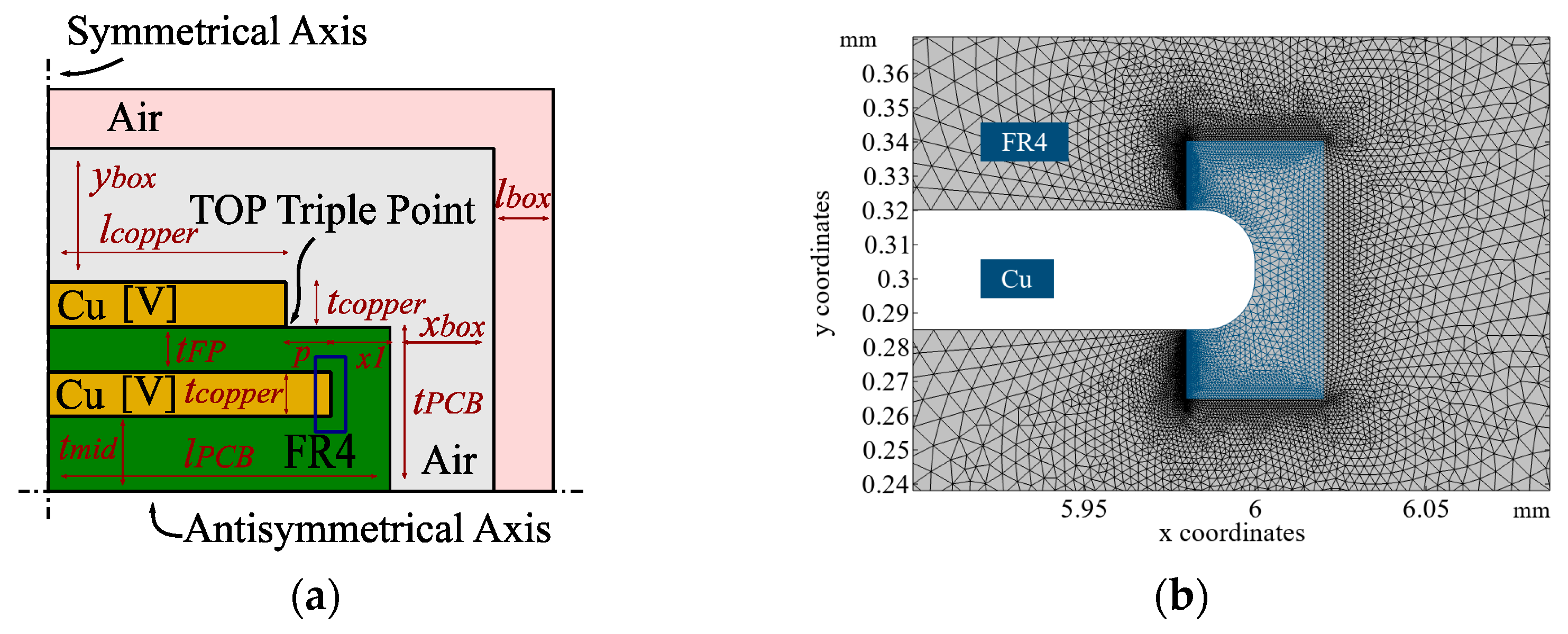
Figure 5.
(a) Schematic pattern of PCB geometry with FPs and (b) zoom (blue square of (a)) and simulation mesh around the FP edge.
Figure 5.
(a) Schematic pattern of PCB geometry with FPs and (b) zoom (blue square of (a)) and simulation mesh around the FP edge.
Figure 6.
Equipotential lines and field mapping results for PCB with FPs.
Figure 6.
Equipotential lines and field mapping results for PCB with FPs.
Figure 7.
(a) Different measurement locations: point A, H line, and V line represent, respectively, the triple point measurement point, horizontal line, and vertical line. (b) Zoom on point A.
Figure 7.
(a) Different measurement locations: point A, H line, and V line represent, respectively, the triple point measurement point, horizontal line, and vertical line. (b) Zoom on point A.
Figure 8.
Numerical simulation results of parametric study for PCB with FPs where (a) represents the simulation results with p variation and lPCB = 10 mm. (b) Simulation results with lPCB variation and p = 1 mm. Efield represents the maximum values along the measurement lines.
Figure 8.
Numerical simulation results of parametric study for PCB with FPs where (a) represents the simulation results with p variation and lPCB = 10 mm. (b) Simulation results with lPCB variation and p = 1 mm. Efield represents the maximum values along the measurement lines.
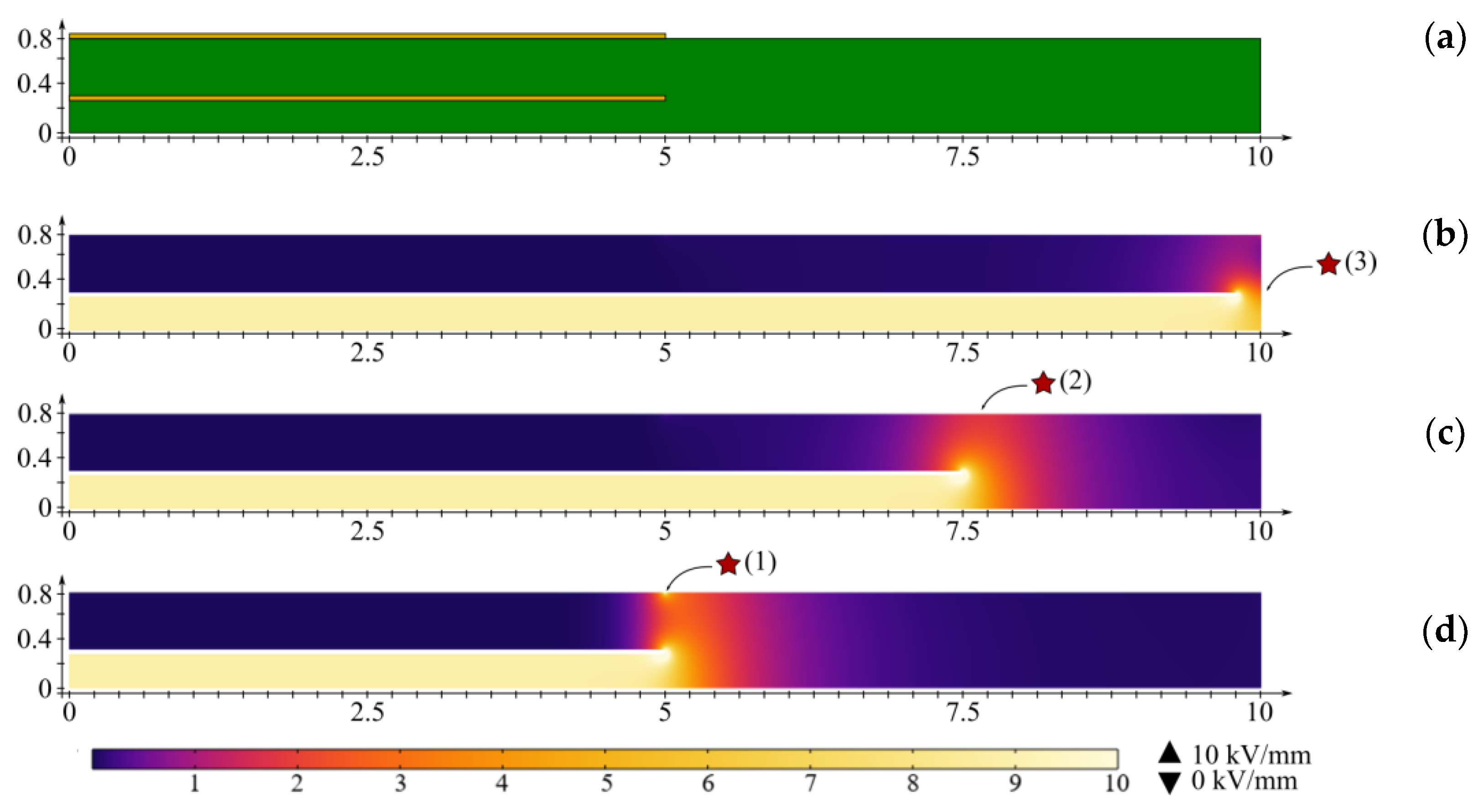
Figure 9.
Numerical simulation Efield map of PCB domain with
p variation: (
a) representation of the PCB domain, (
b) PCB Efield map of marker (3) from
Figure 8a, (
c) PCB Efield map of marker (2), and (
d) PCB Efield map of marker (1).
Figure 9.
Numerical simulation Efield map of PCB domain with
p variation: (
a) representation of the PCB domain, (
b) PCB Efield map of marker (3) from
Figure 8a, (
c) PCB Efield map of marker (2), and (
d) PCB Efield map of marker (1).
Figure 10.
Numerical simulation Efield map of PCB domain with
lPCB variation: (
a) representation of the PCB domain, (
b) PCB Efield map of marker (4) from
Figure 8b, and (
c) PCB Efield map of marker (5).
Figure 10.
Numerical simulation Efield map of PCB domain with
lPCB variation: (
a) representation of the PCB domain, (
b) PCB Efield map of marker (4) from
Figure 8b, and (
c) PCB Efield map of marker (5).
Figure 11.
Synoptic pattern of different PCB configurations with (a) design A, (b) design B, (c) design C, (d) design D, (e) design E, (f) design F, and (g) top view of PCB configurations with FPs where the solid black rectangle represents the edges of the FPs (dimensions in mm).
Figure 11.
Synoptic pattern of different PCB configurations with (a) design A, (b) design B, (c) design C, (d) design D, (e) design E, (f) design F, and (g) top view of PCB configurations with FPs where the solid black rectangle represents the edges of the FPs (dimensions in mm).
Figure 12.
Synoptic pattern of (a) test vessel for PD characterization and (b) AC sinus 50 Hz PD test bench.
Figure 12.
Synoptic pattern of (a) test vessel for PD characterization and (b) AC sinus 50 Hz PD test bench.
Figure 13.
Mean AC PDIV experimental results of each specimen from all configurations: lPCB is the same for designs A, B, C, and D while p is variable, and p is the same for designs C, E, and F while lPCB is variable.
Figure 13.
Mean AC PDIV experimental results of each specimen from all configurations: lPCB is the same for designs A, B, C, and D while p is variable, and p is the same for designs C, E, and F while lPCB is variable.
Figure 14.
Global mean AC PDIV results of all configurations in relation to (a) p parameter and (b) x1 parameter.
Figure 14.
Global mean AC PDIV results of all configurations in relation to (a) p parameter and (b) x1 parameter.
Figure 15.
AC sinus 50 Hz RMS value protocol for PD activity characterization.
Figure 15.
AC sinus 50 Hz RMS value protocol for PD activity characterization.
Figure 16.
(a) Classical PRPD result of PD activity characterization (design A specimen 1), (b) PRPD result of design A with noise band suppression at 10 pC, (c) PRPD result of design B with noise band suppression at 10 pC, and (d) PRPD result of design E with noise band suppression at 80 pC.
Figure 16.
(a) Classical PRPD result of PD activity characterization (design A specimen 1), (b) PRPD result of design A with noise band suppression at 10 pC, (c) PRPD result of design B with noise band suppression at 10 pC, and (d) PRPD result of design E with noise band suppression at 80 pC.
Figure 17.
PD activity quantities global mean results for designs A, B, and E and associated standard deviations.
Figure 17.
PD activity quantities global mean results for designs A, B, and E and associated standard deviations.
Figure 18.
Synoptic pattern of AC sinus PD localization experimental test bench used in [
22]. High voltage applied to the device under test (DUT) is generated by an arbitrary waveform generator (AWG), a low-voltage (LV) alimentation, and a high-voltage (HV) transformer.
Figure 18.
Synoptic pattern of AC sinus PD localization experimental test bench used in [
22]. High voltage applied to the device under test (DUT) is generated by an arbitrary waveform generator (AWG), a low-voltage (LV) alimentation, and a high-voltage (HV) transformer.
Figure 19.
AC sinus 50 Hz PD localization experimental results with (a) focus of design A, (b) focus of design B, (c) focus of design E, (d) PD localization picture of design A at 3.9 kV, (e) PD localization picture of design B at 4.6 kV, and (f) PD localization picture of design E at 7.6 kV. All red solid lines represent the PCB edges and dashed blue lines the inner edges of the outer metallization.
Figure 19.
AC sinus 50 Hz PD localization experimental results with (a) focus of design A, (b) focus of design B, (c) focus of design E, (d) PD localization picture of design A at 3.9 kV, (e) PD localization picture of design B at 4.6 kV, and (f) PD localization picture of design E at 7.6 kV. All red solid lines represent the PCB edges and dashed blue lines the inner edges of the outer metallization.
Table 1.
Geometrical dimensions and physical parameters of numerical simulations.
Table 1.
Geometrical dimensions and physical parameters of numerical simulations.
| Parameter | Value | Description |
|---|
| lPCB | 10 mm | PCB length |
| tPCB | 0.8 mm | PCB thickness |
| lcopper | 5 mm | Metallization length |
| tcopper | 35 µm | Metallization thickness |
| p | 1 mm | Equipotential prolongation |
| tFP | 480 µm | FP depth |
| tmid | 570 µm | Distance between both FPs |
| lbox | 1 mm | Infinite box length |
| xbox | 10 mm | x offset infinite box |
| ybox | 6.7 mm | y offset infinite box |
| V | 5 kV | High voltage value |
| εrFR4 | 4.5 | FR4 relative permittivity |
| εrCu | 1 | Cu relative permittivity |
| εrAir | 1.0006 | Air relative permittivity |
Table 2.
Different interesting cases deduced from numerical simulation parametric studies of PCBs with FPs. The different design parameters and maximum Efield results and localizations are also depicted.
Table 3.
Geometrical dimensions of the PCBs.
Table 3.
Geometrical dimensions of the PCBs.
| Design | A | B | C | D | E | F |
|---|
| lPCB (mm) | 20 | 20 | 20 | 20 | 16 | 17 |
| LPCB (mm) | 50 | 50 | 50 | 50 | 46 | 47 |
| tFP (µm) | - | 480 | 480 | 480 | 480 | 480 |
| tmid (µm) | - | 570 | 570 | 570 | 570 | 570 |
| p (mm) | - | 0 | 2.5 | 4 | 2.5 | 2.5 |
| x1 (mm) | - | 5 | 2.5 | 1 | 0.5 | 1 |
Table 4.
Maximum and minimum PDIV results of each configuration and associated standard deviations. ΔPDIV represents the difference between PDIVmax and PDIVmin.
Table 4.
Maximum and minimum PDIV results of each configuration and associated standard deviations. ΔPDIV represents the difference between PDIVmax and PDIVmin.
| Design | PDIVmin (kV) | σ (kV) | PDIVmax (kV) | σ (kV) | ΔPDIV (kV) |
|---|
| A | 2.84 | 0.0879 | 3.21 | 0.170 | 0.37 |
| B | 2.82 | 0.0953 | 3.10 | 0.0783 | 0.28 |
| C | 8.26 | 1.10 | 8.70 | 0.270 | 0.44 |
| D | 6.68 | 0.300 | 8.63 | 0.388 | 1.95 |
| E | 5.17 | 0.250 | 5.67 | 0.228 | 0.5 |
| F | 6.02 | 0.177 | 7.37 | 0.169 | 1.35 |
Table 5.
Global PDIV experimental results and computing of deviations in relation to p parameter.
Table 5.
Global PDIV experimental results and computing of deviations in relation to p parameter.
| Design | p (mm) | Global PDIV (kV) | Differences |
|---|
| A | - | 3.05 | REF |
| B | 0 | 2.94 | −3.61% |
| C | 2.5 | 8.48 | +178% |
| D | 4 | 7.86 | +158% |
| E | 2.5 | 5.40 | +77% |
| F | 2.5 | 6.71 | +120% |
Table 6.
Global PDIV experimental results and computing of deviations in relation to x1 parameter.
Table 6.
Global PDIV experimental results and computing of deviations in relation to x1 parameter.
| Design | x1 (mm) | Global PDIV (kV) | Differences |
|---|
| E | 0.5 | 5.40 | REF |
| F | 1 | 6.71 | +24% |
| D | 1 | 7.86 | +46% |
| C | 2.5 | 8.48 | +57% |
| B | 5 | 2.94 | −46% |
Table 7.
Global mean PD activity quantities results and comparison.
Table 7.
Global mean PD activity quantities results and comparison.
| Quantities | Design A | Design B | Design E | B/A | E/A |
|---|
| Qmax (nC) | 1.39 | 0.909 | 8.37 | 0.65 | 6.02 |
| Qavg (nC) | 0.354 | 0.300 | 2.76 | 0.85 | 7.80 |
| N (kPD/s) | 0.250 | 0.465 | 0.855 | 1.86 | 3.42 |
| PDEV (kV) | 2.45 | 2.21 | 4.14 | 0.90 | 1.69 |
| PDIV (kV) | 3.05 | 2.94 | 5.40 | 0.96 | 1.77 |
| PDIV/PDEV | 1.24 | 1.33 | 1.30 | - | - |
Table 8.
HV values applied during PD localization characterization and localization at every light spot with different results. Global PDIV from PDIV characterization and maximum Efield location in numerical simulation allow for comparison of every result.
Table 8.
HV values applied during PD localization characterization and localization at every light spot with different results. Global PDIV from PDIV characterization and maximum Efield location in numerical simulation allow for comparison of every result.
| Designs | HV Value (kV) | Global PDIV (kV) | Experimental PD Localization | Numerical Maximum Efield Location |
|---|
| A | 3.9 | 3.05 | Outer metallization corner | Point A |
| B | 4.6 | 2.94 | Outer metallization corners | Point A |
| E | 7.6 | 5.4 | PCB edge | V line |