Heat Transfer and Flow Resistance in Crossflow over Corrugated Tube Banks
Abstract
1. Introduction
2. Numerical Model
2.1. Description of the Tube Geometry
2.2. Governing Equations and Data Reduction
2.3. Boundary Conditions and Numerical Approaches
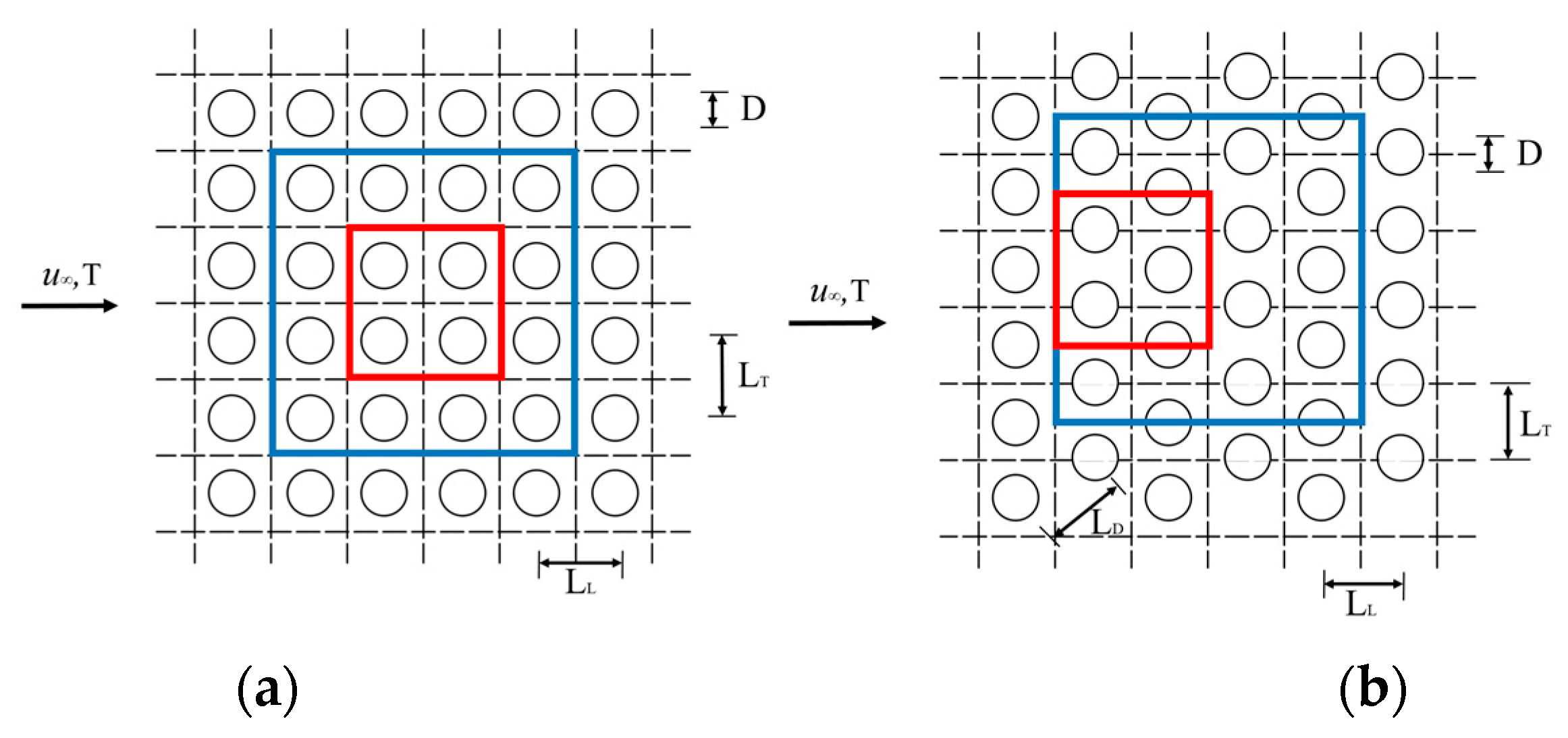
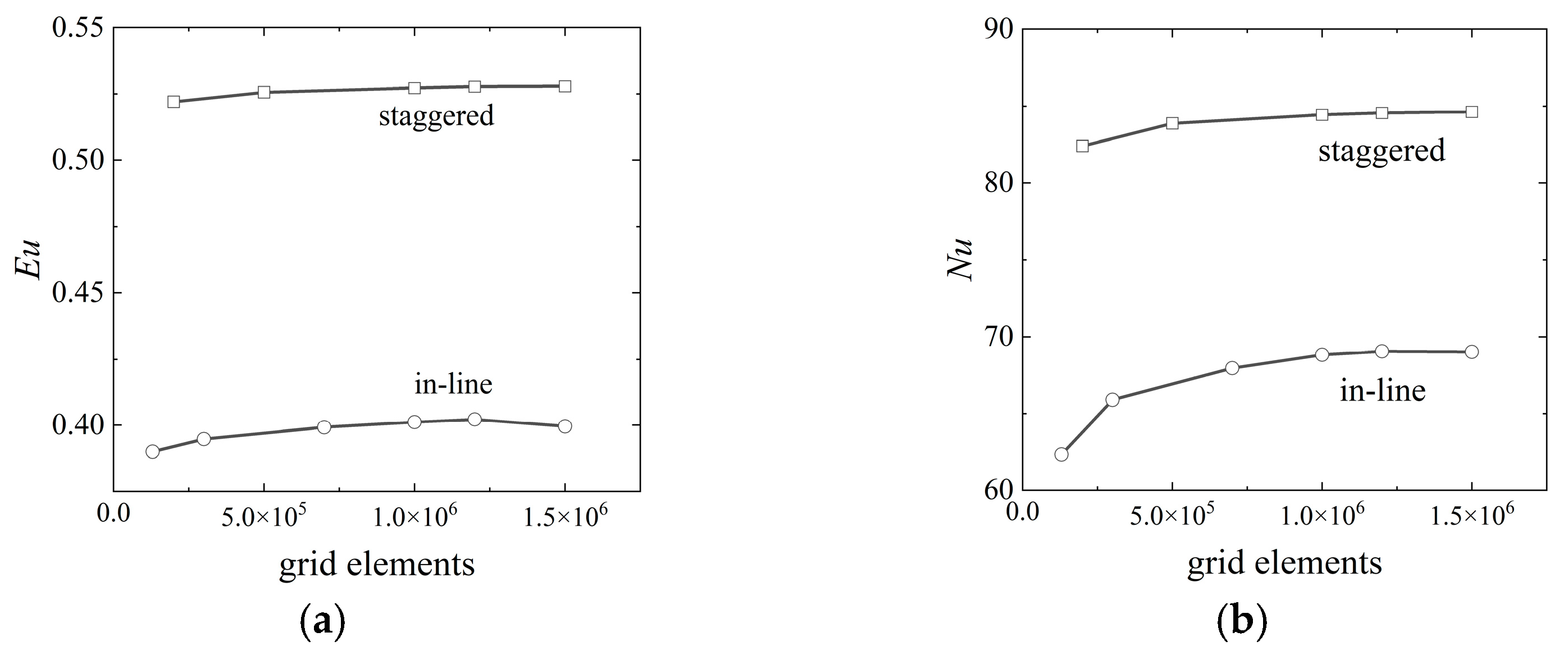
2.4. Computational Domain and Grid Dependency Analysis
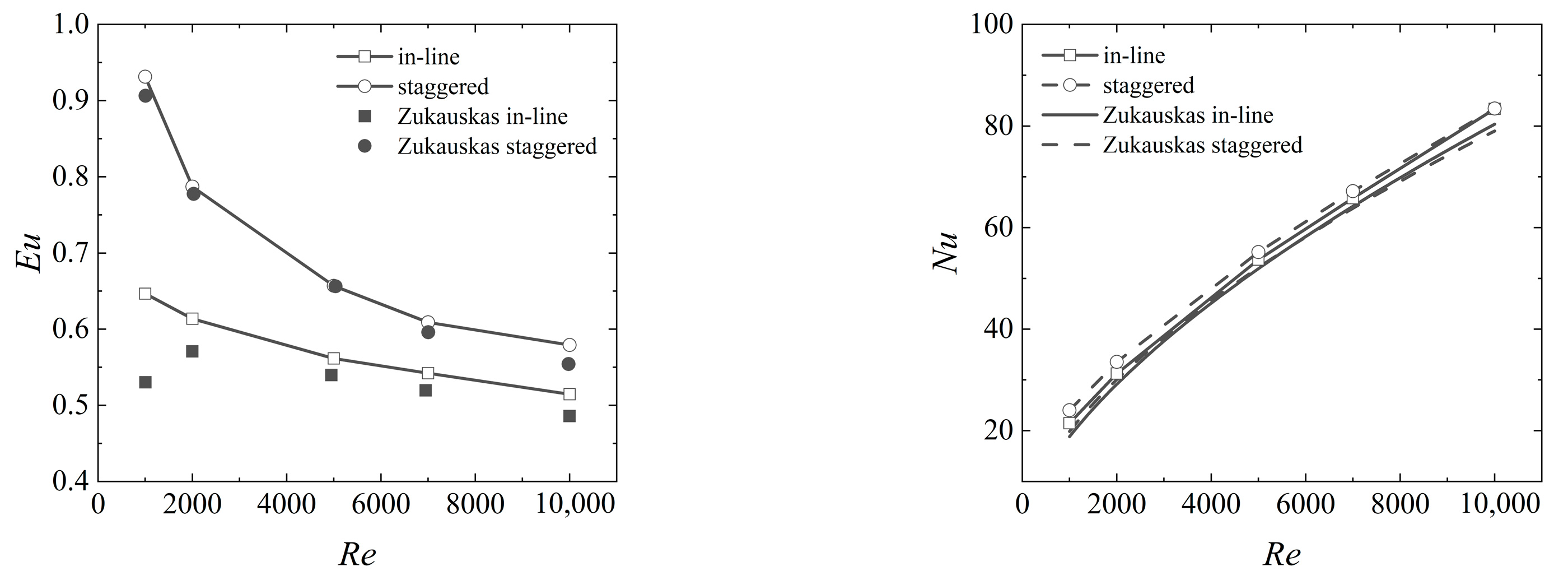
2.5. Validation of Numerical Simulations
3. Results and Discussion
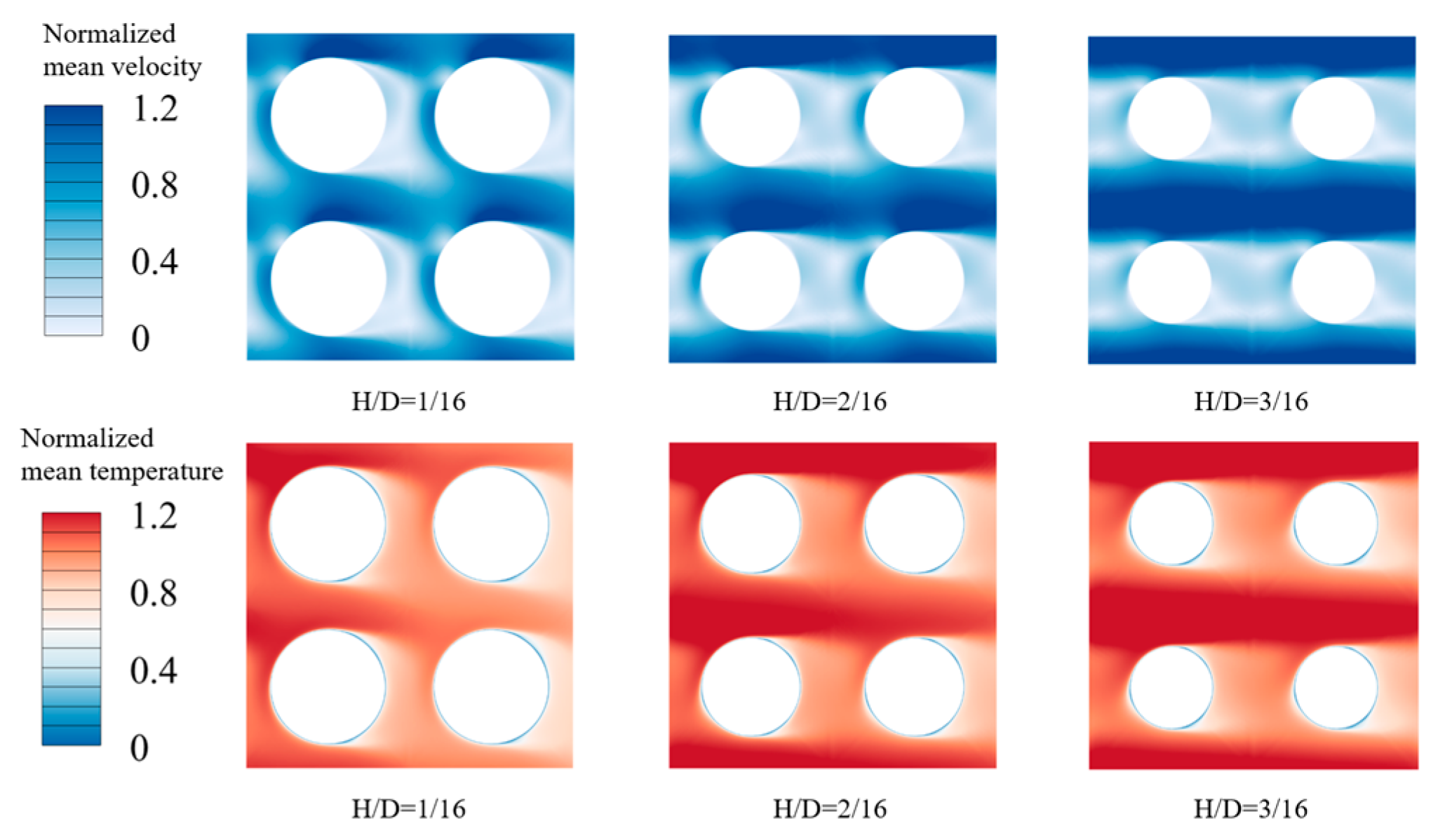
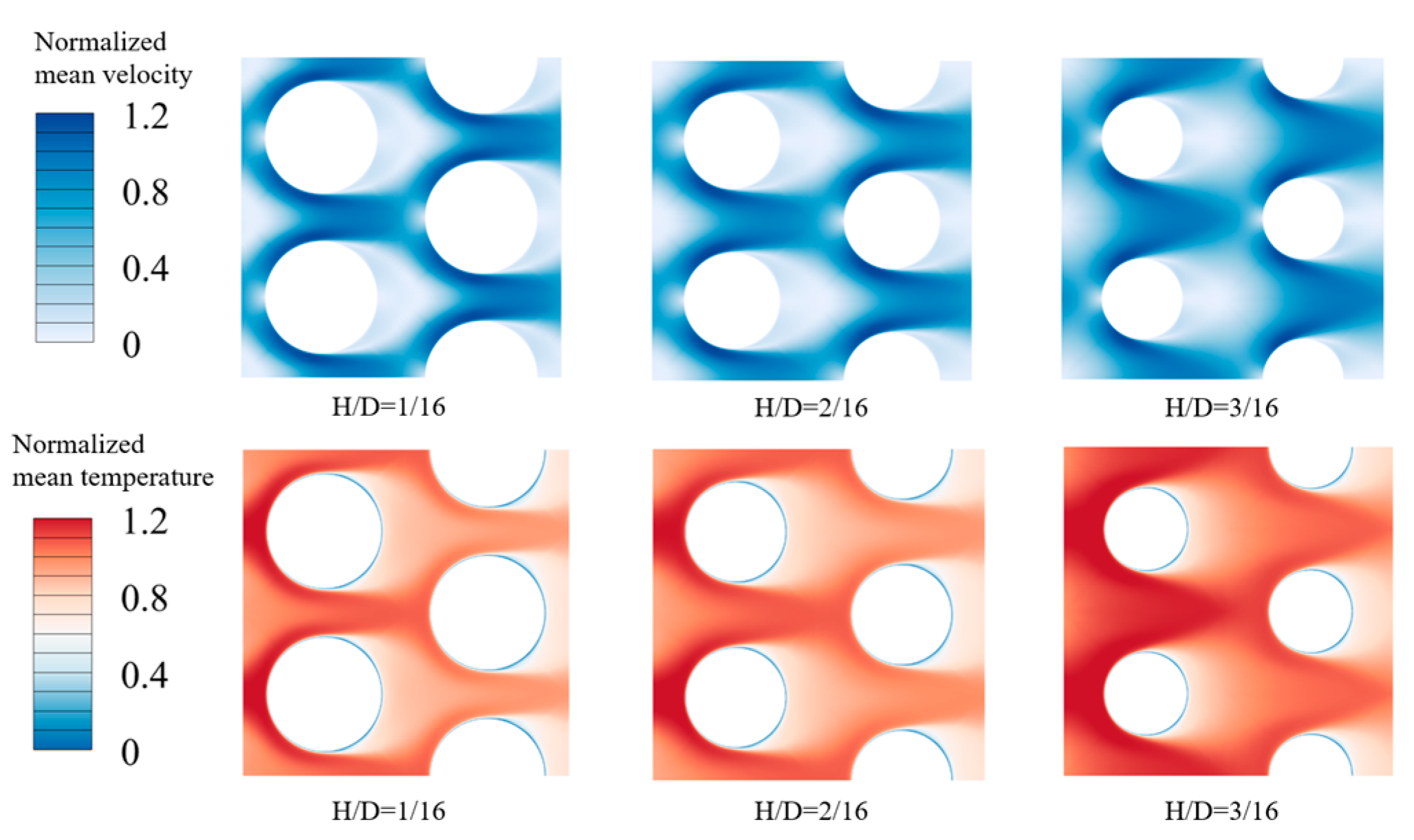
3.1. PIC Tube Banks in In-Line Arrangement
3.2. PIC Tube Banks in Staggered Arrangement
3.3. Global Performance Comparsion of PIC Tube Banks
4. Conclusions
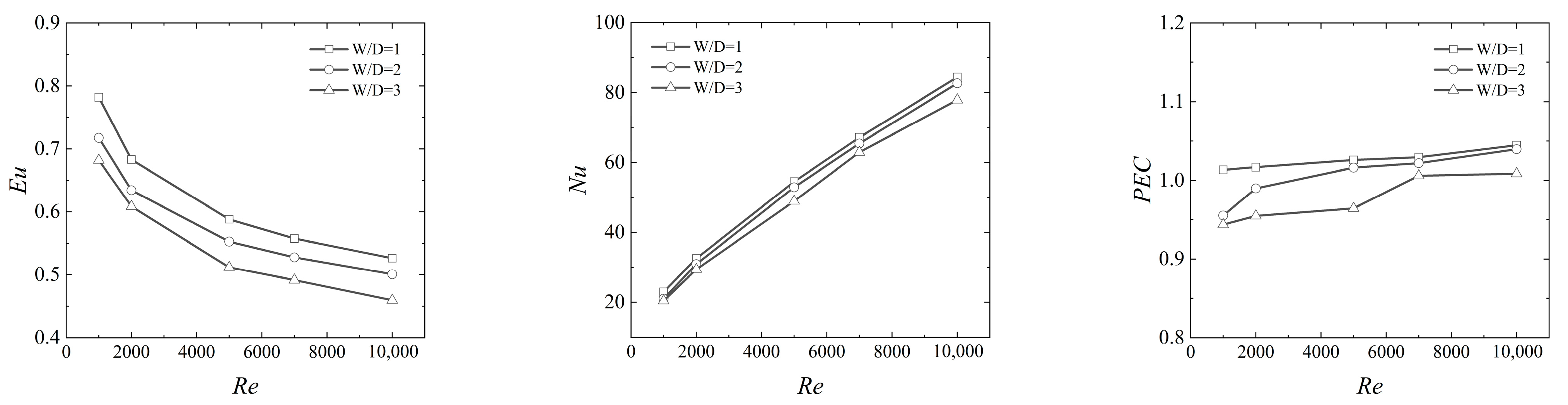
- In an in-line configuration, PIC tubes demonstrate a significant decrease in both pressure drop and heat transfer performance as the corrugation height (H/D) and width (W/D) increase. The Euler number and Nusselt number for PIC tube banks can drop to half those of smooth circular tube banks. An increase in corrugation pitch (P/D) does elevate the pressure drop but diminishes the heat transfer coefficient. Overall, the thermal–hydraulic performance of PIC tube banks is inferior to that of SC tube banks.
- When comparing PIC tube banks with SC tube banks in a staggered arrangement, the global Nusselt number (Nu) remains relatively unchanged, but there is a notable reduction in the Euler number (Eu), leading to a favorable performance evaluation criterion (PEC). Changes in W/D and P/D affect the heat transfer coefficient and pressure drop, but their impact is less pronounced than in the in-line arrangement.
- Our analysis indicates that for enhancing performance in heat exchangers using PIC tubes, a staggered arrangement of tube banks is preferable over an in-line arrangement.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| A | The area of the tube banks, m2 |
| Amin | The minimum cross-section area of the tube banks, m2 |
| cp | Specific heat, J/kg·K |
| D | Tube diameter, m |
| Eu | Euler number |
| H | Corrugation height, m |
| h | Heat transfer coefficient, W/m2·K |
| LT | Transverse spacing |
| LL | Longitudinal spacing |
| Nu | Nusselt number |
| P | Periodic length between corrugations, m |
| PEC | Performance evaluation criteria |
| Δp | Pressure drop per unit length, Pa/m |
| Pr | Prandtl number |
| q | Heat flux, W/m2 |
| qm | Mass flow rate, kg/s |
| Re | Reynolds number |
| ST | The ratio of transverse spacing |
| SL | The ratio of longitudinal spacing |
| T | Temperature, K |
| u | Velocity, m/s |
| W | Corrugation width, m |
| x,y,z | Cartesian coordinate |
| Greek symbols | |
| μ | Viscosity, Pa·s |
| ρ | Fluid density, kg/m3 |
| λ | Thermal conductivity, W/m·K |
| Subscript | |
| in | Inlet |
| out | Outlet |
| w | Wall |
| abbreviation | |
| PIC tube | Periodically inward-corrugated tube |
| SC tube | Smooth circular tube |
References
- Kasim, K.; Muley, A.; Stoia, M.; Ladeinde, F. Advanced Heat Transfer Devices for Aerospace Applications. In Proceedings of the ASME International Mechanical Engineering Congress and Exposition, Tampa, FL, USA, 3–9 November 2017; American Society of Mechanical Engineers: New York, NY, USA, 2017; p. V008T010A027. [Google Scholar]
- Carozza, A. Heat Exchangers in the Aviation Engineering, Heat Exchangers—Advanced Features and Applications; IntechOpen: London, UK, 2017; pp. 149–166. [Google Scholar]
- Konstantinidis, E.; Castiglia, D.; Balabani, S. An experimental study of steady and pulsating cross-flow over a semi-staggered tube bundle. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2005, 219, 283–298. [Google Scholar] [CrossRef]
- Grimison, E.D. Correlation and utilization of new data on flow resistance and heat transfer for cross flow of gases over tube banks. J. Fluids Eng. 1937, 59, 583–594. [Google Scholar] [CrossRef]
- Žkauskas, A. Heat transfer from tubes in crossflow. In Advances in Heat Transfer; Elsevier: Amsterdam, The Netherlands, 1987; pp. 87–159. [Google Scholar]
- Khan, W.; Culham, J.; Yovanovich, M. Convection heat transfer from tube banks in crossflow: Analytical approach. Int. J. Heat Mass Transf. 2006, 49, 4831–4838. [Google Scholar] [CrossRef]
- Wilson, A.S.; Bassiouny, M.K. Modeling of heat transfer for flow across tube banks. Chem. Eng. Process. Process Intensif. 2000, 39, 1–14. [Google Scholar] [CrossRef]
- El-Shaboury, A.M.F.; Ormiston, S.J. Analysis of laminar forced convection of air crossflow in in-line tube banks with nonsquare arrangements. Numer. Heat Transf. Part A Appl. 2005, 48, 99–126. [Google Scholar] [CrossRef]
- Zhang, L.-Z.; Ouyang, Y.-W.; Zhang, Z.-G.; Wang, S.-F. Oblique fluid flow and convective heat transfer across a tube bank under uniform wall heat flux boundary conditions. Int. J. Heat Mass Transf. 2015, 91, 1259–1272. [Google Scholar] [CrossRef]
- Mangrulkar, C.K.; Dhoble, A.S.; Chamoli, S.; Gupta, A.; Gawande, V.B. Recent advancement in heat transfer and fluid flow characteristics in cross flow heat exchangers. Renew. Sustain. Energy Rev. 2019, 113, 109220. [Google Scholar] [CrossRef]
- Bahaidarah, H.M.S.; Anand, N.K.; Chen, H.C. A Numerical Study of Fluid Flow and Heat Transfer over a Bank of Flat Tubes. Numer. Heat Transf. Part A Appl. 2005, 48, 359–385. [Google Scholar] [CrossRef]
- Horvat, A.; Mavko, B. Heat transfer conditions in flow across a bundle of cylindrical and ellipsoidal tubes. Numer. Heat Transf. Part A Appl. 2006, 49, 699–715. [Google Scholar] [CrossRef]
- Ibrahim, T.A.; Gomaa, A. Thermal performance criteria of elliptic tube bundle in crossflow. Int. J. Therm. Sci. 2009, 48, 2148–2158. [Google Scholar] [CrossRef]
- Terukazu, O.; Hideya, N.; Yukiyasu, T. Heat transfer and flow around an elliptic cylinder. Int. J. Heat Mass Transf. 1984, 27, 1771–1779. [Google Scholar] [CrossRef]
- Merker, G.; Hanke, H. Heat transfer and pressure drop on the shell-side of tube-banks having oval-shaped tubes. Int. J. Heat Mass Transf. 1986, 29, 1903–1909. [Google Scholar] [CrossRef]
- Tang, S.; Ding, L.; Sheng, R.; Zhao, K.; Zhang, D.; Shen, B. Parametric analysis on thermal-hydraulic characteristics in variable-direction twisted-oval tube bundle in cross-flow. Int. J. Therm. Sci. 2024, 197, 108761. [Google Scholar] [CrossRef]
- Li, X.; Zhu, D.; Yin, Y.; Liu, S.; Mo, X. Experimental study on heat transfer and pressure drop of twisted oval tube bundle in cross flow. Exp. Therm. Fluid Sci. 2018, 99, 251–258. [Google Scholar] [CrossRef]
- Sparrow, E.; Kang, S. Longitudinally-finned cross-flow tube banks and their heat transfer and pressure drop characteristics. Int. J. Heat Mass Transf. 1985, 28, 339–350. [Google Scholar] [CrossRef]
- Lemouedda, A.; Schmid, A.; Franz, E.; Breuer, M.; Delgado, A. Numerical investigations for the optimization of serrated finned-tube heat exchangers. Appl. Therm. Eng. 2011, 31, 1393–1401. [Google Scholar] [CrossRef]
- Zukauskas, A.; Ulinskas, R. Heat transfer in tube banks in crossflow. Adv. Heat Transf. 1988, 8, 93–160. [Google Scholar]
- Menter, F.R. Two-equation eddy-viscosity turbulence models for engineering applications. AIAA J. 1994, 32, 1598–1605. [Google Scholar] [CrossRef]
- Kim, T. Effect of longitudinal pitch on convective heat transfer in crossflow over in-line tube banks. Ann. Nucl. Energy 2013, 57, 209–215. [Google Scholar] [CrossRef]
- Jiang, H.; Niu, Y.; Yang, P.; Liu, Y. Effect of pulsation parameters on the spatial and temporal variation of flow and heat transfer characteristics in liquid metal cross flow the in-line tube bundle. Int. J. Heat Mass Transf. 2024, 219, 124871. [Google Scholar] [CrossRef]
- Incropera, F.P.; DeWitt, D.P.; Bergman, T.L.; Lavine, A.S. Fundamentals of Heat and Mass Transfer; Wiley: New York, NY, USA, 1996. [Google Scholar]
- Webb, R.L. Performance evaluation criteria for use of enhanced heat transfer surfaces in heat exchanger design. Int. J. Heat Mass Transf. 1981, 24, 715–726. [Google Scholar] [CrossRef]
- Yadav, N.; Gepner, S.W.; Szumbarski, J. Instability in a channel with grooves parallel to the flow. Phys. Fluids 2017, 29, 084104. [Google Scholar] [CrossRef]
- Harikrishnan, S.; Tiwari, S. Unsteady flow and heat transfer characteristics of primary and secondary corrugated. J. Heat Transf. 2020, 142, 031803. [Google Scholar] [CrossRef]



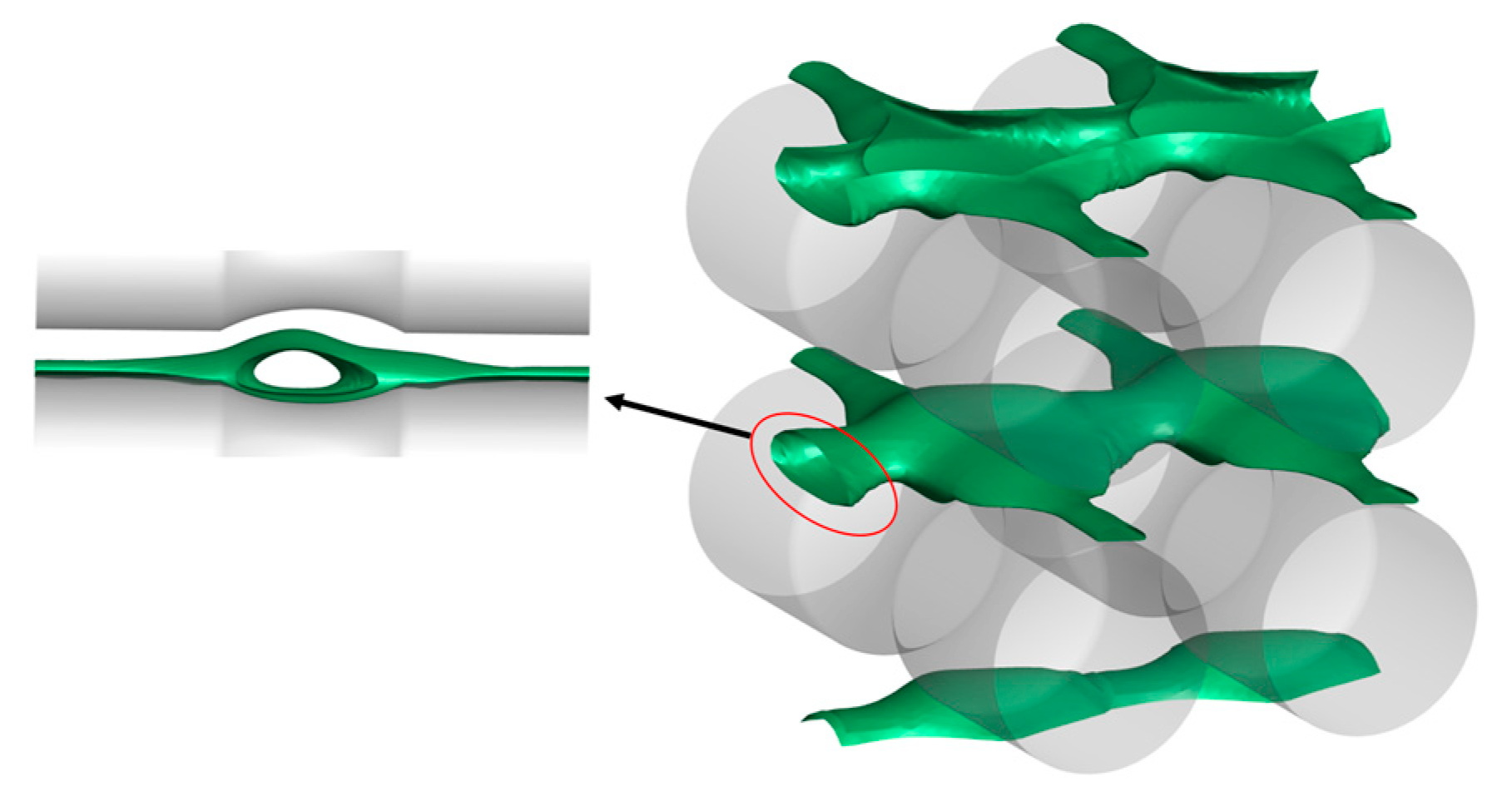
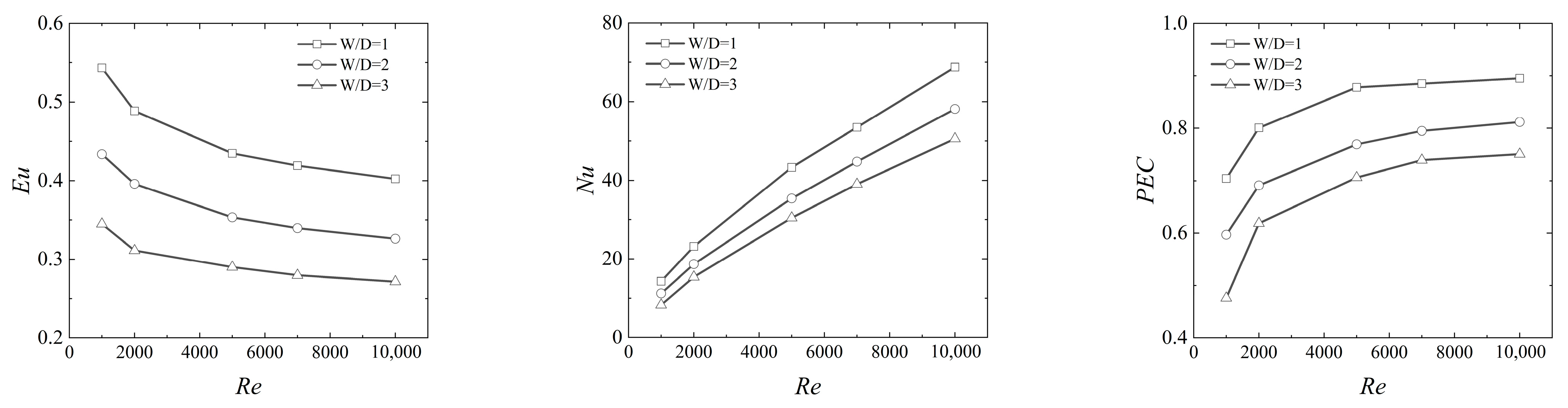

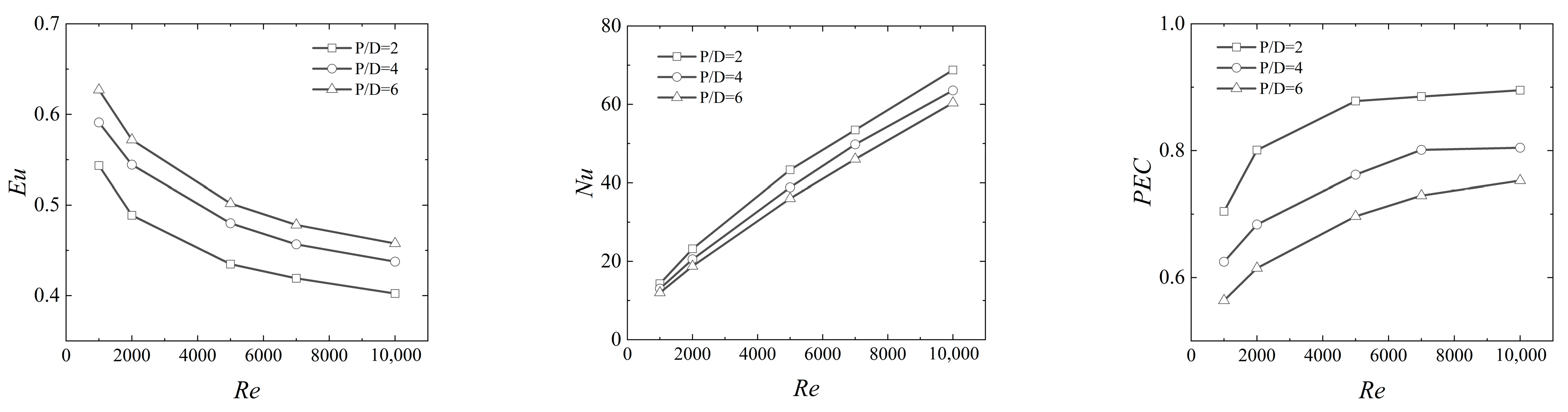
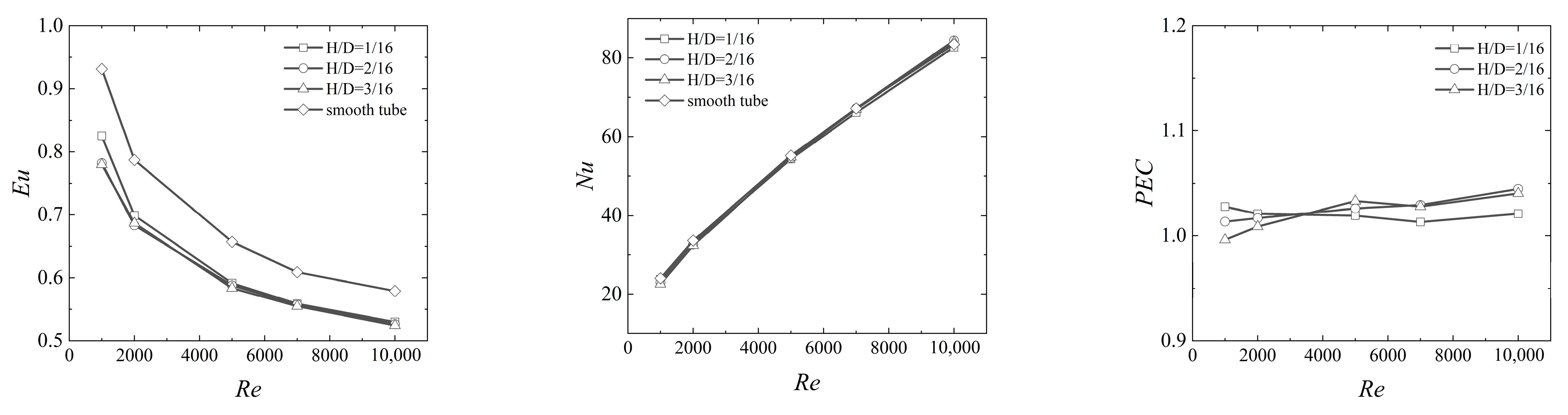


| Case | H/D | W/D | P/D |
|---|---|---|---|
| 1 | 1/16 | 1 | 2 |
| 2 | 2/16 | 1 | 2 |
| 3 | 3/16 | 1 | 2 |
| 4 | 2/16 | 2 | 2 |
| 5 | 2/16 | 3 | 2 |
| 6 | 2/16 | 1 | 4 |
| 7 | 2/16 | 1 | 6 |
| Computed Cases | Eu | Nu |
|---|---|---|
| In-line arrangement 2 × 2 | 0.402 | 68.78 |
| In-line arrangement 4 × 4 | 0.403 | 69.11 |
| Staggered arrangement 2 × 2 | 0.526 | 84.44 |
| Staggered arrangement 4 × 4 | 0.527 | 85.27 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhong, Y.; Zhao, J.; Zhao, L.; Gao, G.; Zhu, X. Heat Transfer and Flow Resistance in Crossflow over Corrugated Tube Banks. Energies 2024, 17, 1641. https://doi.org/10.3390/en17071641
Zhong Y, Zhao J, Zhao L, Gao G, Zhu X. Heat Transfer and Flow Resistance in Crossflow over Corrugated Tube Banks. Energies. 2024; 17(7):1641. https://doi.org/10.3390/en17071641
Chicago/Turabian StyleZhong, Yuzhou, Jingquan Zhao, Lei Zhao, Ge Gao, and Xiaowei Zhu. 2024. "Heat Transfer and Flow Resistance in Crossflow over Corrugated Tube Banks" Energies 17, no. 7: 1641. https://doi.org/10.3390/en17071641
APA StyleZhong, Y., Zhao, J., Zhao, L., Gao, G., & Zhu, X. (2024). Heat Transfer and Flow Resistance in Crossflow over Corrugated Tube Banks. Energies, 17(7), 1641. https://doi.org/10.3390/en17071641






