A Review on Micro-Combustion Flame Dynamics and Micro-Propulsion Systems
Abstract
1. Introduction
2. Definition of Micro-Scale Combustion
- Definition based on Physical length: A generally used characteristic to distinguish between micro-scale and meso-scale combustion is that, when the length scale of the combustor is smaller than 1 mm, the combustion is referred to as micro-combustion. On the other hand, if the physical length scale is greater than 1 mm but around 1 cm, the combustion is referred to as mesoscale combustion, which is usually utilized in the development of micro-engines [7];
- Definition based on the Flame quenching diameter: consists of using the quenching diameter as the largest diameter of a cylindrical tube that will just quench (extinguish) the flame front of a particular fuel–air mixture [8], which is a function of the mixture’s composition and the wall properties (temperature/surface reactivity) [1], and the reference length scale for the flame [9]. Consequently, if the combustor dimensions are smaller (larger) than the quenching diameter, the combustion is referred to as micro-scale (mesoscale). This definition is more sensible in terms of the physical flame regimes and is preferred by researchers for studying the fundamental aspects of micro-combustion;
- Definition based on the Device Scale: Researchers sometimes use the relative size of a device for its intended purpose compared to conventional large-scale devices as a means of defining meso and micro-scale combustion. This approach offers a third way of defining these types of combustion (often utilized by researchers who aim to design micro-thrusters for particular applications).
3. Challenges within Microcombustion
- Short residence time: Micro-combustion, with its small chamber volume, is limited by short residence times due to diffusion and reaction times [16]. For example, the reaction time for hydrogen or hydrocarbon species with air or oxygen is typically 0.1 to 1 ms [17], aligning with small-scale conditions [18]. The Damköhler number (Da), which relates residence time to combustion time, assesses fuel combustion completeness [19,20]. Da is defined as . When Da is less than 1, extinction will occur via incomplete combustion, which reduces combustion and power generation efficiency [21];
- High heat loss ratio: Micro-combustion poses a challenge due to increased heat losses related to high surface-to-volume ratios within the combustion chamber. To address this, understanding the causes of these losses and developing effective mitigation strategies is crucial. Heat loss has been quantified by Kang et al. [15] using the following equation:Here, represents heat loss density, is the average heat loss density, is the inner surface area of the combustor, and is the chamber volume. As the combustion chamber size decreases in the micro-scale, the surface-to-volume ratio () increases substantially, leading to amplified heat loss in the combustor. For conventional burners, is approximately 3 to 5 , while it reaches 500 in micro combustors [22], accelerating heat dissipation from the inner wall;
- The stability of micro-combustion depends on reactions, heat transfer, and the interaction between burned and unburned gases [23,24]. Factors like flow fields, temperature, and species distribution in microchannels can cause combustion instabilities such as flame blowout, oscillations, and asymmetric distribution [25,26]. High heat loss from combustor walls can lead to non-self-sustaining combustion, including thermal or radical extinction in small chambers [27]. Radical extinction occurs when active radicals are absorbed by the inner wall, hindering their reactivity. External wall heating is proposed to enhance flame stability [28]. The increased surface-to-volume ratio in micro-combustion intensifies wall heat losses and radical collisions, affecting flame extinction and micro power systems [29,30]. A review of the advancements in flame stability and residence time in micro-combustion technologies was performed by Nauman et al. [5]. This review compares different methods for improving the stability of a wide range of features in various operational settings, from the use of porous media to increasing the radiation temperature of the burner, using backflow zones formed due to optimized chamber construction encouraging flame anchoring and increasing combustion efficiency, increasing the wall temperature, and using catalytic assisted combustion to speed up the reaction time and residence time.
4. Typical Length and Time Scales
4.1. Flammability Limits in Micro-Combustion and the Quenching Diameter
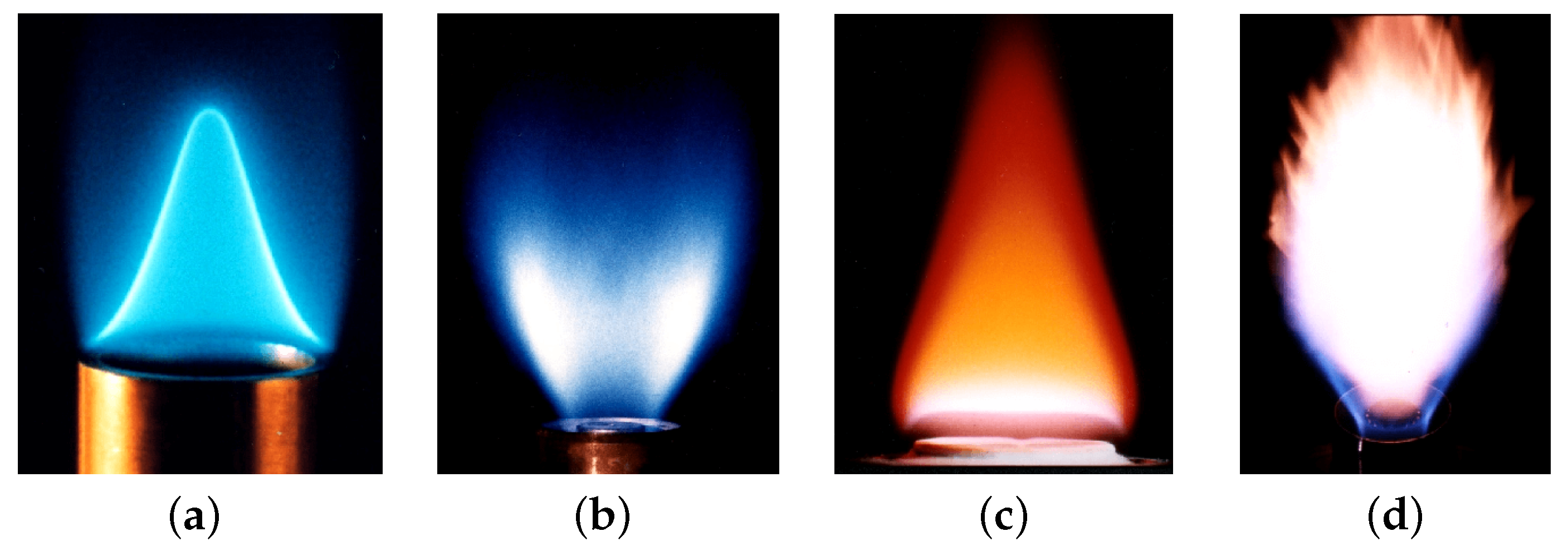
4.2. Classification of Flame Types
5. Classification of Micro-Flame Behaviours
5.1. Flashback and Blow-Off
- Flashback: This occurs when the velocity of the reactants supplied to the burner chamber is lower than the flame speed. As a result, the flame propagates upstream towards the fuel injection system, burning the reactants in a zone increasingly upstream, possibly self-extinguishing (Combustion instability-induced flashback is connected to the phenomenon of the instantaneous axial flow velocity experiencing significant reductions, sometimes even reaching negative values, during large-amplitude oscillations [43]).
- Blow-off: In this scenario, the reactants’ supply velocity is higher than the flame speed. The flame moves along the channel, burning the reactants in a zone increasingly downstream until it “blows off” (possibly self-extinguishing) when the fuel flow rate exceeds a certain limit.

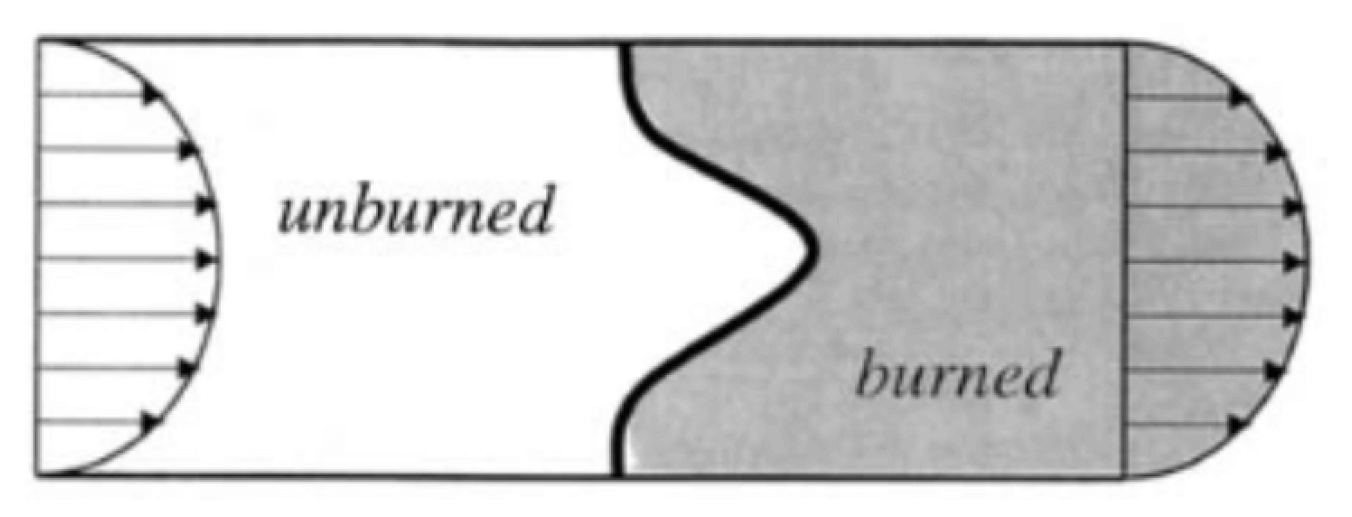
5.2. Steady vs. Non-Steady Flames
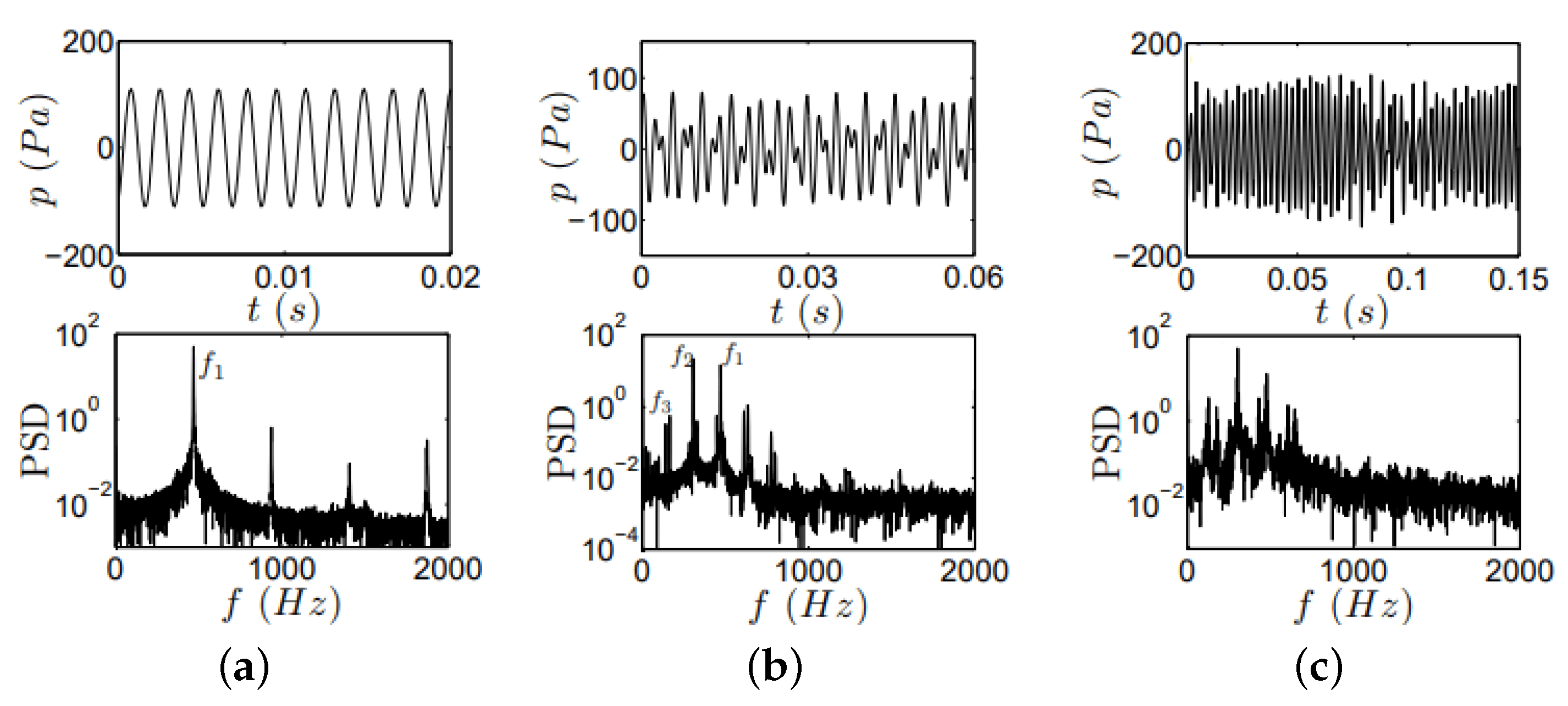
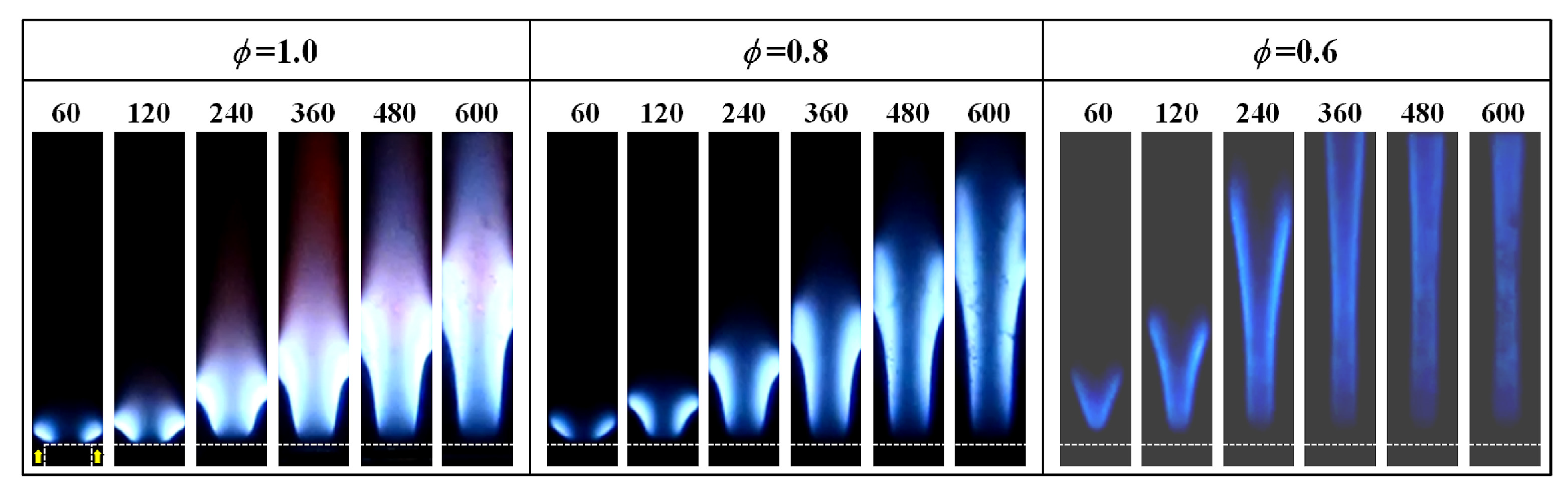
- Periodic flames, which are characterized by fluctuations in their behavior that occur periodically over time, in a constant pattern, Figure 5a;
- Quasi-periodic flames, in which the oscillations present irregular periodicity and recurrence are observed, Figure 5b;
- Chaotic combustion, which can occur when steady combustion waves lose stability and transition to non-steady chaotic burning, exhibiting highly irregular and unpredictable behavior, Figure 5c.
5.3. Mild Combustion
5.4. Stable Flames
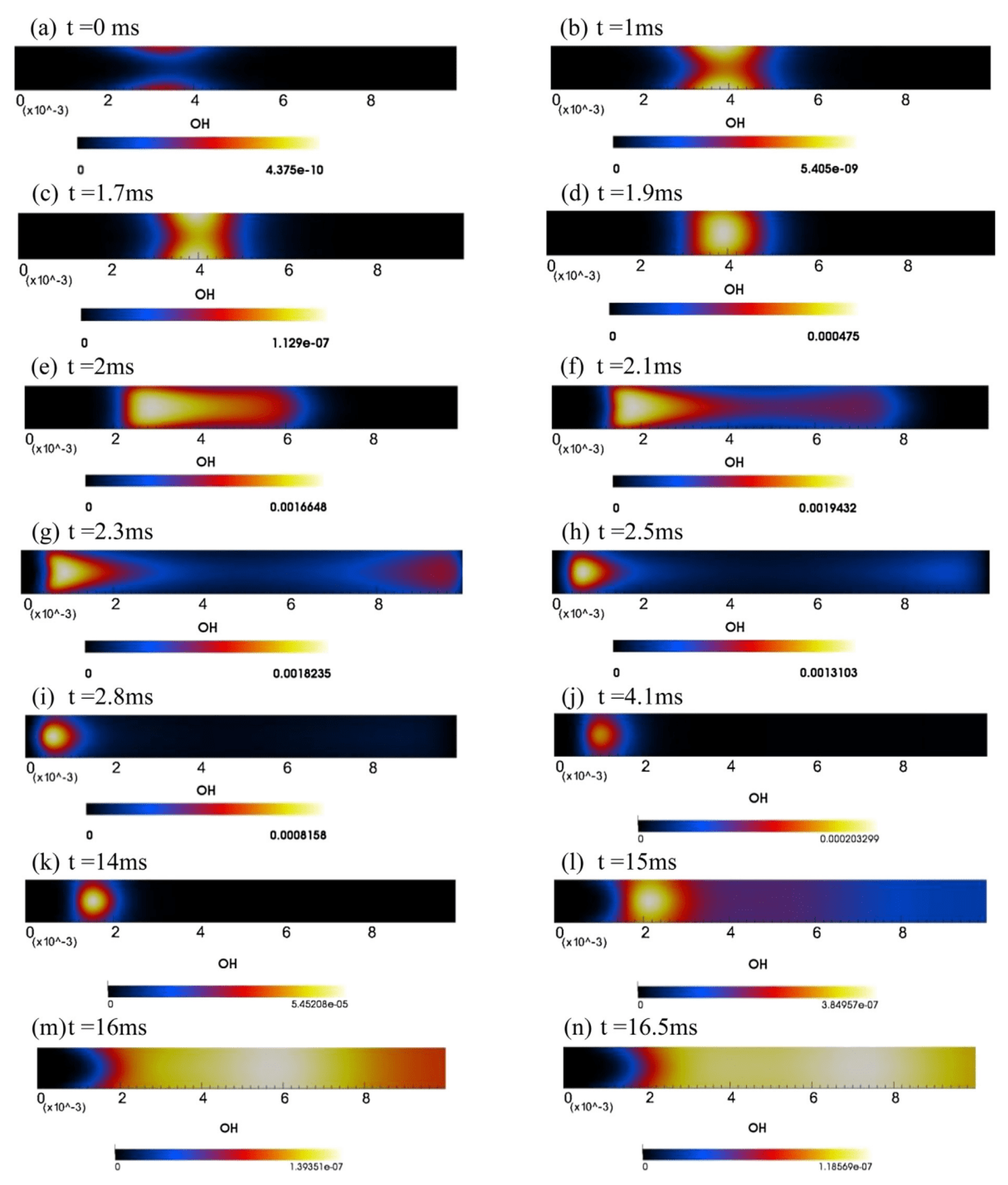
5.5. FREI: Flames with Repetitive Extinction and Ignition
5.6. PFB: Pulsatory Flame Burst
6. Numerical Investigations in Microcombustion
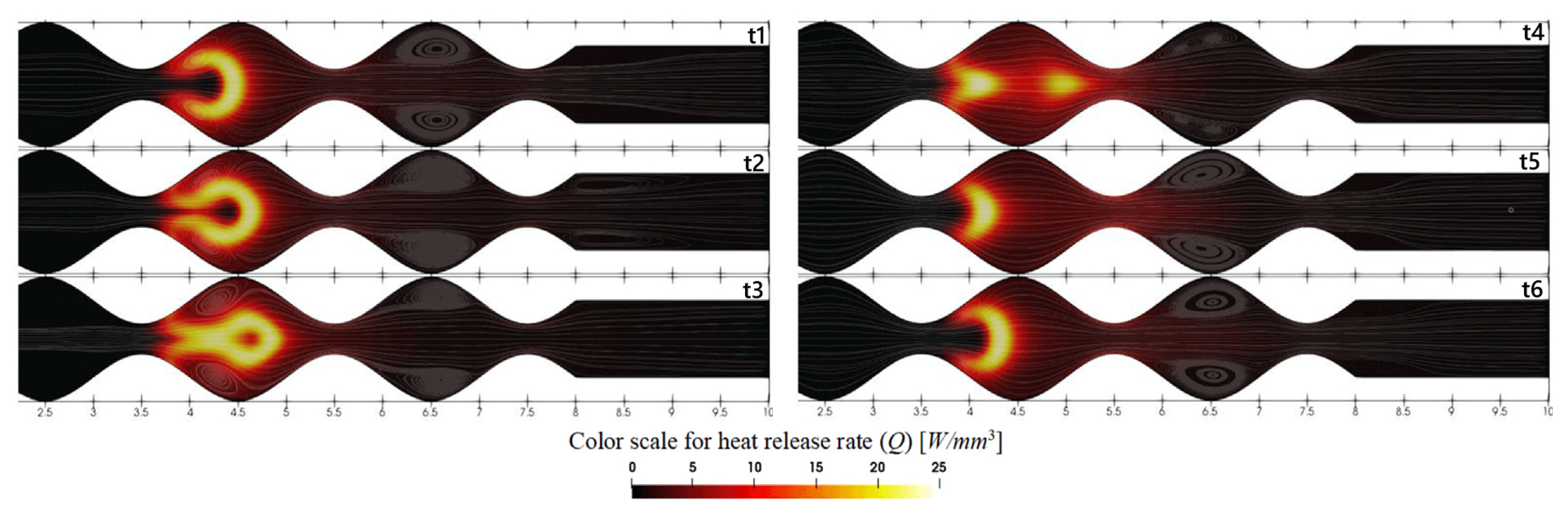
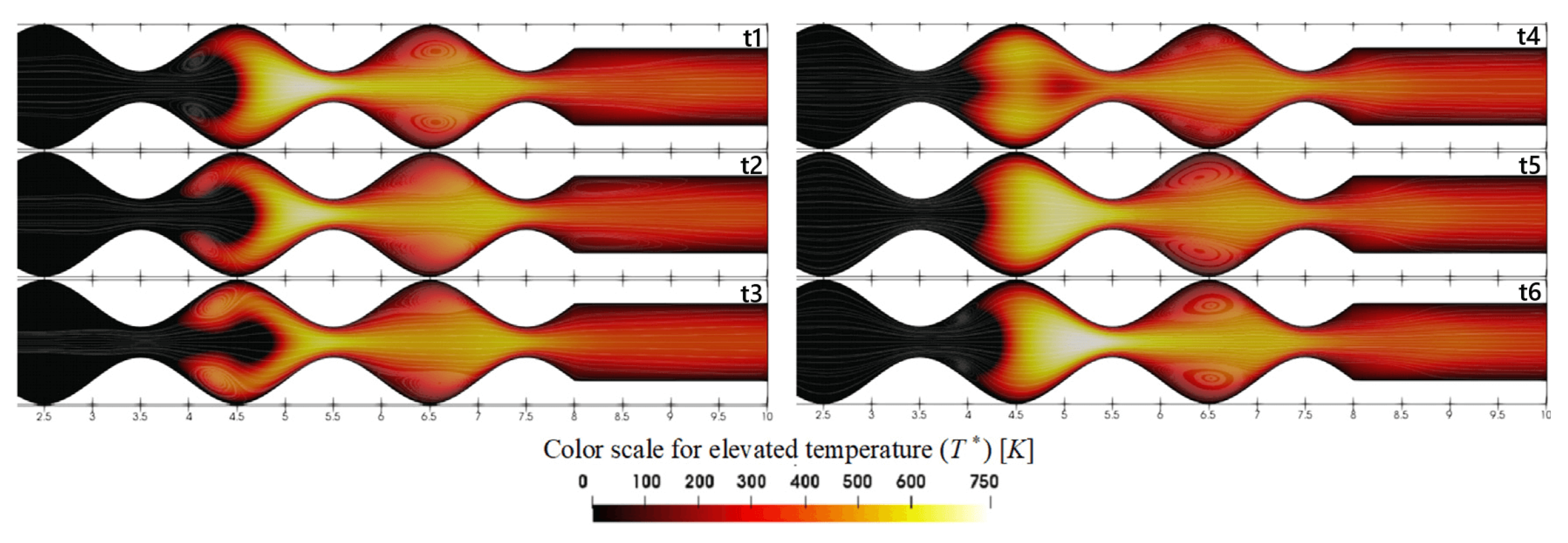
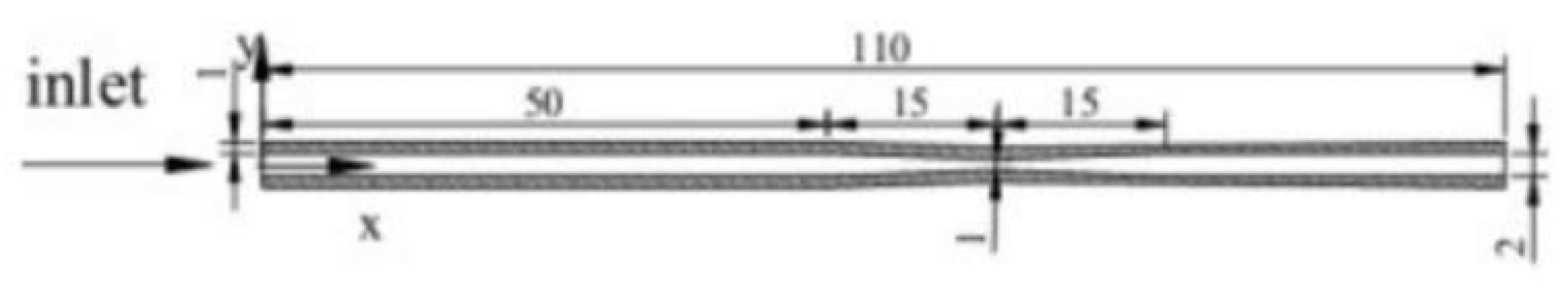
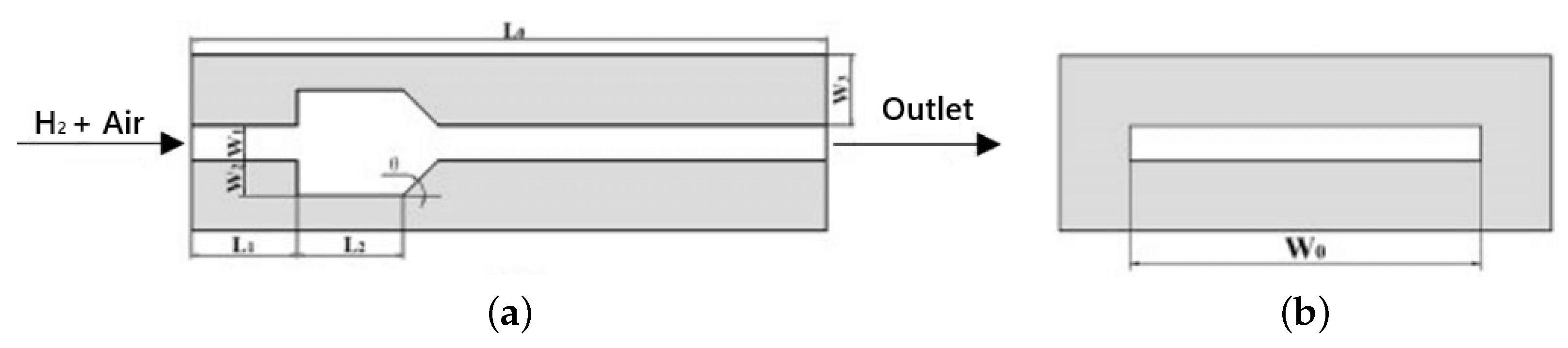
Investigations in Wavy Wall Geometries
7. A Review of Micropropulsion
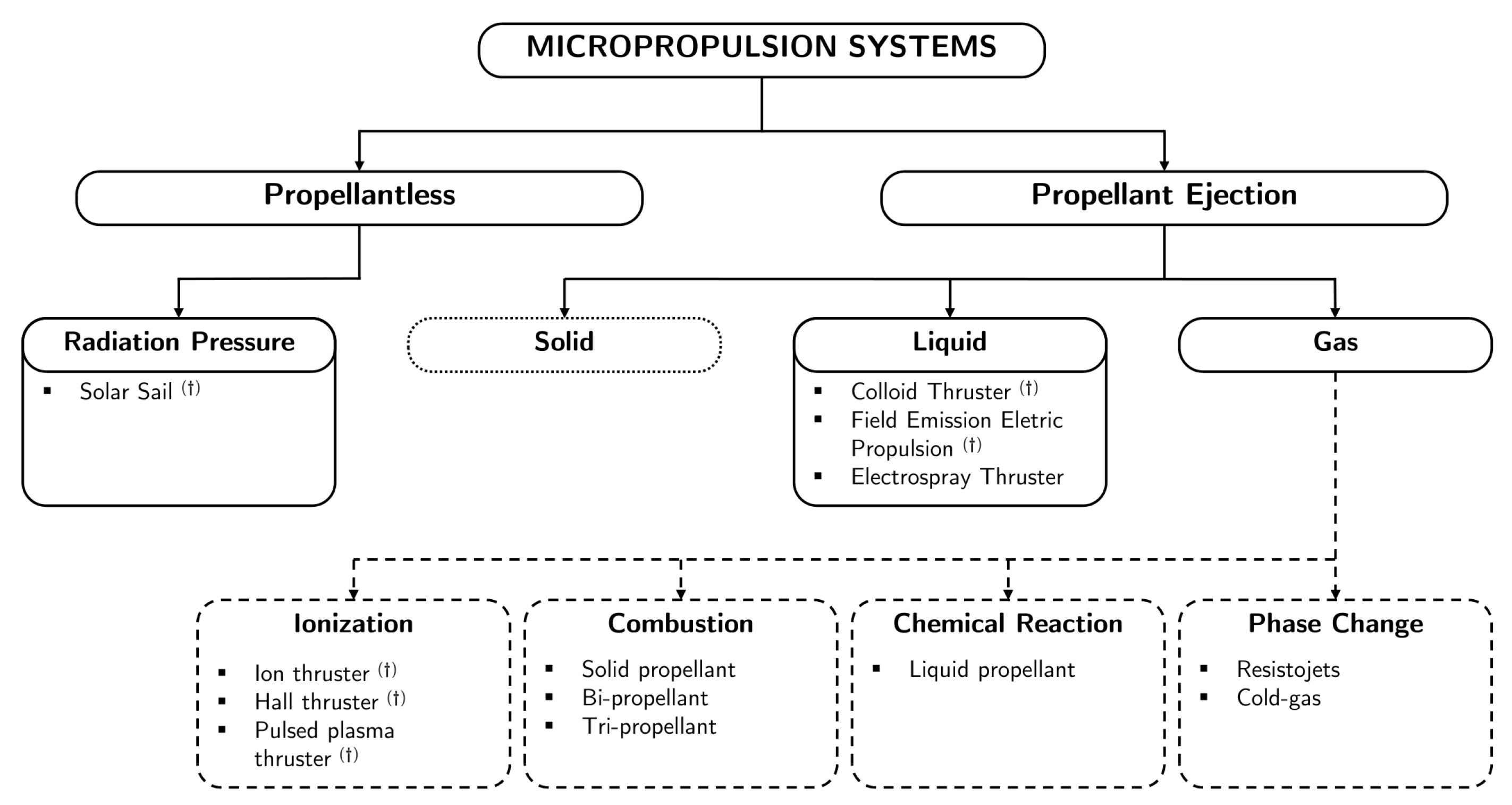
7.1. Overview on Micropropulsion Systems
- (i)
- They possess the capability to generate relatively minor thrust with magnitudes ranging up to and impulse values of μNs up to mNs;
- (ii)
- They display a relatively low volume and weight profile.
7.2. Micropropulsion Systems Performance
7.2.1. Thrust and Specific Impulse
7.2.2. Thrust Considering Chamber Pressure
7.2.3. Thrust Key Indicators
7.3. Conventionally Manufactured Micropropulsion Systems
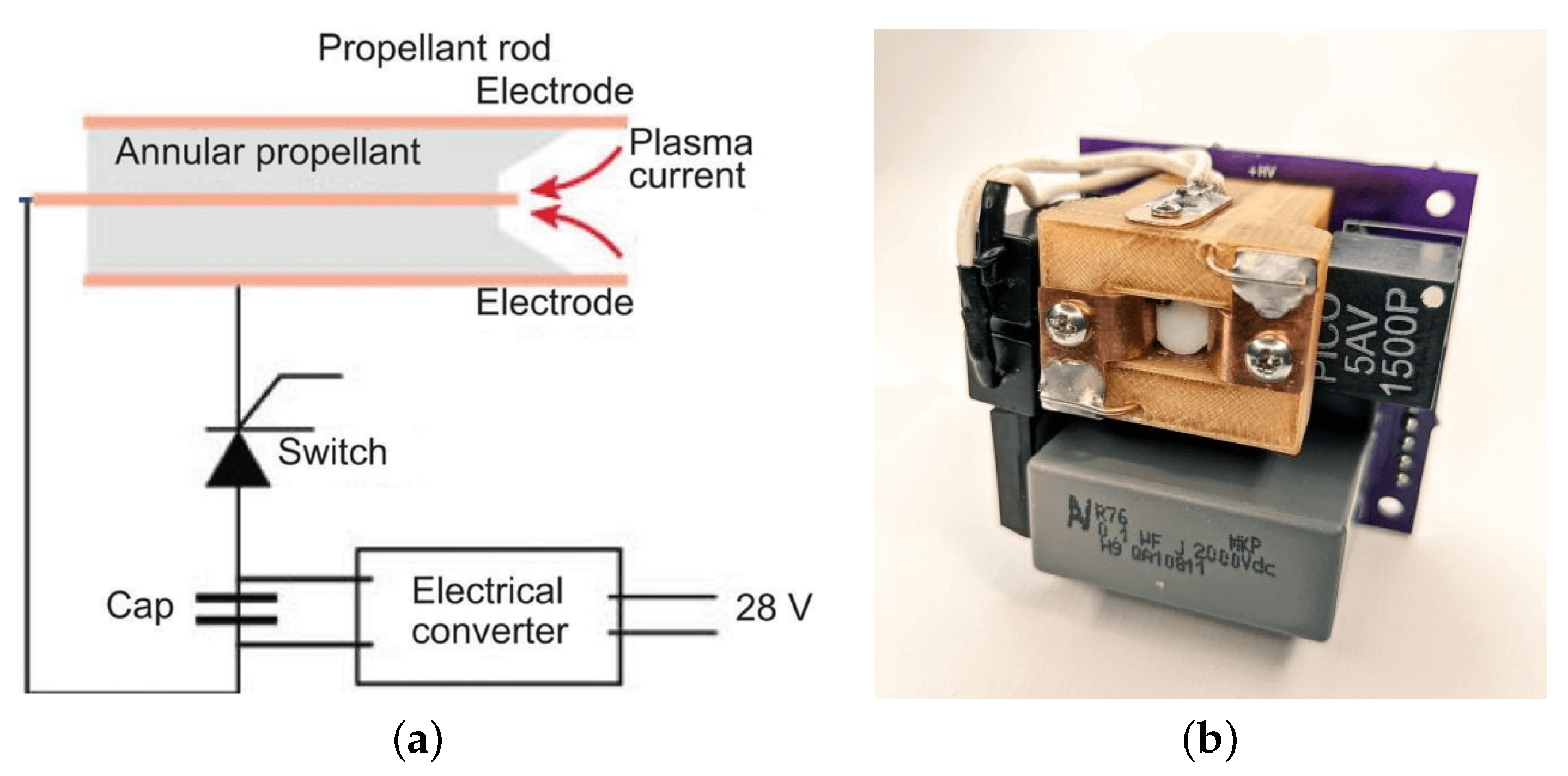
7.3.1. Pulsed Plasma Thruster
7.3.2. Field Emission Electric Propulsion
7.3.3. Other Relevant Micropropulsion System Technologies
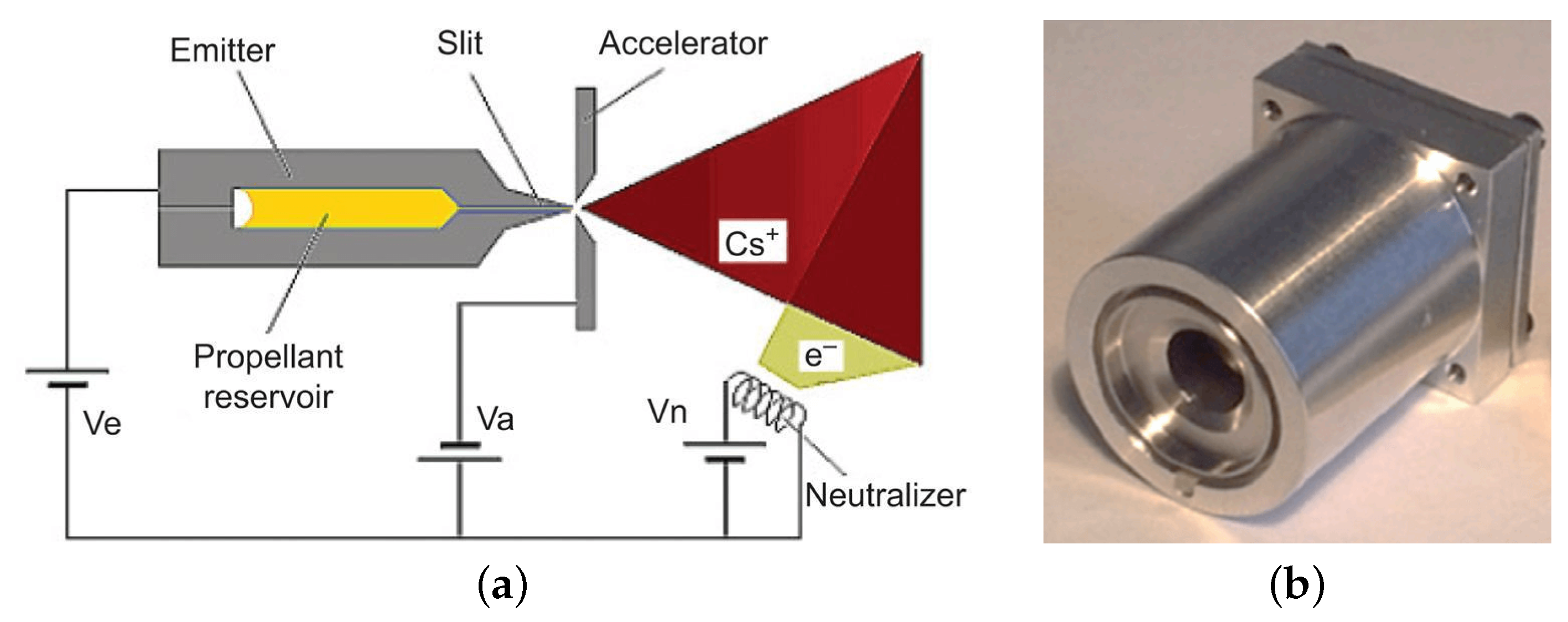
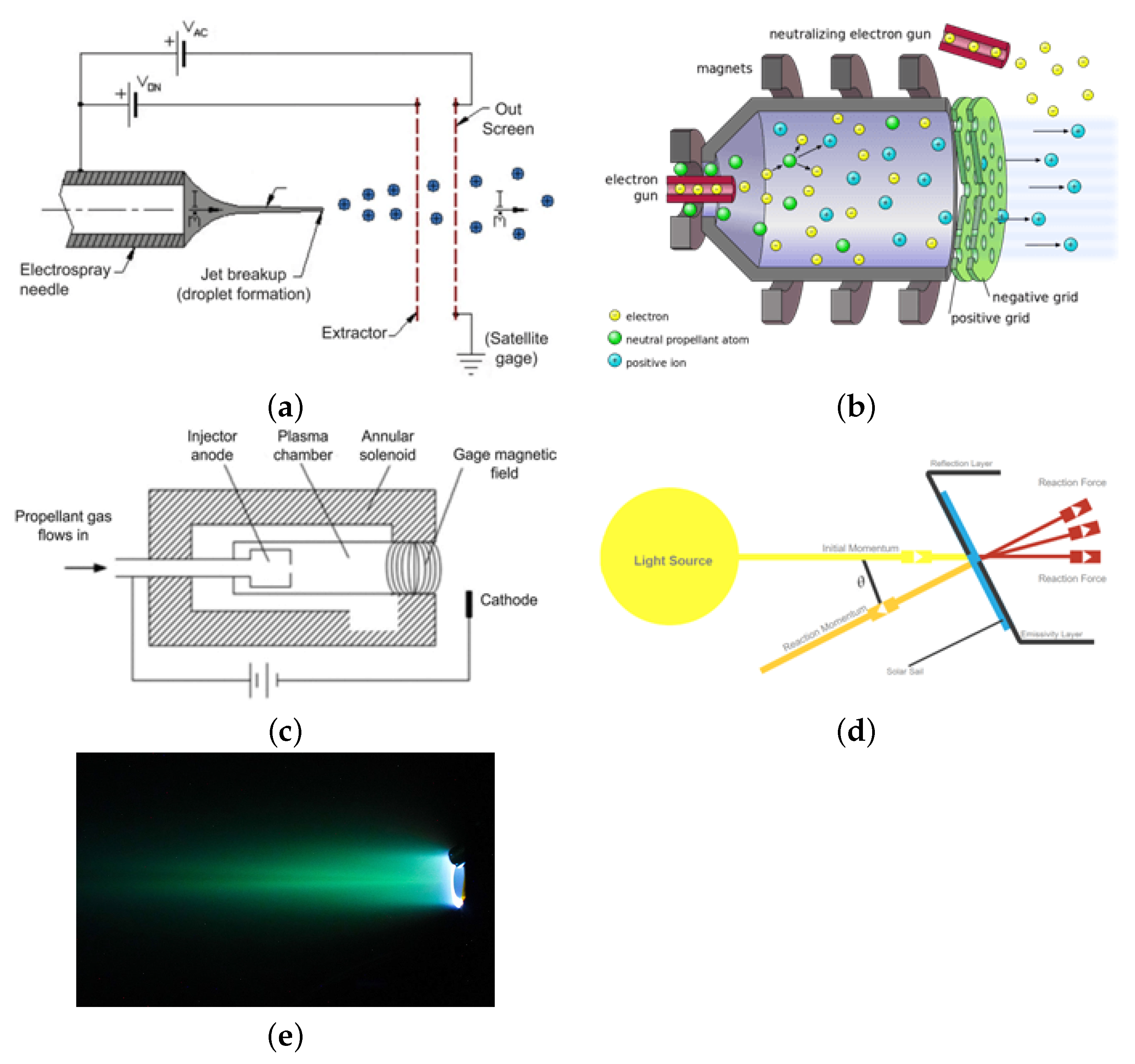
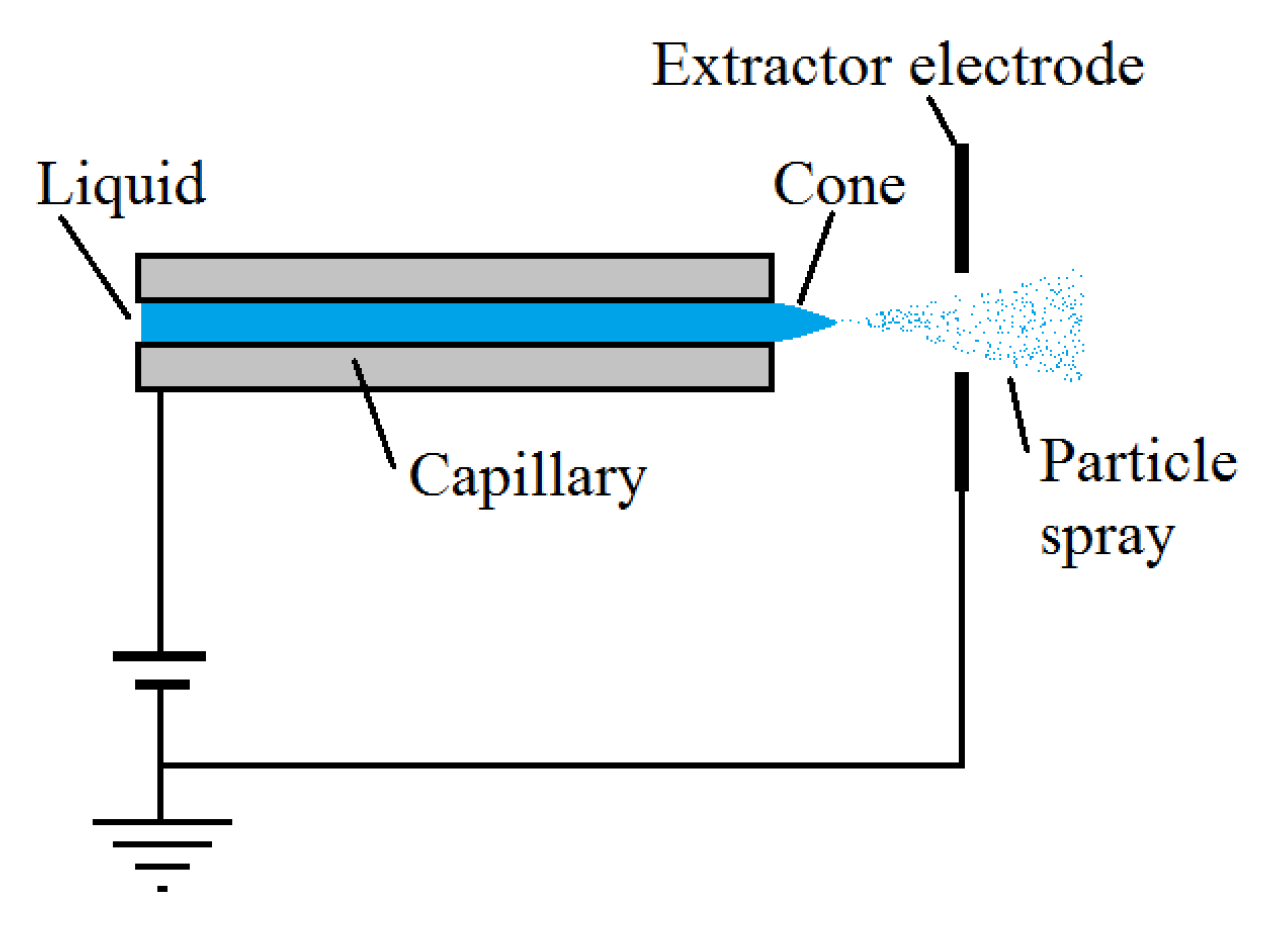
- Colloid Thruster: An electrostatic electric MPS similar to FEEP that uses high voltage and conductive liquids to accelerate charged particles, producing thrust. The thruster comprises charged particle emitters, extraction electrodes, an accelerating network, and a neutralizer to prevent ionic contamination on satellites. Different electrode potentials generate particle streams of varying polarities. The working principle of the colloid thruster [135] is represented in Figure 22a.
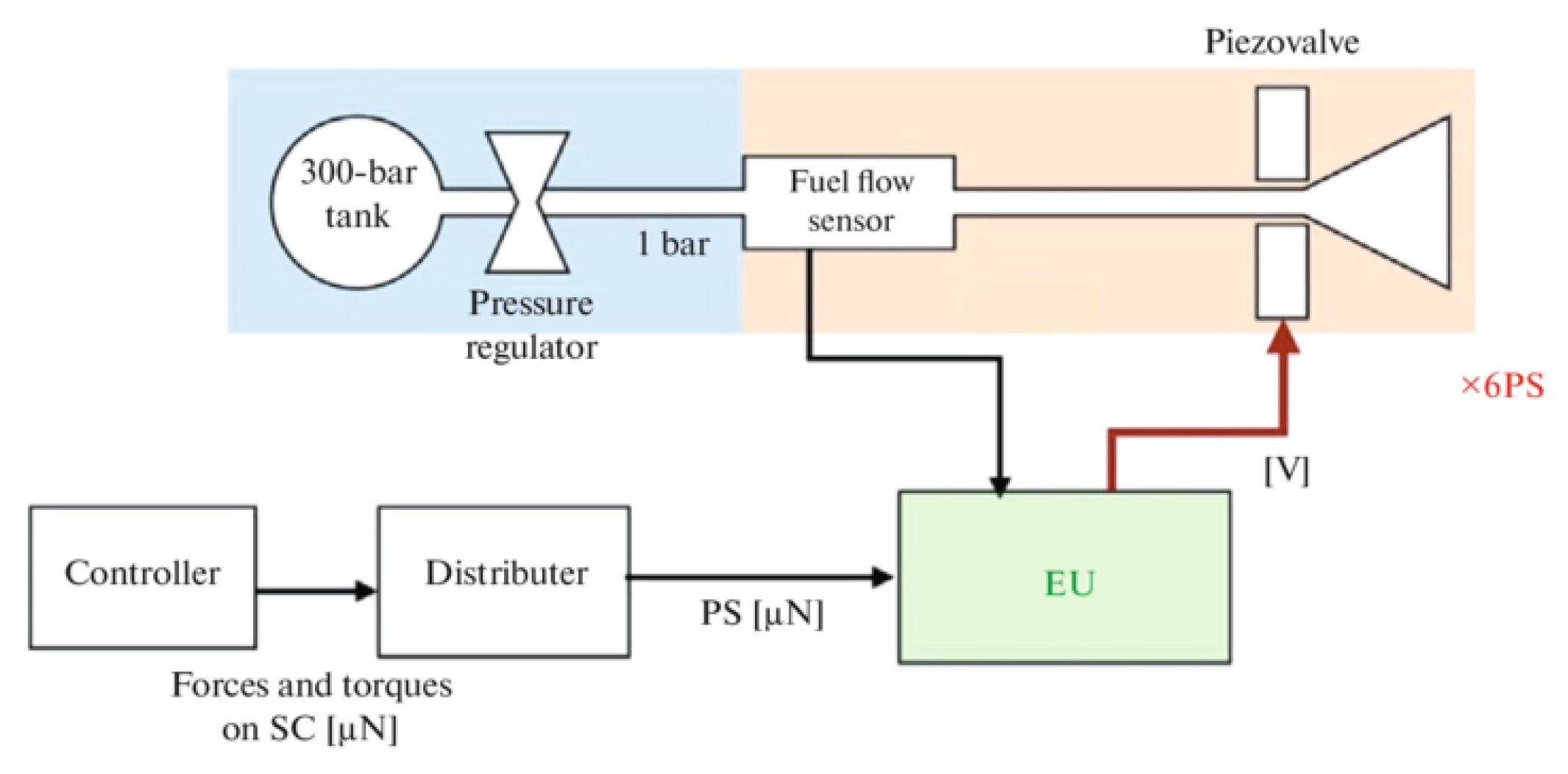
- Hall thruster and Ion Thruster: Devices that generate thrust by ejecting ions at high velocities. They can achieve high specific impulse, but typically produce low thrust levels. There are two main types: Hall thrusters and ion thrusters. Hall thrusters use the Hall effect to trap electrons, ionize the propellant, and then accelerate and exhaust it to create thrust. Ion thrusters, on the other hand, use charged grids to accelerate the ionized propellant [136,137,138]. This propulsion technology has already seen implementation in space missions and is capable of delivering thrust within the appropriate range for CubeSat missions. The working principle of the Ion thruster [139] and the Hall thruster [135] are represented in Figure 22b,c, respectively. Recently Dong et al. [140] proposed an advanced Watt class of Hall micro thrusters (HMT) for space gravitational wave detection. The research involved creating a circuit for thruster discharge and signal acquisition, using a Faraday probe to measure the plasma plume signal generated by the thruster. The discharge of the HMT thruster [140] using xenon is represented in Figure 22e.
- Solar sail: A spacecraft propulsion method that utilizes sunlight momentum to drive the craft forward [141]. It consists of a lightweight, reflective-coated sail with extreme thinness (down to 4.5 microns) and substantial size (hundreds of meters or kilometers) [142]. Photons from the Sun impart momentum to the sail, propelling the spacecraft and this continuous acceleration allows for high speeds over time. Solar sails have various advantages, including cost effectiveness, sustainability, applicability to small satellites, and the potential for long operating lifetimes [143]. This technology shows great promise for efficient and lightweight space travel. The working principle of the Solar sail [144] is represented in Figure 22).
- Laser Micropropulsion (LMP): The concept of laser propulsion was first introduced by Kantrowitz [145], presenting the advantages of being cost effective, with a high specific impulse, and an excellent thrust power ratio, and has gained significant recognition in the applications in micro/nano satellite propulsion. Nevertheless, the widespread adoption of LMP technology is limited by the proper selection of propellant. Recently, Rao et al. [146,147] presented an innovative LMP technology using metal-organic frameworks (MOFs) and also MOF-derived Carbon-encapsulated-Nano-Metal Composites (CNMC) as the propellant. The characteristic structure in CNMC overcomes the challenge of local hot zones caused by uneven physical mixing encountered in traditional LMP approaches. The ability to manipulate the metal content in MOFs allows for the optimization of laser propulsion performance, paving the way for further enhancements.
7.4. Micro-Electro-Mechanical Systems
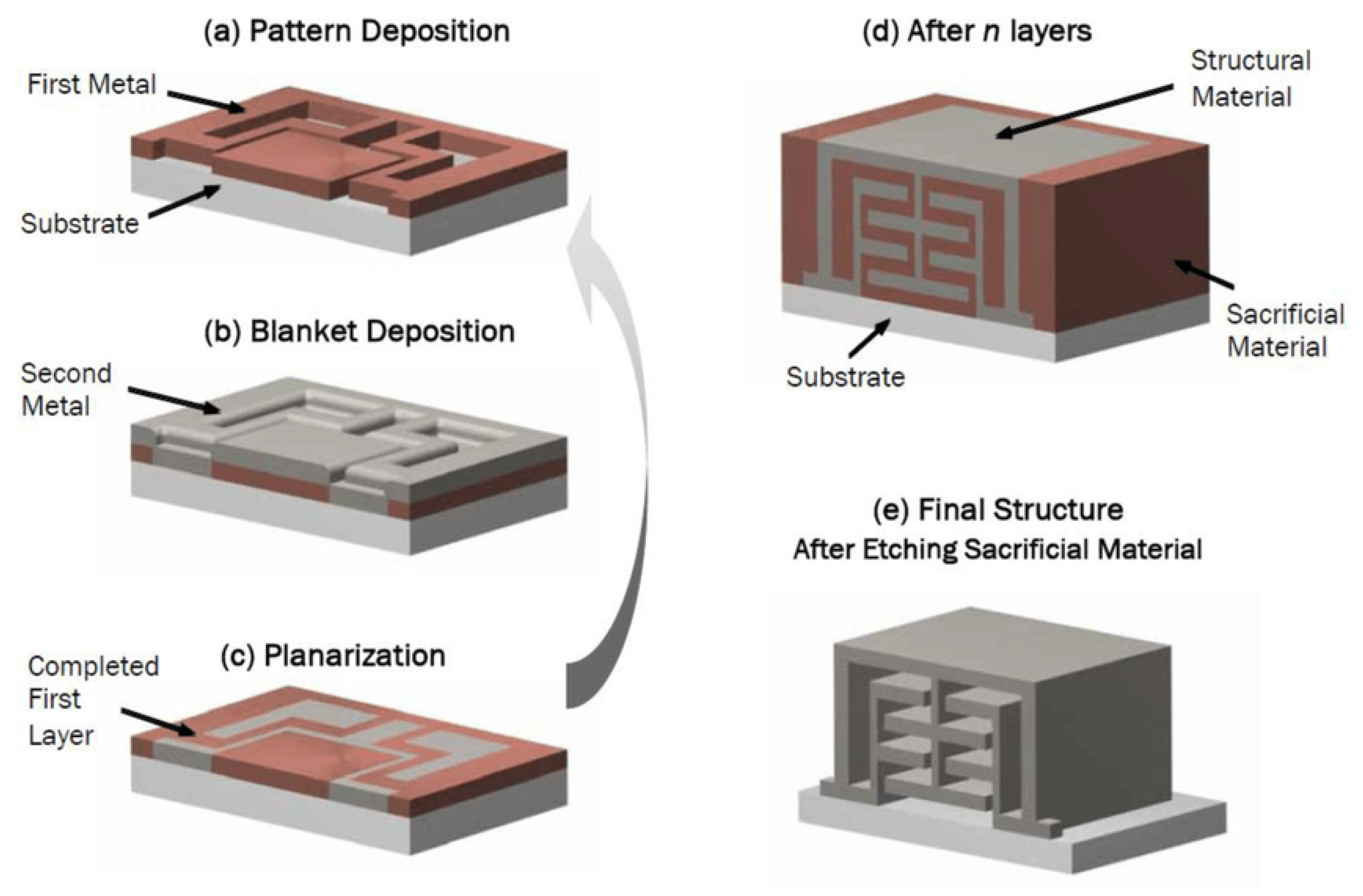
MEMS Manufacturing
- Deposition: This step entails the deposition of minute layers of materials onto the surface of the silicon wafer.
- Patterning: Patterning is employed to imprint a specific design onto the surface, thereby safeguarding certain regions while exposing others to shape the device’s features.
- Etching: Etching serves the purpose of material removal from the exposed areas, employing either isotropic or anisotropic techniques.
7.5. MEMS Categories
7.5.1. Electrothermal MEMS Electrical Thrusters
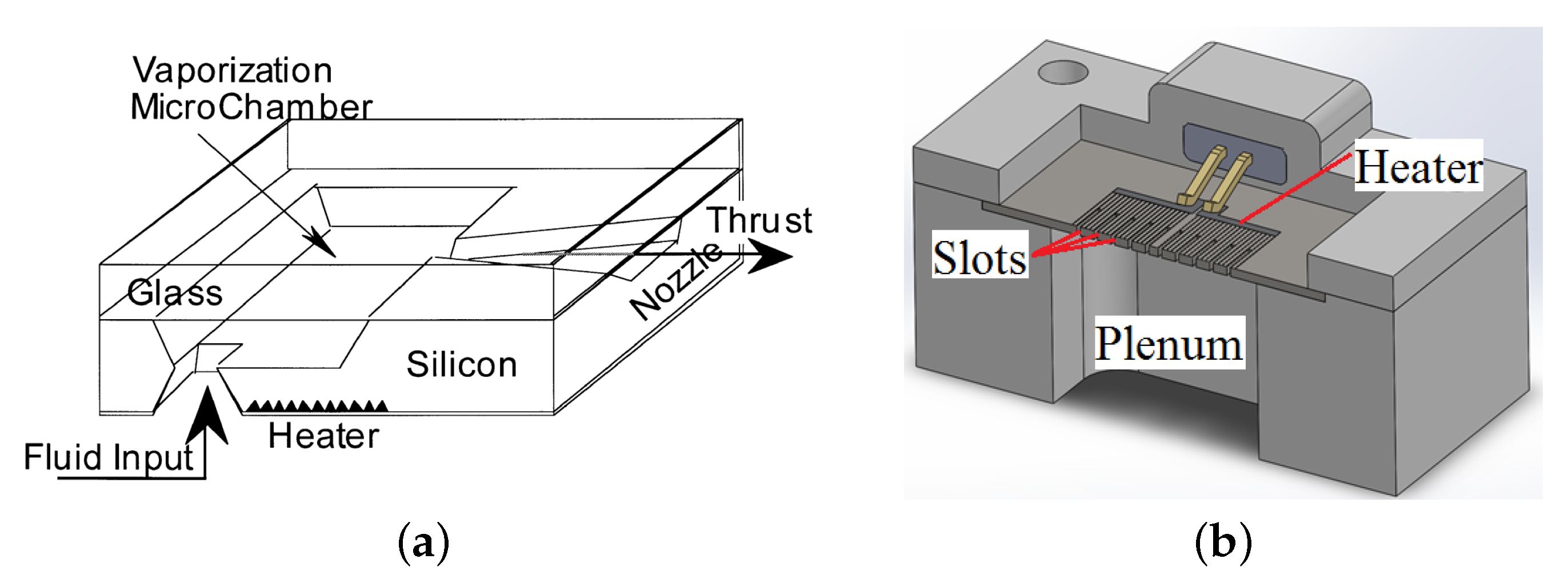
- Vaporizing Liquid Microthruster (VLM): They have been in development since the 1960s [152,153,154,155], and the design comprises an inlet channel for propellant feed, a chamber where a heating element vaporizes the propellant, and a convergent–divergent nozzle to propel the gases to supersonic speeds, as observed in Figure 24a). Several designs demonstrate significant potential, achieving a specific impulse () exceeding 100 s [156,157,158];
- Low-Pressure Microresistojet (LPM, also referred to as the Free Molecule Micro-Resistojet): They operate in the transitional flow regime (0.1 < Kn ≤ 10) [120]. To model and simulate its operation, statistical methods based on gas kinetic theory are required [159,160]. These devices typically consist of an inlet section, a low-pressure plenum (typically below 1000 Pa), and a MEMS-manufactured heater chip with channels to accelerate the gas, as observed in Figure 24b). The design of these channels is critical for efficient heat transfer and overall thruster efficiency [161,162]. The choice of resistance type and manufacturing approach is also essential for the optimal conversion of electrical to thermal energy. Current designs show of around 7∼39 s [163,164,165].
7.5.2. Electrostatic MEMS Electrical Thrusters
7.5.3. MEMS Chemical Thrusters

7.5.4. Cold-Gas Microthrusters
8. Conclusions
Funding
Conflicts of Interest
References
- Ju, Y.; Maruta, K. Microscale combustion: Technology development and fundamental research. Prog. Energy Combust. Sci. 2011, 37, 669–715. [Google Scholar] [CrossRef]
- Nakamura, Y.; Gao, J.; Matsuoka, T. Progress in small-scale combustion. J. Therm. Sci. Technol. 2017, 12, JTST0001. [Google Scholar] [CrossRef][Green Version]
- Cai, L.; Jiaqiang, E.; Li, J.; Ding, J.; Luo, B. A comprehensive review on combustion stabilization technologies of micro/meso-scale combustors for micro thermophotovoltaic systems: Thermal, emission, and energy conversion. Fuel 2023, 335, 126660. [Google Scholar] [CrossRef]
- Jabar, D.; Al-Fahham, M. A review of combustion parameters affecting length and flame structure. AIP Conf. Proc. 2024, 3051, 020001. [Google Scholar]
- Nauman, M.; Pan, J.; Wang, Y.; Li, F.; Ojo, A.O.; Raza, A. A review of recent advancements in micro combustion techniques to enhance flame stability and fuel residence time. Int. J. Hydrogen Energy 2023, 49, 1165–1193. [Google Scholar] [CrossRef]
- Merriam-Webster. Combustion. Available online: https://www.merriam-webster.com/dictionary/combustion (accessed on 28 March 2023).
- Fernandez-Pello, A.C. Micropower generation using combustion: Issues and approaches. Proc. Combust. Inst. 2002, 29, 883–899. [Google Scholar] [CrossRef]
- Thomas, G.; Oakley, G.; Bambrey, R. Fundamental studies of explosion arrester mitigation mechanisms. Process. Saf. Environ. Prot. 2020, 137, 15–33. [Google Scholar] [CrossRef]
- Davy, H., VII. Some researches on flame. Philos. Trans. R. Soc. Lond. 1817, 107, 45–76. [Google Scholar]
- Hossain, A.; Nakamura, Y. Thermal and chemical structures formed in the micro burner of miniaturized hydrogen-air jet flames. Proc. Combust. Inst. 2015, 35, 3413–3420. [Google Scholar] [CrossRef]
- Resende, P.R.; Ayoobi, M.; Afonso, A.M. Numerical investigations of micro-scale diffusion combustion: A brief review. Appl. Sci. 2019, 9, 3356. [Google Scholar] [CrossRef]
- Lee, L.N.; Kim, M.J.; Hwang, W.J. Potential of augmented reality and virtual reality technologies to promote wellbeing in older adults. Appl. Sci. 2019, 9, 3556. [Google Scholar] [CrossRef]
- Glassman, I.; Yetter, R. Flame phenomena in premixed combustible gases. In Combustion, 4th ed.; Academic Press: Cambridge, MA, USA, 2008; pp. 153–162. [Google Scholar]
- Li, Q.; Wang, J.; Meng, L.; Li, J.; Guo, Z. CFD study on stability limits of hydrogen/air premixed flames in planar micro-combustors with catalytic walls. Appl. Therm. Eng. 2017, 121, 325–335. [Google Scholar] [CrossRef]
- Kang, Z.; Shi, Z.; Ye, J.; Tian, X.; Huang, Z.; Wang, H.; Wei, D.; Peng, Q.; Tu, Y. A Review of Micro Power System and Micro Combustion: Present Situation, Techniques and Prospects. Energies 2023, 16, 3201. [Google Scholar] [CrossRef]
- Yun, T.M.; Kottke, P.A.; Anderson, D.M.; Fedorov, A.G. Experimental investigation of hydrogen production by variable volume membrane batch reactors with modulated liquid fuel introduction. Int. J. Hydrogen Energy 2015, 40, 2601–2612. [Google Scholar] [CrossRef]
- Wang, Y. Introduction to Micro-Scale Combustion: University Lecture at Pennsylvania State University. Available online: https://yang.gatech.edu/projects/Meso%20and%20Micro%20Scale%20Propulsion...(Yanxing)/files/micro-combustion%20(for%20webpage%20).pdf (accessed on 19 May 2023).
- Lee, M.J.; Cho, S.M.; Choi, B.I.; Kim, N.I. Scale and material effects on flame characteristics in small heat recirculation combustors of a counter-current channel type. Appl. Therm. Eng. 2010, 30, 2227–2235. [Google Scholar] [CrossRef]
- Tu, Y.; Liu, H.; Yang, W. Flame characteristics of CH4/H2 on a jet-in-hot-coflow burner diluted by N2, CO2, and H2O. Energy Fuels 2017, 31, 3270–3280. [Google Scholar] [CrossRef]
- Mohan, S.; Matalon, M. Diffusion flames and diffusion flame-streets in three dimensional micro-channels. Combust. Flame 2017, 177, 155–170. [Google Scholar] [CrossRef]
- Feldmeier, S.; Schwarz, M.; Wopienka, E.; Pfeifer, C. Categorization of small-scale biomass combustion appliances by characteristic numbers. Renew. Energy 2021, 163, 2128–2136. [Google Scholar] [CrossRef]
- Li, Y.H.; Chen, G.B.; Cheng, T.S.; Yeh, Y.L.; Chao, Y.C. Combustion characteristics of a small-scale combustor with a percolated platinum emitter tube for thermophotovoltaics. Energy 2013, 61, 150–157. [Google Scholar] [CrossRef]
- Kaisare, N.S.; Deshmukh, S.R.; Vlachos, D.G. Stability and performance of catalytic microreactors: Simulations of propane catalytic combustion on Pt. Chem. Eng. Sci. 2008, 63, 1098–1116. [Google Scholar] [CrossRef]
- Bagheri, G.; Hosseini, S.E.; Wahid, M.A. Effects of bluff body shape on the flame stability in premixed micro-combustion of hydrogen–air mixture. Appl. Therm. Eng. 2014, 67, 266–272. [Google Scholar] [CrossRef]
- Akhtar, S.; Khan, M.N.; Kurnia, J.C.; Shamim, T. Investigation of energy conversion and flame stability in a curved micro-combustor for thermo-photovoltaic (TPV) applications. Appl. Energy 2017, 192, 134–145. [Google Scholar] [CrossRef]
- Cao, Z.; Lyu, Y.; Peng, J.; Qiu, P.; Liu, L.; Yang, C.; Yu, Y.; Chang, G.; Yan, B.; Sun, S.; et al. Experimental study of flame evolution, frequency and oscillation characteristics of steam diluted micro-mixing hydrogen flame. Fuel 2021, 301, 121078. [Google Scholar] [CrossRef]
- Aggarwal, S.K. Extinction of laminar partially premixed flames. Prog. Energy Combust. Sci. 2009, 35, 528–570. [Google Scholar] [CrossRef]
- Fu, K.; Knobloch, A.J.; Martinez, F.C.; Walther, D.C.; Fernandez-Pello, C.; Pisano, A.P.; Liepmann, D.; Miyaska, K.; Maruta, K. Design and experimental results of small-scale rotary engines. In Proceedings of the ASME International Mechanical Engineering Congress and Exposition. American Society of Mechanical Engineers, New York, NY, USA, 11–16 November 2001; Volume 35524, pp. 295–301. [Google Scholar]
- Shanbhogue, S.; Sanusi, Y.; Taamallah, S.; Habib, M.; Mokheimer, E.; Ghoniem, A. Flame macrostructures, combustion instability and extinction strain scaling in swirl-stabilized premixed CH4/H2 combustion. Combust. Flame 2016, 163, 494–507. [Google Scholar] [CrossRef]
- Eckart, S.; Yu, C.; Maas, U.; Krause, H. Experimental and numerical investigations on extinction strain rates in non-premixed counterflow methane and propane flames in an oxygen reduced environment. Fuel 2021, 298, 120781. [Google Scholar] [CrossRef]
- Spalding, D.B. A theory of inflammability limits and flame-quenching. Proc. R. Soc. Lond. Ser. A Math. Phys. Sci. 1957, 240, 83–100. [Google Scholar]
- Maruta, K.; Yoshida, M.; Ju, Y.; Niioka, T. Experimental study on methane-air premixed flame extinction at small stretch rates in microgravity. In Proceedings of the Symposium (International) on Combustion, Napoli, Italy, 28 July 1996–2 August 1996; Elsevier: Amsterdam, The Netherlands, 1996; Volume 26-1, pp. 1283–1289. [Google Scholar]
- Ju, Y.; Maruta, K.; Niioka, T. Combustion Limits. Appl. Mech. Rev. 2001, 54, 257–277. [Google Scholar] [CrossRef]
- Lewis, B.; Von Elbe, G. Combustion, Flames and Explosions of Gases; Elsevier: Amsterdam, The Netherlands, 2012. [Google Scholar]
- Daou, J.; Matalon, M. Influence of conductive heat-losses on the propagation of premixed flames in channels. Combust. Flame 2002, 128, 321–339. [Google Scholar] [CrossRef]
- Ferguson, C.R.; Keck, J.C. On laminar flame quenching and its application to spark ignition engines. Combust. Flame 1977, 28, 197–205. [Google Scholar] [CrossRef]
- Law, C.K. Combustion Physics; Cambridge University Press: Cambridge, UK, 2010. [Google Scholar]
- Williams, F.A. Combustion Theory; Benjamin-Cummings Publishing Company: San Francisco, CA, USA, 1985. [Google Scholar]
- Bockhorn, H.; Habisreuther, P.; Hettel, M. Numerical Modelling of Technical Combustion: 100 Volumes of ‘Notes on Numerical Fluid Mechanics’: 40 Years of Numerical Fluid Mechanics and Aerodynamics in Retrospect; Springer: Berlin/Heidelberg, Germany, 2009; pp. 325–340. [Google Scholar] [CrossRef]
- Turns, S.R. Introduction to Combustion; McGraw-Hill Companies: New York, NY, USA, 1996; Volume 287. [Google Scholar]
- Lieuwen, T.C. Unsteady Combustor Physics; Cambridge University Press: Cambridge, UK, 2012. [Google Scholar] [CrossRef]
- Jerzak, W.; Kuźnia, M. Experimental study of impact of swirl number as well as oxygen and carbon dioxide content in natural gas combustion air on flame flashback and blow-off. J. Nat. Gas Sci. Eng. 2016, 29, 46–54. [Google Scholar] [CrossRef]
- Thibaut, D.; Candel, S. Numerical Study of Unsteady Turbulent Premixed Combustion: Application to Flashback Simulation. Combust. Flame 1998, 113, 53–65. [Google Scholar] [CrossRef]
- Fruzza, F.; Lamioni, R.; Tognotti, L.; Galletti, C. Flashback of H2-enriched premixed flames in perforated burners: Numerical prediction of critical velocity. Int. J. Hydrogen Energy 2023, 48, 31790–31801. [Google Scholar] [CrossRef]
- Fruzza, F.; Lamioni, R.; Mariotti, A.; Salvetti, M.V.; Galletti, C. Flashback propensity due to hydrogen blending in natural gas: Sensitivity to operating and geometrical parameters. Fuel 2024, 362, 130838. [Google Scholar] [CrossRef]
- Cai, S.; Yang, W.; Wan, J. Combustion and thermal performances of methane-air premixed flame in a novel preheated micro combustor with a flame holder. Int. J. Therm. Sci. 2024, 197, 108813. [Google Scholar] [CrossRef]
- Margolis, S.B. Chaotic Combustion of Solids and High-Density Fluids near Points of Strong Resonance. Proc. Math. Phys. Sci. 1991, 433, 131–150. [Google Scholar]
- Weng, Y.; Unni, V.R.; Sujith, R.; Saha, A. Synchronization framework for modeling transition to thermoacoustic instability in laminar combustors. Nonlinear Dyn. 2020, 100, 3295–3306. [Google Scholar] [CrossRef]
- Kabiraj, L.; Saurabh, A.; Wahi, P.; Sujith, R. Experimental study of thermoacoustic instability in ducted premixed flames: Periodic, quasi-periodic and chaotic oscillations. In Proceedings of the n3l-Int’l Summer School and Workshop on Non-Normal and Nonlinear Effects in Aero-and Thermoacoustics, Munich, Germany, 17–21 May 2010; p. 12. [Google Scholar]
- Maruta, K.; Muso, K.; Takeda, K.; Niioka, T. Reaction zone structure in flameless combustion. Proc. Combust. Inst. 2000, 28, 2117–2123. [Google Scholar] [CrossRef]
- Cavaliere, A.; De Joannon, M. Mild combustion. Prog. Energy Combust. Sci. 2004, 30, 329–366. [Google Scholar] [CrossRef]
- Wünning, J.; Wünning, J. Flameless oxidation to reduce thermal NO-formation. Prog. Energy Combust. Sci. 1997, 23, 81–94. [Google Scholar] [CrossRef]
- Katsuki, M.; Hasegawa, T. The science and technology of combustion in highly preheated air. Symp. Int. Combust. 1998, 27, 3135–3146. [Google Scholar] [CrossRef]
- Niioka, T. Fundamentals and applications of high-temperature air combustion. In Proceedings of the 5th ASME/JSME Thermal Engineering Joint Conference, San Diego, CA, USA, 15–19 March 1999; pp. 1–6. [Google Scholar]
- Maruta, K.; Kataoka, T.; Kim, N.I.; Minaev, S.; Fursenko, R. Characteristics of combustion in a narrow channel with a temperature gradient. Proc. Combust. Inst. 2005, 30, 2429–2436. [Google Scholar] [CrossRef]
- Pizza, G.; Frouzakis, C.E.; Mantzaras, J.; Tomboulides, A.G.; Boulouchos, K. Three-dimensional simulations of premixed hydrogen/air flames in microtubes. J. Fluid Mech. 2010, 658, 463–491. [Google Scholar] [CrossRef]
- Pizza, G.; Frouzakis, C.E.; Mantzaras, J.; Tomboulides, A.G.; Boulouchos, K. Dynamics of premixed hydrogen/air flames in microchannels. Combust. Flame 2008, 152, 433–450. [Google Scholar] [CrossRef]
- Tsuboi, Y.; Yokomori, T.; Maruta, K. Study on ignition and weak flame in heated meso-scale channel. In Proceedings of the ASME International Mechanical Engineering Congress and Exposition, Proceedings (IMECE), Seattle, WA, USA, 11–15 November 2007; pp. 155–158. [Google Scholar] [CrossRef]
- Di Stazio, A.; Chauveau, C.; Dayma, G.; Dagaut, P. Combustion in micro-channels with a controlled temperature gradient. Exp. Therm. Fluid Sci. 2016, 73, 79–86. [Google Scholar] [CrossRef]
- Li, J.; Chou, S.; Yang, W.; Li, Z. A numerical study on premixed micro-combustion of CH4–air mixture: Effects of combustor size, geometry and boundary conditions on flame temperature. Chem. Eng. J. 2009, 150, 213–222. [Google Scholar] [CrossRef]
- Kim, N.I.; Maruta, K. A numerical study on propagation of premixed flames in small tubes. Combust. Flame 2006, 146, 283–301. [Google Scholar] [CrossRef]
- Norton, D.G.; Vlachos, D.G. Combustion characteristics and flame stability at the microscale: A CFD study of premixed methane/air mixtures. Chem. Eng. Sci. 2003, 58, 4871–4882. [Google Scholar] [CrossRef]
- Lamioni, R.; Lapenna, P.E.; Berger, L.; Kleinheinz, K.; Attili, A.; Pitsch, H.; Creta, F. Pressure-induced hydrodynamic instability in premixed methane-air slot flames. Combust. Sci. Technol. 2020, 192, 1998–2009. [Google Scholar] [CrossRef]
- Kurdyumov, V.; Fernandez-Tarrazo, E. Lewis number effect on the propagation of premixed laminar flames in narrow open ducts. Combust. Flame 2002, 128, 382–394. [Google Scholar] [CrossRef]
- Kurdyumov, V.N.; Pizza, G.; Frouzakis, C.E.; Mantzaras, J. Dynamics of premixed flames in a narrow channel with a step-wise wall temperature. Combust. Flame 2009, 156, 2190–2200. [Google Scholar] [CrossRef]
- Dogwiler, U.; Mantzaras, J.; Benz, P.; Kaeppeli, B.; Bombach, R.; Arnold, A. Homogeneous ignition of methane-air mixtures over platinum: Comparison of measurements and detailed numerical predictions. In Proceedings of the Symposium (International) on Combustion, Boulder, CO, USA, 2–7 August 1998; Elsevier: Amsterdam, The Netherlands, 1998; Volume 27-2, pp. 2275–2282. [Google Scholar]
- Tsai, C.H. The asymmetric behavior of steady laminar flame propagation in ducts. Combust. Sci. Technol. 2008, 180, 533–545. [Google Scholar] [CrossRef]
- Alipoor, A.; Mazaheri, K. Studying the repetitive extinction-ignition dynamics for lean premixed hydrogen-air combustion in a heated microchannel. Energy 2014, 73, 367–379. [Google Scholar] [CrossRef]
- Lapointe, S.; Druzgalski, C.L.; McNenly, M.J. Numerical study of a micro flow reactor at engine pressures: Flames with repetitive extinction and ignition and simulations with a reduced chemical model. Combust. Flame 2018, 197, 102–110. [Google Scholar] [CrossRef]
- Richecoeur, F.; Kyritsis, D.C. Experimental study of flame stabilization in low Reynolds and Dean number flows in curved mesoscale ducts. Proc. Combust. Inst. 2005, 30, 2419–2427. [Google Scholar] [CrossRef]
- Baigmohammadi, M.; Tabejamaat, S.; Farsiani, Y. Experimental study of the effects of geometrical parameters, Reynolds number, and equivalence ratio on methane–oxygen premixed flame dynamics in non-adiabatic cylinderical meso-scale reactors with the backward facing step. Chem. Eng. Sci. 2015, 132, 215–233. [Google Scholar] [CrossRef]
- Minaev, S.; Maruta, K.; Fursenko, R. Nonlinear dynamics of flame in a narrow channel with a temperature gradient. Combust. Theory Model. 2007, 11, 187–203. [Google Scholar] [CrossRef]
- Jackson, T.; Buckmaster, J.; Lu, Z.; Kyritsis, D.; Massa, L. Flames in narrow circular tubes. Proc. Combust. Inst. 2007, 31, 955–962. [Google Scholar] [CrossRef]
- Miyata, E.; Fukushima, N.; Naka, Y.; Shimura, M.; Tanahashi, M.; Miyauchi, T. Direct numerical simulation of micro combustion in a narrow circular channel with a detailed kinetic mechanism. Proc. Combust. Inst. 2015, 35, 3421–3427. [Google Scholar] [CrossRef]
- Alipoor, A.; Mazaheri, K. Combustion characteristics and flame bifurcation in repetitive extinction-ignition dynamics for premixed hydrogen-air combustion in a heated micro channel. Energy 2016, 109, 650–663. [Google Scholar] [CrossRef]
- Alipoor, A.; Mazaheri, K. Maps of flame dynamics for premixed lean hydrogen-air combustion in a heated microchannel. Energy 2020, 194, 116852. [Google Scholar] [CrossRef]
- Biswas, S. Physics of Turbulent Jet Ignition: Mechanisms and Dynamics of Ultra-Lean Combustion; Springer: Berlin/Heidelberg, Germany, 2018. [Google Scholar]
- Nakamura, H.; Fan, A.; Minaev, S.; Sereshchenko, E.; Fursenko, R.; Tsuboi, Y.; Maruta, K. Bifurcations and negative propagation speeds of methane/air premixed flames with repetitive extinction and ignition in a heated microchannel. Combust. Flame 2012, 159, 1631–1643. [Google Scholar] [CrossRef]
- Tsuboi, Y.; Yokomori, T.; Maruta, K. Lower limit of weak flame in a heated channel. Proc. Combust. Inst. 2009, 32, 3075–3081. [Google Scholar] [CrossRef]
- Yamamoto, A.; Oshibe, H.; Nakamura, H.; Tezuka, T.; Hasegawa, S.; Maruta, K. Stabilized three-stage oxidation of gaseous n-heptane/air mixture in a micro flow reactor with a controlled temperature profile. Proc. Combust. Inst. 2011, 33, 3259–3266. [Google Scholar] [CrossRef]
- de Macedo, J.M.R.C. Estudos Numéricos em Micropropulsão. Master’s Thesis, Universidade do Porto, Porto, Portugal, 2021. [Google Scholar]
- Resende, P.R.; Morais, L.C.; Pinho, C.; Afonso, A.M. Combustion characteristics of premixed hydrogen/air in an undulate microchannel. Energies 2022, 15, 626. [Google Scholar] [CrossRef]
- Resende, P.R.; Ferrás, L.L.; Afonso, A.M. Flame dynamics of hydrogen/air mixture in a wavy micro-channel. Int. J. Hydrog. Energy 2023, 48, 13682–13698. [Google Scholar] [CrossRef]
- Li, J.; Chou, S.; Li, Z.; Yang, W. A comparative study of H2-air premixed flame in micro combustors with different physical and boundary conditions. Combust. Theory Model. 2008, 12, 325–347. [Google Scholar] [CrossRef]
- Ronney, P.D. Analysis of non-adiabatic heat-recirculating combustors. Combust. Flame 2003, 135, 421–439. [Google Scholar] [CrossRef]
- Kim, N.I.; Kato, S.; Kataoka, T.; Yokomori, T.; Maruyama, S.; Fujimori, T.; Maruta, K. Flame stabilization and emission of small Swiss-roll combustors as heaters. Combust. Flame 2005, 141, 229–240. [Google Scholar] [CrossRef]
- Kuo, C.; Ronney, P. Numerical modeling of non-adiabatic heat-recirculating combustors. Proc. Combust. Inst. 2007, 31, 3277–3284. [Google Scholar] [CrossRef]
- Kunte, A.; Raghu, A.K.; Kaisare, N.S. A spiral microreactor for improved stability and performance for catalytic combustion of propane. Chem. Eng. Sci. 2018, 187, 87–97. [Google Scholar] [CrossRef]
- Yedala, N.; Raghu, A.K.; Kaisare, N.S. A 3D CFD study of homogeneous-catalytic combustion of hydrogen in a spiral microreactor. Combust. Flame 2019, 206, 441–450. [Google Scholar] [CrossRef]
- Pizza, G.; Mantzaras, J.; Frouzakis, C.E.; Tomboulides, A.G.; Boulouchos, K. Suppression of combustion instabilities of premixed hydrogen/air flames in microchannels using heterogeneous reactions. Proc. Combust. Inst. 2009, 32, 3051–3058. [Google Scholar] [CrossRef]
- Pizza, G.; Mantzaras, J.; Frouzakis, C.E. Flame dynamics in catalytic and non-catalytic mesoscale microreactors. Catal. Today 2010, 155, 123–130. [Google Scholar] [CrossRef]
- Zhou, J.; Wang, Y.; Yang, W.; Liu, J.; Wang, Z.; Cen, K. Combustion of hydrogen–air in catalytic micro-combustors made of different material. Int. J. Hydrogen Energy 2009, 34, 3535–3545. [Google Scholar] [CrossRef]
- Yang, W.M.; Chou, S.; Shu, C.; Li, Z.; Xue, H. Combustion in micro-cylindrical combustors with and without a backward facing step. Appl. Therm. Eng. 2002, 22, 1777–1787. [Google Scholar] [CrossRef]
- Li, J.; Chou, S.; Huang, G.; Yang, W.; Li, Z. Study on premixed combustion in cylindrical micro combustors: Transient flame behavior and wall heat flux. Exp. Therm. Fluid Sci. 2009, 33, 764–773. [Google Scholar] [CrossRef]
- Khandelwal, B.; Deshpande, A.A.; Kumar, S. Experimental studies on flame stabilization in a three step rearward facing configuration based micro channel combustor. Appl. Therm. Eng. 2013, 58, 363–368. [Google Scholar] [CrossRef]
- Faramarzpour, H.; Mazaheri, K.; Alipoor, A. Effect of backward facing step on radiation efficiency in a micro combustor. Int. J. Therm. Sci. 2018, 132, 129–136. [Google Scholar] [CrossRef]
- Fan, A.; Wan, J.; Liu, Y.; Pi, B.; Yao, H.; Liu, W. Effect of bluff body shape on the blow-off limit of hydrogen/air flame in a planar micro-combustor. Appl. Therm. Eng. 2014, 62, 13–19. [Google Scholar] [CrossRef]
- Yang, W.; Deng, C.; Zhou, J.; Liu, J.; Wang, Y.; Cen, K. Experimental and numerical investigations of hydrogen–air premixed combustion in a converging–diverging micro tube. Int. J. Hydrogen Energy 2014, 39, 3469–3476. [Google Scholar] [CrossRef]
- Biswas, S.; Zhang, P.; Wang, H.; Qiao, L. Propagation and extinction behavior of methane/air premixed flames through straight and converging-diverging microchannels. Appl. Therm. Eng. 2019, 148, 1395–1406. [Google Scholar] [CrossRef]
- Rao, S.S.; Raju, V. Effect of Deflector on the Combustion Characteristics of a Micro-Combustor With a Controlled Centrally Slotted Bluff Body. J. Energy Resour. Technol. 2024, 146, 022303-1. [Google Scholar]
- Zhang, C.; Yan, Y.; Shen, K.; Xue, Z.; You, J.; He, Z. Comparative analysis of combustion stability and flow performance in micro combustor based on the synergistic action of slotted blunt body and front-baffle. Appl. Therm. Eng. 2024, 237, 121802. [Google Scholar] [CrossRef]
- Abbaspour, P.; Alipoor, A. Numerical study of combustion characteristics and oscillating behaviors of hydrogen–air combustion in converging–diverging microtubes. Int. J. Heat Mass Transf. 2020, 159, 120127. [Google Scholar] [CrossRef]
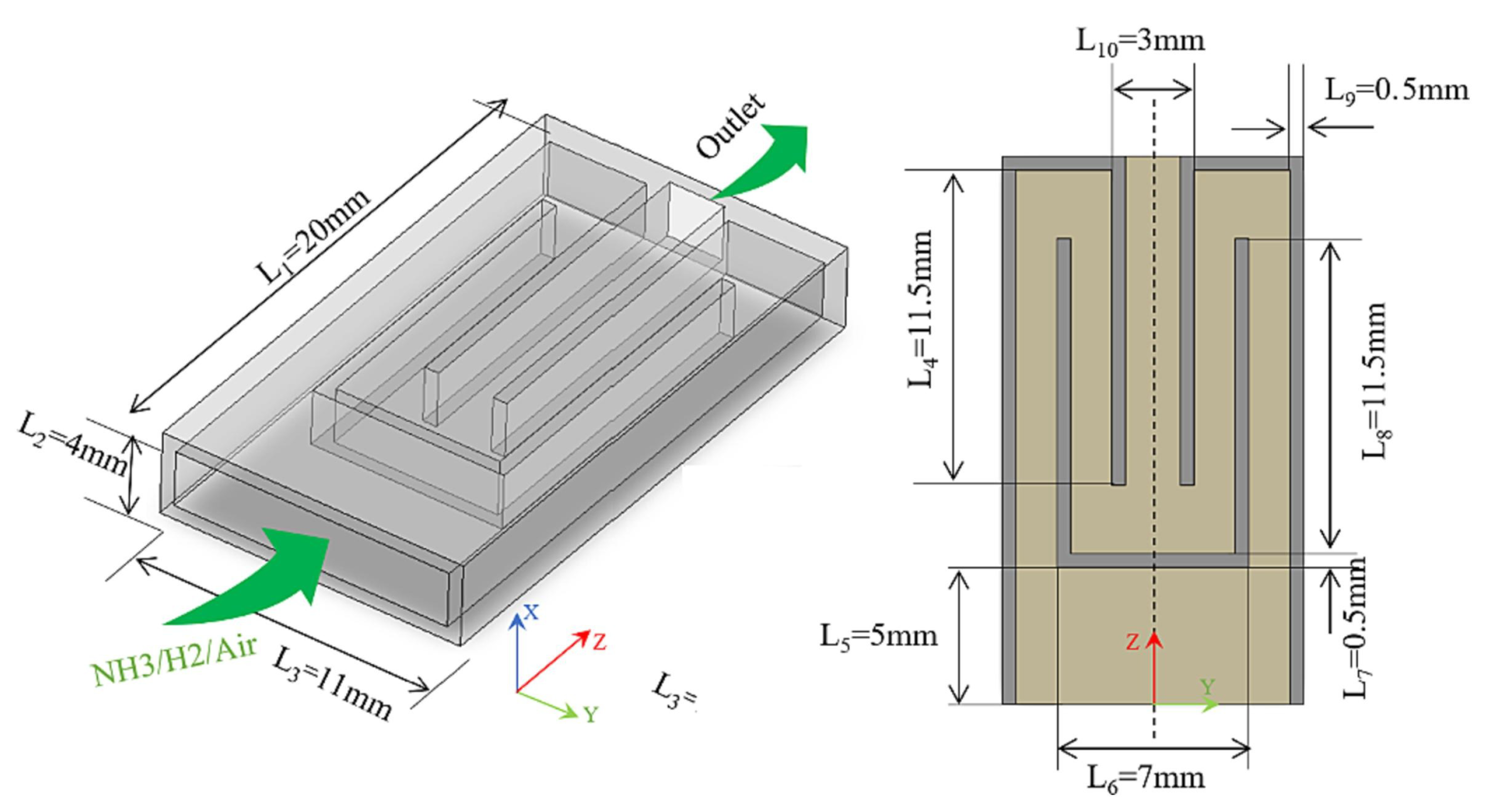
- Zhao, H.; Zhao, D.; Becker, S. Thermal performances investigation on an ammonia-fuelled heat-recirculating micro-combustor with reduced chemical mechanism. Appl. Therm. Eng. 2024, 236, 121685. [Google Scholar] [CrossRef]
- Li, J.; Jiaqiang, E.; Ding, J.; Cai, L.; Luo, B. Effect analysis on combustion performance enhancement of the hydrogen-fueled micro-cylindrical combustors with twisted tapes for micro-thermophotovoltaic applications. Int. J. Hydrogen Energy 2024, 49, 725–743. [Google Scholar] [CrossRef]
- He, Z.; Yan, Y.; Zhao, T.; Feng, S.; Li, X.; Zhang, L.; Zhang, Z. Heat transfer enhancement and exergy efficiency improvement of a micro combustor with internal spiral fins for thermophotovoltaic systems. Appl. Therm. Eng. 2021, 189, 116723. [Google Scholar] [CrossRef]
- Wan, J.; Yang, W.; Fan, A.; Liu, Y.; Yao, H.; Liu, W.; Du, Y.; Zhao, D. A numerical investigation on combustion characteristics of H2/air mixture in a micro-combustor with wall cavities. Int. J. Hydrogen Energy 2014, 39, 8138–8146. [Google Scholar] [CrossRef]
- Yang, W.; Xiang, Y.; Fan, A.; Yao, H. Effect of the cavity depth on the combustion efficiency of lean H2/air flames in a micro combustor with dual cavities. Int. J. Hydrogen Energy 2017, 42, 14312–14320. [Google Scholar] [CrossRef]
- Pizza, G.; Frouzakis, C.E.; Mantzaras, J.; Tomboulides, A.G.; Boulouchos, K. Dynamics of premixed hydrogen/air flames in mesoscale channels. Combust. Flame 2008, 155, 2–20. [Google Scholar] [CrossRef]
- Boyarko, G.A.; Sung, C.J.; Schneider, S.J. Catalyzed combustion of hydrogen–oxygen in platinum tubes for micro-propulsion applications. Proc. Combust. Inst. 2005, 30, 2481–2488. [Google Scholar] [CrossRef]
- Mansouri, Z. Combustion in wavy micro-channels for thermo-photovoltaic applications–Part I: Effects of wavy wall geometry, wall temperature profile and reaction mechanism. Energy Convers. Manag. 2019, 198, 111155. [Google Scholar] [CrossRef]
- Mansouri, Z. A novel wavy micro-combustor for micro-thermophotovoltaic applications. Chem. Eng. Process.-Process Intensif. 2021, 163, 108371. [Google Scholar] [CrossRef]
- Han, L.; Li, J.; Zhao, D.; Xi, Y.; Gu, X.; Wang, N. Effect analysis on energy conversion enhancement and NOx emission reduction of ammonia/hydrogen fuelled wavy micro-combustor for micro-thermophotovoltaic application. Fuel 2021, 289, 119755. [Google Scholar] [CrossRef]
- Abbaspour, P.; Alipoor, A. Numerical study of wavy-wall effects on premixed H2/air flammability limits, propagation modes, and thermal performance of micro combustion chambers. Appl. Energy 2024, 359, 122727. [Google Scholar] [CrossRef]
- You, Z. Chapter 8—Micropropulsion. In Space Microsystems and Micro/Nano Satellites; Micro and Nano Technologies; Butterworth-Heinemann: Oxford, UK, 2018; pp. 295–339. [Google Scholar] [CrossRef]
- Micci, M.; Ketsdever, A. Micropropulsion for Small Spacecraft; American Institute of Aeronautics and Astronautics: Reston, VA, USA, 2000. [Google Scholar]
- Sutton, G.; Biblarz, O. Rocket Propulsion Elements; A Wiley Interscience Publication, Wiley: Hoboken, NJ, USA, 2001. [Google Scholar]
- Merriam-Webster. Propulsion. 2011. Available online: https://www.merriam-webster.com/dictionary/propulsion (accessed on 28 April 2023).
- Rossi, C.; Rouhani, M.D.; Estève, D. Prediction of the performance of a Si-micromachined microthruster by computing the subsonic gas flow inside the thruster. Sens. Actuators A Phys. 2000, 87, 96–104. [Google Scholar] [CrossRef]
- Youngner, D.; Thai Lu, S.; Choueiri, E.; Neidert, J.; Black, R., III; Graham, K.; Fahey, D.; Lucus, R.; Zhu, X. MEMS mega-pixel micro-thruster arrays for small satellite stationkeeping. In Proceedings of the Small Satellite Conference, Logan, UT, USA, 21–24 August 2000. [Google Scholar]
- de Athayde Costa e Silva, M. MEMS Micropropulsion: Design, Modeling and Control of Vaporizing Liquid Microthrusters. Ph.D. Thesis, Delft University of Technology, Delft, The Netherlands, 2018. [Google Scholar] [CrossRef]
- NASA Glenn Research Center. Aeronautics: Aircraft Specifications and Performance. Available online: https://www.grc.nasa.gov/www/k-12/airplane/specimp.html (accessed on 21 July 2023).
- Huh, J.; Park, K.S. Effect of Structural Materials on Monopropellant Thruster Propulsion Performance in Micro Scale. Aerospace 2023, 10, 362. [Google Scholar] [CrossRef]
- Bretti, M. Ais-EPPT1 Pulsed Plasma Thruster. 2020. Available online: https://appliedionsystems.com/portfolio/ais-eppt1-pulsed-plasma-thruster/ (accessed on 10 May 2023).
- Spanjers, G. New Satellite Propulsion System Has Mass Below 100 Grams (0.22 Pounds); Technical Report; Air Force Research Lab Edwards AFB CA Space and Missile Propulsion Division: Kern County, CA, USA, 2001. [Google Scholar]
- Burton, R.L.; Turchi, P. Pulsed plasma thruster. J. Propuls. Power 1998, 14, 716–735. [Google Scholar] [CrossRef]
- Ciaralli, S.; Coletti, M.; Gabriel, S. Performance and lifetime testing of a pulsed plasma thruster for Cubesat applications. Aerosp. Sci. Technol. 2015, 47, 291–298. [Google Scholar] [CrossRef]
- Ciaralli, S.; Coletti, M.; Gabriel, S. Results of the qualification test campaign of a Pulsed Plasma Thruster for Cubesat Propulsion (PPTCUP). Acta Astronaut. 2016, 121, 314–322. [Google Scholar] [CrossRef]
- Coletti, M.; Marques, R.I.; Gabriel, S. Design of a two-stage Pulsed Plasma Thruster for cubesat application. In Proceedings of the 31st IEPC, IEPC-2009-244, Ann Arbor, MI, USA, 20–24 September 2009. [Google Scholar]
- Coletti, M.; Guarducci, F.; Gabriel, S. A micro Pulsed Plasma Thruster for Cubesat application: Design and preliminary experimental results. Acta Astronaut. 2011, 69, 200–208. [Google Scholar] [CrossRef]
- Tanaka, M.; Kisaki, S.; Ikeda, T.; Tahara, H. Research and development of pulsed plasma thruster systems for nano-satellites at Osaka Institute of Technology. In Proceedings of the 2012 IEEE Vehicle Power and Propulsion Conference, Seoul, Republic of Korea, 9–12 October 2012; pp. 517–522. [Google Scholar]
- Fujita, R.; Tahara, H. Development of electrothermal pulsed plasma thruster systems onboard osaka Institute of technology PROITERES nano-satellites. In Proceedings of the 50th AIAA/ASME/SAE/ASEE Joint Propulsion Conference, Cleveland, OH, USA, 28–30 July 2014; p. 3610. [Google Scholar]
- Bock, D.; Tajmar, M. Highly miniaturized FEEP propulsion system (NanoFEEP) for attitude and orbit control of CubeSats. Acta Astronaut. 2018, 144, 422–428. [Google Scholar] [CrossRef]
- Galilei, G. Advanced GG Phase a Study. Available online: http://eotvos.dm.unipi.it/ggweb/phaseA/ (accessed on 22 May 2023).
- Zhong, Y.; Liu, J.; Li, Z.; Wang, Y.; Li, W.; Zhang, H.; Zou, S.; Cai, B.; Gong, Y.; Tu, L.; et al. Study of microchannel structures for measuring emission conductance of indium field emission electric propulsion micro-thrusters. Measurement 2024, 224, 113809. [Google Scholar] [CrossRef]
- Leach, R.; Neal, K. Discussion of micro-newton thruster requirements for a drag-free control system. In Proceedings of the Small Satellite Conference, Logan, UT, USA, 12–15 August 2002. Session VIII: Advanced Technologies & Subsystems, Components & Sensors II. [Google Scholar]
- Mitterauer, J. Micropropulsion for small spacecraft: A new challenge for field effect electric propulsion and microstructured liquid metal ion sources. Surf. Interface Anal. 2004, 36, 380–386. [Google Scholar] [CrossRef]
- Tajmar, M.; Scharlemann, C. Development of electric and chemical microthrusters. Int. J. Aerosp. Eng. 2011, 2011, 361215. [Google Scholar] [CrossRef]
- Rüdenauer, F. Field emission devices for space applications. Surf. Interface Anal. 2007, 39, 116–122. [Google Scholar] [CrossRef]
- Räisänen, O. A Diagram of an Electrostatic Ion Thruster. 2012. Available online: https://en.wikipedia.org/wiki/Gridded_ion_thruster#/media/File:Electrostatic_ion_thruster-en.svg (accessed on 6 May 2023).
- Dong, L.; Lu, S.; Guo, N.; Liang, S.; Long, J.; Luo, W.; Xu, L. Study of the thrust response characteristics of Hall Micro Thruster. Results Phys. 2024, 57, 107338. [Google Scholar] [CrossRef]
- The National Aeronautics and Space Administration. Solar Sail Fact Sheet. 2005. Available online: https://www.nasa.gov/pdf/134645main_solar_sail_fs.pdf (accessed on 27 May 2023).
- The European Space Agency. Solar Sails. Available online: https://www.esa.int/Education/Solar_sails (accessed on 22 May 2023).
- Leipold, M.; Eiden, M.; Garner, C.; Herbeck, L.; Kassing, D.; Niederstadt, T.; Krüger, T.; Pagel, G.; Rezazad, M.; Rozemeijer, H.; et al. Solar sail technology development and demonstration. Acta Astronaut. 2003, 52, 317–326. [Google Scholar] [CrossRef]
- Miller, R. Solar Sails: The Math Behind Solar Sails. 2018. Available online: http://ffden-2.phys.uaf.edu/webproj/212_spring_2015/Robert_Miller/physics.html (accessed on 6 May 2023).
- Kantrowitz, A. Propulsion to orbit by ground based lasers. Aeronaut. Astronaut. 1972, 10, 74–76. [Google Scholar]
- Rao, S.; Xu, Y.; Yuan, J.; Liu, F.; Wang, S.; Jiang, H.; Cheng, G.J. MOFs for Ultrahigh Efficiency Pulsed Laser Micropropulsion. Adv. Mater. 2024, 36, 2306228. [Google Scholar] [CrossRef]
- Rao, S.; Yi, W.; Yuan, J.; Wang, S.; Jiang, H.; Cheng, G.J. Advancing laser micropropulsion: High performance with MOF-derived carbon-encapsulated-nano-metal composites. Matter 2024. [Google Scholar] [CrossRef]
- National Research Council. Microelectromechanical Systems: Advanced Materials and Fabrication Methods; National Academies Press: Washington, DC, USA, 1998. [Google Scholar]
- Müller, J.; Marrese, C.; Polk, J.; Yang, E.H.; Green, A.; White, V.; Bame, D.; Chakraborty, I.; Vargo, S.; Reinicke, R. An overview of MEMS-based micropropulsion developments at JPL. In Proceedings of the 3rd International Symposium of the International Academy of Astronautics (IAA) for Small Satellites for Earth Observation, Berlin, Germany, 2–6 April 2001. [Google Scholar]
- Butler, E.; Folk, C.; Cohen, A.; Vasilyev, N.; Chen, R.; del Nido, P.; Dupont, P. Metal MEMS Tools for Beating-heart Tissue Approximation. IEEE Int. Conf. Robot. Autom. 2011, 2011, 411–416. [Google Scholar] [CrossRef] [PubMed]
- Bayt, R.L. Analysis, Fabrication and Testing of a MEMS-Based Micropropulsion System; Technical Report; Aerospace Computational Design Laboratory, Department of Aeronautics: Cambridge, MA, USA, 1999. [Google Scholar]
- Bennett, S.; Huss, W.; John, R.; Tuchman, A. Experimental propulsion performance of a low power pulsed resistojet. In Proceedings of the 2nd Aerospace Sciences Meeting, New York, NY, USA, 25–27 January 1965; p. 97. [Google Scholar]
- Page, R.J.; Halbach, C.R.; Short, R.A. 3-KW concentric tubular resistojet performance. J. Spacecr. Rocket. 1966, 3, 1669–1674. [Google Scholar] [CrossRef]
- Pugmire, T.K.; Shaw, R.; Enos, G.R. Applied resistojet technology. J. Spacecr. Rocket. 1971, 8, 63–68. [Google Scholar] [CrossRef]
- Page, R.; Short, R. Design of high-performance resistojets for advanced spacecraft. In Proceedings of the 9th Aerospace Sciences Meeting, New York, NY, USA, 25–27 January 1971; p. 195. [Google Scholar]
- Kundu, P.; Bhattacharyya, T.K.; Das, S. Design, fabrication and performance evaluation of a vaporizing liquid microthruster. J. Micromech. Microeng. 2012, 22, 025016. [Google Scholar] [CrossRef]
- Cen, J.; Xu, J. Performance evaluation and flow visualization of a MEMS based vaporizing liquid micro-thruster. Acta Astronaut. 2010, 67, 468–482. [Google Scholar] [CrossRef]
- Silva, M.A.; Guerrieri, D.C.; van Zeijl, H.; Cervone, A.; Gill, E. Vaporizing liquid microthrusters with integrated heaters and temperature measurement. Sens. Actuators A Phys. 2017, 265, 261–274. [Google Scholar] [CrossRef]
- Ahmed, Z.; Gimelshein, S.F.; Ketsdever, A.D. Numerical analysis of free-molecule microresistojet performance. J. Propuls. Power 2006, 22, 749–756. [Google Scholar] [CrossRef]
- Ketsdever, A.; Wadsworth, D.; Vargo, S.; Muntz, E. The free molecule micro-resistojet-An interesting alternative to nozzle expansion. In Proceedings of the 34th AIAA/ASME/SAE/ASEE Joint Propulsion Conference and Exhibit, Cleveland, OH, USA, 13–15 July 1998; p. 3918. [Google Scholar]
- Guerrieri, D.C.; Cervone, A.; Gill, E. Analysis of nonisothermal rarefied gas flow in diverging microchannels for low-pressure microresistojets. J. Heat Transf. 2016, 138, 112403. [Google Scholar] [CrossRef]
- Guerrieri, D.C.; Silva, M.A.; Cervone, A.; Gill, E. Selection and characterization of green propellants for micro-resistojets. J. Heat Transf. 2017, 139, 102001. [Google Scholar] [CrossRef]
- Cervone, A.; Mancas, A.; Zandbergen, B. Conceptual design of a low-pressure micro-resistojet based on a sublimating solid propellant. Acta Astronaut. 2015, 108, 30–39. [Google Scholar] [CrossRef]
- Guerrieri, D.C.; Silva, M.A.; Van Zeijl, H.; Cervone, A.; Gill, E. Fabrication and characterization of low pressure micro-resistojets with integrated heater and temperature measurement. J. Micromech. Microeng. 2017, 27, 125005. [Google Scholar] [CrossRef]
- Guerrieri, D.C.; Silva, M.A.; Cervone, A.; Gill, E. An analytical model for characterizing the thrust performance of a Low-Pressure Micro-Resistojet. Acta Astronaut. 2018, 152, 719–726. [Google Scholar] [CrossRef]
- Mukerjee, E.; Wallace, A.; Yan, K.; Howard, D.; Smith, R.; Collins, S. Vaporizing liquid microthruster. Sens. Actuators A Phys. 2000, 83, 231–236. [Google Scholar] [CrossRef]
- You, Z. Space Microsystems and Micro/Nano Satellites; Butterworth-Heinemann: Oxford, UK, 2017. [Google Scholar]
- Pranajaya, F. Progress on Colloid Micro-Thruster Research and Flight Testing; Space Systems Development Laboratory, Department of Aeronautics and Astronautics, Stanford University: Stanford, CA, USA, 1999. [Google Scholar]
- Berg, S.P.; Rovey, J.; Prince, B.; Miller, S.; Bemish, R. Electrospray of an energetic ionic liquid monopropellant for multi-mode micropropulsion applications. In Proceedings of the 51st AIAA/SAE/ASEE Joint Propulsion Conference, Orlando, FL, USA, 27–29 July 2015; p. 4011. [Google Scholar]
- Berg, S.P.; Rovey, J. Decomposition of a double salt ionic liquid monopropellant on heated metallic surfaces. In Proceedings of the 52nd AIAA/SAE/ASEE Joint Propulsion Conference, Salt Lake City, UT, USA, 25–27 July 2016; p. 4578. [Google Scholar]
- Lewis, D.H., Jr.; Janson, S.W.; Cohen, R.B.; Antonsson, E.K. Digital micropropulsion. Sens. Actuators A Phys. 2000, 80, 143–154. [Google Scholar] [CrossRef]
- Rossi, C.; Do Conto, T.; Esteve, D.; Larangot, B. Design, fabrication and modelling of MEMS-based microthrusters for space application. Smart Mater. Struct. 2001, 10, 1156. [Google Scholar] [CrossRef]
- Rossi, C.; Orieux, S.; Larangot, B.; Do Conto, T.; Esteve, D. Design, fabrication and modeling of solid propellant microrocket-application to micropropulsion. Sens. Actuators A Phys. 2002, 99, 125–133. [Google Scholar] [CrossRef]
- Rossi, C.; Larangot, B.; Lagrange, D.; Chaalane, A. Final characterizations of MEMS-based pyrotechnical microthrusters. Sens. Actuators A Phys. 2005, 121, 508–514. [Google Scholar] [CrossRef]
- Seo, D.; Lee, J.; Kwon, S. The development of the micro-solid propellant thruster array with improved repeatability. J. Micromech. Microeng. 2012, 22, 094004. [Google Scholar] [CrossRef]
- Lee, J.; Jo, H.; Kim, S.; Lee, E.; Son, Y.; Lee, A. Lab-on-PCB for space propulsion: Integrated membraneless micro-ignitor for MEMS solid propellant thruster. Sens. Actuators A Phys. 2023, 363, 114696. [Google Scholar] [CrossRef]
- Lee, J.; Kim, S.; Jo, H.; Lee, E.; Lee, A. Design and fabrication of a scalable solid-propellant micro-thruster array using lab-on-PCB technology. Sens. Actuators A Phys. 2023, 363, 114738. [Google Scholar] [CrossRef]
- Kan, W.; Liu, W.; Lou, W.; Feng, H.; Chu, E.; Wang, J.; Ren, W. High-Safety Energetic Micro-Igniter for Micro-Thrust System. Sens. Actuators A Phys. 2024, 368, 115056. [Google Scholar] [CrossRef]
- Fahd, A.; Baranovsky, A.; Dubois, C.; Chaouki, J.; Elbasuney, S.; Shokry, S. Thrust characteristics of nano-carbon/Al/oxygenated salt nanothermites for micro-energetic applications. Def. Technol. 2023, 30, 55–69. [Google Scholar] [CrossRef]
- Patel, K.D.; Bartsch, M.S.; McCrink, M.H.; Olsen, J.S.; Mosier, B.P.; Crocker, R.W. Electrokinetic pumping of liquid propellants for small satellite microthruster applications. Sens. Actuators B Chem. 2008, 132, 461–470. [Google Scholar] [CrossRef]
- Hitt, D.L.; Zakrzwski, C.M.; Thomas, M.A. MEMS-based satellite micropropulsion via catalyzed hydrogen peroxide decomposition. Smart Mater. Struct. 2001, 10, 1163. [Google Scholar] [CrossRef]
- Kundu, P.; Sinha, A.K.; Bhattacharyya, T.K.; Das, S. MnO2 Nanowire Embedded Hydrogen Peroxide Monopropellant MEMS Thruster. J. Microelectromech. Syst. 2012, 22, 406–417. [Google Scholar] [CrossRef]
- Xu, J.; Zhang, J.; Li, F.; Liu, S.; Ye, Y.; Shen, R. A review on solid propellant micro-thruster array based on MEMS technology. FirePhysChem 2023. [Google Scholar] [CrossRef]
- Chen, C.P.; Chao, Y.C.; Wu, C.Y.; Lee, J.C.; Chen, G.B. Development of a catalytic hydrogen micro-propulsion system. Combust. Sci. Technol. 2006, 178, 2039–2060. [Google Scholar] [CrossRef]
- Armano, M.; Audley, H.; Baird, J.; Binetruy, P.; Born, M.; Bortoluzzi, D.; Castelli, E.; Cavalleri, A.; Cesarini, A.; Cruise, A.M.; et al. LISA Pathfinder micronewton cold gas thrusters: In-flight characterization. Phys. Rev. D 2019, 99, 122003. [Google Scholar] [CrossRef]








| Time Scales | Length Scales |
|---|---|
| Flow residence time, | Combustor scales 1, d |
| Characteristic combustion time, | Combustor structure scales 2, |
| Diffusion time scale of gas-phase, | Flame thickness, |
| Diffusion time scale of solid-phase, | Quenching diameter 3, |
| Time scale of heat loss 4, | Mass diffusion length, |
| Time scale of acoustic wave 5, | Thermal diffusion length, |
| Ignition time scale, | Mean free path, |
| Characteristic flow time, | - |
| Non-Dimensional Numbers | Phenomena |
|---|---|
| or | Flame extinction due to heat loss to the wall |
| Wall catalytic/quenching effects, flame street & cells | |
| Flame-wall thermal coupling, multiple flame regime | |
| Extinction and reignition instability, Weak flame | |
| Flameless combustion | |
| Small wall temperature change | |
| Steady-state temperature distribution in solid phase | |
| Non-equilibrium transport effect | |
| or | Flame extinction due to incomplete combustion |
| Pulsating instability via flame structure coupling | |
| Acoustic instability |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dias, D.M.; Resende, P.R.; Afonso, A.M. A Review on Micro-Combustion Flame Dynamics and Micro-Propulsion Systems. Energies 2024, 17, 1327. https://doi.org/10.3390/en17061327
Dias DM, Resende PR, Afonso AM. A Review on Micro-Combustion Flame Dynamics and Micro-Propulsion Systems. Energies. 2024; 17(6):1327. https://doi.org/10.3390/en17061327
Chicago/Turabian StyleDias, David M., Pedro R. Resende, and Alexandre M. Afonso. 2024. "A Review on Micro-Combustion Flame Dynamics and Micro-Propulsion Systems" Energies 17, no. 6: 1327. https://doi.org/10.3390/en17061327
APA StyleDias, D. M., Resende, P. R., & Afonso, A. M. (2024). A Review on Micro-Combustion Flame Dynamics and Micro-Propulsion Systems. Energies, 17(6), 1327. https://doi.org/10.3390/en17061327