Insulation Resistance Characteristics of Dry DC Link Capacitors in the Presence of High Temperatures and Operating Voltages
Abstract
1. Introduction
2. Insulation Resistance Characteristics of Metalized Polypropylene
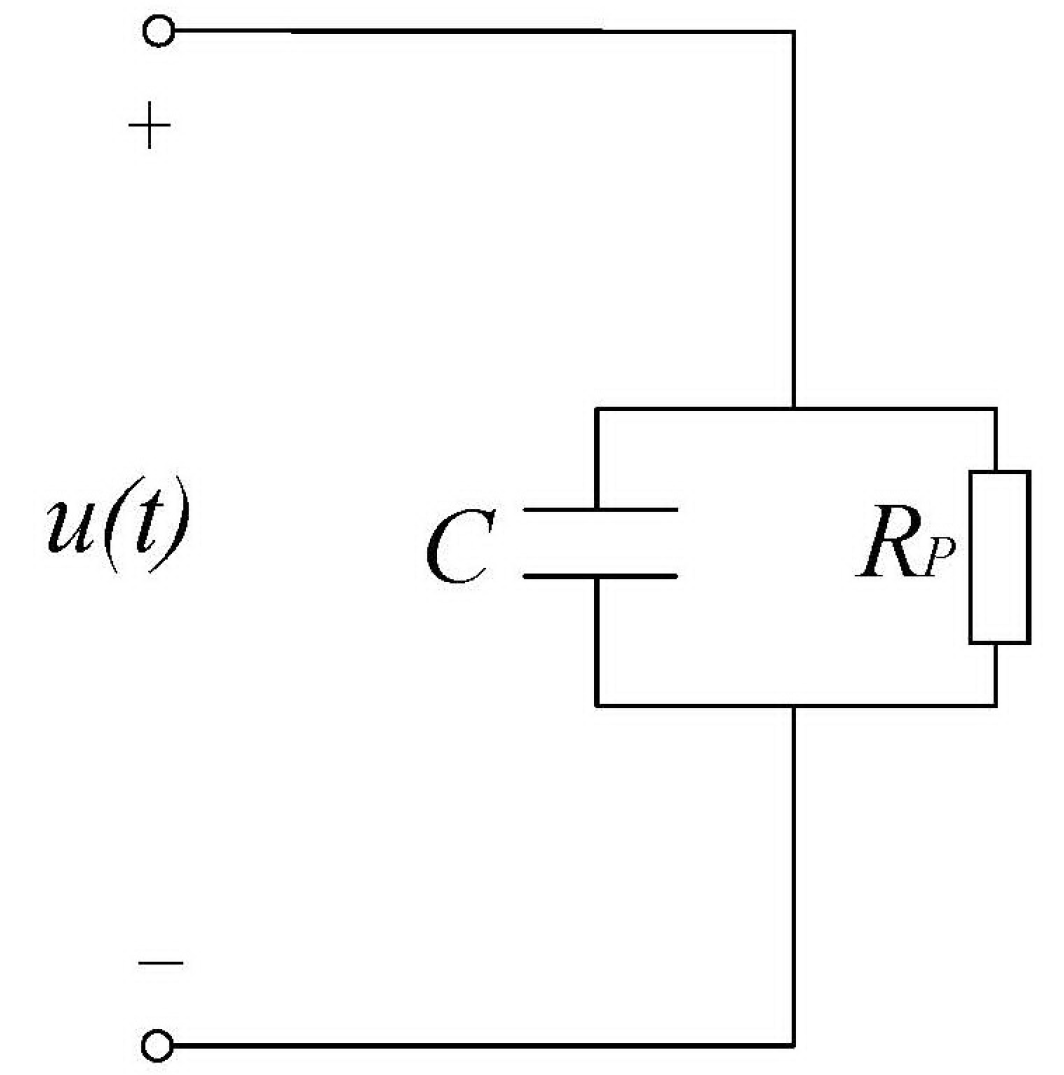
2.1. Insulation Resistance
- (1)
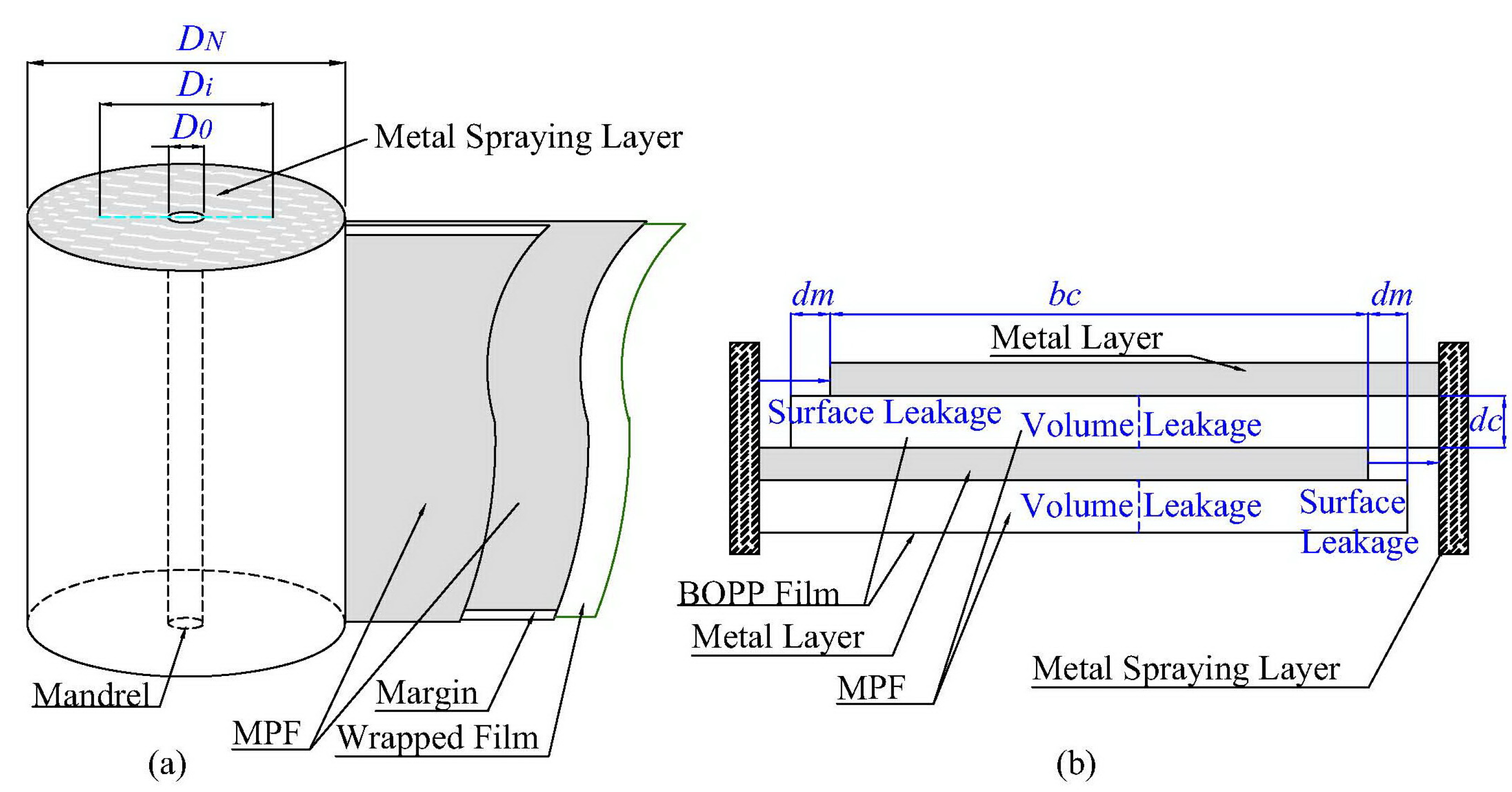
- The insulation resistance of the DCLC is related to the volume resistivity, the thickness and margin width of the MPF, the effective width and length of the electrode, and the surface conductivity.
- (2)
- The DCLC’s self-discharge time constant depends on the dielectric constant, the volume resistivity, the thickness and margin width of the MPF, and the surface conductivity.
- (3)
- Conductivity is the key parameter that affects the equivalent insulation resistance.
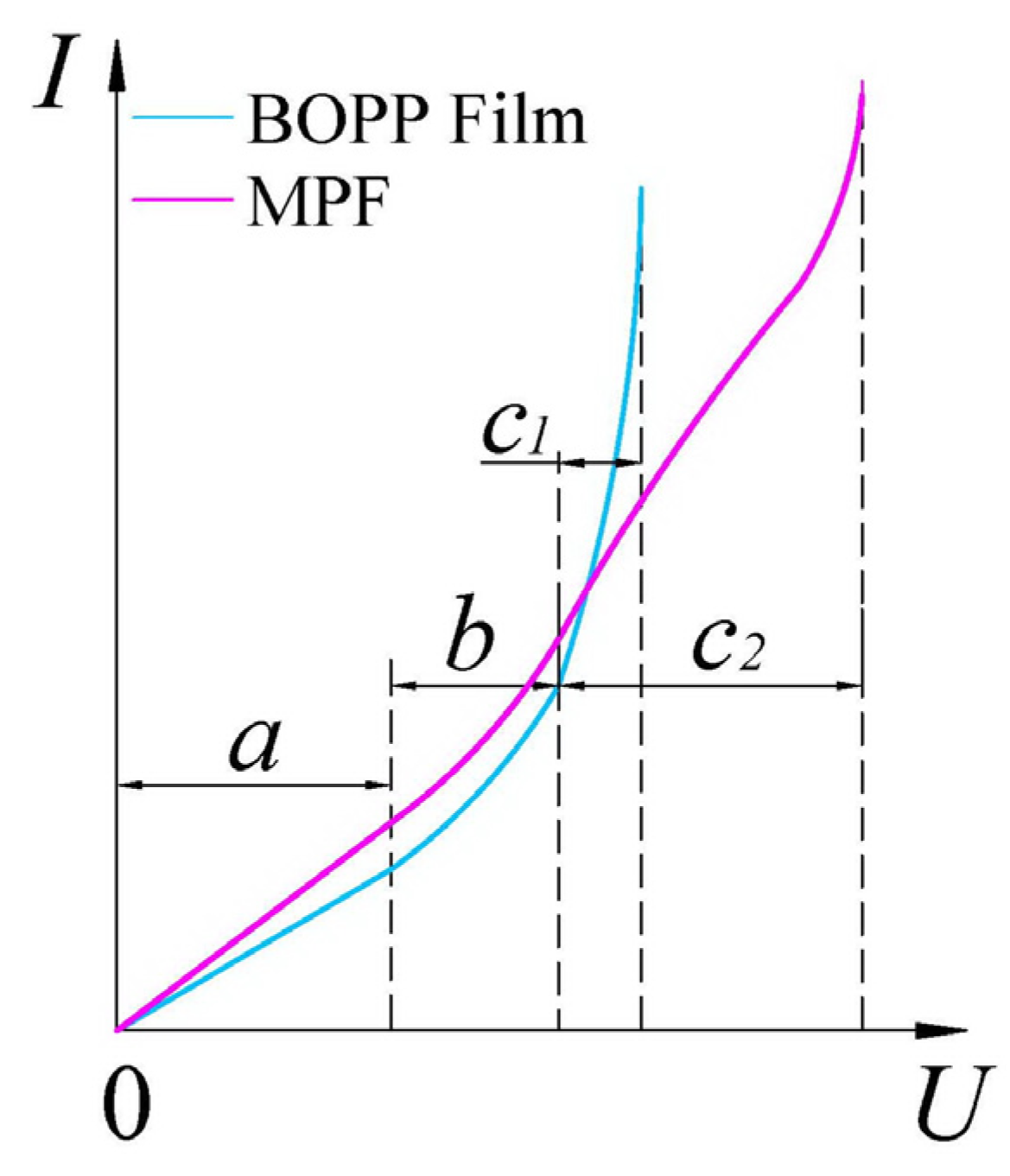
2.2. Dielectric Conductance
3. Experimental Preparation
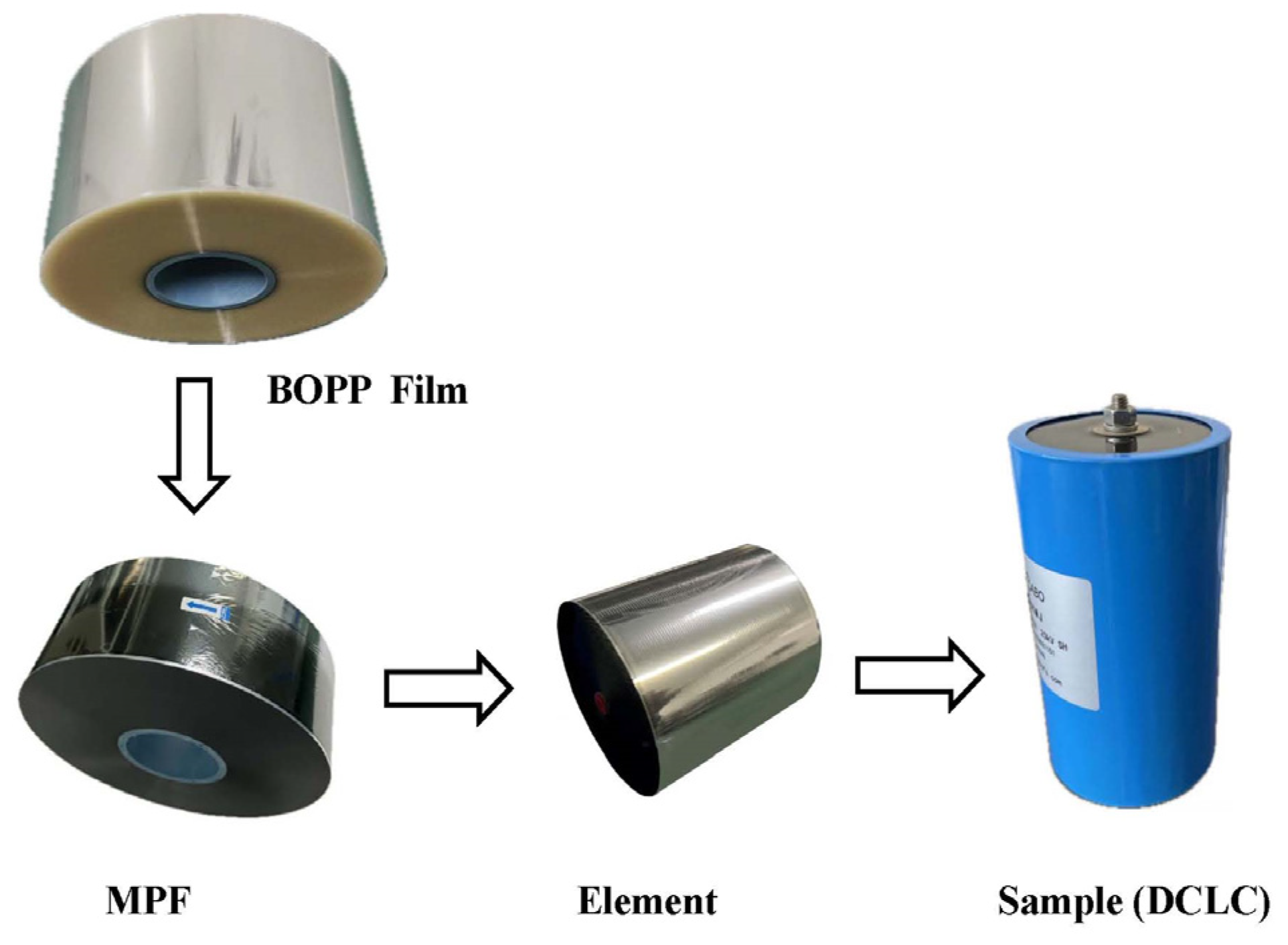
3.1. Sample and Preparation
3.2. Measurement of the Insulation Resistance
4. Experimental Results and Discussion
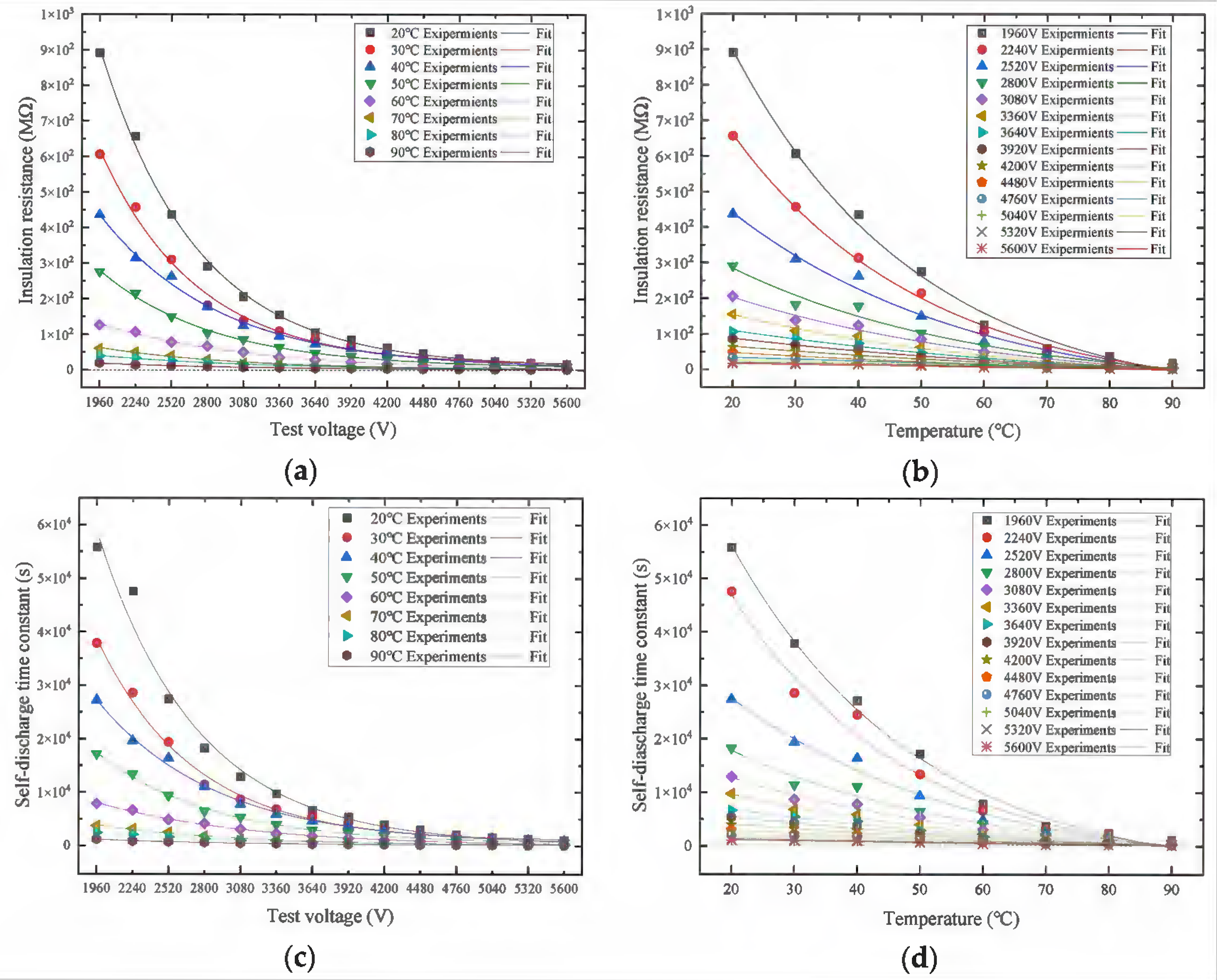
4.1. Insulation Resistance Measurement
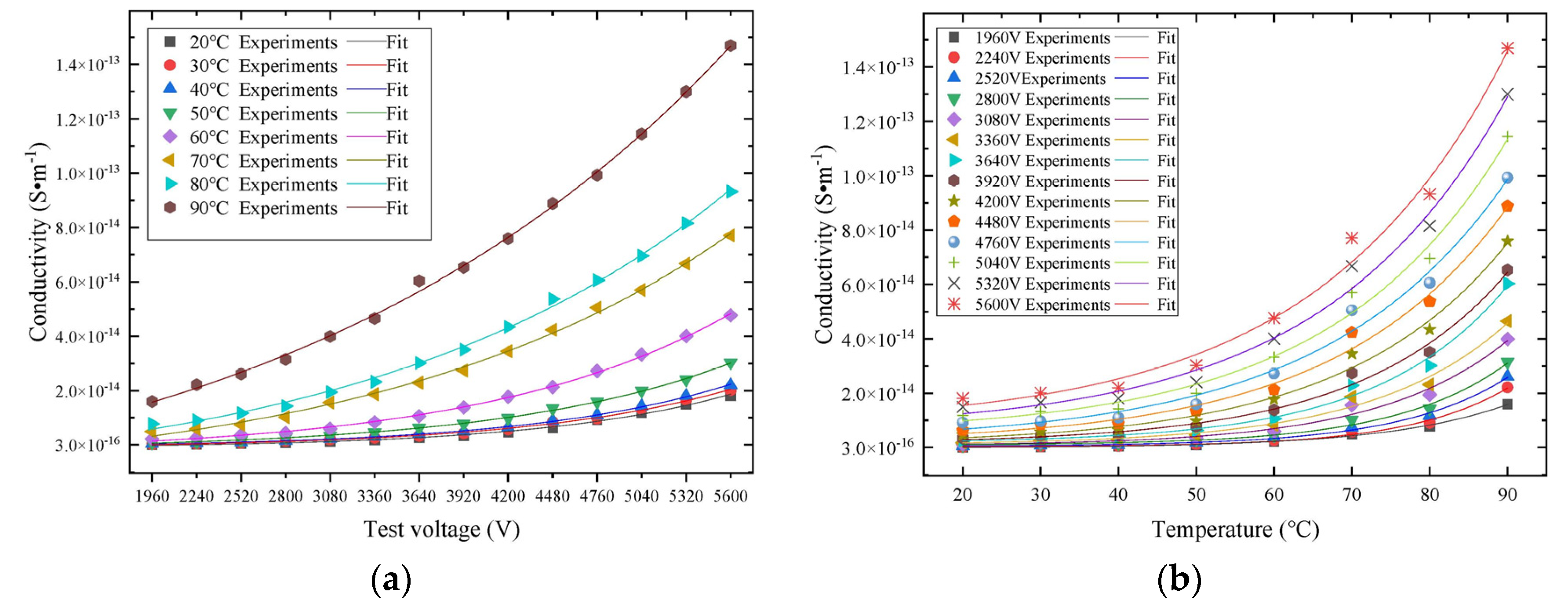
4.2. Conductivity Calculations
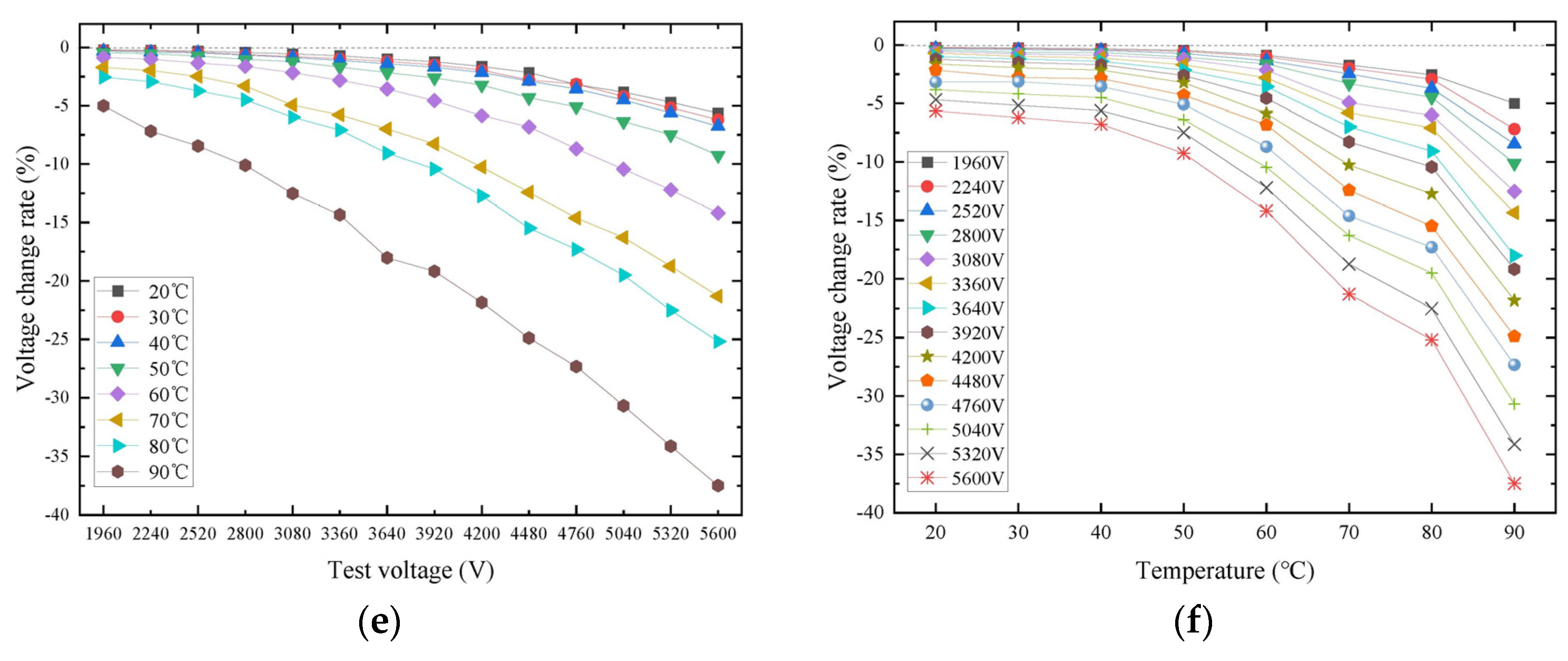
4.3. Effect of Insulation Resistance
5. Conclusions
- (1)
- Under the same operating temperature, the insulation resistance (self-discharge time constant) of the DCLC decreases with the growth in the operating voltage, and the decrease in the insulation resistance (self-discharge time constant) is lessened for the case of operating voltages higher than 4480 V (1.6E0 = 386.21 V/μm).
- (2)
- Under the same operating voltage, the insulation resistance (self-discharge time constant) of the DCLC decreases exponentially with the rise in temperature, and the decrease in the insulation resistance (self-discharge time constant) is lessened when the temperature is higher than 70 °C.
- (3)
- For temperatures lower than 70 °C, the rate of change in the DCLC’s voltage decreases slowly as the operating voltage increases. As the temperature varies in the interval of 70–90 °C, the rate of change in the DCLC’s voltage drastically decreases as the operating voltage increases. Additionally, under the same operating voltage, the rate of change in the DCLC’s voltage increases with the increase in temperature.
- (4)
- The conductivity of the MPF of the DCLC slowly increases in an exponential manner as the temperature increases, and when the temperature is higher than 70–90 °C, the conductivity of the MPF of the DCLC increases sharply with the rise in temperature.
- (5)
- The conductivity of the MPF of the DCLC rises slowly and exponentially with the increase in operating voltage, and when the operating voltage is higher than 4480 V and the temperature is higher than 70 °C, the conductivity of the MPF of the DCLC drastically rises with the growth in the operating voltage.
- (6)
- As the operating voltage increases from 1960 V (0.7E0 = 168.97 V/μm) to 5600 V (2E0 = 482.76 V/μm) and the temperature rises from 20 °C to 90 °C, the DCLC’s insulation resistance reduces from 891. MΩ to 2.14 MΩ. By raising the operating voltage from 1960 V to 5600 V and the temperature from 20 °C to 90 °C, the DCLC’s self-discharge time constant lessens from 55,834 s to 132 s. By increasing the operating voltage from 1960 V to 5600 V and the temperature from 20 °C to 90 °C, the DCLC’s conductivity increases from 3.49 × 10−16 S/m to 1.47 × 10−13 S/m.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Rytöluoto, I.; Niittymäki, M.; Seri, P.; Naderiallaf, H.; Lahti, K.; Saarimäki, E.; Flyktman, T.; Paajanen, M. Biaxially oriented silica-polypropylene nanocomposites for HVDC film capacitors: Morphology-dielectric property relationships, and critical evaluation of the current progress and limitations. J. Mater. Chem. A 2022, 10, 3025–3043. [Google Scholar] [CrossRef]
- Sarjeant, W.J.; Zirnheld, J.; MacDougall, F.W. Capacitors. IEEE T Plasma Sci. 1998, 26, 1368–1392. [Google Scholar] [CrossRef]
- Rabuffi, M.; Picci, G. Status quo and future prospects for metallized polypropylene energy storage capacitors. IEEE Trans. Plasma Sci. 2002, 30, 1939–1942. [Google Scholar] [CrossRef]
- Makdessi, M.; Sari, A.; Venet, P. Metallized polymer film capacitors ageing law based on capacitance degradation. Microelectron. Reliab. 2014, 54, 1823–1827. [Google Scholar] [CrossRef]
- Lv, C.; Liu, J.; Zhang, Y.; Yin, J.; Cao, R.; Li, Y.; Liu, X. A Method to Characterize the Shrinking of Safe Operation Area of Metallized Film Capacitor Considering Electrothermal Coupling and Aging in Power Electronics Applications. IEEE Trans. Ind. Electron. 2022, 70, 1993–2002. [Google Scholar] [CrossRef]
- Du, B. Polymer Insulation Applied for HVDC Transmission; Springer Nature: Berlin/Heidelberg, Germany, 2020. [Google Scholar]
- Xing, Z.; Gu, Z.; Zhang, C.; Guo, S.; Cui, H.; Lei, Q.; Li, G. Influence of Space Charge on Dielectric Property and Breakdown Strength of Polypropylene Dielectrics under Strong Electric Field. Energies 2022, 15, 4412. [Google Scholar] [CrossRef]
- Cheng, L.; Li, Z.; Wang, J.; Xu, Z.; Liu, W.; Li, S. Degradation Behavior and Mechanism of Metalized Film Capacitor under Ultrahigh Field. IEEE Trans. Dielect. Electr. Insul. 2023, 30, 509–517. [Google Scholar] [CrossRef]
- Kurimský, J.; Rajňák, M.; Cimbala, R. Pulse induced failures in bi-axially oriented polypropylene capacitors: Experimental investigation. Ain. Shams. Eng. J. 2023, 14, 102086. [Google Scholar] [CrossRef]
- Umemura, T.; Akiyama, K.; Couderc, D. Morphology and Electrical Properties of Biaxially-oriented Polypropylene Films. IEEE Trans. Dielect. Electr. Insul. 1986, 4, 137–144. [Google Scholar] [CrossRef]
- Karanja, P.K.; Nath, R. Charge trapping and conduction in pure and iodine-doped biaxially-oriented polypropylene. IEEE Trans. Dielectr. Electr. Insul. 1994, 1, 213–223. [Google Scholar] [CrossRef]
- Ho, J.; Jow, T.R. High field conduction in biaxially oriented polypropylene at elevated temperature. IEEE Trans. Dielectr. Electr. Insul. 2012, 19, 990–995. [Google Scholar] [CrossRef]
- Li, H.; Li, Z.; Lin, F.; Liu, D.; Wang, B.; Chen, Y.; Dai, L.; Fan, S. Electrical conduction of metallized BOPP films based on revised Poole–Frenkel effect. J. Electrostat. 2013, 71, 958–962. [Google Scholar] [CrossRef]
- Ran, Z.; Du, B.; Xiao, M.; Li, J. Crystallization Morphology-Dependent Breakdown Strength of Polypropylene Films for Converter Valve Capacitor. IEEE Trans. Dielect. Electr. Insul. 2021, 28, 964–971. [Google Scholar] [CrossRef]
- Liu, H.; Du, B.X.; Xiao, M.; Ran, Z. Improved Conductivity and Breakdown Performance of Polypropylene Film by Parylene Blending for Power Capacitor. IEEE Trans. Dielect. Electr. Insul. 2022, 29, 997–1004. [Google Scholar] [CrossRef]
- Xiao, M.; Zhao, Y.S.; Du, B.X. Dielectric Performance of Furan-Functionalized Polypropylene Film for HVDC Capacitors. IEEE Trans. Dielect. Electr. Insul. 2023, 31, 160–167. [Google Scholar] [CrossRef]
- Zhang, S.; Tian, F.; Liang, J.; Cao, J.; Xing, Z. The Time, Electric Field, and Temperature Dependence of Charging and Discharging Currents in Polypropylene Films. Polymers 2023, 15, 3123. [Google Scholar] [CrossRef]
- Ieda, M.; Sawa, G.; Kato, S. A Consideration of Poole-Frenkel Effect on Electric Conduction in Insulators. J. Appl. Phys. 1971, 42, 3737–3740. [Google Scholar] [CrossRef]
- Karanja, P.; Nath, R. Electrical conduction in biaxially-oriented polypropylene. J. Electrostat. 1993, 31, 51–63. [Google Scholar] [CrossRef]
- Li, H.; Li, Z.; Xu, Z.; Lin, F.; Wang, B.; Li, H.; Zhang, Q.; Wang, W.; Huang, X. Electric Field and Temperature Dependence of Electrical Conductivity in Biaxially Oriented Polypropylene Films. IEEE T Plasma Sci. 2014, 42, 3585–3591. [Google Scholar] [CrossRef]
- Li, H.; Li, Z.; Lin, F.; Chen, Y.; Liu, D.; Wang, B. Insulation resistance of metallized polypropylene film capacitors under different electric fields. IEEE Trans. Dielect. Electr. Insul. 2013, 20, 1315–1320. [Google Scholar] [CrossRef]
- Michelazzi, M.; Fabiani, D. Electrical Conduction in Thin-Film Polypropylene Capacitors. Energies 2023, 16, 6631. [Google Scholar] [CrossRef]
- Wang, Y.; Li, H.; Wang, Z.; Lin, F. Voltage maintaining performance of metallized film capacitors based on crystallinity regulation. High Volt. Eng. 2022, 48, 3643–3650. [Google Scholar]
- Li, Z.; Li, H.; Yang, P.; Lin, F.; Cheng, Y.; Liu, D.; Wang, B.; Zhang, Q. Leakage characteristic of metallized polypropylene film capacitors under different electric fields. Trans. China Electrotech. Soc. 2013, 28, 274–280. [Google Scholar]
- Chen, Y.; Zhang, M.; Li, H.; Lin, F.; Li, Z.; Lu, F.; Liu, D. Insulation resistance characteristics of metallized film capacitor under high electric field. High Power Laser Part. Beams 2012, 24, 797–800. [Google Scholar] [CrossRef]
- Yan, Z.; Zhu, D. High Voltage Insulation Technology; China Electric Power Press: Beijing, China, 2007; pp. 178–182. [Google Scholar]
- Sun, X.; Qiao, Y.; Li, Y.; Guo, X.; Cao, C. Effects of Mechanical Stress on the Life and Insulation Performance of DC-Link Capacitors. Energies 2024, 17, 699. [Google Scholar] [CrossRef]
- JB/T 6168-2023; Pulse Capacitors and DC Capacitors. China Machine Press: Beijing, China, 2023.
- IEC 61071:2017; Capacitors for Power Electronics. IEC: London, UK, 2017.
- Li, H.; Chen, Y.; Lin, F.; Lv, F.; Li, Z.; Zhang, M. Study on voltage maintaining performance of metalized film capacitors under high electric fields. In Proceedings of the 2011 Annual Report Conference on Electrical Insulation and Dielectric Phenomena, Cancun, Mexico, 16–19 October 2011; pp. 699–702. [Google Scholar]
- Kang, S.; Kim, J. Investigation on the Insulation Resistance Characteristics of Low Voltage Cable. Energies 2020, 13, 3611. [Google Scholar] [CrossRef]
- Zhao, Y.S.; Xiao, M.; Du, B.X. High-temperature Breakdown Performance Improvement of Polypropylene Films Based on Furfuryl Sulfide Graft Modification. In Proceedings of the 2022 IEEE 4th International Conference on Dielectrics (ICD), Palermo, Italy, 3–7 July 2022; Volume 3, pp. 717–720. [Google Scholar]
- Du, B.; Zhang, J.; Xiao, M.; Liu, H.; Ran, Z. Dielectric Breakdown Performance of Deashed Polypropylene for HVDC Film Capacitors. IEEE T Dielect. Electr. Insul. 2022, 29, 2209–2217. [Google Scholar] [CrossRef]
- Gallay, R. Metallized film capacitor lifetime evaluation and failure mode analysis. In Proceedings of the CAS-CERN Accelerator School: Power Converters, Baden, Switzerland, 7–14 May 2014; Bailey, R., Ed.; CERN-2015-003; CERN: Geneva, Switzerland, 2014. [Google Scholar]
- Hu, Y.; Ye, X.; Zheng, B.; Zhao, Z.; Zhai, G. Degradation mechanisms-based reliability modeling for metallized film capacitors under temperature and voltage stresses. Microelectron. Reliab. 2022, 138, 114609. [Google Scholar] [CrossRef]
- Tai, Y.; Chen, P.; Jian, Y.; Fang, Q.; Xu, D.; Cheng, J. Failure mechanism and life estimate of metallized film capacitor under high temperature and humidity. Microelectron. Reliab. 2022, 137, 114755. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sun, X.; Qiao, Y.; Li, Y.; Cao, C.; Feng, S. Insulation Resistance Characteristics of Dry DC Link Capacitors in the Presence of High Temperatures and Operating Voltages. Energies 2024, 17, 1147. https://doi.org/10.3390/en17051147
Sun X, Qiao Y, Li Y, Cao C, Feng S. Insulation Resistance Characteristics of Dry DC Link Capacitors in the Presence of High Temperatures and Operating Voltages. Energies. 2024; 17(5):1147. https://doi.org/10.3390/en17051147
Chicago/Turabian StyleSun, Xiaowu, Ying Qiao, Yinda Li, Chongfeng Cao, and Shenrong Feng. 2024. "Insulation Resistance Characteristics of Dry DC Link Capacitors in the Presence of High Temperatures and Operating Voltages" Energies 17, no. 5: 1147. https://doi.org/10.3390/en17051147
APA StyleSun, X., Qiao, Y., Li, Y., Cao, C., & Feng, S. (2024). Insulation Resistance Characteristics of Dry DC Link Capacitors in the Presence of High Temperatures and Operating Voltages. Energies, 17(5), 1147. https://doi.org/10.3390/en17051147





