Air Equalizing Mechanism in Cooling Performance Improvement of Vertical Delta-Type Radiators
Abstract
1. Introduction
2. Methods
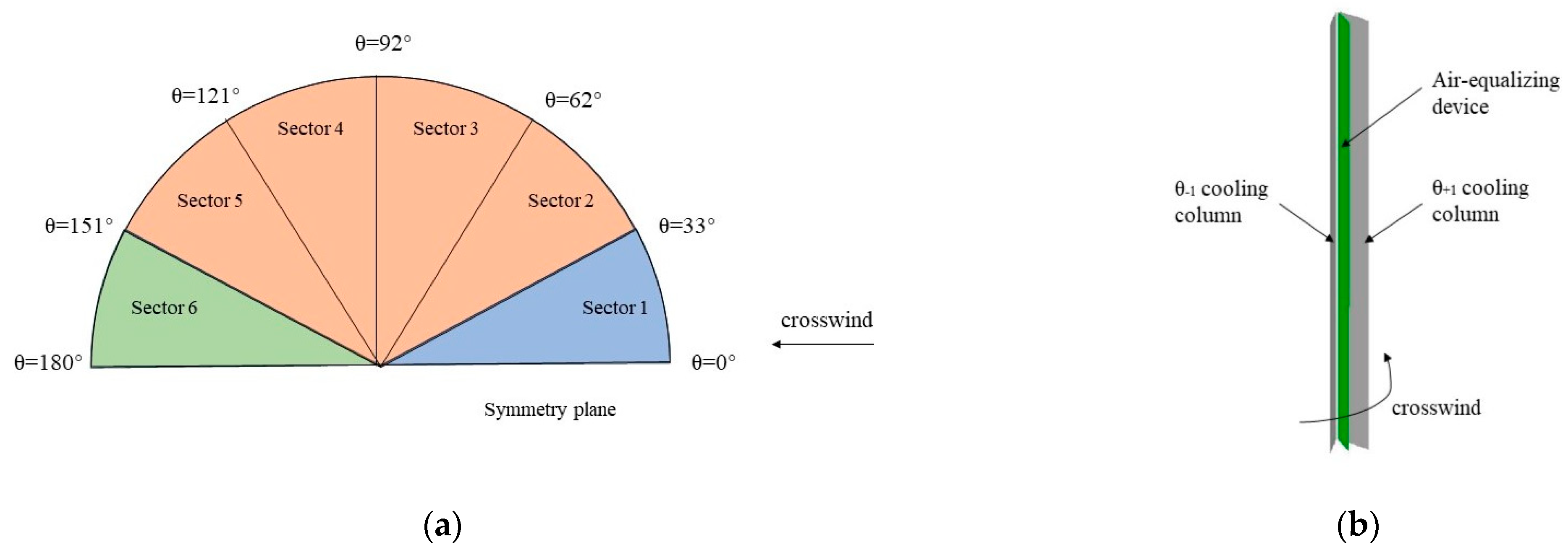
2.1. Geometric Model
2.2. Mathematical Equations
2.3. The CFD and Validation
2.3.1. Solution Method
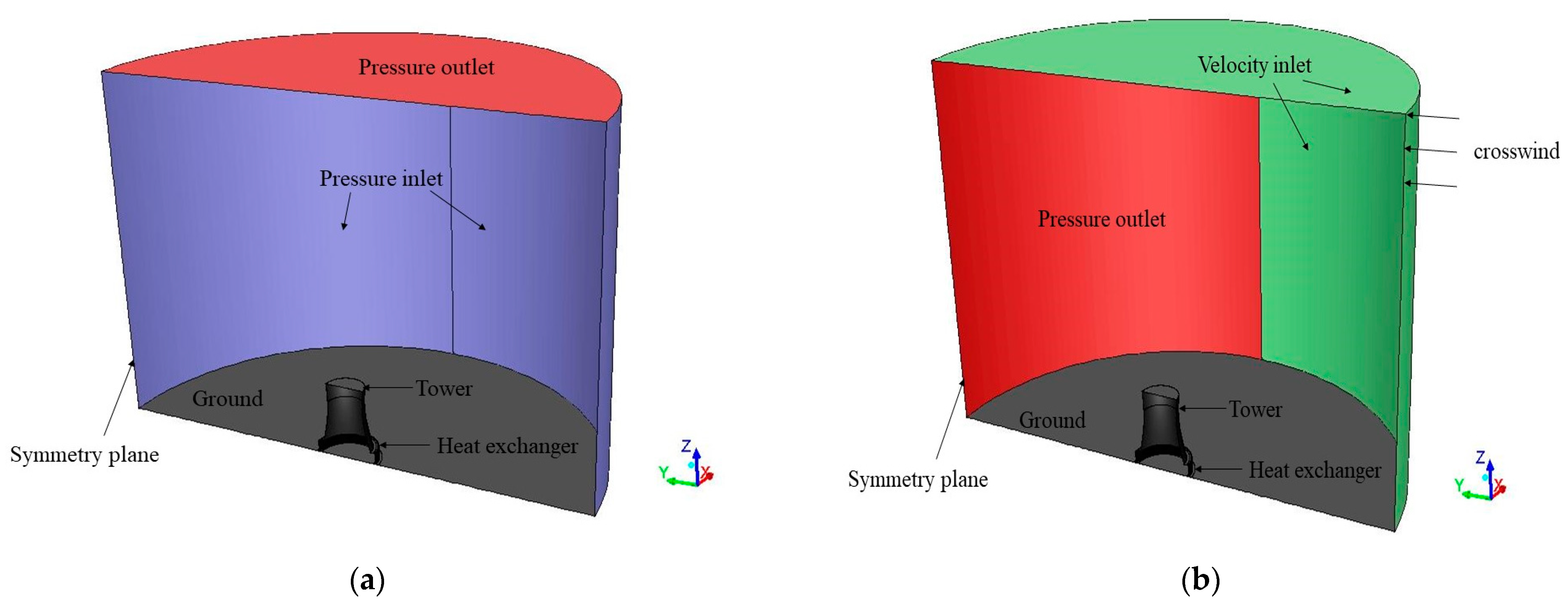
2.3.2. Boundary Conditions
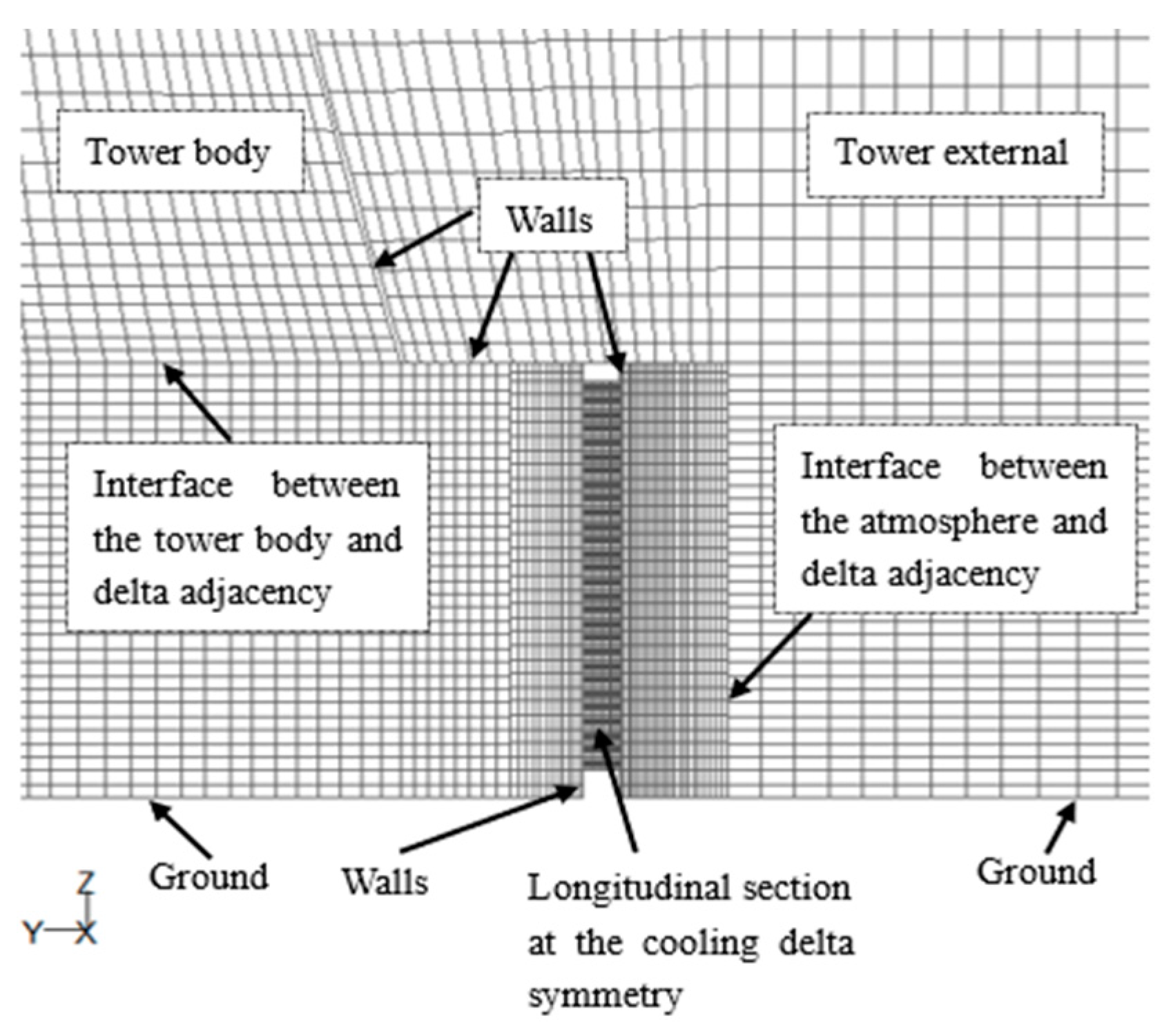
2.3.3. Computing Mesh and Grid Study
2.3.4. Model Validation
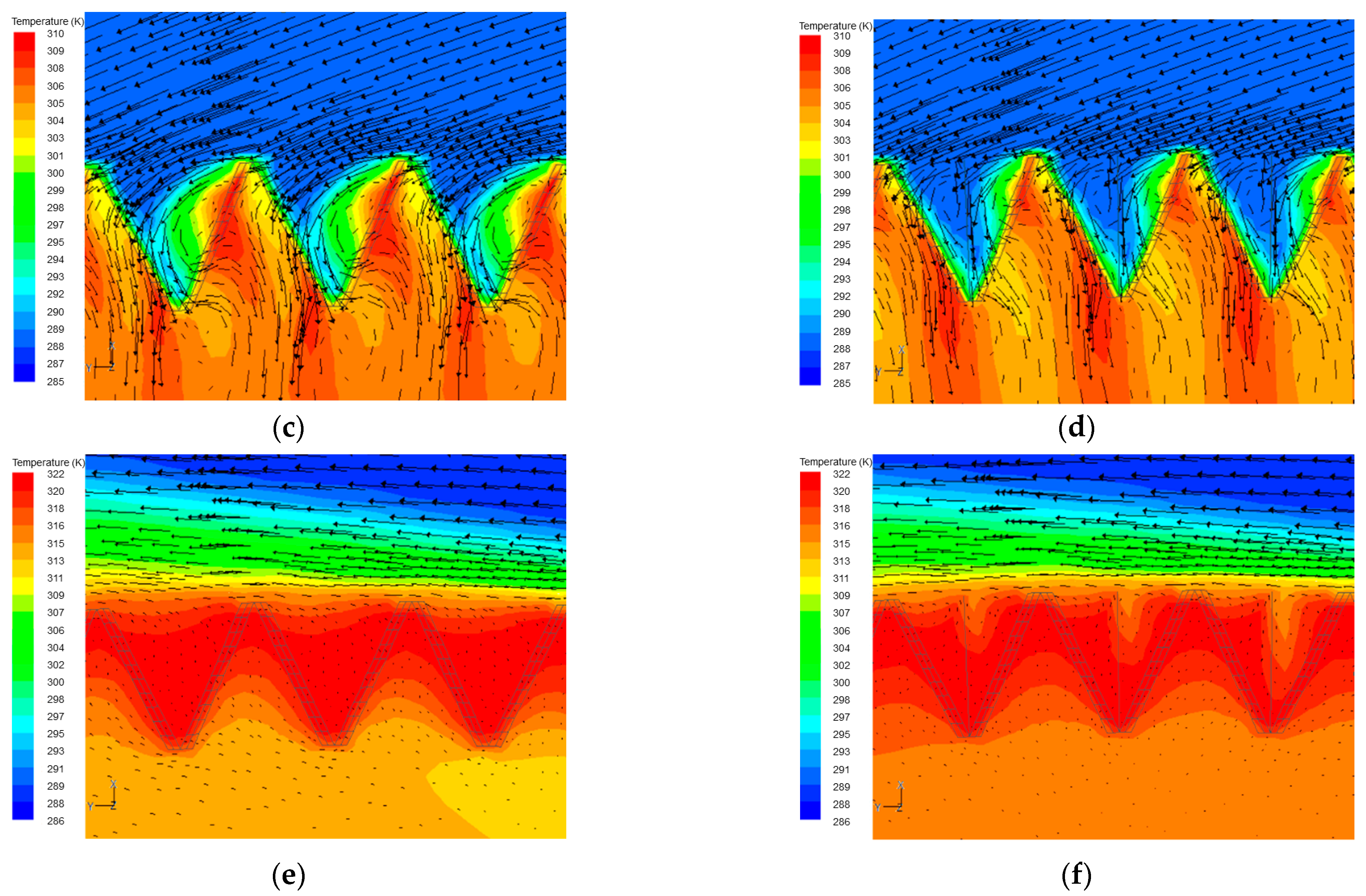
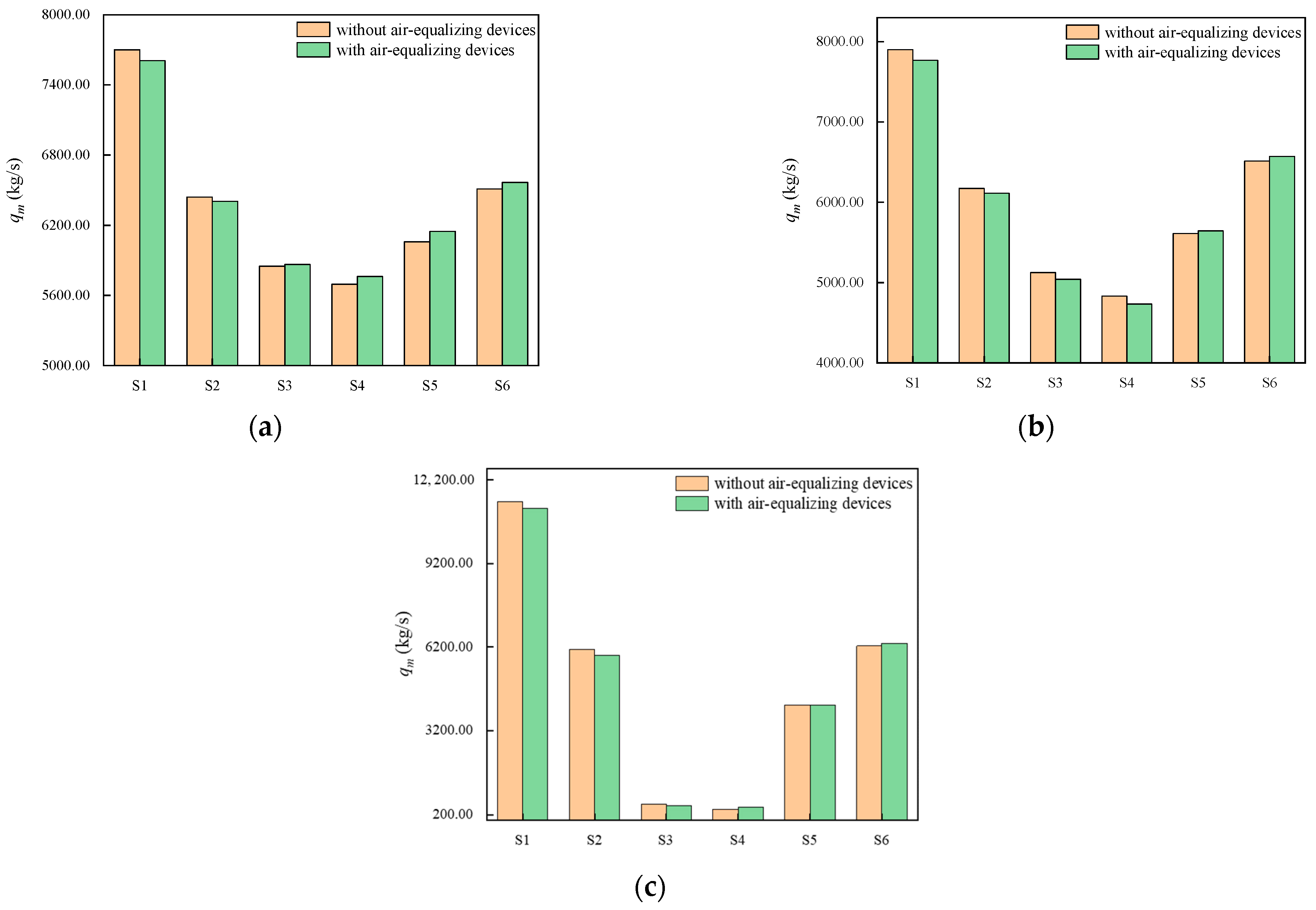
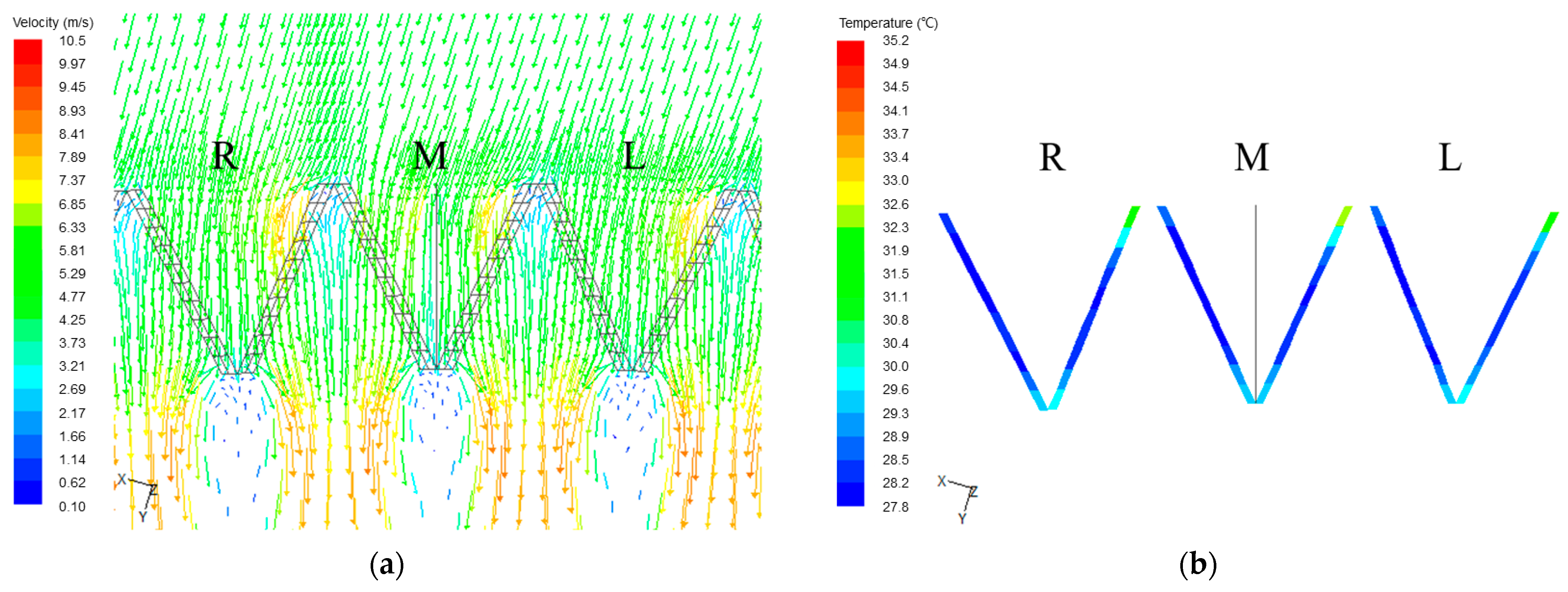
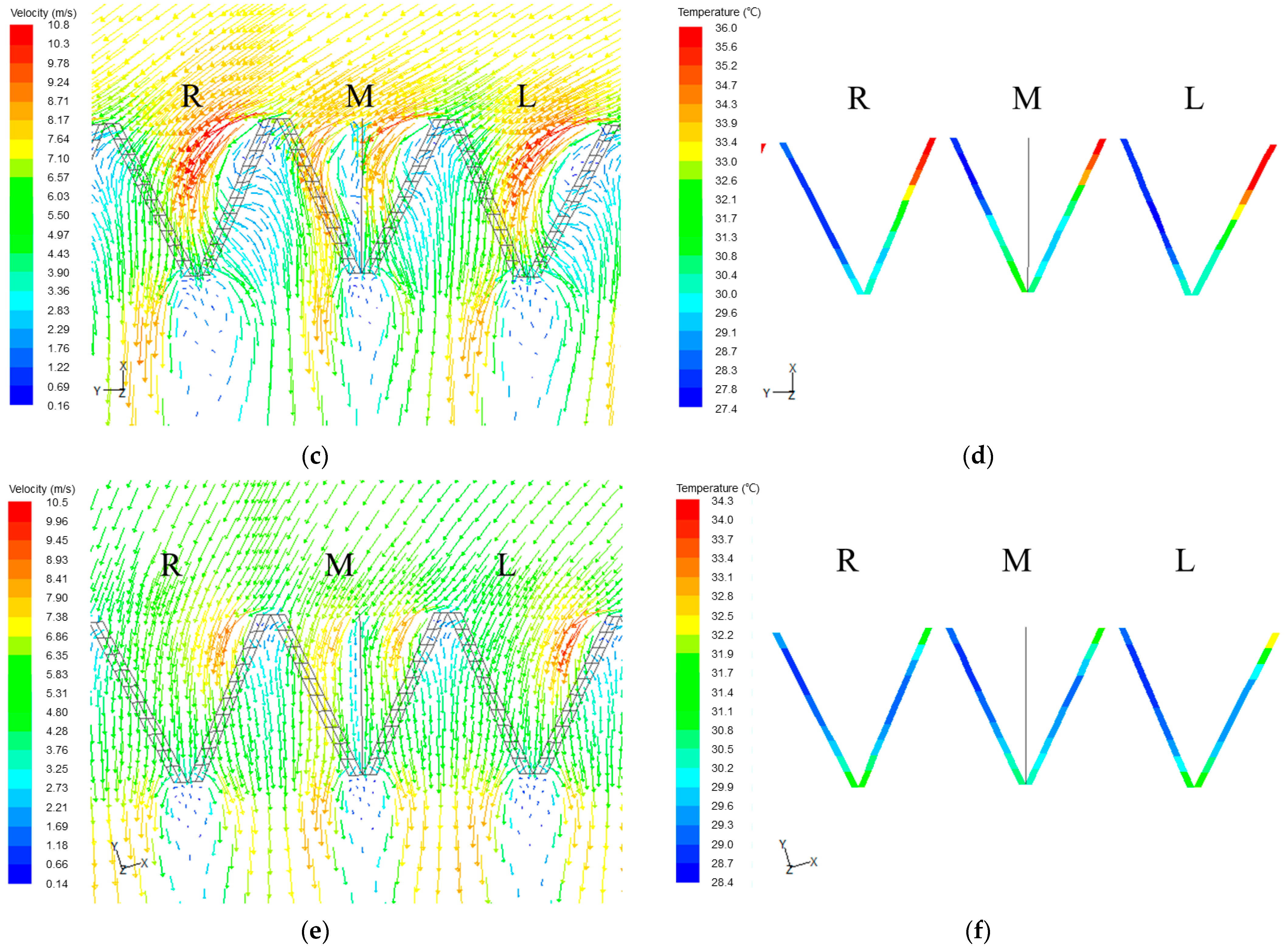
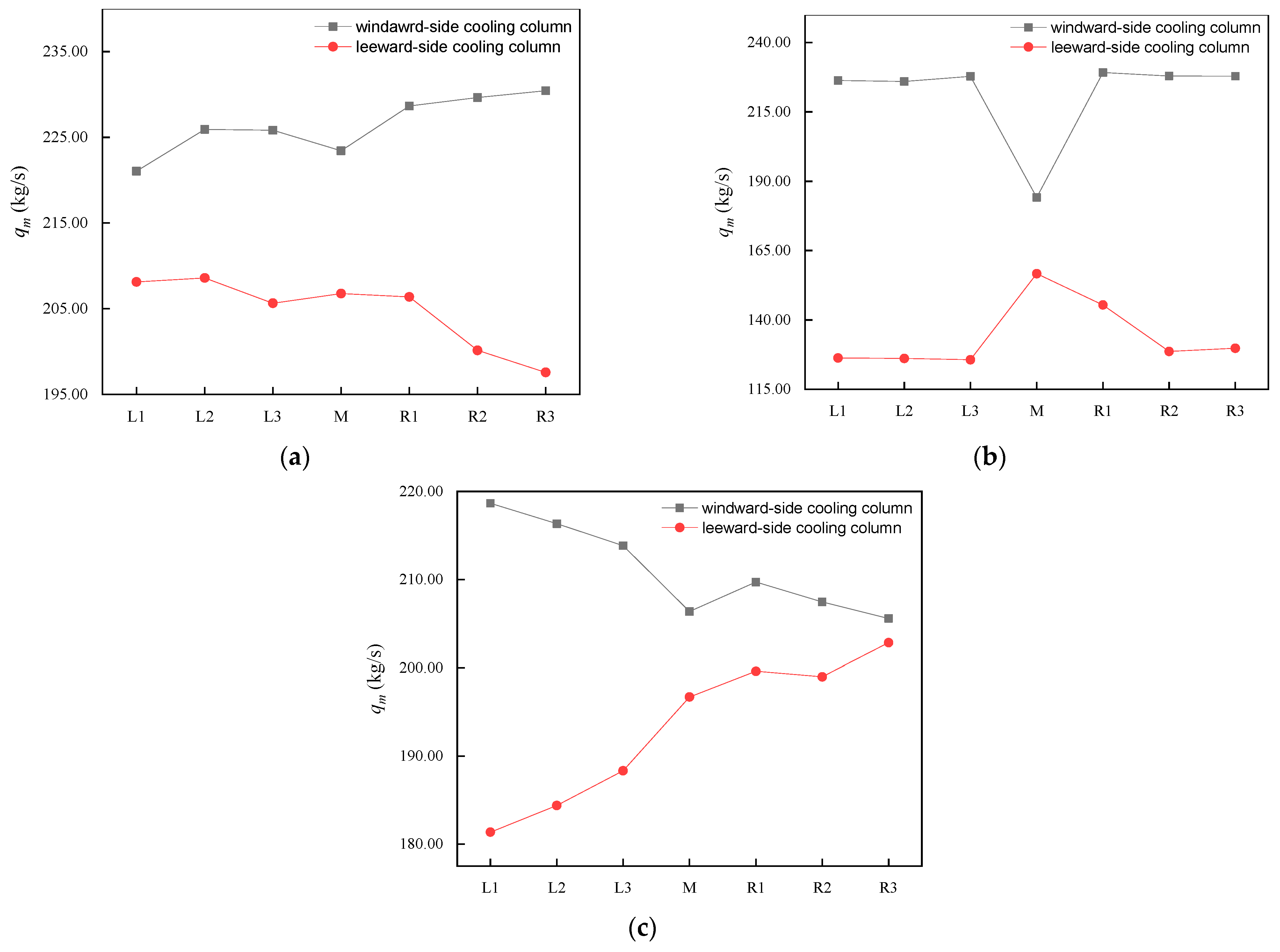
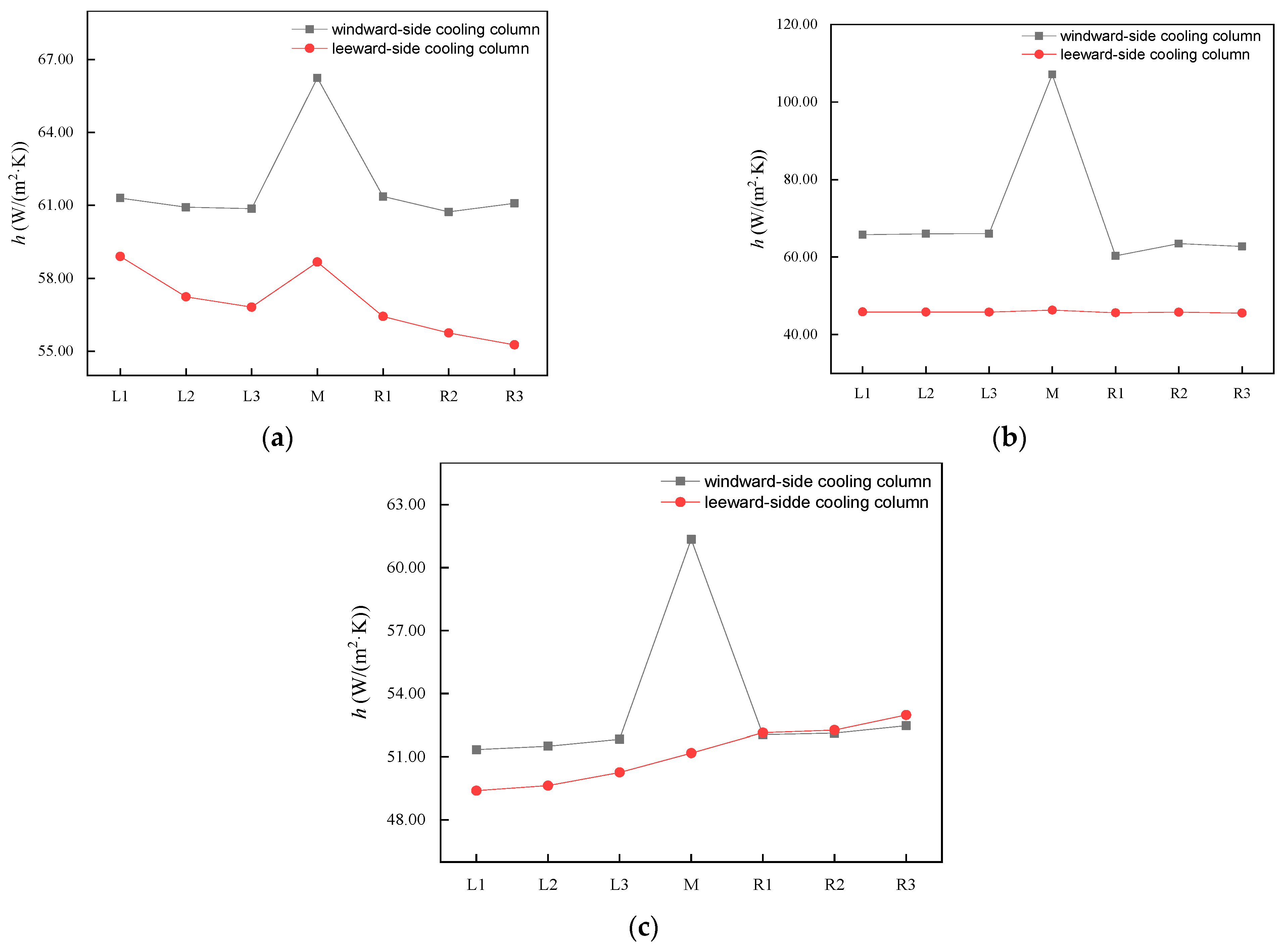
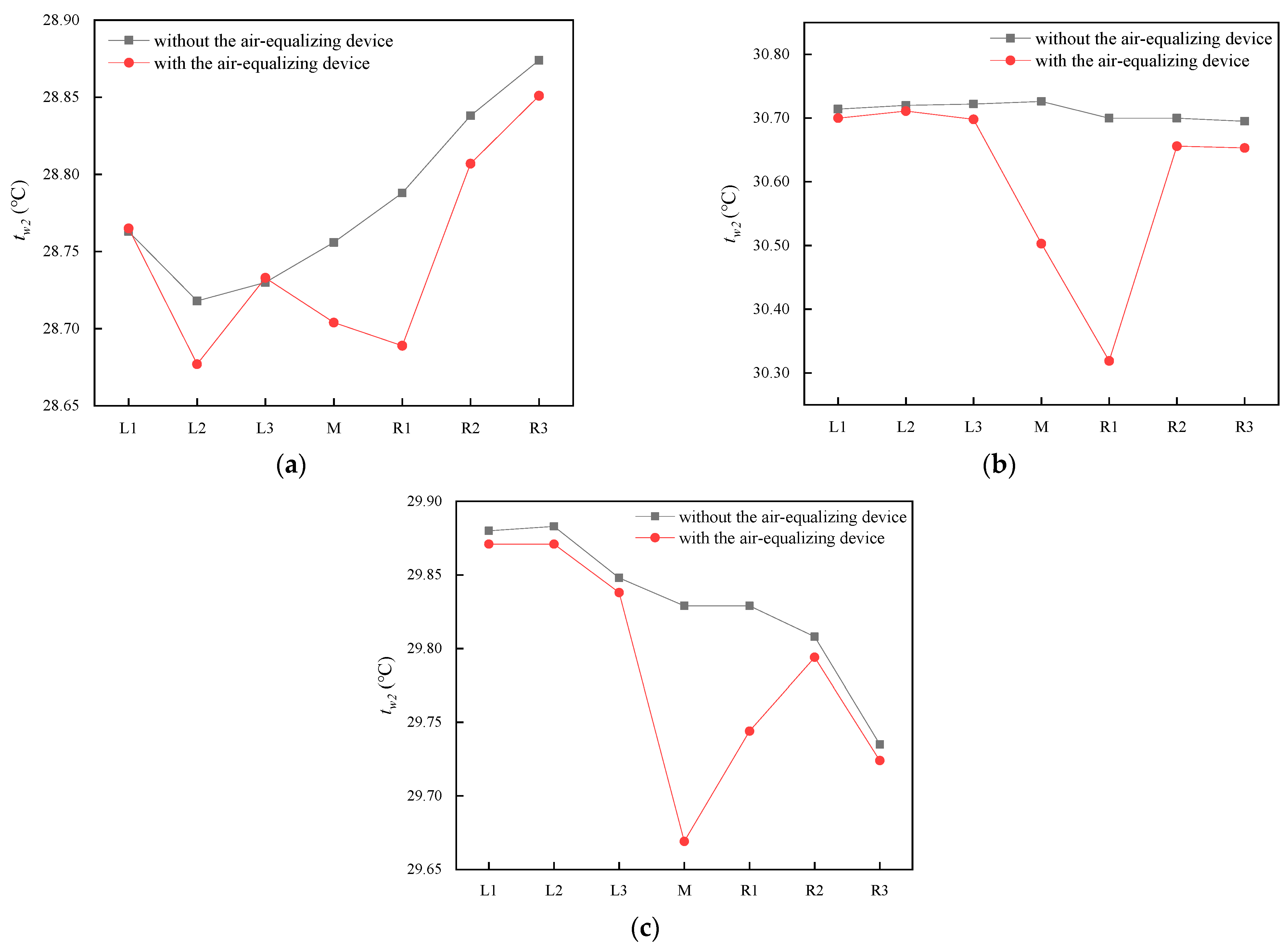
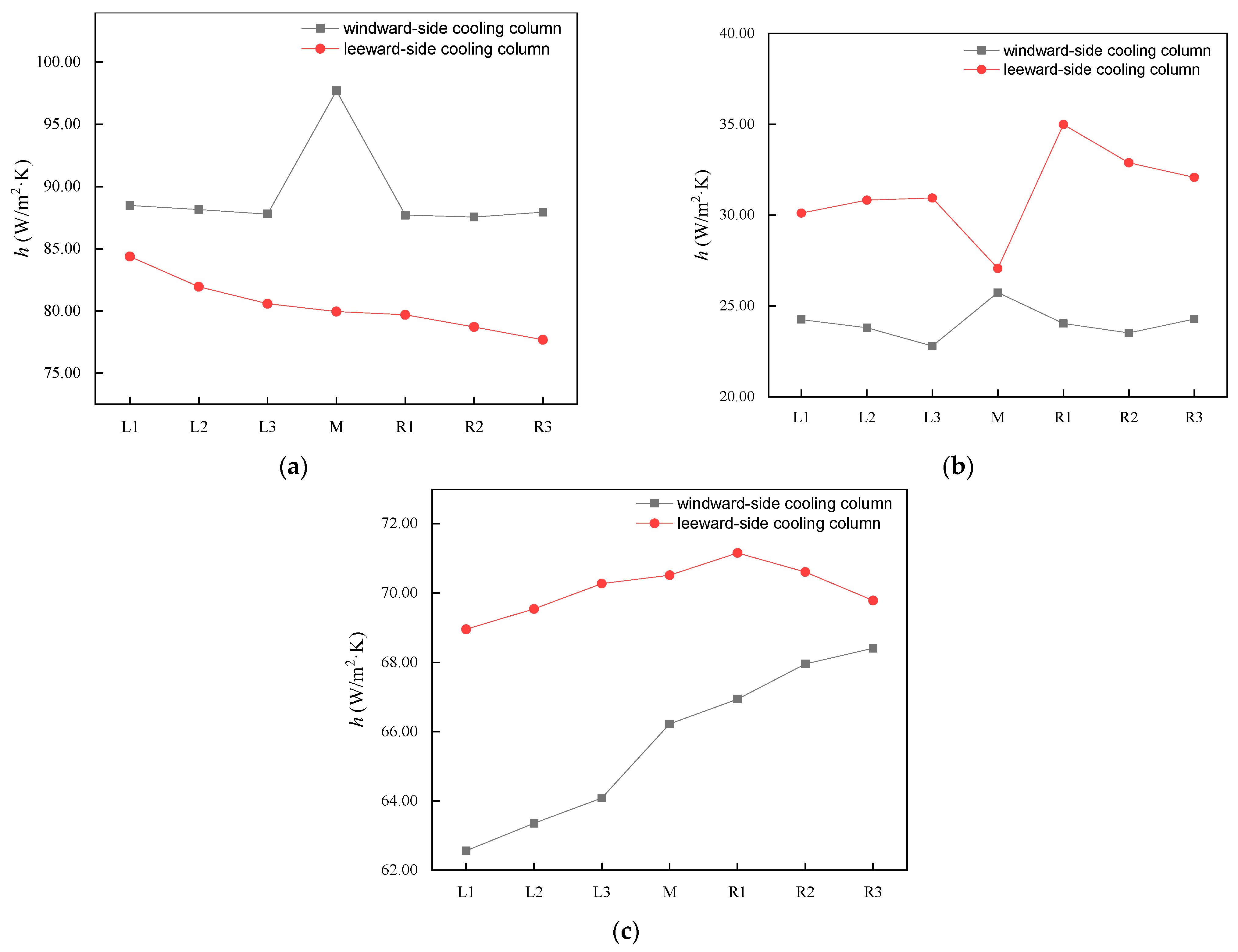
3. Results and Discussion
3.1. Impact on the NDDCT
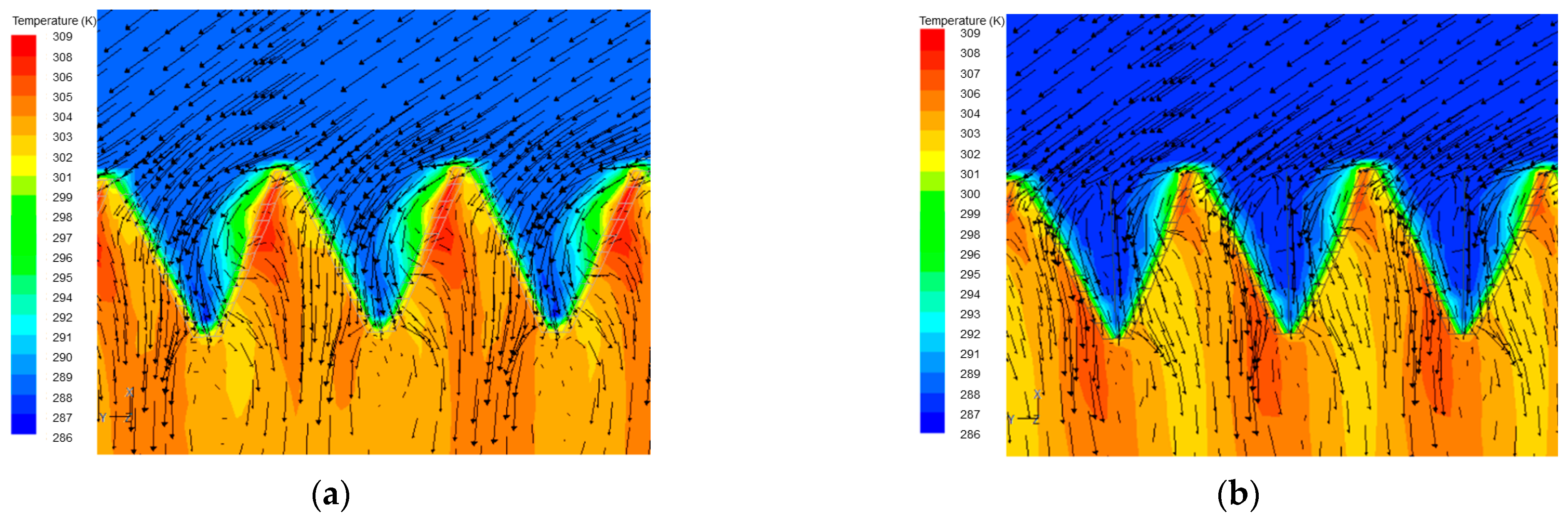
3.2. Impact on the Cooling Deltas at 2.5 m/s Wind Velocity
3.3. Impact on the Cooling Deltas at 12 m/s Wind Velocity
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Zhao, Y.B.; Long, G.Q.; Sun, F.Z.; Li, Y.; Zhang, C.J. Numerical study on the cooling performance of dry cooling tower with vertical two-pass column radiators under crosswind. Appl. Therm. Eng. 2015, 75, 1106–1117. [Google Scholar] [CrossRef]
- Wei, Q.D.; Zhang, B.Y.; Liu, K.Q.; Du, X.D.; Meng, X.Z. A study of the unfavorable effects of wind on the cooling efficiency of dry cooling towers. J. Wind Eng. Ind. Aerodyn. 1995, 54, 633–643. [Google Scholar] [CrossRef]
- Ma, H.; Si, F.Q.; Li, L.; Yan, W.S.; Zhu, K.P. Effects of ambient temperature and crosswind on thermo-flow performance of the tower under energy balance of the indirect dry cooling system. Appl. Therm. Eng. 2015, 78, 90–100. [Google Scholar] [CrossRef]
- Wang, W.L.; Lv, J.F.; Zhang, H.; Liu, Q.; Yue, G.X.; Ni, W.D. A quantitative approach identifies the critical flow characteristics in a natural draft dry cooling tower. Appl. Therm. Eng. 2018, 131, 522–530. [Google Scholar] [CrossRef]
- Wang, W.L.; Wang, Y.Z.; Zhang, H.; Lin, G.M.; Lu, J.F.; Yue, G.X.; Ni, W.D. Fresh breeze cuts down one-third ventilation rate of a natural draft dry cooling tower: A hot state modelling. Appl. Therm. Eng. 2018, 131, 1–7. [Google Scholar] [CrossRef]
- Wang, W.L.; Zhang, H.; Liu, P.; Li, Z.; Lv, J.F.; Ni, W.D. The cooling performance of a natural draft dry cooling tower under crosswind and an enclosure approach to cooling efficiency enhancement. Appl. Energy 2017, 186, 336–346. [Google Scholar] [CrossRef]
- Lu, Y.; Guan, Z.; Gurgenci, H.; Zou, Z. Windbreak walls reverse the negative effect of crosswind in short natural draft dry cooling towers into a performance enhancement. Int. J. Heat Mass Transf. 2013, 63, 162–170. [Google Scholar] [CrossRef]
- Ghasemi Zavaragh, H.; Ceviz, M.A.; Shervani Tabar, M.T. Analysis of windbreaker combinations on steam power plant natural draft dry cooling towers. Appl. Therm. Eng. 2016, 99, 550–559. [Google Scholar] [CrossRef]
- Gu, H.F.; Wang, H.J.; Gu, Y.Q.; Yao, J.N. A numerical study on the mechanism and optimization of wind-break structures for indirect air-cooling towers. Energy Convers. Manag. 2016, 108, 43–49. [Google Scholar] [CrossRef]
- Lu, Y.S.; Gurgenci, H.; Guan, Z.Q.; He, S.Y. The influence of windbreak wall orientation on the cooling performance of small natural draft dry cooling towers. Int. J. Heat Mass Transf. 2014, 79, 1059–1069. [Google Scholar] [CrossRef]
- Al-Waked, R.; Behnia, M. The effect of windbreak walls on the thermal performance of natural draft dry cooling towers. Heat Transf. Eng. 2005, 26, 50–62. [Google Scholar] [CrossRef]
- Chen, L.; Yang, L.J.; Du, X.Z.; Yang, Y.P. Performance improvement of natural draft dry cooling system by interior and exterior windbreaker configurations. Int. J. Heat Mass Transf. 2016, 96, 42–63. [Google Scholar] [CrossRef]
- Shirazi, M.; Jahangiri, A. 3D numerical study using three novel windbreak walls in natural draft dry cooling towers for performance enhancement under various crosswind conditions. Therm. Sci. Eng. Prog. 2021, 25, 100971. [Google Scholar] [CrossRef]
- Seifi, A.R.; Akbari, O.A.; Alrashed, A.A.A.A.; Afshary, F.; Shabani, G.A.S.; Seifi, R.; Goodarzi, M.; Pourfattah, F. Effects of external wind breakers of Heller dry cooling system in power plants. Appl. Therm. Eng. 2018, 129, 1124–1134. [Google Scholar] [CrossRef]
- Wu, T.; Ge, Z.H.; Yang, L.J.; Du, X.Z. Flow deflectors to release the negative defect of natural wind on large scale dry cooling tower. Int. J. Heat Mass Transf. 2019, 128, 248–269. [Google Scholar] [CrossRef]
- Gao, X.F.; Zhang, C.W.; Wei, J.J.; Yu, B. Performance prediction of an improved air-cooled steam condenser with deflector under strong wind. Appl. Therm. Eng. 2010, 30, 2663–2669. [Google Scholar] [CrossRef]
- Zhao, Y.B.; Long, G.Q.; Sun, F.Z.; Li, Y.; Zhang, C.J.; Liu, J.B. Effect mechanism of air deflectors on the cooling performance of dry cooling tower with vertical delta radiators under crosswind. Energy Convers. Manag. 2015, 93, 321–331. [Google Scholar] [CrossRef]
- Ardekani, M.A.; Ranjbar, M.A.; Farhani, F. Use of guide vanes for improvement of flow pattern and enhancement of thermal performance of dry cooling towers. Mech. Ind. 2016, 18, 111. [Google Scholar] [CrossRef]
- Du, X.P.; Han, D.T.; Zhu, Q.M. Heat Transfer Enhancement of the Air-Cooling Tower with Rotating Wind Deflectors under Crosswind Conditions. Appl. Sci. 2018, 8, 544. [Google Scholar] [CrossRef]
- Goodarzi, M.; Keimanesh, R. Heat rejection enhancement in natural draft cooling tower using radiator-type windbreakers. Energy Convers. Manag. 2013, 71, 120–125. [Google Scholar] [CrossRef]
- Ma, H.; Cai, L.; Si, F.Q.; Wang, J.S. Exploratory research on annular-arranged moist media to improve cooling capacity of natural draft dry cooling tower and thermo-flow characteristics of its radiators. Int. J. Heat Mass Transf. 2021, 172, 121123. [Google Scholar] [CrossRef]
- Ma, H.; Si, F.Q.; Zhu, K.P.; Wang, J.S. The adoption of windbreak wall partially rotating to improve thermo-flow performance of natural draft dry cooling tower under crosswind. Int. J. Therm. Sci. 2018, 134, 66–88. [Google Scholar] [CrossRef]
- Abbasi, M.; Alighanbari, F.; Sarvestani, A.B.; Golneshan, A.A. The effect of crosswind and installation of wind-break deflector on the performance of natural draft dry cooling tower (NDDCT). J. Wind Eng. Ind. Aerodyn. 2022, 229, 105146. [Google Scholar] [CrossRef]
- Goodarzi, M.; Keimanesh, R. Numerical analysis on overall performance of Savonius turbines adjacent to a natural draft cooling tower. Energy Convers. Manag. 2015, 99, 41–49. [Google Scholar] [CrossRef]
- Goodarzi, M. A proposed stack configuration for dry cooling tower to improve cooling efficiency under crosswind. J. Wind Eng. Ind. Aerodyn. 2010, 98, 858–863. [Google Scholar] [CrossRef]
- Alizadeh Kheneslu, R.; Jahangiri, A.; Ameri, M. Interaction effects of natural draft dry cooling tower (NDDCT) performance and 4E (energy, exergy, economic and environmental) analysis of steam power plant under different climatic conditions. Sustain. Energy Technol. Assess. 2020, 37, 100599. [Google Scholar] [CrossRef]
- Kong, Y.Q.; Wang, W.J.; Zuo, Z.T.; Yang, L.J.; Du, X.Z.; Xu, C.; Yang, Y.P. Influencing mechanisms of a crosswind on the thermo-hydraulic characteristics of a large-scale air-cooled heat exchanger. Energies 2019, 12, 1128. [Google Scholar] [CrossRef]
- Yang, L.J.; Wu, X.P.; Du, X.Z.; Yang, Y.P. Dimensional characteristics of wind effects on the performance of indirect dry cooling system with vertically arranged heat exchanger bundles. Int. J. Heat Mass Transf. 2013, 67, 853–866. [Google Scholar] [CrossRef]
- Ma, H.; Si, F.Q.; Kong, Y.; Zhu, K.P.; Yan, W.S. Wind-break walls with optimized setting angles for natural draft dry cooling tower with vertical radiators. Appl. Therm. Eng. 2017, 112, 326–339. [Google Scholar] [CrossRef]
- Li, W.D.; Wang, H.T.; Wang, J.; Duan, C.P.; Zhao, Y.B. Effect mechanism of exit-water temperature distribution characteristics on the anti-freezing of natural draft dry cooling tower. Appl. Therm. Eng. 2019, 161, 114078. [Google Scholar] [CrossRef]
- Zhang, K.; Li, M.J.; Liu, H.; Xiong, J.G.; He, Y.L. A general and rapid method to evaluate the effect of flow maldistribution on the performance of heat exchangers. Int. J. Therm. Sci. 2021, 170, 107152. [Google Scholar] [CrossRef]
- Sharifullin, V.N.; Badriev, A.I. Aerodynamic Characteristics of the Cooling Tower under the Nonuniform Distribution of the Water and Air Flows. Therm. Eng. 2019, 66, 569–574. [Google Scholar] [CrossRef]
- Goodarzi, M.; Moradi Maryamnegari, S. A new natural draft dry cooling tower with improved thermal performance during windy condition. Appl. Therm. Eng. 2018, 139, 341–351. [Google Scholar] [CrossRef]
- Ma, H.; Si, F.Q.; Wang, J.S. Study on the effects of apex angle of the delta-type radiator on thermo-flow performance of natural draft dry cooling tower. Int. J. Heat Mass Transf. 2020, 148, 119002. [Google Scholar] [CrossRef]
- Chen, L.; Yang, L.; Du, X.; Yang, Y. Flue gas diffusion for integrated dry-cooling tower and stack system in power plants. Int. J. Therm. Sci. 2017, 114, 257–270. [Google Scholar] [CrossRef]
- Li, X.X.; Gurgenci, H.; Guan, Z.Q.; Wang, X.R.; Xia, L. A review of the crosswind effect on the natural draft cooling towers. Appl. Therm. Eng. 2019, 150, 250–270. [Google Scholar] [CrossRef]
- Liao, H.T.; Yang, L.J.; Du, X.Z.; Yang, Y.P. Triangularly arranged heat exchanger bundles to restrain wind effects on natural draft dry cooling system. Appl. Therm. Eng. 2016, 99, 313–324. [Google Scholar] [CrossRef]
- Wang, W.L.; Zhang, H.; Li, Z.; Lv, J.F.; Ni, W.D.; Li, Y.S. Adoption of enclosure and windbreaks to prevent the degradation of the cooling performance for a natural draft dry cooling tower under crosswind conditions. Energy 2016, 116, 1360–1369. [Google Scholar] [CrossRef]
- Wu, T.; Ge, Z.; Yang, L.; Du, X. Modeling the performance of the indirect dry cooling system in a thermal power generating unit under variable ambient conditions. Energy 2019, 169, 625–636. [Google Scholar] [CrossRef]
- Yang, L.J.; Chen, L.; Du, X.Z.; Yang, Y.P. Effects of ambient winds on the thermo-flow performances of indirect dry cooling system in a power plant. Int. J. Therm. Sci. 2013, 64, 178–187. [Google Scholar] [CrossRef]
- Zhao, Y.B.; Sun, F.Z.; Yang, Y.; Li, Y. Air-Side Flow Equalization Device for Cooling Delta of Natural Draft Dry Cooling Tower. CN104596346B, 11 May 2016. [Google Scholar]
- Al-Waked, R.; Behnia, M. The performance of natural draft dry cooling towers under crosswind: CFD study. Int. J. Energy Res. 2004, 28, 147–161. [Google Scholar] [CrossRef]
- Launder, B.E.; Spalding, D.B. The numerical computation of turbulent flow. Comput. Methods Appl. Mech. ANR Eng. 1974, 3, 269. [Google Scholar] [CrossRef]
- Yang, L.J.; Jia, S.N.; Bu, Y.D.; Du, X.Z.; Yang, Y.P. Numerical study on flow and heat transfer characteristics of finned tube bundles for air-cooled heat exchangers of indirect dry cooling systems in power plants. Proc. CESS 2012, 32, 50–57. [Google Scholar]
- Li, X.G.; Guo, T.; Huang, W.; Wang, L.B.; Zhao, Y.B. Improvement mechanism of wedged column on the cooling performance of vertical delta radiator. Int. J. Heat Mass Transf. 2022, 188, 122619. [Google Scholar] [CrossRef]
- Su, M.D.; Tang, G.F.; Fu, S. Numerical simulation of fluid flow and thermal performance of a dry-cooling tower under cross wind condition. J. Wind Eng. Ind. Aerodyn. 1999, 79, 289–306. [Google Scholar] [CrossRef]
- Zhao, Y.B.; Zhao, Q.; Li, F.; Liang, Q.H.; Li, X.; Chen, Y.S. Effect mechanism of cooling delta aerodynamic field equalizing on the cooling characteristics of dry cooling tower. Int. Commun. Heat Mass Transf. 2023, 148, 107070. [Google Scholar] [CrossRef]





| Grid Number | 3,253,938 | 3,755,643 | 4,910,584 |
|---|---|---|---|
| /°C | 28.770 | 28.543 | 28.498 |
| Temperature difference/°C | 0.270 | 0.043 | −0.002 |
| Relative error (%) | 1.985 | 0.316 | 0.015 |
| Design/Test Work Conditions | Symbol | C1 | C2 |
|---|---|---|---|
| Atmospheric pressure (kPa) | 85.13 | 84.35 | |
| Atmospheric dry-bulb temperature (°C) | 19.07 | 27.39 | |
| Atmospheric relative humidity (%) | 57 | 62 | |
| Ambient crosswind velocity (m/s) | 5.00 | 1.49 | |
| Circulating water volume flow rate (m3/h) | 94,000 | 117,217.72 | |
| Inlet circulating water temperature (°C) | 45.89 | 52.98 | |
| Design/Test outlet water temperature (°C) | 35.68 | 45.11 | |
| 3D numerical outlet water temperature (°C) | 35.32 | 45.03 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, T.; Zhao, Q.; Zhang, Y.; Wang, C.; Li, K.; Li, S.; Quan, L.; Zhao, Y. Air Equalizing Mechanism in Cooling Performance Improvement of Vertical Delta-Type Radiators. Energies 2024, 17, 1111. https://doi.org/10.3390/en17051111
Chen T, Zhao Q, Zhang Y, Wang C, Li K, Li S, Quan L, Zhao Y. Air Equalizing Mechanism in Cooling Performance Improvement of Vertical Delta-Type Radiators. Energies. 2024; 17(5):1111. https://doi.org/10.3390/en17051111
Chicago/Turabian StyleChen, Tiefeng, Qian Zhao, Yaoming Zhang, Chengjun Wang, Kaiming Li, Shixing Li, Longtao Quan, and Yuanbin Zhao. 2024. "Air Equalizing Mechanism in Cooling Performance Improvement of Vertical Delta-Type Radiators" Energies 17, no. 5: 1111. https://doi.org/10.3390/en17051111
APA StyleChen, T., Zhao, Q., Zhang, Y., Wang, C., Li, K., Li, S., Quan, L., & Zhao, Y. (2024). Air Equalizing Mechanism in Cooling Performance Improvement of Vertical Delta-Type Radiators. Energies, 17(5), 1111. https://doi.org/10.3390/en17051111






