A Review of Thermoelectric Generators in Automobile Waste Heat Recovery Systems for Improving Energy Utilization
Abstract
1. Introduction
2. Working Principle
3. TEG Efficiency
4. Figure of Merit
- (i)
- The thermal conductivity of the material can be reduced to maintain a high temperature gradient across the two ends.
- (ii)
- Increasing the electrical conductivity reduces the resistivity and Joule heating.
- (iii)
- Enhancing the Seebeck coefficient leads to a high potential difference.
- (a)
- Improvement of TEG materials.
- (b)
- Implementation of highly efficient heat exchangers.
- (c)
- Structural improvement of TE legs.
5. Thermoelectric Materials Used in Automobiles
5.1. BiTe-Based Materials
5.2. SnSe-Based Materials
5.3. CuSe-Based Materials
5.4. Multi-Component Oxides
5.5. Half-Heusler Alloys
5.6. Organic–Inorganic Composites
5.7. GeTe/PbTe-Based Materials
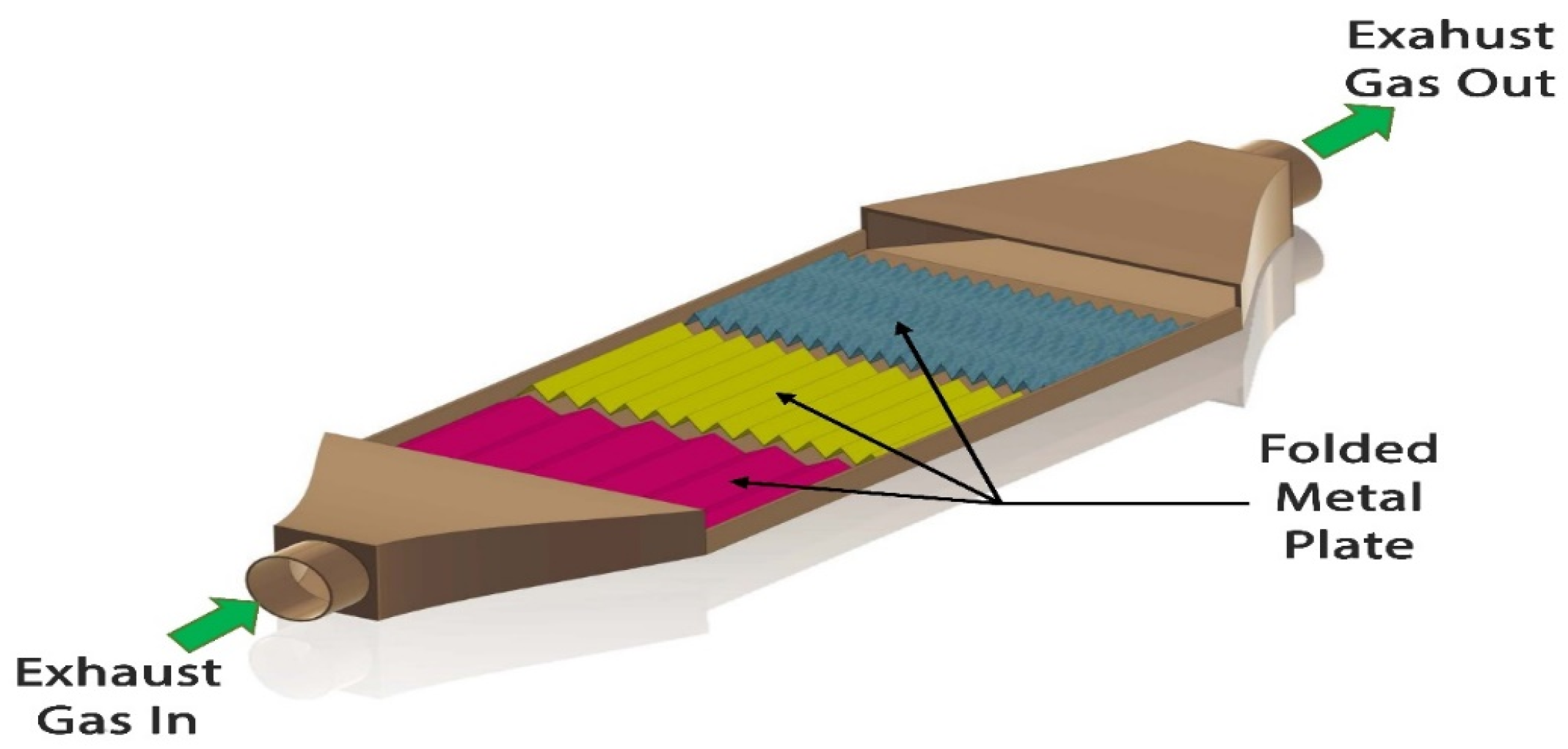
6. Heat Exchanger Design in Automobile Applications
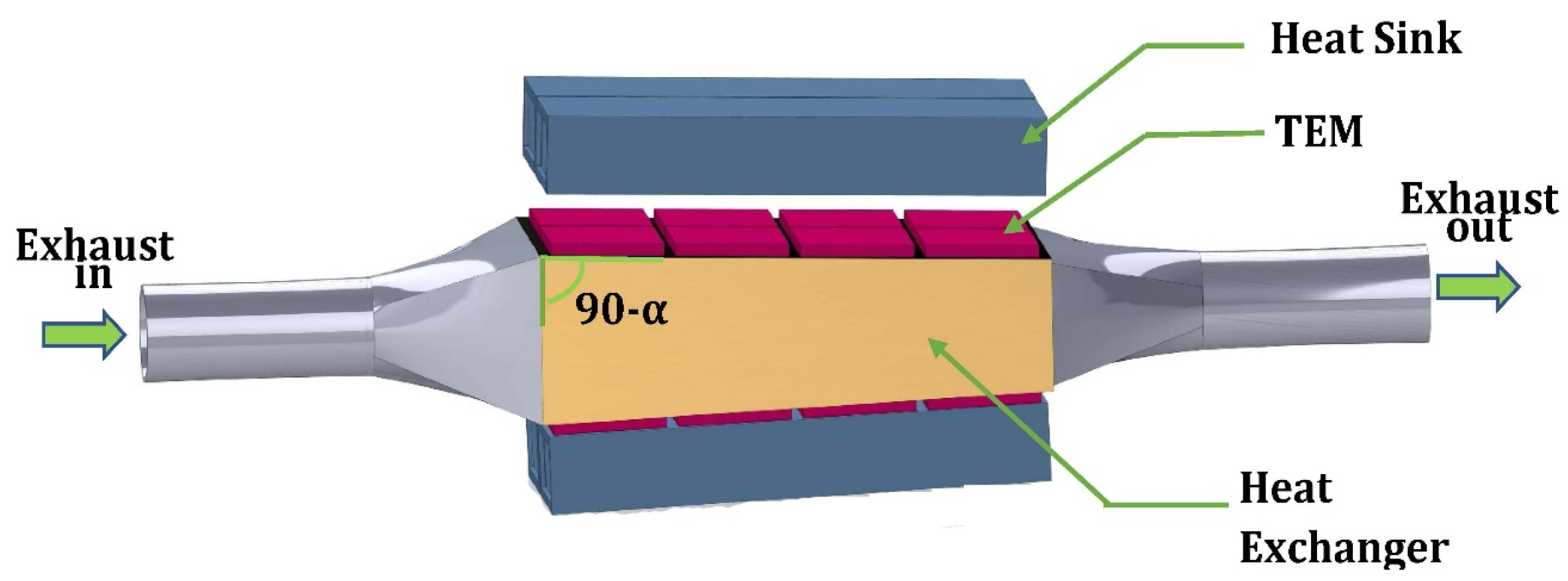

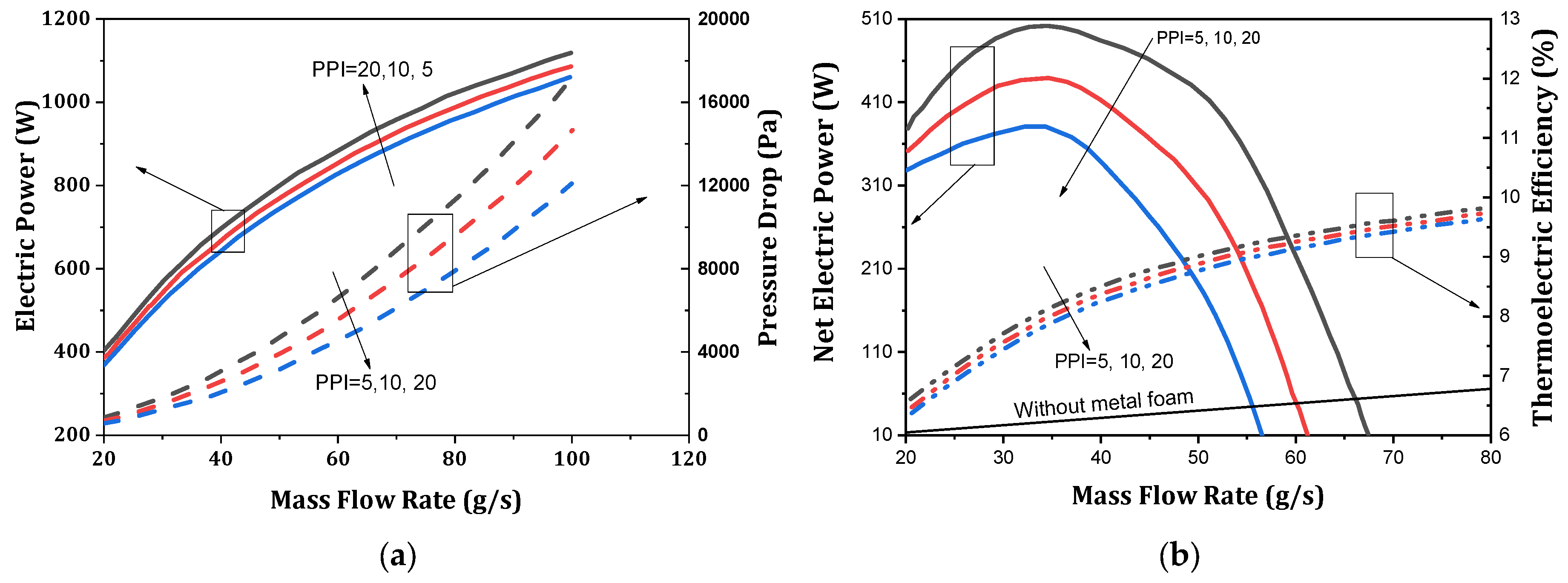
6.1. Metal Foam
6.2. Extended Surface
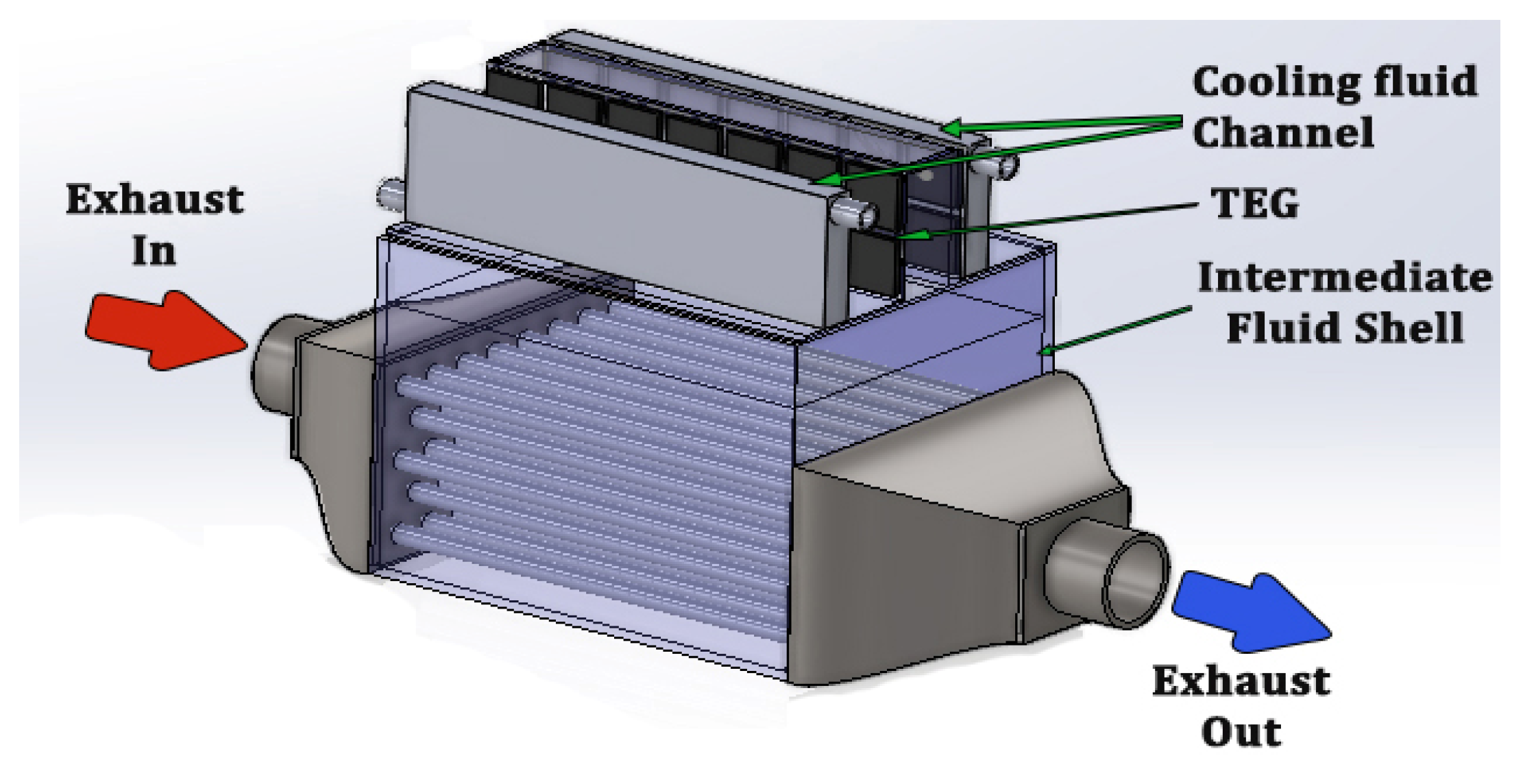
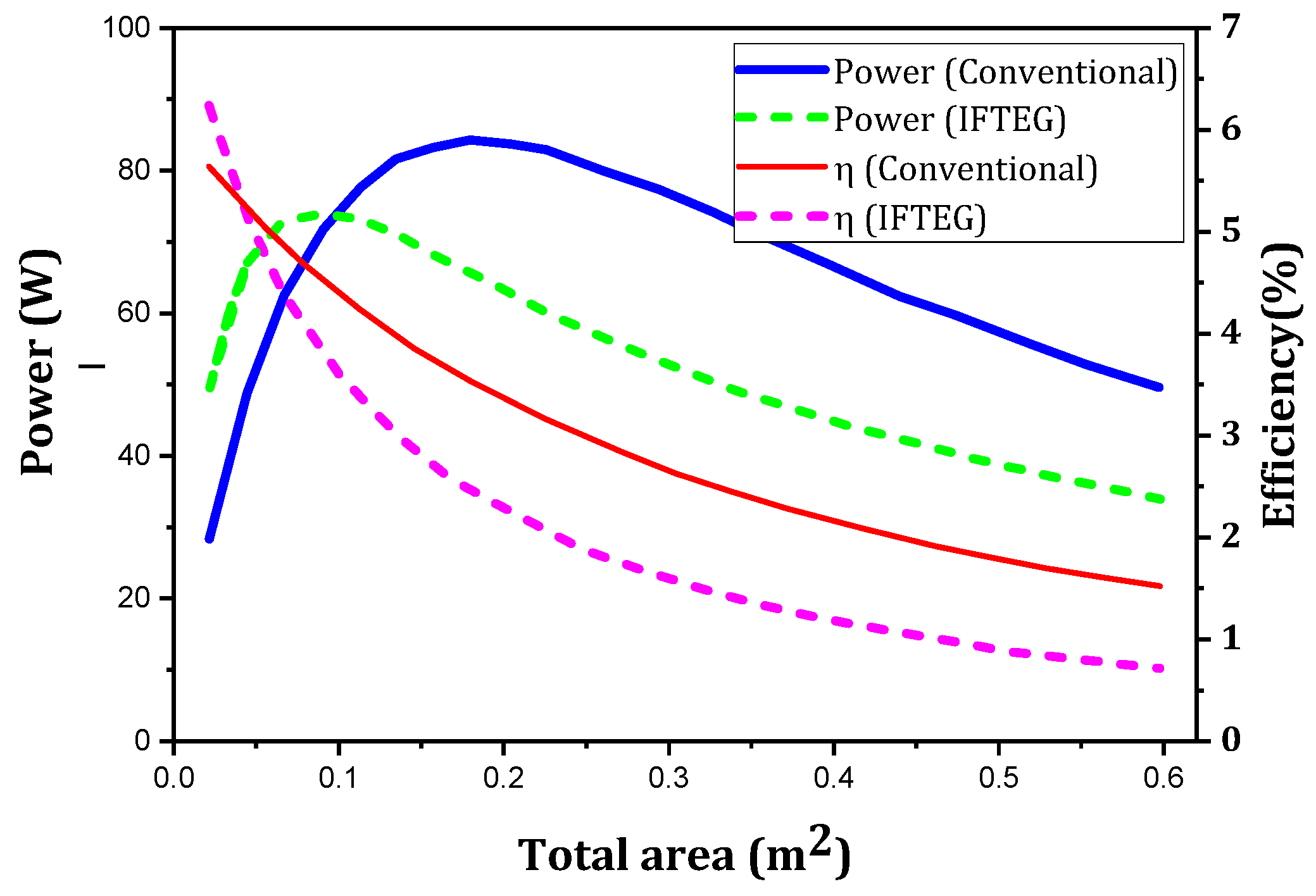
6.3. Intermediate Fluid
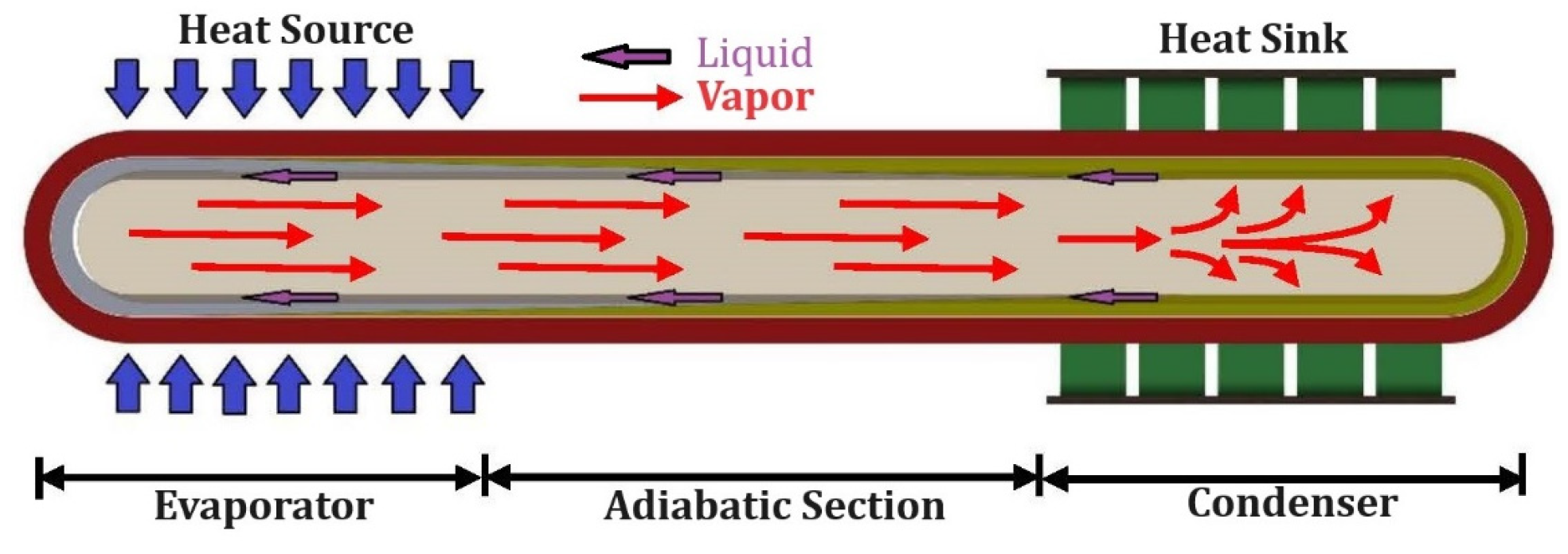
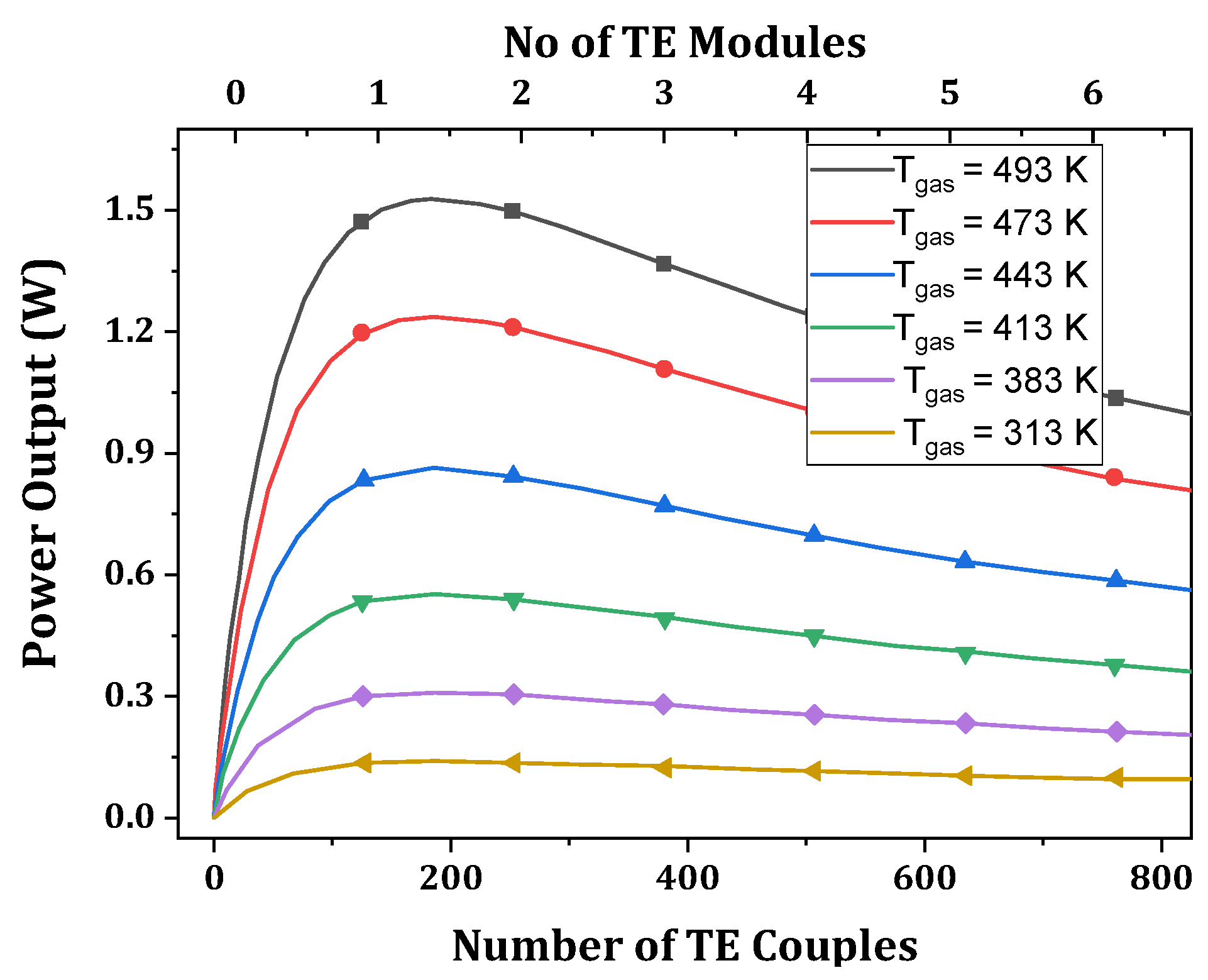
6.4. Heat Pipes
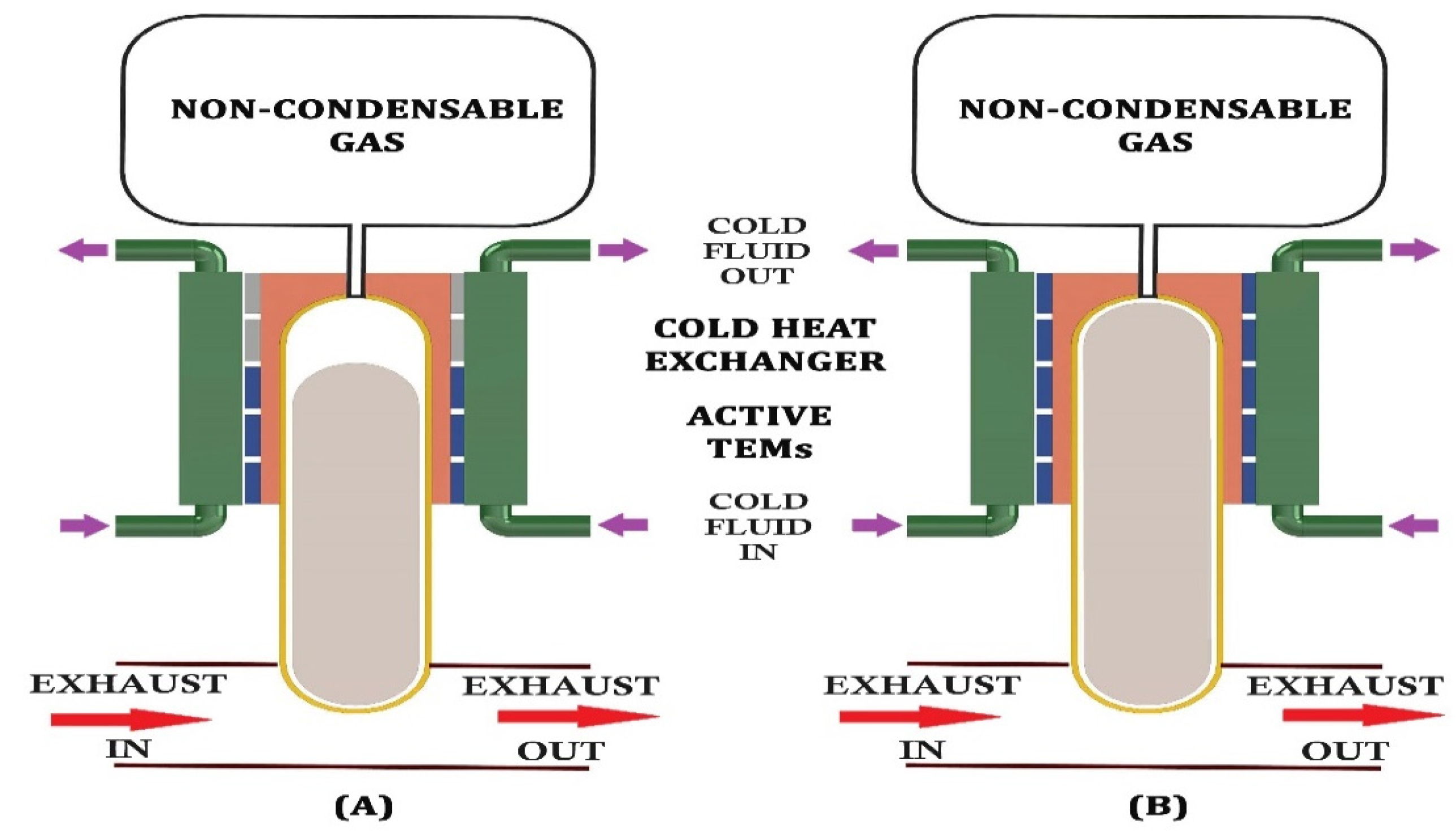
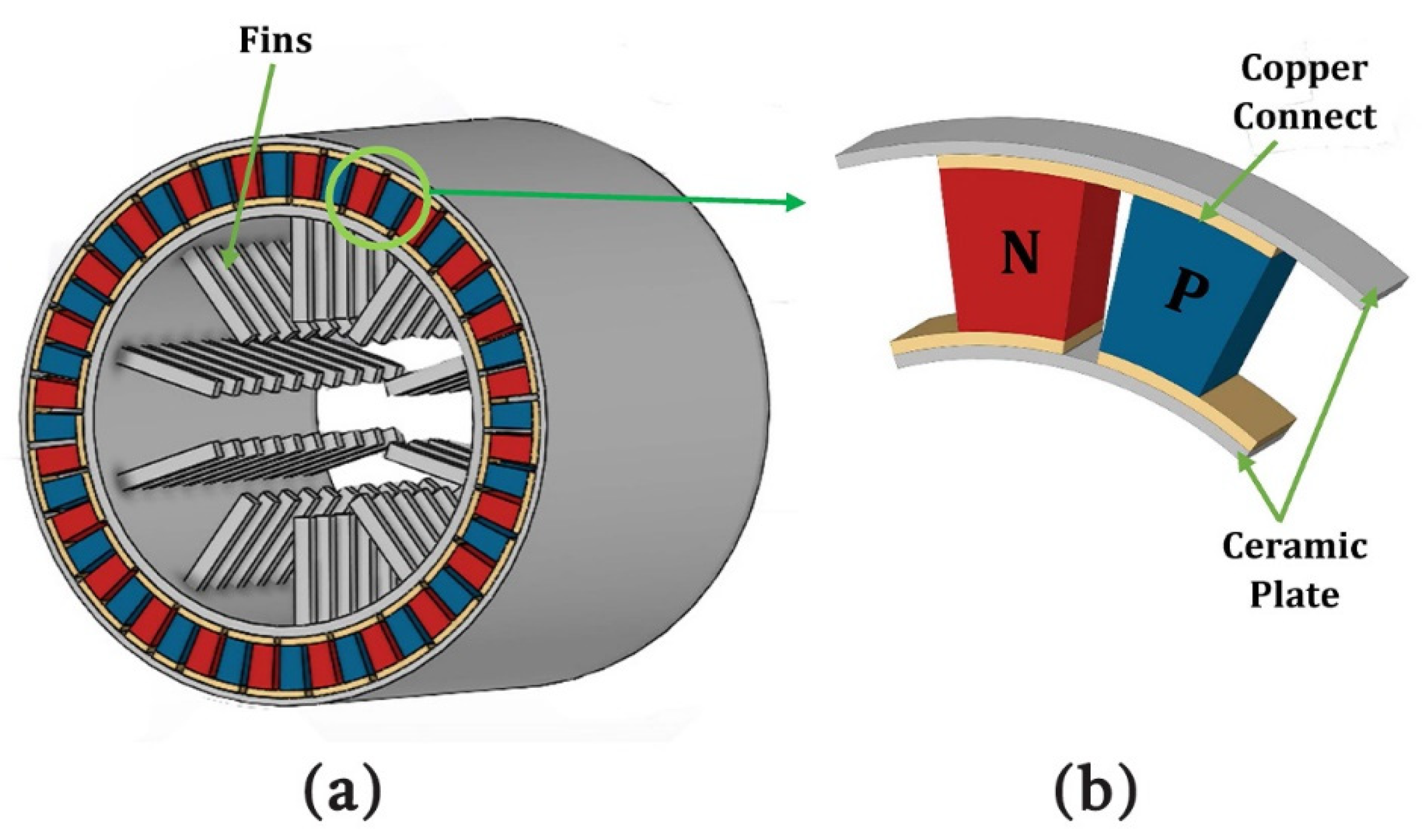
6.5. Annular Thermoelectric Generator
7. Structural Improvement of TE Leg for Automobile Systems
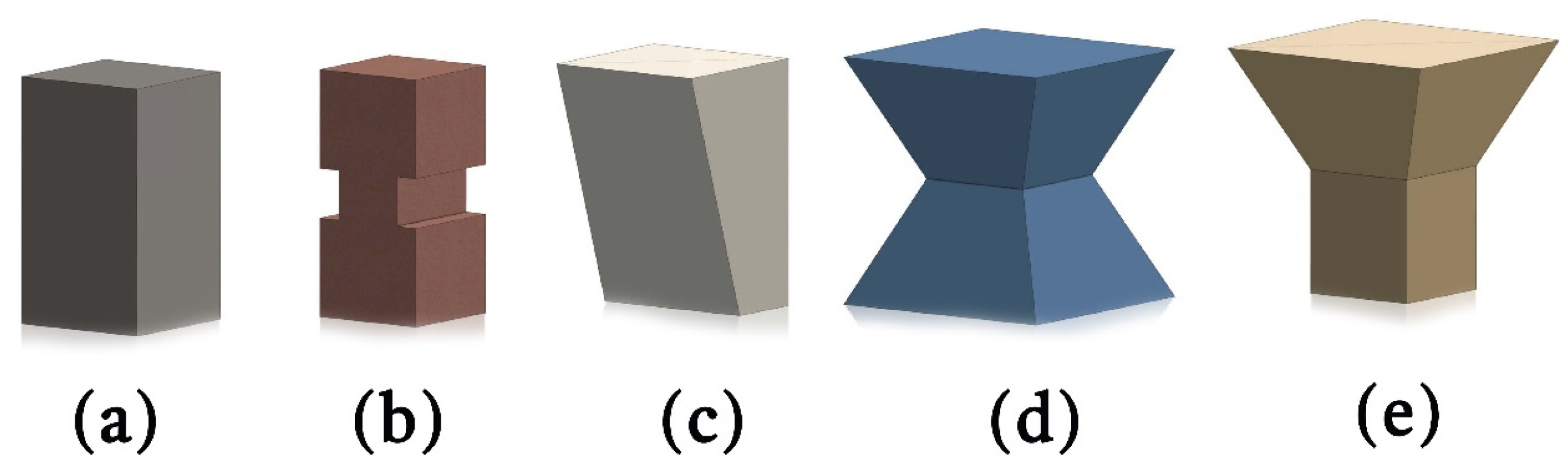
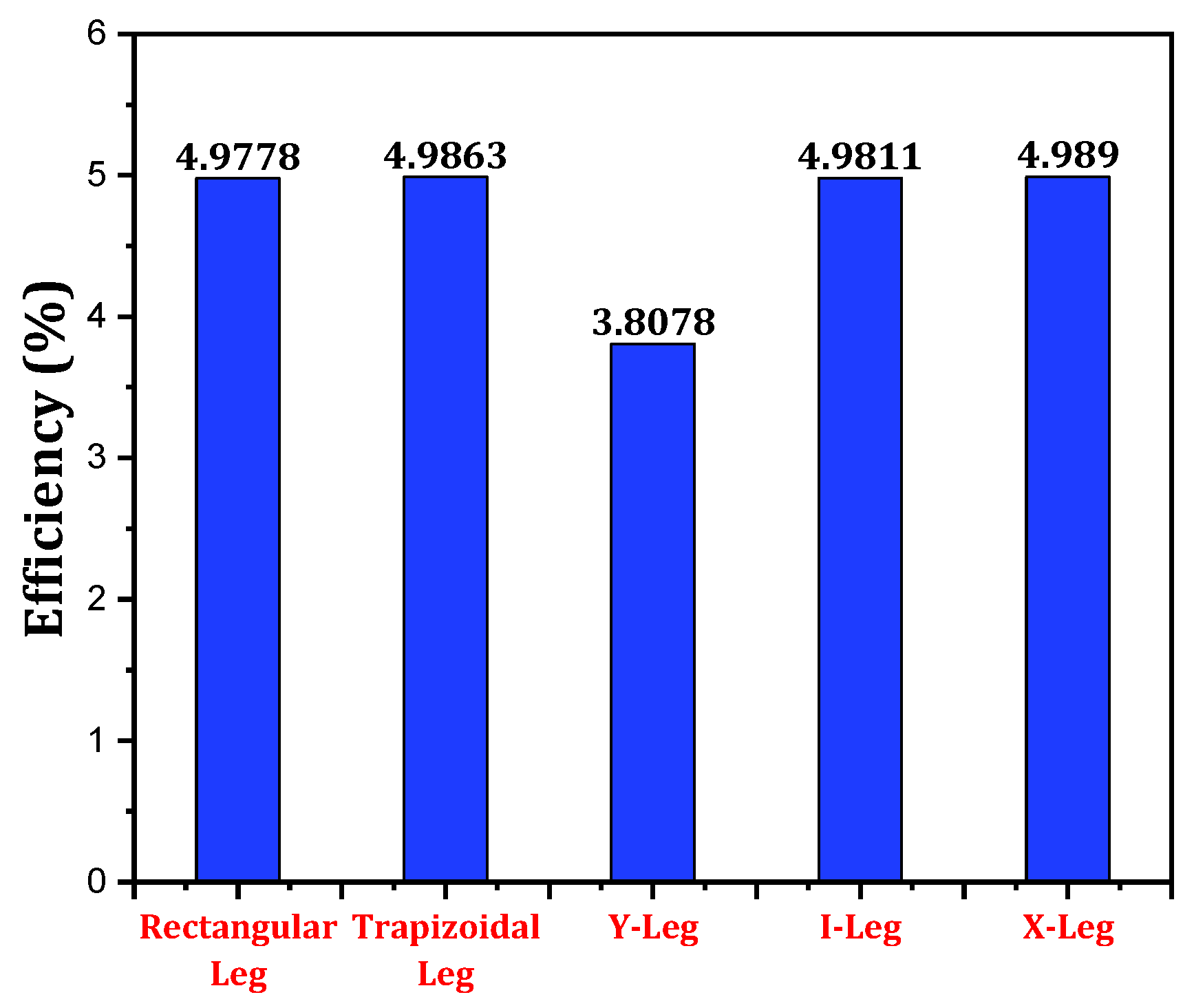
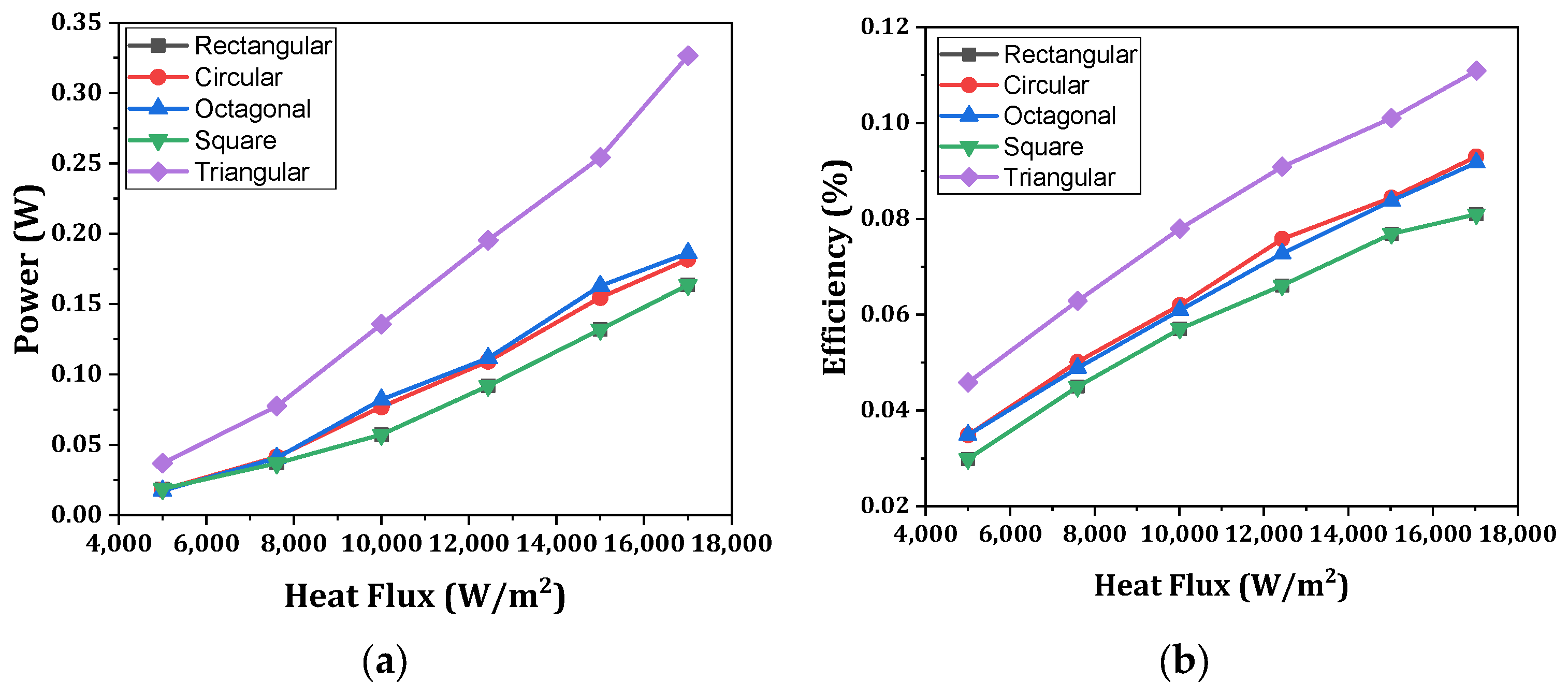
7.1. Leg Geometry Optimization
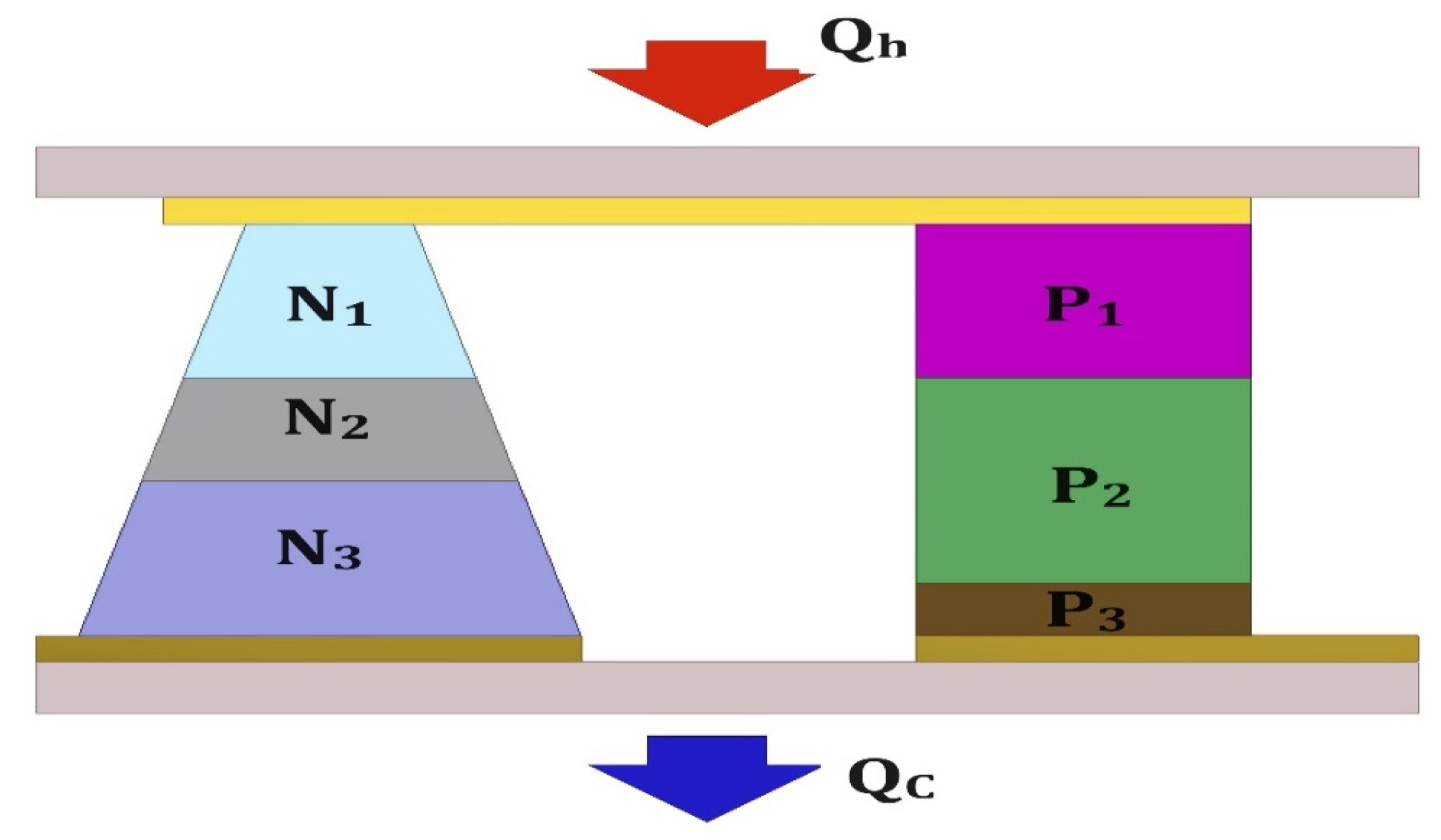
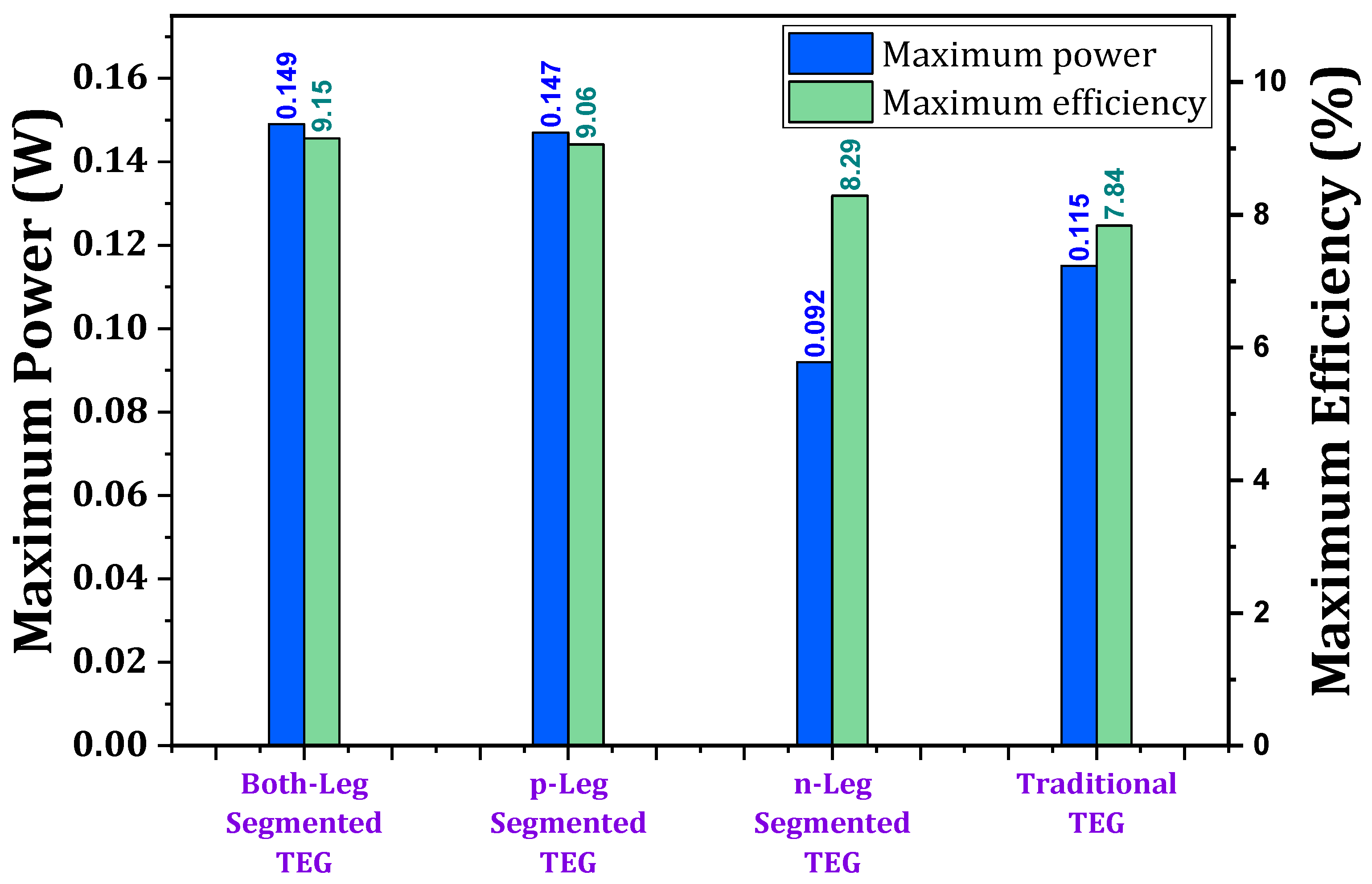
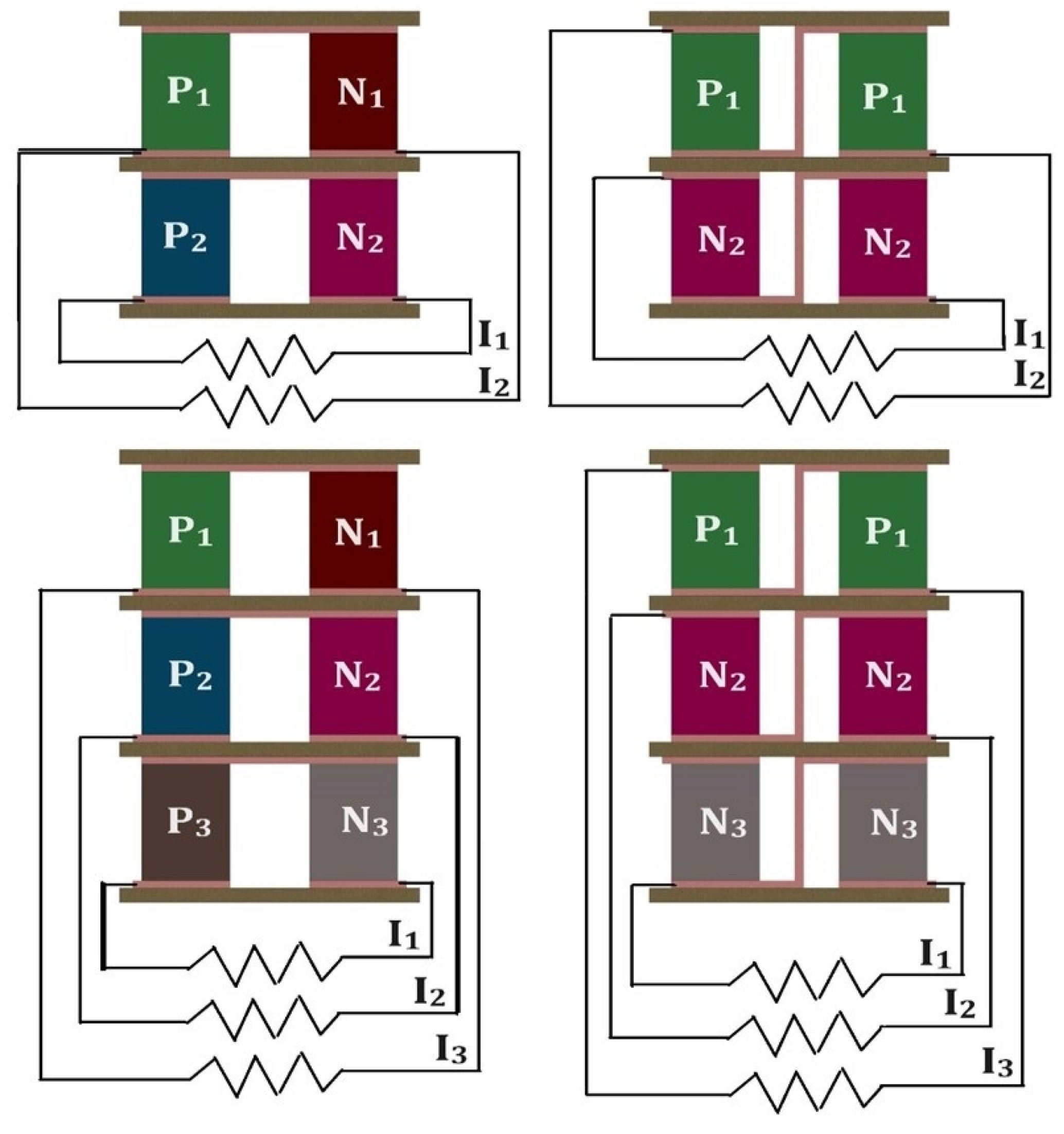
7.2. Segmented Leg Geometry
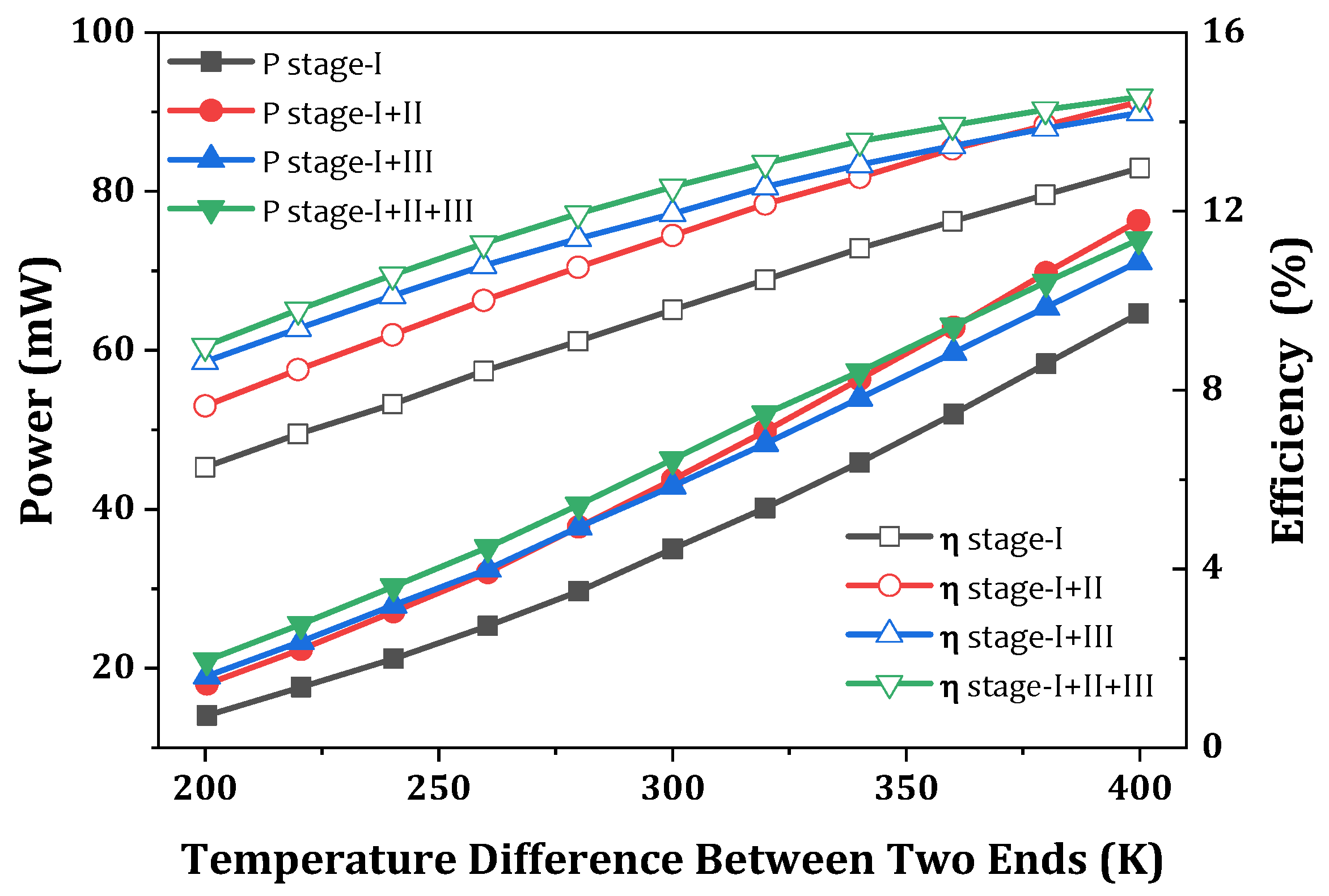
7.3. Multi-Stage TEG
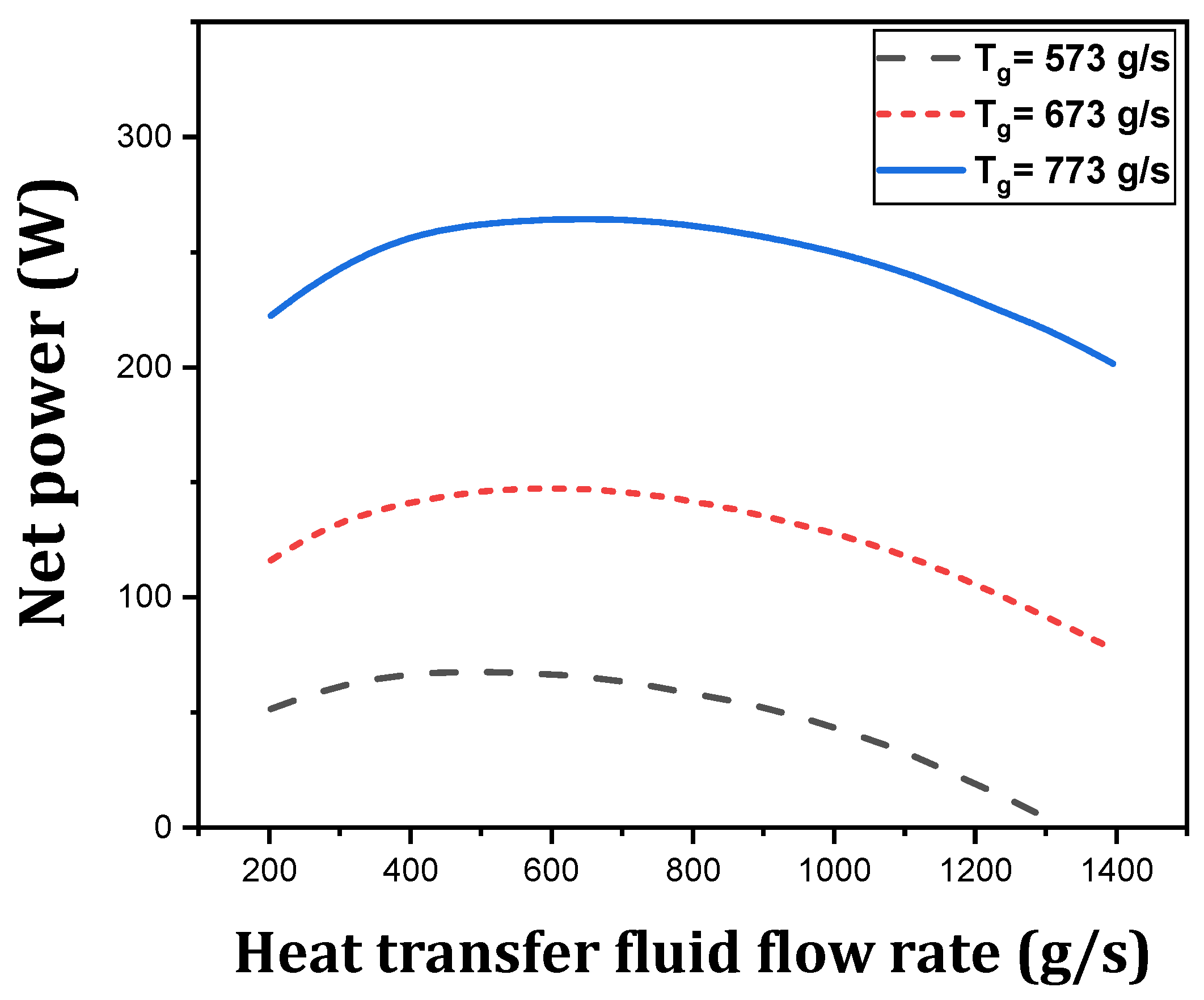
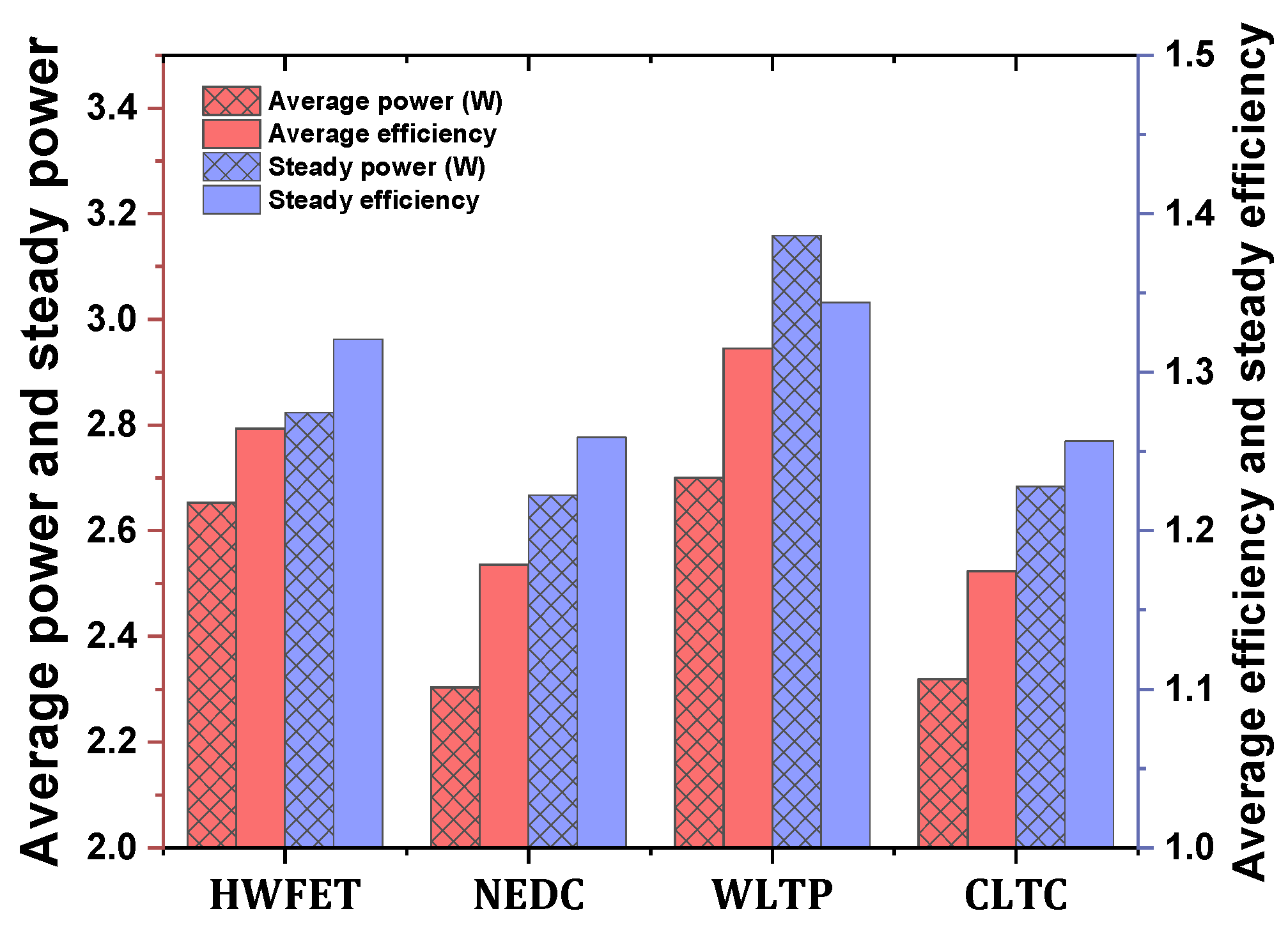
8. Actual Operating Condition
9. Conclusions
- (a)
- The output performance of the TEG system depends on the material that is used, and the material performance depends on the ZT value. BiTe has a high ZT value at room temperature. SnSe is non-toxic and suitable for a medium to high temperature range. Most high-performing materials are used in the range of 500–1000 K, and most are expensive. Organic and inorganic composites are low-cost materials, but the ZT value is low, which makes the materials unsuitable for TEG applications. According to the literature, the highest ZT value is 3 for SnSe-based material. However, they are expensive to synthesize. The ZT value is as high as possible to achieve a higher conversion efficiency. However, no known material has a sufficient ZT value for reasonable performance. For example, for a temperature difference of 350 K and a ZT value of 10, obtaining a Carnot efficiency of ~30% is possible. Thus, we should emphasize research on high-performance and main material synthesis.
- (b)
- The convective heat transfer coefficient of exhaust gas is relatively low. A heat exchanger can enhance the heat absorption capability and improve temperature uniformity. On the other hand, it imposes additional weight and produces back pressure losses that reduce the engine’s combustion efficiency.
- (c)
- Metal foam and extended surfaces that are integrated into heat exchangers can significantly augment the convective heat transfer coefficient and thus improve the heat transfer rate. It was observed that the heat transfer effectiveness was enhanced by 283%, and the power output was doubled, when the foam filling rate was 75%. The integration of vortex generators enhanced the net power by 177.4%. A parabolic fin design improved the temperature uniformity of the TEG system by 91.3%. However, a significant temperature drop may occur along the flow direction. These additional features impose more back pressure losses. Much attention can be paid to selecting the type and dimension of the foam material and extended surface.
- (d)
- A phase change material used as an intermediate fluid promotes temperature uniformity in the system. It also allows the system to operate at a nearly constant temperature. The maximum improvement in power output is 32.6% compared to a conventional one, while the reduction in the thermoelectric optimum module area is 74.2%. The maximum conversion efficiency achieved is 5.4%, a 1.14-time improvement. However, it makes the system bulky and imposes additional weight.
- (e)
- A heat pipe is a super-thermal-conductive device that enhances the heat extraction capacity at both ends of the TEG. It allows the system to operate under a nearly constant temperature difference. It protects the device from high-temperature damage, provides the flexibility to overcome space limitations, and enables the system to accommodate far beyond the exhaust channel, where enough space is available. A VCHP permits the TEG system to operate at a constant temperature, even at part load. The heat pipe has low thermal resistance, and a slight temperature drop occurs between the evaporator and condenser at only 15 °C. Due to this, power output increases by 43%.
- (f)
- The leg shape and leg geometry greatly influence the thermal and electrical resistance, which further influence the overall performance. It also reduces the overall material cost. Despite this, it may lead to a compromise in mechanical strength. A nanomaterial-based trapezoidal-leg geometry has a maximum efficiency of 8.74%, which his 19.73% more than the traditional-leg TEG. Compared with a cylindrical-leg TEG, an hourglass-shaped TEG system has 69.62% greater output and 70.96% enhanced efficiency.
- (g)
- Segmentation and multi-staging lead to better power output and high-quality exhaust heat efficiency, enabling the TE material to operate in its best-performing temperature range. Increasing the number of segments and stages offers more power. However, these make the system more complex. Therefore, for the performance improvement, these changes should be made while selecting the number of segments and the different stages, considering other factors, including material cost, design complexity, and robustness. Segmented designs increase the power output by 30.8%. A system with segmented irregular legs increases the power by 51.71%. A seven-layer segmented TEG system achieves a maximum efficiency of 16.7%. On the other hand, compared to a single-stage TEG, the maximum power and maximum efficiency increase in a two-stage TEG by 44.8% and 6.9%, respectively. Meanwhile, these figures are 36.40% and 34.47% for a three-stage TEG. A unileg multi-stage cascaded TEG outperforms an uncoupled design by 75%.
10. Future Scope
- 1.
- Material research may intensify to gain a higher ZT value with low costs.
- 2.
- Research can be carried out to improve the structural design of heat exchangers further to achieve lower thermal resistance and a high heat transfer coefficient. The catalytic converter can be modified as a heat exchanger by embedding metal foam and coating the metal form with a suitable catalyst.
- 3.
- On the cooling side of the TEM, the dissipated heat can be utilized to preheat the air that is used in engine combustion, thus improving the engine’s combustion efficiency.
- 4.
- At the cooling side of the TEM, the dissipated heat can be utilized to preheat the air that is used in the engine combustion, thus improving the engine’s combustion efficiency.
- 5.
- More work can be focused on optimizing the leg geometry, segmentation, and multi-staging.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| BM | Ball milling |
| BST | Bismuth antimony telluride |
| CFD | Computational fluid dynamics |
| CLTC | China Light-duty vehicle Test Cycle |
| CSP | Cold-sintering process |
| DFT | Density Functional Theory |
| EG | Expanded graphite |
| FEM | Finite element method |
| GA | Genetic algorithm |
| HATEG | Heat transfer fluid in annular TEG |
| HP | Hot press |
| HPS | Hot-press sintering |
| HWFET | Highway Fuel Economy Test |
| ICE | Internal combustion engine |
| IFTEG | Intermediate fluid thermoelectric generator |
| LM | Levitation melting |
| LVG | Longitudinal vortex generator |
| M | Melting |
| MA | Mechanical alloying |
| Max | Maximum |
| MCDM | Multiple criteria decision making |
| MS | Melt-spinning |
| MWCNT | Multi-walled carbon nanotubes |
| NEDC | New European Driving Cycle |
| NTU | Number of Transfer Units |
| OEM | Original Equipment Manufacturer |
| P | Power |
| PCM | Phase change material |
| PPI | Pores per inch |
| PSO | Particle swarm optimization |
| RPH | Rapid Hot-pressing Sintering |
| SHS | Self-propagating High temperature Synthesis |
| SPS | Spark plasma sintering |
| STEG | Segmented thermoelectric generator |
| SUV | Sports utility vehicle |
| TC | Thermocouple |
| TE | Thermoelectric |
| TEG | Thermoelectric generator |
| TEM | Thermoelectric module |
| Temp | Temperature |
| TOPSIS | Technique for Order of Preference by Similarity to Ideal Solution |
| UT | Ultrasonic Treatment |
| VCHP | Variable conductance heat pipe |
| WLTP | Worldwide harmonized Light vehicles Test Procedure |
| ZT | Dimensionless figure of merit |
Nomenclature
| A | cross-sectional area (m2) |
| electric field vector (volt m−1) | |
| I | electric current (amp) |
| current density vector (amp m−2) | |
| k | thermal conductivity (Wm−1K−1) |
| thermal conductance (WK−1) | |
| L | length of thermocouple (m) |
| N | number of thermocouples |
| heat flux vector (Wm−2) | |
| volumetric heat generation rate (Wm−3) | |
| heat rate absorbed at the hot junction (W) | |
| heat rate evolved at the cold junction (W) | |
| electric resistivity (ohm m) | |
| electrical resistance (ohm) | |
| external load resistance (ohm) | |
| Re | Reynolds number |
| temperature (K) | |
| Arithmetic average temperature, (K) | |
| temperature at the hot junction (K) | |
| temperature at the cold junction (K) | |
| total voltage drops for N thermocouples (volt) | |
| net output power (W) | |
| coordinate starts from the hot junction to the cold junction (m) | |
| figure of merit (K−1) | |
| Greek Letters | |
| thermal or conversion efficiency | |
| Seebeck coefficient (volt K−1) | |
| Thomson coefficient (volt K−1) | |
| Subscripts | |
| max | extremum or maximum |
| n | n-type thermocouple |
| p | p-type thermocouple |
References
- Energy Institute. Statistical Review of World Energy 2023. Available online: https://www.energyinst.org/statistical-review (accessed on 13 July 2023).
- Oil Market Report—May 2023. Available online: https://www.iea.org/reports/oil-market-report-may-2023 (accessed on 26 November 2023).
- IEA. CO2 Emissions in 2022, IEA, Paris. 2023. Available online: https://www.iea.org/reports/co2-emissions-in-2022 (accessed on 13 July 2023).
- Hewawasam, L.S.; Jayasena, A.S.; Afnan, M.M.M.; Ranasinghe, R.A.C.P.; Wijewardane, M.A. Waste heat recovery from thermo-electric generators (TEGs). Energy Rep. 2020, 6, 474–479. [Google Scholar] [CrossRef]
- Mohamed, E.S. Development and performance analysis of a TEG system using exhaust recovery for a light diesel vehicle with assessment of fuel economy and emissions. Appl. Therm. Eng. 2019, 147, 661–674. [Google Scholar] [CrossRef]
- Vázquez, J.; Sanz-Bobi, M.A.; Palacios, R.; Arenas, A. State of the art of thermoelectric generators based on heat recovered from the exhaust gases of automobiles. In Proceedings of the 7th European Workshop on Thermoelectrics, Pamplona, Spain, 3–4 October 2002; p. 17. [Google Scholar]
- IB Physics. Available online: https://brettchoypd4.weebly.com/assessment-statements1.html (accessed on 3 July 2023).
- Ping, X.; Yang, F.; Zhang, H.; Xing, C.; Yu, M.; Wang, Y. Investigation and multi-objective optimization of vehicle engine-organic Rankine cycle (ORC) combined system in different driving conditions. Energy 2023, 263, 125672. [Google Scholar] [CrossRef]
- Ping, X.; Yang, F.; Zhang, H.; Xing, C.; Zhang, W.; Wang, Y.; Yao, B. Dynamic response assessment and multi-objective optimization of organic Rankine cycle (ORC) under vehicle driving cycle conditions. Energy 2023, 263, 125551. [Google Scholar] [CrossRef]
- Elmaihy, A.; Rashad, A.; Elweteedy, A.; Nessim, W. Energy and exergy analyses for organic Rankine cycle driven by cooling water of passenger car engine using sixteen working fluids. Energy Convers. Manag.: X 2023, 20, 100415. [Google Scholar] [CrossRef]
- Rêgo, A.T.; Hanriot, S.M.; Oliveira, A.F.; Brito, P.; Rêgo, T.F.U. Automotive exhaust gas flow control for an ammonia–water absorption refrigeration system. Appl. Therm. Eng. 2014, 64, 101–107. [Google Scholar] [CrossRef]
- Farzadi, R.; Bazargan, M. Experimental study of a diffusion absorption refrigeration cycle supplied by the exhaust waste heat of a sedan car at low engine speeds. Heat Mass Transf. 2020, 56, 1353–1363. [Google Scholar] [CrossRef]
- Alklaibi, A.M.; Lior, N. Waste heat utilization from internal combustion engines for power augmentation and refrigeration. Renew. Sustain. Energy Rev. 2021, 152, 111629. [Google Scholar] [CrossRef]
- Peng, Q.; Liu, R.; Zhou, G.; Zhao, X.; Dong, S.; Zhang, Z.; Zhang, H. Summary of Turbocharging as a Waste Heat Recovery System for a Variable Altitude Internal Combustion Engine. ACS Omega 2023, 8, 27932–27952. [Google Scholar] [CrossRef]
- Fu, J.; Liu, J.; Wang, Y.; Deng, B.; Yang, Y.; Feng, R.; Yang, J. A comparative study on various turbocharging approaches based on IC engine exhaust gas energy recovery. Appl. Energy 2014, 113, 248–257. [Google Scholar] [CrossRef]
- Teo, A.E.; Yahya, W.J.; Romagnoli, A.; Rajoo, S.; Noor, A.M. Effectiveness of series and parallel turbo compounding on turbocharged diesel engine. J. Mech. Eng. Sci. 2015, 8, 1448–1459. [Google Scholar] [CrossRef]
- Alwi, E.; Amin, B.; Afnison, W. Electric turbo compounding (ETC) as exhaust energy recovery system on vehicle. GEOMATE J. 2020, 19, 228–234. [Google Scholar]
- Güven, M.; Bedir, H.; Anlaş, G. Optimization and application of Stirling engine for waste heat recovery from a heavy-duty truck engine. Energy Convers. Manag. 2019, 180, 411–424. [Google Scholar] [CrossRef]
- Catapano, F.; Perozziello, C.; Vaglieco, B.M. Heat transfer of a Stirling engine for waste heat recovery application from internal combustion engines. Appl. Therm. Eng. 2021, 198, 117492. [Google Scholar] [CrossRef]
- Kodihal, K.; Sagar, A. Prototype Design of a Small Scale Thermionic Energy Generator for Waste Heat Recovery in Hybrid Electric Vehicle (No. 2019-28-0027); SAE Technical Paper 2019; SAE International: Warrendale, PA, USA, 2019. [Google Scholar] [CrossRef]
- Siddique, A.R.M.; Mahmud, S.; Van Heyst, B. A review of the state of the science on wearable thermoelectric power generators (TEGs) and their existing challenges. Renew. Sustain. Energy Rev. 2017, 73, 730–744. [Google Scholar] [CrossRef]
- Sanin-Villa, D. Recent Developments in Thermoelectric Generation: A Review. Sustainability 2022, 14, 16821. [Google Scholar] [CrossRef]
- Jouhara, H.; Żabnieńska-Góra, A.; Khordehgah, N.; Doraghi, Q.; Ahmad, L.; Norman, L.; Axcell, B.; Wrobel, L.; Dai, S. Thermoelectric generator (TEG) technologies and applications. Int. J. Thermofluids 2021, 9, 100063. [Google Scholar] [CrossRef]
- Zoui, M.A.; Bentouba, S.; Stocholm, J.G.; Bourouis, M. A Review on Thermoelectric Generators: Progress and Applications. Energies 2020, 13, 3606. [Google Scholar] [CrossRef]
- Yan, J.; Liao, X.; Yan, D.; Chen, Y. Review of micro thermoelectric generator. J. Microelectromechanical Syst. 2018, 27, 1–18. [Google Scholar] [CrossRef]
- Orr, B.; Akbarzadeh, A.; Mochizuki, M.; Singh, R. A review of car waste heat recovery systems utilising thermoelectric generators and heat pipes. Appl. Therm. Eng. 2016, 101, 490–495. [Google Scholar] [CrossRef]
- Zhang, L.; Lin, S.; Hua, T.; Huang, B.; Liu, S.; Tao, X. Fiber-based thermoelectric generators: Materials, device structures, fabrication, characterization, and applications. Adv. Energy Mater. 2018, 8, 1700524. [Google Scholar] [CrossRef]
- Muchuweni, E.; Mombeshora, E.T. Enhanced thermoelectric performance by single-walled carbon nanotube composites for thermoelectric generators: A review. Appl. Surf. Sci. Adv. 2023, 13, 100379. [Google Scholar] [CrossRef]
- Champier, D. Thermoelectric generators: A review of applications. Energy Convers. Manag. 2017, 140, 167–181. [Google Scholar] [CrossRef]
- Wright, D.A. Materials for direct-conversion thermoelectric generators. Metall. Rev. 1970, 15, 147–160. [Google Scholar] [CrossRef]
- Shen, Z.G.; Tian, L.L.; Liu, X. Automotive exhaust thermoelectric generators: Current status, challenges and future prospects. Energy Convers. Manag. 2019, 195, 1138–1173. [Google Scholar] [CrossRef]
- Crane, D.T.; LaGrandeur, J.W. Progress report on BSST-Led US department of energy automotive waste heat recovery program. J. Electron. Mater. 2010, 39, 2142–2148. [Google Scholar] [CrossRef]
- LaGrandeur, J.; Crane, D.; Hung, S.; Mazar, B.; Eder, A. Automotive waste heat conversion to electric power using skutterudite, TAGS, PbTe and BiTe. In Proceedings of the 2006 25th International Conference on Thermoelectrics, Vienna, Austria, 6–10 August 2006; IEEE: Piscataway, NJ, USA, 2006; pp. 343–348. [Google Scholar] [CrossRef]
- Espinosa, N.; Lazard, M.; Aixala, L.; Scherrer, H. Modeling a thermoelectric generator applied to diesel automotive heat recovery. J. Electron. Mater. 2010, 39, 1446–1455. [Google Scholar] [CrossRef]
- Mori, M.; Yamagami, T.; Sorazawa, M.; Miyabe, T.; Takahashi, S.; Haraguchi, T. Simulation of fuel economy effectiveness of exhaust heat recovery system using thermoelectric generator in a series hybrid. SAE Int. J. Mater. Manuf. 2011, 4, 1268–1276. [Google Scholar] [CrossRef]
- Hussain, Q.E.; Brigham, D.R.; Maranville, C.W. Thermoelectric exhaust heat recovery for hybrid vehicles. SAE Int. J. Engines 2009, 2, 1132–1142. [Google Scholar] [CrossRef]
- Ikoma, K.; Munekiyo, M.; Furuya, K.; Kobayashi, M.A.K.M.; Izumi, T.A.I.T.; Shinohara, K.A.S.K. Thermoelectric module and generator for gasoline engine vehicles. In Proceedings of the Seventeenth International Conference on Thermoelectrics. Proceedings ICT98 (Cat. No. 98TH8365), Nagoya, Japan, 24–28 May 1998; IEEE: Piscataway, NJ, USA, 1998; pp. 464–467. [Google Scholar] [CrossRef]
- Zulkepli, N.; Yunas, J.; Mohamed, M.A.; Hamzah, A.A. Review of Thermoelectric Generators at Low Operating Temperatures: Working Principles and Materials. Micromachines 2021, 12, 734. [Google Scholar] [CrossRef]
- Soleimani, Z.; Zoras, S.; Ceranic, B.; Cui, Y.; Shahzad, S. A comprehensive review on the output voltage/power of wearable thermoelectric generators concerning their geometry and thermoelectric materials. Nano Energy 2021, 89, 106325. [Google Scholar] [CrossRef]
- Zhu, B.; Su, X.; Shu, S.; Luo, Y.; Tan, X.Y.; Sun, J.; Sun, D.; Zhang, H.; Zhang, Q.; Suwardi, A.; et al. Cold-sintered Bi2Te3-based materials for engineering nanograined thermoelectrics. ACS Appl. Energy Mater. 2022, 5, 2002–2010. [Google Scholar] [CrossRef]
- Shi, Q.; Zhao, X.; Chen, Y.; Lin, L.; Ren, D.; Liu, B.; Zhou, C.; Ang, R. Cu2Te Incorporation-Induced High Average Thermoelectric Performance in p-Type Bi2Te3 Alloys. ACS Appl. Mater. Interfaces 2022, 14, 45582–45589. [Google Scholar] [CrossRef]
- Zhang, Q.; Yuan, M.; Pang, K.; Zhang, Y.; Wang, R.; Tan, X.; Wu, G.; Hu, H.; Wu, J.; Sun, P.; et al. High-Performance Industrial-Grade p-Type (Bi, Sb)2Te3 Thermoelectric Enabled by a Stepwise Optimization Strategy. Adv. Mater. 2023, 35, 2300338. [Google Scholar] [CrossRef] [PubMed]
- Shen, D.; Cheng, R.; Wang, W.; Li, H.; Chen, C.; Zhang, Q.; Chen, Y. Enhanced thermoelectric performance of p-type Bi2Si2Te6 enabled via synergistically optimizing carrier concentration and suppressing bipolar effect. Mater. Today Phys. 2023, 37, 101185. [Google Scholar] [CrossRef]
- Gayner, C.; Menezes, L.T.; Natanzon, Y.; Kauffmann, Y.; Kleinke, H.; Amouyal, Y. Development of Nanostructured Bi2Te3 with High Thermoelectric Performance by Scalable Synthesis and Microstructure Manipulations. ACS Appl. Mater. Interfaces 2023, 15, 13012–13024. [Google Scholar] [CrossRef] [PubMed]
- Shen, T.; Li, K.Y.; Chen, Z.J.; Wu, H.F.; Si, J.X. Enhanced Thermoelectric Performance of n-Type Polycrystalline SnSe via MoCl5 Doping. J. Electron. Mater. 2020, 49, 621–626. [Google Scholar] [CrossRef]
- Abbas, A.; Xu, Z.; Nisar, M.; Li, D.; Li, F.; Zheng, Z.; Liang, G.; Fan, P.; Chen, Y.-X. Achieving weak anisotropy in N-type I-doped SnSe polycrystalline thermoelectric materials. J. Eur. Ceram. Soc. 2022, 42, 7027–7035. [Google Scholar] [CrossRef]
- Zhang, S.; Zhu, C.; He, X.; Wang, J.; Luo, F.; Wang, J.; Liu, H.; Sun, Z. Enhanced thermoelectric performance of n-type polycrystalline SnSe via NdCl3 doping. J. Alloys Compd. 2022, 910, 164900. [Google Scholar] [CrossRef]
- Mao, X.-Y.; Shi, X.-L.; Zhai, L.-C.; Liu, W.-D.; Chen, Y.-X.; Gao, H.; Li, M.; Wang, D.-Z.; Wu, H.; Zheng, Z.-H.; et al. High thermoelectric and mechanical performance in the n-type polycrystalline SnSe incorporated with multi-walled carbon nanotubes. J. Mater. Sci. Technol. 2022, 114, 55–61. [Google Scholar] [CrossRef]
- Nisar, M.; Chen, Y.-X.; Qin, W.; Abbas, A.; Zheng, Z.; Fan, P.; Li, F. Effects of heavy bromine doping on the thermoelectric performance and dynamic stability of SnSe2 polycrystals. J. Alloys Compd. 2023, 959, 170566. [Google Scholar] [CrossRef]
- Li, D.; Tan, X.; Xu, J.; Liu, G.; Jin, M.; Shao, H.; Huang, H.; Zhang, J.; Jiang, J. Enhanced thermoelectric performance in n-type polycrystalline SnSe by PbBr2 doping. RSC Adv. 2017, 7, 17906–17912. [Google Scholar] [CrossRef]
- Mac, T.K.; Manh, D.H.; Nguyen, H.T.; Giang, T.T.H.; Lam, D.S.; Linh, D.C.; Pham, A.T.T.; Phan, B.T.; Thanh, T.D.; Duong, A.T. Synthesis and Thermoelectric Characterization of Sb-Doped Cu2Se by Mechanical Alloying and Solid-State Reaction. J. Electron. Mater. 2023, 53, 693–701. [Google Scholar] [CrossRef]
- Patel, S.R.; Chaki, S.H.; Solanki, M.B.; Kannaujiya, R.M.; Parekh, Z.R.; Khimani, A.J.; Deshpande, M.P. The impact of Ni and Zn doping on the thermal durability and thermoelectric variables of pristine CuSe nanoparticles. Mater. Adv. 2023, 4, 5238–5251. [Google Scholar] [CrossRef]
- Zhao, X.; Li, M.; Ma, R.; Zhang, Y.; Song, H. Effects of introducing MXene on thermoelectric properties of Cu2Se alloys. J. Alloys Compd. 2024, 971, 172787. [Google Scholar] [CrossRef]
- Rapaka, S.S.; Anwar, S.; Singh, J.; Anwar, S. Transport studies of two-step synthesized Cu2Se-Graphene nanocomposites. Phys. B: Condens. Matter 2023, 665, 415044. [Google Scholar] [CrossRef]
- Yang, D.; Zhang, D.; Ao, D.; Nisar, M.; Mansoor, A.; Chen, Y.; Li, F.; Ma, H.; Liang, G.; Zhang, X.; et al. High thermoelectric performance of aluminum-doped cuprous selenide thin films with exceptional flexibility for wearable applications. Nano Energy 2023, 117, 108930. [Google Scholar] [CrossRef]
- Bhat, P.C.; Anusha Ani, A.; Shanubhogue, U.D.; Poornesh, P.; Rao, A.; Chattopadhyay, S. Investigations on Bi Doped Cu2Se Prepared by Solid State Reaction Technique for Thermoelectric Applications. Energies 2023, 16, 3010. [Google Scholar] [CrossRef]
- Ahmed, A.J.; Hossain, M.S.A.; Islam, S.M.K.N.; Yun, F.; Yang, G.; Hossain, R.; Khan, A.; Na, J.; Eguchi, M.; Yamauchi, Y.; et al. Significant improvement in electrical conductivity and figure of merit of nanoarchitectured porous SrTiO3 by La doping optimization. ACS Appl. Mater. Interfaces 2020, 12, 28057–28064. [Google Scholar] [CrossRef]
- Zheng, Y.; Zhang, X.; Xie, W.; Ge, N.; Ren, Y.; Wei, X.; Dai, B. Thermoelectric performance enhancement for Ca3Co4O9 ceramics Co-doped with Ag and Tb. J. Electron. Mater. 2022, 51, 4938–4943. [Google Scholar] [CrossRef]
- Hira, U.; Ali, S.S.; Latif, S.; Pryds, N.; Sher, F. Improved high-temperature thermoelectric properties of dual-doped Ca3Co4O9. ACS Omega 2022, 7, 6579–6590. [Google Scholar] [CrossRef]
- Zhang, Z.; Zhang, C.; Liao, Q.; Qin, L.; Deng, D.; Liang, L. Optimizing electrical and thermal transport properties of Ca3Co4O9 based thermoelectric materials by Ag and Fe co-addition. Mater. Today Commun. 2022, 33, 104866. [Google Scholar] [CrossRef]
- Wu, H.; Shi, X.-L.; Liu, W.-D.; Gao, H.; Wang, D.-Z.; Yin, L.-C.; Liu, Q.; Chen, Z.-G. Ni doping and rational annealing boost thermoelectric performance of nanostructured double perovskite Pr1.8Sr0.2CoFeO6. Appl. Mater. Today 2022, 29, 101580. [Google Scholar] [CrossRef]
- Akram, R.; Khan, J.; Rafique, S.; Hussain, M.; Maqsood, A.; Naz, A.A. Enhanced thermoelectric properties of single-phase Na doped NaXCoO2 thermoelectric material. Mater. Lett. 2021, 300, 130180. [Google Scholar] [CrossRef]
- Van Du, N.; Binh, N.V.; Tran, M.T.; Tu, N.; Huy, P.T.; Rahman, J.U.; Shin, W.H.; Tuan, D.A.; Cho, J.Y.; Lee, S.; et al. Improved thermoelectric properties of Sb-doped Ti0. 5Zr0. 5NiSn alloy with refined structure induced by rapid synthesis processes. J. Alloys Compd. 2024, 970, 172528. [Google Scholar] [CrossRef]
- Tan, C.; Wang, H.; Yao, J.; Chen, T.; Wang, L.; Sun, Y.; Khan, M.; Wang, H.; Wang, C. Synchronously enhanced thermoelectric and mechanical properties of Ti doped NbFeSb based half-heusler alloys by carrier regulation and phonon engineering. J. Eur. Ceram. Soc. 2022, 42, 7010–7016. [Google Scholar] [CrossRef]
- Kahiu, J.N.; Shenoy, U.S.; Kihoi, S.K.; Kim, H.; Yi, S.; Bhat, D.K.; Lee, H.S. Optimized electronic performance in half-Heusler Ti-doped NbFeSb materials by stoichiometric tuning at the Fe and Sb sites. J. Alloys Compd. 2022, 891, 162033. [Google Scholar] [CrossRef]
- Wang, Q.; Xie, X.; Li, S.; Zhang, Z.; Li, X.; Yao, H.; Chen, C.; Cao, F.; Sui, J.; Liu, X.; et al. Enhanced thermoelectric performance in Ti(Fe, Co, Ni)Sb pseudo-ternary Half-Heusler alloys. J. Mater. 2021, 7, 756–765. [Google Scholar] [CrossRef]
- Zhang, X.; Li, S.; Zou, B.; Xu, P.; Song, Y.; Xu, B.; Wang, Y.; Tang, G.; Yang, S. Significant enhancement in thermoelectric properties of half-Heusler compound TiNiSn by grain boundary engineering. J. Alloys Compd. 2022, 901, 163686. [Google Scholar] [CrossRef]
- Sattar, M.A.; Javed, M.; Bouzieh, N.A.; Benkraouda, M.; Amrane, N. First-principles investigation on the novel half-Heusler VXTe (X=Cr, Mn, Fe, and Co) alloys for spintronic and thermoelectric applications. Mater. Sci. Semicond. Process. 2023, 155, 107233. [Google Scholar] [CrossRef]
- Liang, J.; Li, Y.; Yin, S.; Wan, C. Flexible organic-intercalated WSe2 hybrid: Electronic structure modification and thermoelectric performance optimization. Mater. Today Energy 2023, 36, 101355. [Google Scholar] [CrossRef]
- Qu, S.; Ming, C.; Qiu, P.; Xu, K.; Xu, Q.; Yao, Q.; Lu, P.; Zeng, H.; Shi, X.; Chen, L. High-performance n-type Ta4SiTe4/polyvinylidene fluoride (PVDF)/graphdiyne organic–inorganic flexible thermoelectric composites. Energy Environ. Sci. 2021, 14, 6586–6594. [Google Scholar] [CrossRef]
- Liang, J.; Li, Y.; Yin, S.; Wan, C. Organic-SnSe2 Hybrid Superlattice toward Synergistic Electrical Transport Optimization and Thermal Conductance Suppression. ACS Appl. Mater. Interfaces 2023, 15, 34956–34963. [Google Scholar] [CrossRef]
- Wang, Y.; Yao, Q.; Qu, S.; Chen, L. Thermoelectric Properties of n-type Poly (nickel 1,1,2,2-ethenetetrathiolate) Prepared by a New One-step Solvothermal Method. J. Wuhan Univ. Technol.-Mater. Sci. Ed. 2022, 37, 760–764. [Google Scholar] [CrossRef]
- Wang, B.; Zhao, H.; Zhang, B.; Wang, D.; Song, A.; Chen, C.; Yu, F.; Hu, W.; Yu, D.; Xu, B.; et al. Enhanced Thermoelectric Performance of n-Type PbTe via Carrier Concentration Optimization over a Broad Temperature Range. ACS Appl. Mater. Interfaces 2023, 15, 14424–14432. [Google Scholar] [CrossRef] [PubMed]
- Wang, S.; Chang, C.; Bai, S.; Qin, B.; Zhu, Y.; Zhan, S.; Zheng, J.; Tang, S.; Zhao, L.D. Fine Tuning of Defects Enables High Carrier Mobility and Enhanced Thermoelectric Performance of n-Type PbTe. Chem. Mater. 2023, 35, 755–763. [Google Scholar] [CrossRef] [PubMed]
- Lv, F.; Zhong, Y.; Zhao, X.; An, X.; Deng, Q.; Gan, L.; Lin, L.; Ang, R. Lead vacancy promotes sodium solubility to achieve ultra-high ZT in only ternary Pb1-xNaxTe. Small 2023, 19, 2301352. [Google Scholar] [CrossRef] [PubMed]
- Chen, P.; Wu, H.; Zhang, B.; Zhou, Z.; Zheng, S.; Dai, L.; Huo, Y.; Zhang, D.; Yan, Y.; Peng, K.; et al. Intrinsically low lattice thermal conductivity and anisotropic thermoelectric performance in In-doped GeSb2Te4 single crystals. Adv. Funct. Mater. 2023, 33, 2211281. [Google Scholar] [CrossRef]
- Si, R.; Zhang, Z.; Liu, C.; Peng, Y.; Bai, X.; Feng, B.; Chen, J.; Gao, J.; Miao, L. Decoupled electron and phonon transport in thermoelectric GeTe compounded with multi-waled carbon nanotubes. Mater. Today Phys. 2023, 34, 101081. [Google Scholar] [CrossRef]
- Yang, Z.; Tseng, Y.C.; Valiyaveettil, S.M.; Yuan, H.; Smith, E.; Chen, K.H.; Huang, Y.; Zou, T.; Kycia, J.; Mozharivskyj, Y. Optimized electronic properties and nano-structural features for securing high thermoelectric performance in doped GeTe. Dalton Trans. 2023, 52, 10689–10699. [Google Scholar] [CrossRef]
- Kim, S.; Kihoi, S.K.; Kim, H.; Kahiu, J.N.; Lee, H.S. Synergetic effect of Bi and Al co-doping in GeTe-based thermoelectric materials leading to optimized carrier concentration tuning and high ZT. J. Alloys Compd. 2024, 970, 172574. [Google Scholar] [CrossRef]
- Chueachot, R.; Nakhowong, R. Achieving thermoelectric performance of rGO/Bi0.5Sb1.5Te3/Cu2Se1–xTex composites through the scattering engineering strategy. J. Mater. 2023; In Press. [Google Scholar] [CrossRef]
- Ioannou, I.; Ioannou, P.S.; Kyratsi, T.; Giapintzakis, J. Effect of Starting Powder Particle Size on the Thermoelectric Properties of Hot-Pressed Bi0.3Sb1.7Te3 Alloys. Materials 2024, 17, 318. [Google Scholar] [CrossRef] [PubMed]
- Kumar, A.; Thoravat, S.; Jin, H.J.; Park, J.; Jin, H.; Rawat, P.; Rhyee, J.S. Hierarchical nano-/micro-architecture phonon scattering of p-type Bismuth Telluride bulk composites with Ag-TiO2 nano particles synthesized by fluidized bed spray coating method. J. Alloys Compd. 2024, 979, 173503. [Google Scholar] [CrossRef]
- Sun, Y.; Wu, H.; Dong, X.; Xie, L.; Liu, Z.; Liu, R.; Zhang, Q.; Cai, W.; Guo, F.; Sui, J. High Performance BiSbTe Alloy for Superior Thermoelectric Cooling. Adv. Funct. Mater. 2023, 33, 2301423. [Google Scholar] [CrossRef]
- Pang, K.; Yuan, M.; Zhang, Q.; Li, Y.; Zhang, Y.; Zhou, W.; Wu, G.; Tan, X.; Noudem, J.G.; Cui, C.; et al. High Performance Thermoelectric Power of Bi0.5Sb1.5Te3 Through Synergistic Cu2GeSe3 and Se Incorporations. Small 2023, 2306701. [Google Scholar] [CrossRef] [PubMed]
- Li, S.; Yin, L.; Liu, Y.; Wang, X.; Chen, C.; Zhang, Q. Rare earth element Ce enables high thermoelectric performance in n-type SnSe polycrystals. J. Mater. Sci. Technol. 2023, 143, 234–241. [Google Scholar] [CrossRef]
- Howells, G.; Mehraban, S.; McGettrick, J.; Lavery, N.; Carnie, M.J.; Burton, M. Rapid Printing of Pseudo-3D Printed SnSe Thermoelectric Generators Utilizing an Inorganic Binder. ACS Appl. Mater. Interfaces 2023, 15, 23068–23076. [Google Scholar] [CrossRef]
- Wang, Z.C.; Jiang, X.D.; Duan, Y.X.; Wang, X.; Ge, Z.H.; Cai, J.M.; Cai, X.M.; Tan, H.L. p-type Sn0.98Ag0.02Se with low thermal conductivity synthesized by hydrothermal method. J. Eur. Ceram. Soc. 2024, 44, 1636–1646. [Google Scholar] [CrossRef]
- Ma, J.; Chen, Y.; Li, W.; Luo, X. Predictability of thermoelectric figure of merit for the single crystal from first principles. Int. J. Heat Mass Transf. 2024, 221, 125063. [Google Scholar] [CrossRef]
- Wang, T.; Duan, X.; Zhang, H.; Ma, J.; Zhu, H.; Qian, X.; Yang, J.Y.; Liu, T.H.; Yang, R. Origins of three-dimensional charge and two-dimensional phonon transports in Pnma phase PbSnSe2 thermoelectric crystal. InfoMat 2023, 5, 12481. [Google Scholar] [CrossRef]
- Wang, Y.; Bai, S.; Shi, H.; Cao, Q.; Qin, B.; Zhao, L.D. Modulating structures to decouple thermoelectric transport leads to high performance in polycrystalline SnSe. J. Mater. Chem. A 2024, 12, 144–152. [Google Scholar] [CrossRef]
- Liu, D.; Wang, D.; Hong, T.; Wang, Z.; Wang, Y.; Qin, Y.; Su, L.; Yang, T.; Gao, X.; Ge, Z.; et al. Lattice plainification advances highly effective SnSe crystalline thermoelectrics. Science 2023, 380, 841–846. [Google Scholar] [CrossRef] [PubMed]
- Yang, X.; Shi, T.E.; Ma, X.Y.; Wang, Z.Y.; Wang, Y.; Wang, J.; Feng, J.; Ge, Z.H. High thermoelectric properties realized in polycrystalline (Ag, Ga) Co-doped SnSe via two-steps point defects modulation. J. Mater. Res. Technol. 2023, 27, 7606–7613. [Google Scholar] [CrossRef]
- Cao, W.; Wang, Z.; Miao, L.; Shi, J.; Xiong, R. Extremely anisotropic thermoelectric properties of SnSe under pressure. Energy Environ. Mater. 2023, 6, 12361. [Google Scholar] [CrossRef]
- Zhao, X.; Ning, S.; Qi, N.; Li, Y.; Dong, Y.; Zhang, H.; Liu, J.; Ye, B.; Chen, Z. Synergetic optimization of electrical and thermal transport properties by Cu vacancies and nanopores in Cu2Se. ACS Appl. Mater. Interfaces 2021, 13, 58936–58948. [Google Scholar] [CrossRef] [PubMed]
- Tie, J.; Xu, G.; Li, Y.; Fan, X.; Yang, Q.; Nan, B. The effect of SPS sintering temperatures on the structure, thermoelectric properties, and scattering mechanism of Cu2Se. J. Mater. Res. Technol. 2023, 27, 3506–3516. [Google Scholar] [CrossRef]
- Yu, J.; Hu, H.; Jiang, Y.; Zhuang, H.L.; Thong, H.C.; Su, B.; Li, J.W.; Han, Z.; Li, H.; Pei, J.; et al. Interface-Enhanced High-Temperature Thermoelectricity in Cu1.99Se/B4C Composites with Synergistically Improved Mechanical Strength. Adv. Energy Mater. 2024, 2303942. [Google Scholar] [CrossRef]
- Li, G.; Song, G.; Wang, N.; Wu, Y.; Du, H.; You, J.; Zhang, H. Enhancement of the thermoelectric properties in β-Cu2+ xSe/aC nano-multilayer films by heterogeneous interfaces. J. Alloys Compd. 2023, 930, 167432. [Google Scholar] [CrossRef]
- Long, Z.; Wang, Y.; Sun, X.; Li, Y.; Zeng, Z.; Zhang, L.; Chen, H. Band Engineering of the Second Phase to Reach High Thermoelectric Performance in Cu2Se-Based Composite Material. Adv. Mater. 2023, 35, 2210345. [Google Scholar] [CrossRef]
- Zhou, Z.; Huang, Y.; Wei, B.; Yang, Y.; Yu, D.; Zheng, Y.; He, D.; Zhang, W.; Zou, M.; Lan, J.L.; et al. Compositing effects for high thermoelectric performance of Cu2Se-based materials. Nat. Commun. 2023, 14, 2410. [Google Scholar] [CrossRef] [PubMed]
- Piyasin, P.; Palaporn, D.; Kurosaki, K.; Pinitsoontorn, S. High-performance thermoelectric properties of Cu2Se fabricated via cold sintering process. Solid State Sci. 2024, 149, 107448. [Google Scholar] [CrossRef]
- Rudradawong, C.; Khammuang, S.; Kotmool, K.; Bovornratanaraks, T.; Limsuwan, P.; Somdock, N.; Sakdanuphab, R.; Sakulkalavek, A. Enhanced thermoelectric properties of Cu2Se via Sb doping: An experimental and computational study. J. Eur. Ceram. Soc. 2023, 43, 401–406. [Google Scholar] [CrossRef]
- Wang, Z.; Zhao, H.; Luo, X.; Han, W.; Wang, H.; Meng, L.; She, X.; Quan, A.; Peng, Y.; Cai, G.; et al. Study on the Targeted Improvement Mechanism of the Carrier Concentration and Mobility of BiCuSeO Ceramics. Micromachines 2023, 14, 1757. [Google Scholar] [CrossRef] [PubMed]
- Zhou, Z.; Liu, R.; Yang, Y.; Zheng, Y.; Wei, B.; Zhang, W.; Zou, M.; Han, J.; Liu, Y.; Lan, J.; et al. Synergistic effects of CuI doping on enhancing thermoelectric performance for n-type Bi2O2Se fabricated by mechanical alloying. Scr. Mater. 2023, 225, 115163. [Google Scholar] [CrossRef]
- Du, X.; Zhang, G.; Guo, X.; Li, C.; Qi, G.; Yuan, Z. Synergistically optimizing thermoelectric performance of ZnO ceramics by interfacial band alignment and self-doping defects. J. Eur. Ceram. Soc. 2023, 43, 1978–1984. [Google Scholar] [CrossRef]
- Karim, A.M.M.T.; Islam, M.R.; Khatun, H.; Khan, M.K.R.; Rahman, M.M.; Shahjahan; Hossain, F.; Arif, E.M.H.; Nazrul-Islam, S.M.K. Ultrahigh Thermoelectric Performance of ZnO-CdO Thin Films. J. Electron. Mater. 2024, 53, 188–195. [Google Scholar] [CrossRef]
- He, Q.; Yang, D.; Xia, S.; Song, H. Ultra-low thermal conductivity and improved thermoelectric performance in La2O3-dispersed Bi2Sr2Co2Oy ceramics. Mater. Sci. Eng.: B 2024, 299, 116976. [Google Scholar] [CrossRef]
- Brlec, K.; Spooner, K.B.; Skelton, J.M.; Scanlon, D.O. Y2TiO5S2–a promising n-type oxysulphide for thermoelectric applications. J. Mater. Chem. A 2022, 10, 16813–16824. [Google Scholar] [CrossRef]
- Pallavi; Singh, C.P.; Kamlesh, P.K.; Gupta, R.; Verma, A.S. Thermoelectric performance of cadmium-based LiCdX (X= N, P, As, Sb and Bi)-filled tetrahedral semiconductors: Applications in green energy resources. Pramana 2023, 97, 162. [Google Scholar] [CrossRef]
- Ye, X.; Feng, Z.; Zhang, Y.; Zhao, G.; Singh, D.J. Low thermal conductivity and high thermoelectric performance via Cd underbonding in half-Heusler PCdNa. Phys. Rev. B 2022, 105, 104309. [Google Scholar] [CrossRef]
- Tan, C.; Wang, H.; Zhao, L.; Sun, Y.; Yao, J.; Zhai, J.; Wang, C.; Wang, H. Large mass field fluctuation and lattice anharmonicity effects promote thermoelectric and mechanical performances in NbFeSb half-Heusler alloys via Ti/Zr/Hf stepwise doping. J. Mater. Chem. A 2023, 11, 19036–19045. [Google Scholar] [CrossRef]
- Min, R.; Wang, Y.; Jiang, X.; Chen, R.; Li, M.; Kang, H.; Yang, X.; Chen, Z.; Guo, E.; Wang, T. ZrNiSn-based compounds with high thermoelectric performance and ultralow lattice thermal conductivity via introduction of multiscale scattering centers. J. Mater. 2024, 10, 200–209. [Google Scholar] [CrossRef]
- Bo, L.; Wang, W.; Zhu, J.; Li, C.; Zuo, M.; Zhao, D. Stepwise Alloying in Liquid-like Solid Solutions to Achieve Crystallographic Distortion for Regulating Thermoelectric Transport Behavior. ACS Appl. Mater. Interfaces 2023, 15, 54478–54487. [Google Scholar] [CrossRef] [PubMed]
- Singh, M.; Gautam, A.K.; Faraz, M.; Khare, N. Flexible, Freestanding Ternary Nanocomposite Thick Film of Polyaniline/WS2/CNTs for Efficient Thermoelectric Application. ACS Appl. Energy Mater. 2023, 7, 133–144. [Google Scholar] [CrossRef]
- Song, Y.; Dai, X.; Zou, Y.; Li, C.; Di, C.A.; Zhang, D.; Zhu, D. Boosting the Thermoelectric Performance of the Doped DPP-EDOT Conjugated Polymer by Incorporating an Ionic Additive. Small 2023, 19, 2300231. [Google Scholar] [CrossRef]
- Sherchenkov, A.; Borgardt, N.; Shtern, M.; Zaytseva, Y.; Shtern, Y.; Rogachev, M.; Sazonov, V.; Yakubov, A.; Pepelyaev, D. The role of nanostructuring strategies in PbTe on enhancing thermoelectric efficiency. Mater. Today Energy 2023, 37, 101416. [Google Scholar] [CrossRef]
- Zhang, M.; Cai, J.; Gao, F.; Zhang, Z.; Li, M.; Chen, Z.; Wang, Y.; Hu, D.; Tan, X.; Liu, G.; et al. Improved Thermoelectric Performance of p-Type PbTe by Entropy Engineering and Temperature-Dependent Precipitates. ACS Appl. Mater. Interfaces 2023, 16, 907–914. [Google Scholar] [CrossRef]
- Zhang, W.; Zhou, Z.; Yang, Y.; Zheng, Y.; Wei, B.; Lan, J.L.; Nan, C.W.; Lin, Y.H. Enhanced thermoelectric performance of n-type PbTe through introducing PbSe by a fast preparation method. Mater. Today Phys. 2023, 38, 101231. [Google Scholar] [CrossRef]
- Huang, Z.Y.; Wang, F.; Jung, C.; Zhang, S.; Zu, F.; Zhou, C.; Yu, Y. Decorated dislocations lead to dynamically optimized thermoelectric performance in N-type PbTe. Mater. Today Phys. 2023, 37, 101198. [Google Scholar] [CrossRef]
- Hu, Z.; Yu, H.; He, J.; Ran, Y.; Zeng, H.; Zhao, Y.; Yu, Z.; Tai, K. High-Performance Sb-Doped GeTe Thermoelectric Thin Film and Device. Acta Metall. Sin. 2023, 36, 1699–1708. [Google Scholar] [CrossRef]
- Jin, Y.; Ren, D.; Qiu, Y.; Zhao, L.D. Electrical and thermal transport properties of Ge1–xPbxCuySbyTeSe2y. Adv. Funct. Mater. 2023, 33, 2304512. [Google Scholar] [CrossRef]
- Zhang, T.; Qi, N.; Su, X.; Tang, X.; Chen, Z. Vacancy Suppression Induced Synergetic Optimization of Thermoelectric Performance in Sb-Doped GeTe Evidenced by Positron Annihilation Spectroscopy. ACS Appl. Mater. Interfaces 2023, 15, 40665–40675. [Google Scholar] [CrossRef] [PubMed]
- Wu, G.; Guo, Z.; Wang, R.; Tan, X.; Cui, C.; Sun, P.; Hu, H.; Wu, J.; Liu, G.; Jiang, J. Structural modulation and resonant level enable high thermoelectric performance of GeTe in the mid-to-low temperature range. J. Mater. Chem. A 2023, 11, 20497–20505. [Google Scholar] [CrossRef]
- Demir, M.E.; Dincer, I. Development and heat transfer analysis of a new heat recovery system with thermoelectric generator. Int. J. Heat Mass Transf. 2017, 108, 2002–2010. [Google Scholar] [CrossRef]
- Quan, R.; Liu, G.; Wang, C.; Zhou, W.; Huang, L.; Deng, Y. Performance investigation of an exhaust thermoelectric generator for military SUV application. Coatings 2018, 8, 45. [Google Scholar] [CrossRef]
- Asaduzzaman, M.; Ali, M.H.; Pratik, N.A.; Lubaba, N. Exhaust heat harvesting of automotive engine using thermoelectric generation technology. Energy Convers. Manag.: X 2023, 19, 100398. [Google Scholar] [CrossRef]
- Hong, T.D.; Nghiem, Q.T.; Mai, T.V.; Le, L.T. A numerical simulation and experimental study on thermal uniformity of heat exchanger in motorcycle thermoelectric generator unit. JP J. Heat Mass Transf. 2021, 22, 89–105. [Google Scholar] [CrossRef]
- Lu, H.; Wu, T.; Bai, S.; Xu, K.; Huang, Y.; Gao, W.; Yin, X.; Chen, L. Experiment on thermal uniformity and pressure drop of exhaust heat exchanger for automotive thermoelectric generator. Energy 2013, 54, 372–377. [Google Scholar] [CrossRef]
- Kumar, R.C.; Sonthalia, A.; Goel, R. Experimental study on waste heat recovery from an IC engine using thermoelectric technology. Therm. Sci. 2011, 15, 1011–1022. [Google Scholar] [CrossRef]
- Luo, D.; Wang, R.; Yu, W.; Sun, Z.; Meng, X. Modelling and simulation study of a converging thermoelectric generator for engine waste heat recovery. Appl. Therm. Eng. 2019, 153, 837–847. [Google Scholar] [CrossRef]
- Luo, D.; Wang, R.; Yu, W.; Zhou, W. A numerical study on the performance of a converging thermoelectric generator system used for waste heat recovery. Appl. Energy 2020, 270, 115181. [Google Scholar] [CrossRef]
- Buonomo, B.; Di Pasqua, A.; Manca, O.; Nardini, S. Numerical investigation on thermoelectric generators in an exhaust automotive line with aluminium foam. J. Phys.: Conf. Ser. 2020, 1599, 012014. [Google Scholar] [CrossRef]
- Li, Y.; Wang, S.; Zhao, Y.; Lu, C. Experimental study on the influence of porous foam metal filled in the core flow region on the performance of thermoelectric generators. Appl. Energy 2017, 207, 634–642. [Google Scholar] [CrossRef]
- Nithyanandam, K.; Mahajan, R.L. Evaluation of metal foam based thermoelectric generators for automobile waste heat recovery. Int. J. Heat Mass Transf. 2018, 122, 877–883. [Google Scholar] [CrossRef]
- Bai, W.; Yuan, X.; Liu, X. Numerical investigation on the performances of automotive thermoelectric generator employing metal foam. Appl. Therm. Eng. 2017, 124, 178–184. [Google Scholar] [CrossRef]
- Lu, C.; Wang, S.; Chen, C.; Li, Y. Effects of heat enhancement for exhaust heat exchanger on the performance of thermoelectric generator. Appl. Therm. Eng. 2015, 89, 270–279. [Google Scholar] [CrossRef]
- Li, Y.; Wang, S.; Zhao, Y.; Yue, L. Experimental study on the effect of core flow heat transfer enhancement on the performance of TEG. Energy Rep. 2022, 8, 575–580. [Google Scholar] [CrossRef]
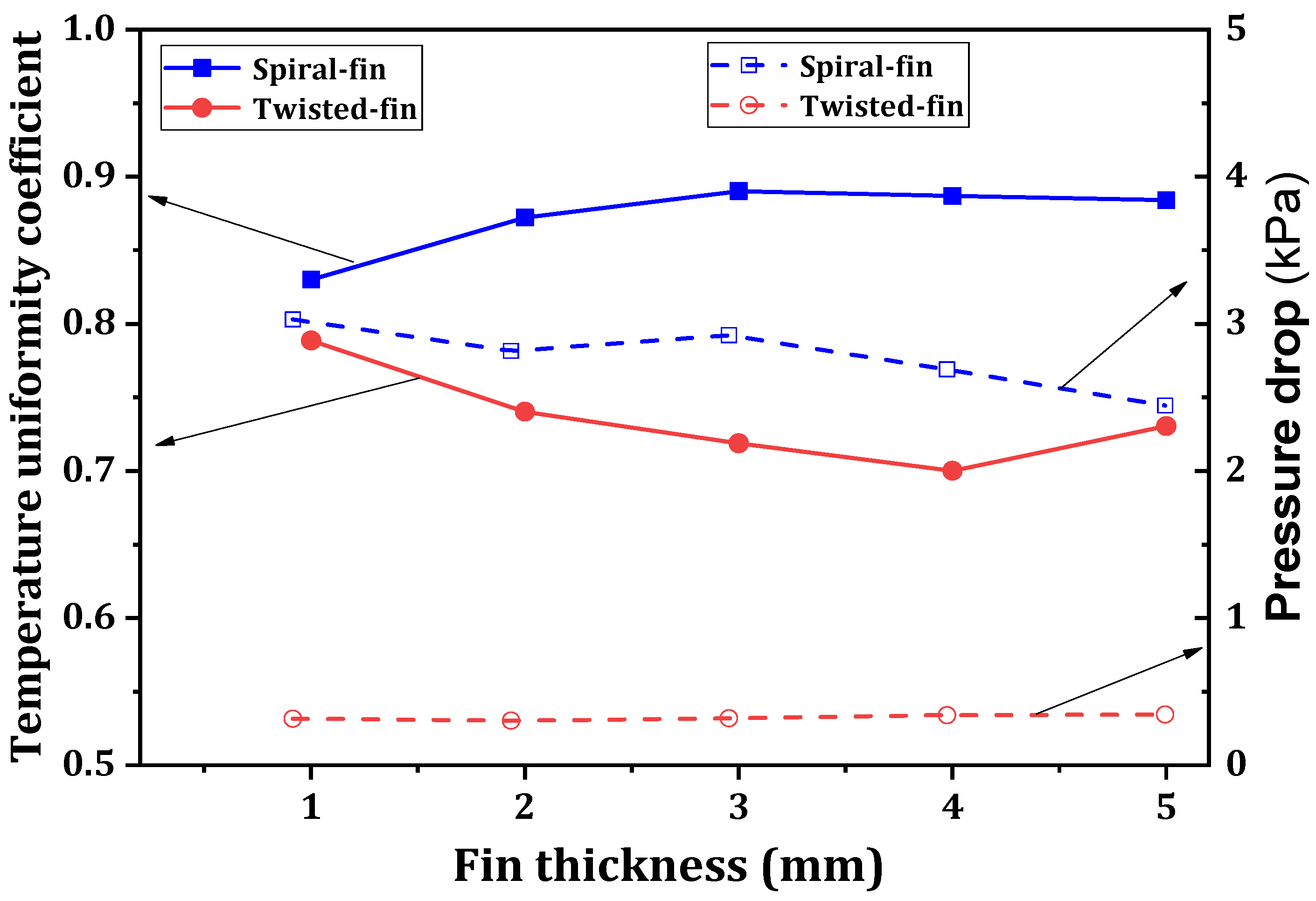
- Wang, Y.; Wu, C.; Tang, Z.; Deng, Y.; Su, C. Optimization of fin distribution to improve the temperature uniformity of a heat exchanger in a thermoelectric generator. J. Electron. Mater. 2015, 44, 1724–1732. [Google Scholar] [CrossRef]
- Tang, Z.B.; Deng, Y.D.; Su, C.Q.; Yuan, X.H. Fluid analysis and improved structure of an ATEG heat exchanger based on computational fluid dynamics. J. Electron. Mater. 2015, 44, 1554–1561. [Google Scholar] [CrossRef]
- Borcuch, M.; Musiał; M.; Gumuła, S.; Sztekler, K.; Wojciechowski, K. Analysis of the fins geometry of a hot-side heat exchanger on the performance parameters of a thermoelectric generation system. Appl. Therm. Eng. 2017, 127, 1355–1363. [Google Scholar] [CrossRef]
- Wang, T.; Ma, S. Thermoelectric generator heat performance study about improved fin structures. Therm. Sci. 2018, 22, 101–112. [Google Scholar] [CrossRef]
- Su, C.Q.; Huang, C.; Deng, Y.D.; Wang, Y.P.; Chu, P.Q.; Zheng, S.J. Simulation and optimization of the heat exchanger for automotive exhaust-based thermoelectric generators. J. Electron. Mater. 2016, 45, 1464–1472. [Google Scholar] [CrossRef]
- Deng, Y.D.; Liu, X.; Chen, S.; Tong, N.Q. Thermal optimization of the heat exchanger in an automotive exhaust-based thermoelectric generator. J. Electron. Mater. 2013, 42, 1634–1640. [Google Scholar] [CrossRef]
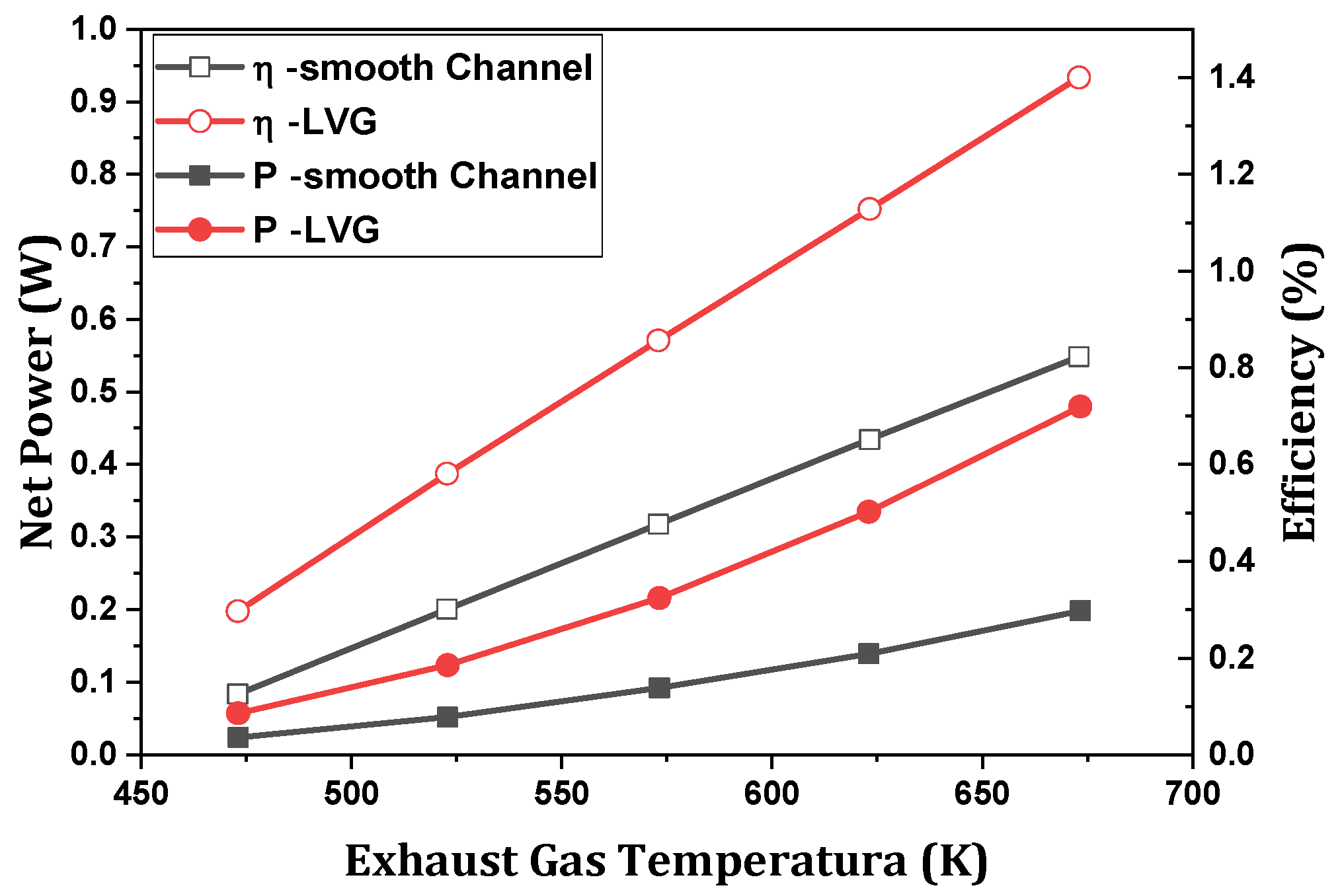
- Lu, X.; Yu, X.; Qu, Z.; Wang, Q.; Ma, T. Experimental investigation on thermoelectric generator with non-uniform hot-side heat exchanger for waste heat recovery. Energy Convers. Manag. 2017, 150, 403–414. [Google Scholar] [CrossRef]
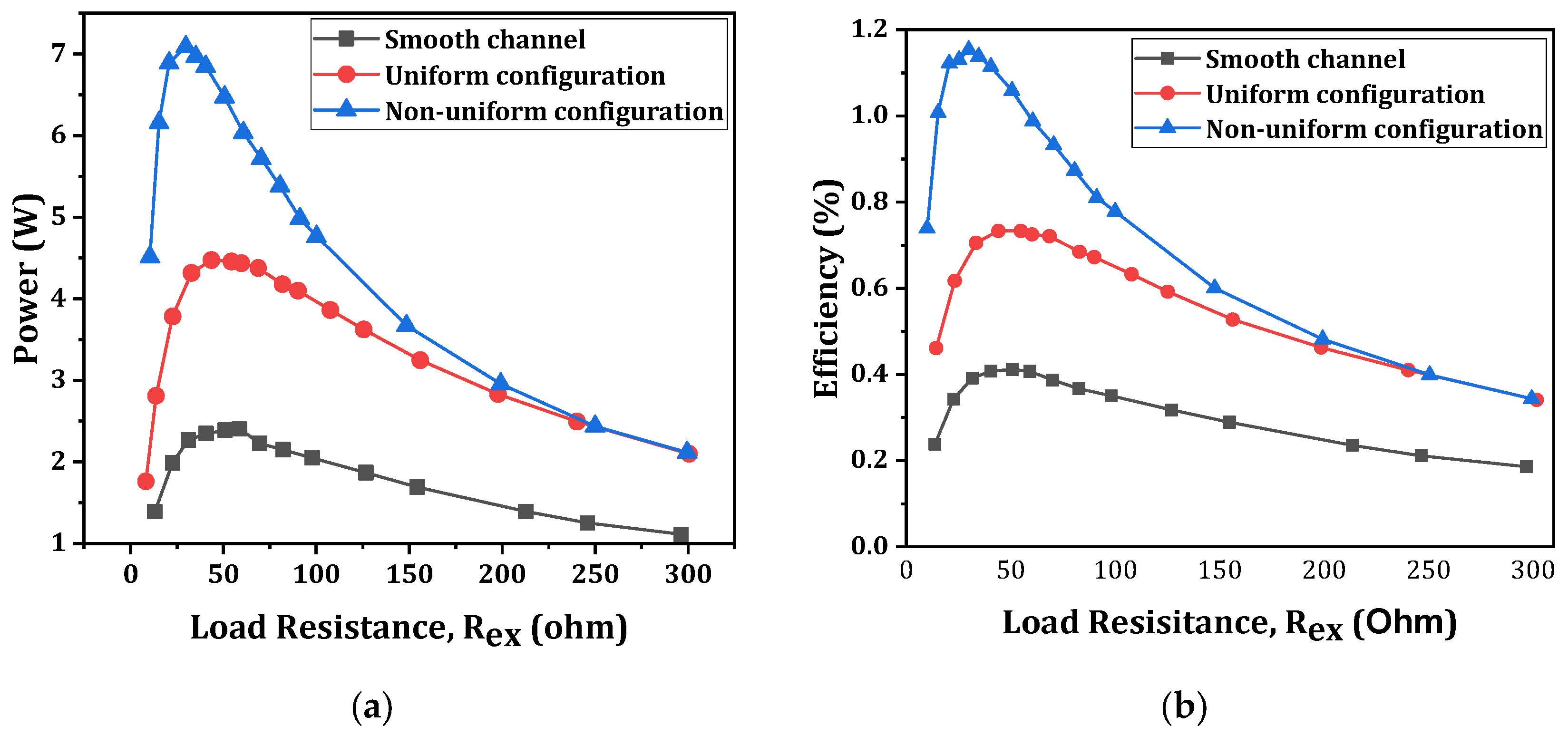
- Ma, T.; Lu, X.; Pandit, J.; Ekkad, S.V.; Huxtable, S.T.; Deshpande, S.; Wang, Q.W. Numerical study on thermoelectric–hydraulic performance of a thermoelectric power generator with a plate-fin heat exchanger with longitudinal vortex generators. Appl. Energy 2017, 185, 1343–1354. [Google Scholar] [CrossRef]
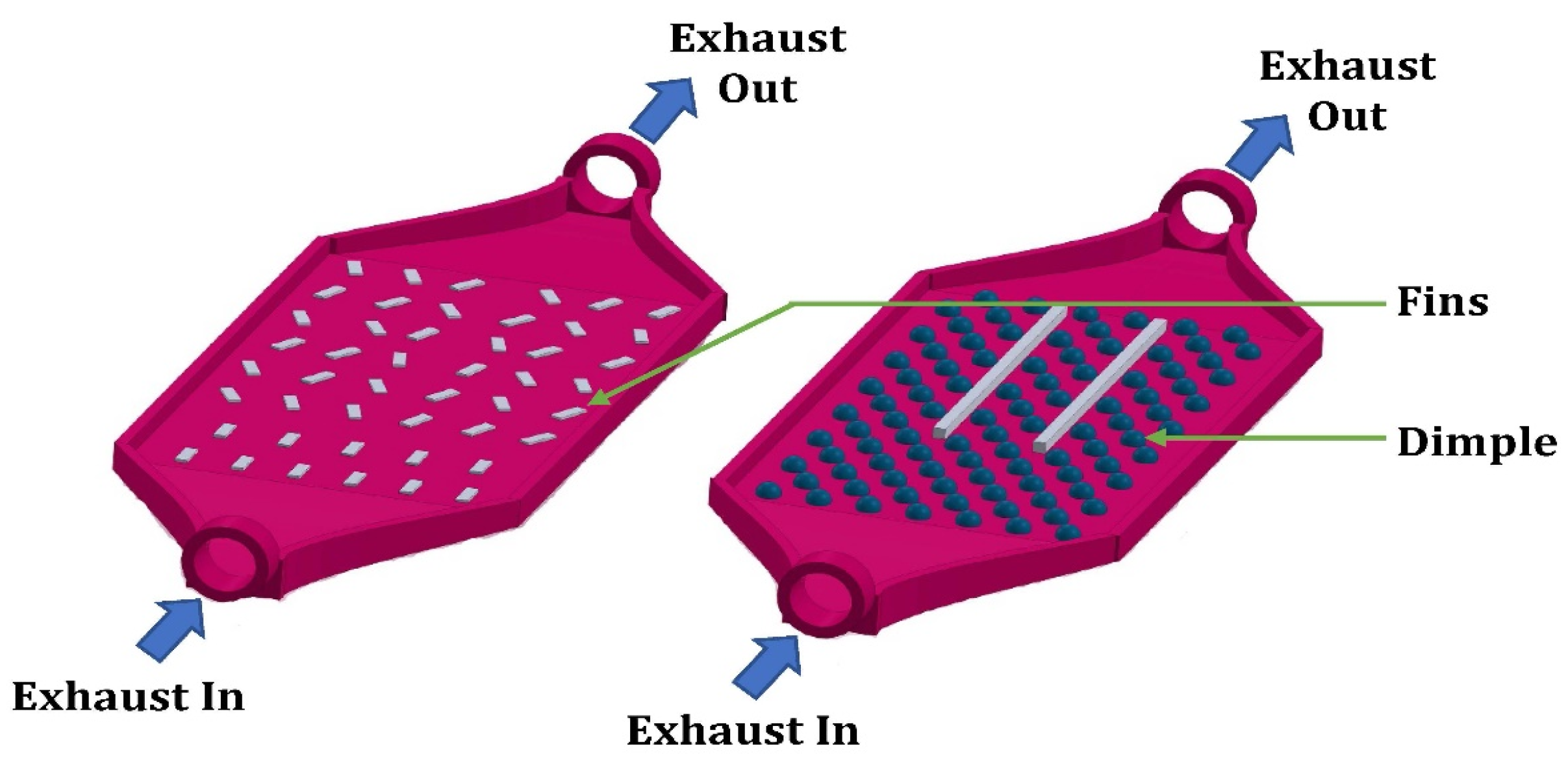
- Wang, Y.; Li, S.; Xie, X.; Deng, Y.; Liu, X.; Su, C. Performance evaluation of an automotive thermoelectric generator with inserted fins or dimpled-surface hot heat exchanger. Appl. Energy 2018, 218, 391–401. [Google Scholar] [CrossRef]
- Wang, Y.; Li, S.; Yang, X.; Deng, Y.; Su, C. Numerical and experimental investigation for heat transfer enhancement by dimpled surface heat exchanger in thermoelectric generator. J. Electron. Mater. 2016, 45, 1792–1802. [Google Scholar] [CrossRef]
- Hong, T.D.; Pham, M.Q.; Nghiem, Q.T.P. Thermal uniformity enhancement of the motorcycle exhaust thermoelectric generator—Theory model for predicting heat exchanger fin profile. Results Eng. 2023, 19, 101324. [Google Scholar] [CrossRef]
- Zhao, Y.; Wang, S.; Ge, M.; Liang, Z.; Liang, Y.; Li, Y. Performance analysis of automobile exhaust thermoelectric generator system with media fluid. Energy Convers. Manag. 2018, 171, 427–437. [Google Scholar] [CrossRef]
- Zhao, Y.; Wang, S.; Ge, M.; Liang, Z.; Liang, Y.; Li, Y. Performance investigation of an intermediate fluid thermoelectric generator for automobile exhaust waste heat recovery. Appl. Energy 2019, 239, 425–433. [Google Scholar] [CrossRef]
- Zhao, Y.; Fan, Y.; Ge, M.; Xie, L.; Li, Z.; Yan, X.; Wang, S. Thermoelectric performance of an exhaust waste heat recovery system based on intermediate fluid under different cooling methods. Case Stud. Therm. Eng. 2021, 23, 100811. [Google Scholar] [CrossRef]
- Ge, M.; Li, Z.; Zhao, Y.; Xie, L.; Wang, S. Effect of exhaust parameters on performance of intermediate fluid thermoelectric generator. Case Stud. Therm. Eng. 2021, 28, 101480. [Google Scholar] [CrossRef]
- Zhang, W.; Li, W.; Li, S.; Xie, L.; Ge, M.; Zhao, Y. Optimization design of an intermediate fluid thermoelectric generator for exhaust waste heat recovery. Processes 2023, 11, 1853. [Google Scholar] [CrossRef]
- Jang, J.-C.; Chi, R.-G.; Rhi, S.-H.; Lee, K.-B.; Hwang, H.-C.; Lee, J.-S.; Lee, W.-H. Heat pipe-assisted thermoelectric power generation technology for waste heat recovery. J. Electron. Mater. 2015, 44, 2039–2047. [Google Scholar] [CrossRef]
- Remeli, M.F.; Tan, L.; Date, A.; Singh, B.; Akbarzadeh, A. Simultaneous power generation and heat recovery using a heat pipe assisted thermoelectric generator system. Energy Convers. Manag. 2015, 91, 110–119. [Google Scholar] [CrossRef]
- Tang, S.; Wang, C.; Liu, X.; Su, G.; Tian, W.; Qiu, S.; Zhang, Q.; Liu, R.; Bai, S. Experimental investigation of a novel heat pipe thermoelectric generator for waste heat recovery and electricity generation. Int. J. Energy Res. 2020, 44, 7450–7463. [Google Scholar] [CrossRef]
- Liu, T.; Wang, T.; Luan, W.; Cao, Q. Optimal number of thermoelectric couples in a heat pipe assisted thermoelectric generator for waste heat recovery. J. Electron. Mater. 2017, 46, 3137–3144. [Google Scholar] [CrossRef]
- Remeli, M.F.; Singh, B.; Affandi, N.D.N.; Ding, L.C.; Date, A.; Akbarzadeh, A. investigation of counter-flow in a heat pipe–thermoelectric generator (HPTEG). J. Electron. Mater. 2017, 46, 3115–3123. [Google Scholar] [CrossRef]
- Aranguren, P.; Astrain, D.; Rodríguez, A.; Martínez, A. Experimental investigation of the applicability of a thermoelectric generator to recover waste heat from a combustion chamber. Appl. Energy 2015, 152, 121–130. [Google Scholar] [CrossRef]
- Kim, P.-J.; Rhi, S.-H.; Lee, K.-B.; Hwang, H.-C.; Lee, J.-S.; Jang, J.-C.; Lee, W.-H.; Lee, K.-W. Heat-pipe-associated localized thermoelectric power generation system. J. Electron. Mater. 2014, 43, 1613–1619. [Google Scholar] [CrossRef]
- Chi, R.-G.; Park, J.-C.; Rhi, S.-H.; Lee, K.-B. Study on heat pipe assisted thermoelectric power generation system from exhaust gas. Heat Mass Transf. 2017, 53, 3295–3304. [Google Scholar] [CrossRef]
- Orr, B.; Akbarzadeh, A.; Lappas, P. An exhaust heat recovery system utilising thermoelectric generators and heat pipes. Appl. Therm. Eng. 2017, 126, 1185–1190. [Google Scholar] [CrossRef]
- Li, T.; Fu, Y.; Dong, C.; Li, D.; Gu, H.; Ye, Y. Factors influencing the thermoelectric characteristics of a thermoelectric generator with cold-side micro heat pipe arrays. J. Power Sources 2023, 557, 232493. [Google Scholar] [CrossRef]
- Brito, F.P.; Martins, J.; Hançer, E.; Antunes, N.; Gonçalves, L.M. Thermoelectric exhaust heat recovery with heat pipe-based thermal control. J. Electron. Mater. 2015, 44, 1984–1997. [Google Scholar] [CrossRef]
- Yang, W.; Jin, C.; Zhu, W.; Li, Y.; Zhang, R.; Huang, L.; Xie, C.; Shi, Y. Taguchi optimization and thermoelectrical analysis of a pin fin annular thermoelectric generator for automotive waste heat recovery. Renew. Energy 2024, 220, 119628. [Google Scholar] [CrossRef]
- Du, K.W.; Wu, C.I. An Innovative Tubular Thermoelectric Generator (TTEG) for Enhanced Waste Heat Recovery in Industrial and Automotive Applications. Appl. Sci. 2024, 14, 685. [Google Scholar] [CrossRef]
- Weng, Z.; Liu, F.; Zhu, W.; Li, Y.; Xie, C.; Deng, J.; Huang, L. Performance improvement of variable-angle annular thermoelectric generators considering different boundary conditions. Appl. Energy 2022, 306, 118005. [Google Scholar] [CrossRef]
- Yang, W.; Zhu, W.; Li, Y.; Zhang, L.; Zhao, B.; Xie, C.; Yan, Y.; Huang, L. Annular thermoelectric generator performance optimization analysis based on concentric annular heat exchanger. Energy 2022, 239, 122127. [Google Scholar] [CrossRef]
- Aljaghtham, M.; Celik, E. Energy conversion and thermal reliability of thermoelectric materials in unileg annular configuration. Mater. Lett. 2021, 300, 130192. [Google Scholar] [CrossRef]
- Yang, W.; Zhu, W.; Du, B.; Wang, H.; Xu, L.; Xie, C.; Shi, Y. Power generation of annular thermoelectric generator with silicone polymer thermal conductive oil applied in automotive waste heat recovery. Energy 2023, 282, 128400. [Google Scholar] [CrossRef]
- Neska, M.; Mrozek, M.; Żurek-Mortka, M.; Majcher, A. Analysis of the Parameters of the Two-Sections Hot Side Heat Exchanger of the Module with Thermoelectric Generators. Energies 2021, 14, 5169. [Google Scholar] [CrossRef]
- Buonomo, B.; Cascetta, F.; di Pasqua, A.; Manca, O. Performance parameters enhancement of a thermoelectric generator by metal foam in exhaust automotive lines. Therm. Sci. Eng. Prog. 2023, 38, 101684. [Google Scholar] [CrossRef]
- Chen, W.H.; Chiou, Y.B.; Chein, R.Y.; Uan, J.Y.; Wang, X.D. Power generation of thermoelectric generator with plate fins for recovering low-temperature waste heat. Appl. Energy 2022, 306, 118012. [Google Scholar] [CrossRef]
- Luo, D.; Wu, Z.; Yan, Y.; Ji, D.; Cheng, Z.; Wang, R.; Li, Y.; Yang, X. Optimal design of a heat exchanger for automotive thermoelectric generator systems applied to a passenger car. Appl. Therm. Eng. 2023, 227, 120360. [Google Scholar] [CrossRef]
- Luo, D.; Yan, Y.; Li, Y.; Yang, X.; Chen, H. Exhaust channel optimization of the automobile thermoelectric generator to produce the highest net power. Energy 2023, 281, 128319. [Google Scholar] [CrossRef]
- Srivastava, K.; Sahoo, R.R. Effect of pitch and angle of attack on thermal performance of new envelope and delta vortex generators for TEG: An experimental and numerical approach. Int. J. Therm. Sci. 2024, 195, 108671. [Google Scholar] [CrossRef]
- Selvam, C.; Manikandan, S.; Krishna, N.V.; Lamba, R.; Kaushik, S.C.; Mahian, O. Enhanced thermal performance of a thermoelectric generator with phase change materials. Int. Commun. Heat Mass Transf. 2020, 114, 104561. [Google Scholar] [CrossRef]
- Tian, Y.; Liu, A.; Wang, J.; Zhou, Y.; Bao, C.; Xie, H.; Wu, Z.; Wang, Y. Optimized output electricity of thermoelectric generators by matching phase change material and thermoelectric material for intermittent heat sources. Energy 2021, 233, 121113. [Google Scholar] [CrossRef]
- Shi, Z.; Wang, G.; Liu, C.; Lv, Q.; Gong, B.; Zhang, Y.; Yan, Y. Optimizing the Transient Performance of Thermoelectric Generator with PCM by Taguchi Method. Energies 2023, 16, 805. [Google Scholar] [CrossRef]
- He, D.; Ou, D.; Gao, H.; Jiao, F. Performance evaluation of a thermoelectric generator-coupled composite phase change material for intermittent aerodynamic heat sources. Int. J. Energy Res. 2022, 46, 2698–2708. [Google Scholar] [CrossRef]
- Wang, P.; Wang, B.; Wang, K.; Gao, R.; Xi, L. An analytical model for performance prediction and optimization of thermoelectric generators with varied leg cross-sections. Int. J. Heat Mass Transf. 2021, 174, 121292. [Google Scholar] [CrossRef]
- Luo, Y.; Li, L.; Chen, Y.; Kim, C.N. Influence of geometric parameter and contact resistances on the thermal-electric behavior of a segmented TEG. Energy 2022, 254, 124487. [Google Scholar] [CrossRef]
- Siddique, A.R.M.; Kratz, F.; Mahmud, S.; Heyst, B.V. Energy conversion by nanomaterial-based trapezoidal-shaped leg of thermoelectric generator considering convection heat transfer effect. J. Energy Resour. Technol. 2019, 141, 082001. [Google Scholar] [CrossRef]
- Doraghi, Q.; Khordehgah, N.; Żabnieńska-Góra, A.; Ahmad, L.; Norman, L.; Ahmad, D.; Jouhara, H. Investigation and computational modelling of variable teg leg geometries. ChemEngineering 2021, 5, 45. [Google Scholar] [CrossRef]
- Khalil, A.; Elhassnaoui, A.; Yadir, S.; Abdellatif, O.; Errami, Y.; Sahnoun, S. Performance comparison of TEGs for diverse variable leg geometry with the same leg volume. Energy 2021, 224, 119967. [Google Scholar] [CrossRef]
- Ibeagwu, I.O. Modelling and comprehensive analysis of TEGs with diverse variable leg geometry. Energy 2019, 180, 90–106. [Google Scholar] [CrossRef]
- Liu, J.; Sun, Y.; Chen, G.; Zhai, P. Performance analysis of variable cross-section TEGs under constant heat flux conditions. Energies 2023, 16, 4473. [Google Scholar] [CrossRef]
- Ge, Y.; He, K.; Xiao, L.; Yuan, W.; Huang, S.M. Geometric optimization for the thermoelectric generator with variable cross-section legs by coupling finite element method and optimization algorithm. Renew. Energy 2022, 183, 294–303. [Google Scholar] [CrossRef]
- Shu, G.; Ma, X.; Tian, H.; Yang, H.; Chen, T.; Li, X. Configuration optimization of the segmented modules in an exhaust-based thermoelectric generator for engine waste heat recovery. Energy 2018, 160, 612–624. [Google Scholar] [CrossRef]
- Zhao, J.; Xu, W.; Kuang, Z.; Long, R.; Liu, Z.; Liu, W. Segmental material design in thermoelectric devices to boost heat-to-electricity performance. Energy Convers. Manag. 2021, 247, 114754. [Google Scholar] [CrossRef]
- Liu, H.-B.; Meng, J.-H.; Wang, X.-D.; Chen, W.-H. A new design of solar thermoelectric generator with combination of segmented materials and asymmetrical legs. Energy Convers. Manag. 2018, 175, 11–20. [Google Scholar] [CrossRef]
- Sharma, S.L.; Debbarma, A. Effect of p-leg material and resistance ratio on a segmented thermoelectric generator. Mater. Today: Proc. 2022, 66, 1023–1030. [Google Scholar] [CrossRef]
- Karana, D.R.; Sahoo, R.R. Influence of geometric parameter on the performance of a new asymmetrical and segmented thermoelectric generator. Energy 2019, 179, 90–99. [Google Scholar] [CrossRef]
- Ramos-Castañeda, C.F.; Olivares-Robles, M.A.; Olivares-Hernandez, A.E.; Hernandez-Gonzalez, L. Performance of a nanofluid-cooled segmented thermoelectric generator: Hollow/filled leg structures and segmentation effects. Processes 2023, 11, 1728. [Google Scholar] [CrossRef]
- Zhang, Y.-Q.; Sun, J.; Wang, G.-X.; Wang, T.-H. Advantage of a thermoelectric generator with hybridization of segmented materials and irregularly variable cross-section design. Energies 2022, 15, 2944. [Google Scholar] [CrossRef]
- Ge, Y.; Lin, Y.; He, Q.; Wang, W.; Chen, J.; Huang, S.M. Geometric optimization of segmented thermoelectric generators for waste heat recovery systems using genetic algorithm. Energy 2021, 233, 121220. [Google Scholar] [CrossRef]
- Zhu, L.; Li, H.; Chen, S.; Tian, X.; Kang, X.; Jiang, X.; Qiu, S. Optimization analysis of a segmented thermoelectric generator based on genetic algorithm. Renew. Energy 2020, 156, 710–718. [Google Scholar] [CrossRef]
- Ge, Y.; Liu, Z.; Sun, S.; Liu, W. Optimal design of a segmented thermoelectric generator based on three-dimensional numerical simulation and multi-objective genetic algorithm. Energy 2018, 147, 1060–1069. [Google Scholar] [CrossRef]
- Yin, T.; Li, Z.-M.; Peng, P.; Liu, W.; Shao, Y.-Y.; He, Z.-Z. Performance analysis of a novel two-stage automobile thermoelectric generator with the temperature-dependent materials. Appl. Therm. Eng. 2021, 195, 117249. [Google Scholar] [CrossRef]
- Maduabuchi, C.; Singh, S.; Ozoegwu, C.; Njoku, H.; Eke, M. The combined impacts of leg geometry configuration and multi-staging on the exergetic performance of thermoelectric modules in a solar thermoelectric generator. J. Energy Resour. Technol. 2022, 144, 041303. [Google Scholar] [CrossRef]
- Kanimba, E.; Pearson, M.; Sharp, J.; Stokes, D.; Priya, S.; Tian, Z. A modeling comparison between a two-stage and three-stage cascaded thermoelectric generator. J. Power Sources 2017, 365, 266–272. [Google Scholar] [CrossRef]
- Aljaghtham, M.; Celik, E. Design of cascade thermoelectric generation systems with improved thermal reliability. Energy 2022, 243, 123032. [Google Scholar] [CrossRef]
- Yang, H.; Li, M.; Wang, Z.; Ren, F.; Yang, Y.; Ma, B.; Zhu, Y. Performance optimization for a novel two-stage thermoelectric generator with different PCMs embedding modes. Energy 2023, 281, 128307. [Google Scholar] [CrossRef]
- Zhao, Z.; Zuo, Z.; Wang, W.; Liu, R.; Kuang, N. Performance optimization for a combustion-based micro thermoelectric generator with two-stage thermoelectric module. Appl. Therm. Eng. 2021, 198, 117464. [Google Scholar] [CrossRef]
- Yin, T.; Li, W.-T.; Li, K.; He, Z.-Z. Multi-parameter optimization and uncertainty analysis of multi-stage thermoelectric generator with temperature-dependent materials. Energy Rep. 2021, 7, 7212–7223. [Google Scholar] [CrossRef]
- Zhao, Y.; Li, W.; Zhao, X.; Wang, Y.; Luo, D.; Li, Y.; Ge, M. Energy and exergy analysis of a thermoelectric generator system for automotive exhaust waste heat recovery. Appl. Therm. Eng. 2024, 239, 122180. [Google Scholar] [CrossRef]
- Yin, T.; Ren, D.; Ma, X.; Wei, Y.; Gao, Q.; Han, X. Optimal Design and Multi-Parameter Sensitivity Analysis of a Segmented Thermoelectric Generator. Processes 2023, 11, 3352. [Google Scholar] [CrossRef]
- Wang, X.; Qi, J.; Deng, W.; Li, G.; Gao, X.; He, L.; Zhang, S. An optimized design approach concerning thermoelectric generators with frustum-shaped legs based on three-dimensional multiphysics model. Energy 2021, 233, 120810. [Google Scholar] [CrossRef]
- Cheng, K.; Qin, J.; Jiang, Y.; Zhang, S.; Bao, W. Performance comparison of single-and multi-stage onboard thermoelectric generators and stage number optimization at a large temperature difference. Appl. Therm. Eng. 2018, 141, 456–466. [Google Scholar] [CrossRef]
- Cheng, K.; Qin, J.; Jiang, Y.; Lv, C.; Zhang, S.; Bao, W. Performance assessment of multi-stage thermoelectric generators on hypersonic vehicles at a large temperature difference. Appl. Therm. Eng. 2018, 130, 1598–1609. [Google Scholar] [CrossRef]
- Luo, D.; Wang, R.; Yan, Y.; Yu, W.; Zhou, W. Transient numerical modelling of a thermoelectric generator system used for automotive exhaust waste heat recovery. Appl. Energy 2021, 297, 117151. [Google Scholar] [CrossRef]
- Luo, D.; Yan, Y.; Li, Y.; Chen, W.-H.; Yang, X.; Wang, X.; Cao, B. Dynamic behaviour of automobile thermoelectric waste heat recovery under different driving cycles. Appl. Therm. Eng. 2023, 232, 121039. [Google Scholar] [CrossRef]
- Lan, S.; Yang, Z.; Chen, R.; Stobart, R. A dynamic model for thermoelectric generator applied to vehicle waste heat recovery. Appl. Energy 2018, 210, 327–338. [Google Scholar] [CrossRef]









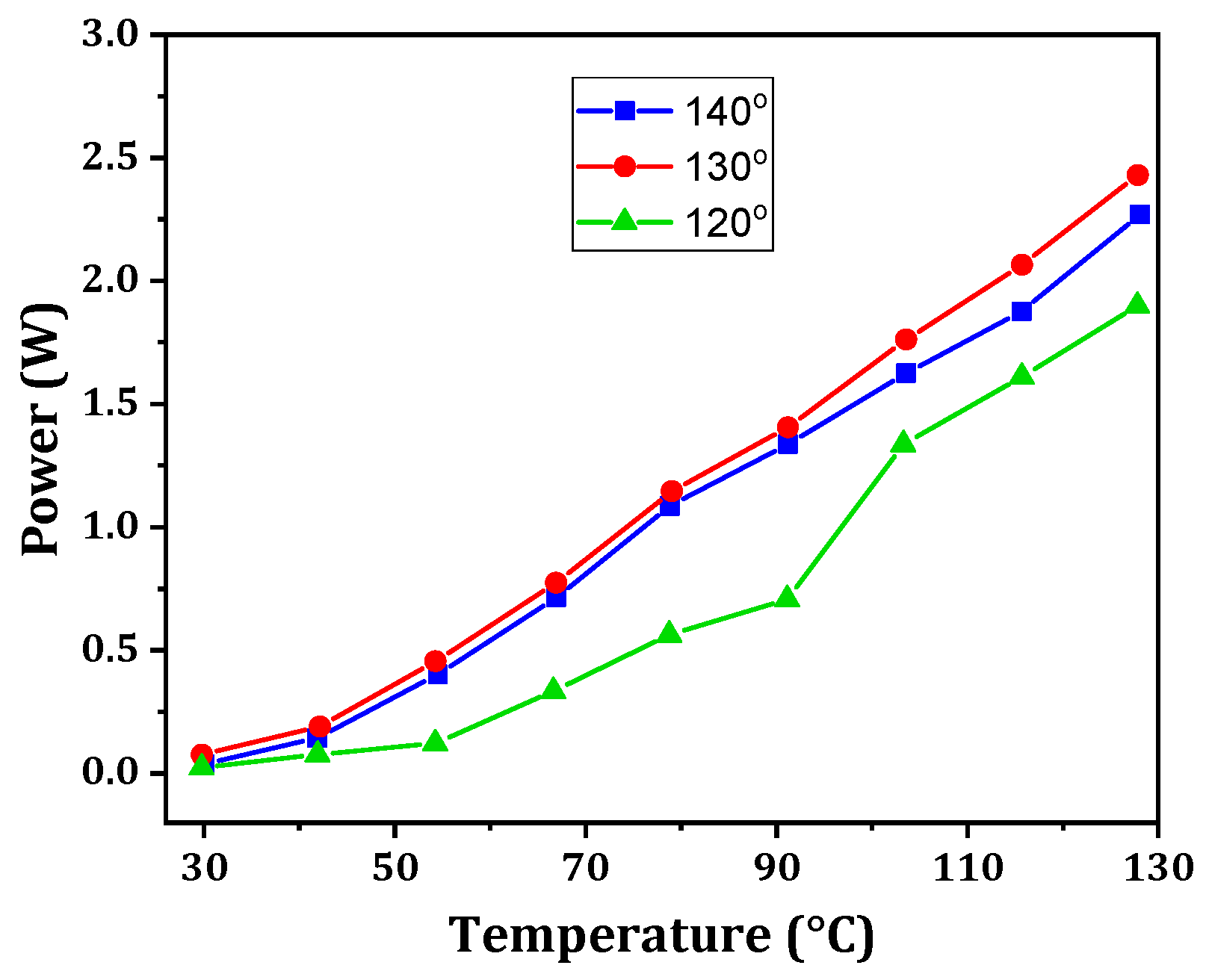

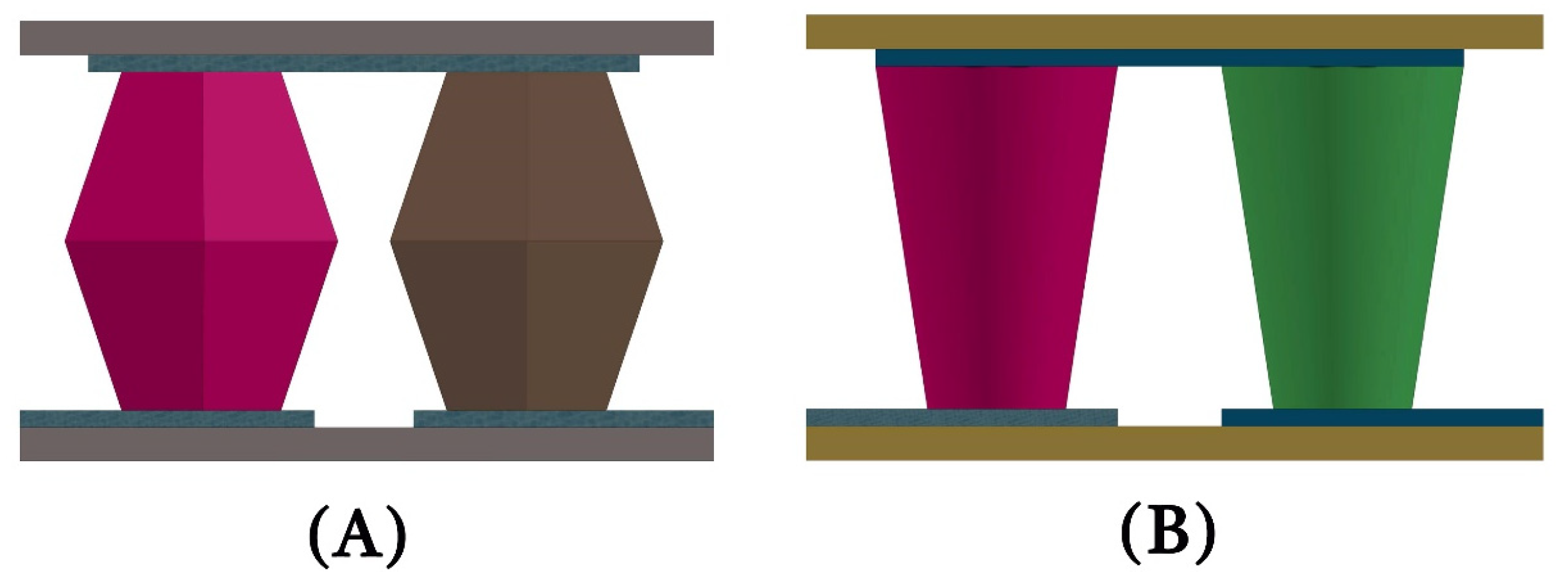




| Author | Year | Base Material | Material | Technique | Max ZT | Temp. |
|---|---|---|---|---|---|---|
| Chueachot and Nakhowong [80] | 2023 | BiTe | rGO/Bi0.5Sb1.5Te3-0.3% Cu2Se0.98Te0.02 | Solid-State Technique | 1.64 | 448 K |
| Ioannou et al. [81] | 2024 | BiTe | Bi0.3Sb1.7Te3 | M, MA, BM, HP | 1.13 | 350 K |
| Kumar et al. [82] | 2024 | BiTe | 0.90 wt% Ag-TiO2/BST | Spray Coating | 1.28 | 350 K |
| Sun et al. [83] | 2023 | BiTe | Bi0.4Sb1.6Te3 + 0.01 MnO2 | BM and SPS | 1.43 | 75 °C |
| Pang et al. [84] | - | BiTe | Bi0.5Sb1.5Te2.94Se0.06 + 0.11 wt.% Cu2GeSe3 | BM and SPS | 1.41 | 350 K |
| Li et al. [85] | 2023 | SnSe | Sn0.9Pb0.07Ce0.03Se0.93Te0.07 | BM, SPS | 1.3 | 823 K |
| Howells et al. [86] | 2023 | SnSe | SnSe + 1.5% Na2SiO3 | BM, Pseudo-3D Printing | 0.751 | 823 K |
| Wang et al. [87] | 2024 | SnSe | Sn0.97Ag0.03Se | Hydrothermal Method and HP | 1.14 | 773 K |
| Ma et al. [88] | 2024 | SnSe | Pnma phase SnSe | First Principles Calculated | 1.2 | Room Temp. |
| Wang et al. [89] | 2023 | SnSe | Pnma phase PbSnSe2 | DFT and Boltzmann Equation | 3 | 700 K |
| Wang et al. [90] | 2024 | SnSe | (Sn0.9925Ge0.0075Se)0.985 (-AgInSe2)0.0015 | M, SPS | 1.6 | 773 K |
| Liu et al. [91] | 2023 | SnSe | SnCu0.001Se | Two-Step Modified Bridgman Method | 2.2 | 300–773 K |
| Yang et al. [92] | 2023 | SnSe | Sn0⋅98Ag0.01Ga0.01Se | M, SPS | 1.53 | 823 K |
| Cao et al. [93] | 2023 | SnSe | Pnma phase SnSe | DFT | 1.64 | 300 K |
| Zhao et al. [94] | 2021 | CuSe | Cu1.99In0.01Se | M and SPS | 1.44 | 873 K |
| Tie et al. [95] | 2023 | CuSe | Cu2Se | Vacuum Melting, Annealing, and SPS | 1.34. | 873 K |
| Yu et al. [96] | 2024 | CuSe | Cu1.99Se + 0.9 vol.% B4C | MA and SPS | 2.6 | 1025 K |
| Li et al. [97] | 2023 | CuSe | β-Cu2+xSe/a-C nano-multi-layer | Magnetron Sputtering | 0.42 | Room Temp. |
| Long et al. [98] | 2023 | CuSe | Cu2Se/5 wt% Cu2Se0.88S0.06Te0.06 | HP and Sintering | 2.04 | 850 K |
| Zhou et al. [99] | 2023 | CuSe | Cu2Se-BiCuSeO–graphene composites | SHS and SPS | 2.82 | 1000 K |
| Piyasin et al. [100] | 2024 | CuSe | Cu2Se + 3 wt% thiol-amine | CSP | 2.13 | 800 K |
| Rudradawong et al. [101] | 2023 | CuSe | Cu2 Se0.995 Sb0.005 | Solid-State Reaction | 0.47 | 523 K |
| Wang et al. [102] | 2023 | Oxide | Bi0.98Pb0.02Cu0.98Al0.02SeO | BM and SPS | 1.14 | 873 K |
| Zhou et al. [103] | 2023 | Multi-Component Oxides | CuI-doped Bi2O2Se | MA, SPS, SSR | 0.45 | 823 K |
| Du et al. [104] | 2023 | Multi-Component Oxides | 0.95 ZnO/0.05 ZnS | Hydrothermal Synthesis and Ion Exchange Reaction | 0.94 | 900 K |
| Karim at al. [105] | 2024 | Multi-Component Oxides | ZnO-CdO thin film | Spray Pyrolysis | 0.59 | 413 K |
| He et al. [106] | 2024 | Multi-Component Oxides | Bi2Sr2Co2Oy + 0.25wt% La2O3 | SSR | 0.28 | 923 K |
| Brlec et al. [107] | 2022 | Multi-Component Oxides | Y2Ti2O5S2 | DFT | 1.18 | 1000 K |
| Pallavi et al. [108] | 2023 | Half-Heusler | LiCdP | DFT | 1.0 | 300 K |
| Ye et al. [109] | 2022 | Half-Heusler | PCdNa | DFT | 3.3 | 900 K |
| Tan et al. [110] | 2023 | Half-Heusler | Nb0.82Ti0.06Zr0.06Hf0.06FeSb | M, SPS | 0.74 | 973 K |
| Min et al. [111] | 2024 | Half-Heusler | Zr0.66Hf0.30Nb0.04NiSn | LM, SPS | 1.06 | 1123 K |
| Bo et al. [112] | 2023 | Half-Heusler | Cu1.9Se—1 wt % (SnSe)0.75(AgBiSe2)0.25. | Cold Press, RPH | 1.23 | 750 |
| Singh et al. [113] | 2023 | Organic–Inorganic | PANI/WS2/CNT | Drop-Cast Method, Chemical Vapor Deposition | 0.17 | 323 K |
| Song et al. [114] | 2023 | Organic–Inorganic | PDPP-EDOT thin film | Drop Casting | 0.28 | 130 °C |
| Sherchenkov et al. [115] | 2023 | PbTe | PbTe + 0.2 wt% PbI2 + 0.3 wt% Ni | BM and SPS | 1.35 | 850 K |
| Zhang et al. [116] | 2023 | PbTe | Pb0.9Na0.04Mn0.06Te0.9S0.05Se0.05 | BM, HPS | 2.1 | 825 K |
| Zhang et al. [117] | 2023 | PbTe | Pb0.995Bi0.005Te | SHS, SPS | 0.57 | 300–623 K |
| Huang et al. [118] | 2023 | PbTe | Sb and AgTe2-doped PbTe | SPS | 1.5 | 750 K |
| Hu et al. [119] | 2023 | GeTe | Ge0.907Sb0.093Te | MS | 1.53 | 703 K |
| Jin et al. [120] | 2023 | GeTe | Ge0.925Pb0.075Cu0.005Sb0.005TeSe0.01 | M, SPS | 2.2 | 723 K |
| Zhang et al. [121] | 2023 | GeTe | Ge0.94Sb0.06Te | BM, MA, SPS | 2.2 | 723 K |
| Wu et al. [122] | 2023 | GeTe | (Ge0.91Sb0.09Te)0.99(InSe)0.01 | M, Annealing, HP | 2.1 | 773 K |
| Author | Year | Heat Exchanger Type | Notable Results | Increase in Performance Compared with the Primary Heat Exchanger |
|---|---|---|---|---|
| Neska et al. [170] | 2021 | With fluid circulation channel | 28 W maximum power and 0.93% maximum efficiency | Power output increases by 14%, efficiency increases by 16% |
| Buonomo et al. [171] | 2023 | With metal foam | 13.4% maximum efficiency with filling ratio equal to one | Maximum efficiency is 3.6% with no metal foam |
| Chen et al. [172] | 2022 | With plate fins | 0.411 W maximum power and 0.95% maximum efficiency | 105.5% improvement in power and 43.94% improvement in efficiency over a case with no fin |
| Luo et al. [173] | 2023 | With plate fins | 35.94 W net power and 1.22% net efficiency | Net power is 16.49 W, and net efficiency is 0.79% for a system with no fin |
| Luo et al. [174] | 2023 | With plate fins | 45.02 W maximum net power and 1.5% maximum net conversion efficiency | 9.52% increase in net power and 22.95% increase in net efficiency compared with a case without fin optimization |
| Srivastava and Sahoo [175] | 2024 | With vortex generator | 2 W maximum power | Maximum power increased by 267.24% against a case with no vortex generator |
| Selvam et al. [176] | 2020 | With PCM | 4.5% maximum efficiency | 36.7% improvement from no PCM |
| Tian et al. [177] | 2021 | With PCM | - | Optimization enhanced the power output by 15.6% |
| Shi et al. [178] | 2023 | With PCM | 1.472% overall conversion efficiency | |
| He et al. [179] | 2022 | With EG/paraffin composite PCM | 0.764 W maximum power with 10 wt% EG | 30.8% more than the system with only paraffin |
| Author | Year | TEM Design | Major Results | Remarks |
|---|---|---|---|---|
| Zhao et al. [205] | 2024 | Variable cross-section leg geometry | Cross-vertical leg geometry generated a power output of 0.0025 W/mm3 | Conventional leg produced a power output between 0.0012 and 0.016 W/mm3 |
| Yin et al. [206] | 2023 | Segmented leg geometry | 24.63 mW maximum power based on the optimization analysis | Maximum power 22.18 mW without optimization |
| Zhang et al. [194] | Segmented and variable cross-section leg geometry | 59.91 mW power developed for segmented and variable designs | 39.49 mW power produced from conventional design | |
| Wang et al. [207] | 2021 | Variable leg cross-section area | For 241 pairs of legs: 9.65 W power output and 3.83% efficiency. For 97 pairs: 15.58 W power output and 3.26% efficiency | With the increase in number of pairs, the power increases and conversion efficiency declines |
| Cheng et al. [208] | 2018 | Multi-stage | 4912.2 W/m2 maximum power density and 12.67% efficiency for two-stage TEG | 2743.3 W/m2 maximum power density and 6.45% efficiency for single-stage TEG |
| Cheng et al. [209] | 2018 | Multi-stage | Maximum conversion efficiency of 18.38%.for four-stage TEG system | - |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bhakta, S.; Kundu, B. A Review of Thermoelectric Generators in Automobile Waste Heat Recovery Systems for Improving Energy Utilization. Energies 2024, 17, 1016. https://doi.org/10.3390/en17051016
Bhakta S, Kundu B. A Review of Thermoelectric Generators in Automobile Waste Heat Recovery Systems for Improving Energy Utilization. Energies. 2024; 17(5):1016. https://doi.org/10.3390/en17051016
Chicago/Turabian StyleBhakta, Sourav, and Balaram Kundu. 2024. "A Review of Thermoelectric Generators in Automobile Waste Heat Recovery Systems for Improving Energy Utilization" Energies 17, no. 5: 1016. https://doi.org/10.3390/en17051016
APA StyleBhakta, S., & Kundu, B. (2024). A Review of Thermoelectric Generators in Automobile Waste Heat Recovery Systems for Improving Energy Utilization. Energies, 17(5), 1016. https://doi.org/10.3390/en17051016







