Author Contributions
Conceptualization, M.C. and J.-P.W.; methodology, M.C.; software, M.C.; validation, M.C., Z.S. and J.-P.W.; formal analysis, M.C. and Z.S.; investigation, M.C. and Z.S.; resources, M.C. and J.-P.W.; data curation, M.C. and Z.S.; writing—original draft preparation, M.C.; writing—review and editing, Z.S. and J.-P.W.; visualization, M.C. and Z.S.; supervision, J.-P.W.; project administration, J.-P.W.; funding acquisition, J.-P.W. All authors have read and agreed to the published version of the manuscript.
Figure 1.
Flow chart within a time step in BYCFoam. Calculations carried out in Cantera are denoted by the blue font.
Figure 1.
Flow chart within a time step in BYCFoam. Calculations carried out in Cantera are denoted by the blue font.
Figure 2.
Specific heat capacity of stoichiometric DME/air mixtures.
Figure 2.
Specific heat capacity of stoichiometric DME/air mixtures.
Figure 3.
Homogeneous autoignition of stoichiometric DME/air mixtures: (a) ignition delay time with different initial temperatures and initial pressures , (b) temperature evolution during autoignition with = 625 K and = 0.5 atm.
Figure 3.
Homogeneous autoignition of stoichiometric DME/air mixtures: (a) ignition delay time with different initial temperatures and initial pressures , (b) temperature evolution during autoignition with = 625 K and = 0.5 atm.
Figure 4.
Solutions of 1D Sod shock-tube problem at time : (a) pressure, (b) density. Black solid line: Riemann exact solution. Red solid line: rhoCentralFoam. Dashed lines: BYCFoam with four flux schemes.
Figure 4.
Solutions of 1D Sod shock-tube problem at time : (a) pressure, (b) density. Black solid line: Riemann exact solution. Red solid line: rhoCentralFoam. Dashed lines: BYCFoam with four flux schemes.
Figure 5.
The close-up of (a) pressure distributions behind the shock wave; (b) density distributions near the contact discontinuity. Black solid line: Riemann exact solution. Red solid line: rhoCentralFoam. Dashed lines: BYCFoam with four flux schemes.
Figure 5.
The close-up of (a) pressure distributions behind the shock wave; (b) density distributions near the contact discontinuity. Black solid line: Riemann exact solution. Red solid line: rhoCentralFoam. Dashed lines: BYCFoam with four flux schemes.
Figure 6.
Density contours of shock-wave diffraction.
Figure 6.
Density contours of shock-wave diffraction.
Figure 7.
Temperature evolution during autoignition with three different solvers (a) DME/air (b) n-heptane/air.
Figure 7.
Temperature evolution during autoignition with three different solvers (a) DME/air (b) n-heptane/air.
Figure 8.
Laminar flame speeds of premixed hydrogen/air mixture with varying equivalence ratios.
Figure 8.
Laminar flame speeds of premixed hydrogen/air mixture with varying equivalence ratios.
Figure 9.
One-dimensional planar flame structure of stoichiometric hydrogen/air mixture: (a) temperature and density, (b) mass fraction of and .
Figure 9.
One-dimensional planar flame structure of stoichiometric hydrogen/air mixture: (a) temperature and density, (b) mass fraction of and .
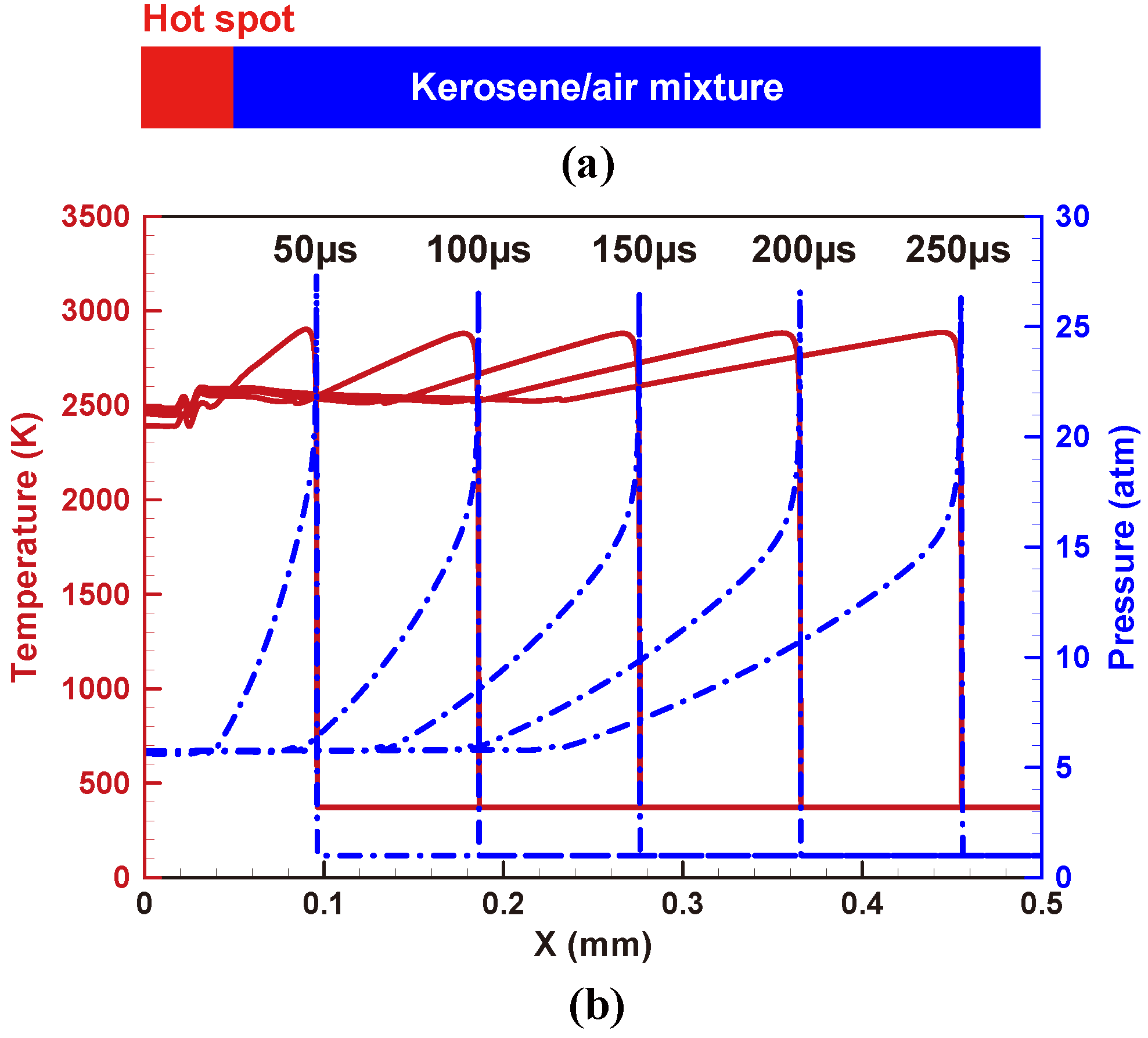
Figure 10.
Numerical simulation of one-dimensional detonation tube with kerosene/air mixture at 1 atm and 373 K: (a) schematic of the detonation tube, (b) temperature and pressure distributions at different simulation times.
Figure 10.
Numerical simulation of one-dimensional detonation tube with kerosene/air mixture at 1 atm and 373 K: (a) schematic of the detonation tube, (b) temperature and pressure distributions at different simulation times.
Figure 11.
The computational domain and initial setup in the simulation of a detonation cellular structure.
Figure 11.
The computational domain and initial setup in the simulation of a detonation cellular structure.
Figure 12.
The final flow fields of a mode-four detonation: (a) pressure, (b) temperature, (c) mesh refinement, (d) mass fraction of .
Figure 12.
The final flow fields of a mode-four detonation: (a) pressure, (b) temperature, (c) mesh refinement, (d) mass fraction of .
Figure 13.
Numerical simulation of a two-dimensional detonation cellular structure: (a) schlieren plot by density gradient, (b) triple-point trajectories.
Figure 13.
Numerical simulation of a two-dimensional detonation cellular structure: (a) schlieren plot by density gradient, (b) triple-point trajectories.
Figure 14.
Schematic of an RDC in a two-dimensional computational domain.
Figure 14.
Schematic of an RDC in a two-dimensional computational domain.
Figure 15.
Schematic of the initial conditions. A hot spot is located at the bottom of the computational domain to ignite the reactants. The rest of the mixture is air at 1 atm and 300 K.
Figure 15.
Schematic of the initial conditions. A hot spot is located at the bottom of the computational domain to ignite the reactants. The rest of the mixture is air at 1 atm and 300 K.
Figure 16.
The mass flow rates of the inlet and outlet. Solid lines: Case 0 with a static mesh. Dashed lines: Case 5 with AMR.
Figure 16.
The mass flow rates of the inlet and outlet. Solid lines: Case 0 with a static mesh. Dashed lines: Case 5 with AMR.
Figure 17.
The well-developed flow fields of an RDC. (a) Temperature and (b) mass fraction of in Case 0. (c) Temperature and (d) mass fraction of in Case 5. (e) Temperature and pressure distributions in the circumferential direction at 0.2 cm. Solid lines: Case 0 with static mesh. Dashed lines: Case 5 with AMR.
Figure 17.
The well-developed flow fields of an RDC. (a) Temperature and (b) mass fraction of in Case 0. (c) Temperature and (d) mass fraction of in Case 5. (e) Temperature and pressure distributions in the circumferential direction at 0.2 cm. Solid lines: Case 0 with static mesh. Dashed lines: Case 5 with AMR.
Table 1.
The distinctions between rhoCentralFoam and BYCFoam.
Table 1.
The distinctions between rhoCentralFoam and BYCFoam.
| Solver | Reconstruction Variables | Flux Schemes |
|---|
| rhoCentralFoam | () * | Kurganov |
| BYCFoam | () | Kurganov, HLL, HLLC, AUSM+M |
Table 2.
Initial conditions of auto-ignition.
Table 2.
Initial conditions of auto-ignition.
| Fuel | Initial Temperature [K] | Initial Pressure [atm] | Chemical Mechanisms |
|---|
| DME | 650 | 1 | Bhagatwala et al. [44] |
| n-heptane | 700 | 3 | LLNL (Version 3) [45] |
Table 3.
Comparisons of one-dimensional detonation properties with varying equivalence ratios . U is the detonation velocity, p and T are the pressure and temperature at the CJ points of the detonation wave, respectively. is the maximum relative error between 1D simulations and the theoretical CJ properties of ().
Table 3.
Comparisons of one-dimensional detonation properties with varying equivalence ratios . U is the detonation velocity, p and T are the pressure and temperature at the CJ points of the detonation wave, respectively. is the maximum relative error between 1D simulations and the theoretical CJ properties of ().
| [m/s] | [m/s] | [atm] | [atm] | [K] | [K] | |
|---|
| 0.8 | 1723.71 | 1716.24 | 13.67 | 13.51 | 2709.56 | 2703.75 | 1.17% |
| 1.0 | 1795.04 | 1789.31 | 15.07 | 14.94 | 2876.97 | 2880.14 | 0.86% |
| 1.2 | 1830.44 | 1827.93 | 15.73 | 15.68 | 2913.65 | 2906.93 | 0.32% |
| 1.4 | 1832.18 | 1822.06 | 15.67 | 15.48 | 2828.18 | 2822.31 | 1.21% |
Table 4.
Refinement criteria used in the simulation of the detonation cellular structure.
Table 4.
Refinement criteria used in the simulation of the detonation cellular structure.
| Criteria | Refined Regions |
|---|
| 0.04 or 0.01 | Leading shock, transverse waves and slip lines |
| and 1000 K | Induction zone and reaction zone |
Table 5.
Comparisons of the detonation cell size with other numerical results. L and are the length and the width of the detonation cells, respectively.
Table 5.
Comparisons of the detonation cell size with other numerical results. L and are the length and the width of the detonation cells, respectively.
| Study | Base Grid [µm] | Finest Grid [µm] | Cell Size [mm] | Comments |
|---|
| | |
|---|
| Current study | | | | AMR |
| Deiterding et al. [38] | | | | AMR |
| Oran et al. [56] | | - | | Uniform grids |
| Wang et al. [57] | | - | | Uniform grids |
Table 6.
Test cases for the AMR and DLB algorithms. Case 0 is a benchmark without using any acceleration methods. Cases 0–1 are calculated with uniform static grids. Cases 2–5 are calculated with AMR. The CPU time costs in the computations are listed with a relative form.
Table 6.
Test cases for the AMR and DLB algorithms. Case 0 is a benchmark without using any acceleration methods. Cases 0–1 are calculated with uniform static grids. Cases 2–5 are calculated with AMR. The CPU time costs in the computations are listed with a relative form.
| Case | Base Grid [mm] | Finest Grid [mm] | DLB_Mesh | DLB_Chem | Relative CPU Time |
|---|
| 0 | 0.1 | - | - | - | 100% |
| 1 | 0.1 | - | - | ✓ | 80% |
| 2 | 0.4 | 0.1 | - | - | 63% |
| 3 | 0.4 | 0.1 | - | ✓ | 20% |
| 4 | 0.4 | 0.1 | ✓ | - | 14% |
| 5 | 0.4 | 0.1 | ✓ | ✓ | 11% |