Optimizing CO2-Water Injection Ratio in Heterogeneous Reservoirs: Implications for CO2 Geo-Storage
Abstract
1. Introduction
2. Methodology
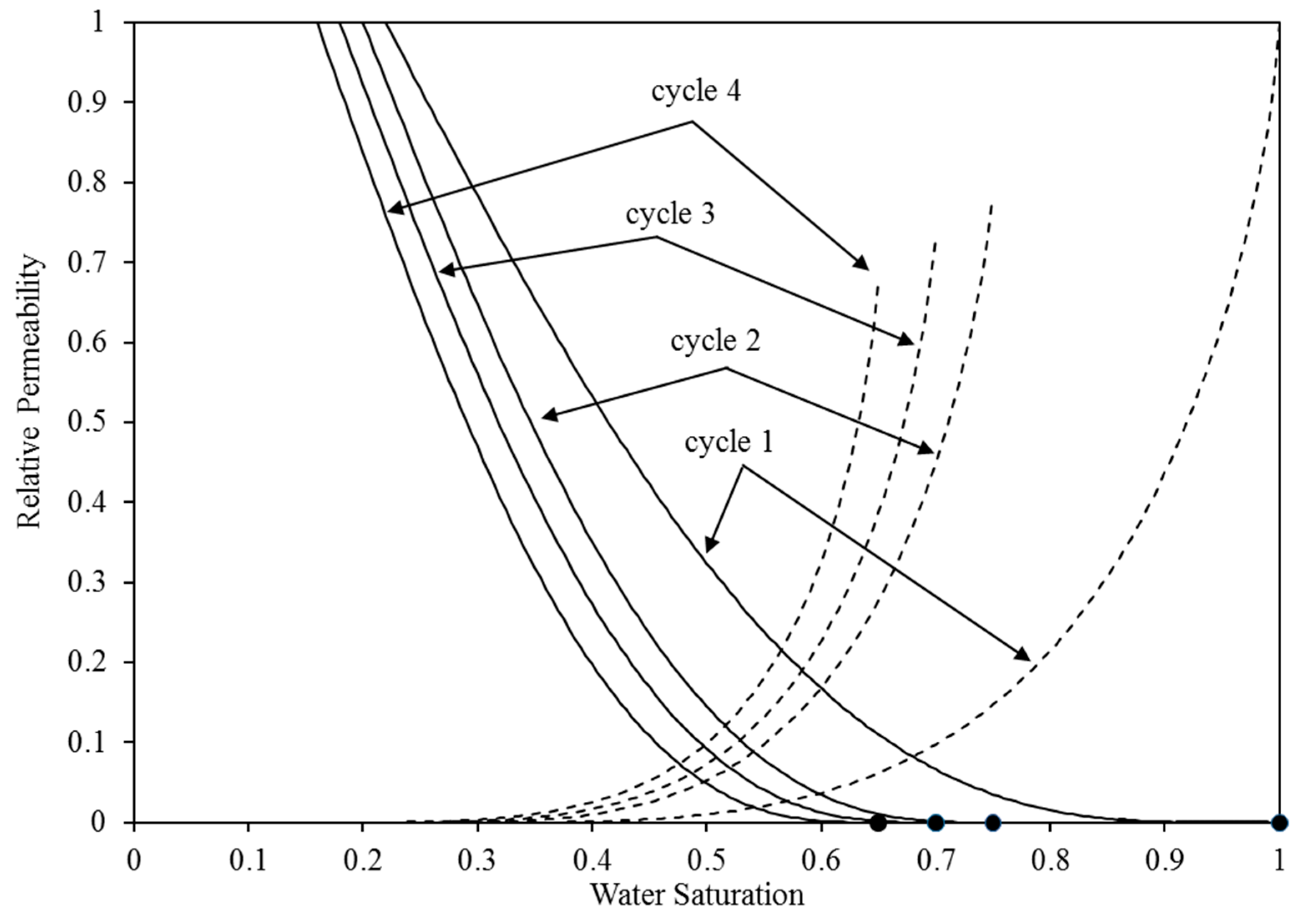
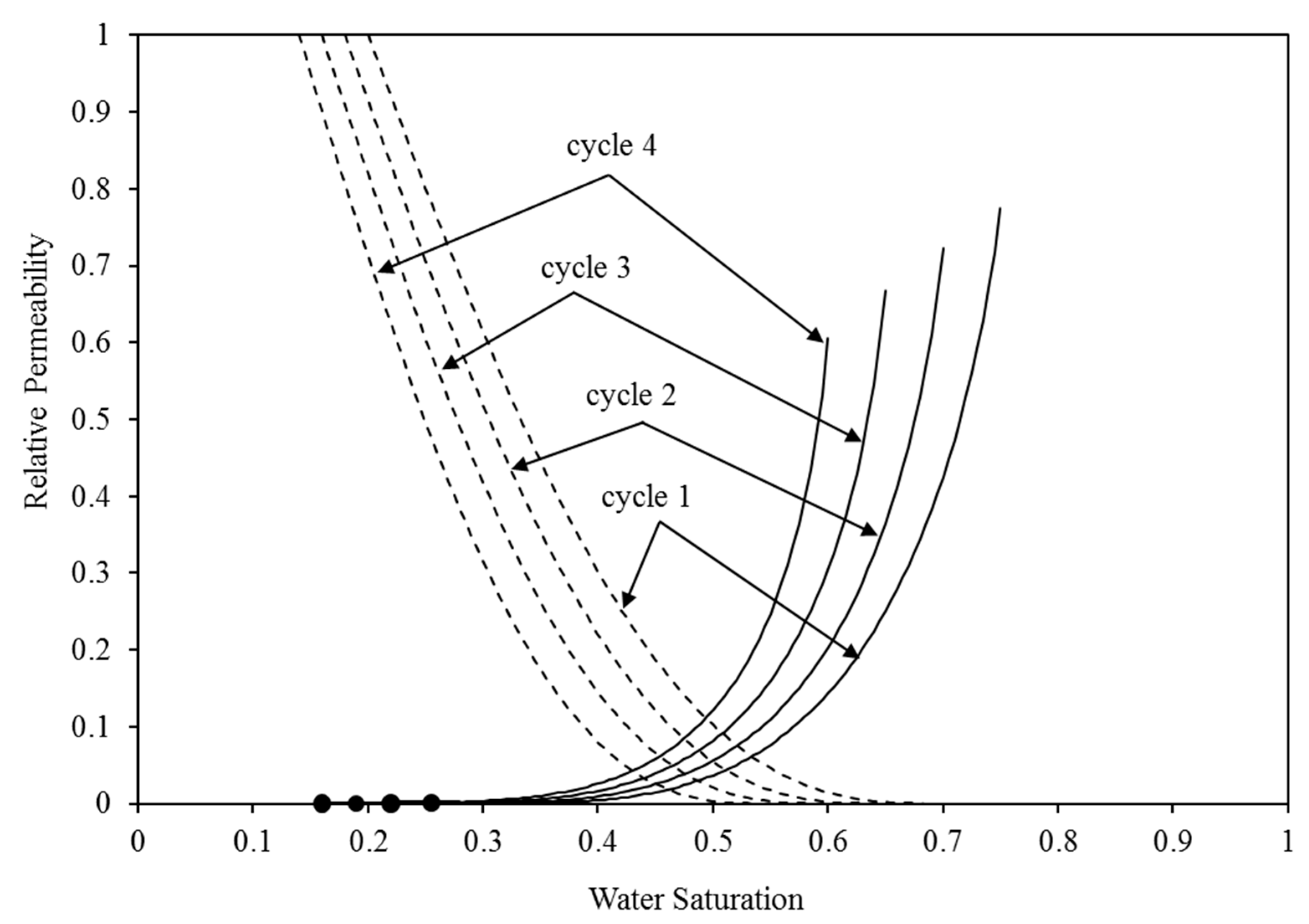
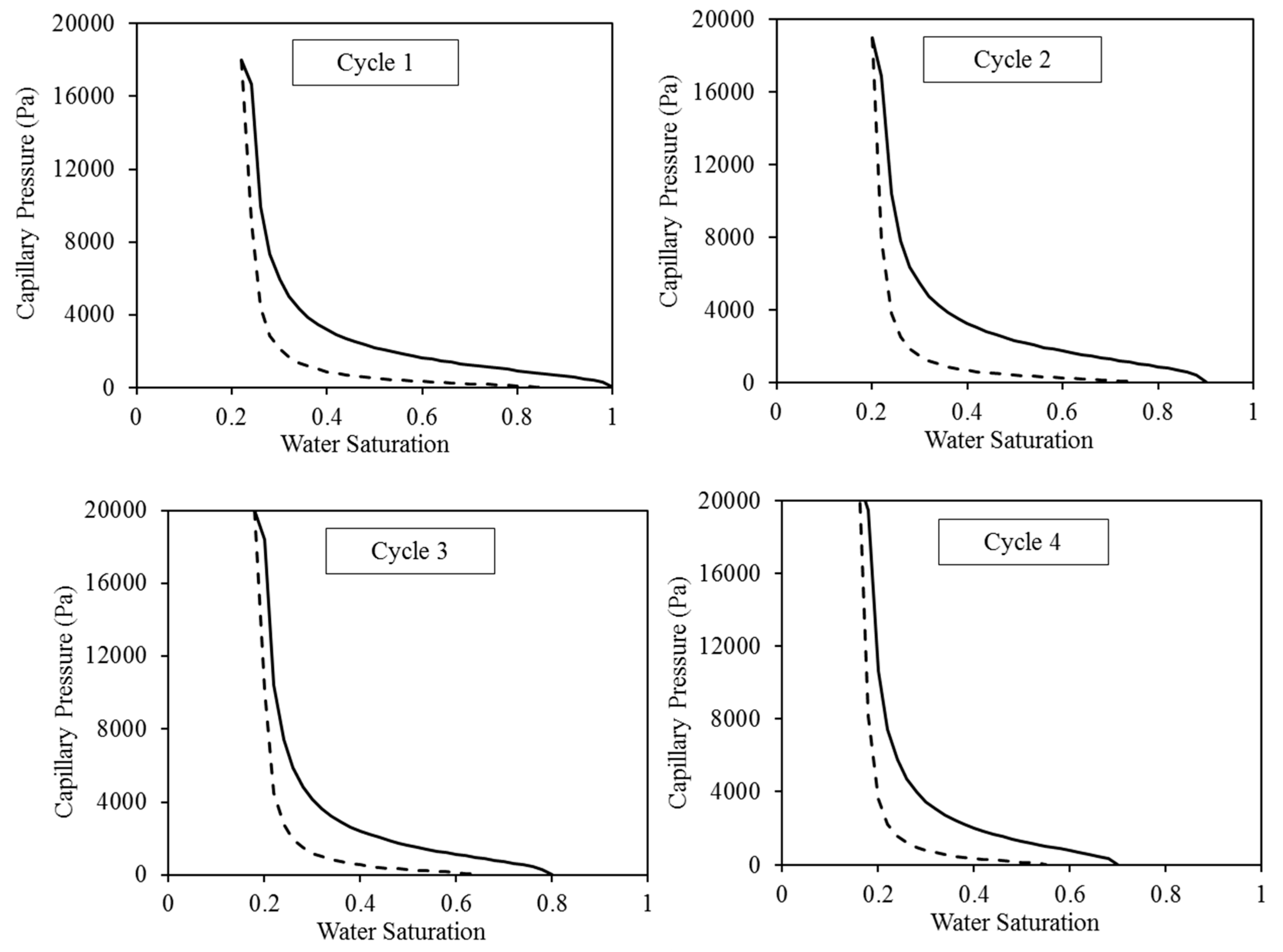
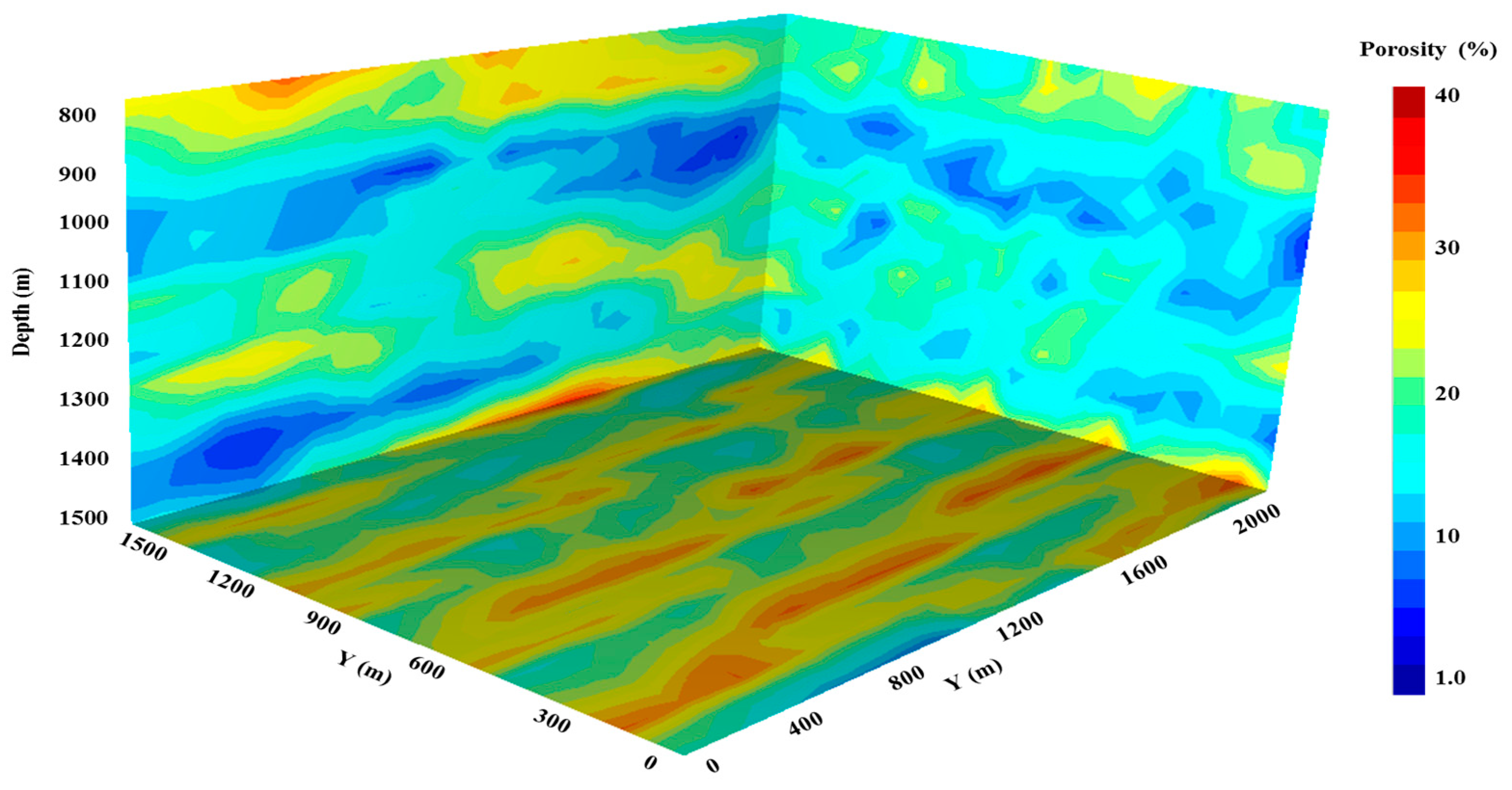
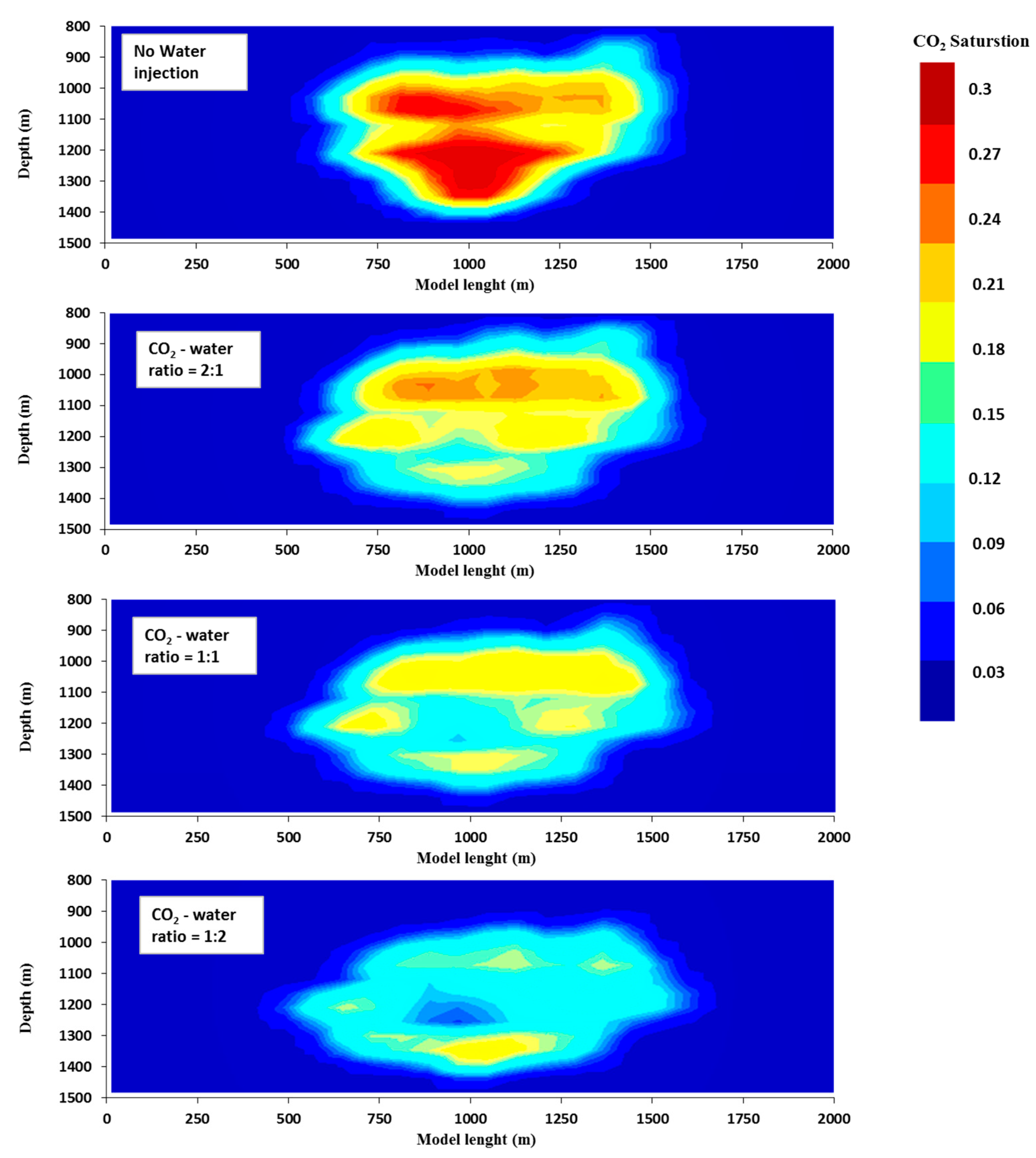
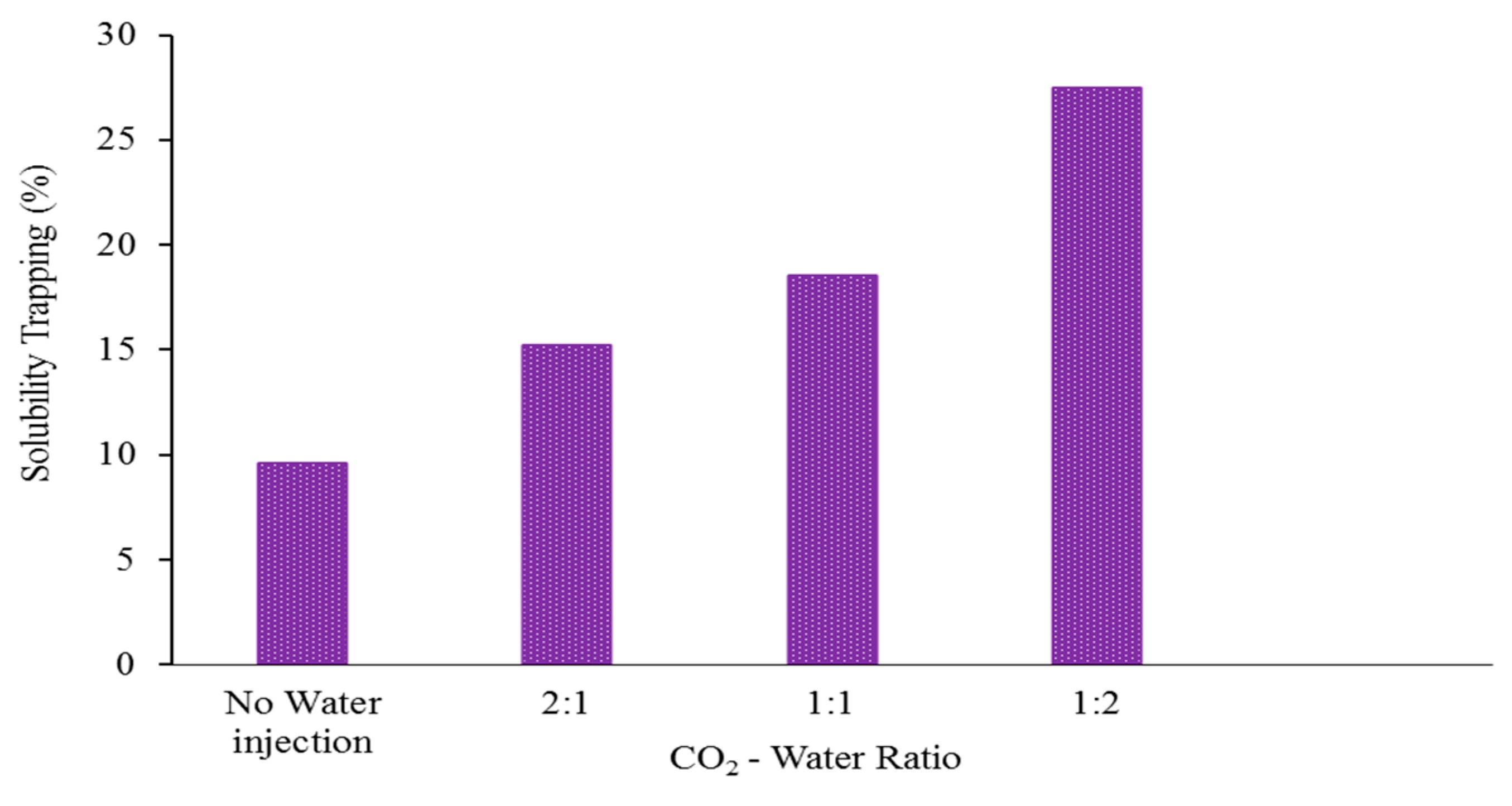
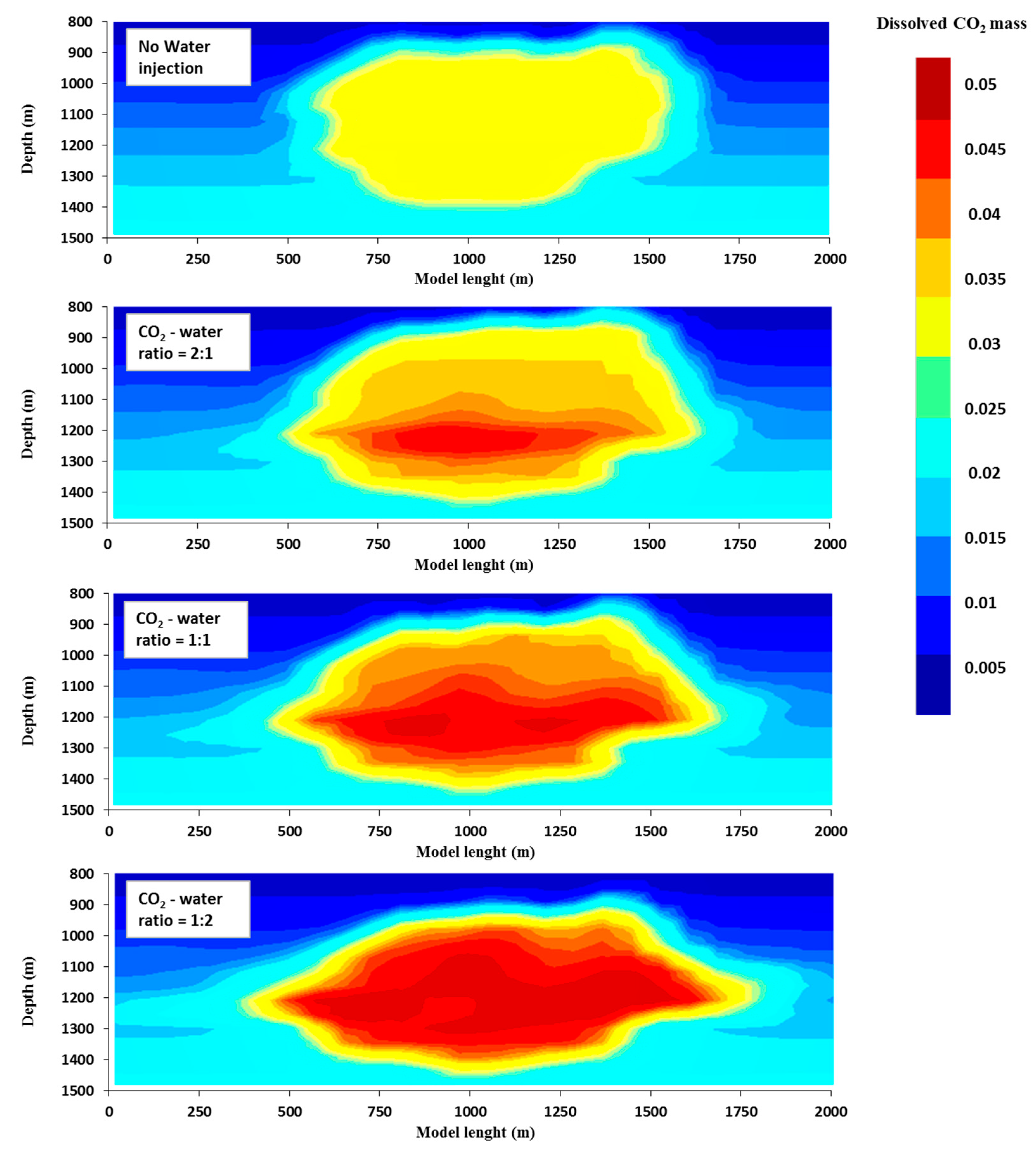
3. Results and Discussion
4. Conclusions
Funding
Data Availability Statement
Conflicts of Interest
References
- Riemer, P. Greenhouse gas mitigation technologies, an overview of the CO2 capture, storage and future activities of the IEA Greenhouse Gas R&D programme. Energy Convers. Manag. 1996, 37, 665–670. [Google Scholar]
- Kou, Z.; Wang, H.; Alvarado, V. Reservoir characterization and multiphase flow property in the upper Minnelusa sandstone: Implication for geological carbon storage. Adv. Geo-Energy Res. 2022, 6, 535–536. [Google Scholar] [CrossRef]
- Weir, G.J.; White, S.P.; Kissling, W.M. Reservoir storage and containment of greenhouse gases. Transp. Porous Media 1996, 23, 37–60. [Google Scholar] [CrossRef]
- Holloway, S. Underground sequestration of carbon dioxide—A viable greenhouse gas mitigation option. Energy 2005, 30, 2318–2333. [Google Scholar] [CrossRef]
- Zhang, L.; Li, X.; Ren, B.; Cui, G.; Zhang, Y.; Ren, S.; Chen, G.; Zhang, H. CO2 storage potential and trapping mechanisms in the H-59 block of Jilin oilfield China. Int. J. Greenh. Gas Control 2016, 49, 267–280. [Google Scholar] [CrossRef]
- Al-Khdheeawi, E.A.; Mahdi, D.S.; Yuan, Y.; Iglauer, S. Influence of Clay Content on CO2-Rock Interaction and Mineral-Trapping Capacity of Sandstone Reservoirs. Energies 2023, 16, 3489. [Google Scholar] [CrossRef]
- Xu, T.; Apps, J.A.; Pruess, K. Numerical simulation of CO2 disposal by mineral trapping in deep aquifers. Appl. Geochem. 2004, 19, 917–936. [Google Scholar] [CrossRef]
- Al-Khdheeawi, E.A.; Mahdi, D.S.; Hasan, M.M. Reservoir scale CO2-water-rock interactions and geochemical evolution of sandstone reservoirs due to CO2 geo-storage process. In AIP Conference Proceedings; AIP Publishing: Melville, NY, USA, 2022. [Google Scholar]
- Ning, Y.; Tura, A. Economic and operational investigation of CO2 sequestration through enhanced oil recovery in unconventional reservoirs in Colorado, USA. Geoenergy Sci. Eng. 2023, 226, 211820. [Google Scholar] [CrossRef]
- Song, H.; Huang, G.; Li, T.; Zhang, Y.; Lou, Y. Analytical model of CO2 storage efficiency in saline aquifer with vertical heterogeneity. J. Nat. Gas Sci. Eng. 2014, 18, 77–89. [Google Scholar] [CrossRef]
- Ashirbekov, A.; Kabdenova, B.; Kuljabekov, A.; Monaco, E.; Wang, L.; Rojas-Solórzano, L. Lattice Boltzmann pseudopotential multiphase modeling of transcritical CO2 flow using a crossover formulation. Adv. Geo-Energy Res. 2022, 6, 539–540. [Google Scholar] [CrossRef]
- Shariatipour, S.M.; Pickup, G.E.; Mackay, E.J. Simulations of CO2 storage in aquifer models with top surface morphology and transition zones. Int. J. Greenh. Gas Control 2016, 54, 117–128. [Google Scholar] [CrossRef]
- Prévost, J.H.; Fuller, R.; Altevogt, A.S.; Bruant, R.; Scherer, G. Numerical modeling of carbon dioxide injection and transport in deep saline aquifers. Greenh. Gas Control Technol. 2005, 7, 2189–2193. [Google Scholar]
- Metz, B.; Davidson, O.; De Coninck, H.C.; Loos, M.; Meyer, L. IPCC, 2005: IPCC Special Report on Carbon Dioxide Capture and Storage; Working Group III of the Intergovernmental Panel on Climate Change: Cambridge, UK; New York, NY, USA, 2005; 442p. [Google Scholar]
- Iglauer, S.; Al-Yaseri, A.Z.; Rezaee, R.; Lebedev, M. CO2 wettability of caprocks: Implications for structural storage capacity and containment security. Geophys. Res. Lett. 2015, 42, 9279–9284. [Google Scholar] [CrossRef]
- Al-Khdheeawi, E.A.; Mahdi, D.S.; Ali, M.; Fauziah, C.A.; Barifcani, A. Impact of caprock type on geochemical reactivity and mineral trapping efficiency of CO2. In Offshore Technology Conference Asia; OnePetro: Richardson, TX, USA, 2020. [Google Scholar]
- Al-Khdheeawi, E.A.; Vialle, S.; Barifcani, A.; Sarmadivaleh, M.; Iglauer, S. Impact of reservoir wettability and heterogeneity on CO2-plume migration and trapping capacity. Int. J. Greenh. Gas Control 2017, 58, 142–158. [Google Scholar] [CrossRef]
- Al-Khdheeawi, E.A.; Vialle, S.; Barifcani, A.; Sarmadivaleh, M.; Iglauer, S. Influence of CO2-wettability on CO2 migration and trapping capacity in deep saline aquifers. Greenh. Gases Sci. Technol. 2017, 7, 328–338. [Google Scholar] [CrossRef]
- Iglauer, S. Dissolution Trapping of Carbon Dioxide in Reservoir Formation Brine-a Carbon Storage Mechanism; INTECH Open Access Publisher: London, UK, 2011. [Google Scholar]
- Bachu, S.; Gunter, W.; Perkins, E. Aquifer disposal of CO2: Hydrodynamic and mineral trapping. Energy Convers. Manag. 1994, 35, 269–279. [Google Scholar] [CrossRef]
- Xu, T.; Apps, J.A.; Pruess, K. Reactive geochemical transport simulation to study mineral trapping for CO2 disposal in deep arenaceous formations. J. Geophys. Res. Solid Earth 2003, 108, 1–13. [Google Scholar] [CrossRef]
- Ambrose, W.A.; Lakshminarasimhan, S.; Holtz, M.H.; Núñez-López, V.; Hovorka, S.D.; Duncan, I. Geologic factors controlling CO2 storage capacity and permanence: Case studies based on experience with heterogeneity in oil and gas reservoirs applied to CO2 storage. Environ. Geol. 2008, 54, 1619–1633. [Google Scholar] [CrossRef]
- Arif, M.; Barifcani, A.; Lebedev, M.; Iglauer, S. Structural trapping capacity of oil-wet caprock as a function of pressure, temperature and salinity. Int. J. Greenh. Gas Control 2016, 50, 112–120. [Google Scholar] [CrossRef]
- Johnson, J.W.; Nitao, J.J.; Knauss, K.G. Reactive transport modeling of CO2 storage in saline aquifers to elucidate fundamental processes, trapping mechanisms and sequestration partitioning. Geol. Storage Carbon Dioxide 2004, 233, 107–128. [Google Scholar]
- Al-Khdheeawi, E.A.; Vialle, S.; Barifcani, A.; Sarmadivaleh, M.; Iglauer, S. Effect of brine salinity on CO2 plume migration and trapping capacity in deep saline aquifers. APPEA J. 2017, 57, 100–109. [Google Scholar] [CrossRef]
- Al-Khdheeawi, E.A.; Vialle, S.; Barifcani, A.; Sarmadivaleh, M.; Iglauer, S. Effect of wettability heterogeneity and reservoir temperature on CO 2 storage efficiency in deep saline aquifers. Int. J. Greenh. Gas Control 2018, 68, 216–229. [Google Scholar] [CrossRef]
- Blunt, M.J. Effects of heterogeneity and wetting on relative permeability using pore level modeling. SPE J. 1997, 2, 70–87. [Google Scholar] [CrossRef]
- Doughty, C.; Pruess, K. Modeling Supercritical CO2 Injection in Heterogneous Porous Media; Lawrence Berkeley National Laboratory: Berkeley, CA, USA, 2003. [Google Scholar]
- Flett, M.; Gurton, R.; Weir, G. Heterogeneous saline formations for carbon dioxide disposal: Impact of varying heterogeneity on containment and trapping. J. Pet. Sci. Eng. 2007, 57, 106–118. [Google Scholar] [CrossRef]
- Al-Khdheeawi, E.A.; Vialle, S.; Barifcani, A.; Sarmadivaleh, M.; Iglauer, S. Enhancement of CO2 trapping efficiency in heterogeneous reservoirs by water-alternating gas injection. Greenh. Gases Sci. Technol. 2018, 8, 920–931. [Google Scholar] [CrossRef]
- Joodaki, S.; Niemi, A.; Rasmusson, K.; Yang, Z.; Bensabat, J. Enhancement of CO2 Trapping in Saline Aquifers Using a Water-Alternating-Gas Method. 2016. Available online: https://ui.adsabs.harvard.edu/abs/2016EGUGA..18.8216J/abstract (accessed on 1 April 2016).
- Ahmadi, Y.; Eshraghi, S.E.; Bahrami, P.; Hasanbeygi, M.; Kazemzadeh, Y.; Vahedian, A. Comprehensive Water–Alternating-Gas (WAG) injection study to evaluate the most effective method based on heavy oil recovery and asphaltene precipitation tests. J. Pet. Sci. Eng. 2015, 133, 123–129. [Google Scholar] [CrossRef]
- Al-Bayati, D.; Al-Khdheeawi, E.; Saeedi, A.; Myers, M.; Xie, Q. Investigations of Miscible Water Alternating Gas Injection Efficiency in Layered Sandstone Porous Media. Iraqi J. Oil Gas Res. 2022, 2, 31–44. [Google Scholar] [CrossRef]
- Al-Ghanim, W.; Gharbi, R.; Algharaib, M.K. Designing a simultaneous water alternating gas process for optimizing oil recovery. In EUROPEC/EAGE Conference and Exhibition; Society of Petroleum Engineers: London, UK, 2009. [Google Scholar]
- Lei, H.; Yang, S.; Zu, L.; Wang, Z.; Li, Y. Oil Recovery Performance and CO2 Storage Potential of CO2 Water-Alternating-Gas Injection after Continuous CO2 Injection in a Multilayer Formation. Energy Fuels 2016, 30, 8922–8931. [Google Scholar] [CrossRef]
- Chen, S.; Li, H.; Yang, D.; Tontiwachwuthikul, P. Optimal parametric design for water-alternating-gas (WAG) process in a CO2-miscible flooding reservoir. J. Can. Pet. Technol. 2010, 49, 75–82. [Google Scholar] [CrossRef]
- Shahverdi, H.; Sohrabi, M. An improved three-phase relative permeability and hysteresis model for the simulation of a water-alternating-gas injection. SPE J. 2013, 18, 841–850. [Google Scholar] [CrossRef]
- Le Van, S.; Chon, B. Applicability of an Artificial Neural Network for Predicting Water-Alternating-CO2 Performance. Energies 2017, 10, 842. [Google Scholar] [CrossRef]
- Laochamroonvorapongse, R.; Kabir, C.; Lake, L.W. Performance assessment of miscible and immiscible water-alternating gas floods with simple tools. J. Pet. Sci. Eng. 2014, 122, 18–30. [Google Scholar] [CrossRef]
- Nghiem, L.; Shrivastava, V.; Kohse, B.; Hassam, M.; Yang, C. Simulation and optimization of trapping processes for CO2 storage in saline aquifers. J. Can. Pet. Technol. 2010, 49, 15–22. [Google Scholar] [CrossRef]
- Dake, L.P. Fundamentals of Reservoir Engineering; Elsevier: Amsterdam, The Netherlands, 2007; Volume 8. [Google Scholar]
- Pruess, K. ECO2M: A TOUGH2 Fluid Property Module for Mixtures of Water, NaCl, and CO2, Including Super-and Sub-Critical Conditions, and Phase Change between Liquid and Gaseous CO2; Lawrence Berkeley National Laboratory: Berkeley, CA, USA, 2011. [Google Scholar]
- Pruess, K.; Oldenburg, C.; Moridis, G. TOUGH2 User’s Guide Version 2; Lawrence Berkeley National Laboratory: Berkeley, CA, USA, 1999. [Google Scholar]
- Pruess, K. ECO2N: A TOUGH2 Fluid Property Module for Mixtures of Water, NaCl, and CO2; Lawrence Berkeley National Laboratory: Berkeley, CA, USA, 2005. [Google Scholar]
- Doster, F.; Nordbotten, J.; Celia, M. Impact of capillary hysteresis and trapping on vertically integrated models for CO2 storage. Adv. Water Resour. 2013, 62, 465–474. [Google Scholar] [CrossRef]
- Doughty, C. Modeling geologic storage of carbon dioxide: Comparison of non-hysteretic and hysteretic characteristic curves. Energy Convers. Manag. 2007, 48, 1768–1781. [Google Scholar] [CrossRef]
- Al-Khdheeawi, E.A.; Vialle, S.; Barifcani, A.; Sarmadivaleh, M.; Iglauer, S. Influence of injection well configuration and rock wettability on CO2 plume behaviour and CO2 trapping capacity in heterogeneous reservoirs. J. Nat. Gas Sci. Eng. 2017, 43, 190–206. [Google Scholar] [CrossRef]
- Craig, F.F. The Reservoir Engineering Aspects of Waterflooding; Henry L. Doherty Memorial Fund of AIME, Society of Petroleum Engineers: Richardson, TX, USA, 1993; Volume 3. [Google Scholar]
- McCaffery, F.; Bennion, D. The Effect of Wettability on Two-Phase Relative Pemeabilities. J. Can. Pet. Technol. 1974, 13. [Google Scholar] [CrossRef]
- Batycky, J.P.; McCaffery, F.G.; Hodgins, P.K.; Fisher, D.B. Interpreting relative permeability and wettability from unsteady-state displacement measurements. Soc. Pet. Eng. J. 1981, 21, 296–308. [Google Scholar] [CrossRef]
- Pentland, C.H.; El-Maghraby, R.; Iglauer, S.; Blunt, M.J. Measurements of the capillary trapping of super-critical carbon dioxide in Berea sandstone. Geophys. Res. Lett. 2011, 38. [Google Scholar] [CrossRef]
- Van Genuchten, M.T. A closed-form equation for predicting the hydraulic conductivity of unsaturated soils. Soil Sci. Soc. Am. J. 1980, 44, 892–898. [Google Scholar] [CrossRef]
- Mualem, Y. A new model for predicting the hydraulic conductivity of unsaturated porous media. Water Resour. Res. 1976, 12, 513–522. [Google Scholar] [CrossRef]
- Leverett, M. Capillary behavior in porous solids. Trans. AIME 1941, 142, 152–169. [Google Scholar] [CrossRef]



| CO2—Water Ratio | CO2 Injection Rate (Million Ton/Year) | Total CO2 Injected (Million Ton) | Water Injection Rate (Million Ton/Year) | Total Injected Water (Million Ton) |
|---|---|---|---|---|
| No water injection | 1.0 | 4.0 | not applicable | not applicable |
| 2:1 | 1.0 | 4.0 | 0.5 | 2.0 |
| 1:1 | 1.0 | 4.0 | 1.0 | 4.0 |
| 1:2 | 1.0 | 4.0 | 2.0 | 8.0 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Al-Khdheeawi, E.A. Optimizing CO2-Water Injection Ratio in Heterogeneous Reservoirs: Implications for CO2 Geo-Storage. Energies 2024, 17, 678. https://doi.org/10.3390/en17030678
Al-Khdheeawi EA. Optimizing CO2-Water Injection Ratio in Heterogeneous Reservoirs: Implications for CO2 Geo-Storage. Energies. 2024; 17(3):678. https://doi.org/10.3390/en17030678
Chicago/Turabian StyleAl-Khdheeawi, Emad A. 2024. "Optimizing CO2-Water Injection Ratio in Heterogeneous Reservoirs: Implications for CO2 Geo-Storage" Energies 17, no. 3: 678. https://doi.org/10.3390/en17030678
APA StyleAl-Khdheeawi, E. A. (2024). Optimizing CO2-Water Injection Ratio in Heterogeneous Reservoirs: Implications for CO2 Geo-Storage. Energies, 17(3), 678. https://doi.org/10.3390/en17030678







