Numerical Study of Supercritical Opposed Wall-Fired Boiler Furnace Temperature and High-Temperature Heating Surface Stress under Variable Load Operation
Abstract
1. Introduction
2. Calculating the Condition of the Boiler Equipment
2.1. Flue Gas and Air Supply
2.2. Main Boiler Parameters
2.3. Burner Condition
3. Modeling Methodology
3.1. Modeling and Grid
3.2. Numerical Methods
3.3. Mathematical Model and Model Parameters for Numerical Simulation
3.3.1. Realizable k-ε Model
3.3.2. Lagrangian Stochastic Tracking Model
3.3.3. P-1 Model
3.4. Stress Solution
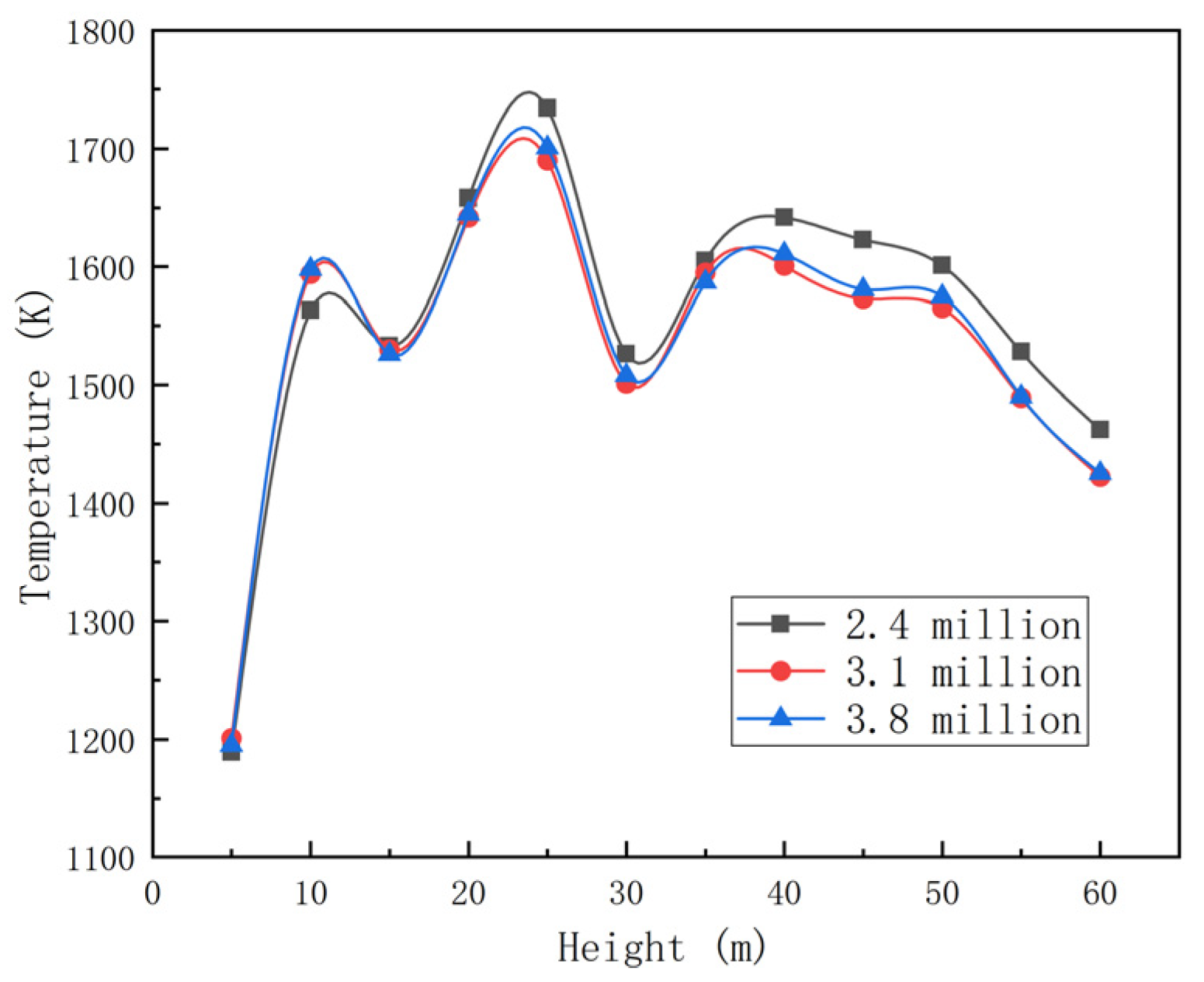
3.5. Grid Independence Testing
3.6. Boundary Condition
3.7. Differential Approximations for Radiative Heat Transfer in Combustion Systems Containing Particles
3.8. Case Conditions
4. Results and Discussion
4.1. Verification of Numerical Simulation Accuracy
4.2. Numerical Simulation of the Furnace
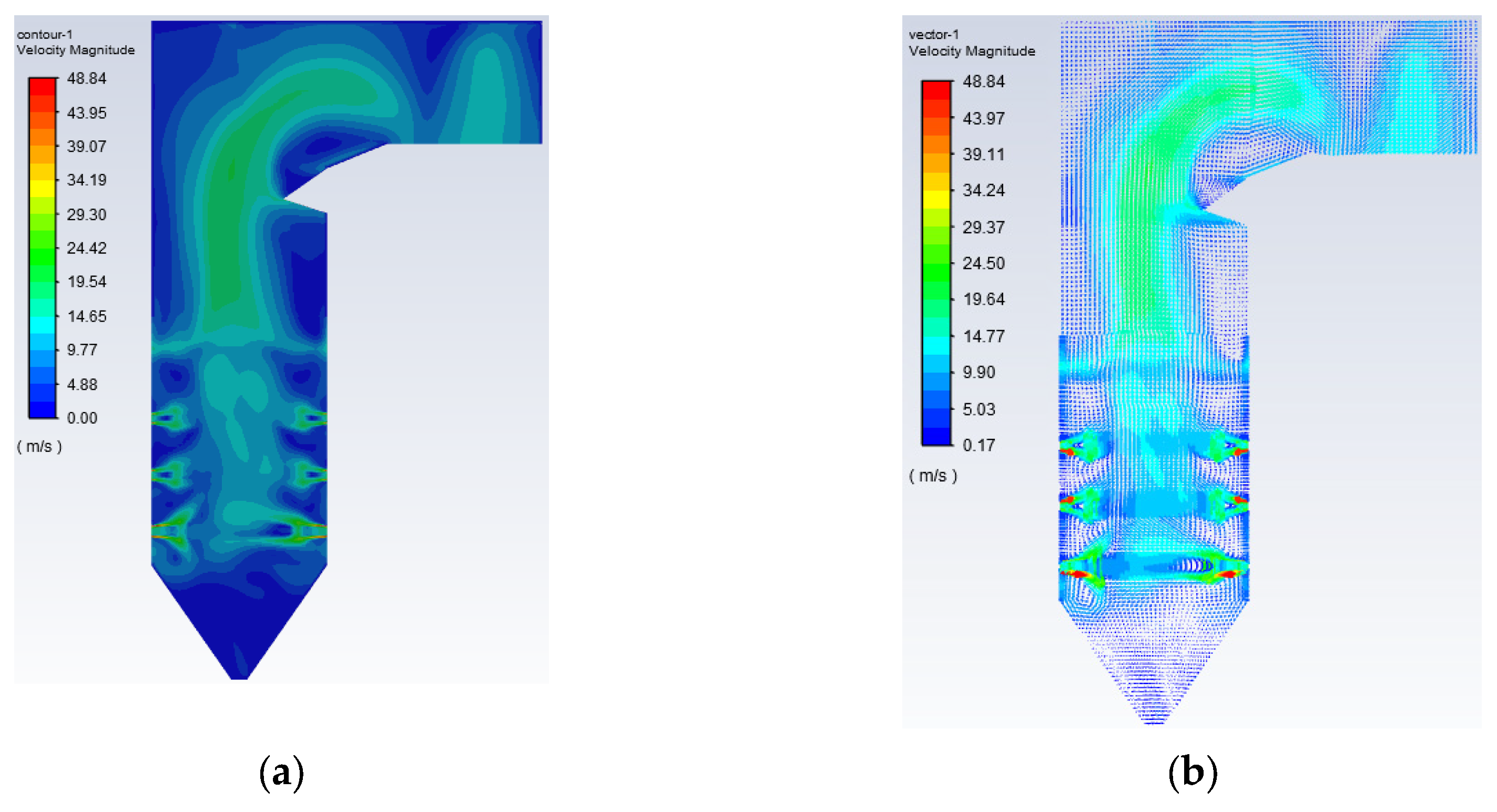
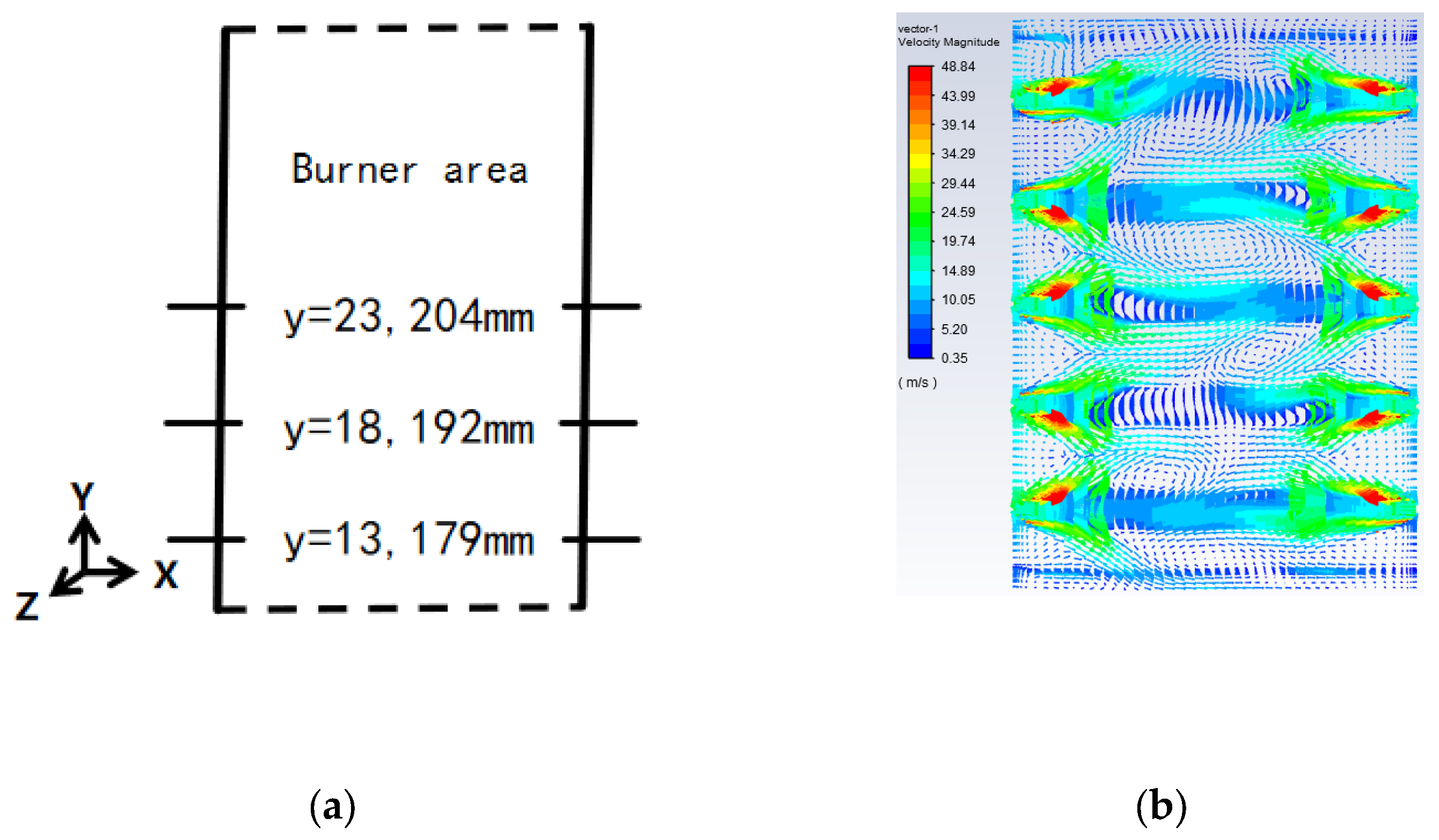
4.2.1. Furnace Flow Distribution
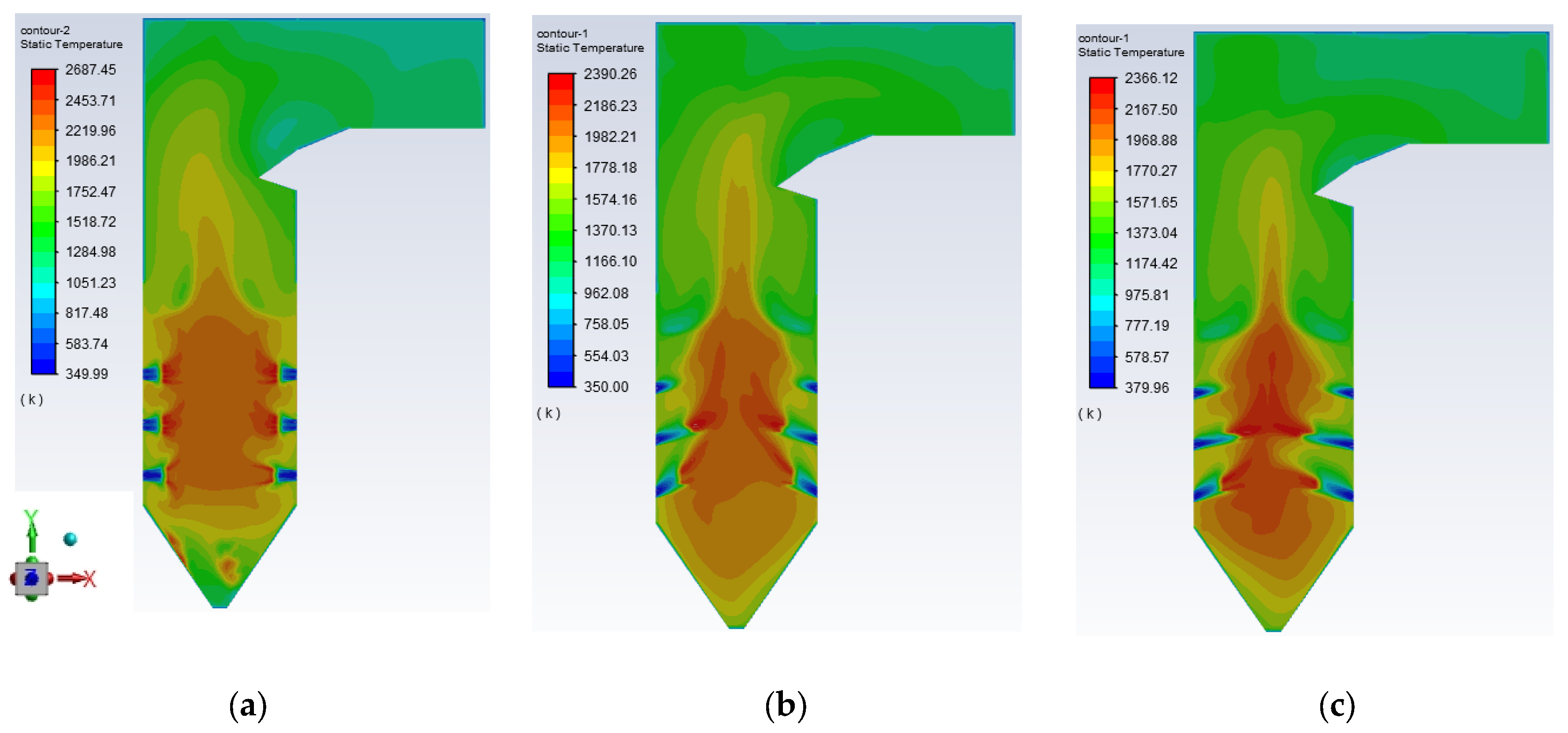
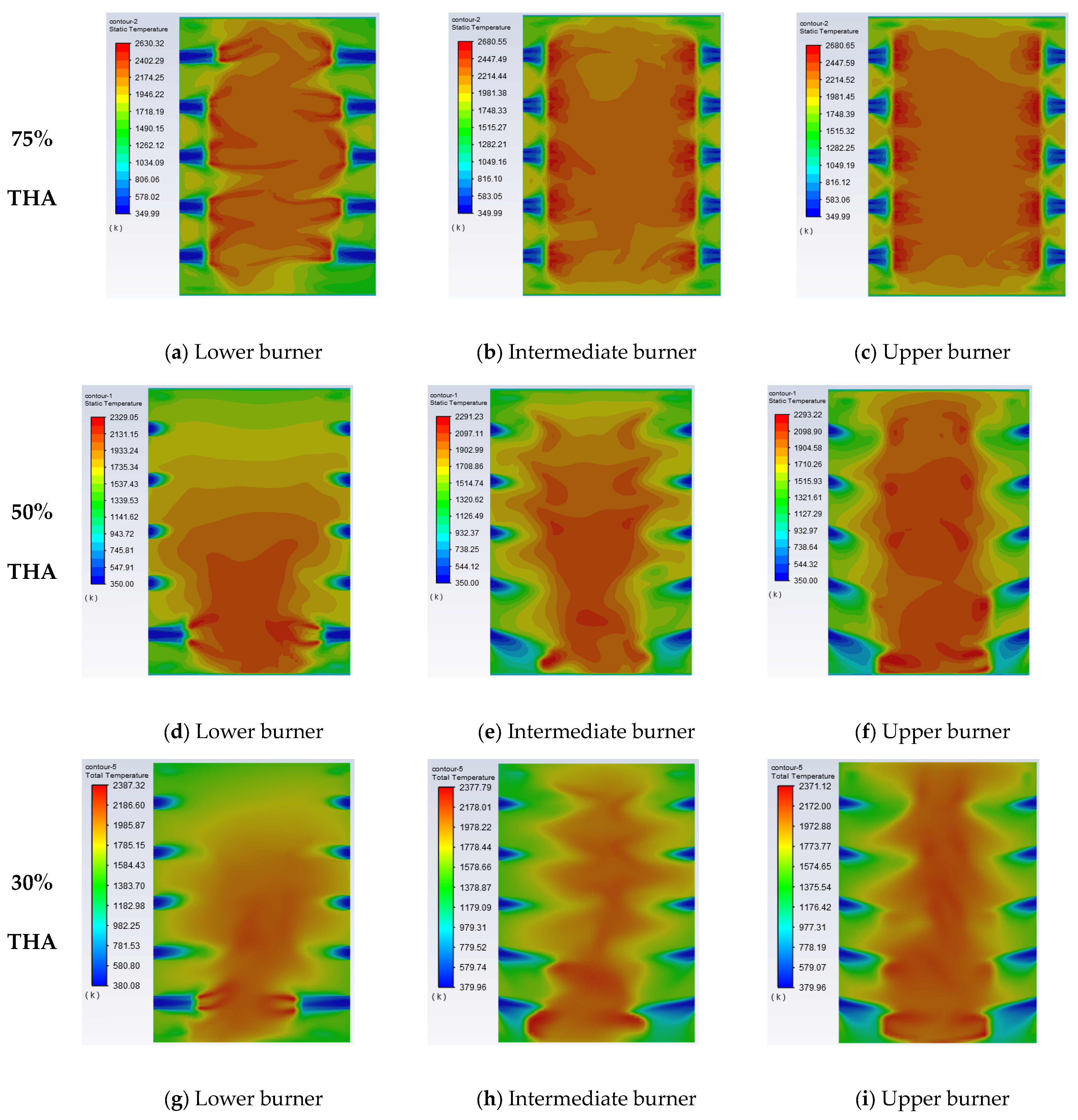
4.2.2. Temperature of the Furnace under Different Loads
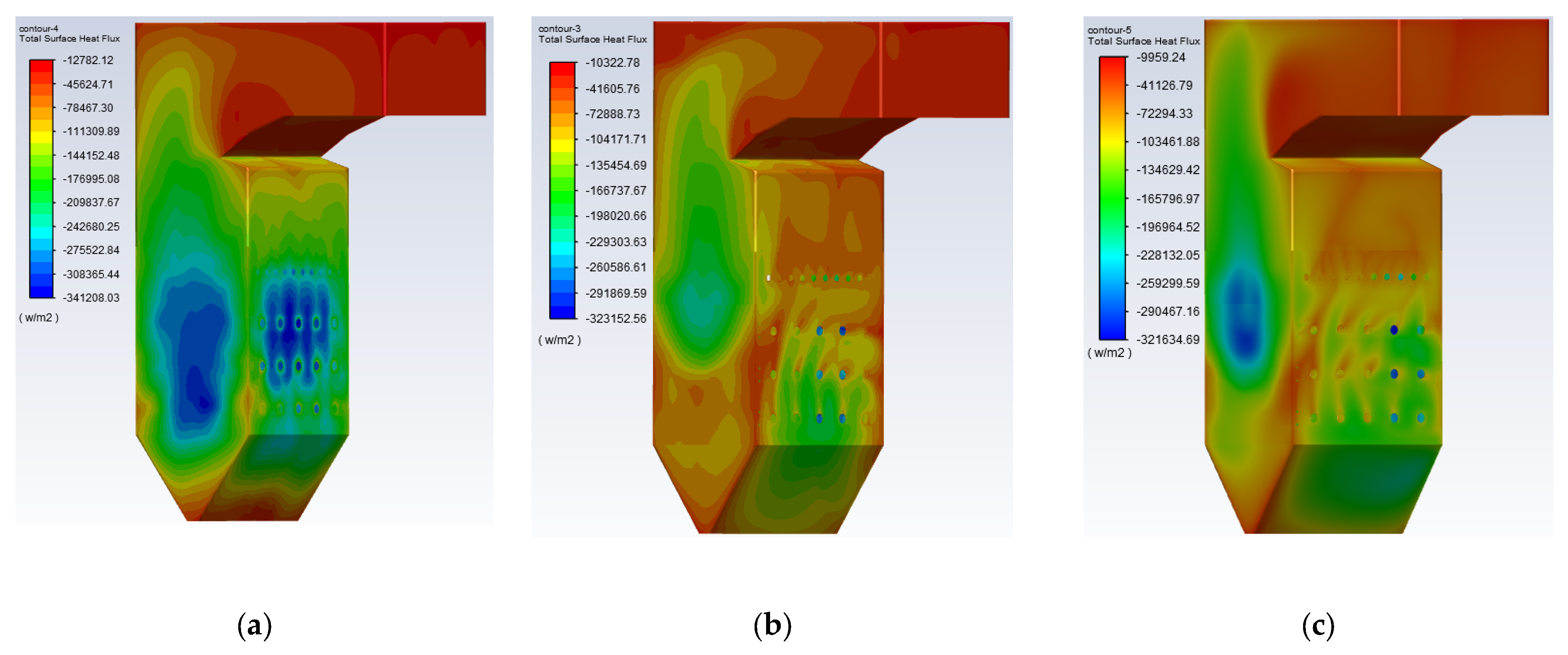
4.2.3. Heat Flux Distributions of the Furnace Wall under Different Loads
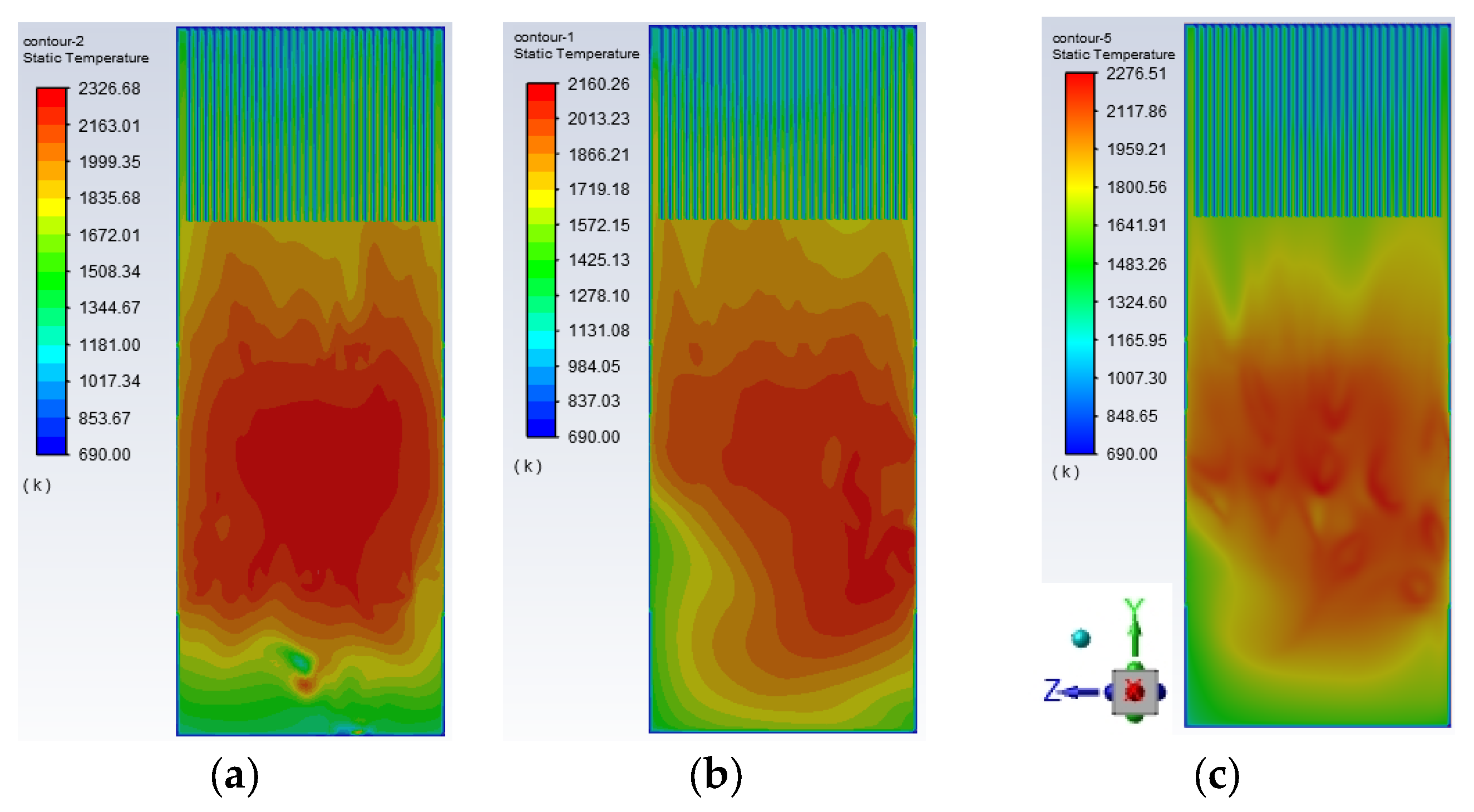
4.3. Numerical Simulation of Heating Surfaces 1 and 2
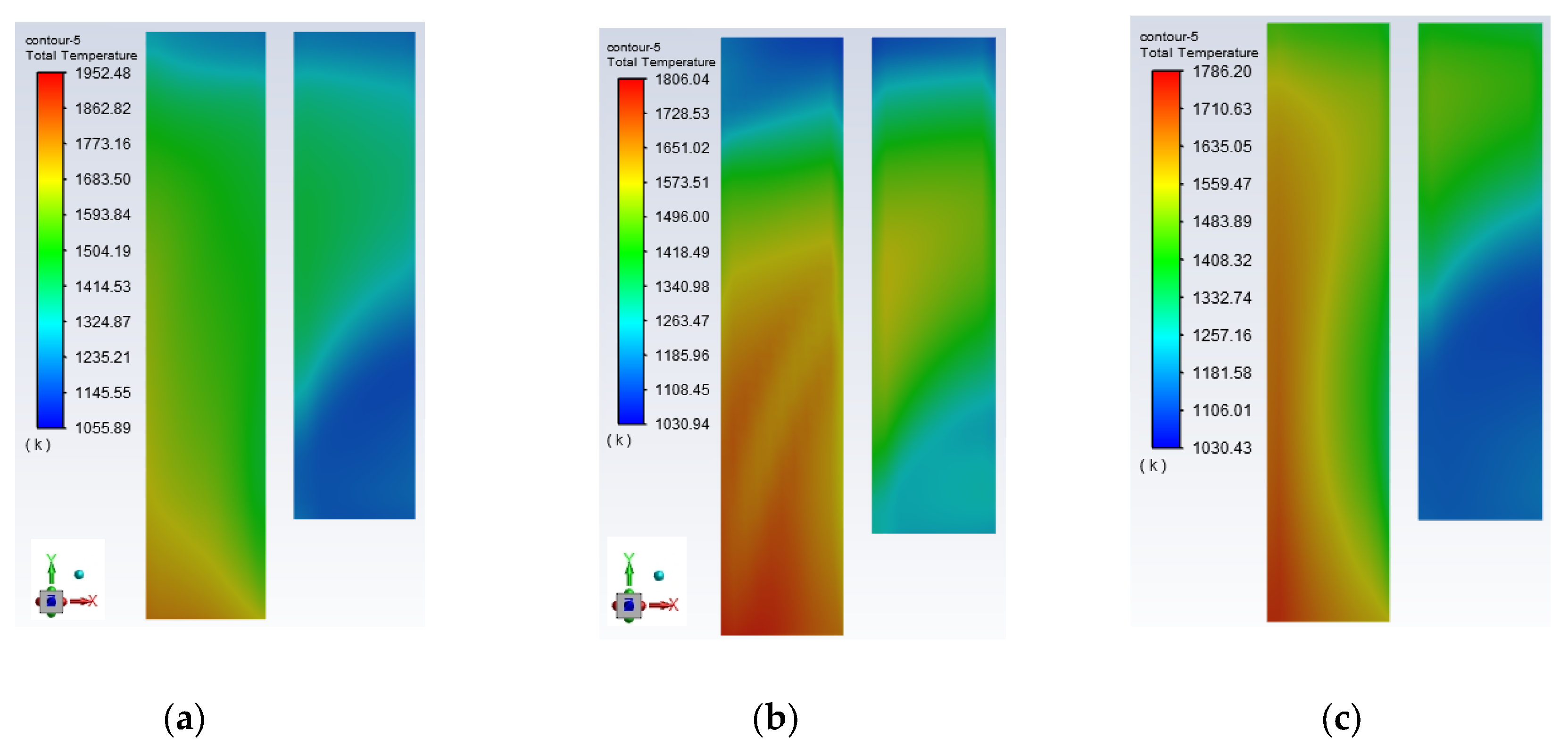
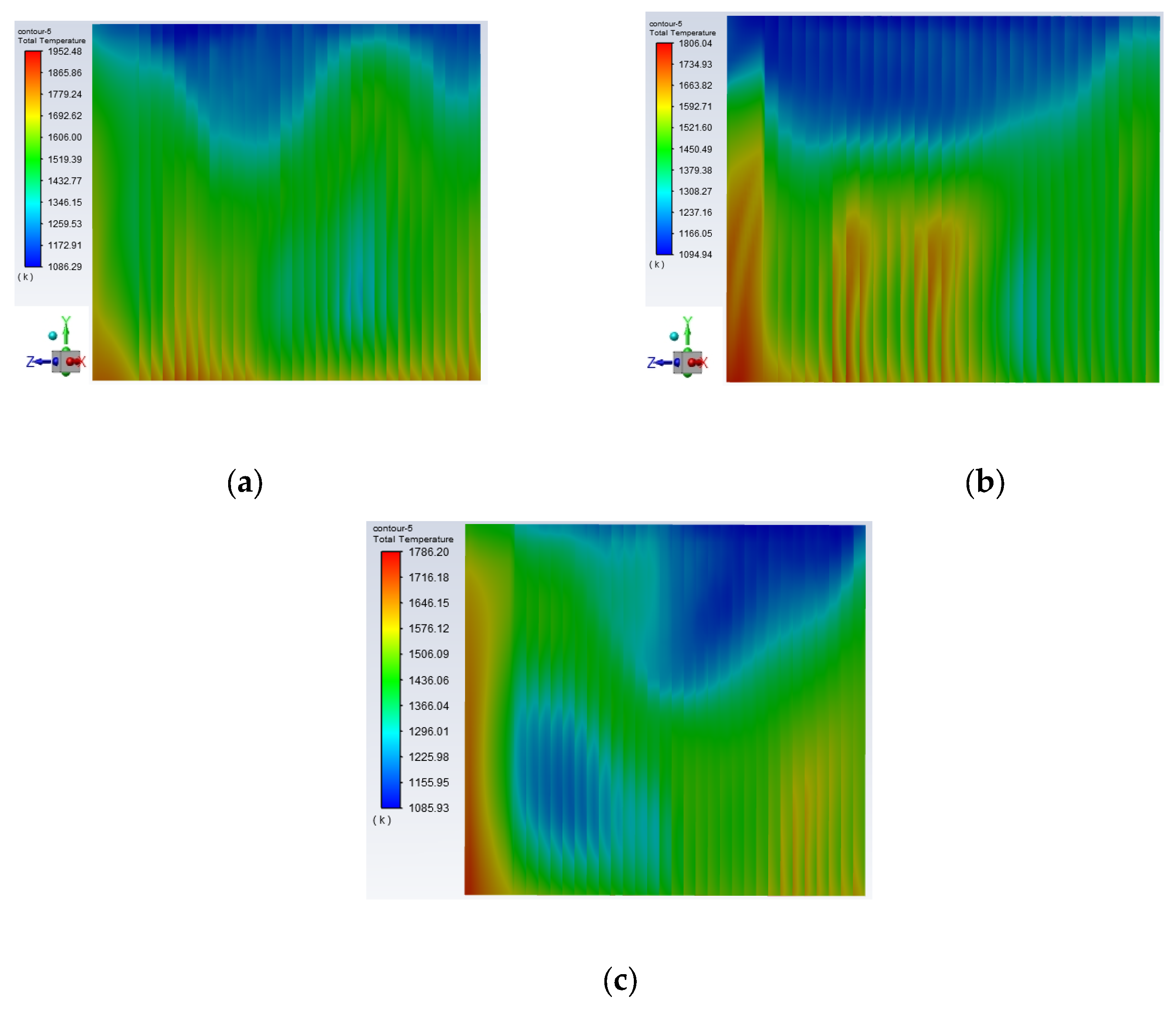
4.3.1. Temperature Distribution Analysis of Heating Surfaces 1 and 2 under Different Loads
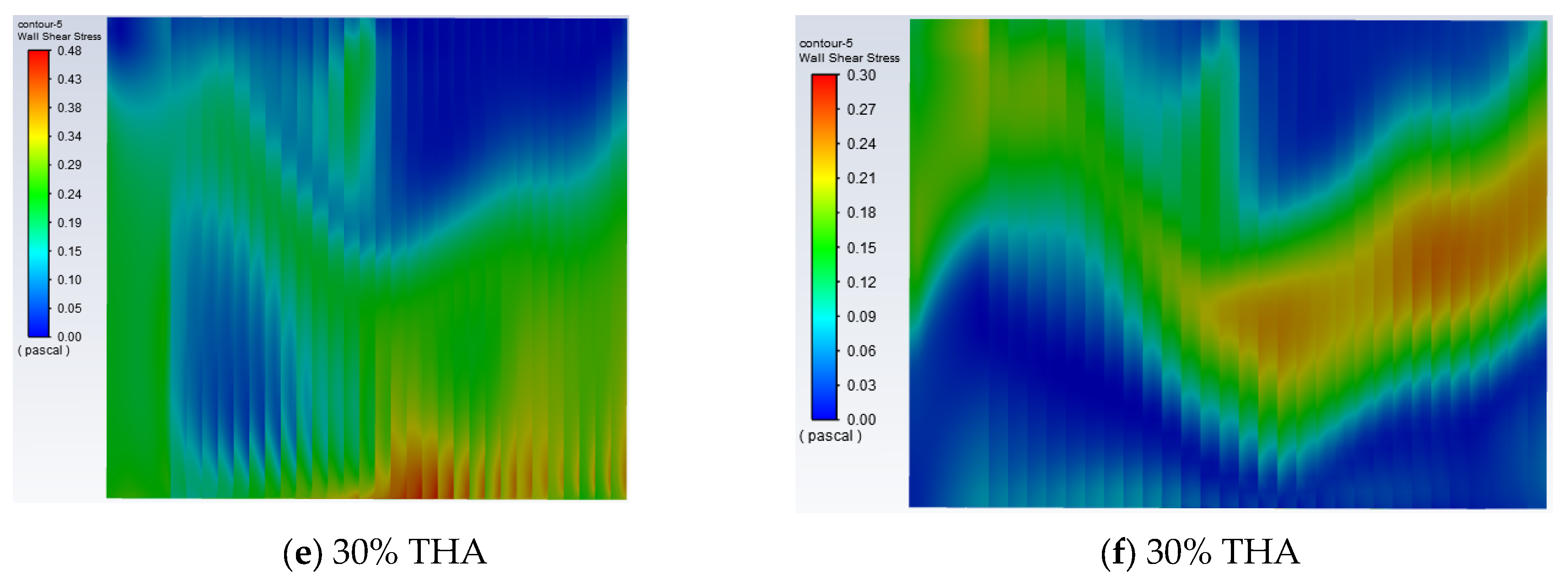
4.3.2. Stress Distributions of Heating Surfaces under Different Loads
5. Conclusions
- (1)
- In a supercritical opposed wall-fired boiler under high load conditions, the furnace flame is filled with a high degree of uniform temperature distribution in the furnace chamber, the distance of the fuel ignition point from the burner outlet of each layer is shorter, the fuel comes from the primary air outlet after rapid combustion, and the pulverized coal combustion rate is high. When the load is reduced, the furnace flame is filled with a reduced degree of narrowing of the combustion area. The burner can effectively combust the fuel under high loads, but the temperature inside the furnace chamber is too high when the load is high, so it is necessary to have burner protection measures to prevent the burner exit temperature from being too high and damaging the burner. When the load is lowered, the flame is close to the left and right walls of the furnace chamber, so there are certain requirements for the temperature resistance of the left and right walls and their water-cooled walls.
- (2)
- Upon reducing the operational load of the boiler, several notable changes are observed. Firstly, the outlet temperature of the furnace experiences a decrease, transitioning from 1158 K to 1009 K. Concurrently, the average temperature within the platen superheater area decreases from 1800 K to 1570 K. With the decrease of the airflow velocity in the furnace, the enrolling capacity of the swirling secondary air on the high-temperature flue gas and the mixing capacity of the exhaust air on the rising flue gas are gradually reduced. Consequently, these changes result in a gradual decrease in temperature within the upper region of the furnace, facilitating the progressive concentration of the high-temperature flame on the operating burner within the main combustion zone.
- (3)
- At elevated operational loads, the temperature distribution across the heating surface 1 exhibits uniformity, primarily around 1600 K. Notably, the bottom platen registers notably high temperatures, with the maximum temperature reaching 1900 K. However, as the load diminishes, a notable shift occurs in the temperature distribution across heating surface 1. Specifically, an uneven temperature distribution emerges, marked by a substantial temperature gradient between the middle and lower segments, with the maximum temperature differential reaching 400 K. This transition precipitates a significant surge in stress within this region, consequently yielding excessive expansion of heating surface 1. In the case of heating surface 2, the higher-temperature region predominantly occupies the central segment, with an overall temperature level inferior to that of heating surface 1. Remarkably, the temperature distribution maintains relative uniformity during load variations, with the maximum stress manifesting in the central region of heating surface 2.
- (4)
- Under high-load conditions, the focal point of maximum heat flux within the furnace wall primarily resides in the sidewall of the primary combustion region. Notably, the burner area and the upper section of the burner receive a substantial heat flux. However, as the operational load decreases, the heat flux gradually converges toward the main combustion zone. In the range of 75% to 50% load, the heat absorption within the main combustion zone remains relatively constant. In the platen superheater area, when the load is reduced, the radiation heat absorption gradually decreases and the convection heat absorption increases.
Author Contributions
Funding
Conflicts of Interest
References
- Choi, H.; Choi, Y.; Moon, U.-C.; Lee, K.Y. Supplementary Control of Conventional Coordinated Control for 1000 MW Ultra-Supercritical Thermal Power Plant Using One-Step Ahead Control. Energies 2023, 16, 6197. [Google Scholar] [CrossRef]
- Hu, P.; Cao, C.; Dai, S. Optimal dispatch of combined heat and power units based on particle swarm optimization with genetic algorithm. AIP Adv. 2020, 10, 045008. [Google Scholar] [CrossRef]
- Wang, J.; Yang, J.; Yang, F.; Cheng, F. Numerical and Experimental Investigation of the Decoupling Combustion Characteristics of a Burner with Flame Stabilizer. Energies 2023, 16, 4474. [Google Scholar] [CrossRef]
- Ning, X.; Yue, Y.; Huang, J.; Ding, H.; Li, B.; Deng, L.; Li, Y. Numerical study on optimization of secondary air box in a 600 MW opposed wall-fired boiler. AIP Adv. 2023, 13, 105119. [Google Scholar] [CrossRef]
- Xie, X.-Q.; Yang, J.-G.; Zhu, C.-Y.; Liu, C.-H.; Zhao, H.; Wang, Z.-H. Numerical analysis of reasons for the CO distribution in an opposite-wall-firing furnace. J. Zhejiang Univ. Sci. A 2020, 21, 193–208. [Google Scholar] [CrossRef]
- Nawaz, M.; Hamzaoui, M.; Bukhari, M.D.; Mustafa, G.; Khan, S.U.; Abdelkarim, A.; Kolsi, L. A numerical study on the superheater tubes bundle of a 660 MW coal-fired supercritical boiler. Int. J. Mod. Phys. B 2023, 2450226. [Google Scholar] [CrossRef]
- Li, X.; Zeng, L.; Zhang, N.; Chen, Z.; Li, Z.; Qin, Y. Effects of the air-staging degree on performances of a supercritical down-fired boiler at low loads: Air/particle flow, combustion, water wall temperature, energy conversion and NOx emissions. Fuel 2022, 308, 121896. [Google Scholar] [CrossRef]
- Wang, H.; Liu, J. Influence of operation of industrial boilers under low load on overheaters. Metall Power. 2001, 6, 47–48. [Google Scholar]
- Kabouris, J.; Kanellos, F.D. Impacts of Large-Scale Wind Penetration on Designing and Operation of Electric Power Systems. IEEE Trans. Sustain. Energy 2010, 1, 107–114. [Google Scholar] [CrossRef]
- Wen, D.; Pan, Y.; Chen, X.; Aziz, M.; Zhou, Q.; Li, N. Analysis and prediction of thermal stress distribution on the membrane wall in the arch-fired boiler based on machine learning technology. Therm. Sci. Eng. Prog. 2022, 28, 101137. [Google Scholar] [CrossRef]
- Pronobis, M. Environmentally Oriented Modernization of Power Boilers; Elsevier: Amsterdam, The Netherlands, 2020. [Google Scholar]
- Li, Z.; Miao, Z.; Shen, X.; Li, J. Effects of momentum ratio and velocity difference on combustion performance in lignite-fired pulverized boiler. Energy 2018, 165, 825–839. [Google Scholar] [CrossRef]
- Liu, H.; Wang, Y.; Zhang, W.; Wang, H.; Deng, L.; Che, D. Coupled modeling of combustion and hydrodynamics for a 1000 MW double-reheat tower-type boiler. Fuel 2019, 255, 115722. [Google Scholar] [CrossRef]
- Yu, C.; Xiong, W.; Ma, H.; Zhou, J.; Si, F.; Jiang, X.; Fang, X. Numerical investigation of combustion optimization in a tangential firing boiler considering steam tube overheating. Appl. Therm. Eng. 2019, 154, 87–101. [Google Scholar] [CrossRef]
- Laubscher, R.; Rousseau, P. CFD study of pulverized coal-fired boiler evaporator and radiant superheaters at varying loads. Appl. Therm. Eng. 2019, 160, 114057. [Google Scholar] [CrossRef]
- Gomez, A.; Fueyo, N.; Diez, L.I. Modelling and simulation of fluid flow and heat transfer in the convective zone of a power-generation boiler. Appl. Therm. Eng. 2008, 28, 532–546. [Google Scholar] [CrossRef]
- Ludowski, P.; Taler, D.; Taler, J. Identification of thermal boundary conditions in heat exchangers of fluidized bed boilers. Appl. Therm. Eng. 2013, 58, 194–204. [Google Scholar] [CrossRef]
- Madejski, P.; Taler, D.; Korzeń, A. Modelling of platen superheaters in a circulating fluidized bed boiler at different loads. Arch. Energ. 2012, 2012, 85–92. [Google Scholar]
- Madejski, P.; Taler, D. Analysis of temperature and stress distribution of superheater tubes after attemperation or sootblower activation. Energy Convers. Manag. 2013, 71, 131–137. [Google Scholar] [CrossRef]
- Modliński, N.; Szczepanek, K.; Nabagło, D.; Madejski, P.; Modliński, Z. Mathematical procedure for predicting tube metal temperature in the second stage reheater of the operating flexibly steam boiler. Appl. Therm. Eng. 2019, 146, 854–865. [Google Scholar] [CrossRef]
- Zima, W.; Nowak-Ocłoń, M.; Ocłoń, P. Simulation of fluid heating in combustion chamber waterwalls of boilers for supercritical steam parameters. Energy 2015, 92, 117–127. [Google Scholar] [CrossRef]
- Luo, R.; Fu, J.; Li, N.; Zhang, Y.; Zhou, Q. Combined control of secondary air flaring angle of burner and air distribution for opposed-firing coal combustion. Appl. Therm. Eng. 2015, 79, 44–53. [Google Scholar] [CrossRef]
- Ameri, M.; Shamshirgaran, S.R. A case study: The effects of the design factors on the thermal profile of Shahid Rajaiee boiler. Appl. Therm. Eng. 2008, 28, 955–961. [Google Scholar] [CrossRef]
- Fu, J.; Zhou, Q.; Li, N.; Liu, Z.; Liu, T. Effects of external stresses on hot corrosion behavior of stainless steel TP347HFG. Corros. Sci. 2016, 104, 103–111. [Google Scholar] [CrossRef]
- Fu, J.; Li, N.; Zhou, Q.; Guo, P. Impacts of applied stresses on high temperature corrosion behavior of HR3C in Molten salt. Oxid. Met. 2015, 83, 317–333. [Google Scholar] [CrossRef]
- Zhong, X.; Wu, X.; Han, E.-H. Effects of exposure temperature and time on corrosion behavior of a ferritic–martensitic steel P92 in aerated supercritical water. Corros. Sci. 2015, 90, 511–521. [Google Scholar] [CrossRef]
- Gruber, T.; Schulze, K.; Scharler, R.; Obernberger, I. Investigation of the corrosion behaviour of 13CrMo4–5 for biomass fired boilers with coupled online corrosion and deposit probe measurements. Fuel 2015, 144, 15–24. [Google Scholar] [CrossRef]
- Wu, X.; Fan, W.; Liu, Y.; Bian, B. Numerical simulation research on the unique thermal deviation in a 1000 MW tower type boiler. Energy 2019, 173, 1006–1020. [Google Scholar] [CrossRef]
- Madejski, P. Numerical study of a large-scale pulverized coal-fired boiler operation using CFD modeling based on the probability density function method. Appl. Therm. Eng. 2018, 145, 352–363. [Google Scholar] [CrossRef]
- Tan, P.; Fang, Q.; Zhao, S.; Yin, C.; Zhang, C.; Zhao, H.; Chen, G. Causes and mitigation of gas temperature deviation in tangentially fired tower-typeboilers. Appl. Therm. Eng. 2018, 139, 135–143. [Google Scholar] [CrossRef]
- Laubscher, R.; Rousseau, P. Coupled simulation and validation of a utility-scale pulverized coal-fired boiler radiant final-stage superheater. Therm. Sci. Eng. Prog. 2020, 18, 100512. [Google Scholar] [CrossRef]
- Zheng, S.; Luo, Z.; Deng, Y.; Zhou, H. Development of a distributed parameter model for the evaporation system in a super-critical W-shaped boiler. Appl. Therm. Eng. 2014, 62, 123–132. [Google Scholar]
- Shih, T.H.; Liou, W.W.; Shabbir, A.; Yang, Z.; Zhu, J. A new k-ε eddy viscosity model for high Reynolds number turbulent flows. Comput. Fluids 1995, 24, 227–238. [Google Scholar] [CrossRef]
- Wang, Y.Q.; Zhou, Y.G. Numerical optimization of the influence of multiple deep air-staged combustion on the NOx emission in an opposed firing utility boiler using lean coal. Fuel 2020, 269, 116996. [Google Scholar] [CrossRef]
- Coelho, P.J. Numerical simulation of radiative heat transfer from non-gray gases in three-dimensional enclosures. J. Quant. Spectrosc. Radiat. Trans. 2002, 74, 307–328. [Google Scholar] [CrossRef]
- Zhou, Z.; Han, X.; Jin, G.; Wang, X.; Yu, J.; Shan, S. New coefficients of the weighted-sum-of-gray-gases model for gas radiation characteristics of hydrogen/natural gas blends combustion. Int. Commun. Heat Mass Transf. 2023, 149, 107090. [Google Scholar] [CrossRef]
- Kobayashi, H.; Howard, J.B.; Sarofim, A.F. Coal devolatilization at high temperatures. Symp. Combust. 1977, 16, 411–425. [Google Scholar] [CrossRef]
- Li, Y.; Zhu, Q.; Kong, D.; Han, H.; Kang, Y.; Liang, J.; Zhao, W.; Zhu, M.; Huang, S. Numerical Simulation of Ash and Slag Deposition in Supercritical Tangential Boiler. In Proceedings of the 2023 International Conference on Smart Electrical Grid and Renewable Energy (SEGRE), Changsha, China, 16–19 June 2023. [Google Scholar]
- Wang, H.P.; Jin, H.Z.; Yang, Z.; Deng, S.S.; Wu, X.H.; An, J.X.; Sheng, R.R.; Ti, S.G. CFD modeling of flow, combustion and NOx emission in a wall-fired boiler at different low-load operating conditions. Appl. Therm. Eng. 2024, 236, 121824. [Google Scholar] [CrossRef]
- Lage, M.; Lopes, H.; da Silveira Carvalho, M. Flows with suspended and floating particles. J. Comput. Phys. 2011, 230, 7736–7754. [Google Scholar] [CrossRef]
- Hu, H.H. Direct simulation of flows of solid–liquid mixtures. Int. J. Multiph. Flow 1996, 22, 335–352. [Google Scholar] [CrossRef]
- Hu, H.H.; Joseph, D.D.; Crochet, M.J. Direct simulation of fluid particle motions. Theor. Comput. Fluid Dyn. 1992, 3, 285–306. [Google Scholar] [CrossRef]
- Liu, F.; Consalvi, J.-L.; Coelho, P.J.; Andre, F.; Gu, M.; Solovjov, V.; Webb, B.W. The impact of radiative heat transfer in combustion processes and its modeling—With a focus on turbulent flames. Fuel 2020, 281, 118555. [Google Scholar] [CrossRef]
- Viskanta, R. Radiative Transfer in Combustion Systems: Fundamentals and Applications; Begell House: New York, NY, USA, 2005. [Google Scholar]
- Modest, M.F. Radiative Heat Transfer, 2nd ed.; Academic Press: New York, NY, USA, 2003. [Google Scholar]
- Dombrovsky, L.A. Radiation Heat Transfer in Disperse Systems; Begell House: New York, NY, USA, 1996. [Google Scholar]
- Dombrovsky, L.A.; Baillis, D. Thermal Radiation in Disperse Systems: An Engineering Approach; Begell House: New York, NY, USA, 2010. [Google Scholar]
- Kang, W.; Jo, H.; Lee, J.; Jang, K.; Ryu, C. Numerical investigations on overfire air design for improved boiler operation and lower NOx emission in commercial wall-firing coal power plants. Appl. Therm. Eng. 2023, 219, 119604. [Google Scholar] [CrossRef]
- Zhang, Y.; Liu, Y.; Yang, X.; Chen, G.; Jin, B. Numerical investigation on optimization of wall jet to reduce high temperature corrosion in 660 MW opposed wall fired boiler. Int. J. Chem. React. Eng. 2022, 20, 305–323. [Google Scholar] [CrossRef]

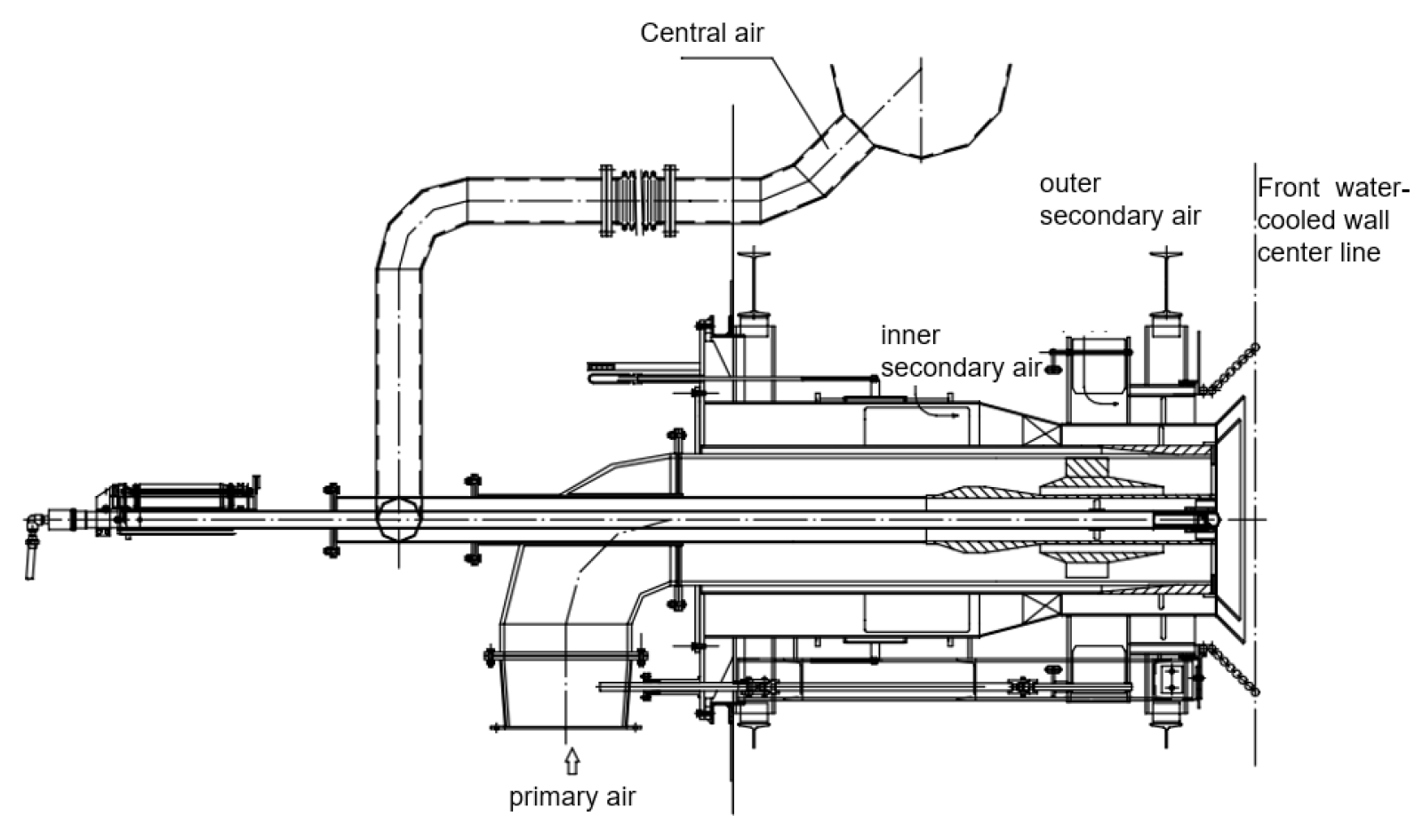

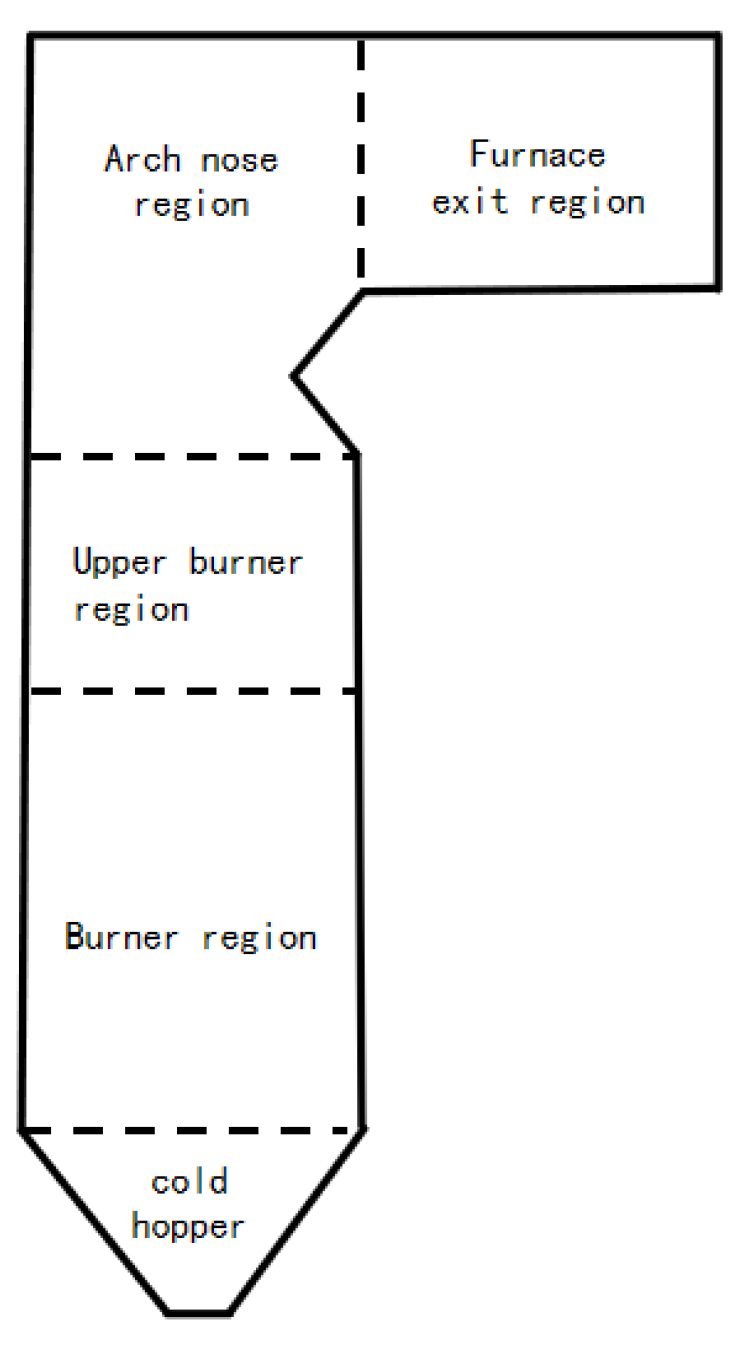
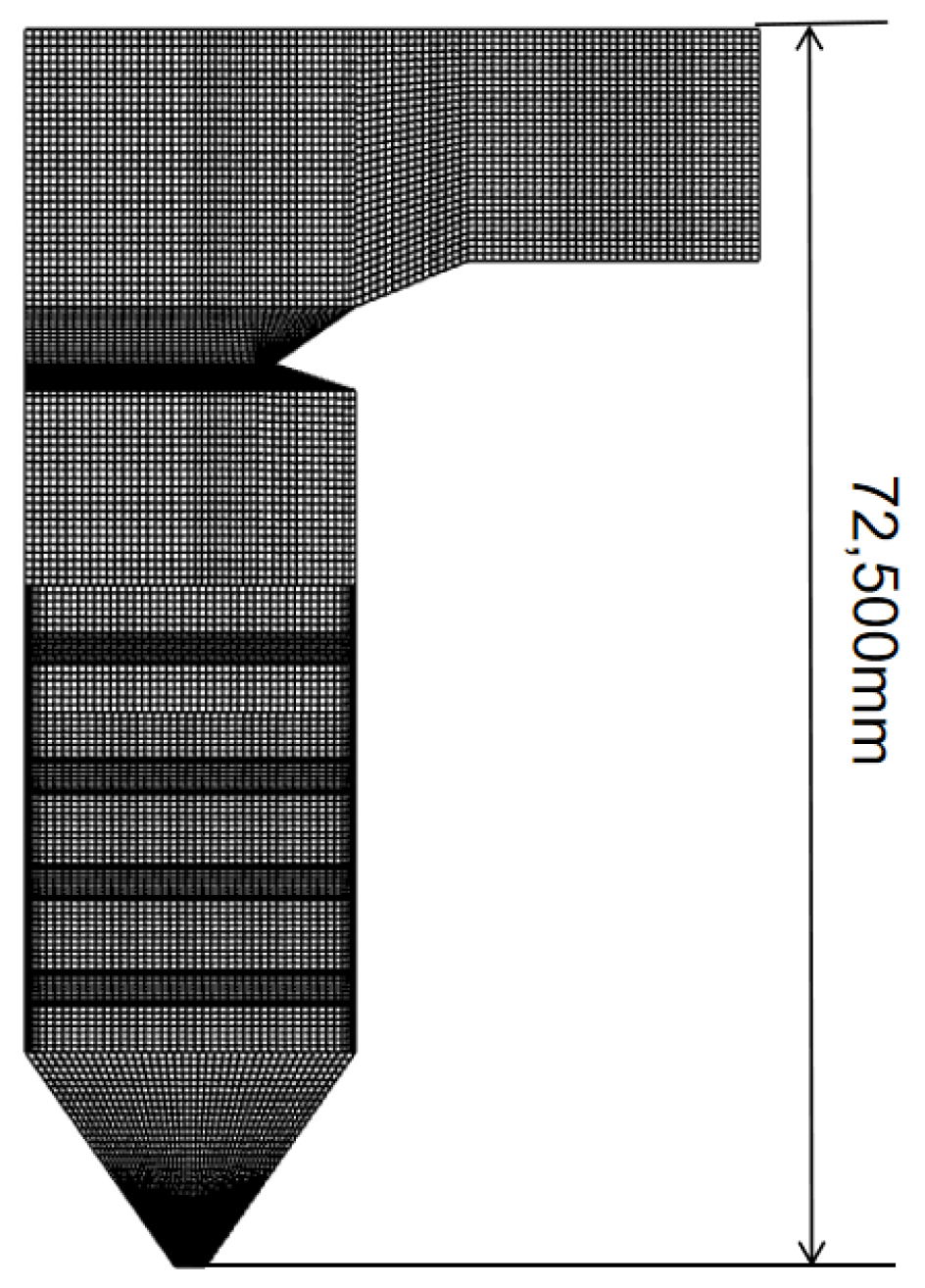
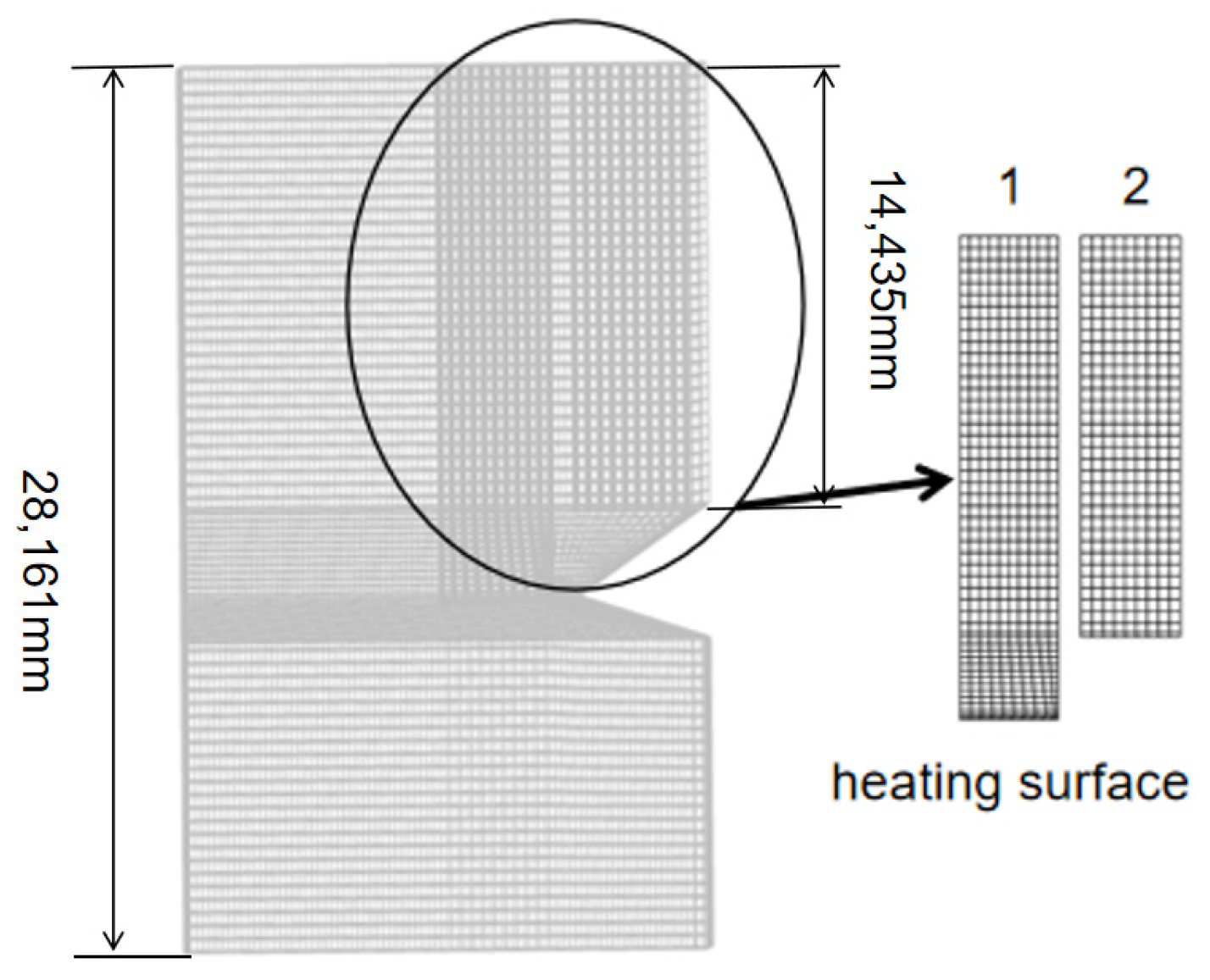


| Name | Unit | B-MCR (Boiler Maximum Continuous Rating) | BRL (Boiler Rated Load) |
|---|---|---|---|
| Superheated Steam Flow Rate | t h−1 | 2025 | 1913.1 |
| Superheater outlet steam pressure | MPa | 25.4 | 25.26 |
| Superheater Outlet Steam Temperature | °C | 571 | 571 |
| Reheat Steam Flow Rate | t h−1 | 1712.8 | 1621 |
| Reheater inlet steam pressure | MPa | 4.66 | 4.42 |
| Reheater Outlet Steam Pressure | MPa | 4.46 | 4.23 |
| Reheater Inlet Steam Temperature | °C | 324 | 318 |
| Reheater Outlet Steam Temperature | °C | 569 | 569 |
| Economizer inlet feedwater temperature | °C | 283 | 279 |
| Name | Primary Air | Internal Secondary Air | Outer Secondary Air | Center Air | Overfire Air 1 | Overfire Air 2 |
|---|---|---|---|---|---|---|
| Duct equivalent diameter (m) | 0.597 | 0.178 | 0.207 | 0.105 | 0.588 | 0.172 |
| Element | Cdaf | Hdaf | Odaf | Ndaf | Sdaf |
|---|---|---|---|---|---|
| Daf (%) | 77.94 | 5.41 | 14.5 | 0.95 | 1.2 |
| Name | FCar | Var | Mar | Aar | Qar,net |
| Percentage (%) | 29.27 | 34.09 | 19.00 | 17.64 | 18.41 (MJ·kg−1) |
| Comparison Items | Measurement Point Value | Numerical Simulation Value | Relative Error (%) |
|---|---|---|---|
| Furnace outlet temperature (K) | 1197 | 1185 | 1.02 |
| Outlet temperature in the area of arch nose (K) | 1687 | 1664 | 1.38 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Du, J.; Li, Y.; Zhao, Y.; Da, Y.; Che, D. Numerical Study of Supercritical Opposed Wall-Fired Boiler Furnace Temperature and High-Temperature Heating Surface Stress under Variable Load Operation. Energies 2024, 17, 663. https://doi.org/10.3390/en17030663
Du J, Li Y, Zhao Y, Da Y, Che D. Numerical Study of Supercritical Opposed Wall-Fired Boiler Furnace Temperature and High-Temperature Heating Surface Stress under Variable Load Operation. Energies. 2024; 17(3):663. https://doi.org/10.3390/en17030663
Chicago/Turabian StyleDu, Jiajun, Yilong Li, Yonggang Zhao, Yaodong Da, and Defu Che. 2024. "Numerical Study of Supercritical Opposed Wall-Fired Boiler Furnace Temperature and High-Temperature Heating Surface Stress under Variable Load Operation" Energies 17, no. 3: 663. https://doi.org/10.3390/en17030663
APA StyleDu, J., Li, Y., Zhao, Y., Da, Y., & Che, D. (2024). Numerical Study of Supercritical Opposed Wall-Fired Boiler Furnace Temperature and High-Temperature Heating Surface Stress under Variable Load Operation. Energies, 17(3), 663. https://doi.org/10.3390/en17030663





