Thermophysical Modeling of the Vaporization Process in a Motive Nozzle with a Profiled Supersonic Part
Abstract
1. Introduction
2. Materials and Methods
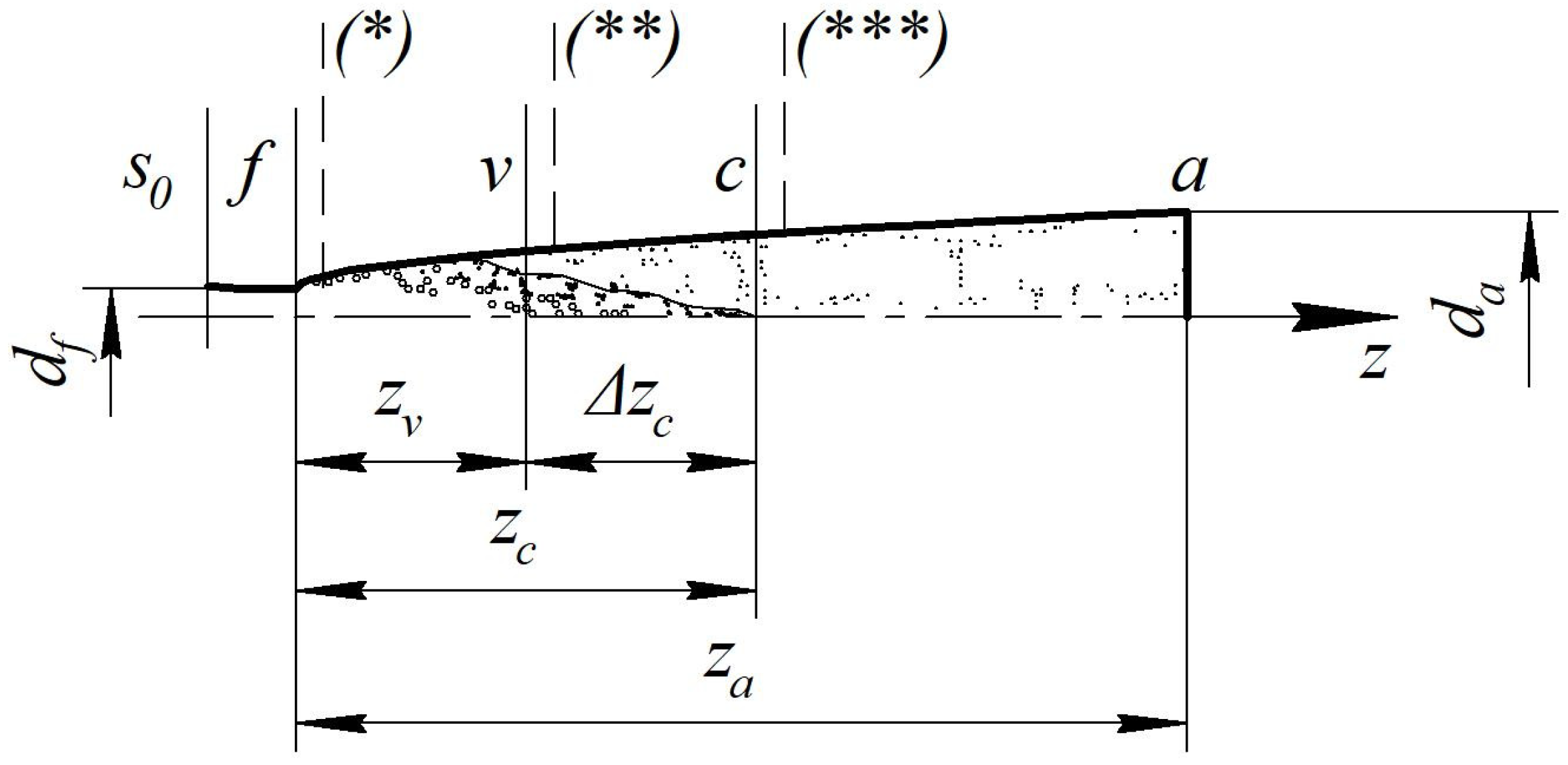
2.1. Design Schemes
2.2. A Mathematical Model
- (1)
- for a conical shape (Figure 1a):
- (2)
- for a parabolic shape (Figure 1b):
- (3)
- for a hyperbolic shape (Figure 1c):
- (4)
- for an elliptic shape (Figure 1d):
- (5)
- for a Vitoshynskyi shape (Figure 1e):
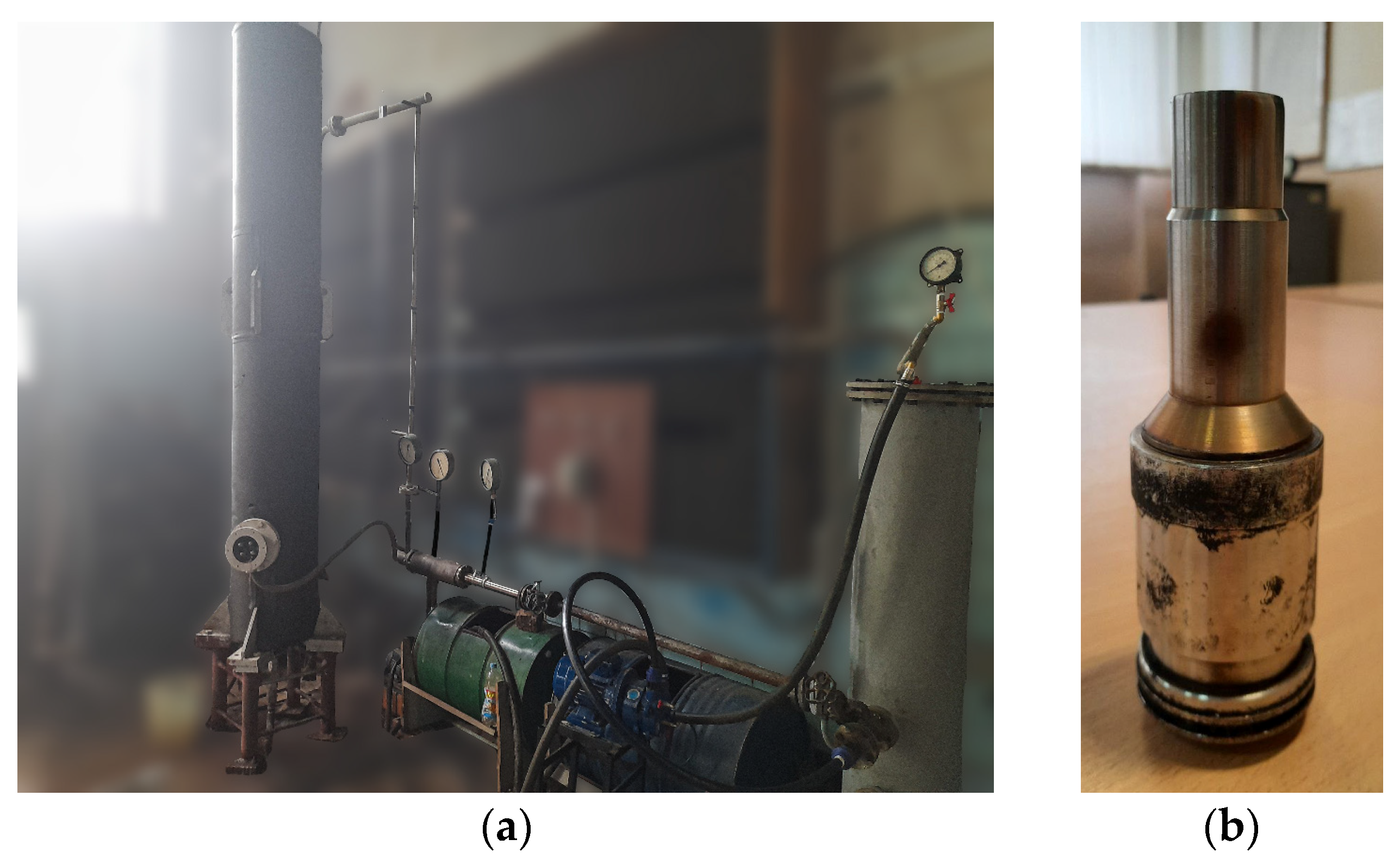
2.3. An Experimental Stand
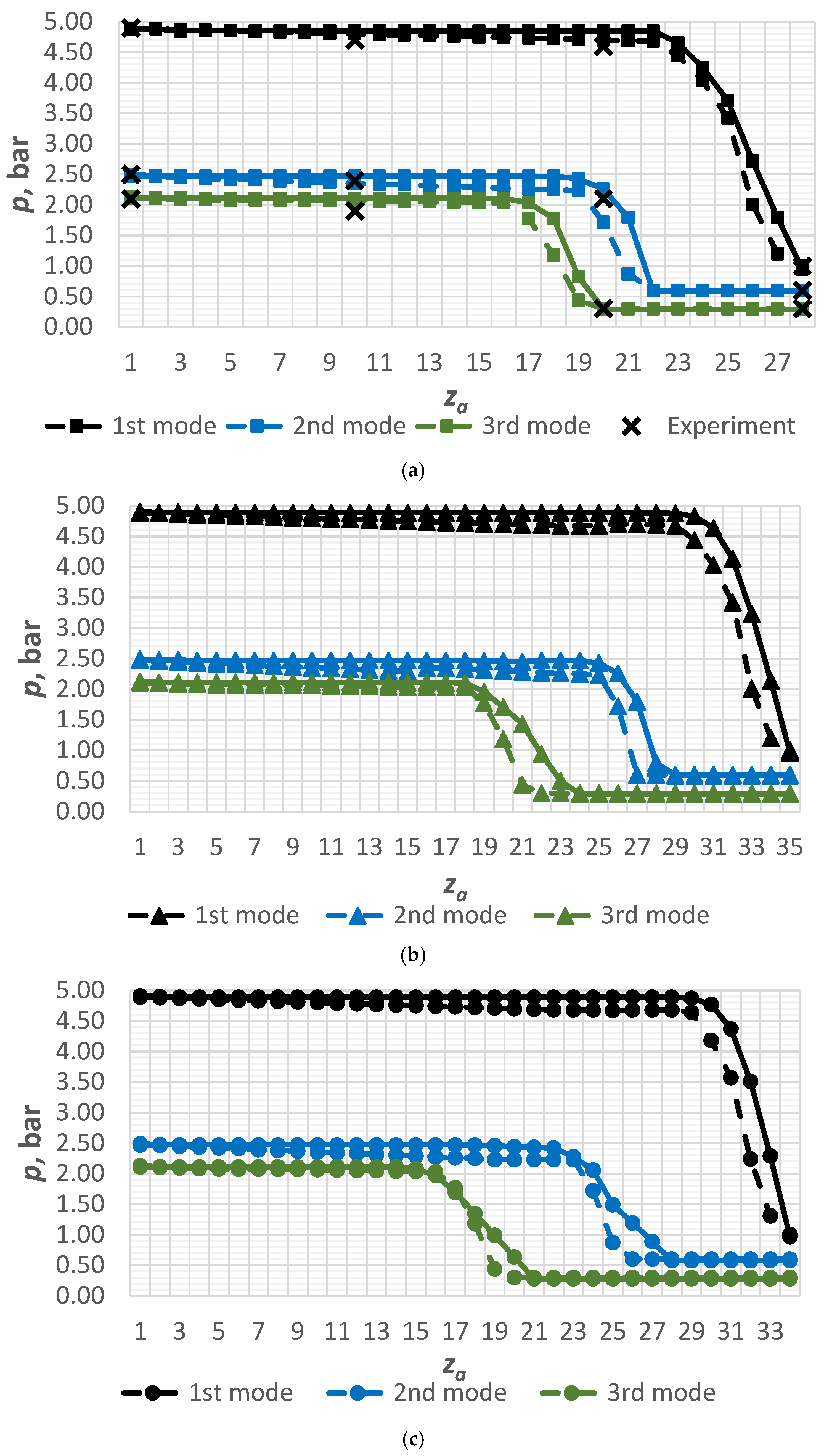
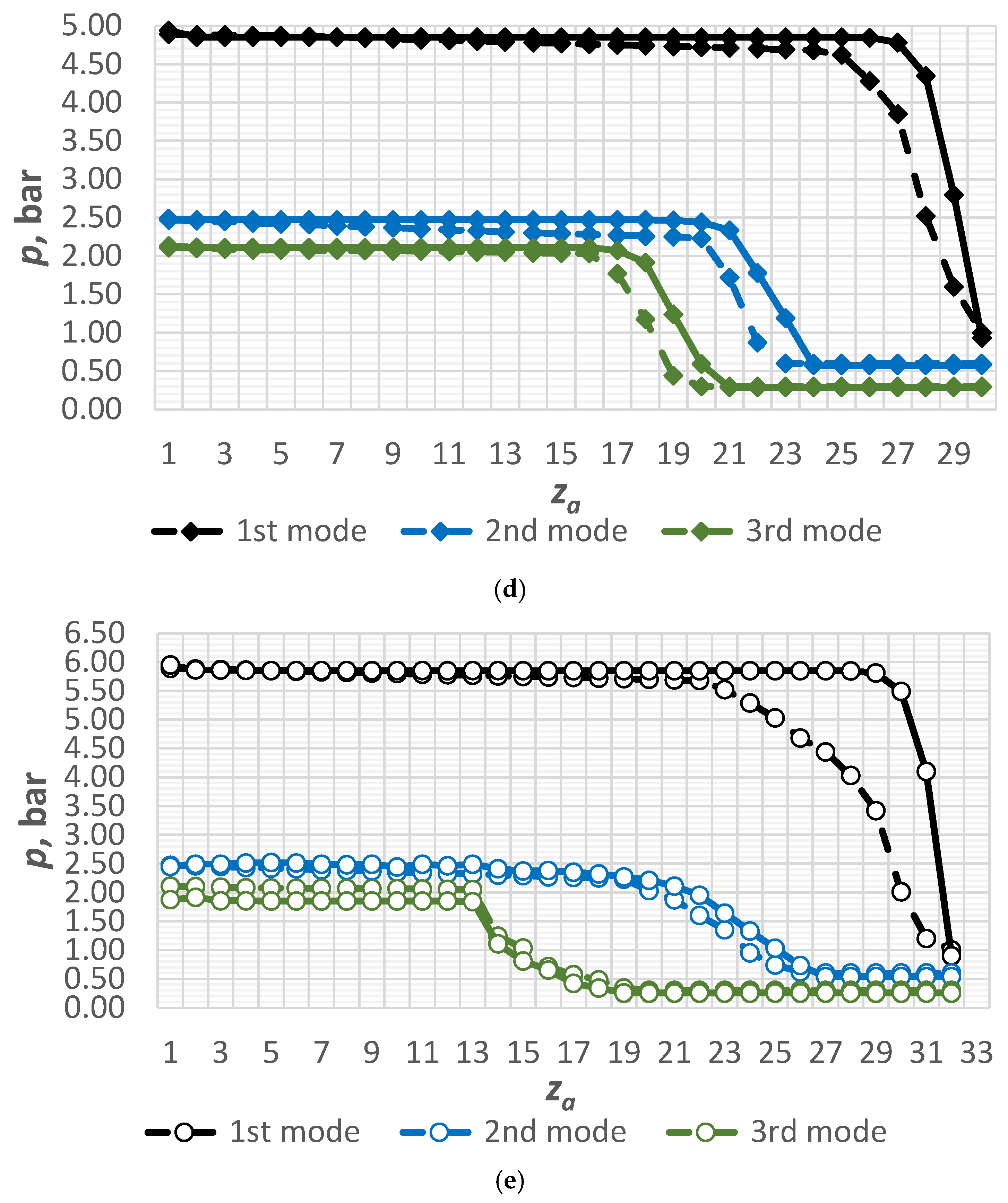
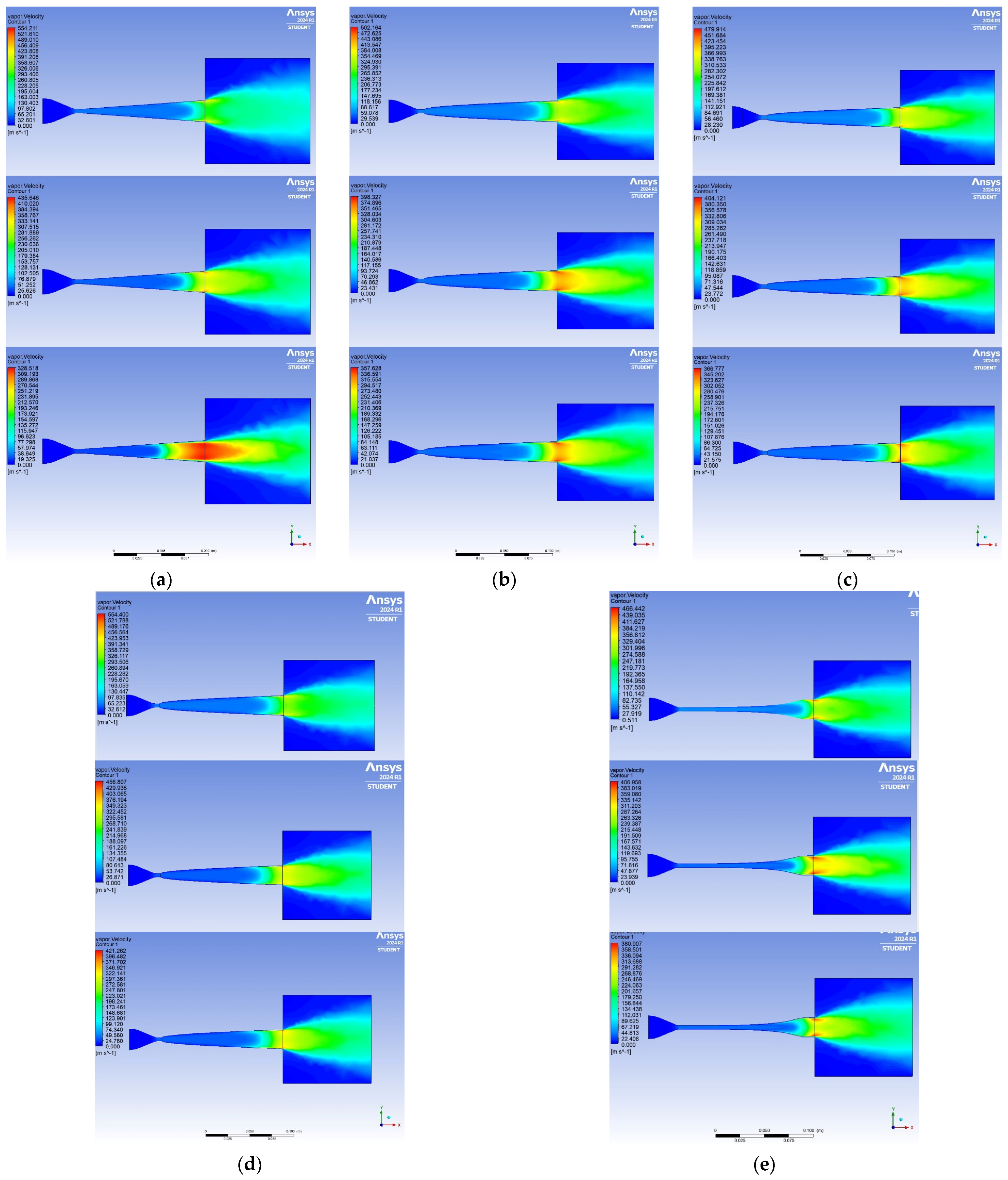
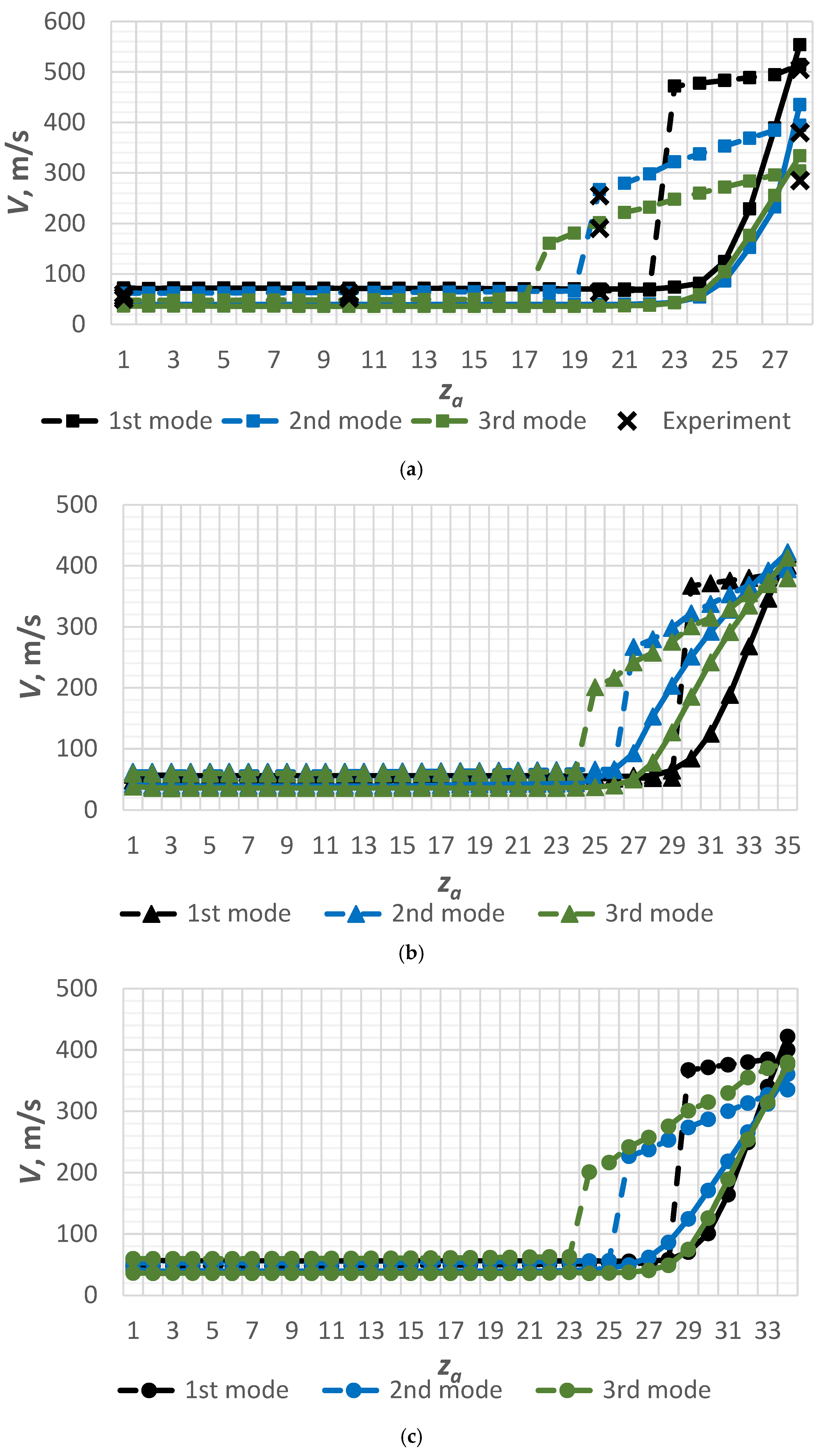
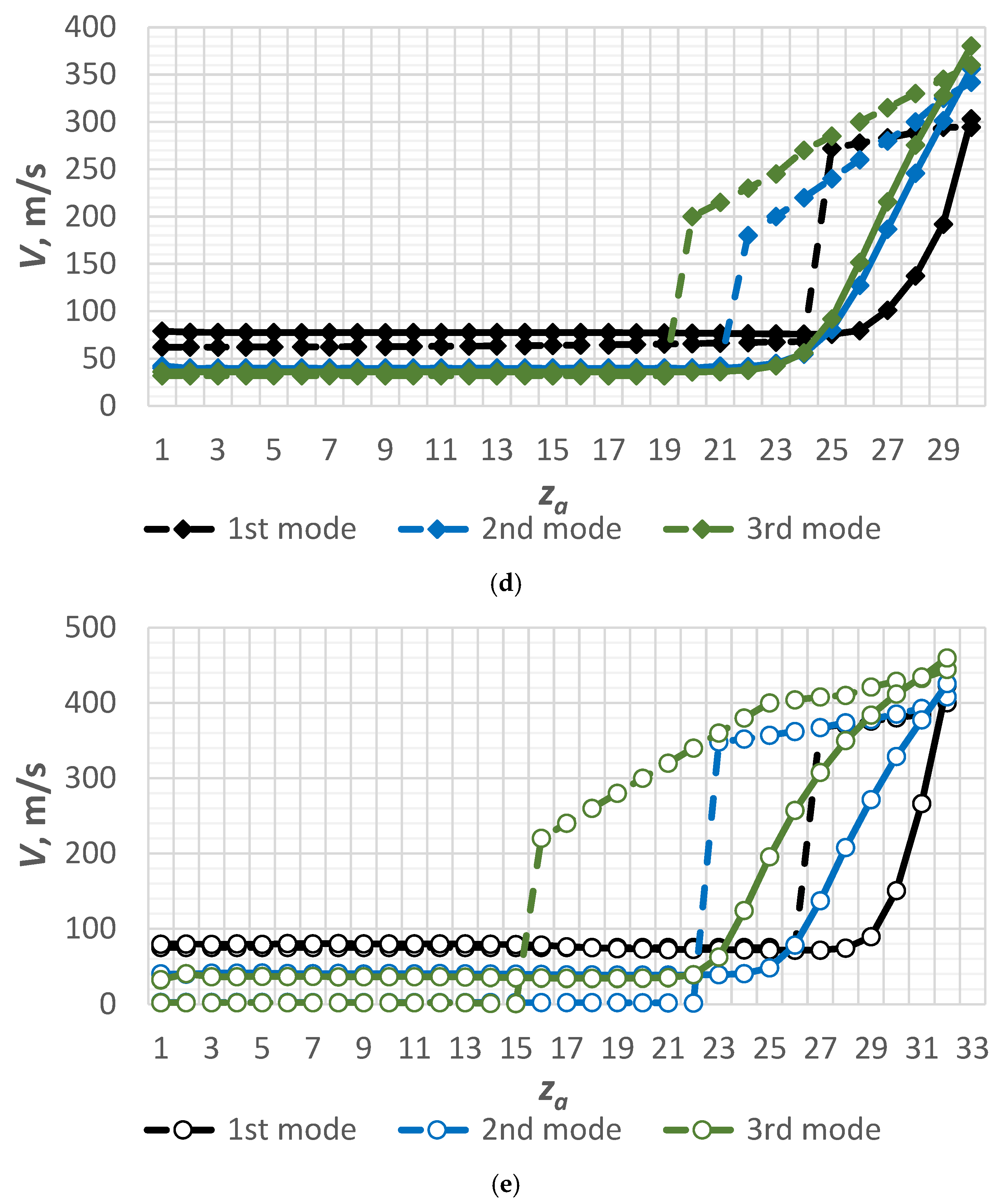
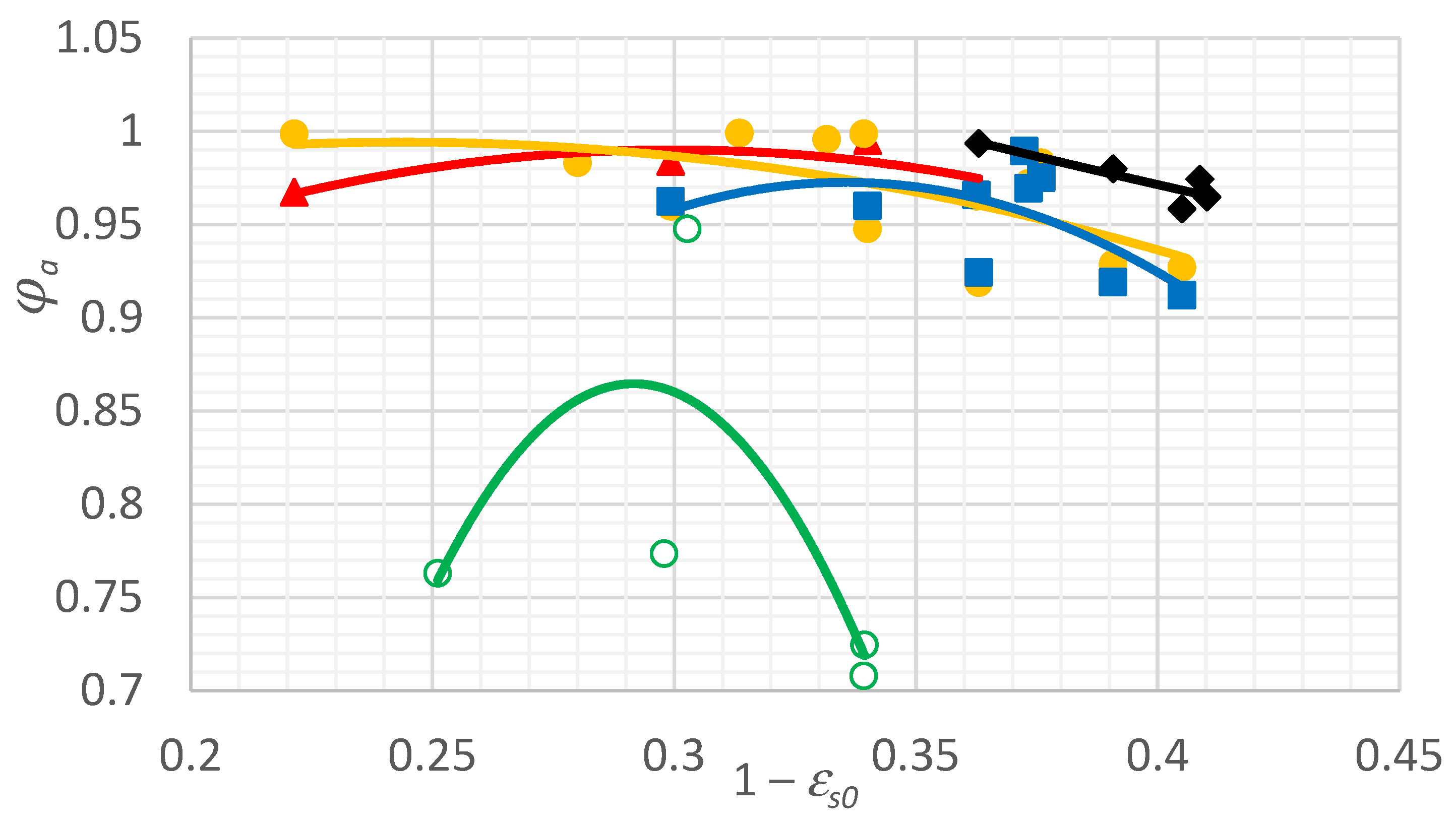
3. Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Tashtoush, B.M.; Al-Nimr, M.A.; Khasawneh, M.A. A comprehensive review of ejector design, performance, and applications. Appl. Energy 2019, 240, 138–172. [Google Scholar] [CrossRef]
- Colarossi, M.; Trask, N.; Schmidt, D.P.; Bergander, M.J. Multidimensional modeling of condensing two-phase ejector flow. Int. J. Refrig. 2012, 35, 290–299. [Google Scholar] [CrossRef]
- Prokopov, M. The Thermophysical Design of Working Process of Liquid-Steam Stream Compressor. Ph.D. Thesis, Sumy State University, Sumy, Ukraine, 2012. [Google Scholar]
- Lorentzen, G. Revival of carbon dioxide as a refrigerant. Int. J. Refrig. 1994, 17, 292–301. [Google Scholar] [CrossRef]
- Liu, J.; Zhou, L.; Cheng, J.; Lin, Z.; Zhang, X. A novel two-stage compression air-source heat pump cycle combining space heating, cooling, and domestic hot water production. Energy Build. 2023, 285, 112863. [Google Scholar] [CrossRef]
- Huang, C.; Li, Z.; Ye, Z.; Wang, R. Thermodynamic study of carbon dioxide transcritical refrigeration cycle with dedicated subcooling and cascade recooling. Int. J. Refrig. 2022, 137, 80–90. [Google Scholar] [CrossRef]
- Welzl, M.; Heberle, F.; Brüggemann, D. Experimental evaluation of nucleate pool boiling heat transfer correlations for R245fa and R1233zd(E) in ORC applications. Renew. Energy 2020, 147, 2855–2864. [Google Scholar] [CrossRef]
- Dai, B.; Liu, S.; Sun, Z.; Ma, Y. Thermodynamic performance analysis of CO2 transcritical refrigeration cycle assisted with mechanical subcooling. Energy Procedia 2017, 105, 2033–2038. [Google Scholar] [CrossRef]
- Sharma, D.K.; Pegallapati, A.S.; Ramgopal, M. Performance of a CO2 based transcritical air conditioning system under summer and winter conditions. Therm. Sci. Eng. Prog. 2023, 41, 101847. [Google Scholar] [CrossRef]
- Mahmoudian, J.; Mazzelli, F.; Milazzo, A.; Malpress, R.; Buttsworth, D. Experiments on water vapour condensation within supersonic nozzle flow generated by an impulse tunnel. Int. J. Multiph. Flow 2021, 134, 103473. [Google Scholar] [CrossRef]
- Sarevski, V.N.; Sarevski, M.N. Characteristics of R718 Thermocompression refrigerating/heat pump systems with two-phase ejectors. In Proceedings of the International Refrigeration and Air Conditioning Conference, West Lafayette, IN, USA, 16–19 July 2012; Available online: https://docs.lib.purdue.edu/iracc/1214/ (accessed on 1 November 2024).
- Sutthivirode, K.; Thongtip, T. Performance improvement of ejector refrigerator–based water chiller working with different mixing chamber profiles. Alex. Eng. J. 2021, 60, 3693–3707. [Google Scholar] [CrossRef]
- Butrymowicz, D.; Śmierciew, K.; Karwacki, J.; Gagan, J. Experimental investigations of low-temperature driven ejection refrigeration cycle operating with isobutane. Int. J. Refrig. 2014, 39, 196–209. [Google Scholar] [CrossRef]
- Butrymowicz, D.; Śmierciew, K.; Karwacki, J.; Borsukiewicz, A.; Gagan, J. Experimental investigations of flow boiling heat transfer under near-critical pressure for selected working fluids. Sustainability 2022, 14, 14029. [Google Scholar] [CrossRef]
- Sharapov, S.; Mižáková, J.; Husiev, D.; Panchenko, V.; Ivanov, V.; Pavlenko, I.; Židek, K. Vapor overproduction condition monitoring in a liquid–vapor ejector. Processes 2022, 10, 2383. [Google Scholar] [CrossRef]
- Majchrzyk, M.; Dziurowicz, D.; Haida, M.; Palacz, M.; Bodys, J.; Fingas, R.; Smolka, J.; Nowak, A. Detailed numerical investigation of the CO2 two-phase ejector 3-D CFD model based on the flow visualisation experiments. Chem. Eng. Process.-Process Intensif. 2022, 182, 109195. [Google Scholar] [CrossRef]
- Chekh, O.; Sharapov, S.; Prokopov, M.; Kozin, V.; Butrymowicz, D. Cavitation in nozzle: The effect of pressure on the vapor content. In Advances in Design, Simulation and Manufacturing II. DSMIE 2019; Ivanov, V., Trojanowska, J., Machado, J., Liaposhchenko, O., Zajac, J., Pavlenko, I., Edl, M., Perakovic, D., Eds.; Lecture Notes in Mechanical Engineering; Springer: Cham, Switzerland, 2020; pp. 522–530. [Google Scholar] [CrossRef]
- Sharapov, S.O.; Krmela, J.; Husiev, D.M.; Verbytskiy, A.R.; Bocko, J. Heat utilization in boiler plants by using liquid-vapor jet apparatus. J. Eng. Sci. 2024, 11, G1–G8. [Google Scholar] [CrossRef]
- Łapka, P.; Seredyński, M.; Grzebielec, A.; Szelągowski, A. Numerical investigation of nozzle shape effect on the flash boiling phenomenon. In Numerical Heat Transfer, Part A: Applications; Taylor & Francis: Abingdon, UK, 2023; pp. 1–23. [Google Scholar] [CrossRef]
- Le, A.D. Study of thermodynamic effect on the mechanism of flashing flow under pressurized hot water by a homogeneous model. J. Fluids Eng. 2022, 144, 011206. [Google Scholar] [CrossRef]
- Kumar, A.; Tammone, C.; Haglind, F. Numerical investigation of flashing flow in a convergent-divergent nozzle. In Turbo Expo: Power for Land, Sea, and Air; American Society of Mechanical Engineers: New York, NY, USA, 2023; Volume 6, p. V006T09A009. [Google Scholar] [CrossRef]
- Liu, Z.; Wang, S.; Lin, Z.; Wang, X.; Wang, D.; Ouyang, Y.; Zhang, D. A non-equilibrium phase change model based on the Lee model for flashing flows in converging–diverging nozzles. Nucl. Eng. Des. 2024, 423, 113185. [Google Scholar] [CrossRef]
- Quang, D.L.; Mereu, R.; Besagni, G.; Dossena, V.; Inzoli, F. Computational fluid dynamics modeling of flashing flow in convergent-divergent nozzle. J. Fluids Eng. 2018, 140, 101102. [Google Scholar] [CrossRef]
- Le, A.D.; Thang, H.P.; The, H.T. Assessment of a homogeneous model for simulating a cavitating flow in water under a wide range of temperatures. J. Fluids Eng. 2021, 143, 101204. [Google Scholar] [CrossRef]
- Hung, K.-S.; Hsiao, W.-C.; Li, Y.-C.; Kuan, Y.-D. 150USRT class R-513A refrigerant two-stage centrifugal compressor design point and separation point flow field simulation analysis. Processes 2023, 11, 253. [Google Scholar] [CrossRef]
- Ayad, F.; Bouaichaoui, Y.; Aboshighiba, H. Fluid-to-fluid similarity and CFD predictions of surrogate fluid as a replacement for R11 refrigerant used in a subcooled flow boiling analysis: Validation against reference experimental data. Int. J. Heat Mass Transf. 2024, 230, 125772. [Google Scholar] [CrossRef]
- Praveen, R.; Kumaran, P.; Anandu, S.; Gnana Gokulprasath, S.; Karthickraja, N. Aerodynamic and flow optimization in turbo charger nozzle using ANSYS CFX. AIP Conf. Proc. 2023, 2523, 020006. [Google Scholar] [CrossRef]



| Mode | pin, bar | tin, °C | pout, bar | m, kg/s |
|---|---|---|---|---|
| 1 | 20 | 200 | 1.00 | 0.83 |
| 2 | 10 | 150 | 0.50 | 0.44 |
| 3 | 6 | 110 | 0.29 | 0.24 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sharapov, S.; Husiev, D.; Klymenko, V.; Pavlenko, I.; Ginter-Kramarczyk, D.; Krupińska, A.; Ochowiak, M.; Włodarczak, S. Thermophysical Modeling of the Vaporization Process in a Motive Nozzle with a Profiled Supersonic Part. Energies 2024, 17, 6465. https://doi.org/10.3390/en17246465
Sharapov S, Husiev D, Klymenko V, Pavlenko I, Ginter-Kramarczyk D, Krupińska A, Ochowiak M, Włodarczak S. Thermophysical Modeling of the Vaporization Process in a Motive Nozzle with a Profiled Supersonic Part. Energies. 2024; 17(24):6465. https://doi.org/10.3390/en17246465
Chicago/Turabian StyleSharapov, Serhii, Danylo Husiev, Volodymyr Klymenko, Ivan Pavlenko, Dobrochna Ginter-Kramarczyk, Andżelika Krupińska, Marek Ochowiak, and Sylwia Włodarczak. 2024. "Thermophysical Modeling of the Vaporization Process in a Motive Nozzle with a Profiled Supersonic Part" Energies 17, no. 24: 6465. https://doi.org/10.3390/en17246465
APA StyleSharapov, S., Husiev, D., Klymenko, V., Pavlenko, I., Ginter-Kramarczyk, D., Krupińska, A., Ochowiak, M., & Włodarczak, S. (2024). Thermophysical Modeling of the Vaporization Process in a Motive Nozzle with a Profiled Supersonic Part. Energies, 17(24), 6465. https://doi.org/10.3390/en17246465









