Analysis of an Axial Field Hybrid Excitation Synchronous Generator

Abstract

An axial field hybrid excitation synchronous generator (AF-HESG) is proposed for an independent power supply system, and its electromagnetic performance is studied in this paper. The distinguishing feature of the proposed generator is the addition of static magnetic bridges at both ends to place the field windings and the use of a sloping surface to increase the additional air-gap cross-sectional area. The advantage of the structure is that it achieves brushless excitation and improves the flux-regulation range. The structure and magnetic circuit characteristics are introduced in detail. Theoretical analysis of the flux-regulation principle is conducted by studying the relationship between field magnetomotive force, rotor reluctance, and air-gap flux density. Quantitative calculation is performed using a magnetomotive force (MMF)-specific permeance model, and the influence of the main parameters on the air-gap flux density and flux-regulation range is analyzed. Subsequently, magnetic field, no-load, and load characteristics are investigated through three-dimensional finite element analysis. The loss distribution is analyzed, and the temperature of the generator under rated conditions is simulated. Finally, a 30 kW, 1500 r/min prototype is developed and tested. The test results show good flux-regulation capability and stable voltage output performance of the proposed generator.

1. Introduction

The electric excitation generator has a simple structure and is low cost. By changing the field winding current, the air-gap magnetic field can be easily adjusted, achieving a wide range of output voltage and speed regulation characteristics. Disconnecting the excitation circuit can effectively demagnetize and achieve short-circuit and fault protection of the machine system. However, the field winding loss makes the system efficiency relatively low, and it is difficult to achieve high power density. Moreover, due to the use of a brush and slip ring structure, the reliability is low [1,2].

The permanent magnet (PM) generator eliminates the structure of the brush and slip ring and also eliminates the excitation power, resulting in higher efficiency. Compared with other types of electric excitation machines with the same power and speed, the efficiency can usually be improved by 3–5%. However, it is difficult to adjust the voltage of the permanent magnet generator itself, and it is also difficult to demagnetize during faults. It needs to rely on a converter to achieve voltage regulation [3].

Hybrid excitation technology combines two excitation methods in a reasonable way, combining the advantages of the high-power density of permanent magnet machines and the adjustable magnetic field of electric excitation machines [4]. The development of electrification (such as independent power supply systems) has become the most popular trend to promote energy conservation. The independent power sources are influenced by both the engine and the load. To ensure the stability of power generation systems, the research focus is on generators with wide power regulation ranges and stable voltage output. Domestic and foreign scholars have conducted research on hybrid excitation technology and have made significant progress in the field of independent power generation, such as aircraft, vehicle power supplies, and mobile power supplies [5,6].

In order to realize the brushless excitation technology of the hybrid excitation machine, there are usually three methods based on the placement of PM and field winding. The first is that both the PM and field winding are on the rotor [7,8,9,10], such as two-stage excitation generators and harmonic excitation hybrid excitation generators. This method is equipped with an additional set of windings on the stator or rotor and provides dc power through the rotating rectifier. It has high excitation efficiency and good waveform quality [11]. However, the use of a rotating rectifier limits some applications.

The second is that the PM and the field winding are both on the stator [12,13,14,15,16], such as in a doubly salient hybrid excitation machine. This method has a simple rotor structure without PM and winding. The rotor structure is simple, the mechanical strength is high, and it is easy to achieve a brushless structure. This type of machine has a wide range of application prospects in high-speed situations. In order to ensure effective slot filling, the stator slots need to be deepened. And to ensure that the magnetic circuit of the stator yoke is not saturated, it is necessary to increase the outer diameter of the stator, thereby increasing the volume of the entire generator.

The third is to place the PM on the rotor and the field winding on the stator [17,18,19,20], such as in a dual-direction hybrid excitation synchronous generator [21]. The characteristic of this machine is that the axial flux path passes through the shell and cover. This method has an axial magnetic circuit and an additional air gap by adding the magnetic parts, and the structure is relatively complex, but the excitation method is simple and reliable.

An AF-HESG is presented in this paper, with an additional axial magnetic bridge. The rotor adopts a claw-pole-like structure, with the field winding placed in the axial magnetic bridge, and the field flux path does not pass through the permanent magnet. It achieves brushless excitation. The flux-regulation principle is analyzed based on the relationship between MMF, reluctance, and air-gap flux density. Analytical calculation of air-gap flux density using the MMF-specific permeance model. Finite element analysis is utilized to analyze magnetic field distribution, operating characteristics, and temperature field. Finally, a prototype is constructed and tested to verify the theoretical and simulation results.

2. Machine Topology and Operating Principle

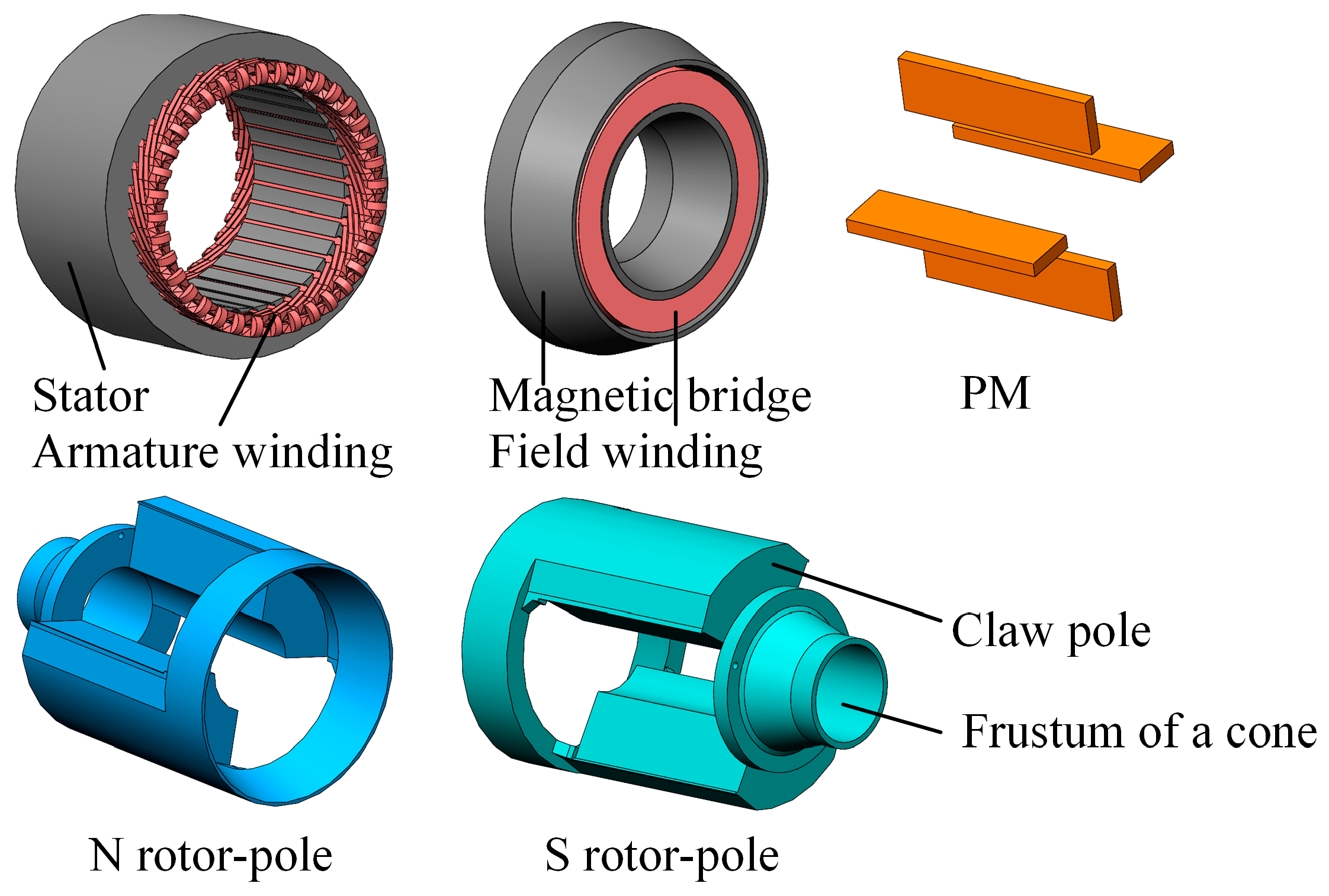
2.1. Topology

The sectional view of the AF-HESG proposed in this paper is shown in Figure 1. And the diagram of various components of the AF-HESG is shown in Figure 2. The stator is mainly composed of a stator core, an armature winding, an axial magnetic bridge with a side opening, and the ring-type field winding. The rotor is mainly composed of an N rotor-pole, an S rotor-pole, and a permanent magnet. The claw pole and the frustum of a cone together form a rotor pole. The permanent magnet is located between the N-pole and S-pole rotor claw poles. In particular, the stator core is made of silicon steel sheet, and the rest are solid steel. The field windings are placed in the opening of the axial magnetic bridge, and there are additional air gaps between the axial magnetic bridge and the rotor. The additional air gap is a sloping surface. The schematic diagram of the generator system is shown in Figure 3. The field windings at both ends are connected in series and powered by a DC power supply, and the armature winding is connected to the load.

Table 1. The structure parameters of AF-HESG.

Parameters	Value
Rated power	30 kW
Rated speed	1500 r/min
Rated phase voltage	220 V
Number of pole pairs	2
Width of PM	40 mm
Thickness of PM	10 mm
Length of stator core	250 mm
Number of stator slots	36
The field current	6 A

shows the structure parameters of the AF-HESG.

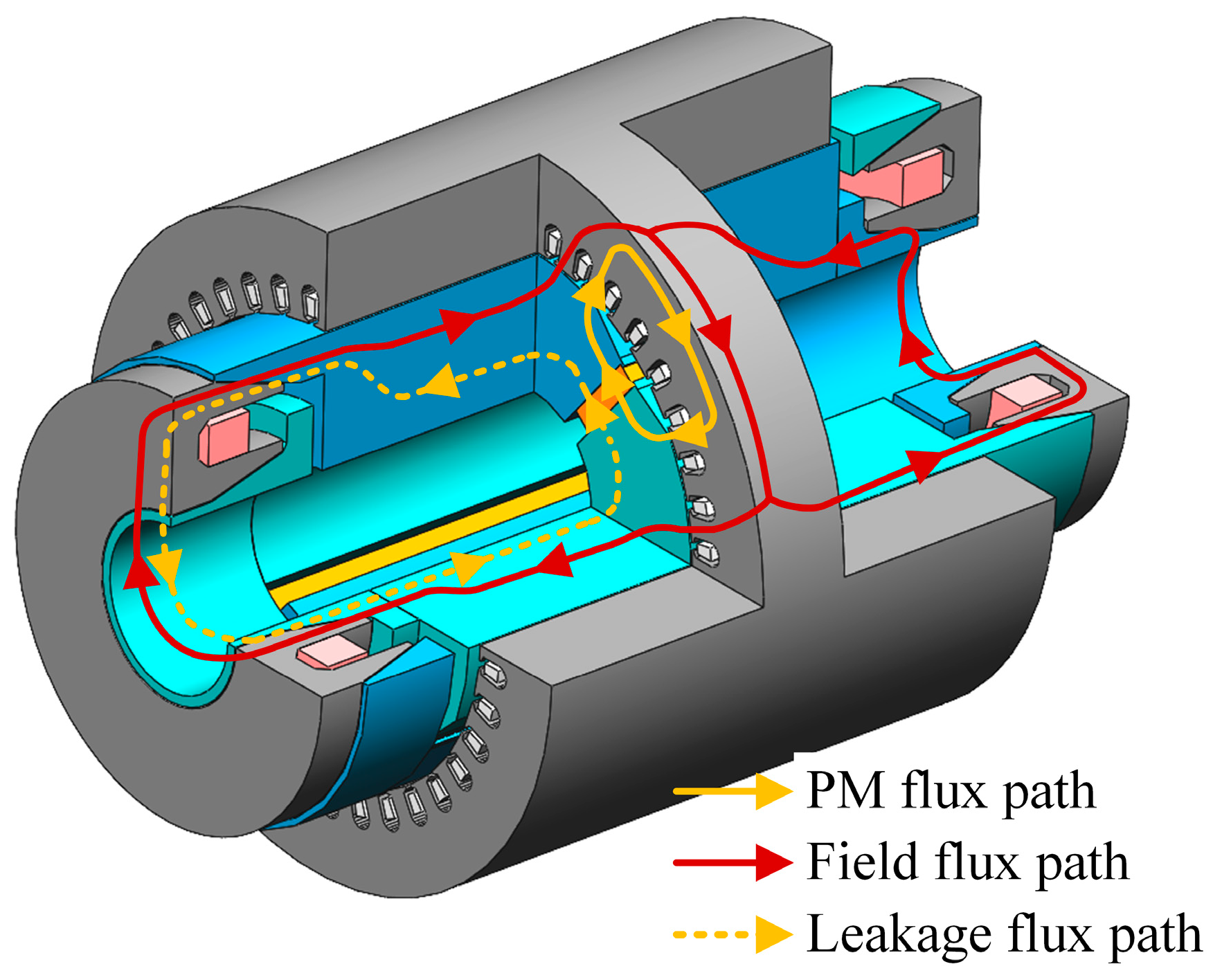
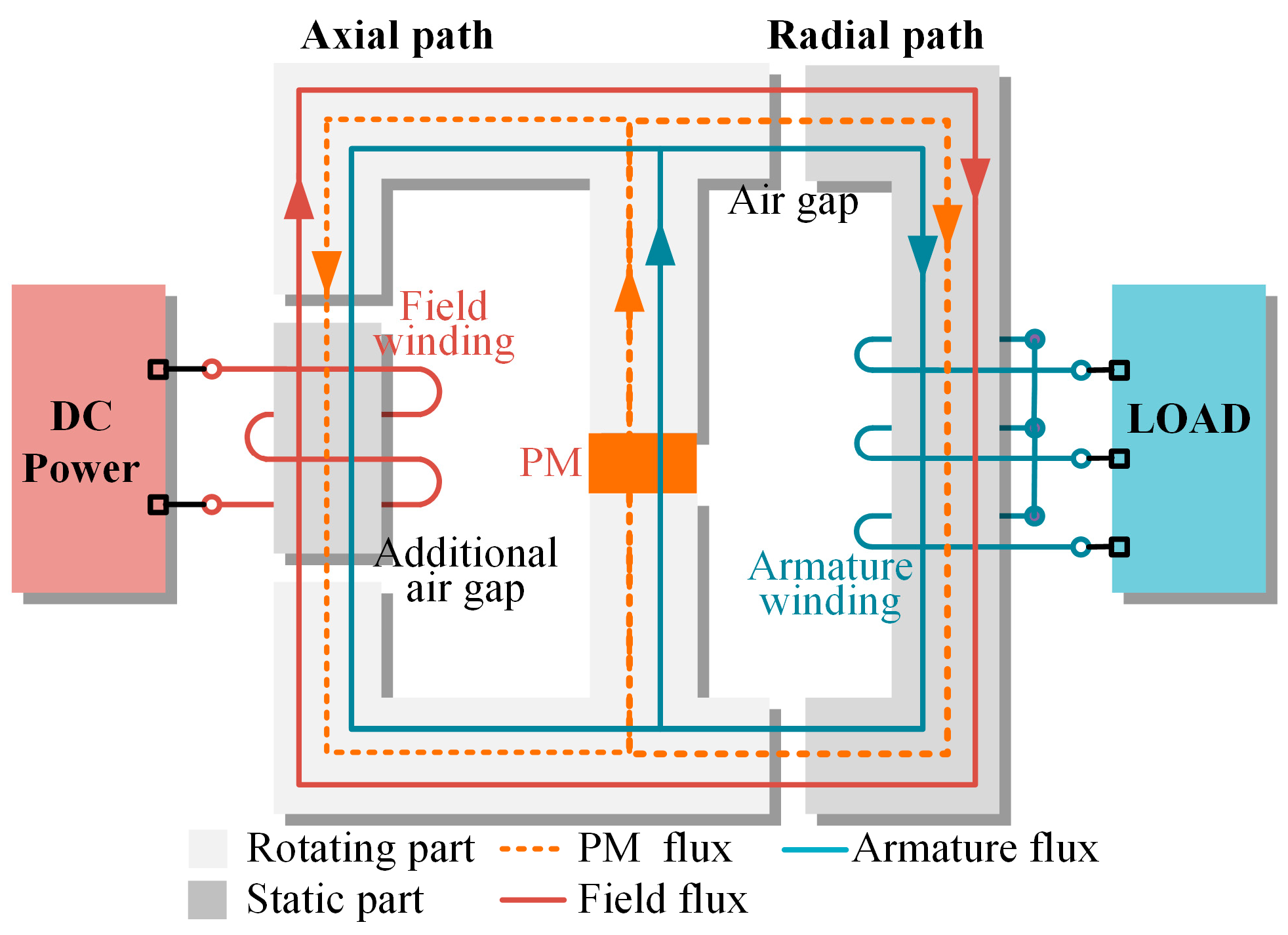
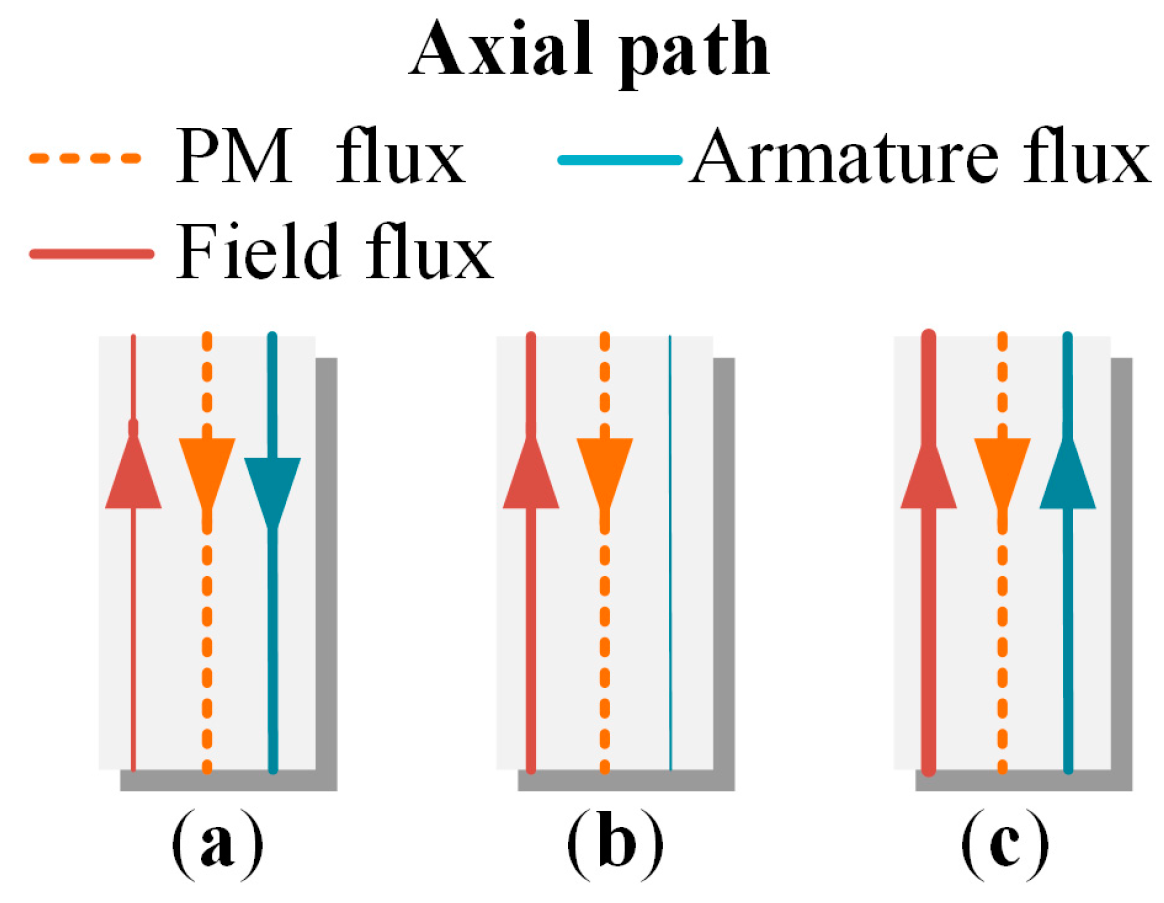
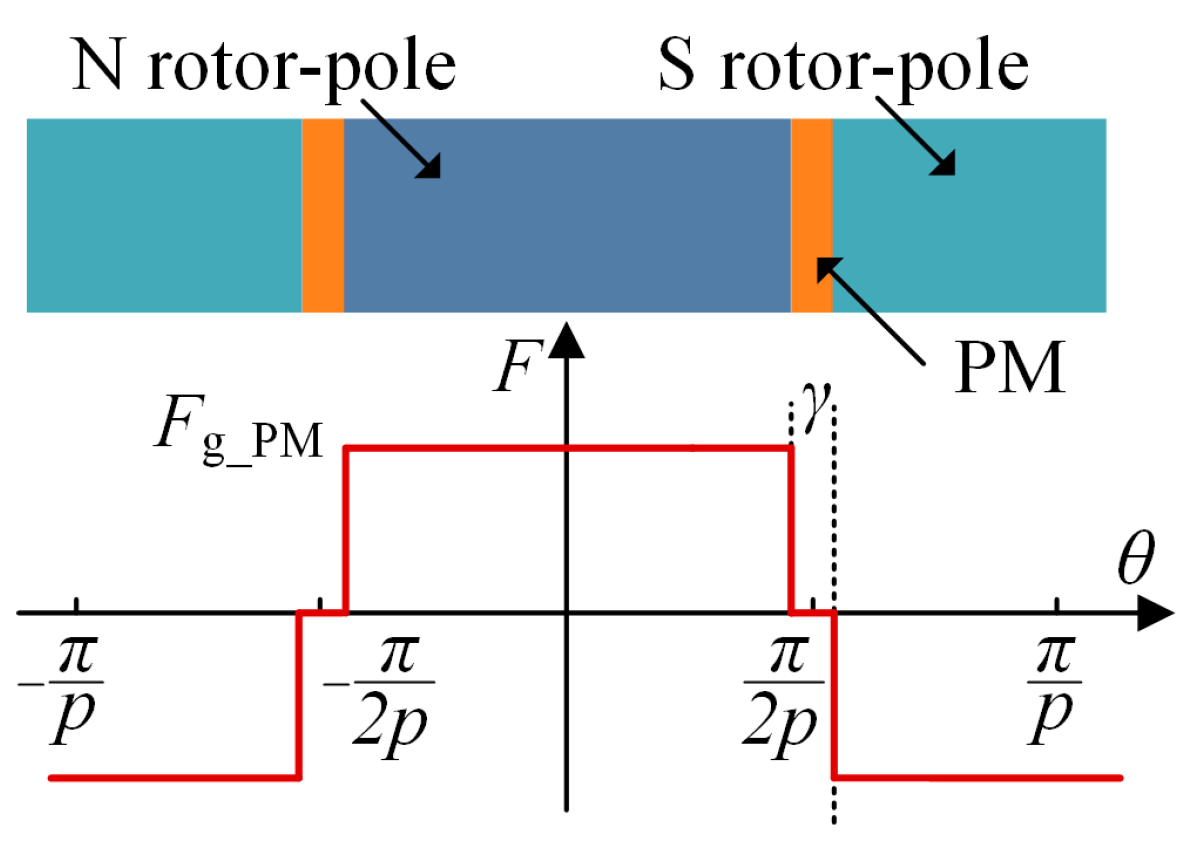
2.2. Operating Principle

The AF-HESG is primarily composed of three flux paths: the PM flux path, the field flux path, and the leakage flux path, as depicted in Figure 4. The PM generates radial air-gap flux and axial leakage flux. The leakage flux passes through the axial magnetic bridge, additional air gaps, and then returns to the PM. The field flux path is in parallel with the PM flux path. This enables the generator to have flexible flux-regulation capability.

In order to have a simpler understanding of the principle of the AF-HESG, Figure 5 shows the equivalent magnetic circuit and electrical circuit under flux-enhancing. Considering leakage flux as PM flux, it can be seen that the armature flux mainly depends on the PM flux and field flux. The direction of the armature flux in the radial flux path is clear, but in the axial flux path, its direction depends on the field flux. As shown in Figure 6, when the field flux is small, the direction of the armature flux is opposite to that of the field flux. As the field flux increases, the direction of the armature flux becomes the same as that of the field flux. By weakening the PM flux through the axial magnetic circuit, not only can parallel brushless excitation be achieved, but also a larger flux-regulation range can be obtained.

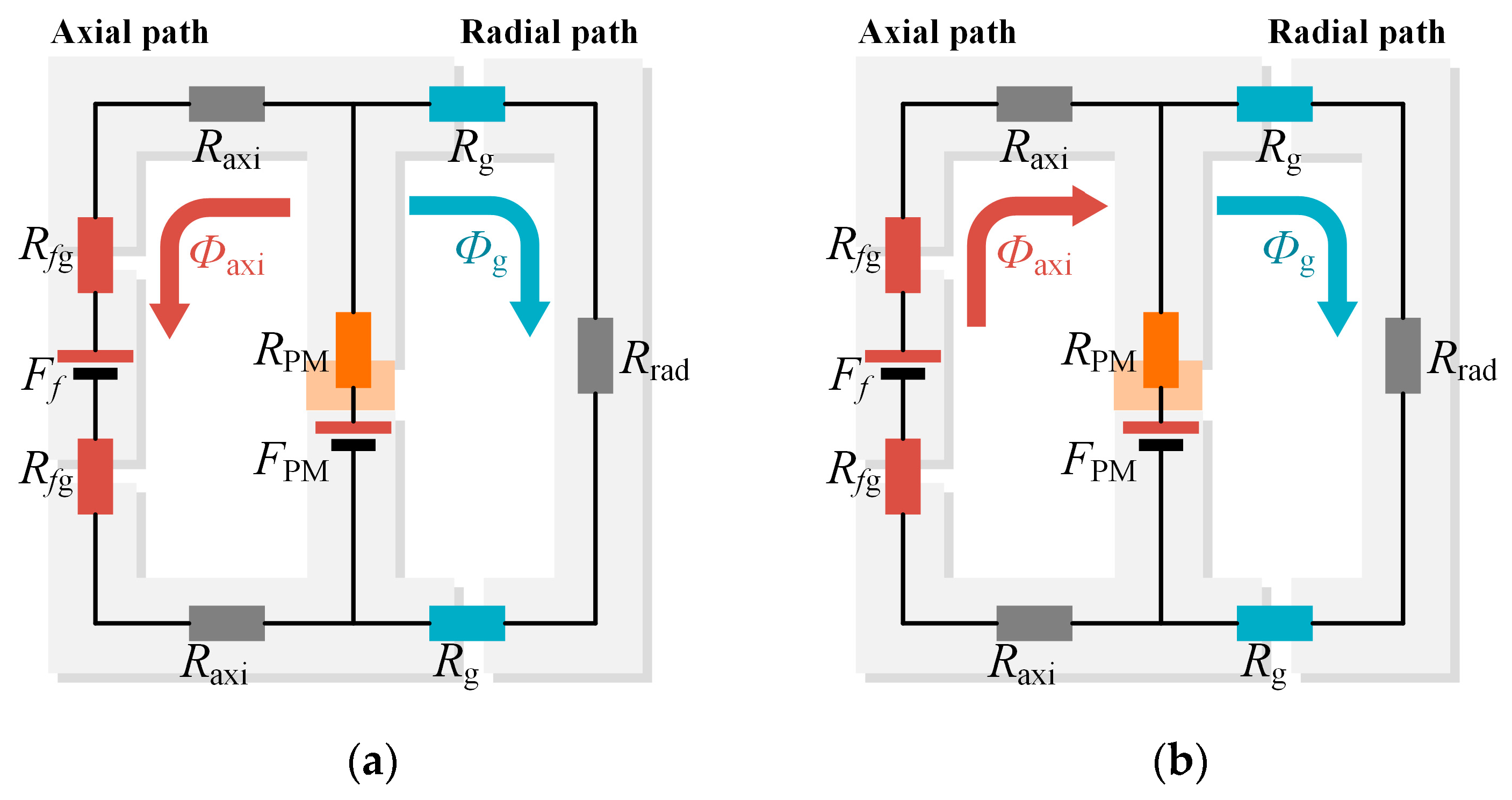
2.3. Equivalent Magnetic Circuit Model

In order to analyze the flux-regulation ability of the AF-HESG more accurately, it is necessary to conduct a quantitative analysis. Equivalent magnetic circuit (MEC) models are established for Case 1 and Case 3, as shown in Figure 7. R_g and R_fg are the main air-gap reluctances and the additional air-gap reluctances, respectively; ϕ_g and ϕ_axi are the air-gap flux and axial flux, respectively; ϕ_PM is the PM flux, R_PM and F_PM are the equivalent internal reluctance and the equivalent MMF of the PM, respectively, F_f is the field MMF, and R_rad and R_axi are the equivalent reluctances of the radial and axial core. As the field MMF increases, the direction of axial flux ϕ_axi changes.

Based on the MEC, the relationship between the air-gap flux, MMF, and reluctance generated by PMs ϕ_{g_PM} and field windings (FW) ϕ_{g_f} can be expressed as follows:

$$\left\{\begin{array}{l}{\varphi }_{\mathrm{g}\text{\_}\mathrm{PM}}={\displaystyle \frac{F_{\mathrm{PM}}\left(2R_{f\mathrm{g}}+2R_{\mathrm{axi}}\right)}{\left[\begin{array}{l}\left(2R_{\mathrm{g}}+R_{\mathrm{rad}}\right)R_{\mathrm{PM}}+\left(2R_{f\mathrm{g}}+2R_{\mathrm{axi}}\right)R_{\mathrm{PM}}\\ +\left(2R_{\mathrm{g}}+R_{\mathrm{rad}}\right)\left(2R_{f\mathrm{g}}+2R_{\mathrm{axi}}\right)\end{array}\right]}}\\ {\varphi }_{\mathrm{g}\text{\_}f}={\displaystyle \frac{F_f{R}_{\mathrm{PM}}}{\left[\begin{array}{l}\left(2R_{\mathrm{g}}+R_{\mathrm{rad}}\right)R_{\mathrm{PM}}+\left(2R_{f\mathrm{g}}+2R_{\mathrm{axi}}\right)R_{\mathrm{PM}}\\ +\left(2R_{\mathrm{g}}+R_{\mathrm{rad}}\right)\left(2R_{f\mathrm{g}}+2R_{\mathrm{axi}}\right)\end{array}\right]}}\end{array}\right.$$

(1)

where the PM MMF can be calculated as follows:

$$F_{\mathrm{PM}}={\displaystyle \frac{B_{\mathrm{r}}{h}_{\mathrm{PM}}}{{\mu }_0{\mu }_{\mathrm{PM}}}}$$

(2)

where B_r is the PM remanent flux density, h_PM is the thickness of PM, and μ_PM is the relative permeability of PM. And the field MMF can be calculated as follows:

$$F_f=N_f{I}_f$$

(3)

where N_f is the number of turns of field winding. I_f is the dc field current. The main air-gap flux is the sum of the air-gap flux generated by the PM and the field winding. The corresponding air gap MMF can be expressed as follows:

$$\left\{\begin{array}{l}{F}_{\mathrm{g}\text{\_}\mathrm{PM}}=2R_{\mathrm{g}}{\varphi }_{\mathrm{g}\text{\_}\mathrm{PM}}\\ F_{\mathrm{g}\text{\_}f}=2R_{\mathrm{g}}{\varphi }_{\mathrm{g}\text{\_}f}\\ F_{\mathrm{g}}=F_{\mathrm{g}\text{\_}\mathrm{PM}}+F_{\mathrm{g}\text{\_}f}\end{array}\right.$$

(4)

The MMF-specific permeance method can obtain the waveform of air-gap flux density by calculating the air-gap MMF and permeance. To simplify the model, iron core saturation and edge effects are ignored. Figure 8 shows the waveform of the ideal air-gap MMF, where γ is the angle corresponding to the thickness of the PM and p is the number of pole pairs.

The air-gap MMF generated by PM is an even function with a period of 2π/p. Its waveform is expanded into a Fourier series as follows [22]:

$$\left\{\begin{array}{l}{F}_{\mathrm{g}\text{\_}\mathrm{PM}}(\theta )={\displaystyle \sum _{i=1,3\dots }^{\infty }{F}_{\mathrm{g}\text{\_}\mathrm{PM}i}\cos \left(ip\theta \right)}\\ F_{\mathrm{g}\text{\_}\mathrm{PM}i}={\displaystyle \frac{4F_{\mathrm{g}\text{\_}\mathrm{PM}}}{i\pi }}\sin {\displaystyle \frac{i\pi }{2}}\cos {\displaystyle \frac{ip\gamma }{2}}\end{array}\right.$$

(5)

The air-gap MMF generated by FW is the same. The total MMF of the air gap can be calculated by the following:

$$F_{\mathrm{g}}(\theta )=F_{\mathrm{g}\text{\_}\mathrm{PM}}(\theta )+F_{\mathrm{g}\text{\_}f}(\theta )$$

(6)

The air-gap specific permeance can be expressed as follows:

$${\Lambda }_{\mathrm{g}}(\theta )={\displaystyle \frac{{\delta }_g}{{\mu }_0}}{\Lambda }_{\mathrm{gs}}(\theta ){\Lambda }_{\mathrm{gr}}(\theta )$$

(7)

where Λ_gs(θ) and Λ_gr(θ) represent the air-gap specific permeances considering stator slotting and rotor pole arc, respectively. They can be calculated by the following:

$$\left\{\begin{array}{l}{\Lambda }_{\mathrm{gs}}\left(\theta \right)={\Lambda }_{\mathrm{gs}0}+{\displaystyle \sum _{m=1}^{\infty }{\Lambda }_{\mathrm{gsm}}\cos \left(m{Z}_{\mathrm{s}}\theta \right)}\\ {\Lambda }_{\mathrm{gr}}\left(\theta \right)={\displaystyle \frac{{\Lambda }_{\mathrm{gr}0}}{2}}+{\displaystyle \sum _{n=1}^{\infty }{\Lambda }_{\mathrm{grn}}\cos \left(np\theta \right)}\end{array}\right.$$

(8)

where Λ_gs0 and Λ_gr0 are the dc components and Λ_gsm and Λ_grm are the mth and nth harmonic amplitudes. The air-gap flux density can be calculated by the following:

$$B_{\mathrm{g}}(\theta )=F_{\mathrm{g}}(\theta )\times {\Lambda }_{\mathrm{g}}(\theta )$$

(9)

Figure 9 shows the calculation results of the MMF-specific permeance method. The distribution of the air-gap MMF generated by PM and FW is shown in Figure 9a. The waveform of the air-gap specific permeances, considering stator slotting and rotor pole arc, obtained by adding up 100 terms of the Fourier series, is shown in Figure 9b. The waveform of the air-gap flux density is shown in Figure 9c. Figure 10 shows the relationship of the air-gap flux density and field current. It can be observed that as the field current increases, the air-gap flux density increases. Due to the neglect of iron core saturation and leakage flux during calculation, there is a certain difference from the simulation results, but the overall trend is similar.

According to the above calculation, the air-gap flux density is affected by the parameters of AF-HESG, and the main parameters and affected parameters are shown in

Table 2. The main parameters and their range of variation.

Parameters	Affected Parameters	Variation Range
PM height hPM	FPM, RPM	5~15 mm
PM width wPM	RPM	30~50 mm
Air-gap length δg	Rg, Λg	0.5~1 mm
Additional air-gap length δfg	Rfg	0.45~0.95 mm
Multiple of additional air-gap cross-sectional area k_sgf	Rfg	0.5~1

. And the variation range of these parameters is normalized to obtain the influence of the main parameters on the air-gap MMF, air-gap flux density, and flux-regulation ability, as shown in Figure 11. The flux-regulation range k_f is defined as follows:

$$k_f={\displaystyle \frac{B_{\mathrm{g}}-B_{\mathrm{g}\text{\_}\mathrm{PM}}}{B_{\mathrm{g}\text{\_}\mathrm{PM}}}}\times 100\%$$

(10)

where B_g is the air-gap flux density with field current applied and B_{g_PM} is the air-gap flux density under PM excitation only.

From Figure 11, it can be observed that the width of the PM w_PM mainly affects the air-gap MMF generated by the PM, thereby increasing the air-gap flux density. Both B_g and B_{g_PM} are increasing, reducing the k_f. The length of the main air-gap δ_g also has a significant impact on the air-gap flux density, but it has little effect on the k_f. The reason is that the air gap permeance Λ_g is also changing, as shown in Figure 12. The longer the additional air-gap length δ_fg, the lower the air-gap MMF generated by the FW, the lower the air-gap flux density, and the B_{g_PM} remains unchanged. So, the k_f is significantly reduced. The larger the cross-sectional area of the additional air gap, the higher the air gap flux density and the k_f. Therefore, the AF-HESG adopts an inclined additional air gap, which can effectively increase the flux-regulation capability.

3. Performance Analysis of AF-HESG

In order to analyze the flux-regulation ability of the AF-HESG, the magnetic field and the operating characteristics are analyzed by using a three-dimensional finite element model. The loss distribution is analyzed, and the temperature of the generator under rated conditions is simulated.

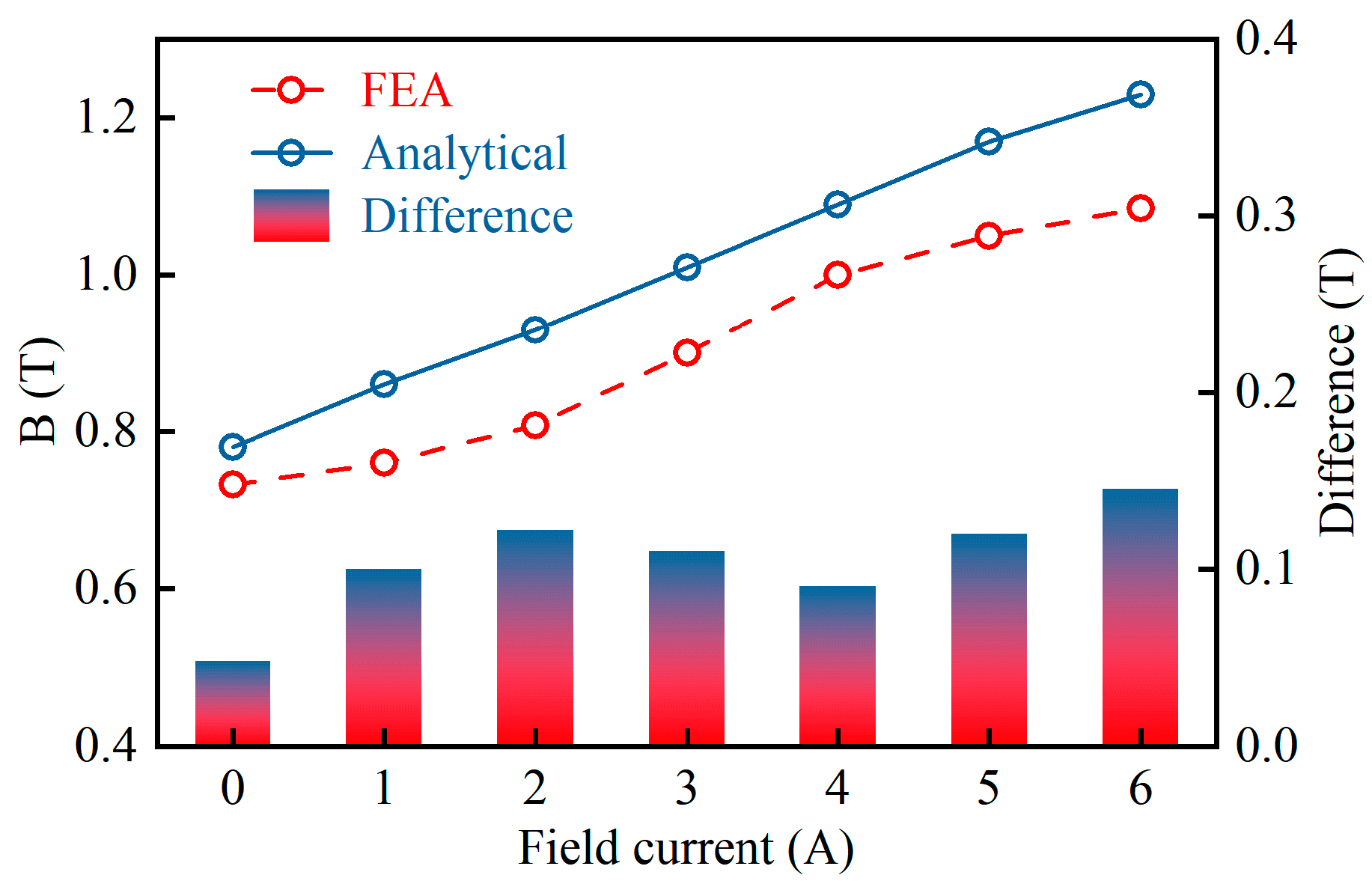
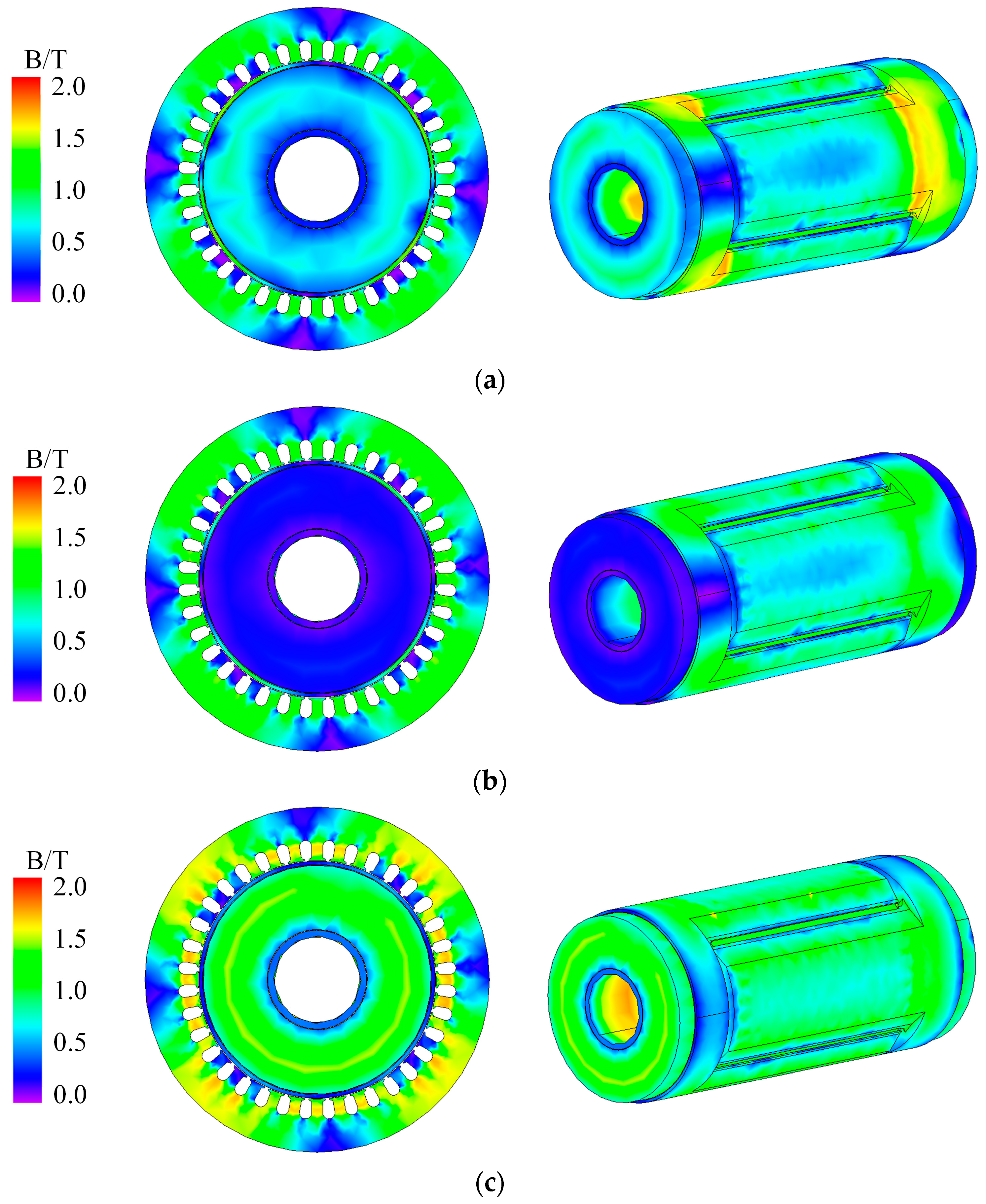
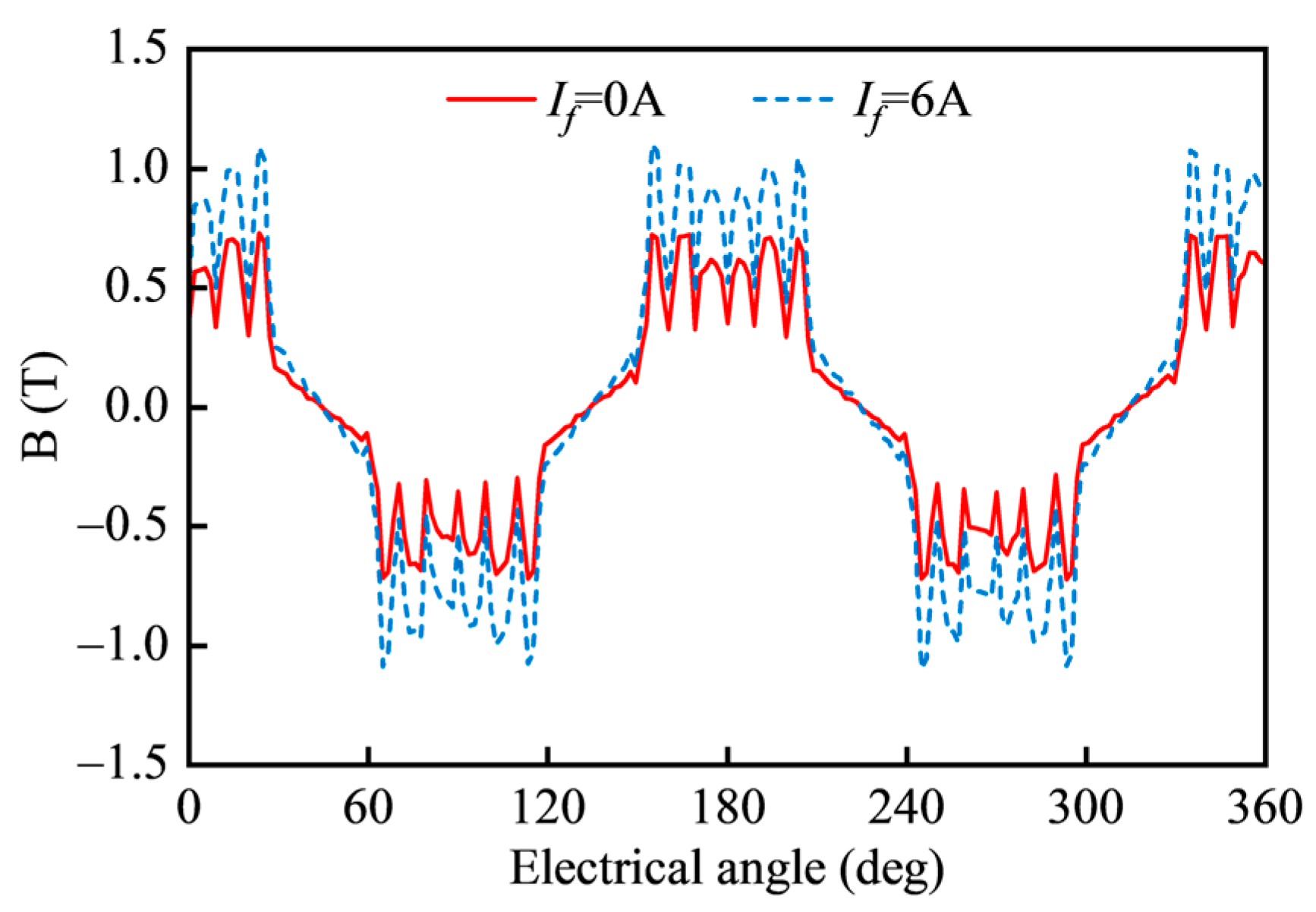
3.1. Magnetic Field Analysis

Through static finite element analysis (FEA), magnetic field distributions with different I_f are obtained, as shown in Figure 13. Figure 14 shows the distribution waveform of the air-gap flux density under different field currents. It can be observed that when the field current I_f is 0 A, part of the PM flux passes through the axial magnetic circuit. When I_f increases, the flux density of the radial path increases, while the axial path decreases. As the field current continues to increase, the main air-gap flux increases significantly. When I_f is 6 A, the AF-HESG reaches a saturated state. The result of magnetic field distributions conforms to the operating principle of Figure 6.

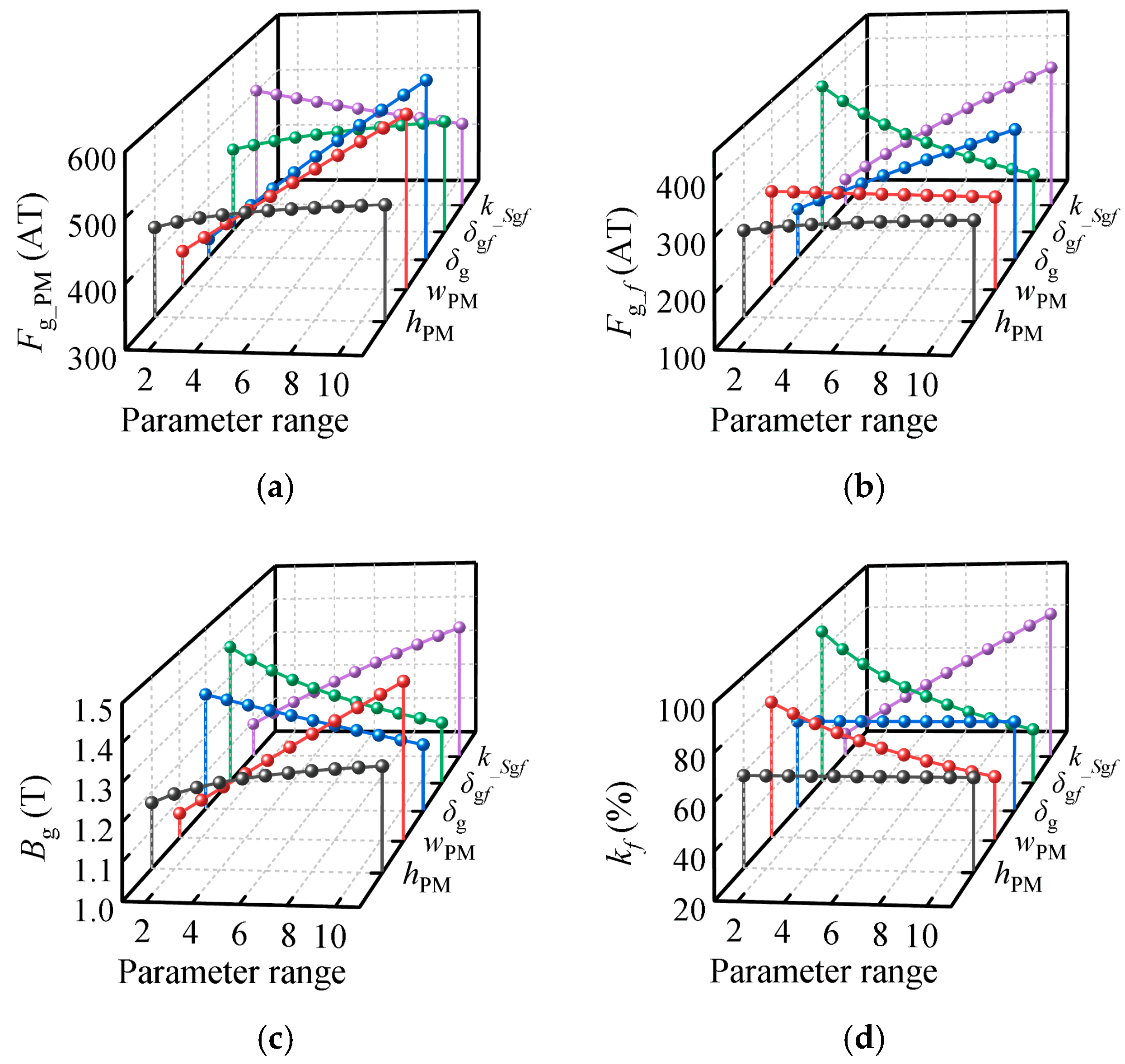
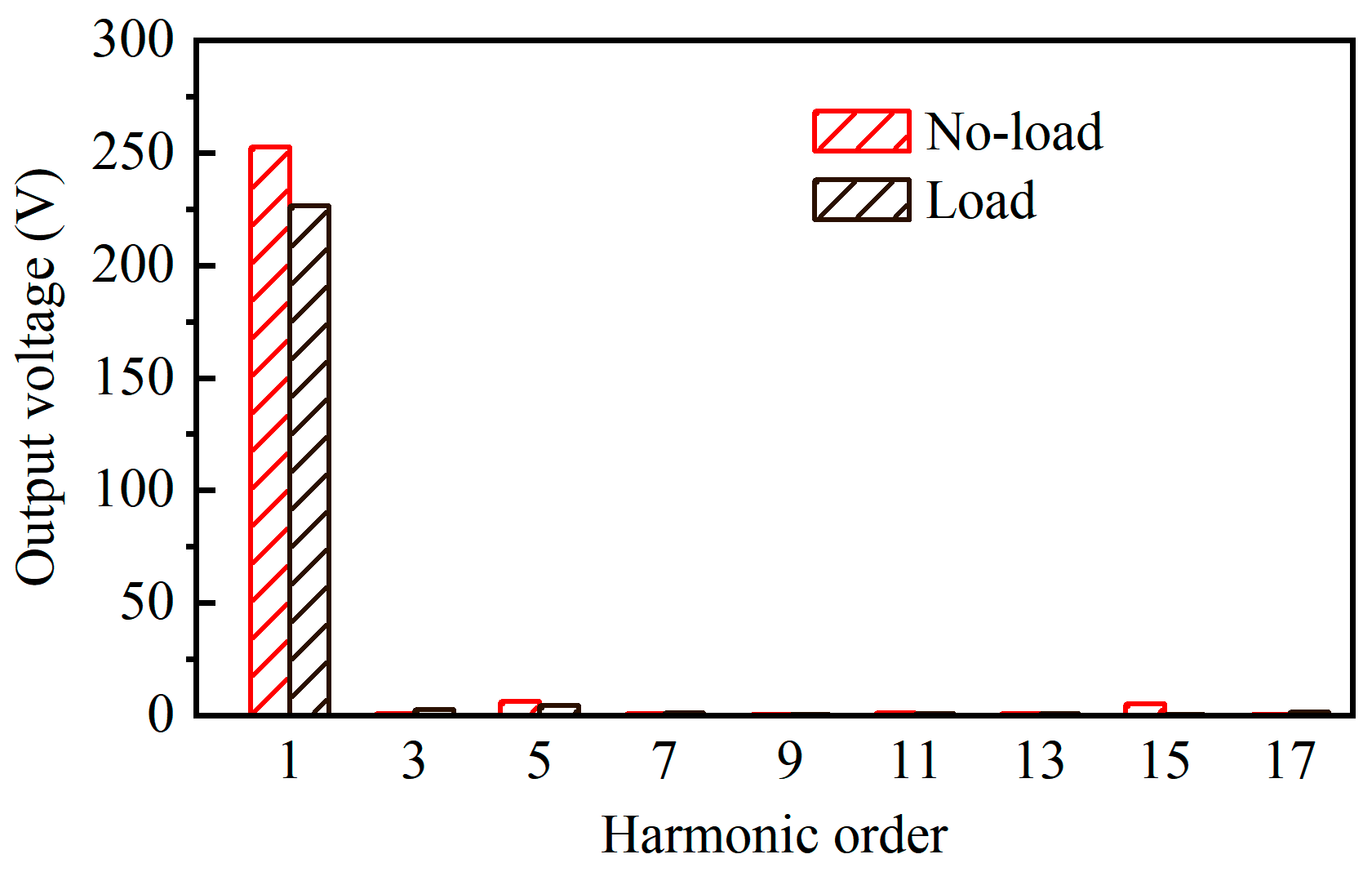
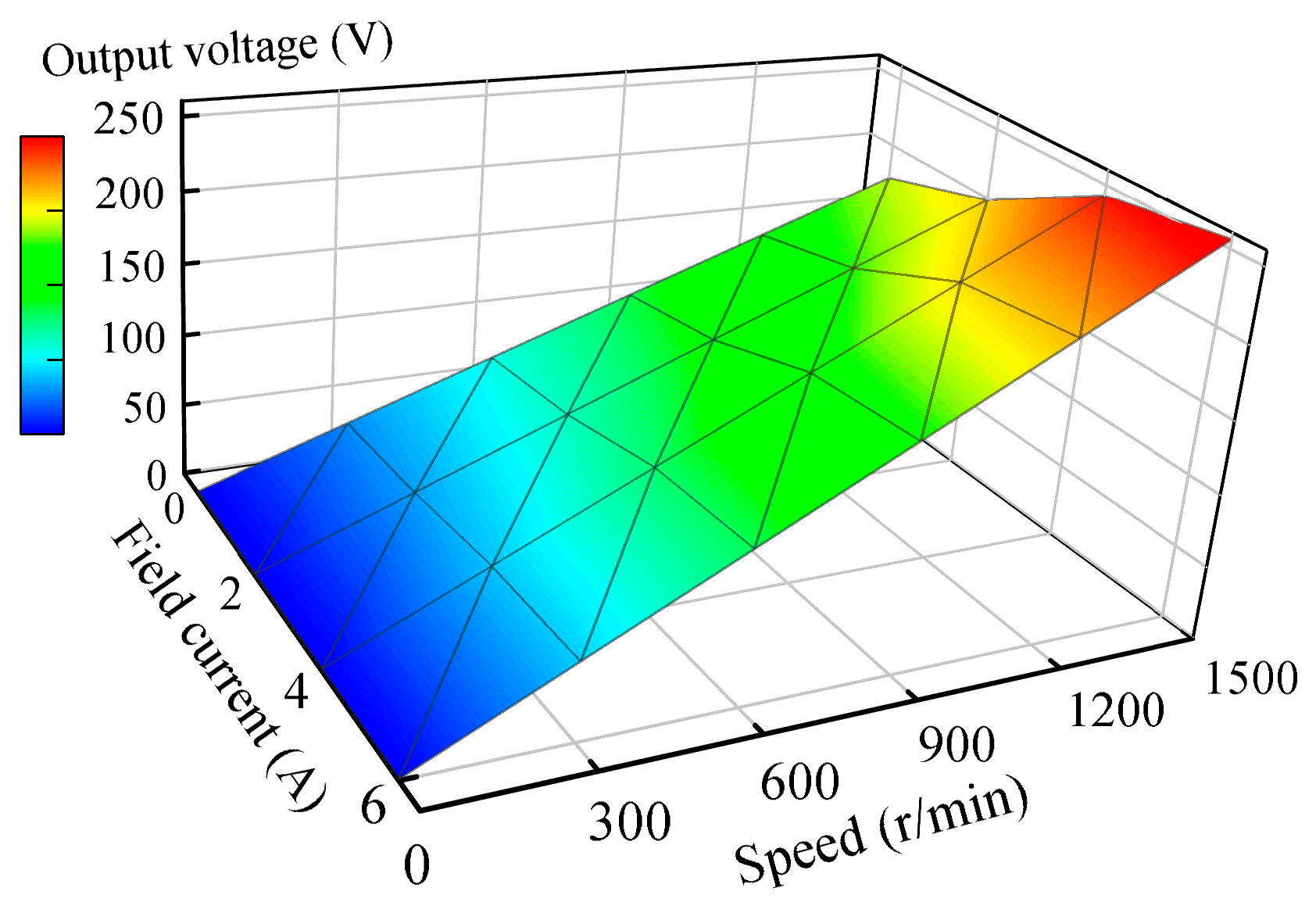
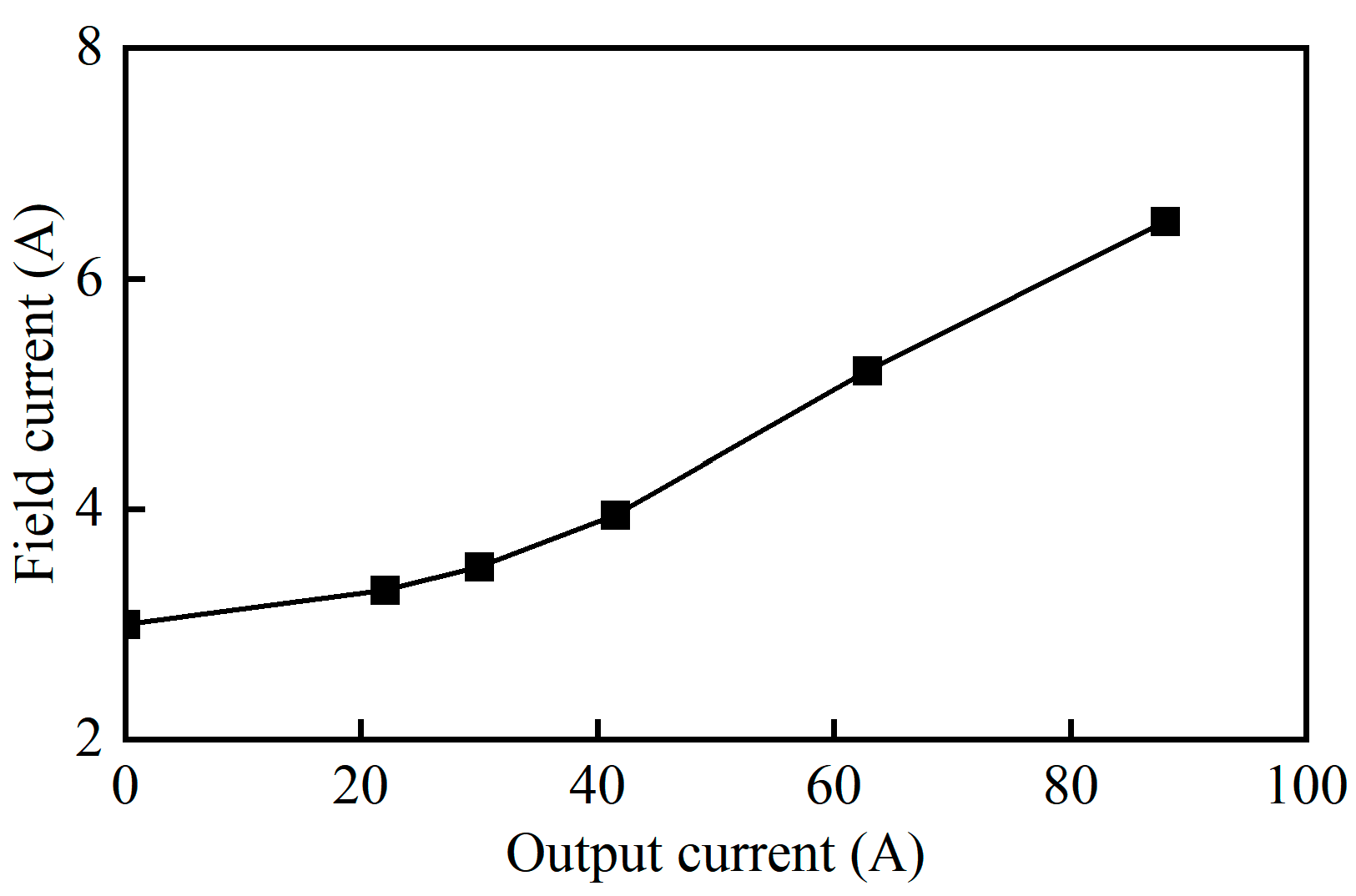
3.2. Operating Characteristics Analysis

Through transient FEA, the no-load voltage and the rated load voltage under different field currents are obtained, as shown in Figure 15. When the field current is 6 A, the effective value of the no-load voltage is 250 V, and the rated load voltage is 234 V. Due to the filtering effect of the armature winding, the sine degree of the rated load voltage waveform is higher. Figure 16 shows the voltage harmonics under no-load and loaded conditions.

Figure 17 shows the relationship among the no-load output voltage, the field current, and speed, i.e., the no-load characteristics of the AF-HESG. It is obvious that the no-load output voltage increases linearly with the speed. When I_f increases from 0 A to 2 A, the output voltage increases slowly because the field MMF needs to partially offset the PM leakage flux. When I_f reaches 2 A, the output voltage increases faster, while when I_f is between 4 A and 6 A, the voltage increases slowly because the iron core is beginning to saturate.

The external characteristics at rated speed for different field currents are depicted in Figure 18. It can be observed that with constant field current, the output voltage decreases as the load current increases. The voltage adjustment rate represents the degree of steady-state voltage drop when the load changes, and can be defined as follows:

$$\Delta U={\displaystyle \frac{U_{\mathrm{no}\text{-}\mathrm{load}}-U_{\mathrm{N}}}{U_{\mathrm{N}}}}\times 100\%$$

(11)

The voltage adjustment rate of the generator is relatively low, approximately 9.36%. As the field current increases, the output voltage increases while the load current remains constant. The voltage regulation range is 46.5%.

To maintain stable voltage output, it is necessary to adjust the field current. The regulation characteristics, i.e., the relationship between field current and load current with the output voltage U_out set to 220 V, are shown in Figure 19. It can be observed that as the load current increases, the required field current also increases.

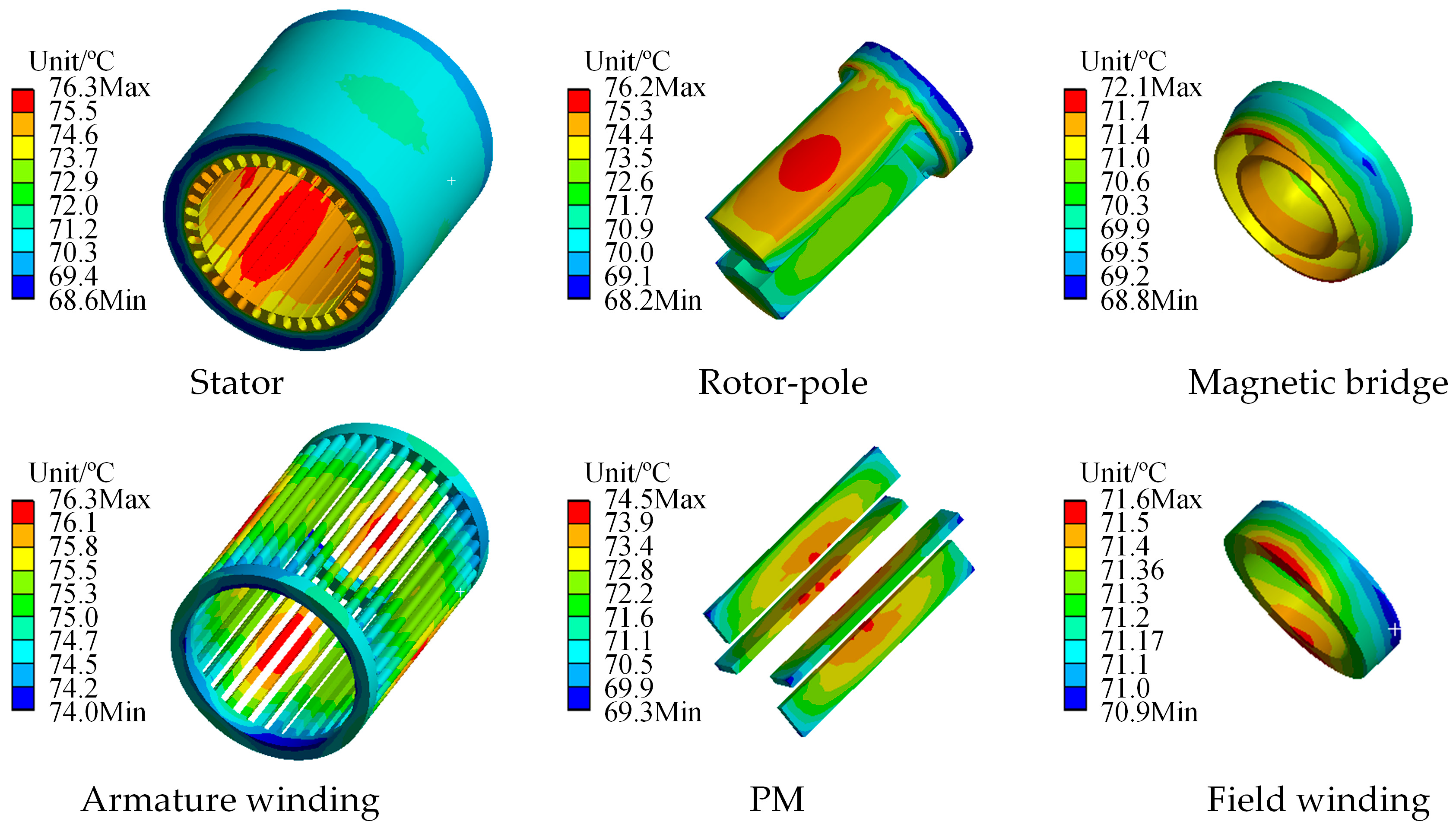
3.3. Loss and Temperature Field Analysis

The component of the loss under different field currents at the rated speed and load is shown in Figure 20. The main loss is the iron loss of the rotor and the copper loss of the armature winding W_A because the rotor is made of solid steel with high eddy current loss. With the addition of field current, the losses of all components have increased, except for the magnetic bridge, because, without field current, most of the PM flux passes through the magnetic bridge. The PM loss is relatively small. The efficiency of the AF-HESG under rated conditions is about 89%.

In order to avoid damage to the generator caused by high temperature, the temperature field is checked. Due to the complexity of the winding end model, the winding end is equivalent to a circular ring. A three-dimensional temperature field finite element model is established. The losses during AF-HESG will serve as a heat source in temperature field analysis, achieving electromagnetic thermal unidirectional coupling calculation. The method is to input the loss results in the form of heat generation rate into the temperature field model. The heat generation rate is the heat generation power per unit volume, and it can be calculated by the following:

$$Q={\displaystyle \frac{P_{\mathrm{loss}}}{V}}$$

(12)

where P_loss is the losses of each part of the generator, and V represents the effective volume of the corresponding part. The results are shown in

Table 3. The result of the heat generation rate.

Components	Ploss (W)	V (m3)	Q (W/m3)
Stator	319	6.58 × 10−3	48,443
Rotor-pole	1296	5.87 × 10−3	220,783
Magnetic bridge	48	1.28 × 10−3	37,500
PM	38	4 × 10−4	95,000
Armature winding	1452	1.52 × 10−3	954,007
Field winding	187	7.05 × 10−4	265,531

.

The accurate selection of thermal conductivity and convective heat transfer coefficient on the surface of each component of the AF-HESG determines the accuracy of temperature calculation. The calculation method for the equivalent thermal conductivity of the air gap is as follows [23]:

$$\left\{\begin{array}{l}{\lambda }_{\mathrm{g}}=0.0019{\eta }^{-2.9084}{R{e}_{\mathrm{g}}}^{0.4614\ln (3.33361\eta )}\\ \eta =D_2/D_{\mathrm{i}1}\end{array}\right.$$

(13)

where D₂ is the outer diameter of the rotor, D_i1 is the inner diameter of the stator, and R_eg is the Reynolds number of the air gap. Based on relevant engineering practice experience, the thermal conductivity λ_t and convective heat transfer coefficients α_t of each component are shown in

Table 4. The result of thermal conductivity and convective heat transfer coefficients.

Components	Material	λt (W/m·K)	αt (W/m2·K)
Stator	50WW600	27.2	/
Rotor/Magnetic bridge	Steel No. 10	45	/
PM	N35UH	7.6	/
Winding	copper	400	/
Air-gap	Equivalent air	0.098	/
Shell/shaft	Aluminum	237	/
Surface of shell	/	/	41.76
Stator end	/	/	69.2
Rotor end	/	/	100.25

.

The FEA results of the steady-state temperature field of each component under rated load conditions are shown in Figure 21. It can be observed that the temperature is relatively average, with a maximum temperature of around 76 degrees. Therefore, the result conforms to the temperature range for normal operation of the generator.

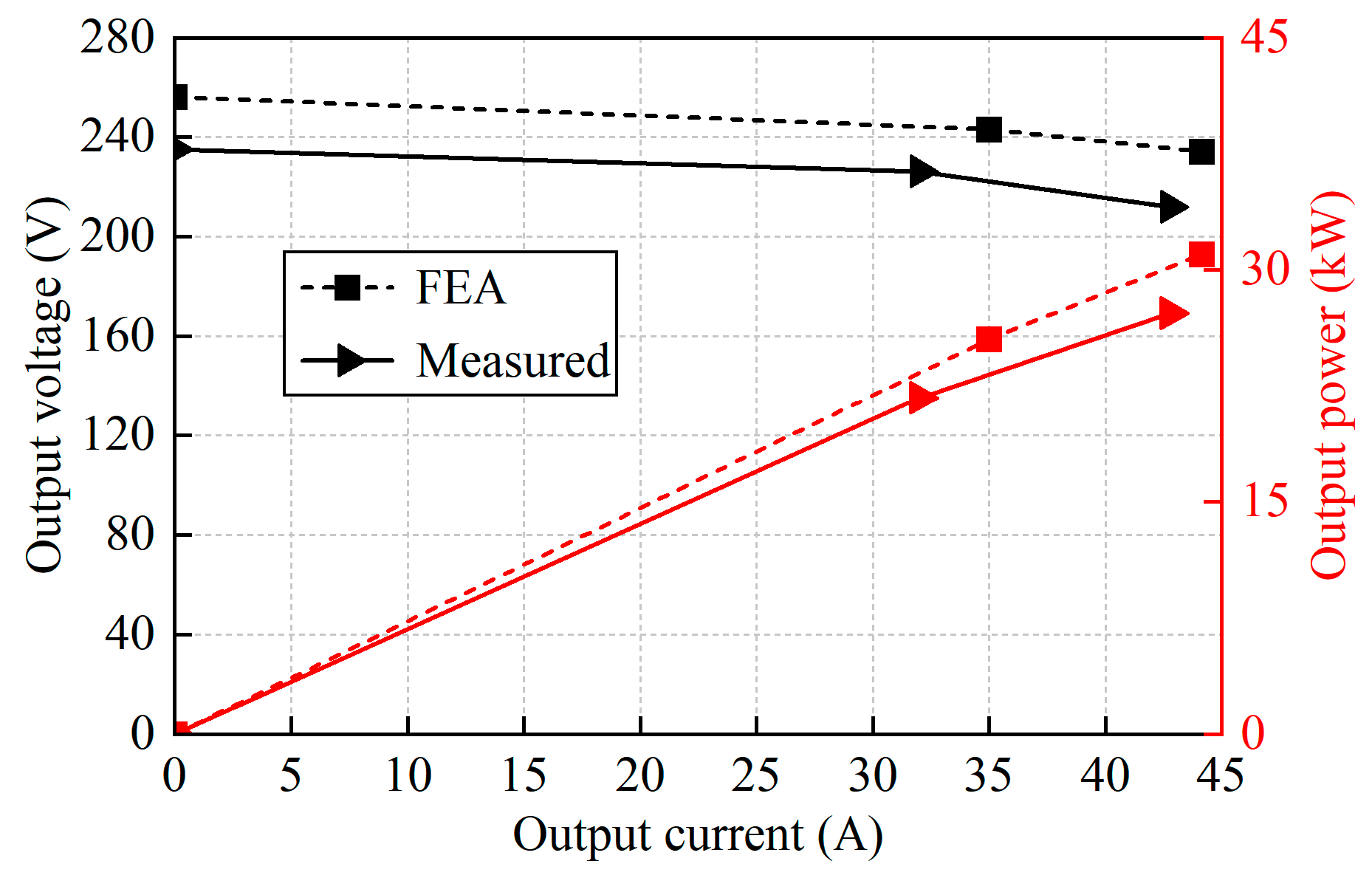
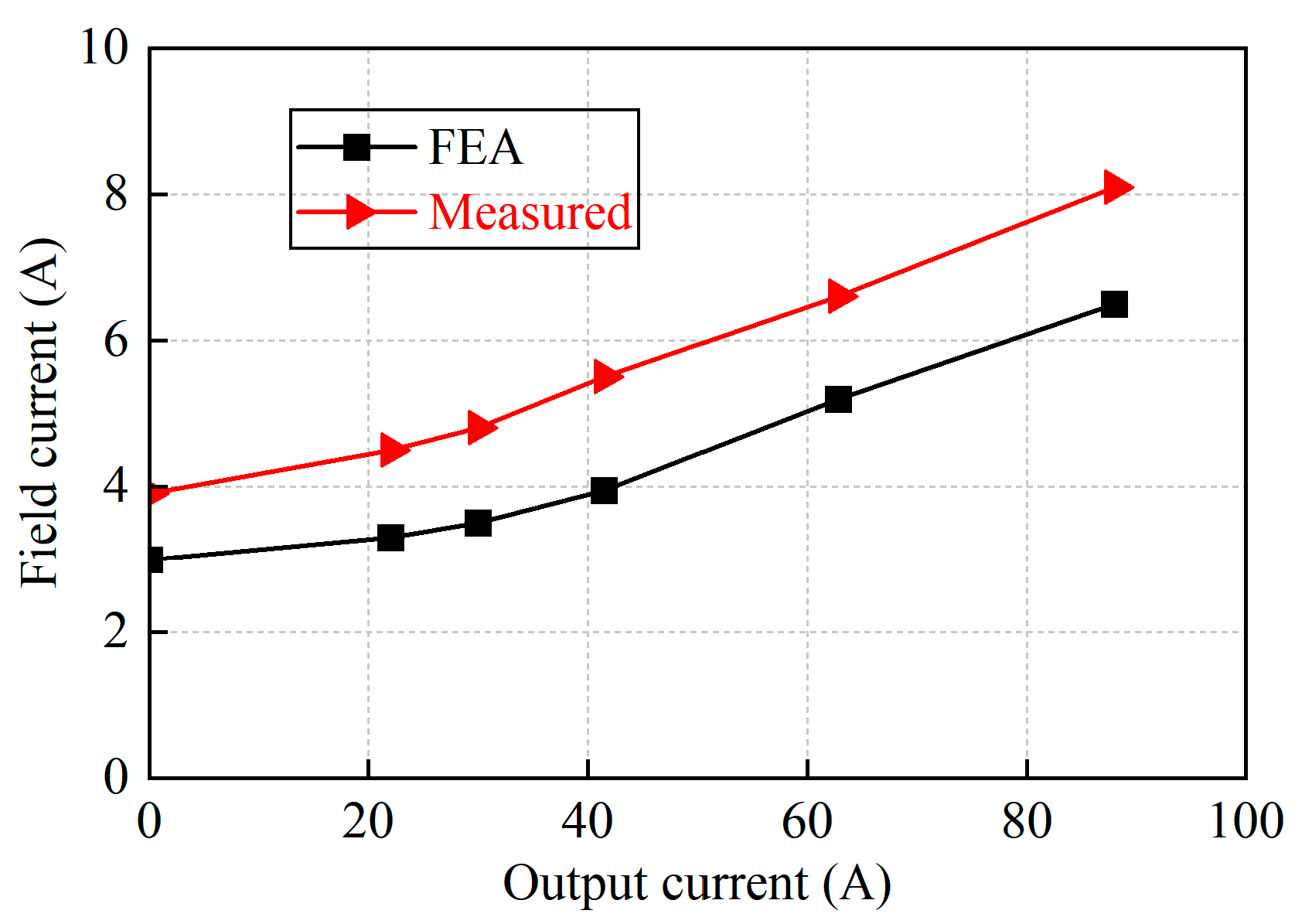
4. Experimental Verification

The prototype of the AF-HESG is manufactured and the test platform is built, as shown in Figure 22. The platform mainly consists of the AF-HESG, the prime motor, a dc power supply, and the three-phase load. The proposed generator is driven by the prime mover during testing. The dc power supply provides the dc field current.

Figure 23 shows the measured no-load output voltage waveform under I_f = 0 A and I_f = 6 A. It is observed that the three-phase voltage is symmetrical, and as the field current increases, the effective value of no-load output voltage increases from 166 V to 235 V. Figure 24 shows the measured load output voltage waveform under I_f = 0 A and I_f = 6 A. As the field current increases, the effective value of load output voltage increases from 155 V to 212 V. It can be concluded that the voltage waveform is generally smoother than no-load voltage due to the filtering effect of the windings.

By varying the speed and field currents, the no-load characteristics at different speeds can be obtained, as shown in Figure 25. Compared to the simulation, the measured output voltage is relatively small, but the overall trend conforms to the simulation results because it is difficult to ensure that the structural parameters and additional air gap size are completely consistent with the design values in actual processing. Figure 26 shows the measured external characteristics under I_f = 6 A. It can be observed that as the load current increases, the output voltage decreases and the output power increases. Under rated conditions, the actual output power of the AF-HESG is 27.3 kW, and the efficiency is about 85%. Figure 27 shows a comparison of the regulation characteristics at rated speed with an output voltage of 220 V. It can be observed that as the load current increases, a higher field current is required to maintain voltage stability in the experiment, yet the overall trend remains consistent.

5. Conclusions

In this paper, a detailed study was conducted on the operating principle and electromagnetic performance of the AF-HESG through the MMF-specific permeance method, three-dimensional finite element analysis, and prototype testing. The conclusions are as follows:

(1)

AF-HESG provides a field flux path without passing through permanent magnets by adding an axial magnetic circuit, achieving brushless excitation.

(2)

According to the analysis of the air-gap flux density, it can be concluded that AF-HESG can increase the flux-regulation range by changing the additional air-gap length and cross-sectional area, so the AF-HESG adopts an inclined additional air gap, which can effectively increase the flux-regulation capability.

(3)

Finite element simulations are conducted to validate the model and analyze the output characteristics of the generator. The load voltage regulation efficiency is 46.5%. The voltage adjustment rate is 9.36%. Due to the axial magnetic circuit of the generator, the rotor is made of solid materials. Therefore, the eddy current loss of the rotor is relatively large. And the temperature is relatively average under rated load conditions.

(4)

A prototype is manufactured. Due to the relatively complex structure of the generator, processing is difficult, and it is not easy to mass produce. The feasibility of the generator principle is verified through comparison between the measured results and simulations. And it reflects that AF-HESG has good magnetic field regulation ability and stable voltage output ability.