Numerical Analysis of Soot Dynamics in C₃H₈ Oxy-Combustion with CO₂ and H₂O

Abstract

Oxygen-enriched combustion is increasingly recognized as a viable approach for clean energy production and carbon capture, offering substantial benefits for boosting combustion efficiency and mitigating pollutant emissions, which makes it widely adopted in various industrial applications. Liquefied petroleum gas (LPG), predominantly consisting of propane (C₃H₈), is commonly utilized in numerous combustion systems, yet its emissions of soot particulates have raised considerable environmental concerns. This study delves into the combustion dynamics and soot formation behavior of propane, the principal component of LPG, under oxy-fuel combustion conditions, with the inclusion of H₂O and CO₂, utilizing both experimental techniques and numerical simulations. The results reveal that CO₂ and H₂O suppress soot formation through distinct mechanisms. CO₂ decreases soot nucleation and surface growth by lowering flame temperature and H atom concentration, but it minimally enhances soot oxidation. H₂O significantly reduces soot formation by chemically increasing OH radical concentration, thereby enhancing soot oxidation. A detailed decoupling analysis further shows that CO₂’s influence is predominantly thermal and chemical, resulting in lower OH levels and an elongated flame shape. In contrast, H₂O’s substantial thermal and chemical effects decrease flame height and promote soot reduction. These insights advance the understanding of soot formation control in oxy-fuel combustion, offering strategies to optimize combustion efficiency and minimize environmental impact.

1. Introduction

Despite the ongoing global energy transition, fossil fuels remain essential to modern society [1]. However, the soot particles generated from fuel combustion pose significant risks to both the environment and human health [2]. In alignment with China’s ‘carbon neutrality’ strategy, optimizing the use of widely prevalent hydrocarbon fuels while minimizing soot formation is a critical research focus. In this situation, oxy-fuel combustion-based carbon capture technology has received a lot of attention from industries. Exhaust gas recirculation (EGR) and flue gas recirculation (FGR) are also being used by many industries [3,4]. Recent research indicates that soot formation on hydrocarbon fuels in O₂/CO₂ and O₂/H₂O environments significantly differs from its formation in air-based combustion [5,6]. Park J et al. [7] examined the impact of adding H₂O, CO₂, and N₂ into CH₄/air counterflow diffusion flames. Their results indicated that CO₂ addition reduced the flame temperature due to a combination of thermal and chemical influences, whereas H₂O promoted combustion primarily through its chemical interactions. Wang et al. [8] conducted numerical simulations and experiments to investigate the effects CO₂ on soot formation in C₂H₄/air opposed-flow flames, discovering that CO₂ reduced concentrations of key species like H radicals, methyl, propargyl, and acetylene, which in turn decreased soot nucleation and surface growth rates. Chen et al. [9] also investigated the impacts of CO₂ on laminar co-flow ethylene diffusion flames. Their results indicated that CO₂ decreases the nucleation size of soot particles and modified the boundary between soot growth and oxidation regions on the flame surface. Zhang et al. [10] used a two-color flame emission method with R-band and G-band spectra to measure flame temperature and soot volume fraction in an O₂/CO₂ environment. Their results showed that as the O₂ concentration increased from 21% to 50%, the formation of soot by CO₂ was effectively stopped. Xie et al. [11] studied flames that were mixed with CO₂, H₂, and air. The study found that introducing CO₂ and H₂O altered the primary chemical reaction from OH + H₂ ⇋ H + H₂O to HO₂ + H ⇋ OH + OH, with CO₂ exerting a more significant chemical influence than H₂O. Xu H et al. [12] performed experiments and computer simulations on laminar synthetic gas diffusion flames with 30% CO₂ as the oxidant. Their results showed that CO₂’s thermal and chemical effects change the intensity of combustion by lowering the concentration of OH.

The effect of adding water vapor on soot formation has not been extensively studied, though some research indicates that introducing water vapor into hydrocarbon fuel combustion can suppress soot production. Liu F et al. [13] conducted numerical simulations to examine the effects of water vapor on flame characteristics and soot volume fraction in a laminar co-flow ethylene/air diffusion flame at 1–2 bar. They employed a model combining polycyclic aromatic hydrocarbons (PAHs) with the hydrogen-abstraction/carbon-addition (HACA) mechanism to simulate soot formation’s surface growth and the oxidation processes involved in soot formation. The study demonstrated that adding water vapor affected flame dynamics and soot generation mainly through dilution and thermal impacts, particularly by decreasing H radical concentrations, which in turn reduced the initial rate of soot formation. Ying et al. [14] investigated the effect of H₂O addition on soot morphology, nanostructure, and oxidation activity in the fuel-rich region of an ethylene reverse diffusion flame. The findings indicated that higher H₂O concentrations decreased soot, implying that H₂O effectively inhibited its formation by decreasing the size of the primary particles and aggregates, confirming changes in soot morphology. Cepeda F et al. [15] investigated the influence of water vapor on soot formation in laminar co-flow diffusion flames across varying oxygen concentrations, utilizing both experimental methods and numerical simulations. The findings showed a strong alignment between measured data and simulation results, indicating that water vapor influenced H and OH radical concentrations and altered the formation and oxidation pathways of soot precursors. Qiu L et al. [16] performed numerical simulations on ethanol/air co-flow diffusion flames and examined the impact of water vapor on flame structure and soot production. The authors proposed a stepwise decoupling method to isolate the impacts of dilution, density, transport, thermal, radiative, and chemical effects of water vapor through the use of various virtual species. The study indicated that flame temperature distribution is chiefly affected by the dilution and thermal properties of water vapor, whereas the decrease in soot volume fraction was primarily due to its dilution and chemical effects.

Liquefied petroleum gas (LPG), primarily composed of propane, is extensively utilized in various combustion systems, making the control of its soot particle emissions a critical concern. However, the mechanisms underlying soot formation in propane laminar diffusion flames remain underexplored and require further investigation. Hu X et al. [17]. investigated the laminar flame speed of propane in O₂/CO₂ atmospheres using both experimental and numerical simulations. Their results showed that the laminar flame speed increases with the oxygen content in the air but decreases when there are more CO₂ contents, due to the thermal, radiative, and chemical effects of CO_2, which slow it down. Zhang et al. [18] investigated oxygen-enriched combustion characteristics and soot formation in laminar coaxial jet diffusion flames of ethylene/O₂/N₂. The results revealed that oxygen-enriched combustion significantly raises the flame temperature, expanding the soot formation zone and increasing the soot volume fraction as oxygen concentration rises. Wu et al. [19] employed optical diagnostics to examine how CO₂ addition affects soot formation in ethylene and propane diffusion flames. Their measurements encompassed the spatial distributions of polycyclic aromatic hydrocarbons (PAHs), temperature, soot volume fraction, primary particle size, and particle number density. The results showed that with increasing CO₂ concentration, the ethylene flame’s overall height decreases, the temperatures at the lower regions drop, while the upper temperatures rise. In contrast, the propane flame height remained relatively constant, with a slight overall temperature drop.

This study investigates the efficient and clean use of fossil energy through fundamental experiments and numerical simulations. The study focuses on the combustion characteristics and soot formation of propane (the primary component of liquefied petroleum gas) in CO₂/H₂O atmospheres. Furthermore, there is an in-depth examination into the effects of varying flame temperature, height, and the amount of soot that forms in propane co-flow diffusion flames in O₂/N₂, O₂/CO₂, and O₂/H₂O environments. Several virtual and key species were incorporated into the calculations to analyze the influence of CO₂ and H₂O on propane flame structure and soot formation.

2. Experiment and Simulation

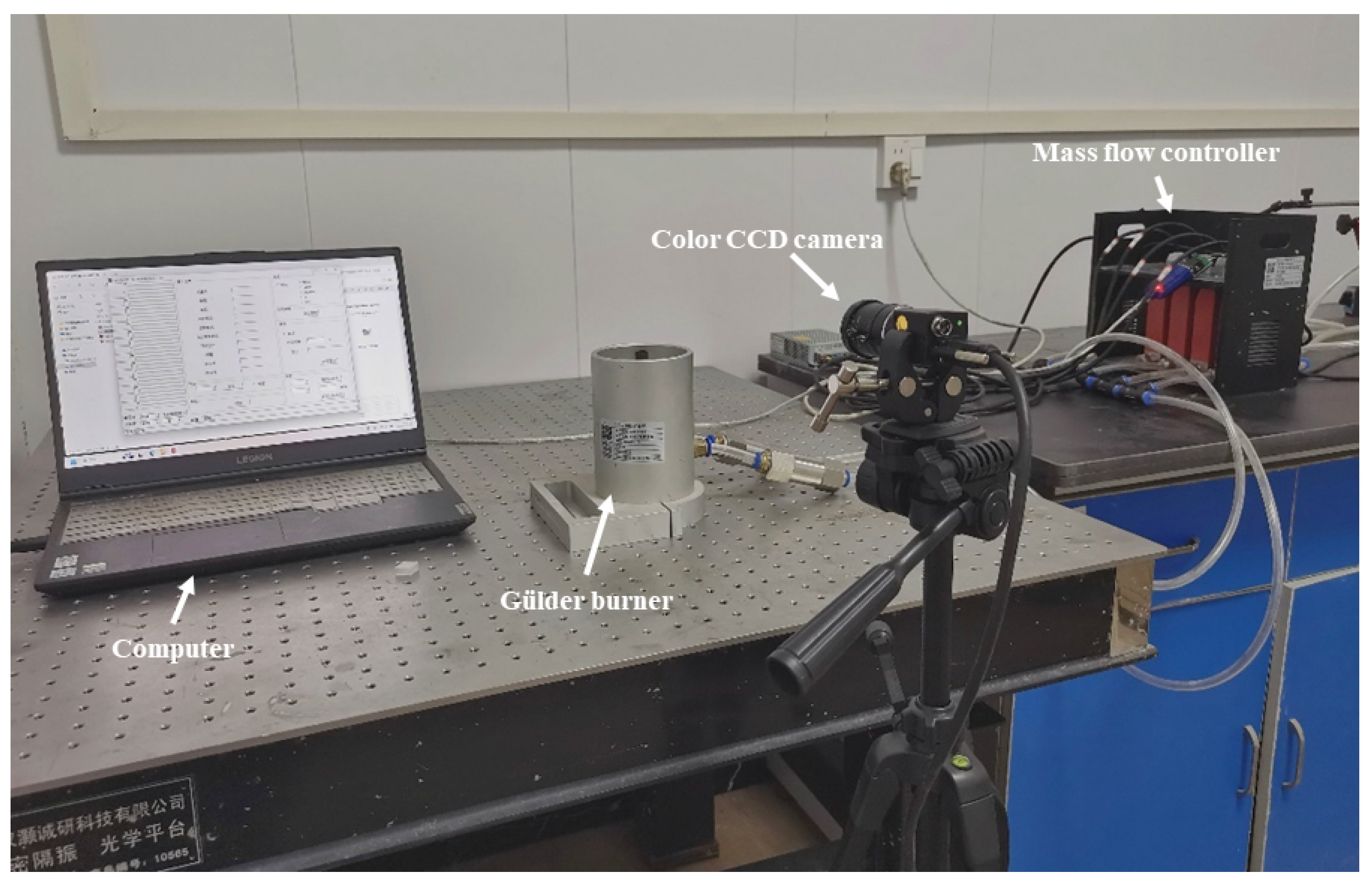
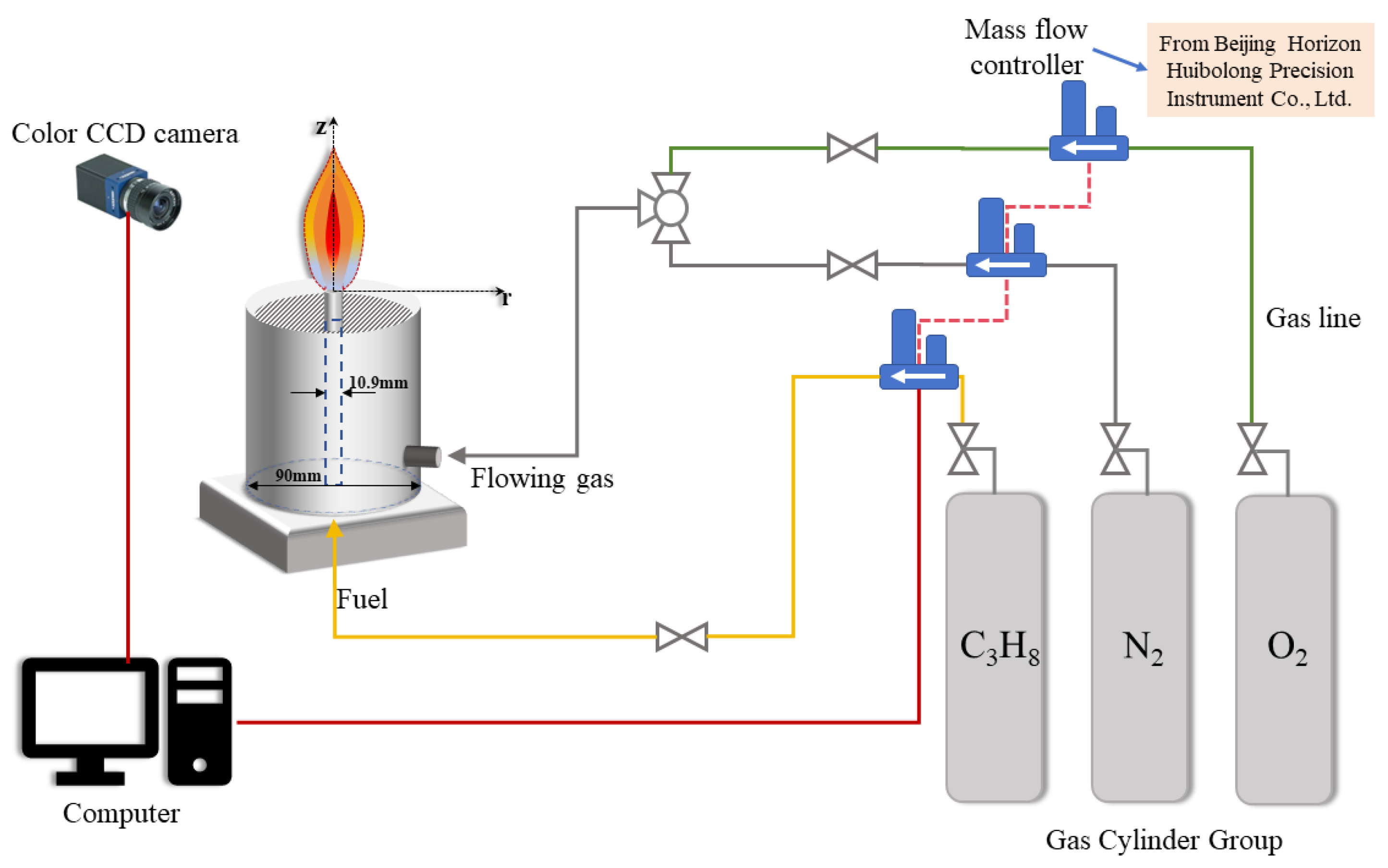
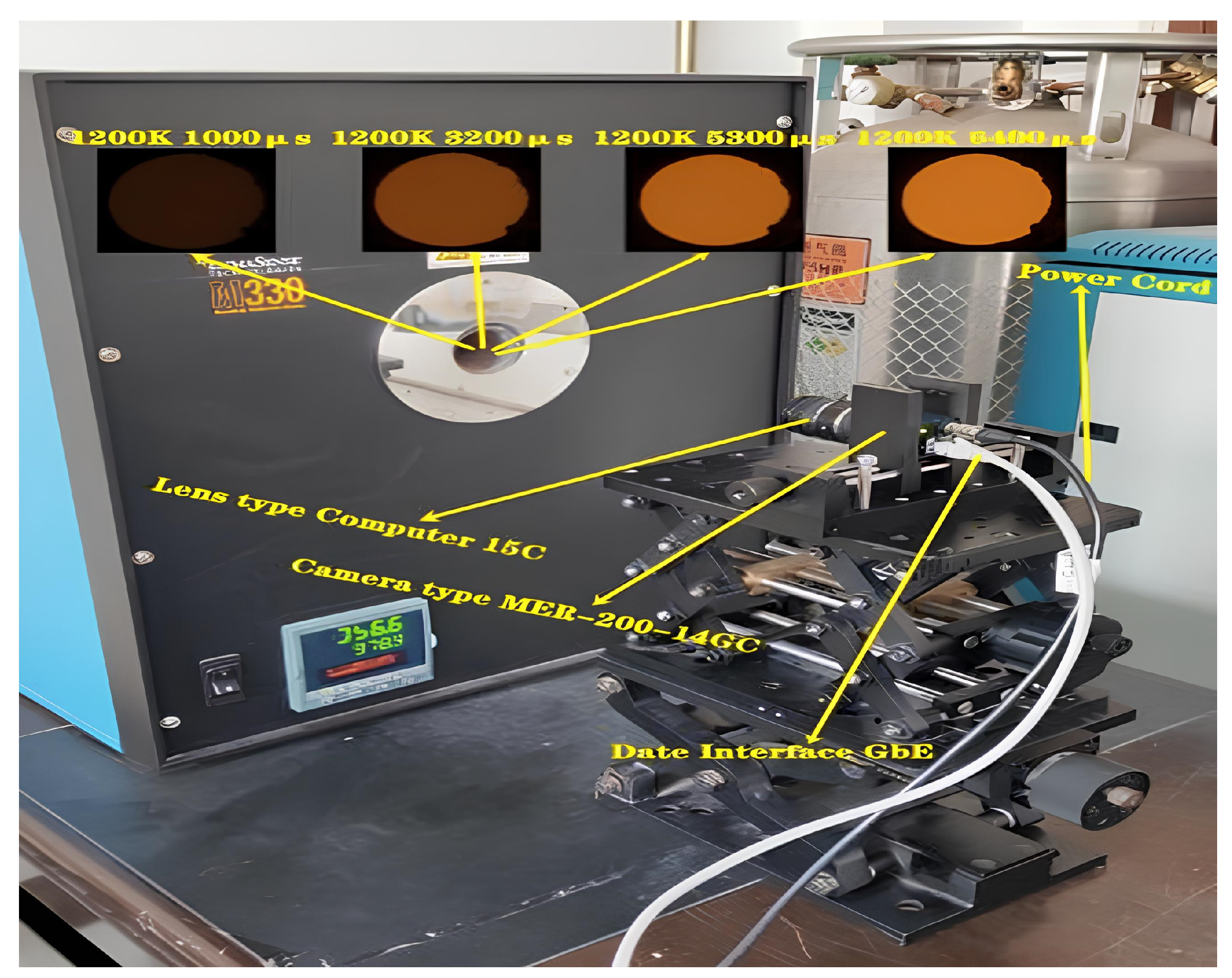
2.1. Experimental Instrument

The experiment utilizes a laminar diffusion flame combustion setup, depicted in Figure 1. This system consists of a burner, gas distribution pipeline, gas cylinder, flow control mechanism, and an optical measurement apparatus. The burner used in the experiment is a Gülder burner. The fuel tube features an inner diameter of 10.9 mm and a wall thickness of 0.95 mm, while the accompanying gas pipe has an inner diameter of 90 mm, consistent with configurations reported in previous studies [20].

The experimental setup, depicted in Figure 2, features a hydrocarbon fuel laminar diffusion flame system with a Gülder burner. The central tube emits propane, while the surrounding annular space releases co-flow gas. The co-flow gas flow is homogenized through small glass beads and a porous metal filter, creating a coaxial laminar diffusion flame at atmospheric pressure. O₂ concentration variations, which are achieved by adjusting the O₂/N₂ mix, allow for different suitable working conditions. A Mercury series industrial camera (MER-200-14GC) which has a 1/1.8” global exposure CCD sensor with a 1628 H × 1236 V resolution, and exposure times were adjusted to ensure precise data capture. While a 15c fixed-focus lens was used to capture visible light thermal radiation images transmitted via a Gigabit Ethernet, the gas flow was regulated using the S48 300/HMT gas mass flow controller from Beijing Horizon Huibolong Precision Instrument Co., Ltd., Beijing, China.

2.2. Experiment Principle

This study employs the two-color method, a non-contact technique for temperature measurement, which enables the temperature sensor to detect radiation signals from the combustion process without physically interacting with the target. This approach, which uses a CCD camera to capture thermal radiation images, preserves the integrity of the temperature field and its distribution, allowing for real-time temperature monitoring through continuous signal capture [21].

The study concentrates on a propane laminar diffusion flame, which exhibits absorption and emission in the absence of scattering or incident radiation. In the visible spectrum, the study focuses on the radiation given off by soot particles, leaving out the effects of triatomic gasses like CO₂ and H₂O. By utilizing intensity data from single and unidirectional boundary images, the study simultaneously reconstructs both the flame temperature and soot volume concentration. Figure 3 illustrates the segmentation of the horizontal cross-section of the axisymmetric flame, establishing a geometric correlation between the flame’s computational units and the camera’s imaging pixel units [22]. The jth line of sight records the following spectral radiation intensity:

$$I_{\lambda }\left({\theta }_j\right)={\displaystyle {\int }_{l_{j0}}^{l_j}{\kappa }_{\lambda }\left(l\right)}{I}_{b,\lambda }\left(l\right)\exp \left[-{\displaystyle {\int }_l^{l_j}{\kappa }_{\lambda }\left(l\prime \right)dl\prime }\right]dl,$$

(1)

where $l$ is the path length of direct radiation intensity, ${\kappa }_{\lambda }\left(l\right)I_{b,\lambda }\left(l\right)$ is the spectral emission source term, $\exp \left[-{\displaystyle {\int }_l^{l_j}{\kappa }_{\lambda }\left(l\prime \right)dl\prime }\right]dl$ is the self-absorption, $I_{b,\lambda }(l)$ is the blackbody radiation intensity of soot particles, and ${\kappa }_{\lambda }$ is the spectral absorption coefficient of the soot particles. The approximate representation based on the Mie theory is typically constrained by Rayleigh conditions [23]:

$$k_{\lambda }={\displaystyle \frac{6\pi \cdot E\left(m\right)\cdot f_v}{\lambda }},$$

(2)

here $f_v$ is the volume fraction of soot, $E\left(m\right)$ is the function of the optical constant of soot, and m is the gradient refractive index function with real and imaginary parts.

$$E\left(m\right)=\mathrm{Im}\left|{\displaystyle \frac{m^2-1}{m^2+1}}\right|={\displaystyle \frac{6nk}{{\left(n^2-k^2+2\right)}^2+4n^2{k}^2}},$$

(3)

where n and k represent the refractive index and absorptivity, respectively. According to the literature [24], $E\left(m\right)$ = 0.26 (n = 1.57, k = 0.56).

The blackbody spectral radiation intensity $I_{b,\lambda }(l)$ can be determined using Planck’s radiation law:

$$I_{b,\lambda }={\displaystyle \frac{c_1{\lambda }^{-5}}{e^{\left(c2/\lambda T\right)}-1}}.$$

(4)

This study assumes that the image pixel values in the R and G channels are proportional to the monochromatic radiation intensity, despite the broader spectral response curves of these bands. By considering the camera’s spectral response characteristics in the R and G bands, the directional emission power E_R and E_G can be calculated as follows [25]:

$$\begin{array}{l}{E}_R\left({\theta }_j\right)={\displaystyle {\int }_{{\lambda }_{R1}}^{{\lambda }_{R2}}}{\eta }_R\left(\lambda \right)I_{\lambda }\left({\theta }_j,\lambda \right)d\lambda \\ E_G\left({\theta }_j\right)={\displaystyle {\int }_{{\lambda }_{G1}}^{{\lambda }_{G2}}{\eta }_R\left(\lambda \right)I_{\lambda }\left({\theta }_j,\lambda \right)d\lambda .}\end{array}$$

(5)

The equations provided above can be used to determine both the temperature and soot volume fraction.

The reliability of these measurements significantly depends on the accuracy of image acquisition. To achieve precise mapping between radiation intensity and the color values in flame images, visible light radiation calibration of the imaging system is essential. This calibration process establishes a functional relationship between the radiation intensity channels and the image’s color values. A blackbody furnace, known for its stable radiation coefficient, is employed as the standard radiation source, with its temperature serving as the brightness temperature. Following the calibration method proposed by Wu Yonghua, thermal radiation images of the blackbody furnace are captured using a color CCD camera, allowing for the correlation between temperature and RGB values [21]. This correlation produces a fitting curve that is used to calibrate the monochromatic intensity values (R, G, B) in relation to the blackbody temperature. The calibration setup requires equipment such as a high-temperature blackbody furnace, CCD camera, computer, and transmission lines, as illustrated in Figure 4.

2.3. Experimental Condition

Flame images were captured using a CCD camera mounted on a laminar combustion test bench designed for hydrocarbon fuels. The two-color flame emission method, employing R and G channel response bands, was used to reconstruct the two-dimensional distribution of flame temperature and soot volume fraction for propane in a C₃H₈/(O₂/N₂/CO₂) atmosphere. The experimental conditions for this study are provided in

Table 1. Experimental condition.

Test Conditions	Fuel Flow/(mL·min−1)	OI	Co-Flow Air Flow Rate/(L·min−1)
	C3H8		Total	N2
Case 1	109.8	19%	40	32.4
Case 2	109.8	21%	40	31.6
Case 3	109.8	25%	40	30
Case 4	109.8	29%	40	28.4
Case 5	109.8	33%	40	26.8

.

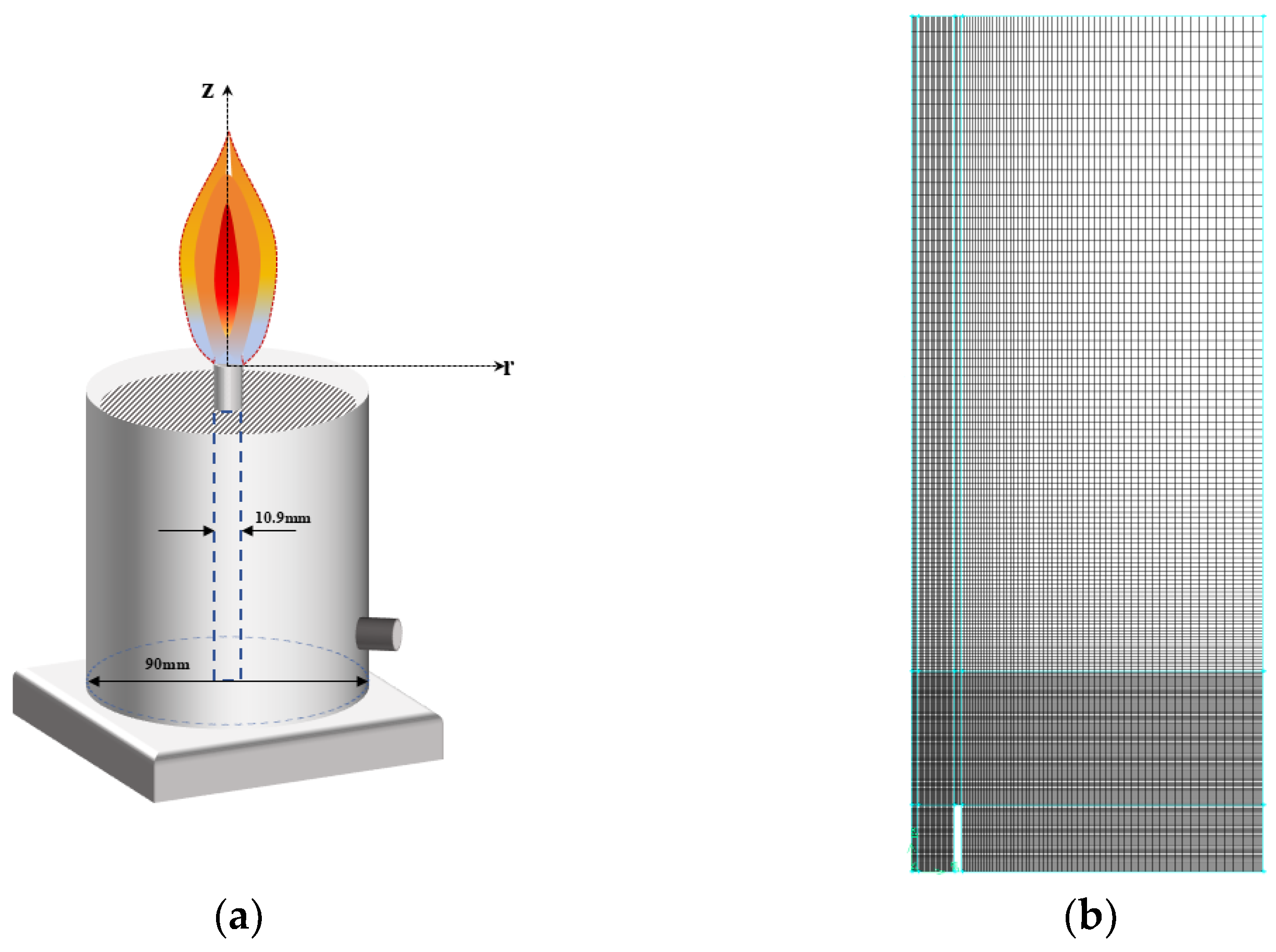
2.4. Numerical Simulation

The numerical simulations for laminar co-flow diffusion flames involving C₃H₈/(O₂/CO₂) and C₃H₈/(O₂/H₂O) mixtures were conducted using a computational domain structured to reflect the geometry of the actual Gülder burner. As illustrated in Figure 5, a two-dimensional axisymmetric domain was utilized to optimize computational efficiency and ensure accuracy of the simulation results. The domain has axial and radial dimensions of 118 mm and 45 mm. Furthermore, this is discretized into axial and radial control volumes of 194 and 88 mm, with total quadrilateral cells of 20,052. A structured grid with non-uniform spacing was used, focusing refinement in the main reaction area. Initially, a uniform fine grid with 0.2 mm spacing was used for the first 30 mm in the axial direction, followed by a gradual expansion with a factor of 1.0205 across 94 nodes. In the radial direction, the grid initially had a uniform spacing of 0.2 mm for the first 0.8 mm, followed by 19 evenly spaced nodes from 0.80 to 5.45 mm. The interval from 5.45 to 6.45 mm was divided into 4 nodes, with spacing gradually increasing on the gas side, utilizing a total of 61 equidistant nodes and an expansion factor of 1.025. To ensure accurate resolution of boundary layer phenomena, the computational domain extended 10 mm upstream of the fuel nozzle to better capture the fuel outlet velocity profile [26]. The quadrilateral mesh provided the geometric flexibility necessary for structured grid refinement while maintaining computational efficiency.

A grid independence study was conducted to validate the reliability and accuracy of the computational results. This analysis involved comparing the peak flame temperature profiles obtained using three different grid resolutions: a coarse grid with 10,026 cells, a medium grid with 20,052 cells, and a fine grid with 40,104 cells. These grids were systematically refined, particularly in the primary reaction zone where steep gradients in temperature and species concentrations are present.

The peak flame temperature, a critical indicator of combustion dynamics, was selected as the key metric for evaluating the grid sensitivity. The temperature distribution along the flame centerline of the propane axial diffusion flame in an air atmosphere was calculated for each grid resolution (see Figure 6). The medium and fine grids exhibited negligible differences in peak temperature values, with a relative deviation of less than 1%. In contrast, the coarse grid showed noticeable deviations, indicating insufficient resolution for capturing the combustion physics accurately. The findings confirmed that the medium grid with 20,052 cells provides a satisfactory balance between computational efficiency and result accuracy. Based on this validation, the medium grid was adopted for all simulations in this study.

For the boundary conditions, a velocity inlet was designated for both the fuel and accompanying gas inlets. The propane was introduced at a velocity of 1.96 cm/s, while the velocity of the accompanying gas varied depending on the specific working conditions, as detailed in Table 1. The side boundaries were treated as walls with an isothermal condition set at 300 K. To account for fuel preheating, the nozzle wall temperature was elevated to 400 K, while the outlet was maintained at 300 K with a pressure outlet condition, ensuring a smooth return at the outlet boundary. The chemical reaction mechanism used for the gas phase was GRI-Mech3.0, developed by the University of California, Berkeley, CA, USA, which was optimized for simulating the combustion of C1–C3 hydrocarbons and included a 219 elementary reaction with 36 species [27]. All simulations were conducted using ANSYS FLUENT 14.0, where the discrete ordinates (DO) radiation model was applied for radiation heat transfer calculations. The radiative properties of the gas and soot were determined using the weighted sum of the gray gasses model (WSGGM), as formulated by Smith et al. [28] Although the default WSGGM has limitations when applied to oxy-flames, the dominant mechanisms in this study, such as chemical kinetics and thermal effects, remain unaffected. This was verified by the consistent results observed in the experimental and numerical comparisons, as discussed in Section 3.1 and Section 3.2. The Moss–Brookes soot model was employed, which incorporates the polymerization of acetylene as the initial nucleation step for soot formation and utilizes acetylene to promote surface growth. This model effectively captures the soot nucleation, surface growth, and oxidation processes. For the numerical solution, a solver based on pressure–velocity coupling was used, specifically applying the SIMPLE algorithm to manage the coupling between pressure and velocity fields. Temperature monitoring was implemented to ensure stability; once the temperature reached equilibrium, the simulation results were considered converged. The simulation process began with a cold-state simulation, which was followed by the introduction of chemical reactions. To simulate ignition, a high-temperature area was established within the ethylene and oxygen cold mixing zone.

This study employed a distribution separation technique to distinguish the density, transport, thermal, and chemical effects of CO₂ by incorporating multiple virtual species into the simulation model. The design conditions for different oxidant flow compositions, using CO₂ as a reference, are presented in

Table 2. Design conditions of different oxidant components.

Case	Oxidant Composition (Mole Fraction)							Remark
	XO2 **	XN2	XF1CO2	XF2CO2	XFCO2	XCO2	The Velocity of Co-Current Gas Flow/(m·s−1)
3-1	0.3	0.7					0.1783	Bench
3-2			0.7				0.1783	1 and 2, Density effect
3-3				0.7			0.1783	2 and 3, Transport effect
3-4					0.7		0.1783	3 and 4, Thermal effect
3-5						0.7	0.1783	4 and 5, Chemical effect

** X is used to refer to virtual matter, and these symbols in Table 2 and Table 3 (e.g., XF, XF1, XF2) are only used to identify virtual species with different effects and have no actual physical meaning.

. Alongside the baseline conditions, four additional cases (3-2 through 3-5) were defined. In Case 3-2, the virtual species F1CO₂ was introduced with identical thermal and transport properties to N₂. Also, chemically inert and non-reactive F1CO₂ mirrored the elemental composition of CO₂ and has a greater molar mass than N₂. Consequently, the oxidant’s mixing density exceeded that of the reference condition. The variation in calculation outcomes between Case 3-2 and Case 3-1 is thus attributed to the density effect of CO₂. In Case 3-3, the virtual species F2CO₂ was introduced, which has the same transport properties and elemental composition as CO₂, but with the thermal properties of N₂ and chemically inert. The difference in outcomes between Case 3-3 and Case 3-2 highlights the transport effect of CO₂. Furthermore, in Case 3-4, the virtual species FCO₂ replicates the thermal, transport properties, and elemental composition of real CO₂ without engaging in chemical reactions. The thermal effect of CO₂ is identified by comparing Case 3-4 with Case 3-3, whereas the chemical effect is determined by the difference between Case 3-5 and Case 3-4 reveals the chemical effect of CO₂. Similarly, to isolate the effects of H₂O, multiple virtual species were introduced, forming different oxidant flow compositions under similar design conditions, As shown in

Table 3. Design conditions of different oxidant components.

Case	Oxidant Composition (Mole Fraction)							Remark
	XO2	XN2	XF1H2O	XF2H2O	XFH2O	XH2O	The Velocity of Co-Current Gas Flow/(m·s−1)
3-6	0.3	0.7					0.1783	Bench
3-7			0.7				0.1783	1 and 2, Density effect
3-8				0.7			0.1783	2 and 3, Transport effect
3-9					0.7		0.1783	3 and 4, Thermal effect
3-10						0.7	0.1783	4 and 5, Chemical effect

.

Table 4. Physical properties of N₂, CO₂ and H₂O (1000 K, 0.1 MPa).

Cas	Density (kg/m3)	Heat Capacity (J/mol·K)	Isobaric Specific Heat Capacity (J/mol·K)	Coefficient of Thermal Conductivity (W/m·K)	Thermal Diffusivity (m2/s)
N2	0.34	32.963	41.293	0.097085	1.95 × 10−4
CO2	0.54	46	54.322	0.070571	1.08 × 10−4
H2O	0.22	24.386	32.703	0.065991	1.68 × 10−4

summarize the physical properties of the three diluents.

3. Results and Analysis

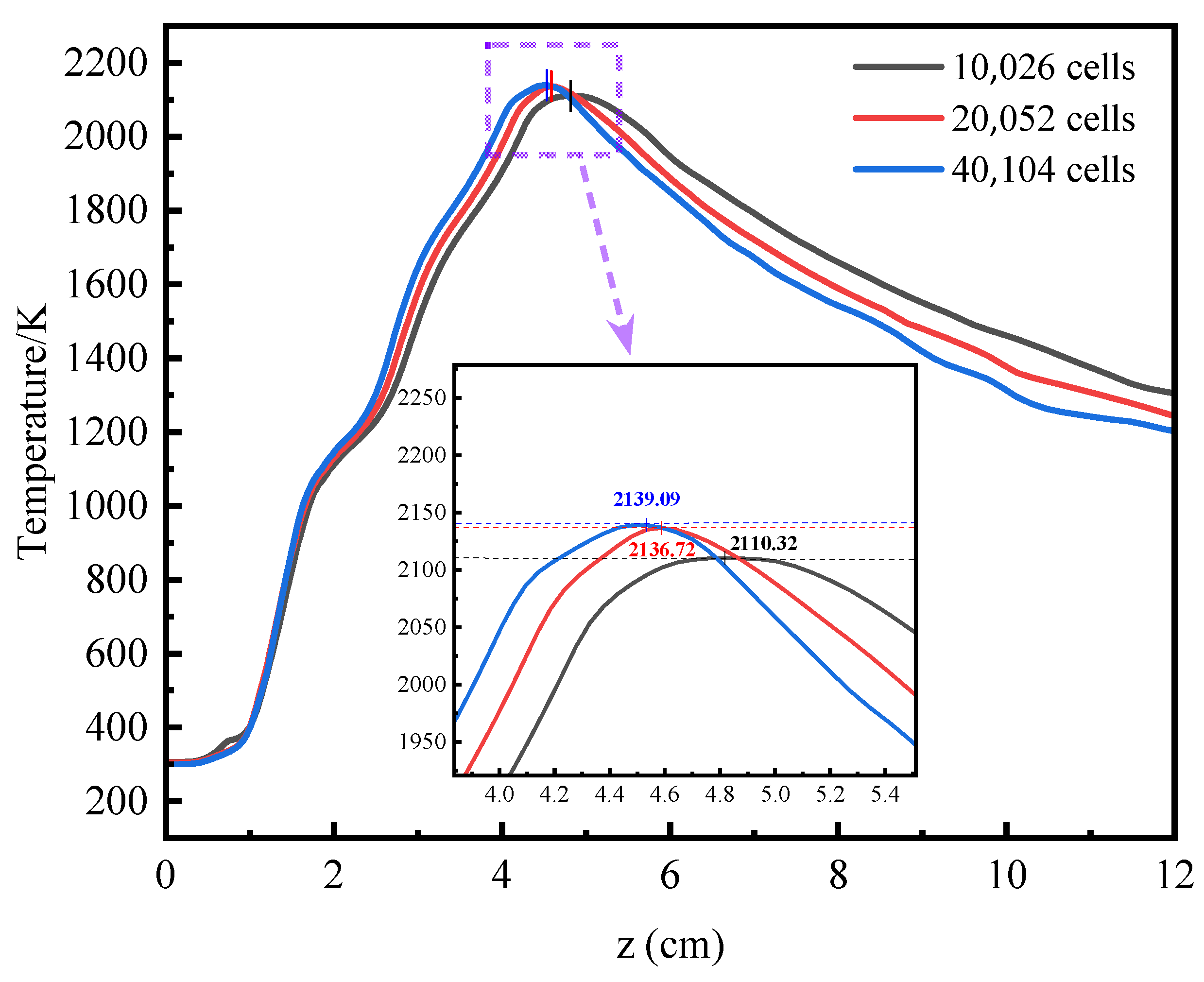
3.1. Verification

A comparative analysis was performed to validate the accuracy of the visible light imaging measurement technique, the reconstruction algorithm, and the numerical calculations. The reconstructed and numerically calculated results for propane combustion at oxygen concentrations of 21% and 25% were evaluated against the experimental data presented by Chu et al. [29]. This comparison serves to verify the reliability of the methods and algorithms employed in the study. The experimental conditions and numerical calculations for Case 2 and Case 3 largely align with those reported in the previous literature, with the primary distinction being the different oxidant flow rate settings used in this study. However, given that the oxidant flow rate has a negligible impact on the overall characteristics of conventional diffusion flames, the soot volume fraction (SVF) and temperature distribution in Case 2 and Case 3 can still be reliably compared with other technical measurement results. At a flame height of 30 mm, Figure 7 and Figure 8 illustrate the radial distribution of temperature and SVF. The experimental measurements and numerical calculations for Case 2 and Case 3 exhibit trends consistent with those reported in the literature, confirming the effectiveness of the measurement method and numerical approach in accurately capturing the SVF and temperature distribution in the diffusion flame. Although radiative heat transfer can contribute significantly to the energy balance, the close agreement between experimental and simulated peak SVF and temperature values suggests that the key conclusions of this study are primarily influenced by chemical kinetics and thermal mechanisms. The potential uncertainties in radiative heat transfer calculations, due to the default WSGGM, do not significantly alter the observed trends in soot formation and temperature distribution. Measurement noise near the flame’s center axis during the experimental process causes minor unevenness in SVF and temperature distributions. While the experimentally predicted peak SVF area at the two wings is marginally closer to the flame center axis compared to the numerical predictions, the predicted peak SVF and peak temperature are closely aligned. To further substantiate the accuracy of the numerical model,

Table 5. Error analysis of peak temperature and soot volume fraction.

OI	Parameter	Current Numerical Results	Experimental Results	Chu et al. [29] Results	Relative Error (%): Current vs. Experimental	Relative Error (%): Current vs. Chu et al. [29]
21%	Peak Temperature (K)	1986.58	1933.31	1922.31	2.76	3.34
	Peak SVF (ppm)	2.65	2.70	2.51	1.85	5.58
25%	Peak Temperature (K)	2161.71	2106.57	2172.41	2.62	0.49
	Peak SVF (ppm)	4.71	4.86	4.52	3.09	4.20

provides an error analysis of the peak temperature and peak SVF values. The table demonstrates that the numerical predictions are within acceptable error margins, further validating the reliability of the numerical calculations and reconstruction algorithm employed in this study.

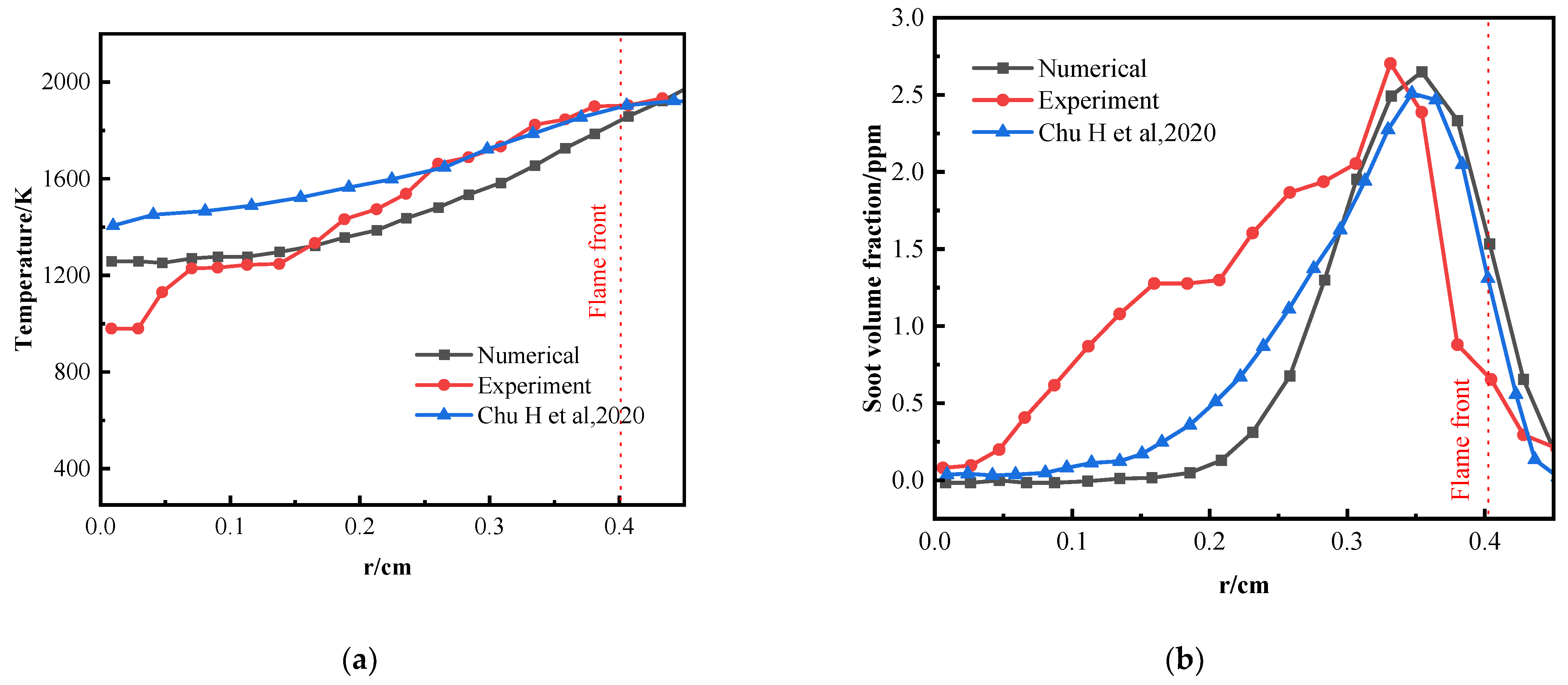
3.2. Numerical and Experimental Comparisons

Figure 9 shows the visual images of propane flames in an O₂/N₂ atmosphere, illustrating the flame characteristics at different oxygen concentrations (19%, 21%, 25%, 29%, and 33%). The figure reveals that, under a constant propane flow rate, increasing the oxygen concentration from 19% to 33%, with a co-flow gas total flow rate of 40 L/min, causes the flame height to decrease significantly from 82 mm to 39 mm, marking a reduction of 52.4%. The most pronounced change occurs when the oxygen concentration rises from 21% to 25%, resulting in a decrease in flame height from 72 mm to 58 mm, a reduction of 19.4%. As the oxygen concentration increases, the flame’s brightness intensifies, shifting from a dark yellow hue to a bright white. This change in thermal radiation characteristics is indicative of the higher emissivity of soot particles compared to gasses, which enhances the flame’s radiative heat transfer. Even a small amount of soot can emit a strong yellow light, consistent with the phenomena observed by Chu et al. [29] in a C₃H₈/(O₂/N₂) atmosphere. As oxygen concentration rises, the dark shadow area at the flame’s lower part diminishes, which shows that increased oxygen levels promote soot formation.

Due to the inability of experimental methods to capture the detailed processes of soot formation, numerical simulations were employed for a more in-depth analysis. Based on both experimental measurements and numerical simulations, Figure 10 and Figure 11 present the two-dimensional distribution of soot volume fraction distribution in a C₃H₈/(O₂/N₂) flame, derived from experimental measurements and numerical simulations. Comparing these results reveals that both methods produce consistent distribution areas. Both approaches predict that the peak soot concentration occurs in the annular regions of the flame wings. Nevertheless, the numerical simulation forecasts the soot peak at a lower axial position compared to the experimental observations. This discrepancy may be due to the numerical simulation’s inability to accurately capture the soot concentration along the flame’s centerline. The likely cause of the variance is the intrinsic limitations of the soot model and the sensitivity of the current two-color measurement method. The difference may be due to the absence of polycyclic aromatic hydrocarbon (PAH) formation pathways in the simulation, which are crucial for the formation of soot [30]. The comparison of temperature distributions, as illustrated in Figure 12 and Figure 13, reveals that the high-temperature regions are primarily concentrated in the wings of the flame. The peak flame temperature of the C₃H₈/(O₂/N₂) mixture rises significantly with increasing oxygen concentration. This increase can be attributed to the higher mole fraction of O₂ in the co-flow gas, which enhances the flame’s capacity to entrain O₂. As a result, there is a greater production of reactive free radicals such as O and OH, which in turn accelerates the combustion reactions occurring downstream [31].

Figure 14 depicts the rates of soot mass nucleation and surface growth in both O₂/N₂ and O₂/CO₂ environments. Higher oxygen concentrations enhance soot formation by increasing nucleation and surface growth rates. A notable decrease in soot nucleation and surface growth rates is evident when comparing O₂/N₂ and O₂/CO₂ atmospheres with identical O₂ concentrations, underscoring CO₂’s suppressive effect. The observed suppression of soot formation in CO₂-rich atmospheres can be attributed to several plausible mechanisms: (1) CO₂ likely acts as a thermal buffer due to its high specific heat capacity, which reduces flame temperatures and consequently limits the thermal decomposition of fuel into soot precursors. (2) CO₂ may participate in chemical interactions that consume intermediate hydrocarbon species, thereby decreasing the availability of key precursors for soot nucleation and growth. (3) the radiative properties of CO₂ could enhance heat losses from the flame, further diminishing soot formation processes. From an environmental perspective, reducing soot nucleation and growth can lower particulate matter emissions, a major contributor to air pollution and adverse health effects. Additionally, replacing N₂ with CO₂ in oxidizing atmospheres may support the development of sustainable combustion strategies, particularly in applications such as carbon capture and storage (CCS), where CO₂ is recycled or sequestered. These findings suggest that CO₂ addition offers a promising approach to achieving cleaner and more efficient energy production.

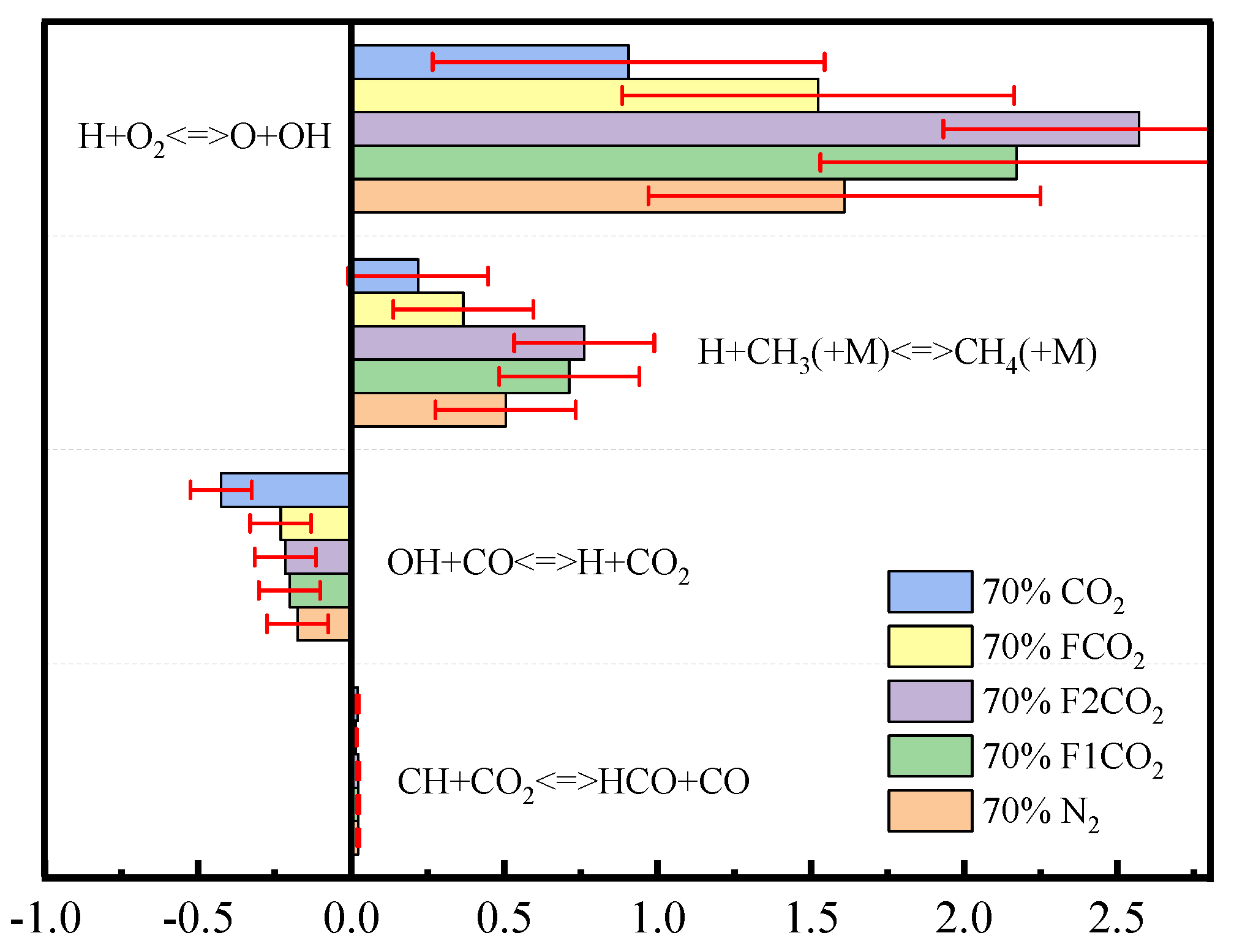
3.3. Impacts of CO₂ Addition: Combustion Sensitivity Analysis

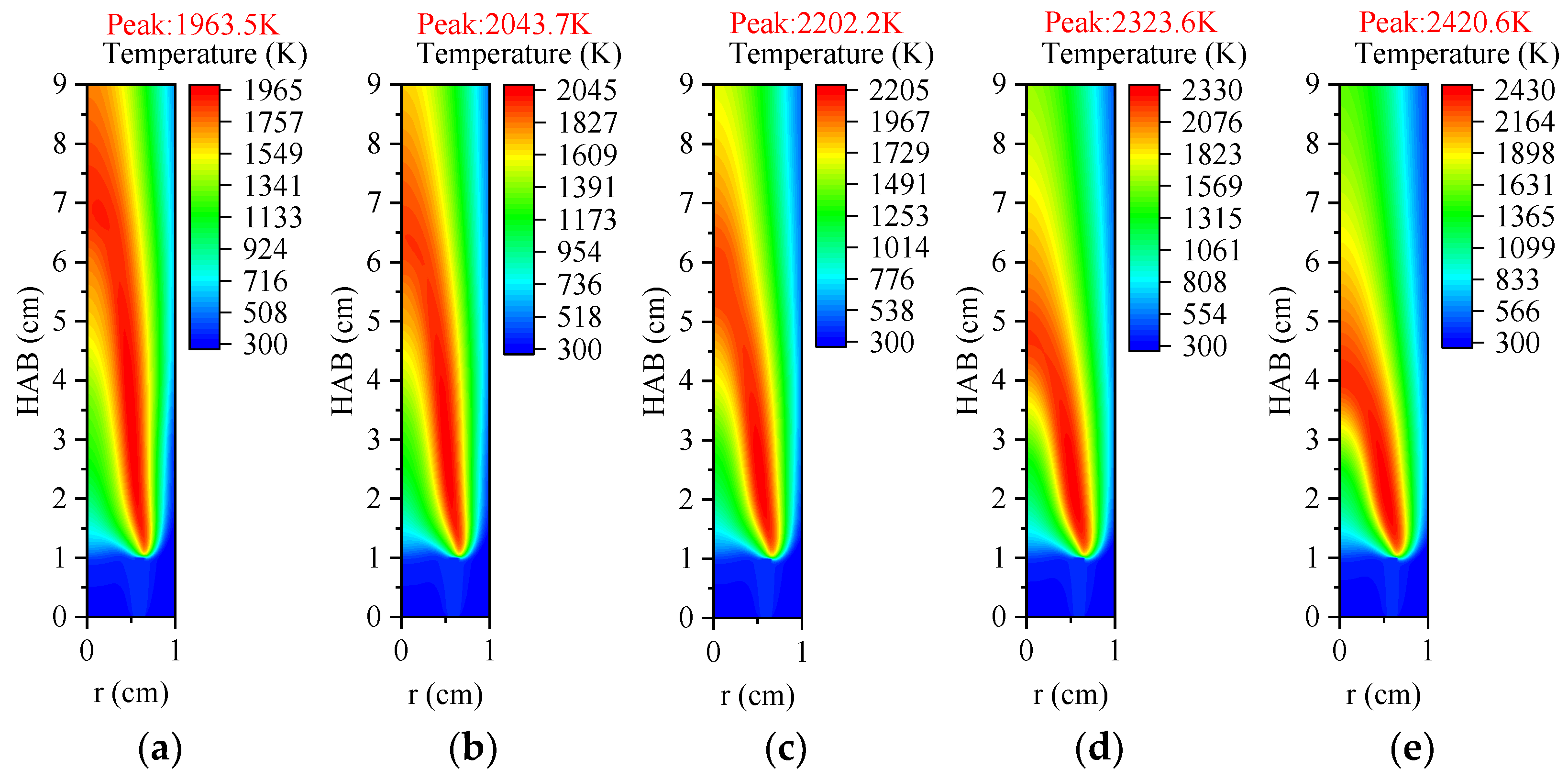
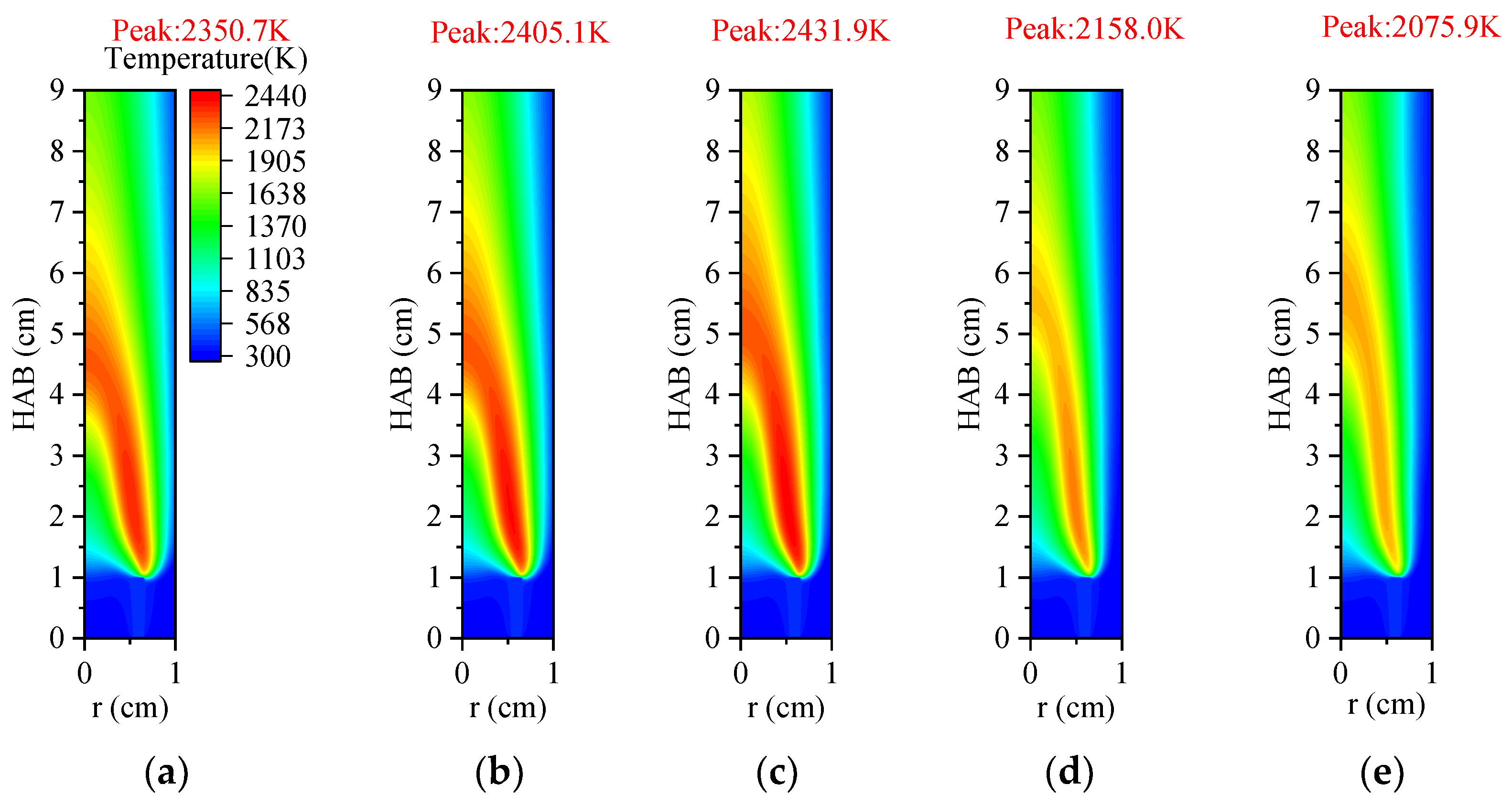
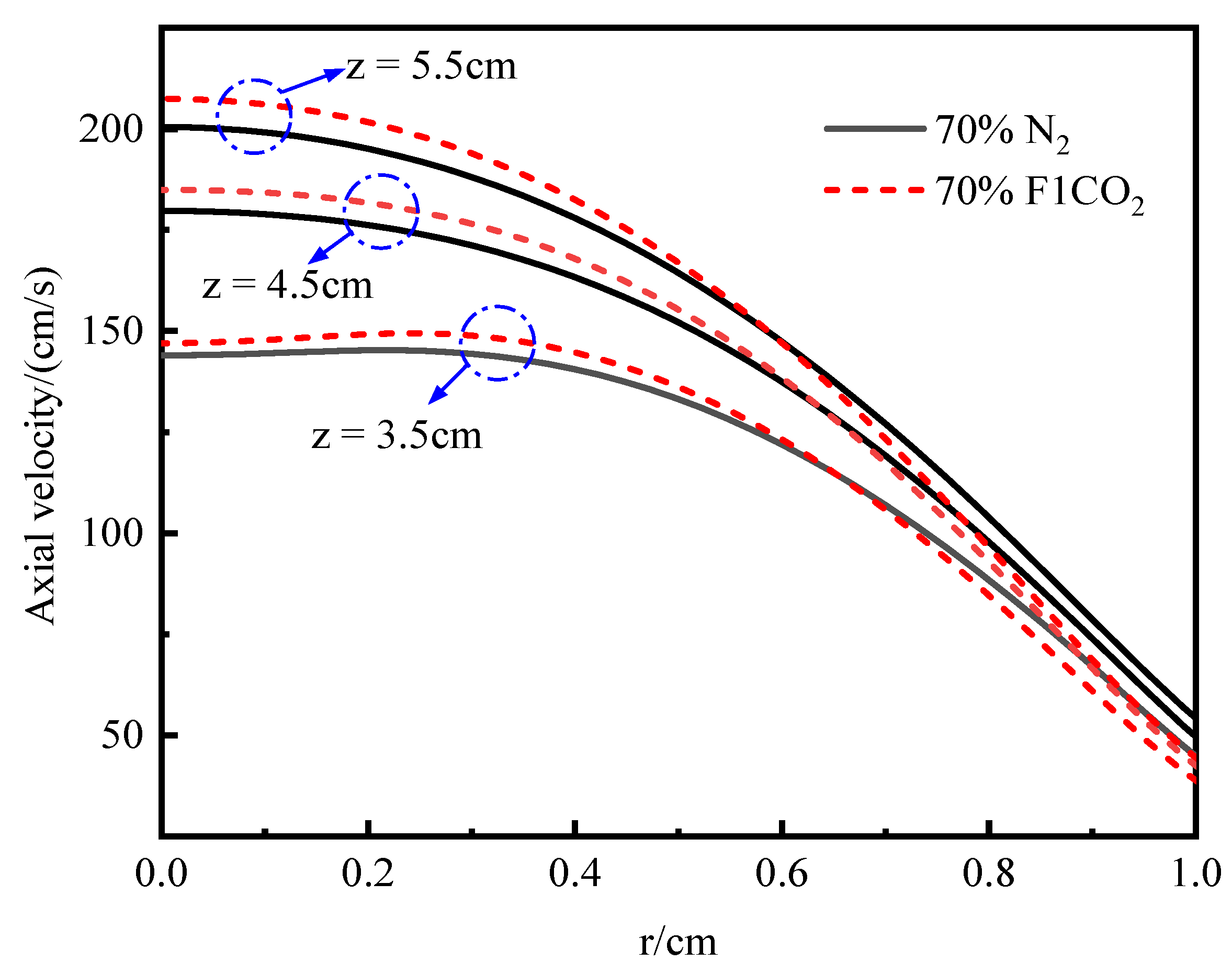
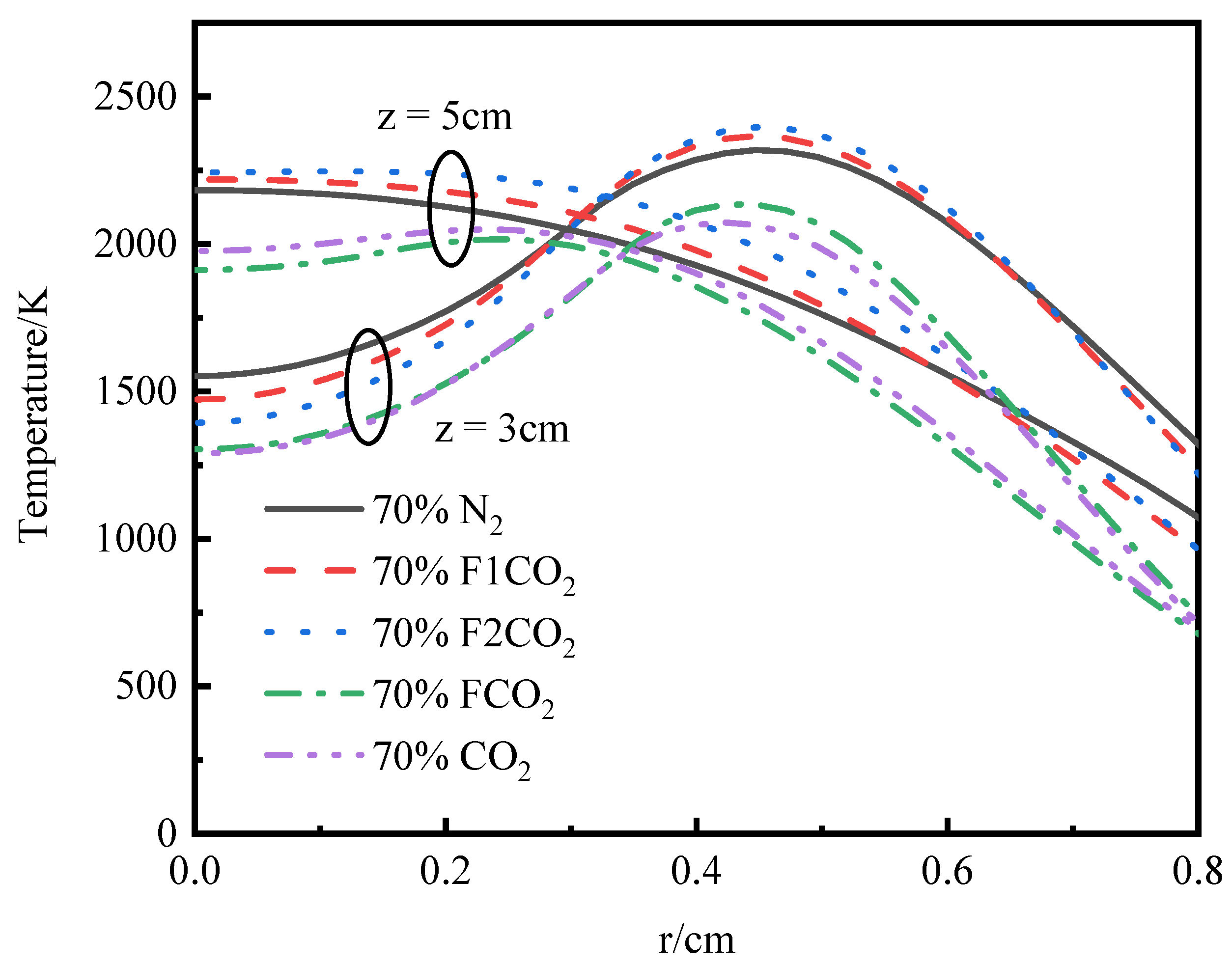
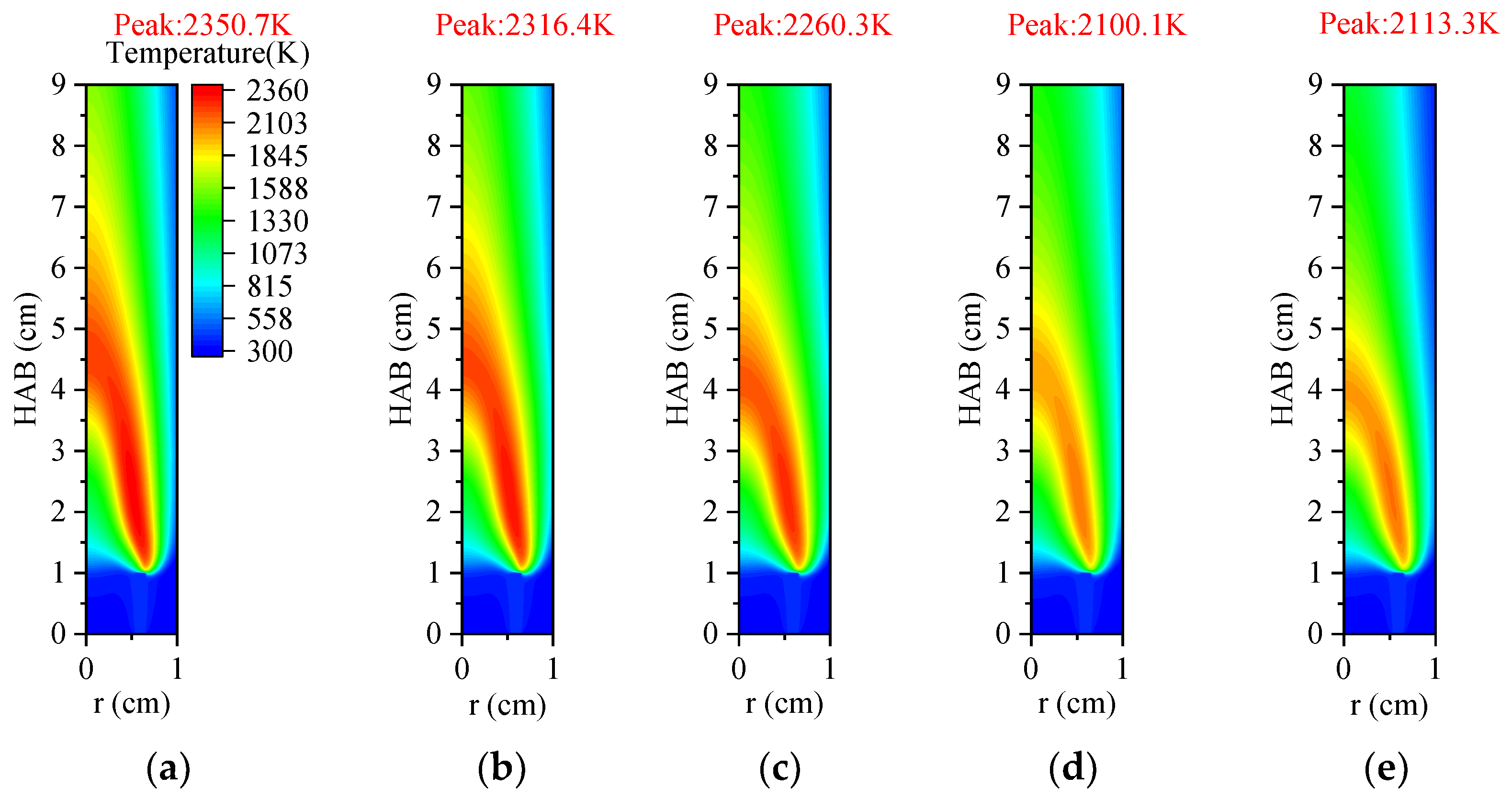
3.3.1. Temperature and Axial Flow Velocity

Figure 15 and Figure 16 present the two-dimensional temperature distributions and central axis temperatures under different conditions to examine the effect of CO₂. As shown in Figure 15, the temperature variations are predominantly influenced by the thermal effect of CO₂, which is due to its higher heat capacity. Subsequently, the chemical and density effects contribute to the temperature variations, whereas the transport effect has a minimal influence on the overall temperature distribution, typically leading to a modest increase in flame temperature. These results align with the findings reported by Mahmoud et al. [32] which examined the impact of CO₂ dilution on n-heptane co-flow diffusion flames in oxygen-rich conditions. Moreover, when CO₂ is substituted with FCO₂, a noticeable increase in peak temperature occurs, indicating that the influence of chemical interactions from CO₂ significantly lowers the peak temperature. This observation also aligns with the conclusions of Wang et al. [33] from their experimental and numerical analysis of soot formation in a laminar co-flow H₂/C₂H₄ diffusion flame under O₂/CO₂ conditions.

Figure 16 illustrates that, with flame heights under 6 cm and a constant outlet velocity, the axial velocity of the flame is greater in the atmospheres of O₂/N₂, relative to O₂/CO₂. This difference is primarily due to the temperature reduction resulting from the thermal and chemical effects of CO₂.

The influence of CO₂’s density on velocity and temperature fields is examined by analyzing the radial distribution of axial velocity at different heights above the burner, as illustrated in Figure 17. Near the flame axis, the axial velocity for Case 3-2 (with 70% F1CO₂) is marginally higher than in the reference Case 3-1. For positions beyond 0.6 cm from the flame axis, the axial velocity in Case 3-2 is marginally less than in Case 3-1. This difference is due to the higher molar mass of F1CO₂ (44.009 g/mol) compared to N₂ (28.013 g/mol), resulting in a denser co-flow gas in Case 3-2. In the oxidant region, the increased density away from the reaction zone reduces the mixture’s buoyancy, resulting in a reduced axial velocity. Additionally, the extended mixing time between the fuel and oxidant enhances combustion intensity, leading to an increase in flame temperature.

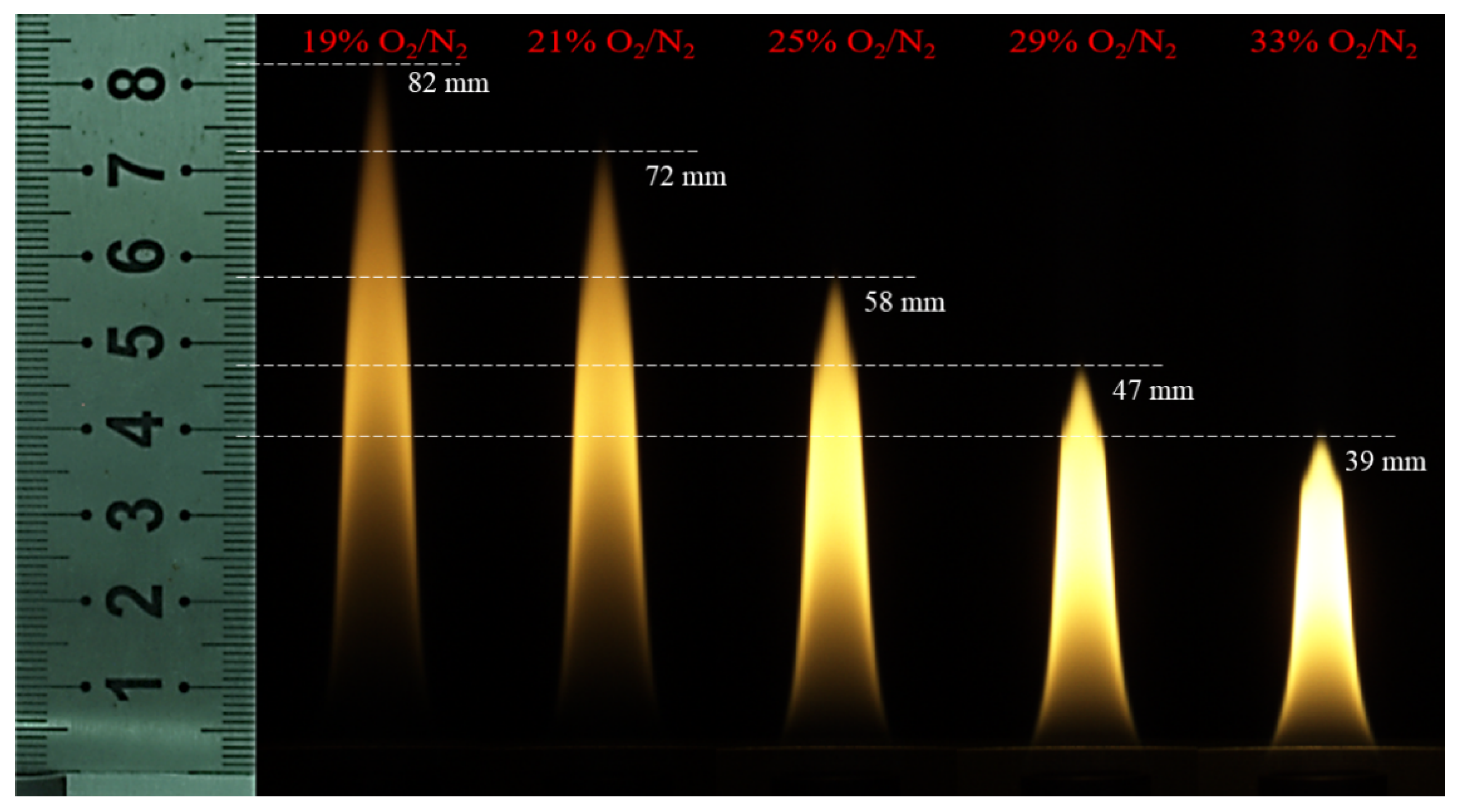
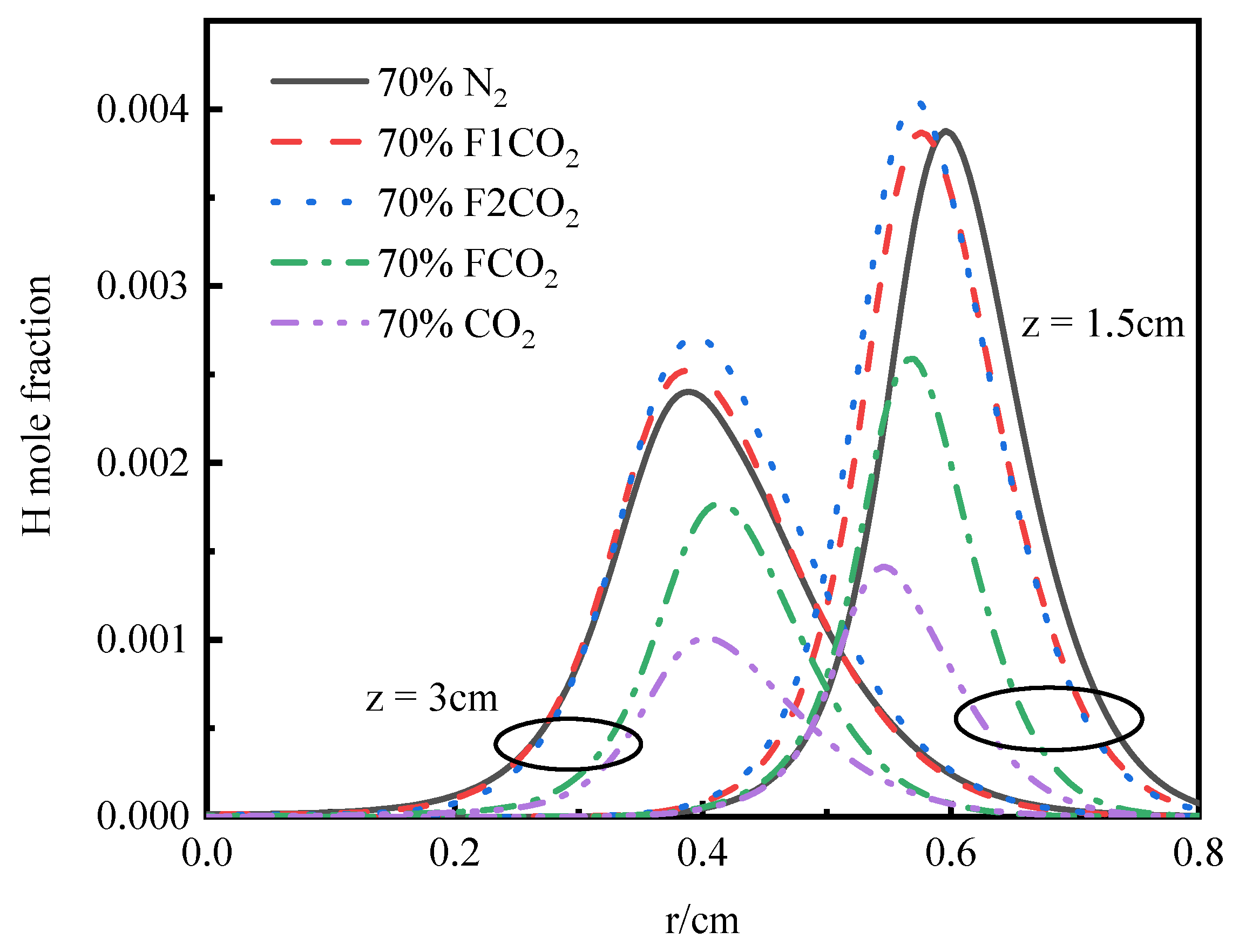
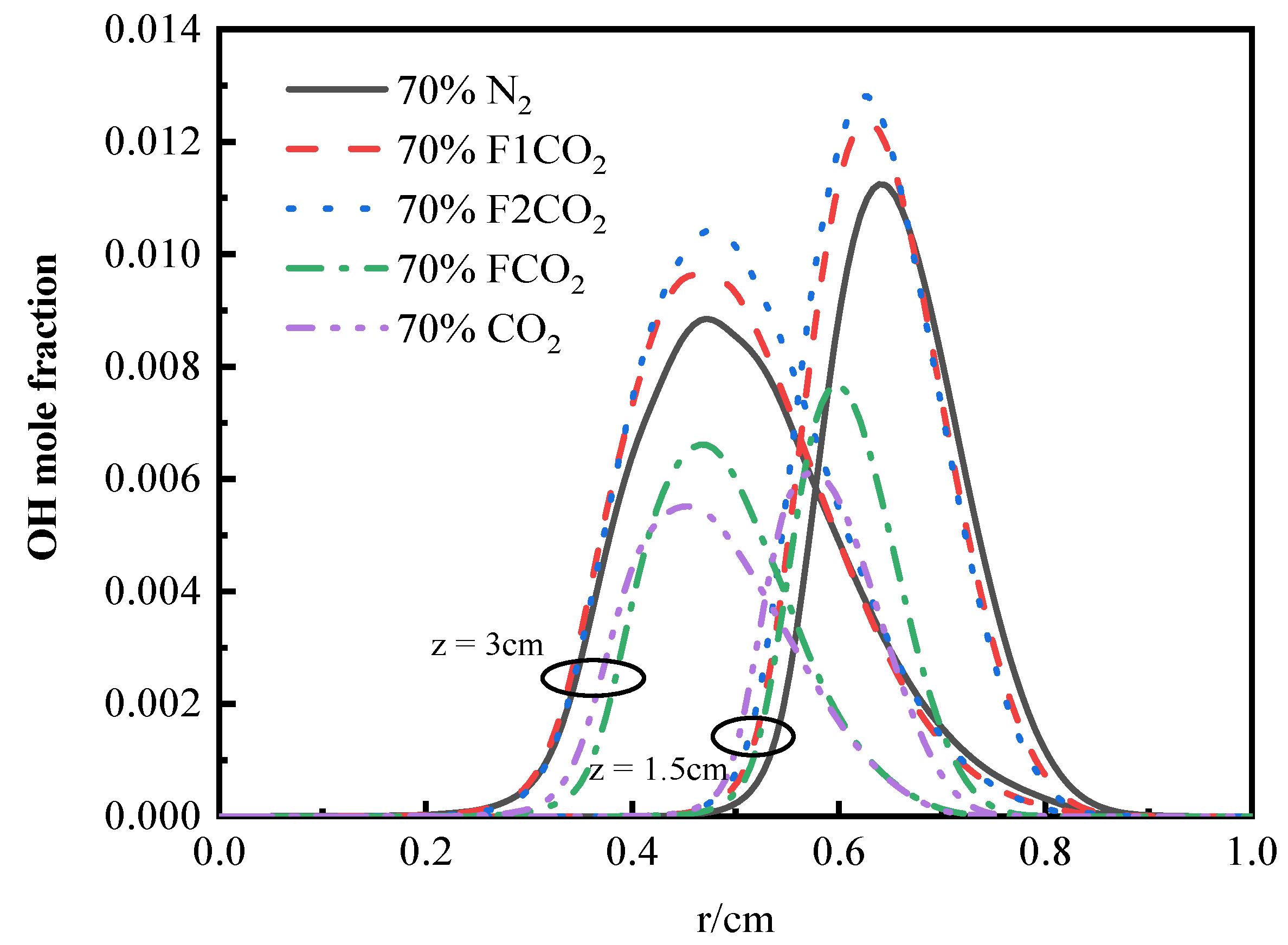
3.3.2. OH Radical Distribution and Flame Height

Lee’s soot oxidation model highlights OH radicals as key oxidants in combustion, representing the fragmentation state within a flame [34]. Figure 18 illustrates the two-dimensional distribution of OH radical mole fractions under various calculation conditions. The results reveal that OH radicals are predominantly located in the flame’s wings, with peak concentrations occurring less than 2 cm above the burner outlet. The effect of CO₂ on the distribution of OH radicals mirrors its impact on the temperature distribution, suggesting that OH concentration serves as a reliable combustion intensity indicator. When O₂/CO₂ is present in the atmosphere, the peak mole fraction of OH is noticeably lower than when O₂/N₂ is present. Specifically, CO₂ reduces the peak OH mole fraction by 37.4% due to its thermal effect, relative to 22.0% due to its chemical effect. Conversely, CO₂ raises the peak OH concentration by 9.6% and 3.1%, respectively. Although CO₂’s density and transport effects slightly elevate OH concentrations, its thermal and chemical impacts cause a substantial decrease.

Flame height can be defined in a variety of ways, with some researchers describing it as where the temperature reaches its maximum value along the flame centerline [13,16,35]. Using this definition, Figure 19 illustrates the impact of various CO₂ effects on flame height. The increased axial velocity in the reaction zone, due to CO₂’s density effect, allows the flow to travel a greater distance within the same residence time. The transport effect of CO₂ also results in a taller flame height, which occurs because the binary diffusion coefficient of CO₂–O₂ mixtures is less than that of N₂-O₂ mixtures. As a result, CO₂’s transport properties replace those of N₂ in the oxidant, reducing oxygen diffusion and requiring a higher flame position for complete combustion. Also, the thermal effect of CO₂ increases flame height, possibly due to CO₂’s high specific heat capacity, which absorbs more heat, reduces combustion intensity, and consequently raises flame height. This phenomenon aligns with the reduced combustion intensity associated with lower OH concentrations. In addition, CO₂ contributes chemically to an increased flame height and diminished combustion intensity, as illustrated by the OH distribution in Figure 18.

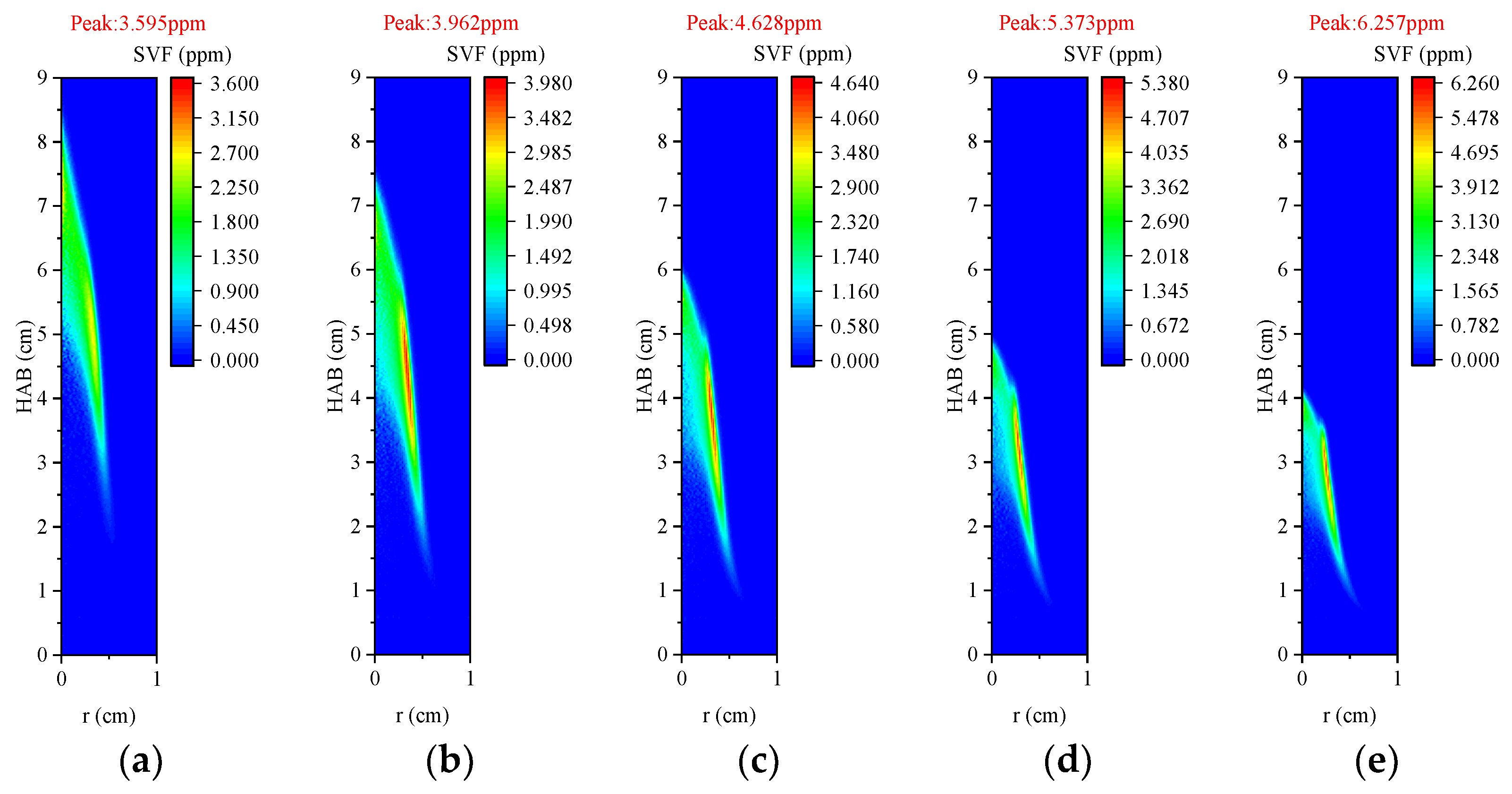
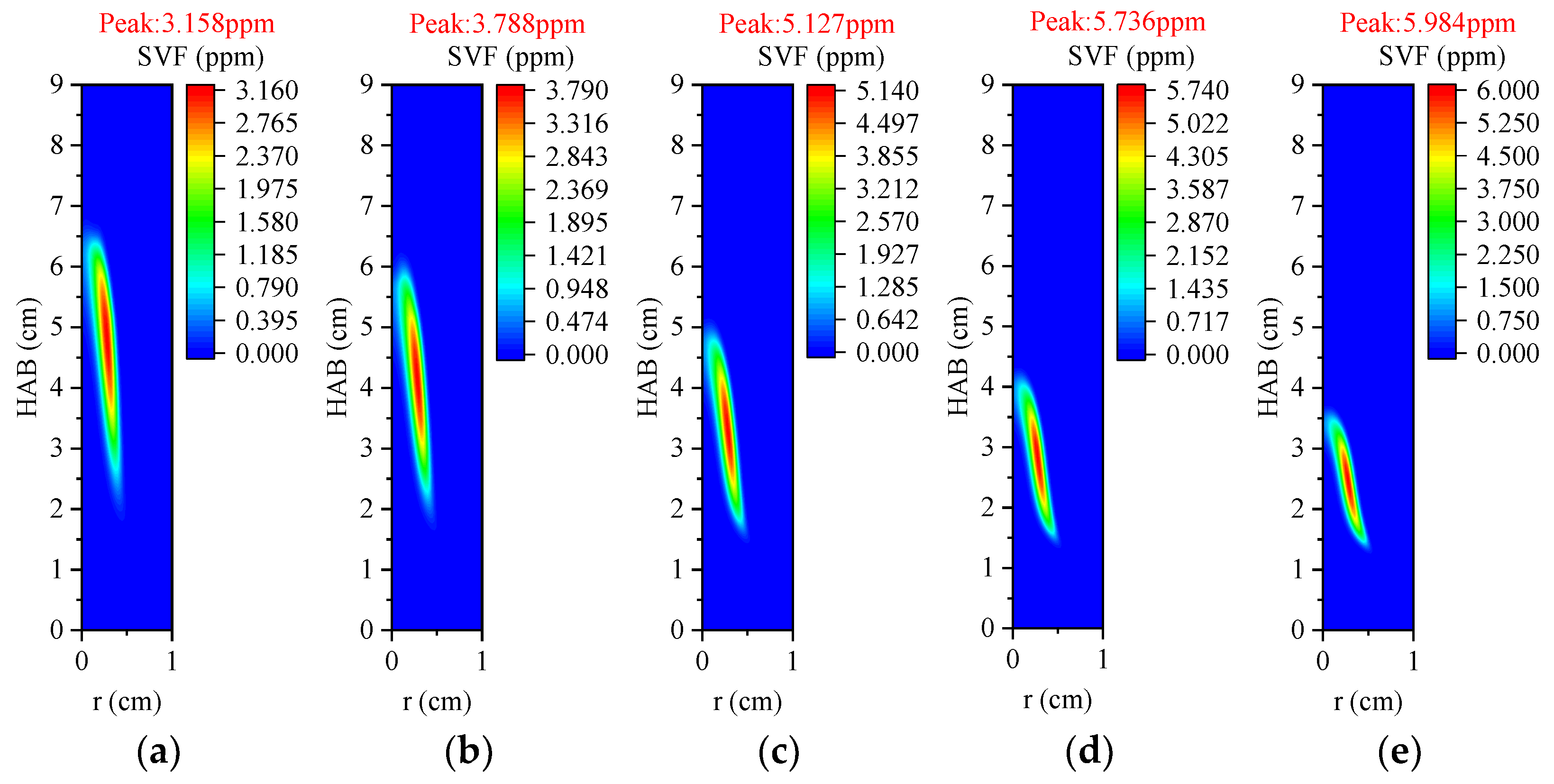
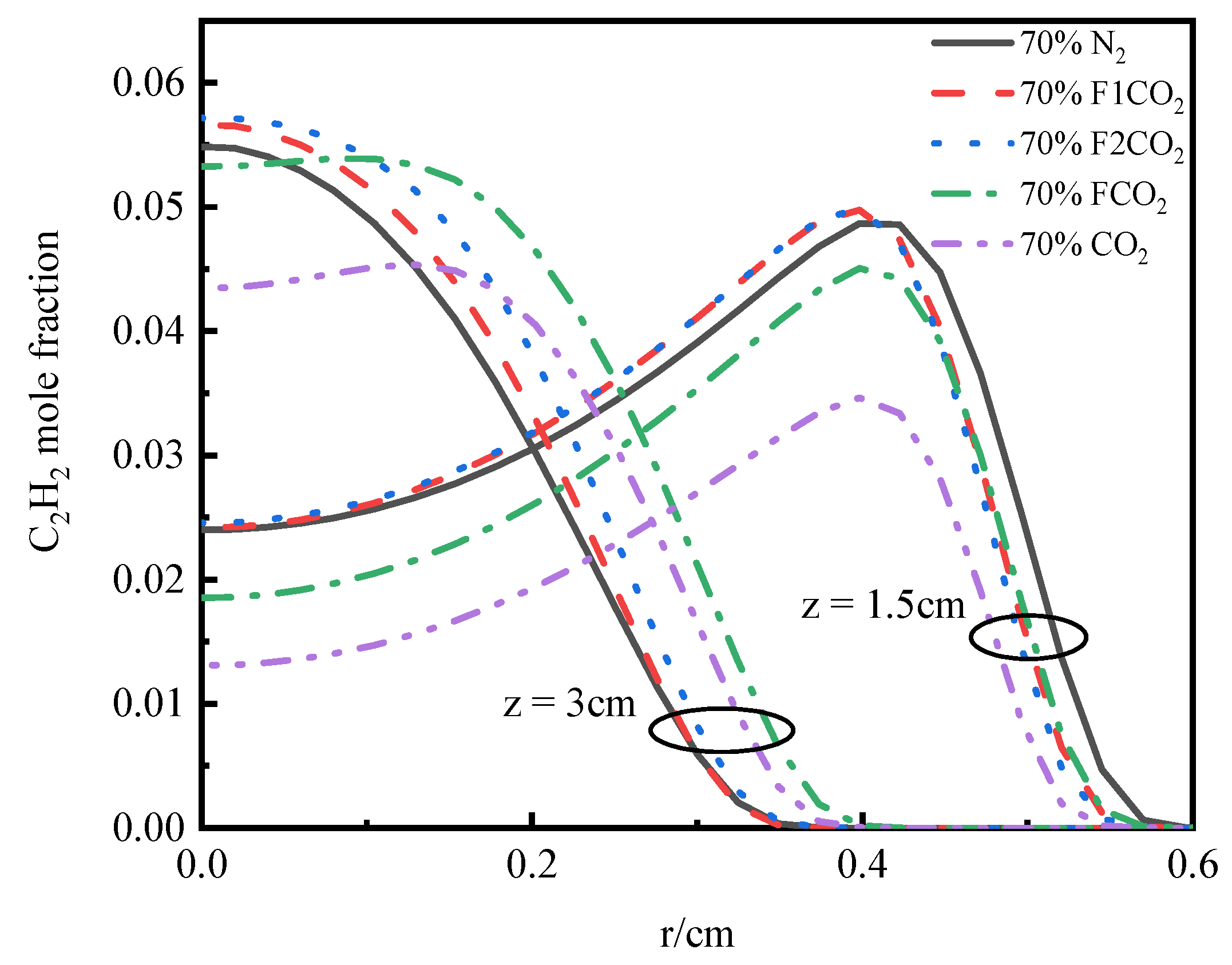
3.3.3. Volume Fraction of Soot

Figure 20 presents the distribution of soot volume fraction in two dimensions, with soot mainly concentrated along the flame’s wings. By defining the visible flame height as the centerline position where soot disappears [36], we can evaluate how the density, transport, thermal, and chemical effects of CO₂ affect the visible flame height.

Based on the results, CO₂ has the most significant thermal impact. The flame height measured at 4 cm in an O₂/N₂ atmosphere, increasing to 4.2 cm and 4.4 cm under the influence of density and transport effects, respectively, and reaching approximately 5 cm due to the thermal effect. However, the chemical effect results in a flame height of approximately 4.7 cm. While CO₂ moderately inhibits soot formation, this effect is less pronounced compared to its chemical influence. Soot formation is primarily influenced by CO₂ through the reaction OH + CO ⇋ H + CO₂. While some researchers argue that high concentrations of CO₂ enhance soot oxidation by increasing the reverse reaction rate of this elementary reaction and boosting OH radical concentration, others suggest that the primary mechanism is a reduction in H radical concentration [37].

Soot formation is primarily governed by the nucleation of soot particles at the early stages of combustion. This process plays a critical role in determining the density of primary soot particles, even though its contribution to the total soot mass is relatively smaller compared to surface growth [13]. Consequently, nucleation is crucial to the whole process of soot formation, since it dictates the initial surface area available for subsequent surface growth. Figure 21 illustrates the impact of various CO₂ effects—thermal, chemical, density, and transport—on the nucleation rate, surface growth rate, and oxidation rate of soot. It can be seen that while the transport effect specifically reduces the oxidation rate, the other effects exhibit similar influences across the nucleation, surface growth, and oxidation stages. The consistency between these processes emphasizes the complex interplay between them and highlights the nuanced role that CO₂ plays in modulating the overall dynamics of soot formation.

The study examined the impact of CO₂ on soot mass nucleation, surface growth, and oxidation rates at various burner heights by analyzing the radial distributions of temperature, acetylene (C₂H₂) concentration, and the mole fractions of H and OH radicals, (see Figure 22, Figure 23, Figure 24 and Figure 25). According to the soot formation model, nucleation is primarily initiated by the condensation of C₂H₂, making the initiation rate highly sensitive to temperature and C₂H₂ concentration. Figure 22 presents the radial temperature distributions at 3 cm and 5 cm above the burner under varying CO₂ effects. The addition of CO₂ alters the flame temperature through a combination of density, chemical, and thermal effects. At 3 cm, in the lower flame region, the high specific heat capacity of CO₂ induces a pronounced thermal buffering effect, leading to a significant reduction in flame temperature. This reduction is further intensified by radiative heat losses and chemical interactions between CO₂ and intermediate hydrocarbon species, including C₂H₂, which suppress exothermic reactions that typically sustain higher temperatures. Additionally, the proximity to the burner introduces localized flow disturbances, contributing to a more uneven temperature distribution at this height. At 5 cm, the flame enters a more stable development phase, where convective heat transfer plays a dominant role, facilitating a more uniform redistribution of thermal energy across the radial profile. This stabilizing effect results in a smoother temperature distribution and reduces the influence of CO₂ observed at lower heights. When combined with the findings in Figure 21, it becomes evident that the CO₂-induced temperature reduction at lower flame heights significantly diminishes soot nucleation rates, as these rates are highly dependent on temperature and C₂H₂ availability. The surface growth of soot particles, primarily influenced by the condensation of PAHs and the incorporation of C₂H₂, is likewise impacted by these thermal conditions. The temperature, C₂H₂ concentration, and H radical availability are key factors influencing the contribution of C₂H₂ to surface growth. The thermal effect of CO₂ reduces the radial temperature in the lower flame region, thereby further inhibiting soot surface growth.

The effects of CO₂ on the radial distribution of acetylene (C₂H₂) concentration vary with flame height as shown in Figure 23. The chemical effect of CO₂ is particularly significant, leading to a marked reduction in C₂H₂ concentration within the lower regions of the flame. Even though the C₂H₂ concentration in a C₃H₈/(O₂/CO₂) atmosphere is higher than in a C₃H₈/(O₂/N₂) atmosphere, the chemical effect of CO₂ continues to inhibit the C₂H₂ concentration at z = 3 cm. The chemical properties of CO₂ exert a persistent suppressive impact on acetylene concentration, even when overall C₂H₂ levels are high.

Hydrogen (H) is crucial in both the initial nucleation and subsequent growth of soot particles. Its role is key in determining the rate of formation of active centers within the HACA mechanism, which influences the addition of C₂H₂ to soot [10]. The radial distribution of radical concentration in Figure 24 shows that in a C₃H₈/(O₂/CO₂) flame, there is a low concentration of radical H. This is primarily attributed to the thermal and chemical effects of CO₂, which reduce the rate of C₂H₂ addition, thereby influencing the overall process of soot formation.

The critical chain reaction influencing soot formation and related intermediate components is OH + CO ⇋ H + CO₂. CO₂ shifts the equilibrium of the reaction towards the consumption of H and the production of more OH. According to Figure 25, the thermal effect of CO₂ exerts the greatest influence on OH concentration, whereas its chemical effect also contributes to a lesser degree.

Both the thermal and chemical impacts of CO₂ lead to a reduction in OH formation, which correlates with the observed decrease in oxidation rate as shown in Figure 21. This suggests that instead of enhancing soot oxidation, CO₂ in the co-flow gas primarily influences the formation and growth of soot particles by decreasing the flame temperature and the H molar fraction. This finding aligns with the conclusions drawn by Guo et al. [38] in their study on C₂H₄/(O₂/CO₂) flames.

Figure 26 presents a sensitivity analysis of the various effects of adding the virtual component CO₂ to key elementary reactions. The reaction OH + CO ⇋ H + CO₂ exhibits the highest sensitivity to the chemical effects of CO₂. Increasing the mole fraction of reactive CO₂ accelerates the reverse reaction rate, leading to reduced CO oxidation heat release and a subsequent decrease in H concentration. When the mole fraction of non-reactive CO₂ increases, the reverse reaction rate of the elementary reaction also increases slightly compared to the reference condition. It should be noted that this increase is significantly less pronounced than the effect caused by the chemical action of CO₂. The reaction H + O₂ ⇋ O+ OH is pivotal in the oxidation of propane, yet its reaction rate in an O₂/CO₂ atmosphere is lower compared to O₂/N₂ and O₂/FCO₂ atmospheres. This disparity may be attributed to the simultaneous competition for H radicals between the reactions H + CH₃ (+M) ⇋ CH₄ (+M) and H + O₂ ⇋ O + OH, which collectively diminish the overall H concentration and, consequently, reduce the reaction rate [39].

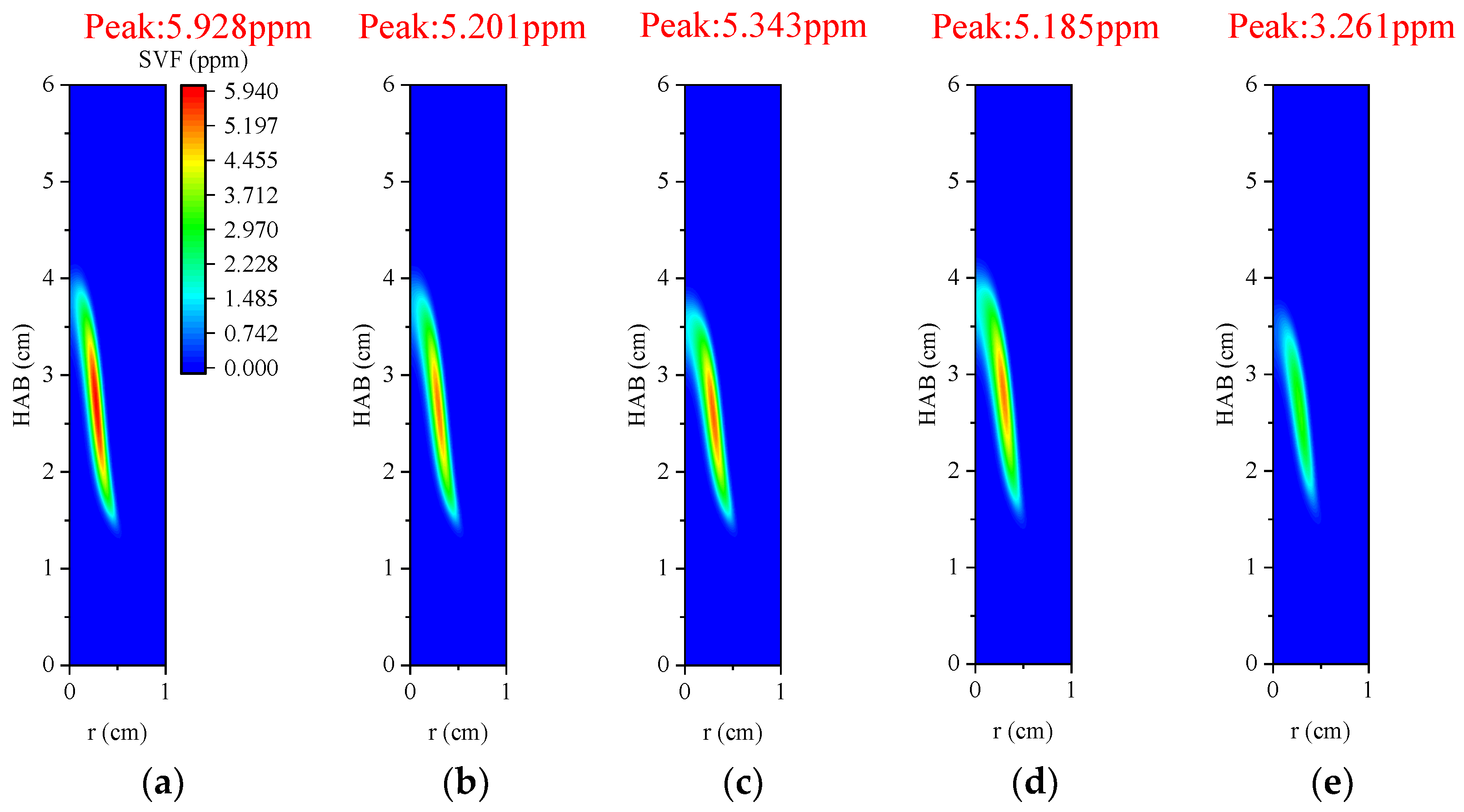
3.4. Impacts of H₂O Addition: Combustion Sensitivity Analysis

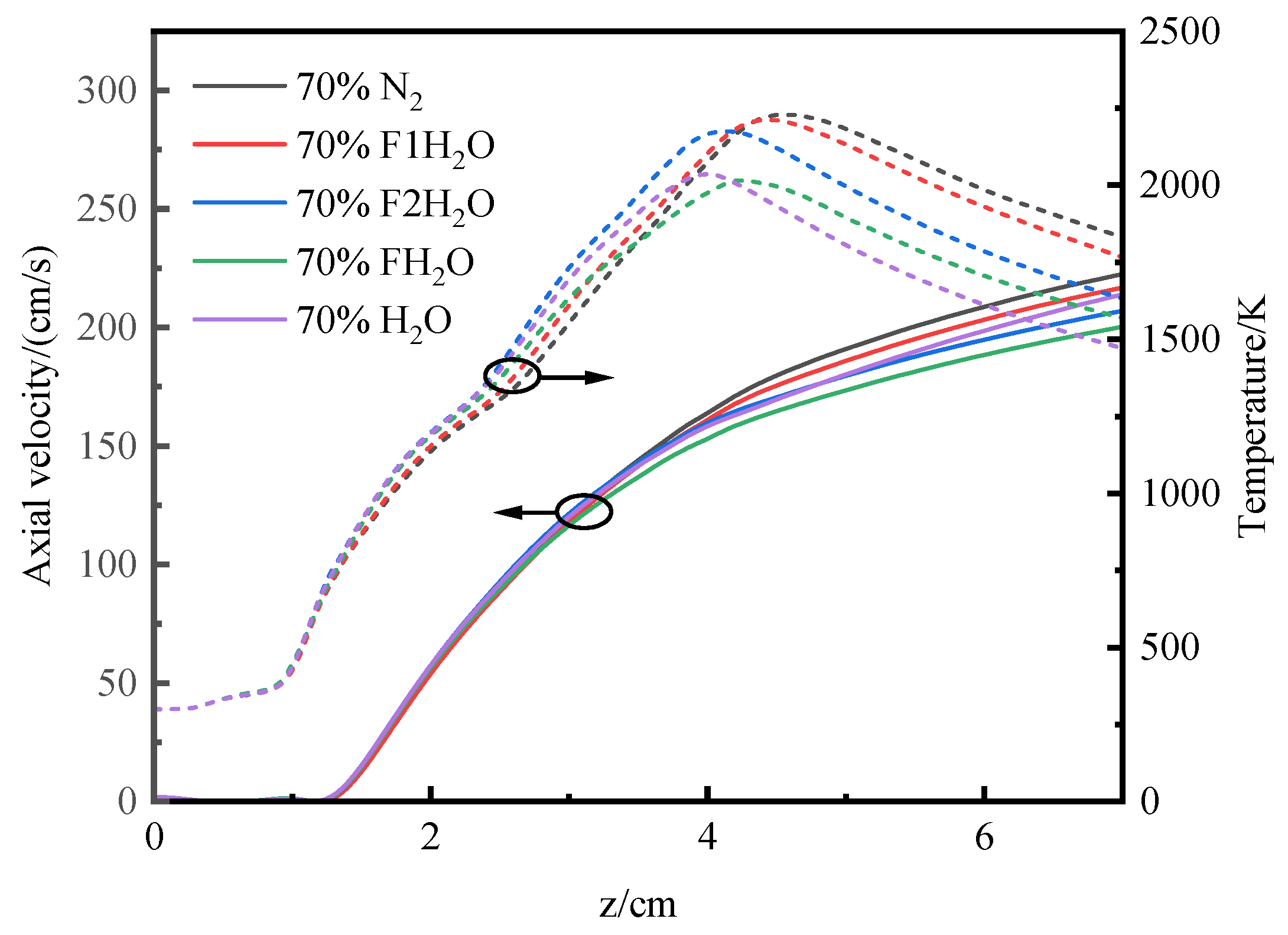
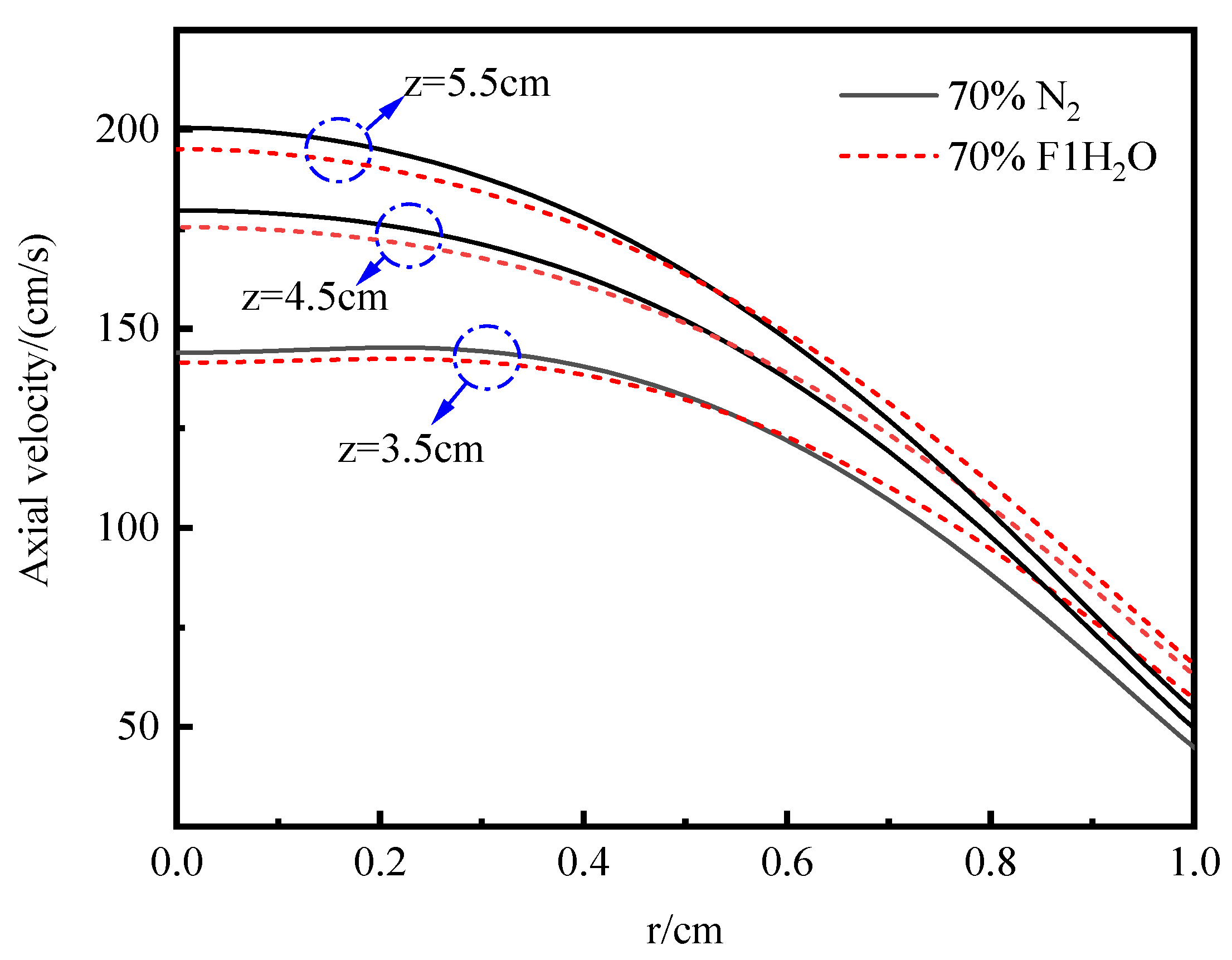
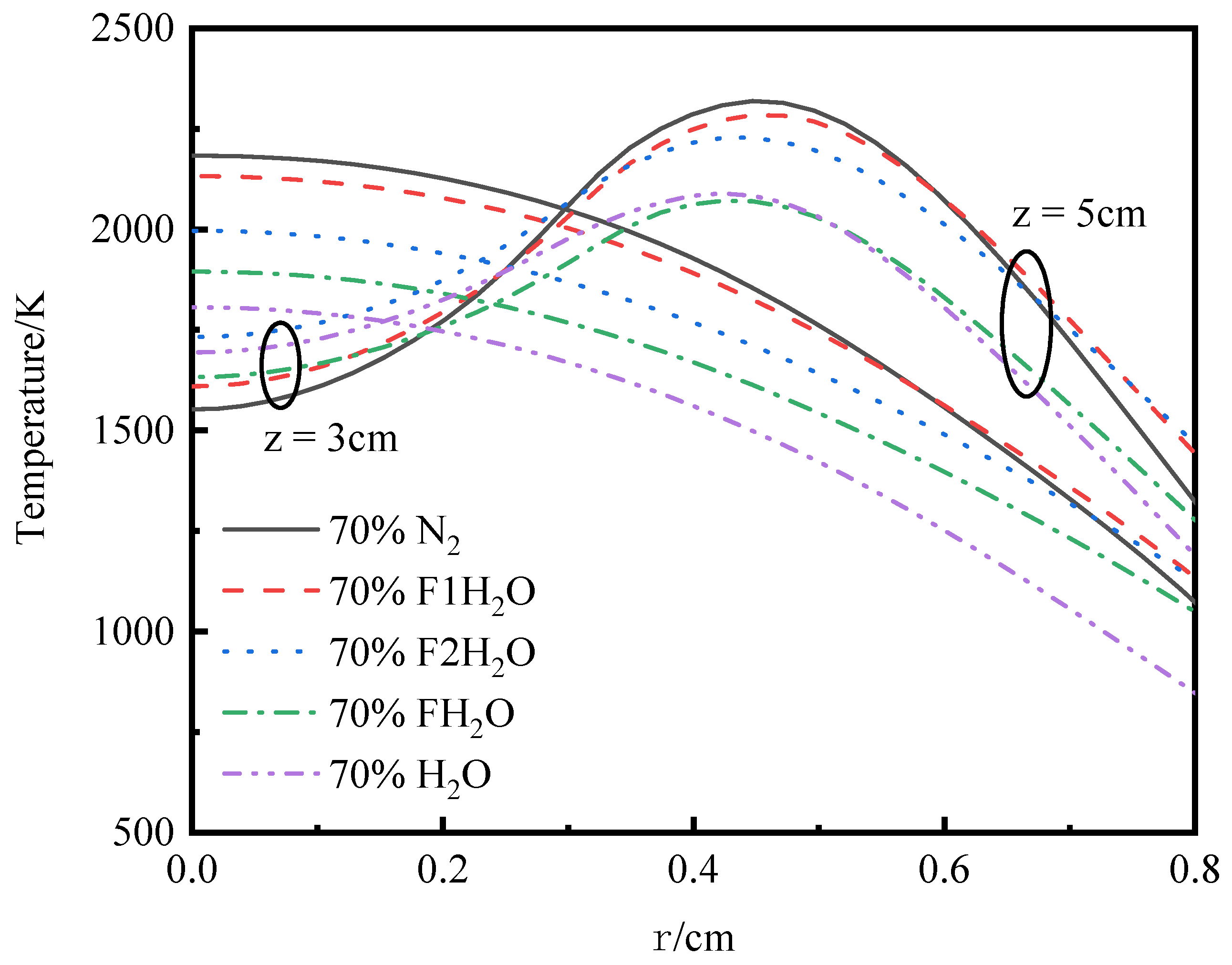
3.4.1. Temperature and Axial Flow Velocity

Figure 27 provides a two-dimensional depiction of the temperature distribution following the introduction of a virtual H₂O component into the system. Notably, the density and transport effects exhibit minimal influence on the peak temperature. This outcome is in stark contrast with the behavior observed when CO₂ is involved. Among the factors, thermal effect has the most significant impact on flame temperature. Additionally, chemical influence further diminishes the peak temperature. Observing the peak temperature in Figure 27 alongside the axial temperature at the flame center in Figure 28 reveals that the observed temperature differences are primarily due to the thermal effect of H₂O, resulting from its high heat capacity. Chemical and transport effects also affect the overall temperature distribution, though to a lesser degree, whereas the density effect has a minimal impact. At constant outlet velocity, Figure 28 further shows that flame height axial velocity under the baseline conditions is greater, relative to other conditions, particularly around the 3.5 cm mark. In this case, the temperature discrepancy is primarily due to the thermal and chemical effects of H₂O.

To investigate the impact of water density on velocity and temperature fields, the axial velocity’s radial distribution at different elevations above the burner is analyzed, as shown in Figure 29. According to the findings, the axial velocity in Case 3-7 (70% F1H₂O) near the flame axis is slightly lower than Case 3-6. However, the axial velocity in Case 3-7 is greater than that of the reference case in regions far from the flame axis (r > 0.6 cm). This behavior is attributed to the lower $\dot{m}$ of F1H₂O (18.015 g/mol) compared to N₂ (28.013 g/mol), which results in a reduced oxidant density under Case 3-7. There is a rise in axial velocity within these areas due to the increased buoyancy of the mixture distant from the reaction flame. This change shortens the mixing time between oxidant and fuel airflows, subsequently affecting the intensity of flame combustion. Under the density effect, a slight reduction in peak temperature may be due to such an impact.

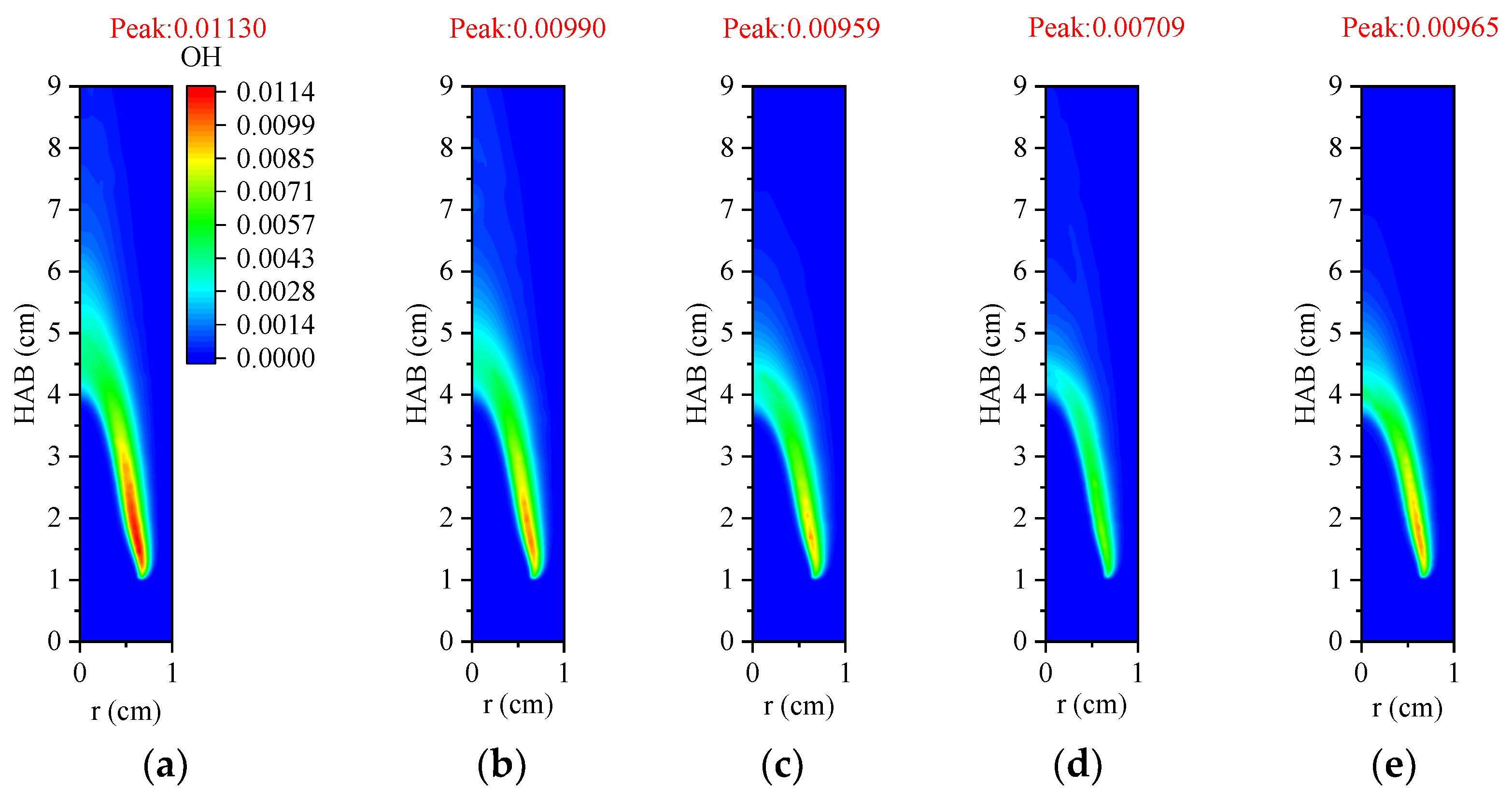
3.4.2. OH Radical Distribution and Flame Height

Under varying conditions, Figure 30 illustrates the two-dimensional distribution of OH radical mole fractions. The OH radicals are predominantly concentrated on either side of the flame, with their highest concentration observed at a point below 2 cm above the burner outlet. The impact of H₂O on OH distribution mirrors its effect on temperature, signifying that OH concentration is indicative of combustion intensity. Notably, in an O₂/H₂O atmosphere, the peak OH mole fraction is markedly lower than in an O₂/N₂ environment, primarily due to thermal effects which diminishes OH peak by 26.1%. In addition, OH peak is further reduced by 12.4% due to the density effect of H₂O, while the transport effect is negligible. Conversely, the chemical effect of H₂O substantially elevates the OH peak by 36.1%, highlighting its role in enhancing OH concentration. Overall, the chemical influence of H₂O tends to increase OH concentration, whereas thermal and density effects are significant contributors to its reduction.

Figure 31 depicts the influence of H₂O on flame height, analyzed under the same criteria used for the addition of CO₂. The chemical, thermal, and transport effects of H₂O on flame height are consistent with findings made by Xu H et al. [12] in their study of the impact of H₂O-diluted oxidants on the structure and shape of laminar co-flow syngas diffusion flames. As seen in Figure 28, the axial velocity in the presence of H₂O (F1H₂O) is generally lower than the baseline condition, resulting in a shorter reaction path within the same residence time, which leads to a reduction in flame height due to H₂O’s density effect. Furthermore, the transport effect of H₂O contributes to a further decrease in flame height, which is attributed to the binary diffusion coefficient of the H₂O–O₂ mixture being much higher than that of the N₂–O₂ mixture. [16] Consequently, the transport characteristics of H₂O in the oxidant enhance the oxygen diffusion process, replacing the role of N₂ and reducing the required surface area for complete combustion, ultimately leading to a shortened flame height.

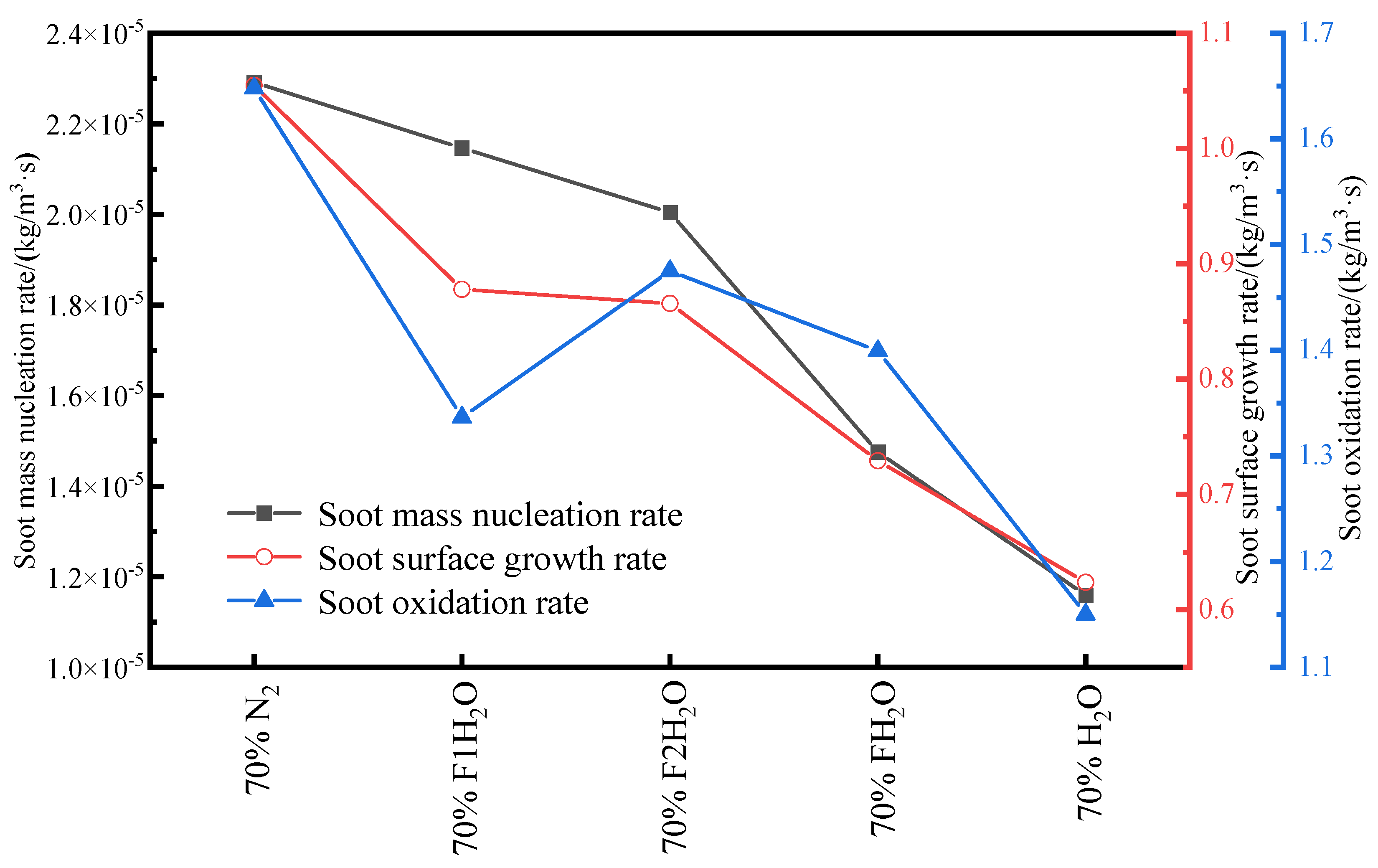
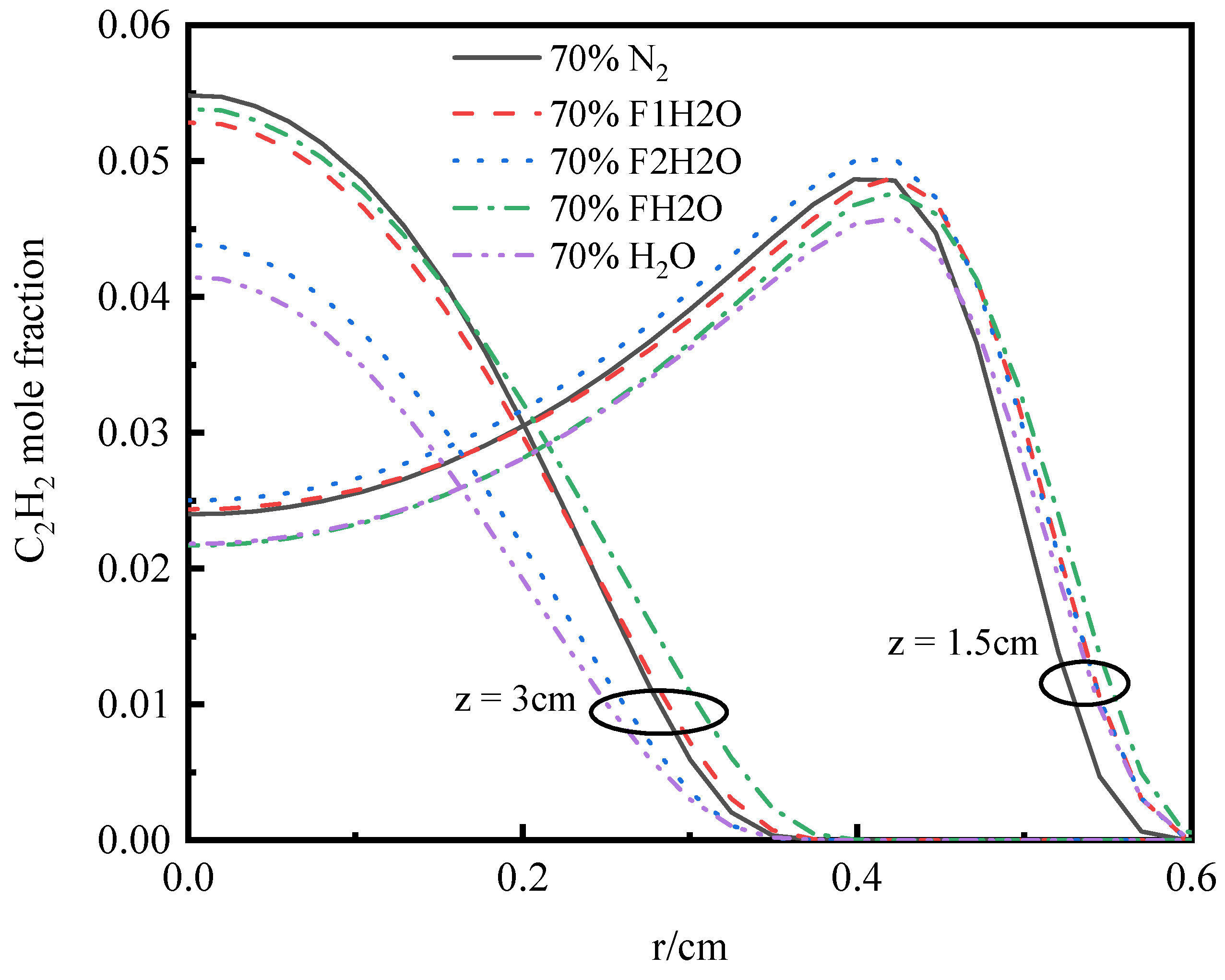
3.4.3. Volume Fraction of Soot

A two-dimensional layout of soot volume fraction in Figure 32 illustrates that soot primarily accumulates along the flame’s wings. The thermal effect of H₂O is the primary factor influencing flame height, as demonstrated by a comparison of visible flame height variations caused by different effects of H₂O. Specifically, the visible flame height which measures 3.9 cm in an O₂/N₂ atmosphere decreases slightly to 3.8 cm, and 3.7 cm under density and transport effects. However, under thermal and chemical effect, it increases to approximately 4.1 cm, and 3.6 cm. While the thermal effect exerts a mild inhibitory influence on soot formation, its impact is considerably less significant compared to the chemical effect.

The effect of H₂O on nucleation, surface growth, and oxidation rates of soot particles can be seen in Figure 33. It appears that the soot mass nucleation rate is highly sensitive to thermal and chemical effects. Surface growth is notably inhibited by multiple factors, including the density, transport, thermal, and chemical effects, with the density, thermal, and chemical effects exerting the most pronounced decelerating influence. Generally, the oxidation rate depends primarily on density and chemical effects. Despite the chemical effect lowering the oxidation rate, it still surpasses the rates of soot mass nucleation and surface growth.

To investigate the influence of H₂O on the mass nucleation, surface growth, and oxidation rates of soot, Figure 34, Figure 35, Figure 36 and Figure 37 show a comparative analysis of temperature, acetylene concentration, and mole fractions of OH and H radicals at various heights above the burner. The soot model, which assumes particle initiation through acetylene condensation, establishes that the initiation rate is contingent on both temperature and acetylene concentration. As shown in Figure 34, the reduced soot nucleation rate is primarily attributed to the thermal effects of water vapor, rather than its chemical properties, in the low flame region. This is due to the negligible temperature differences between H₂O and FH₂O flames. Soot surface growth is primarily influenced by the addition of C₂H₂ and polycyclic aromatic hydrocarbon condensation, with C₂H₂ being the predominant mechanism. The rate of C₂H₂ is also significantly influenced by temperature, C₂H₂ concentration, and H radical concentration. The heat impact of water vapor causes the radial temperature to lower in areas where soot accumulates, especially in the lower flame zone, as shown in Figure 33.

Figure 35 reveals that H₂O exerts varying effects on the radial distribution of C₂H₂ concentration at different flame heights. In the low flame region, the chemical effect of H₂O significantly lowers the C₂H₂ concentration. As the radial distance increases, C₂H₂ concentration in the C₃H₈/(O₂/H₂O) atmosphere surpasses that in the C₃H₈/(O₂/N₂) atmosphere. However, despite this increase, the chemical effect of H₂O continues to exert an inhibitory influence on the overall C₂H₂ concentration.

It is crucial to understand H radicals in both soot nucleation and growth since they affect the formation of active centers in the HACA mechanism. As shown in Figure 36, the concentration of H radicals which is lower in the C₃H₈/(O₂/H₂O) atmosphere is primarily due to the combined thermal and chemical effects of H₂O. This reduced H concentration lowers the C₂H₂ rate, subsequently inhibiting the soot surface growth.

In the C₃H₈/(O₂/H₂O) flame, the chain reaction H₂ + OH ⇋ H₂O + H is crucial in influencing soot formation and key intermediates. The addition of H₂O drives the reaction toward increased consumption and production of H and OH. As shown in Figure 37, the H₂O chemical effect significantly elevates the OH mole fraction. As a primary oxidizing species, OH directly impacts the soot oxidation rate. As a result, the chemical effect of water vapor is dominant, with increased OH concentrations intensifying soot oxidation.

Figure 38 illustrates the sensitivity analysis of various key elementary reactions under the introduction of an additional virtual component, H₂O. It is clear that the elementary reaction OH + H₂ ⇋ H + H₂O shows the highest sensitivity to the chemical influence of water vapor. As the molar fraction of reactive H₂O increases, these reactions predominantly proceed towards consuming H₂O to produce more OH. Conversely, an increase in the molar fraction of non-reactive water vapor slightly elevates the reverse reaction rate of OH +H₂ ⇋ H + H₂O compared to the benchmark condition, although this effect remains significantly less pronounced than the impact of reactive H₂O. The elementary reaction 2OH ⇋ O + H₂O also demonstrates considerable sensitivity to the chemical presence of H₂O, potentially synergizing with OH + H₂ ⇋ H + H₂O in the reverse direction to augment OH concentrations. Meanwhile, H + O₂ ⇋ O + OH stands out as the principal elementary reaction in the oxidation process of C₃H₈, where the addition of H₂O promotes the forward reaction and enhances OH concentrations. Furthermore, the elementary reaction OH + CO ⇋ H + CO₂ serves as a primary pathway for the conversion of CO to CO₂. Overall, the pivotal roles of OH + H₂ ⇋H + H₂O and 2OH ⇋ O + H₂O in the chemical inhibition of soot formation by H₂O are widely supported in the literature. Specifically, studies [12,22] consistently affirm that the chemical impact of H₂O primarily inhibits soot formation by augmenting OH concentrations.

4. Conclusions

A laminar diffusion flame under oxygen-enriched combustion conditions was investigated experimentally and numerically for the impact of CO₂. Comparing experimentally observed temperature and SVF distributions with numerical predictions in an O₂/N₂/CO₂ atmosphere is presented in this study. Although the simulation accurately captured the flame temperature, it overestimated the SVF in the O₂/N₂/CO₂ environment. Additionally, the influence of O₂/CO₂ and O₂/H₂O oxy-fuel combustion on flame structure and soot formation was investigated through numerical simulations of laminar propane co-diffusion flames. By applying a sequential decoupling approach, the distinct effects of diluents—specifically density, transport, thermal, and chemical effects—were isolated, leading to the following conclusions:

(1)

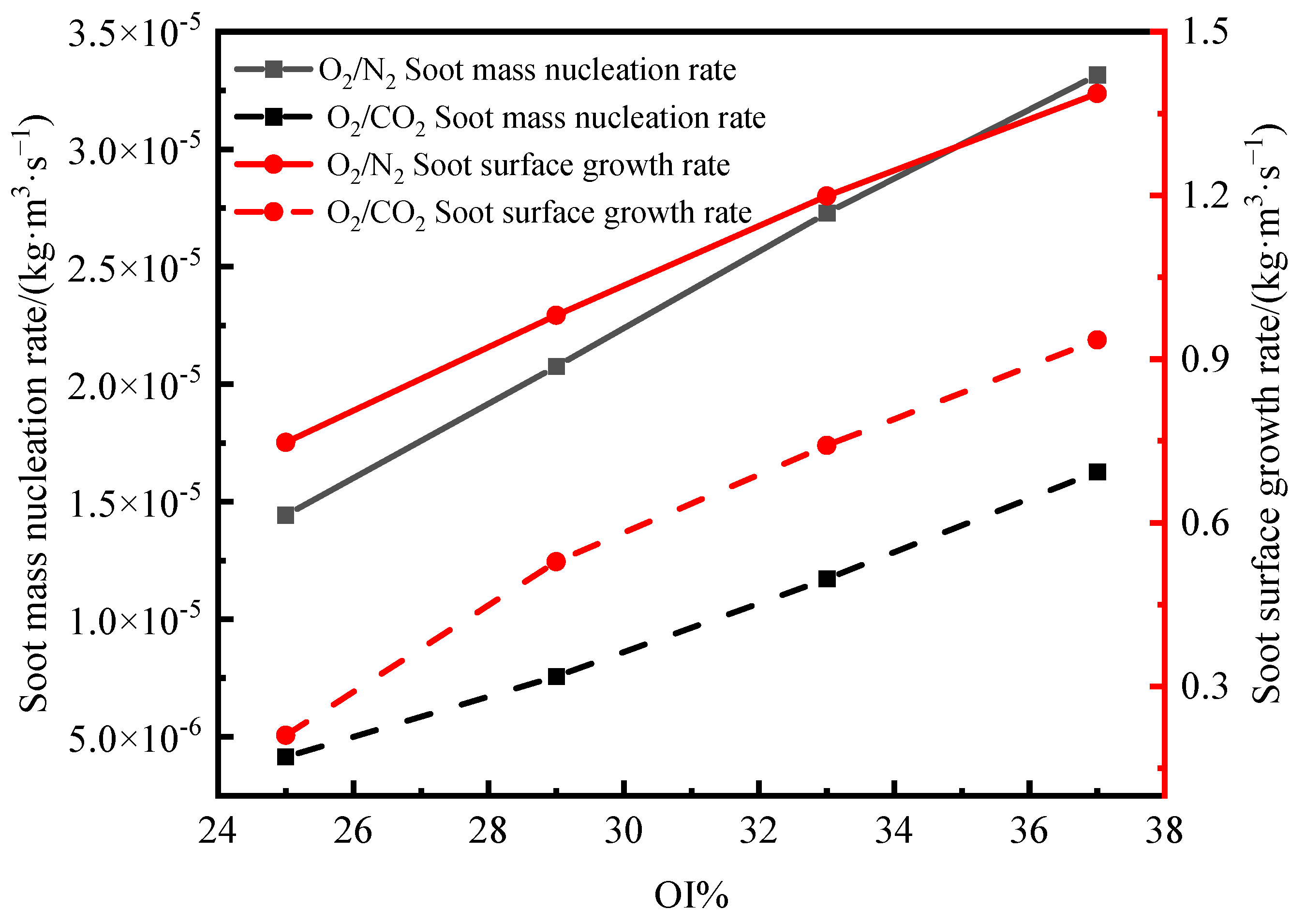
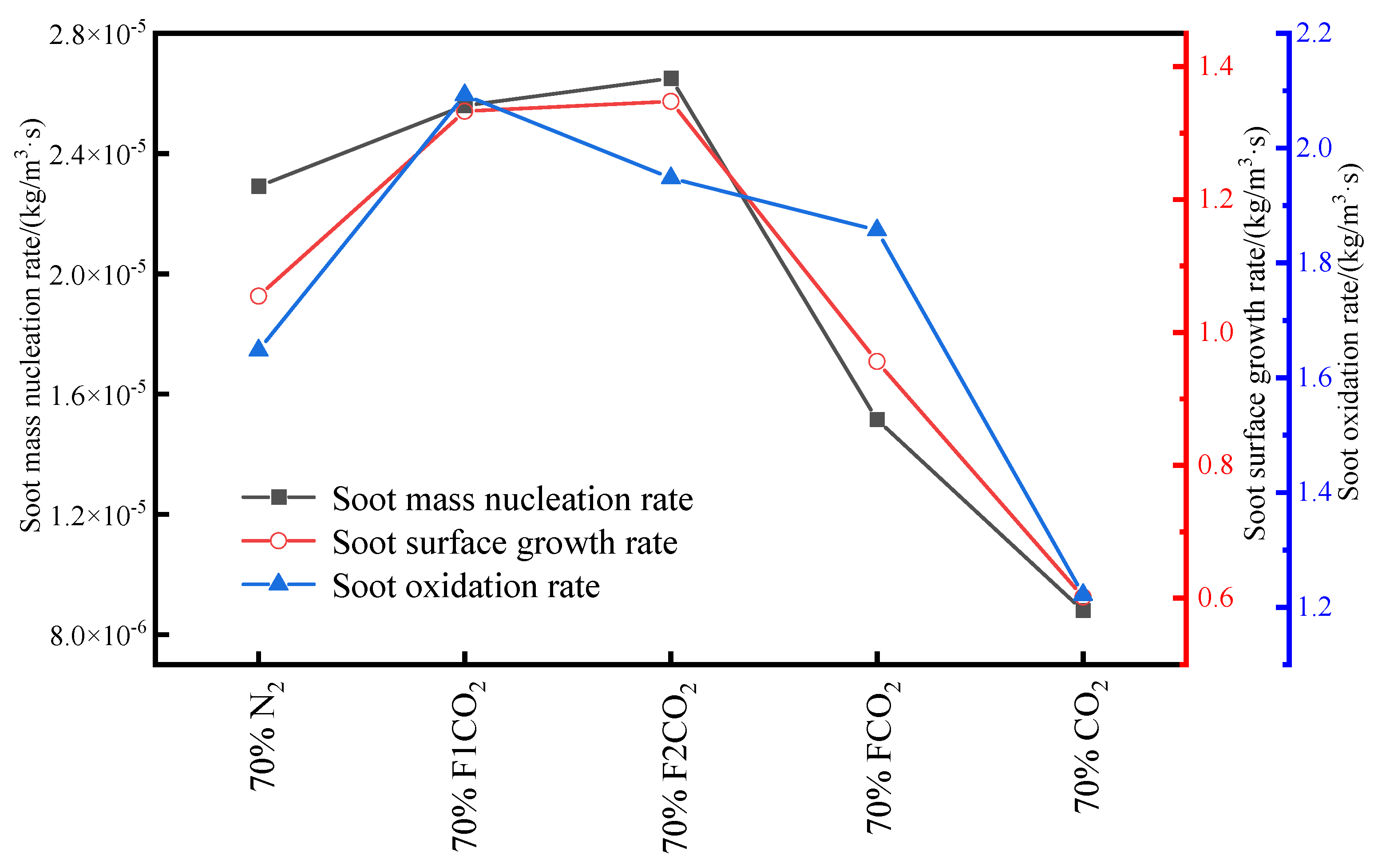
Under an O₂ index (OI) of 30%, substituting CO₂ for N₂ effectively suppresses soot formation. This replacement moves the high-temperature zone from the flame edges to the tip, thus, removing the splitting effect of the flame tip. The reduction in SVF is a result of decreased soot mass nucleation and surface growth rates, which occur when N₂ is substituted with CO₂.

(2)

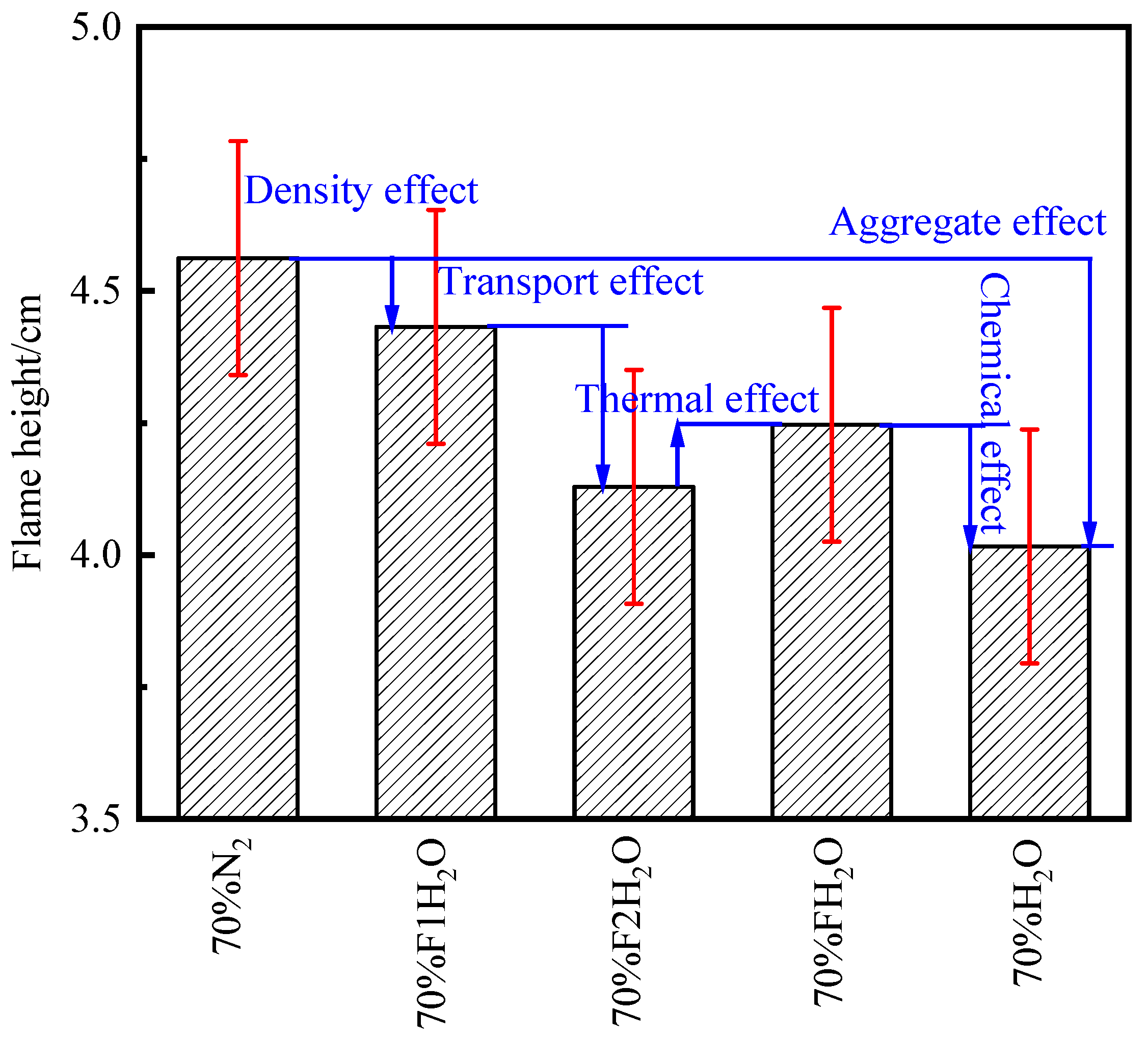
Thermal and chemical interactions with CO₂ drive the temperature differences in O₂/CO₂ combustion mode, while transport and density effects play a less important role. The chemical role of CO₂ leads to a reduction in OH radical concentration and temperature, which in turn results in an elongated flame height.

(3)

The differences in temperature distribution during O₂/H₂O combustion are primarily attributed to the thermal effects and chemical interactions of water vapor. In addition to its chemical effects, H₂O has significant thermal effects. The replacement of N₂ with H₂O leads to a reduction in flame height. The thermal effects of both CO₂ and H₂O lead to a reduction in OH concentration and a decrease in temperature. However, H₂O’s density effect diverges from that of CO₂, and its influence on transport processes is less pronounced, highlighting the distinct roles these species play in the system’s dynamics.

(4)

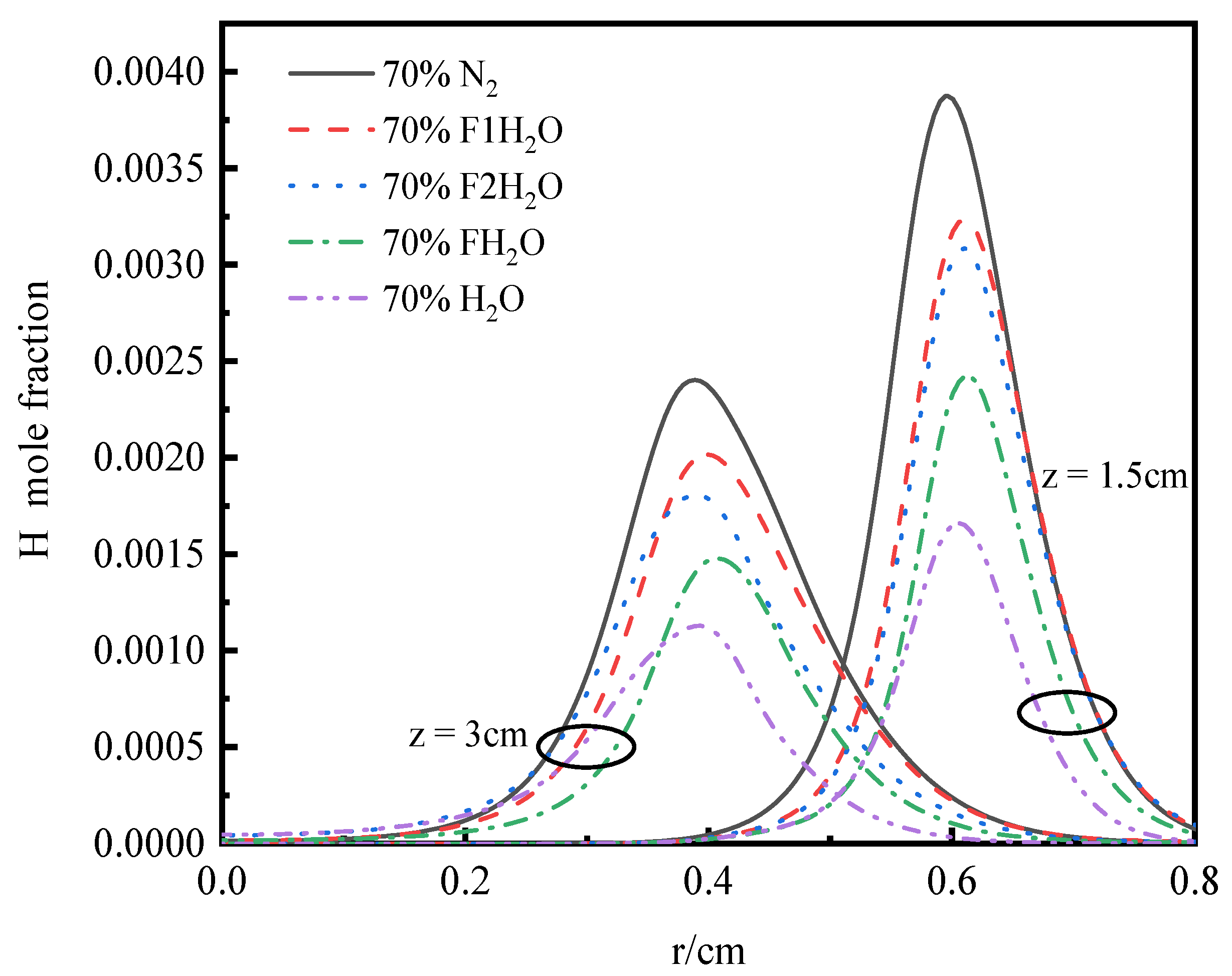
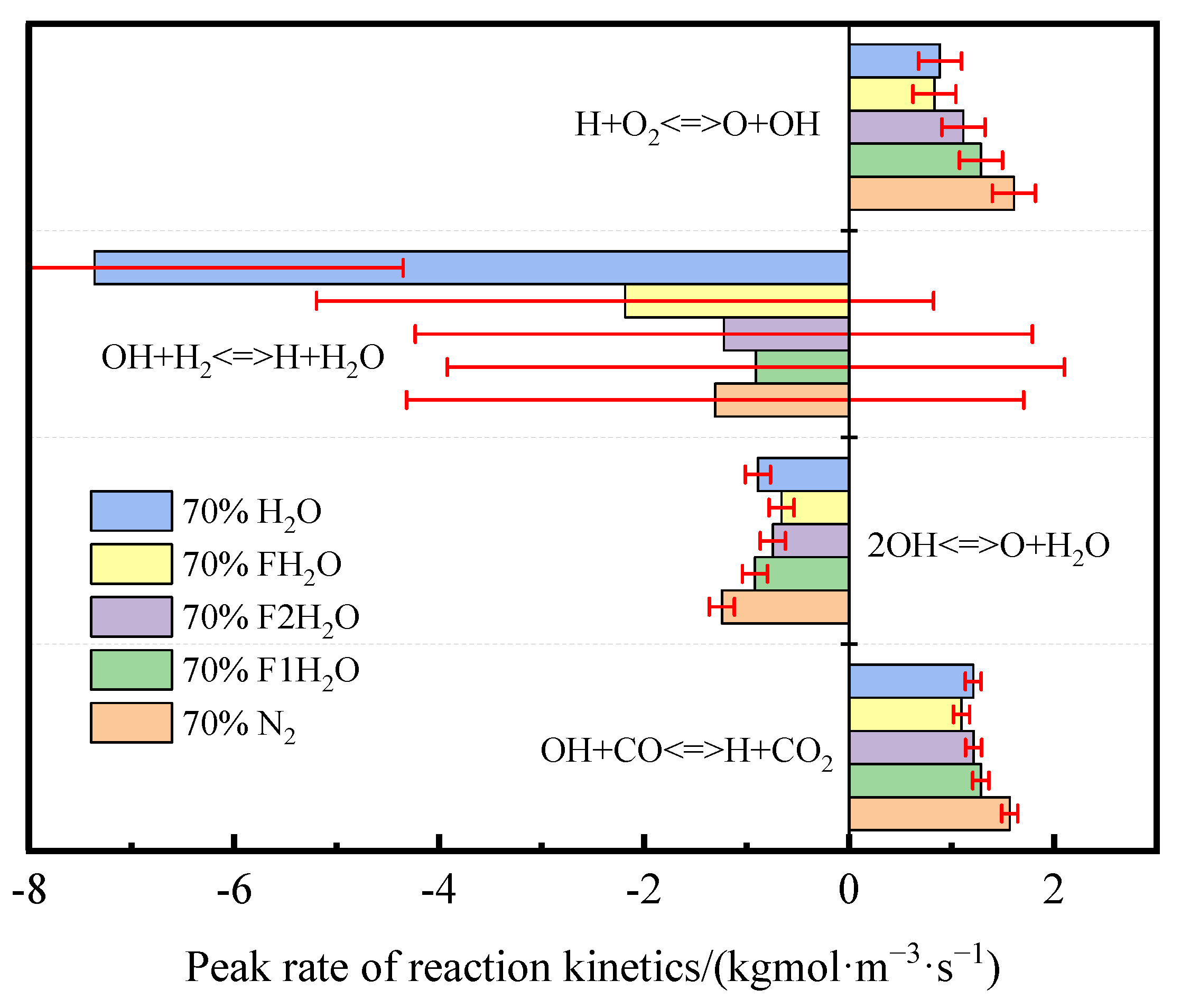
In the oxidizer, CO₂ primarily impacts soot nucleation and surface growth rates by lowering flame temperature and reducing the mole fraction of H radicals, rather than by enhancing soot oxidation. In contrast, in oxy-steam combustion, the reaction OH + H₂ ⇋ H + H₂O plays a crucial role in suppressing soot formation. Here, the chemical effects of water vapor significantly inhibit soot generation by increasing OH radical concentrations.