Fast Analytic–Numerical Algorithms for Calculating Mutual and Self-Inductances of Air Coils
Abstract
1. Introduction
2. Principles for Calculating the Mutual and Self-Inductances of Air Coils
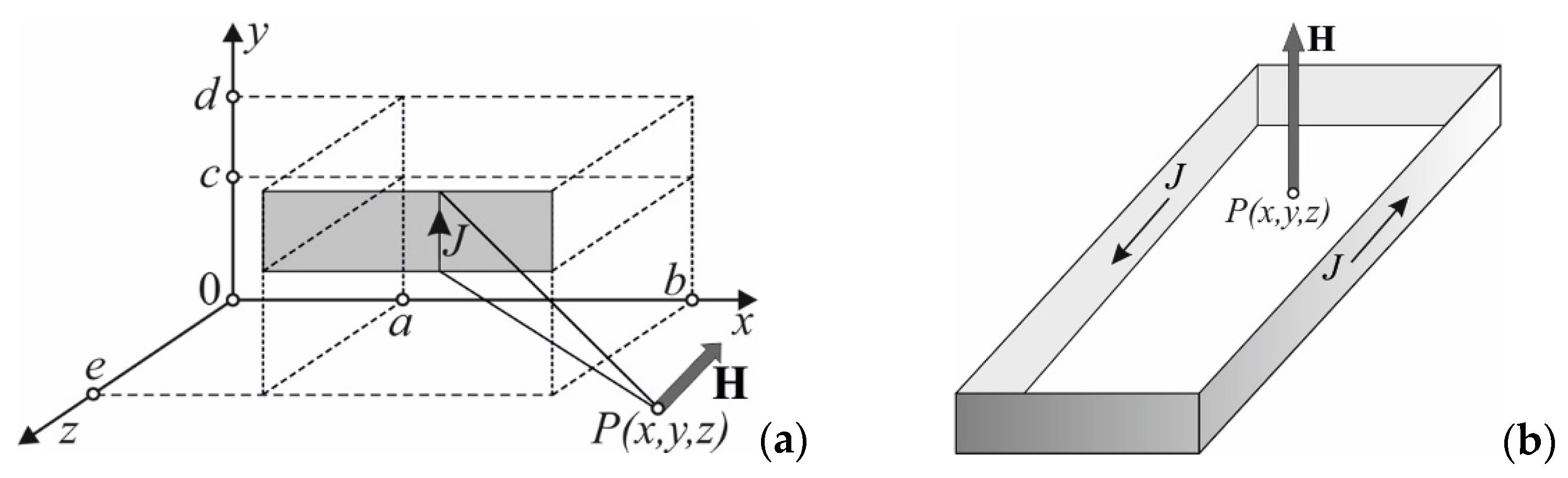
Basics of Magnetic Field Theory Used for Inductance Calculations
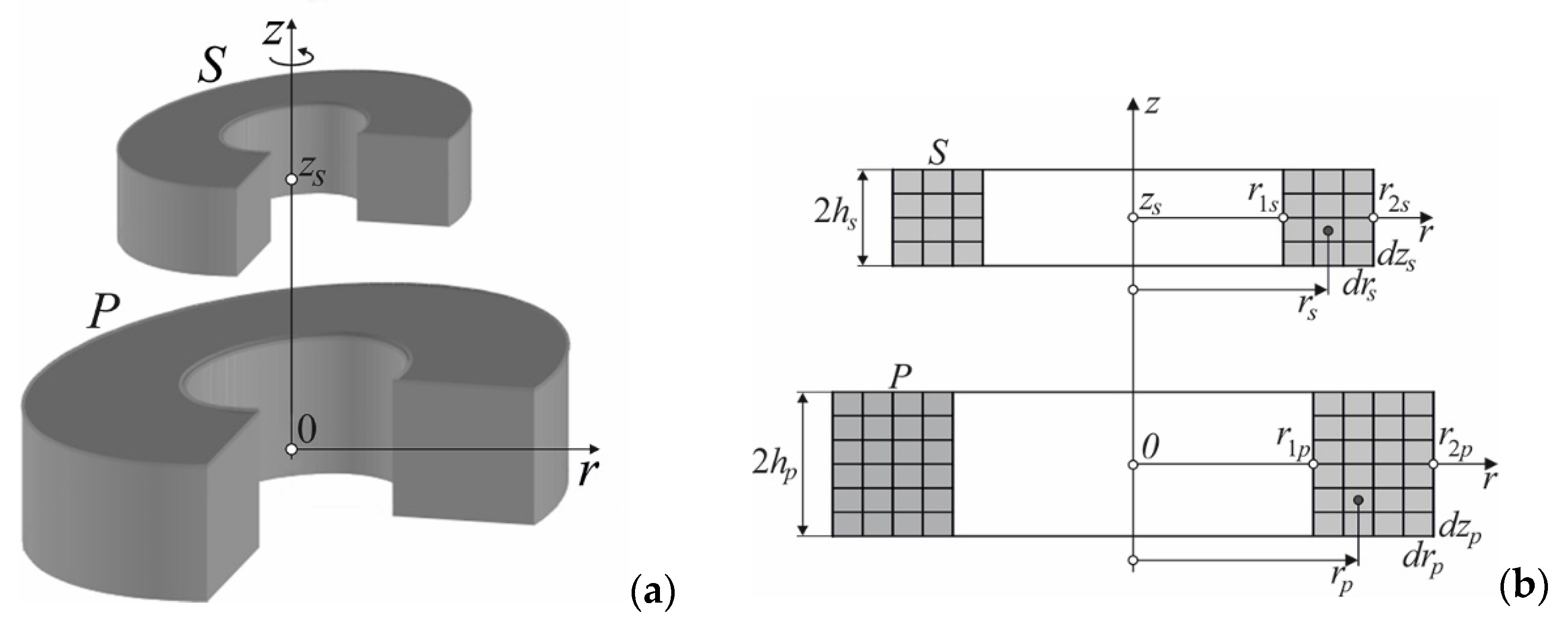
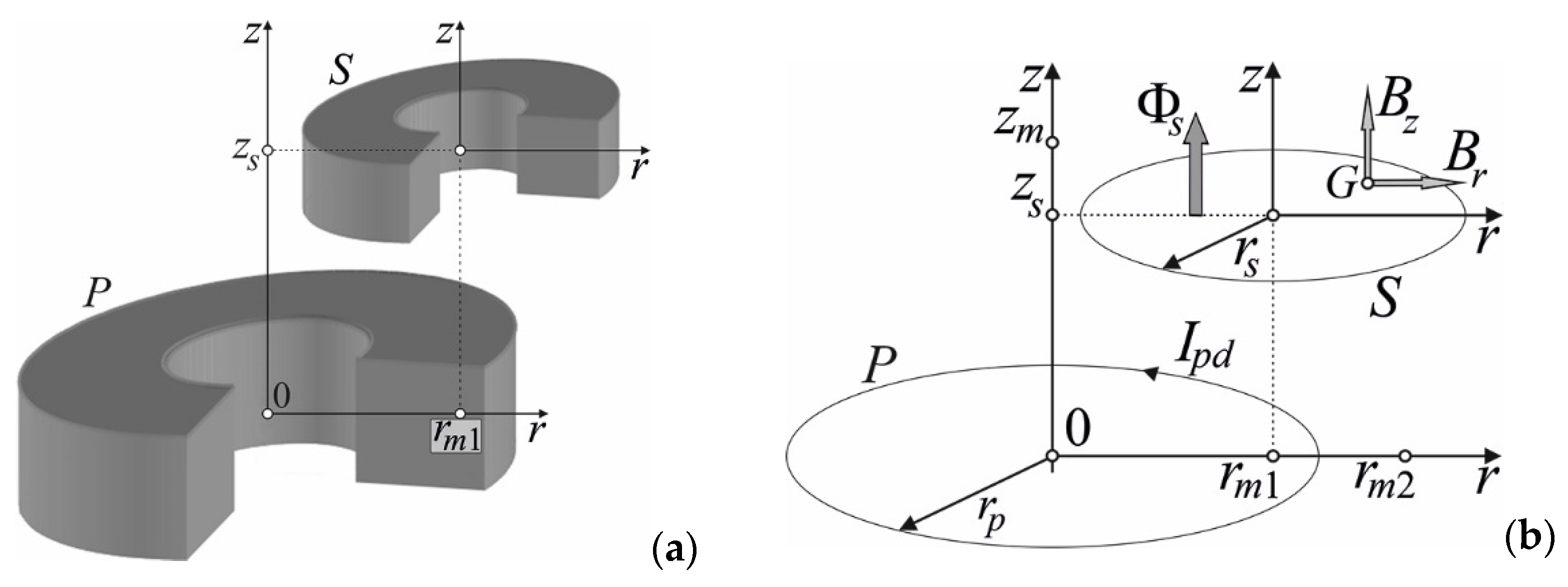
3. Cylindrical Air Coils
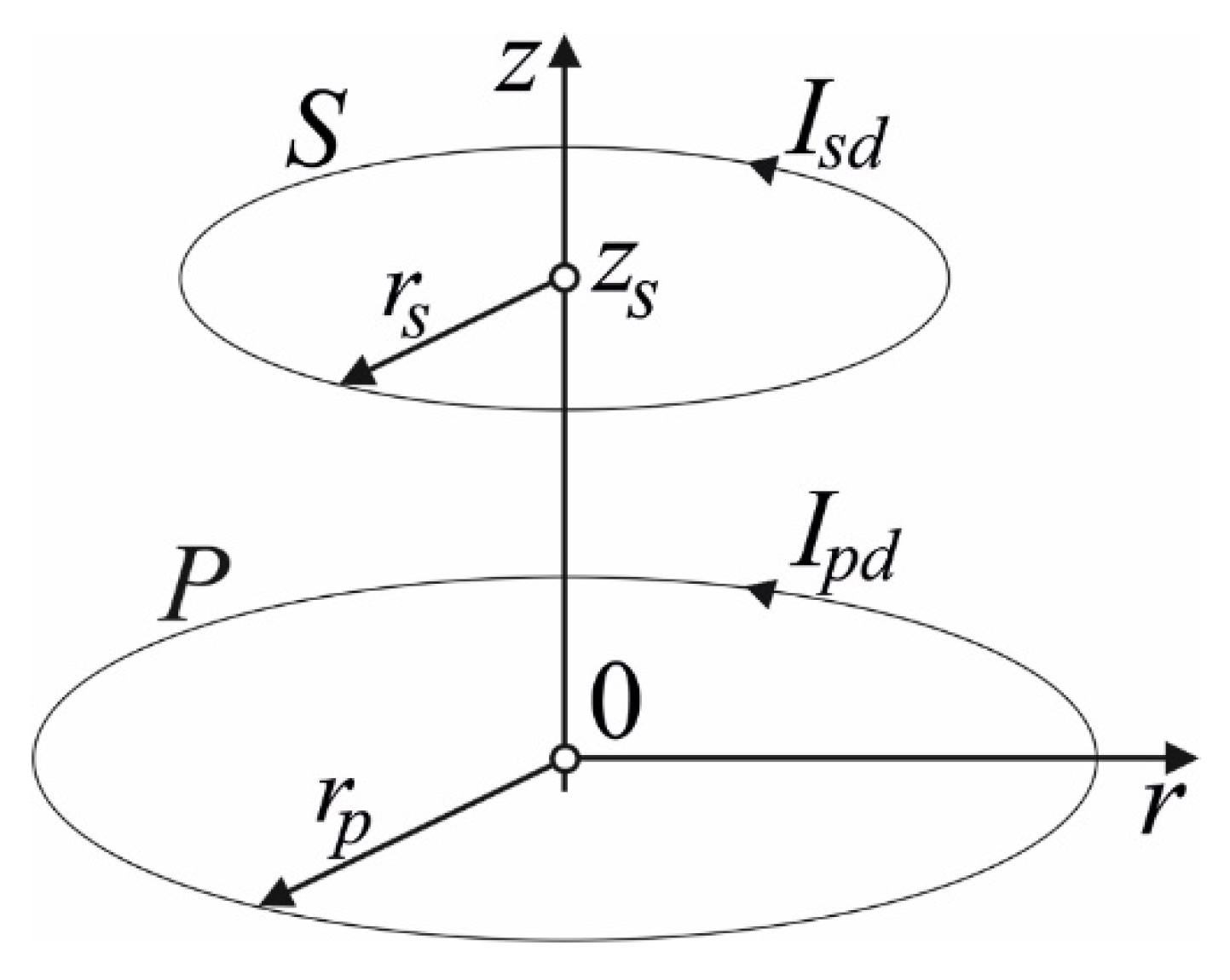
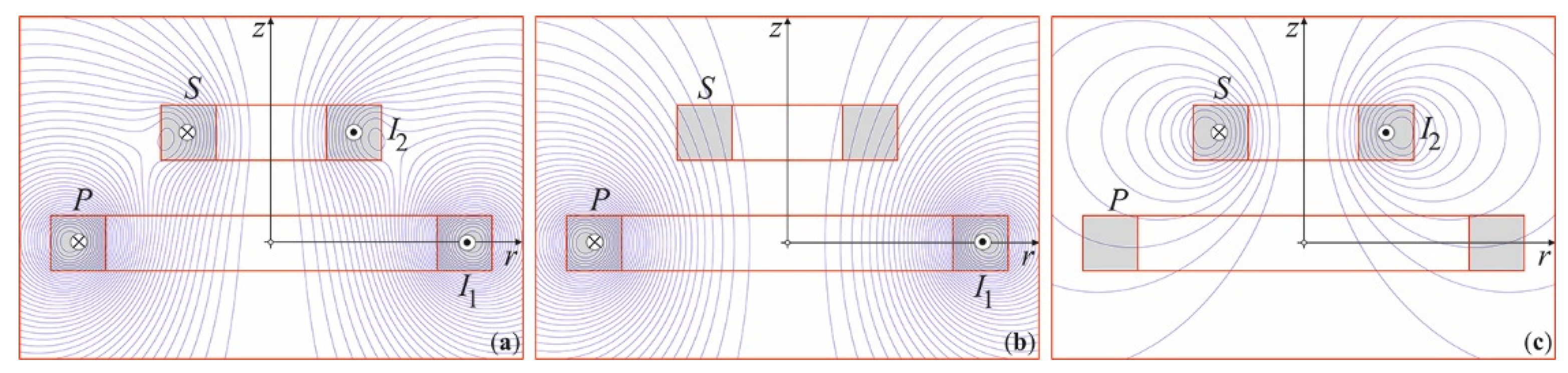
3.1. Magnetic Field of Cylindrical Coils
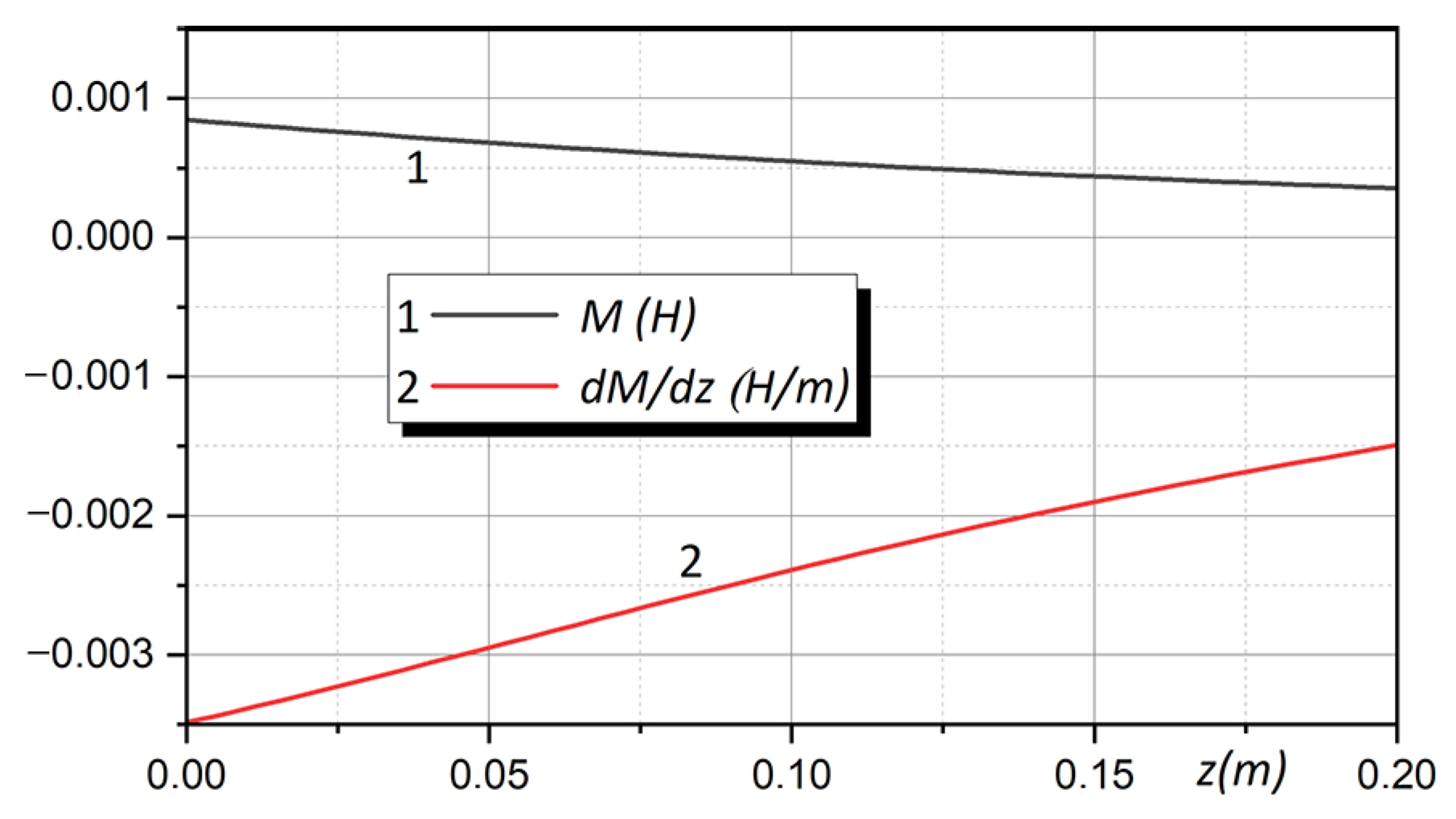
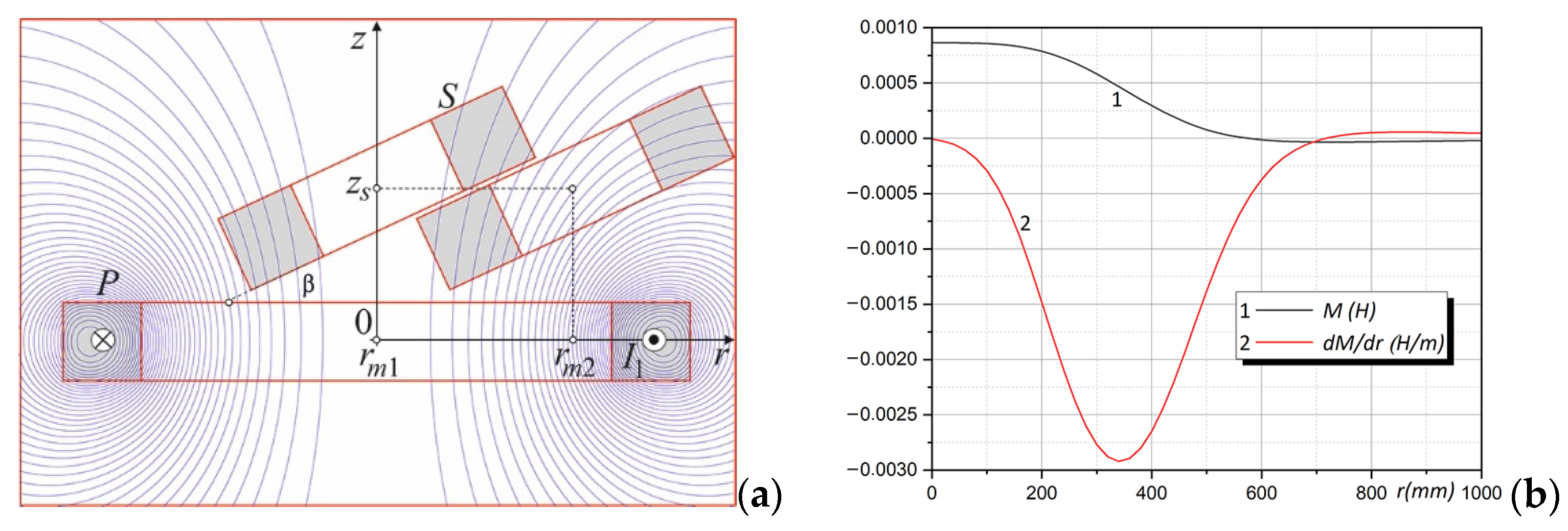
3.2. Comparison with Results Obtained by Other Authors and the Finite Element Method
3.3. Non-Coaxial Cylindrical Coils
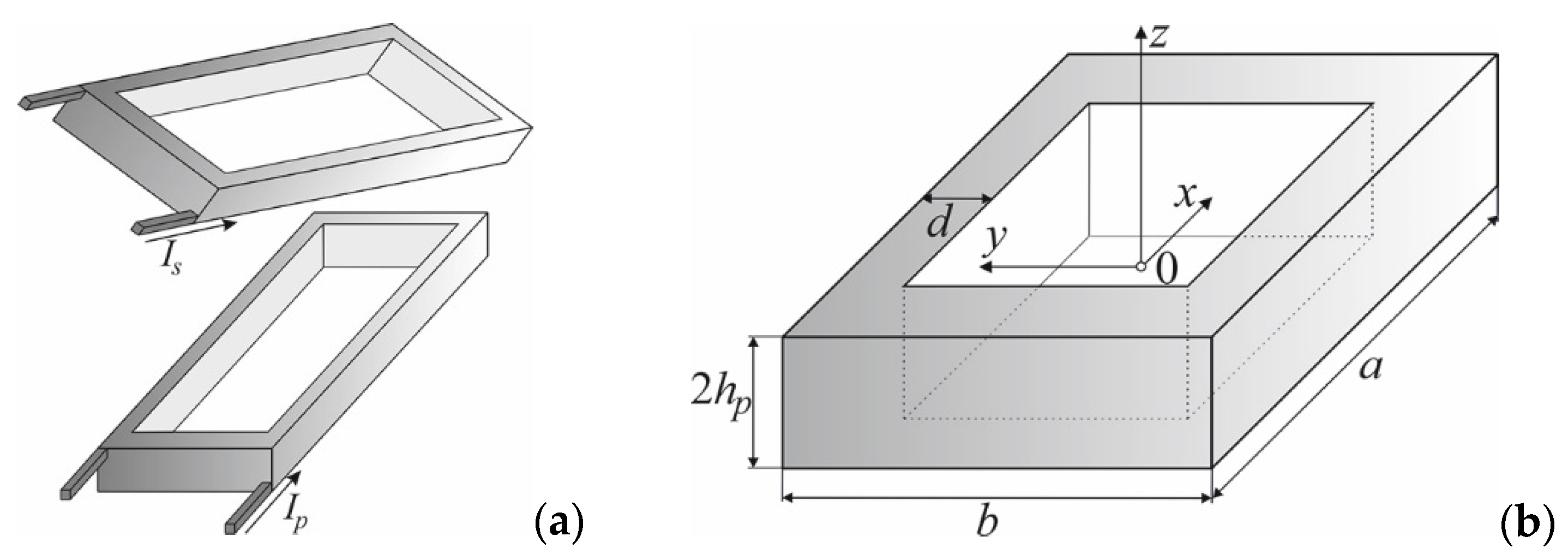
4. Rectangular Air Coils
5. Magnetic Field of Current Coils, Permanent Magnets, and High-Temperature Superconductors
6. Moving Coils: Induced Voltages and Forces
7. Conclusions
Funding
Data Availability Statement
Conflicts of Interest
References
- Triviño, A.; González, J.M.; Aguado, J.A. Wireless Power Transfer Technologies Applied to Electric Vehicles: A Review. Energies 2021, 14, 1547. [Google Scholar] [CrossRef]
- Razu, R.R.; Mahmud, S.; Uddin, M.J.; Islam, S.S.; Bais, B.; Misran, N.; Islam, M.T. Wireless Charging of Electric Vehicle While Driving. IEEE Access 2021, 9, 157973–157983. [Google Scholar] [CrossRef]
- Carvalho, N.B.; Georgiadis, A.; Costanzo, A.; Rogier, H.; Collado, A.; Garcia, J.A.; Lucyszyn, S.; Mezzanotte, P.; Kracek, J.; Masotti, D.; et al. Wireless Power Transmission: R&D Activities within Europe. IEEE Trans. Microw. Theory Tech. 2014, 62, 1031–1043. [Google Scholar]
- Schormans, M.; Valente, V.; Demosthenous, A. Practical Inductive Link Design for Biomedical Wireless Power Transfer: A Tutorial. IEEE Trans. Biomed. Circuits Syst. 2018, 12, 1112–1130. [Google Scholar] [CrossRef] [PubMed]
- Gong, C.; Liu, D.; Miao, Z.; Li, M. A Magnetic-Balanced Inductive Link for the Simultaneous Uplink Data and Power Telemetry. Sensors 2017, 17, 1768. [Google Scholar] [CrossRef] [PubMed]
- Wardach, M.; Bonislawski, M.; Palka, R.; Paplicki, P.; Prajzendanc, P. Hybrid Excited Synchronous Machine with Wireless Supply Control System. Energies 2019, 12, 3153. [Google Scholar] [CrossRef]
- Kalisiak, S.; Marcinek, M.; Hołub, M.; Pałka, R. Contactless power supply system with resonant circuit parameter change compensation. In Proceedings of the 14th European Conference on Power Electronics and Applications, Birmingham, UK, 30 August–1 September 2011. [Google Scholar]
- Marcinek, M.; Hołub, M.; Kalisiak, S.; Pałka, R. Resonant frequency stabilization technique in series-series contactless energy transfer systems. Arch. Electr. Eng. 2017, 66, 547–558. [Google Scholar] [CrossRef]
- Panchal, C.; Stegen, S.; Lu, J. Review of static and dynamic wireless electric vehicle charging system. Eng. Sci. Technol. Int. J. 2018, 21, 922–937. [Google Scholar] [CrossRef]
- Grover, F.W. The Calculation of the Mutual Inductance of Circular Filaments in any Desired Positions. Proc. IRE 1944, 32, 620–629. [Google Scholar] [CrossRef]
- Conway, J.T. Inductance Calculations for Noncoaxial Coils Using Bessel Functions. IEEE Trans. Magn. 2007, 43, 1023–1034. [Google Scholar] [CrossRef]
- Conway, J.T. Noncoaxial Inductance Calculations Without the Vector Potential for Axisymmetric Coils and Planar Coils. IEEE Trans. Magn. 2008, 44, 453–462. [Google Scholar] [CrossRef]
- Conway, J.T. Inductance Calculations for Circular Coils of Rectangular Cross Section and Parallel Axes Using Bessel and Struve Functions. IEEE Trans. Magn. 2010, 46, 75–81. [Google Scholar] [CrossRef]
- Babic, S.; Salon, S.; Akyel, C. The Mutual Inductance of Two Thin Coaxial Disk Coils in Air. IEEE Trans. Magn. 2004, 40, 822–825. [Google Scholar] [CrossRef]
- Babic, S.; Akyel, C. New analytic-numerical solutions for the mutual inductance of two coaxial circular coils with rectangular cross section in air. IEEE Trans. Magn. 2006, 42, 1661–1669. [Google Scholar] [CrossRef]
- Babic, S.; Akyel, C. Magnetic Force Calculation Between Thin Coaxial Circular Coils in Air. IEEE Trans. Magn. 2008, 44, 445–452. [Google Scholar] [CrossRef]
- Babic, S.; Sirois, F.; Akyel, C.; Girardi, C. Mutual Inductance Calculation Between Circular Filaments Arbitrarily Positioned in Space: Alternative to Grover’s Formula. IEEE Trans. Magn. 2010, 46, 3591–3600. [Google Scholar] [CrossRef]
- Babic, S.; Sirois, F.; Akyel, C.; Lemarquand, G.; Lemarquand, V.; Ravaud, R. New Formulas for Mutual Inductance and Axial Magnetic Force Between a Thin Wall Solenoid and a Thick Circular Coil of Rectangular Cross-Section. IEEE Trans. Magn. 2011, 47, 2034–2044. [Google Scholar] [CrossRef]
- Babic, S.; Akyel, C. New Formulas for Mutual Inductance and Axial Magnetic Force Between Magnetically Coupled Coils: Thick Circular Coil of the Rectangular Cross-Section-Thin Disk Coil (Pancake). IEEE Trans. Magn. 2013, 49, 860–868. [Google Scholar] [CrossRef]
- Babic, S.I.; Martinez, J.; Akyel, C.; Babic, B. Mutual Inductance Calculation between Misalignment Coils for Wireless Power Transfer of Energy. Prog. Electromagn. Res. M 2014, 38, 91–102. [Google Scholar] [CrossRef]
- Babic, S.I.; Smith, M.; Fokas, N.; Langer, Y.; Selvaggi, J.P. The Analytical Formula for Calculating the Self-Inductance for the Circular Coil of the Rectangular Cross-Section with a Nonuniform Current Density. Prog. Electromagn. Res. M 2021, 103, 15–23. [Google Scholar] [CrossRef]
- Župan, T.; Štih, Ž.; Trkulja, B. Fast and Precise Method for Inductance Calculation of Coaxial Circular Coils with Rectangular Cross Section Using the One-Dimensional Integration of Elementary Functions Applicable to Superconducting Magnets. IEEE Trans. Appl. Supercond. 2014, 24, 4901309. [Google Scholar] [CrossRef]
- Zhang, X.; Meng, H.; Wie, B.; Wang, S.; Yang, Q. Mutual inductance calculation for coils with misalignment in wireless power transfer. J. Eng. 2019, 16, 1041–1044. [Google Scholar] [CrossRef]
- Kushwaha, B.K.; Rituraj, G.; Kumar, P. 3-D Analytical Model for Computation of Mutual Inductance for Different Misalignments with Shielding in Wireless Power Transfer System. IEEE Trans. Transp. Electrif. 2017, 3, 332–342. [Google Scholar] [CrossRef]
- Pankrac, V. Generalization of Relations for Calculating the Mutual Inductance of Coaxial Coils in Terms of Their Applicability to Non-Coaxial Coils. IEEE Trans. Magn. 2011, 47, 4552–4563. [Google Scholar] [CrossRef]
- Pankrac, V.; Kracek, J. Simple Algorithms for the Calculation of the Intensity of the Magnetic Field of Current Loops and Thin-Wall Air Coils of a General Shape Using Magnetic Dipoles. IEEE Trans. Magn. 2012, 48, 4767–4778. [Google Scholar] [CrossRef]
- Dehui, W.; Qisheng, S.; Xiaohong, W.; Fang, C. Calculation of self- and mutual inductances of rounded rectangular coils with rectangular cross-sections and misalignments. IET Electr. Power Appl. 2018, 12, 1014–1019. [Google Scholar] [CrossRef]
- Wu, D.; Cheng, F.; Huang, C. Analytical computation of mutual inductance between two rectangular spiral coils with misalignments for wireless power applications. Microw. Opt. Technol. Lett. 2020, 62, 637–642. [Google Scholar] [CrossRef]
- Liang, S. Analysis of inductance calculation of coaxial circular filamentary coils, thin-wall solenoids, and disk coils using inverse hyperbolic functions. IET Sci. Meas. Technol. 2016, 10, 754–760. [Google Scholar] [CrossRef]
- Alkasir, A. Analytical Modeling of Self- and Mutual Inductances of DD Coils in Wireless Power Transfer Applications. J. Electromagn. Eng. Sci. 2022, 22, 162–170. [Google Scholar] [CrossRef]
- Cheng, Y.; Shu, Y. A New Analytical Calculation of the Mutual Inductance of the Coaxial Spiral Rectangular Coils. IEEE Trans. Magn. 2014, 50, 7026806. [Google Scholar] [CrossRef]
- Ollendorff, F. Berechnung Magnetischer Felder; Springer: Vienna, Austria, 1952. [Google Scholar]
- Abramowitz, M.; Stegun, I.A. Handbook of Mathematical Functions: With Formulas, Graphs, and Mathematical Tables; Dover Publications, INC.: New York, NY, USA, 1964. [Google Scholar]
- Mohan, S.S.; del Hershenson, M.M.; Boyd, S.P.; Lee, T.H. Simple accurate expressions for planar spiral inductances. IEEE J. Solid-State Circuits 1999, 34, 1419–1424. [Google Scholar] [CrossRef]
- Hussain, I.; Woo, D.-K. Self-Inductance Calculation of the Archimedean Spiral Coil. Energies 2022, 15, 253. [Google Scholar] [CrossRef]
- Aditya, K. Analytical design of Archimedean spiral coils used in inductive power transfer for electric vehicles application. Electr. Eng. 2018, 100, 1819–1826. [Google Scholar] [CrossRef]
- May, H.; Palka, R.; Portabella, E.; Canders, W.R. Evaluation of the magnetic field—High temperature superconductor interactions. COMPEL Int. J. Comput. Math. Electr. Electron. Eng. 2004, 23, 286–304. [Google Scholar] [CrossRef]
- Patel, A. The Use of an MgB2 Hollow Cylinder and Pulse Magnetized (RE)BCO Bulk for Magnetic Levitation Applications. IEEE Trans. Appl. Supercond. 2013, 23, 3. [Google Scholar] [CrossRef]
- Palka, R. Synthesis of Application-Optimized Air Gap Field Distributions in Synchronous Machines. Energies 2022, 15, 2322. [Google Scholar] [CrossRef]
- Sikora, R.; Palka, R. Synthesis of Magnetic-Fields. IEEE Trans. Magn. 1982, 18, 385–390. [Google Scholar] [CrossRef]
- Palka, R. Synthesis of magnetic fields by optimization of the shape of areas and source distributions. Electr. Eng. 1991, 75, 1–7. [Google Scholar] [CrossRef]
- Patil, D.; McDonough, M.K.; Miller, J.M.; Fahimi, B.; Balsara, P.T. Wireless Power Transfer for Vehicular Applications: Overview and Challenges. IEEE Trans. Transp. Electrif. 2018, 4, 3–37. [Google Scholar] [CrossRef]
- Hussain, I.; Woo, D.-K. Simplified Mutual Inductance Calculation of Planar Spiral Coil for Wireless Power Applications. Sensors 2022, 22, 1537. [Google Scholar] [CrossRef]
- Yang, M.; Li, Z.; Zhang, M.; Wan, J. Mutual Inductance Calculation of Circular Coils Sandwiched between 3-Layer Magnetic Mediums for Wireless Power Transfer Systems. Electronics 2021, 10, 3043. [Google Scholar] [CrossRef]
- Babic, S. Analytical and Semi-Analytical Formulas for the Self and Mutual Inductances of Concentric Coplanar Ordinary and Bitter Disk Coils. Physics 2021, 3, 240–254. [Google Scholar] [CrossRef]
- Parise, M.; Loreto, F.; Romano, D.; Antonini, G.; Ekman, J. Accurate Computation of Mutual Inductance of Non Coaxial Pancake Coils. Energies 2021, 14, 4907. [Google Scholar] [CrossRef]



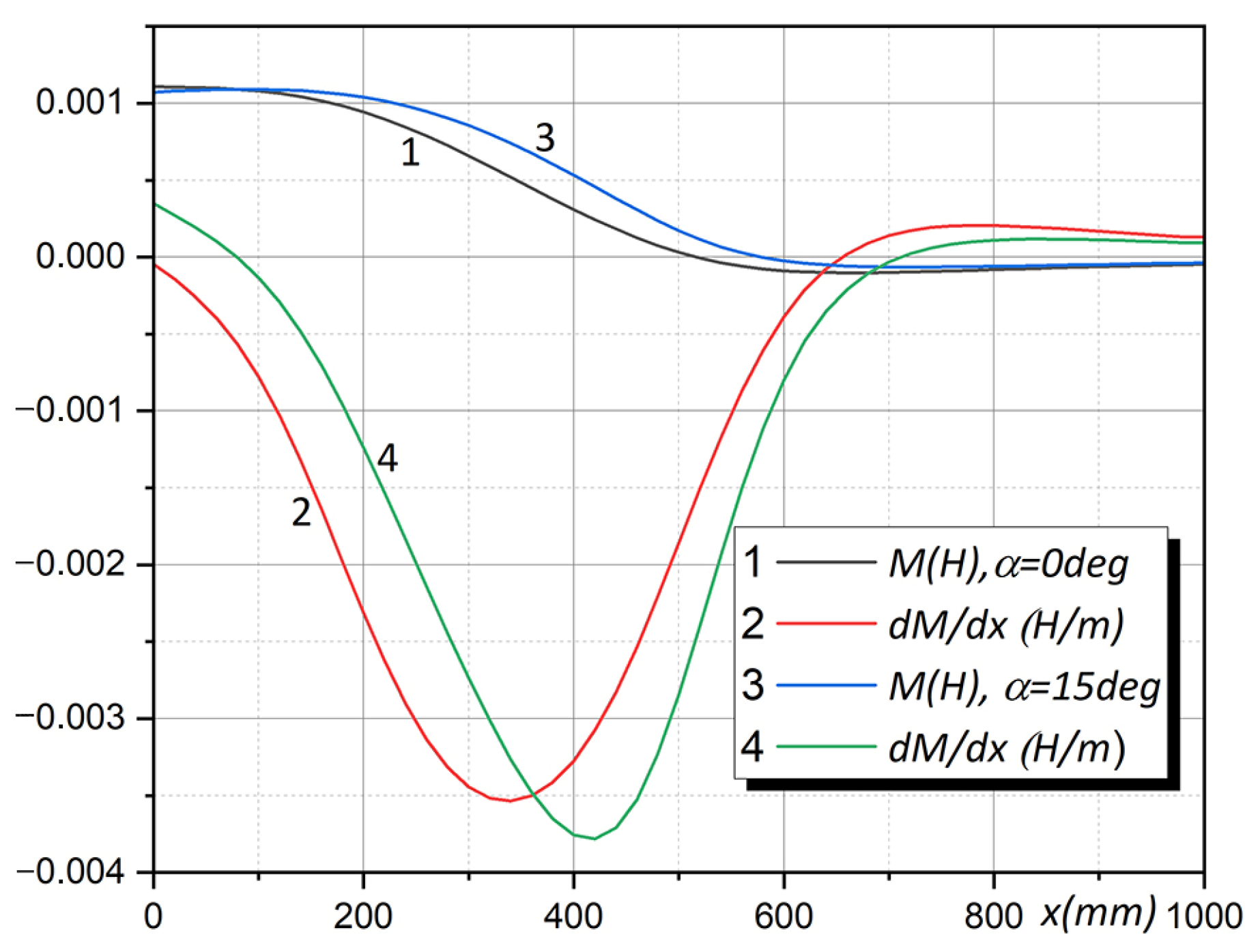

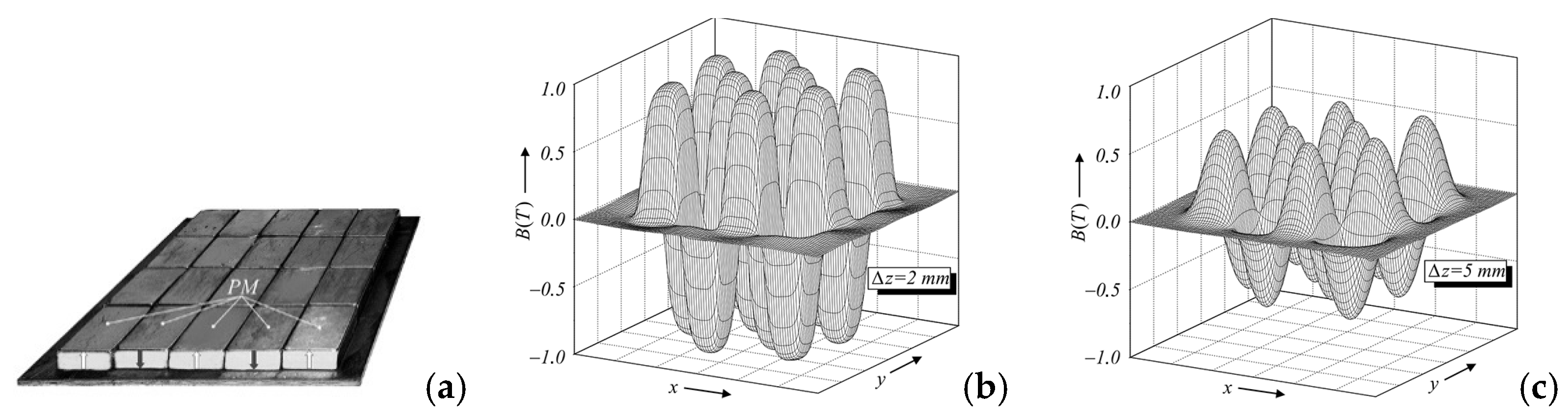

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Palka, R. Fast Analytic–Numerical Algorithms for Calculating Mutual and Self-Inductances of Air Coils. Energies 2024, 17, 325. https://doi.org/10.3390/en17020325
Palka R. Fast Analytic–Numerical Algorithms for Calculating Mutual and Self-Inductances of Air Coils. Energies. 2024; 17(2):325. https://doi.org/10.3390/en17020325
Chicago/Turabian StylePalka, Ryszard. 2024. "Fast Analytic–Numerical Algorithms for Calculating Mutual and Self-Inductances of Air Coils" Energies 17, no. 2: 325. https://doi.org/10.3390/en17020325
APA StylePalka, R. (2024). Fast Analytic–Numerical Algorithms for Calculating Mutual and Self-Inductances of Air Coils. Energies, 17(2), 325. https://doi.org/10.3390/en17020325





