Impact of Phase Angle Jump on a Doubly Fed Induction Generator under Low-Voltage Ride-Through Based on Transfer Function Decomposition
Abstract
1. Introduction
- (1)
- There is a coupling relationship between different variables in a DFIG, which increases the difficulty of solving the transfer function. In this paper, the differential-algebraic equations of the DFIG are linearized, and the transfer functions are obtained by separating the input and output variables from the equations;
- (2)
- Voltage magnitude and the phase of the point of common coupling are not explicitly included in the DFIG model equation, so it is necessary to establish the relationship between the voltage component of the xy axis, voltage amplitude, and phase;
- (3)
- The transfer function model based on the detailed DFIG model is of a high order, and it is difficult to perform inverse Laplace transformation directly to obtain the analytic expression of the output variable of a DFIG. A transfer-function-reduction method for DFIGs based on Schur decomposition is proposed.
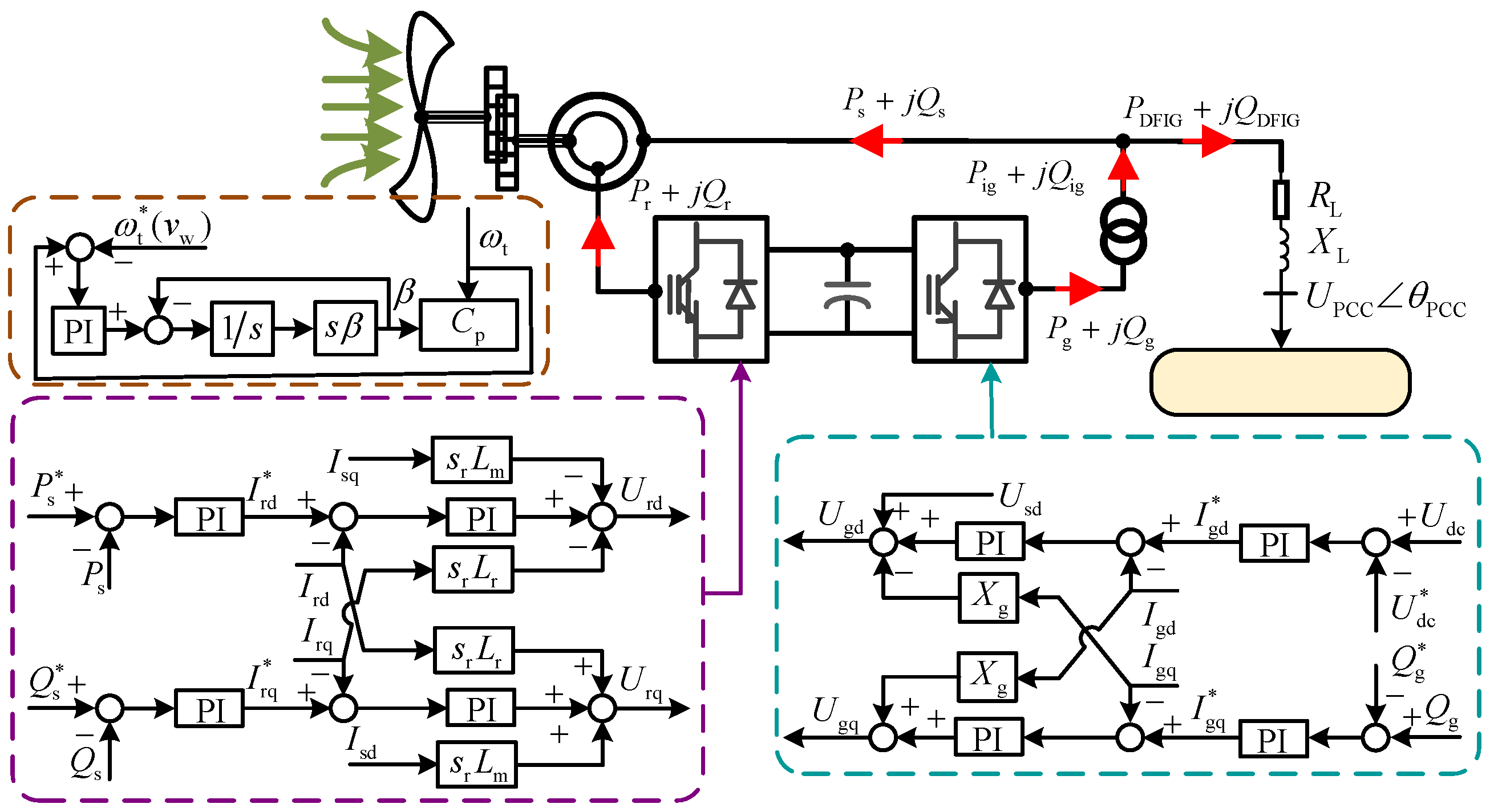
2. Transfer Function Model of the DFIG
2.1. Linearized Model of the DFIG
2.2. Transfer Function Model Based on the Laplace Transform
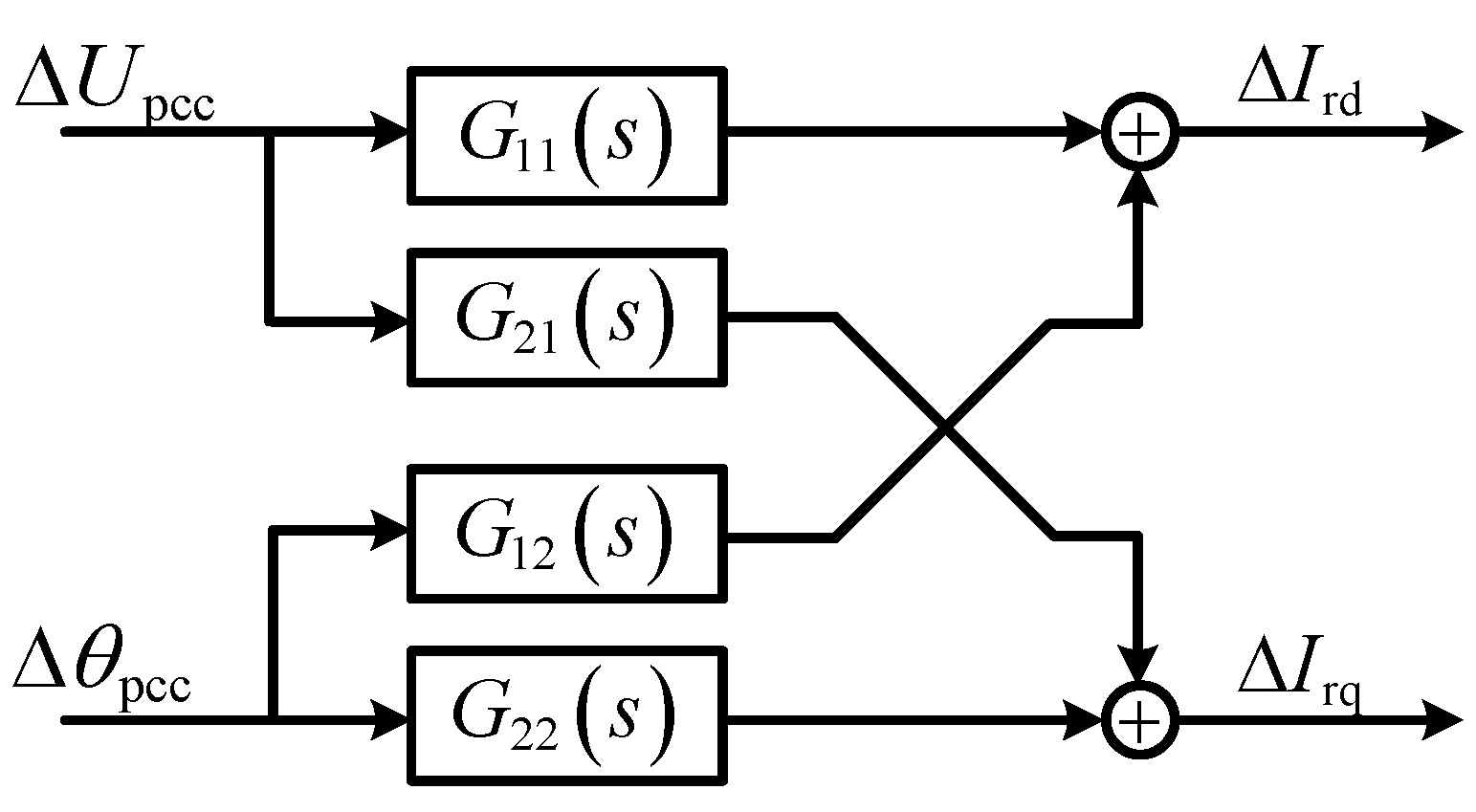
3. The Reduced Model of the Transfer Function
4. Numerical Validation
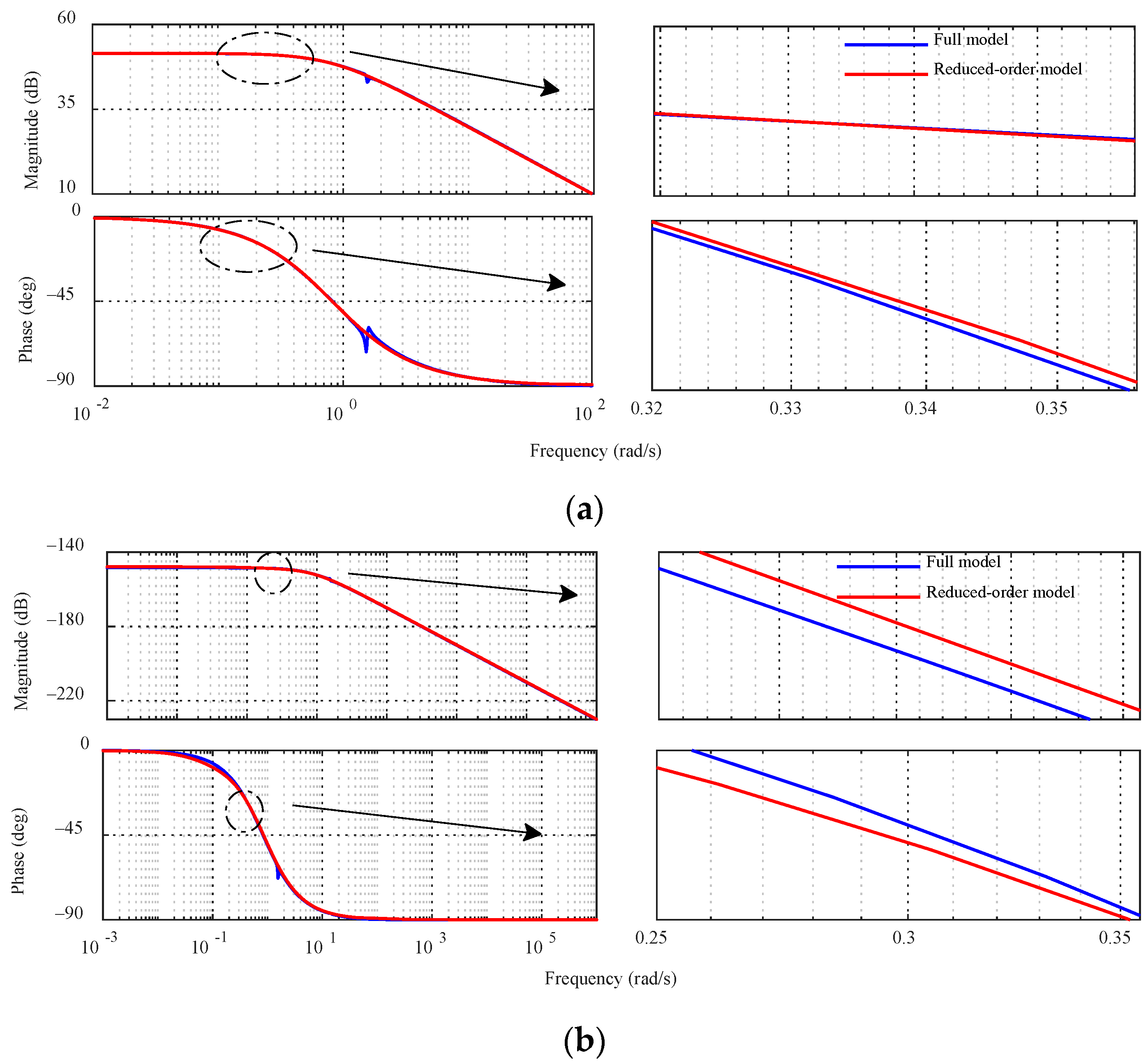
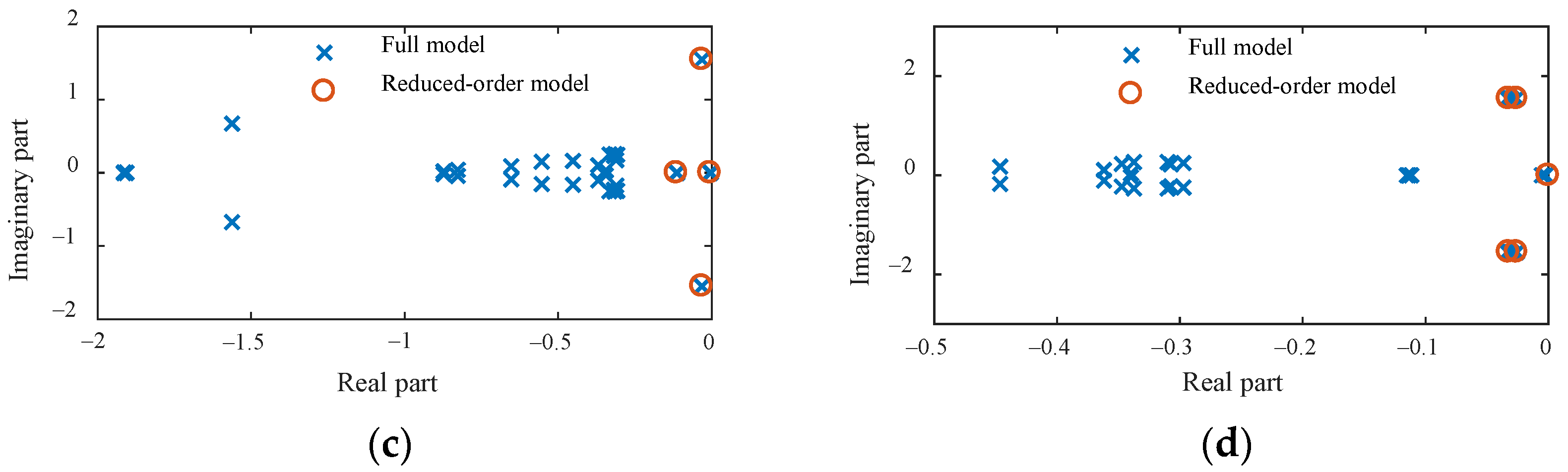
4.1. Accuracy Verification of the Reduced-Order Model of the Transfer Function
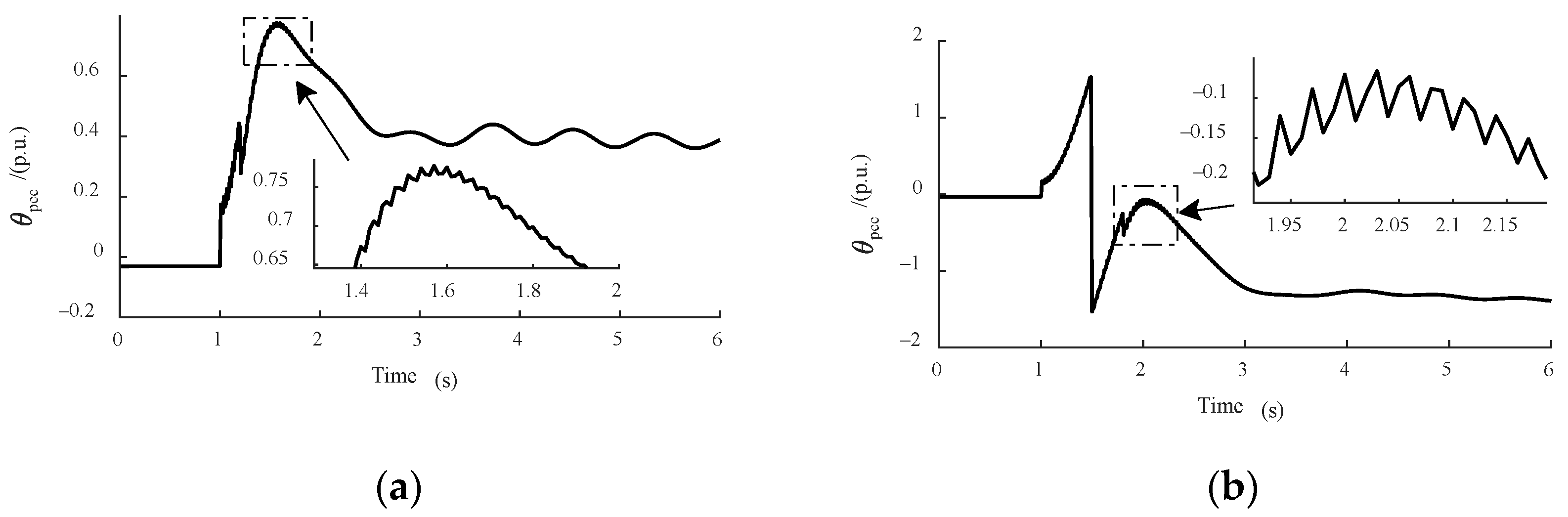
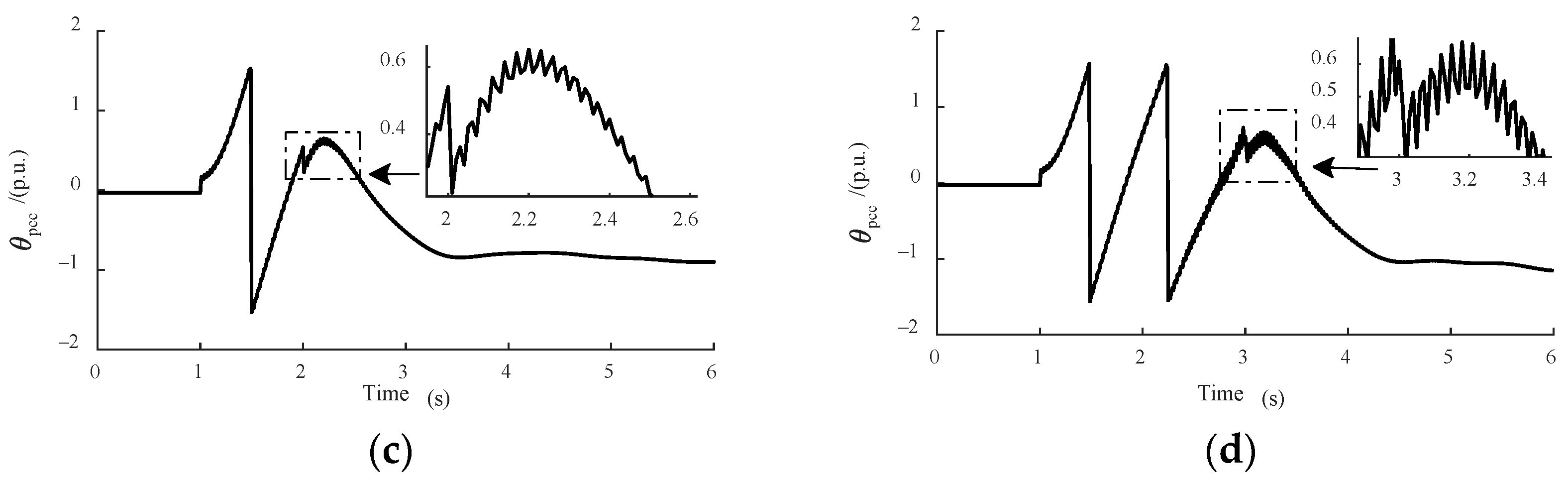
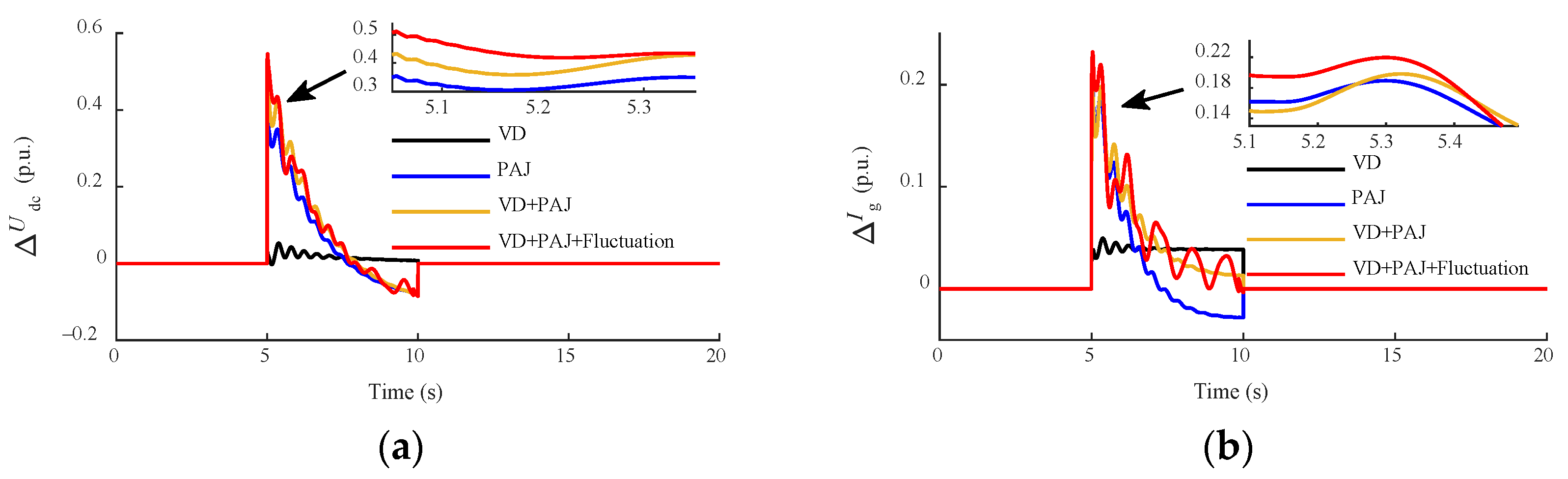
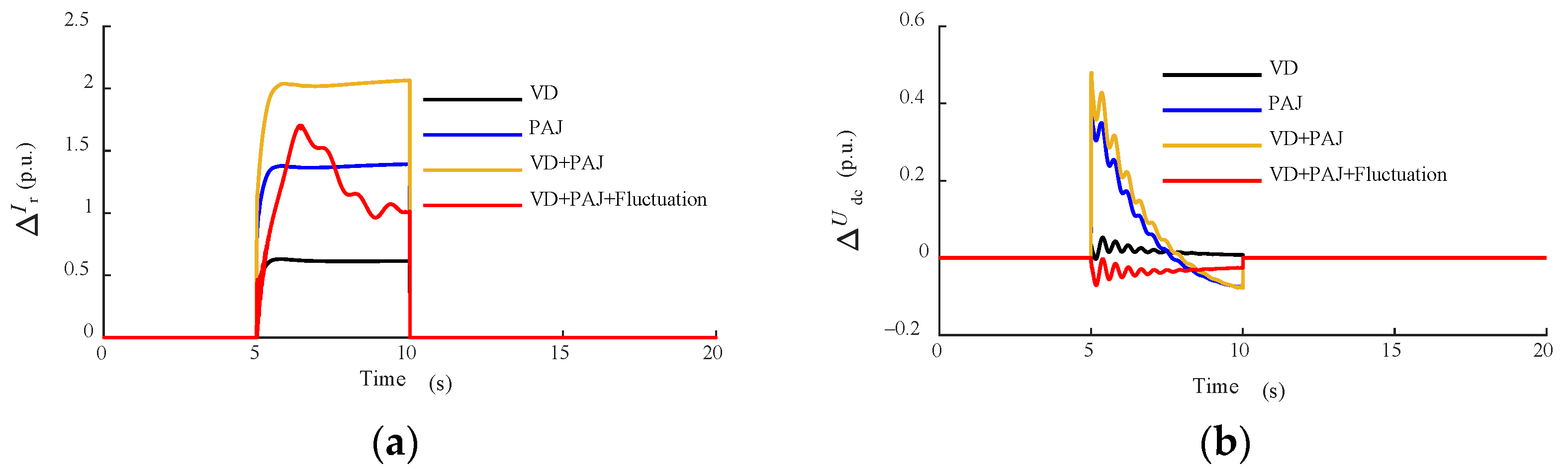
4.2. DFIG Response Characteristic Analysis with the Phase Angle Jump and Its Fluctuation
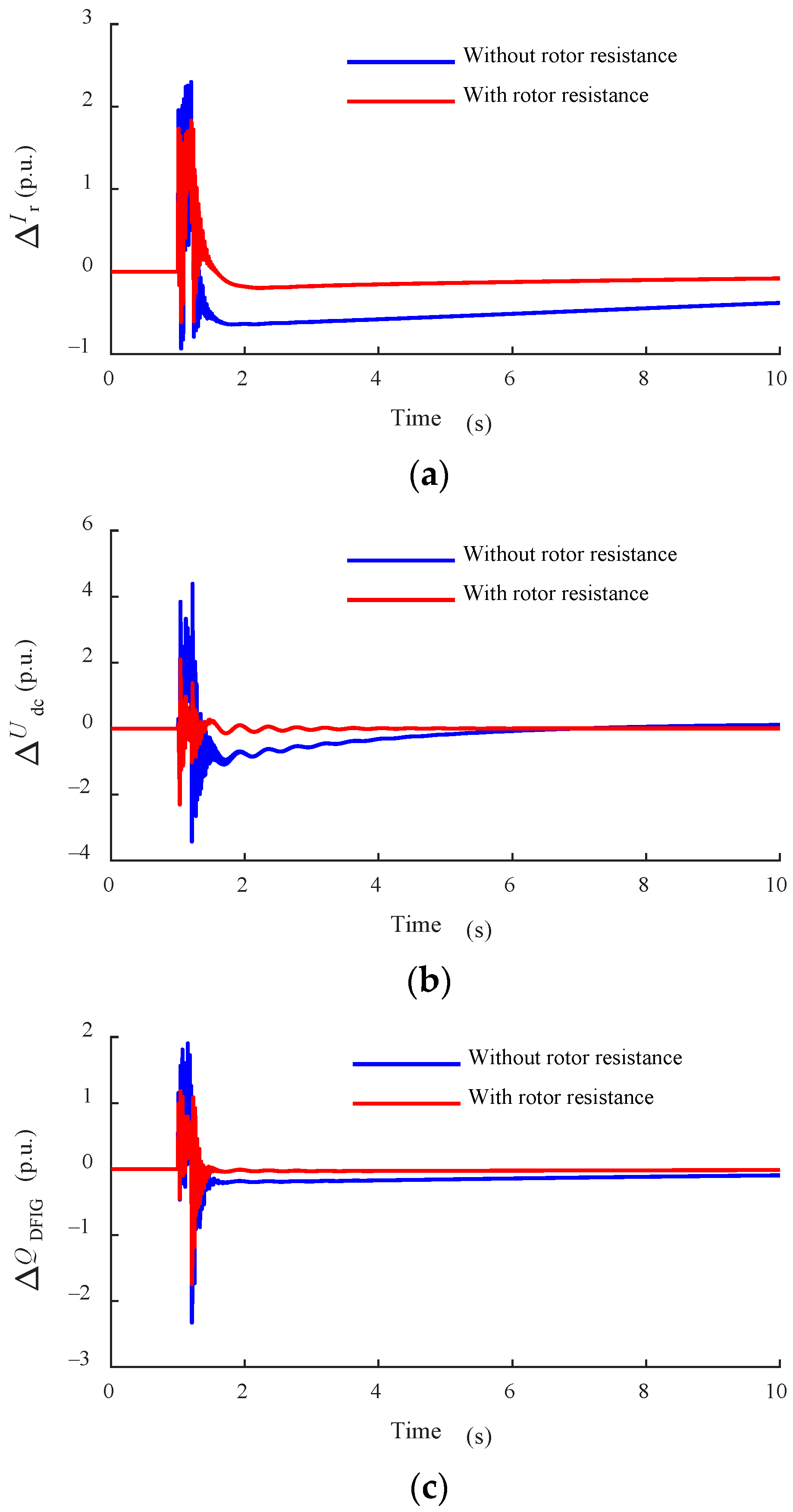
4.3. Validity Verification of Rotor String Resistance Switching Strategy
5. Conclusions
- (1)
- The model reduction method based on Schur decomposition maintains the dominant characteristics of the DFIG, and the response characteristics within the middle- and high-frequency bands are consistent with the actual response;
- (2)
- The response curves of the stator current, the rotor current, and the DC voltage based on the reduced transfer function are consistent with those of the time-domain simulation results, which verifies the accuracy of the proposed reduced transfer function of the DFIG. Compared with the time domain simulation, the transfer function is computationally more efficient, and the simulation time is about 4% of that using the time domain simulation;
- (3)
- The rotor current of the DFIG and the PCC voltage with phase angle jump and its fluctuation increase significantly compared with those with the voltage drop only. Hence, a large phase angle jump will threaten the security of the DFIG and the integrated power system. The existing LVRT measures against a voltage drop should be improved by considering the negative impact of the phase angle jump.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Gao, X.; Xie, Z.; Li, M.; Yang, S.; Zhang, X. Analysis and mitigation of electromechanical oscillations in drivetrain for hybrid synchronization control of DFIG-based wind turbines. IEEE Trans. Power Electron. 2024, 39, 3002–3013. [Google Scholar] [CrossRef]
- Saha, S.; Saleem, M.I.; Roy, T.K. Impact of high penetration of renewable energy sources on grid frequency behaviour. Int. J. Electr. Power Energy Syst. 2023, 145, 142–615. [Google Scholar] [CrossRef]
- Alsmadi, Y.M.; Xu, L.; Blaabjerg, F.; Ortega, A.J.P.; Abdelaziz, A.Y.; Wang, A.; Albataineh, Z. Detailed investigation and performance improvement of the dynamic behavior of grid-connected DFIG-based wind turbines under LVRT conditions. IEEE Trans. Ind. Appl. 2018, 54, 4795–4812. [Google Scholar] [CrossRef]
- Loulijat, A.; Ababssi, N.; Mohamed, M. Kalman observer contribution to a second order sliding mode control for wind turbine based on DFIG during the network voltage dip. Int. J. Intell. Eng. Syst. 2021, 14, 88–101. [Google Scholar] [CrossRef]
- Qin, B.; Li, H.; Zhou, X.; Li, J.; Liu, W. Low-voltage ride-through techniques in DFIG-Based wind turbines: A Review. Appl. Sci. 2020, 10, 2154. [Google Scholar] [CrossRef]
- Tian, X.; Li, G.; Chi, Y. Voltage phase angle jump characteristic of DFIGs in case of weak grid connection and grid fault. J. Mod. Power Syst. Clean Energy 2016, 4, 256–264. [Google Scholar] [CrossRef]
- Reddy, K.; Saha, A.K. A heuristic approach to optimal crowbar setting and low voltage ride through of a doubly fed induction generator. Energies 2022, 15, 9307. [Google Scholar] [CrossRef]
- Jiang, D.; Yu, W.; Wang, J.; Zhong, G.; Zhou, Z. Dynamic analysis of DFIG fault detection and its suppression using sliding mode control. IEEE J. Emerg. Sel. Top. Power Electron. 2023, 11, 643–656. [Google Scholar] [CrossRef]
- Yin, J.; Huang, X.; Qian, W. Analysis and research on short-circuit current characteristics and grid access faults of wind farms with multi-type fans. Energy Rep. 2024, 11, 1161–1170. [Google Scholar] [CrossRef]
- Wang, Y.; Bollen, M.H.J.; Xiao, X.-Y. Calculation of the phase-angle jump for voltage dips in three-phase systems. IEEE Trans. Power Deliv. 2015, 30, 480–487. [Google Scholar] [CrossRef]
- Ren, J.; Xiao, X.; Zheng, Z.; Wang, Y.; Ma, Z.; Liu, K. Impact of phase angle jump on DFIG under LVRT conditions: Challenges and recommendations. IEEE Trans. Power Deliv. 2021, 36, 3701–3713. [Google Scholar] [CrossRef]
- Geng, H.; Liu, C.; Yang, G. LVRT capability of DFIG-based WECS under asymmetrical grid fault condition. IEEE Trans. Ind. Electron. 2013, 60, 2495–2509. [Google Scholar] [CrossRef]
- Ren, J.; Xiao, X.; Liu, Y.; Zheng, Z.; Chen, W. Impact of PAJ with varying POW in voltage sag on rotor over-voltage in DFIG-based wind generator. CIRED-Open Access Proc. J. 2017, 2017, 715–718. [Google Scholar] [CrossRef][Green Version]
- Tian, X.; Chi, Y.; Wang, W.; Li, G.; Tang, H.; Wang, Z. Transient characteristics and adaptive fault ride through control strategy of DFIGs considering voltage phase angle jump. J. Mod. Power Syst. Clean Energy 2017, 5, 757–766. [Google Scholar] [CrossRef]
- Taul, M.; Wang, X.; Davari, P.; Blaabjerg, F. Robust fault ride through of converter-based generation during severe faults with phase jumps. IEEE Trans. Ind. Appl. 2020, 56, 570–583. [Google Scholar] [CrossRef]
- Pan, X.; Liang, W.; Guo, J.; Sun, X.; Chen, H.; He, D. Parameter estimation by considering both voltage dip and phase angle jump for DFIG-based WTGs in distribution grids. IEEE Trans. Sustain. Energy 2024. [Google Scholar] [CrossRef]
- Liu, X.; Zhang, Z.; Liu, Y.; Liu, Z.; Su, M.; Li, C.; Ge, L.; Zhang, X.; Wang, P. Fault current unified calculation method for whole process fault ride-through of DFIG-based wind farms. IEEE Trans. Smart Grid 2024, 15, 485–503. [Google Scholar] [CrossRef]
- Xiong, X.; Luo, B.; Li, L.; Sun, Z.; Blaabjerg, F. Impedance reshaping method of DFIG system based on compensating rotor current dynamic to eliminate PLL influence. IEEE Trans. Power Electron. 2024, 39, 4006–4016. [Google Scholar] [CrossRef]
- Zhang, D.; Ma, J.; Wang, S.; Ji, Y.; Qu, C. Analysis on the characteristic of the instantaneous inrush current of brushless doubly fed induction generator. IEEE Trans. Energy Convers. 2023, 38, 747–758. [Google Scholar] [CrossRef]
- Yang, Y.; Zhu, D.; Zhou, D.; Zou, X.; Hu, J.; Kang, Y. Synchronization instability mechanism and damping enhancement control for DFIG-based wind turbine during grid faults. IEEE Trans. Power Electron. 2023, 38, 12104–12115. [Google Scholar] [CrossRef]
- Du, C.; Du, X.; Tong, C. SSR Stable Wind speed range quantification for DFIG-based wind power conversion system considering frequency coupling. IEEE Trans. Sustain. Energy 2023, 14, 125–139. [Google Scholar] [CrossRef]
- Yang, H.; Yuan, X. Modeling and analyzing the effect of frequency variation on weak grid-connected VSC system stability in DC voltage control timescale. Energies 2019, 12, 4458. [Google Scholar] [CrossRef]
- Abo-Khalil, A.G.; Alghamdi, A.; Tlili, I.; Eltamaly, A.M. Current controller design for DFIG-based wind turbines using state feedback control. IET Renew. Power Gener. 2019, 13, 1938–1948. [Google Scholar] [CrossRef]
- Zhang, Y.; Tang, W.; Fang, Y.; Liu, Y.; He, W.; Li, Y. Reduced-order model of frequency response including PLL-based DFIG wind turbine. In Proceedings of the 2023 2nd International Conference on Smart Grids and Energy Systems (SGES), Guangzhou, China, 25–27 August 2023; pp. 156–162. [Google Scholar]
- Huang, J.; Sang, S.; Zhang, L.; Xue, X.; Sun, T. Control of a variable-impedance fault current limiter to assist low-voltage ride-through of doubly fed induction generators. Electronics 2021, 10, 2364. [Google Scholar] [CrossRef]
- Kaloi, G.S.; Baloch, M.H.; Kumar, M.; Soomro, D.M.; Chauhdary, S.T.; Memon, A.A.; Ishak, D. An LVRT scheme for grid connected DFIG based WECS using state feedback linearization control technique. Electronics 2019, 8, 777. [Google Scholar] [CrossRef]
- Sabzevari, K.; Khosravi, N.; Abdelghany, M. Low-voltage ride-through capability in a DFIG using FO-PID and RCO techniques under symmetrical and asymmetrical faults. Sci. Rep. 2023, 13, 17534. [Google Scholar] [CrossRef]
- Mohsen, G.; Mehdi, F.; Babak, M.; Farzad, G. Power flow management and LVRT enhancement by using multi-functional capacitive bridge-type fault current limiter in DFIG system. Int. J. Electr. Power Energy Syst. 2023, 148, 108810. [Google Scholar]
- Zhou, N.; Ma, H.; Chen, J.; Fang, Q.; Jiang, Z.; Li, C. Equivalent modeling of LVRT characteristics for centralized DFIG wind farms based on PSO and DBSCAN. Energies 2023, 16, 2551. [Google Scholar] [CrossRef]
- Xian, L.; Wu, L.; Li, W.; Wu, Y.; Xie, X. Enhancing FRT capability of DFIG based on RC-crowbar considering the resonance and matching control strategies for different fault degrees. Electr. Power Syst. Res. 2024, 232, 110434. [Google Scholar] [CrossRef]
- Li, S.; Fang, T.; Zhang, H.; Ye, J. Damping the electromechanical oscillation modes (EOMs) in DFIG-integrated power systems with sensitivity analysis and optimization to outputs of SGs. Int. J. Electr. Power Energy Syst. 2022, 135, 107565. [Google Scholar] [CrossRef]
- Li, S. Low-frequency oscillations of wind power systems caused by doubly-fed induction generators. Renew. Energy 2017, 104, 129–138. [Google Scholar] [CrossRef]
- Liu, X.; Zhang, P.; Deng, X.; Sun, D. Hierarchical overvoltage predictive control scheme for a DFIG-based wind farm. Electr. Power Syst. Res. 2023, 217, 109172. [Google Scholar] [CrossRef]
- Yuan, H.; Wang, D.; Zhou, X. Frequency support of DFIG-based wind turbine via virtual synchronous control of inner voltage vector. Electr. Power Syst. Res. 2023, 225, 109823. [Google Scholar] [CrossRef]



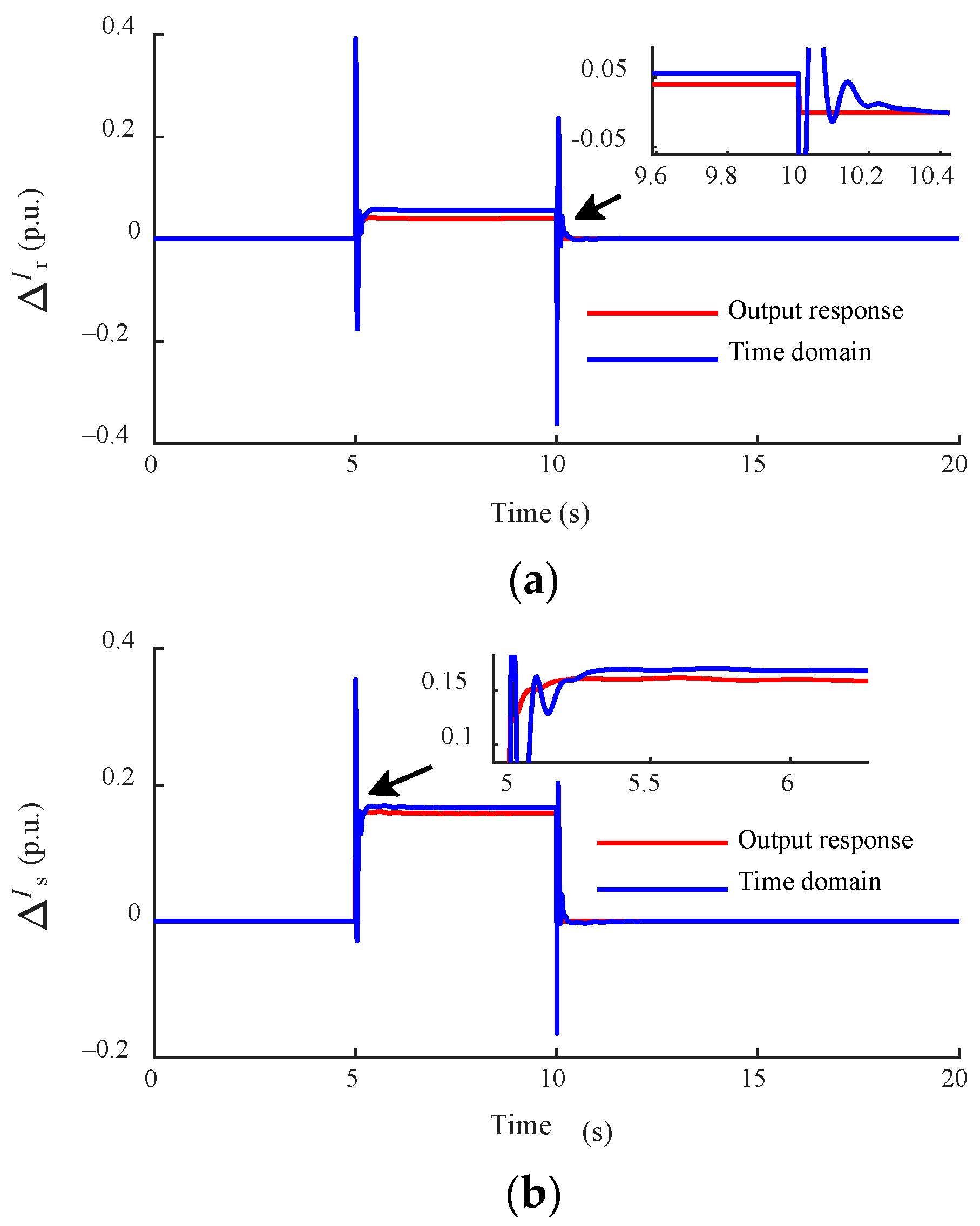
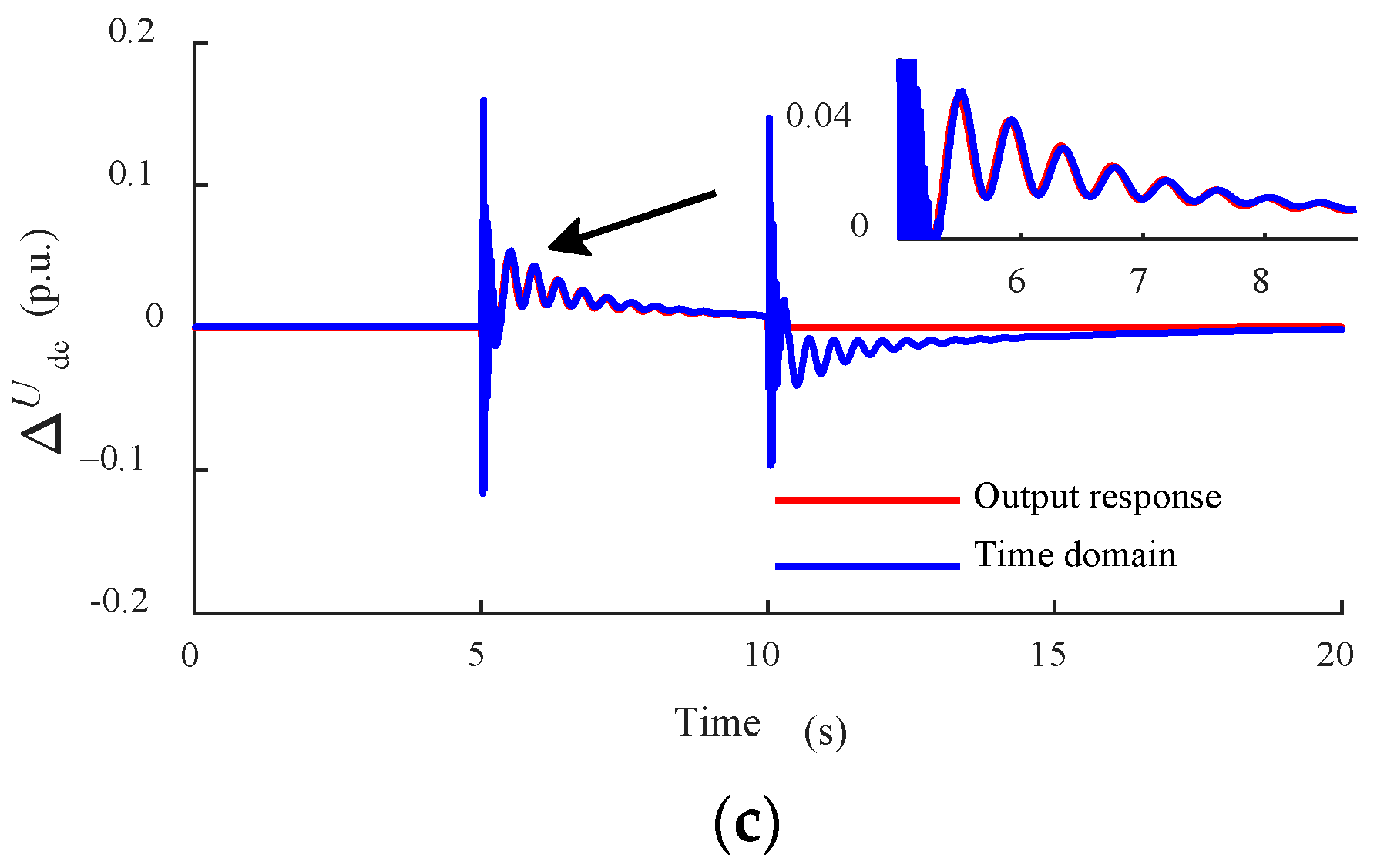

| Output Response | Time Domain | |
|---|---|---|
| Simulation time (s) | 0.25 | 5.98 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Feng, P.; Xu, J.; Wang, Z.; Li, S.; Shen, Y.; Gui, X. Impact of Phase Angle Jump on a Doubly Fed Induction Generator under Low-Voltage Ride-Through Based on Transfer Function Decomposition. Energies 2024, 17, 4778. https://doi.org/10.3390/en17194778
Feng P, Xu J, Wang Z, Li S, Shen Y, Gui X. Impact of Phase Angle Jump on a Doubly Fed Induction Generator under Low-Voltage Ride-Through Based on Transfer Function Decomposition. Energies. 2024; 17(19):4778. https://doi.org/10.3390/en17194778
Chicago/Turabian StyleFeng, Peiru, Jiayin Xu, Zhuang Wang, Shenghu Li, Yuming Shen, and Xu Gui. 2024. "Impact of Phase Angle Jump on a Doubly Fed Induction Generator under Low-Voltage Ride-Through Based on Transfer Function Decomposition" Energies 17, no. 19: 4778. https://doi.org/10.3390/en17194778
APA StyleFeng, P., Xu, J., Wang, Z., Li, S., Shen, Y., & Gui, X. (2024). Impact of Phase Angle Jump on a Doubly Fed Induction Generator under Low-Voltage Ride-Through Based on Transfer Function Decomposition. Energies, 17(19), 4778. https://doi.org/10.3390/en17194778




