The Influence of Reduced Frequency on H-VAWT Aerodynamic Performance and Flow Field Near Blades

Abstract

Studies demonstrate that the reduced frequency k is influenced by the incoming wind speed U₀ and the rotor speed n. As a dimensionless parameter, k characterizes the stability of the flow field, which is a critical factor affecting the performance of vertical-axis wind turbines (VAWTs). This paper investigates the impact of k on the performance of straight-blade vertical-axis wind turbines (H-VAWT). The findings indicate that 0.05 is the critical value of k. The same k results in a similar flow field structure, yet the performance changes vary with different U₀. A decrease in n or an increase in U₀ leads to an increase in the average value and fluctuation of k, which subsequently reduces the rotor rotation torque C_m and decreases the maximum wind energy utilization rate Cp_max. This reduction in Cp_max weakens the stability of the flow field. Additionally, the high-speed area of the blade’s trailing edge velocity trajectory at $\theta =0°$, $\theta =120°$, and $\theta =240°$ expands with increasing range. Velocity dissipation in the high-speed area of the trailing edge affects the stability of the flow field within the rotor.

1. Introduction

Since the introduction of the “dual carbon” objective, enhancing wind energy efficiency has become a hot research topic [1]. Horizontal-axis wind turbines (HAWT) and vertical-axis wind turbines (VAWT) are the main devices utilizing wind energy. Compared to HAWTs, VAWTs have been developed as an important component for utilizing wind energy in urban and marine wind applications due to their simple design, adaptability to various environments, and high efficiency of wind energy conversion [2,3,4,5,6,7]. However, during the operation of VAWTs, the substantially varying angle of attack leads to unstable aerodynamic performance. The dimensionless parameter—reduced frequency ($k$) has an effect on the unstable aerodynamic performance of VAWTs based on research, which is the main factor affecting its performance [8,9].

Numerous experimental and theoretical investigations have examined the influence of reduced frequency on wind turbine performance with the rapid advancement of wind energy technology [10,11]. Nidiana et al. [12] conducted a study on the dynamic stall of VAWTs with NACA0015, and results indicate that with the increase in the reduced frequency, the calculated stall-onset angle increases. Galera et al. [13] have aimed to explore the relationship between reduced frequency, aerodynamic forces, and the torque generated by the blades. Ullah et al. [14] have also investigated the impacts of reduced frequency on the dynamic stall of VAWTs. They found that at the low angle of attacks, increasing the reduced frequency had little influence on the lift coefficient, while at a higher angle of attacks, the slope of the lift coefficient decreased with decreasing reduced frequency. Zhu et al. [9] conducted a study on the effects of the reduced frequency on the aerodynamic performance and dynamic stall behaviors of a VAWT. This investigation indicates that increasing reduced frequency can significantly affect the dynamic stall behavior and effectively improve the VAWT performance. As reduced frequency increases, the onset of dynamic stall is gradually delayed to a higher angle of attack, which effectively suppresses the separated flow.

In addition, changes in reduced frequency directly impact flow fields, such as the evolution of the tip vortex and the formation of wake structures. Li et al. [15] examined the vortex behaviors and aerodynamic forces in dynamic stall. The research has shown that leading edge vortices are sufficient to stabilize the airflow at higher reduced frequencies even when the airfoil is in the downstroke phase. Ambrogi et al. [16] investigated the unsteady separation characteristics in the turbulent boundary layer. They found that the reduced frequency has a significant effect on the transient flow-separation process. These factors significantly influence the overall energy capture efficiency of H-type vertical-axis wind turbines (H-VAWTs) [17]. Specifically, higher reduced frequencies typically result in larger unsteady aerodynamic forces, which can lead to more severe fatigue cycles in wind turbine blades. Conversely, lower reduced frequencies may result in insufficient energy capture in the wake, thereby affecting the output performance of downstream wind turbines [18].

Although considerable investigations have been carried out, it remains to be investigated how the reduced frequency affects the performance and flow field of an H-VAWT rather than an airfoil during its operation. Further research is also necessary on the effect of the critical value of the reduced frequency on H-VAWTs. The main purpose of the present work is to deepen the understanding of performance variations in H-VAWTs and the evolution of the flow field within the wind turbine influenced by reduced frequency through 3D numerical simulations.

This article is organized as follows: Section 2 covers the validation of the geometric model, the numerical simulation approach, and the comparison with field experimental data. Section 3 explores the impact of reduced frequency on H-VAWT performance and examines how this performance interacts with the internal flow field of the wind rotor. Finally, Section 4 summarizes the main achievements and conclusions.

2. Models and Methods

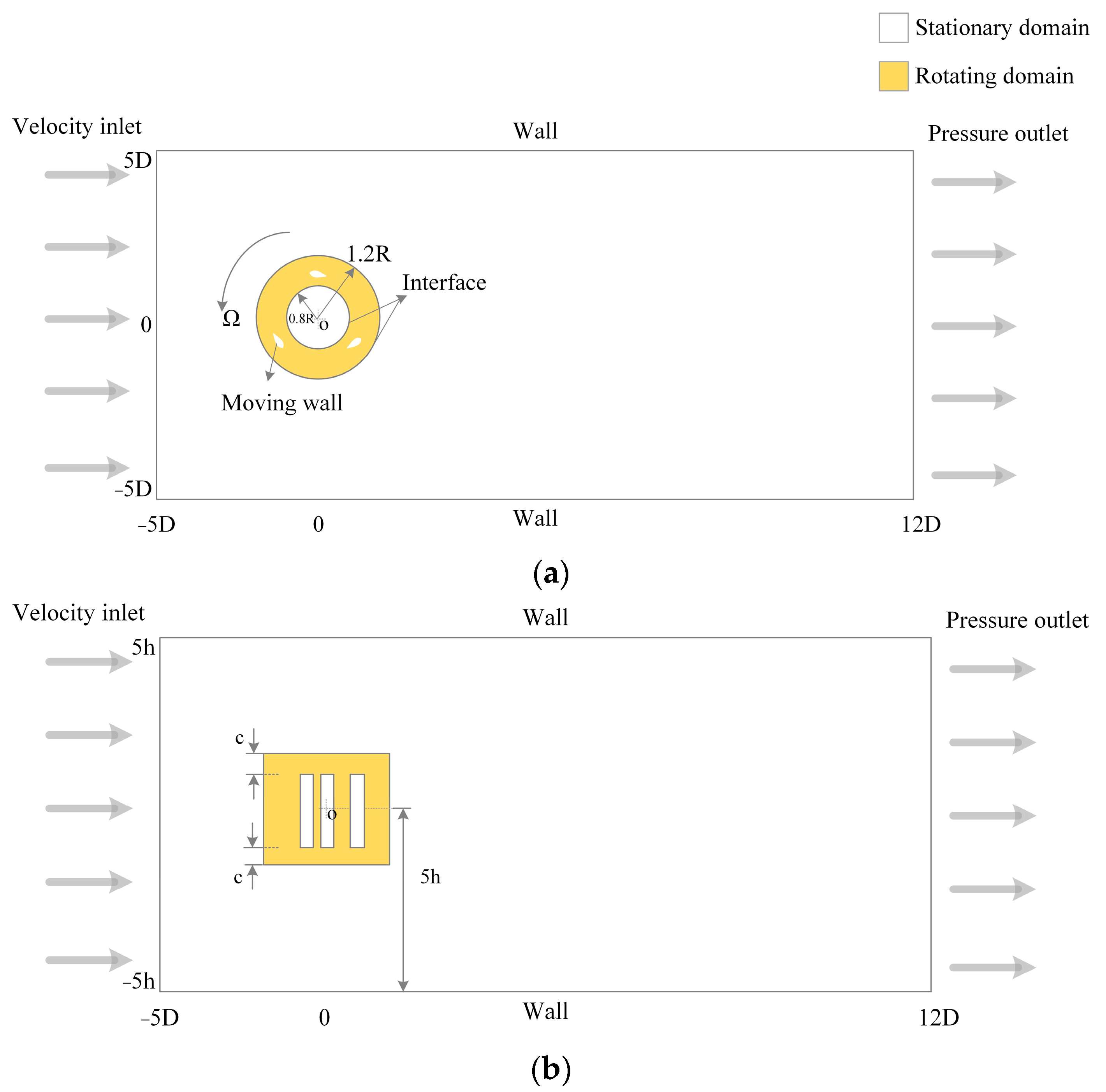
2.1. Physical Model

The H-VAWT model is based on a design from Uppsala University, as comprehensive field experiment data are available [19,20]. The detailed parameters of the H-VAWT are outlined in

Table 1. Main parameters of 12 kW H-VAWT.

Parameter	Value
Airfoil	NACA 0021
$\mathrm{Installation} \mathrm{angle} (\alpha$)	2 (°)
Chord length (c)	0.25 (m)
Number of blades	3
Blade length (h)	5 (m)
Radius (R)	3 (m)
Diameter (D)	6 (m)
Rated wind speed	12 (m/s)
Rated speed	127 (rpm)

, and the numerical model is shown in Figure 1. The rotor shaft and blade bracket are excluded from the numerical model to create high-quality structured grids [21]. Figure 2 shows the top view of the model along with an analysis of blade speed.

2.2. Turbulence Model

This research employed a 3D Unsteady Reynolds-averaged Navier–Stokes (URANS) method due to the 3D loss of power coefficient ($C_p$) in VAWTs, which cannot be ignored as shown in the investigation of Madrigal et al. [22]. Balduzzi et al. [9] demonstrated that the 3D URANS method can effectively analyze complex aerodynamic phenomena, including dynamic stalls. Therefore, the 3D URANS method has been widely employed for studying the aerodynamic characteristics of H-VAWTs [23,24,25,26,27,28,29].

To ensure consistency with field experiment data, the $SSTk-\omega$ turbulence model is applied. Li et al. [24] published a review on numerical simulation of VAWTs, summarizing the application of turbulence models. $SSTk-\omega$ turbulence model retains the advantages of $k-\epsilon$ model and $k-\omega$ model [30]. Daroczy et al. [14] demonstrated the suitability of the $SSTk-\omega$ model for numerically simulating H-VAWTs. When the 3D method is used for numerical simulation, the calculated results of $SSTk-\omega$ are well consistent with the experimental values at low tip speed ratios [31]. Compared with Direct Numerical Simulation and Large Eddy Simulation, the $SSTk-\omega$ turbulence model has been proven to reduce computational resources as well as ensure accuracy [9,32].

Therefore, the $SSTk-\omega$ turbulence model is applied in this research.

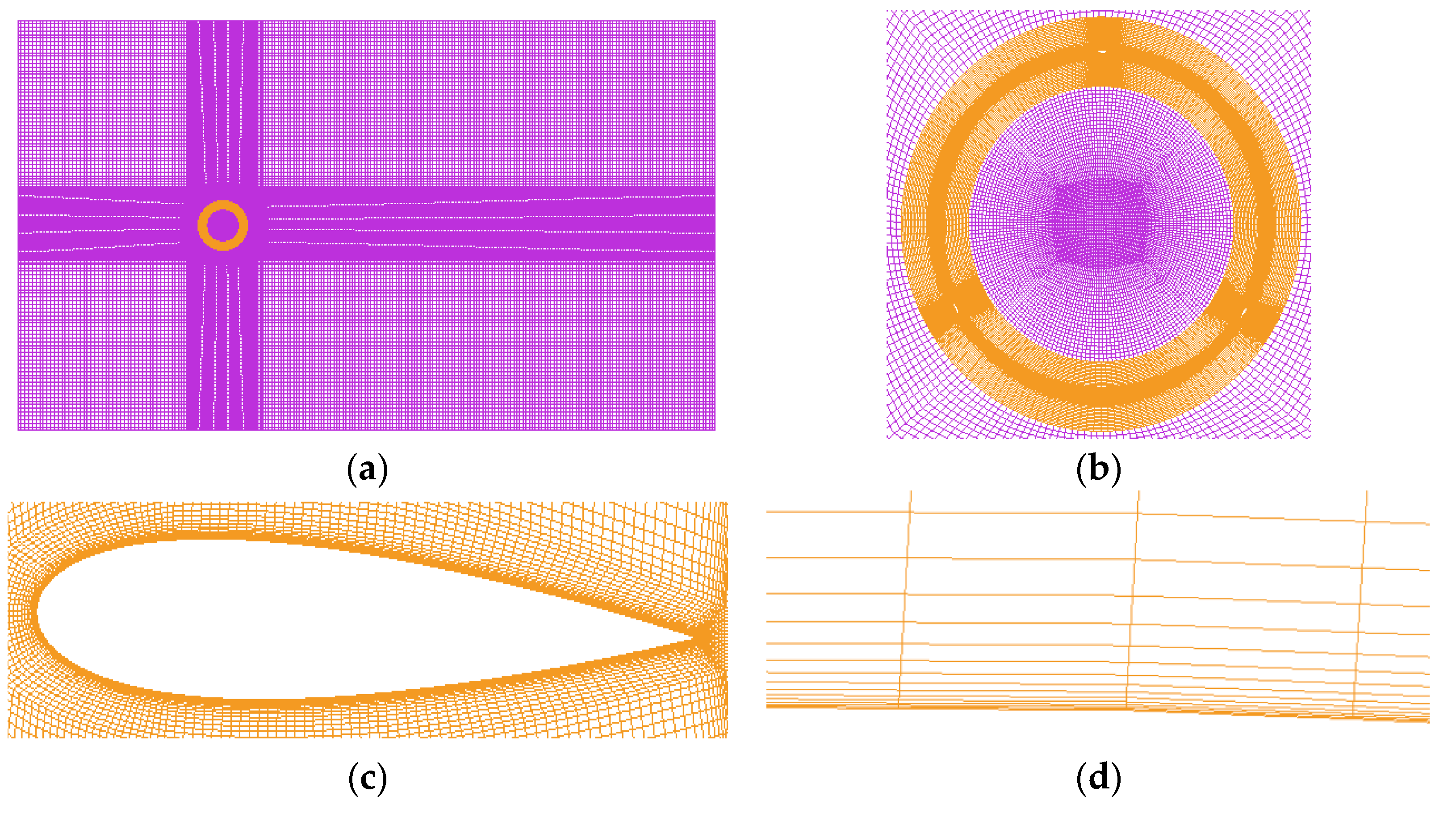
2.3. Grid Division

The size of the computational domain and grid configuration were determined based on prior research [31,33]. The key grid features are summarized in

Table 2. Grid features.

Parameter	Value
Distance from the inlet to the center of wind wheel	5D
Distance from the exit to the center of wind wheel	12D
Distance from wall to the center of wind wheel	5D
Height of stationary domain	10 h
Inner radius of rotating domain	0.8R
Outer radius of rotating domain	1.2R
Height of first layer grid (m)	2 × 10−5
growth rate	1.05
$y^{+}$	<1
Number of airfoil nodes	200

, and the grid division is illustrated in Figure 3. The computational domain is divided into a stationary domain and a rotating domain. Figure 3a shows an overall schematic of the grid division, while Figure 3b shows the grid at the interface. Figure 3c shows that O-type segmentation is employed near the wall to maintain grid orthogonality. To ensure y⁺ < 1, the boundary grid includes 30 layers near the wall, with the first layer grid height controlled at 2 × 10⁻⁵ m and a growth rate of 1.05. The boundary layer grid is shown in Figure 3d. The boundary conditions are set to facilitate data transfer between the two computational domains, as shown in Figure 4.

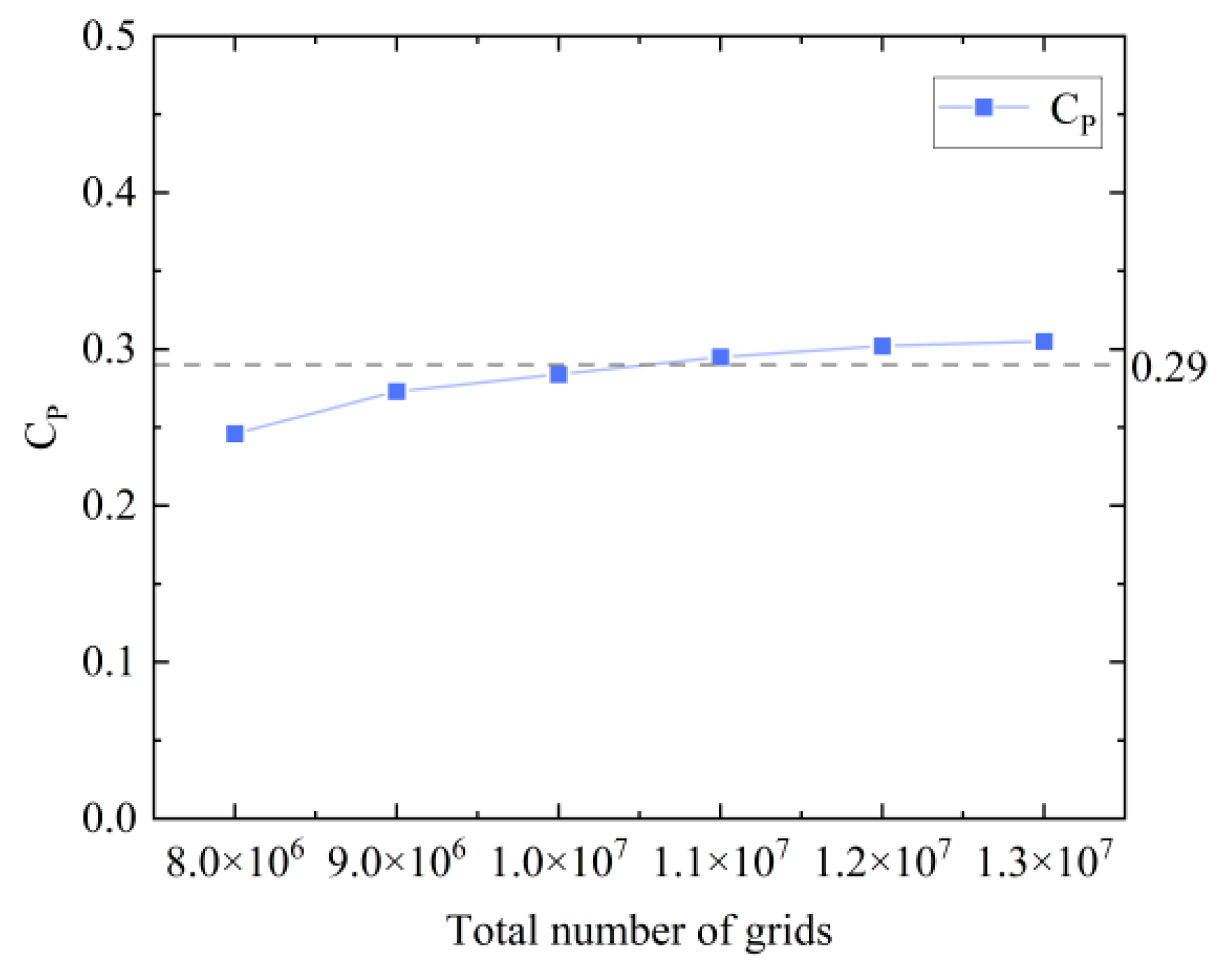
2.4. Result Verification

In this research, the ANSYS FLUENT software 15.0 is used to solve the governing equations. The second-order upwind scheme is employed for the spatial discretization of both the URANS and turbulence equations. Temporal discretization is achieved through a bounded second-order implicit scheme, which guarantees high simulation accuracy and theoretical unconditional stability for the time step. The pressure-based coupled approach is utilized, directly solving the Navier–Stokes equations using pressure within the implicit discrete momentum equation. This coupled algorithm is preferred over pre-corrector–corrector systems like the SIMPLE algorithms due to its superior robustness and convergence, particularly for large time steps or grids of poor quality. This method is chosen for its effectiveness in pressure–velocity coupling and its reduced sensitivity to time discretization [9]. Based on previous studies, the time step is set to 720 steps per revolution with 20 internal iterations per step [24]. This setup corresponds to a 0.5° azimuthal increment of blade rotation for each time step.

To verify the reliability of the numerical calculations, the power coefficient $C_P$ was computed under different tip speed ratios ($\lambda$) and rated rotational speeds by varying the incoming wind speed ($U_0$). The working condition settings are detailed in

Table 3. Turbulence model and algorithm accuracy verification condition setting.

Tip Speed Ratio λ	Wind Speed U0 (m/s)
2.5	16.0
3.0	13.3
3.3	12.1
3.5	11.4
4.0	10.0

. The errors between these calculated values and experimental results were compared as shown in Figure 5. The error between the calculated results and the experimental data [19] is less than 10%, indicating that the calculation method is accurate.

To verify the accuracy of the turbulence model and computational grid, experimental results were used as a reference [19]. The grid independence of the numerical calculations was assessed under the conditions of $\lambda =3.3$, a rotational speed ($n$) of 127 rpm, and an incoming wind speed of 12 m/s. Since the grid size near the blade significantly impacts the accuracy of the results, particular attention was given to the number of grid nodes near the airfoil during the grid independence verification [34]. The results of the grid independent validation are shown in Figure 6. To balance accuracy and computational efficiency, this study employs 200 nodes on the airfoil surface and 30 boundary layers; the total number of grids is $1.2\times {10}^7$.

3. Results and Discussion

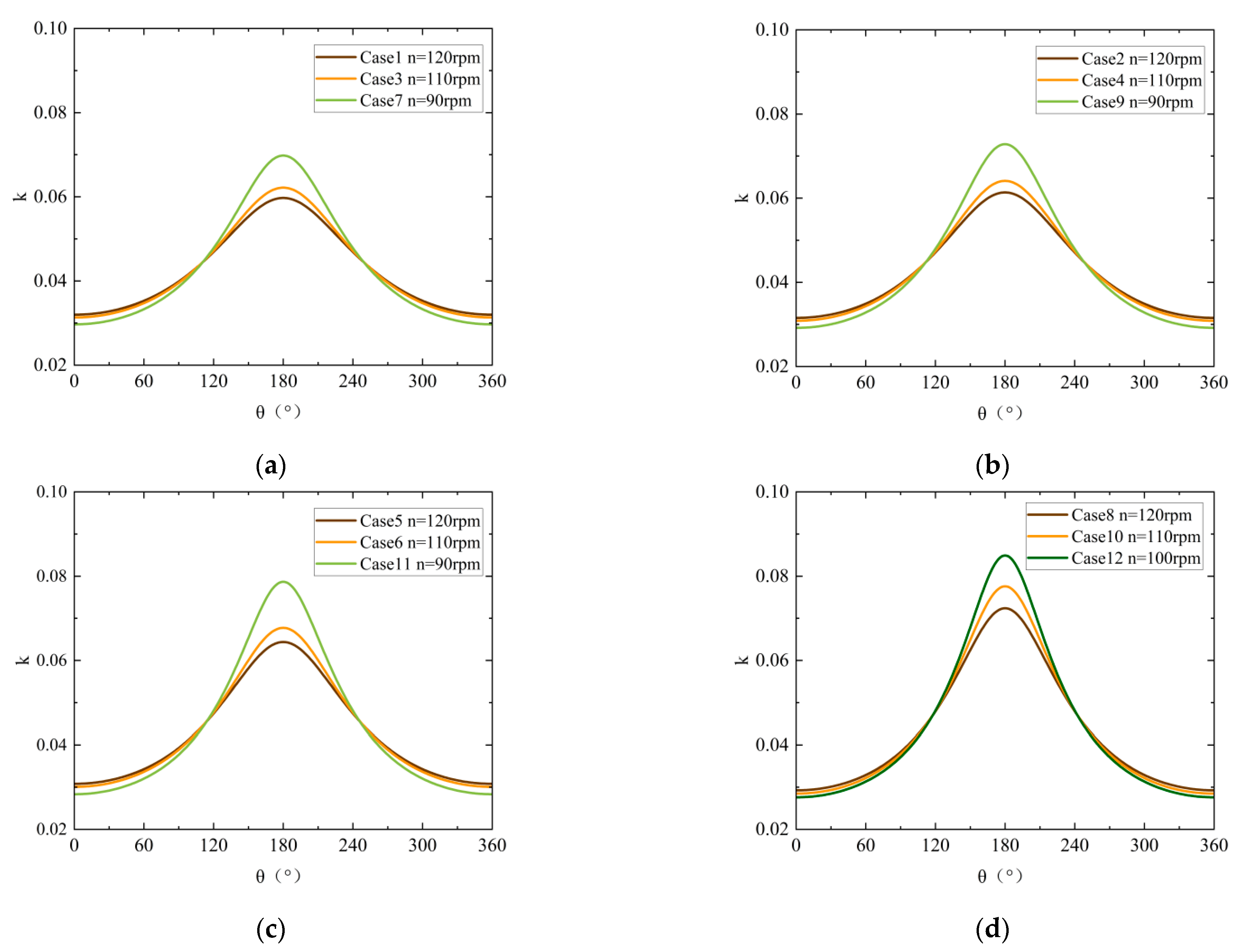
3.1. Variations of Reduced Frequency k during H-VAWT Operation

The tip speed ratio $\lambda$ is defined as:

$$\lambda ={\displaystyle \frac{\Omega R}{U_0}}$$

(1)

The velocity decomposition is conducted as the triangle shown in Figure 2, in which the $\alpha$ and the resultant velocity $W$ at different azimuths can be calculated using the following expressions:

$$\alpha =\phi +\beta ={tan}^{-1}\left({\displaystyle \frac{sin \theta }{cos \theta +\lambda }}\right)+\beta$$

(2)

$$W=U_0\sqrt{{sin}^2\theta +{(cos \theta +\lambda )}^2}$$

(3)

The reduced frequency ($k$) significantly affects the aerodynamic performance of the airfoil during pitch motion. This parameter is typically used to characterize the degree of unsteadiness and describe unsteady aerodynamic problems [35]:

$$k={\displaystyle \frac{\Omega c}{2W}}={\displaystyle \frac{c}{2\sqrt{{sin}^2\theta +{\left(cos \theta +\lambda \right)}^2}}}{\displaystyle \frac{\Omega }{U_0}}$$

(4)

where the relationship between angular velocity $\Omega$ and rotating speed $n$ is:

$$\Omega ={\displaystyle \frac{2\pi n}{60}}$$

(5)

To investigate the influence of $k$, the angle of attack ($\alpha$) and the azimuth angle of the resultant velocity ($\theta$) can be maintained constant by keeping the incoming wind speed $U_0$ and tip speed ratio $\lambda$ fixed. Different values of $k$ can be achieved by varying the rotational speed n. The settings for the case study, including the average value of $k$ ($k_{ave})$ and its fluctuation range within an operational cycle ($k_f)$, are detailed in

Table 4. Settings of different case studies.

Case	Tip Speed Ratio λ	Wind Speed U0 (m/s)	Rotating Speed n (rpm)	Reduced Frequency k
Case 1	3.3	11.4	120	0.046 ± 0.014
Case 2	3.1	12.1	120	0.046 ± 0.015
Case 3	3.0	11.4	110	0.047 ± 0.015
Case 4	2.9	12.1	110	0.047 ± 0.017
Case 5	2.8	13.3	120	0.048 ± 0.017
Case 6	2.6	13.3	110	0.049 ± 0.019
Case 7	2.5	11.4	90	0.050 ± 0.020
Case 8	2.4	16.0	120	0.051 ± 0.022
Case 9	2.3	12.1	90	0.051 ± 0.022
Case 10	2.2	16.0	110	0.053 ± 0.025
Case 11	2.1	13.3	90	0.054 ± 0.025
Case 12	2.0	16.0	100	0.056 ± 0.029

. The wind speeds were set from the verified operating conditions in Table 3, and the rotational speeds were set based on the range of speeds common to H-VAWTs.

The variation of $k$ with $\theta$ is illustrated in Figure 7, and the correlation between each parameter is shown in Figure 8. Under constant rotational speed $n$ and varying incoming wind speed $U_0$, when $\theta =0°$, the blade tip speed $\Omega R$ is opposite to the direction of $U_0$, resulting in the minimum relative wind speed $W$ and the minimum value of $k$:$k_{min}$. Conversely, when $\theta =180°$, the blade tip speed $\Omega R$ aligns with the direction of $U_0$, resulting in the maximum $W$ and the maximum value of k: $k_{max}$. Under the same $n$ and different values of $U_0$, $k_{max}$, and $k_{ave}$, the fluctuations in $k$ increase with $U_0$, while $k_{min}$ shows the opposite trend. According to Figure 8, the correlation between $k_{ave}$ and $U_0$ is 0.72, whereas its correlation with $n$ is −0.58. This indicates that as $n$ increases or $U_0$ decreases, the incoming flow struggles to enter the wind rotor, leading to a more stable flow field inside the wind rotor and a corresponding decrease in $k$.

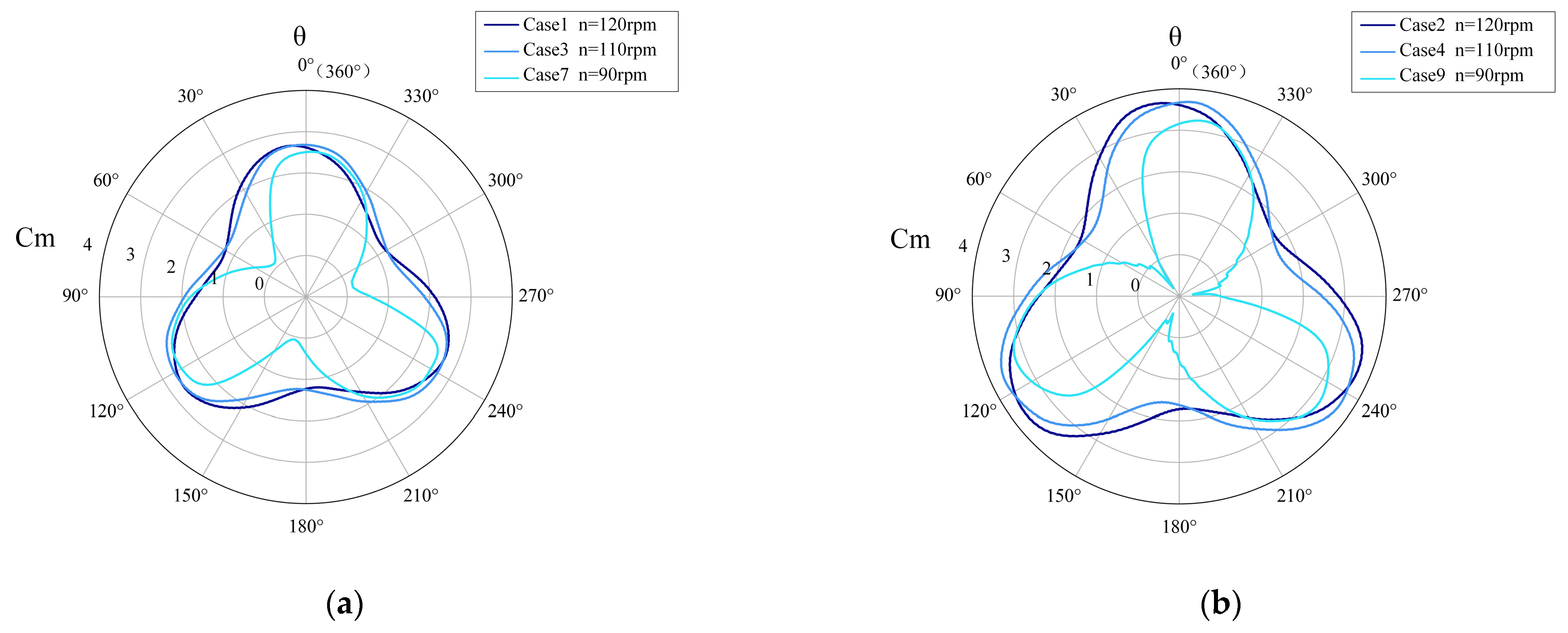
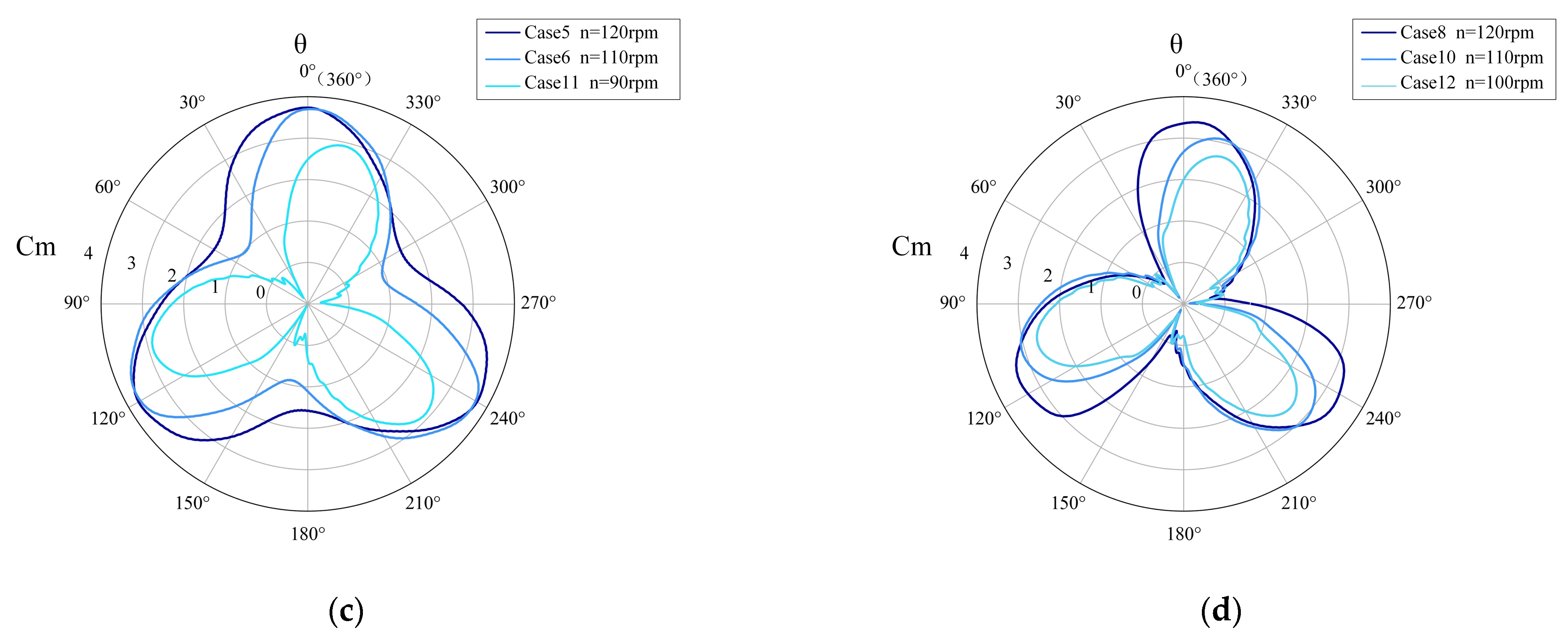
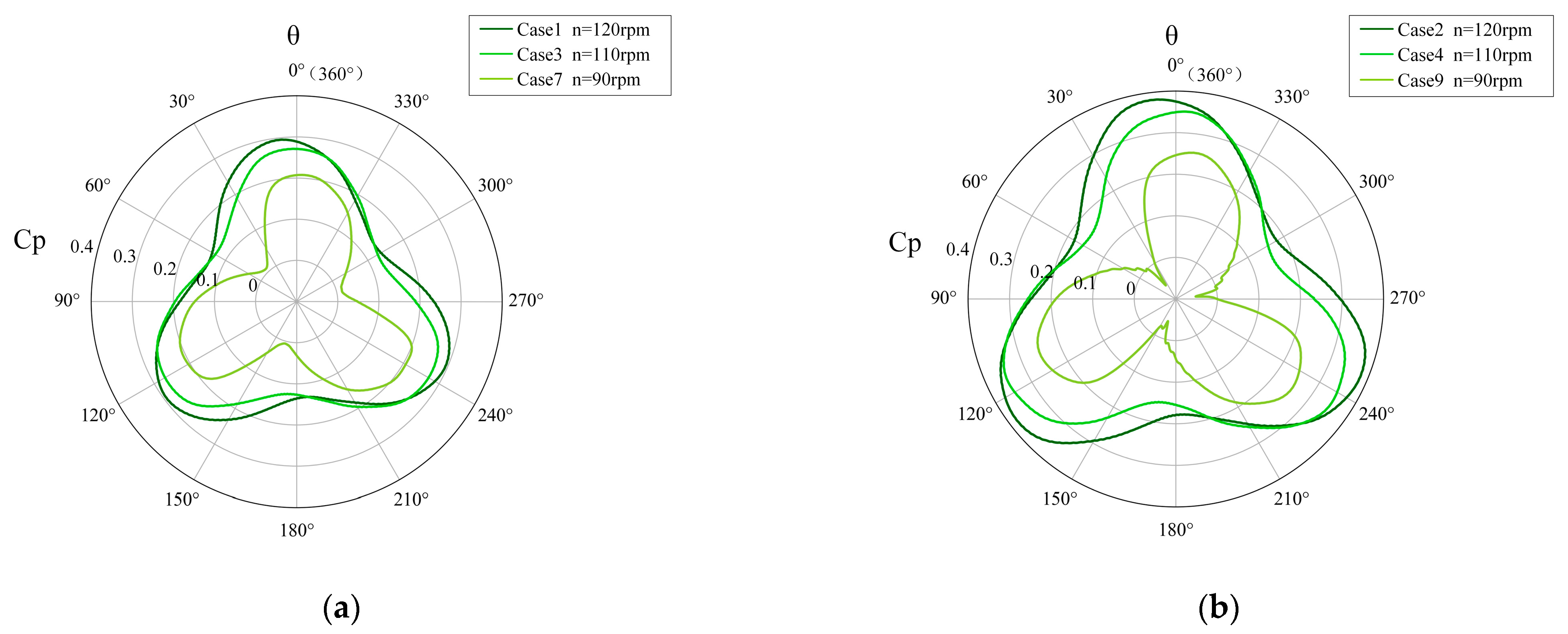
3.2. Effect of Reduced Frequency k on H-VAWT Performance

H-VAWT performance is evaluated by the rotation moment coefficient ($C_m$) and wind energy utilization factor ($C_P$), which are defined as follows:

$$M={\displaystyle \frac{1}{2\pi }}{\int }_0^{2\pi }{F}_{T}Rd\theta$$

(6)

$$C_m={\displaystyle \frac{M}{0.5\rho Dh{U_0}^2}}$$

(7)

$$C_P={\displaystyle \frac{\Omega M}{0.5\rho Dh{U_0}^3}}$$

(8)

where $F_{T}R$ represents the instantaneous torque at a given azimuth angle $\theta$. The wind wheel torque $M$ denotes the average value of $F_{T}R$ over a single rotation. The numerator $\Omega M$ represents the power of H-VAWT, while the denominator refers to the kinetic energy of the wind passing through the wind wheel.

Combining with Equation (4), the relationship between $k$, $C_m$, and $C_P$ can be expressed in the form below:

$$C_m=8k{\displaystyle \frac{MW}{\rho \Omega ch{D}^2{U_0}^2}}$$

(9)

$$C_p=4k{\displaystyle \frac{MW}{\rho chD{U_0}^3}}$$

(10)

Figure 9 shows the variation of the $C_m$ with $\theta$. Under a constant rotational speed $n$, the overall value of $C_m$ increases as the reduced frequency $k$ rises. Specifically, for every 1% increase of $k$, the maximum value of $C_M$ increases by up to 22.39%. However, the rate of increase slows as $k$ continues to rise. This deacceleration may be attributed to increased airflow disturbance losses caused by greater interaction between the blade and the airflow at higher $k$ values, which diminishes the growth rate of the maximum $C_m$. Conversely, the minimum value of $C_m$ increases by approximately 25.22% for every 1% increase in $k$. When $k_{ave}>0.05$, a decrease in $k$ results in a reduction in the minimum $C_m$. This correlation suggests that higher $k$ may enhance momentum transfer and aerodynamic effects, leading to improved performance in terms of torque generation.

The variation of $C_m$ precedes the changes in the reduced frequency $k$. Figure 9 indicates that the maximum value of $C_m$ is obtained when the H-VAWT operates near 0°, 120°, and 240°. The $k_{min}$ is reached when the H-VAWT operates at 0° (or 360°). During the operational cycle, the angle at which the maximum $C_m$ occurs precedes by about 16%. The minimum value of $C_m$ is obtained when the H-VAWT runs near 60°,180°, and 300°. The $k_{max}$ is obtained when the H-VAWT runs at 180°. During the operational cycle, the angle at which the minimum $C_m$ occurs precedes by about 15%. Under the same rotational speed $n$, higher values of $k_{ave}$ cause the extreme values of $C_m$ to occur earlier in the cycle. The complex changes in the flow field during the rotation of the blades introduce a lag effect on the lift and torque generated by the blades. That means that the variation in $k$ first influences the flow field, which then manifests in the performance changes in the H-VAWT. The detailed changes in the flow field near the blades will be discussed in Section 3.3.

Figure 10 shows the variation of the $C_p$ with $\theta$. The trend of $C_p$ is the same as that of $C_m$. When $k_{ave}<0.05$, the angle at which $C_p$ obtains the extreme value lags behind $k$. However, when $k_{ave}>0.05$, the angle at which $C_p$ obtains the extreme value precedes $k$. For $k_{ave}<0.05$ and at the same rotational speed $n$, $C_P$ shows an increasing trend as $k_{ave}$ and $k_f$ increase. For every 1% increase in $k$, the maximum increase in ${Cp}_{min}$ is 20%, and the maximum increases in ${Cp}_{max}$ are 18%. When $k_{ave}>0.05$ and $n$ remains constant, ${Cp}_{min}$ begins to decline as $k_{ave}$ and $k_f$ increase, and the growth rate of ${Cp}_{max}$ slows down. $C_m$ exhibits significant changes during the startup phase when $k_{ave}>0.05$, which in turn affects $C_P$. In the startup phase, the rapidly changing blade angle may cause flow separation, forming vortices and turbulence. These unstable flow field structures significantly impact lift and torque.

Figure 11 shows the correlation between $k$, $C_m$ and $C_P$. As shown in Table 4, for each operating condition, $k_{min}<0.05$, so $k_{min}$ shows a strong positive correlation with the $C_m$, and $C_P$. As $k_{ave}$ and $k_f$ increase, the instability of the flow field becomes larger, resulting in a negative growth phenomenon of the performance indexes $C_m$ and $C_P$.

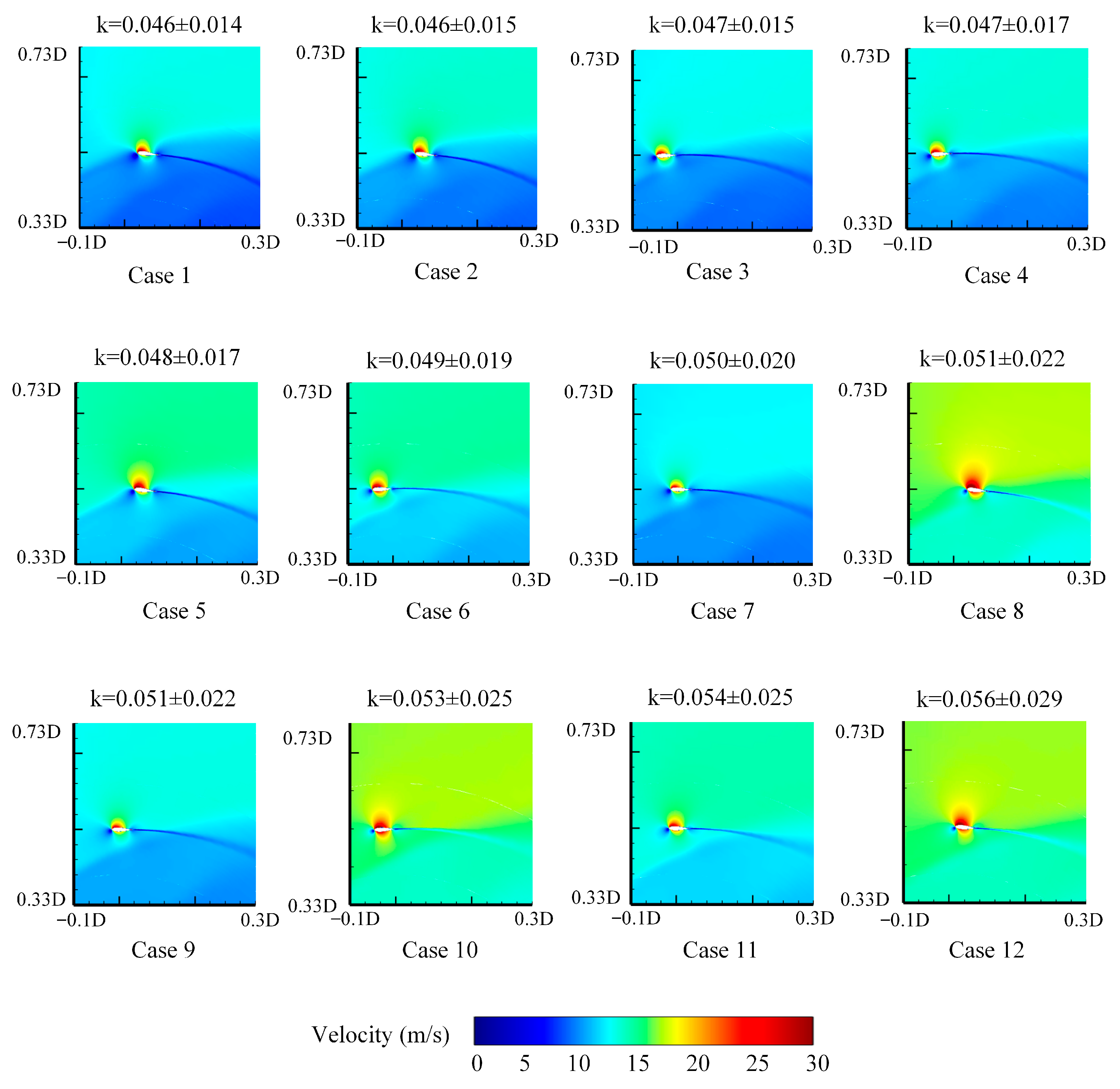
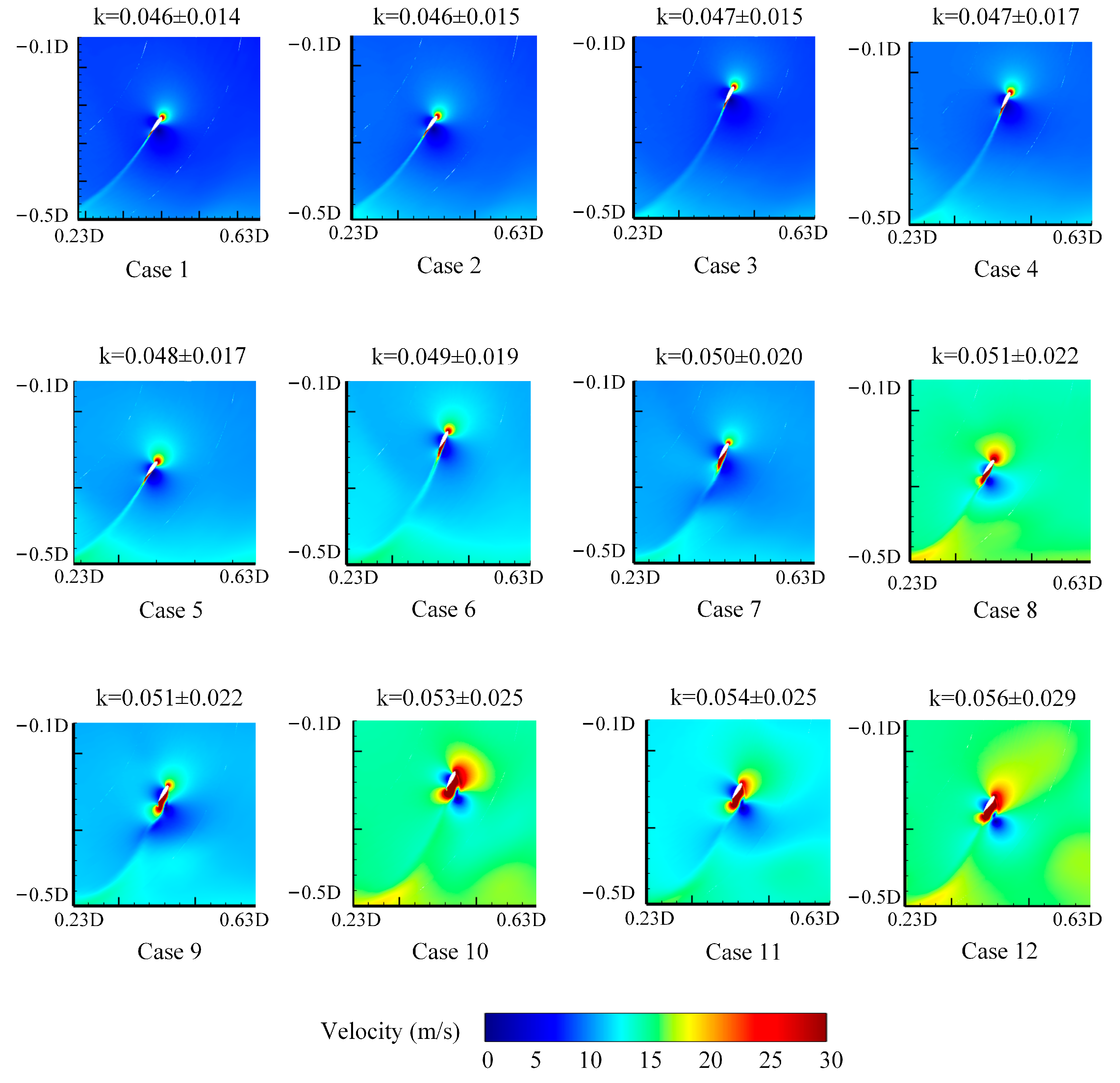
3.3. Effect of Reduced Frequency k on the Flow Field near the Blade

To investigate the H-VAWT aerodynamic performance, this section examines the changes in the flow field within the wind wheel influenced by the average reduced frequency $k_{ave}$, revealing the interaction between the flow field near the blades and the overall performance of the H-VAWT. To avoid the influence of tip vortices, the analysis in this study was conducted on the Z = 0 plane.

Figure 12 shows the variations in the flow field pattern around the blade with $k_{ave}$ at the position of $\theta =0°$. It is observed that there is a high-speed area located at 1/10 of the chord length $c$ behind the trailing edge, but the velocity trajectory behind the trailing edge generally exhibits a velocity loss. When $k_{ave}<0.048$, the velocity loss trajectory of the blade’s trailing edge at $\theta =0°$ is longer, and this trajectory intersects with the velocity loss trajectory of the wind rotor. Consequently, the flow field around the blade is significantly affected by the trailing edge velocity loss, and the velocity loss area on the lower surface of the blade extends beyond the airfoil range. As $k_f$ and $k_{ave}$ increase, the velocity loss trajectory of the blade’s trailing edge at $\theta =0°$ becomes shorter. The intersection point of the trailing edge velocity loss trajectory and the wind rotor’s trajectory moves back, eventually separating from the blade. When $k_{ave}>0.050$, the trailing edge velocity loss trajectory within the wind rotor intersects with the velocity loss trajectory at $\theta =0°$, thereby reducing its impact on the flow field around the blade. Therefore, the velocity loss area on the lower surface of the blade gradually shrinks to within the airfoil range.

Figure 13 shows the variation in the flow field around the blade with $k_{ave}$ at $\theta =120°$. Due to inertia, the high-speed area of the velocity trajectory behind the blade’s trailing edge becomes longer compared to the position at $\theta =0°$ and the high-speed area on the upper surface of the blade gradually moves forward toward the leading edge. When $k_{ave}<0.048$, the high-speed trajectory of the blade’s trailing edge at $\theta =120°$ is distinctly elongated, and the high-speed area behind the trailing edge is smaller than the airfoil. A low-speed area appears near the inner region of the wind rotor in the wake, intersecting with the lower surface of the wing, and a low-speed area is also present on the upper surface of the wing. As $k_{ave}$ and $k_f$ increase, the low-speed area on both the upper and lower surfaces of the wing decreases in size. Simultaneously, the high-speed areas at the leading and trailing edges of the airfoil expand and gradually tilt towards the inner side of the wind rotor. When $0.048<k_{ave}<0.050$, velocity dissipation begins to appear on the windward side of the high-speed trajectory of the trailing edge at $\theta =120°$, but this does not affect the stability of the flow field within the wind rotor. The low-speed area in the wake near the inner region of the wind rotor becomes separated from the lower surface of the wing. When $k_{ave}>0.050$ at $\theta =120°$, the high-speed trajectory of the blade’s trailing edge becomes deformed due to velocity dissipation on the windward side, affecting the stability of the flow field within the wind rotor. The high-speed area behind the trailing edge gradually becomes larger than the area of the airfoil. The low-speed area on the lower surface of the wing gradually detaches along the high-speed area in the wake, forming a vortex. The high-speed area at the leading edge of the airfoil moves further towards the inside of the wind rotor.

Figure 14 shows the variation in the flow field around the blade with $k_{ave}$ at $\theta =240°$. When $k_{ave}<0.048$, the high-speed trajectory of the blade’s trailing edge is noticeably elongated, and the high-speed area behind the trailing edge is smaller than the airfoil. There are also low-speed areas on both the upper and lower surfaces of the airfoil. As $k_{ave}$ and $k_f$ increase, the low-speed areas on the upper and lower surfaces of the airfoil decrease in size. Concurrently, the high-speed areas at the leading and trailing edges of the airfoil expand and gradually tilt towards the outer side of the wind rotor. When $0.048<k_{ave}<0.050$, velocity dissipation starts to appear on the windward side of the high-speed trajectory of the blade’s trailing edge at $\theta =240°$. The high-speed areas expand, but the stability of the flow field outside the wind rotor remains unaffected. The low-speed area on the upper surface of the airfoil begins to separate from the airfoil. For $k_{ave}>0.050$, the high-speed trajectory of the blade’s trailing edge at $\theta =240°$ becomes deformed due to velocity dissipation on the windward side, which affects the stability of the flow field both inside and outside the wind rotor. The high-speed area behind the trailing edge gradually becomes larger than the airfoil area. The low-speed area on the upper surface of the airfoil detaches along the high-speed area in the wake, forming a vortex. Additionally, the high-speed area at the leading edge of the airfoil moves towards the outside of the wind rotor.

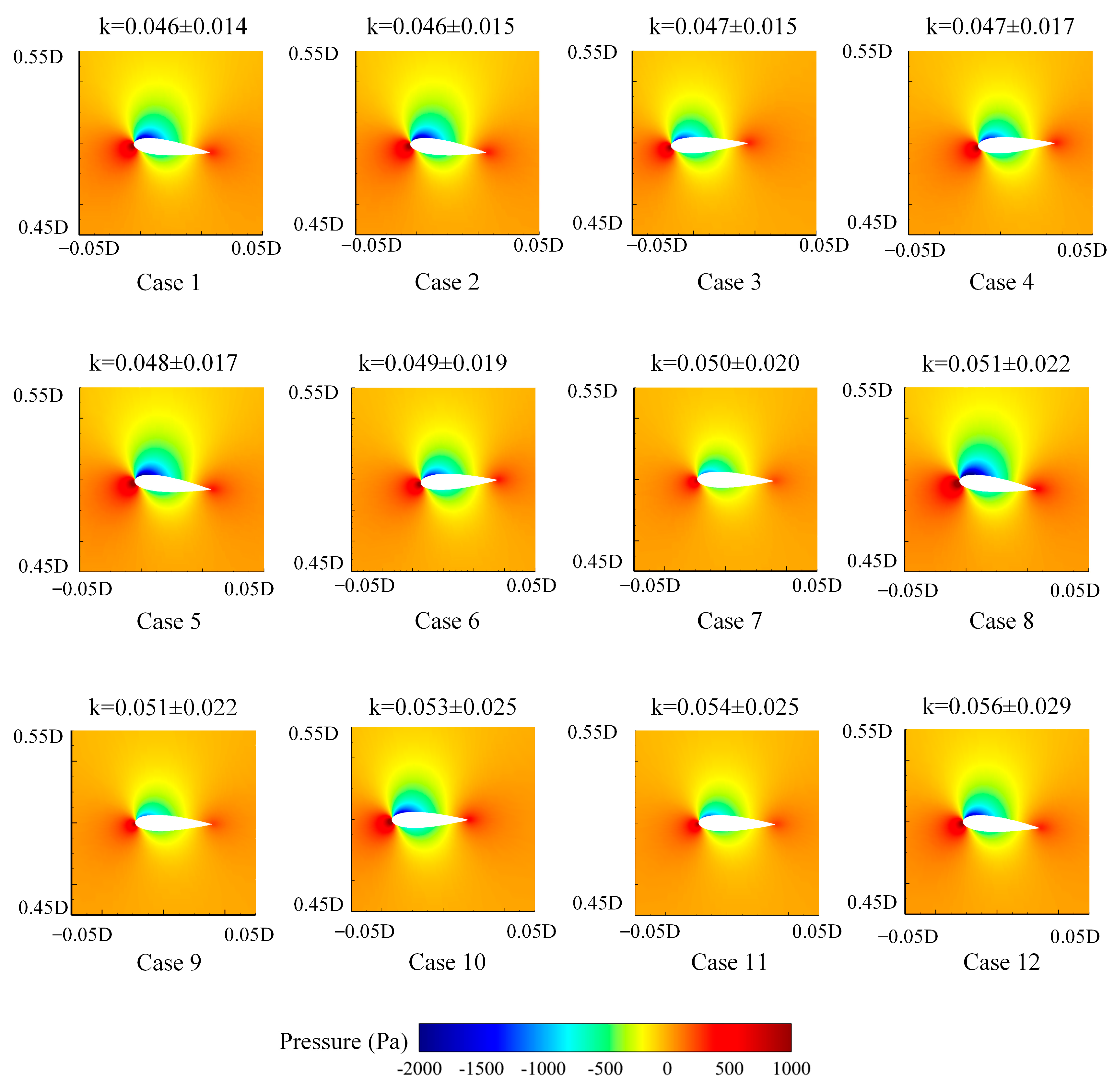
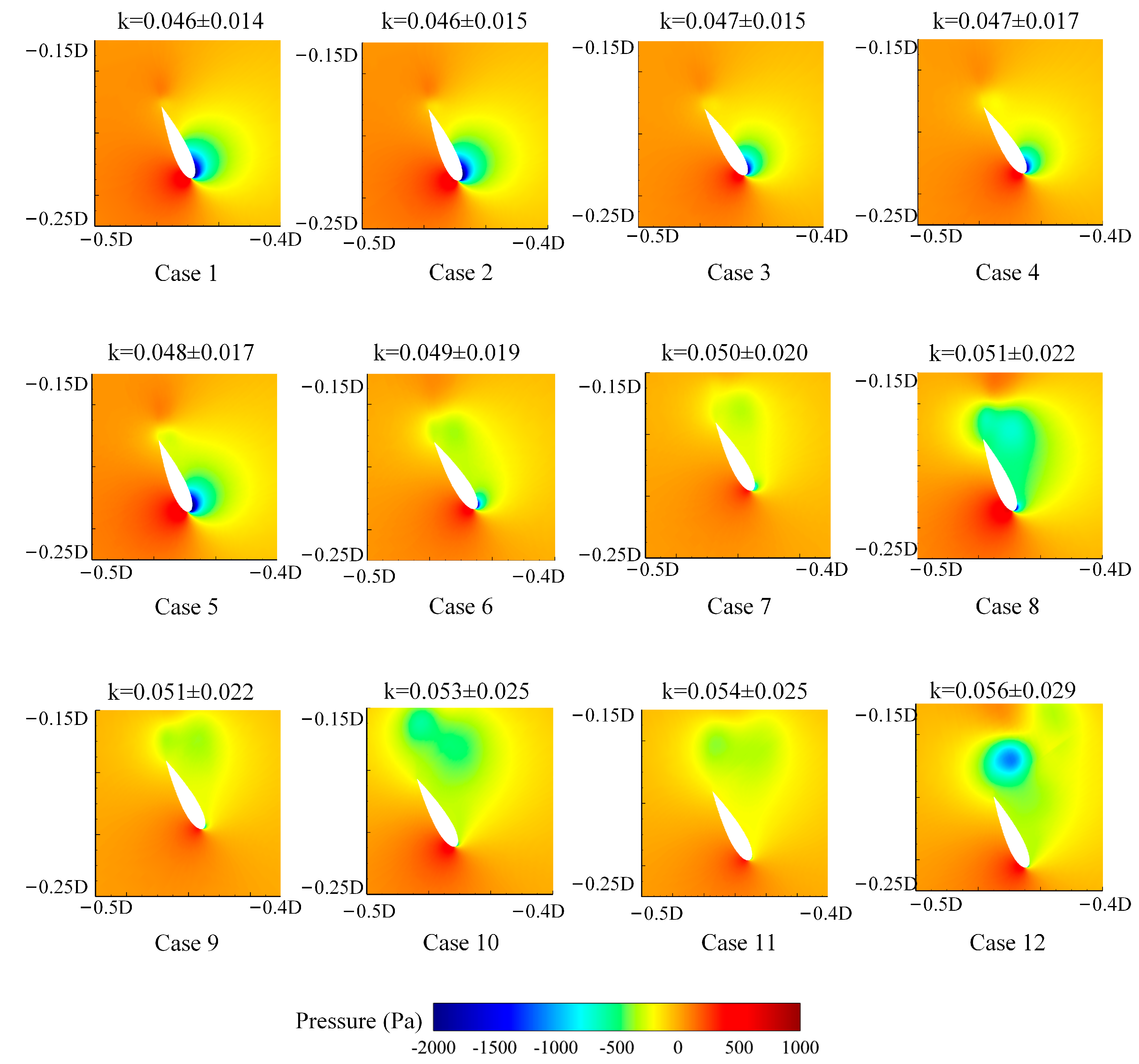
3.4. Effect of Reduced Frequency k on the Pressure around the Blade

To investigate the H-VAWT performance, this section examines the changes in the pressure around the blade influenced by $k_{ave}$. To avoid the influence of tip vortices, the analysis in this study was conducted on the Z = 0 plane.

Figure 15 shows that at $\theta =0°$, the pressure around the blade changes with $k_{ave}$. There is no significant difference in pressure around the blades for all operating conditions. The pressure is higher at the leading and trailing edges of the airfoil, and the area of the low-pressure region is larger on the upper airfoil than on the lower airfoil. The airflow hits the leading edge of the blade directly, resulting in higher pressure in the leading edge area. At the same time, the airflow converges at the trailing edge after passing through the blade surface, forming a larger dynamic pressure region, so the pressure at the trailing edge is also higher. The response of the upper and lower airfoils to the airflow disturbance is more consistent, and the influence on the pressure distribution under different working conditions is small, resulting in insignificant differences in the pressure distribution.

Figure 16 shows that at $\theta =120°$, the pressure around the blade changes with $k_{ave}$. As the H-VAWT rotates, the upper airfoil rotates to the windward side, where it is directly hit by the incoming flow, and the upper airfoil pressure is higher. The lower airfoil pressure becomes significantly smaller. As $k_{ave}$ increases, the low-pressure region expands at the trailing edge. When $k_{ave}>0.050$, the low-pressure area at the trailing edge becomes larger, and low-pressure vortices are gradually developed. Under the influence of higher reduced frequency, the airflow cannot attach to the airfoil surface effectively, and the resulting separation vortex or vortex area becomes significant. These low-pressure vortices not only affect the pressure distribution of the blade, but also lead to additional aerodynamic drag and vibration.

Figure 17 shows that at $\theta =240°$, the pressure around the blade changes with $k_{ave}$. As the H-VAWT rotates, the lower airfoil rotates to the windward side. As a result, the lower airfoil has a distinct high-pressure region, while the upper airfoil develops a low-pressure region. With the increase of $k_{ave}$, the low-pressure region shifts and expands towards the trailing edge. When $k_{ave}>0.050$, the low-pressure area becomes larger at the trailing edge, and the low-pressure vortex develops gradually.

Based on the H-VAWT, some researchers have investigated the boundary layer separation characteristics and performance of the optimized blades in different reduced frequencies [36,37,38,39]. Kiefer et al. [40] found that beyond the critical reduced frequency, neither the load magnitude nor the vortex evolution is related to the reduced frequency but depends on the geometry of the motion and the convective time scale. This indicates that at the same reduced frequency, different blade designs exhibit different performance and flow field evolution than H-VAWTs. However, both the aerodynamic performance and the flow field structure change at critical values. Therefore, some of the conclusions drawn from this investigation may be applicable with other blade shapes.

4. Conclusions

By adjusting $U_0$ and $n$, the $SST k-\omega$ turbulence model was employed to investigate the influence of $k$ on the performance of H-VAWT and explore the interaction between performance and flow field dynamics. The findings indicate that 0.05 is the critical value of $k$.The main achievements can be summarized as follows:

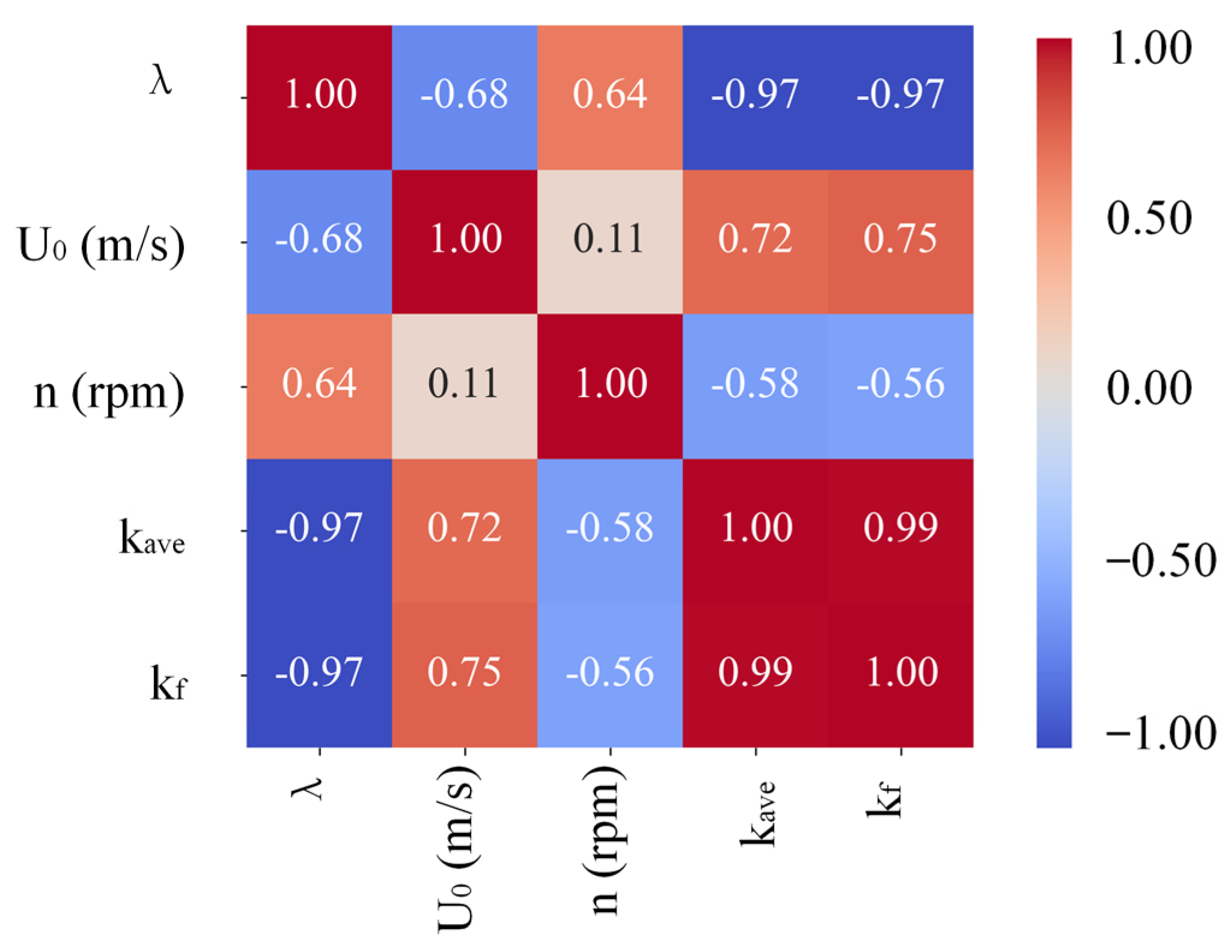
• The variations in $k$: This parameter is affected by both $U_0$ and $n$, with $U_0$ exhibiting a greater impact. As $n$ increases or $U_0$ decreases, the incoming flow struggles to enter the wind rotor, stabilizing the flow field within the rotor and causing $k$ to decline.
• Impact of $k$ on H-VAWT performance: When $k<0.05$, a 1% increase in $k$ can lead to a maximum $C_m$ increase of 22.39%. Conversely, the minimum value of $C_m$ increases by 25.22% for every 1% increase in $k$. As 1% increase in $k$ can maximally increase ${Cp}_{min}$ by 20%, and ${Cp}_{max}$ by 18%. For $k>0.05$, higher $k$ enhances momentum transfer and aerodynamic effects, leading to a decrease in performance.
• Impact of $k$ on the flow field near the blades: The change in the flow field can be visualized as $k_{ave}=0.05$ is the critical value. The stability of the flow field in the vicinity of the blades is weakened with the increase of $k_{ave}$. The structure of the flow field is the same when $k_{ave}$ is the same. When $k_{ave}<0.048$, there is an obvious trajectory of blade trailing edge velocity loss inside the wind turbine, and $U_0$ does not affect the flow field inside the wind turbine; when $0.048<k_{ave}<0.050$, there is still a trajectory of blade trailing edge velocity loss inside the wind turbine, and the velocity of blade trailing edge at the position of airfoil 2 is significantly increased by the influence of $U_0$. When $k_{ave}>0.050$, there is no blade trailing edge velocity loss trajectory inside the wind turbine, and flow separation occurs at airfoil 2 and airfoil 3, and vortices are formed.
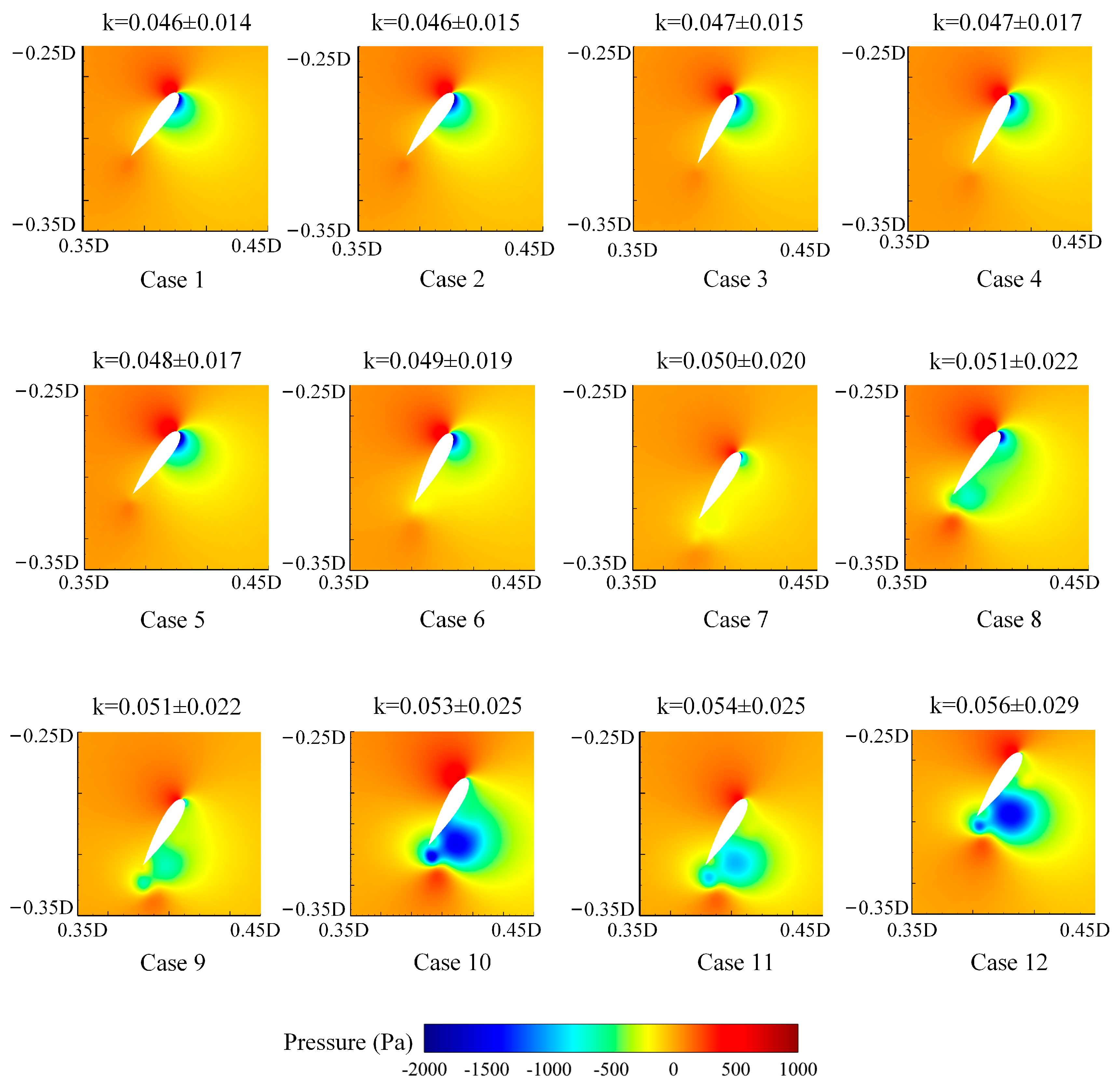
• Impact of $k$ on pressure near the blades: The change in pressure around the blade is not significant at $\theta =0°$. As the H-VAWT rotates, the pressure difference between the upper and lower airfoil surfaces of the blade becomes larger, the airflow disturbance is enhanced, and the pressure begins to change with $k_{ave}$. The pressure is higher at the trailing edge of the blade than at the trailing edge of the blade. As $k_{ave}$ increases, the low-pressure region expands at the trailing edge. When $k_{ave}>0.050$, the low-pressure region becomes significantly larger at the trailing edge, and a low-pressure vortex develops gradually.
• Directions for future work: The current investigation focuses on the effect of $k$ on the performance of H-VAWTs and the flow field near the blades. In the future, the effects of $k$ on the wake field of H-VAWTs and the relationships between the incoming flow and wake will be further investigated. In addition, different blade designs might have altered the conclusions of this investigation. This issue will also be discussed to broaden the application of the investigation.