Numerical Study on Internal Flow and Cavitation Characteristics of GDI Injectors for Different Nozzle Orifice Geometries
Abstract
1. Introduction
2. Numerical Methodology
2.1. Model Setup and Meshing
2.2. Nozzle Structure Setting
3. Results and Discussion
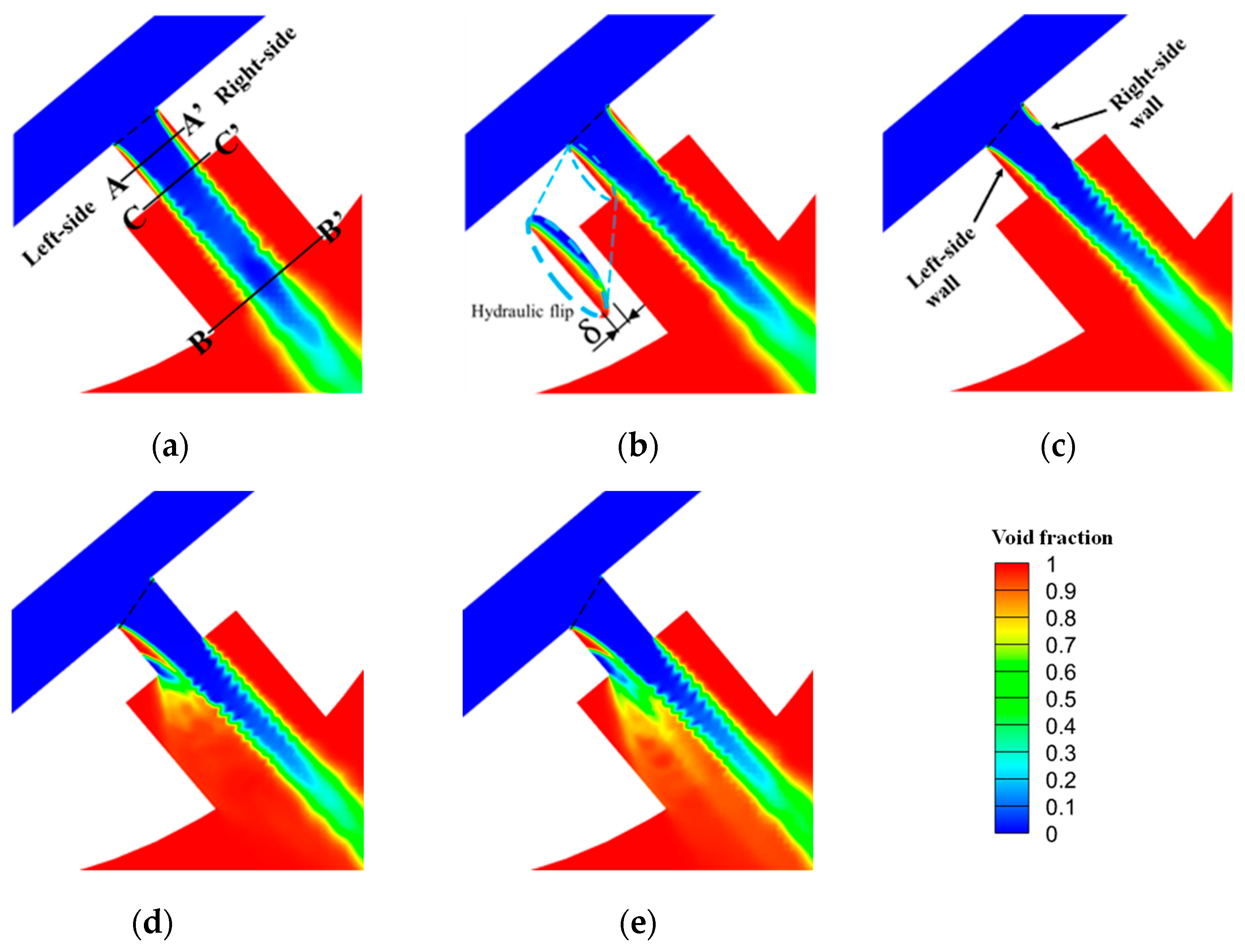
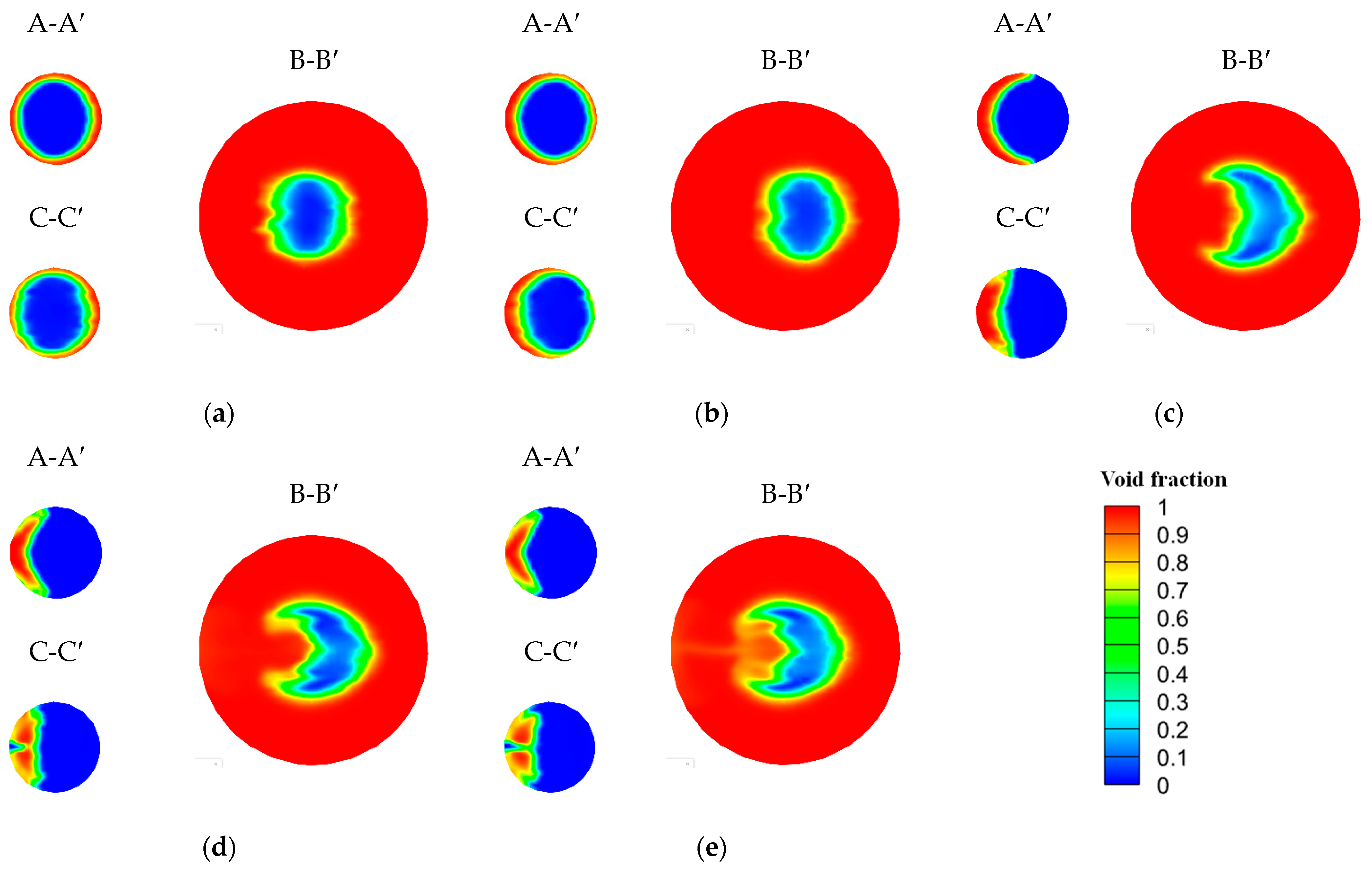
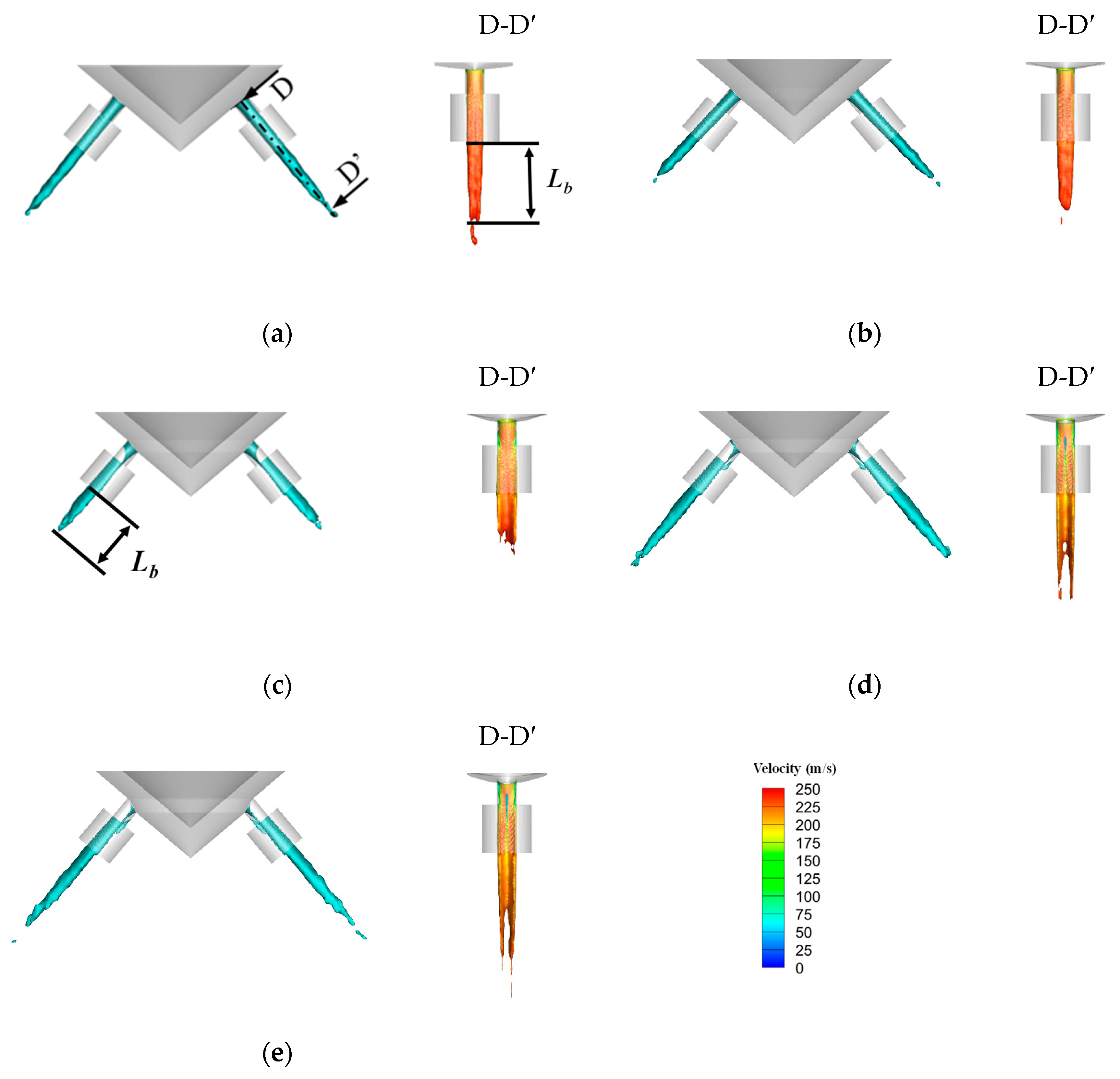
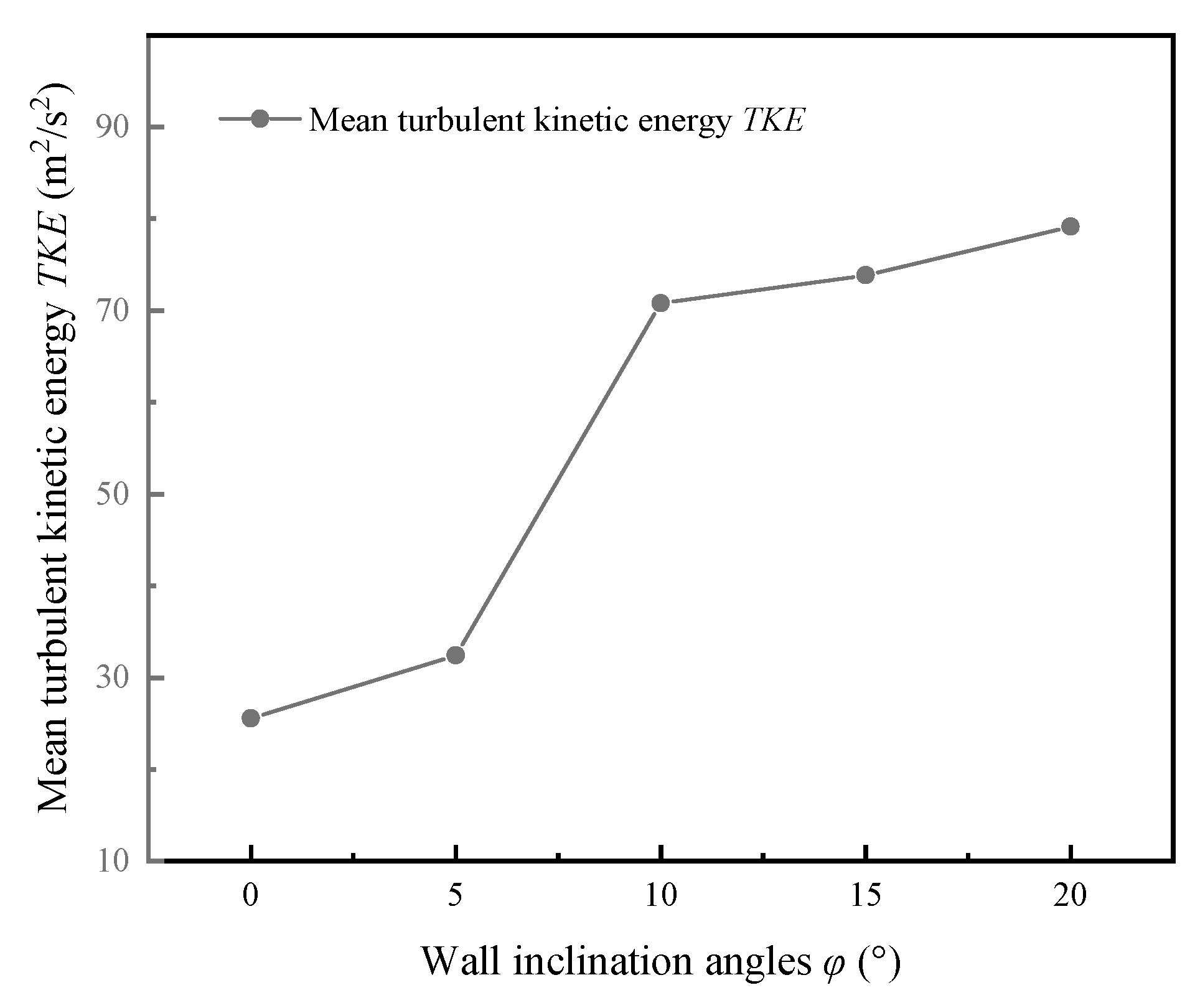
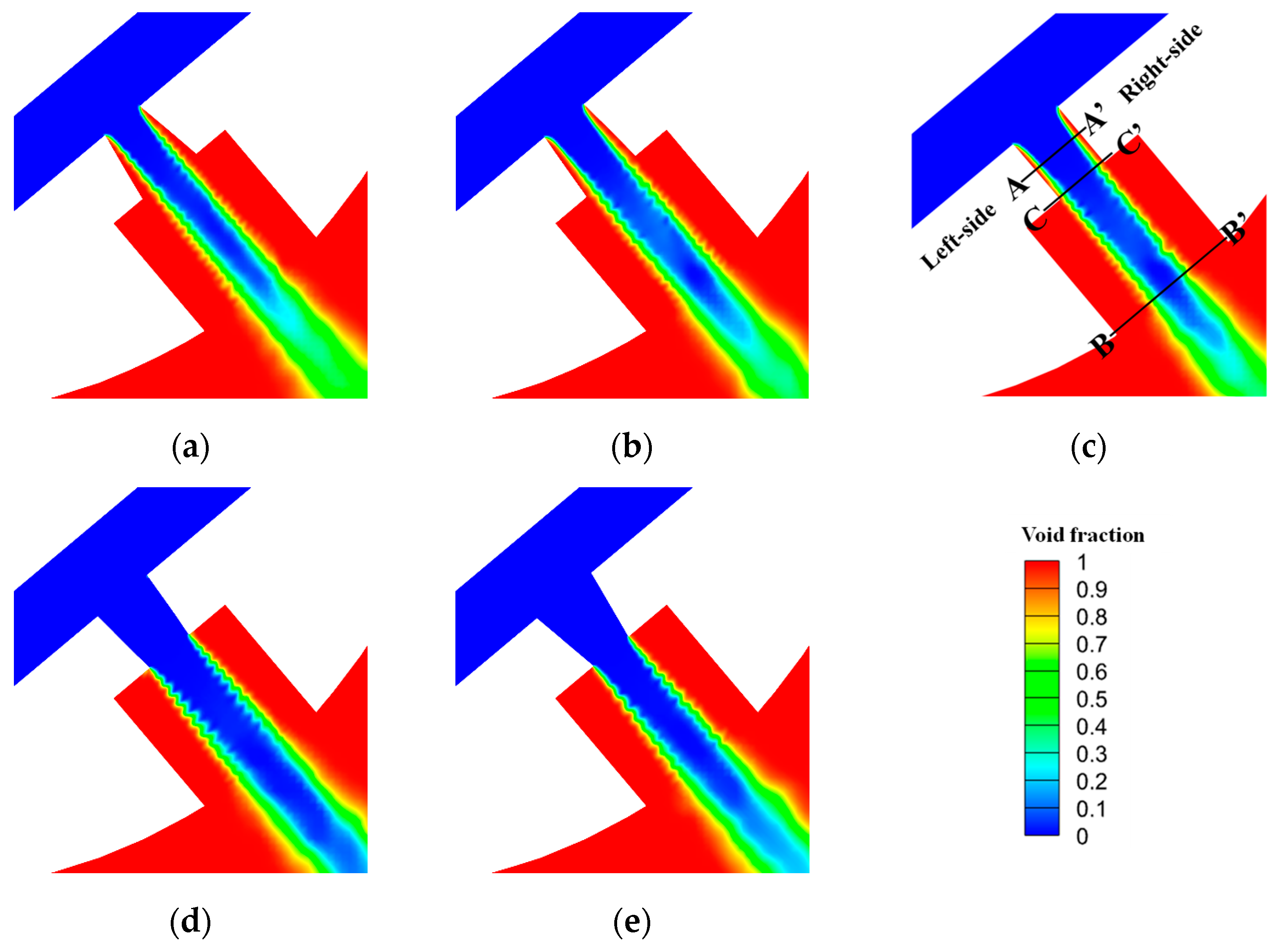
3.1. Influence of the Wall Inclination Angle on Internal Flow and Cavitation Characteristics in Nozzle
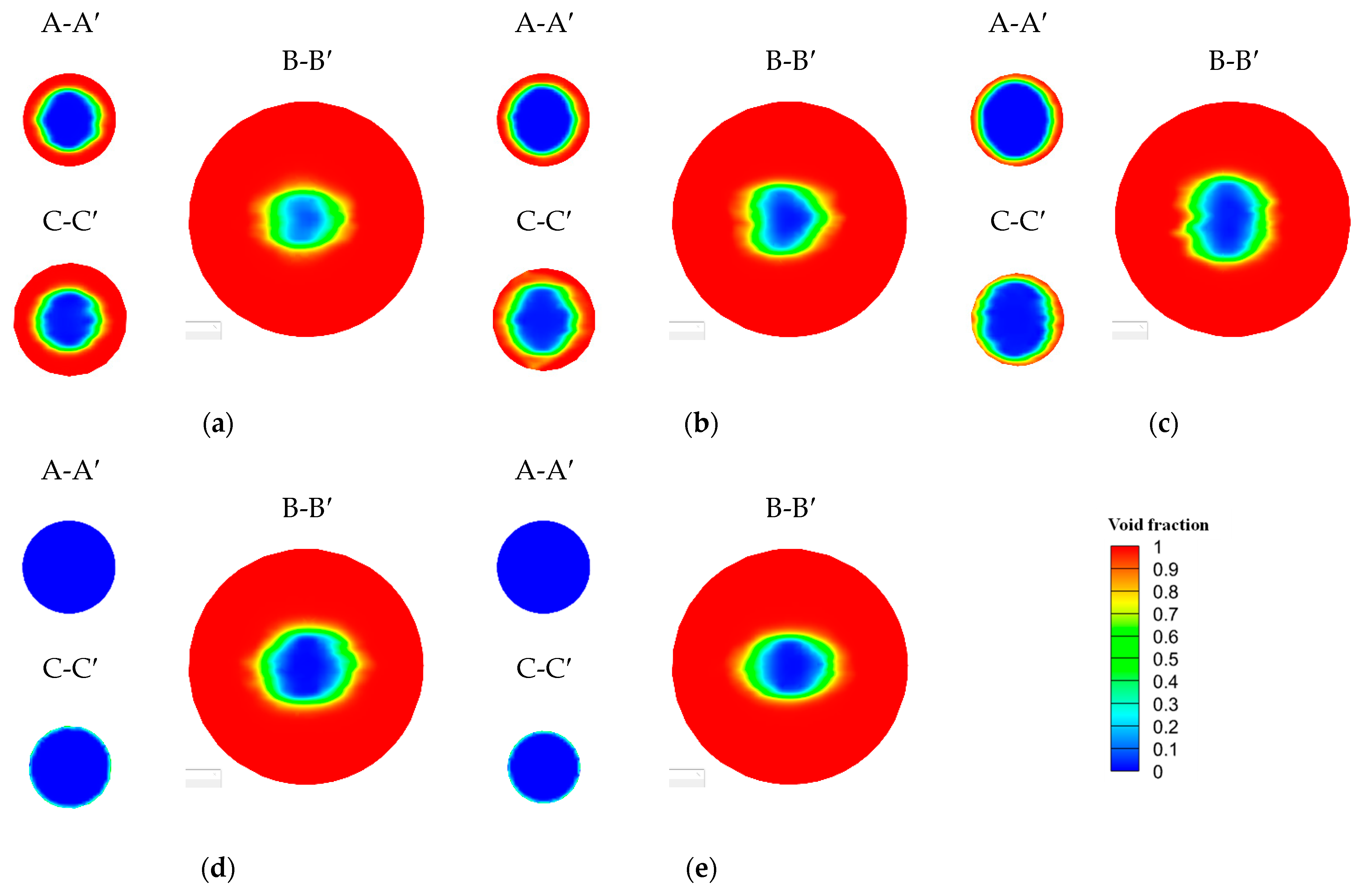
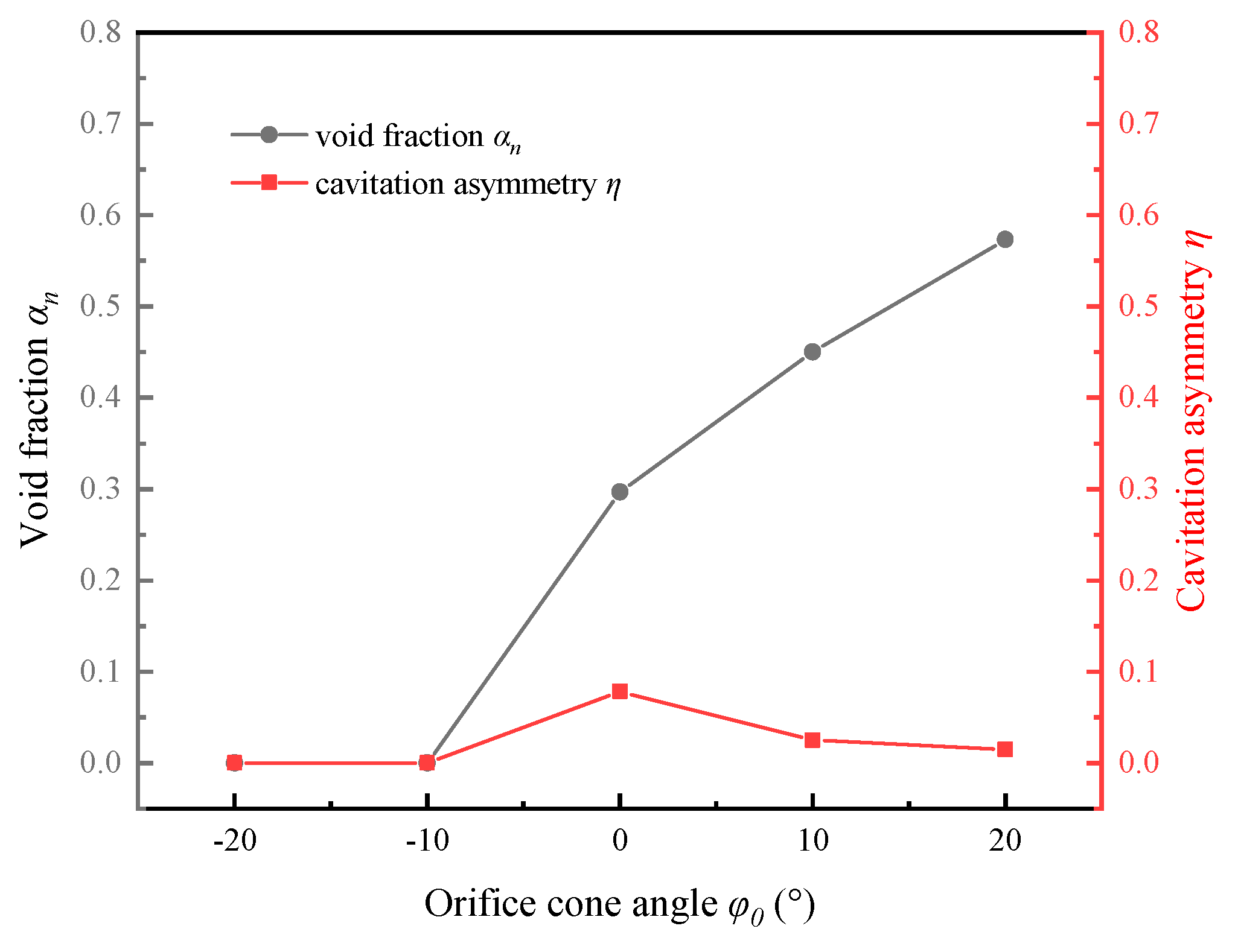
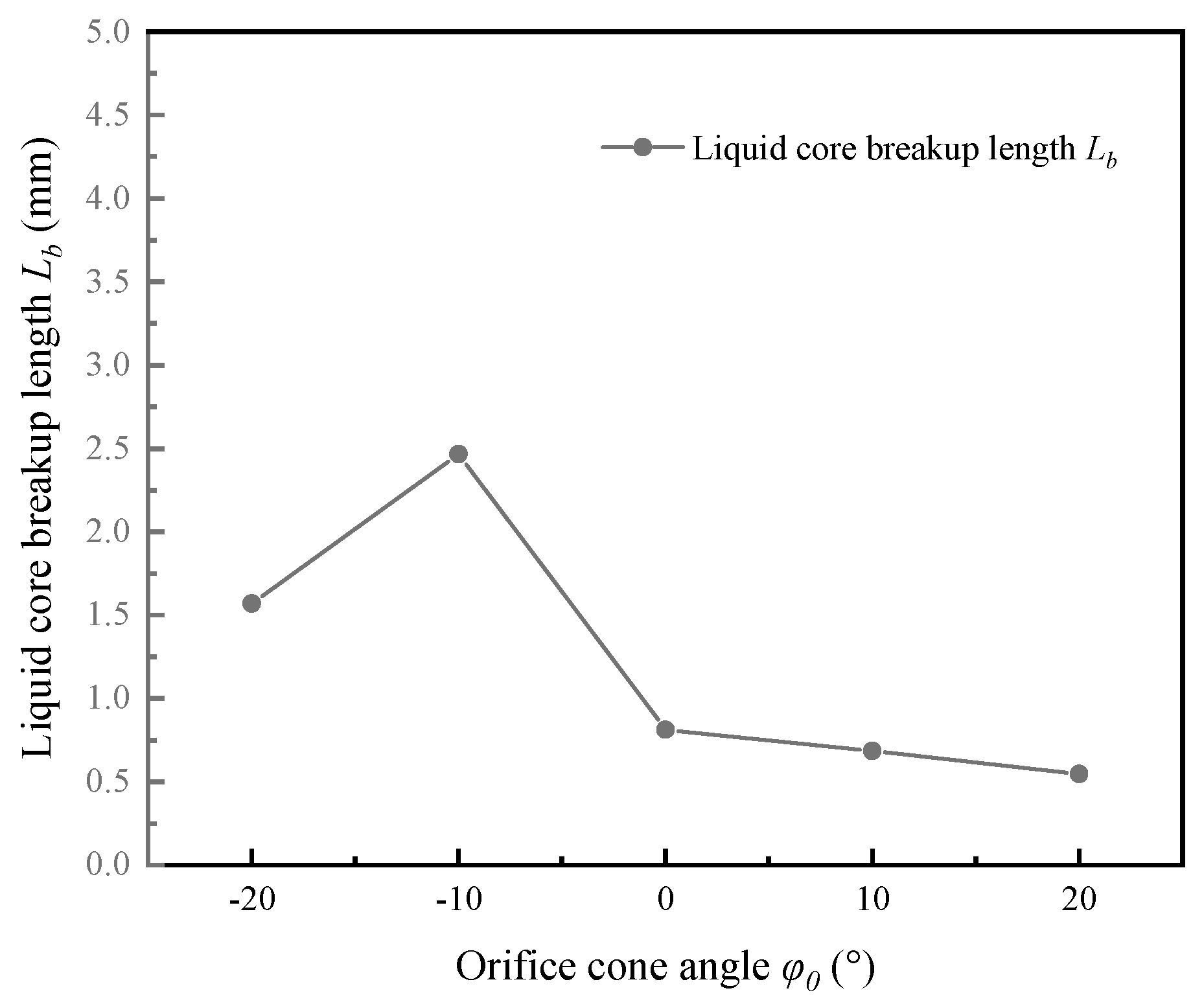
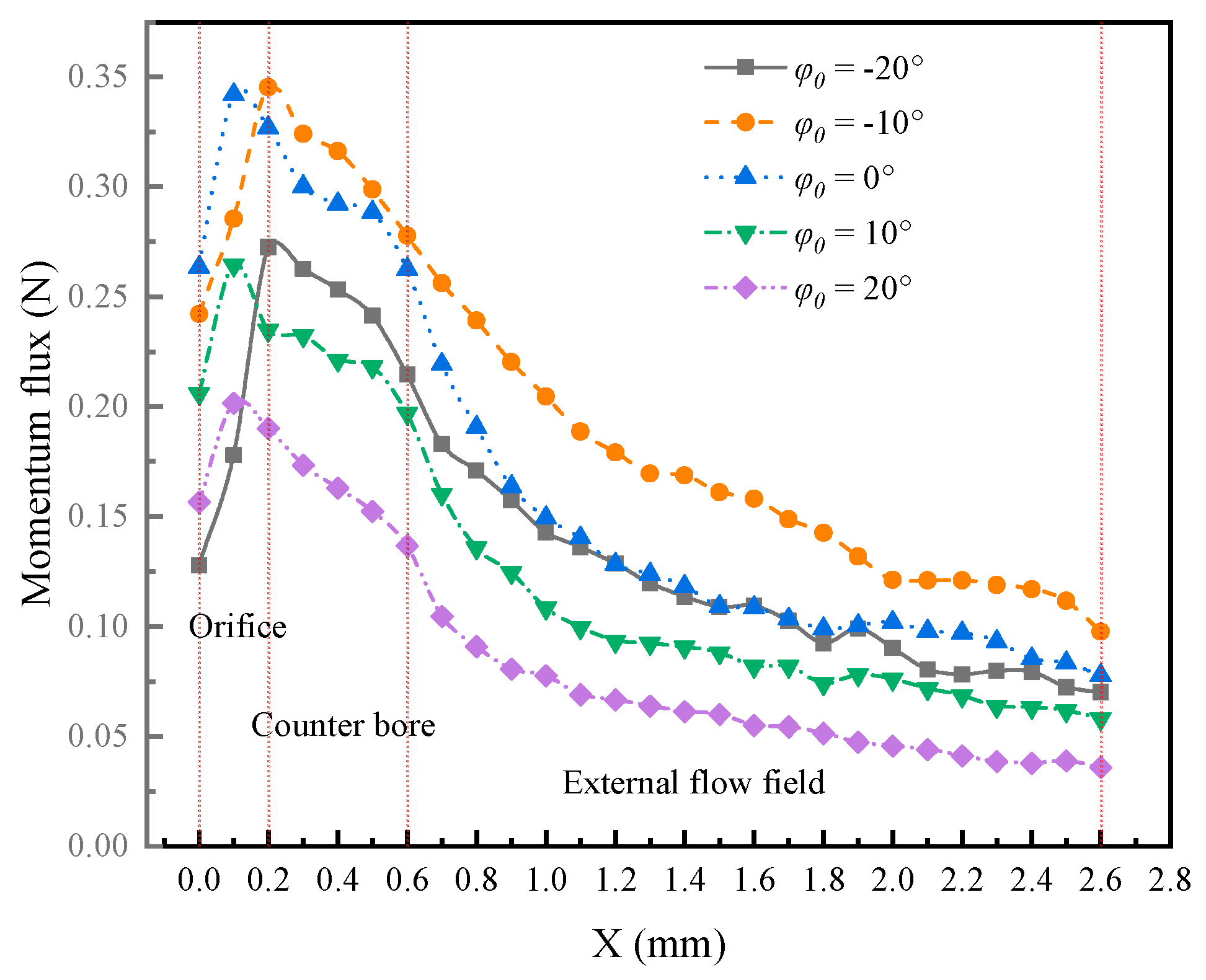
3.2. Influence of Orifice Cone Angle on Internal Flow and Cavitation Characteristics in the Orifice
4. Conclusions
- (1)
- As the wall inclination angle increases, the cavitation asymmetry within the orifice intensifies, accompanied by a decrease in the gas volume fraction. On one side of the injection hole, the occurrence of cavitation is suppressed. On the other side of the injection hole, cavitation is intensified. Furthermore, the width of the hydraulic flip on one side of the orifice expands, while the flow state on the other side of the orifice gradually changes from a hydraulic flip to super-cavitation flow, transitional cavitation and an almost fully reattached flow.
- (2)
- When the wall inclination angle φ exceeds 10°, the cross-sectional profile of the jet at the counter-bore exit undergoes a transition from an ellipse to a crescent. Simultaneously, the shape of the fuel liquid core transforms from cylindrical to hollow semi-circular. Furthermore, as the wall inclination angle reaches 15°, the mass flow rate and penetration of the nozzle become larger.
- (3)
- In comparison to the cylindrical orifice, the presence of a divergent orifice intensifies fuel cavitation and leads to an enlarged hydraulic flip width as the orifice cone angle φ0 increases. Conversely, the convergent orifice configuration mitigates cavitation effects, and the complete absence of the gas region within the orifice is achieved when the orifice cone angle φ0 is below −10°.
- (4)
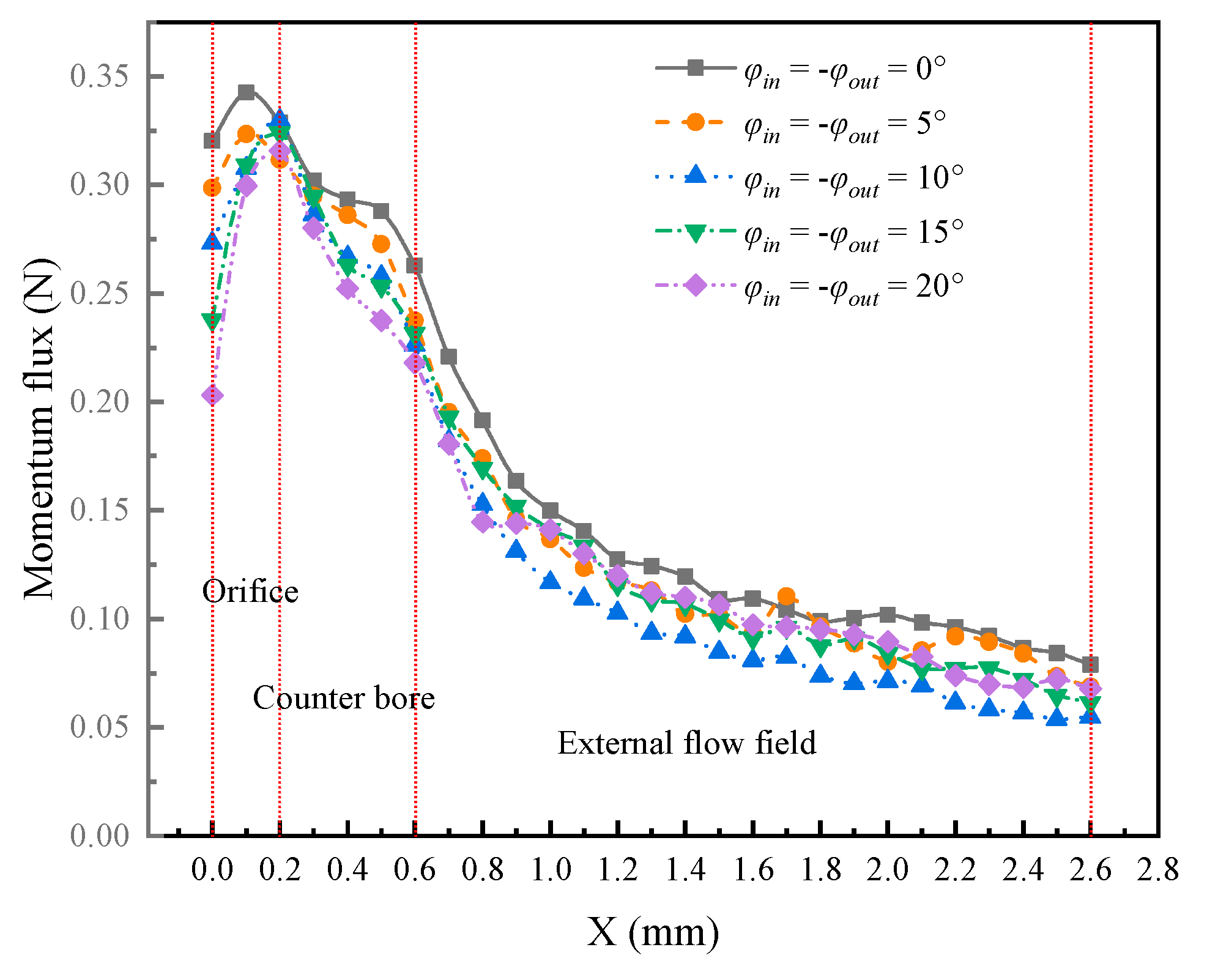
- When the orifice layout changes to a divergent type, the flow coefficient of the orifice reduces, together with a diminution in the fuel momentum flux and the mass flow rate. As the orifice cone angle decreases, the orifice layout becomes convergent, and the flow coefficient and momentum flux increase. The spray performance of a nozzle with an orifice cone angle of −10° is superior in terms of mass flow rate and liquid penetration.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Shah, K.J.; Pan, S.; Lee, I.; Kim, H.; You, Z.; Zheng, J.; Chiang, P. Green transportation for sustainability: Review of current barriers, strategies, and innovative technologies. J. Clean Prod. 2021, 326, 129392. [Google Scholar] [CrossRef]
- Zahoor, A.; Mehr, F.; Mao, G.; Yu, Y.; Sápi, A. The carbon neutrality feasibility of worldwide and in China’s transportation sector by E-car and renewable energy sources before 2060. J. Energy Storage 2023, 61, 106696. [Google Scholar] [CrossRef]
- Ferrari, A.; Novara, C.; Vento, O.; Violante, M.; Zhang, T. A novel fuel injected mass feedback-control for single and multiple injections in direct injection systems for CI engines. Fuel 2023, 334, 126670. [Google Scholar] [CrossRef]
- Ferrari, A.; Novara, C.; Paolucci, E.; Vento, O.; Violante, M.; Zhang, T. Design and rapid prototyping of a closed-loop control strategy of the injected mass for the reduction of CO2, combustion noise and pollutant emissions in diesel engines. Appl. Energy 2018, 232, 358–367. [Google Scholar] [CrossRef]
- Ferrari, A.; Novara, C.; Paolucci, E.; Vento, O.; Violante, M.; Zhang, T. A new closed-loop control of the injected mass for a full exploitation of digital and continuous injection-rate shaping. Energy Convers. Manag. 2018, 177, 629–639. [Google Scholar] [CrossRef]
- Finesso, R.; Marello, O.; Spessa, E. Development of a pressure-based technique to control IMEP and MFB50 in a 3.0 L diesel engine. Energy Procedia 2018, 148, 424–430. [Google Scholar] [CrossRef]
- Ambrosio, S.; Finesso, R.; Hardy, G.; Manelli, A.; Mancarella, A.; Marello, O.; Mittica, A. Model-Based Control of Torque and Nitrogen Oxide Emissions in a Euro VI 3.0 L Diesel Engine through Rapid Prototyping. Energies 2021, 14, 1107. [Google Scholar] [CrossRef]
- Ambrosio, S.; Mancarella, A.; Manelli, A.; Mittica, A.; Hardy, G. Experimental analysis on the effects of multiple injection strategies on pollutant emissions, combustion noise, and fuel consumption in a premixed charge compression ignition engine. Sae Int. J. Engines 2021, 14, 611–630. [Google Scholar]
- Jin, Z.; Vento, O.; Zhang, T.; Ferrari, A.; Mittica, A.; Ouyang, L.; Tan, S. Numerical-experimental optimization of the common-feeding injection system concept for application to light-duty commercial vehicles. J. Energy Resour. Technol. 2021, 143, 122304. [Google Scholar] [CrossRef]
- Mancarella, A.; Marello, O. Effect of coolant temperature on performance and emissions of a compression ignition engine running on conventional diesel and hydrotreated vegetable oil (HVO). Energies 2022, 16, 144. [Google Scholar] [CrossRef]
- Ueckerdt, F.; Bauer, C.; Dirnaichner, A.; Everall, J.; Sacchi, R.; Luderer, G. Potential and risks of hydrogen-based e-fuels in climate change mitigation. Nat. Clim. Chang. 2021, 11, 384–393. [Google Scholar] [CrossRef]
- Lindstad, E.; Lagemann, B.; Rialland, A.; Gamlem, G.M.; Valland, A. Reduction of maritime GHG emissions and the potential role of E-fuels. Transp. Res. Part D Transp. Environ. 2021, 101, 103075. [Google Scholar] [CrossRef]
- Ravi, S.S.; Brace, C.; Larkin, C.; Aziz, M.; Leach, F.; Turner, J.W. On the pursuit of emissions-free clean mobility–Electric vehicles versus e-fuels. Sci. Total Environ. 2023, 875, 162688. [Google Scholar] [CrossRef] [PubMed]
- Ferrari, A.; Pizzo, P.; Vento, O. Investigation of a GDI injector with an innovative flowmeter for high-pressure transient flows. Int. J. Engine Res. 2023, 24, 4287–4296. [Google Scholar] [CrossRef]
- Fontanesi, S.; Shamsudheen, F.A.; Gonzalez, E.G.; Sarathy, S.M.; Berni, F.; D Adamo, A.; Borghi, M.; Breda, S. Impact of fuel surrogate formulation on the prediction of knock statistics in a single cylinder GDI engine. Int. J. Engine Res. 2024, 25, 405–423. [Google Scholar] [CrossRef]
- Huang, W.; Moon, S.; Gao, Y.; Wang, J.; Ozawa, D.; Matsumoto, A. Hole Number Effect on Spray Dynamics of Multi-Hole Diesel Nozzles: An Observation from Three- to Nine-Hole Nozzles. Exp. Therm. Fluid Sci. 2018, 102, 387–396. [Google Scholar] [CrossRef]
- Huang, W.; Pratama, R.H.; Oguma, M.; Kinoshita, K.; Takeda, Y.; Suzuki, S. Spray dynamics of synthetic dimethyl carbonate and its blends with gasoline. Fuel 2023, 341, 127696. [Google Scholar] [CrossRef]
- Huang, W.; Gong, H.; Moon, S.; Wang, J.; Murayama, K.; Taniguchi, H.; Arima, T.; Arioka, A.; Sasaki, Y. Nozzle Tip Wetting in GDI Injector at Flash-boiling Conditions. Int. J. Heat Mass Transf. 2021, 169, 120935. [Google Scholar] [CrossRef]
- Sou, A.; Hosokawa, S.; Tomiyama, A. Effects of cavitation in a nozzle on liquid jet atomization. Int. J. Heat Mass Transf. 2007, 50, 3575–3582. [Google Scholar] [CrossRef]
- Qiu, T.; Song, X.; Lei, Y.; Liu, X.; An, X.; Lai, M. Influence of inlet pressure on cavitation flow in diesel nozzle. Appl. Therm. Eng. 2016, 109, 364–372. [Google Scholar] [CrossRef]
- Giannadakis, E.; Gavaises, M.; Arcoumanis, C. Modelling of cavitation in diesel injector nozzles. J. Fluid Mech. 2008, 616, 153–193. [Google Scholar] [CrossRef]
- Pratama, R.H.; Huang, W.; Moon, S.; Wang, J.; Murayama, K.; Taniguchi, H.; Arima, T.; Sasaki, Y.; Arioka, A. Hydraulic flip in a gasoline direct injection injector and its effect on injected spray. Fuel 2022, 310, 122303. [Google Scholar] [CrossRef]
- Ge, M.; Zhang, G.; Petkovšek, M.; Long, K.; Coutier-Delgosha, O. Intensity and regimes changing of hydrodynamic cavitation considering temperature effects. J. Clean Prod. 2022, 338, 130470. [Google Scholar] [CrossRef]
- Ge, M.; Sun, C.; Zhang, G.; Coutier-Delgosha, O.; Fan, D. Combined suppression effects on hydrodynamic cavitation performance in Venturi-type reactor for process intensification. Ultrason. Sonochem. 2022, 86, 106035. [Google Scholar] [CrossRef]
- Yin, B.; Yu, S.; Jia, H.; Yu, J. Numerical research of diesel spray and atomization coupled cavitation by Large Eddy Simulation (LES) under high injection pressure. Int. J. Heat Fluid Flow 2016, 59, 1–9. [Google Scholar] [CrossRef]
- Wang, Z.; Dai, X.; Li, F.; Li, Y.; Lee, C.; Wu, H.; Li, Z. Nozzle internal flow and spray primary breakup with the application of closely coupled split injection strategy. Fuel 2018, 228, 187–196. [Google Scholar] [CrossRef]
- Zhang, L.; He, Z.; Guan, W.; Wang, Q.; Som, S. Simulations on the cavitating flow and corresponding risk of erosion in diesel injector nozzles with double array holes. Int. J. Heat Mass Transf. 2018, 124, 900–911. [Google Scholar] [CrossRef]
- Gong, H.; Huang, W.; Gao, Y.; Wang, J.; Arioka, A.; Sasaki, Y. End-of-injection fuel dribbling dynamics of multi-hole GDI injector. Fuel 2022, 317, 123406. [Google Scholar] [CrossRef]
- Gao, Y.; Huang, W.; Pratama, R.; Wang, J. Transient Nozzle-Exit Velocity Profile in Diesel Spray and Its Influencing Parameters. Int. J. Automot. Manuf. Mater. 2022, 1, 8. [Google Scholar] [CrossRef]
- Ji, M.; Wu, Z.; Ferrari, A.; Fu, L.; Vento, O. Experimental Investigation on Gasoline—Water Mixture Fuel Impingement Preparation Method and Spray Characteristics with High Injection Temperatures and Pressures. Energies 2023, 16, 6026. [Google Scholar] [CrossRef]
- Payri, F.; Bermúdez, V.; Payri, R.; Salvador, F.J. The influence of cavitation on the internal flow and the spray characteristics in diesel injection nozzles. Fuel 2004, 83, 419–431. [Google Scholar] [CrossRef]
- Benajes, J.; Pastor, J.V.; Payri, R.; Plazas, A.H. Analysis of the influence of diesel nozzle geometry in the injection rate characteristic. J. Fluids Eng. 2004, 126, 63–71. [Google Scholar] [CrossRef]
- Soyama, H. Effect of nozzle geometry on a standard cavitation erosion test using a cavitating jet. Wear 2013, 297, 895–902. [Google Scholar] [CrossRef]
- He, Z.; Guo, G.; Tao, X.; Zhong, W.; Leng, X.; Wang, Q. Study of the effect of nozzle hole shape on internal flow and spray characteristics. Int. Commun. Heat Mass Transf. 2016, 71, 1–8. [Google Scholar] [CrossRef]
- Chen, Z.; He, Z.; Shang, W.; Duan, L.; Zhou, H.; Guo, G.; Guan, W. Experimental study on the effect of nozzle geometry on string cavitation in real-size optical diesel nozzles and spray characteristics. Fuel 2018, 232, 562–571. [Google Scholar] [CrossRef]
- Liao, H.; Zhao, S.; Cao, Y.; Zhang, L.; Yi, C.; Niu, J.; Zhu, L. Erosion characteristics and mechanism of the self-resonating cavitating jet impacting aluminum specimens under the confining pressure conditions. J. Hydrodyn. 2020, 32, 375–384. [Google Scholar] [CrossRef]
- He, Z.; Hu, B.; Wang, J.; Guo, G.; Feng, Z.; Wang, C.; Duan, L. The cavitation flow and spray characteristics of gasoline-diesel blends in the nozzle of a high-pressure common-rail injector. Fuel 2023, 350, 128786. [Google Scholar] [CrossRef]
- Liu, H.; Xu, Y.; Wang, Z.; Zhang, J.; Wang, J. Experimental and numerical simulations to examine the mechanism of nozzle geometry affecting cavitation water jets. Geoenergy Sci. Eng. 2024, 233, 212511. [Google Scholar] [CrossRef]
- Balz, R.; Nagy, I.G.; Weisser, G.; Sedarsky, D. Experimental and numerical investigation of cavitation in marine Diesel injectors. Int. J. Heat Mass Transf. 2021, 169, 120933. [Google Scholar] [CrossRef]
- Wu, Z.; Zhao, W.; Li, Z.; Deng, J.; Hu, Z.; Li, L. A review of engine fuel injection studies using synchrotron radiation x-ray imaging. Automot. Innov. 2019, 2, 79–92. [Google Scholar] [CrossRef]
- Li, Z.; Zhao, W.; Wu, Z. Understanding transient internal flow processes in high-pressure nozzles using synchrotron radiation X-ray phase contrast imaging technology. At. Sprays 2021, 31, 1–14. [Google Scholar] [CrossRef]
- Hu, B.; He, Z.; Li, C.; Deng, Y.; Guan, W.; Zhang, L.; Guo, G. Study of the effect of cavitation flow patterns in diesel injector nozzles on near-field spray atomization characteristics using a LES-VOF method. Int. J. Multiph. Flow. 2024, 174, 104791. [Google Scholar] [CrossRef]
- Yu, S.; Yin, B.; Jia, H.; Chen, C.; Xu, B. Investigation of inner cavitation and nozzle exit flow patterns for elliptical orifice GDI injectors with various aspect ratios. Int. Commun. Heat Mass 2021, 129, 105682. [Google Scholar] [CrossRef]
- Guo, G.; Lu, K.; Xu, S.; Yuan, J.; Bai, T.; Yang, K.; He, Z. Effects of in-nozzle liquid fuel vortex cavitation on characteristics of flow and spray: Numerical research. Int. Commun. Heat Mass 2023, 148, 107040. [Google Scholar] [CrossRef]
- Yang, G.; Hu, L.; Qiu, F.; Li, W.; Long, F.; Hu, D.; Cheng, Z. Numerical simulation of the effect of jet small orifice structure on cavitation characteristic and jet impact flow field. Chem. Eng. Process. Process Intensif. 2024, 200, 109775. [Google Scholar] [CrossRef]
- Zhao, W.; Li, Z.; Deng, J.; Li, L.; Wu, Z. Experimental and Numerical Study on the Effects of Nozzle Geometry Features on the Nozzle Internal Flow and Cavitation Characteristics. At. Sprays 2021, 31, 67–95. [Google Scholar] [CrossRef]
- Wu, Z.; Hu, C.; Leng, P.; Zhao, W.; Deng, J.; Li, K.; Li, L. Effect of nozzle geometry features on the nozzle internal flow and cavitation characteristics based on X-ray dynamic imaging. Nucl. Instrum. Methods Phys. Res. Sect. A Accel. Spectrometers Detect. Assoc. Equip. 2024, 1058, 168831. [Google Scholar] [CrossRef]
- Saha, K.; Som, S.; Battistoni, M.; Li, Y.; Quan, S.; Kelly Senecal, P. Modeling of Internal and Near-Nozzle Flow for a Gasoline Direct Injection Fuel Injector. J. Energy Resour. Technol. 2016, 138, 52208. [Google Scholar] [CrossRef]
- Tu, P.W.; Xu, H.; Srivastava, D.K.; Dean, K.; Jing, D.; Cao, L.; Weall, A.; Venus, J.K. Numerical Investigation of GDI Injector Nozzle Geometry on Spray Characteristics; SAE Technical Paper 2015-01-1906; SAE International: Warrendale, PA, USA, 2015. [Google Scholar] [CrossRef]
- Dong, P.; Chen, Q.; Liu, G.; Zhang, B.; Yan, G.; Wang, R. Effects of geometric parameters on flow and atomization characteristics of swirl nozzles for artificial snowmaking. Int. J. Refrig. 2023, 154, 56–65. [Google Scholar] [CrossRef]
- Bal, M.; Kayansalçik, G.; Ertunç, Ö; Böke, Y.E. Benchmark study of 2D and 3D VOF simulations of a simplex nozzle using a hybrid RANS-LES approach. Fuel 2022, 319, 123695. [Google Scholar] [CrossRef]
- Guan, W.; He, Z.; Zhang, L.; EL-Seesy, A.I.; Wen, L.; Zhang, Q.; Yang, L. Effect of asymmetric structural characteristics of multi-hole marine diesel injectors on internal cavitation patterns and flow characteristics: A numerical study. Fuel 2021, 283, 119324. [Google Scholar] [CrossRef]
- Ferrari, A.; Vento, O. Thermal effects on Common Rail injection system hydraulic performance. Int. J. Engine Res. 2023, 24, 3602–3612. [Google Scholar] [CrossRef]
- Payri, R.; Salvador, F.J.; Gimeno, J.; Venegas, O. Study of cavitation phenomenon using different fuels in a transparent nozzle by hydraulic characterization and visualization. Exp. Therm. Fluid. Sci. 2013, 44, 235–244. [Google Scholar] [CrossRef]
- Ferrari, A. Fluid dynamics of acoustic and hydrodynamic cavitation in hydraulic power systems. Proc. R. Soc. A 2017, 473, 20160345. [Google Scholar] [CrossRef]
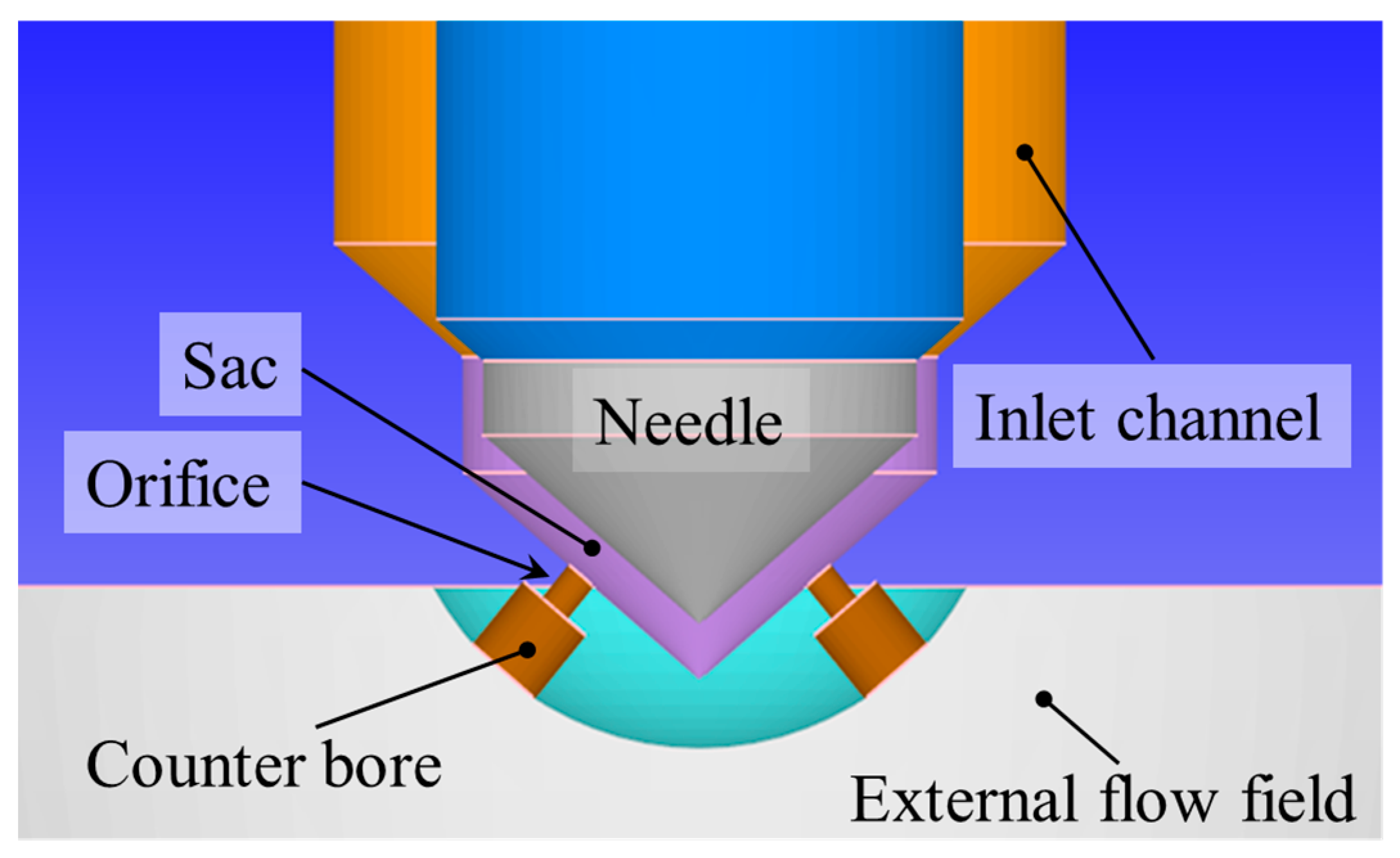

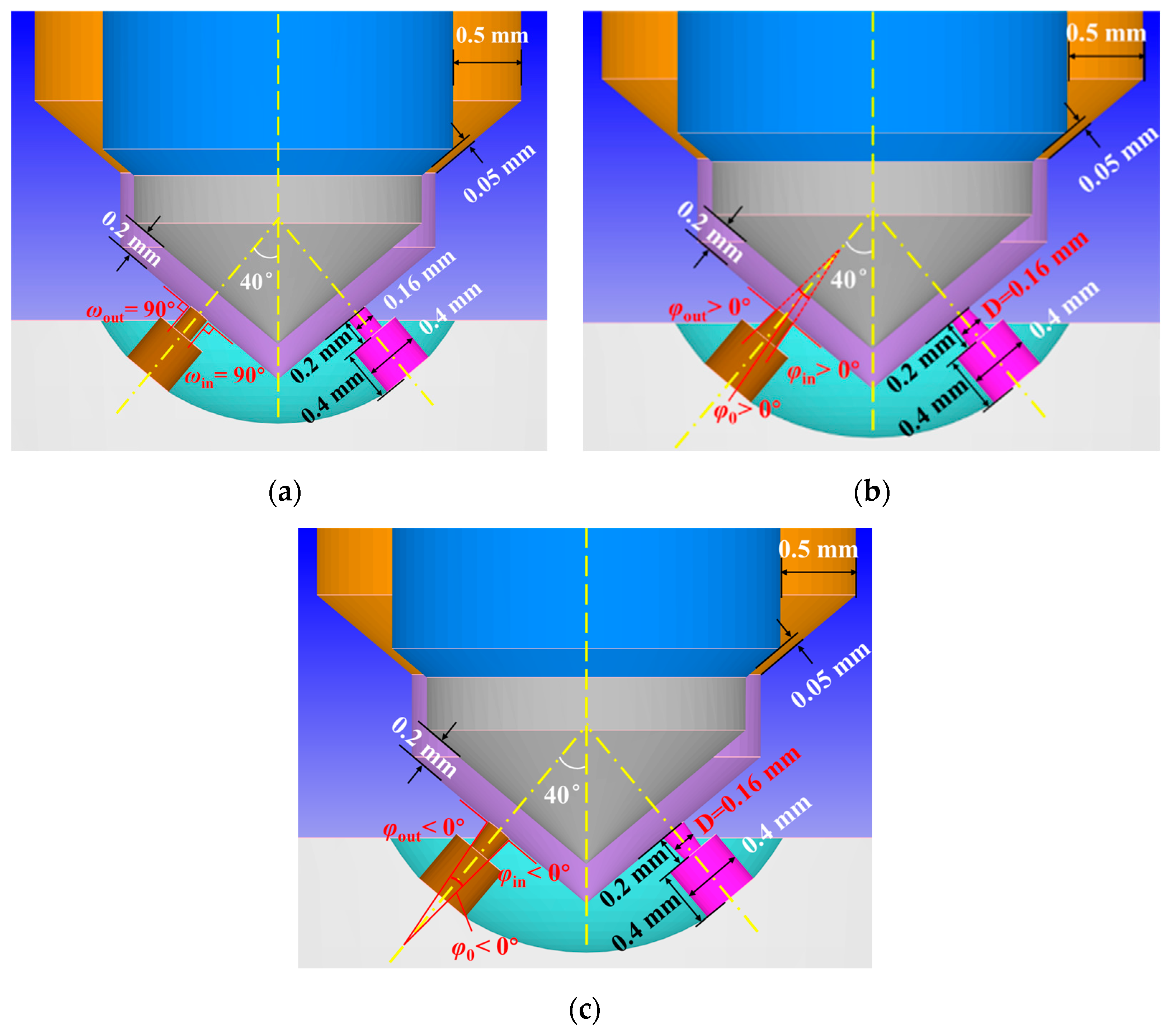





| Computational domain | Domain | Species | P [bar] | T [K] |
| Inlet channel | isooctane: 99.998%; air: 0.002% | 200 | 300 | |
| Sac | isooctane: 99.998%; air: 0.002% | 1 | ||
| Orifice | air (77% N2; 23% O2) | |||
| Counter bore | air (77% N2; 23% O2) | |||
| External flow field | air (77% N2; 23% O2) | |||
| Boundary conditions | Boundary | Species | P [bar] | T [K] |
| Inlet | isooctane: 99.998%; air: 0.002% | 200 | 300 | |
| Outlet | air (77% N2; 23% O2) | 1 | ||
| Wall | —— | —— |
| Viscosity Ns/m2 | Density kg/m3 | Vapor Pressure kPa | Vaporization Heat J/kg | Specific Heat Factor J/(kg·K) | |
|---|---|---|---|---|---|
| Isooctane | 6.13 × 10−4 | 698 | 11 | 3.35 × 105 | 2130 |
| air | 2.97 × 10−5 | 1.18 | 3.24 | 1 × 103 | 1047 |
| Nozzle | Na | Nb | Nc | Nd | Ne |
|---|---|---|---|---|---|
| φin [deg] | 0 | 5 | 10 | 15 | 20 |
| φout [deg] | 0 | −5 | −10 | −15 | −20 |
| Nozzle | N1 | N2 | N3 | N4 | N5 |
|---|---|---|---|---|---|
| ωin,out [deg] | 80 | 85 | 90 | 95 | 100 |
| φin,out [deg] | 10 | 5 | 0 | −5 | −10 |
| φ0 [deg] | 20 | 10 | 0 | −10 | −20 |
| Orifice type | Divergent | Divergent | Cylindrical | Convergent | Convergent |
| D [mm] | Dout [mm] | Aout [mm2] | φ0 [deg] | Nozzle Type | |
|---|---|---|---|---|---|
| Nozzle N1 | 0.16 | 0.195 | 0.030 | +20 | Divergent |
| Nozzle N2 | 0.16 | 0.177 | 0.025 | +10 | Divergent |
| Nozzle N3 | 0.16 | 0.16 | 0.020 | 0 | Cylindrical |
| Nozzle N4 | 0.16 | 0.143 | 0.016 | −10 | Convergent |
| Nozzle N5 | 0.16 | 0.125 | 0.012 | −20 | Convergent |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hu, C.; Wu, Z.; Ferrari, A.; Ji, M.; Deng, J.; Vento, O. Numerical Study on Internal Flow and Cavitation Characteristics of GDI Injectors for Different Nozzle Orifice Geometries. Energies 2024, 17, 4114. https://doi.org/10.3390/en17164114
Hu C, Wu Z, Ferrari A, Ji M, Deng J, Vento O. Numerical Study on Internal Flow and Cavitation Characteristics of GDI Injectors for Different Nozzle Orifice Geometries. Energies. 2024; 17(16):4114. https://doi.org/10.3390/en17164114
Chicago/Turabian StyleHu, Chaoqun, Zhijun Wu, Alessandro Ferrari, Meng Ji, Jun Deng, and Oscar Vento. 2024. "Numerical Study on Internal Flow and Cavitation Characteristics of GDI Injectors for Different Nozzle Orifice Geometries" Energies 17, no. 16: 4114. https://doi.org/10.3390/en17164114
APA StyleHu, C., Wu, Z., Ferrari, A., Ji, M., Deng, J., & Vento, O. (2024). Numerical Study on Internal Flow and Cavitation Characteristics of GDI Injectors for Different Nozzle Orifice Geometries. Energies, 17(16), 4114. https://doi.org/10.3390/en17164114






