Improved Performance of Low-Frequency Harmonic Current Suppression Based on Hybrid Active Power Filter
Abstract
1. Introduction
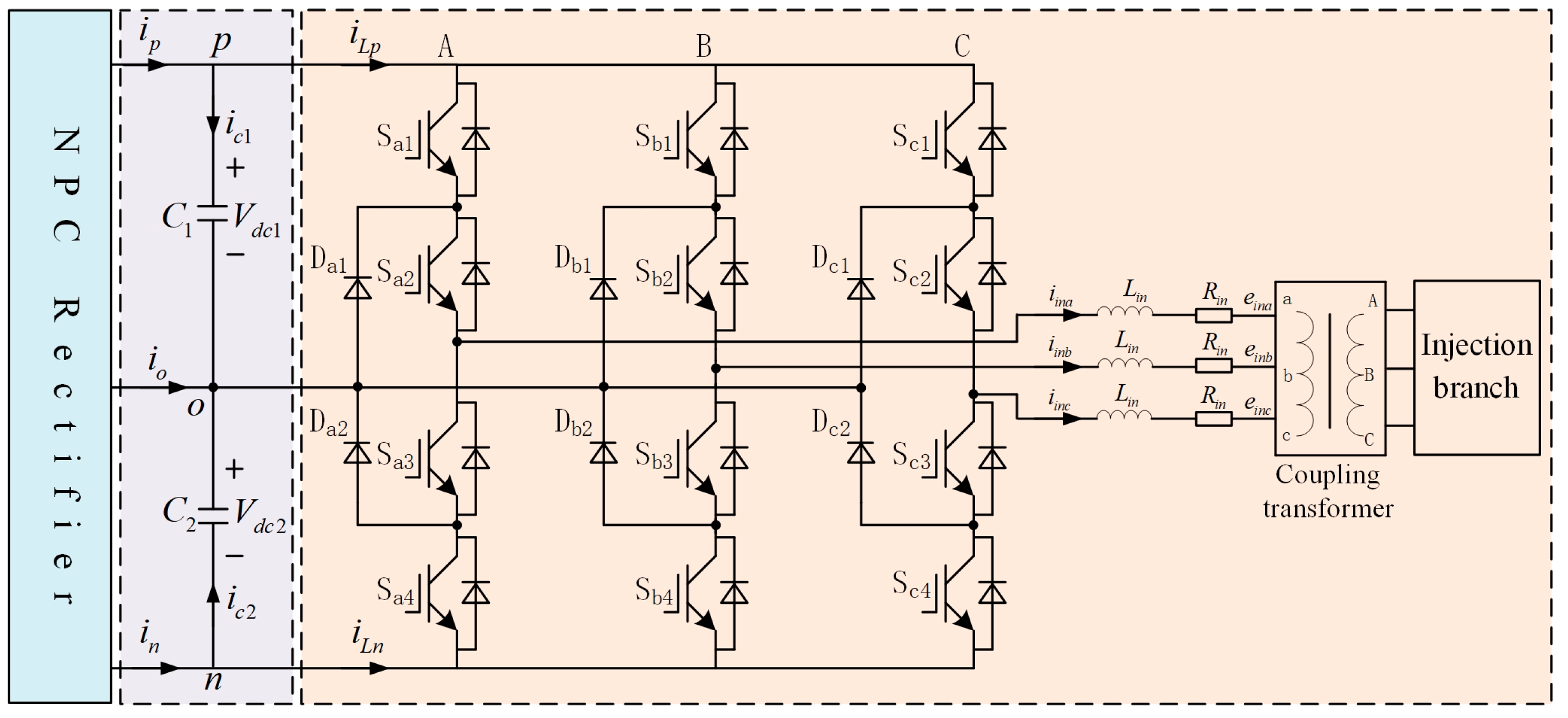
2. Hybrid Active Power Filter
3. HAPF Mathematical Model
3.1. APF Mathematical Model
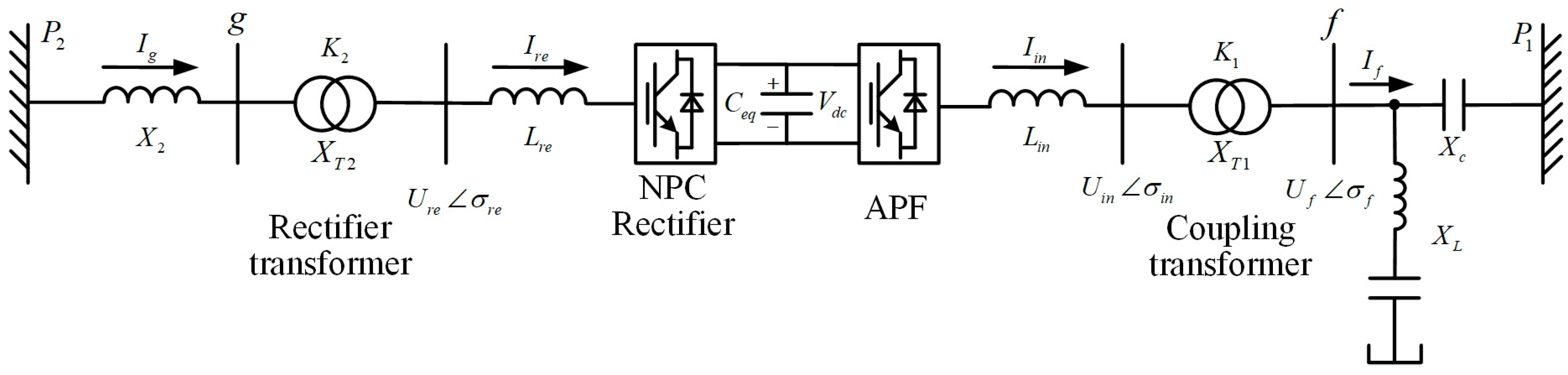
3.2. HAPF System and Power Grid Connection Model
3.3. DC-Side Mathematical Model of HAPF
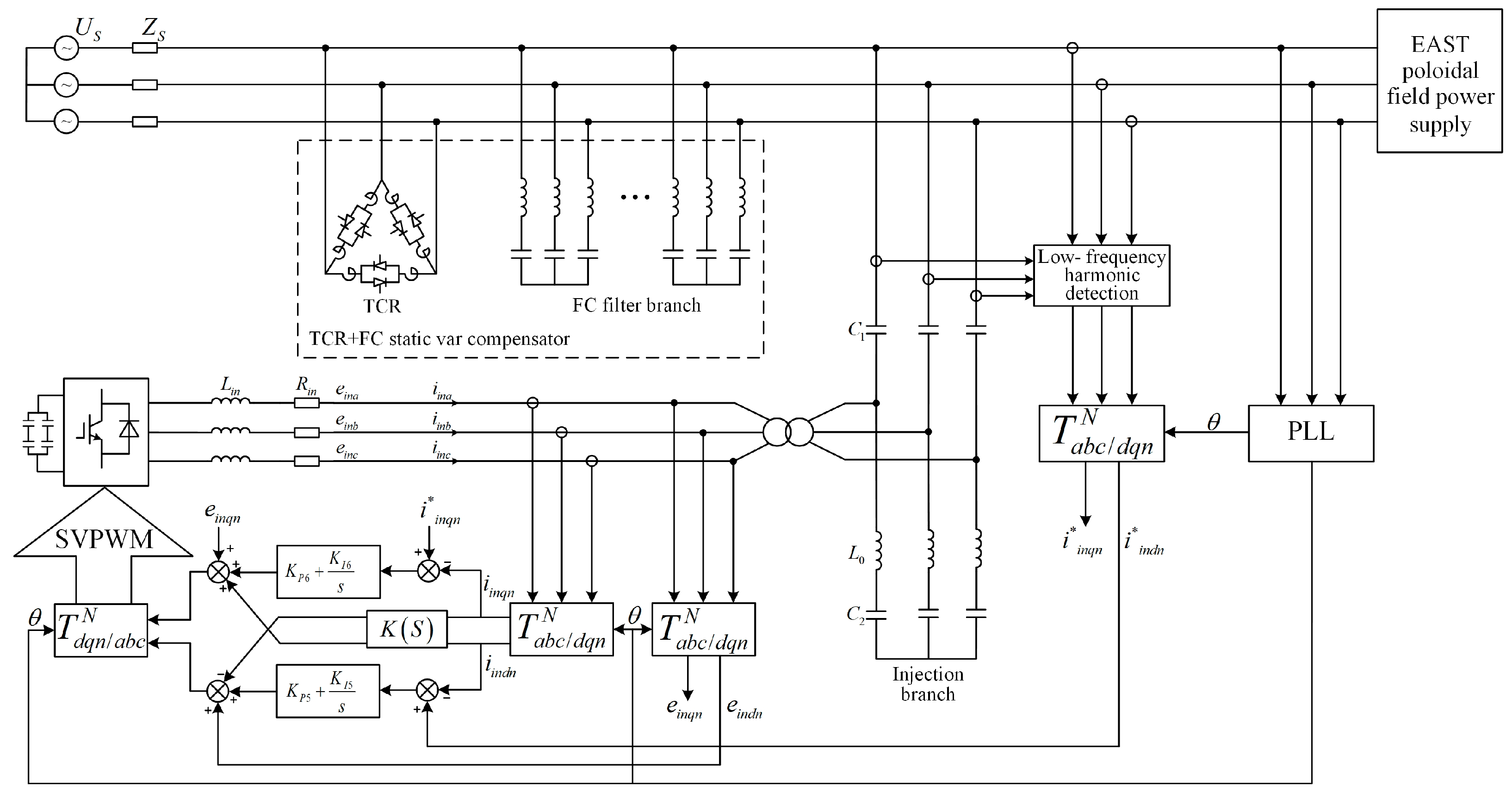
3.4. Mathematical Model of HAPF Control System
3.5. Mathematical Model of the HAPF
4. HAPF Stability Analysis
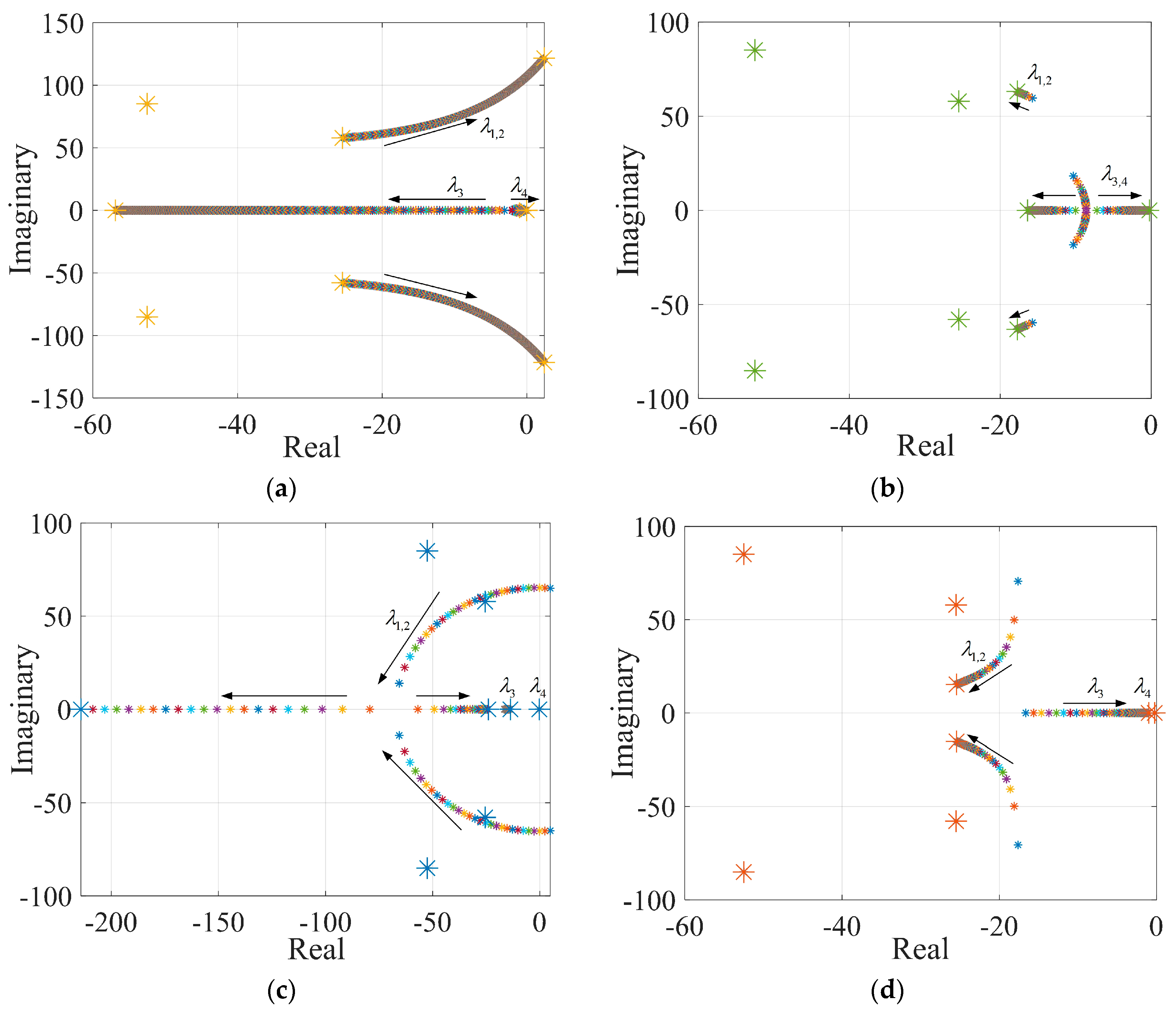
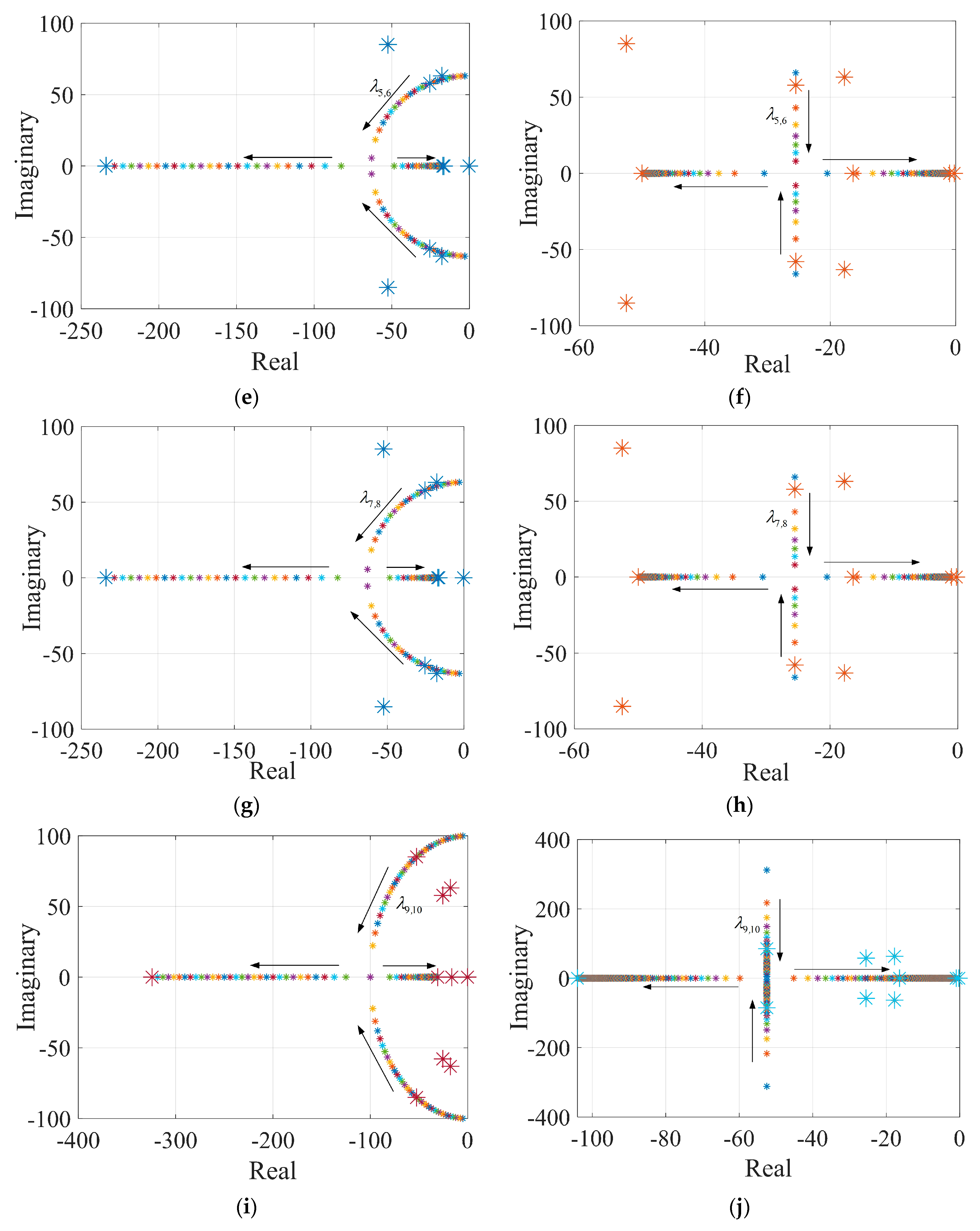
4.1. Eigenvalues and Stability Analysis of the HAPF
4.2. Eigenvalue Analysis and Control Parameter Optimization
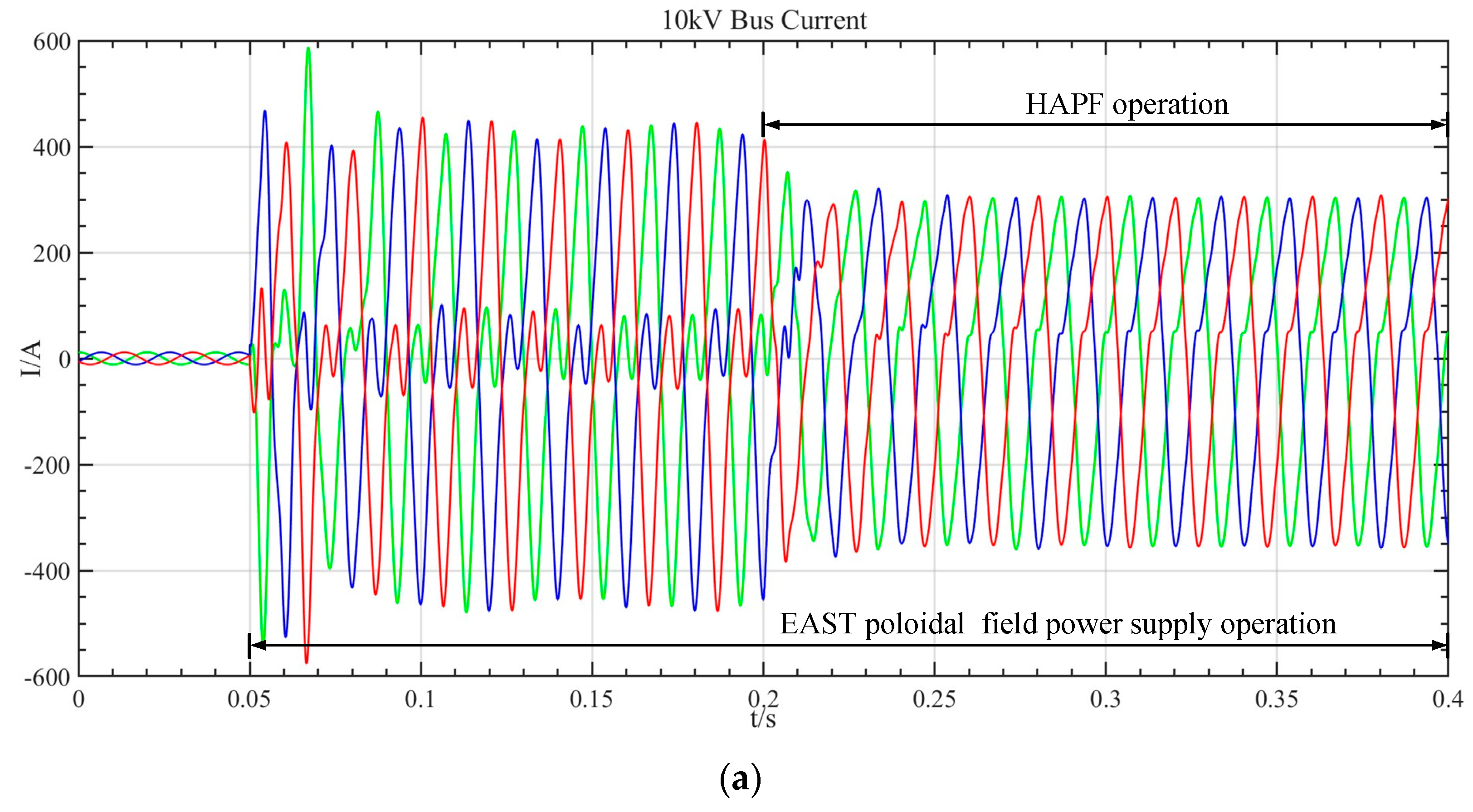
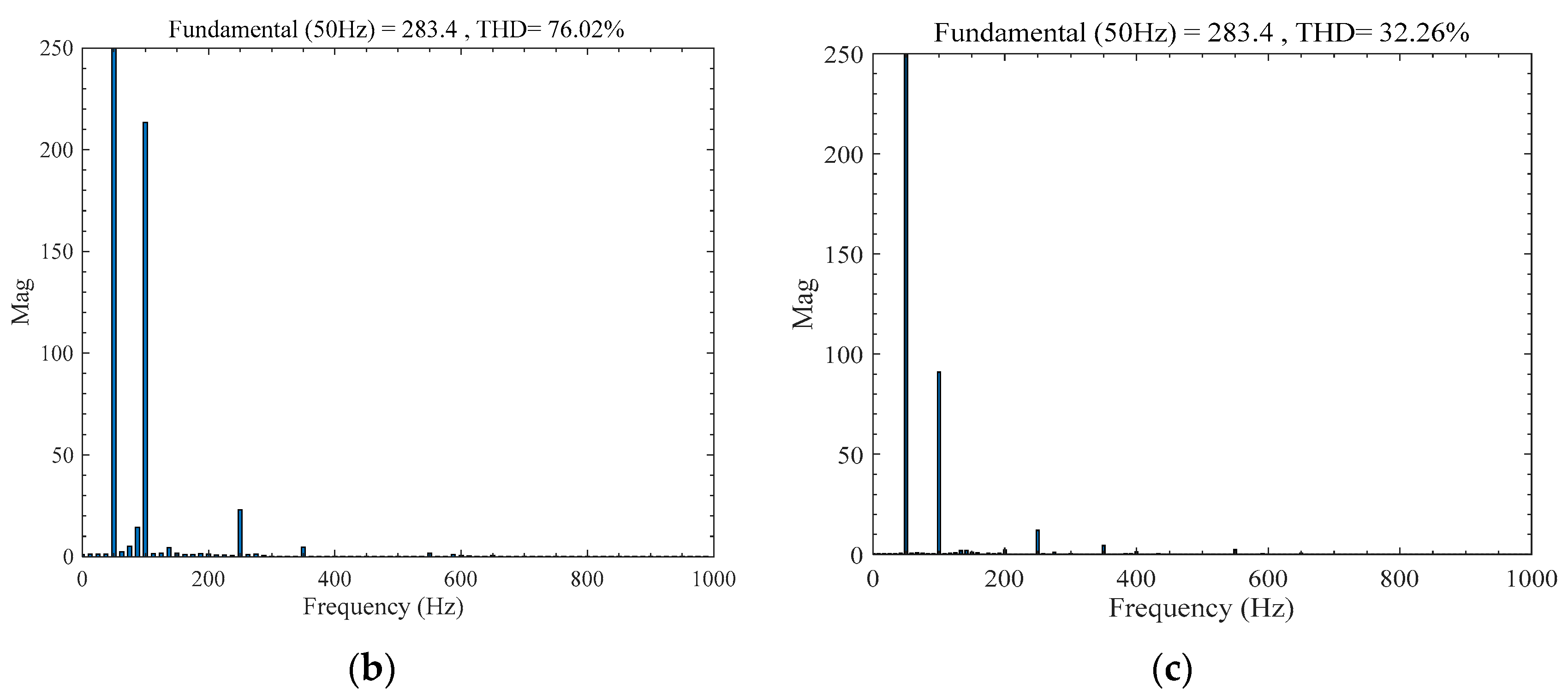
5. Simulation Verification
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Li, J.; Guo, H.Y.; Wan, B.N.; Liang, Y.F.; Xu, G.S. Recent progress on EAST. Fusion Sci. Technol. 2017, 64, 417–423. [Google Scholar] [CrossRef]
- Hu, L.; Zhou, Z.; Zhuang, M.; Zhang, Q.; Zhu, Z. Alarm and interlock system in cryogenic system of EAST. IEEE Trans. Appl. Supercond. 2020, 30, 4203105. [Google Scholar] [CrossRef]
- Wan, B.N.; Xu, G.S. Advances in experimental research towards high confinement and steady state operation on the experimental advanced superconducting Tokamak. Sci. Sin.-Phys. Mech. Astron. 2019, 49, 47–59. [Google Scholar] [CrossRef]
- Wan, B.; Gong, X.; Liang, Y.; Xiang, N.; Xu, G.S.; Sun, Y.; Wang, L.; Qian, J.; Liu, H.; Zhang, B.; et al. Advances in the long-pulse steady-state high beta H-mode scenario with active controls of divertor heat and particle fluxes in EAST. Nucl. Fusion 2022, 62, 042010. [Google Scholar] [CrossRef]
- Liu, Y.; Fu, P.; Zhang, X.; Huang, L.; He, S.; Chen, X. Design the phase output bar for improving static and dynamic current distribution among parallel IGBTs in high power rectifier bridge arm of the super-conducting Tokamak (EAST). IEEE Trans. Plasma Sci. 2024; Early Access. [Google Scholar] [CrossRef]
- Ali, M.; Labouré, E.; Costa, F. Integrated active filter for differential-mode noise suppression. IEEE Trans. Power Electron. 2014, 29, 1503–1507. [Google Scholar] [CrossRef]
- Huang, R.L.; Zhu, Y.F.; Fu, P.; Huang, L.S.; Wang, Z.M.; Xu, L.W.; Gao, G. Research on short circuit operation control strategy of a superconducting magnet power supply for CRAFT. J. Fusion Energy 2021, 40, 21. [Google Scholar] [CrossRef]
- He, S.; Huang, L.; Gao, G.; Wang, G.; Wang, Z.; Chen, X. Design of real-time control in poloidal field power supply based on finite-state machine. IEEE Trans. Plasma Sci. 2019, 47, 1878–1883. [Google Scholar] [CrossRef]
- Ji, Y.; Yuan, H.W.; Xu, L.W.; Wu, Y.N.; Guo, Q.; Mao, H.F.; Li, J. Control and operation mode of ITER high-power DC testing platform. J. Fusion Energy 2015, 34, 1483–1488. [Google Scholar] [CrossRef]
- Qu, J.; Fu, P.; Wu, Y.N.; Lu, J.; Zhu, M.X.; Liang, Y.; Tian, Y.X. Power quality analysis for poloidal field power supply system in EAST. Fusion Sci. Technol. 2021, 77, 316–326. [Google Scholar] [CrossRef]
- Miao, P.C.; Li, G.; Weng, Z.Y. Analysis of the vertical field on EAST for long-pulse H-mode discharges. IEEE Trans. Plasma Sci. 2020, 48, 280–284. [Google Scholar] [CrossRef]
- Shen, X.S.; Tao, J.; Deng, T.B.; Wu, Y.N.; Wu, S.Y. Research on harmonic suppression characteristics and control methods for low-frequency resonance suppressor. Fusion Eng. Des. 2024, 204, 114462. [Google Scholar] [CrossRef]
- Lu, J.; Fu, P.; Li, J.; Mao, H.F.; Xu, L.W.; Wu, Y.N. A new hybrid filter based on differential current control method for low-order harmonic suppression in Tokamak power system. Int. J. Energy Res. 2018, 42, 82–90. [Google Scholar] [CrossRef]
- Ai, L.; Hu, H.; Li, Z.; Wang, Y.; Guo, X. A novel frequency tuning scheme for a C-type filter based on the best filtering frequency. IEEE Trans. Power Deliv. 2023, 38, 3021–3032. [Google Scholar] [CrossRef]
- Aleem, S.H.E.A.; Zobaa, A.F.; Aziz, M.M.A. Optimal C-type passive filter based on minimization of the voltage harmonic distortion for nonlinear loads. IEEE Trans. Ind. Electron. 2012, 59, 281–289. [Google Scholar] [CrossRef]
- Mishra, R.; Saha, T.K. Control of a stand-alone distributed generation system with unbalanced and nonlinear load. Int. Trans. Electr. Energy Syst. 2020, 30, e12286. [Google Scholar] [CrossRef]
- Moradian, M.; Soltani, J. An isolated three-phase induction generator system with dual stator winding sets under unbalanced load condition. IEEE Trans Energy Convers. 2016, 31, 531–539. [Google Scholar] [CrossRef]
- Kraimia, M.N.; Boudour, M. Harmonic interaction of a static var compensator with AC power system containing multiple non-linear loads. Eng. Rev. 2020, 40, 21–31. [Google Scholar] [CrossRef]
- Liu, Y.; Xu, W.; Zhu, J.; Blaabjerg, F. Sensorless Control of standalone brushless doubly fed induction generator feeding unbalanced loads in a ship shaft power generation system. IEEE Trans. Ind. Electron. 2018, 66, 739–749. [Google Scholar] [CrossRef]
- Wang, W. SVC Power Supply Method of High Power AC EAF and Power Quality Control; Shanghai Jiao Tong University: Shanghai, China, 2008. [Google Scholar]
- Gu, J.J.; Li, K.; Zheng, Y.Z. Research on model of DC furnace system of Baosteel. Metall. Power 2011, 4, 1–3, 42. [Google Scholar]
- Xue, L.N. Study on Operating Characteristic Model and Virtual Control of a 350 MW Thermal Power Unit; Southeast University: Nanjing, China, 2018. [Google Scholar]
- Aravena, P.; Moran, L.; Dixon, J.; Espinoza, J.; Godoy, O. A new hybrid filter topology for sub and inter-harmonic attenuation in cycloconverter-fed drives applications. In Proceedings of the IEEE Industry Applications Society Annual Meeting, Houston, TX, USA, 4–8 October 2009; pp. 1–7. [Google Scholar]
- Popescu, M.; Bitoleanu, A.; Constantin, V.S.; Linca, M.; Subtirelu, G.E. Adaptive control of DC voltage in three-phase three-wire shunt active power filters systems. Energies 2020, 13, 3147. [Google Scholar] [CrossRef]
- Lumbreras, D.; Gálvez, E.; Collado, A.; Zaragoza, J. Trends in power quality, harmonic mitigation and standards for light and heavy industries: A review. Energies 2020, 13, 5792. [Google Scholar] [CrossRef]
- Wu, Y.; Chen, R.; Li, C.; Zhang, L.; Dai, W. An adaptive sine-cosine moth-flame optimization algorithm for parameter identification of hybrid active power filters in power systems. IEEE Access 2020, 8, 156378–156393. [Google Scholar] [CrossRef]
- Akagi, H. New trends in active filters for power conditioning. IEEE Trans. Ind. Appl. 1996, 32, 1312–1322. [Google Scholar] [CrossRef]
- Tiwari, A.K.; Dubey, S.P. Ant colony optimization based hybrid active power filter for harmonic compensation. In Proceedings of the 2016 International Conference on Electrical, Electronics, and Optimization Techniques (ICEEOT), Chennai, India, 3–5 March 2016; pp. 777–782. [Google Scholar]
- Sou, W.K.; Choi, W.H.; Chao, C.W.; Lam, C.S.; Gong, C.; Wong, C.K.; Wong, M.C. A deadbeat current controller of LC-hybrid active power filter for power quality improvement. IEEE J. Emerg. Sel. Top. Power Electron. 2020, 8, 3891–3905. [Google Scholar] [CrossRef]
- Karbasforooshan, M.-S.; Monfared, M. Adaptive predictive deadbeat current control of single-phase multi-tuned shunt hybrid active power filters. IEEE Trans. Power Deliv. 2024, 39, 446–454. [Google Scholar] [CrossRef]
- Herman, L.; Knez, K.; Blažič, B. Design and performance evaluation of a hybrid active power filter controller. Energies 2024, 17, 2492. [Google Scholar] [CrossRef]
- Liu, Q.Y.; Li, Y.; Hu, S.J.; Luo, L.F. A transformer integrated filtering system for power quality improvement of industrial DC supply system. IEEE Trans. Ind. Electron. 2020, 67, 3329–3339. [Google Scholar] [CrossRef]
- Shen, X.S.; Gao, G.; Wu, Y.N.; Lu, J.; Tan, L.; Tian, Y.X.; Dai, M.Y.; Zou, T.; Liang, Y. Control parameters optimization of three-level neutral-point clamp rectifier for EAST low-frequency resonance suppressor. Fusion Eng. Des. 2021, 170, 112486. [Google Scholar] [CrossRef]





| Control Parameters | Value | APF Parameters | Value |
| Power parameters | Value | ||
| Eigenvalues | Oscillation Frequency (Hz) | Damping Ratio | |
|---|---|---|---|
| 10.05 | 0.27 | ||
| 0 | 1 | ||
| 0 | 1 | ||
| 9.21 | 0.40 | ||
| 9.21 | 0.40 | ||
| 13.55 | 0.53 | ||
| 13.55 | 0.53 |
| Scale factor | ||||||
| Integral coefficient | ||||||
| Eigenvalues | Oscillation Frequency (Hz) | Damping Ratio | |
|---|---|---|---|
| 3.45 | 0.91 | ||
| 0 | 1 | ||
| 0 | 1 | ||
| 2.25 | 0.96 | ||
| 2.25 | 0.96 | ||
| 3.54 | 0.98 | ||
| 3.54 | 0.98 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shen, X.; Tao, J.; Wu, Y.; Deng, T.; Zhu, Q.; Peng, F. Improved Performance of Low-Frequency Harmonic Current Suppression Based on Hybrid Active Power Filter. Energies 2024, 17, 3740. https://doi.org/10.3390/en17153740
Shen X, Tao J, Wu Y, Deng T, Zhu Q, Peng F. Improved Performance of Low-Frequency Harmonic Current Suppression Based on Hybrid Active Power Filter. Energies. 2024; 17(15):3740. https://doi.org/10.3390/en17153740
Chicago/Turabian StyleShen, Xianshun, Jun Tao, Yanan Wu, Tianbai Deng, Qianlong Zhu, and Feixiang Peng. 2024. "Improved Performance of Low-Frequency Harmonic Current Suppression Based on Hybrid Active Power Filter" Energies 17, no. 15: 3740. https://doi.org/10.3390/en17153740
APA StyleShen, X., Tao, J., Wu, Y., Deng, T., Zhu, Q., & Peng, F. (2024). Improved Performance of Low-Frequency Harmonic Current Suppression Based on Hybrid Active Power Filter. Energies, 17(15), 3740. https://doi.org/10.3390/en17153740





