In-Depth Analysis of the Burst of a Liquefied Petroleum Gas Tank in Gravedona, Italy
Abstract
1. Introduction
2. Materials and Methods
2.1. Description of the Accident
- After maintenance, the tanks had been left open, causing the inter gas to exit. That is, the filling procedure may have taken place when the tank was filled with air at ambient pressure.
- The standard filling procedure had not been adopted.
- A safety valve had not been properly mounted.
- The tanks had not been grounded.
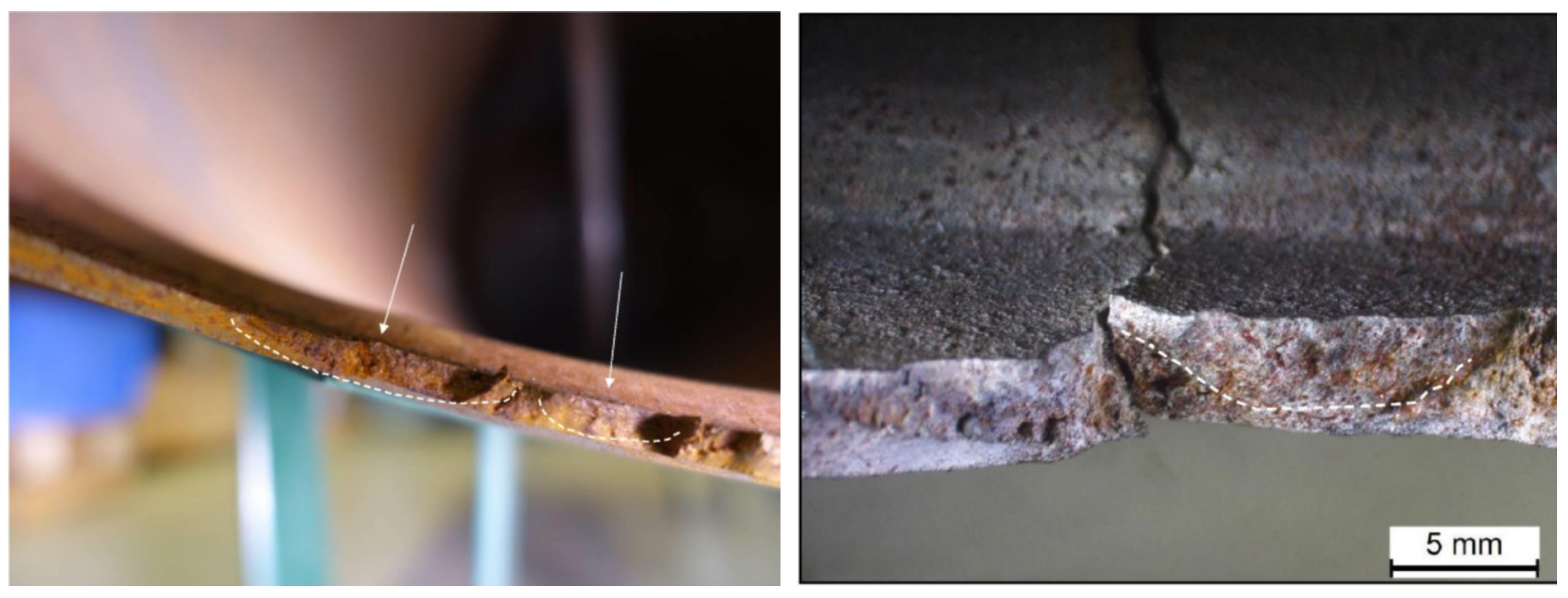
- A crack was present.
- The tank had not been properly positioned and installed.
- The air–LPG mixture inside the tank became flammable during the filling procedure. The flammable mixture was somehow ignited, and the resulting internal overpressure led to the tank bursting.
- The normal pressure rise due to the filling procedure led to the rupture of the tank due to the presence of a crack.
2.2. Analytical Model for Studying Mixture Flammability
- The behavior of the gases inside the tank can be described through the ideal gas law, and the gas mixture is homogeneous.
- Prior to filling, the tank contains air at pressure and volume . Initially, the liquefied LPG flowing into the tank undergoes a change of state and becomes gas. The air partial pressure remains constant, while the propane and butane partial pressure is determined by the moles inserted into the tank. This filling phase is referred to as phase 1.
- When the conditions inside the tank allow for the formation of liquefied LPG, a dynamic equilibrium between gaseous and liquefied LPG determines the thermodynamic state in the tank. The LPG vapor pressure at temperature T is constant at , while the air pressure increases as the ullage is reduced in size. This filling phase is referred to as phase 2.
2.3. Numerical Model for Studying Mixture Flammability
2.4. Analytical and Numerical Models for Studying the Possibility That the Burst Was Caused by Mechanical Failure
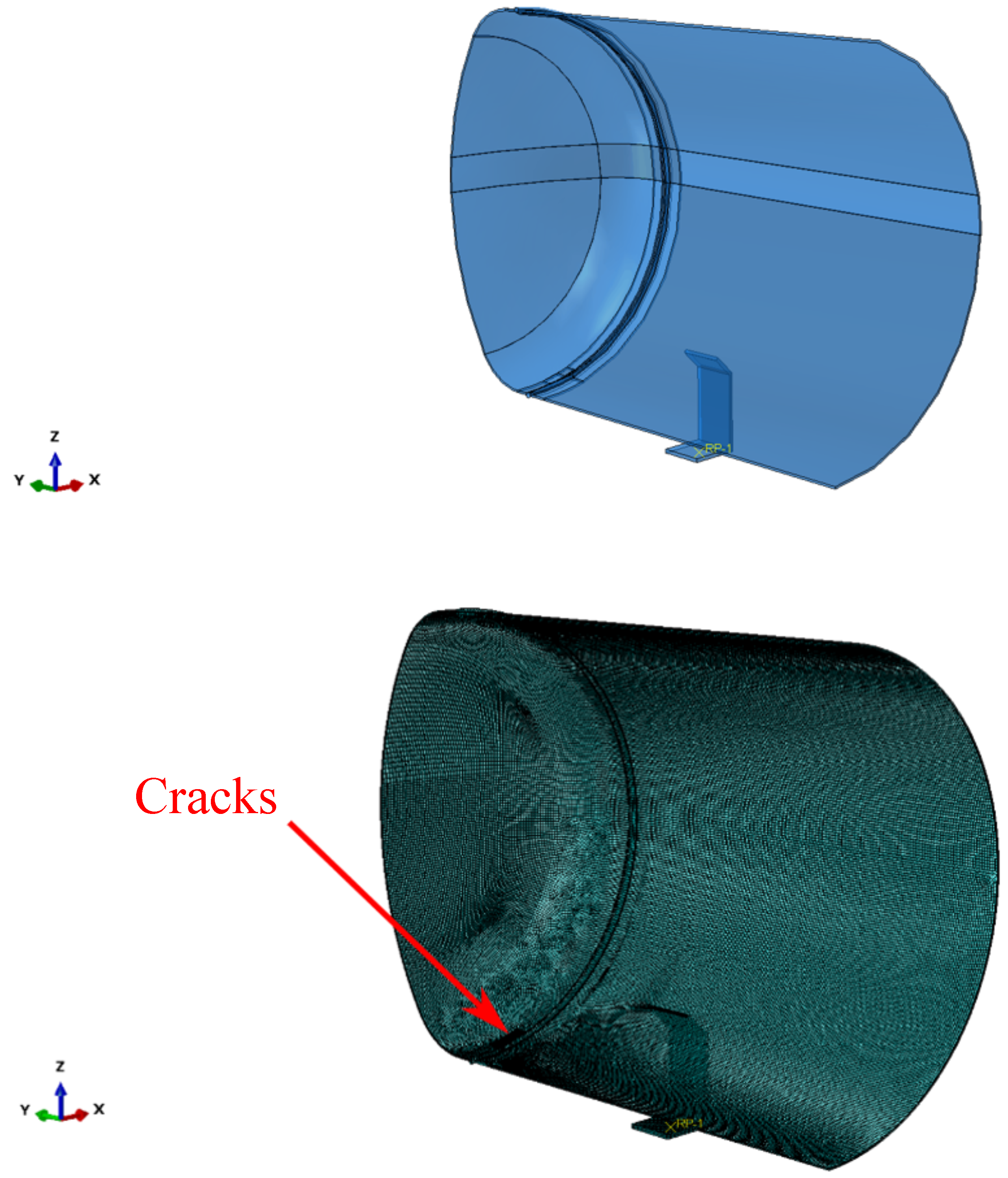
2.5. Analytical Model for Studying the Mechanics of the Tank after the Burst
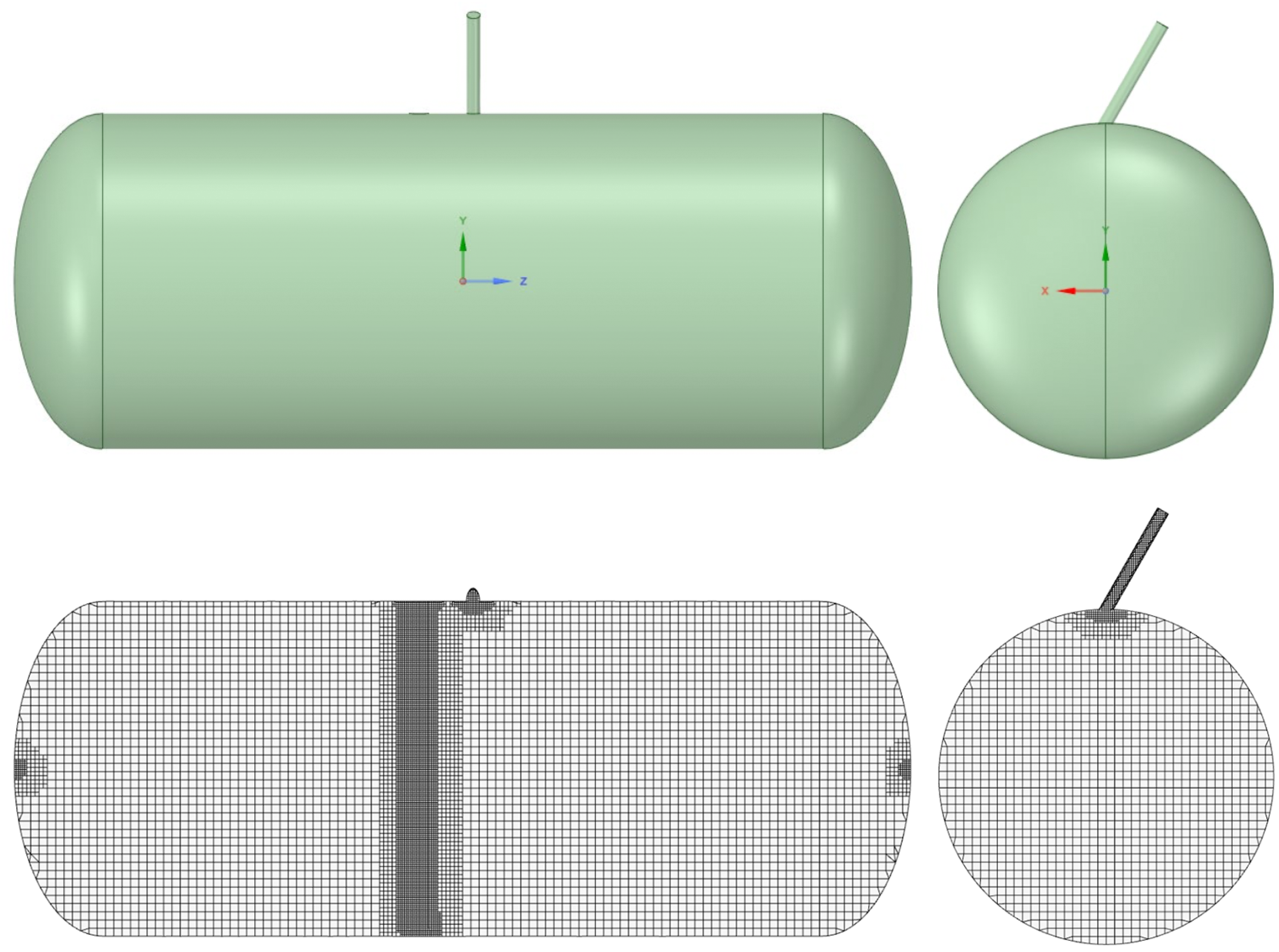
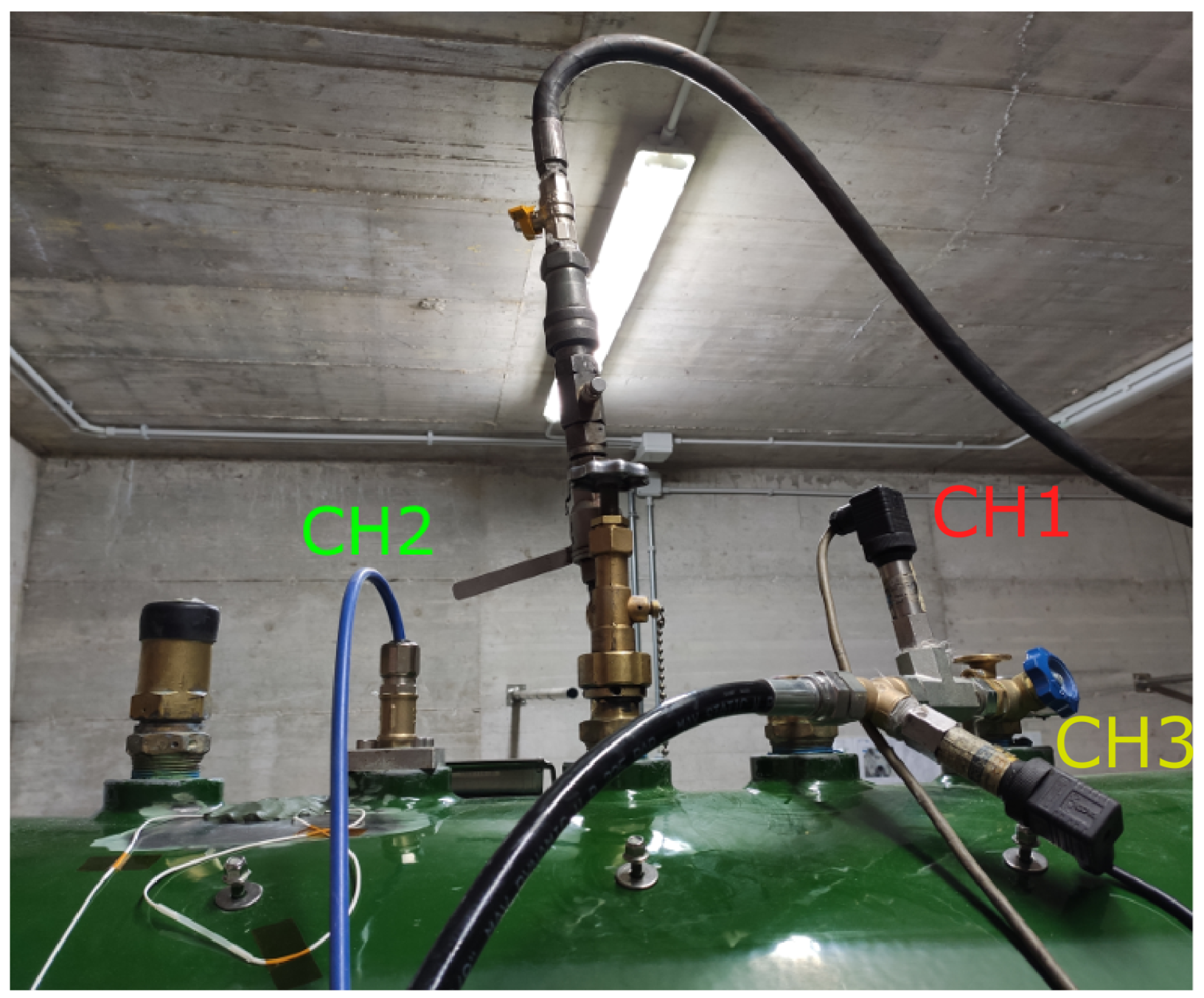
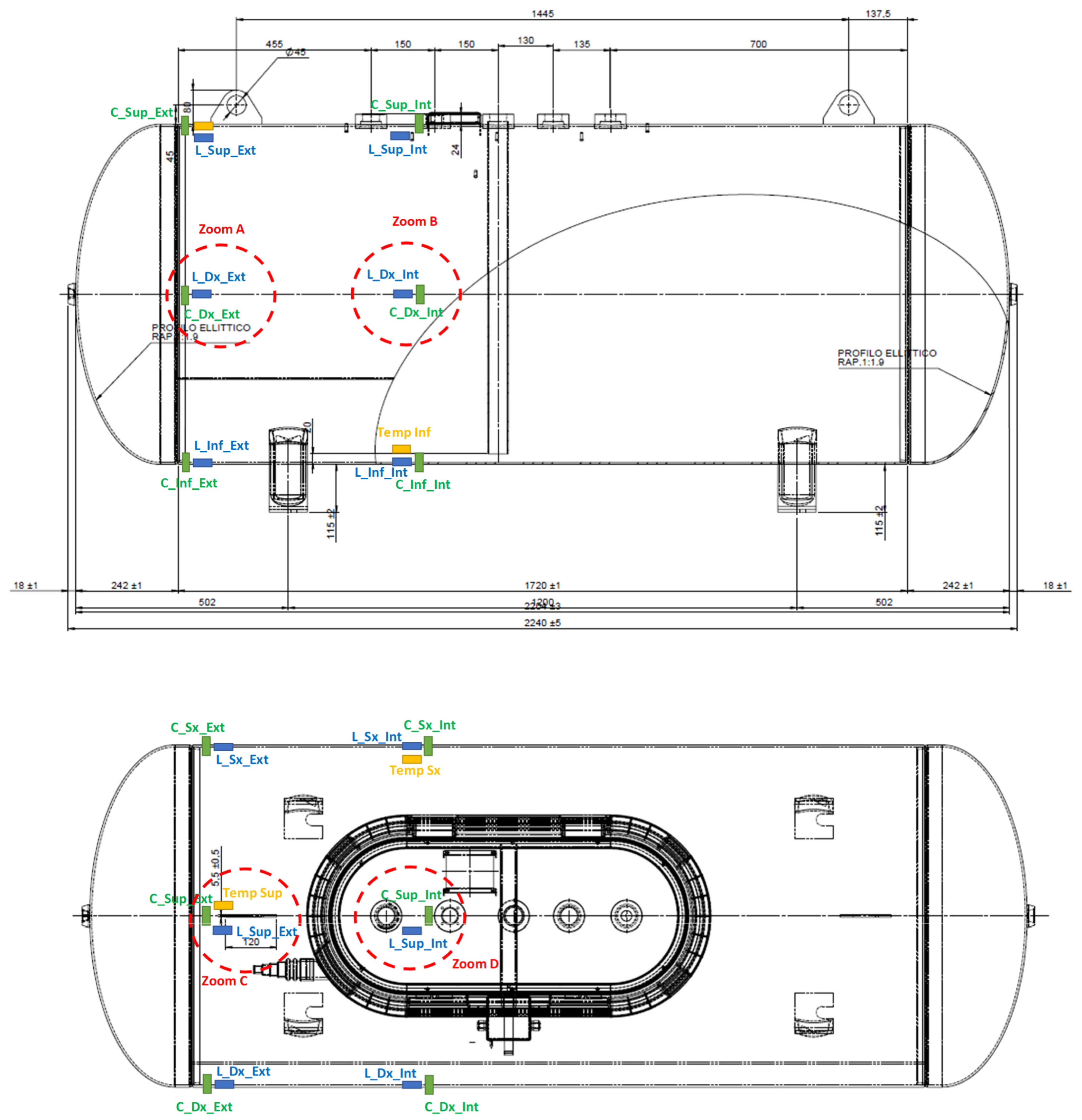
2.6. Experimental Campaign
- RSN-OUT-60
- RSA-IN-20
- RSA-IN-60
- RVA-IN-20
- RVA-IN-60
- RLA-IN-20
- RLA-IN-60
- Connect the empty hose and pump in 20 L of liquefied LPG. Wait for 120 s.
- Pump in 5 L of liquefied LPG. Wait for 120 s.
- Pump in the remaining quantity of LPG at a flow rate of .
3. Results
- The air pressure inside the tank before filling was varied between 1 and 7 , with step 0.2 . This choice was made to study the influence of this parameter on the flammability conditions. Only the initial condition was considered relevant with regard to the accident described in this work.
- The tank temperature when the burst occurred was varied between −25 °C and 22 °C, with step 1 °C. This choice allowed us to consider the influence of the non-standard filling procedure on the tank temperature [19].
4. Conclusions
- lammability Analysis: According to the analytical lumped system model, the air–LPG mixture did not reach global flammable conditions. Instead, the local assessment performed by means of computational fluid dynamics (CFD) simulations revealed the presence of flammable regions, particularly around the gas–liquid interface. However, given the absence of burnt areas found in the tank, and considering the small extension of the identified flammable region, the ignition of the mixture was not the primary cause of the burst. Still, the results show that both global and local analyses should be carried out to fully investigate failures.
- Mechanical Failure Analysis: A critical crack length of 58mm was identified, beyond which the tank would fail due to a combination of static and fatigue failure mechanisms. This finding, along with the presence of chevron marks, suggest that the burst was primarily caused by mechanical failure rather than an explosive mixture ignition.
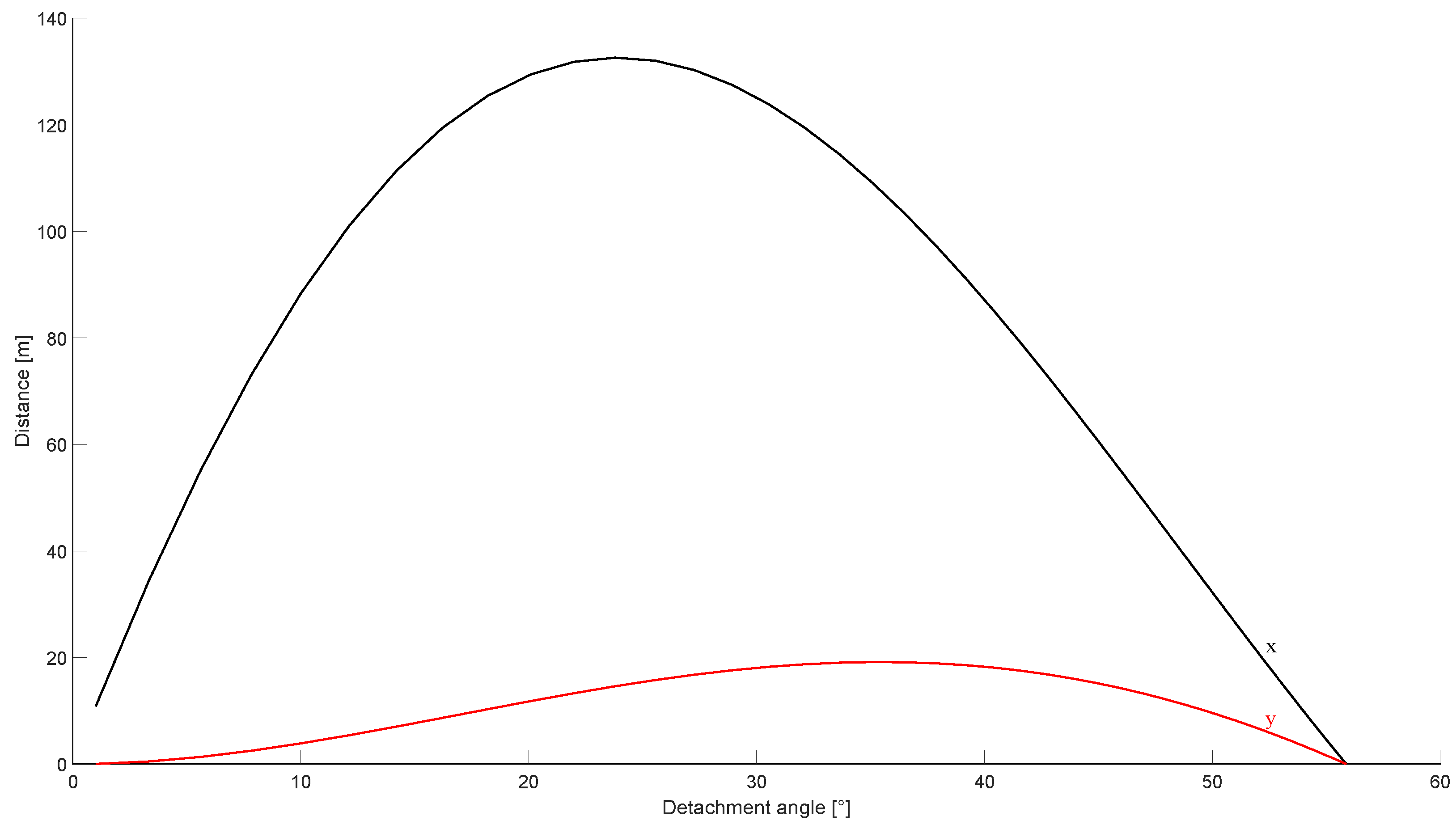
- Dynamic Response Analysis: The analytical model developed for describing the tank’s dynamics after the burst confirmed that the observed displacement of the operator’s body was consistent with the forces and moments generated by the sudden release of pressurized gases. This model validated the proposed scenario and demonstrated the plausibility of the observed accident dynamics.
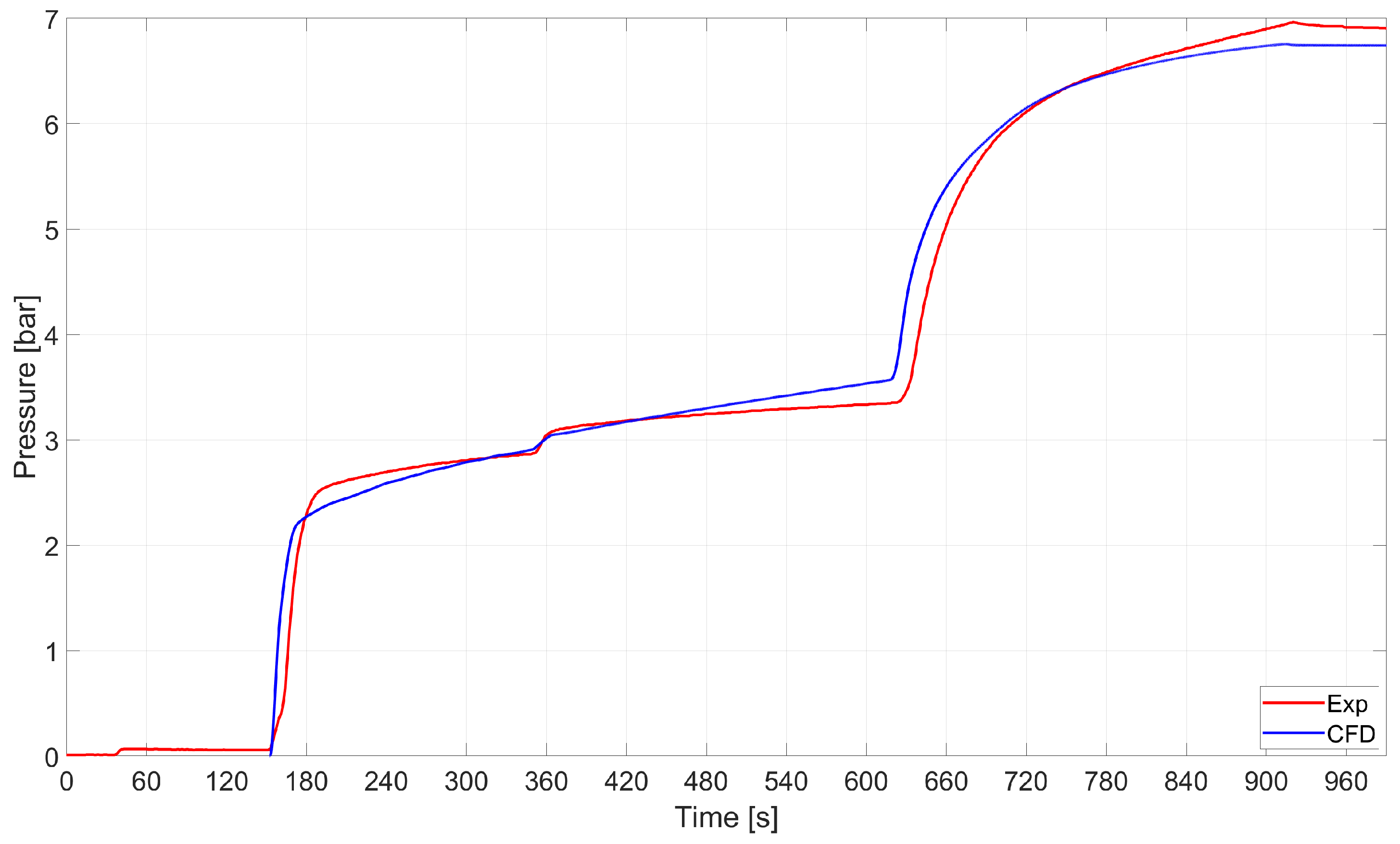
- Experimental Validation: Experiments were carried out to validate the developed models. The pressure and temperature data collected during controlled filling procedures matched the analytical and numerical results. Similarly, the gas mixture composition from the experiments was accurately predicted by the analytical lumped system model.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Arturson, C. Analysis of severe fire disasters. In The Management of Mass Burn Casualties and Fire Disasters: Proceedings of the First International Conference on Burns and Fire Disasters; Masellis, M., Gunn, S., Eds.; Springer: Dordrecht, The Netherlands, 1992; pp. 24–33. [Google Scholar] [CrossRef]
- Wang, K.; Hu, Q.; Qian, X.; Li, M.; Shi, T. Cause analysis and damage mechanism of explosive destruction with case investigation involving LPG tank trailer. Eng. Fail. Anal. 2022, 133, 106002. [Google Scholar] [CrossRef]
- Gamas, E.D.; Magdaleno, M.; Diaz, L.; Schifter, I.; Ontiveros, L.; Alvarez-Cansino, G. Contribution of Liquefied Petroleum Gas to Air Pollution in the Metropolitan Area of Mexico City. J. Air Waste Manag. Assoc. 2000, 50, 188–198. [Google Scholar] [CrossRef] [PubMed]
- Arturson, G. The tragedy of San Juanico—The most severe LPG disaster in history. Burns 1987, 13, 87–102. [Google Scholar] [CrossRef] [PubMed]
- Arturson, G. The los alfaques disaster: A boiling-liquid, expanding-vapour explosion. Burns 1981, 7, 233–251. [Google Scholar] [CrossRef]
- Lyu, S.; Zhang, S.; Huang, X.; Peng, S.; Li, J. Investigation and modeling of the LPG tank truck accident in Wenling, China. Process Saf. Environ. Prot. 2022, 157, 493–508. [Google Scholar] [CrossRef]
- Yang, D.; Peng, K.; Zheng, J.; Xie, B.; Wang, J.; Xu, B.; Li, F. Consequences analysis of the LPG tank truck traffic accident: A case study of the Wenling explosion accident. J. Loss Prev. Process Ind. 2024, 87, 105228. [Google Scholar] [CrossRef]
- Jiao, Y.; Chen, R.; Wang, K.; Fan, X.; Liu, F. Simulation of scenario characteristics of LPG tank explosion accident and its contribution to emergency planning: A case study. J. Loss Prev. Process Ind. 2023, 83, 105066. [Google Scholar] [CrossRef]
- Terzioglu, L.; Iskender, H. Modeling the consequences of gas leakage and explosion fire in liquefied petroleum gas storage tank in Istanbul technical university, Maslak campus. Process Saf. Prog. 2021, 40, 319–326. [Google Scholar] [CrossRef]
- Akpi, A.; MMom, P.; Olanrewaju, L. Liquefied Petroleum Gas Stations Disaster Risk Preparedness Assessment of Port Harcourt City, Nigeria. J. Risk Anal. Crisis Response 2023, 13, 209–219. [Google Scholar] [CrossRef]
- Wang, X.; Shen, X.; Qian, X.; Hu, Q.; Yuan, M.; Li, M.; Jiang, J. Case study of fire and explosion accident based on damage consequence and numerical results: Explosion medium traceability. Case Stud. Therm. Eng. 2023, 49, 103171. [Google Scholar] [CrossRef]
- Database of Weather Forecasts From 3B Meteo. Available online: https://www.3bmeteo.com/meteo/gravedona+ed+uniti/storico/201907 (accessed on 7 April 2020).
- Zabetakis, M.G. Flammability Characteristics of Combustible Gases and Vapors; Technical Report; Bureau of Mines: Pittsburgh, PA, USA, 1964. [Google Scholar]
- Lee, W.H. A pressure iteration scheme for two-phase flow modeling. Multiph. Transp. Fundam. React. Safety, Appl. 1980, 1, 407–431. [Google Scholar]
- Fluent, A. 2020R2 Theory Guide; ANSYS Inc.: Canonsburg, PA, USA, 2020. [Google Scholar]
- Lemmon, E.W. Thermophysical properties of fluid systems. NIST Chemistry WebBook. Available online: https://webbook.nist.gov/chemistry/fluid/ (accessed on 17 July 2024).
- BS 7910:2005; Guide to Methods for Assessing the Acceptability of Flaws in Metallic Structures. Institution, B.S.: London, UK, 2005.
- United States Department of Agriculture N.R.C.S. Soil Bulk Density Moisture Aeration. Available online: https://www.nrcs.usda.gov/sites/default/files/2022-10/Soil%20Bulk%20Density%20Moisture%20Aeration.pdf (accessed on 17 July 2024).
- ASSOGASLIQUIDI. PRIMO RIFORNIMENTO DI SERBATOI DESTINATI ALL’INSTALLAZIONE IN DEPOSITI DI GPL SOGGETTI AL D.M. 14.5.2004.









| Component | [K] | |||
|---|---|---|---|---|
| Propane | 2.1 | 9.5 | 298.15 | 101,325 |
| Butane | 1.9 | 8.5 | 298.15 | 101,325 |
| Component | [K] | [Pa] | [KJ/mol] |
|---|---|---|---|
| Propane | 293.15 | 14.79 | |
| Butane | 294.25 | 22.39 |
| Characteristic | Value | Units |
|---|---|---|
| No. Elements | 238.1 | cells |
| Minimum element size | 5 | mm |
| Maximum element size | 25 | mm |
| Minimum orthogonal quality | 0.257 | - |
| Maximum skewness | 0.584 | - |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lomazzi, L.; Passoni, S.; Mereu, R.; Cadini, F.; Giglio, M. In-Depth Analysis of the Burst of a Liquefied Petroleum Gas Tank in Gravedona, Italy. Energies 2024, 17, 3741. https://doi.org/10.3390/en17153741
Lomazzi L, Passoni S, Mereu R, Cadini F, Giglio M. In-Depth Analysis of the Burst of a Liquefied Petroleum Gas Tank in Gravedona, Italy. Energies. 2024; 17(15):3741. https://doi.org/10.3390/en17153741
Chicago/Turabian StyleLomazzi, Luca, Stefano Passoni, Riccardo Mereu, Francesco Cadini, and Marco Giglio. 2024. "In-Depth Analysis of the Burst of a Liquefied Petroleum Gas Tank in Gravedona, Italy" Energies 17, no. 15: 3741. https://doi.org/10.3390/en17153741
APA StyleLomazzi, L., Passoni, S., Mereu, R., Cadini, F., & Giglio, M. (2024). In-Depth Analysis of the Burst of a Liquefied Petroleum Gas Tank in Gravedona, Italy. Energies, 17(15), 3741. https://doi.org/10.3390/en17153741








