Research on Photovoltaic Power Generation Characteristics of Small Ocean Observation Unmanned Surface Vehicles
Abstract
1. Introduction
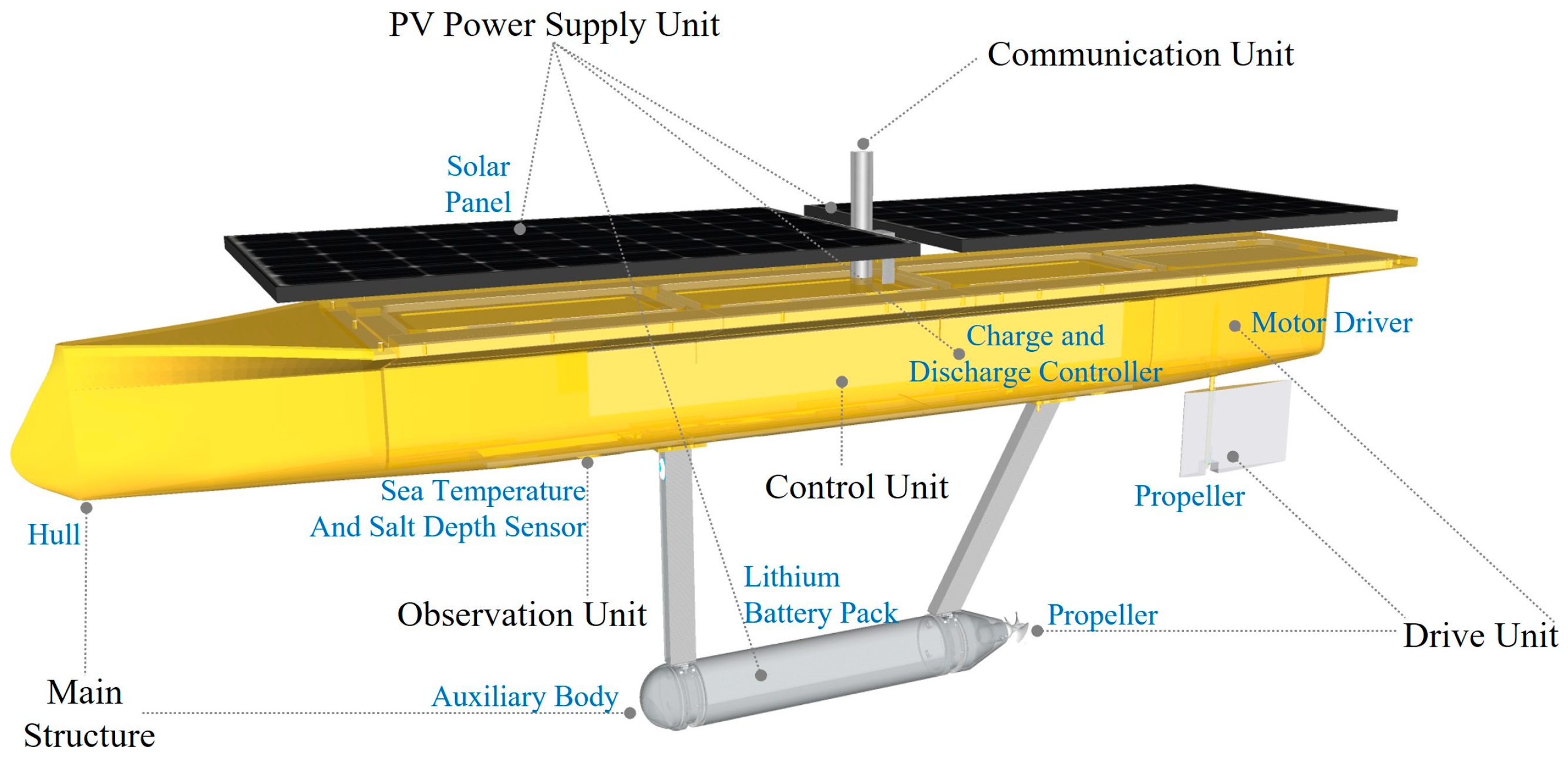
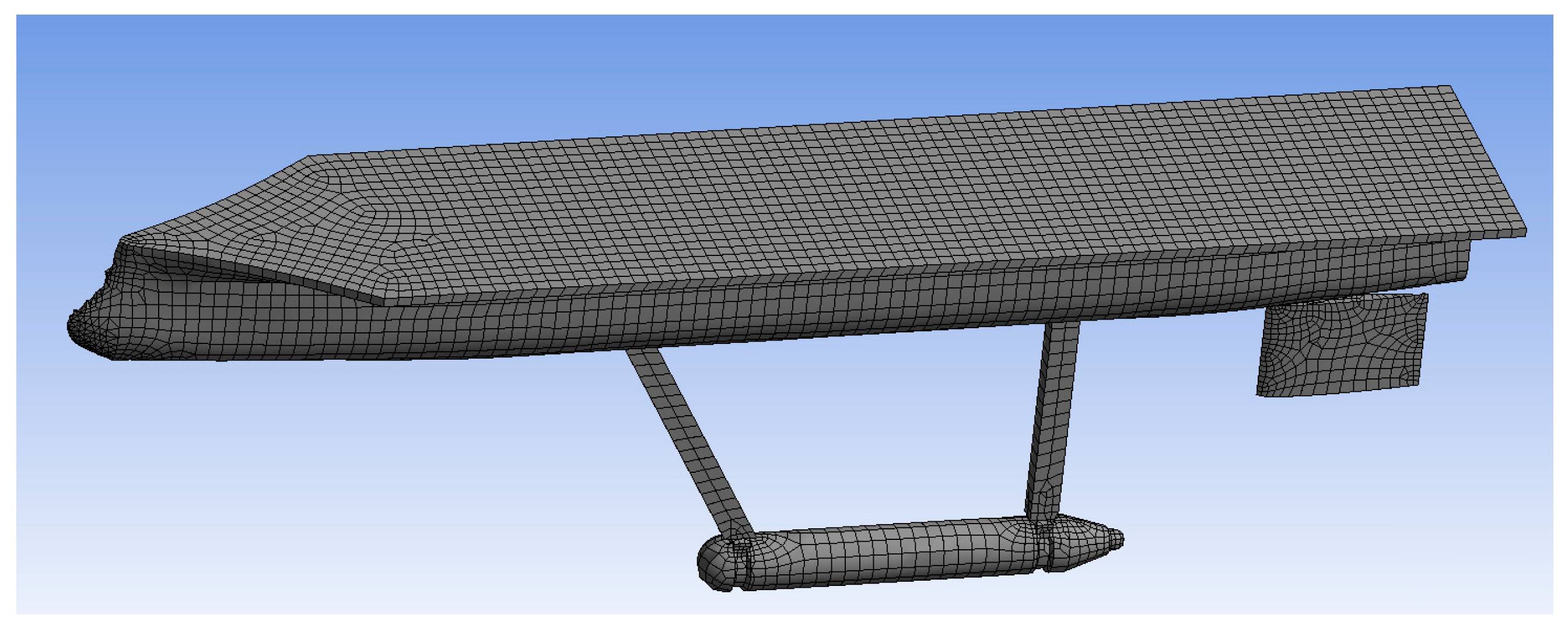
2. Small Long-Endurance USV
3. Simulation Model of USV PV System
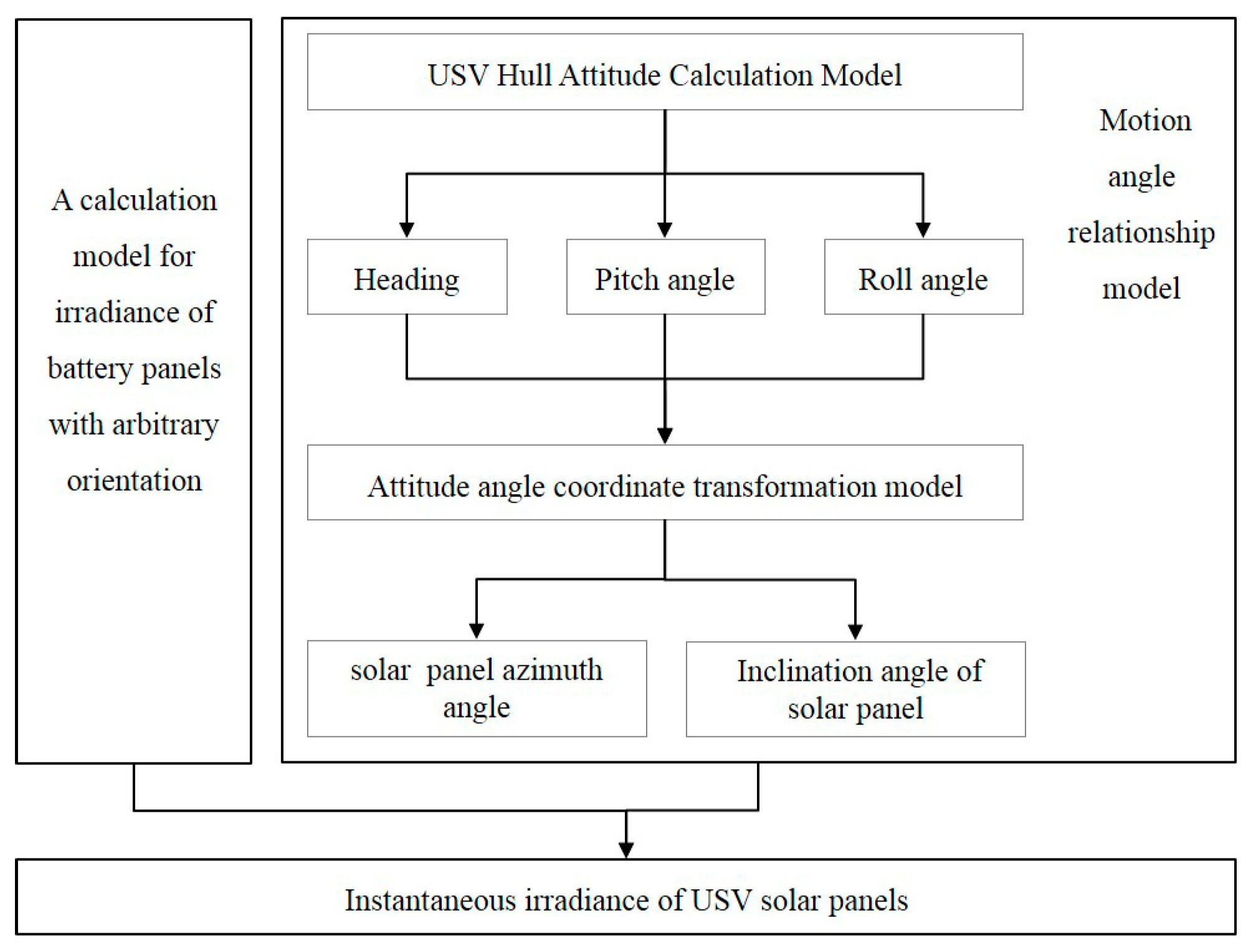
3.1. Irradiance Calculation Model
3.1.1. Irradiance Calculation Model of Arbitrary Orientation Panel
3.1.2. USV Attitude Calculation Model
3.1.3. Attitude Angle Coordinate Transformation Model
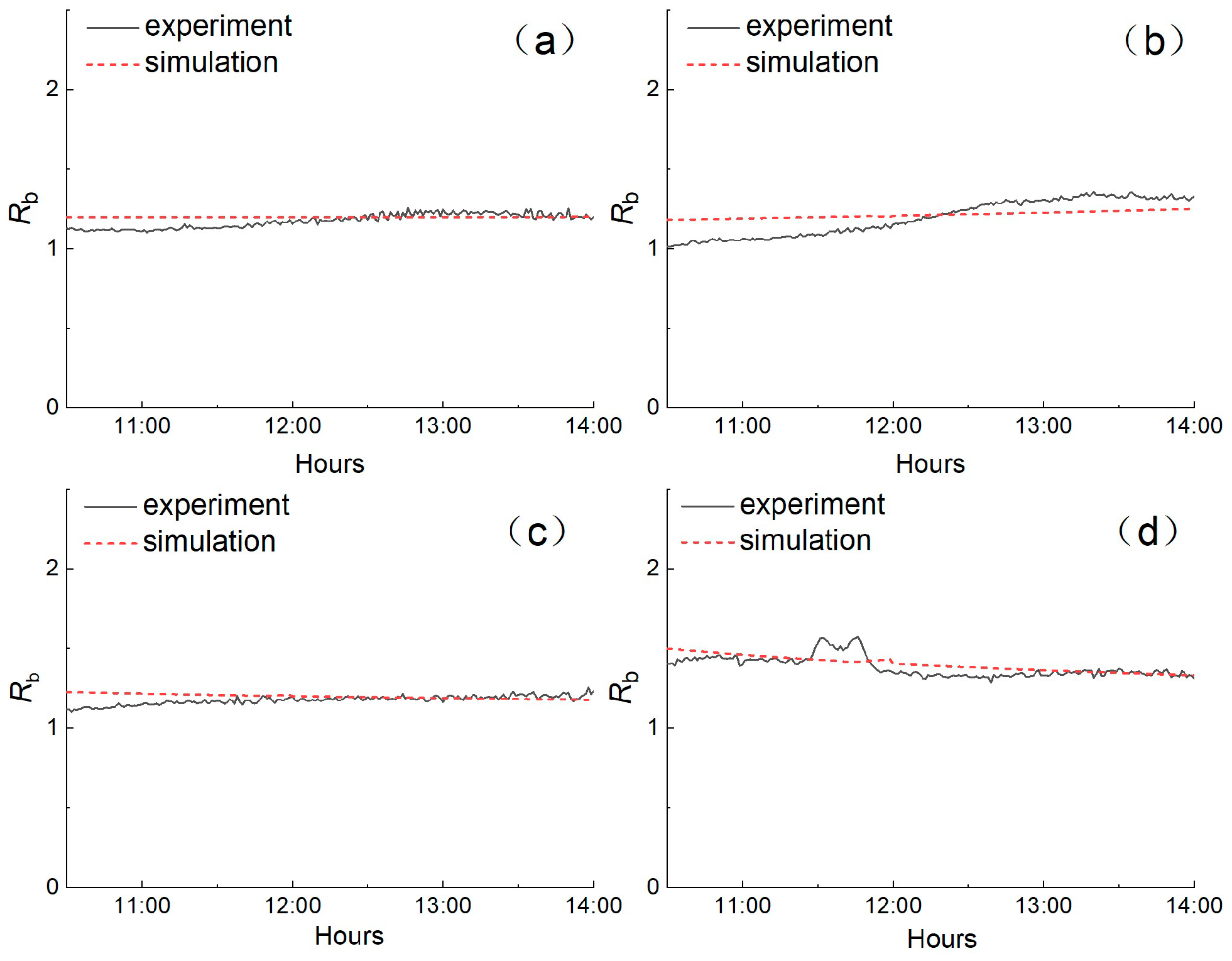
3.1.4. Computational Model Verification
3.2. Photovoltaic Power System
3.2.1. Power System and Simulation Model
3.2.2. Experimental Verification
4. Irradiance Characteristics of USV PV Modules under Typical Wave Conditions
4.1. Simulation Condition
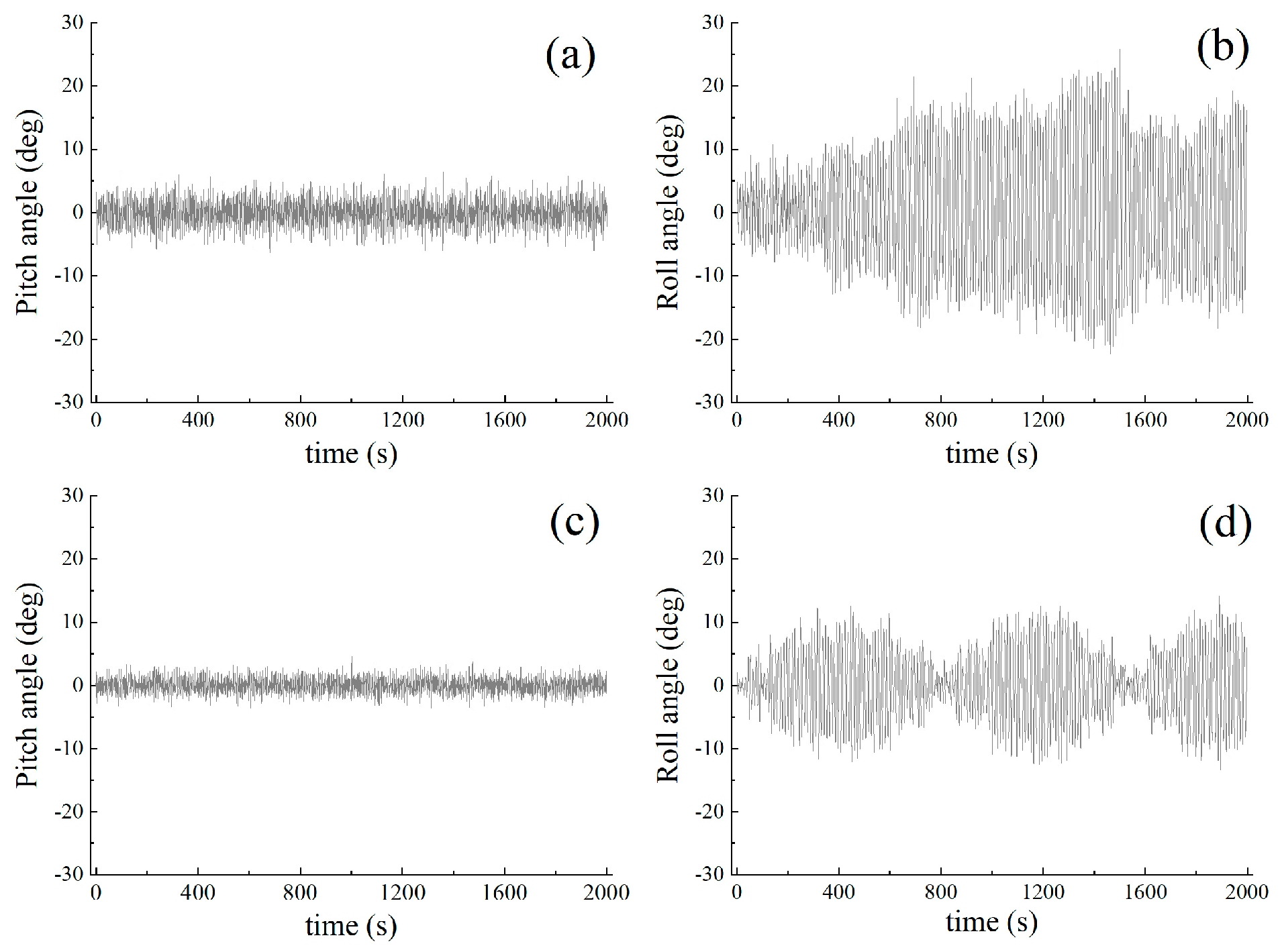
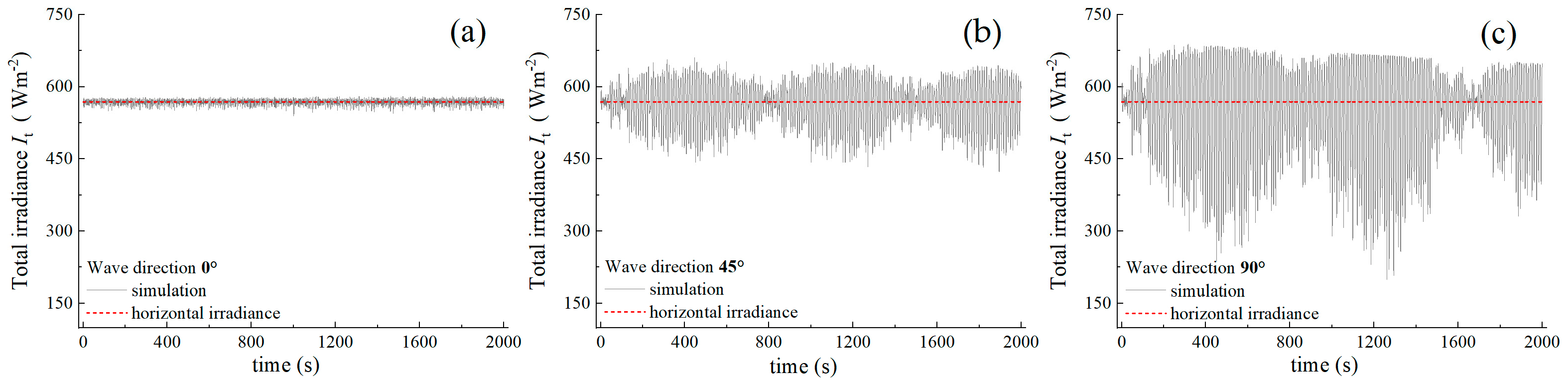
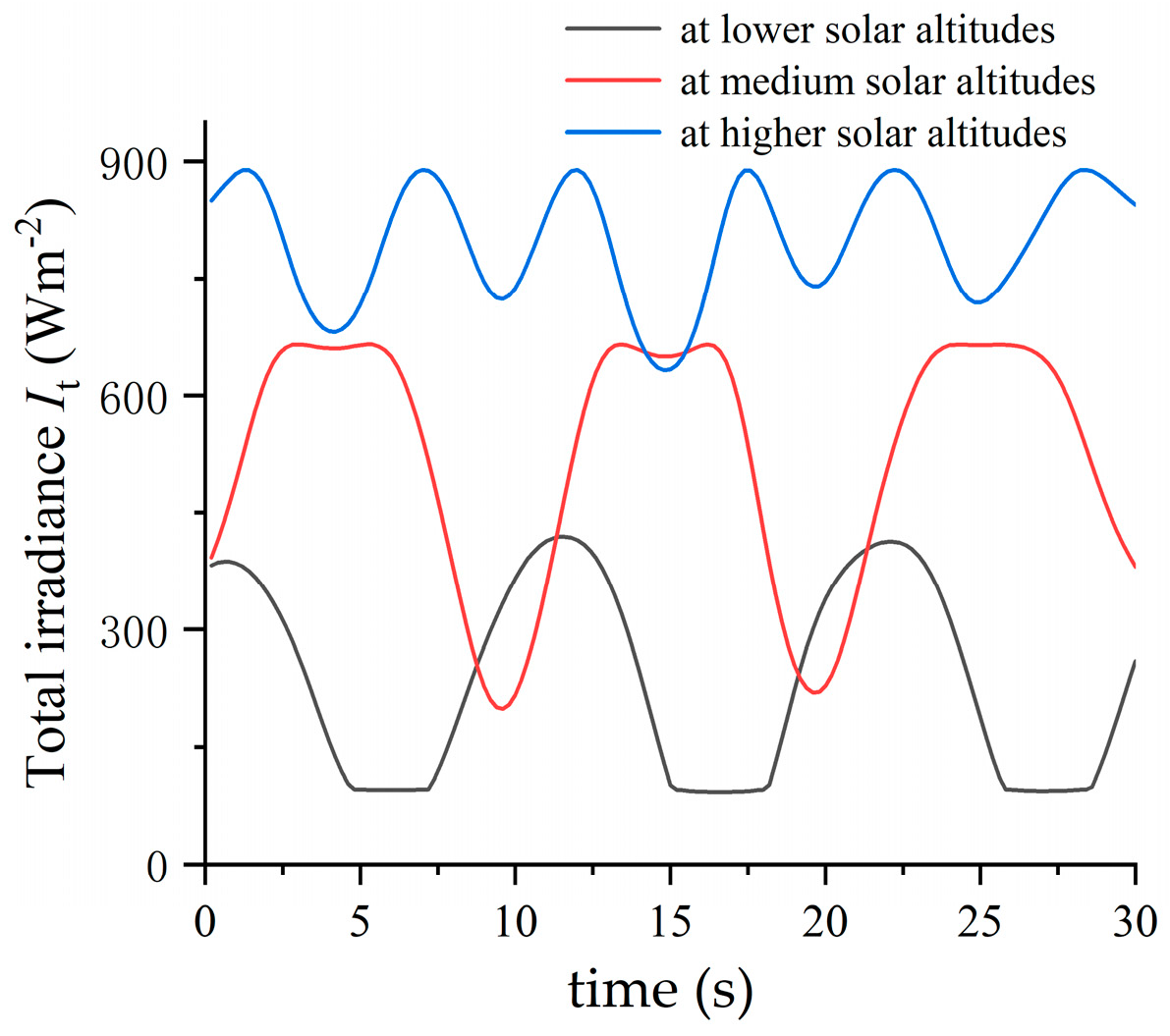
4.2. Effect of Waves on Irradiance at Lower Solar Altitude Angles
4.3. Effect of Waves on Irradiance at Medium Solar Altitude Angle
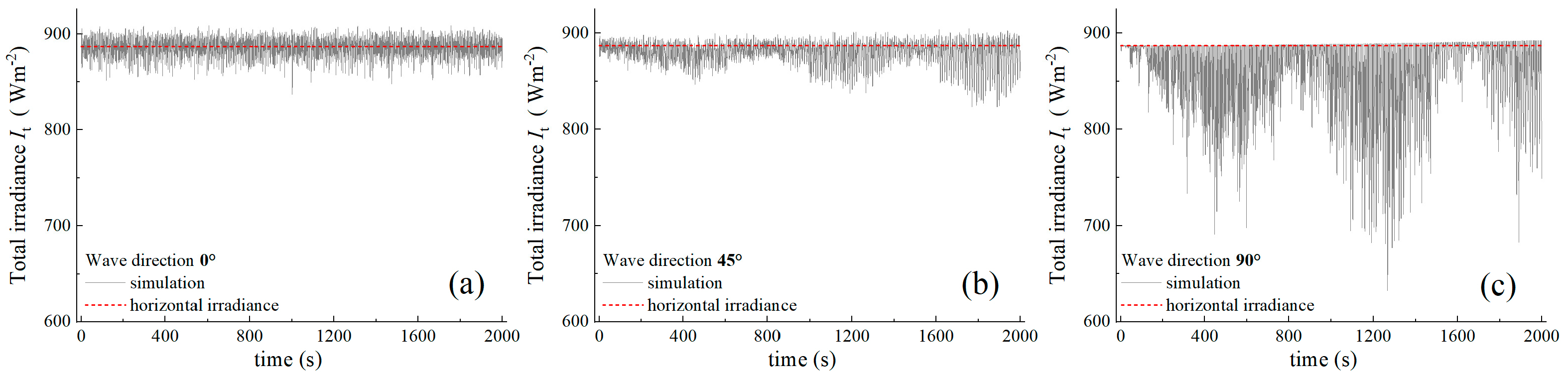
4.4. Effect of Waves on Irradiance at Higher Solar Altitude Angle
5. Characteristics of Photovoltaic MPPT under Wave Excitation
5.1. Changes in Irradiance
5.2. Simulation Analysis of MPPT under Wave Influence
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| CFD | Computational Fluid Dynamics |
| FOCV | fractional open circuit voltage |
| MPP | maximum power point |
| MPPT | maximum power point tracking |
| PV | photovoltaic |
| PWM | Pulse-Width Modulation |
| P&O | Perturb and Observe |
| USV | unmanned surface vehicle |
| A | sun’s azimuth (deg) |
| CR | rotational motion damping (N·m·s/rad) |
| Cu | translational motion damping (N·s/m) |
| Fc | the resistance around the flow (N) |
| Fb | the buoyancy force of USV (N) |
| Fw | indicates wave force (N) |
| G | the gravity of USV (N) |
| h° | the altitude angle of the sun (deg) |
| I | the total irradiance of the horizontal plane (W/m2) |
| Ib | horizontal direct irradiance (W/m2) |
| Id | horizontal scattered irradiance (W/m2) |
| It | the total irradiance of the surface of the solar panel (W/m2) |
| Itb | direct radiation (W/m2) |
| Itd | scattered radiation (W/m2) |
| Itr | reflected radiation (W/m2) |
| J | the moment of inertia (N·m) |
| ΔJ | the additional moment of inertia (N·m) |
| KR | rotational motion damping stiffness (N·m/rad) |
| Ku | translational motion damping stiffness (N/m) |
| M | the mass of USV (kg) |
| ΔM | the additional mass of USV (kg) |
| Pi | the instantaneous output power of the photovoltaic panel at a certain moment (W) |
| the high-frequency oscillation component of the output power at that moment (W) | |
| R | the motion variable of USV (rad) |
| Rb | radiation factor (W/m2) |
| Rx | the roll angle of the solar panel (deg) |
| Ry | the pitch angle of the solar panel (deg) |
| Rz | the yaw angle of the solar panel (deg) |
| Tc | flow resistance moment (N·m) |
| Tb | the buoyancy moment of USV (N·m) |
| Tw | wave action moment (N·m) |
| ui | the displacement along the coordinate axis of USV (m) |
| β | the solar panel inclination angle (deg) |
| γ | the solar panel azimuth angle (deg) |
| δ | the declination angle of the sun on the observed day (deg) |
| z | the time from noon (deg) |
| ρ | the reflection factor (deg) |
| φ | the latitude of the measurement site (deg) |
| ω | time angle (deg) |
References
- Cokelet, E.D.; Meinig, C.; Lawrence-Slavas, N.; Stabeno, P.J.; Mordy, C.W.; Tabisola, H.M.; Jenkins, R.; Cross, J.N. The use of Saildrones to examine spring conditions in the Bering sea. In Proceedings of the OCEANS 2015-MTS/IEEE Washington, Washington, DC, USA, 19–22 October 2015; pp. 1–7. [Google Scholar]
- Wang, P.; Wang, D.; Zhang, X.; Li, X.; Peng, T.; Lu, H.; Tian, X. Numerical and experimental study on the maneuverability of an active propeller control based wave glider. Appl. Ocean Res. 2020, 104, 102369. [Google Scholar] [CrossRef]
- Setiawan, F.; Kadir, R.; Gamayanti, N.; Santoso, A.; Bilfaqih, Y.; Hidayat, Z. Dynamic modelling and controlling unmanned surface vehicle. IOP Conf. Ser. Earth Environ. Sci. 2021, 649, 012056. [Google Scholar] [CrossRef]
- Setiawan, J.D.; Septiawan, M.A.; Ariyanto, M.; Caesarendra, W.; Munadi, M.; Alimi, S.; Sulowicz, M. Development and performance measurement of an affordable unmanned surface vehicle (USV). Automation 2022, 3, 27–46. [Google Scholar] [CrossRef]
- Liu, P.; Su, Y.-m.; Liao, Y.-l. Numerical and experimental studies on the propulsion performance of a Wave Glide propulsor. China Ocean Eng. 2016, 30, 393–406. [Google Scholar] [CrossRef]
- Yang, T.; Guo, Y.; Zhou, Y.; Wei, S. Joint communication and control for small underactuated USV based on mobile computing technology. IEEE Access 2019, 7, 160610–160622. [Google Scholar] [CrossRef]
- Leifeng, W.; Ye, L.; Yulei, L.; Kaiwen, P.; Weixin, Z. Adaptive heading control of unmanned wave glider with heading information fusion. Control Eng. Pract. 2019, 85, 216–224. [Google Scholar] [CrossRef]
- Liu, Y.; Lin, M.; Jiang, X.; Sun, X.; Song, X. A comparison of multiplatform wind products in the South China Sea during summer and autumn in 2019. J. Oceanol. Limnol. 2021, 39, 2181–2194. [Google Scholar] [CrossRef]
- Li, C.; Sang, H.; Sun, X.; Qi, Z. Hydrographic and meteorological observation demonstration with wave glider “black pearl”. In Proceedings of the Intelligent Robotics and Applications: 10th International Conference, ICIRA 2017, Wuhan, China, 16–18 August 2017; Proceedings, Part I 10. pp. 790–800. [Google Scholar]
- Yunisa, Y.; Zhimwang, J.T.; Aminu, I.; Shaka, O.S.; Frank, L.M. Design and Construction of 5KVA Solar Power Inverter System. Int. J. Adv. Eng. Manag. 2022, 4, 1355–1358. [Google Scholar]
- Asnil, K.; Husnaini, I.; Astrid, E. Design and Performance of Dual Axis Solar Tracker Based on Light Sensors to Maximize the Photovoltaic Energy Output. J. Theor. Appl. Inf. Technol. 2022, 100, 6554–6564. [Google Scholar]
- Eke, R.; Senturk, A. Performance comparison of a double-axis sun tracking versus fixed PV system. Sol. Energy 2012, 86, 2665–2672. [Google Scholar] [CrossRef]
- Fathi, M.; Amjadifard, R.; Eshghi, F.; Kelarestaghi, M. Design and implementation of a novel multi-faceted-efficient pneumatic dual-axis solar tracker. World J. Eng. 2024, 21, 254–266. [Google Scholar] [CrossRef]
- Ye, L.; Sun, H.B.; Song, X.R.; Li, L.C. Dynamic modeling of a hybrid wind/solar/hydro microgrid in EMTP/ATP. Renew. Energy 2012, 39, 96–106. [Google Scholar] [CrossRef]
- Motahhir, S.; El Hammoumi, A.; El Ghzizal, A. The most used MPPT algorithms: Review and the suitable low-cost embedded board for each algorithm. J. Clean. Prod. 2020, 246, 118983. [Google Scholar] [CrossRef]
- Robles Algarín, C.; Taborda Giraldo, J.; Rodriguez Alvarez, O. Fuzzy logic based MPPT controller for a PV system. Energies 2017, 10, 2036. [Google Scholar] [CrossRef]
- Bollipo, R.B.; Mikkili, S.; Bonthagorla, P.K. Hybrid, optimal, intelligent and classical PV MPPT techniques: A review. CSEE J. Power Energy Syst. 2020, 7, 9–33. [Google Scholar]
- Karami, N.; Moubayed, N.; Outbib, R. General review and classification of different MPPT Techniques. Renew. Sustain. Energy Rev. 2017, 68, 1–18. [Google Scholar] [CrossRef]
- Sumathi, V.; Jayapragash, R.; Bakshi, A.; Akella, P.K. Solar tracking methods to maximize PV system output—A review of the methods adopted in recent decade. Renew. Sustain. Energy Rev. 2017, 74, 130–138. [Google Scholar] [CrossRef]
- Subudhi, B.; Pradhan, R. A comparative study on maximum power point tracking techniques for photovoltaic power systems. IEEE Trans. Sustain. Energy 2012, 4, 89–98. [Google Scholar] [CrossRef]
- Podder, A.K.; Roy, N.K.; Pota, H.R. MPPT methods for solar PV systems: A critical review based on tracking nature. IET Renew. Power Gener. 2019, 13, 1615–1632. [Google Scholar] [CrossRef]
- Bendib, B.; Belmili, H.; Krim, F. A survey of the most used MPPT methods: Conventional and advanced algorithms applied for photovoltaic systems. Renew. Sustain. Energy Rev. 2015, 45, 637–648. [Google Scholar] [CrossRef]
- El Gouri, R.; Brahim, M.; Hlou, L. A Comparative Study of Mppt Technical Based on Fuzzy Logic and Perturb Observe Algorithms for Photovoltaic Systems. J. Theor. Appl. Inf. Technol. 2013, 58, 337–346. [Google Scholar]
- Rezk, H.; Eltamaly, A.M. A comprehensive comparison of different MPPT techniques for photovoltaic systems. Sol. Energy 2015, 112, 1–11. [Google Scholar] [CrossRef]
- Esram, T.; Chapman, P.L. Comparison of photovoltaic array maximum power point tracking techniques. IEEE Trans. Energy Convers. 2007, 22, 439–449. [Google Scholar] [CrossRef]
- Krishnaram, K.; Sivamani, S.; Alaas, Z.; Ahmed, M.; Senthilkumar, S.; Raj, S.A. Experimental design and analysis of advanced three phase converter for PV application with WCO-P&O MPPT controller. Sci. Rep. 2024, 14, 10984. [Google Scholar] [PubMed]
- Dolara, A.; Faranda, R.; Leva, S. Energy comparison of seven MPPT techniques for PV systems. J. Electromagn. Anal. Appl. 2009, 2009, 725. [Google Scholar] [CrossRef]
- Chen, P.-C.; Chen, P.-Y.; Liu, Y.-H.; Chen, J.-H.; Luo, Y.-F. A comparative study on maximum power point tracking techniques for photovoltaic generation systems operating under fast changing environments. Sol. Energy 2015, 119, 261–276. [Google Scholar] [CrossRef]
- Lagdani, O.; Trihi, M.; Bossoufi, B. PV array connected to the grid with the implementation of MPPT algorithms (INC, P&O and FL method). Int. J. Power Electron. Drive Syst. 2019, 10, 2084. [Google Scholar]
- Sharmin, R.; Chowdhury, S.S.; Abedin, F.; Rahman, K.M. Implementation of an MPPT technique of a solar module with supervised machine learning. Front. Energy Res. 2022, 10, 932653. [Google Scholar] [CrossRef]
- Manna, S.; Singh, D.K.; Akella, A.K.; Kotb, H.; AboRas, K.M.; Zawbaa, H.M.; Kamel, S. Design and implementation of a new adaptive MPPT controller for solar PV systems. Energy Rep. 2023, 9, 1818–1829. [Google Scholar] [CrossRef]
- Hassan, A.; Bass, O.; Masoum, M.A. An improved genetic algorithm based fractional open circuit voltage MPPT for solar PV systems. Energy Rep. 2023, 9, 1535–1548. [Google Scholar] [CrossRef]
- Hai, T.; Aksoy, M.; Nishihara, K. Optimized MPPT model for different environmental conditions to improve efficacy of a photovoltaic system. Soft Comput. 2024, 28, 2161–2179. [Google Scholar] [CrossRef]
- Mbarki, B.; Fethi, F.; Chrouta, J.; Zaafouri, A. Adaptive neuro-fuzzy inference system algorithm-based robust terminal sliding mode control MPPT for a photovoltaic system. Trans. Inst. Meas. Control 2024, 46, 316–325. [Google Scholar] [CrossRef]
- Chellakhi, A.; Beid, S.E.; Abouelmahjoub, Y. A novel theta MPPT approach based on adjustable step size for photovoltaic system applications under various atmospheric conditions. Energy Syst. 2024, 15, 89–114. [Google Scholar] [CrossRef]
- Ibrahim, O.; Yahaya, N.Z.; Saad, N.; Umar, M.W. Matlab/Simulink model of solar PV array with perturb and observe MPPT for maximising PV array efficiency. In Proceedings of the 2015 IEEE conference on energy conversion (CENCON), Johor Bahru, Malaysia, 19–20 October 2015; pp. 254–258. [Google Scholar]
- De Dieu Nguimfack-Ndongmo, J.; Harrison, A.; Alombah, N.H.; Kuate-Fochie, R.; Asoh, D.A.; Kenné, G. Adaptive terminal synergetic-backstepping technique based machine learning regression algorithm for MPPT control of PV systems under real climatic conditions. ISA Trans. 2024, 145, 423–442. [Google Scholar] [CrossRef]
- Yadav, I.; Sachan, S.; Gholian-Jouybari, F.; Maurya, S.K.; Hajiaghaei-Keshteli, M. A performance analysis of step-voltage and step-duty size-based MPPT controller used for solar PV applications. Soft Comput. 2024, 28, 7465–7479. [Google Scholar] [CrossRef]
- Kolakaluri, V.K.; Aalam, M.N.; Sarkar, V. Sampling Time Modulation of a Photovoltaic Power Tracking Controller Based upon Real-time Monitoring of Converter Dynamics. IEEE Trans. Power Electron. 2023, 39, 2822–2834. [Google Scholar] [CrossRef]
- Schmidt, H.; Burger, B.; Bussemas, U.; Elies, S. How fast does an MPP tracker really need to be? In Proceedings of the 24th European Photovoltaic Solar Energy Conference and Exhibition, Hamburg, Germany, 21–25 September 2009. [Google Scholar]
- Rojas, C.A.; Kouro, S.; Inzunza, R.; Mitsugi, Y.; Alcaide, A.M. Harmonic impedance model of multiple utility-interactive multilevel photovoltaic inverters. Energies 2022, 15, 9462. [Google Scholar] [CrossRef]
- de Jesus, V.M.R.; Cupertino, A.F.; Xavier, L.S.; Pereira, H.A.; Mendes, V.F. Comparison of MPPT strategies in three-phase photovoltaic inverters applied for harmonic compensation. IEEE Trans. Ind. Appl. 2019, 55, 5141–5152. [Google Scholar] [CrossRef]
- Hou, T.; Wang, S. Research on the MPPT of Photovoltaic Power Generation Based on the CSA-INC Algorithm. Energy Eng. 2023, 120, 87–106. [Google Scholar] [CrossRef]
- Manoharan, P.; Subramaniam, U.; Babu, T.S.; Padmanaban, S.; Holm-Nielsen, J.B.; Mitolo, M.; Ravichandran, S. Improved perturb and observation maximum power point tracking technique for solar photovoltaic power generation systems. IEEE Syst. J. 2020, 15, 3024–3035. [Google Scholar] [CrossRef]
- Ahmed, M.; Harbi, I.; Kennel, R.; Abdelrahem, M. Maximum Power Point Tracking Implementation under Partial Shading Conditions Using Low-Cost Photovoltaic Emulator. Eng 2022, 3, 424–438. [Google Scholar] [CrossRef]
- Soulatiantork, P. Experimental Performance Evaluation of P&O and IC MPPT Algorithms for Photovoltaic Systems under Constant and Variable Environmental Conditions. Int. J. Energy Eng. 2020, 10, 46–58. [Google Scholar]
- Soon, T.K.; Mekhilef, S. A fast-converging MPPT technique for photovoltaic system under fast-varying solar irradiation and load resistance. IEEE Trans. Ind. Inform. 2014, 11, 176–186. [Google Scholar] [CrossRef]
- Paulescu, E.; Paulescu, M. A Semi-Analytical Model for Separating Diffuse and Direct Solar Radiation Components. Appl. Sci. 2022, 12, 12759. [Google Scholar] [CrossRef]
- El Mghouchi, Y.; El Bouardi, A.; Choulli, Z.; Ajzoul, T. Models for obtaining the daily direct, diffuse and global solar radiations. Renew. Sustain. Energy Rev. 2016, 56, 87–99. [Google Scholar] [CrossRef]
- Li, Z.H.Y.; Zhang, X.S. Research on seakeeping performance of catamaran based on potential flow model. Ocean Eng. 2023, 41, 57–67. [Google Scholar]
- Niu, J. Research on Seakeeping Performance of Longitudinal Motion of SWATH Ship. Master’s Thesis, Dalian University of Technology, Dalian, Chian, 2021. [Google Scholar]
- Wang, B.Z.; Zhang, Y.F.; Chen, X.Y. Study on Characteristics of 30 kW PV system in ZHONGSHAN Station, Antarctica. Acta Energiae Solaris Sin. 2021, 42, 272–277. [Google Scholar]





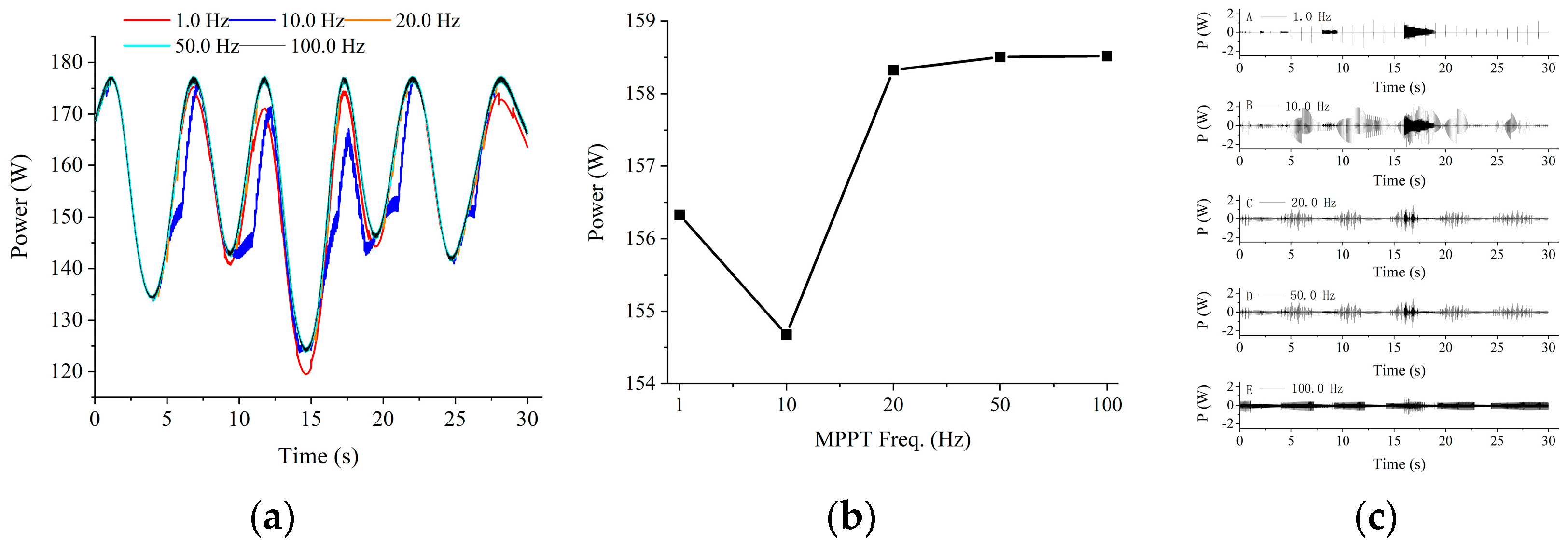

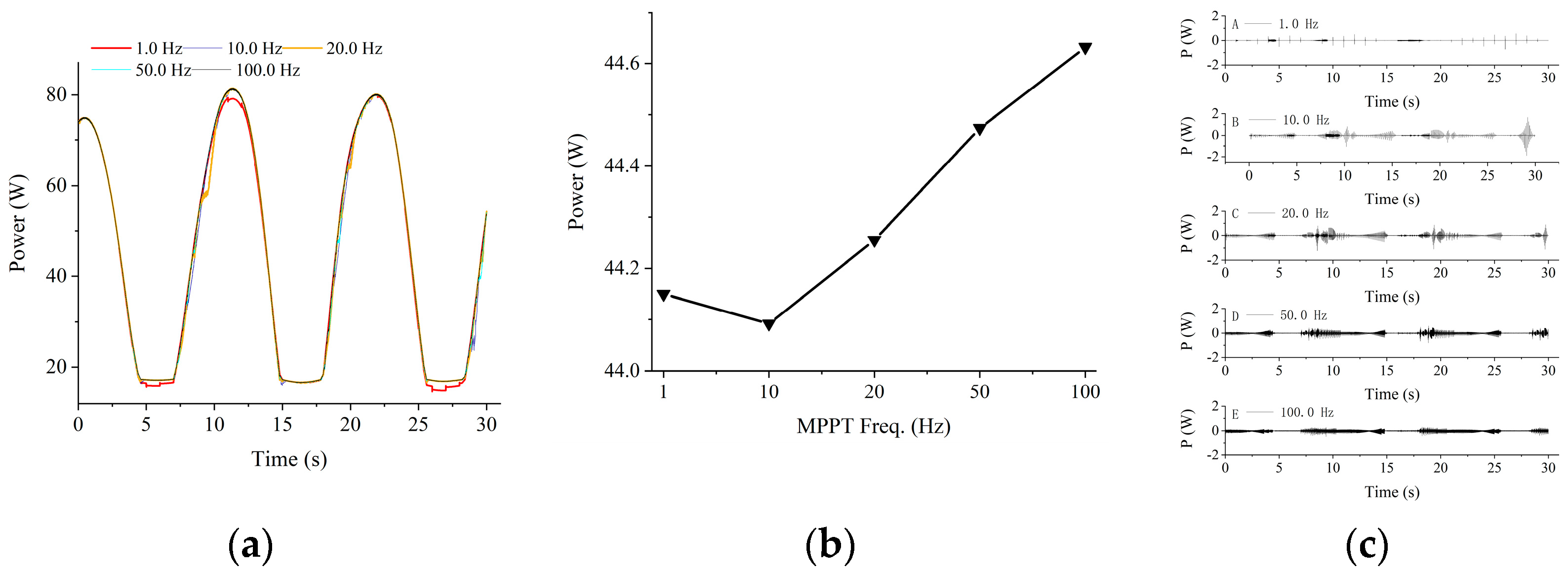
| Working Condition | Speed/Knot | Significant Wave Height/m | Spectrum Peak Period/s | Wave Direction/deg | Wave Condition |
|---|---|---|---|---|---|
| 1 | 1 | 1.5 | 8 | 0 | Level 4 |
| 2 | 1 | 1.5 | 8 | 45 | Level 4 |
| 3 | 1 | 1.5 | 8 | 90 | Level 4 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, W.; Wang, B.; Ke, W.; Shen, S.; Wu, X. Research on Photovoltaic Power Generation Characteristics of Small Ocean Observation Unmanned Surface Vehicles. Energies 2024, 17, 3699. https://doi.org/10.3390/en17153699
Yang W, Wang B, Ke W, Shen S, Wu X. Research on Photovoltaic Power Generation Characteristics of Small Ocean Observation Unmanned Surface Vehicles. Energies. 2024; 17(15):3699. https://doi.org/10.3390/en17153699
Chicago/Turabian StyleYang, Weiwei, Bingzhen Wang, Wei Ke, Shuyuan Shen, and Xiao Wu. 2024. "Research on Photovoltaic Power Generation Characteristics of Small Ocean Observation Unmanned Surface Vehicles" Energies 17, no. 15: 3699. https://doi.org/10.3390/en17153699
APA StyleYang, W., Wang, B., Ke, W., Shen, S., & Wu, X. (2024). Research on Photovoltaic Power Generation Characteristics of Small Ocean Observation Unmanned Surface Vehicles. Energies, 17(15), 3699. https://doi.org/10.3390/en17153699





