Study on Compatibility Evaluation of Multilayer Co-Production to Enhance Recovery of Water Flooding in Oil Reservoir
Abstract
1. Introduction
2. Experiment
2.1. Materials
2.2. Experimental Model Design
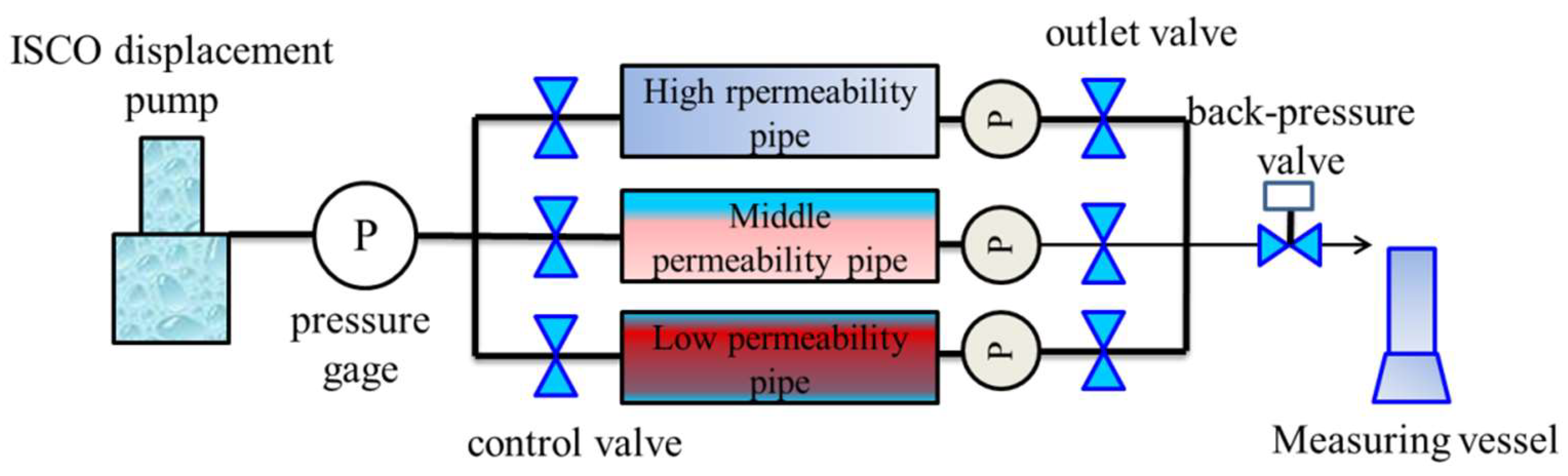
2.3. Experimental Procedure
- (1)
- Quartz sand of different particle sizes is mixed in a certain proportion and placed in a sand-filling pipe to obtain a sand-filling pipe core model. If the proportion of quartz sand particle size is different, the permeability of the sand-filling pipe core model is different. By changing the filling proportion of quartz sand particle size, other sand-filling pipe core models are obtained in turn.
- (2)
- Wash oil, dry, and weigh the sand-filled pipe. The porosity and permeability of each sand-filled tube are measured, respectively.
- (3)
- The sand-filled tubes were pumped out and saturated with salt water, then the effective volume of the pores was obtained, and the absolute liquid phase permeability of each tube was measured. The size and permeability of the sand-filled tubes are shown in Table 2.
- (4)
- The above saltwater-saturated pipes were displaced with crude oil. Data were recorded during the displacing process until only crude oil was produced at the liquid-producing end. At this time, the bound water saturation of each pipe was obtained, and the oil phase permeability under this saturation was measured.
- (5)
- The viscosity of crude oil is 5 mPa·s and the viscosity of aqueous solution is 1.50 mPa·s.
- (6)
- Three sand-filled pipe models were connected in parallel and water flooding was carried out. A fixed flow rate of 3 mL/min was maintained for displacement until the water content at the outlet of the high-permeability layer reached more than 98%. Liquid production and oil production at the outlet of each sand-filled pipe were collected and recorded, and the contribution rate of liquid production at the low-permeability layer was calculated.
- (7)
- Parallel models with different differences were replaced and the experiment was repeated. The physical simulation experimental device is shown in Figure 2.
| Scheme | Permeability Max-Min Ratio | Permeability (mD) | ||
|---|---|---|---|---|
| High-Permeability Layer | Middle-Permeability Layer | Low-Permeability Layer | ||
| 1 | 2.8 | 136.4 | 92.7 | 48.9 |
| 2 | 4.2 | 207.9 | 135.2 | 49.5 |
| 3 | 5.1 | 255.51 | 153.1 | 50.1 |
| 4 | 6.2 | 308.14 | 180.5 | 49.7 |
| 5 | 7.4 | 369.26 | 212.58 | 49.9 |
| Length (cm) | 50.98~51.23 | |||
| Diameter (cm) | 3.80 | |||
2.4. Experimental Results
3. Establishment of Theoretical Model
3.1. Assumptions
- ①
- Constant injection fluid volume, injection, and production balance.
- ②
- Rigid porous media, rock, and fluid are incompressible.
- ③
- Non-piston displacement creates an oil–water two-phase zone.
- ④
- In the stable compartment, interlayer channeling is not considered.
- ⑤
- Starting pressure gradient is not taken into account.
3.2. Governing Equation
3.3. Model Solving
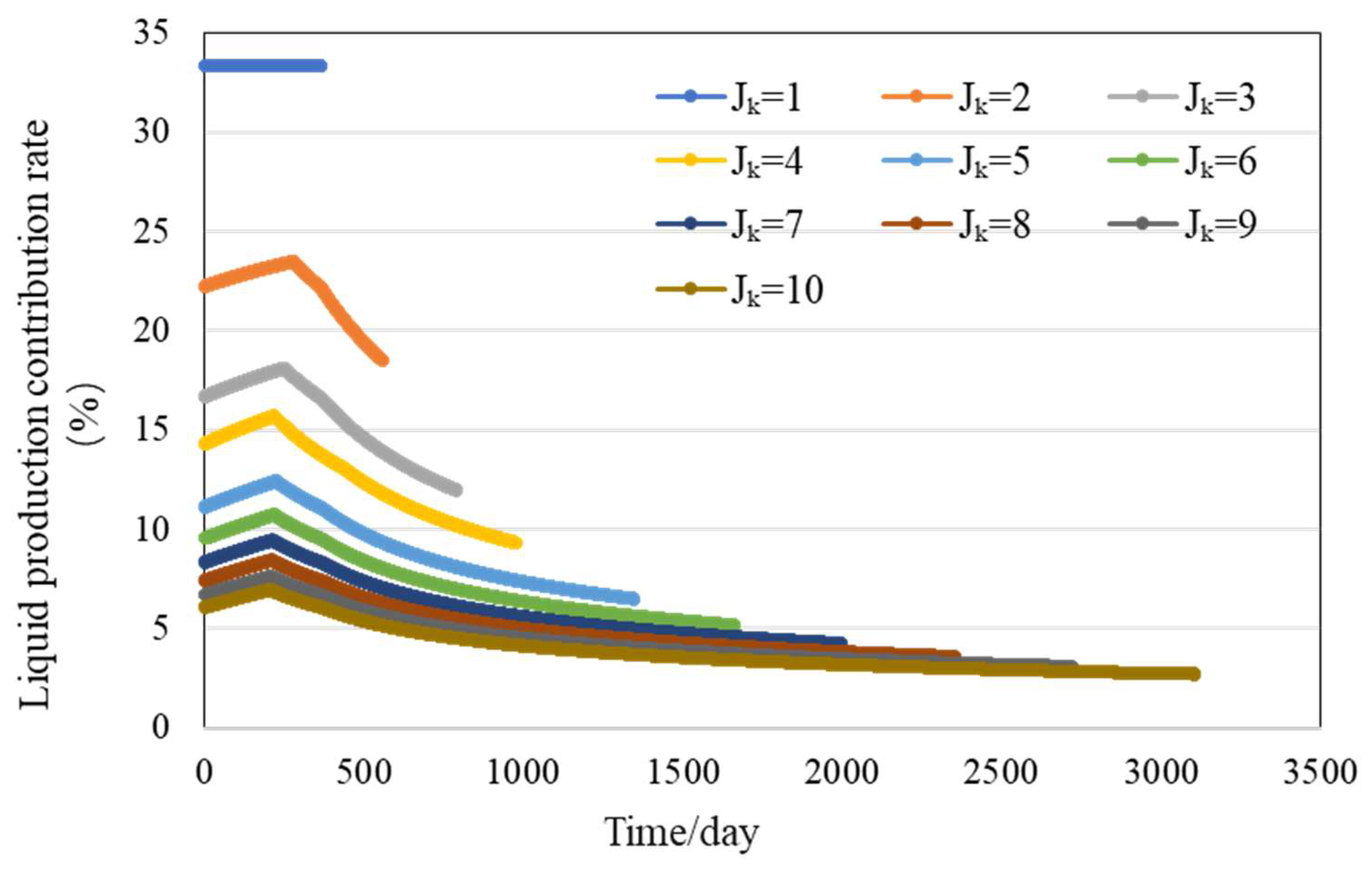
3.4. Study on Permeability Differential Limit
4. Verification and Analysis of Mathematic Model
5. Conclusions
- (1)
- The results of the displacement experiment conducted in the parallel core demonstrate that as permeability contrast increases, the production ratios of high- and low-permeability will also improve. This is primarily due to the fact that the production contribution rate for the low-permeability layer will decrease.
- (2)
- Using the Buckley–Lever (B-L) seepage equation, the mathematical model of multilayer combined production for water flooding in oil reservoirs has been established. When comparing simulated and experiment results, the error is less than 5%, demonstrating the accuracy of the established model.
- (3)
- When the permeability contrast is less than three, the experiment and the simulated results demonstrate that the low-permeability layer’s water flooding effect is superior and can be utilized to some extent. However, when the gradient difference is greater than six, the low-permeability layer’s production contribution rate is less than five percent.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| Oil phase flow velocity, m/s; | |
| Water phase seepage velocity, m/s; | |
| Total seepage velocity, m/s; | |
| Viscosity of crude, mPa·s; | |
| Viscosity of water, mPa·s; | |
| Porosity, %; | |
| Absolute permeability, mD; | |
| Oil phase relative permeability; | |
| Water phase relative permeability; | |
| Total flow, m3/s; | |
| Seepage cross section area, m2; | |
| Thickness of each reservoir, m; | |
| Seepage resistance of each layer, mPa·s/(D·m); | |
| Pressure drop in oil–water two-phase zone, MPa; | |
| Pressure drop in oil–water two-phase zone, MPa; | |
| Distance from supply edge to well row, m; | |
| Location of oil–water front in each layer, m; | |
| Water saturation, %; | |
| Oil saturation, %; | |
| Irreducible water saturation, %; | |
| Exit side water saturation, %; | |
| Derivative of fractional rate corresponding to water flooding front saturation; | |
| Pore volume of the hypertonic layer, m3; | |
| Cumulative fluid production of each reservoir, m3. |
References
- Wei, C.; Raad SM, J.; Leonenko, Y.; Hassanzadeh, H. Correlations for prediction of hydrogen gas viscosity and density for production, transportation, storage, and utilization applications. Int. J. Hydrogen Energy 2023, 48, 34930–34944. [Google Scholar] [CrossRef]
- Salmo, I.C.; Sorbie, K.S.; Skauge, A. The impact of rheology on viscous oil displacement by polymers analyzed by pore-scale network modelling. Polymers 2021, 13, 1259. [Google Scholar] [CrossRef]
- Schluter, S.; Berg, S.; Rucker, M.; Armstrong, R.T.; Vogel, H.; Hilfer, R.; Wildenschild, D. Pore-scale displacement mechanisms as a source of hysteresis for two-phase flow in porous media. Water Resour. Res. 2016, 52, 2194–2205. [Google Scholar] [CrossRef]
- Sorbie, K.S.; Al Ghafri, A.Y.; Skauge, A.; Mackay, E.J. On the modelling of immiscible viscous fingering in two-phase flow in porous media. Transp. Porous Media 2020, 135, 331–359. [Google Scholar] [CrossRef]
- Chai, X.; Tian, L.; Dong, P.; Wang, C.; Peng, L.; Wang, H. Study on recovery factor and interlayer interference mechanism of multilayer co-production in tight gas reservoir with high heterogeneity and multi-pressure systems. J. Pet. Sci. Eng. 2022, 210, 109699. [Google Scholar] [CrossRef]
- Cui, C.Z.; Xu, J.P.; Wang, D.P.; Liu, Z.-H.; Huang, Y.-S.; Geng, Z.-L. Layer regrouping for water-flooded commingled reservoirs at a high water-cut stage. Pet. Sci. 2016, 13, 272–279. [Google Scholar] [CrossRef][Green Version]
- Shen, F.; Cheng, L.; Sun, Q.; Huang, S. Evaluation of the vertical producing degree of commingled production via waterflooding for multilayer offshore heavy oil reservoirs. Energies 2018, 11, 2428. [Google Scholar] [CrossRef]
- Xu, J.; Hu, X.; Ning, B. Dynamic interference behaviors of arbitrary multilayer commingling production in heavy oil reservoirs with water flooding. ACS Omega 2021, 6, 10005–10012. [Google Scholar] [CrossRef]
- Guo, W.; Fu, S.; Li, A.; Xie, H.; Cui, S.; Nangendo, J. Experimental research on the mechanisms of improving water flooding in fractured-vuggy reservoirs. J. Pet. Sci. Eng. 2022, 213, 110383. [Google Scholar] [CrossRef]
- Chai, R.; Liu, Y.; Xue, L.; Rui, Z.; Zhao, R.; Wang, J. Formation damage of sandstone geothermal reservoirs: During decreased salinity water injection. Appl. Energy 2022, 322, 119465. [Google Scholar] [CrossRef]
- Yang, Y.; Liao, G.; Xiong, W.; Shen, R.; Zhang, J.; Li, Q.; Wang, S.; Zhang, J.; Tan, L.; Shao, G. Physical and numerical simulation of inter-fracture flooding in heterogeneous tight oil reservoirs. Energy Rep. 2022, 8, 12970–12978. [Google Scholar] [CrossRef]
- Fu, D.; Fu, Y.; Zhang, Y.J.; Wang, M. Experimental simulation study on influencing factors of liquid production capacity in heterogeneous water drive reservoirs. Phys. Fluids 2024, 36, 016612. [Google Scholar] [CrossRef]
- Sun, L.; Li, B.; Jiang, H.; Li, Y.; Jiao, Y. An injectivity evaluation model of polymer flooding in offshore multilayer reservoir. Energies 2019, 12, 1444. [Google Scholar] [CrossRef]
- Huang, S.J.; Kang, B.T.; Cheng, L.S.; Zhou, W.; Chang, S. Quantitative characterization of interzonal interference and productivity prediction of directional well in offshore common heavy oil reservoirs. Pet. Explor. Dev. 2015, 42, 488–495. [Google Scholar] [CrossRef]
- Kucuk, F.; Karakas, M.; Ayestaran, L. Well testing and analysis techniques for layered reservoirs. SPE Form. Eval. 1986, 1, 342–354. [Google Scholar] [CrossRef]
- Zhong, H.; He, Y.; Zhao, X.; Peng, X. Theoretical study on the micro-flow mechanism of polymer flooding in a double heterogeneous oil layer. Energies 2022, 15, 3236. [Google Scholar] [CrossRef]
- Chai, R.; Liu, Y.; Wang, J.; Liu, Q.; Rui, Z. CO2 utilization and sequestration in Reservoir: Effects and mechanisms of CO2 electrochemical reduction. Appl. Energy 2022, 323, 119584. [Google Scholar] [CrossRef]
- Xiong, W.; Gao, S.S.; Gao, H.J.; Long, H. Application of physical simulation results of interlayer heterogeneous reservoir to flow unit division. Daqing Pet. Geol. Dev. 2005, 2, 34–36+105. [Google Scholar]
- Mo, J.W.; Sun, W.; Yang, X.P.; Han, Z.Y.; Wu, M.J. Study on water flooding effect and influencing factors of severe interlayer heterogeneous reservoir. J. Northwest Univ. (Nat. Sci. Ed.) 2011, 41, 113–118. [Google Scholar]
- Deng, X.; Huang, X.; Ye, Q.; Li, S.; Yu, C.; Zhang, X.; Wang, Z. Experimental research on production law of multilayer heterogeneous reservoirs. Front. Earth Sci. 2022, 10, 990554. [Google Scholar] [CrossRef]
- Tariq, S.M.; Ramey, H.J. Drawdown Behavior of a Well with Storage and Skin Effect Communicating with Layers of Different Radii and Other Characteristics. In Proceedings of the SPE Annual Fall Technical Conference and Exhibition, Houston, TX, USA, 1 October 1978. [Google Scholar]
- Tompang, R.; Kelkar, B.G. Prediction of Waterflood Performance in Stratified Reservoirs. Chest 1988, 92, 657–662. [Google Scholar]
- Wei, C.; Liu, Y.; Deng, Y.; Cheng, S.; Hassanzadeh, H. Temperature transient analysis of naturally fractured geothermal reservoirs. SPE J. 2022, 27, 2723–2745. [Google Scholar] [CrossRef]





| Ion Type | Na+ | K+ | Ca2+ | Mg2+ | Cl− | SO42− |
|---|---|---|---|---|---|---|
| Ions content (mg/L) | 11,842.76 | 184.46 | 1490.91 | 137.87 | 21,133.35 | 94.64 |
| Permeability Max-Min Ratio | Q1/Q3 | Contribution Ratio of Q3 (%) |
|---|---|---|
| 2.8 | 3.92 | 14.34 |
| 4.2 | 7.21 | 8.12 |
| 5.1 | 10.53 | 6.23 |
| 6.2 | 14.12 | 4.31 |
| 7.4 | 16.85 | 3.64 |
| Permeability Max-Min Ratio | Permeability of Low-Permeability Layer (mD) | Permeability of Medium Permeability Layer (mD) | Permeability of High-Permeability Layer (mD) |
|---|---|---|---|
| 1 | 40 | 40 | 40 |
| 2 | 40 | 60 | 80 |
| 3 | 40 | 80 | 120 |
| 4 | 40 | 100 | 160 |
| 5 | 40 | 120 | 200 |
| 6 | 40 | 140 | 240 |
| 7 | 40 | 160 | 280 |
| 8 | 40 | 180 | 320 |
| 9 | 40 | 200 | 360 |
| 10 | 40 | 220 | 400 |
| Viscosity of Crude (mPa·s) | 5 |
|---|---|
| Water viscosity (mPa·s) | 1.5 |
| Reservoir length (m) | 300 |
| Reservoir width (m) | 200 |
| Reservoir thickness (m) | 15 m (Each reservoir is 5 m thick) |
| Porosity (%) | 20 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tian, L.; Chai, X.; Zhang, L.; Zhang, W.; Zhu, Y.; Wang, J.; Wang, J. Study on Compatibility Evaluation of Multilayer Co-Production to Enhance Recovery of Water Flooding in Oil Reservoir. Energies 2024, 17, 3667. https://doi.org/10.3390/en17153667
Tian L, Chai X, Zhang L, Zhang W, Zhu Y, Wang J, Wang J. Study on Compatibility Evaluation of Multilayer Co-Production to Enhance Recovery of Water Flooding in Oil Reservoir. Energies. 2024; 17(15):3667. https://doi.org/10.3390/en17153667
Chicago/Turabian StyleTian, Leng, Xiaolong Chai, Lei Zhang, Wenbo Zhang, Yuan Zhu, Jiaxin Wang, and Jianguo Wang. 2024. "Study on Compatibility Evaluation of Multilayer Co-Production to Enhance Recovery of Water Flooding in Oil Reservoir" Energies 17, no. 15: 3667. https://doi.org/10.3390/en17153667
APA StyleTian, L., Chai, X., Zhang, L., Zhang, W., Zhu, Y., Wang, J., & Wang, J. (2024). Study on Compatibility Evaluation of Multilayer Co-Production to Enhance Recovery of Water Flooding in Oil Reservoir. Energies, 17(15), 3667. https://doi.org/10.3390/en17153667





