Numerical Investigation of Hydraulic Fractures Vertical Propagation Mechanism for Enhanced Tight Gas Recovery
Abstract
1. Introduction
- Numerical simulation methods are used to explore the vertical expansion law of hydraulic fractures from two aspects: geological lithology and engineering operation. The consodered geological factors mainly consist of barrier/reservoir stress, thickness, elastic modulus, Poisson’s ratio, tensile strength, and the difference in fracture toughness; The engineering factors mainly include fracturing fluid volume, discharge, and viscosity which are verified through laboratory experiments as well;
- Based on the influence pattern and extent of various factors on the vertical extension of hydraulic fractures, we clarify the main controlling factors of fracture height and establish the fracture height prediction map. Finally, the feasibility and effect of three fracture height control technologies, e.g., multi-stage intermittent sand addition, low-viscosity pre-fluid, and multi-stage variable-displacement, are verified through field application.
2. Research on the Vertical Expansion Law of Hydraulic Fractures
2.1. Establishment of Numerical Models
2.2. Analysis of Single-Factor Influence on Vertical Expansion of Hydraulic Fractures
2.2.1. Influence of Geological Lithology Factors
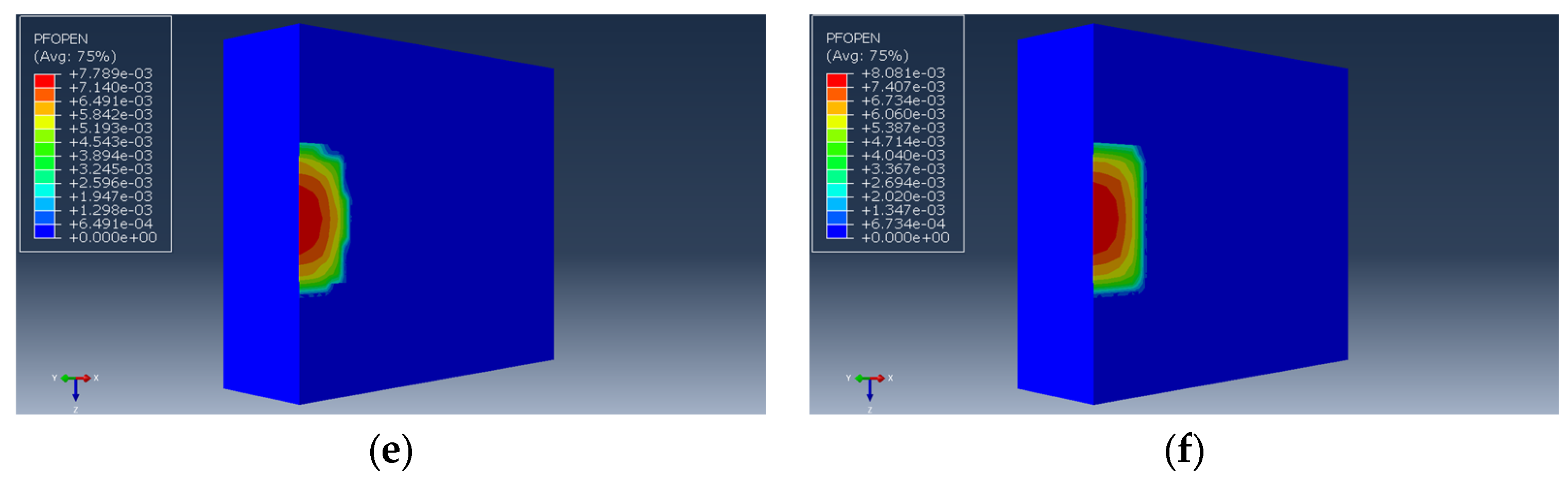
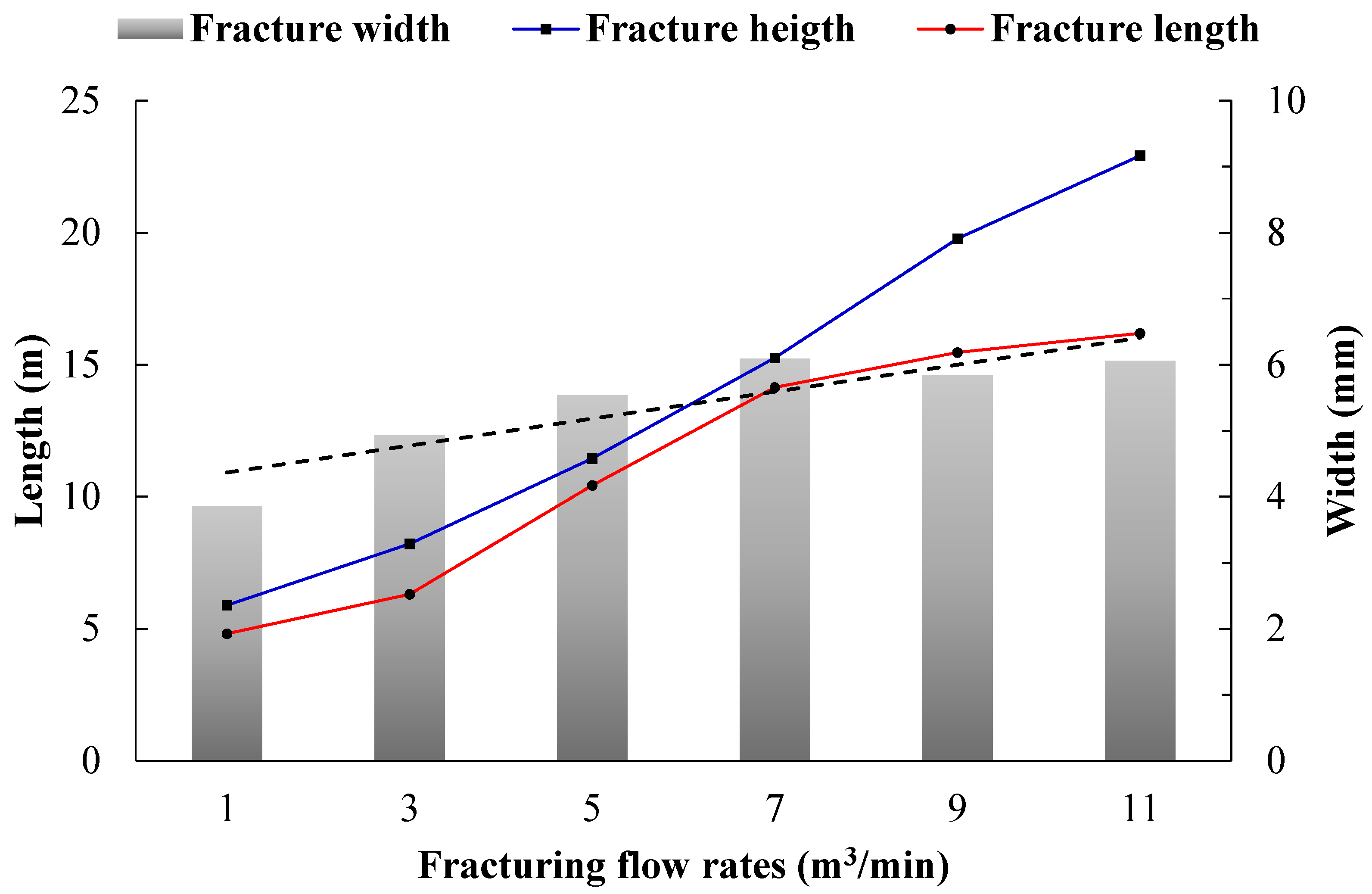
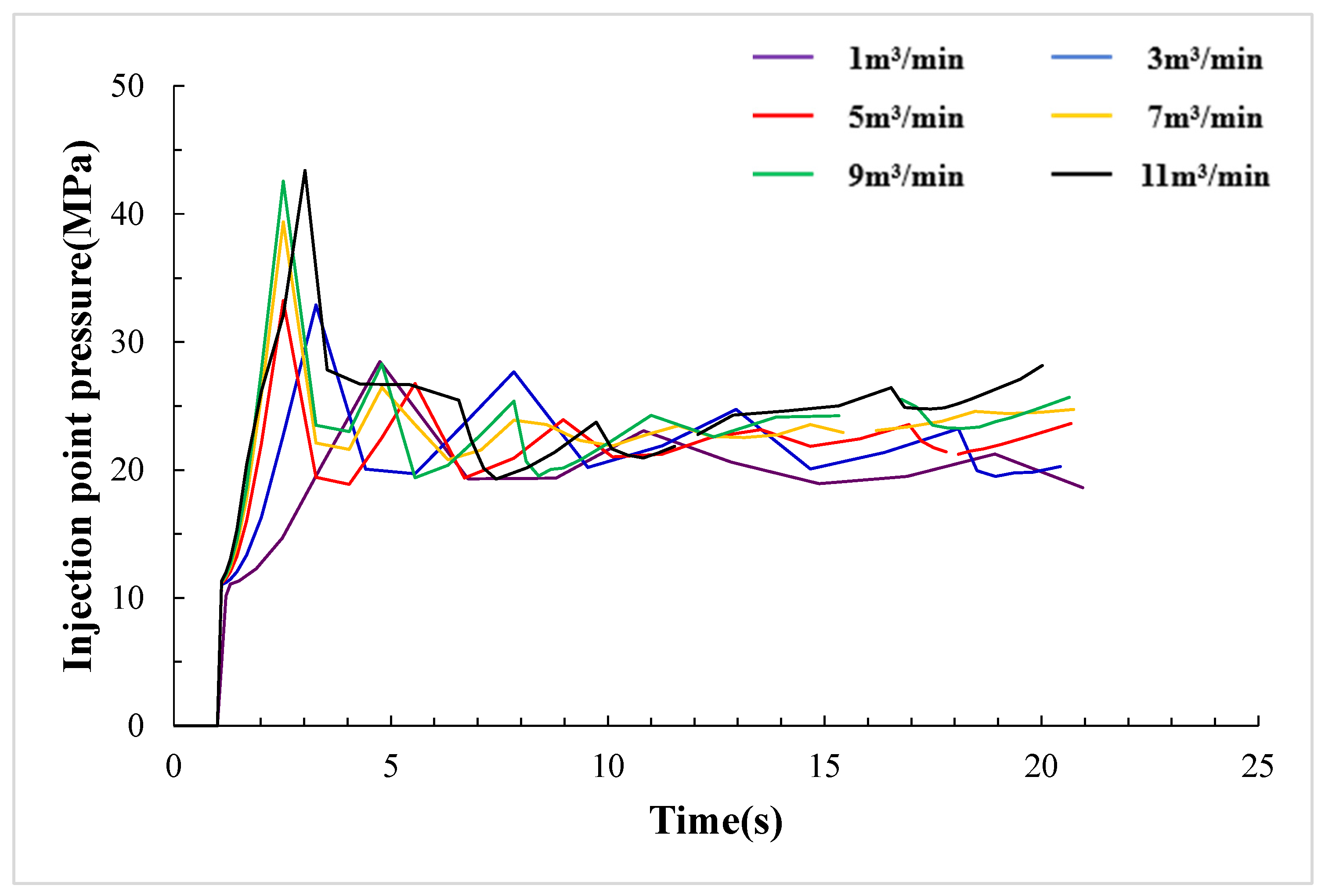
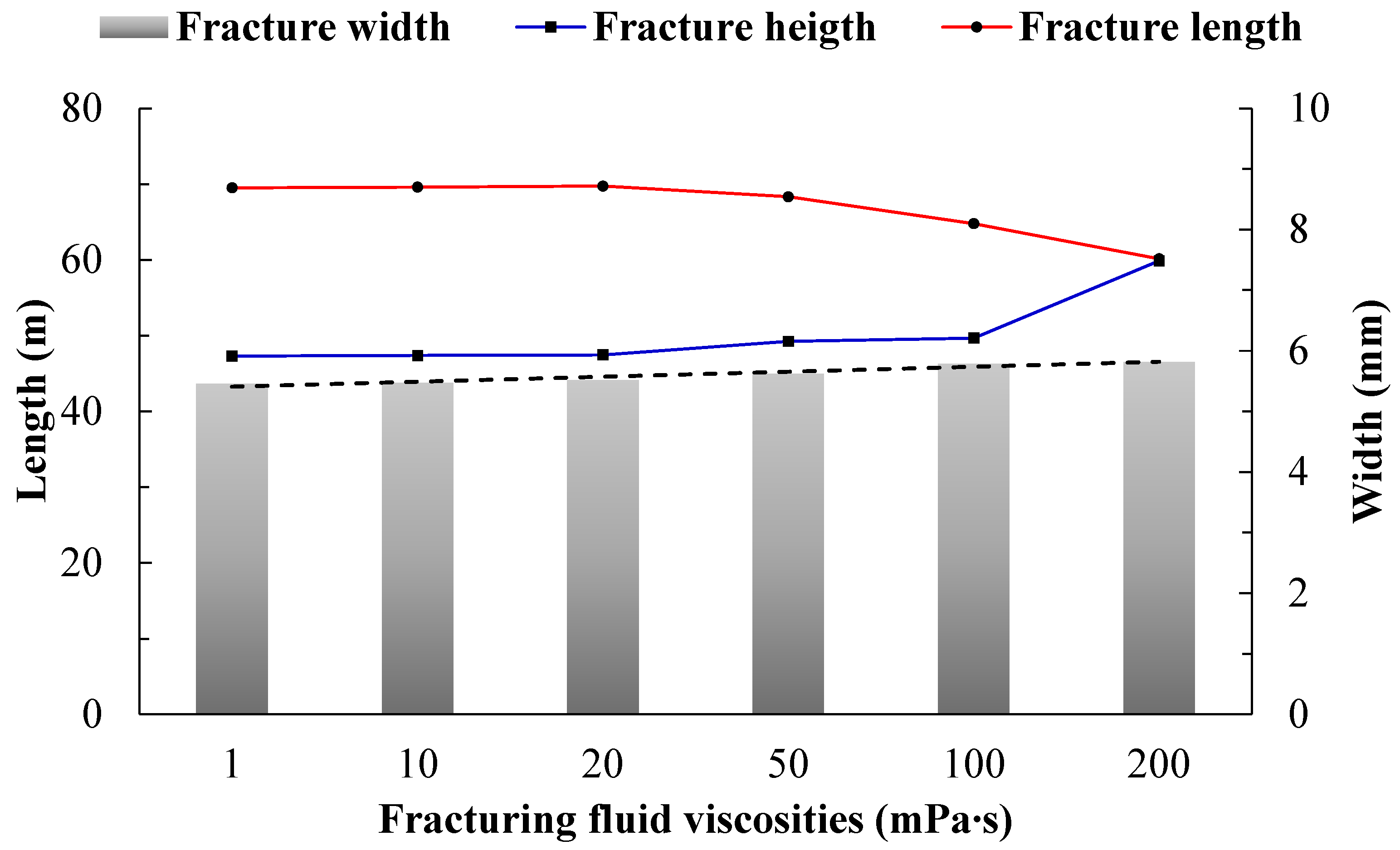
2.2.2. Engineering Construction Parameters Influence
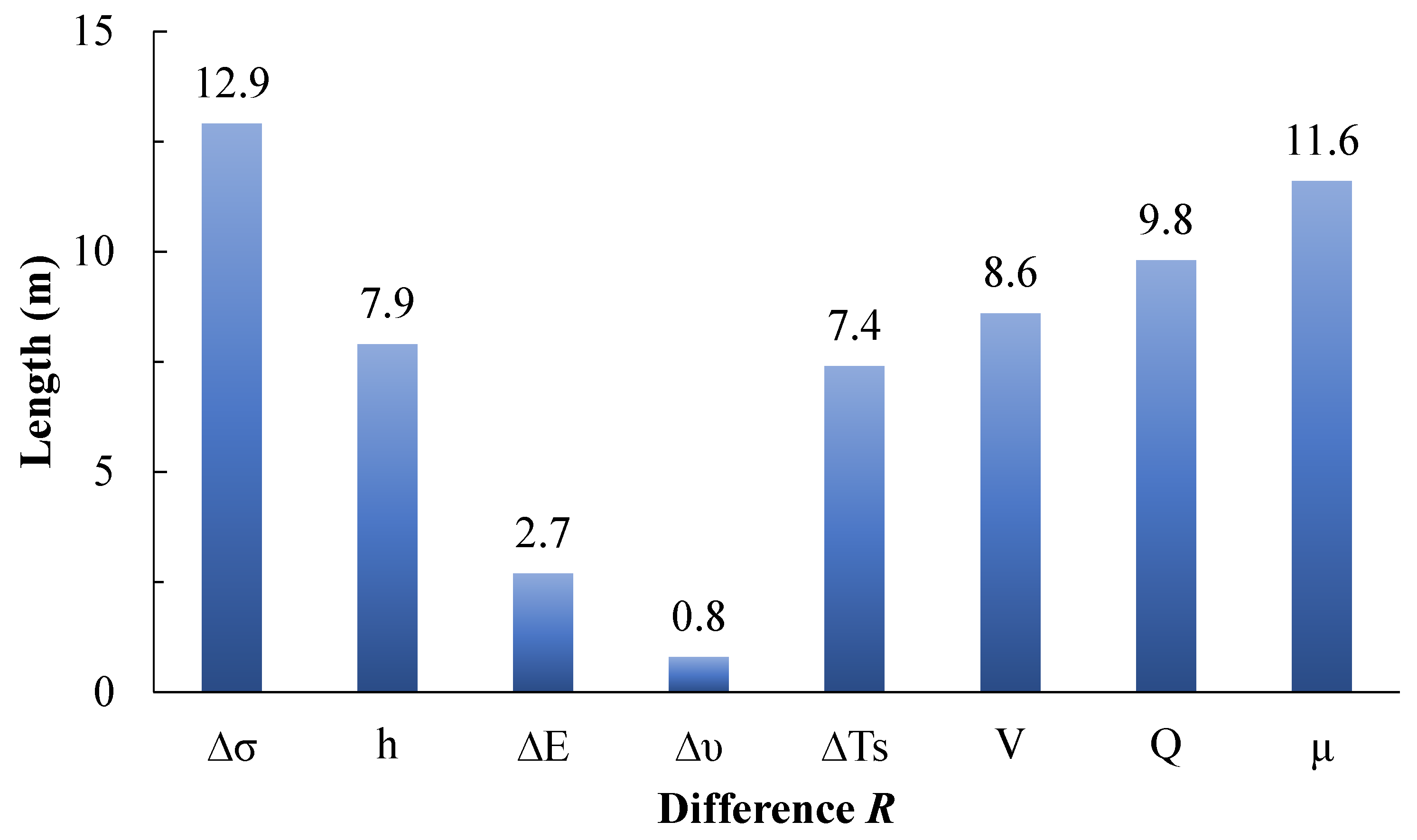
2.3. Orthogonal Analysis of Multi-Factor Vertical Expansion of Hydraulic Fractures
3. Prediction Study on Hydraulic Fracture Height
4. Conclusions
- (1)
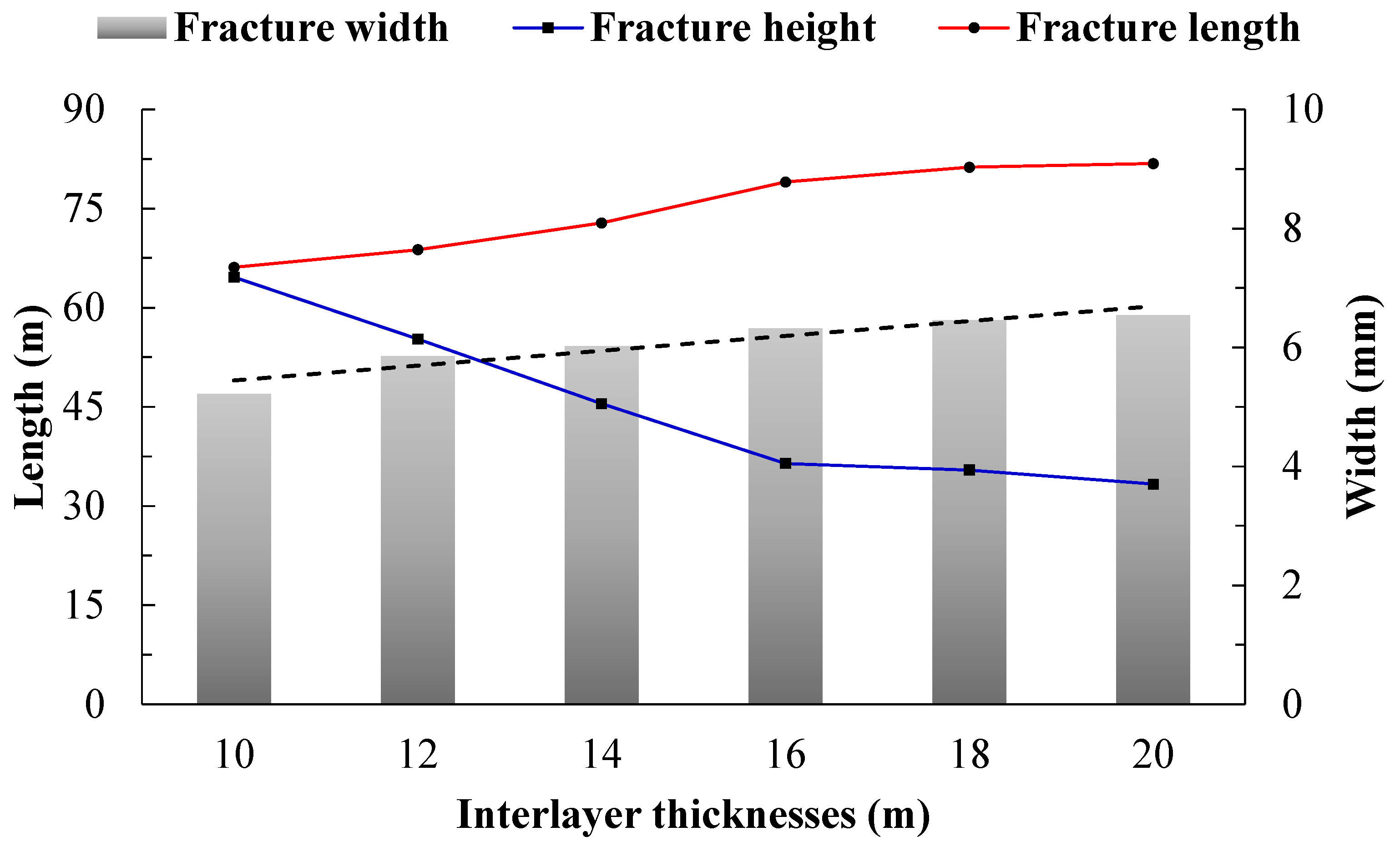
- During the initial stage of fracture formation, there is a competition between vertical and horizontal propagation of hydraulic fractures. Once the initial shape of the fracture is determined, subsequent propagation of the fracture will more easily follow the direction of competitive advantage. Therefore, under the same conditions, the height and length of the fracture are inversely proportional, meaning that the smaller the fracture height, the greater the length of the fracture in the reservoir, and the larger the effective fracture volume;
- (2)
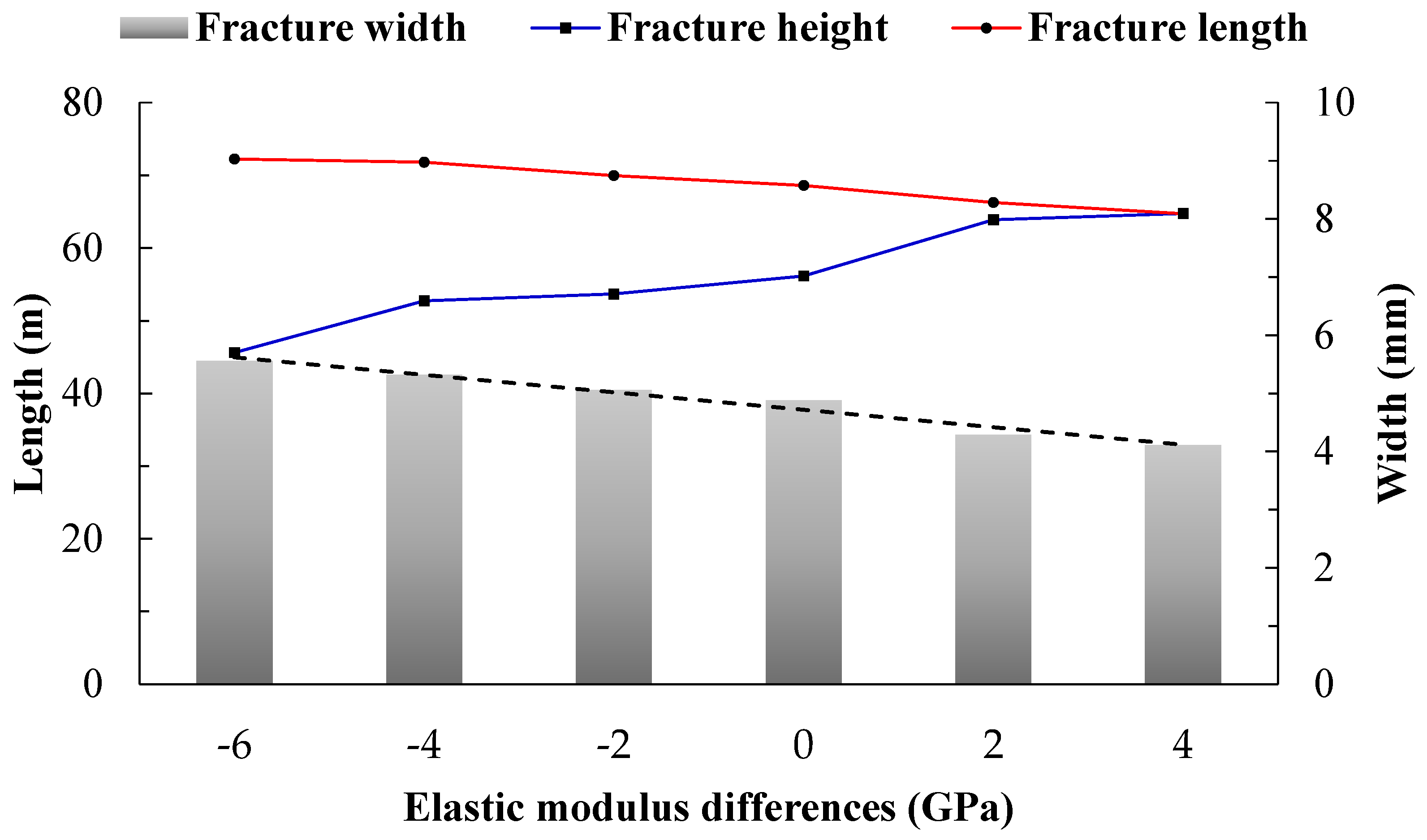
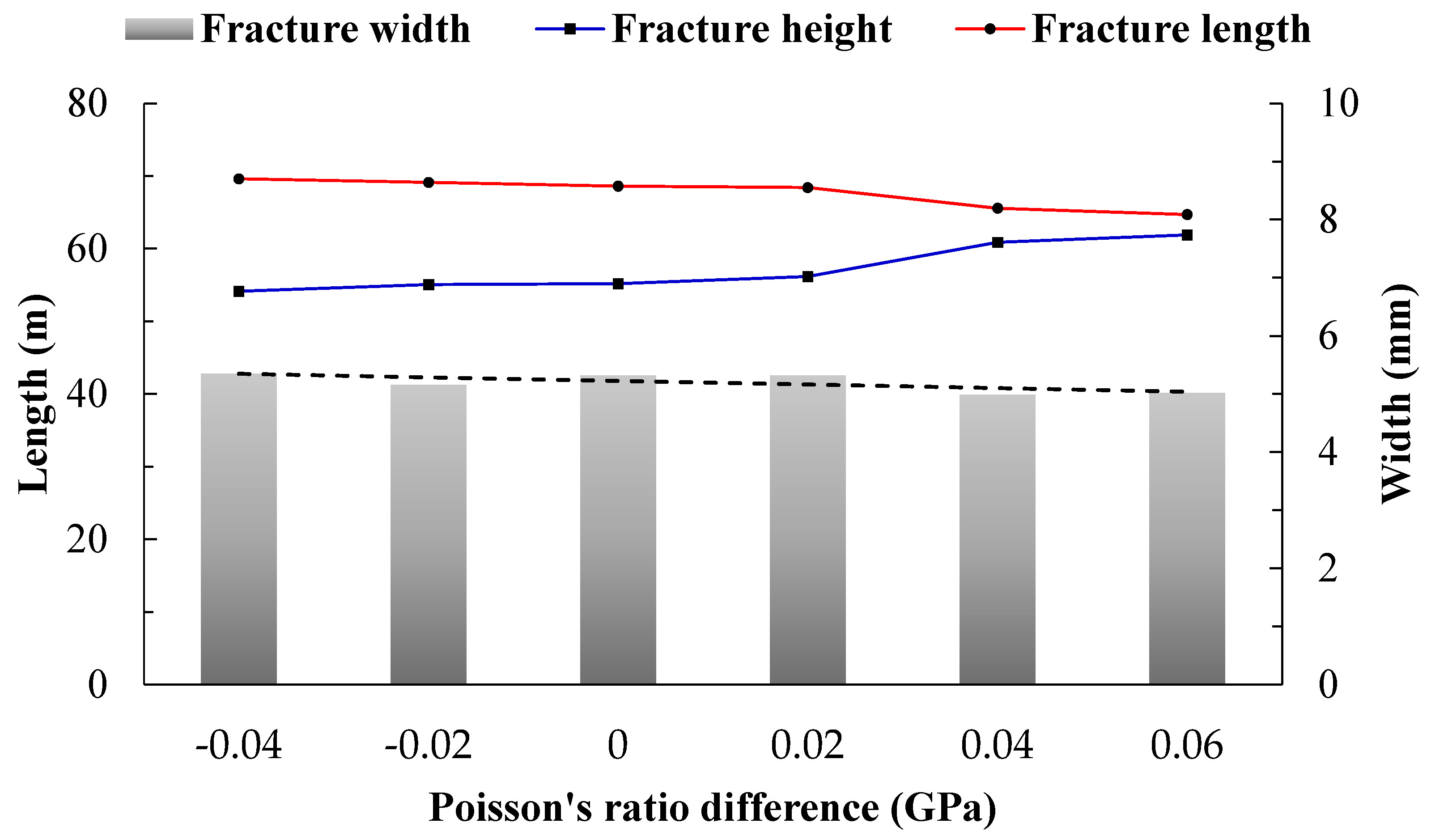
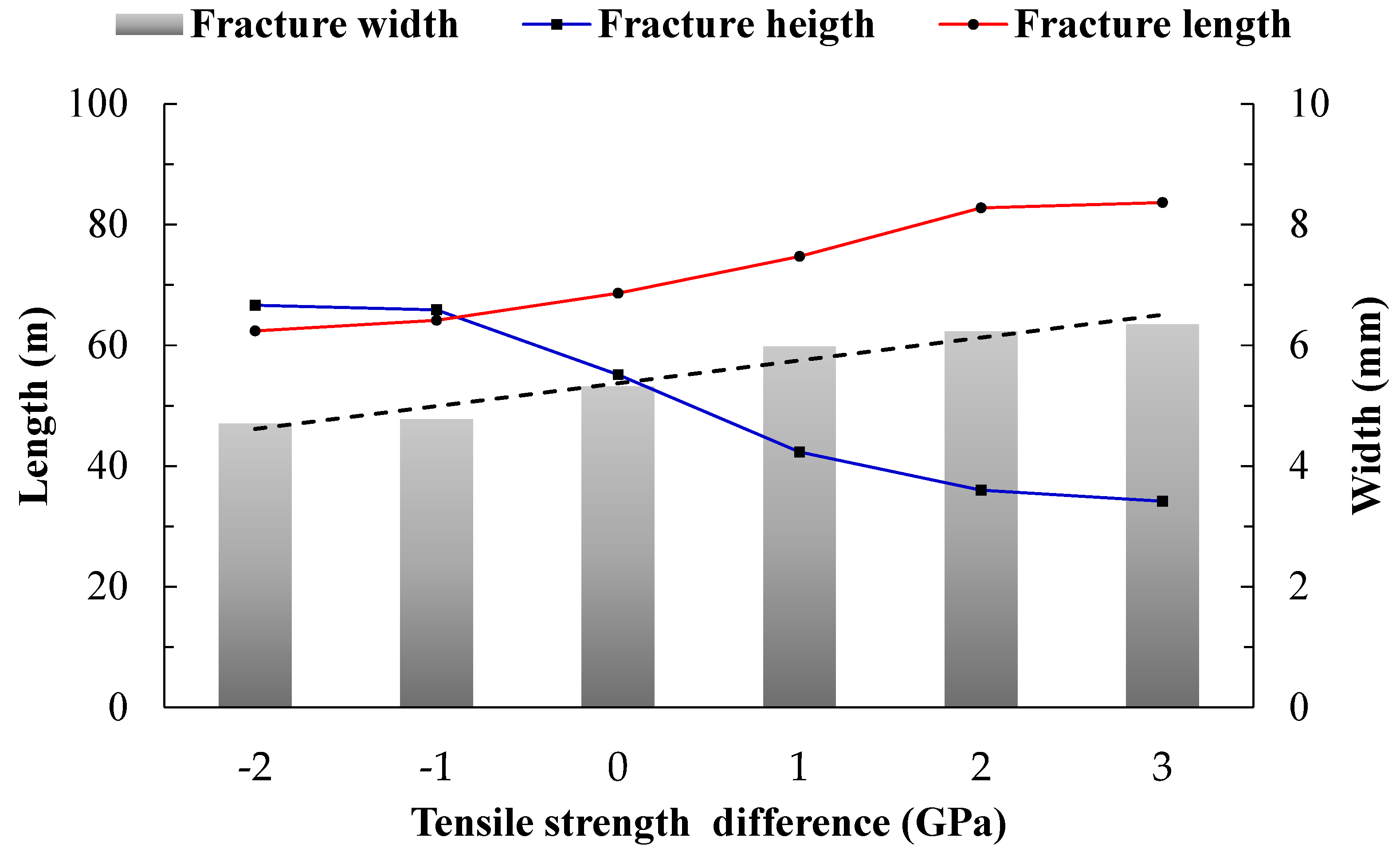
- For geological lithological factors, the height of hydraulic fractures decreases with increasing thickness of interlayers, difference in stress between interlayers and reservoirs, difference in tensile strength, and difference in fracture toughness, while it increases with increasing difference in elastic modulus between interlayers and reservoirs. The influence of the difference in Poisson’s ratio between interlayers and reservoirs is not significant. Among these factors, the thickness of interlayers and the difference in stress and tensile strength between interlayers and reservoirs have a greater impact on fracture height. Compared to reservoirs, thicker interlayers with higher stress and tensile strength are more conducive to controlling fracture height;
- (3)
- Regarding engineering construction parameters, the height of hydraulic fractures increases with increasing volume, rate, and viscosity of fracturing fluid. Among these factors, rate and viscosity have a greater impact on the initial vertical propagation of hydraulic fractures. A low rate and low viscosity during fracturing are conducive to promoting the longitudinal extension and propagation of hydraulic fractures, thereby avoiding uncontrolled increases in fracture height;
- (4)
- For the development of tight gas hydraulic fracturing in the Linxing–Shifu shale formation, the factors affecting fracture height are in the following order: stress difference between interlayers and reservoirs > fracturing fluid viscosity > fracturing rate > fracturing fluid volume > thickness of interlayers > difference in tensile strength between interlayers and reservoirs > difference in elastic modulus between interlayers and reservoirs > difference in Poisson’s ratio between interlayers and reservoirs;
- (5)
- A preliminary prediction chart for fracture height in the Linxing–Shifu shale formation has been established, with an average prediction error of about 10.8%. The reliability is relatively high, providing some guidance for optimizing fracturing design in the area.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Naceur, K.B.; Touboul, E. Mechanisms Controlling Fracture-Height Growth in Layered Media. SPE Prod. Eng. 1990, 5, 142–150. [Google Scholar] [CrossRef]
- Simonson, E.R.; Abou-Sayed, A.S.; Clifton, R.J. Containment of massive hydraulic fractures. Soc. Pet. Eng. J. 1978, 18, 27–32. [Google Scholar] [CrossRef]
- Huang, L.; Dontsov, E.; Fu, H.; Lei, Y.; Weng, D.; Zhang, F. Hydraulic fracture height growth in layered rocks: Perspective from DEM simulation of different propagation regimes. Int. J. Solids Struct. 2022, 238, 111395. [Google Scholar] [CrossRef]
- Warpinski, N.R.; Schmidt, R.A.; Northrop, D.A. In-situ stresses: The predominant influence on hydraulic fracture containment. J. Pet. Technol. 1982, 34, 653–664. [Google Scholar] [CrossRef]
- Gu, H.; Siebrits, E. Effect of Formation Modulus Contrast on Hydraulic Fracture Height Containment. SPE Prod. Oper. 2008, 23, 170–176. [Google Scholar] [CrossRef]
- Gu, H.; Siebrits, E.; Sabourov, A. Hydraulic fracture modeling with bedding plane interfacial slip. In Proceedings of the SPE Eastern Regional/AAPG Eastern Section Joint Meeting, Pittsburgh, PA, USA, 11–15 October 2008. Society of Petroleum Engineers. [Google Scholar]
- Eekelen, V. Hydraulic Fracture Geometry: Fracture Containment in Layered Formations. Soc. Pet. Eng. J. 1982, 22, 341–349. [Google Scholar] [CrossRef]
- Smith, M.B.; Bale, A.B.; Britt, L.K.; Klein, H.H.; Siebrits, E.; Dang, X. Layered Modulus Effects on Fracture Propagation, Proppant Placement, and Fracture Modeling. In Proceedings of the SPE Annual Technical Conference and Exhibition, Denver, CO, USA, 30 October–2 November 2011. Society of Petroleum Engineers. [Google Scholar]
- Huang, J.; Morris, J.P.; Fu, P.; Settgast, R.R.; Sherman, C.S.; Ryerson, F.J. Hydraulic-fracture-height growth under the combined influence of stress barriers and natural fractures. SPE J. 2019, 24, 302–318. [Google Scholar] [CrossRef]
- Salah, M.; Gabry, M.A.; ElSebaee, M.; Mohamed, N. Control of hydraulic fracture height growth above water zone by inducing artificial barrier in Western Desert, Egypt. In Proceedings of the Abu Dhabi International Petroleum Exhibition and Conference, Abu Dhabi, United Arab Emirates, 7–10 November 2016. Society of Petroleum Engineers. [Google Scholar]
- Li, J.; Wu, K. An efficient model for hydraulic fracture height growth considering the effect of bedding layers in unconventional shale formations. SPE J. 2022, 27, 3740–3756. [Google Scholar] [CrossRef]
- Gao, Q.; Ghassemi, A. Three dimensional finite element simulations of hydraulic fracture height growth in layered formations using a coupled hydro-mechanical model. Int. J. Rock Mech. Min. Sci. 2020, 125, 104137. [Google Scholar] [CrossRef]
- Detounay, E. Propagation regimes of fluid-driven fractures in impermeable rocks. Int. J. Geomech. 2004, 4, 35–45. [Google Scholar] [CrossRef]
- Chen, Z.X.; Chen, M.; Huang, R.Z.; Shen, Z.H. Vertical propagation of hydraulic fractures in layered formation. J. China Univ. Pet. (Ed. Nat. Sci.) 1997, 21, 23–26, 32. [Google Scholar]
- Qin, M.; Yang, D. Numerical investigation of hydraulic fracture height growth in layered rock based on peridynamics. Theor. Appl. Fract. Mech. 2023, 125, 103885. [Google Scholar] [CrossRef]
- Cong, Z.; Li, Y.; Tang, J.; Martyushev, D.A.; Yang, F. Numerical simulation of hydraulic fracture height layer-through propagation based on three-dimensional lattice method. Eng. Fract. Mech. 2022, 264, 108331. [Google Scholar] [CrossRef]
- Tang, J.; Wu, K. A 3-D model for simulation of weak interface slippage for fracture height containment in shale reservoirs. Int. J. Solids Struct. 2018, 144, 248–264. [Google Scholar] [CrossRef]
- Kresse, O.; Weng, X.; Mohammadnejad, T. Modeling the effect of fracture interference on fracture height growth by coupling 3D displacement discontinuity method in hydraulic fracture simulator. In ARMA US Rock Mechanics/Geomechanics Symposium; ARMA: San Francisco, CA, USA, 2017; p. ARMA–2017. [Google Scholar]
- Mehrabi, M.; Pei, Y.; Haddad, M.; Javadpour, F.; Sepehrnoori, K. Quasi-static fracture height growth in laminated reservoirs: Impacts of stress and toughness barriers, horizontal well landing depth, and fracturing fluid density. In Proceedings of the Unconventional Resources Technology Conference (URTeC), Houston, TX, USA, 26–28 July 2021; pp. 1027–1046. [Google Scholar]
- Rho, S.; Noynaert, S.; Bunger, A.P.; Zolfaghari, N.; Xing, P.; Abell, B.; Suarez-Rivera, R. Finite-element simulations of hydraulic fracture height growth on layered mudstones with weak interfaces. In ARMA US Rock Mechanics/Geomechanics Symposium; ARMA: San Francisco, CA, USA, 2017; p. ARMA–2017. [Google Scholar]
- Yang, L.; Chen, B. Extended finite element-based cohesive zone method for modeling simultaneous hydraulic fracture height growth in layered reservoirs. J. Rock Mech. Geotech. Eng. 2024. [Google Scholar] [CrossRef]
- Du, J.; Chen, X.; Liu, P.; Zhao, L.; Chen, Z.; Yang, J.; Chen, W.; Wang, G.; Lou, F.; Miao, W. Numerical Modeling of Fracture Height Propagation in Multilayer Formations Considering the Plastic Zone and Induced Stress. ACS Omega 2022, 7, 17868–17880. [Google Scholar] [CrossRef] [PubMed]
- Li, H.; Zou, Y.; Valko, P.P.; Ehlig-Economides, C. Hydraulic fracture height predictions in laminated shale formations using finite element discrete element method. In Proceedings of the SPE Hydraulic Fracturing Technology Conference and Exhibition, Woodlands, TX, USA, 9–11 February 2016; SPE: The Woodlands, TX, USA, 2016; p. D011S001R002. [Google Scholar]
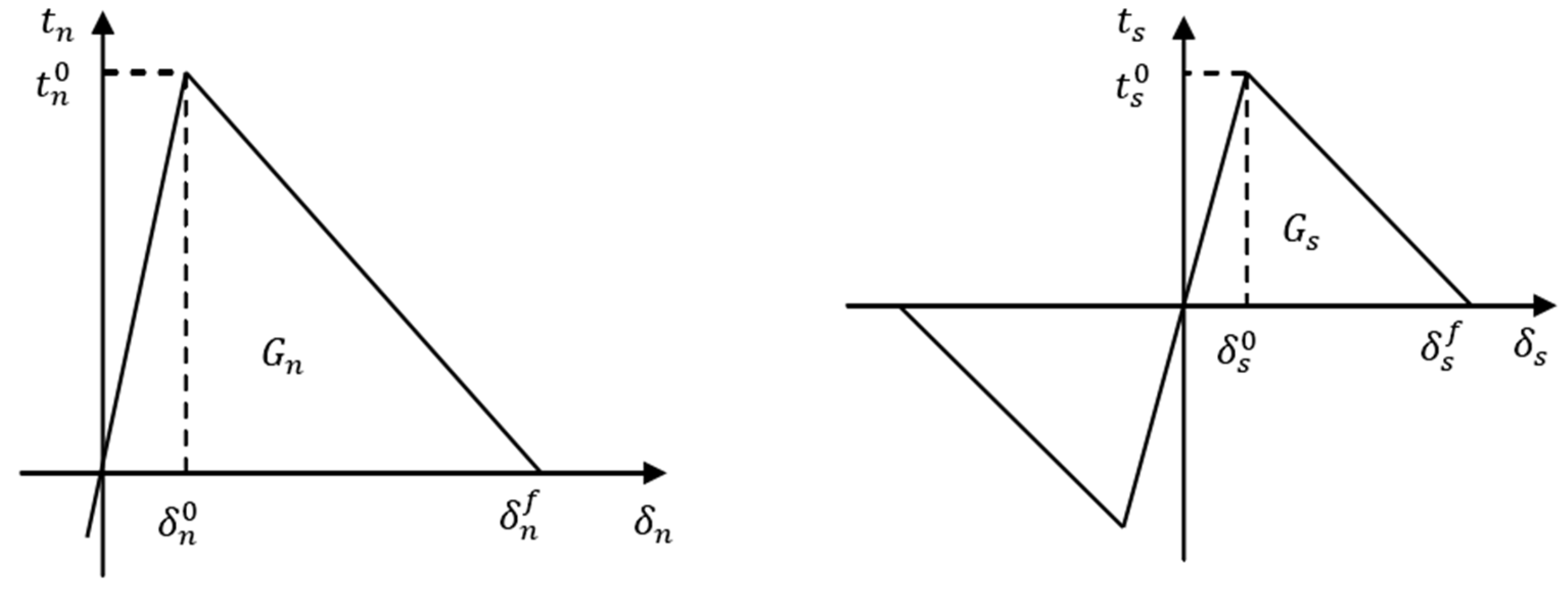
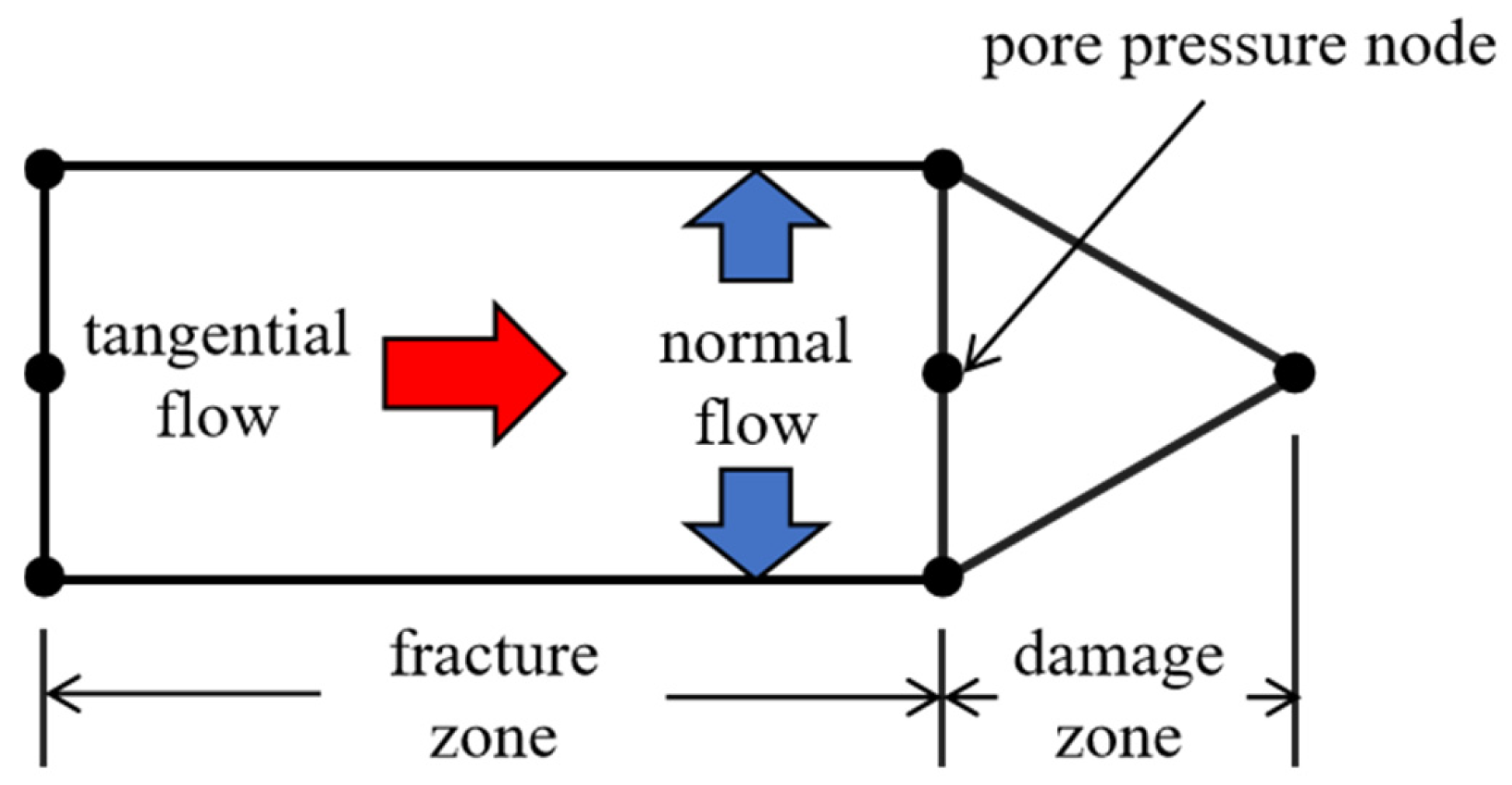
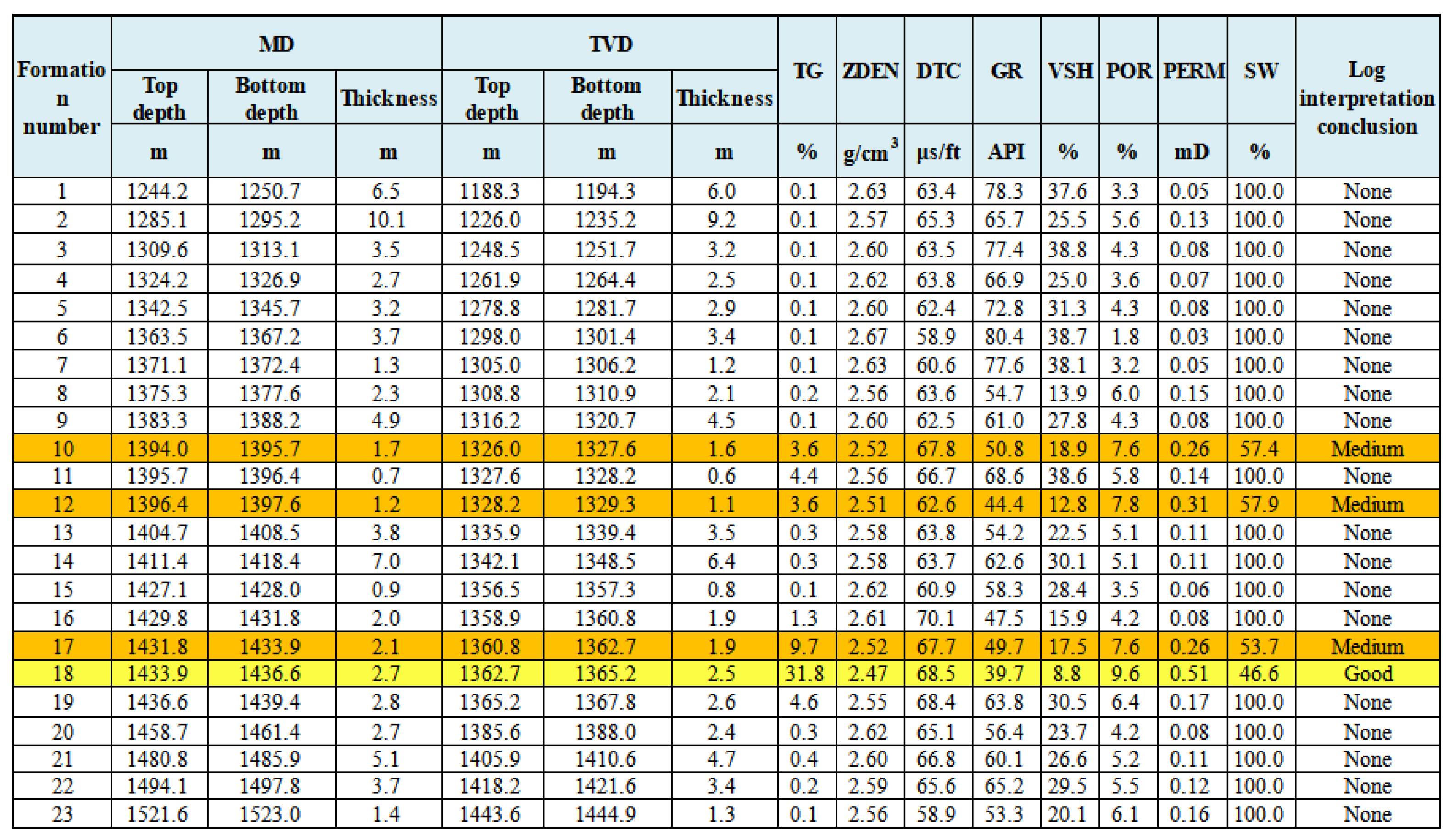
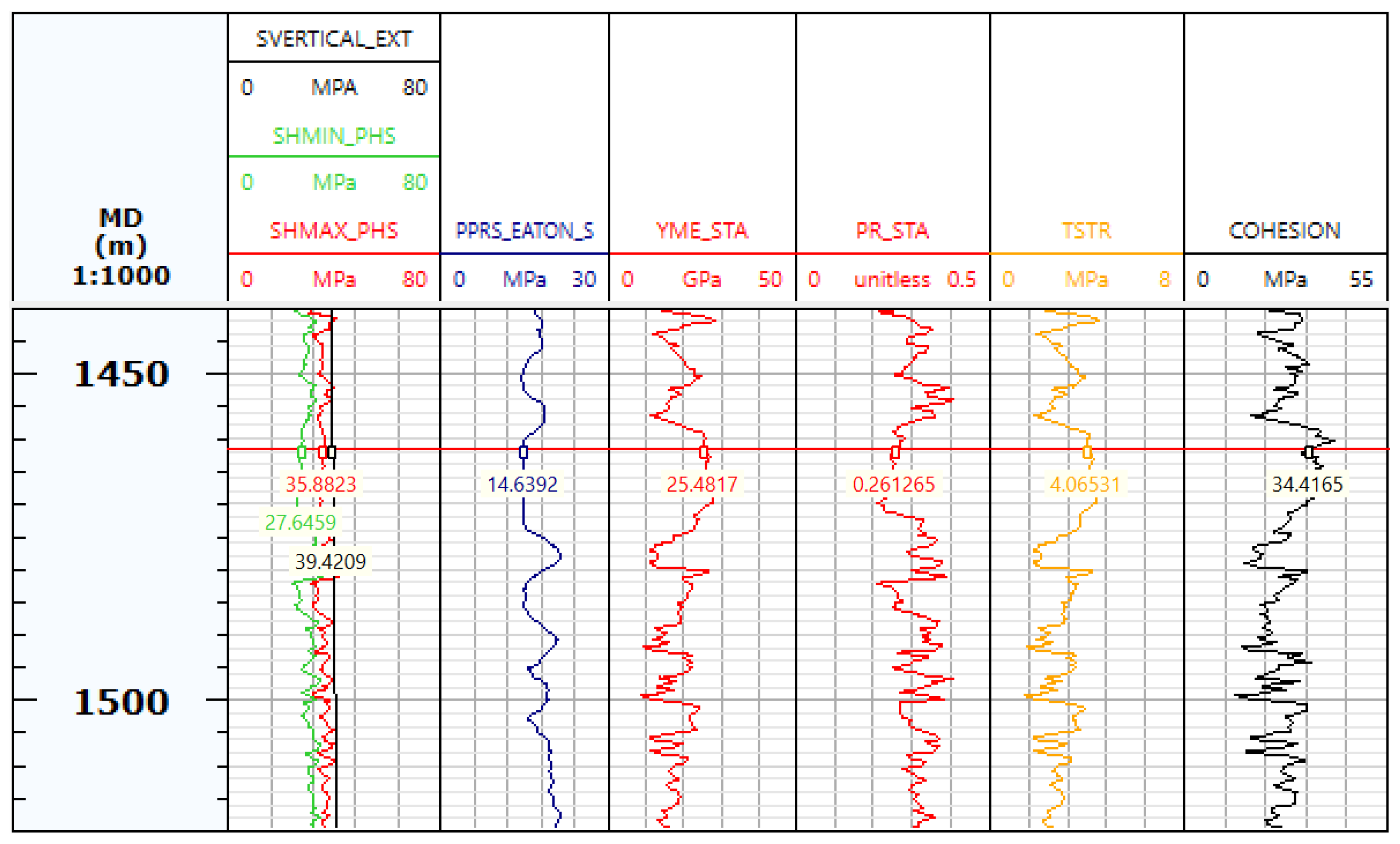

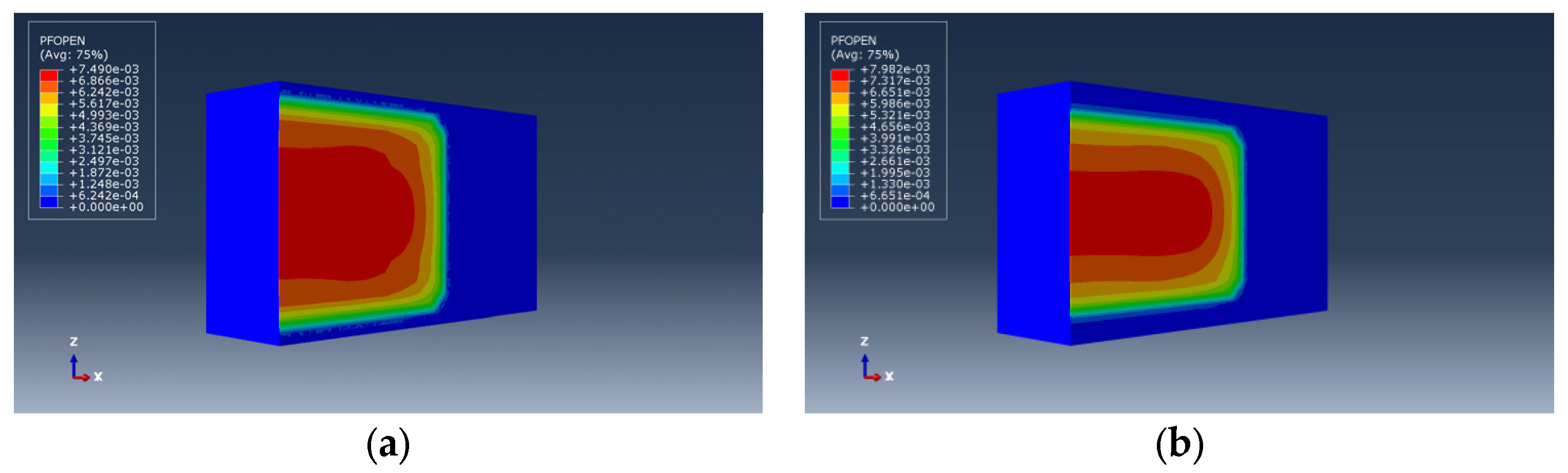
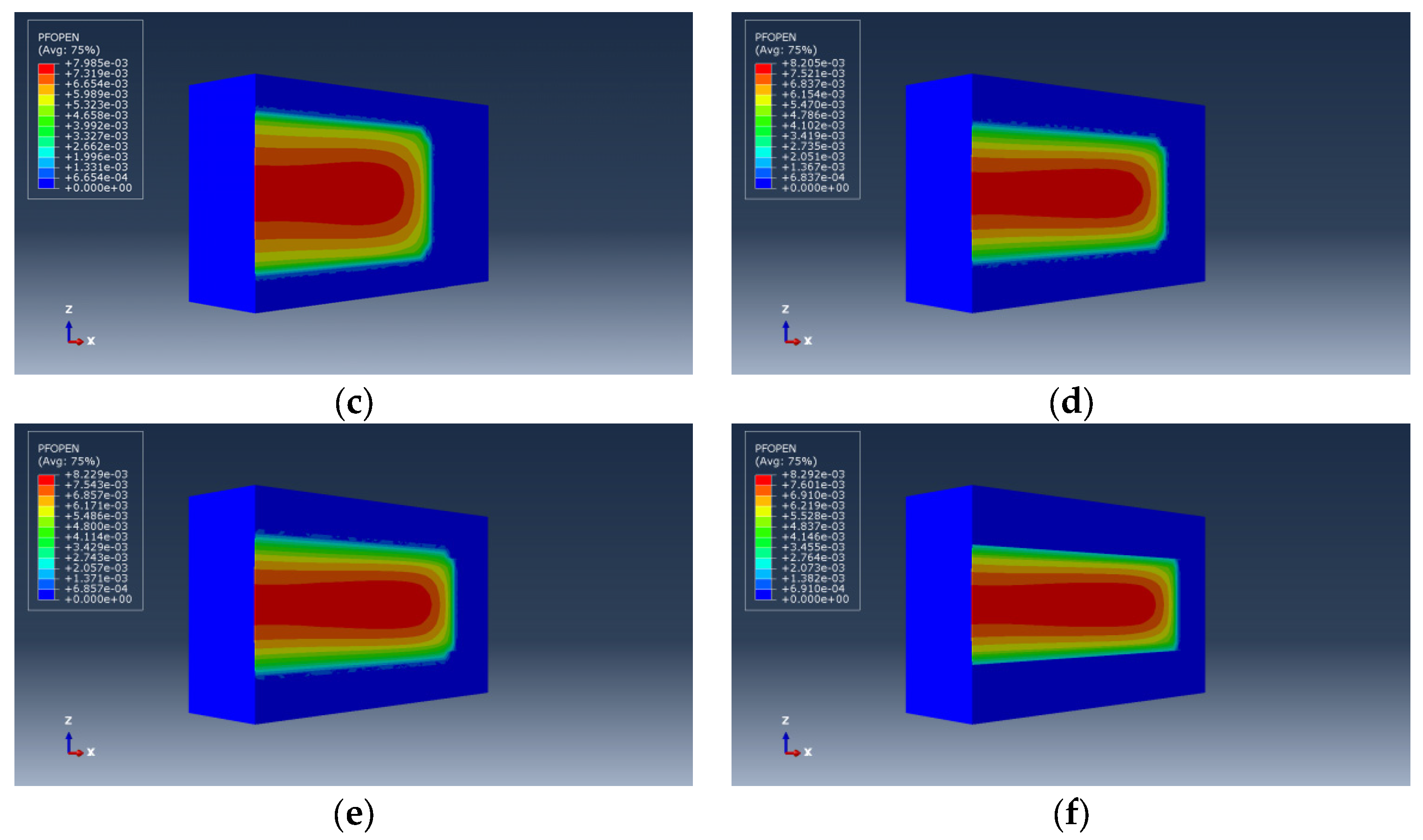

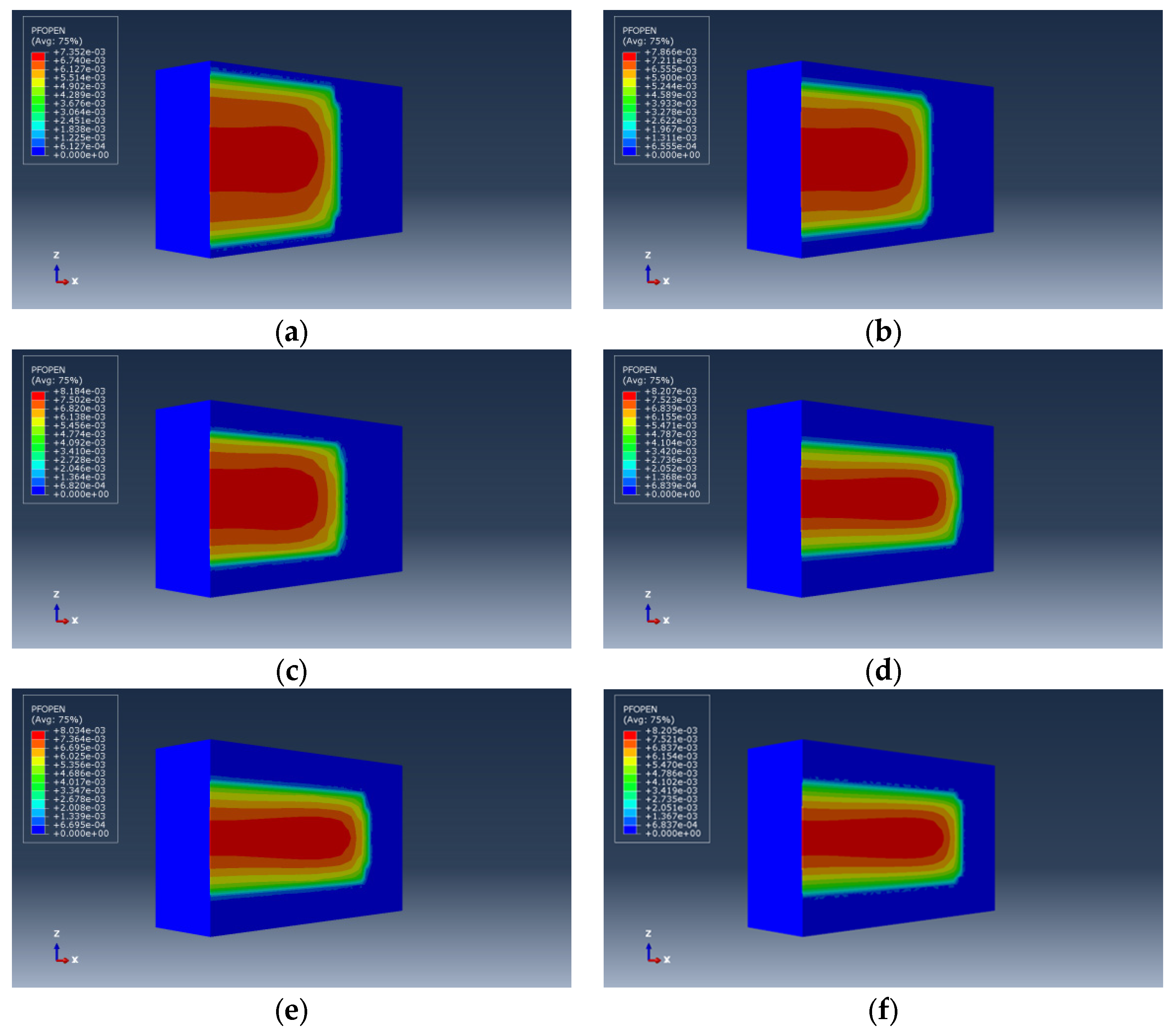







| Parameter Category | Parameter Name/Units | Parameter Value |
|---|---|---|
| Formation parameters | Simulation depth/m | 1450~1520 |
| Porosity/% | 10 | |
| Permeability/mD | 0.5 | |
| Elastic modulus/GPa | 25 | |
| Poisson’s ratio | 0.25 | |
| Tensile strength/MPa | 4 | |
| Shear strength/MPa | 34 | |
| Pressure parameters | Pore pressure/MPa | 15 |
| Overburden stress/MPa | 40 | |
| Maximum horizontal stress/MPa | 34 | |
| Minimum horizontal stress/MPa | 26 | |
| Construction parameters | Fluid volume/m3 | 100 |
| Displacement/m3∙min−1 | 3 | |
| Viscosity/mPa∙s | 1 | |
| Perforation location | Mid-reservoir |
| Parameter Category | Parameter Name/Unit | Parameter Value |
|---|---|---|
| Subsurface parameter | Porosity/% | 5 |
| Permeability/mD | 0.05 | |
| Elastic modulus/GPa | 21 | |
| Poisson’s ratio | 0.23 | |
| Tensile strength/MPa | 5 | |
| Shear strength/MPa | 35 | |
| Pressure parameter | Pore pressure/MPa | 15 |
| Overburden stress/MPa | 40 | |
| Maximum horizontal stress/MPa | 34 | |
| Minimum horizontal stress/MPa | 28 |
| Factors | /MPa | /m | /GPa | /MPa | /m3 | /m3∙min−1 | /mPa∙s | ||
|---|---|---|---|---|---|---|---|---|---|
| Levels | |||||||||
| I | 2 | 5 | −2 | 0.02 | 2 | 250 | 3 | 20 | |
| II | 4 | 10 | −4 | 0.04 | 4 | 300 | 3.5 | 100 | |
| III | 6 | 15 | −6 | 0.06 | 6 | 350 | 4 | 200 | |
| Parameter Categories | Parameter Name/Unit | Reservoir/Formation Parameters |
|---|---|---|
| Strata parameters | Thickness/m | 2~20/20 |
| Porosity/% | 7.8/2.4 | |
| Permeability/mD | 0.45/0.05 | |
| Elastic modulus/GPa | 29.8/22.6 | |
| Poisson’s ratio | 0.23/0.31 | |
| Tensile strength/MPa | 3.8/6.4 | |
| Shear strength/MPa | 32.3/46.7 | |
| Fracture toughness/MPa∙m1/2 | 0.75 | |
| Pressure parameters | Interlaminar shear strength/MPa | 1~7 |
| Construction parameters | Net liquid volume/m3 | 300~350 |
| Displacement/m3∙min−1 | 3~3.5 | |
| Fracturing fluid viscosity/mPa∙s | 200 |
| Well Number | Interlayer Stress DifferenceMPa | Reservoir Thickness m | Displacement m3/min | Net Liquid Volume m3 | Predicted Fracture Height m | Actual Fracture Height m | Relative Error % |
|---|---|---|---|---|---|---|---|
| LX-A | 1.35 | 14.0 | 3.5 | 356.4 | 44.9 | 40 | 12.3 |
| LX-B | 3.50 | 12.8 | 3.0 | 294.0 | 26.4 | 30 | 12.0 |
| LX-C | 1.70 | 20.4 | 3.5 | 289.7 | 26.8 | 30 | 10.7 |
| LX-D | 4.25 | 19.3 | 3.5 | 338.4 | 27.3 | 30 | 9.0 |
| LX-E | 2.96 | 21.7 | 3.5 | 294.6 | 27.6 | 30 | 8.0 |
| LX-F | 1.71 | 13.3 | 3.5 | 348.9 | 37.3 | 40 | 6.8 |
| LX-G | 2.00 | 10.0 | 3.0 | 304.8 | 35.1 | 30 | 17.0 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wu, J.; Fan, B.; Wu, G.; Peng, C.; Chen, Z.; Yan, W.; Xiao, C.; Liu, W.; Wu, M.; Zou, L. Numerical Investigation of Hydraulic Fractures Vertical Propagation Mechanism for Enhanced Tight Gas Recovery. Energies 2024, 17, 3785. https://doi.org/10.3390/en17153785
Wu J, Fan B, Wu G, Peng C, Chen Z, Yan W, Xiao C, Liu W, Wu M, Zou L. Numerical Investigation of Hydraulic Fractures Vertical Propagation Mechanism for Enhanced Tight Gas Recovery. Energies. 2024; 17(15):3785. https://doi.org/10.3390/en17153785
Chicago/Turabian StyleWu, Jianshu, Baitao Fan, Guangai Wu, Chengyong Peng, Zhengrong Chen, Wei Yan, Cong Xiao, Wei Liu, Mingliang Wu, and Lei Zou. 2024. "Numerical Investigation of Hydraulic Fractures Vertical Propagation Mechanism for Enhanced Tight Gas Recovery" Energies 17, no. 15: 3785. https://doi.org/10.3390/en17153785
APA StyleWu, J., Fan, B., Wu, G., Peng, C., Chen, Z., Yan, W., Xiao, C., Liu, W., Wu, M., & Zou, L. (2024). Numerical Investigation of Hydraulic Fractures Vertical Propagation Mechanism for Enhanced Tight Gas Recovery. Energies, 17(15), 3785. https://doi.org/10.3390/en17153785








