Research on a Three-Phase Soft-Switching Inverter Based on a Simple Auxiliary Snubber Circuit
Abstract
1. Introduction
- (1)
- All switches can reliably achieve soft-switching, reducing switching losses and improving efficiency;
- (2)
- The ASC is designed with a simple structure, requiring fewer components, which simplifies the overall system design;
- (3)
- The proposed inverter operates with fewer modes and achieves higher transmission efficiency, further enhancing performance and reducing complexity.
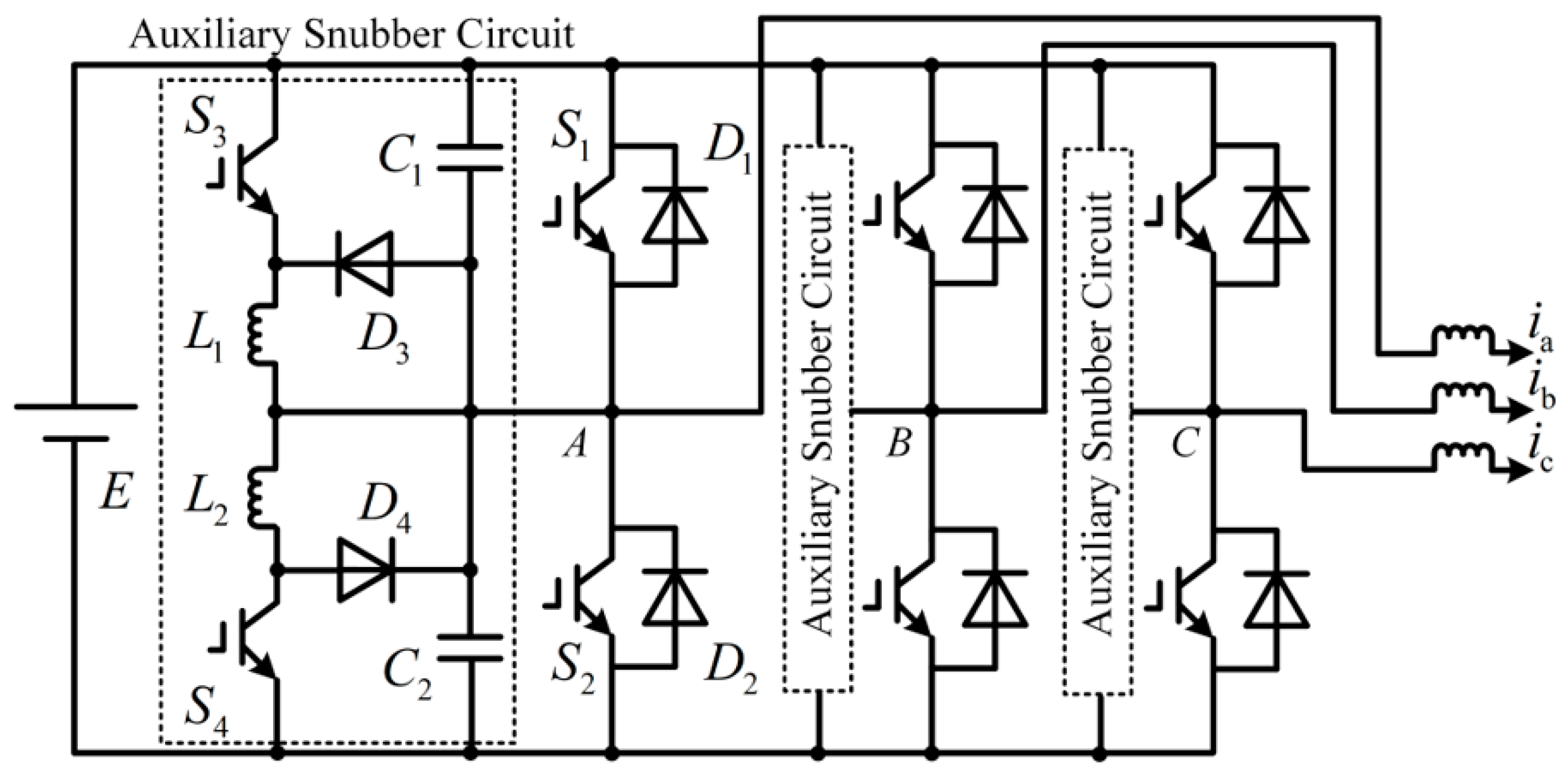
2. Circuit Topology and Operation Principle
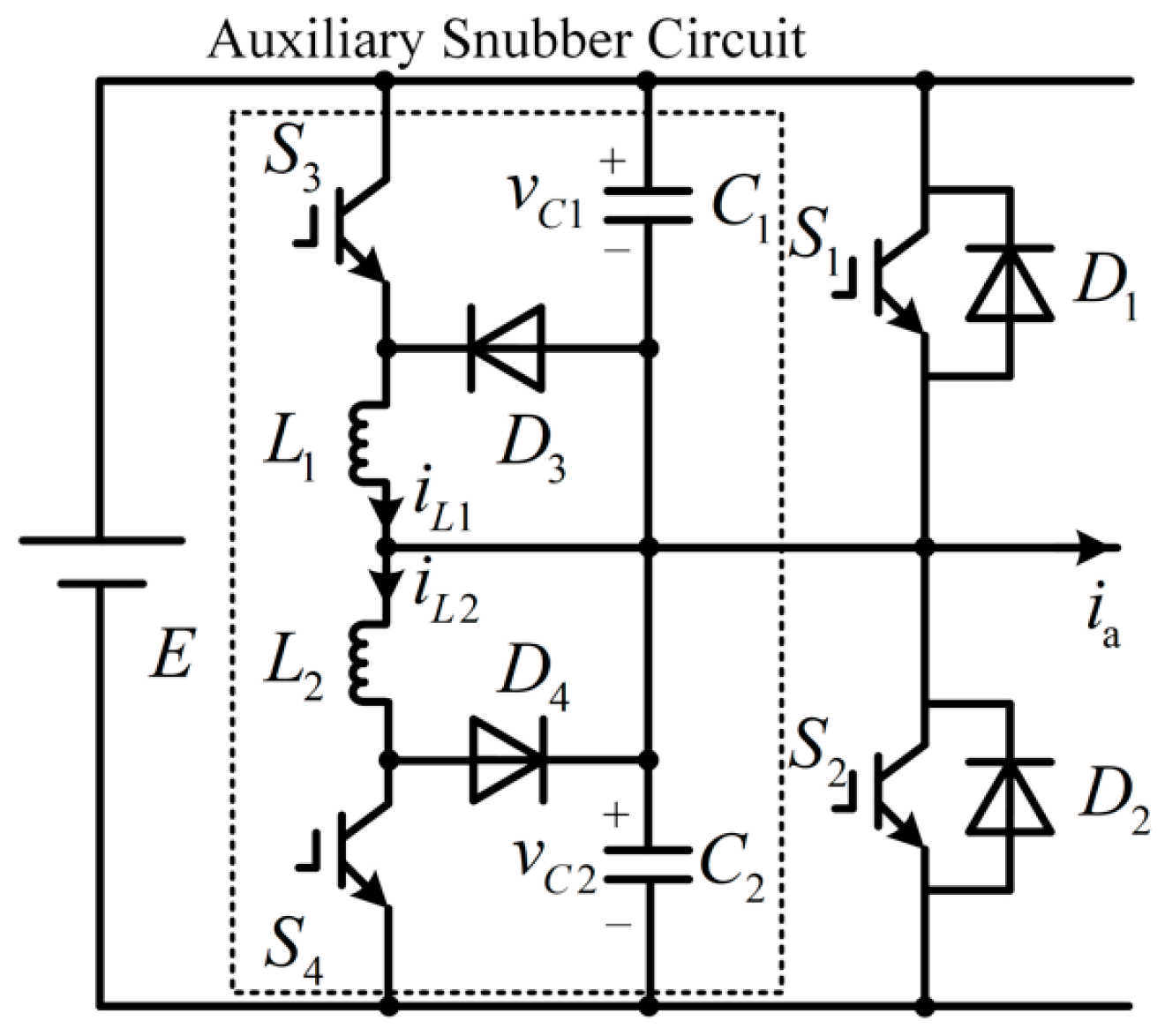
2.1. Circuit Topology
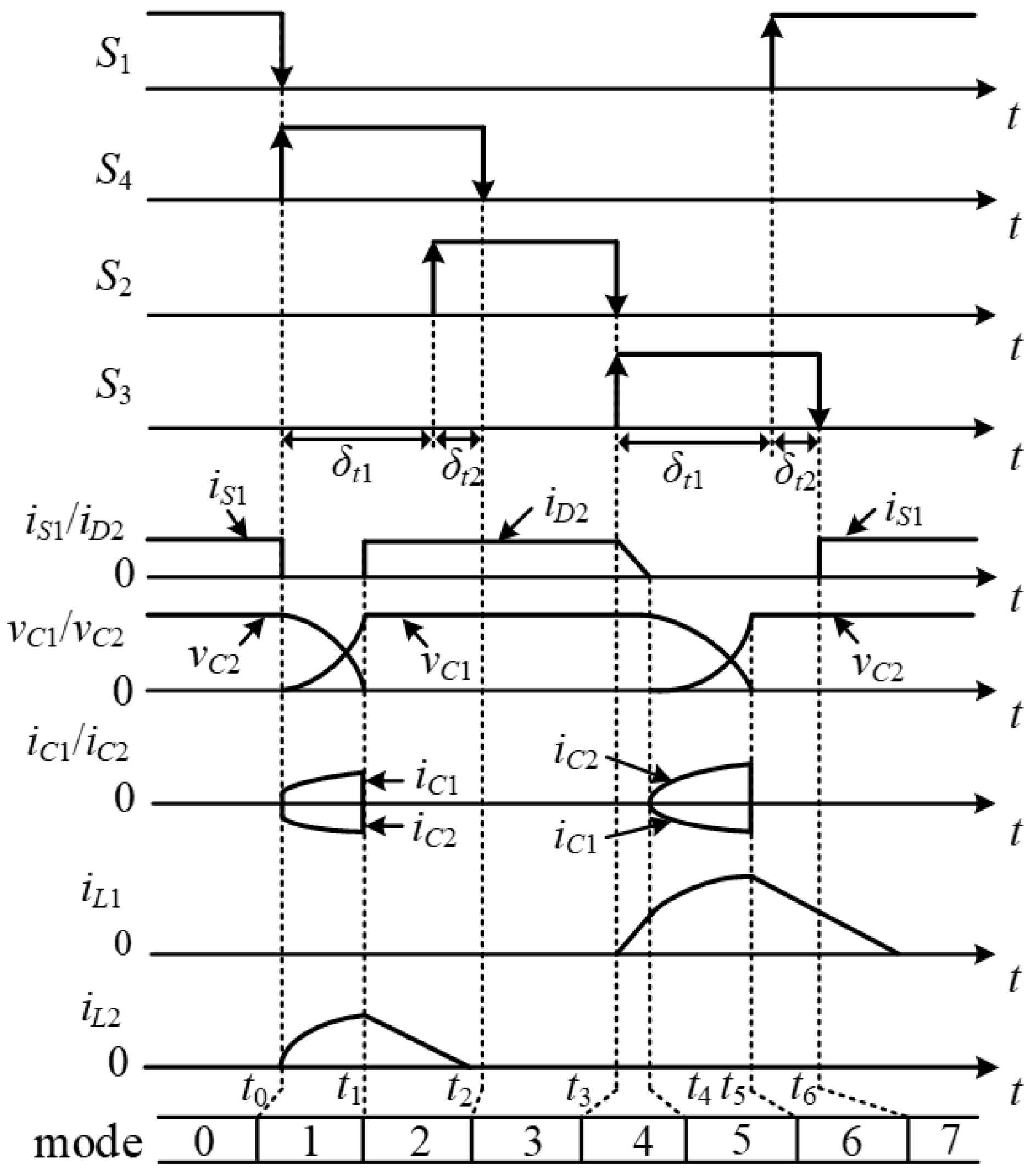
2.2. Operation Principle
3. Steady-State Characteristics
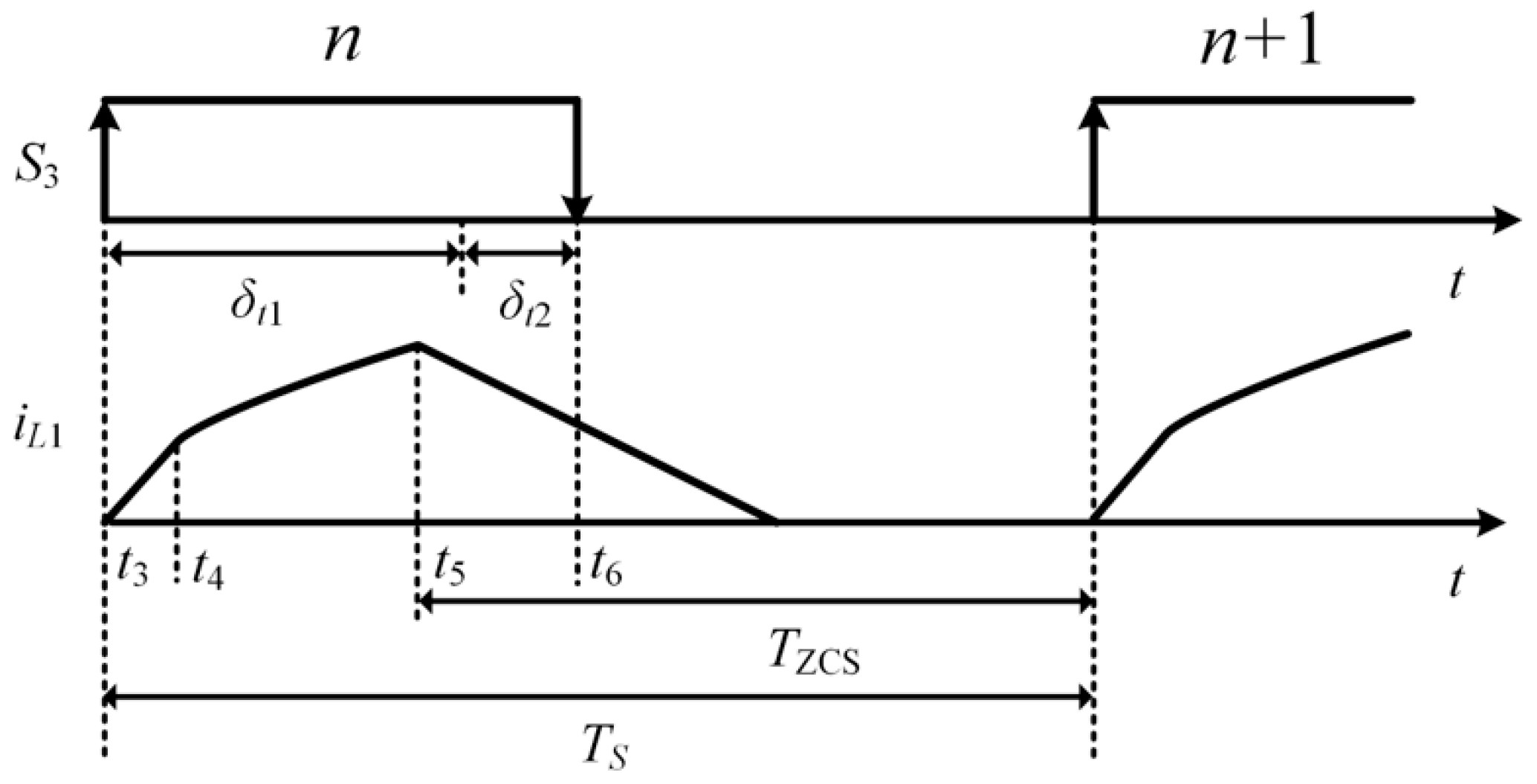
3.1. Soft-Switching Realization Condition
3.2. Number of Components and Soft-Switching Action
3.3. Voltage and Current Stress of Components
- (1)
- (2)
- (3)
- (4)
- The auxiliary switches in the proposed inverter are subjected to the lowest current stress. This stress can be further minimized by increasing the auxiliary resonant inductance L and decreasing the auxiliary resonant capacitance C.
4. Parameter Design
- (1)
- To facilitate soft-switching, the conditions specified in Equations (18)–(20) must be met;
- (2)
- The change rate of voltage should be constrained to a set limit (dv/dt)set, and similarly, the current change rate should remain within the set limit (di/dt)set. These conditions can be mathematically represented as follows:
- (3)
- To optimize the efficiency of the system, this paper tries to minimize the circulation loss of inductance current. The total circulation loss is as follows:
5. Experimental Results
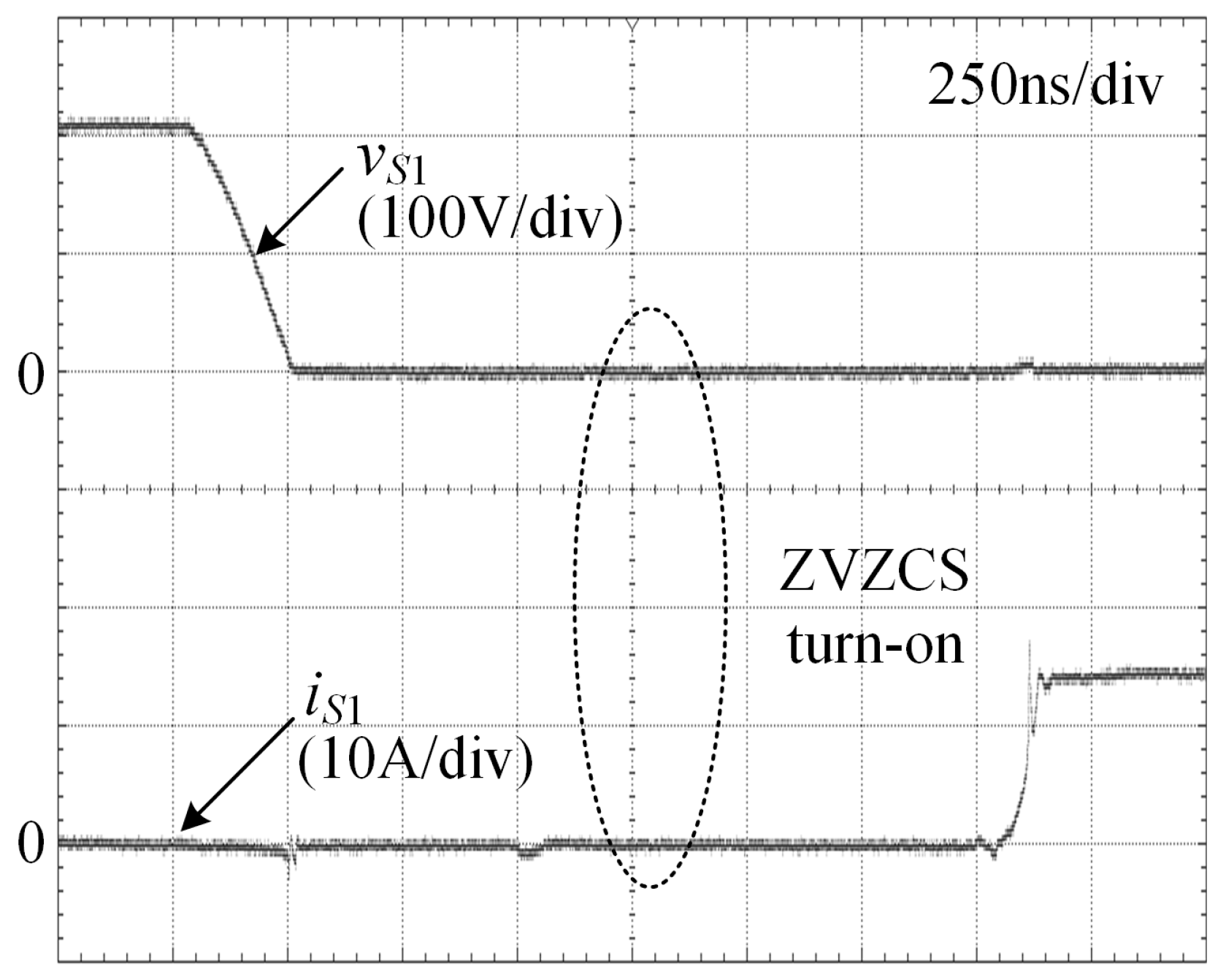
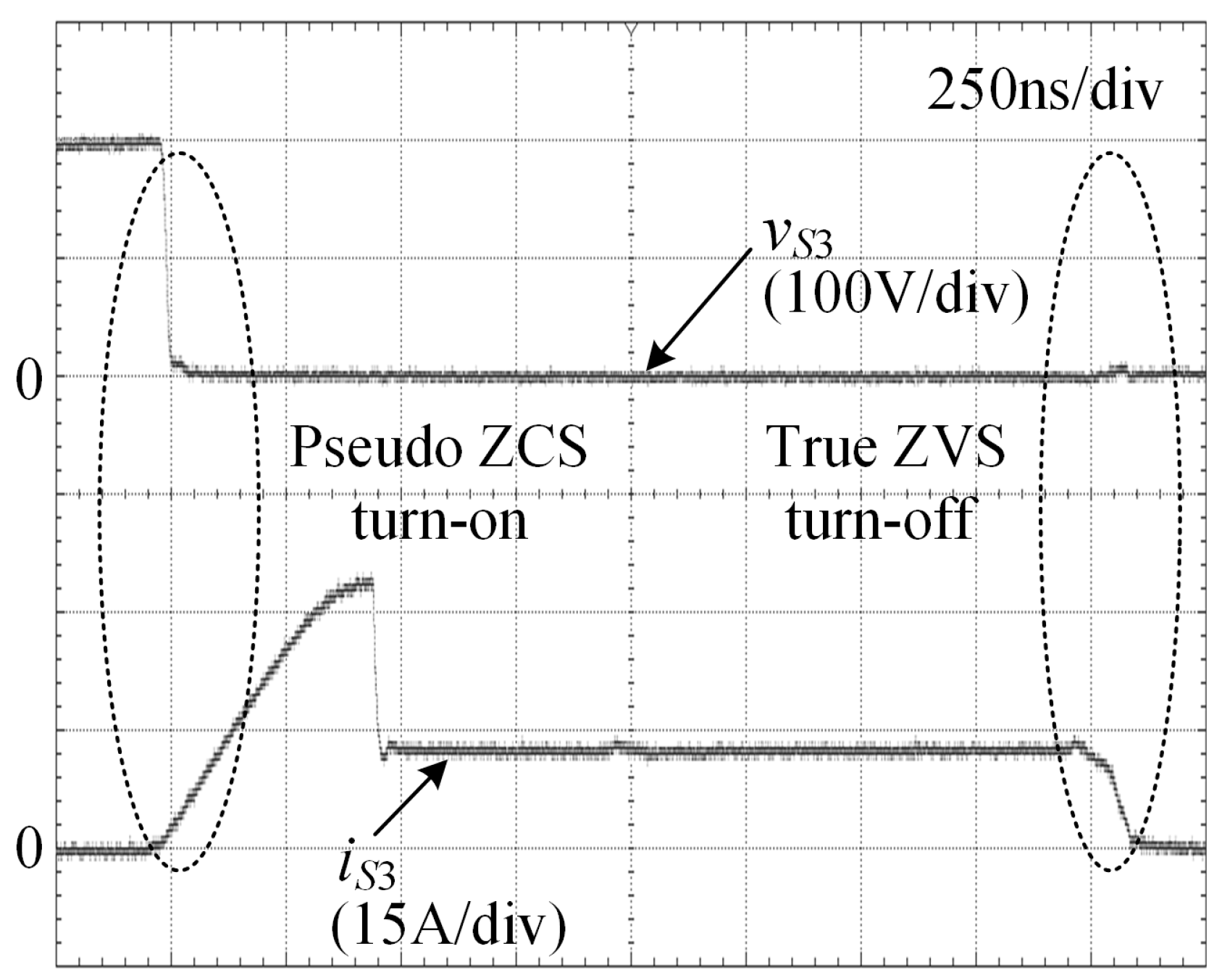
5.1. Waveforms Evaluation
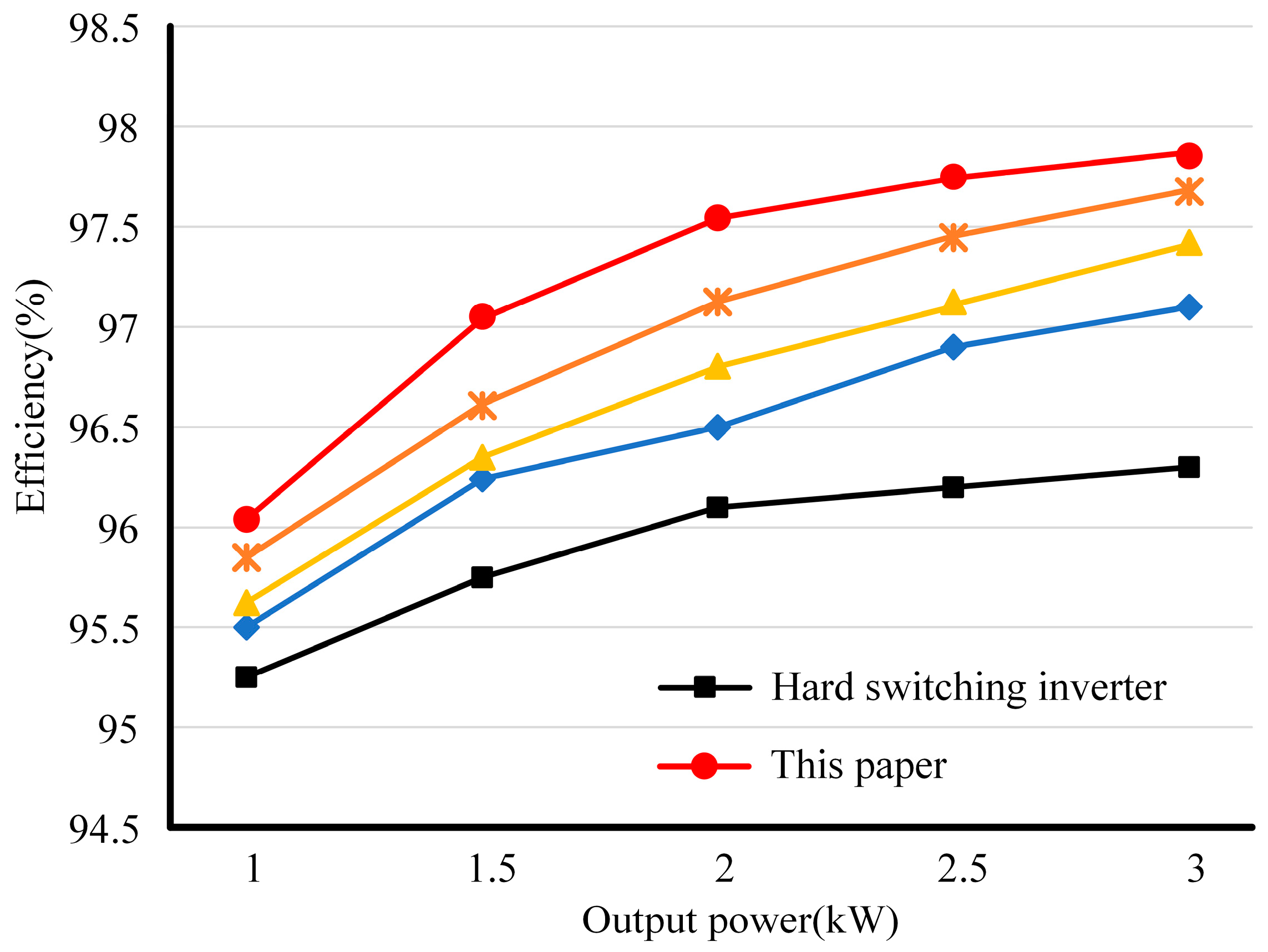
5.2. Efficiency Comparison
6. Conclusions
- (1)
- The proposed topology features a simplified structure with fewer components and reduced operating modes. This simplification not only lowers the overall cost but also enhances the inverter’s performance by making it more efficient and reliable;
- (2)
- All switches are capable of achieving soft-switching. Specifically, while auxiliary switches in [22,23,24] manage the pseudo-ZVS turn-off, the auxiliary switches in the proposed inverter achieve a true ZVS turn-off. This true ZVS turn-off significantly reduces switching losses and improves the overall efficiency and longevity of the switches by minimizing stress during transitions;
- (3)
- When compared to the ARCP inverters detailed in [22,23,24], the proposed inverter demonstrates superior transmission efficiency. This improvement is primarily due to the enhanced soft-switching capabilities and the reduced number of components, which collectively contribute to lower power losses and higher operational efficiency.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Chu, E.; Kang, Y.; Zhang, P.; Wang, Z.; Zhang, T. An SVPWM method for parallel resonant DC-Link inverter with the smallest loss in the auxiliary commutation circuit. IEEE Trans. Power Electron. 2022, 37, 1772–1787. [Google Scholar] [CrossRef]
- Chen, Y.; Xu, D. Review of Soft-Switching Topologies for Single-Phase Photovoltaic Inverters. IEEE Trans. Power Electron. 2022, 37, 1926–1944. [Google Scholar] [CrossRef]
- Li, R.; Xu, D. A zero-voltage switching three-phase inverter. IEEE Trans. Power Electron. 2014, 29, 1200–1210. [Google Scholar]
- Kedarisetti, J.; Mutschler, P. A motor-friendly quasi-resonant DC-link inverter with lossless variable zero-voltage duration. IEEE Trans. Power Electron. 2012, 27, 2613–2622. [Google Scholar] [CrossRef]
- Jafar, J.; Fernandes, B. A new quasi-resonant DC-link PWM inverter using single switch for soft switching. IEEE Trans. Power Electron. 2002, 17, 1010–1016. [Google Scholar] [CrossRef]
- Wang, Q.; Guo, G.; Wang, Y.; Chen, J. An Efficient Three-Phase Resonant DC-Link Inverter with Low Energy Consumption. IEEE Trans. Power Electron. 2021, 36, 702–715. [Google Scholar] [CrossRef]
- Divan, D. The resonant DC link converter-a new concept in static power conversion. IEEE Trans. Ind. Appl. 1989, 25, 317–325. [Google Scholar] [CrossRef]
- Chu, E.; Zhang, T.; Wang, Z. Auxiliary resonant commutated pole soft-switching inverter with simple topology. J. Power Electron. 2022, 22, 198–209. [Google Scholar] [CrossRef]
- McMurray, W. Resonant snubbers with auxiliary switches. In Proceedings of the Conference Record of the IEEE Industry Applications Society Annual Meeting, San Diego, CA, USA, 1–5 October 1989; pp. 289–834. [Google Scholar]
- Doncker, R.W.D.; Lyons, J.P. The auxiliary resonant commutated pole converter. In Proceedings of the Conference Record of the 1990 IEEE Industry Applications Society Annual Meeting, Seattle, WA, USA, 7–12 October 1990; pp. 1228–1235. [Google Scholar]
- Lai, J.; Young, S.; Ott, J.; McKeever, J.; Fang, Z. A delta-configured auxiliary resonant snubber inverter. IEEE Trans. Ind. Appl. 1996, 32, 518–525. [Google Scholar]
- Lai, J. Practical design methodology of auxiliary resonant snubber inverters. In Proceedings of the PESC Record. 27th Annual IEEE Power Electronics Specialists Conference, Baveno, Italy, 23–27 June 1996; pp. 432–437. [Google Scholar]
- Yamamoto, H.; Kaneda, M.; Nakaoka, M. Three-phase soft-switching inverter resonant with unique resonant snubbers. In Proceedings of the IEEE 1999 International Conference on Power Electronics and Drive Systems, Hong Kong, China, 27–29 July 1999; pp. 1078–1083. [Google Scholar]
- Lai, J.; Zhang, J.; Yu, H.; Kouns, H. Source and load adaptive design for a high-power soft-switching inverter. IEEE Trans. Power Electron. 2006, 21, 1667–1675. [Google Scholar] [CrossRef]
- Russi, J.; Martins, M.; Hey, H. Coupled-filter-inductor soft-switching techniques: Principles and topologies. IEEE Trans. Ind. Electron. 2008, 55, 3361–3373. [Google Scholar] [CrossRef]
- Yu, W.; Lai, J.; Park, S. An improved zero-voltage switching inverter using two coupled magnetics in one resonant pole. IEEE Trans. Power Electron. 2010, 25, 952–961. [Google Scholar]
- Yuan, X.; Barbi, I. Analysis, designing, and experimentation of a transformer-assisted PWM zero-voltage switching pole inverter. IEEE Trans. Power Electron. 2000, 15, 72–82. [Google Scholar] [CrossRef]
- Inaba, C.; Yamazaki, T.; Yoshida, M.; Hiraki, E.; Konishi, Y.; Nakaoka, M. Three phase soft switching inverter with pulse current transformer-assisted resonant snubbers. IEEE Int. Conf. Ind. Electron. 2001, 2, 1106–1111. [Google Scholar]
- Turzynski, M.; Chrzan, P.; Kolincio, M.; Burkiewicz, S. Quasi resonant DC-link voltage inverter with enhanced zero-voltage switching control. In Proceedings of the 19th European Conference on Power Electronics and Applications (EPE’17 ECCE Europe), Warsaw, Poland, 11–14 September 2017; pp. 1–8. [Google Scholar]
- Wang, Q.; Wang, Y. Research on a novel high-efficiency three-phase resonant pole soft-switching inverter. IEEE Trans. Power Electron. 2021, 36, 5845–5857. [Google Scholar] [CrossRef]
- Chu, E.; Wu, M.; Huang, L.; Hou, X.; Zhang, H. Research on a novel modulation strategy for auxiliary resonant commutated pole inverter with the smallest loss in auxiliary commutation circuits. IEEE Trans. Power Electron. 2014, 29, 1103–1117. [Google Scholar]
- Chu, E.; Zhang, X.; Sun, Q.; Li, S.; Xiong, H.; Yang, X. Three-phase double auxiliary resonant commutated pole inverter topology and analysis of its working principle. IET Power Electron. 2016, 9, 1536–1545. [Google Scholar] [CrossRef]
- Chu, E.; Zhang, X.; Huang, L. Research on a novel modulation strategy for double auxiliary resonant commutated pole soft-switching inverter with the shunt dead time. IEEE Trans. Power Electron. 2016, 31, 6855–6869. [Google Scholar] [CrossRef]
- Chu, E.; Chen, Z.; Xie, H.; Zhang, H. Modified double auxiliary resonant commutated pole inverter and its modulation strategy. IEEE J. Emerg. Sel. Topics Power Electron. 2020, 8, 4467–4481. [Google Scholar] [CrossRef]



| Components | Auxiliary Switch | Auxiliary Diode | Resonant Inductor | Resonant Capacitor |
|---|---|---|---|---|
| [22] | 2 | 8 | 4 | 6 |
| [23] | 4 | 8 | 4 | 6 |
| [24] | 4 | 4 | 2 | 6 |
| This paper | 2 | 2 | 2 | 2 |
| Switching | (S1, S2) | (S3, S4) | (S5, S6) | |
| [22] | Turn-on | ZVZCS | Pseudo ZCS | — |
| Turn-off | Pseudo ZVS | Pseudo ZVS | — | |
| [23] | Turn-on | ZVZCS | Pseudo ZCS | ZVZCS |
| Turn-off | Pseudo ZVS | Pseudo ZVS | ZVZCS | |
| [24] | Turn-on | ZVZCS | Pseudo ZCS | ZVZCS |
| Turn-off | Pseudo ZVS | Pseudo ZVS | True ZVS | |
| This paper | Turn-on | ZVZCS | Pseudo ZCS | — |
| Turn-off | Pseudo ZVS | True ZVS | — | |
| Voltage Stress | (S1, S2) | (S3, S4) | (S5, S6) |
| [22] | E | E | — |
| [23] | E | E | E |
| [24] | E | E | E |
| This paper | E | E | — |
| Current stress | (S1, S2) | (S3, S4) | (S5, S6) |
| [22] | iamax | — | |
| [23] | iamax | ||
| [24] | iamax | ||
| This paper | iamax | — |
| Components | Parameters |
|---|---|
| Control board | DSP TMS320F2812 |
| Driver chip | SKHI23/17 |
| Input DC voltage (E) | 200 V |
| Switching frequency (fs) | 16 kHz |
| S1(D1)–S2(D2) | SKM75GB063D (600 V, 100 A) |
| S3–S4 | IRG4PC50K (600 V, 52 A) |
| D3~D4 | RHRG7560(600 V, 75 A) |
| C1–C2 | 10 nF |
| L1–L2 | 2 μH |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dai, D.; Zheng, H. Research on a Three-Phase Soft-Switching Inverter Based on a Simple Auxiliary Snubber Circuit. Energies 2024, 17, 3653. https://doi.org/10.3390/en17153653
Dai D, Zheng H. Research on a Three-Phase Soft-Switching Inverter Based on a Simple Auxiliary Snubber Circuit. Energies. 2024; 17(15):3653. https://doi.org/10.3390/en17153653
Chicago/Turabian StyleDai, Dawei, and Hua Zheng. 2024. "Research on a Three-Phase Soft-Switching Inverter Based on a Simple Auxiliary Snubber Circuit" Energies 17, no. 15: 3653. https://doi.org/10.3390/en17153653
APA StyleDai, D., & Zheng, H. (2024). Research on a Three-Phase Soft-Switching Inverter Based on a Simple Auxiliary Snubber Circuit. Energies, 17(15), 3653. https://doi.org/10.3390/en17153653





