Advanced Static Var Generator in the Reactive Power Automatic Compensation System of an Underground Mine with a Thyristor Hoisting Machine
Abstract
1. Introduction
2. Materials and Methods
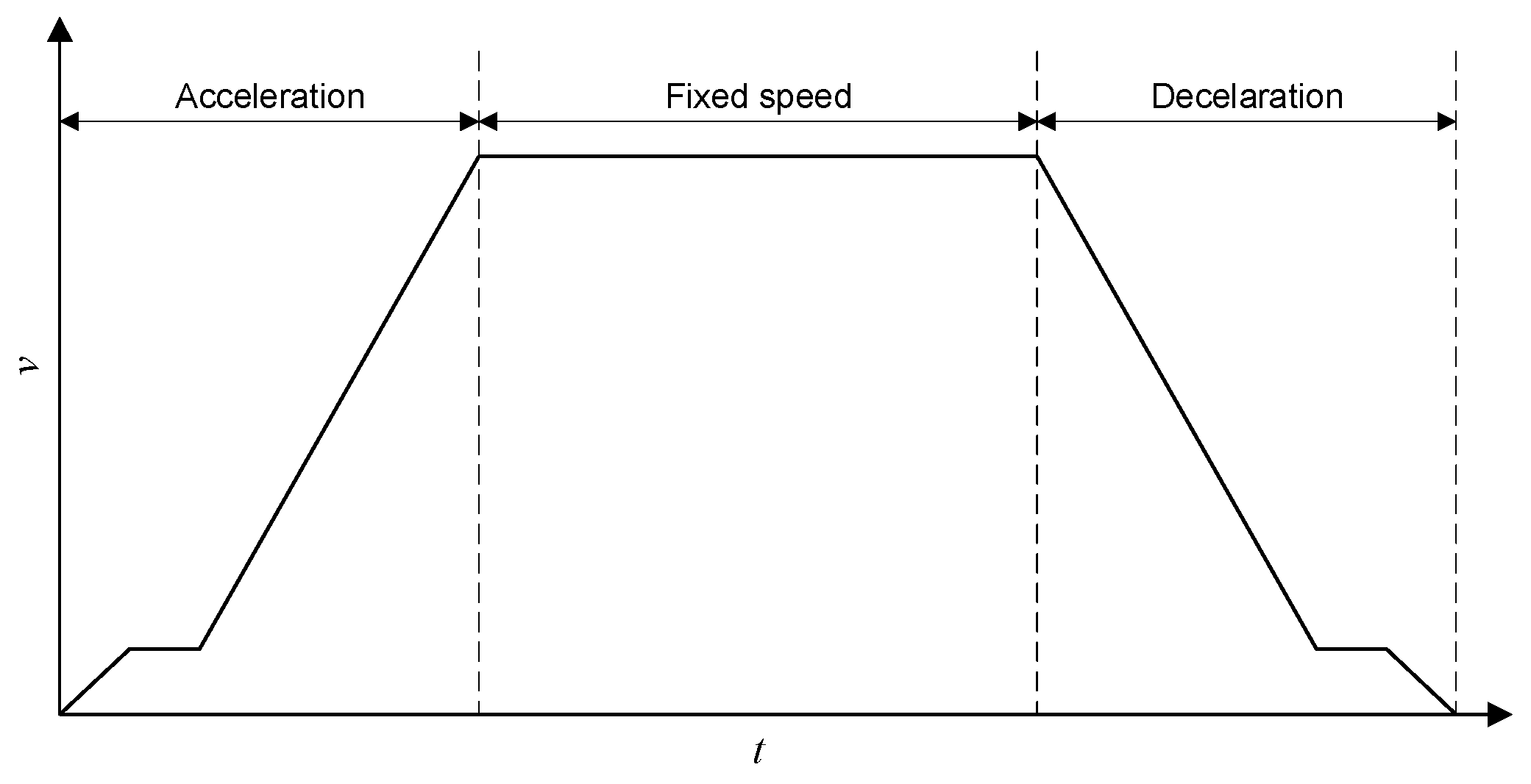
2.1. The Impact of Hoisting Machines on the PQ of the Mine Power Grid
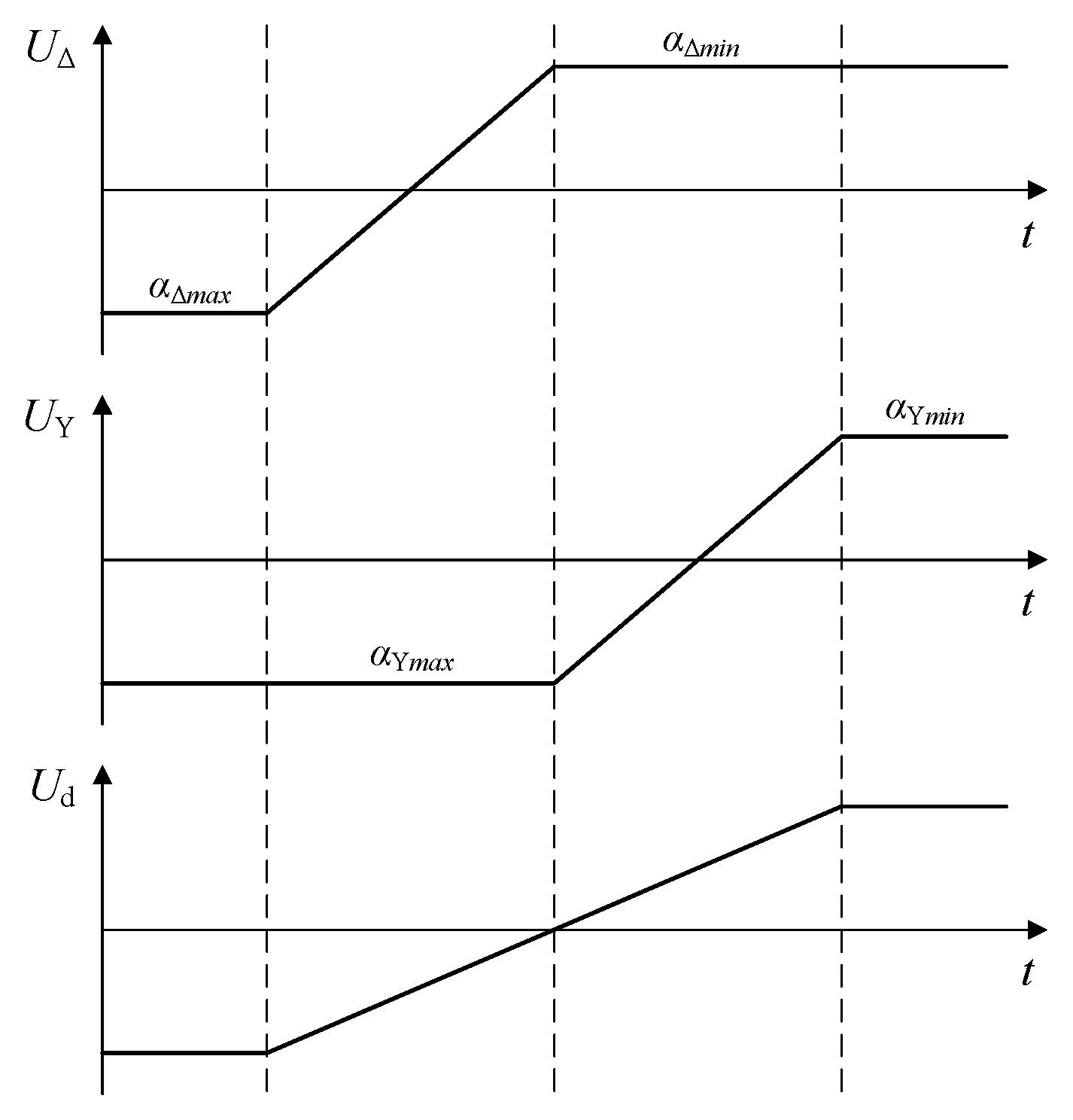
2.2. The Thyristor Hoisting Machine Higher Current Harmonics
- i(t)—current of the primary side of the transformer;
- Id—current in the DC circuit;
- υi—transformer current ratio;
- αY, αΔ—firing angles for Y and Δ rectifiers;
- n—harmonic order.
2.3. Static Var Generator
- Reduction (for reduction in a wider range, APF is used) of higher current harmonics in the grid;
- Reducing (or completely eliminating) the possibility of occurrence of resonance phenomena in the grid for higher harmonics;
- Stepless regulation of reactive power;
- Reactive power compensation, both inductive and capacitive loads;
- Reducing grid voltage fluctuations and light flickering;
- Reduction of asymmetry (balancing) in multiphase systems;
- Support for the power grid in FRT (Fault Ride Through), e.g., LVRT (Low Voltage Ride Through) or O(H)VRT (Over Voltage or High Voltage Ride Through).
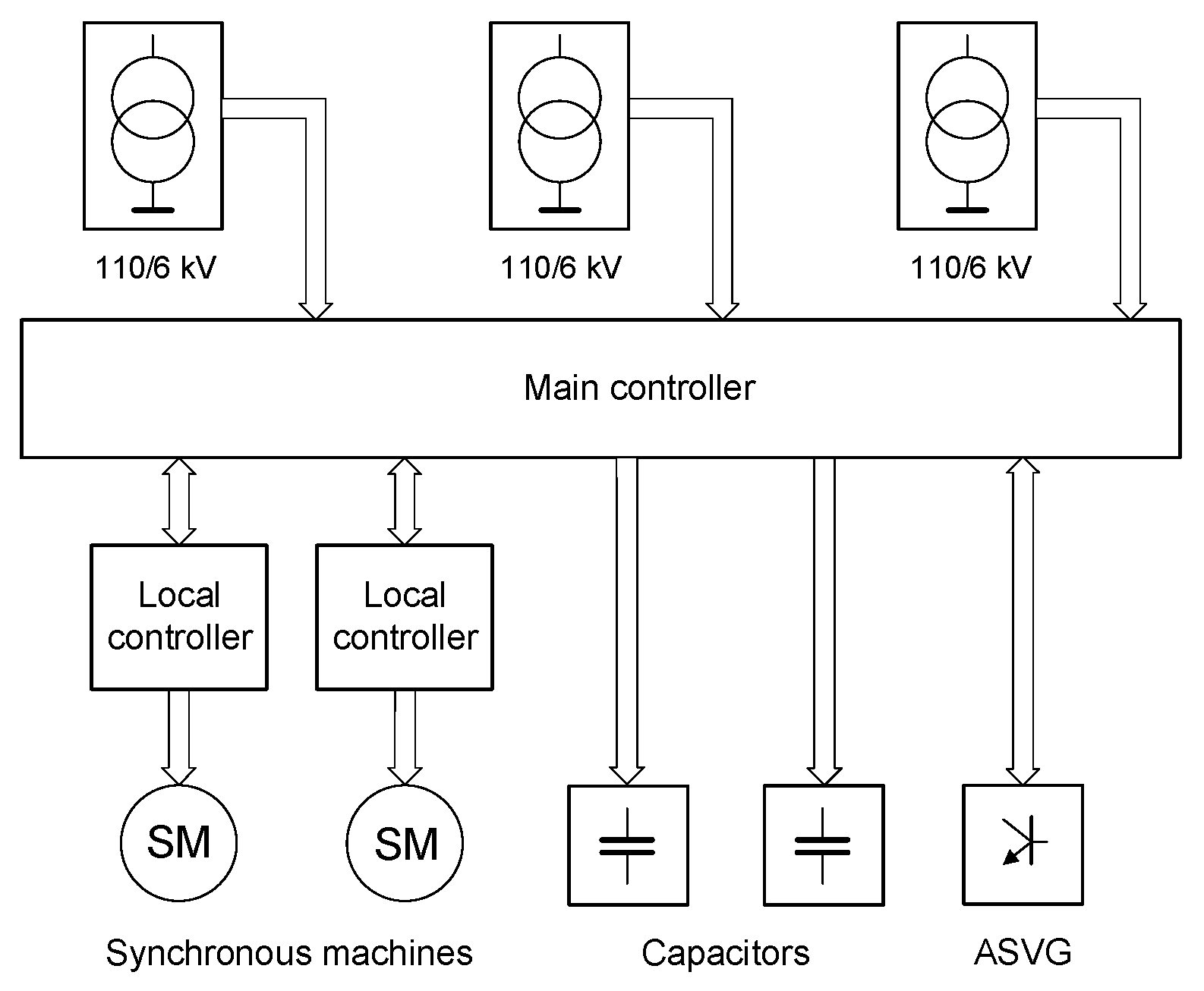
2.4. Reactive Power Automatic Compensation System
- tg φ—power coefficient;
- P—actual fundamental active power at the plant PCC;
- Q—actual fundamental reactive power at the plant PCC.
- ΔQz—required change in the fundamental reactive power at the plant PCC;
- tg φz—set value of power coefficient at the plant PCC.
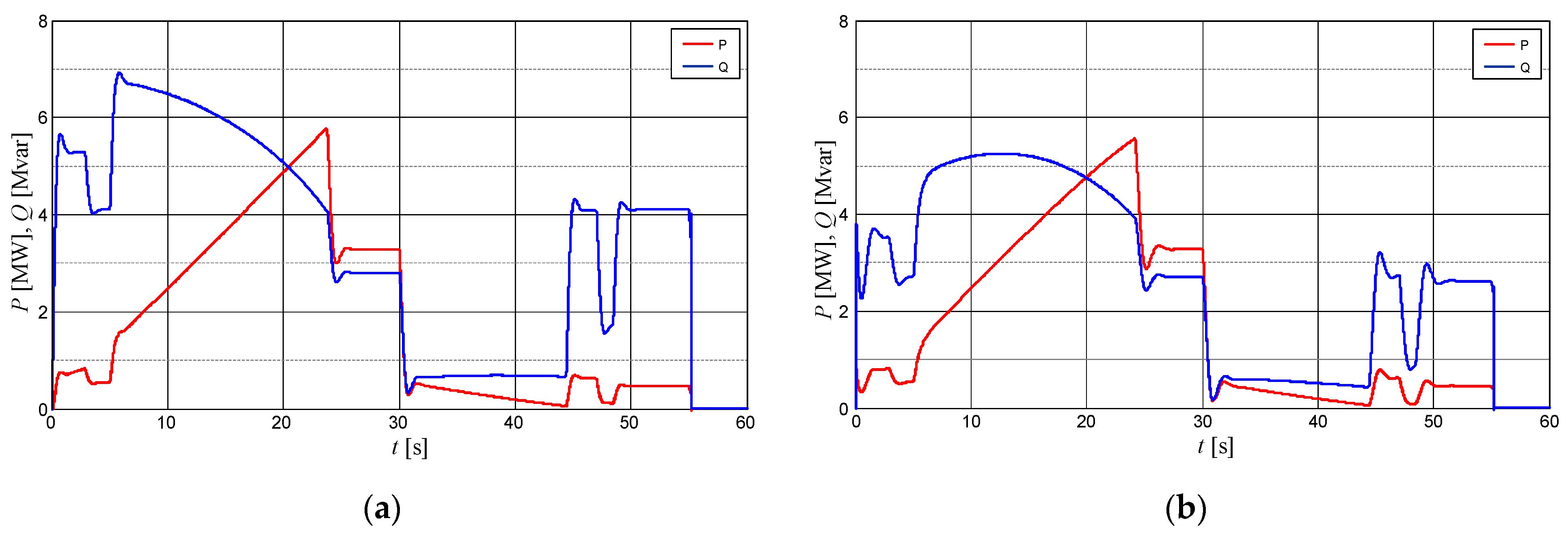
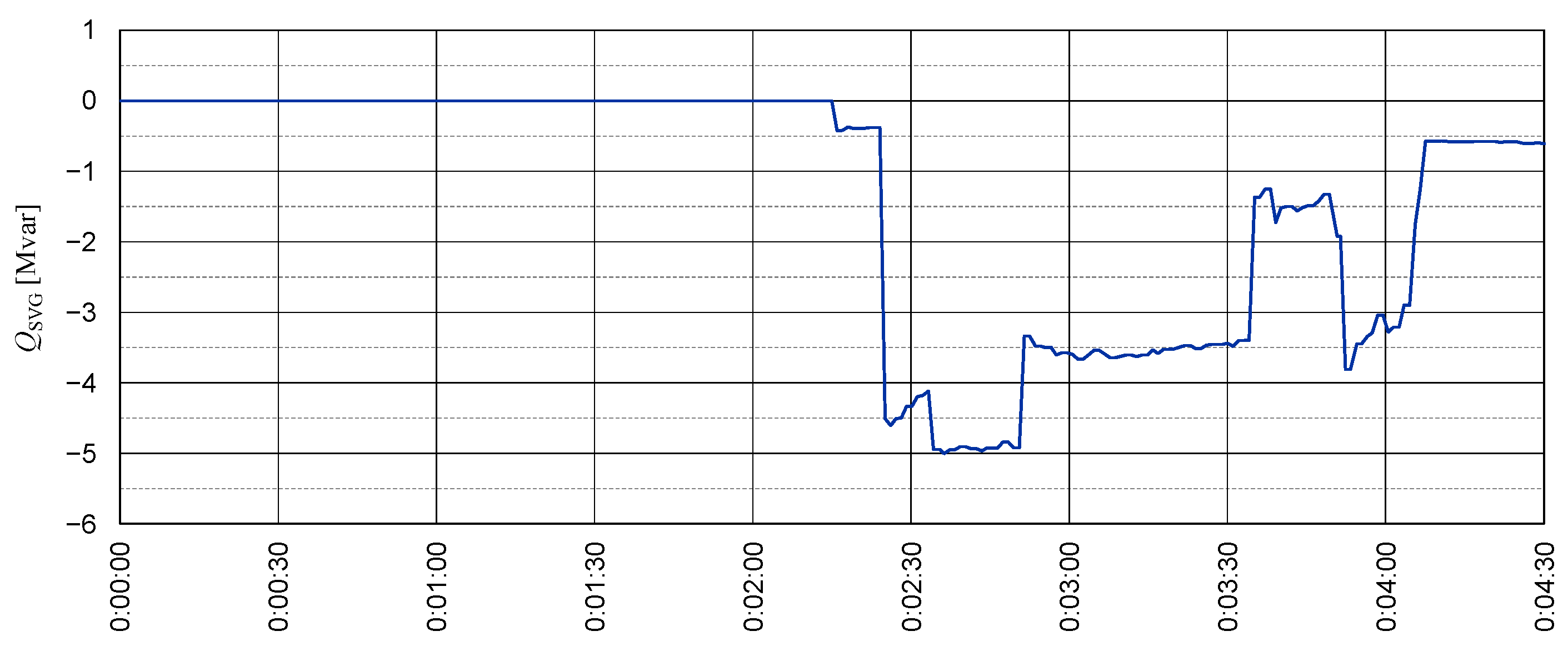
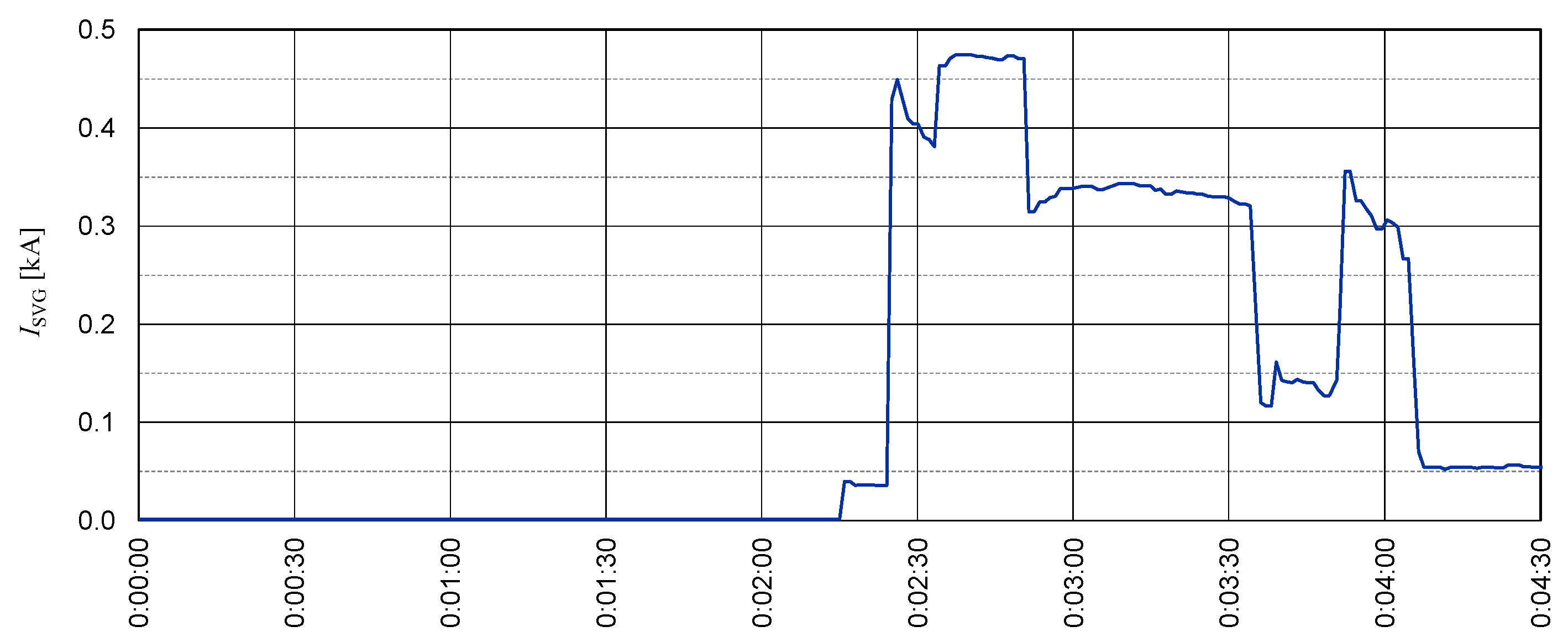
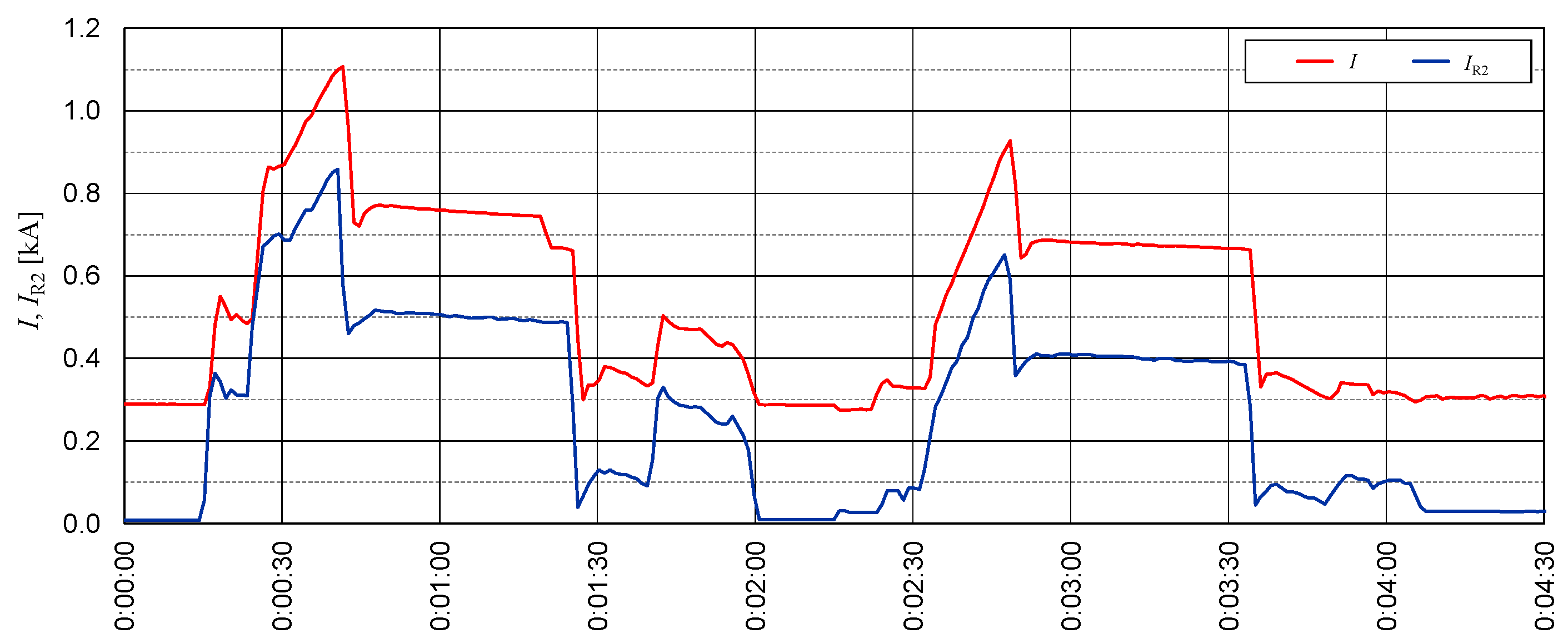
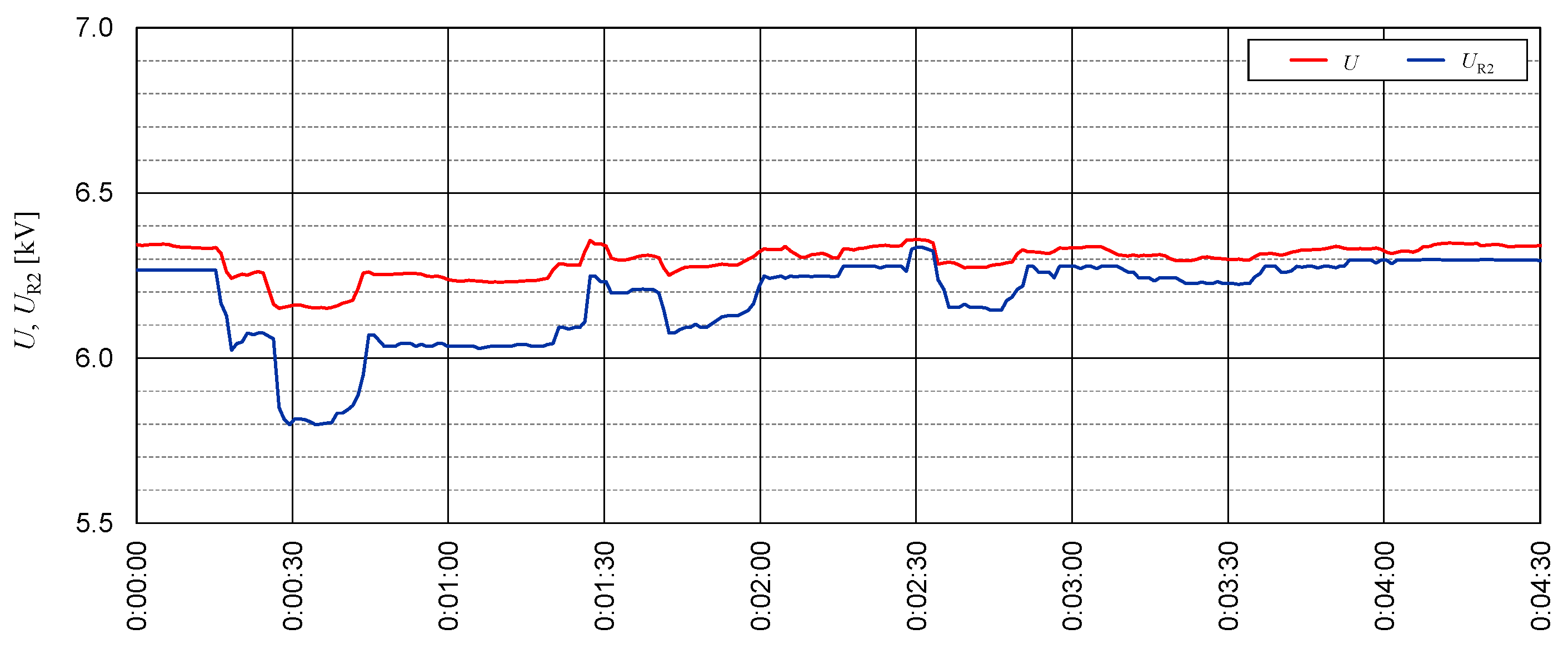
2.5. Case Study
3. Results and Discussion
4. Summary and Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Motlanthe, N.; Muremi, L. Application of static var compensator with harmonic filters in the heavy industry. In Proceedings of the 2019 Southern African Universities Power Engineering Conference/Robotics and Mechatronics/Pattern Recognition Association of South Africa (SAUPEC/RobMech/PRASA), Bloemfontein, South Africa, 28–30 January 2019; pp. 235–240. [Google Scholar]
- Roos, F.; Bansal, R.C. Reactive power and harmonic compensation: A case study for the coal-mining industry. J. Energy S. Afr. 2019, 30, 34–48. [Google Scholar] [CrossRef]
- Kgori, P.; Okojie, D.E.; Akuru, U.B. Design and Analysis of a Proposed Multistage Capacitor Bank Compensation Scheme. In Proceedings of the 2022 IEEE PES/IAS PowerAfrica, Kigali, Rwanda, 22–26 August 2022; pp. 1–5. [Google Scholar]
- Dixon, J.; Moran, L.; Rodriguez, J.; Domke, R. Reactive Power Compensation Technologies: State-of-the-Art Review. Proc. IEEE 2005, 93, 2144–2164. [Google Scholar] [CrossRef]
- Aviña-Corral, V.; Rangel-Magdaleno, J.; Hernandez-Perez, J. 63-Level Asymmetric Capacitor an Inductor Bank without Discharge Resistors and Reduced Transients. In Proceedings of the 2023 IEEE International Autumn Meeting on Power, Electronics and Computing (ROPEC), Ixtapa, Mexico, 18–20 October 2023; pp. 1–6. [Google Scholar]
- Varetsky, Y.; Hanzelka, Z. Filter characteristics in a DC drive power supply system. In Proceedings of the 2008 13th International Conference on Harmonics and Quality of Power, Woolongon, NSW, Australia, 28 September–1 October 2008; pp. 1–6. [Google Scholar]
- Jiangzi, M.L. Measure and Suppression of Harmonic Currents for Heavy-Duty Hoisting Machines. In Proceedings of the 2005 IEEE/PES Transmission & Distribution Conference & Exposition: Asia and Pacific, Dalian, China, 18 August 2005; pp. 1–4. [Google Scholar]
- Pravitasari, D.; Firmansyah, E.; Haryono, T. Harmonic current elimination in industrial power systems. In Proceedings of the 2015 2nd International Conference on Information Technology, Computer, and Electrical Engineering (ICITACEE), Semarang, Indonesia, 16–18 October 2015; pp. 358–362. [Google Scholar]
- Li, D.; Wang, T.; Pan, W.; Ding, X.; Gong, J. A comprehensive review of improving power quality using active power filters. Electr. Power Syst. Res. 2021, 199, 107389. [Google Scholar] [CrossRef]
- Habrych, M.; Wisniewski, G.; Miedzinski, B.; Wosik, J.; Kozlowski, A. Power loss due to high harmonics in power transformer when use for dc drive of hoisting machines. In Proceedings of the 2014 International Conference on Information Science, Electronics and Electrical Engineering, Sapporo, Japan, 26–28 April 2014; pp. 2065–2070. [Google Scholar]
- Semenov, A.S. Analyzing Impacts of Higher Harmonics on Electricity Quality Parameters at Mining Facilities. In Proceedings of the 2024 International Conference on Industrial Engineering, Applications and Manufacturing (ICIEAM), Sochi, Russia, 20–24 May 2024; pp. 321–325. [Google Scholar]
- Bebikhov, Y.V.; Egorov, A.N.; Semenov, A.S. How Higher Harmonics Affect the Electrical Facilities in Mining Power Systems. In Proceedings of the 2020 International Conference on Industrial Engineering, Applications and Manufacturing (ICIEAM), Sochi, Russia, 18–22 May 2020; pp. 1–7. [Google Scholar]
- IEEE Std 519-2022 (Revision of IEEE Std 519-2014); IEEE Standard for Harmonic Control in Electric Power Systems. IEEE: Piscataway, NJ, USA, 2022.
- EN 50160:2023-10; Voltage Characteristics of Electricity Supplied by Public Distribution Networks. CENELEC: Brussels, Belgium, 2023.
- Siostrzonek, T.; Chmielowiec, K.; Piątek, K.; Dutka, M.; Firlit, A. The use of multi-pulse systems in the power supply of hoisting machine drives to improve voltage parameters in mining plants. In Proceedings of the 2020 12th International Conference and Exhibition on Electrical Power Quality and Utilisation (EPQU), Cracow, Poland, 14–15 September 2020; pp. 1–6. [Google Scholar]
- Hyla, M. Reactive power compensation in a 6 kV power grid supplying a 12-pulse thyristor hoisting machine. Prz. Elektrotech. 2022, 98, 55–63. [Google Scholar] [CrossRef]
- Ahsan, F.M.; Chatterjee, J.K.; Das, A. Operation of a 12-pulse converter in closed loop for controlled P-Q operation. In Proceedings of the 2006 International Conference on Power Electronic, Drives and Energy Systems, New Delhi, India, 12–15 December 2006; pp. 1–6. [Google Scholar]
- Das, A.; Chatterjee, J.K.; Gaja, A.K. Asymmetrical firing of 12-pulse converter for controlled P-Q operation using PIC microcontroller. In Proceedings of the 2006 IEEE Power India Conference, New Delhi, India, 10–12 April 2006. [Google Scholar]
- Hamad, M.S.; Masoud, M.I.; Finney, S.J.; Williams, B.W. Performance analysis of medium voltage series connected 12-pulse current source controlled rectifier using static VAR compensator. In Proceedings of the 2008 IEEE International Symposium on Industrial Electronics, Cambridge, UK, 30 June–2 July 2008; pp. 282–286. [Google Scholar]
- Modi, P.S.; Joshi, S.K. New combined Hybrid active filter for twelve pulse converter operating under asymmetrical operation. In Proceedings of the Australasian Universities Power Engineering Conference AUPEC, Brisbane, QLD, Australia, 25–28 September 2011; pp. 1–6. [Google Scholar]
- Modi, P.S.; Joshi, S.K. Effect of source inductance on controlled var operation of 12-pulse converter. In Proceedings of the 2009 International Conference on Control, Automation, Communication and Energy Conservation, Perundurai, India, 4–6 June 2009; pp. 1–7. [Google Scholar]
- Hamad, M.S.; Masoud, M.I.; Williams, B.W. Medium-Voltage 12-Pulse Converter: Output Voltage Harmonic Compensation Using a Series APF. IEEE Trans. Ind. Electron. 2014, 61, 43–52. [Google Scholar] [CrossRef]
- Hamad, M.S.; Masoud, M.I.; Massoud, A.M.; Finney, S.J.; Williams, B.W. A new power locus for the p-q operation of series connected 12-pulse current source controlled converters. In Proceedings of the 2008 IEEE Power Electronics Specialists Conference, Rhodes, Greece, 15–19 June 2008; pp. 2264–2270. [Google Scholar]
- Kowalak, R. Modelling of Power Electronic Compensators for the Analysis of Power System Operation. Acta Energ. Power Eng. Q. 2013, 17, 48–55. [Google Scholar] [CrossRef]
- Igbinovia, F.O.; Fandi, G.; Švec, J.; Müller, Z.; Tlusty, J. Comparative review of reactive power compensation technologies. In Proceedings of the 16th International Scientific Conference on Electric Power Engineering EPE, Kouty and Desnou, Czech Republic, 20–22 May 2015; pp. 2–7. [Google Scholar]
- Buła, D.; Michalak, J.; Zygmanowski, M.; Adrikowski, T.; Jarek, G.; Jeleń, M. Control Strategy of 1 kV Hybrid Active Power Filter for Mining Applications. Energies 2021, 14, 4994. [Google Scholar] [CrossRef]
- Buła, D.; Jarek, G.; Michalak, J.; Zygmanowski, M. Control Method of Four Wire Active Power Filter Based on Three-Phase Neutral Point Clamped T-Type Converter. Energies 2021, 14, 8427. [Google Scholar] [CrossRef]
- Li, Y.; Wang, C.; Zhao, X.; Zhang, K. Research of Mining STATCOM Based on Hybrid Multilevel H-bridge Inverter. Energy Power Eng. 2013, 5, 636–641. [Google Scholar] [CrossRef]
- Sun, R.; Qiu, H.; Chen, B.; Yuan, X.; Luo, S.; Gu, W. Voltage sag improvement using DVR and SVG in power systems. In Proceedings of the 2016 China International Conference on Electricity Distribution (CICED), Xi’an, China, 10–13 August 2016; pp. 1–5. [Google Scholar]
- Ma, Y.; Huang, A.; Zhou, X. A review of STATCOM on the electric power system. In Proceedings of the 2015 IEEE International Conference on Mechatronics and Automation (ICMA), Beijing, China, 2–5 August 2015; pp. 162–167. [Google Scholar]
- Ren, L.; Wang, F.; Shi, Y.; Gao, L. Coupling Effect Analysis and Design Principle of Repetitive Control Based Hybrid Controller for SVG with Enhanced Harmonic Current Mitigation. IEEE J. Emerg. Sel. Top. Power Electron. 2022, 10, 5659–5669. [Google Scholar] [CrossRef]
- Peng, F.Z.; Lai, J.-S. Dynamic performance and control of a static VAr generator using cascade multilevel inverters. IEEE Trans. Ind. Appl. 1997, 33, 748–755. [Google Scholar] [CrossRef]
- Cheng, H.; Yin, X.; Wang, Z.; Chen, S. Control Analysis and Stability for Static VAR Generator. IFAC Proc. Vol. 2003, 36, 1025–1030. [Google Scholar] [CrossRef]
- Luo, R.; He, Y.; Tu, Y.; Chen, X.; Liu, J. Research on the Unbalanced Compensation Range of Delta-connected Cascaded H-bridge Multilevel SVG. In Proceedings of the 2018 International Power Electronics Conference (IPEC-Niigata 2018-ECCE Asia), Niigata, Japan, 20–24 May 2018; pp. 3439–3444. [Google Scholar]
- Fujun, M.; An, L.; Qiaopo, X.; Zhixing, H.; Qianming, X. Derivation of zero-sequence circulating current and the compensation of delta-connected static var generators for unbalanced load. IET Power Electron. 2016, 9, 576–588. [Google Scholar] [CrossRef]
- Yu, Y.; Xu, D.; Wu, J.; Xu, R.; Yu, Y.; Yang, R.; Yan, J. Research and design on multi-target current tracking control strategy of cascaded H-bridge static var generator. In Proceedings of the 2014 17th International Conference on Electrical Machines and Systems (ICEMS), Hangzhou, China, 22–25 October 2014; pp. 2471–2477. [Google Scholar]
- Peng, F.Z.; Lai, J.-S.; McKeever, J.W.; VanCoevering, J. A multilevel voltage-source inverter with separate DC sources for static VAr generation. IEEE Trans. Ind. Appl. 1996, 32, 1130–1138. [Google Scholar] [CrossRef]
- Yu, K.; Shi, Z.; Zang, Y.; Lu, Q.; Xiang, Y.; Lai, H. Reactive Voltage Control in Photovoltaic Power Stations Considering Source-Load Uncertainty. In Proceedings of the 2024 IEEE 6th Advanced Information Management, Communicates, Electronic and Automation Control Conference (IMCEC), Chongqing, China, 24–26 May 2024; pp. 1934–1938. [Google Scholar]
- Su, S.; Li, P.; Yan, Y.-t.; Yang-Yang; Gao, F.; Xue, A.-c. Analysis of the impact of SVG on the voltage stability of an actual wind farm. In Proceedings of the International Conference on Renewable Power Generation (RPG 2015), Beijing, China, 17–18 October 2015; pp. 1–4. [Google Scholar]
- Zhou, Z.; Mastoi, M.S.; Wang, D.; Haris, M. Control strategy of DFIG and SVG cooperating to regulate grid voltage of wind power integration point. Electr. Power Syst. Res. 2023, 214, 108862. [Google Scholar] [CrossRef]
- Wei, Z.; Runqing, B.; Yong, Z.; Chen, L. Research on application of SVG for reactive power control in wind farm. In Proceedings of the 2012 Power Engineering and Automation Conference, Wuhan, China, 18–20 September 2012; pp. 1–3. [Google Scholar]
- Kashani, M.G.; Babaei, S.; Bhattacharya, S. SVC and STATCOM application in Electric Arc Furnace efficiency improvement. In Proceedings of the 2013 4th IEEE International Symposium on Power Electronics for Distributed Generation Systems (PEDG), Rogers, AR, USA, 8–11 July 2013; pp. 1–7. [Google Scholar]
- Abdelmohsen, A.M.; Hebala, A.M.; Hamad, M.S. Comparative Study of SVC and STATCOM Performance on Reactive Power Compensation in Hot Strip Mill. In Proceedings of the 2023 IEEE Conference on Power Electronics and Renewable Energy (CPERE), Luxor, Egypt, 19–21 February 2023; pp. 1–7. [Google Scholar]
- Raju, M.N.; Sreedevi, J.; Mandi, R.P.; Meera, K.S. Modular multilevel converters technology: A comprehensive study on its topologies, modelling, control and applications. IET Power Electron. 2019, 12, 149–169. [Google Scholar] [CrossRef]
- Tian, S.; Jia, Q.; Xue, S.; Yu, H.; Qu, Z.; Gu, T. Collaborative optimization allocation of VDAPFs and SVGs for simultaneous mitigation of voltage harmonic and deviation in distribution networks. Int. J. Electr. Power Energy Syst. 2020, 120, 106034. [Google Scholar] [CrossRef]
- Tian, S.; Jia, Q.; Cui, Y.; Xue, S.; Yu, H.; Liu, W. Multi-objective Collaborative Optimization of VDAPFs and SVGs Allocation Considering MFGCIs Contribution for Voltage Partitioning Mitigation in Distribution Networks. Electr. Power Syst. Res. 2022, 207, 107830. [Google Scholar] [CrossRef]
- Wang, D.; Cui, T.; Hu, Z.; Shen, Y. Impacts of SVG Location and Capacity on Voltage Recovery Characteristics in Hunan Network. In Proceedings of the 2019 4th International Conference on Intelligent Green Building and Smart Grid (IGBSG), Yichang, China, 6–9 September 2019; pp. 418–421. [Google Scholar]


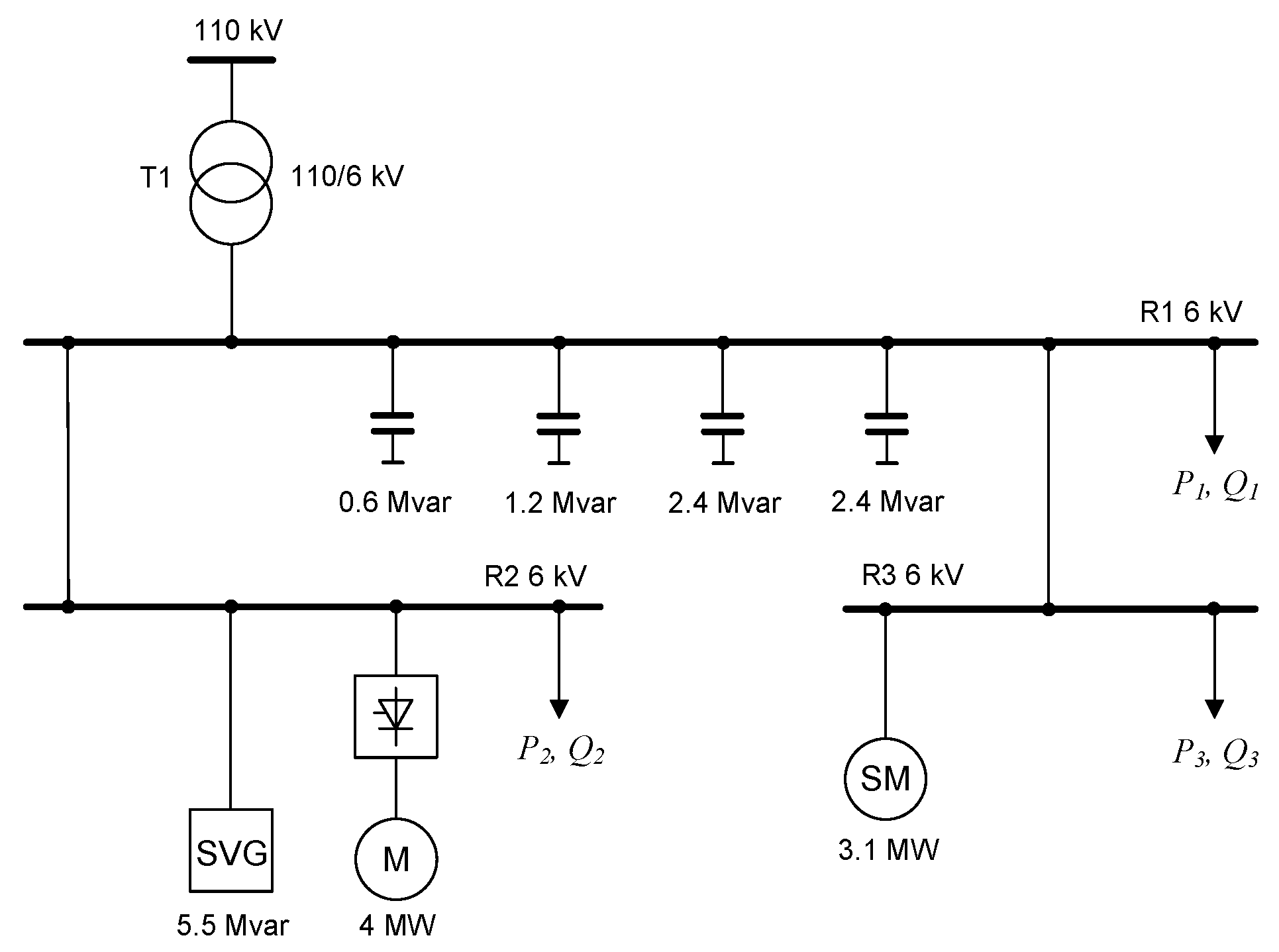
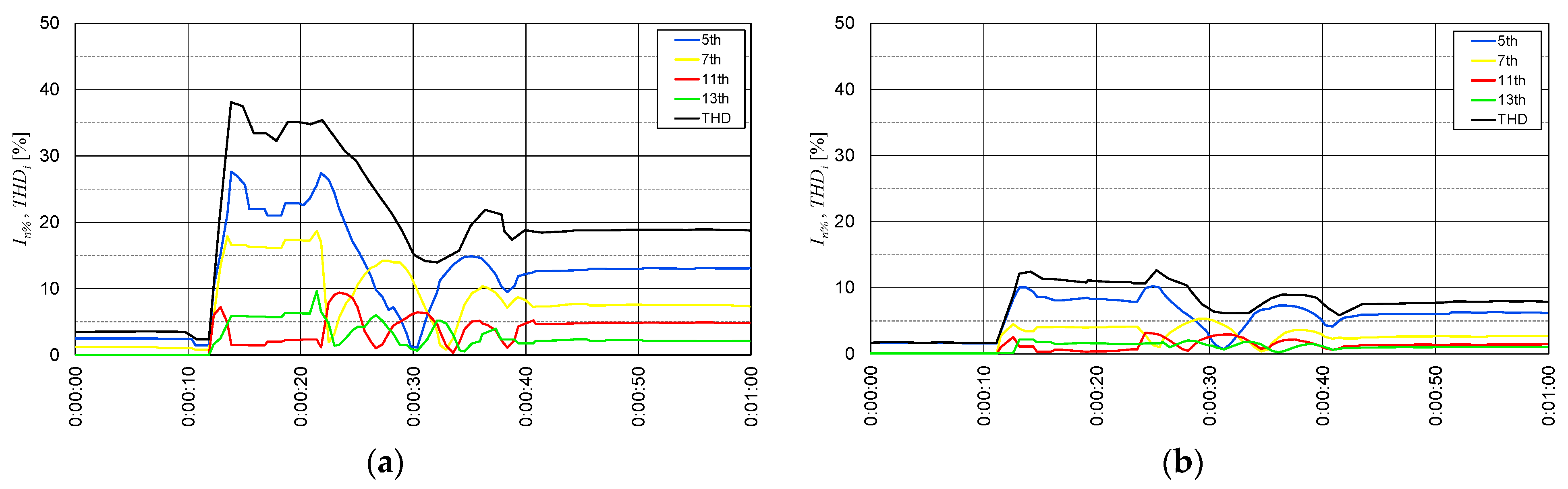
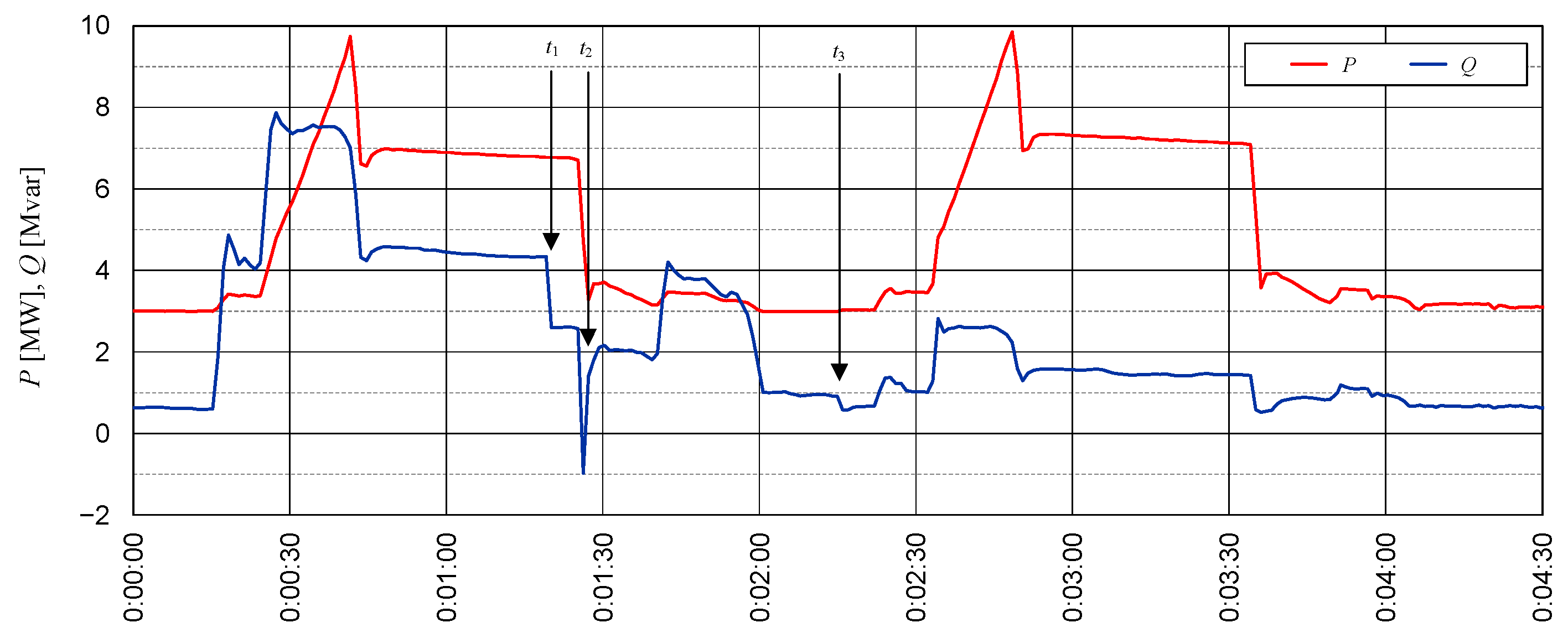

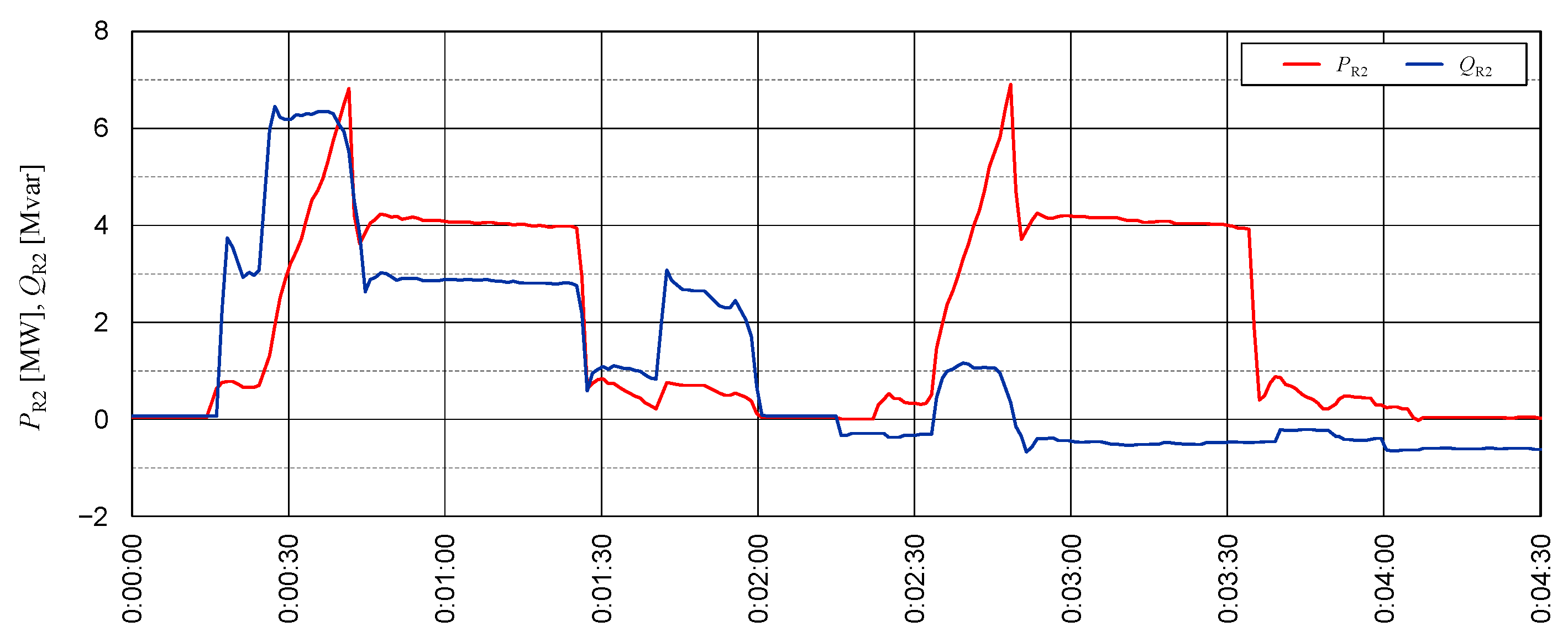
| Parameters | Value |
|---|---|
| compensator power | 5.5 Mvar |
| current RMS | 530 A |
| working voltage | 6 kV |
| filtering capacity | 2–25th harmonic |
| transient response | <0.1 ms |
| complete response | <10 ms |
| efficiency | >97% |
| frequency | 50 Hz ± 5% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hyla, M.; Latko, A. Advanced Static Var Generator in the Reactive Power Automatic Compensation System of an Underground Mine with a Thyristor Hoisting Machine. Energies 2024, 17, 3628. https://doi.org/10.3390/en17153628
Hyla M, Latko A. Advanced Static Var Generator in the Reactive Power Automatic Compensation System of an Underground Mine with a Thyristor Hoisting Machine. Energies. 2024; 17(15):3628. https://doi.org/10.3390/en17153628
Chicago/Turabian StyleHyla, Marian, and Andrzej Latko. 2024. "Advanced Static Var Generator in the Reactive Power Automatic Compensation System of an Underground Mine with a Thyristor Hoisting Machine" Energies 17, no. 15: 3628. https://doi.org/10.3390/en17153628
APA StyleHyla, M., & Latko, A. (2024). Advanced Static Var Generator in the Reactive Power Automatic Compensation System of an Underground Mine with a Thyristor Hoisting Machine. Energies, 17(15), 3628. https://doi.org/10.3390/en17153628






