A Study on the Problem of AC Corrosion of Power Umbilical Cables Caused by Electromagnetic Induction Phenomena
Abstract
1. Introduction
2. Materials and Methods
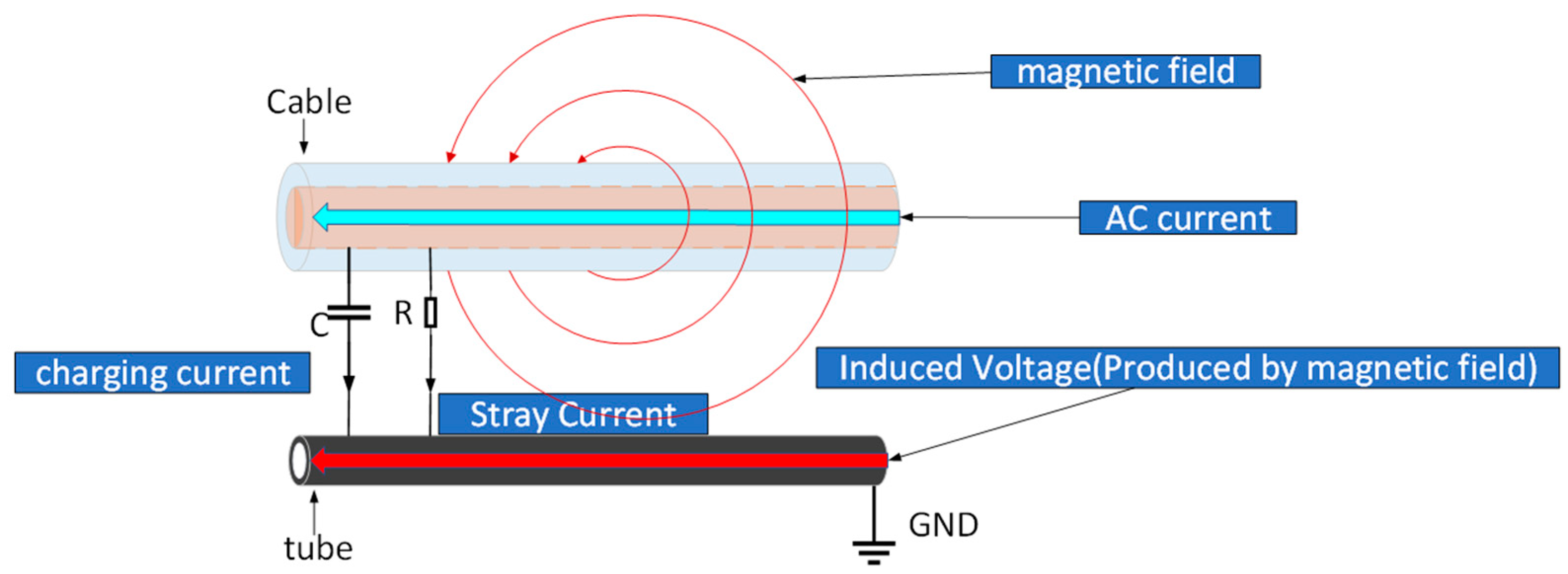
2.1. Mechanism of Induced Potential Generation in Steel Tube of Power Umbilical Cable
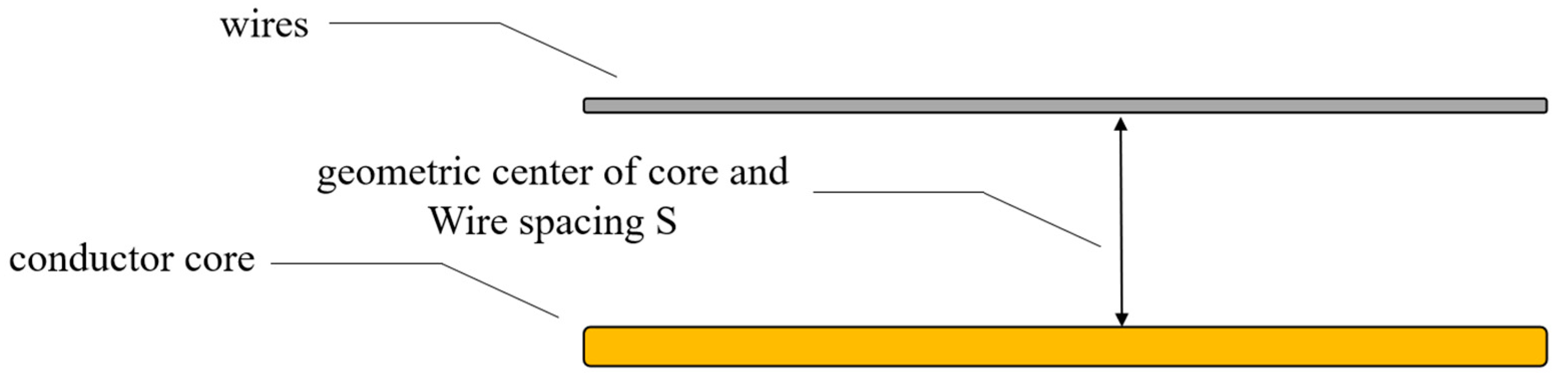
2.2. Effect of Spatial Distribution on Induced Potentials
3. Results
3.1. Finite Element Simulation Design of Power Umbilical Cable
3.2. Study of Induced Potential in Steel Tubes of Power Umbilical Cables
3.2.1. Calculation of the Magnetic Field Distribution of a Power Umbilical Cable
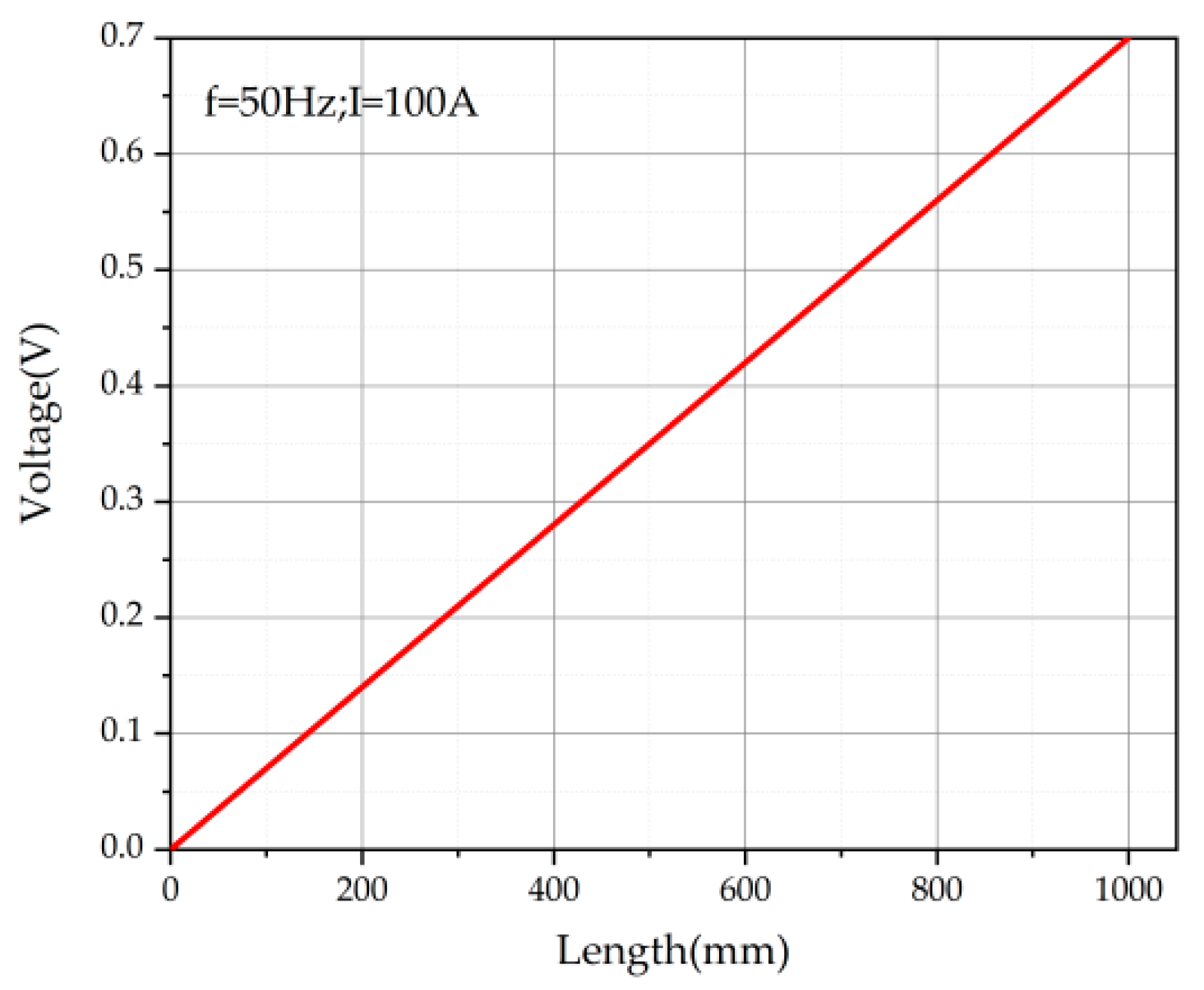
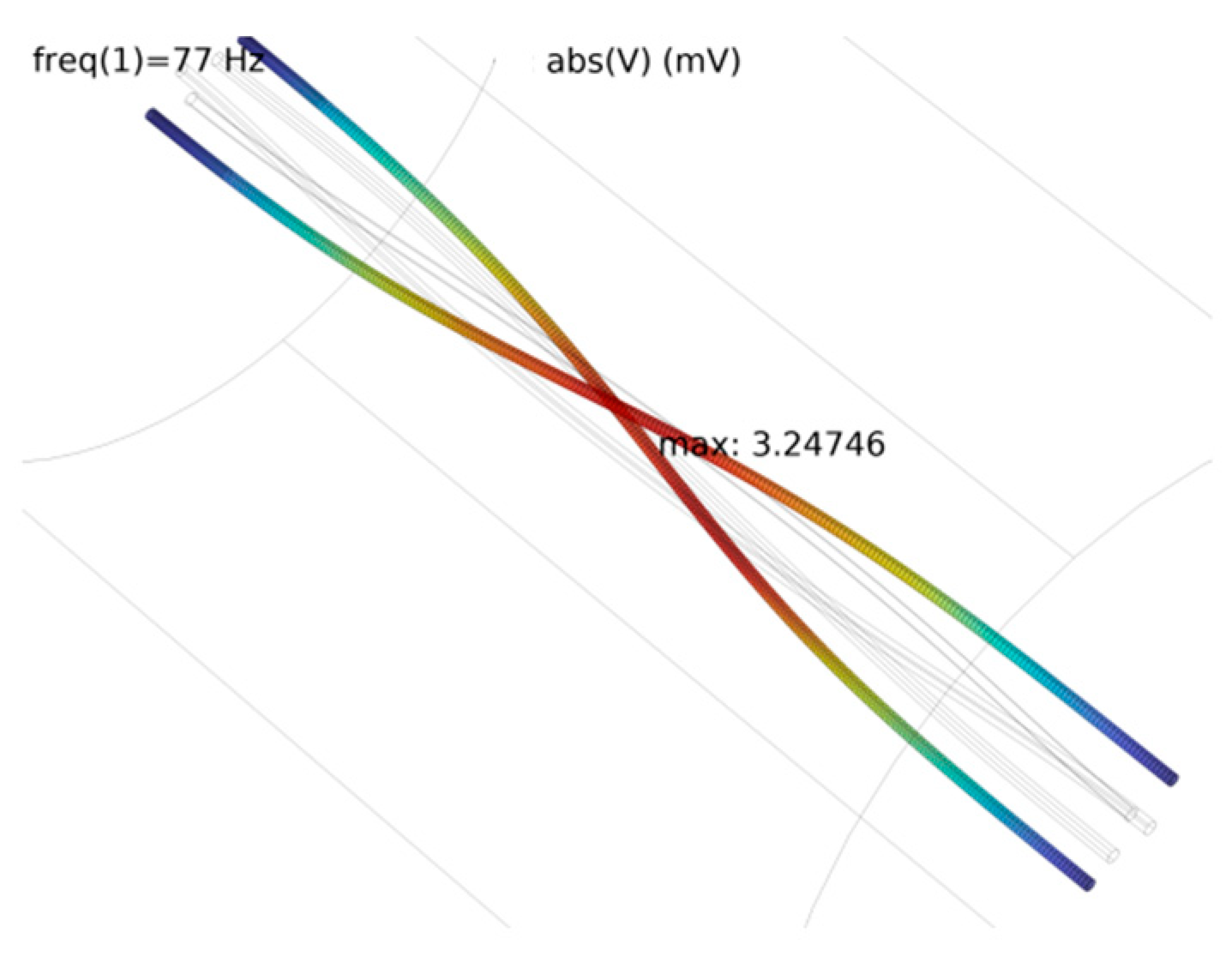
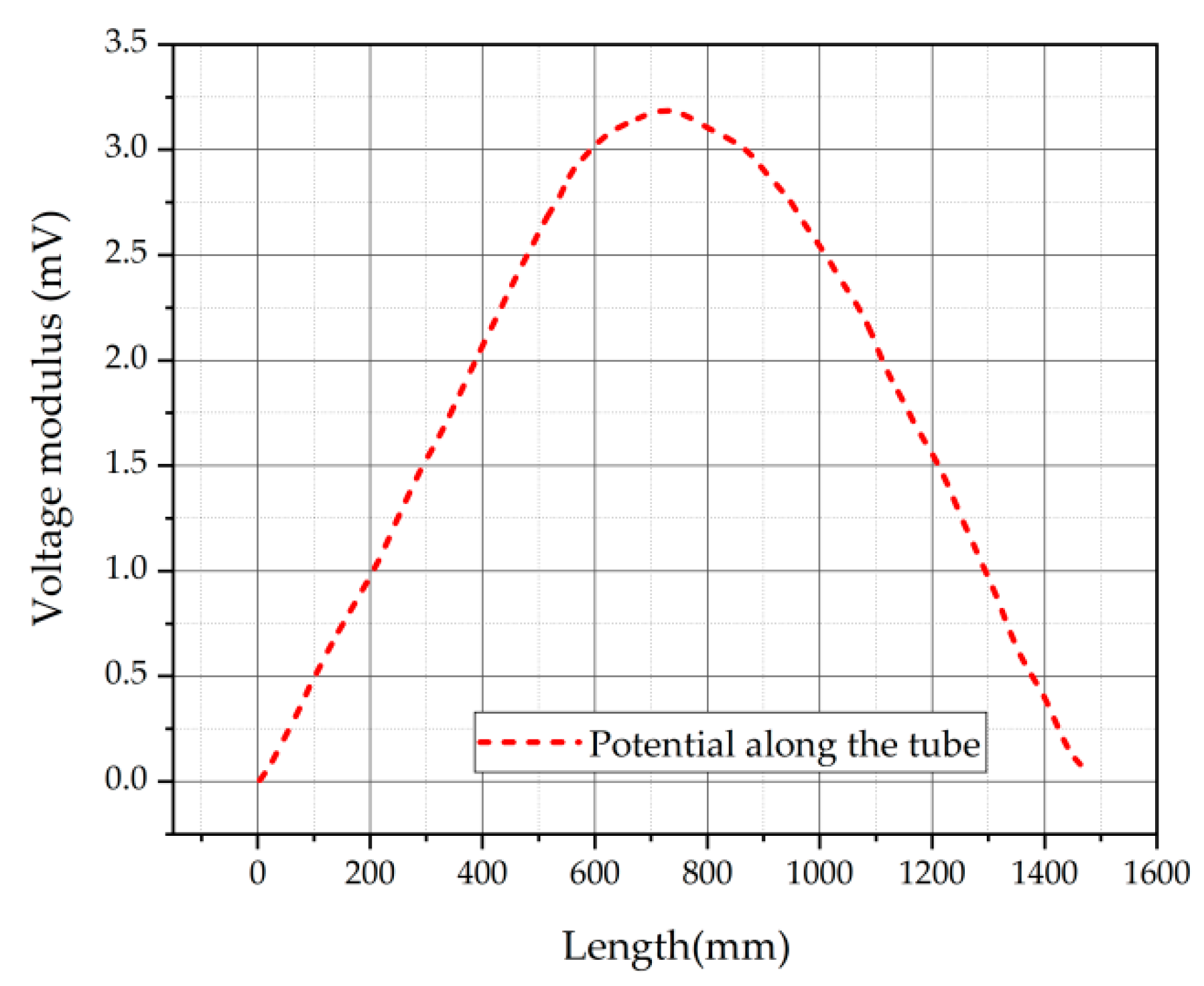
3.2.2. Calculation of Induced Potential Distribution for Steel Tubes of Power Umbilical Cables
4. Discussion
4.1. AC Electrochemical Corrosion Mechanism
- Positive half-cycle:
- Negative half-cycle:
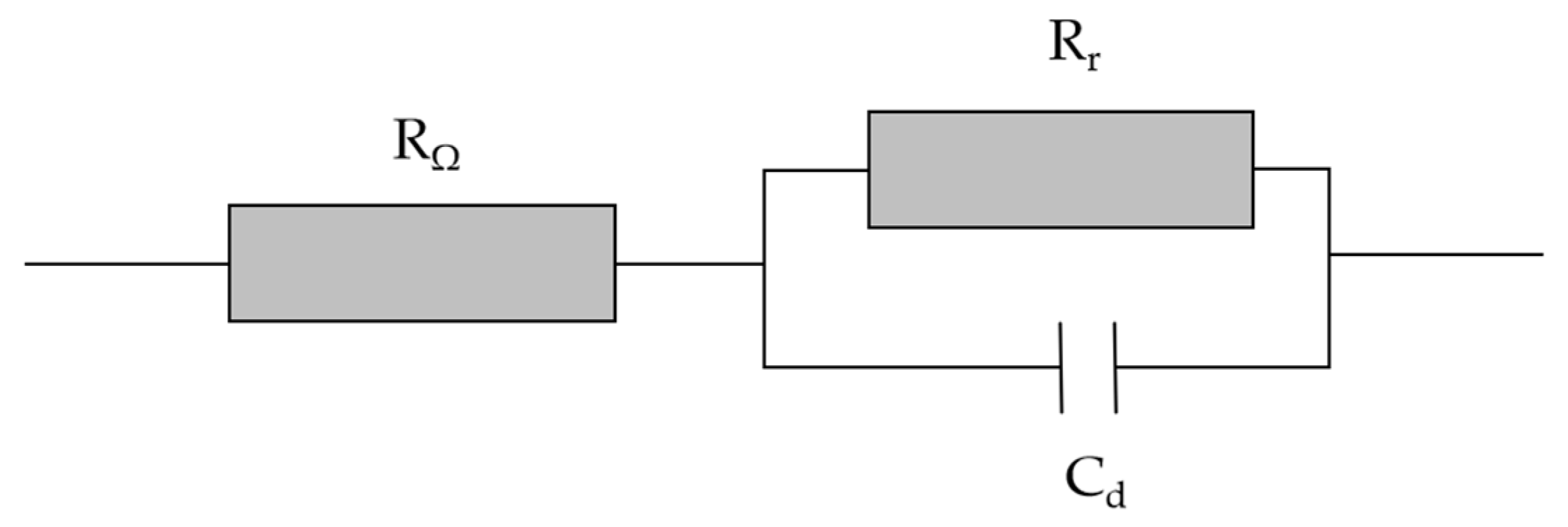
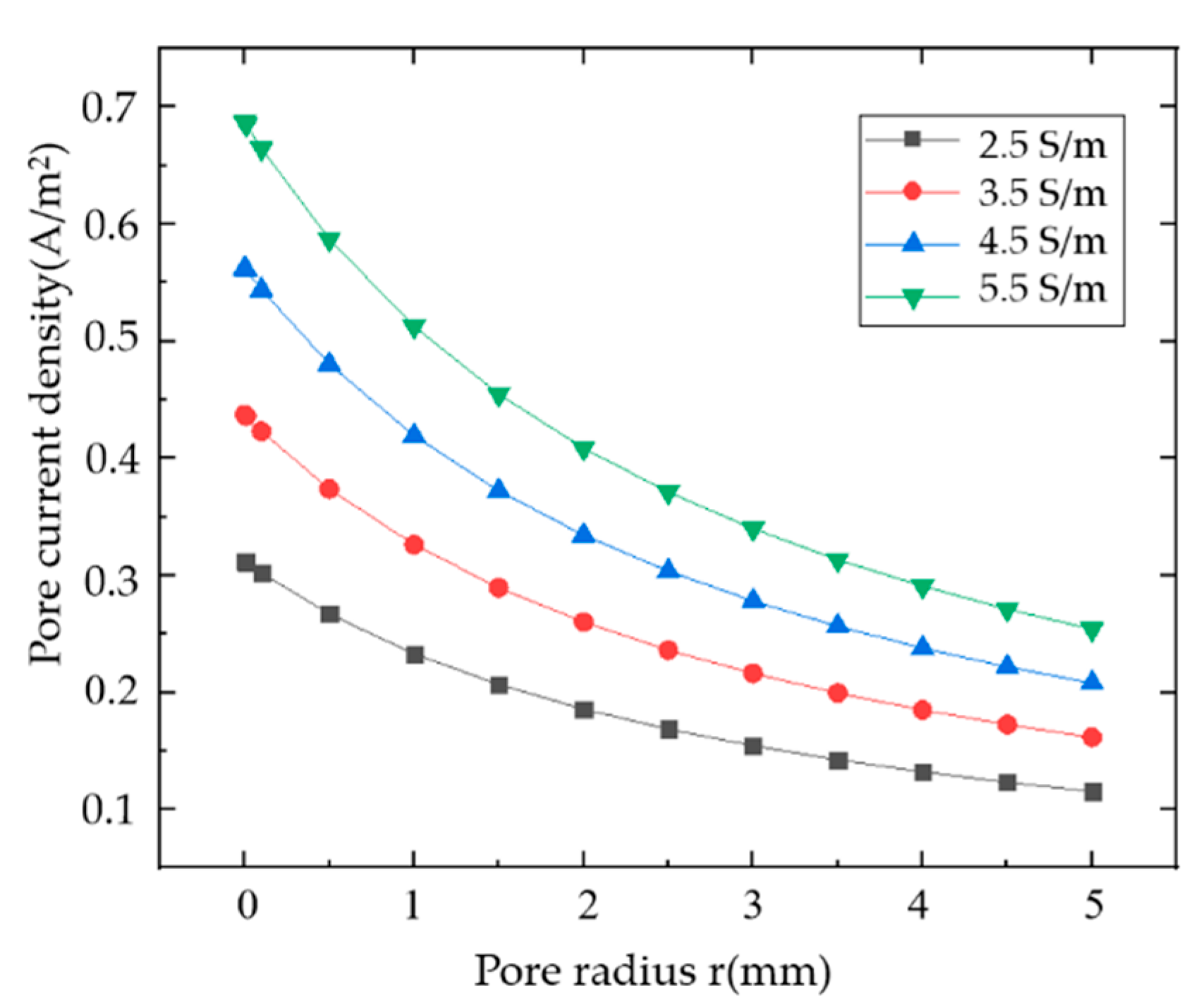
4.2. Factors Affecting Power Umbilical Cable Steel Tube Current Density
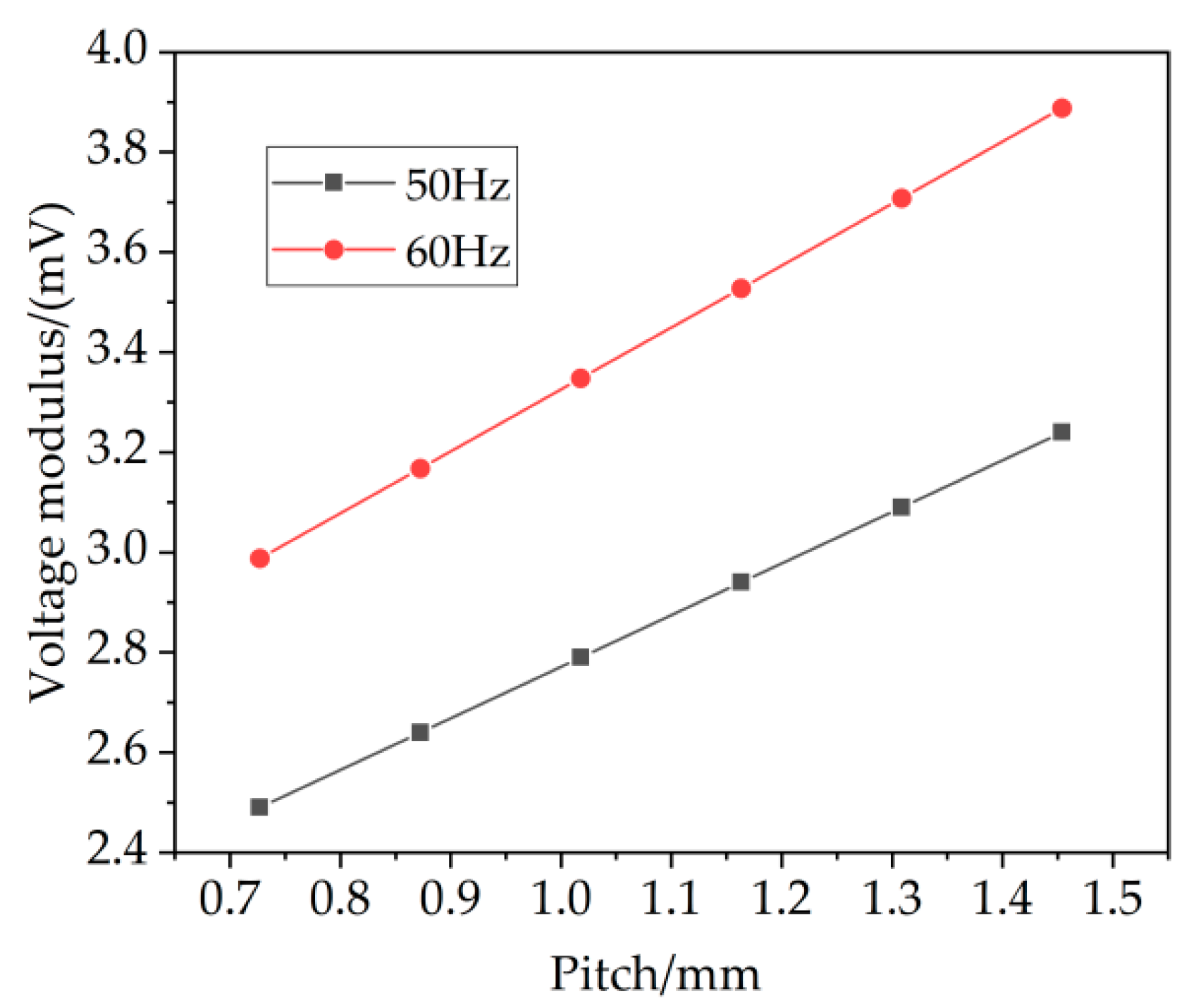
4.3. Effect of Different Pitches and AC Frequencies on Current Density of Steel Tubes
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Guo, H.; Li, Y.; Zheng, L.J.; Yan, J.; Zhang, Q.; Xu, Q. Development of open umbilical structure integrated aided design analysis software platform. Shipbuild. China 2022, 63, 224–236. [Google Scholar]
- Cai, R.; Yang, S. Analysis and Calculation of Crosstalk for Twisted Communication Cables in Umbilical Cable. Energies 2022, 15, 3501. [Google Scholar] [CrossRef]
- Gao, H.; Guo, H.; Sun, K.F.; Qu, Y.; Guo, Y.; Li, B. Preliminary Physical Design of Subsea Umbilical Cable for Production System. Wire Cable 2011, 6, 12–16. [Google Scholar]
- Li, Y.J.; Han, Y.S.; Pang, J.J.; Jin, D.; Sun, Y.L.; Li, Z.H. Electric Field Assist on Enhancing the Electrical Breakdown Strength of Cross-Linked Polyethylene for Power Cable Insulation. Macromolecules 2024, 57, 5497–5506. [Google Scholar] [CrossRef]
- Li, Y.J.; Han, Y.S.; Sun, Y.L.; Li, Z.H. Characterization of Migration Behavior of Organic Electric-field Regulator in Polyethylene Composite Based On the Synchronous Spectra of Space Charge and Polarization Current. Compos. Sci. Technol. 2024, 247, 110437. [Google Scholar] [CrossRef]
- Dieumegard, C.; Fellows, P. Installation of metallic tube umbilicals in 3000 meters water. In Proceedings of the Offshore Technology Conference, Houston, TX, USA, 5–8 May 2003. [Google Scholar]
- Sara, G.; Lazzari, L.; Ormellese, M. AC corrosion. Part 2: Parameters influencing corrosion rate. Corros. Sci. 2010, 52, 916–922. [Google Scholar]
- Wang, X.H.; Song, X.T.; Chen, Y.C.; Wang, Z.Q.; Zhang, L.W. Corrosion Behavior of X70 and X80 tubeline Steels in Simulated Soil Solution. Int. J. Electrochem. Sci. 2018, 13, 6436–6450. [Google Scholar] [CrossRef]
- Qin, Q.Y.; Wei, B.X.; Bai, Y.L.; Qi, F.; Jin, X.; Cheng, S. Effect of alternating current frequency on corrosion behavior of X80 pipeline steel in coastal saline soil. Eng. Fail. Anal. 2021, 120, 105065. [Google Scholar] [CrossRef]
- Wang, H.R.; Du, C.W.; Liu, Z.Y.; Wang, L.T.; De, D. Effect of alternating current on the cathodic protection and interface structure of X80 steel. Materials 2017, 10, 851. [Google Scholar] [CrossRef]
- Guo, A.L.; Zheng, J.Z. Detection, Evaluation and Preventive Measures of Stray Current Interference of Product Oil tubelines in Hunan. Oil-Gas Field Surf. Eng. 2018, 37, 77–81. [Google Scholar]
- Moravcik, I.; Peighambardoust, N.S. Interstitial nitrogen enhances corrosion resistance of an equiatomic CoCrNi medium-entropy alloy in sulfuric acid solution. Mater. Charact. 2021, 172, 110869. [Google Scholar] [CrossRef]
- Bjørn, G.; Martin, H.; Marius, H.; Steinar, M. Voltages and AC Corrosion on Metallic Tubes in Umbilical Cables Caused by Magnetic Induction From Power Cable Charging Currents. IEEE Trans. Power Deliv. 2019, 34, 596–605. [Google Scholar]
- Ouadah, M.; Ziouche, A.; Omar, T.; Ibtiouen, R.; Bouyegsh, S.; Charif, D. AC Corrosion Induced by High Voltage Power Line on Cathodically Protected Pipeline. In International Conference on Control, Engineering & Information Technology; Research Gate: Sousse, Tunisia, 2014. [Google Scholar]
- Zanotto, F.; Grassi, V.; Balbo, A.; Monticelli, C.; Zucchi, F. Stress-Corrosion Cracking Behaviour of Lean-Duplex Stainless Steels in Chloride/Thiosulphate Environments. Metals 2018, 8, 237. [Google Scholar] [CrossRef]
- Muralidharan, S.; Kim, D.; Ha, T.; Bae, J.; Ha, Y.; Lee, H.; Scantlebury, J. Influence of alternating, direct and superimposed alternating and direct current on the corrosion of mild steel in marine environments. Desalination 2007, 216, 103–115. [Google Scholar] [CrossRef]
- Huang, X.Y.; Zhou, L.; Li, Y.K. The synergistic effect of temperature, H2S/CO2 partial pressure and stress toward corrosion of X80 pipeline steel. Eng. Fail. Anal. 2023, 146, 107079. [Google Scholar] [CrossRef]
- Liu, L.Y.; Wen, X.R.; Shi, R.C.; Li, P.; Wen, Y.M.; Han, T. High-efficiency magnetic field energy harvesting from a three-core cable. Sens. Actuators A Phys. 2023, 360, 114501. [Google Scholar] [CrossRef]
- Li, X.; Zhang, H.Z.; Zhang, X.; Liu, S.G.; Yao, S.J. Electromagnetic interference and corrosion on the buried metal oil and gas tubelines caused by the high voltage AC transmission lines and protection measures. J. Shandong Univ. Technol. 2017, 31, 65–70. [Google Scholar]
- Gao, J.G.; Yu, P.L.; Li, Z.Y.; Zhang, X.H.; Liu, T.; Liu, Z.H. Simulation Analysis of Induced Voltage on Metal Sheath of Power Cable Based on Finite Element Method. High Volt. Eng. 2014, 40, 714–720. [Google Scholar]
- Bremnes, J.J.; Evebset, G.; Stolan, R. Power loss and inductance of steel armoured multi-core cables: Comparison of IEC values with “2,5D” FEA results and measurements. In Cigre Session; CIGRE: London, UK, 2010. [Google Scholar]
- Wang, X.H.; Xu, C.; Chen, Y.C.; Tu, C.Y.; Wang, Z.Q.; Song, X.T. Effects of stray AC on corrosion of 3-layer polyethylene coated X70 tubeline steel and cathodic delamination of coating with defects in 3.5 wt% NaCl solution. Corros. Eng. Sci. Technol. 2018, 53, 214–225. [Google Scholar] [CrossRef]
- Zhang, S.X.; Li, Z.L.; Yang, C.; Gou, J.X. The AC corrosion mechanisms and models-A review. Corrosion 2019, 76, 188–201. [Google Scholar] [CrossRef]
- Zhu, M.; Du, C.W.; Li, X.G.; Liu, Z.Y.; Wu, X.G. Effect of AC on corrosion behavior of X80 tubeline steel in high pH solution. Mater. Corros. 2015, 66, 486–493. [Google Scholar] [CrossRef]
- Berkowitz, B.J.; Horowitz, H.H. The Role of in the Corrosion and Hydrogen Embrittlement of Steel. J. Electrochem. Soc. 1982, 129, 468–474. [Google Scholar] [CrossRef]
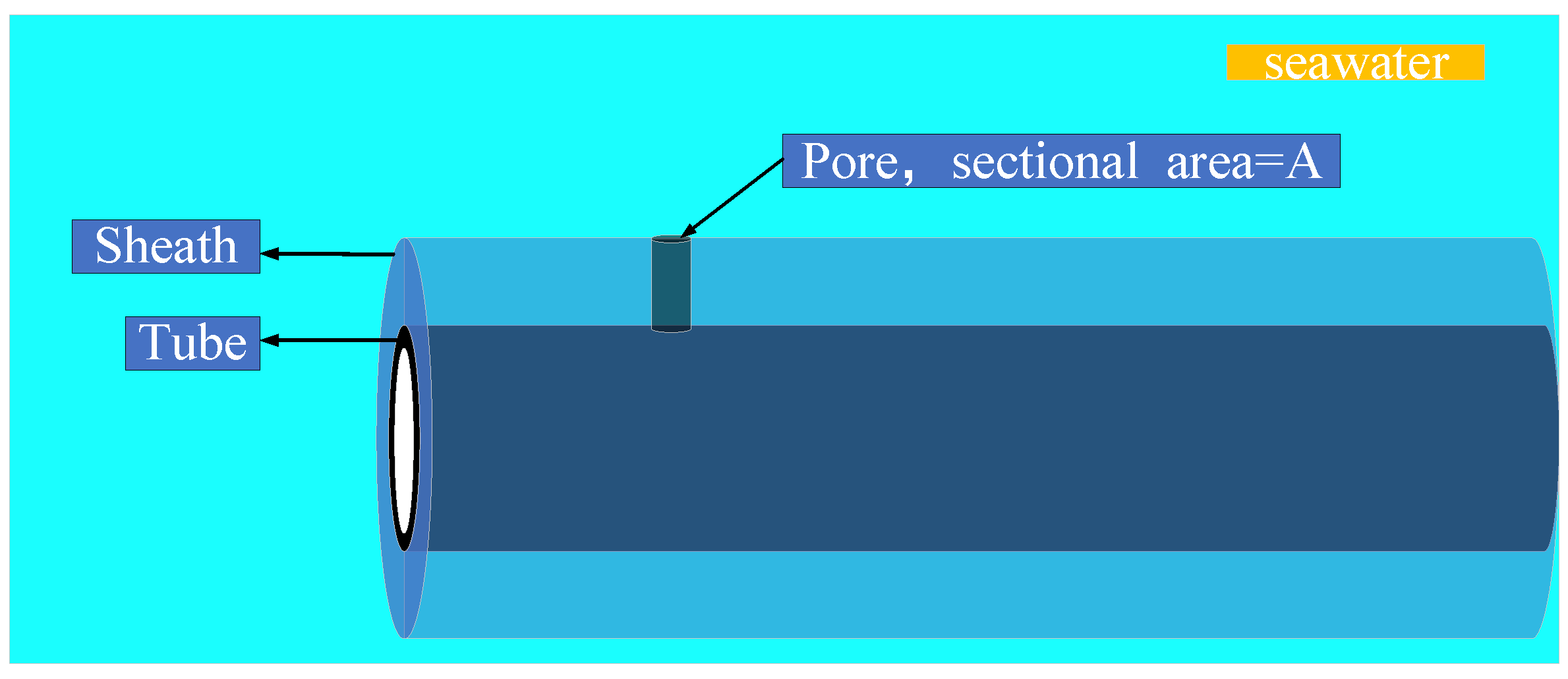
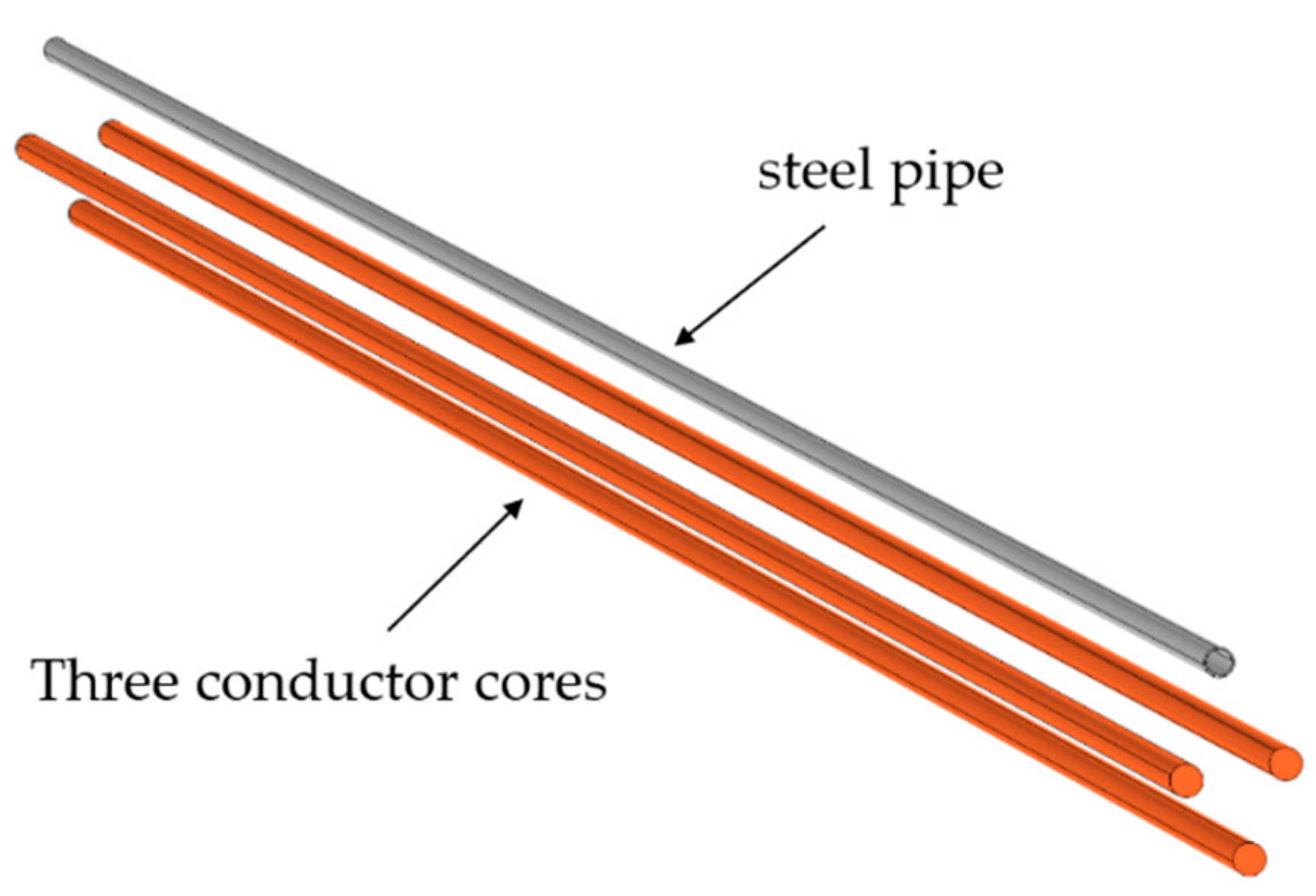






| Framework | External Diameter (mm) | Thickness (mm) | Conductivity (S/m) | Relative Permittivity |
|---|---|---|---|---|
| Copper conductor core | 16.2 | none | 5.89 × 107 | 100,000 |
| Conductor shielding | 19.2 | 1.5 | 100 | 2.5 |
| XLPE insulation | 39.2 | 10.0 | 1 × 10−17 | 2.3 |
| Insulating shield | 40.8 | 0.8 | 100 | 2.5 |
| Semi-conducting water blocking tape | 41.8 | 0.5 | 100 | 2.5 |
| Metallic screen (copper) | 42.2 | 0.2 | 5.89 × 107 | 100,000 |
| Semi-conductive sheath | 48.8 | 3.3 | 1–10−12 | 2.5 |
| Steel tube | 14.7 | 1.00 | 1.3 × 107 | 33 |
| Steel tube PE sheathing | 25.4 | 5.35 | 1–10−12 | 1 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shao, P.; Li, H.; Pan, P.; Shao, Q.; Li, Z.; Yang, J. A Study on the Problem of AC Corrosion of Power Umbilical Cables Caused by Electromagnetic Induction Phenomena. Energies 2024, 17, 3588. https://doi.org/10.3390/en17143588
Shao P, Li H, Pan P, Shao Q, Li Z, Yang J. A Study on the Problem of AC Corrosion of Power Umbilical Cables Caused by Electromagnetic Induction Phenomena. Energies. 2024; 17(14):3588. https://doi.org/10.3390/en17143588
Chicago/Turabian StyleShao, Pengjin, Haijun Li, Pan Pan, Qibing Shao, Zhen Li, and Jiaming Yang. 2024. "A Study on the Problem of AC Corrosion of Power Umbilical Cables Caused by Electromagnetic Induction Phenomena" Energies 17, no. 14: 3588. https://doi.org/10.3390/en17143588
APA StyleShao, P., Li, H., Pan, P., Shao, Q., Li, Z., & Yang, J. (2024). A Study on the Problem of AC Corrosion of Power Umbilical Cables Caused by Electromagnetic Induction Phenomena. Energies, 17(14), 3588. https://doi.org/10.3390/en17143588






