Design Considerations and Experimental Testing of a Wide-Area Inductive Power Transfer (IPT) System for Body-Worn Electronics
Abstract
1. Introduction
2. IPT Theory
2.1. Tuned-Coupled Circuits—Key Design Parameters
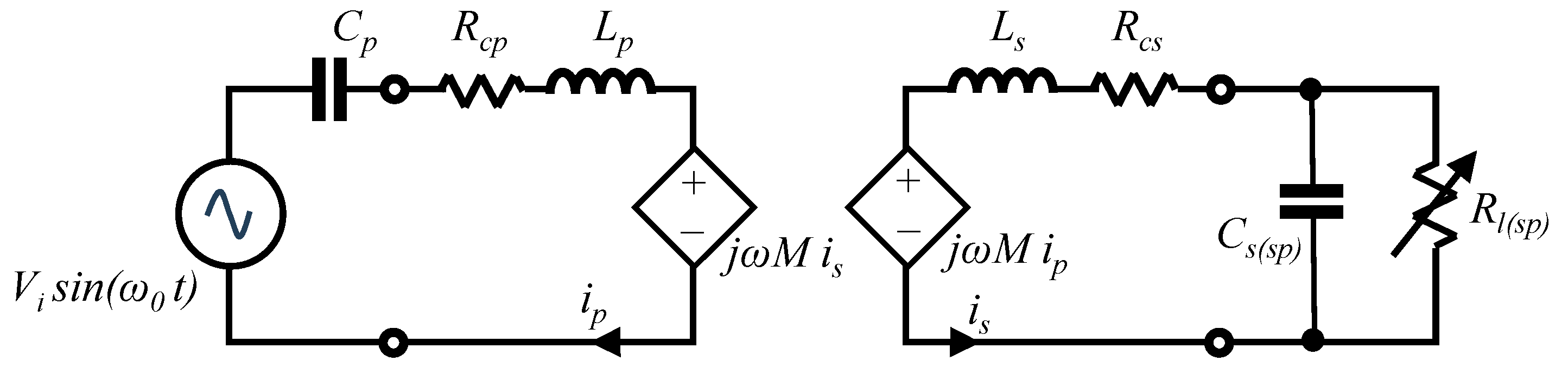
2.2. Series—Parallel (‘SP’) Tuned Circuits
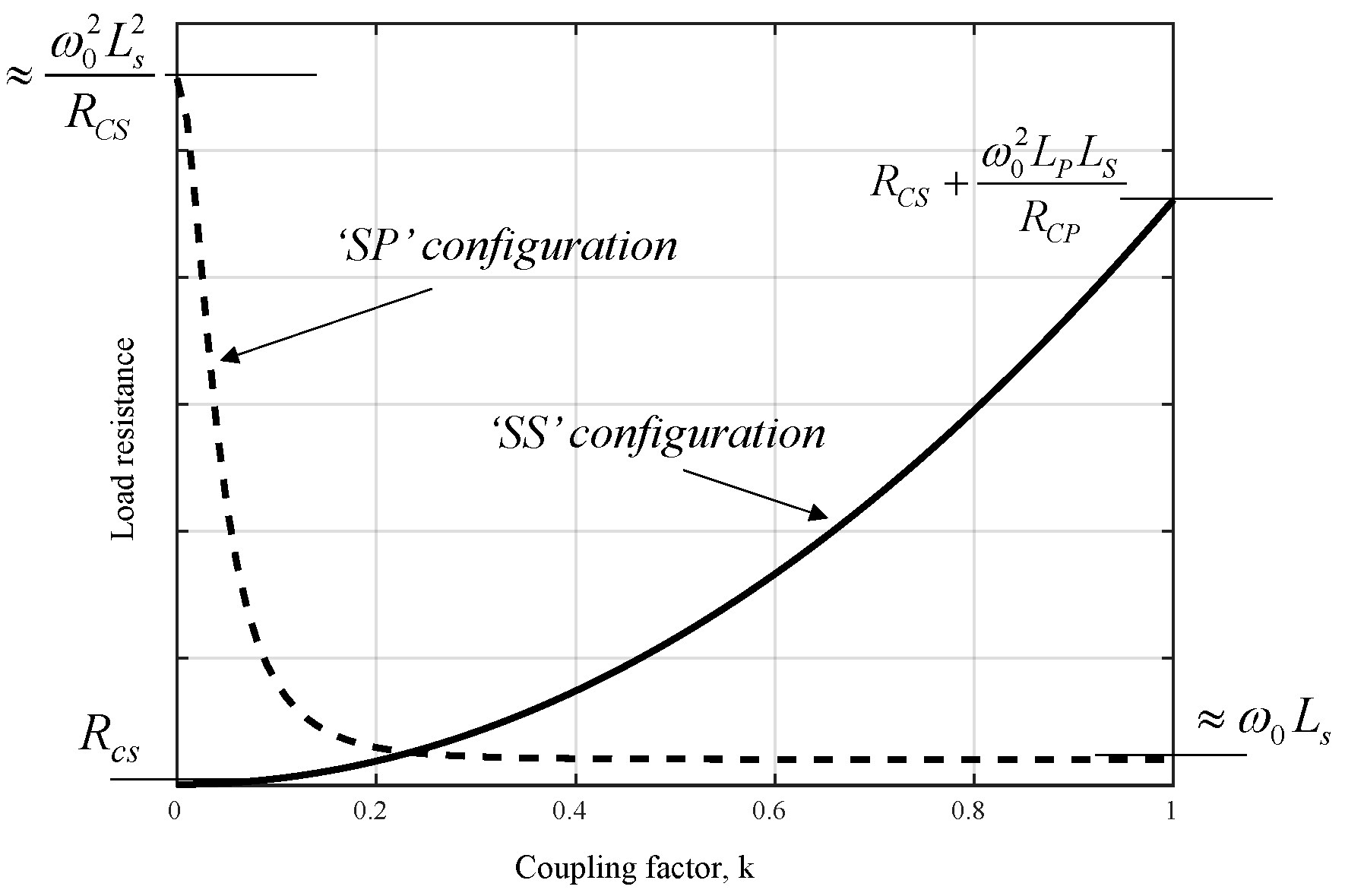
2.3. Optimum Load for Maximum Power Transfer with Variable Coupling
2.4. Operation with a Peak Rectifier
3. TX and RX Coils
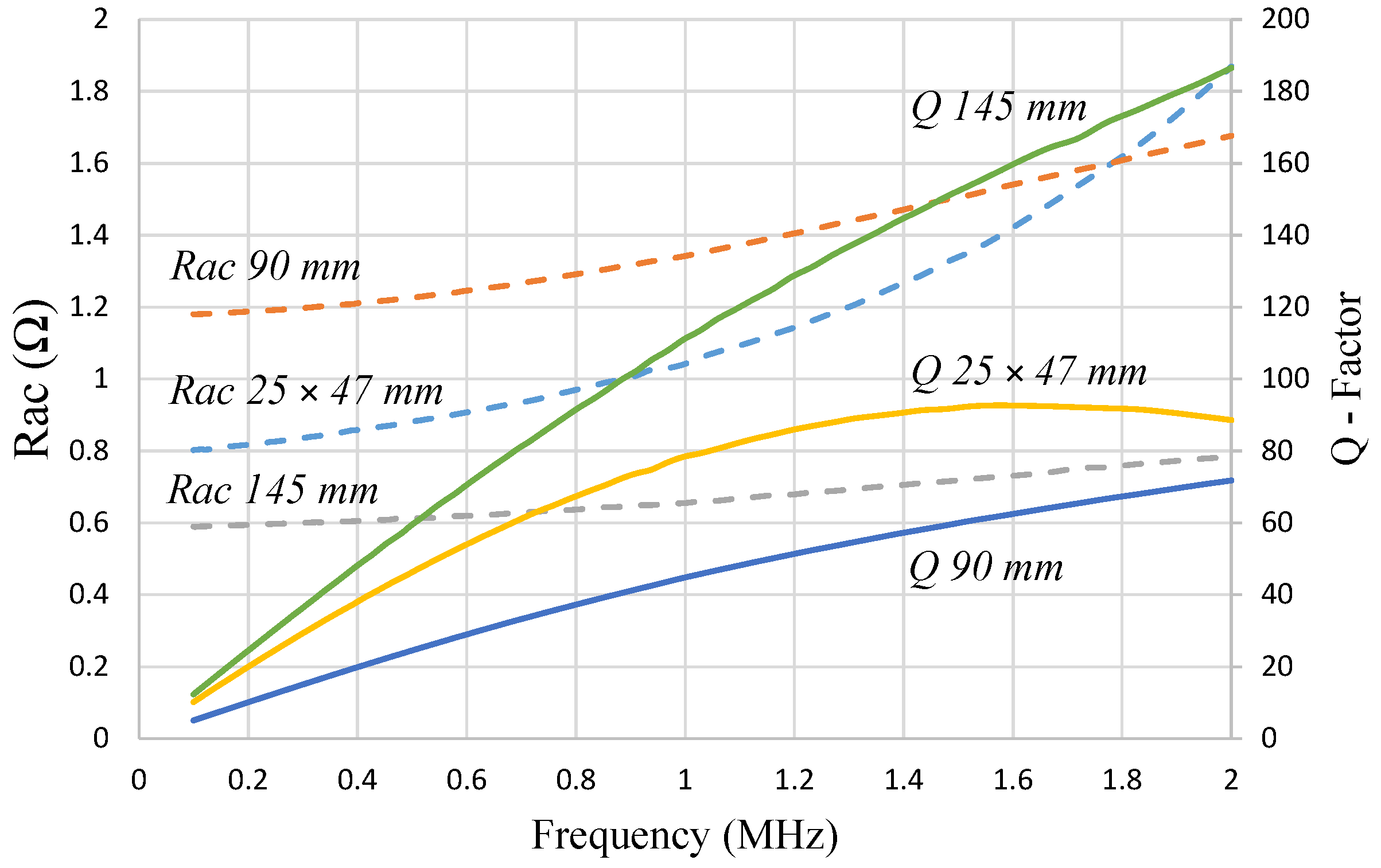
3.1. Coil Design and Properties
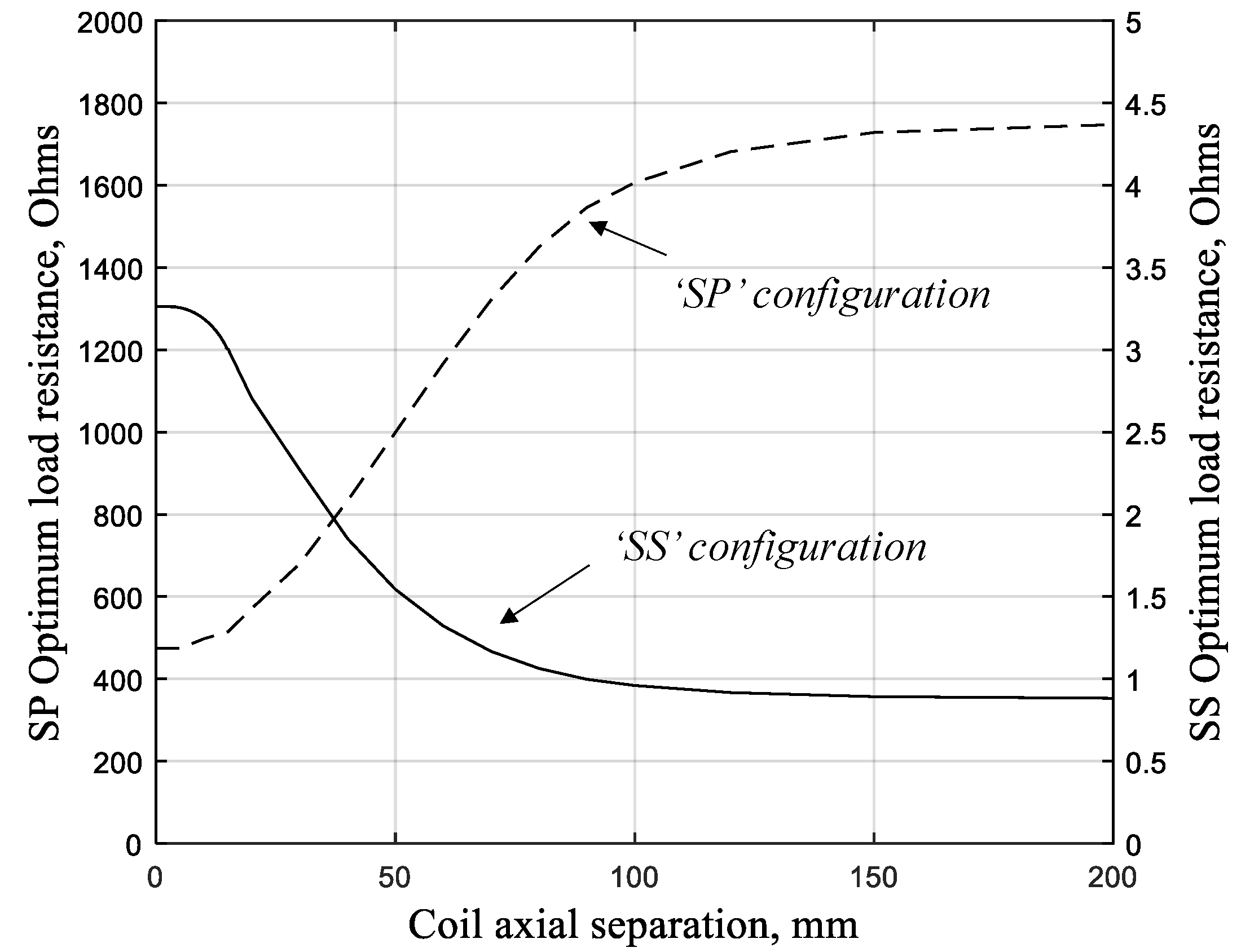
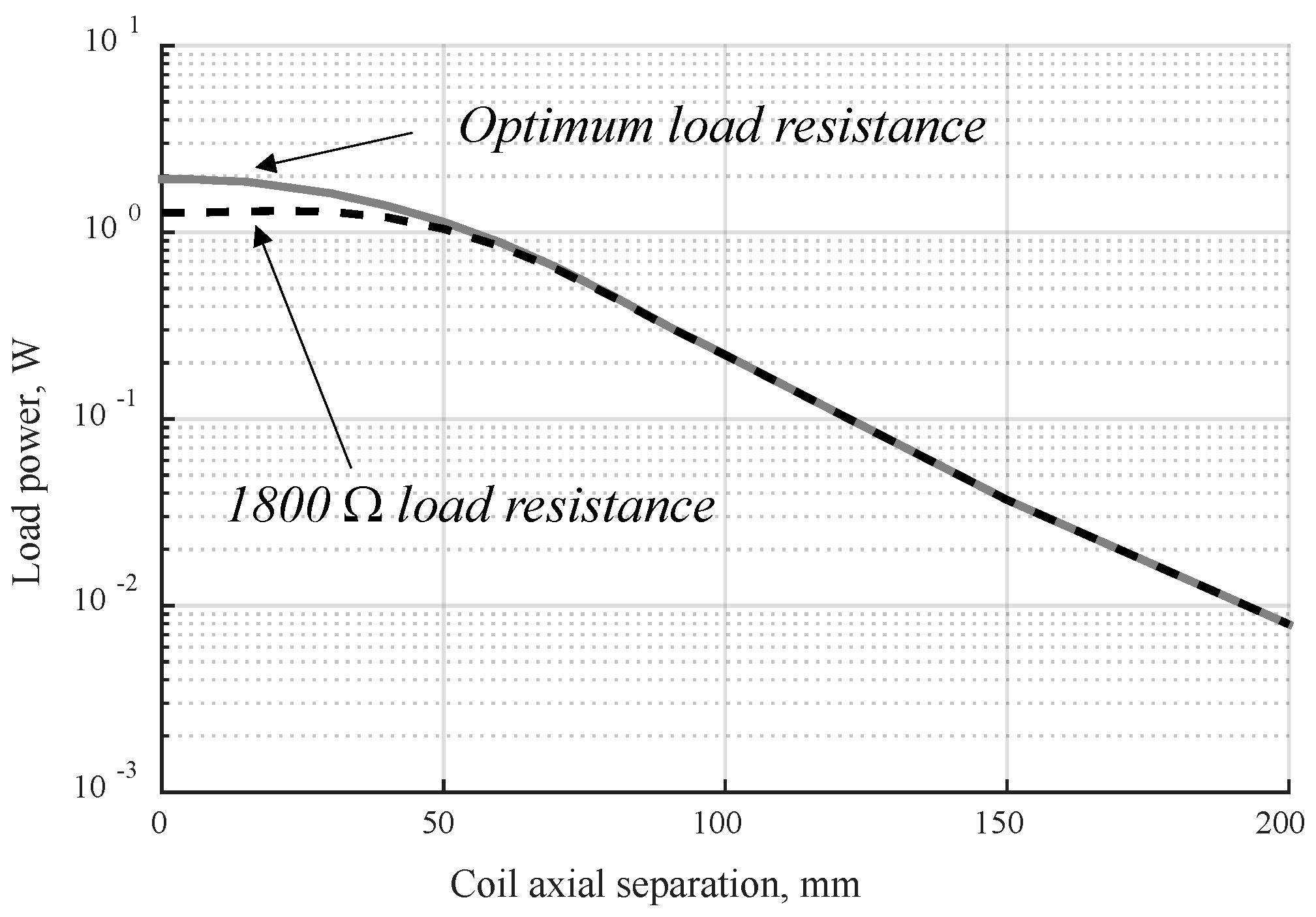
3.2. Influence of Coil Separation on Coupling and Choice of Load
3.3. Compliance with Human Exposure Guidelines
4. Implementation
4.1. System Design Discussion
4.2. Transmit Coil Drive Circuit
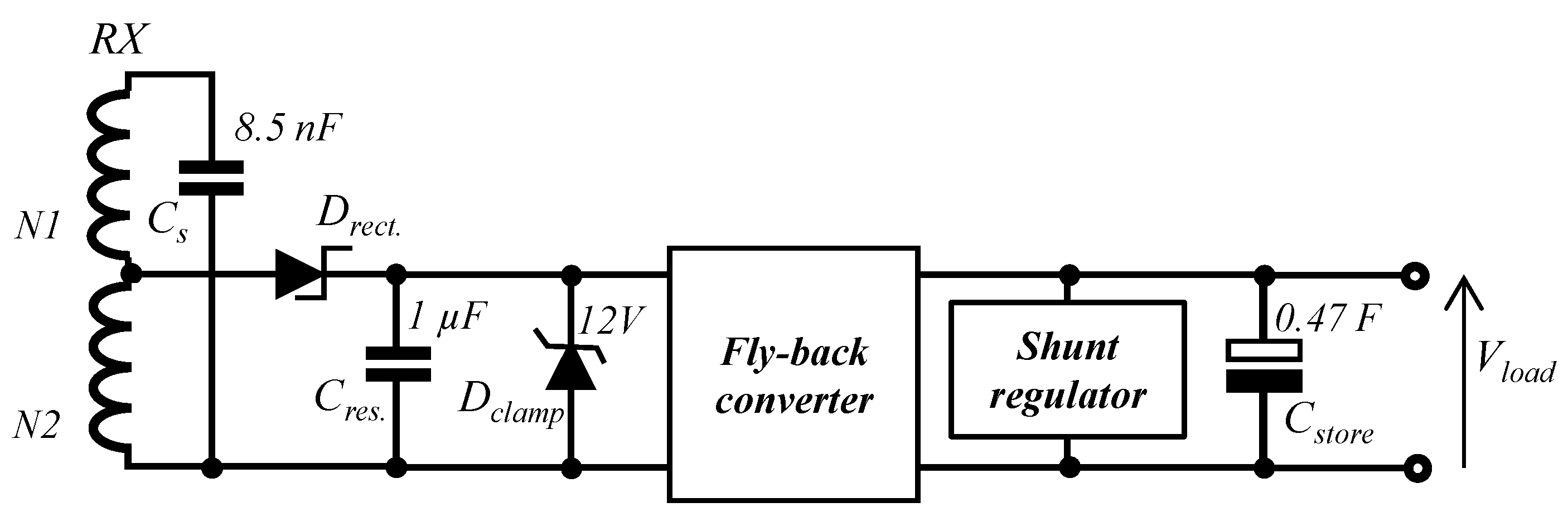
4.3. Receive Circuit and Power-Conditioning
4.4. Complete System
5. Experimental Results
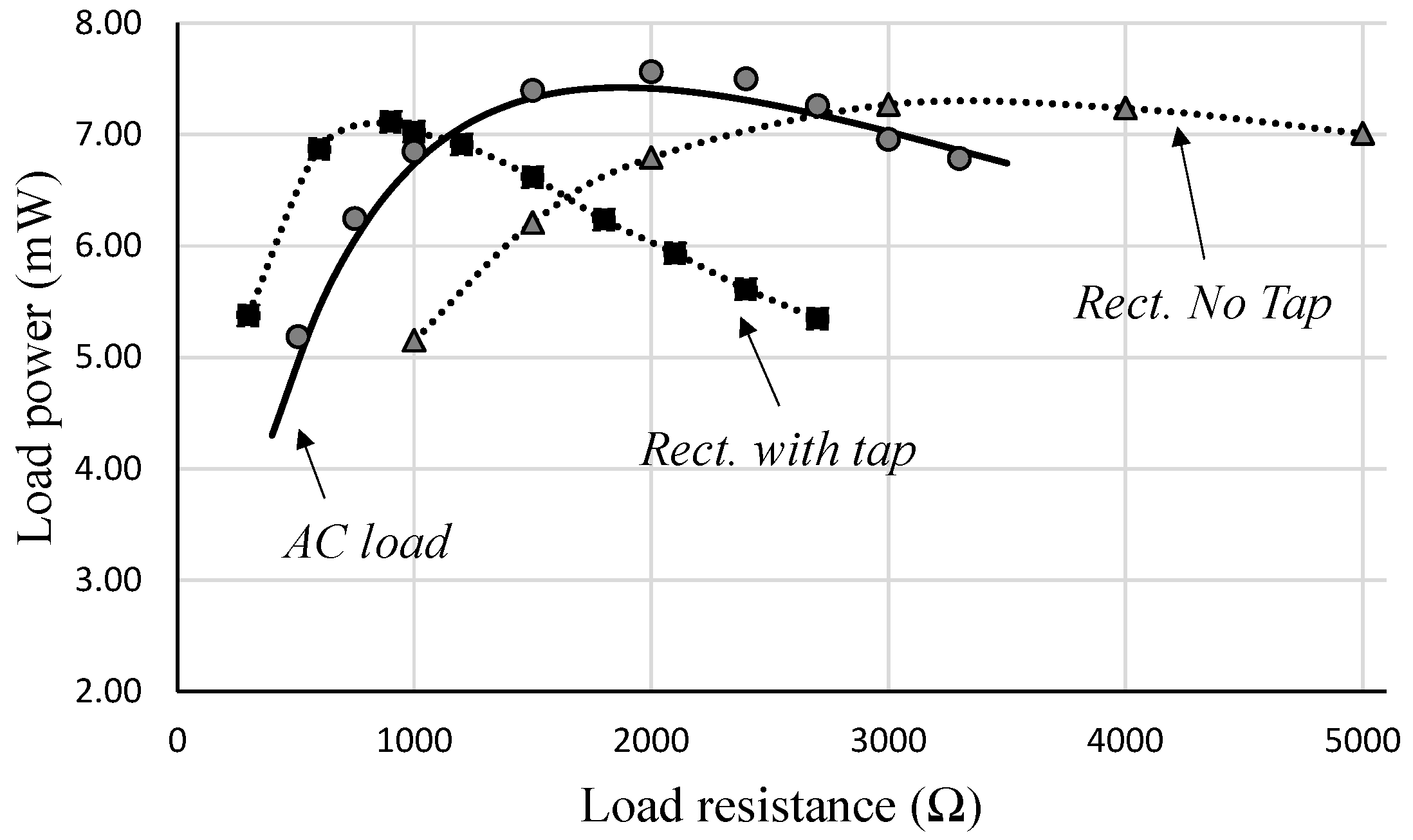
5.1. Load Power Plots
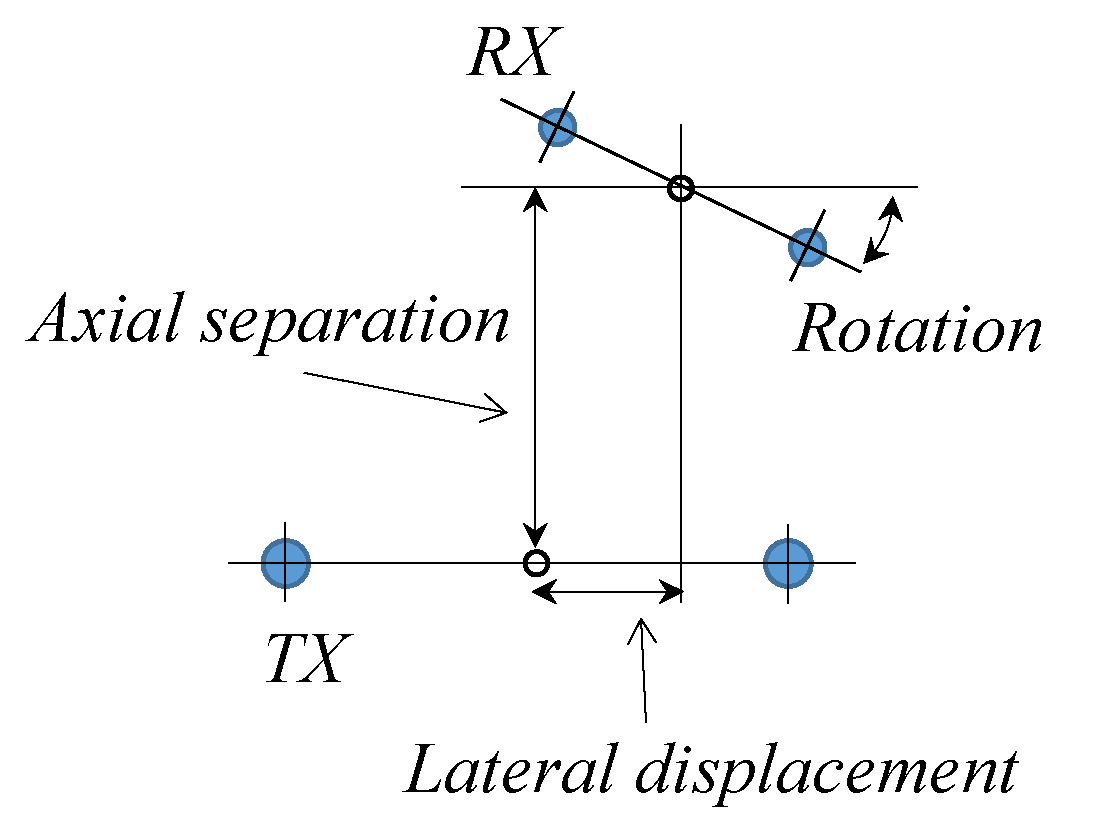
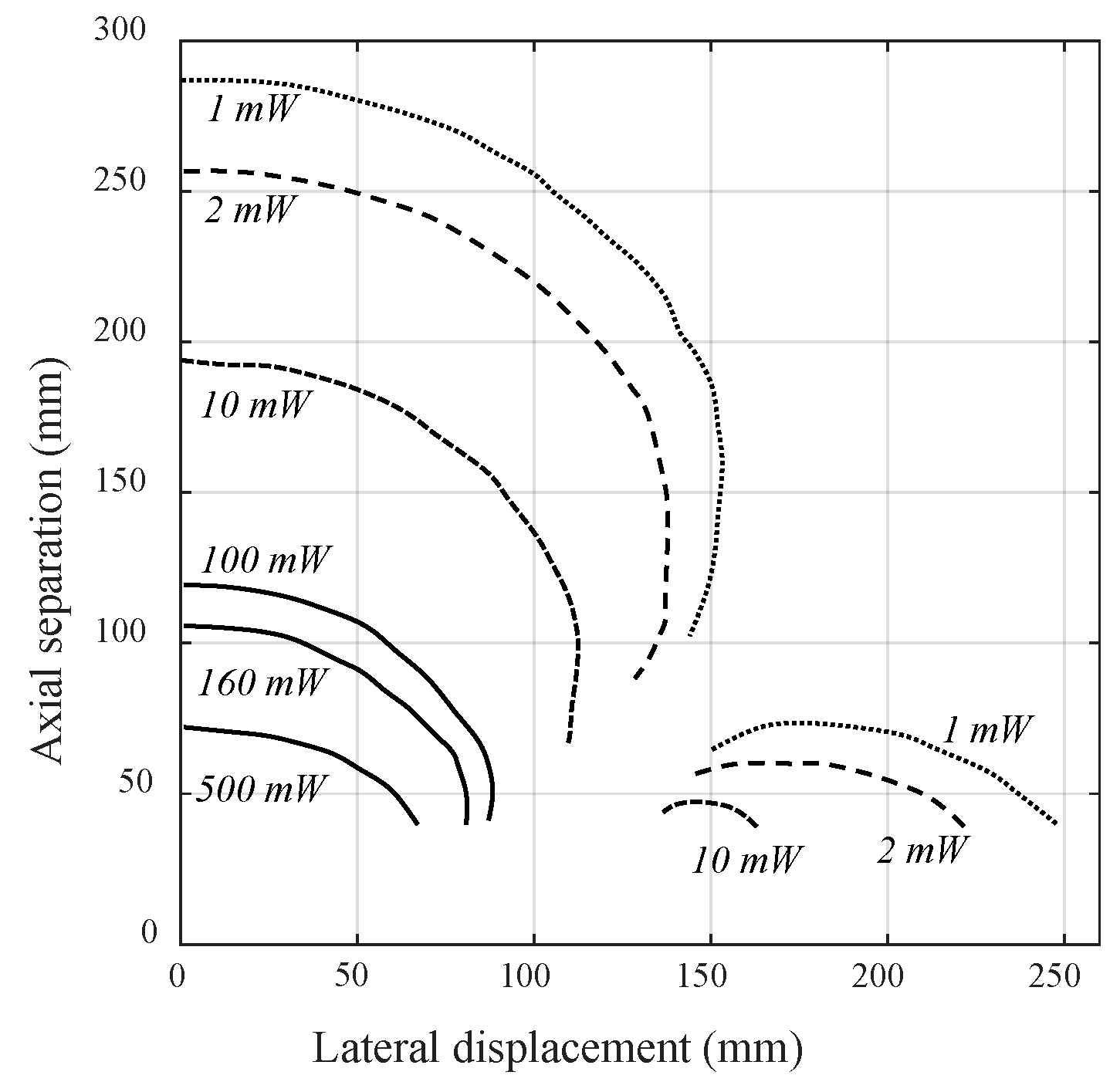
5.2. AC Load (No Rectifier) Power versus Axial Separation and Lateral Misalignment
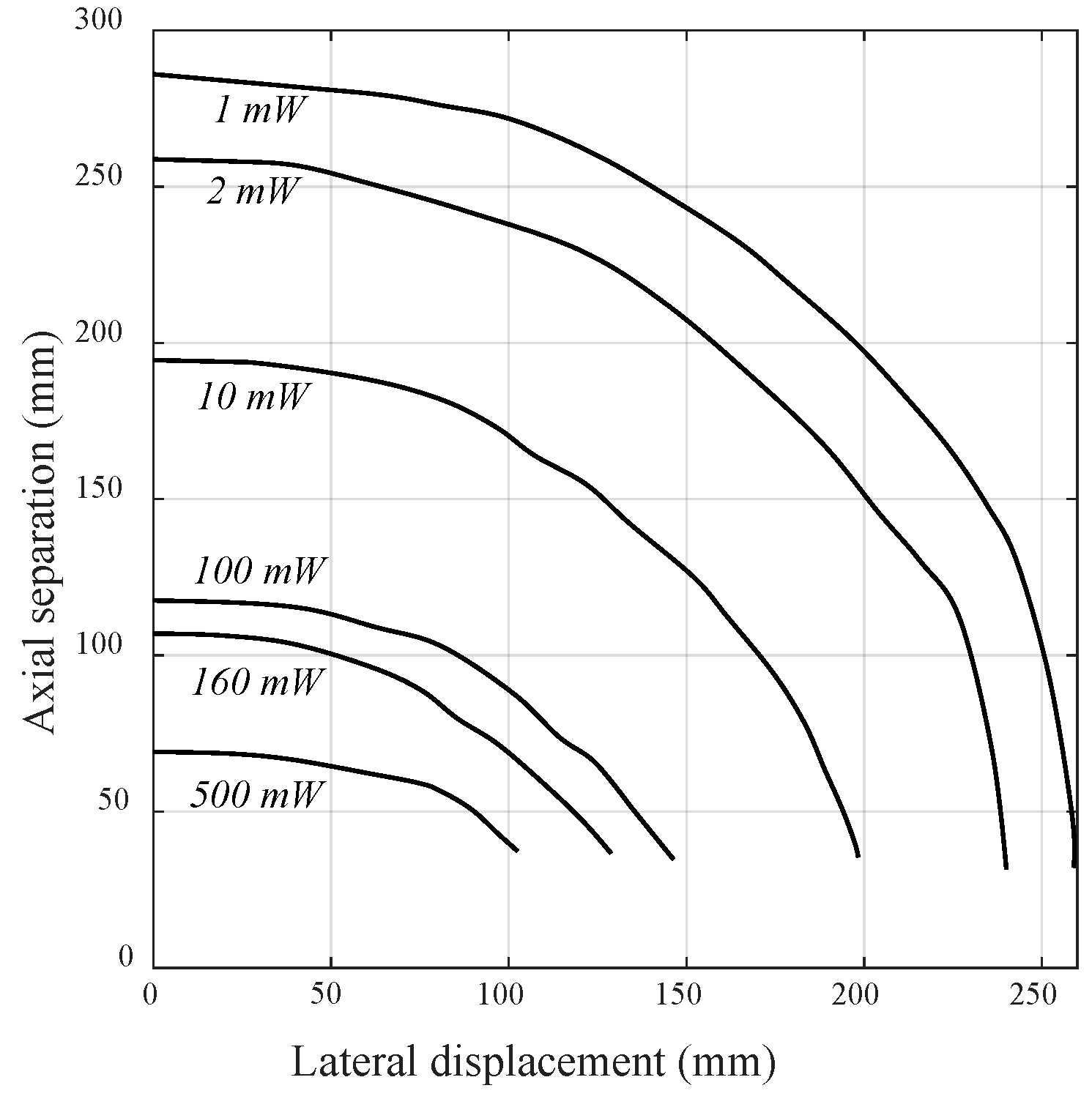
5.3. DC Load Power (with Rectifier) and Optimum Load at 200 mm Axial Separation
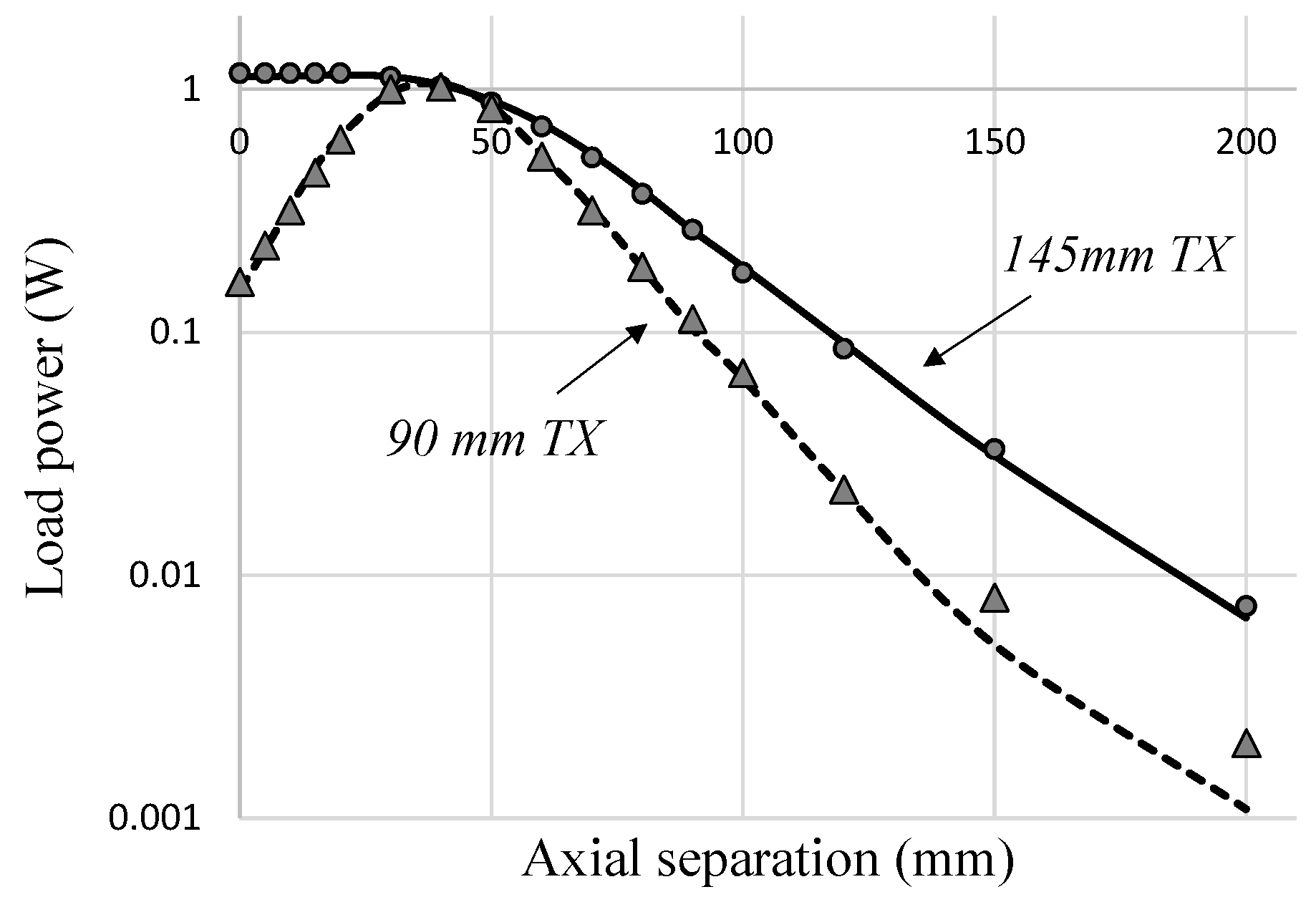
5.4. Power Spatial Envelope Tests
5.5. System Power Consumption and Losses
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Budhia, M.L.; Covic, G.A.; Boys, J. Design and Optimization of Circular Magnetic Structures for Lumped Inductive Power Transfer Systems. IEEE Trans. Power Electron. 2011, 26, 3096–3108. [Google Scholar] [CrossRef]
- Aydin, E.; Aydemir, M.T.; Aksoz, A.; El Baghdadi, M.; Hegazy, O. Inductive Power Transfer for Electric Vehicle Charging Applications: A Comprehensive Review. Energies 2022, 15, 4962. [Google Scholar] [CrossRef]
- Jayalath, S.; Khan, M. Design, Challenges, and Trends of Inductive Power Transfer Couplers for Electric Vehicles: A Review. IEEE J. Emerg. Sel. Top. Power Electron. 2020, 9, 6196–6218. [Google Scholar] [CrossRef]
- Sergeant, P.; Van Den Bossche, A. Inductive coupler for contactless power transmission. IET Electr. Power Appl. 2008, 2, 1–7. [Google Scholar] [CrossRef]
- Hui, S.R.; Ho, W.W. A New Generation of Universal Contactless Battery Charging Platform for Portable Consumer Electronic Equipment. IEEE Trans. Power Electron. 2005, 20, 620–627. [Google Scholar] [CrossRef]
- Liu, X.; Hui, S.Y. Optimal Design of a Hybrid Winding Structure for Planar Contactless Battery Charging Platform. IEEE Trans. Power Electron. 2008, 23, 455–463. [Google Scholar]
- Roshan, Y.M.; Park, E.J. Design approach for a wireless power transfer system for wristband wearable devices. IET Power Electron. 2017, 10, 931–937. [Google Scholar] [CrossRef]
- Choi, J.; Seo, C. Analysis on transmission efficiency of wireless energy transmission resonator based on magnetic resonance. Prog. Electromagn. Res. M 2011, 19, 221–237. [Google Scholar] [CrossRef][Green Version]
- Silay, K.M.; Dehollain, C.; Declercq, M. Inductive Power Link for a Wireless Cortical Implant with Biocompatible Packaging. In Proceedings of the IEEE SENSORS 2010 Conference, Waikoloa, HI, USA, 1–4 November 2010. [Google Scholar]
- Chen, L.; Liu, S.; Zhou, Y.; Cui, T. An Optimizable Circuit Structure for High-Efficiency Wireless Power Transfer. IEEE Trans. Ind. Electron. 2013, 60, 339–349. [Google Scholar] [CrossRef]
- Lee, S.-H.; Lorenz, R.D. A Design Methodology for Multi-kW, Large Airgap, MHz Frequency, Wireless Power Transfer Systems. In Proceedings of the ECCE 2011, Phoenix, AZ, USA, 17–22 September 2011. [Google Scholar]
- Pinuela, M.; Yates, D.C.; Lucyszyn, S.; Mitcheson, P.D. Maximizing DC-to-Load Efficiency for Inductive Power Transfer. IEEE Trans. Power Electron. 2013, 28, 2437–2447. [Google Scholar] [CrossRef]
- Clare, L.; Worgan, P.; Stark, B.H.; Adami, S.E.; Coyle, D. Influence of Exposure Guidelines on the Design of On-Body Inductive Power Transfer. In Proceedings of the WPT 2015, Boulder, CO, USA, 13–15 May 2015. [Google Scholar]
- Kim, N.Y.; Kim, K.Y.; Ryu, Y.H.; Choi, J.; Kim, D.Z.; Yoon, C.; Park, Y.K.; Kwon, S. Automated Adaptive Frequency Tracking System for Efficient Mid-Range Wireless Power Transfer via Magnetic Resonance Coupling. In Proceedings of the 42nd European Microwave Conference, Amsterdam, The Netherlands, 29 October–1 November 2012. [Google Scholar]
- Sample, P.; Meyer, D.A.; Smith, J.R. Analysis, Experimental Results, and Range Adaptation of Magnetically Coupled Resonators for Wireless Power Transfer. IEEE Trans. Ind. Electron. 2011, 58, 544–554. [Google Scholar] [CrossRef]
- Zhong, W.; Lee, C.K.; Hui, S.Y.R. General Analysis on the Use of Tesla’s Resonators in Domino Forms for Wireless Power Transfer. IEEE Trans. Ind. Electron. 2013, 60, 261–270. [Google Scholar]
- Terman, F.E. Electronic and Radio Engineering; McGraw-Hill Book Company Inc.: New York, NY, USA, 1955; pp. 63–70. [Google Scholar]
- Albert, A.L. Radio Fundamentals; McGraw Hill Book Company Inc.: New York, NY, USA, 1948; pp. 101–108. [Google Scholar]
- Carter, R.H.A. Admiralty Handbook of Wireless Telegraphy; H.M. Stationery Office: London, UK, 1938; Volume 1, pp. 330–339. [Google Scholar]
- Low, Z.N.; Chinga, R.A. Design and Test of a High-Power High-Efficiency Loosely Coupled Planar Wireless Power Transfer System. IEEE Trans. Ind. Electron. 2009, 56, 1801–1812. [Google Scholar]
- Wang, C.; Stielau, O.H.; Covic, G.A. Design Considerations for a Contactless Electric Vehicle Battery Charger. IEEE Trans. Ind. Electron. 2005, 52, 1308–1314. [Google Scholar] [CrossRef]
- Sen, P.C. Power Electronics; Tata McGraw Publishing Company Limited: New Delhi, India, 2007; pp. 229–237. [Google Scholar]
- Knecht, O.; Bosshard, R.; Kolar, J.W. High-Efficiency Transcutaneous Energy Transfer for Implantable Mechanical Heart Support Systems. IEEE Trans. Power Electron. 2015, 30, 6221–6236. [Google Scholar] [CrossRef]
- Wheeler, H.A. Formulas for the Skin Effect. Proc. IRE 1942, 30, 412. [Google Scholar] [CrossRef]
- Morecroft, J.H. Resistance and capacitance of coils at radio frequencies. IRE 1922, 10, 261–286. [Google Scholar] [CrossRef]
- Rosa, E.B.; Cohen, L. Formulae and tables for the calculation of mutual and self-inductance. Bull. Bur. Stand. 1907, 5. [Google Scholar] [CrossRef]
- Grover, F.W. Inductance Calculations; Dover Publications, Inc.: Mineola, NY, USA, 1946; pp. 77–87. [Google Scholar]
- Lin, J.; Saunders, R.; Schulmeister, K.; Söderberg, P.; Swerdlow, A.; Taki, M.; Veyret, B.; Ziegelberger, G.; Repacholi, M.H.; Matthes, R.; et al. ICNIRP guidelines for limiting exposure to time-varying electric and magnetic fields. Health Phys. 1998, 74, 494–522. [Google Scholar]
- C95.1-2005; IEEE Standard for Safety Levels with Respect to Human Exposure to Radio Frequency Electromagnetic Fields, 3 kHz to 300 GHz. IEEE: Piscataway, NJ, USA, 2005.
- Hui, S.Y.R.; Zhong, W.; Lee, C.K. A Critical Review of Recent Progress in Mid-Range Wireless Power Transfer. IEEE Trans. Power Electron. 2014, 29, 4500–4511. [Google Scholar] [CrossRef]
- Gilgenstein, O. Switched-Mode Power Supplies in Practice; Wiley: Hoboken, NJ, USA, 1989; pp. 161–200. [Google Scholar]
- TDK. Metallized Polypropylene Film Capacitors (MKP) Datasheet; Series/Type: B32651 … B32656; TDK Electronics AG: Woodbridge, NJ, USA, 2015. [Google Scholar]
- Fafoutis, X.; Clare, L.R.; Grabham, N.; Beeby, S.; Stark, B.H.; Piechocki, R.; Craddock, I. Energy-Neutral Activity Monitoring: Wearables Powered by Smart Inductive Charging Surfaces. In Proceedings of the IEEE International Conference on Sensing, Communication and Networking, London, UK, 27–30 June 2016. [Google Scholar]
- Burrow, S.; Clare, L. Open-loop power conditioning for vibration energy harvesters. Electron. Lett. 2009, 45, 999–1000. [Google Scholar] [CrossRef]

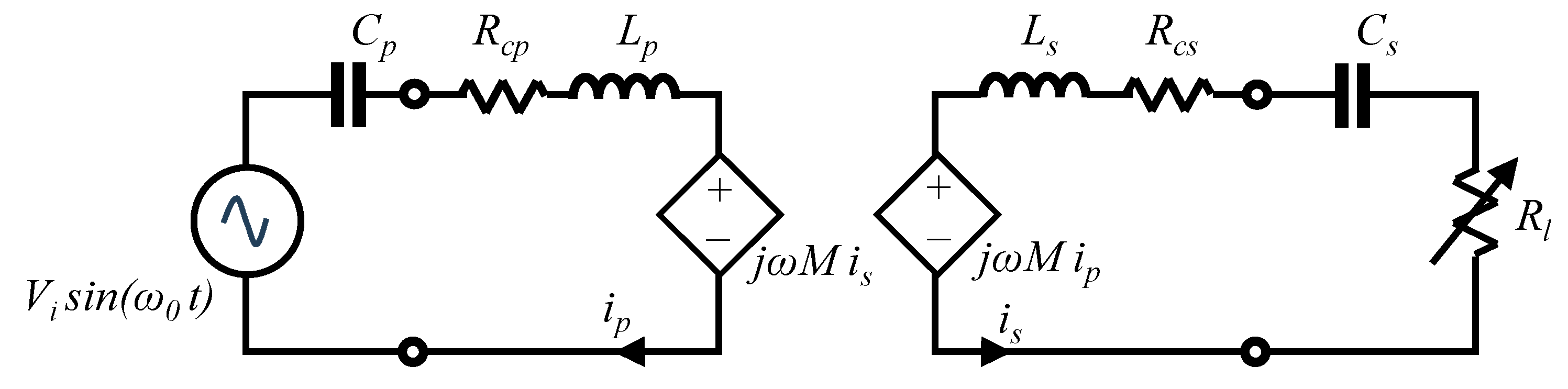






Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Burrow, S.; Clare, L.; Stark, B.; Beeby, S.; Grabham, N. Design Considerations and Experimental Testing of a Wide-Area Inductive Power Transfer (IPT) System for Body-Worn Electronics. Energies 2024, 17, 3367. https://doi.org/10.3390/en17143367
Burrow S, Clare L, Stark B, Beeby S, Grabham N. Design Considerations and Experimental Testing of a Wide-Area Inductive Power Transfer (IPT) System for Body-Worn Electronics. Energies. 2024; 17(14):3367. https://doi.org/10.3390/en17143367
Chicago/Turabian StyleBurrow, Steve, Lindsay Clare, Bernard Stark, Steve Beeby, and Neil Grabham. 2024. "Design Considerations and Experimental Testing of a Wide-Area Inductive Power Transfer (IPT) System for Body-Worn Electronics" Energies 17, no. 14: 3367. https://doi.org/10.3390/en17143367
APA StyleBurrow, S., Clare, L., Stark, B., Beeby, S., & Grabham, N. (2024). Design Considerations and Experimental Testing of a Wide-Area Inductive Power Transfer (IPT) System for Body-Worn Electronics. Energies, 17(14), 3367. https://doi.org/10.3390/en17143367







