Comparison of Maximum Heat Transfer Rate of Thin Vapor Chambers with Different Wicks under Multiple Heat Sources and Sinks
Abstract
1. Introduction
2. Analytical Model
2.1. Temperature Distribution Model
2.2. Hydrodynamic Models
2.3. Theoretical Approach for Maximum Heat Transfer Rate of TVCs
2.4. Validation
3. Results and Discussion
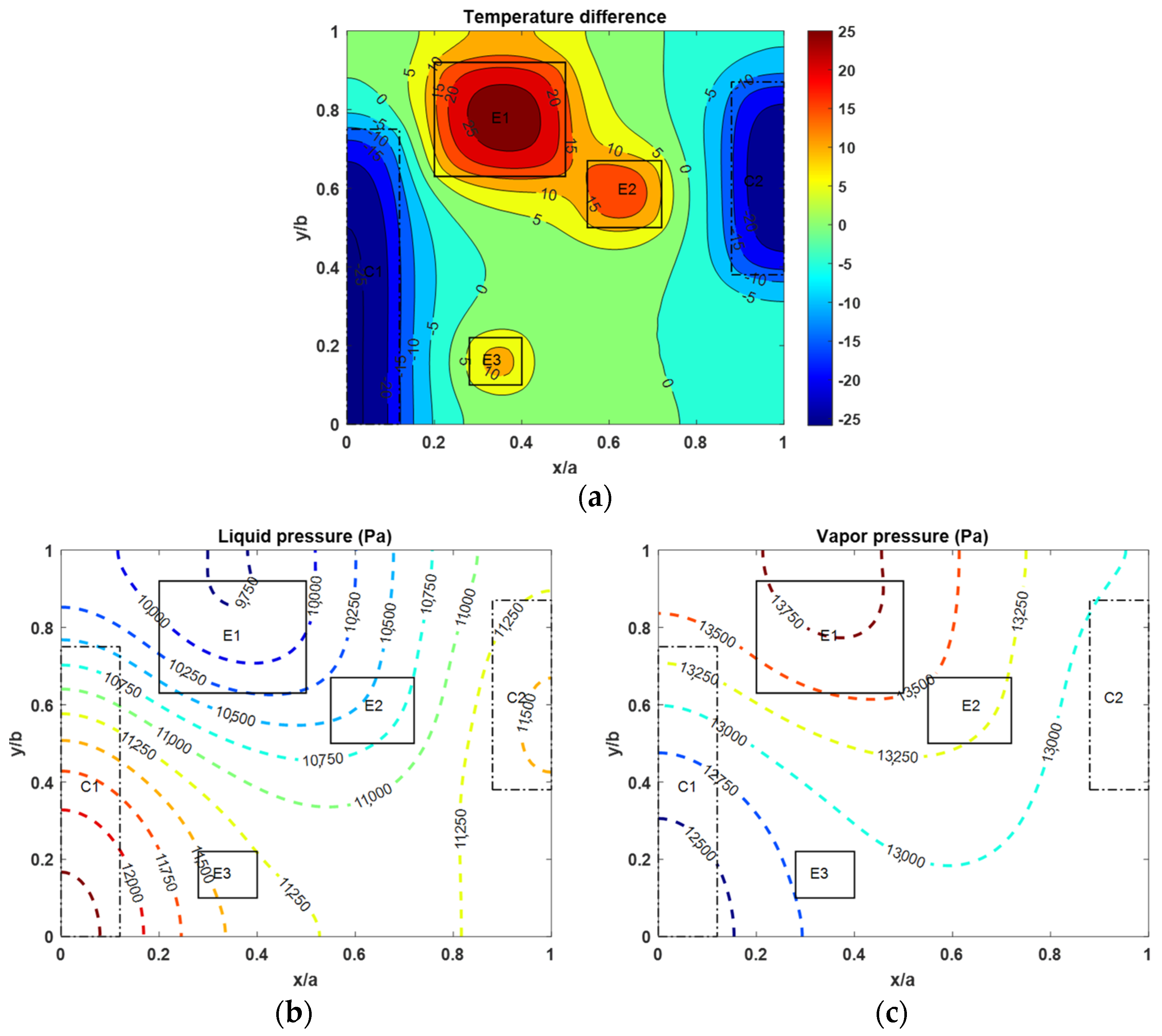
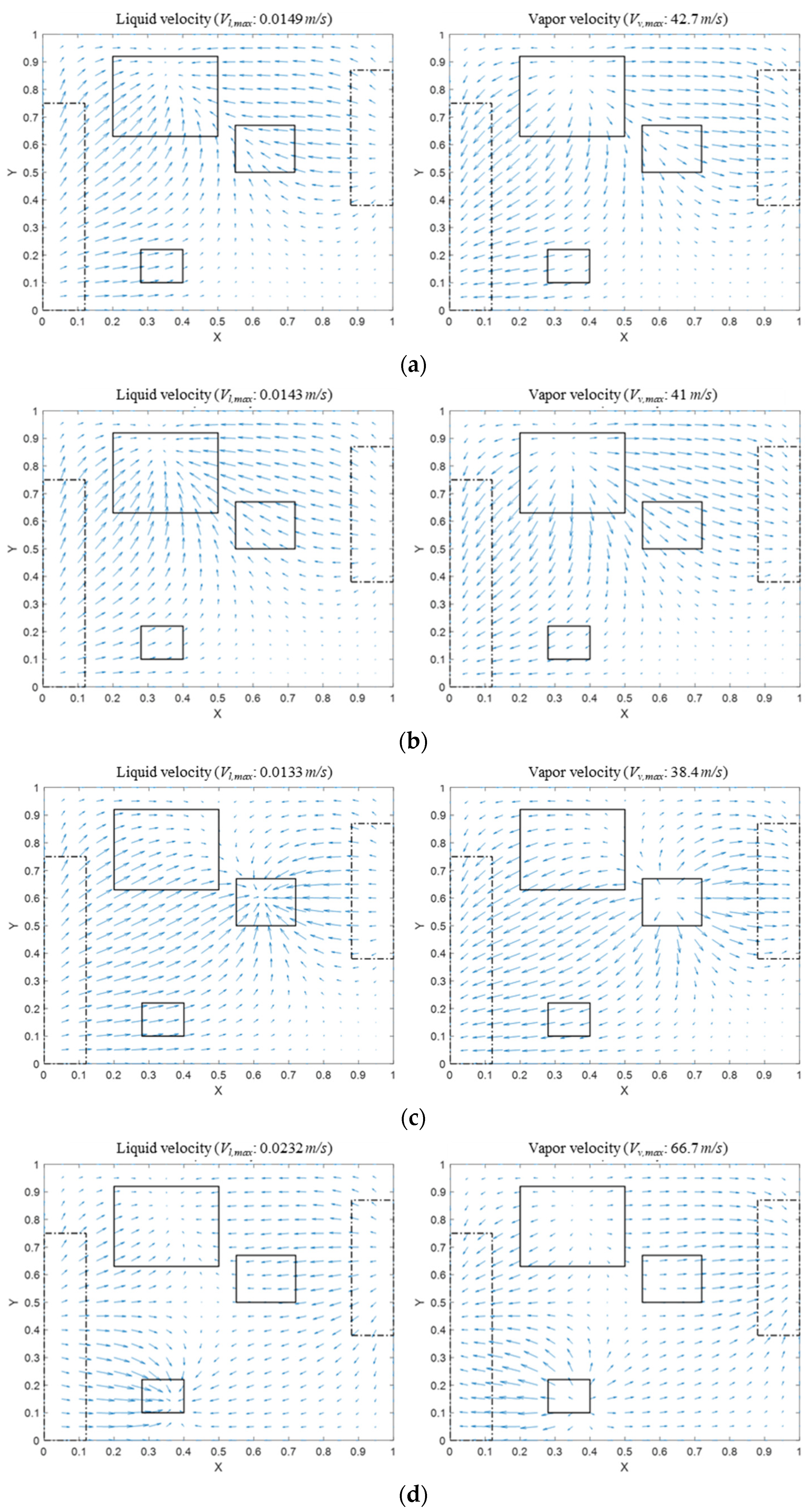
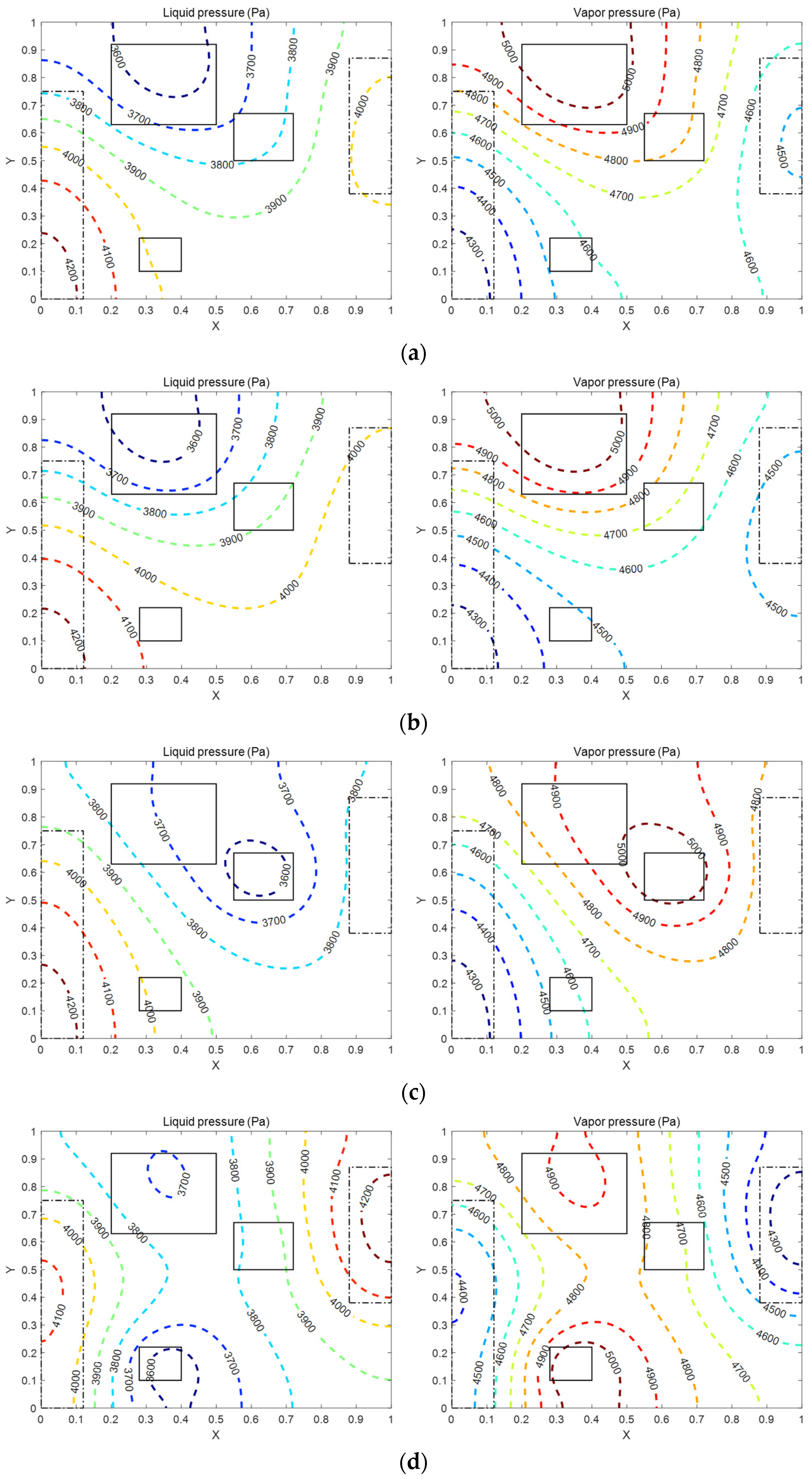
3.1. Flow Characteristics in Vapor Chamber under Different Heat Flux Conditions for Each Heat Source
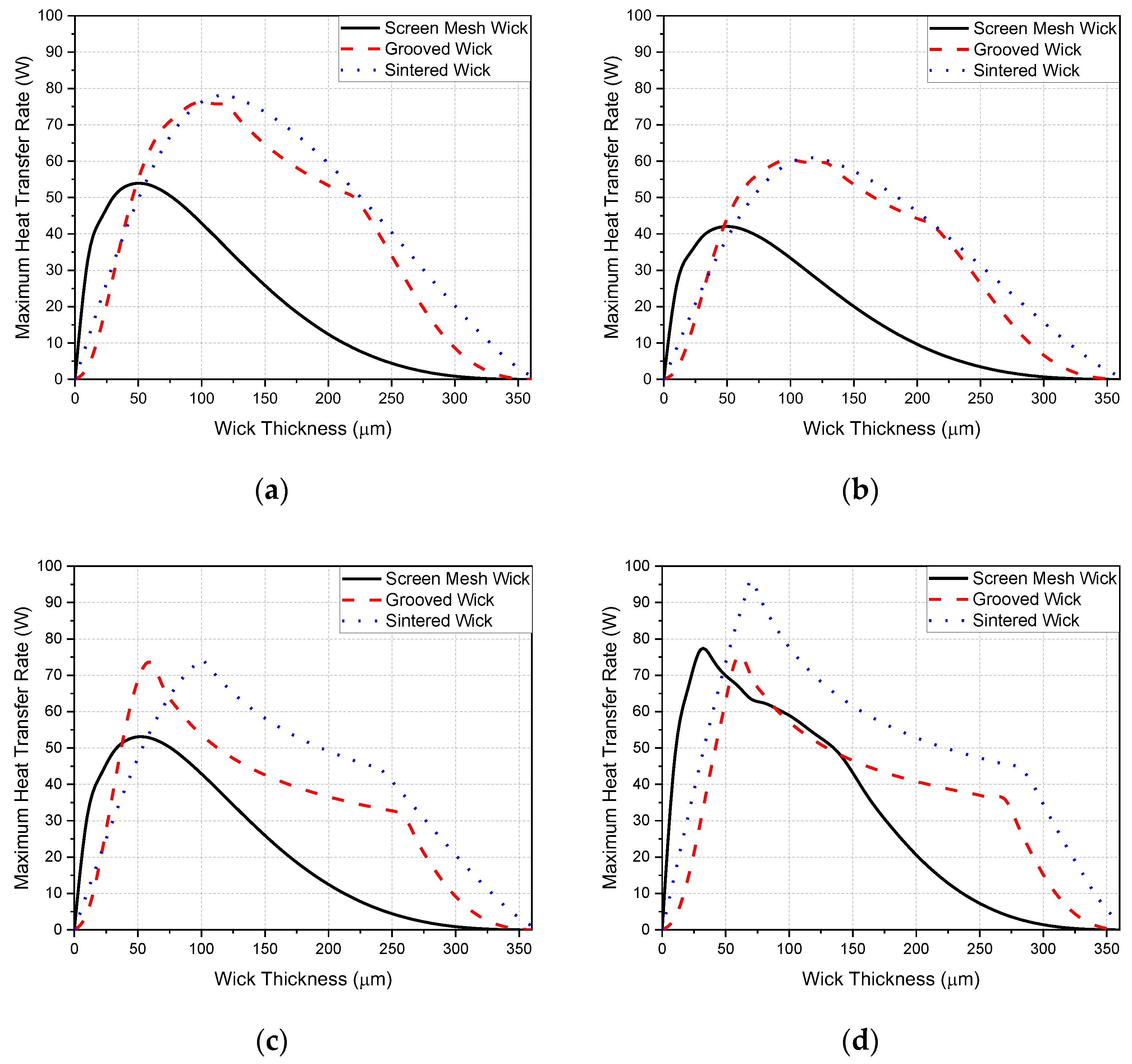
3.2. The Effect of Wick Type on the Maximum Heat Transfer Rate
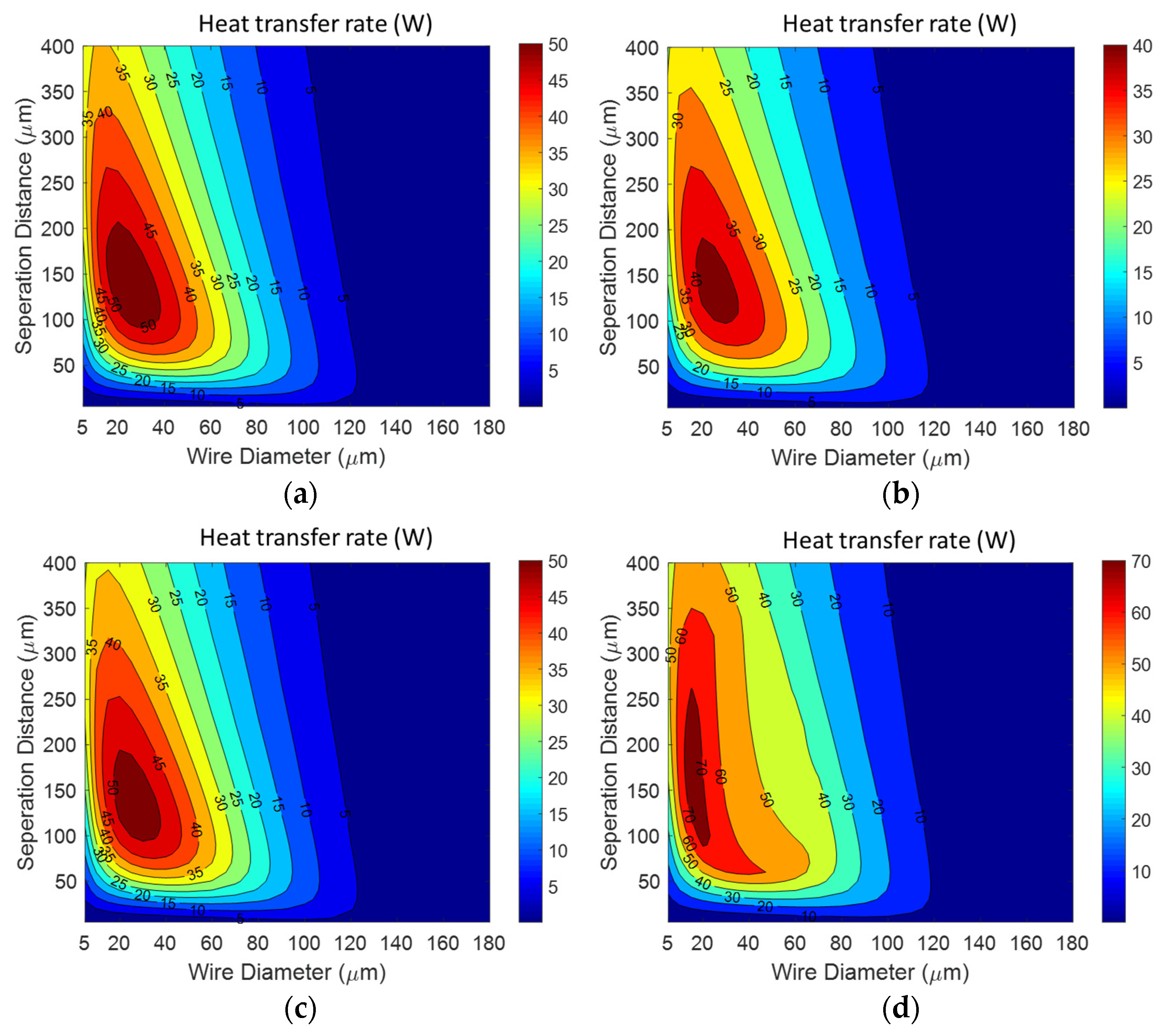
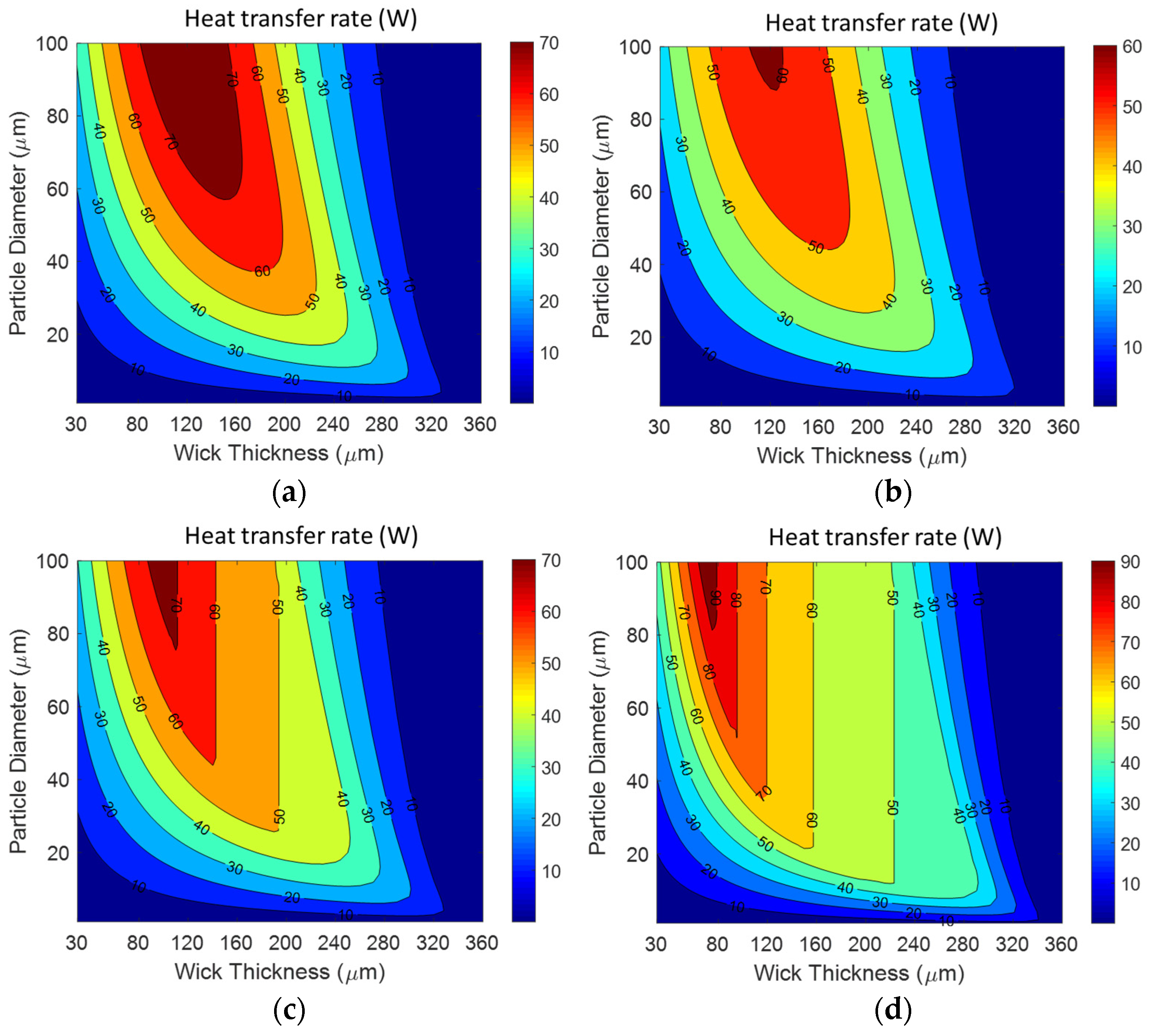
3.3. The Effects of Engineering Key Parameters on the Maximum Heat Transfer Rate
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| a, b, c | TVC dimensions [m] |
| A | total area [m2] |
| Am0, A0n, Amn | Fourier coefficients of temperature |
| Bi | Biot number (hc/kwall) |
| Bm0, B0n, Bmn | Fourier coefficients of heat flux |
| Cm0, C0n, Cmn | Fourier coefficients of pressure |
| Cp | specific heat [J·kg−1K−1] |
| d | wire diameter [m] |
| dg | distance of groove [m] |
| D | diameter of particle [m] |
| Dh | hydraulic diameter [m] |
| D* | effective diameter of particle [m] |
| f Re | Poiseuille number |
| h | equivalent heat transfer coefficient [W·m−2K−1] |
| hlv | latent heat of vaporization [J·kg−1] |
| H | thickness or height [m] |
| kwall | thermal conductivity [W·m−1K−1] |
| K | permeability [m2] |
| np | number of particles |
| N | mesh number |
| P | pressure [Pa] |
| Q | heat transfer rate [W] |
| r | pore radius [m] |
| T | temperature [K] |
| T* | dimensionless temperature |
| u | velocity [m·s−1] |
| V | volume [m3] |
| w | separation distance of wires [m] |
| wf | groove width [m] |
| x, y, z | coordinates [m] |
| X, Y, Z | dimensionless coordinates |
| Greek Symbols | |
| α | overlap ratio of particles |
| β, γ | dimensionless lengths |
| ε | porosity |
| η | ratio of the heat source area to the heat sink area |
| θ | contact angle |
| κ | weighting factor |
| μ | dynamic viscosity [kg·m−1s−1] |
| ρ | density [kg·m−3] |
| σ | surface tension [N·m−1] |
| φ | heat flux [W·m−2] |
| ϕ | dimensionless heat flux |
| ω | liquid pressure drop coefficient by no-slip condition |
| Subscripts | |
| b | bond |
| c | condenser |
| cap | capillary |
| e | evaporator |
| eff | effective |
| g | groove |
| l | liquid |
| o | imposed |
| p | particle |
| sat | saturation |
| v | vapor |
| w | wick |
References
- Ramadass, P.; Haran, B.; White, R.; Popov, B.N. Capacity fade of Sony 18650 cells cycled at elevated temperatures: Part I. Cycling performance. J. Power Sources 2002, 112, 606–613. [Google Scholar] [CrossRef]
- Bodenes, L.; Naturel, R.; Martinez, H.; Dedryvère, R.; Menetrier, M.; Croguennec, L.; Pérès, J.P.; Tessier, C.; Fischer, F. Lithium secondary batteries working at very high temperature: Capacity fade and understanding of aging mechanisms. J. Power Sources 2013, 236, 265–275. [Google Scholar] [CrossRef]
- Gurrum, S.P.; Edwards, D.R.; MarchandGolder, T.; Akiyama, J.; Yokoya, S.; Drouard, J.; Dahan, F. Generic thermal analysis for phone and tablet systems. In Proceedings of the 2012 IEEE 62nd Electronic Components and Technology Conference, San Diego, CA, USA, 29 May–1 June 2012. [Google Scholar]
- Kwon, H.K.; Hwang, J.; Chung, H.; Kang, M.; Cho, H.D.; Kim, Y.M. Thermal Power of Mobile Application Processor. Int. Symp. Microelectron. 2012, 1, 000866–000872. [Google Scholar] [CrossRef]
- Ahamed, M.S.; Saito, Y.; Mashiko, K.; Mochizuki, M. Characterization of a high performance ultra-thin heat pipe cooling module for mobile handheld electronic devices. Heat Mass Transf. 2017, 53, 3241–3247. [Google Scholar] [CrossRef]
- Choi, W.; Kang, M.; Han, S. Analysis of thermal characteristics of a battery module using a novel graphite material. In Proceedings of the 2019 IEEE Transportation Electrification Conference and Expo, Asia-Pacific, Seogwipo-si, Republic of Korea, 8–11 May 2019. [Google Scholar]
- Li, J.; Lv, L.; Zhou, G.; Li, X. Mechanism of a microscale flat plate heat pipe with extremely high nominal thermal conductivity for cooling high-end smartphone chips. Energy Convers. Manag. 2019, 201, 112202. [Google Scholar] [CrossRef]
- Huang, G.; Liu, W.; Luo, Y.; Deng, T.; Li, Y.; Chen, H. Research and optimization design of limited internal cavity of ultra-thin vapor chamber. Int. J. Heat Mass Transf. 2020, 148, 119101. [Google Scholar] [CrossRef]
- Li, B.; Yin, X.; Tang, W.; Zhang, J. Optimization design of grooved evaporator wick structures in vapor chamber heat spreaders. Appl. Therm. Eng. 2020, 166, 114657. [Google Scholar] [CrossRef]
- Ranjan, R.; Murthy, J.Y.; Garimella, S.V.; Altman, D.H.; North, M.T. Modeling and design optimization of ultrathin vapor chambers for high heat flux applications. IEEE Trans. Compon. Packag. Manuf. Technol. 2012, 2, 1465–1479. [Google Scholar] [CrossRef]
- Li, D.; Huang, Z.; Zhao, J.; Jian, Q.; Chen, Y. Analysis of heat transfer performance and vapor–liquid meniscus shape of ultra-thin vapor chamber with supporting columns. Appl. Therm. Eng. 2021, 193, 117001. [Google Scholar] [CrossRef]
- Lefevre, F.; Lallemand, M. Coupled thermal and hydrodynamic models of flat micro heat pipes for the cooling of multiple electronic components. Int. J. Heat Mass Transf. 2006, 49, 1375–1383. [Google Scholar] [CrossRef]
- Subedi, B.; Kim, S.H.; Jang, S.P. Effect of mesh wick geometry on the maximum heat transfer rate of flat-micro heat pipes with multiple heat sources and sinks. Int. J. Heat Mass Transf. 2019, 131, 537–545. [Google Scholar] [CrossRef] [PubMed]
- Kim, S.J.; Seo, J.K.; Do, K.H. Analytical and experimental investigation on the operational characteristics and the thermal optimization of a miniature heat pipe with a grooved wick structure. Int. J. Heat Mass Transfer. 2003, 46, 2051–2063. [Google Scholar] [CrossRef]
- Khrustalev, D.; Faghri, A. Thermal analysis of a micro heat pipe. ASME J. Heat Transf. 1994, 116, 189–198. [Google Scholar] [CrossRef]
- Kim, S.B.; Kim, K.H.; Jang, S.P.; Kedzierski, M.A. Thermal characteristics of silicon wafer-based TVCs (thin vapor chambers) with disk-shape using DI water. Int. J. Heat Mass Transf. 2018, 127, 526–534. [Google Scholar] [CrossRef]
- Faghri, A. Heat Pipe Science and Technology, 1st ed.; Taylor & Francis: Washington, DC, USA, 1995. [Google Scholar]
- Kakaç, S.; Shah, R.K.; Aung, W. Handbook of Single-Phase Convective Heat Transfer; John-Wiley Sons: New York, NY, USA, 1987. [Google Scholar]
- Revellin, R.; Rulliere, R.; Lefèvre, F.; Bonjour, J. Experimental validation of an analytical model for predicting the thermal and hydrodynamic capabilities of flat micro heat pipes. Appl. Therm. Eng. 2009, 29, 1114–1122. [Google Scholar] [CrossRef]
- Kaviany, M. Principles of Heat Transfer in Porous Media; Springer: New York, NY, USA, 1995. [Google Scholar]
- Chi, S.W. Heat Pipe Theory and Practice: A Sourcebook; Hemisphere Pub.: Washington, DC, USA, 1976. [Google Scholar]
- Slobozhanin, L.A.; Alexander, J.I.D.; Collicott, S.H.; Gonzalez, S.R. Capillary pressure of a liquid in a layer of close-packed uniform spheres. Phys. Fluids 2006, 18, 082104. [Google Scholar] [CrossRef]
- Byon, C.; Kim, S.J. Capillary performance of bi-porous sintered metal wicks. Int. J. Heat Mass Transf. 2012, 55, 4096–4103. [Google Scholar] [CrossRef]
- Qu, Y.; Zhou, K.; Zhang, K.F.; Tian, Y. Effects of multiple sintering parameters on the thermal performance of bi-porous nickel wicks in Loop Heat Pipes. Int. J. Heat Mass Transf. 2016, 99, 638–646. [Google Scholar] [CrossRef]
- Xiao, B.; Faghri, A. A three-dimensional thermal-fluid analysis of flat heat pipes. Int. J. Heat Mass Transf. 2008, 51, 3113–3126. [Google Scholar] [CrossRef]
- Weibel, J.A.; Garimella, S.V. Visualization of vapor formation regimes during capillary-fed boiling in sintered-powder heat pipe wicks. Int. J. Heat Mass Transf. 2012, 55, 3498–3510. [Google Scholar] [CrossRef]
- Byon, C.; Kim, S.J. Effects of geometrical parameters on the boiling limit of bi-porous wicks. Int. J. Heat Mass Transf. 2012, 55, 7884–7891. [Google Scholar] [CrossRef]
- Hong, F.J.; Cheng, P.; Wu, H.Y.; Sun, Z. Evaporation/boiling heat transfer on capillary feed copper particle sintered porous wick at reduced pressure. Int. J. Heat Mass Transf. 2013, 63, 389–400. [Google Scholar] [CrossRef]
- Li, Y.; Li, Z.; Zhou, W.; Zeng, Z.; Yan, Y.; Li, B. Experimental investigation of vapor chambers with different wick structures at various parameters. Exp. Therm. Fluid Sci. 2016, 77, 132–143. [Google Scholar] [CrossRef]
- Deng, D.; Huang, Q.; Xie, Y.; Huang, X.; Chu, X. Thermal performance of composite porous vapor chambers with uniform radial grooves. Appl. Therm. Eng. 2017, 125, 1334–1344. [Google Scholar] [CrossRef]
- Zhang, S.; Chen, C.; Chen, G.; Sun, Y.; Tang, Y.; Wang, Z. Capillary performance characterization of porous sintered stainless steel powder wicks for stainless steel heat pipes. Int. Commun. Heat Mass Transf. 2020, 116, 104702. [Google Scholar] [CrossRef]



| Parameters | Specification |
|---|---|
| Vapor chamber length (a) | 40 mm |
| Vapor chamber breadth (b) | 40 mm |
| Height of wall (c) | 265 µm |
| Total height (Hw + Hv) | 370 µm |
| Wick material | Copper |
| Working fluid | D.I. water |
| Saturation temperature (Tsat) | 30 °C |
| Position of heat source 1 (E1) | 0.25 ≤ x/a ≤ 0.4, 0.65 ≤ y/b ≤ 0.8 |
| Position of heat source 2 (E2) | 0.6 ≤ x/a ≤ 0.75, 0.55 ≤ y/b ≤ 0.7 |
| Position of heat source 3 (E3) | 0.25 ≤ x/a ≤ 0.4, 0.2 ≤ y/b ≤ 0.35 |
| Position of heat sink 1 (C1) | 0 ≤ x/a ≤ 0.1, 0 ≤ y/b ≤ 1 |
| Position of heat sink 2 (C2) | 0.9 ≤ x/a ≤ 1, 0 ≤ y/b ≤ 1 |
| Screen mesh wick | |
| Wire diameter (d) | 5–180 µm |
| Wire separation distance (w) | 5–400 µm |
| Wick thickness (Hw) | 10–360 µm |
| Grooved wick | |
| Fin width (wf) | 4–86 µm |
| Groove height (Hg) | 10–360 µm |
| Groove distance (dg) | 5–200 µm |
| Sintered wick | |
| Particle diameter (D) | 1–100 µm |
| Wick thickness (Hw) | 30–360 µm |
| Overlap ratio of particles (α) | 0.025 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kim, S.H.; Kang, S.Y.; Park, S.J.; Jang, S.P. Comparison of Maximum Heat Transfer Rate of Thin Vapor Chambers with Different Wicks under Multiple Heat Sources and Sinks. Energies 2024, 17, 3330. https://doi.org/10.3390/en17133330
Kim SH, Kang SY, Park SJ, Jang SP. Comparison of Maximum Heat Transfer Rate of Thin Vapor Chambers with Different Wicks under Multiple Heat Sources and Sinks. Energies. 2024; 17(13):3330. https://doi.org/10.3390/en17133330
Chicago/Turabian StyleKim, Sung Hyoun, Seo Yeon Kang, Sung Jun Park, and Seok Pil Jang. 2024. "Comparison of Maximum Heat Transfer Rate of Thin Vapor Chambers with Different Wicks under Multiple Heat Sources and Sinks" Energies 17, no. 13: 3330. https://doi.org/10.3390/en17133330
APA StyleKim, S. H., Kang, S. Y., Park, S. J., & Jang, S. P. (2024). Comparison of Maximum Heat Transfer Rate of Thin Vapor Chambers with Different Wicks under Multiple Heat Sources and Sinks. Energies, 17(13), 3330. https://doi.org/10.3390/en17133330






