Experimental Study for Understanding the Characteristics of a Floating Axis Wind Turbine under Wind and Wave Conditions
Abstract
1. Introduction
2. Methods
2.1. FAWT Model for Water Tank Experiment
2.2. Experimental Setup
2.3. Experimental Conditions
- (1)
- regular waves: RAO of the FAWT (parking or operating)
- (2)
- irregular waves: response of the FAWT (parking or operating)
- (3)
- rapid decrease of wind velocity: wind velocity changes from 2.0 to 0.0 [m/s]
- (4)
- transient response in start-up: number of rotations changes from 0 to 70 [rpm]
- (5)
- transient response in shutdown: number of rotations changes from 70 to 0 [rpm]
- (6)
- unbalanced blades weight: an additional weight is attached on the top of one blade
- (7)
- passive braking system for rotor over speed: drag parts are attached on the spar
- (8)
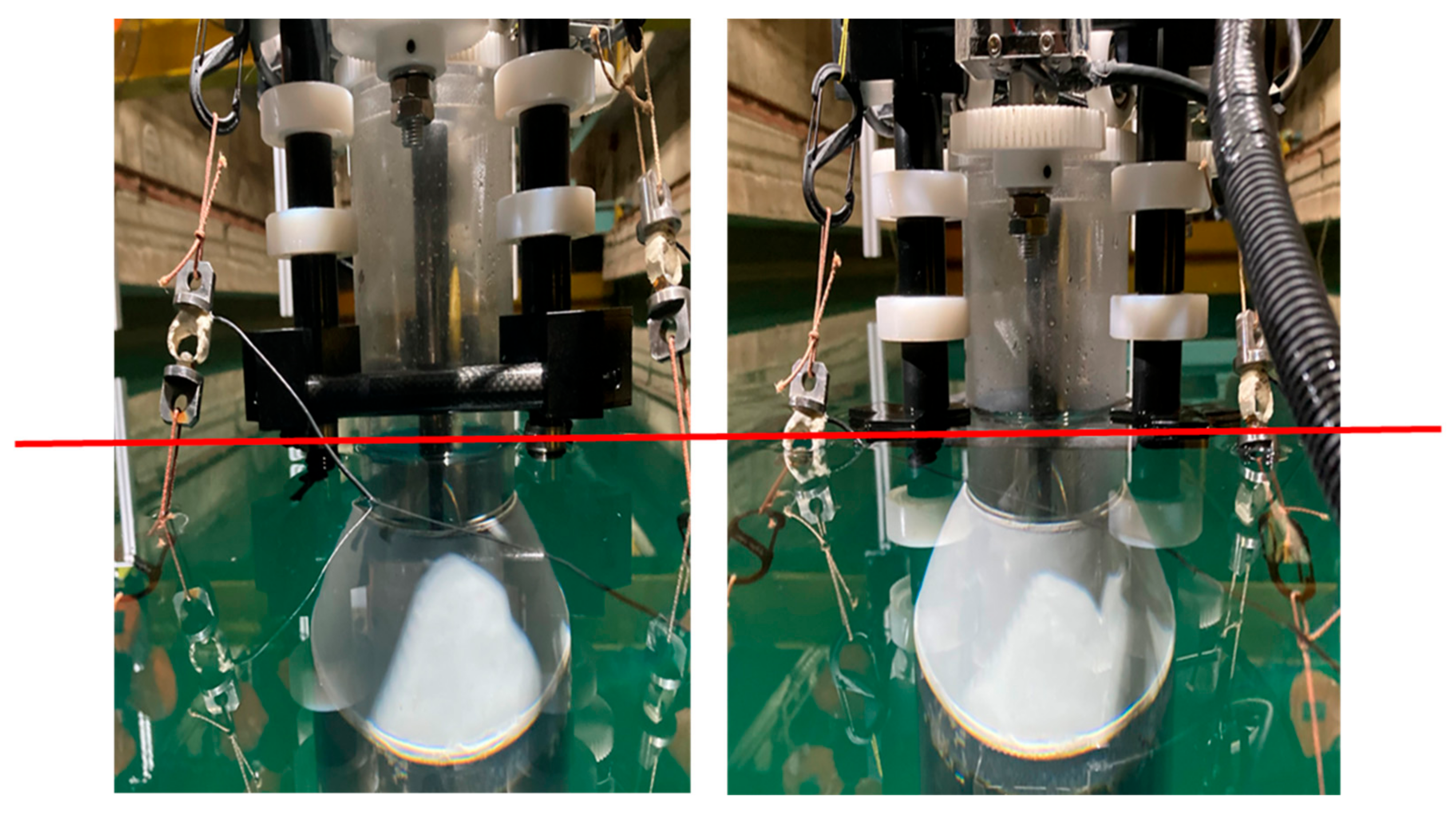
- damaged case: one block of the spar near the sea surface is assumed to be flooded
3. Experiment Results and Discussions
3.1. Decay Tests
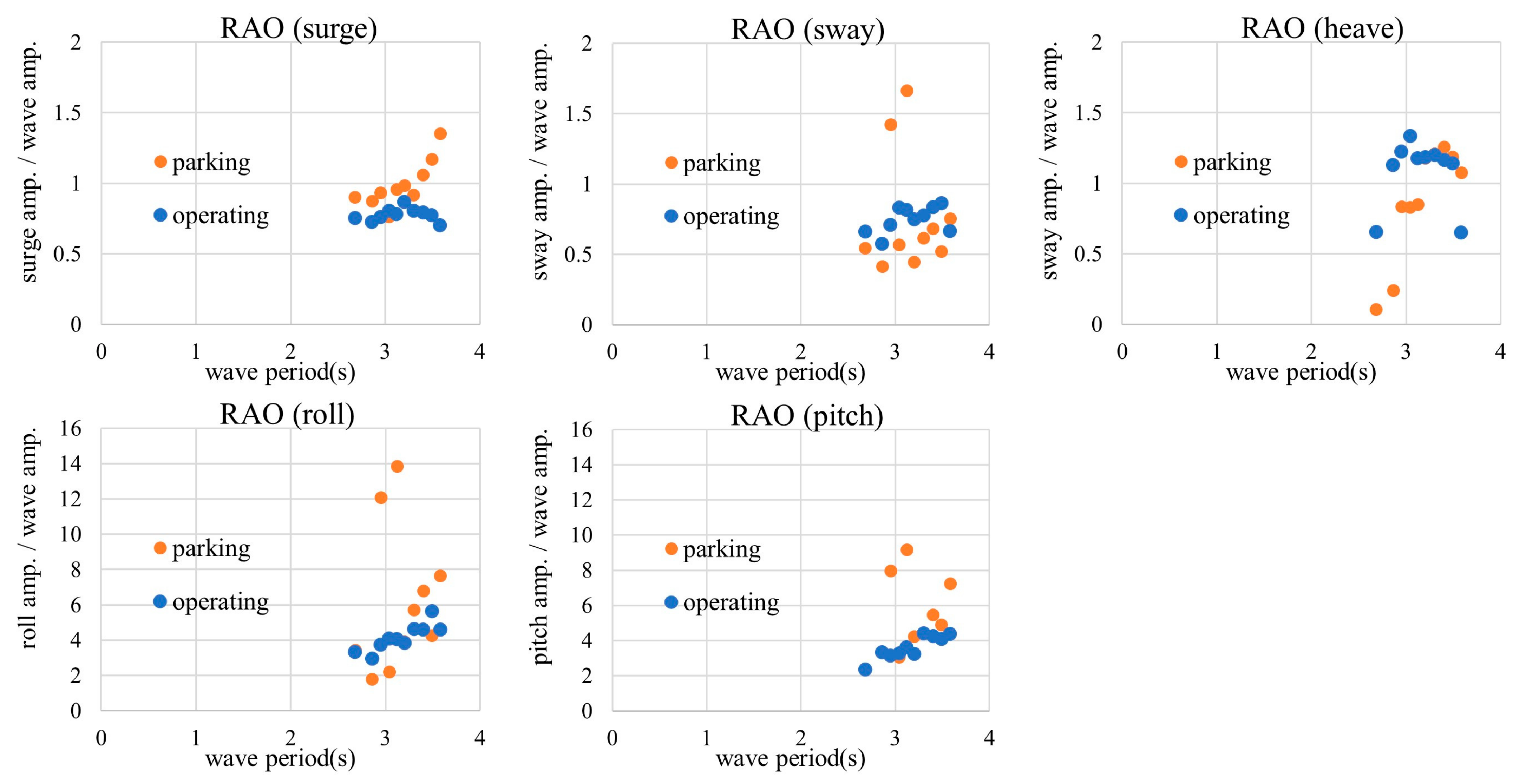
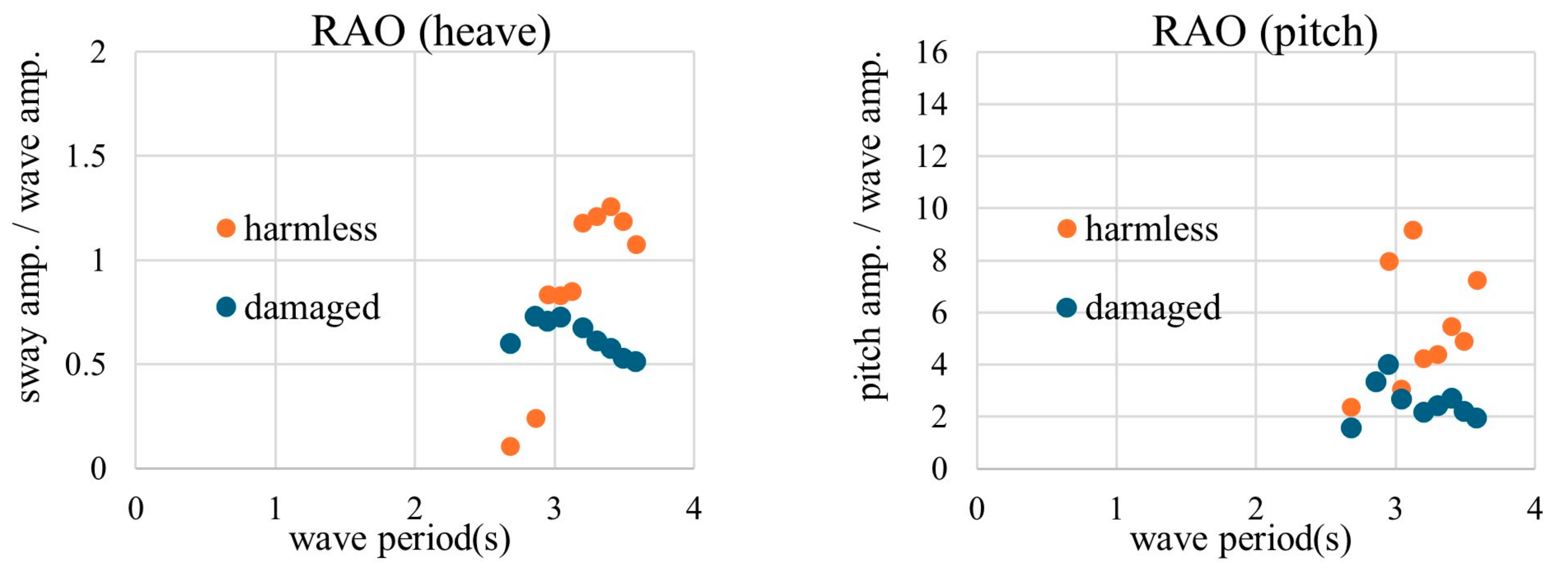
3.2. RAO of the FAWT
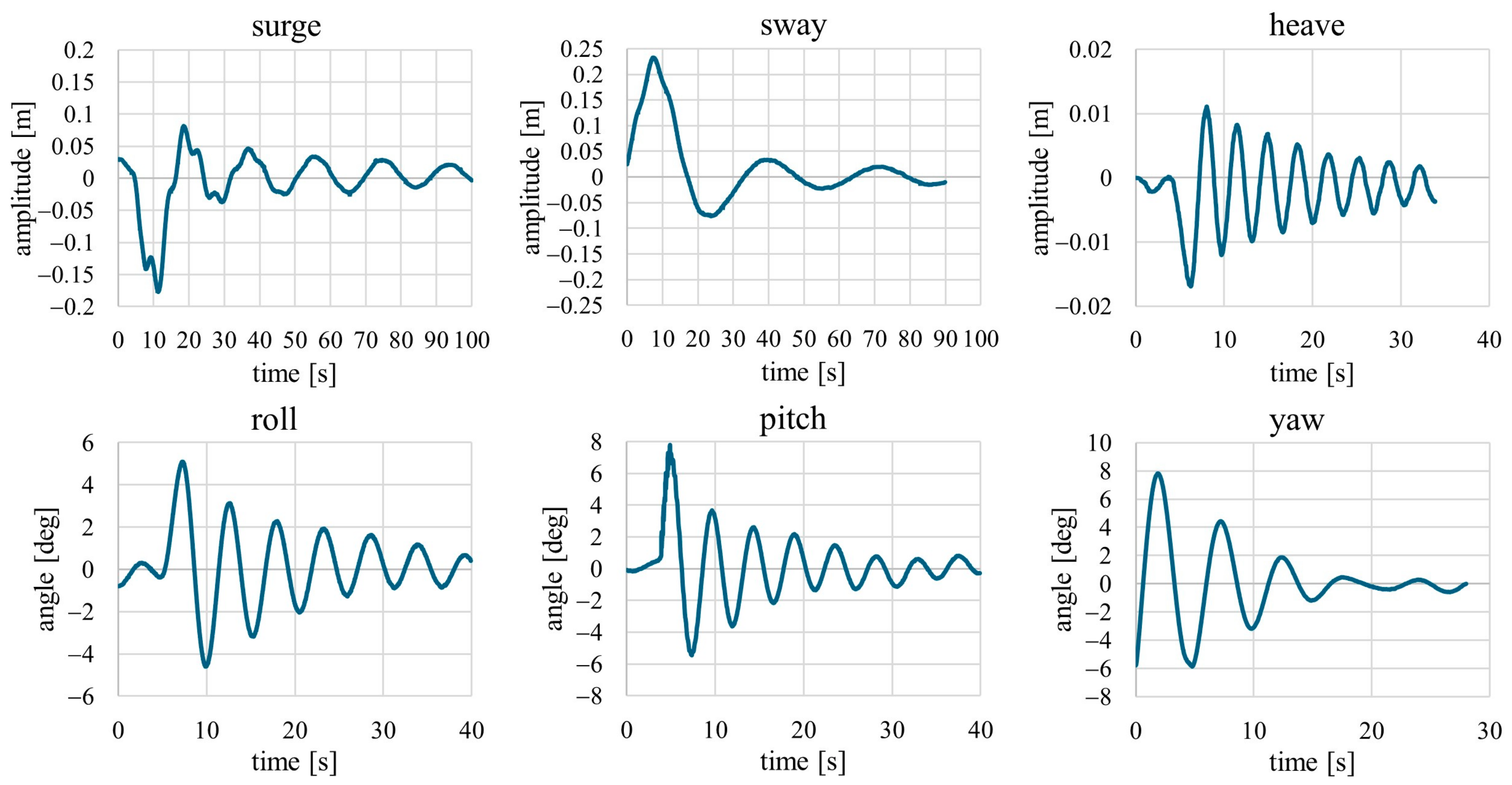
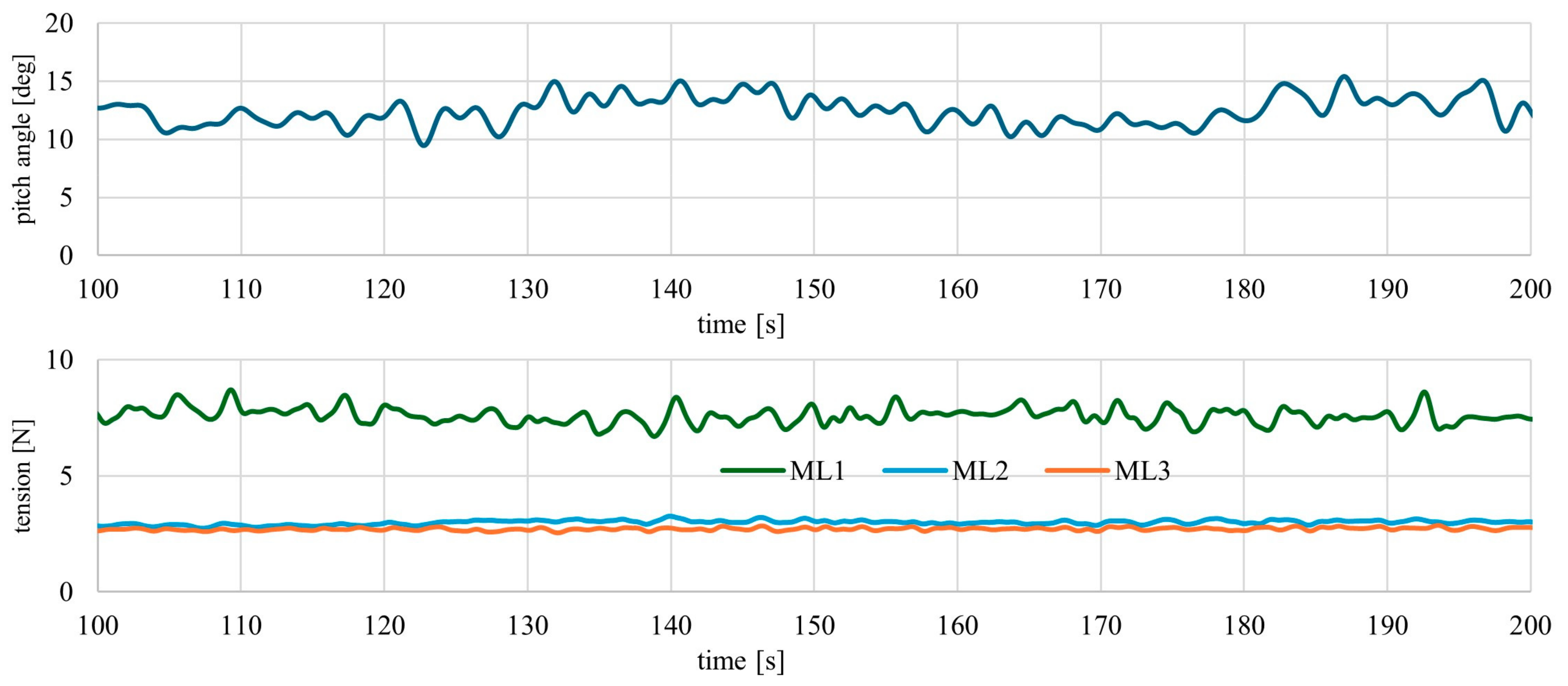
3.3. Response of the FAWT under the Irregular Waves
3.4. Response under the Rapid Decrease of Wind Velocity Condition
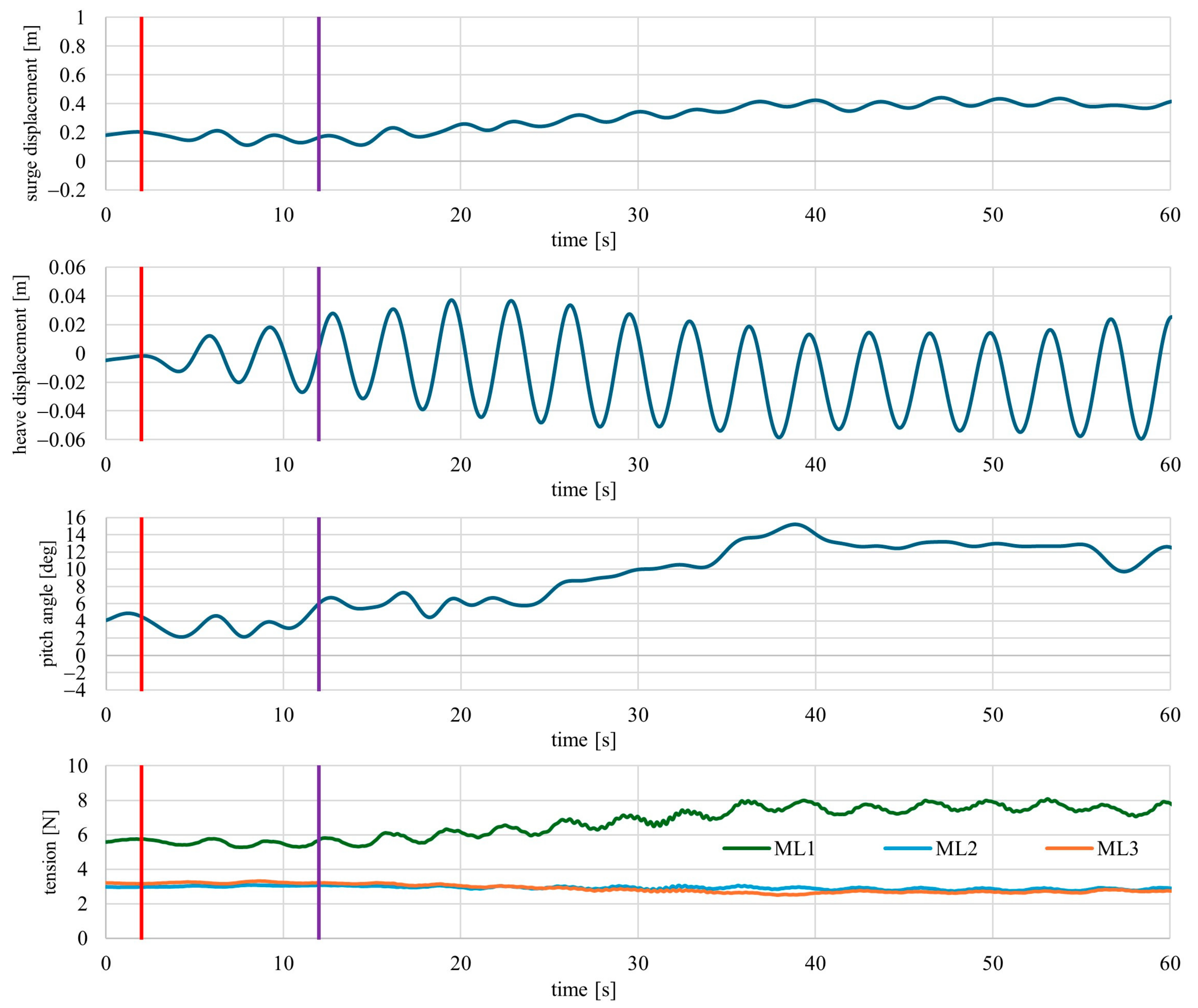
3.5. Transient Response in Start-Up or Shutdown
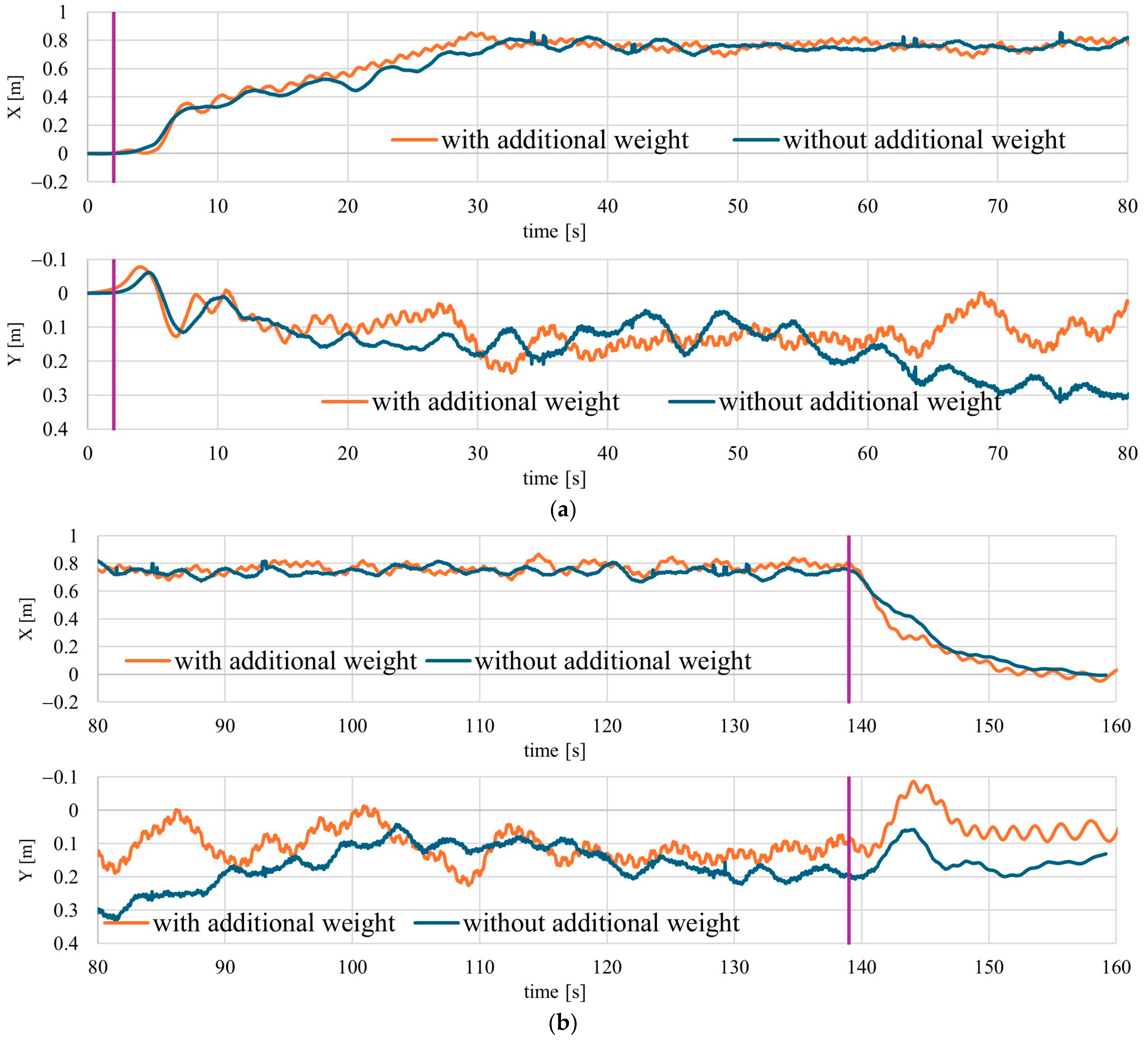
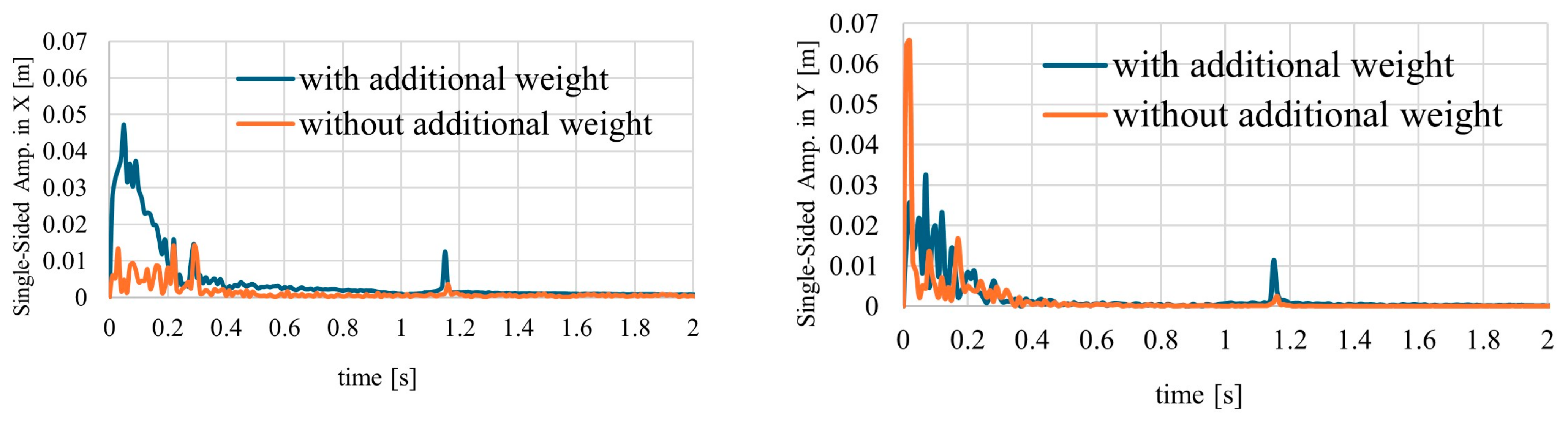
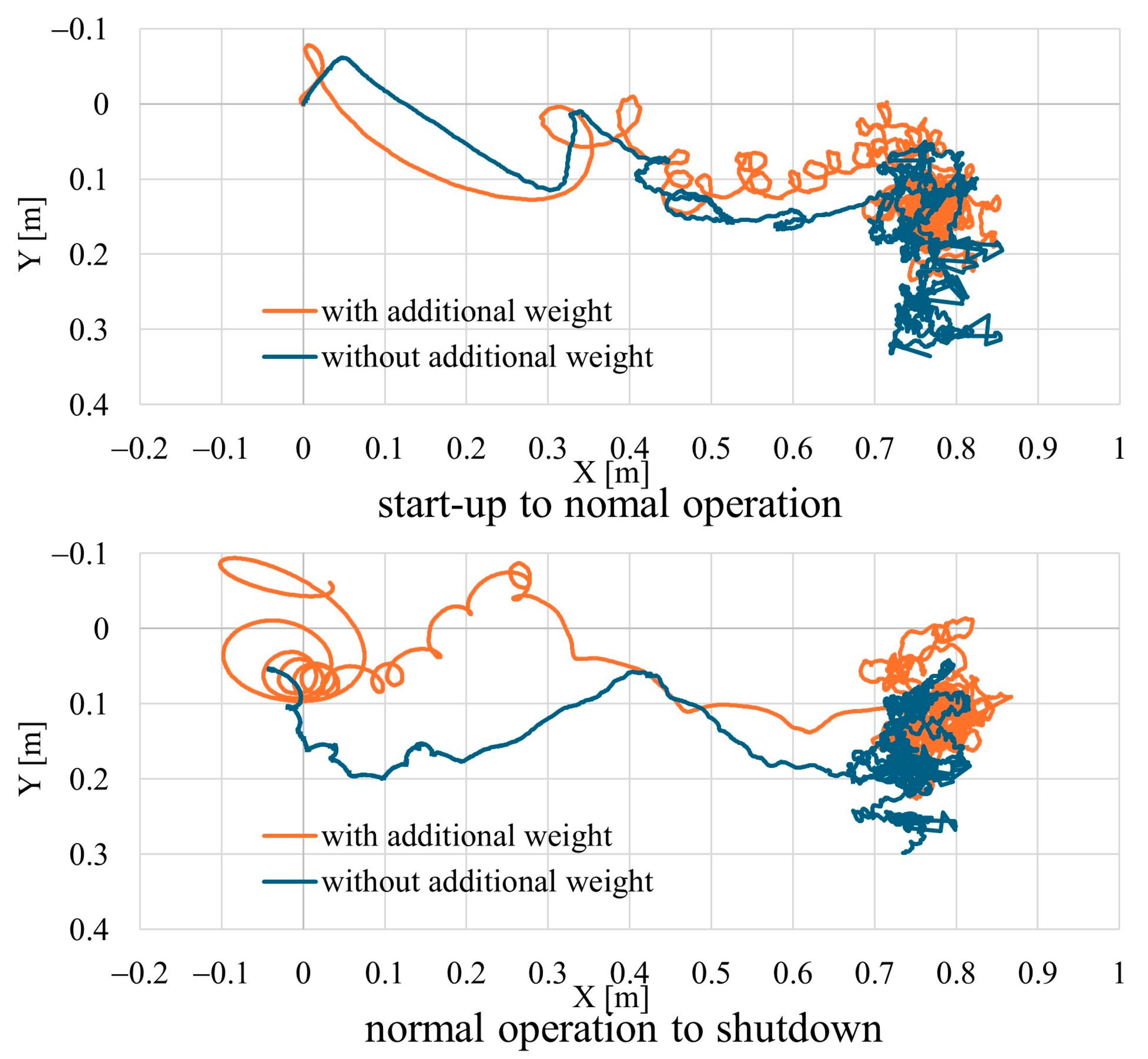
3.6. Unbalanced Blades Weight Case
3.7. Passive Braking System for the Rotor Overspeed
3.8. Damaged Case
4. Conclusions
- The heave RAO of the FAWT model is approximately 1.3—a value that is comparable to those of the other FOWTs, whose floaters are relatively large compared with that of the FAWT.
- Pitch and roll RAOs under the rated operation are smaller than those under the parking condition due to the rotor gyroscopic effect.
- Under the irregular waves, normalized heave amplitude by the incoming significant wave height is approximately 1.0.
- In case of the operating FAWT under irregular waves, the maximum tension of the mooring line to the upwind side is approximately twice of its initial one in still water. Meanwhile, the mean tensions of mooring lines to the downwind side decrease by approximately 10–20% percent from their initial ones.
- A sudden decrease of incoming wind does not affect the dynamics of the operating FAWT because of its inertia.
- There are no unsafe responses during the start-up or shutdown phase. However, the disruption of blade weight balance leads to a slewing motion of the center shaft.
- Appendages that submerge in the water only when the rotor overspeed occurs can work as a passive braking system.
- The damping force caused by the submergence of PTO frame will contribute to the safety of the damaged case.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| wavelength [m] | |
| gravity acceleration [m/s2] | |
| wave height [m] (irregular wave case: significant wave height [m]) | |
| wave period [s] (irregular wave case: mean wave period [s]) | |
| wave slope angle [rad] | |
| Abbreviations | |
| FOWTs | Floating offshore wind turbines |
| FAWT | Floating axis wind turbine |
| HAWT | Horizontal axis wind turbine |
| VAWT | Vertical axis wind turbine |
| TLP | Tension-leg platform |
| FVAWT | Floating vertical axis wind turbine |
| CFD | Computational fluid dynamics |
| LDV | Laser doppler velocity |
| PTO | Power take-off |
| DTU | Technical University of Denmark |
| BSG | Bulk specific gravity |
| NOWPHAS | Nationwide Ocean Wave information network for Ports and HArbourS |
| RAO | Response amplitude operator |
| ITTC | International Towing Tank Conference |
References
- Takata, T.; Takaoka, M.; Gonçalves, R.T.; Houtani, H.; Yoshimura, Y.; Hara, K.; Oh, S.; Dotta, R.; Malta, E.B.; Iijima, K.; et al. Dynamic Behavior of a Flexible Multi-Column FOWT in Regular Waves. J. Mar. Sci. Eng. 2021, 9, 124. [Google Scholar] [CrossRef]
- Leroy, V.; Delacroix, S.; Merrien, A.; Bachynski-Polić, E.E.; Gilloteaux, J.-C. Experimental investigation of the hydro-elastic response of a spar-type floating offshore wind turbine. Ocean Eng. 2022, 255, 111430. [Google Scholar] [CrossRef]
- Vittori, F.; Azcona, J.; Eguinoa, I.; Pires, O.; Rodríguez, A.; Morató, Á.; Garrido, C.; Desmond, C. Model tests of a 10MW semi-submersible floating wind turbine under waves and wind using hybrid method to integrate the rotor thrust and moments. Wind Energy Sci. 2022, 7, 5. [Google Scholar] [CrossRef]
- Zhang, H.; Wen, B.; Tian, X.; Li, X.; Dong, Y.; Wang, M.; Peng, Z. Experimental study on mitigating vibration of floating offshore wind turbine using tuned mass damper. Ocean Eng. 2023, 288, 115974. [Google Scholar] [CrossRef]
- Ghigo, A.; Faraggiana, E.; Giorgi, G.; Mattiazzo, G.; Bracco, G. Floating Vertical Axis Wind Turbines for offshore applications among potentialities and challenges: A review. Renew. Sustain. Energy Rev. 2024, 193, 114302. [Google Scholar] [CrossRef]
- Paulsena, U.S.; Vita, L.; Madsenb, H.A.; Hattelc, J.; Ritchie, E.; Leban, K.M.; Berthlsen, P.A.; Carstensen, S. 1st DeepWind 5 MW baseline design. Energy Procedia 2012, 24, 27–35. [Google Scholar] [CrossRef]
- Paulsena, U.S.; Madsenb, H.A.; Hattelc, J.H.; Baranc, I.; Nielsenb, P.H. Design Optimization of a 5 MW Floating Offshore Vertical-Axis Wind Turbine. Energy Procedia 2014, 53, 268–279. [Google Scholar] [CrossRef]
- Borg, M.; Collu, M. A comparison on the dynamics of a floating vertical axis wind turbine on three different floating support structures. Energy Procedia 2013, 35, 22–32. [Google Scholar] [CrossRef]
- Ishie, J.; Wang, K.; Ong, M.C. Structural Dynamic Analysis of Semi-Submersible Floating Vertical Axis Wind Turbines. Energies 2016, 9, 1047. [Google Scholar] [CrossRef]
- Robertson, A.; Jonkman, J.; Masciola, M.; Song, H.; Goupee, A.; Coulling, A.; Luan, C. Definition of the Semisubmersible Floating System for Phase II of OC4; National Renewable Energy Laboratory: Golden, CO, USA, 2012. [Google Scholar]
- Battisti, L.; Benini, E.; Brighenti, A.; Castelli, M.R.; Dell’Anna, S.; Dossena, V.; Persico, G.; Schmidt Paulsen, U.; Pedersen, T.F. Wind tunnel testing of the DeepWind demonstrator in design and tilted operating conditions. Energy 2016, 111, 484–497. [Google Scholar] [CrossRef]
- Iwamatsu, S.; Suzuki, H.; Nihei, Y. Study on Elastic Response of Double-Rotor VAWTs. J. Mar. Sci. Eng. 2022, 10, 1400. [Google Scholar] [CrossRef]
- Hand, B.; Cashman, A. Conceptual design of a large-scale floating offshore vertical axis wind turbine. Energy Procedia 2017, 142, 83–88. [Google Scholar] [CrossRef]
- Yang, Y.; Guo, Z.; Zhang, Y.; Jinyama, H.; Li, Q. Numerical Investigation of the Tip Vortex of a Straight-Bladed Vertical Axis Wind Turbine with Double-Blades. Energies 2017, 10, 1721. [Google Scholar] [CrossRef]
- Huijs, F.; Vlasveld, E.; Gormand, M.; Savenije, F.; Caboni, M.; LeBlanc, B.; Simao Ferreira, C.; Lindenburg, K.; Gueydon, S.; Otto, W.; et al. Integrated design of a semi-submersible floating vertical axis wind turbine (VAWT) with active blade pitch control. In Proceedings of the Journal of Physics: Conference Series, 1104, Trondheim, Norway, 17–19 January 2018. [Google Scholar]
- Akimoto, H.; Tanaka, K.; Uzawa, K. Floating axis wind turbines for offshore power generation-a conceptual study. Environ. Res. Lett. 2011, 6, 044017. [Google Scholar] [CrossRef]
- SeaTwirl. Available online: https://seatwirl.com/ (accessed on 30 May 2024).
- Hmedi, M.; Uzunoglu, E.; Zeng, C.; Gaspar, J.F.; Guedes Soares, C. Experimental Challenges and Modelling Approaches of Floating Wind Turbines. J. Mar. Sci. Eng. 2023, 11, 2048. [Google Scholar] [CrossRef]
- Russo, V.; Contestabile, P.; Bardazzi, A.; Leone, E.; Iglesias, G.; Tomasicchio, G.R.; Vicinanza, D. Dynamic Loads and Response of a Spar Buoy Wind Turbine with Pitch-Controlled Rotating Blades: An Experimental Study. Energies 2021, 14, 3598. [Google Scholar] [CrossRef]
- Takata, T.; Takaoka, M.; Houtani, H.; Hara, K.; Oh, S.; Malta, E.B.; Iijima, K.; Suzuki, H.; Gonçalves, R.T. Effect of Heave Plates on the Wave Motion of a Flexible Multicolumn FOWT. Energies 2022, 15, 7605. [Google Scholar] [CrossRef]
- Robertson, A.; Wang, L. OC6 Phase Ib: Floating Wind Component Experiment for Difference-Frequency Hydrodynamic Load Validation. Energies 2021, 14, 6417. [Google Scholar] [CrossRef]
- Tagliafierro, B.; Karimirad, M.; Altomare, C.; Goteman, M.; Martinez-Estevez, I.; Capasso, S.; Dominguez, J.M.; Viccione, G.; Gomez-Gesteira, M.; Crespo, A.J.C. Numerical validations and investigation of a semi-submersible floating offshore wind turbine platform interacting with ocean waves using an SPH framework. Appl. Ocean Res. 2023, 141, 103757. [Google Scholar] [CrossRef]
- Borg, M.; Pegalajar-Jurado, A.; Stiesdal, H.; Madsen, F.J.; Nielsen, T.R.L.; Mikkelsen, R.F.; Mirzaei, M.; Lomholt, A.K.; Bredmose, H. Dynamic response analysis of the TetraSpar floater in waves Experiment and numerical reproduction. Mar. Struct. 2024, 94, 103546. [Google Scholar] [CrossRef]
- Bak, C.; Zahle, F.; Bitsche, R.; Kim, T.; Yde, A.; Henriksen, L.C.; Hansen, M.H.; Blasques, J.P.A.A.; Gaunaa, M.; Natarajan, A. Description of the DTU 10MW Reference Wind Turbine; DTU Wind Energy Report-I-0092; DTU Wind Energy: Kongens Lyngby, Denmark, 2013. [Google Scholar]
- Akimoto, H.; Iijima, K.; Takata, Y. Feasibility study of the floating axis wind turbine preliminary model experiments. In Proceedings of the ASME 2017 36th International Conference on Ocean, Offshore and Arctic Engineering, Trondheim, Norway, 25–30 June 2017. [Google Scholar]
- Real-Time NOWPHAS (The Nationwide Ocean Wave Information Network for Ports and HArbourS). Available online: https://www.mlit.go.jp/kowan/nowphas/ (accessed on 30 May 2024).
- Ishihara, T.; Liu, Y. Dynamic Response Analysis of a Semi-Submersible Floating Wind Turbine in Combined Wave and Current Conditions Using Advanced Hydrodynamic Models. Energies 2020, 13, 5820. [Google Scholar] [CrossRef]
- Koo, B.J.; Gouppe, A.J.; Kimball, R.W.; Lambrakos, K.F. Model tests for a floating wind turbine on three different floaters. J. Offshore Mech. Arct. Eng. 2014, 136, 020907. [Google Scholar] [CrossRef]
- Ohta, M.; Komatsu, M.; Ito, H.; Kumamoto, H. Development of a V-shaped Semi-submersible Floating Structure for 7MW Offshore Wind Turbine. In Proceedings of the International Symposium on Marine and Offshore Renewable Energy, Tokyo, Japan, 28–30 October 2013. [Google Scholar]
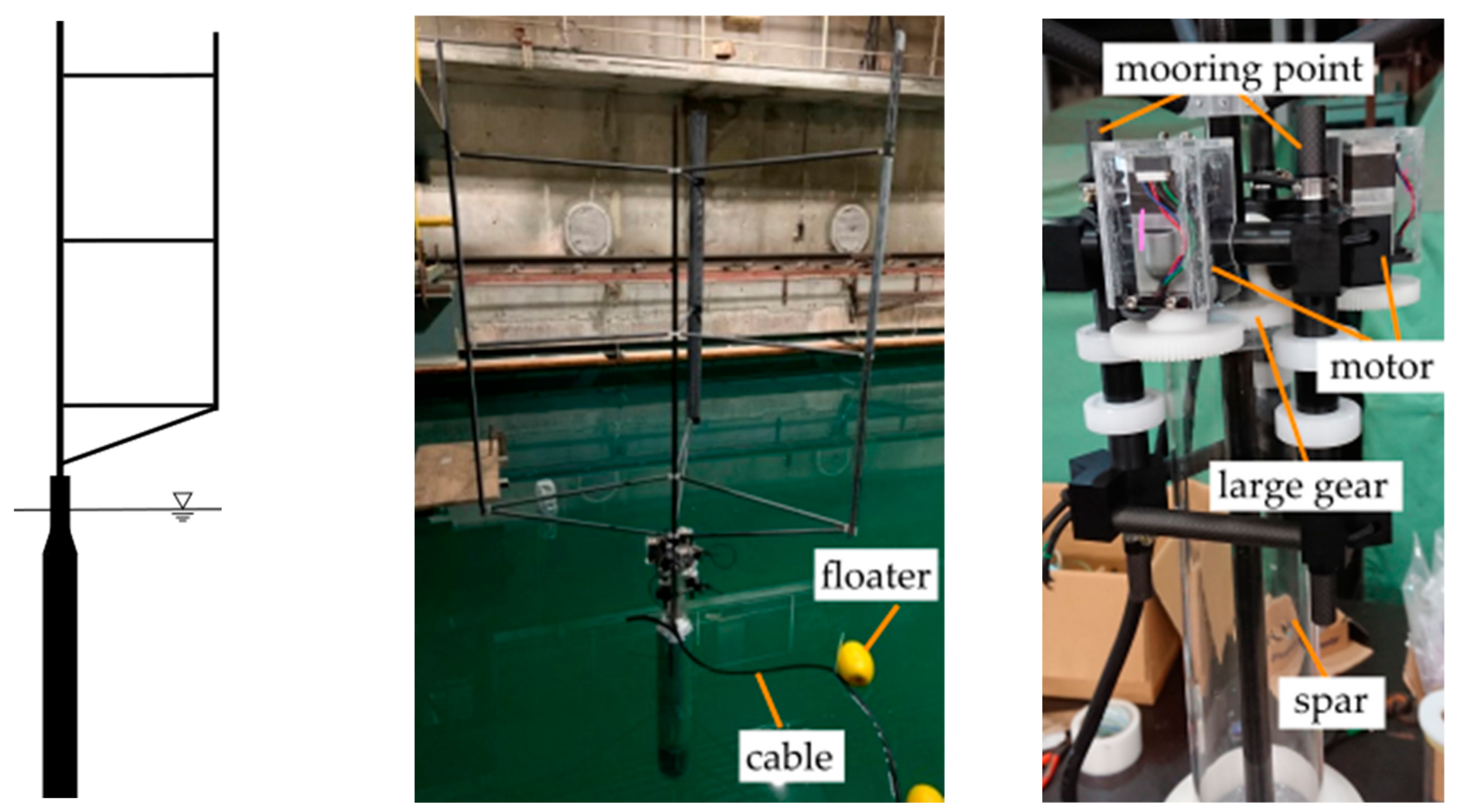









| Item | |
|---|---|
| Height of Rotor, H [m] | 1.38 |
| Diameter of Rotor, D [m] | 1.38 |
| Number of Blade | 3 |
| Blade Cross-section | NACA0021 |
| Blade Chord Length [m] | 0.07 |
| Solidity | 0.048 |
| Height of Spar [m] | 1.42 |
| Diameter of Spar [m] | 0.15 |
| Diameter of Spar (slender part) | 0.089 |
| Total Height of the model [m] | 3.13 |
| Draft [m] | 1.28 |
| Total Weight in Air [kg] | 20.0 |
| Center of Gravity from bottom [m] | 0.34 |
| Center of Buoyancy from bottom [m] | 0.57 |
| Item | |
|---|---|
| Length of polyethylene rope [m] | 3.16 |
| Weight of polyethylene rope per unit length [kg/m] | 0.003 |
| Length of stainless chain [m] | 10.0 |
| Weight of stainless chain per unit length [kg/m] | 0.147 |
| Operating Limit | 20 kW FAWT | Scale Model |
| Significant wave height [m] | 0.5 | 0.068 |
| Mean wave period [s] | 7.5 | 2.77 |
| Extreme Condition | 20 kW FAWT | Scale Model |
| Significant wave height [m] | 1.0 | 0.136 |
| Mean wave period [s] | 13.0 | 4.80 |
| Case | Rotor | Wave | [m] | [s] |
|---|---|---|---|---|
| (1) | P | R | 0.05 | 2.68, 2.86, 2.95, 3.04, 3.12, 3.20, 3.30, 3.40, 3.49, 3.58 |
| O | R | 0.05 | 2.68, 2.86, 2.95, 3.04, 3.12, 3.20, 3.30, 3.40, 3.49, 3.58 | |
| (2) | P | I | 0.068, 0.136 | 2.77 |
| O | I | 0.068, 0.136 | 2.77 | |
| (3) | O | R | 0.068 | 3.04, 3.40, 3.58 |
| (4) | PO | R | 0.068 | 3.04, 3.40, 3.58 |
| (5) | OP | R | 0.068 | 3.04, 3.40, 3.58 |
| (6) | O | R | 0.05 | 3.40 |
| (7) | O | I | 0.068 | 2.77 |
| (8) | P | R | 0.05 | 2.68, 2.86, 2.95, 3.04, 3.12, 3.20, 3.30, 3.40, 3.49, 3.58 |
| Direction | Scale Model | 20 kW FAWT |
|---|---|---|
| Surge | 20.5 | 55.5 |
| Sway | 32.8 | 88.8 |
| Heave | 3.4 | 9.2 |
| Roll | 5.1 | 13.9 |
| Pitch | 4.6 | 12.3 |
| Yaw | 5.1 | 13.9 |
| Wave Condition | Rotor | Normalized Max. Heave Amplitude [-] | Max. Pitch Angle [deg] | |
|---|---|---|---|---|
| [m] | [s] | |||
| 0.068 | 2.77 | Parking | 0.85 | 8.0 |
| Operating | 1.01 | 16.1 | ||
| 0.136 | 2.77 | Parking | 0.89 | 10.0 |
| Operating | 0.81 | 16.1 | ||
| Wave Condition | Rotor | ML1 | ML2 | ML3 | |
|---|---|---|---|---|---|
| [m] | [s] | ||||
| 0.068 | 2.77 | Parking | 1.36 (4.58) | 0.96 (3.12) | 0.92 (3.51) |
| Operating | 2.02 (4.57) | 0.92 (3.13) | 0.77 (3.49) | ||
| 0.136 | 2.77 | Parking | 1.53 (4.56) | 0.97 (3.14) | 0.93 (3.49) |
| Operating | 2.00 (4.57) | 0.92 (3.26) | 0.78 (3.49) | ||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Senga, H.; Kunishi, K.; Fujita, G.; Imaoka, T.; Ohira, H.; Akimoto, H. Experimental Study for Understanding the Characteristics of a Floating Axis Wind Turbine under Wind and Wave Conditions. Energies 2024, 17, 3285. https://doi.org/10.3390/en17133285
Senga H, Kunishi K, Fujita G, Imaoka T, Ohira H, Akimoto H. Experimental Study for Understanding the Characteristics of a Floating Axis Wind Turbine under Wind and Wave Conditions. Energies. 2024; 17(13):3285. https://doi.org/10.3390/en17133285
Chicago/Turabian StyleSenga, Hidetaka, Keitaro Kunishi, Gaku Fujita, Tomotake Imaoka, Hiroyuki Ohira, and Hiromichi Akimoto. 2024. "Experimental Study for Understanding the Characteristics of a Floating Axis Wind Turbine under Wind and Wave Conditions" Energies 17, no. 13: 3285. https://doi.org/10.3390/en17133285
APA StyleSenga, H., Kunishi, K., Fujita, G., Imaoka, T., Ohira, H., & Akimoto, H. (2024). Experimental Study for Understanding the Characteristics of a Floating Axis Wind Turbine under Wind and Wave Conditions. Energies, 17(13), 3285. https://doi.org/10.3390/en17133285





