Thermal and Hydrodynamic Measurements of a Novel Chaotic Micromixer to Enhance Mixing Performance
Abstract
1. Introduction
2. Materials and Methods
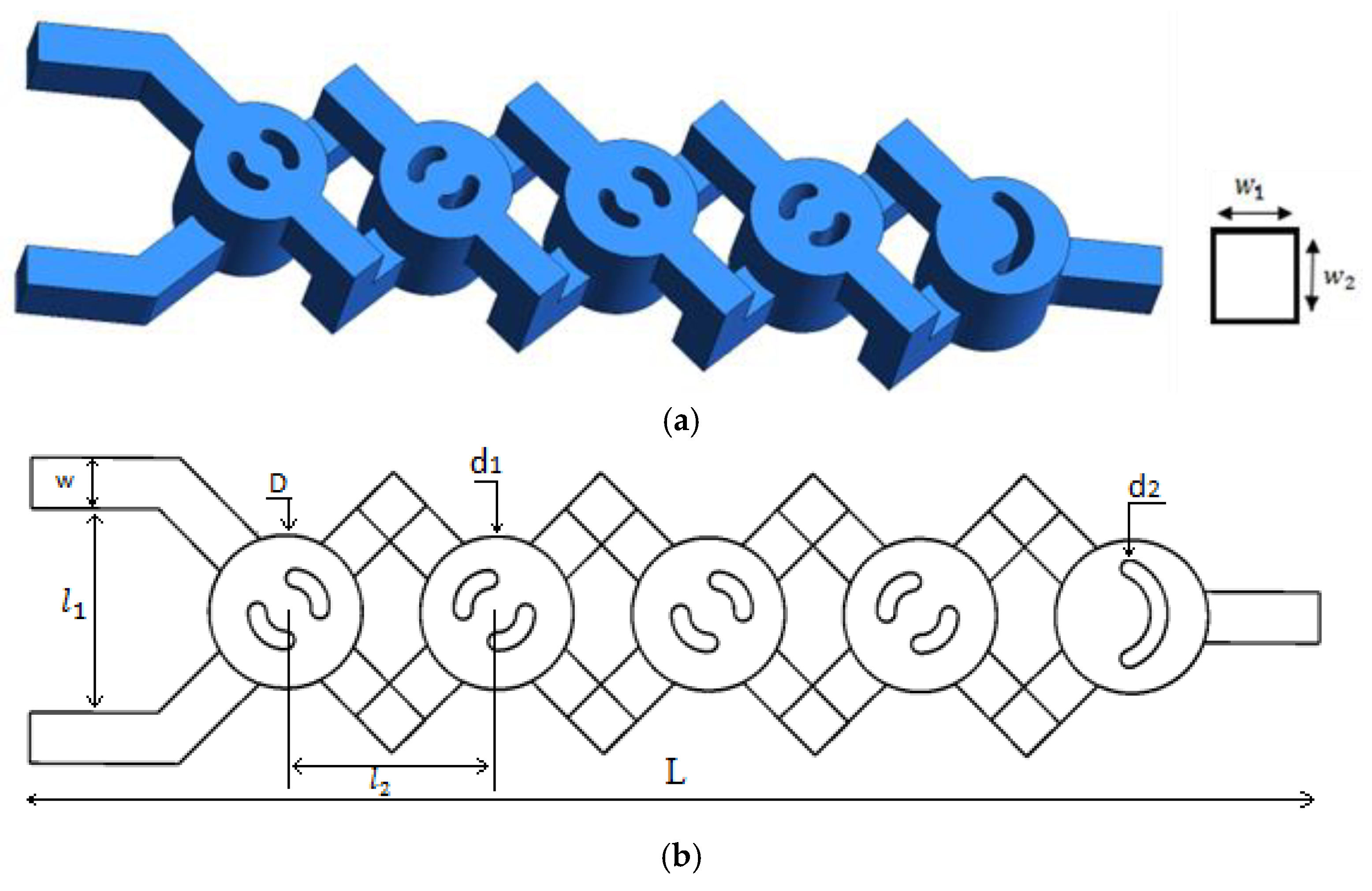
2.1. Micromixer Design
2.2. Numerical Simulations
2.2.1. Properties of Fluids
2.2.2. Governing Equations
2.3. Characterization of the Thermal Mixing
2.4. Boundary Conditions and Mesh-Independent Test
3. Results and Discussion
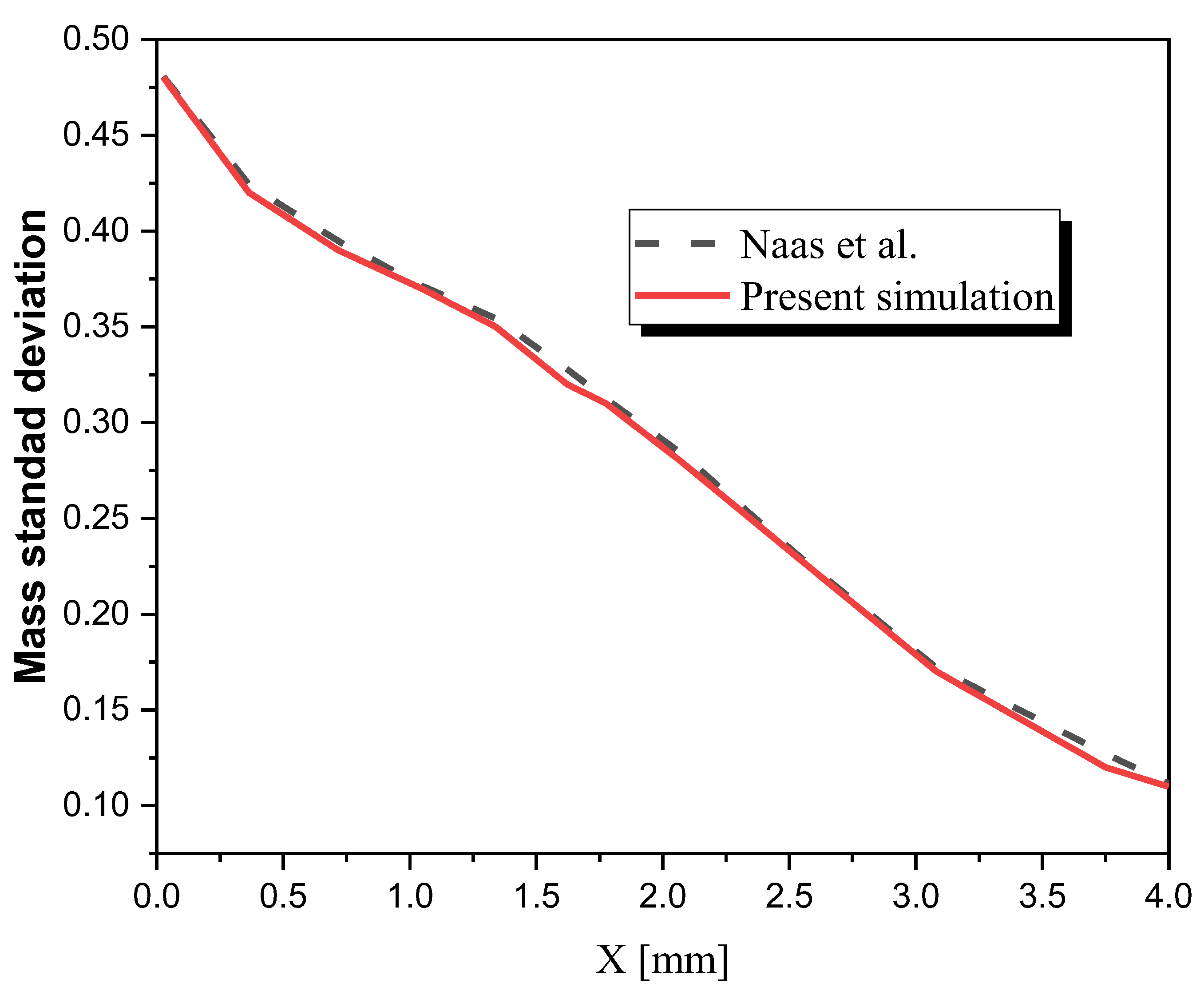
3.1. CFD Validation Case
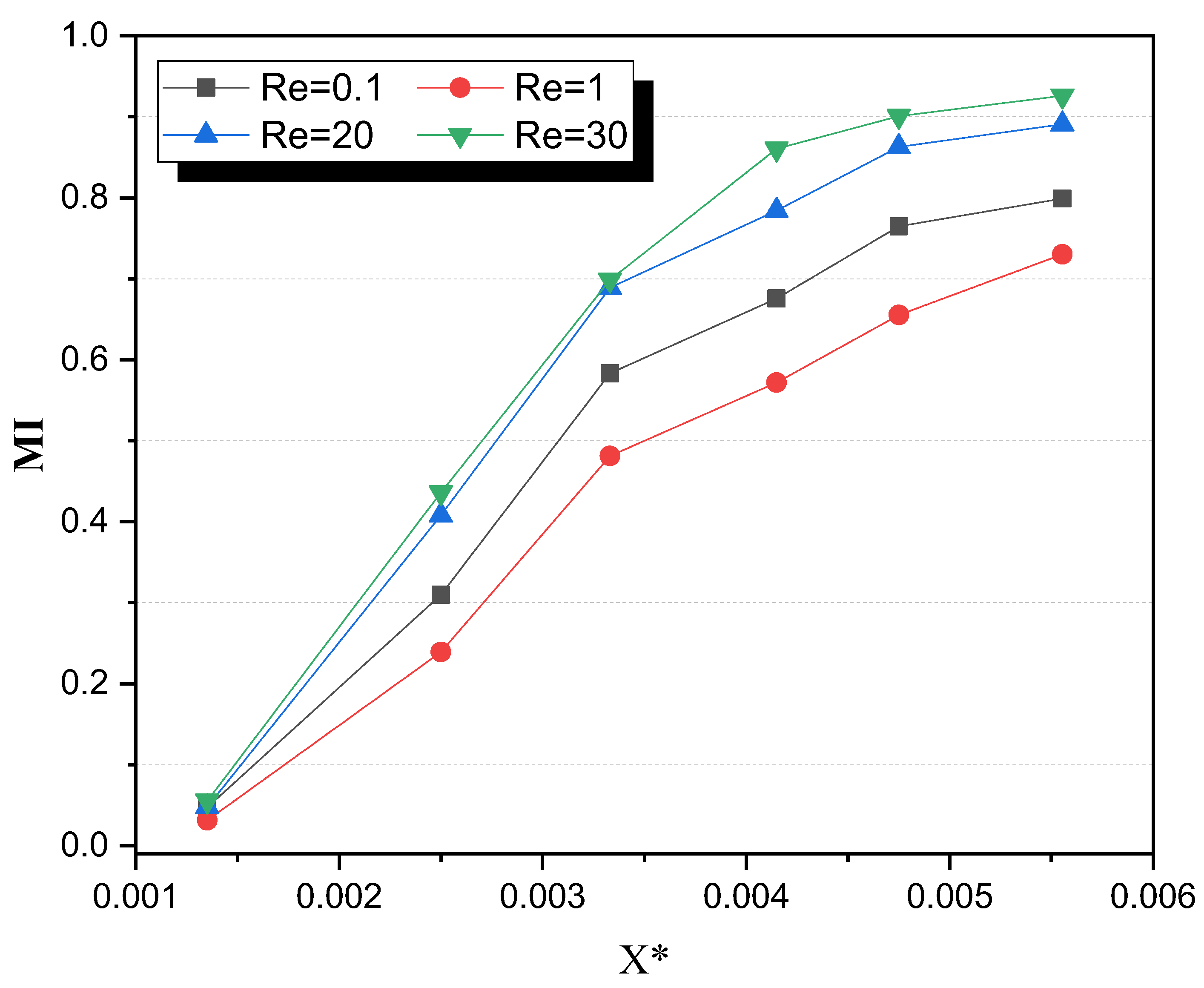
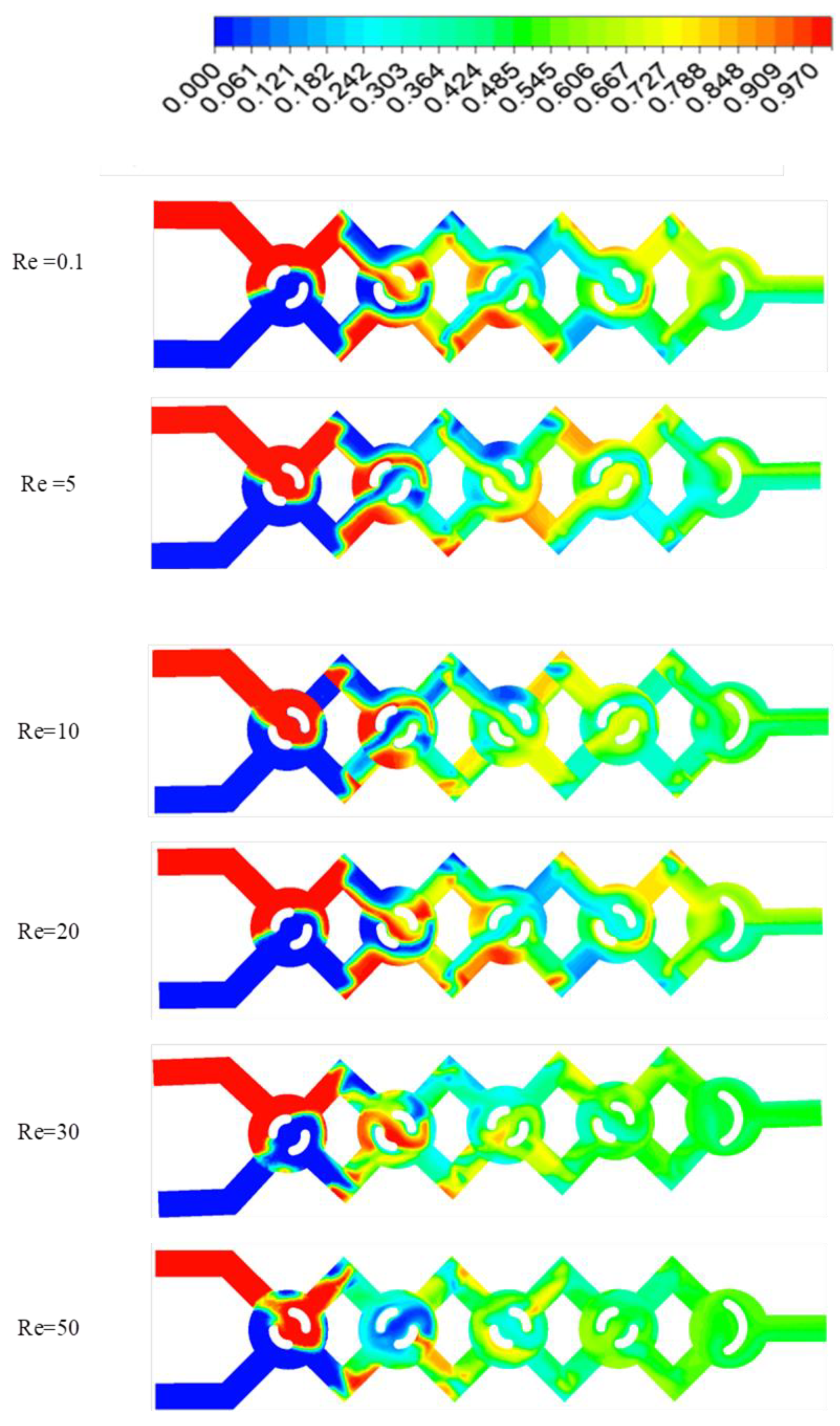
3.2. Analysis of the Newtonian Fluid
Micromixer Mixing Performance
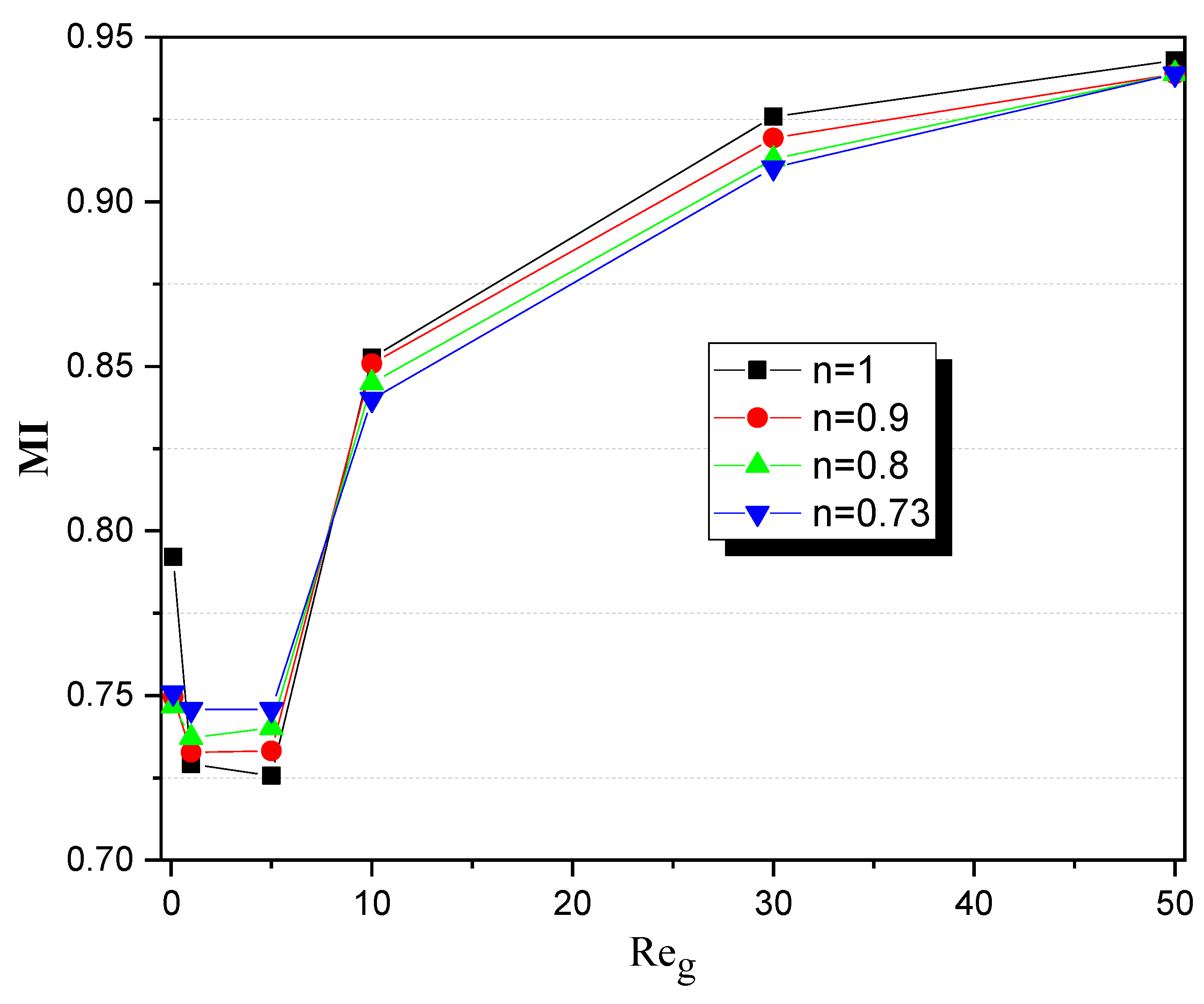
3.3. Analysis of the Non-Newtonian Fluid
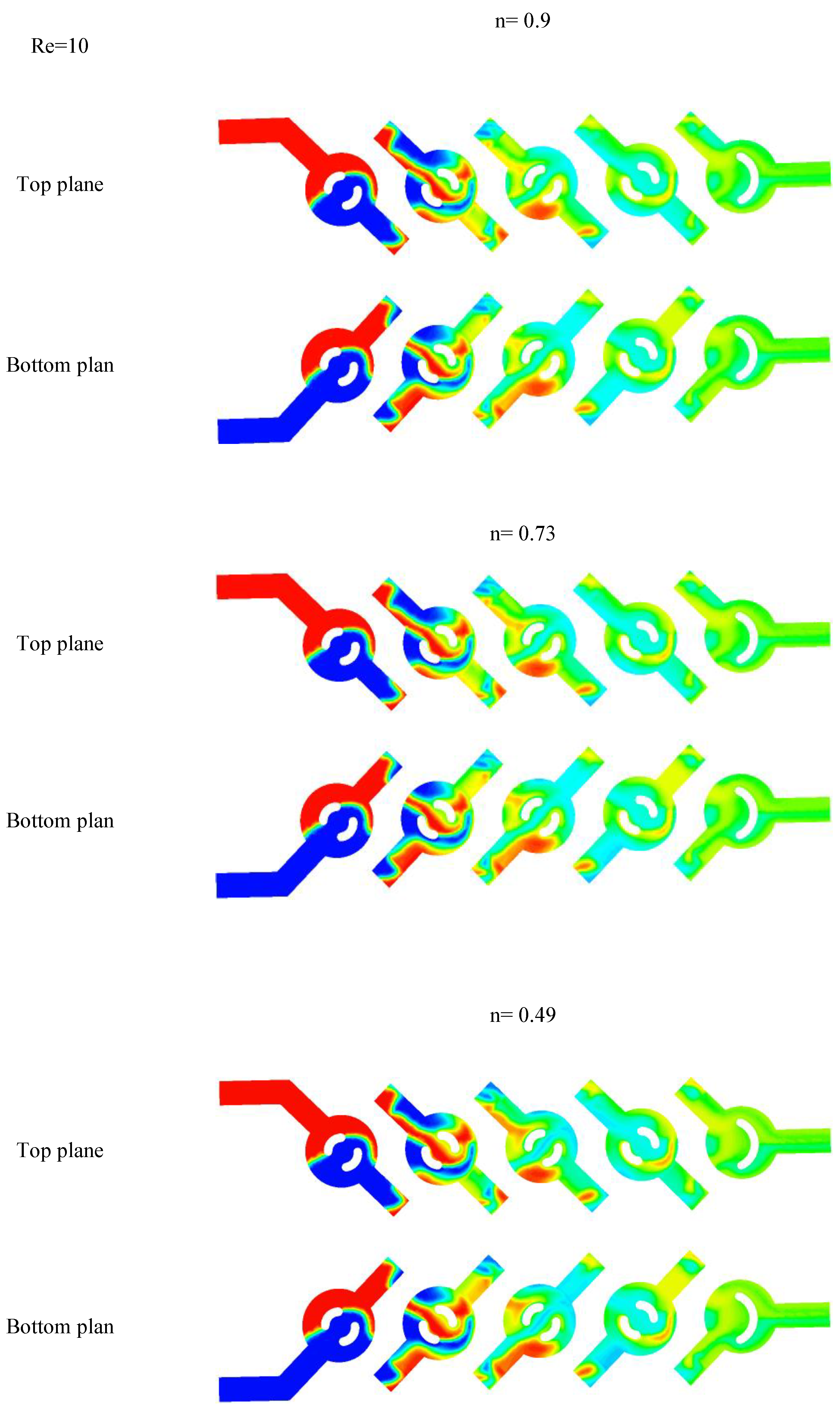
3.3.1. Micromixer Mixing Performance
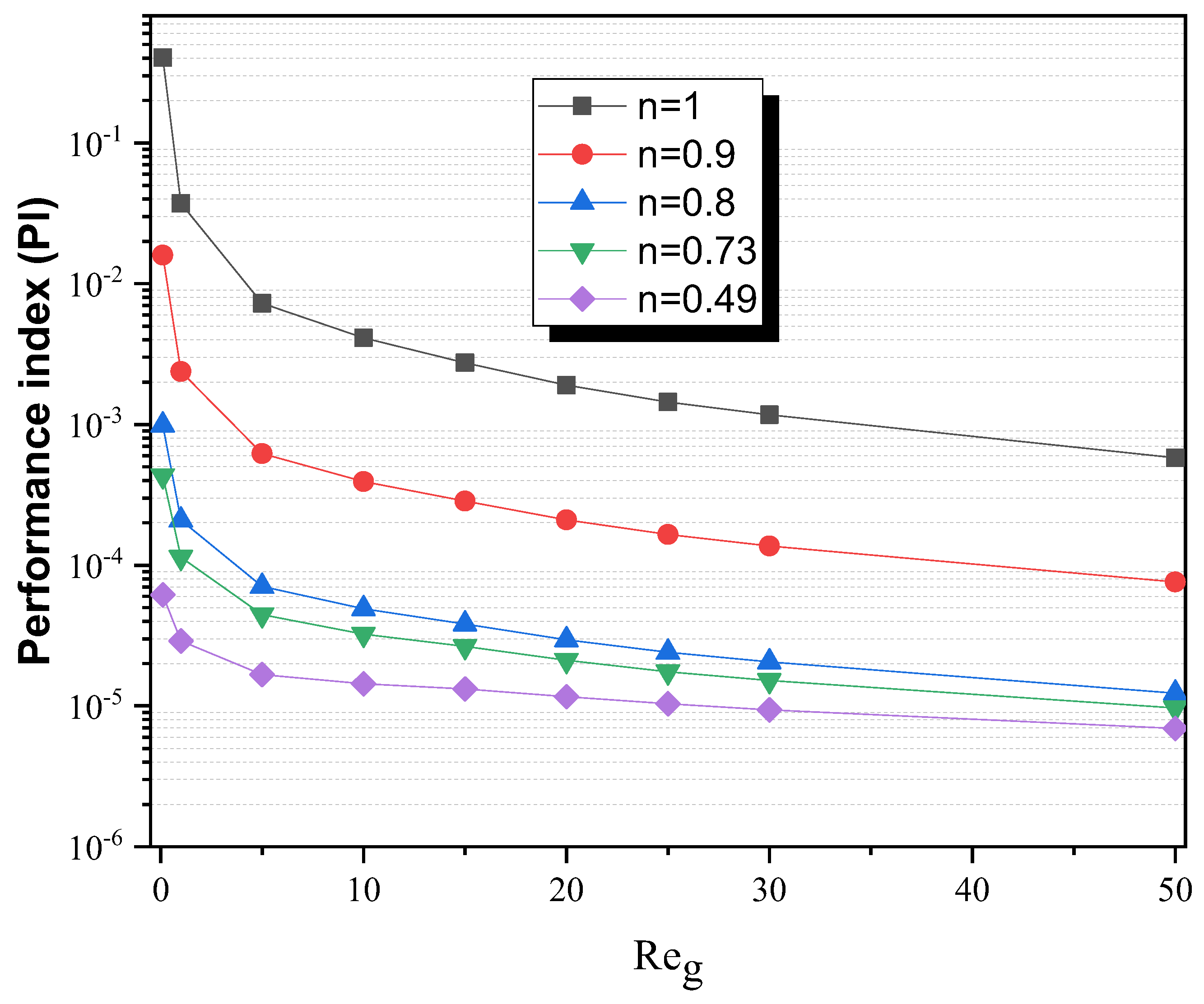
3.3.2. Pressure Drop and Performance Index
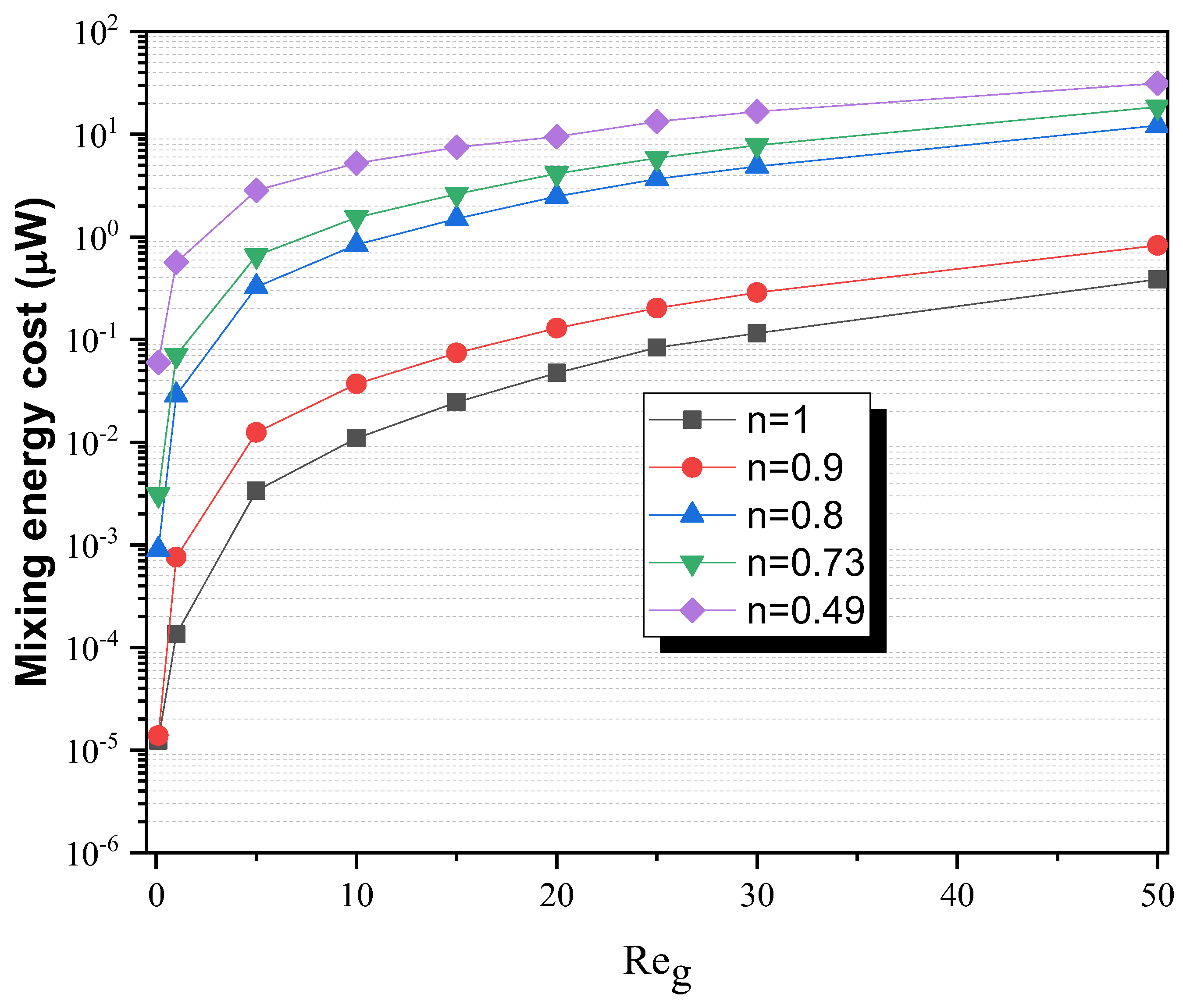
3.3.3. Mixing Energy Cost
3.3.4. Mass Distribution of the Non-Newtonian Fluids
3.3.5. Temperature Contours
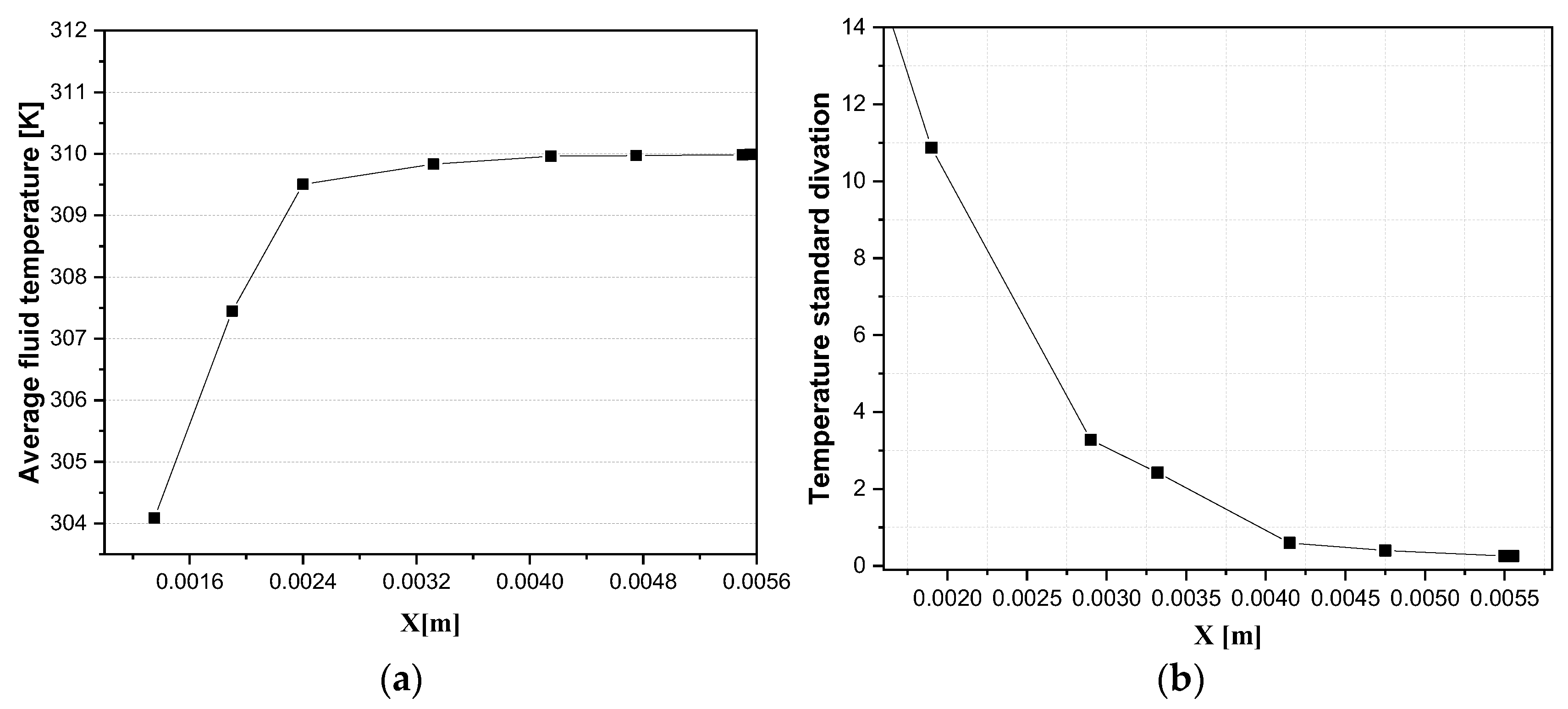
3.3.6. Average Fluid Temperature
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| Symbol | Description |
| ∇ | Gradient operator |
| c | Concentration |
| C | Average mole fraction |
| ci | Mole fraction at a specific location |
| CFD | Computational fluid dynamics |
| CMC | Carboxy methyl cellulose |
| D | Diffusion coefficient |
| d1, d2 | Diameter of grooves |
| Dh | Hydraulic diameter |
| k | Consistency index |
| L | Axial length of channel |
| l1, l2 | Length of grooves |
| MEC | Mixing energy cost |
| MI | Mixing index |
| n | Power law index |
| N | Number of nodes in the cross-section. |
| P | Pressure |
| PI | Performance index |
| Q | Flow rate |
| Reg | Generalized Reynolds number |
| T | Cross-section mean temperature |
| Ti | Temperature at the i-th node |
| TMI | Thermal mixing index |
| u | Velocity vector |
| w1, w2 | Width of inlet channels |
| γ | Shear rate tensor |
| ΔP | Pressure drop |
| Μ | Dynamic viscosity |
| μa | Apparent viscosity (for non-Newtonian fluids) |
| ξ | Dimensionless geometrical parameter |
| ρ | Fluid density |
| σ | Standard deviation |
| σ0 | Standard deviation at inlet section |
| τ | Shear stress tensor |
References
- Chen, X.; Shen, J. Design and Simulation of a Chaotic Micromixer with Diamond-Like Micropillar Based on Artificial Neural Network. Int. J. Chem. React. Eng. 2017, 15, 20160039. [Google Scholar] [CrossRef]
- Stroock, A.D.; Dertinger, S.K.W.; Ajdari, A.; Mezić, I.; Stone, H.A.; Whitesides, G.M. Chaotic Mixer for Microchannels. Science 2002, 295, 647–651. [Google Scholar] [CrossRef] [PubMed]
- Rahimi, M.; Azimi, N.; Parsamogadam, M.A.; Rahimi, A.; Masahy, M.M. Mixing Performance of T, Y, and Oriented Y-Micromixers with Spatially Arranged Outlet Channel: Evaluation with Villermaux/Dushman Test Reaction. Microsyst. Technol. 2017, 23, 3117–3130. [Google Scholar] [CrossRef]
- Nguyen, N.-T.; Wu, Z. Micromixers—A Review. J. Micromech. Microeng. 2005, 15, 2. [Google Scholar] [CrossRef]
- Hessel, V.; Löwe, H.; Schönfeld, F. Micromixers—A Review on Passive and Active Mixing Principles. Chem. Eng. Sci. 2005, 60, 2479–2501. [Google Scholar] [CrossRef]
- Glasgow, I.; Aubry, N. Enhancement of Microfluidic Mixing Using Time Pulsing. Lab Chip 2003, 3, 114–120. [Google Scholar] [CrossRef] [PubMed]
- Chen, X.; Li, T.; Zeng, H.; Hu, Z.; Fu, B. Numerical and Experimental Investigation on Micromixers with Serpentine Microchannels. Int. J. Heat Mass Transf. 2016, 98, 131–140. [Google Scholar] [CrossRef]
- Chen, X.; Wang, X. Optimized Modular Design and Experiment for Staggered Herringbone Chaotic Micromixer. Int. J. Chem. React. Eng. 2015, 13, 305–309. [Google Scholar] [CrossRef]
- Ianovska, M.A.; Mulder, P.P.M.F.A.; Verpoorte, E. Development of Small-Volume, Microfluidic Chaotic Mixers for Future Application in Two-Dimensional Liquid Chromatography. RSC Adv. 2017, 7, 9090–9099. [Google Scholar] [CrossRef]
- Ober, T.J.; Foresti, D.; Lewis, J.A. Active Mixing of Complex Fluids at the Microscale. Proc. Natl. Acad. Sci. USA 2015, 112, 12293–12298. [Google Scholar] [CrossRef]
- The, H.L.; Ta, B.Q.; Thanh, H.L.; Dong, T.; Thoi, T.N.; Karlsen, F. Geometric Effects on Mixing Performance in a Novel Passive Micromixer with Trapezoidal-Zigzag Channels. J. Micromech. Microeng. 2015, 25, 094004. [Google Scholar] [CrossRef]
- Chen, J.-K.; Yang, R.-J. Electroosmotic Flow Mixing in Zigzag Microchannels. Electrophoresis 2007, 28, 975–983. [Google Scholar] [CrossRef]
- Liu, R.H.; Stremler, M.A.; Sharp, K.V.; Olsen, M.G.; Santiago, J.G.; Adrian, R.J.; Aref, H.; Beebe, D.J. Passive Mixing in a Three-Dimensional Serpentine Microchannel. J. Microelectromech. Syst. 2000, 9, 190–197. [Google Scholar] [CrossRef]
- Vanka, S.P.; Luo, G.; Winkler, C.M. Numerical Study of Scalar Mixing in Curved Channels at Low Reynolds Numbers. AIChE J. 2004, 50, 2359–2368. [Google Scholar] [CrossRef]
- Johnson, T.J.; Ross, D.; Locascio, L.E. Rapid Microfluidic Mixing. Anal. Chem. 2002, 74, 45–51. [Google Scholar] [CrossRef]
- Chen, J.J.; Shie, Y.S. Interfacial Configurations and Mixing Performances of Fluids in Staggered Curved-Channel Micromixers. Microsyst. Technol. 2012, 18, 1823–1833. [Google Scholar] [CrossRef]
- Kim, D.S.; Lee, S.H.; Kwon, T.H.; Ahn, C.H. A Serpentine Laminating Micromixer Combining Splitting/Recombination and Advection. Lab Chip 2005, 5, 739–747. [Google Scholar] [CrossRef]
- Tayeb, N.T.; Hossain, S.; Khan, A.H.; Mostefa, T.; Kim, K.-Y. Evaluation of Hydrodynamic and Thermal Behaviour of Non-Newtonian-Nanofluid Mixing in a Chaotic Micromixer. Micromachines 2022, 13, 933. [Google Scholar] [CrossRef]
- Wu, S.-J.; Hsu, H.-C.; Feng, W.-J. Novel Design and Fabrication of a Geometrical Obstacle-Embedded Micromixer with Notched Wall. Jpn. J. Appl. Phys. 2014, 53, 097201. [Google Scholar] [CrossRef]
- Mengeaud, V.; Josserand, J.; Girault, H.H. Mixing Processes in a Zigzag Microchannel: Finite Element Simulations and Optical Study. Anal. Chem. 2002, 74, 4279–4286. [Google Scholar] [CrossRef]
- Tsai, R.-T.; Wu, C.-Y. An Efficient Micromixer Based on Multidirectional Vortices Due to Baffles and Channel Curvature. Biomicrofluidics 2011, 5, 014103. [Google Scholar] [CrossRef]
- Tsai, R.-T.; Wu, C.-Y.; Chang, C.-Y.; Kuo, M.-Y. Mixing Behaviors of Shear-Thinning Fluids in Serpentine-Channel Micromixers. Int. J. Aerosp. Mech. Eng. 2015, 9, 1329–1335. [Google Scholar]
- Raza, W.; Kim, K.-Y. Unbalanced Split and Recombine Micromixer with Three-Dimensional Steps. Ind Eng Chem Res 2020, 59, 3744–3756. [Google Scholar] [CrossRef]
- Alam, A.; Kim, K.-Y. Mixing Performance of a Planar Micromixer with Circular Chambers and Crossing Constriction Channels. Sens. Actuators B Chem. 2013, 176, 639–652. [Google Scholar] [CrossRef]
- Xia, H.M.; Wan, S.Y.M.; Shu, C.; Chew, Y.T. Chaotic Micromixers Using Two-Layer Crossing Channels to Exhibit Fast Mixing at Low Reynolds Numbers. Lab Chip 2005, 5, 748–755. [Google Scholar] [CrossRef]
- Hossain, S.; Lee, I.; Kim, S.M.; Kim, K.-Y. A Micromixer with Two-Layer Serpentine Crossing Channels Having Excellent Mixing Performance at Low Reynolds Numbers. Chem. Eng. J. 2017, 327, 268–277. [Google Scholar] [CrossRef]
- Wang, J.; Liu, G.; Li, X.; He, F.; Ma, X. A Micromixer with Two-Layer Crossing Microchannels Based on PMMA Bonding Process. Int. J. Chem. React. Eng. 2019, 17, 20180265. [Google Scholar] [CrossRef]
- Afzal, A.; Kim, K.-Y. Flow and Mixing Analysis of Non-Newtonian Fluids in Straight and Serpentine Microchannels. Chem. Eng. Sci. 2014, 116, 263–274. [Google Scholar] [CrossRef]
- Baheri Islami, S.; Khezerloo, M.; Gharraei, R. The Effect of Chaotic Advection on Mixing Degree and Pressure Drop of Non-Newtonian Fluids Flow in Curved Micromixers. J. Braz. Soc. Mech. Sci. Eng. 2017, 39, 813–831. [Google Scholar] [CrossRef]
- Baheri Islami, S.; Khezerloo, M. Enhancement of Mixing Performance of Non-Newtonian Fluids Using Curving and Grooving of Microchannels. J. Appl. Fluid Mech. 2017, 10, 127–141. [Google Scholar] [CrossRef]
- He, M.; Li, W.; Zhang, M.Q.; Zhang, J. Numerical Investigation on the Efficient Mixing of Overbridged Split-and-Recombine Micromixer at Low Reynolds Number. Microsyst. Technol. 2019, 25, 3447–3461. [Google Scholar] [CrossRef]
- Mashaei, P.R.; Hosseinalipour, S.M.; Esmailpour, K. Numerical Investigation of Thermal Mixing of Shear Thinning Fluids in One-Way Opposing Jets. J. Comput. Appl. Res. Mech. Eng. 2014, 3, 95–103. [Google Scholar]
- Maurya, A.; Tiwari, N.; Chhabra, R.P. Thermal Mixing of Impinging Laminar Streams of Shear-Thinning Fluids. Heat Transf. Eng. 2020, 41, 1576–1595. [Google Scholar] [CrossRef]
- Naas, T.T.; Lasbet, Y.; Aidaoui, L.; Boukhalkhal, A.L.; Loubar, K. High Performance in Terms of Thermal Mixing of Non-Newtonian Fluids Using Open Chaotic Flow: Numerical Investigations. Therm. Sci. Eng. Prog. 2020, 16, 100454. [Google Scholar] [CrossRef]
- Kouadri, A.; Douroum, E.; El Ouederni, A.R.; Benazza, A.; Laouedj, S.; Khelladi, S. Assessment of Mixing Behaviors of Non-Newtonian Pseudoplastic Fluids in Short Microdevices. Int. Commun. Heat Mass Transf. 2024, 155, 107500. [Google Scholar] [CrossRef]
- Li, A.; Yao, Y.; Tang, X.; Liu, P.; Zhang, Q.; Li, Q.; Li, P.; Zhang, F.; Wang, Y.; Tao, C.; et al. Experimental and Computational Investigation of Chaotic Advection Mixing in Laminar Rectangular Stirred Tanks. Chem. Eng. J. 2024, 485, 149956. [Google Scholar] [CrossRef]
- Fellouah, H.; Castelain, C.; Ould-El-Moctar, A.; Peerhossaini, H. The Dean Instability in Power-Law and Bingham Fluids in a Curved Rectangular Duct. J. Non-Newton Fluid Mech. 2010, 165, 163–173. [Google Scholar] [CrossRef]
- Pinho, F.T.; Whitelaw, J.H. Flow of Non-Newtonian Fluids in a Pipe. J. Non-Newton Fluid Mech. 1990, 34, 129–144. [Google Scholar] [CrossRef]
- Huang, H.-C.; Li, Z.-H.; Zheng-Hua, L. Finite Element Analysis of Non-Newtonian Flow: Theory and Software; Springer: London, UK, 1998. [Google Scholar]
- Byron Bird, R.; Armstrong, R.C.; Hassager, O. Dynamics of Polymeric Liquids, Volume 1: Fluid Mechanics; Wiley: Hoboken, NJ, USA, 1987. [Google Scholar]
- Metzner, A.B.; Reed, J.C. Flow of Non-newtonian Fluids—Correlation of the Laminar, Transition, and Turbulent-flow Regions. AIChE J. 1955, 1, 434–440. [Google Scholar] [CrossRef]
- Delplace, F.; Leuliet, J.C. Generalized Reynolds Number for the Flow of Power Law Fluids in Cylindrical Ducts of Arbitrary Cross-Section. Chem. Eng. J. Biochem. Eng. J. 1995, 56, 33–37. [Google Scholar] [CrossRef]
- Mahammedi, A.; Tayeb, N.T.; Kim, K.-Y.; Hossain, S. Mixing Enhancement of Non-Newtonian Shear-Thinning Fluid for a Kenics Micromixer. Micromachines 2021, 12, 1494. [Google Scholar] [CrossRef] [PubMed]
- Lasbet, Y.; Auvity, B.; Castelain, C.; Peerhossaini, H. Thermal and Hydrodynamic Performances of Chaotic Mini-Channel: Application to the Fuel Cell Cooling. Heat Transf. Eng. 2007, 28, 795–803. [Google Scholar] [CrossRef]
- Gidde, R.R.; Pawar, P.M.; Ronge, B.P.; Shinde, A.B.; Misal, N.D.; Wangikar, S.S. Flow Field Analysis of a Passive Wavy Micromixer with CSAR and ESAR Elements. Microsyst. Technol. 2019, 25, 1017–1030. [Google Scholar] [CrossRef]
- Falk, L.; Commenge, J.-M. Performance Comparison of Micromixers. Chem. Eng. Sci. 2010, 65, 405–411. [Google Scholar] [CrossRef]
- Gidde, R.R.; Pawar, P.M. Flow Feature and Mixing Performance Analysis of RB-TSAR and EB-TSAR Micromixers. Microsyst. Technol. 2020, 26, 517–530. [Google Scholar] [CrossRef]





| CMC (%) | n | k (Pa·sn) |
|---|---|---|
| 0 | 1 | 0.00092 |
| 0.1 | 0.9 | 0.0075 |
| 0.2 | 0.8 | 0.06 |
| 0.3 | 0.73 | 0.15 |
| 0.7 | 0.49 | 2.75 |
| Mesh | Standard Deviation | Pressure Drop (Pa) |
|---|---|---|
| 138,768 | 0.0581 | 200.5 |
| 245,810 | 0.0637 | 204.36 |
| 316,116 | 0.0682 | 205.9 |
| 472,970 | 0.0727 | 209.56 |
| 540,824 | 0.0762 | 210.43 |
| 652,462 | 0.0784 | 211.3 |
| 793,495 | 0.0784 | 211.4 |
| Re | MEC (μW) This Work | MEC (μW) in Ref. [18] | MEC (μW) in Ref. [47] |
|---|---|---|---|
| 1 | 0.00134 | 0.0064 | 0.0297 |
| 5 | 0.336 | 0.194 | 0.8346 |
| 15 | 2.456 | 1.548 | 10.2599 |
| 30 | 11.529 | 7.43 | 55.1685 |
| Line | P1 | P2 | P3 | P4 | P5 | Outlet |
|---|---|---|---|---|---|---|
| Y (m) | 0.00135 | 0.00247 | 0.00333 | 0.00415 | 0.00475 | 0.0056 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mahammedi, A.; Kouider, R.; Tayeb, N.T.; Kassir Al-Karany, R.; Cuerda-Correa, E.M.; Al-Kassir, A. Thermal and Hydrodynamic Measurements of a Novel Chaotic Micromixer to Enhance Mixing Performance. Energies 2024, 17, 3248. https://doi.org/10.3390/en17133248
Mahammedi A, Kouider R, Tayeb NT, Kassir Al-Karany R, Cuerda-Correa EM, Al-Kassir A. Thermal and Hydrodynamic Measurements of a Novel Chaotic Micromixer to Enhance Mixing Performance. Energies. 2024; 17(13):3248. https://doi.org/10.3390/en17133248
Chicago/Turabian StyleMahammedi, Abdelkader, Rahmani Kouider, Naas Toufik Tayeb, Raúl Kassir Al-Karany, Eduardo M. Cuerda-Correa, and Awf Al-Kassir. 2024. "Thermal and Hydrodynamic Measurements of a Novel Chaotic Micromixer to Enhance Mixing Performance" Energies 17, no. 13: 3248. https://doi.org/10.3390/en17133248
APA StyleMahammedi, A., Kouider, R., Tayeb, N. T., Kassir Al-Karany, R., Cuerda-Correa, E. M., & Al-Kassir, A. (2024). Thermal and Hydrodynamic Measurements of a Novel Chaotic Micromixer to Enhance Mixing Performance. Energies, 17(13), 3248. https://doi.org/10.3390/en17133248









